Influence of Thermal Sensitivity of Functionally Graded Materials on Temperature during Braking
Abstract
:1. Introduction
2. Statement to the Problem
- The braking process with constant deceleration is considered;
- At the initial time moment, the temperature of a brake is equal to the ambient temperature Ta;
- In the heat conduction equation, only the change in temperature gradient in the perpendicular direction to the disc-pad contact surfaces is taken into consideration;
- The thermal contact on the friction surfaces is perfect, i.e., the temperatures of its contact surfaces are equal, and the sum of frictional heat fluxes intensities, acting along the normal direction to the contact surface to the insides of the elements equal to the specific friction power;
- Due to the symmetry of the system with respect to the mid plane of the disc, when determining the brake temperature, the contact of one pad and a disc with half of its thickness is considered;
- The pads and the disc are made of two-component thermally sensitive functionally graded materials, in such a way that their friction surfaces are materials with low thermal conductivity (i.e., cermet), while the core materials are characterized by higher thermal conductivity (titanium alloys, aluminum, etc.);
- The thermal conductivity of the disc and pads materials increases exponentially with the distance from the contact surface;
- The whole initial kinetic energy of the vehicle is transformed into heat during braking, neglecting the small part of energy associated with wear on the contact surfaces of the disc and pads;
3. Solution with Temperature-Independent FGMs Properties
4. Volume Temperature
5. Numerical Analysis
- (1)
- the values of the input parameters were given (Table 1), and then from Equations (8) and (9) the area of the nominal contact was calculated , specific friction power , friction power and stop time ;
- (2)
- using the dependencies (40)–(51) the materials properties , and , at the initial temperature were established (Table 2);
- (3)
- the effective values of: the specific heat , density , thermal diffusivity , the effective depths of heat penetration and the dimensionless gradient parameters of materials , were found from Equations (3) and (5)–(7). Then, the dimensionless parameters and were determined from the Formulas (16) and (17), and also the weight and heat partition ratios , were calculated from the Equations (38) and (39) (Table 3);
- (4)
- the volume temperature values of the disc and the pad were obtained from the Equations (36) and (37);
- (5)
- the values of materials properties , , , , corresponding to the volume temperature were determined from the Formulas (40)–(51);
- (6)
- the steps (3)–(5) were repeated resulting in the corrected values for the volume temperature , and ;
- (7)
- by means of the formula , final values of the volume temperature and were found;
- (8)
- (9)
- the temperature field (23)–(26), the temperature evolution (27), and temporal profiles of heat fluxes intensities , (30)–(32) were determined.
6. Conclusions
- the influence of thermal sensitivity on the temperature of FGMs may be more significant than in the case of homogeneous materials;
- for the selected friction pair, taking into account the thermal sensitivity caused an almost threefold reduction in the maximum temperature in comparison to the appropriate temperature values, found with the same properties of the materials;
- the influence of thermal sensitivity on the intensity of heat fluxes directed from the friction surface to the interior of the friction pair elements is insignificant. This means that to estimate the amount of heat absorbed by the individual elements of the friction pair, appropriate solutions to linear problems can be used.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| Effective depth of heat penetration () | |
| Area of the nominal contact region () | |
| Specific heat capacity () | |
| Coefficient of friction (dimensionless) | |
| Weight of the friction elements (kg) | |
| The Bessel functions of the first kind of the kth order | |
| Thermal diffusivity () | |
| Thermal conductivity () | |
| Contact pressure () | |
| Nominal value of the contact pressure () | |
| External radius of the pads (m) | |
| Internal radius of the pads (m) | |
| Specific power of friction () | |
| q0 | Nominal value of the specific power of friction () |
| Nominal friction power () | |
| Time () | |
| Stop time () | |
| Temperature () | |
| Initial temperature () | |
| Velocity () | |
| Volume fractions of the material phases | |
| Initial velocity () | |
| Initial kinetic energy of the system () | |
| Spatial coordinate in axial direction () | |
| lower | Number of the main () and frictional () elements of the friction pair |
| lower | Number of the component material of selected friction element |
| Heat partition ratio (dimensionless) | |
| Cover angle of the pads (rad) | |
| Parameter of material gradient () | |
| Parameter of material gradient (dimensionless) | |
| Temperature rise () | |
| Temperature rise (dimensionless) | |
| Temperature scaling factor () | |
| Density () | |
| Time (dimensionless) | |
| Time of braking (dimensionless) | |
| Spatial coordinate in axial direction (dimensionless) | |
| Volume temperature () |
References
- Balakin, V.; Sergienko, V. Thermal Calculation of Brakes and Frictional Assemblies; Belyi Metal-Polymer Research Institute of the National Academy of Sciences of Belarus: Gomel, Belarus, 1999. (In Russian) [Google Scholar]
- Chichinadze, A.V.; Braun, E.D.; Ginzburg, A.G.; Ignat’eva, E.V. Calculation, Testing and Selection of Friction Couples; Nauka: Moscow, Russia, 1979. (In Russian) [Google Scholar]
- Adamowicz, A.; Grześ, P. Three-dimensional FE model for calculation of temperature of a thermosensitive disc. Appl. Therm. Eng. 2013, 50, 572–581. [Google Scholar] [CrossRef]
- Yevtushenko, A.A.; Grześ, P.; Adamowicz, A. The temperature mode of the carbon-carbon multi-disc brake in the view of the interrelations of its operating characteristics. Materials 2020, 13, 1878. [Google Scholar] [CrossRef] [PubMed]
- Kushnir, R.M.; Popovych, V.S. Heat Conduction Problems of Thermosensitive Solids under Complex Heat Exchange; Vyacheslav, V., Ed.; Heat Conduction-Basic Research: Rijeka, Croatia, 2011; ISBN 978-953-307-404-7. [Google Scholar]
- Yevtushenko, A.A.; Kuciej, M.; Och, E. Some Methods for Calculating Temperature during the Friction of Thermosensitive Materials. Numer. Heat Transf. Part A Appl. 2015, 67, 696–718. [Google Scholar] [CrossRef]
- Yevtushenko, A.; Kuciej, M.; Och, E.; Yevtushenko, O. Effect of the thermal sensitivity in modeling of the frictional heating during braking. Adv. Mech. Eng. 2016, 8, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Popovich, V.S.; Garmatii, G.Y. Solution of nonstationary heat conduction problems for thermosensitive bodies under convective heat exchange. J. Math. Sci. 1998, 90, 2037–2041. [Google Scholar] [CrossRef]
- Evtushenko, O.; Kuciej, M.; Topczewska, K. Determination of the maximal temperature of a pad-disk tribosystem during one-time braking. Mater. Sci. 2020, 56, 152–159. [Google Scholar] [CrossRef]
- Zelentsov, V.B.; Lapina, P.A.; Mitrin, B.I. Wear of Functionally Graded Coatings under Frictional Heating Conditions. Nanomaterials 2022, 12, 142. [Google Scholar] [CrossRef]
- Yevtushenko, A.; Topczewska, K.; Zamojski, P. The Effect of Functionally Graded Materials on Temperature during Frictional Heating: Under Uniform Sliding. Materials 2021, 14, 4285. [Google Scholar] [CrossRef]
- Abbas, I.A. Nonlinear transient thermal stress analysis of thick-walled FGM cylinder with temperature-dependent material properties. Meccanica 2014, 49, 1697–1708. [Google Scholar] [CrossRef]
- Azadi, M.; Azadi, M. Nonlinear transient heat transfer and thermoelastic analysis of thick-walled FGM cylinder with temperature-dependent material properties using Hermitian transfinite element. J. Mech. Sci. Technol. 2009, 23, 2635. [Google Scholar] [CrossRef]
- Tanigawa, Y.; Akai, T.; Kawamura, R.; Oka, N. Transient heat conduction and thermal stress problems of a nonhomogeneous plate with temperature-dependent material properties. J. Therm. Stresses 1996, 19, 77–102. [Google Scholar] [CrossRef]
- Ding, S.; Wu, C.-P. Optimization of material composition to minimize the thermal stresses induced in FGM plates with temperature-dependent material properties. Int. J. Mech. Mater. Des. 2018, 14, 527–549. [Google Scholar] [CrossRef]
- Noda, N. Thermal stresses in functionally graded materials. J. Therm. Stresses 1999, 22, 477–512. [Google Scholar] [CrossRef]
- Ueda, S. Thermoelastic analysis of W-Cu functionally graded materials subjected to a thermal shock using a micromechanical model. J. Therm. Stresses 2001, 24, 19–46. [Google Scholar] [CrossRef]
- Yevtushenko, A.; Topczewska, K.; Zamojski, P. The Effect of Functionally Graded Materials on Temperature during Frictional Heating at Single Braking. Materials 2021, 14, 6241. [Google Scholar] [CrossRef]
- Chichinadze, A.V. Polymers in Friction Assembles of Machines and Devices: A Handbook; Allerton Press Inc.: New York, NY, USA, 1984. [Google Scholar]
- Yevtushenko, A.; Kuciej, M.; Topczewska, K. Frictional Heating during Braking of the C/C Composite Disc. Materials 2020, 13, 2691. [Google Scholar] [CrossRef]
- Yevtushenko, A.; Topczewska, K.; Kuciej, M. Analytical Determination of the Brake Temperature Mode during Repetitive Short-Term Braking. Materials 2021, 14, 1912. [Google Scholar] [CrossRef]
- Watson, G.N. A Treatise of the Theory of Bessel Functions, 2nd ed.; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Yevtushenko, A.A.; Kuciej, M. Two heat conduction problems with frictional heating during braking. J. Theor. Appl. Mech. 2010, 48, 367–380. [Google Scholar]
- Fazekas, G.A.G. Temperature gradients and heat stresses in brake drums. SAE Trans. 1953, 61, 279–284. [Google Scholar]
- Strojny-Nędza, A.; Pietrzak, K.; Gili, F.; Chmielewski, M. FGM based on copper–alumina composites for brake disc applications. Arch. Civ. Mech. Eng. 2020, 20, 83. [Google Scholar] [CrossRef]
- Burghartz, S.; Schulz, B. Thermophysical properties of sapphire, AlN and MgAl2O4 down to 70 K. J. Nucl. Mater. 1994, 212–215 Pt B, 1065–1068. [Google Scholar] [CrossRef]
- Kingery, W.D.; Francl, J.; Coble, R.L.; Vasilos, T. Thermal Conductivity: X, Data for Several Pure Oxide Materials Corrected to Zero Porosity. J. Am. Ceram. Soc. 1954, 37, 107–110. [Google Scholar] [CrossRef]
- Archer, D.A. Thermodynamic Properties of Synthetic Sapphire (α-Al2O3), Standard Reference Material 720 and the Effect of Temperature-Scale Differences on Thermodynamic Properties. J. Phys. Chem. Ref. Data 1993, 22, 1441–1453. [Google Scholar] [CrossRef] [Green Version]
- Simon, N.J.; Drexler, E.S.; Reed, R.P. Properties of Copper and Copper Alloys at Cryogenic Temperatures; Monograph 177; National Institute of Standards and Technology: New York, NY, USA, 1992.
- Pankratz, L.B. Thermodynamic Properties of Elements and Oxides; Bulletin 672; U.S. Bureau of Mines: Washington, DC, USA, 1982. [Google Scholar]
- Taylor, D. Thermal expansion data. II: Binary oxides with the fluorite and rutile structures, MO2, and the antifluorite structure, M2O. Trans. J. Br. Ceram. Soc. 1984, 83, 32–37. [Google Scholar]
- Deem, H.W.; Wood, W.D.; Lucks, C.F. The relationship between Electrical and Thermal Conductivities of Titanium Alloys. Trans. Metall. Soc. AIME 1958, 212, 520–523. [Google Scholar]
- Cezairrliyan, A.; McClure, J.L.; Taylor, R.J. Thermophysical Measurements on 90Ti-6Al-4V Alloy Above 1450 K Using a Transient (Subsecond) Technique. J. Res. Nat. Bur. Stand.-A Phys. Chem. 1977, 81, 251–256. [Google Scholar] [CrossRef]
- Press, W.H.; Teukolsky, S.A.; Flannery, B.P.; Vetterling, W.T. Numerical Recipes in Fortran 77: Volume 1, Volume 1 of Fortran Numerical Recipes: The Art of Scientific Computing; Cambridge University Press: Cambridge, UK, 1992. [Google Scholar]

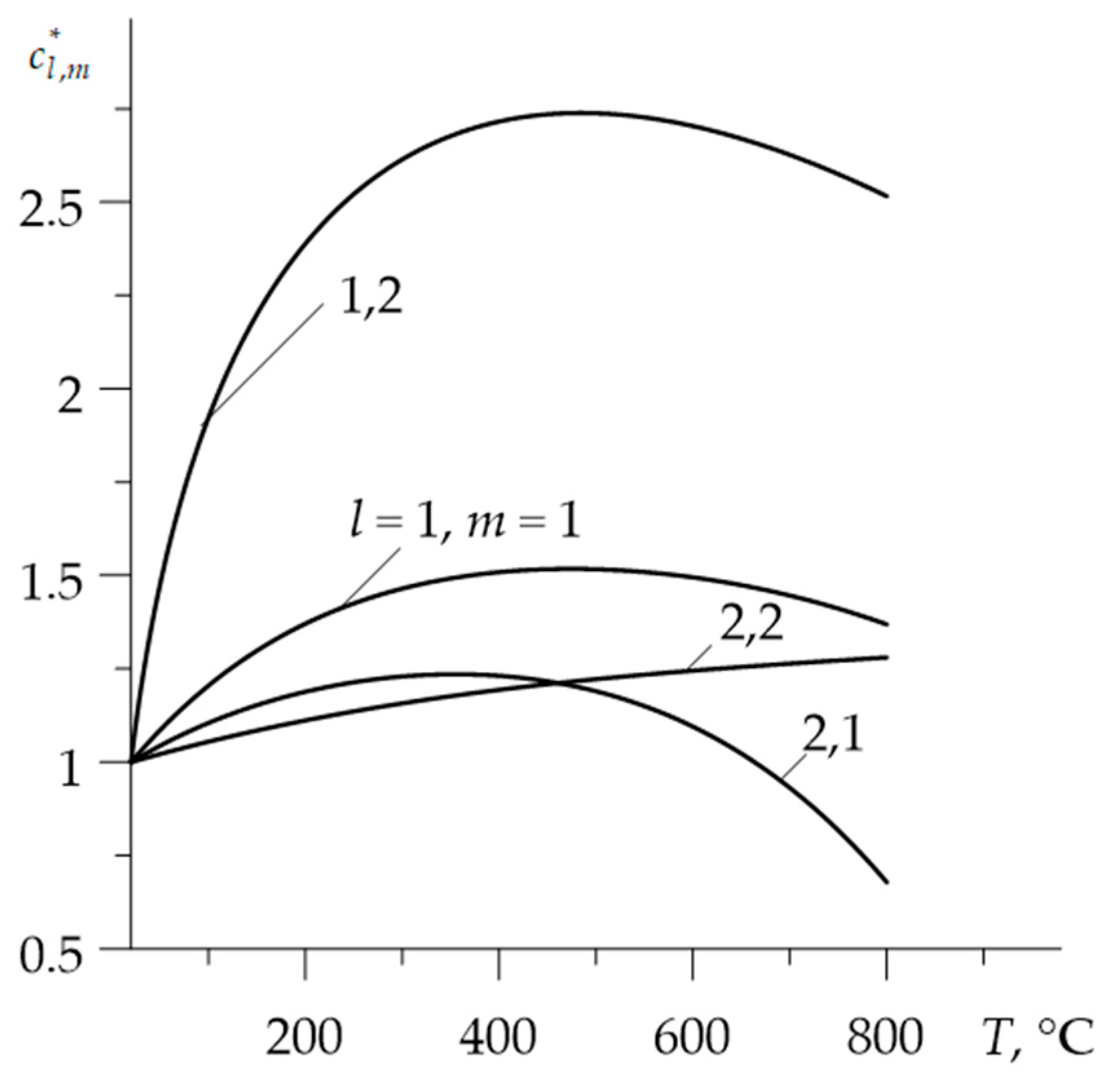
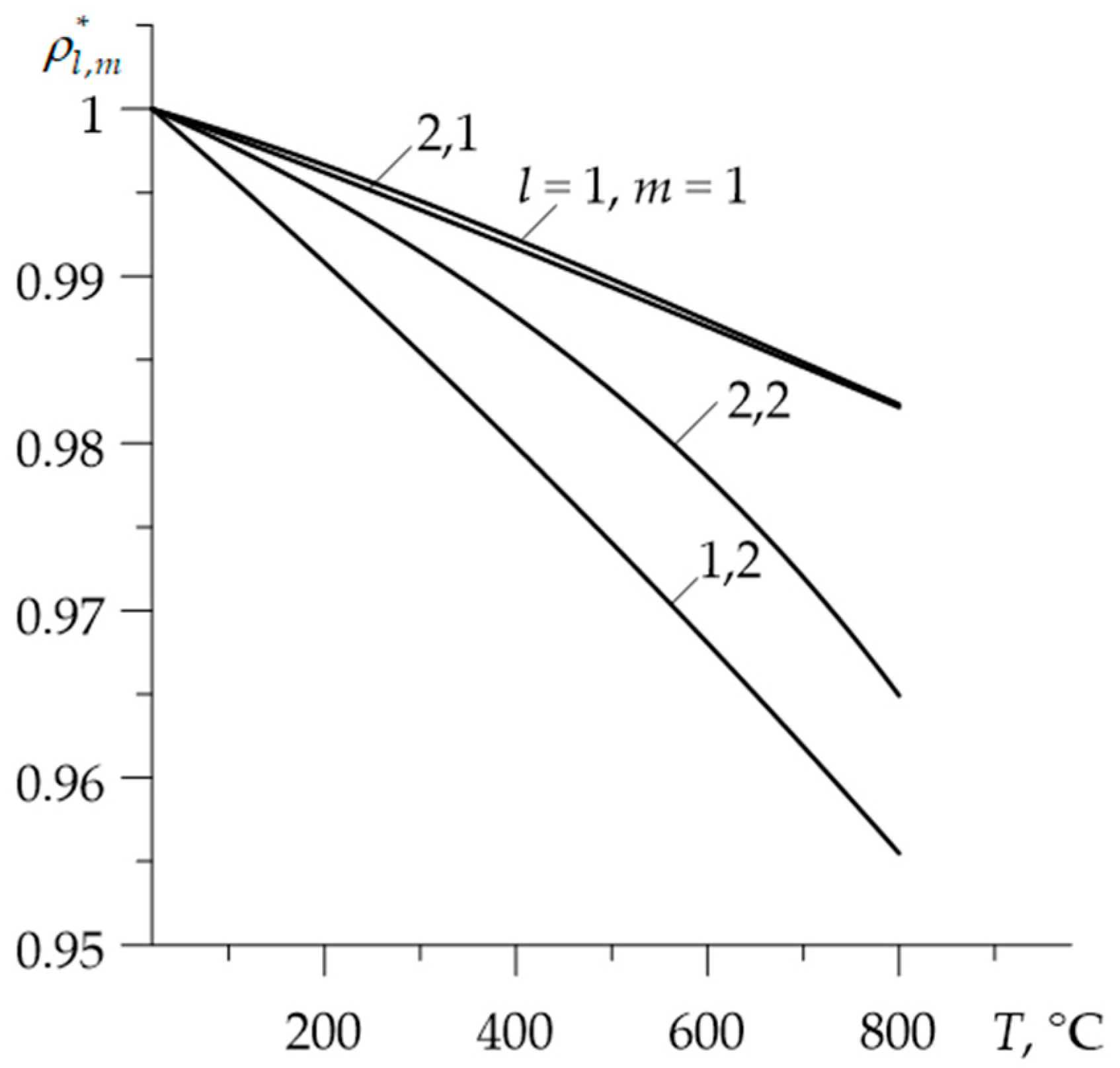
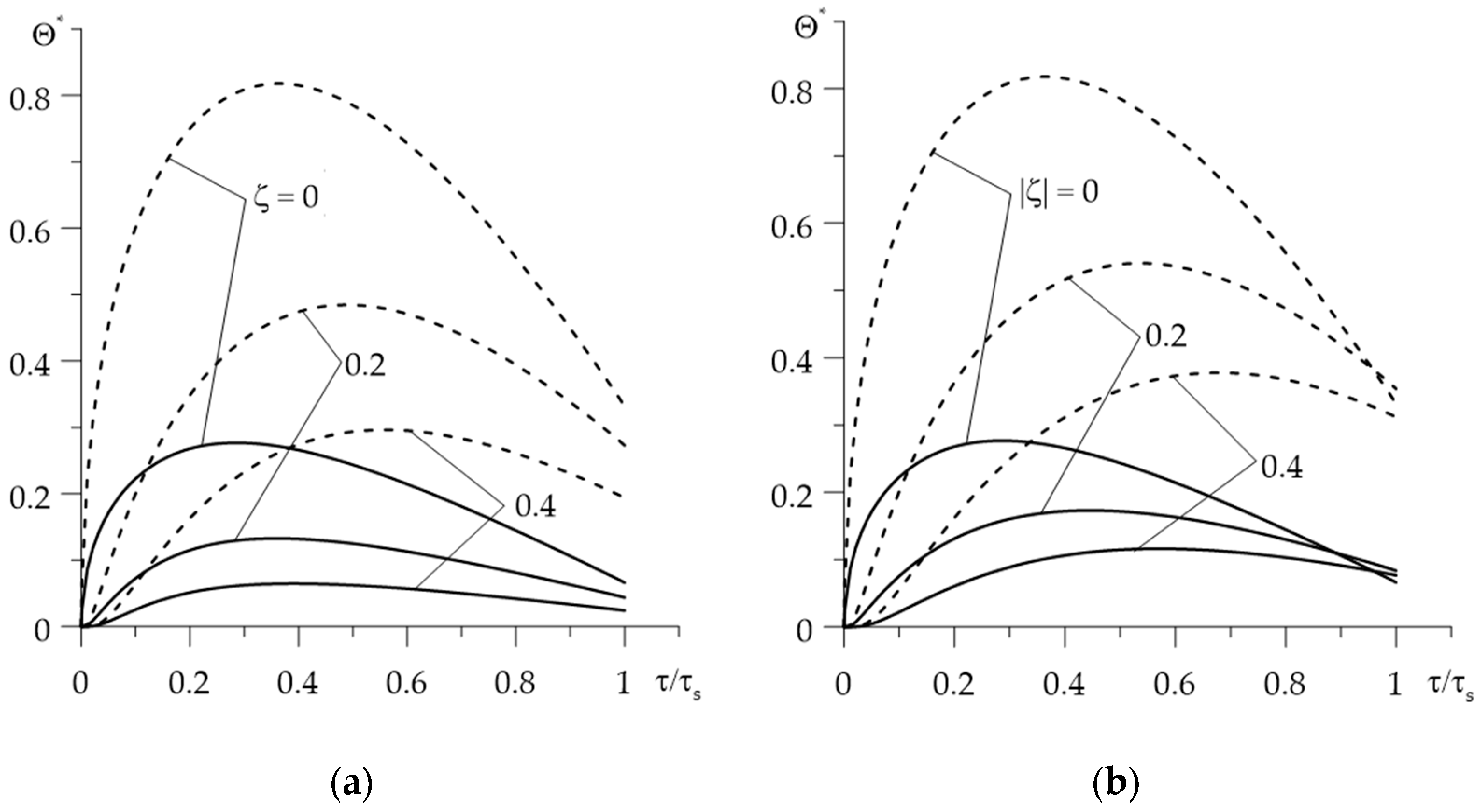
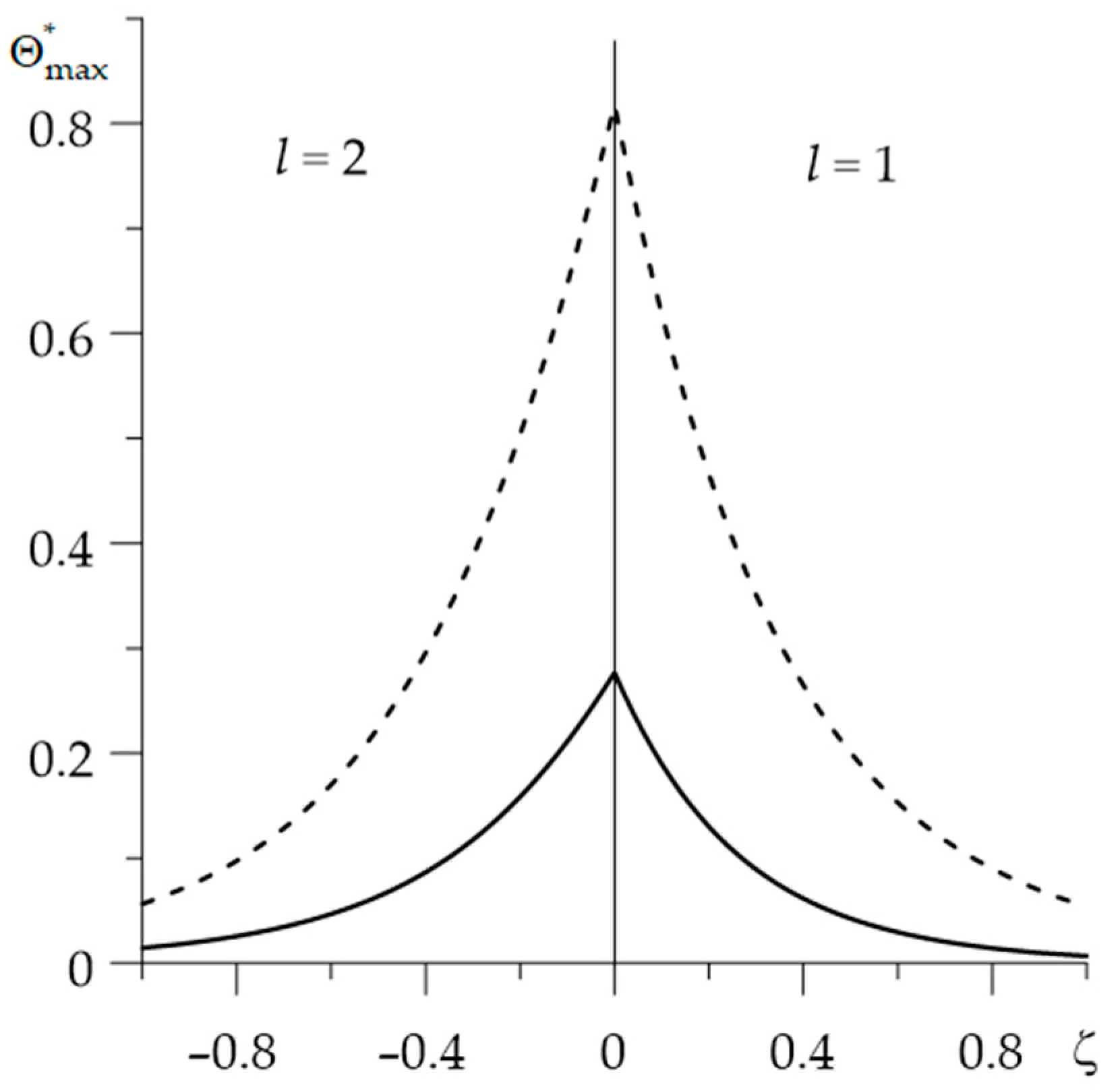
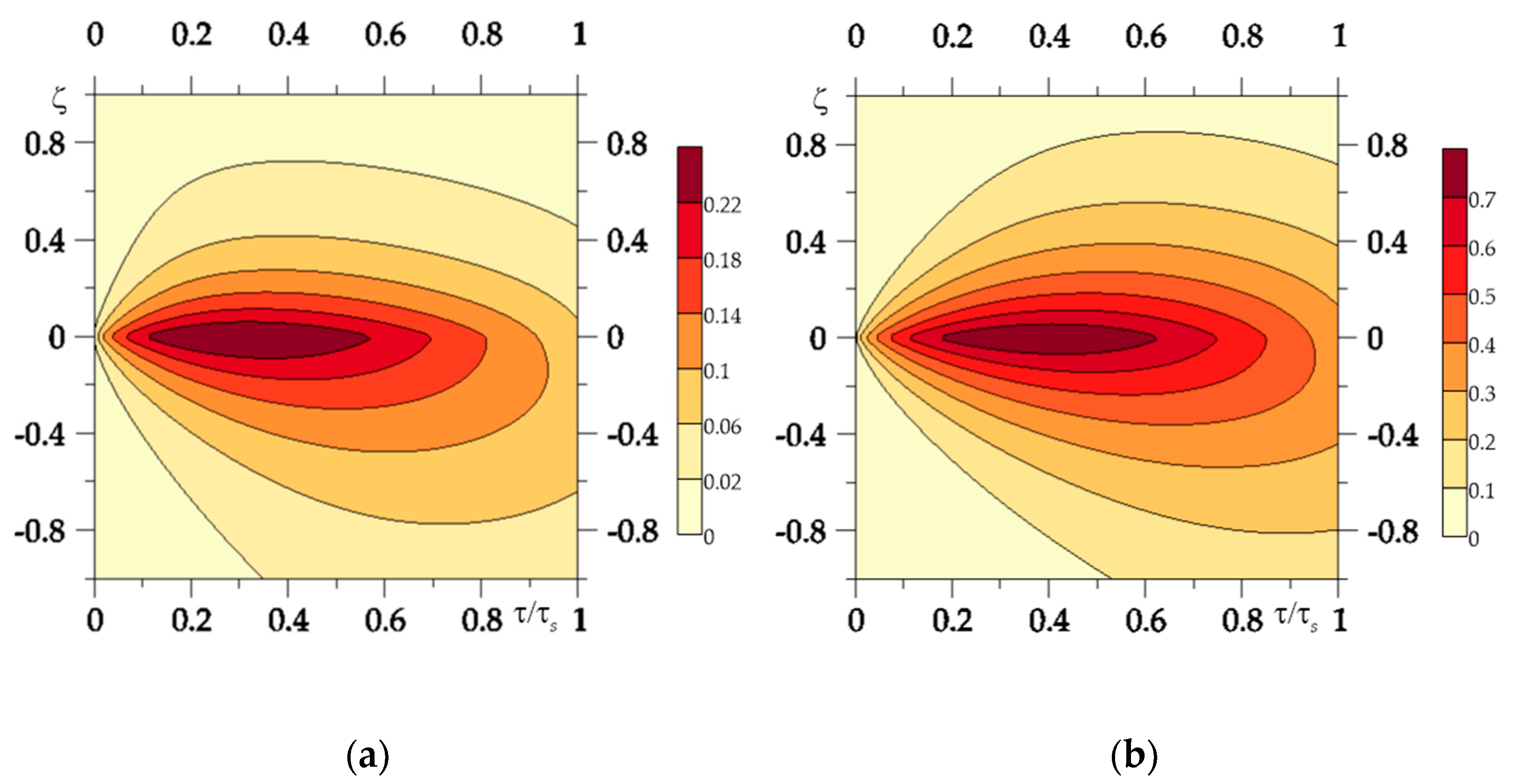
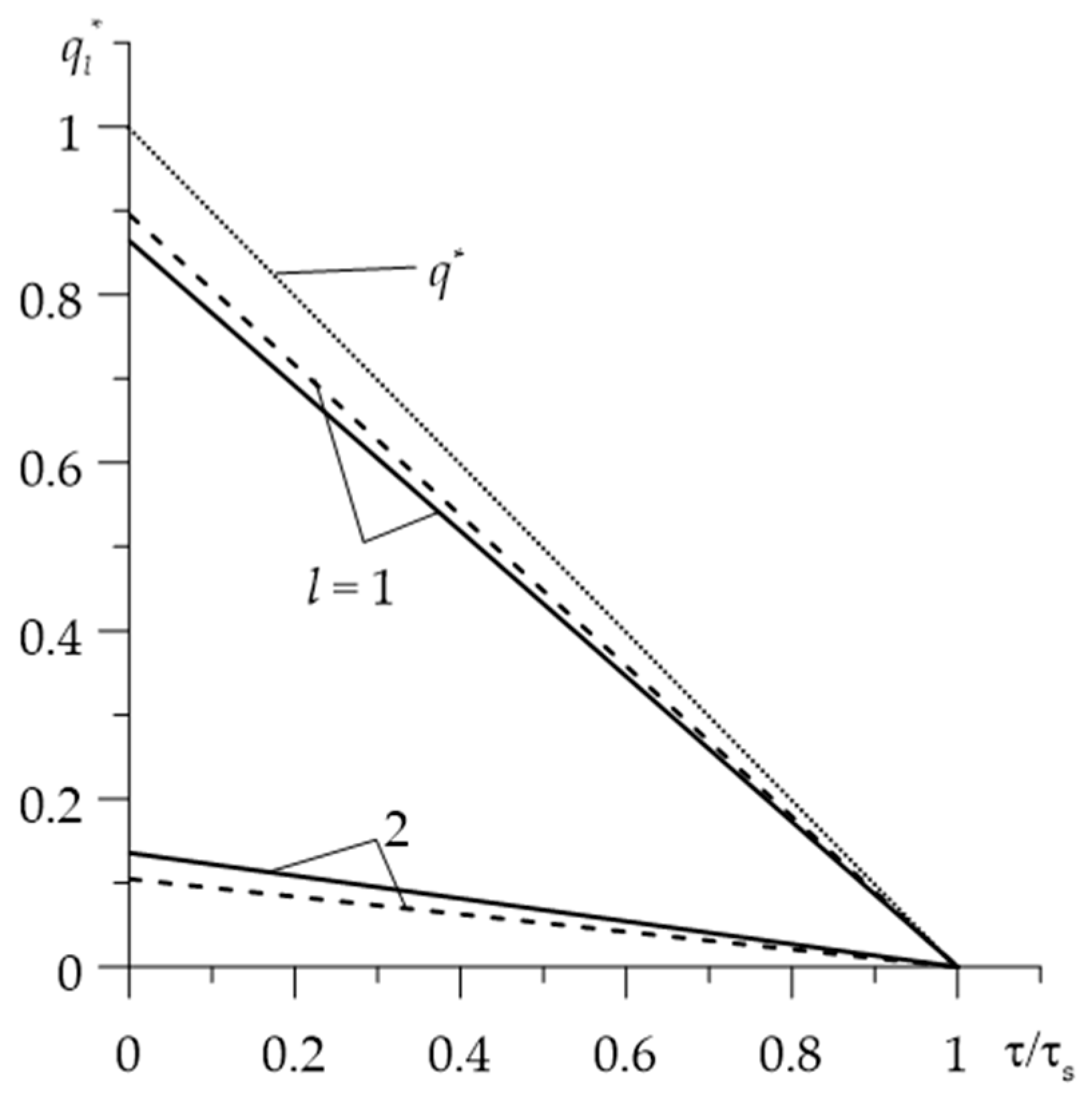
| Friction Coefficient | Nominal Pressure | Initial Sliding Speed | Initial Kinetic Energy | Outer Radius | Inner Radius | Initial Temperature |
|---|---|---|---|---|---|---|
| 0.27 | 0.602 | 23.8 | 103.54 | 37.5 | 26.5 | 20 |
| Element Index | Material Index | Material | Thermal Conductivity | Specific Heat Capacity | Density |
|---|---|---|---|---|---|
| l = 1 | m = 1 | Al2O3 | 37.24 | 727.29 | 3990.92 |
| m = 2 | Cu | 402.65 | 147.35 | 8947.92 | |
| l = 2 | m = 1 | ZrO2 | 1.94 | 452.83 | 6102.16 |
| m = 2 | Ti-6Al-4V | 6.87 | 538.08 | 4431.79 |
| Element Index | l = 1 | l = 2 |
|---|---|---|
| 437.3 | 495.5 | |
| 6469.4 | 5267 | |
| 13.2 | 0.743 | |
| 2.381 | 1.266 | |
| 21.854 | 5.193 | |
| 0.3127 | 0.0605 | |
| 0.896 | 0.104 |
| Element Index | Material Index | Material | Thermal Conductivity | Specific Heat Capacity | Density |
|---|---|---|---|---|---|
| l = 1 | m = 1 | Al2O3 | 10.19 | 1097.93 | 3945.59 |
| m = 2 | Cu | 367.15 | 401.89 | 8690.20 | |
| l = 2 | m = 1 | ZrO2 | 1.99 | 552.67 | 6069.84 |
| m = 2 | Ti-6Al-4V | 9.57 | 615.44 | 4399.06 |
| Element Index | l = 1 | l = 2 |
|---|---|---|
| 749.7 | 584.9 | |
| 6317.2 | 5233.8 | |
| 2.15 | 0.65 | |
| 3.585 | 1.583 | |
| 8.834 | 4.854 | |
| 0.1234 | 0.0562 | |
| 0.863 | 0.137 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yevtushenko, A.; Topczewska, K.; Zamojski, P. Influence of Thermal Sensitivity of Functionally Graded Materials on Temperature during Braking. Materials 2022, 15, 963. https://doi.org/10.3390/ma15030963
Yevtushenko A, Topczewska K, Zamojski P. Influence of Thermal Sensitivity of Functionally Graded Materials on Temperature during Braking. Materials. 2022; 15(3):963. https://doi.org/10.3390/ma15030963
Chicago/Turabian StyleYevtushenko, Aleksander, Katarzyna Topczewska, and Przemysław Zamojski. 2022. "Influence of Thermal Sensitivity of Functionally Graded Materials on Temperature during Braking" Materials 15, no. 3: 963. https://doi.org/10.3390/ma15030963
APA StyleYevtushenko, A., Topczewska, K., & Zamojski, P. (2022). Influence of Thermal Sensitivity of Functionally Graded Materials on Temperature during Braking. Materials, 15(3), 963. https://doi.org/10.3390/ma15030963








