Identification of Vibration Modes and Wave Propagation of Operational Rails by Multipoint Hammering and Reciprocity Theorem
Abstract
1. Introduction
- In rail mode identification, the number of accelerometers (spatial resolution) is insufficient, especially when identifying rail mode shapes. Even in the latest literature [20,21] using a relatively large number of sensors, the mode shape of the pinned-pinned mode has not been completely experimentally identified.
- The verification is limited to short-length rails in the laboratory, and the influence on experimental conditions such as the free ends cannot be denied. In particular, the identification of the wavelength-frequency relationship of actual rails is deeply related to the rail corrugation in [4], but they have remained unknown until now.
2. Methods of Measurements and Analysis
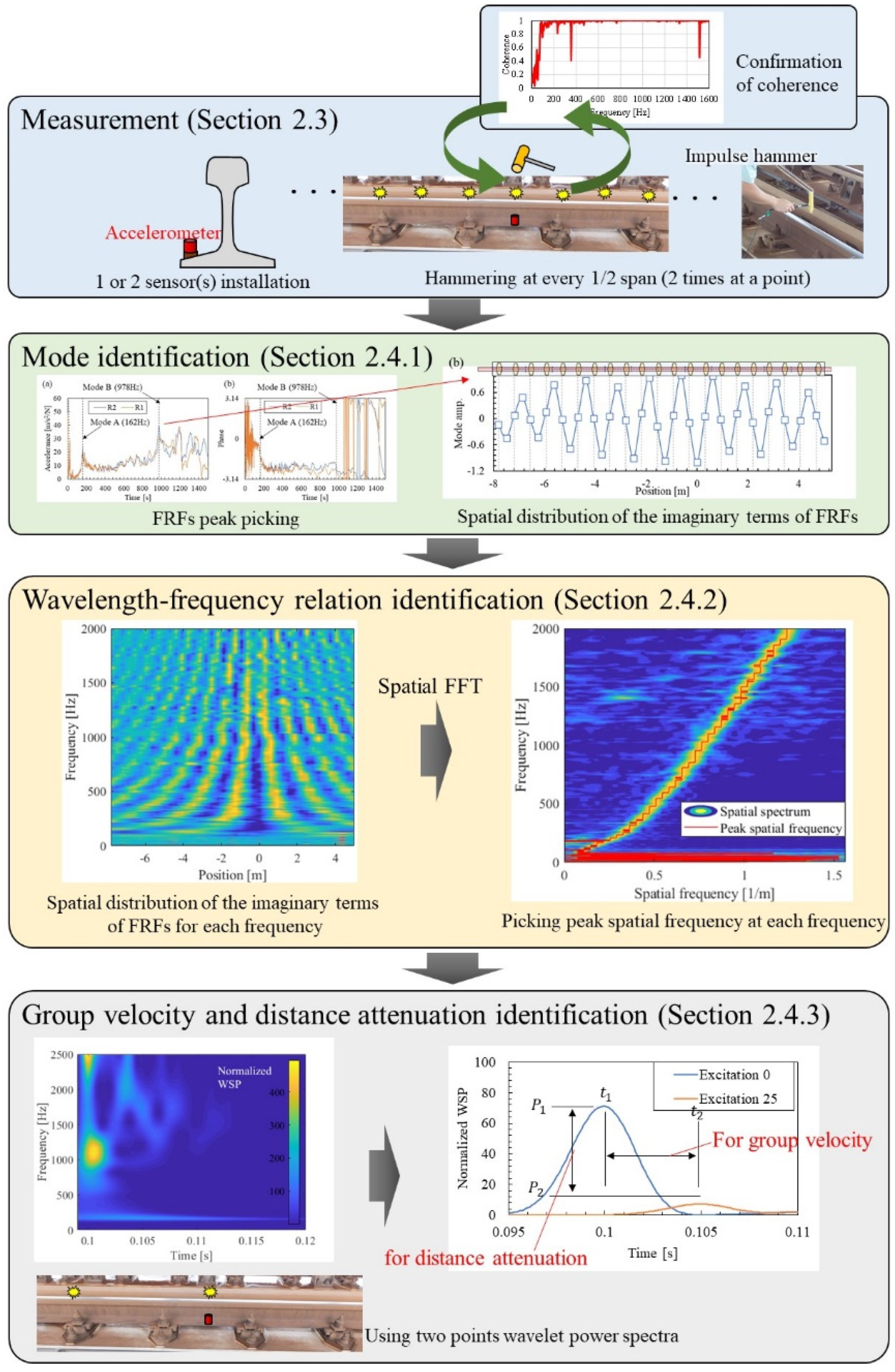
2.1. Outline of the Proposed Method
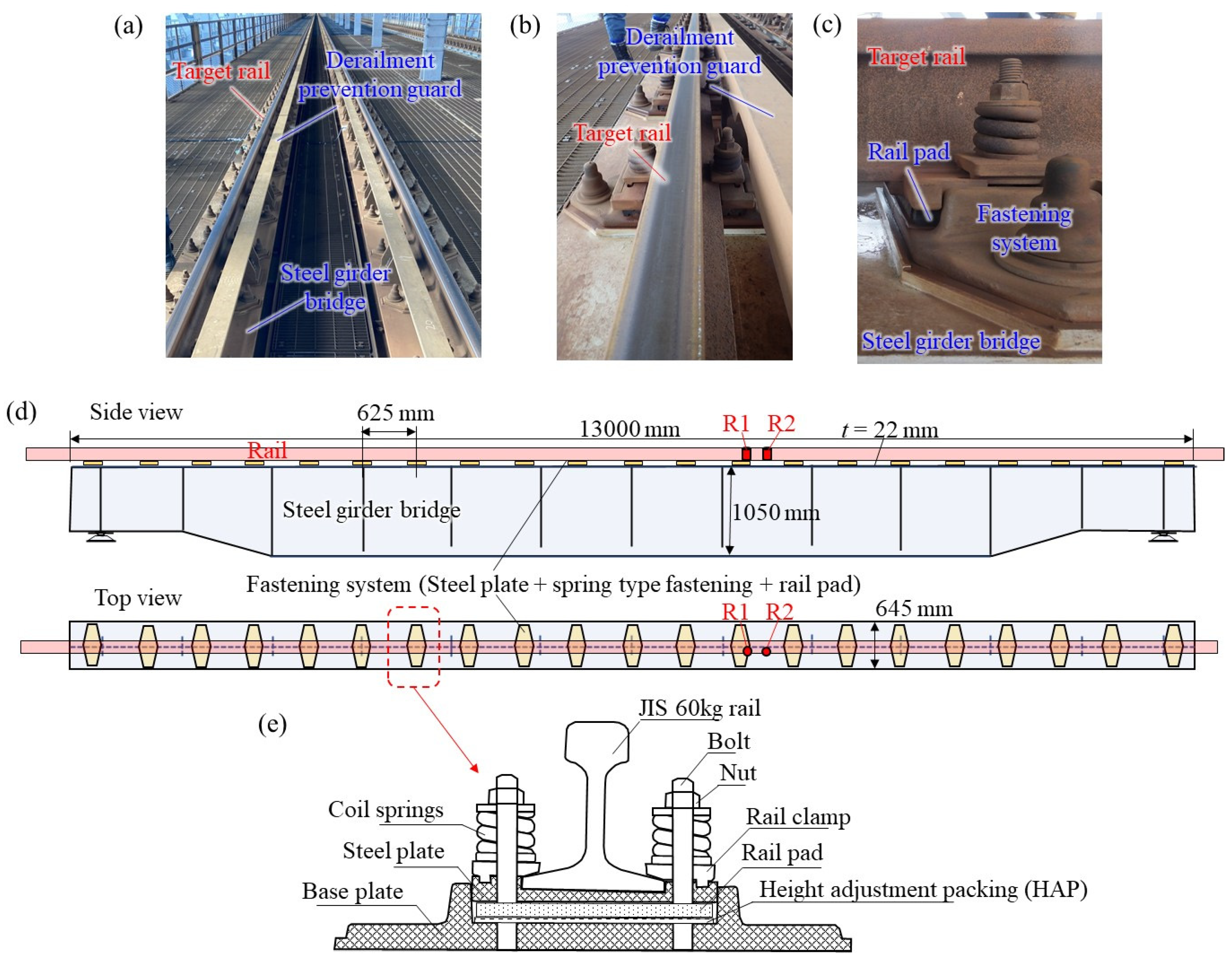
2.2. Tested Rail
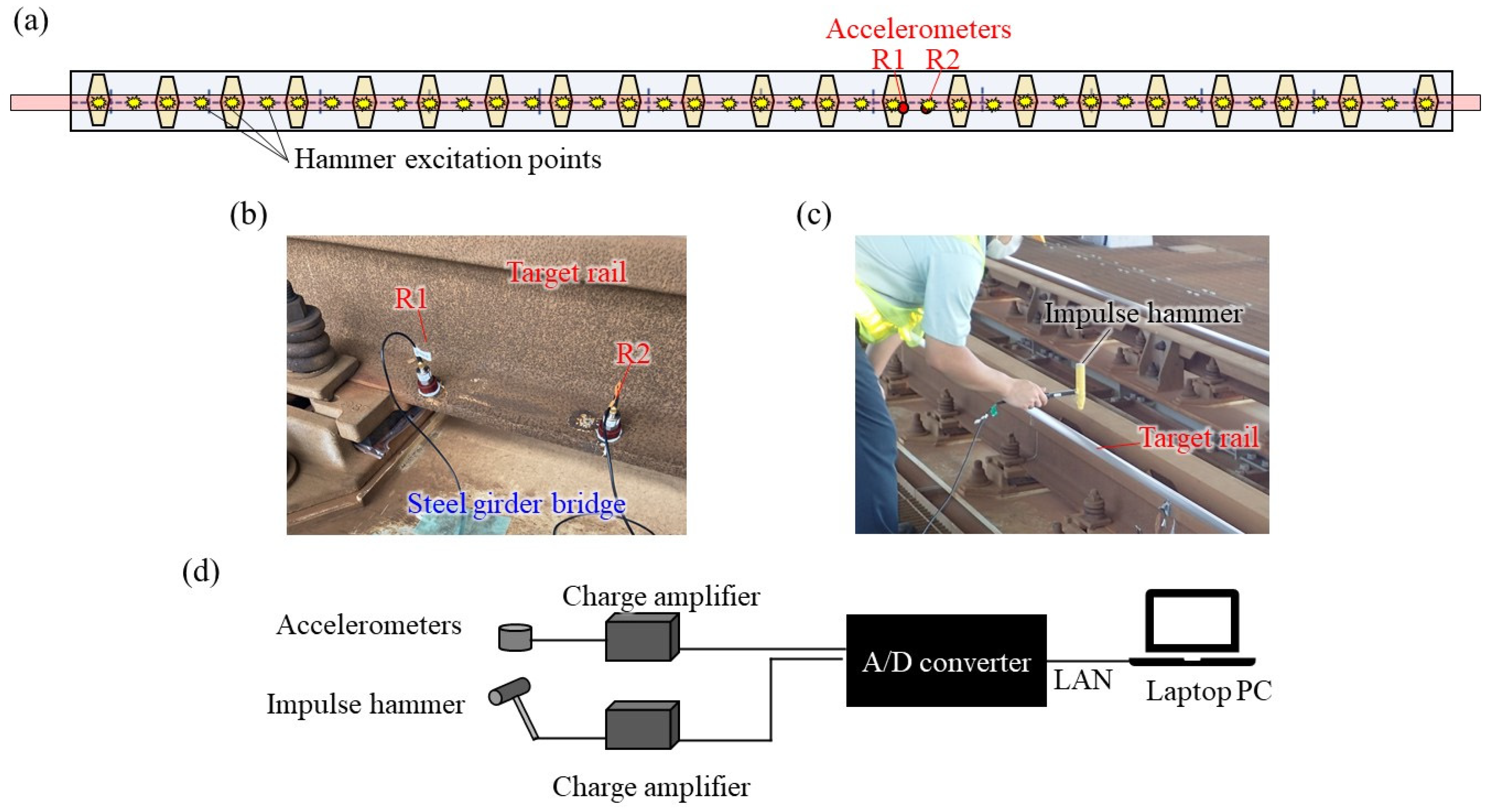
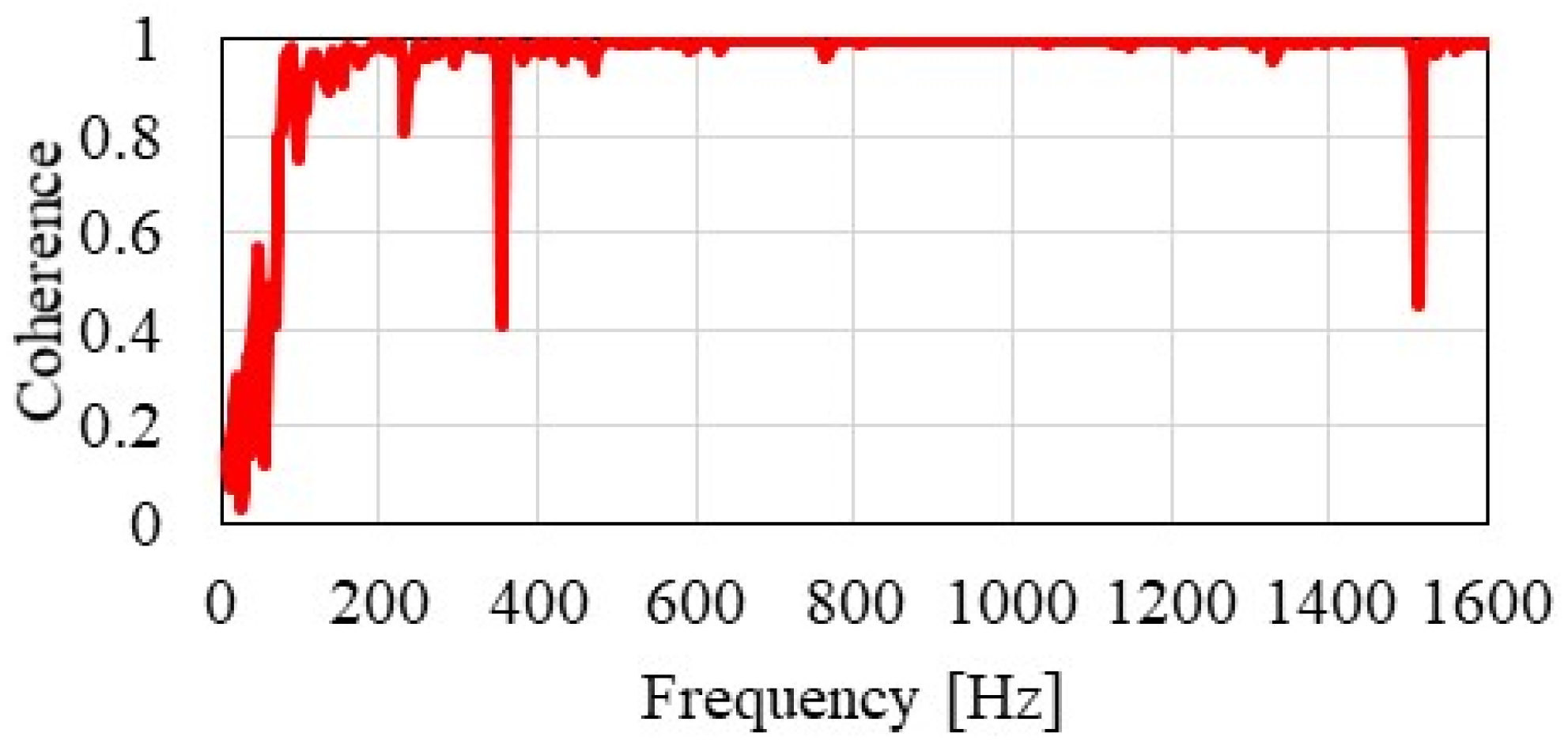
2.3. Measurement Methods
2.4. Identification Methods of Vibration Modes and Wave Propagation
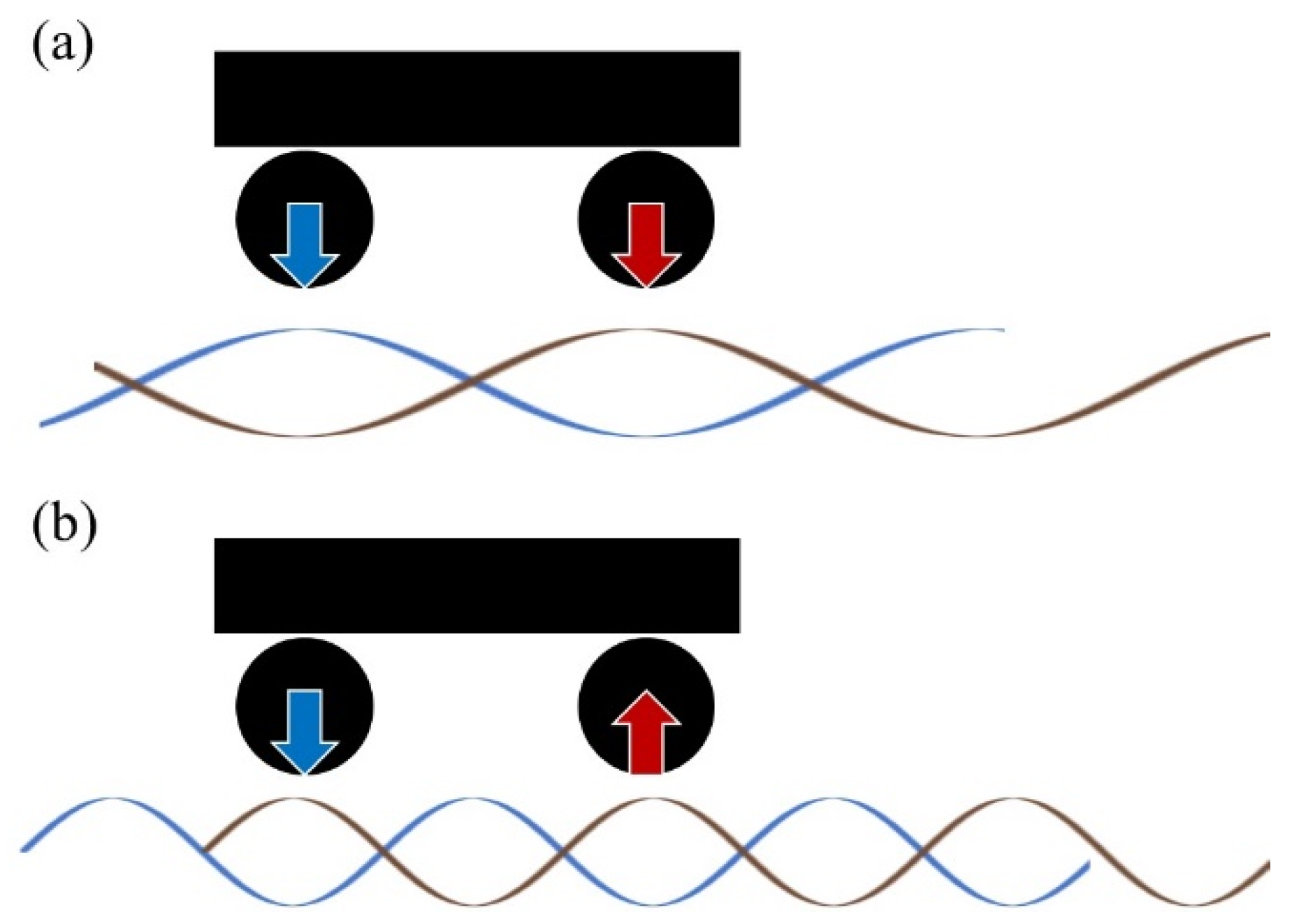
2.4.1. Modal Identification Method by Multipoint Vibration and Reciprocity Theorem
2.4.2. Wavelength-Frequency Relation Identification Method by Multipoint Vibration and Reciprocity Theorem
2.4.3. Group Velocity and Distance Attenuation Identification Method by SMEW Measurement
3. Results of Measurements and Identifications
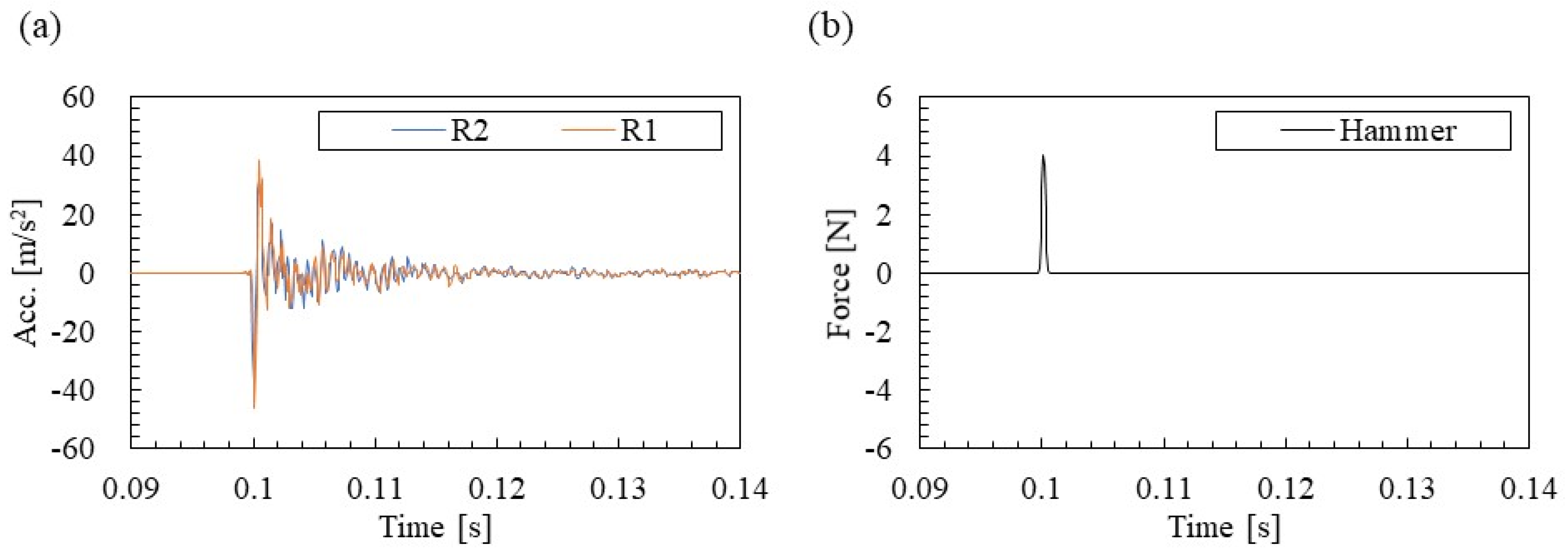
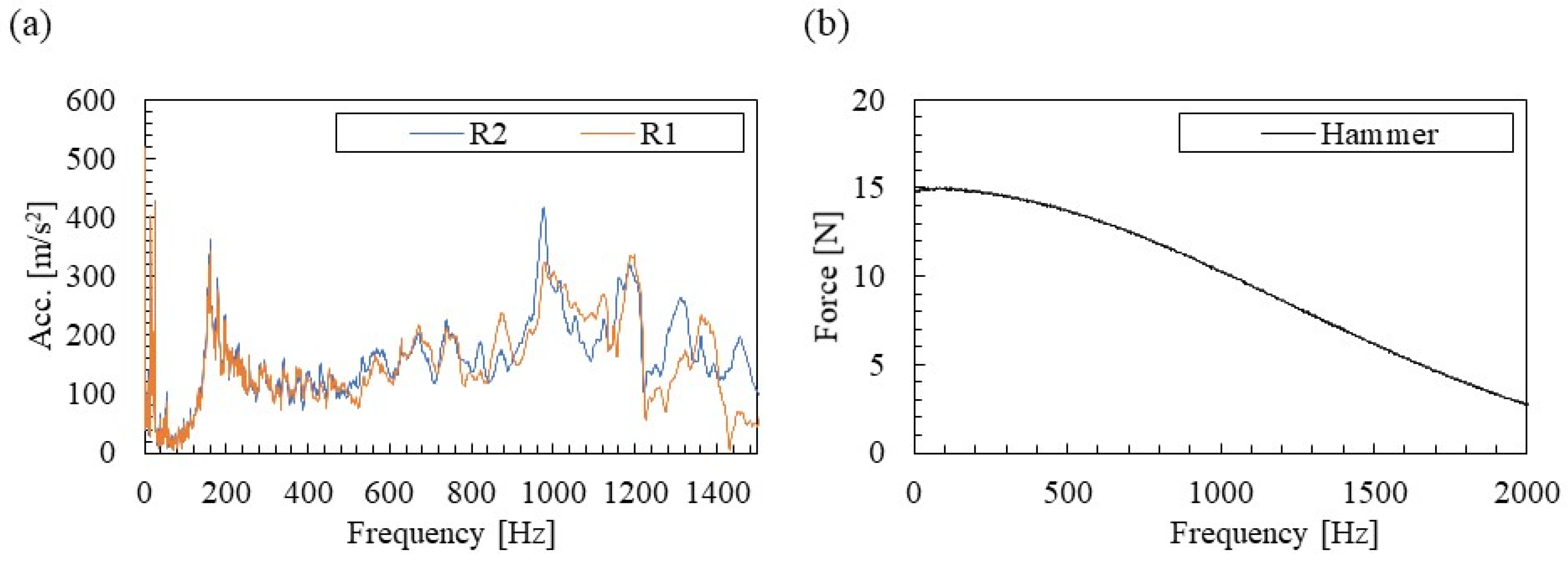
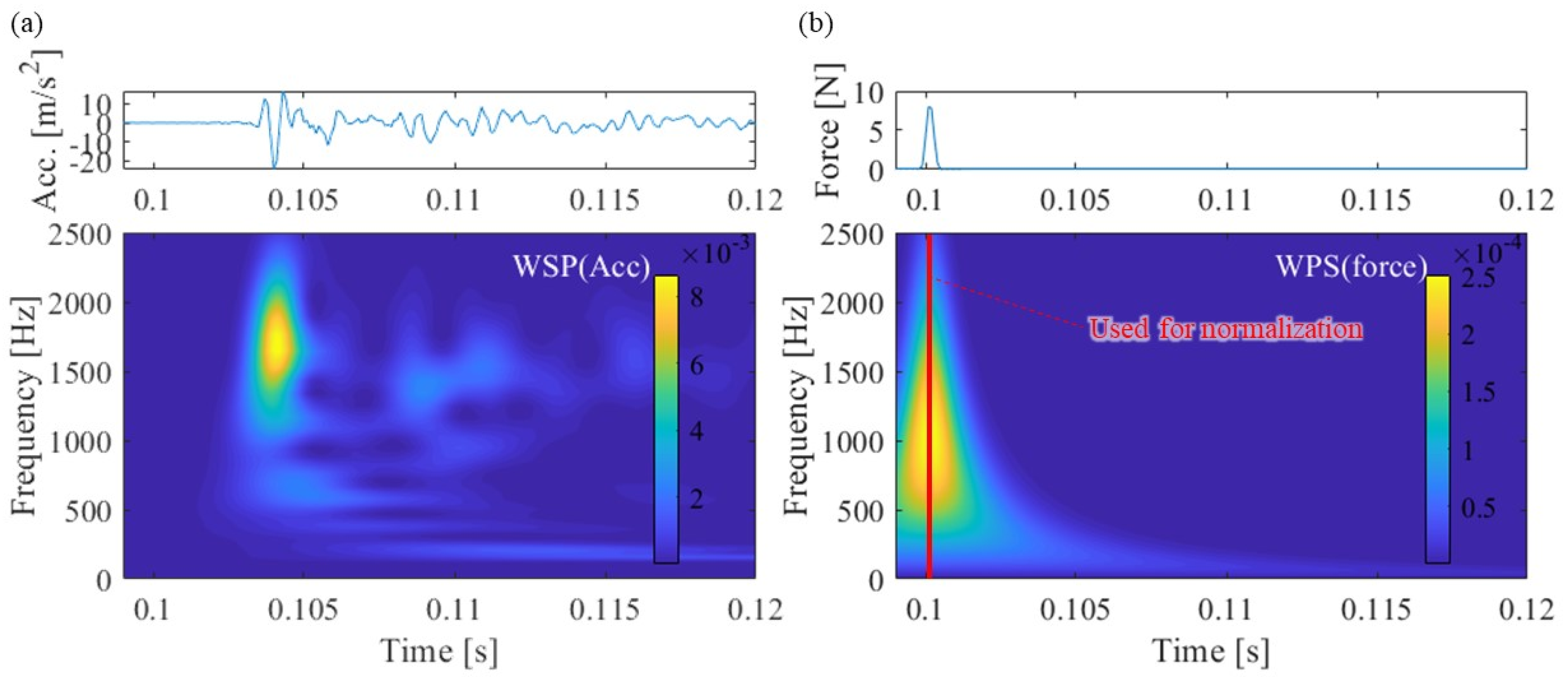
3.1. Acceleration and Excitation Force Measurement Results
3.2. Eigen Frequency Identification Results
3.3. Mode Shape Identification Results
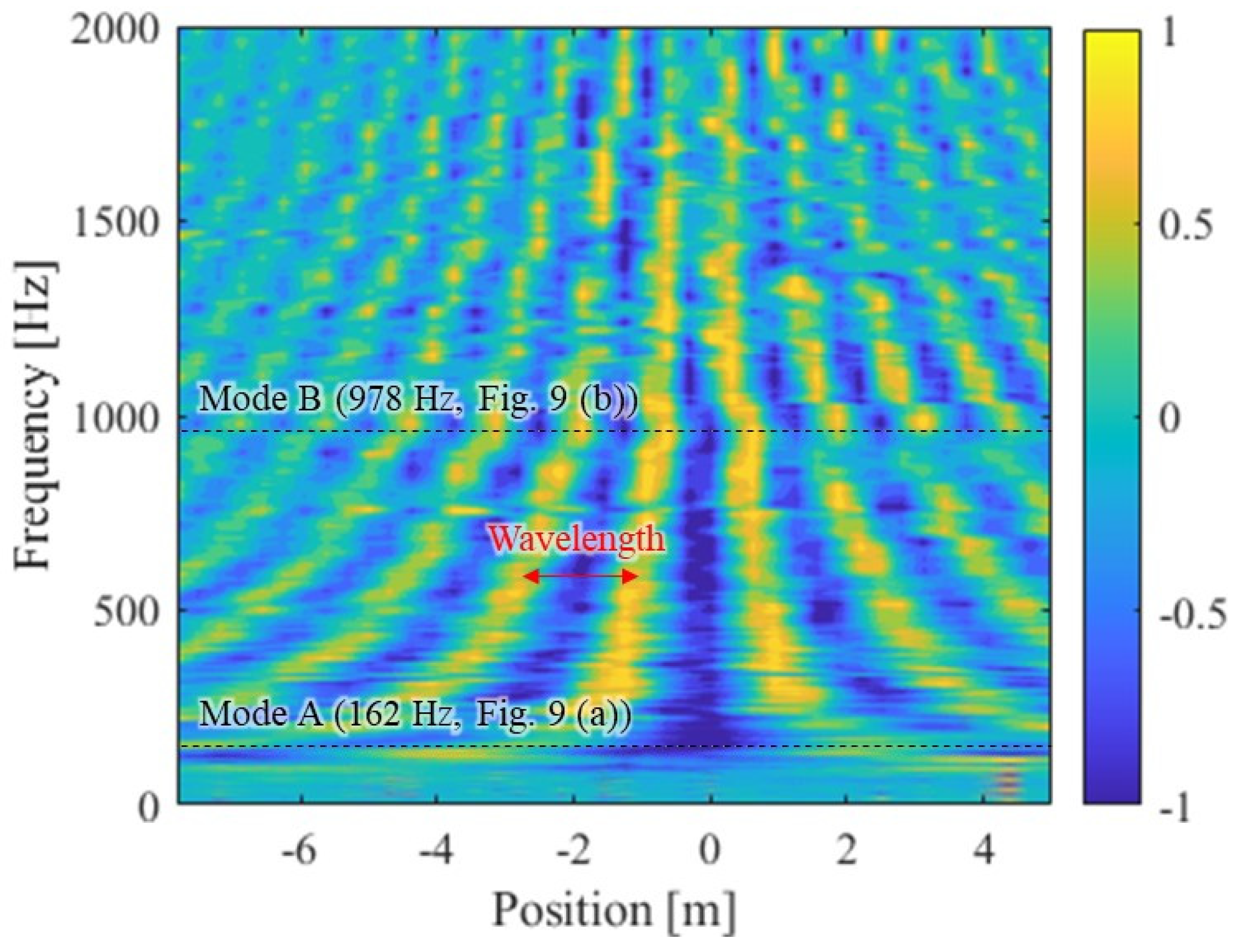
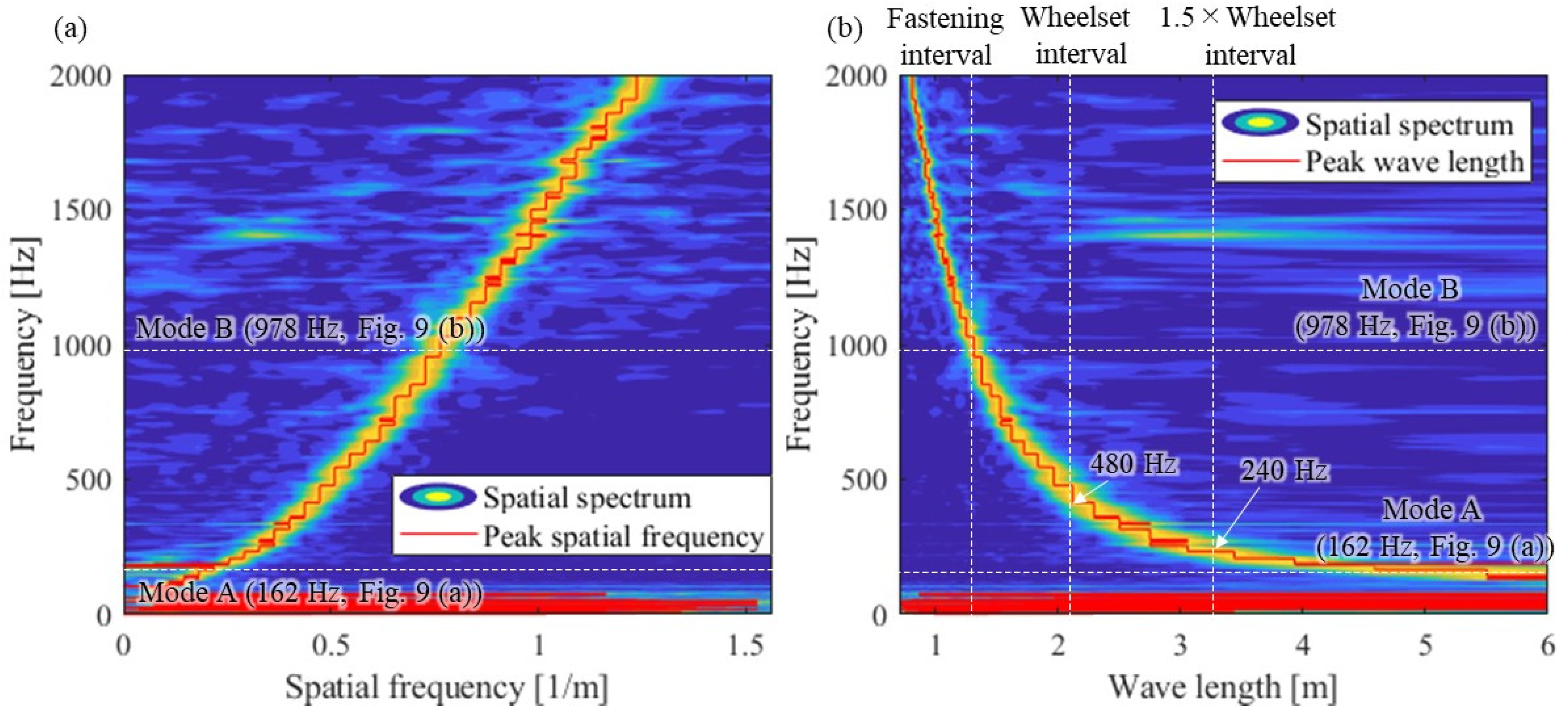
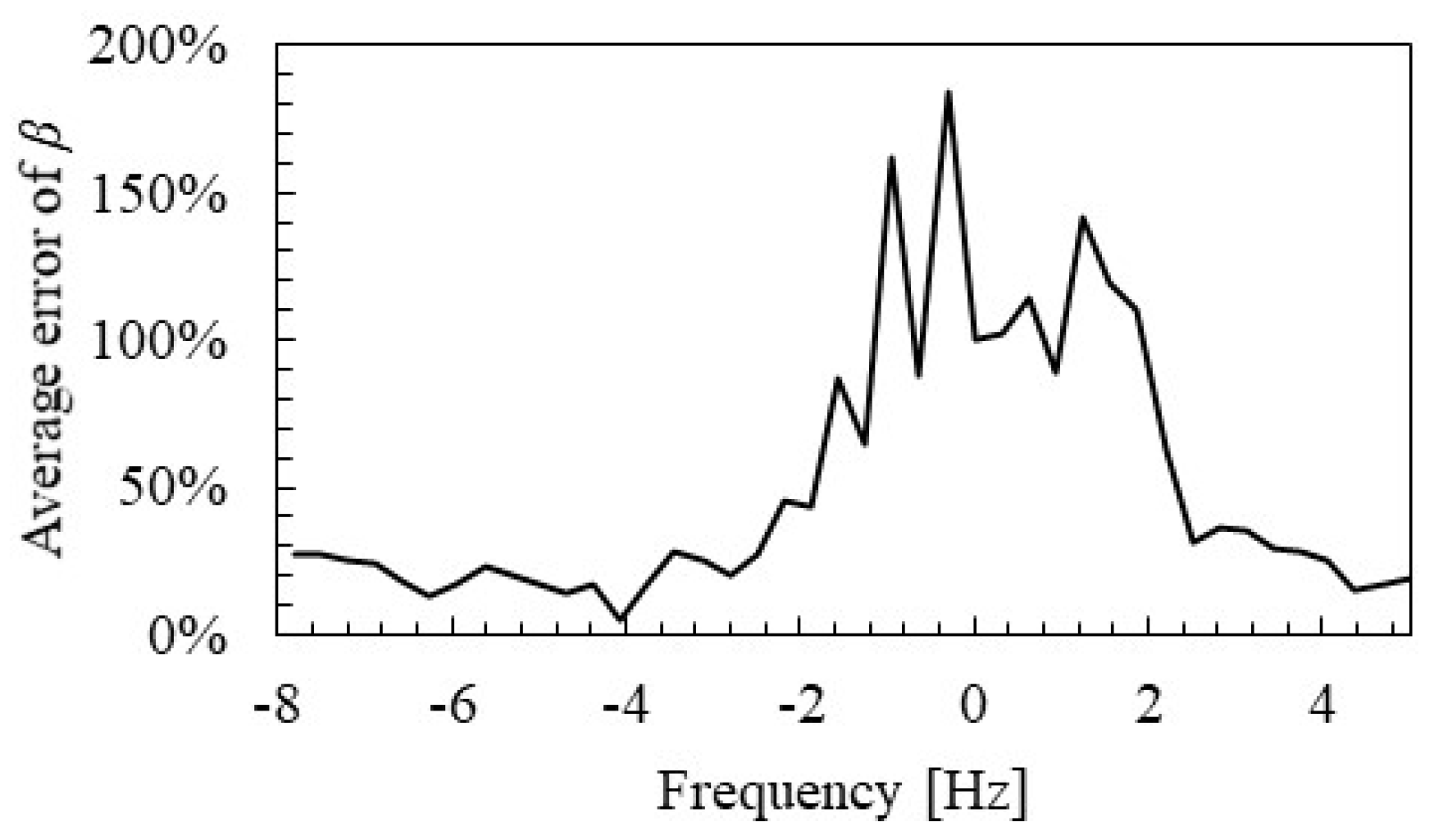
3.4. Wavelength-Frequency Relation Identification Results
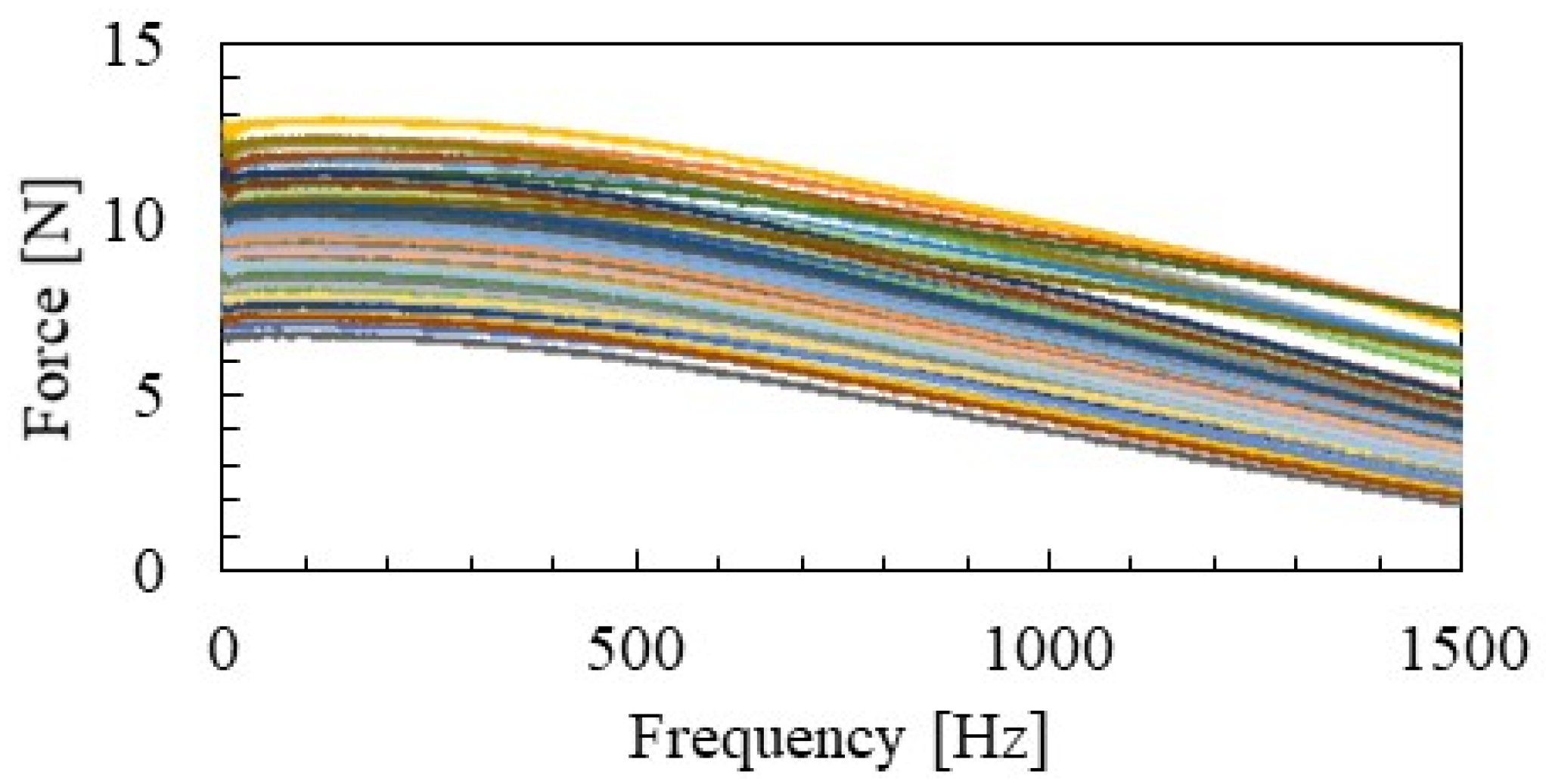
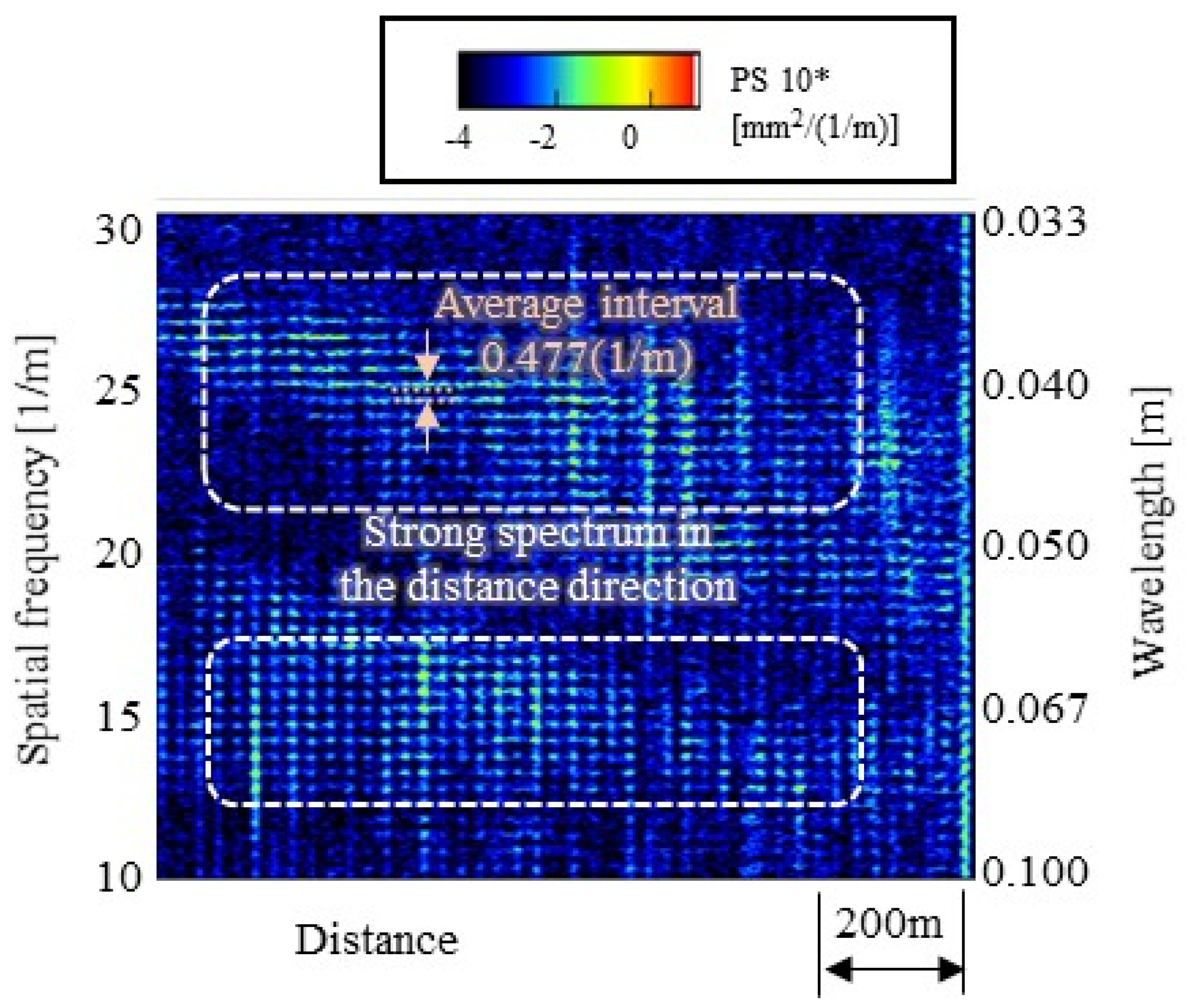
3.5. SMEW Measurement Results: NWPS
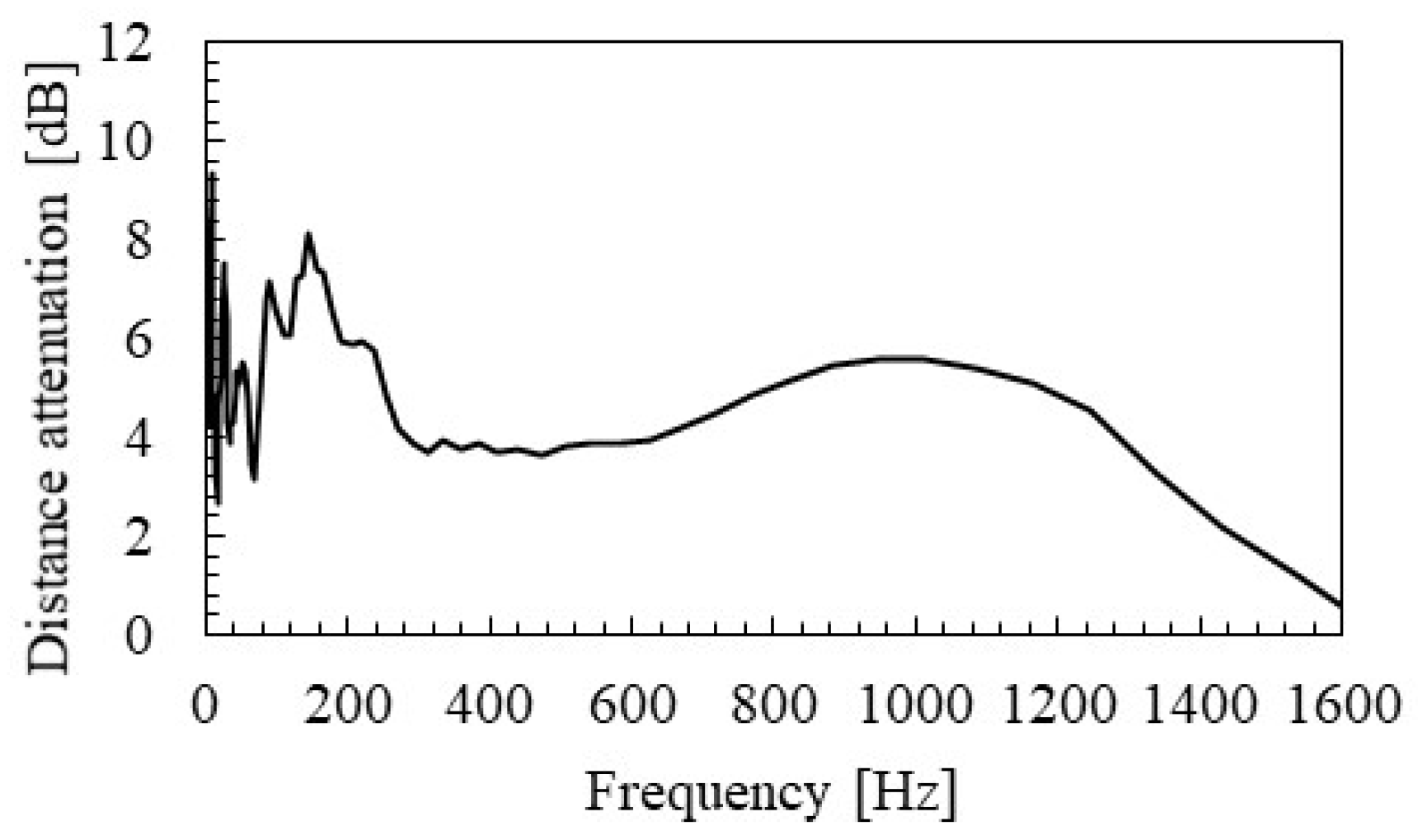
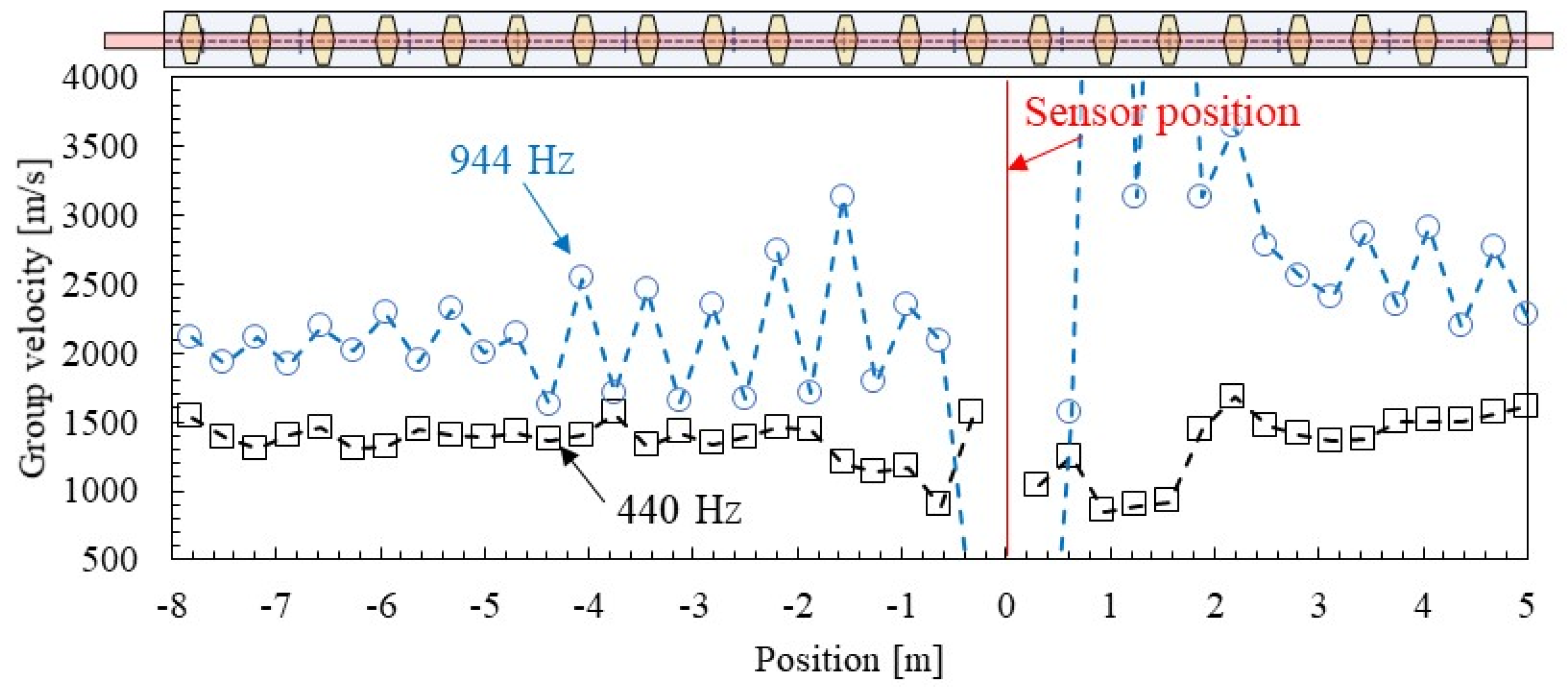
3.6. SMEW Measurement Results: Group Velocity and Distance Attenuation
4. Discussions
4.1. Spatial Distribution of Group Velocity and Distance Attenuation
4.2. Measurement Distance of Group Velocity and Distance Attenuation
4.3. Generation Mechanism of the Rail Corrugation
5. Conclusions
- The new method developed to identify the vibration modes and wave propagation characteristics by multipoint hammering uses the reciprocity theorem to exchange the excitation and the measurement points, solving the existing method’s number-of-sensors limitation problem.
- It was empirically clarified that the pinned–pinned mode and wave propagation characteristics such as the wavelength-frequency relation up to approximately 1500 Hz can be identified only from the field tests by the high-density hammering point arrangement.
- The identified eigen frequencies were in good agreement with simple theoretical calculations.
- The SMEW measuring method that uses WPS normalized by excitation force at each frequency was proposed as a method for identifying group velocity and distance attenuation caused by multipoint hammering.
- The group velocity and distance attenuation of an actual rail identified by SMEW measurement significantly increased due to the influence of the stationary mode near the eigen modes.
- In addition, the group velocity and distance attenuation can be identified with high accuracy by the distance between the hammering point and the measurement point of over 2 m.
- From the identified wavelength-frequency relationship and the rail irregularity measurement result, the experiment confirmed that the rail corrugation with a wavelength of approximately 0.04 m was caused by the interference of the waves generated between the two wheelsets in a bogie.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hempelmann, K.; Knothe, K. An extended linear model for the prediction of short pitch corrugation. Wear 1996, 191, 161–169. [Google Scholar] [CrossRef]
- Grassie, S.L.; Kalousek, J. Rail corrugation: Characteristics, causes and treatments. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 1993, 7, 57–68. [Google Scholar] [CrossRef]
- Grassie, S.L. Rail corrugation: Characteristics, causes, and treatments. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2009, 223, 581–596. [Google Scholar] [CrossRef]
- Manabe, K. A hypothesis on a wavelength fixing mechanism of rail corrugation. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2000, 14, 21–26. [Google Scholar] [CrossRef]
- Thompson, D.J. Wheel-rail noise generation, part III: Rail vibration. J. Sound Vib. 1993, 61, 421–446. [Google Scholar] [CrossRef]
- Thompson, D.; Hemsworth, B.; Vincent, N. Experimental validation of the TWINS prediction program for rolling noise, part 1: Description of the model and method. J. Sound Vib. 1996, 193, 123–135. [Google Scholar] [CrossRef]
- Thompson, D.; Fodiman, P.; Mahé, H. Experimental validation of the TWINS prediction program for rolling noise, part 2: Results. J. Sound Vib. 1996, 193, 137–147. [Google Scholar] [CrossRef]
- Ilias, H. The influence of railpad stiffness on wheelset/track interaction and corrugation growth. J. Sound Vib. 1999, 227, 935–948. [Google Scholar] [CrossRef]
- Giannakos, K. Modeling the influence of short wavelength defects in a railway track on the dynamic behavior of the Non-Suspended Masses. Mech. Syst. Signal Process. 2016, 68–69, 68–83. [Google Scholar] [CrossRef]
- Grassie, S.L.; Gregory, R.W.; Harrison, D.; Johnson, K.L. The Dynamic Response of Railway Track to High Frequency Vertical Excitation. J. Mech. Eng. Sci. 1982, 24, 77–90. [Google Scholar] [CrossRef]
- Xu, L.; Zhai, W. A three-dimensional model for train-track-bridge dynamic interactions with hypothesis of wheel-rail rigid contact. Mech. Syst. Signal Process. 2019, 132, 471–489. [Google Scholar] [CrossRef]
- Koziol, P. Experimental validation of wavelet based solution for dynamic response of railway track subjected to a moving train. Mech. Syst. Signal Process. 2016, 79, 174–181. [Google Scholar] [CrossRef]
- Gavrić, L. Computation of propagative waves in free rail using a finite element technique. J. Sound Vib. 1995, 185, 531–543. [Google Scholar] [CrossRef]
- Cettour-Janet, R.; Barbarulo, A.; Letourneaux, F.; Puel, G. An Arnoldi reduction strategy applied to the semi-analytical finite element method to model railway track vibrations. Mech. Syst. Signal Process. 2019, 16, 997–1016. [Google Scholar] [CrossRef]
- Sale, M.; Rizzo, P.; Marzani, A. Semi-analytical formulation for the guided waves-based reconstruction of elastic moduli. Mech. Syst. Signal Process. 2011, 25, 2241–2256. [Google Scholar] [CrossRef]
- Setshedi, I.I.; Loveday, P.W.; Long, C.S.; Wilke, D.N. Estimation of rail properties using semi-analytical finite element models and guided wave ultrasound measurements. Ultrasonics 2019, 96, 240–252. [Google Scholar] [CrossRef]
- Oregui, M.; Li, Z.; Dollevoet, R. An investigation into the vertical dynamics of tracks with monoblock sleepers with a 3D finite-element model. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2015, 230, 891–908. [Google Scholar] [CrossRef]
- Knothe, K.; Strzyzakowski, Z.; Willner, K. Rail Vibrations in the High Frequency Range. J. Sound Vib. 1994, 169, 111–123. [Google Scholar] [CrossRef]
- Ryue, J.; Thompson, D.; White, P. Investigations of propagating wave types in railway tracks at high frequencies. J. Sound Vib. 2008, 315, 157–175. [Google Scholar] [CrossRef]
- Zhang, P.; Li, S.; Núñez, A.; Li, Z. Multimodal dispersive waves in a free rail: Numerical modeling and experimental investigation. Mech. Syst. Signal Process. 2021, 50, 107305. [Google Scholar] [CrossRef]
- Zhang, P.; Li, S.; Núñez, A.; Li, Z. Vibration modes and wave propagation of the rail under fastening constraint. Mech. Syst. Signal Process. 2021, 160, 107933. [Google Scholar] [CrossRef]
- Hayashi, T.; Song, W.-J.; Rose, J.L. Guided wave dispersion curves for a bar with an arbitrary cross-section, a rod and rail example. Ultrasonics 2003, 41, 175–183. [Google Scholar] [CrossRef]
- Aktan, A.E.; Farhey, D.N.; Helmicki, A.J.; Brown, D.L.; Hunt, V.J.; Lee, K.-L.; Levi, A. Structural Identification for Condition Assessment: Experimental Arts. J. Struct. Eng. 1997, 123, 1674–1684. [Google Scholar] [CrossRef]
- Van Der Seijs, M.V.; Pasma, E.A.; Bosch, D.D.V.D.; Wernsen, M.W.F. A Benchmark Structure for Validation of Experimental Substructuring, Transfer Path Analysis and Source Characterisation Techniques. Dyn. Coupled Struct. 2017, 68, 295–305. [Google Scholar] [CrossRef]
- Contartese, N.; Nijman, E.; Desmet, W. A procedure to restore measurement induced violations of reciprocity and passivity for FRF-based substructuring. Mech. Syst. Signal Process. 2021, 167, 108556. [Google Scholar] [CrossRef]
- Schmerr, L.W.; Song, J.S. Ultrasonic Nondestructive Evaluation Systems: Models and Measurements; Springer Science & Business Media: Hoboken, NJ, USA, 2007; 602p. [Google Scholar]
- Kubrusly, A.C.; Dixon, S. Application of the reciprocity principle to evaluation of mode-converted scattered shear horizontal (SH) wavefields in tapered thinning plates. Ultrasonics 2021, 17, 106544. [Google Scholar] [CrossRef] [PubMed]
- Yang, Z.; Boogaard, A.; Chen, R.; Dollevoet, R.; Li, Z. Numerical and experimental study of wheel-rail impact vibration and noise generated at an insulated rail joint. Int. J. Impact Eng. 2018, 113, 29–39. [Google Scholar] [CrossRef]
- Oregui, M.; Li, Z.; Dollevoet, R. Identification of characteristic frequencies of damaged railway tracks using field hammer test measurements. Mech. Syst. Signal Process. 2015, 54–55, 224–242. [Google Scholar] [CrossRef]
- Triepaischajonsak, N.; Thompson, D.J.; Jones, C.J.C.; Ryue, J.; Priest, J.A. Ground vibration from trains: Experimental parameter characterization and validation of a numerical model. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2011, 25, 140–153. [Google Scholar] [CrossRef]
- Lombaert, G.; Degrande, G.; Kogut, J.; François, S. The experimental validation of a numerical model for the prediction of railway induced vibrations. J. Sound Vib. 2006, 297, 512–535. [Google Scholar] [CrossRef]
- Foti, S.; Lai, C.G.; Rix, G.J.; Strobbia, C. Surface Wave Methods for Near-Surface Site Characterization; CRC Press: London, UK, 2014. [Google Scholar]
- Takahashi, K.; Nakahata, K. Visualization of dynamic behavior in a structural component based on reciprocity and flaw detection using a selective wave propagation mode. J. Struct. Eng. A 2019, 5A, 264–274. (In Japanese) [Google Scholar]
- Walnut, D.F. An Introduction to Wavelet Analysis; Springer Nature: Berlin/Heidelberg, Germany, 2004. [Google Scholar] [CrossRef]
- di Scalea, F.L.; McNamara, J. Measuring high-frequency wave propagation in railroad tracks by joint time–frequency analysis. J. Sound Vib. 2004, 73, 637–651. [Google Scholar] [CrossRef]
- Aboshi, M.; Tanaka, H. Growth Mechanism and Evolution Process of Rail Corrugation. Q. Rep. RTRI 2021, 62, 55–60. [Google Scholar] [CrossRef]
- Schevenels, M.; Lombaert, G.; Degrande, G.; Clouteau, D. The wave propagation in a beam on a random elastic foundation. Probabilistic Eng. Mech. 2007, 22, 150–158. [Google Scholar] [CrossRef]
- Sheng, X.; Zeng, H.; Zhang, S.Y.; Wang, P. Numerical Study on Propagative Waves in a Periodically Supported Rail Using Periodic Structure Theory. J. Adv. Transp. 2021, 2021, 6635198. [Google Scholar] [CrossRef]
- Oregui, M.; de Man, A.; Woldekidan, M.; Li, Z.; Dollevoet, R. Obtaining railpad properties via dynamic mechanical analysis. J. Sound Vib. 2016, 363, 460–472. [Google Scholar] [CrossRef]
- Aboshi, M.; Tanaka, H. Theoretical analyses of growth mechanism of rail corrugation. Trans. JSME 2019, 5, 875. (In Japanese) [Google Scholar] [CrossRef][Green Version]
- Tanaka, H.; Shimizu, A.; Sano, K. Development and verification of monitoring tools for realizing effective maintenance of rail corrugation. In Proceedings of the 6th IET Conference on Railway Condition Monitoring (RCM 2014), Birmingham, UK, 17–18 September 2014; pp. 1–6. [Google Scholar]
- Matsuoka, K.; Tanaka, H.; Kawasaki, K.; Somaschini, C.; Collina, A. Drive-by methodology to identify resonant bridges using track irregularity measured by high-speed trains. Mech. Syst. Signal Process. 2021, 158, 107667. [Google Scholar] [CrossRef]
- Matsuoka, K.; Tokunaga, M.; Kaito, K. Bayesian estimation of instantaneous frequency reduction on cracked concrete railway bridges under high-speed train passage. Mech. Syst. Signal Process. 2021, 61, 107944. [Google Scholar] [CrossRef]
- Somaschini, C.; Matsuoka, K.; Collina, A. Experimental analysis of a composite bridge under high-speed train passages. Procedia Eng. 2017, 199, 3071–3076. [Google Scholar] [CrossRef]
- Matsuoka, K.; Kaito, K.; Sogabe, M. Bayesian time–frequency analysis of the vehicle–bridge dynamic interaction effect on simple-supported resonant railway bridges. Mech. Syst. Signal Process. 2019, 135, 106373. [Google Scholar] [CrossRef]









| Equipment | Types | Specifications |
|---|---|---|
| Piezoelectric accelerometer | PV95 (Rion CO., LTD., Kokubunji-shi, Japan) | Range: 1–10,000 Hz Sensitivity: 0.714 pC/(m/s2) |
| Charge amplifier | UV16 (Rion CO., LTD., koKubunji-shi, Japan) | Range: 1–15,000 Hz |
| Impulse hammer | 086C03 (PCB Piezotronics, New York, NY, USA) | Sensitivity: 9.68 mV/N Mass: 136 g |
| Recording system | NicDAQ-9189 Ni9233, Ni9239 LabVIEW (National Instruments Japan Corp., Tokyo, Japan) | Sampling frequency: 10,000 Hz |
| Modes | Identified Frequencies | Calculated Frequencies |
|---|---|---|
| A (fundamental mode) | 162.0 Hz | 162.2 Hz |
| B (pinned–pinned mode) | 978.0 Hz | 994.1 Hz |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Matsuoka, K.; Kajihara, K.; Tanaka, H. Identification of Vibration Modes and Wave Propagation of Operational Rails by Multipoint Hammering and Reciprocity Theorem. Materials 2022, 15, 811. https://doi.org/10.3390/ma15030811
Matsuoka K, Kajihara K, Tanaka H. Identification of Vibration Modes and Wave Propagation of Operational Rails by Multipoint Hammering and Reciprocity Theorem. Materials. 2022; 15(3):811. https://doi.org/10.3390/ma15030811
Chicago/Turabian StyleMatsuoka, Kodai, Kazuhiro Kajihara, and Hirofumi Tanaka. 2022. "Identification of Vibration Modes and Wave Propagation of Operational Rails by Multipoint Hammering and Reciprocity Theorem" Materials 15, no. 3: 811. https://doi.org/10.3390/ma15030811
APA StyleMatsuoka, K., Kajihara, K., & Tanaka, H. (2022). Identification of Vibration Modes and Wave Propagation of Operational Rails by Multipoint Hammering and Reciprocity Theorem. Materials, 15(3), 811. https://doi.org/10.3390/ma15030811






