Systematic Evaluation of Permeability of Concrete Incorporating Coconut Shell as Replacement of Fine Aggregate
Abstract
1. Introduction
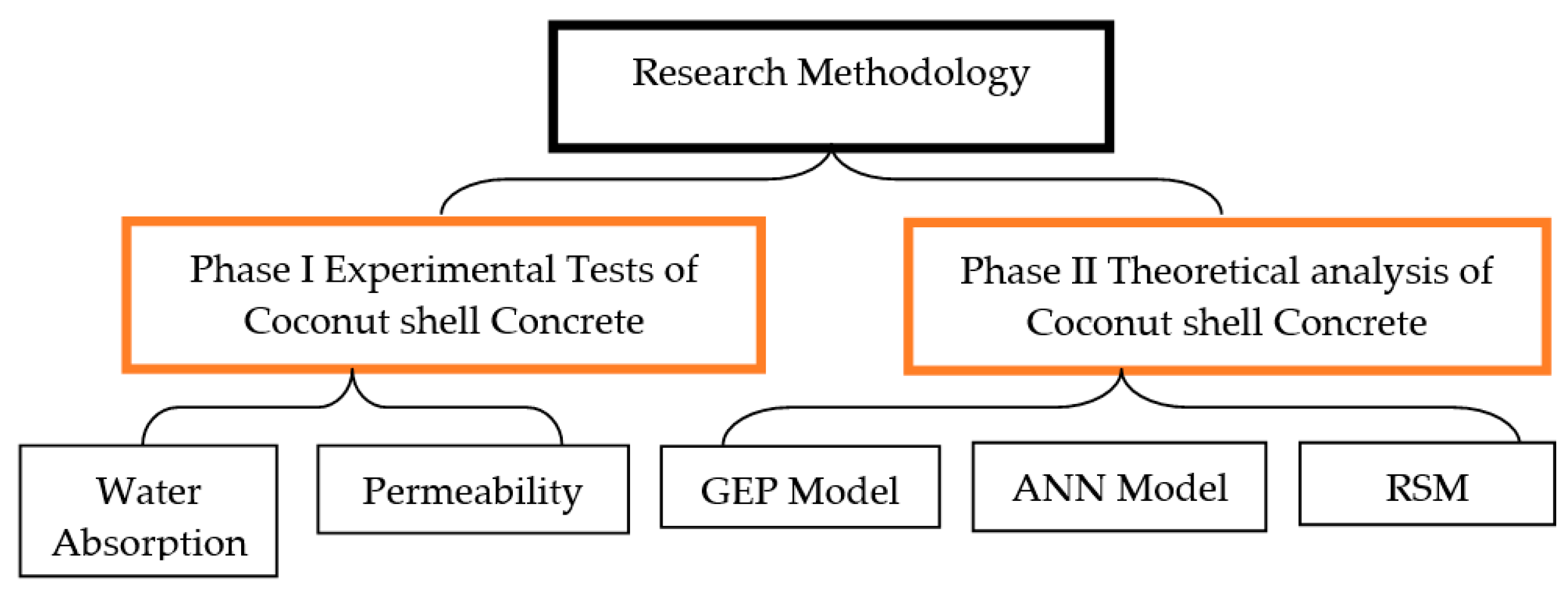
2. Experimental and Informational Modeling
2.1. Experiment Design
2.2. Preparation of Concrete Mix Design
2.3. Water Absorption Test
2.4. Permeability Test
2.5. Prediction Model Using ANN
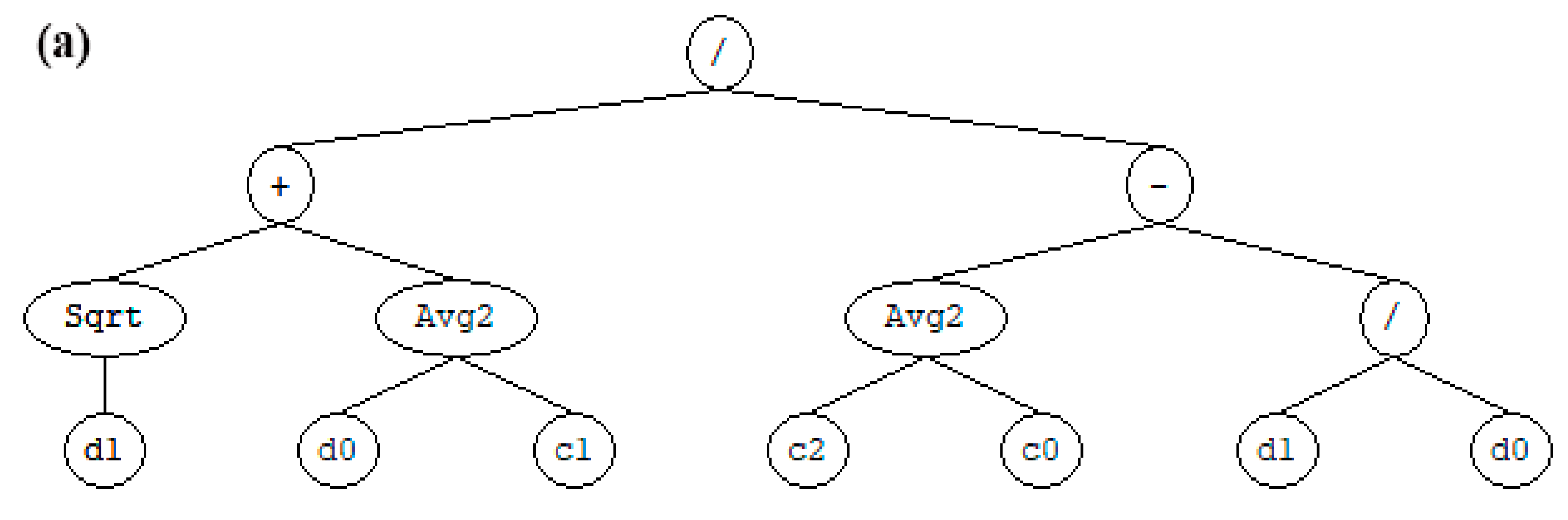
2.6. Prediction Model Using GEP
3. Result and Discussion
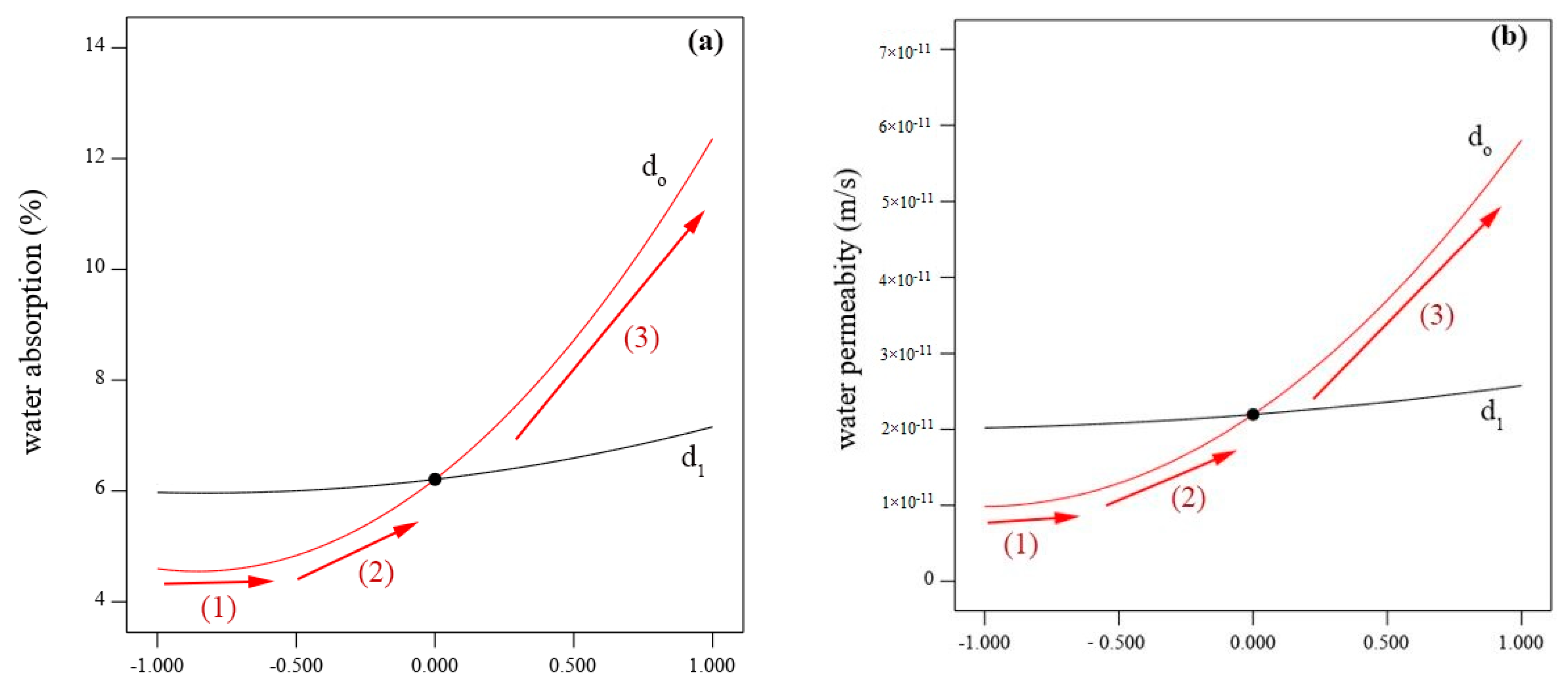
3.1. Parametric Analysis
3.1.1. Water Absorption
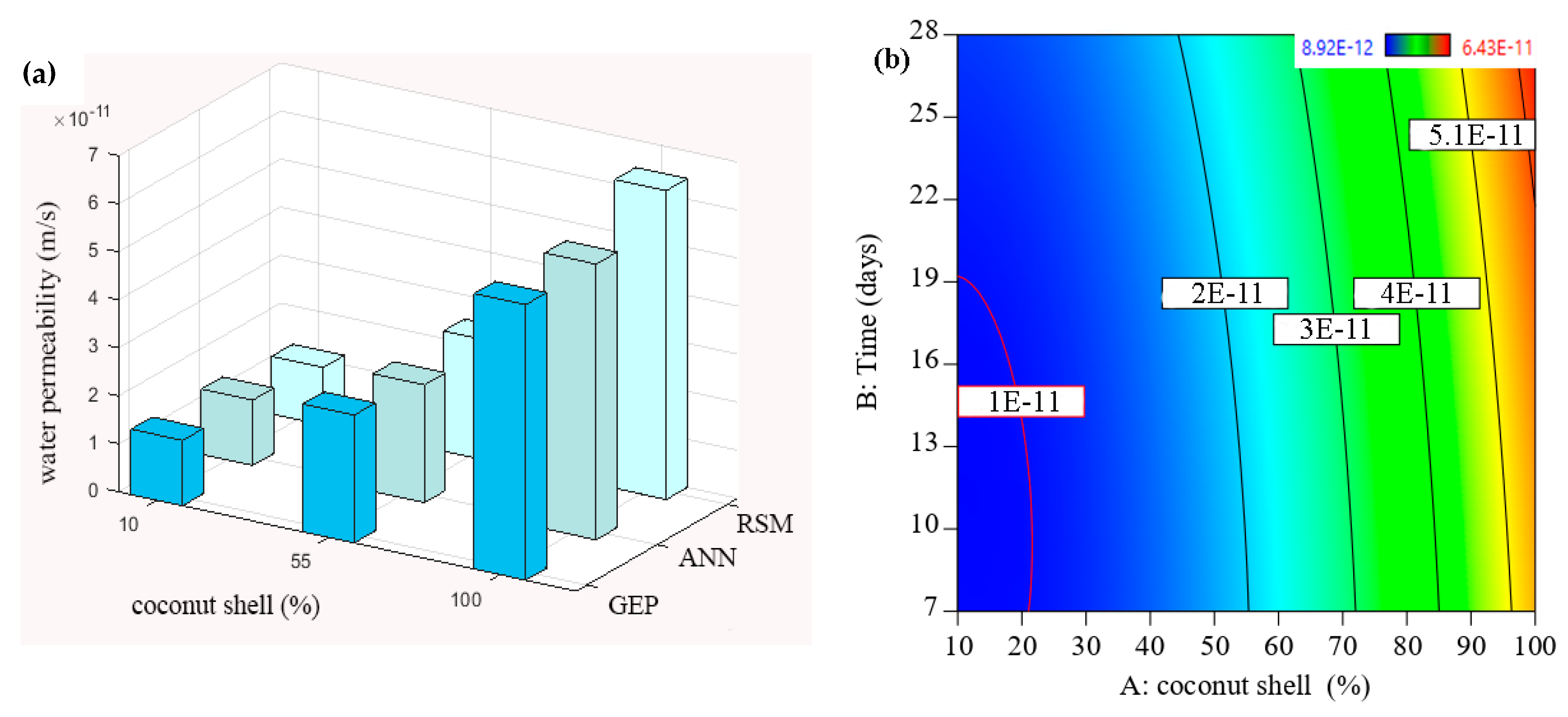
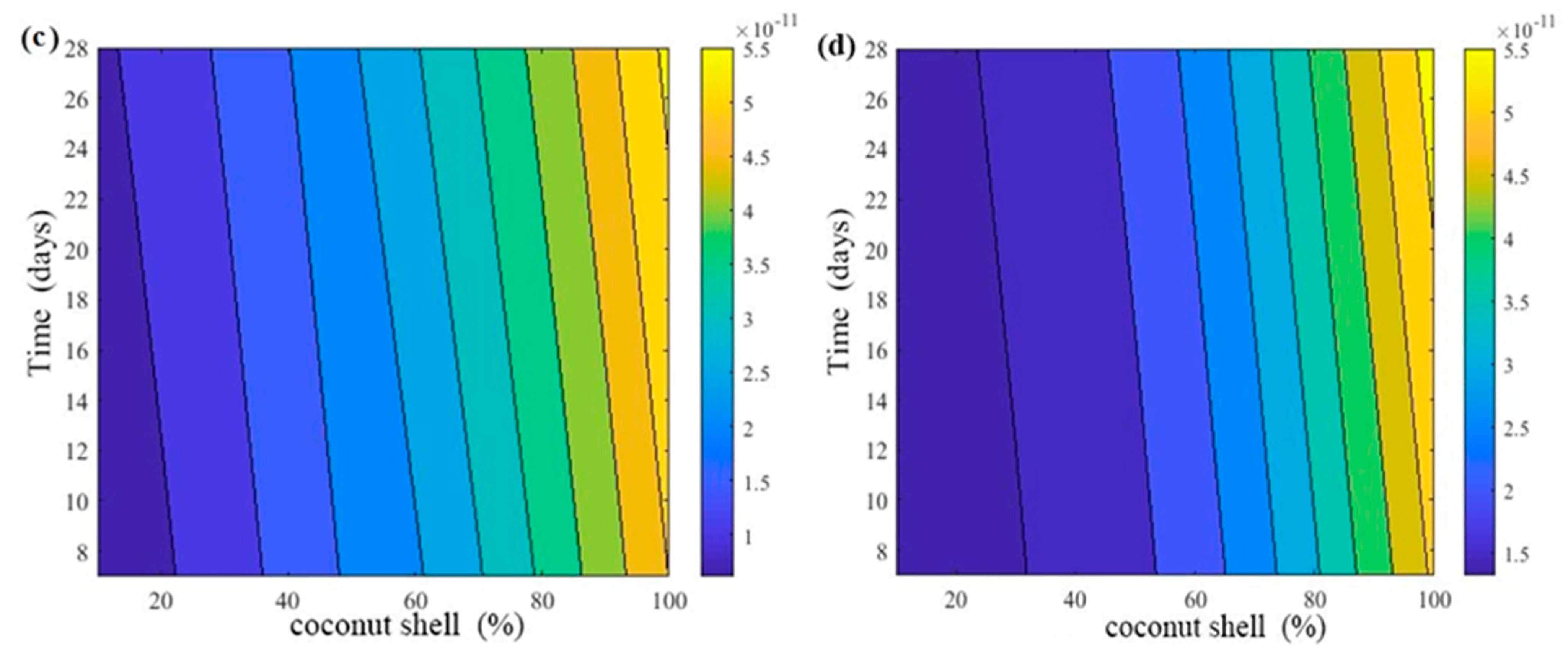
3.1.2. Water Permeability
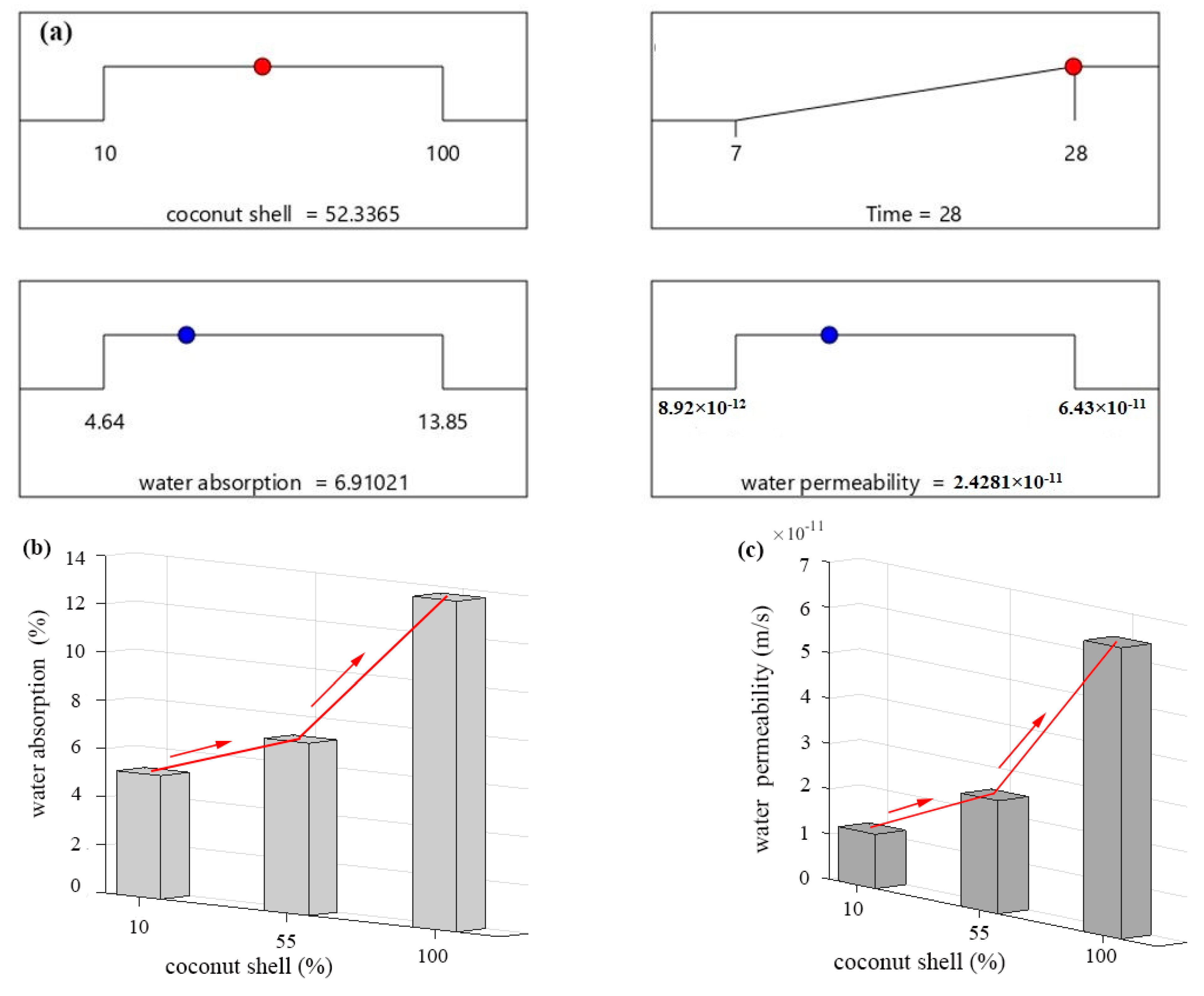
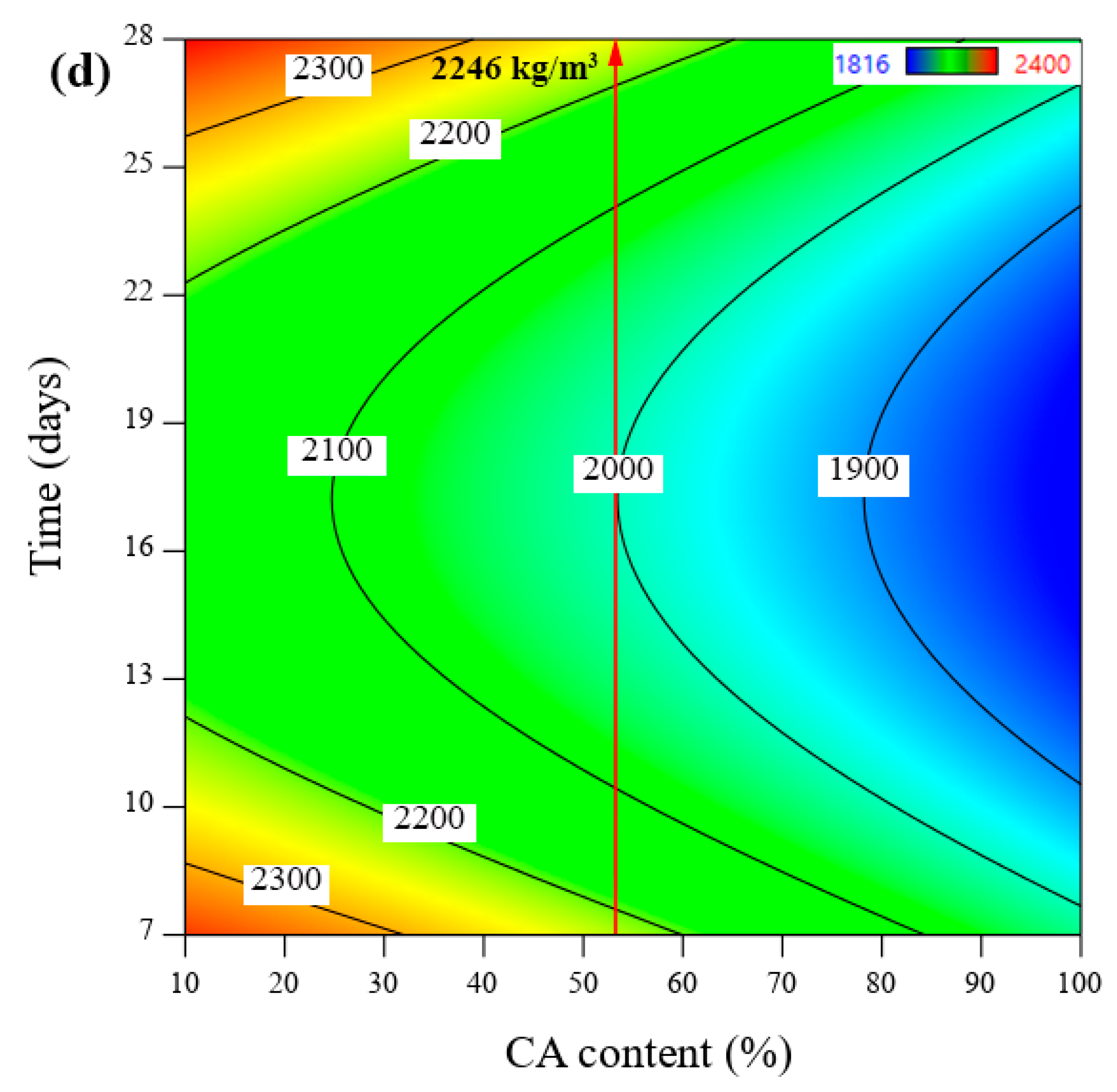
3.2. Informational Modeling Using RSM
3.3. Informational Modeling Using GEP and AMM
4. Conclusions
- All mathematical models of RSM, ANN, and GEP proved their ability to evaluate the behavior of CA-based concrete, in which the predicted data and the actual data were consistent.
- Based on ANN, GEP, and RSM models, the replacement percentage of fine aggregate by coconut shell up to 50% produce a good quality concrete in which the permeability and water absorption were less than 2.7 × 10−11 m/s and 5%, receptively.
- The ANN, RSM, and GEP also revealed that the high replacement of fine aggregate by coconut shell produced a concrete with high permeability (greater than 4.5 × 10−11 m/s) and high water absorption (greater than 10%).
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Aziz, W.; Aslam, M.; Ejaz, M.F.; Ali, M.J.; Ahmad, R.; Raza, M.W.U.H.; Khan, A. Mechanical properties, drying shrinkage and structural performance of coconut shell lightweight concrete. In Structures; Elsevier: Amsterdam, The Netherlands, 2022. [Google Scholar]
- Tangadagi, R.B.; Manjunatha, M.; Preethi, S.; Bharath, A.; Reshma, T.V. Strength characteristics of concrete using coconut shell as a coarse aggregate—A sustainable approach. Mater. Today: Proc. 2021, 47, 3845–3851. [Google Scholar] [CrossRef]
- Tiwari, A.; Singh, S.; Nagar, R. Feasibility assessment for partial replacement of fine aggregate to attain cleaner production perspective in concrete: A review. J. Clean. Prod. 2016, 135, 490–507. [Google Scholar] [CrossRef]
- Janani, S.; Kulanthaivel, P.; Sowndarya, G.; Srivishnu, H.; Shanjayvel, P.G. Study of coconut shell as coarse aggregate in light weight concrete—A review. Mater. Today Proc. 2022, 65, 2003–2006. [Google Scholar] [CrossRef]
- Alengaram, U.J.; Jumaat, M.Z.; Mahmud, H. Ductility behaviour of reinforced palm kernel shell concrete beams. Eur. J. Sci. Res. 2008, 23, 406–420. [Google Scholar]
- Verma, D.; Gope, P. The use of coir/coconut fibers as reinforcements in composites. In Biofiber Reinforcements in Composite Materials; Elsevier: Amsterdam, The Netherlands, 2015; pp. 285–319. [Google Scholar]
- Gunasekaran, K.; Kumar, P.; Lakshmipathy, M. Mechanical and bond properties of coconut shell concrete. Constr. Build. Mater. 2011, 25, 92–98. [Google Scholar] [CrossRef]
- Nor, M.; A’liah, N.A.; Engku Ariff, E.E.; Nik Omar, N.R.; Zainol Abidin, A.Z.; Muhammad, R.M.; Rahim, H.; Nazmi, M.S.; Sulaiman, N.H. Total productivity and technical efficiency of coconuts in Malaysia. Econ. Technol. Manag. Rev. 2020, 15, 11–22. [Google Scholar]
- Gunasekaran, K.; Annadurai, R.; Kumar, P. Long term study on compressive and bond strength of coconut shell aggregate concrete. Constr. Build. Mater. 2012, 28, 208–215. [Google Scholar] [CrossRef]
- Bušić, R.; Miličević, I.; Šipoš, T.K.; Strukar, K. Recycled rubber as an aggregate replacement in self-compacting concrete—Literature overview. Materials 2018, 11, 1729. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Zhang, S.; Wang, R.; Dang, F. Potential use of waste tire rubber as aggregate in cement concrete—A comprehensive review. Constr. Build. Mater. 2019, 225, 1183–1201. [Google Scholar] [CrossRef]
- Mhaya, A.; Abidin, A.R.Z.; Sarbini, N.N.; Ismail, M. Role of crumb tyre aggregates in rubberised concrete contained granulated blast-furnace slag. In IOP Conference Series: Earth and Environmental Science; IOP Publishing: Bristol, UK, 2019; pp. 1–12. [Google Scholar]
- Singh, N.; Mithulraj, M.; Arya, S. Influence of coal bottom ash as fine aggregates replacement on various properties of concretes: A review. Resour. Conserv. Recycl. 2018, 138, 257–271. [Google Scholar] [CrossRef]
- Ibrahim, M.H.B.W.; Shahidan, S.; Algaifi, H.A.; Hamzah, A.F.B.; Jaya, R.P. CBA Self-compacting Concrete Exposed to Chloride and Sulphate. In Properties of Self-Compacting Concrete with Coal Bottom Ash under Aggressive Environments; Springer: Berlin/Heidelberg, Germany, 2021; pp. 33–57. [Google Scholar]
- Ibrahim, M.H.B.W.; Shahidan, S.; Amer Algaifi, H.; Bin Hamzah, A.F.; Putra Jaya, R. CBA Self-compacting Concrete Exposed to Seawater by Wetting and Drying Cycles. In Properties of Self-Compacting Concrete with Coal Bottom Ash under Aggressive Environments; Springer: Berlin/Heidelberg, Germany, 2021; pp. 59–75. [Google Scholar]
- Małek, M.; Łasica, W.; Jackowski, M.; Kadela, M. Effect of waste glass addition as a replacement for fine aggregate on properties of mortar. Materials 2020, 13, 3189. [Google Scholar] [CrossRef] [PubMed]
- Harrison, E.; Berenjian, A.; Seifan, M. Recycling of waste glass as aggregate in cement-based materials. Environ. Sci. Ecotechnol. 2020, 4, 100064. [Google Scholar] [CrossRef] [PubMed]
- Mhaya, A.M.; Baharom, S.; Huseien, G.F. Improved strength performance of rubberized Concrete: Role of ground blast furnace slag and waste glass bottle nanoparticles amalgamation. Constr. Build. Mater. 2022, 342, 128073. [Google Scholar] [CrossRef]
- Medina, C.; Frías, M.; De Rojas, M.S.; Thomas, C.; Polanco, J.A. Gas permeability in concrete containing recycled ceramic sanitary ware aggregate. Constr. Build. Mater. 2012, 37, 597–605. [Google Scholar] [CrossRef]
- Jiao, H.; Chen, W.; Wu, A.; Yu, Y.; Ruan, Z.; Honaker, R.; Chen, X.; Yu, J. Flocculated unclassified tailings settling efficiency improvement by particle collision optimization in the feedwell. Int. J. Miner. Metall. Mater. 2022, 29, 2126–2135. [Google Scholar] [CrossRef]
- Chen, F.; Xu, B.; Jiao, H.; Chen, X.; Shi, Y.; Wang, J.; Li, Z. Triaxial mechanical properties and microstructure visualization of BFRC. Constr. Build. Mater. 2021, 278, 122275. [Google Scholar] [CrossRef]
- Pan, X.; Shi, C.; Jia, L.; Zhang, J.; Wu, L. Effect of inorganic surface treatment on air permeability of cement-based materials. J. Mater. Civ. Eng. 2016, 28, 04015145. [Google Scholar] [CrossRef]
- Jia, L.; Shi, C.; Pan, X.; Zhang, J.; Wu, L. Effects of inorganic surface treatment on water permeability of cement-based materials. Cem. Concrete Composites 2016, 67, 85–92. [Google Scholar] [CrossRef]
- Baghban, M.H.; Mhaya, A.M.; Faridmehr, I.; Huseien, G.F. Carbonation Depth and Chloride Ion Penetration Properties of Rubberised Concrete Incorporated Ground Blast Furnace Slag. In Solid State Phenomena; Trans Tech Publications Ltd.: Bach, Switzerland, 2022; Volume 329, pp. 101–108. [Google Scholar]
- Shaaban, I.G.; Rizzuto, J.P.; El-Nemr, A.; Bohan, L.; Ahmed, H.; Tindyebwa, H. Mechanical properties and air permeability of concrete containing waste tires extracts. J. Mater. Civ. Eng. 2021, 33, 04020472. [Google Scholar] [CrossRef]
- Mhaya, A.M.; Baghban, M.H.; Faridmehr, I.; Huseien, G.F. Performance Evaluation of Modified Rubberized Concrete Exposed to Aggressive Environments. Materials 2021, 14, 1900. [Google Scholar] [CrossRef]
- El Mir, A.S.; Nehme, G.; Assaad, J.J. Durability of self-consolidating concrete containing natural waste perlite powders. Heliyon 2020, 6, e03165. [Google Scholar] [CrossRef] [PubMed]
- Sharma, N.K.; Kumar, P.; Kumar, S.; Thomas, B.S.; Gupta, R.C. Properties of concrete containing polished granite waste as partial substitution of coarse aggregate. Constr. Build. Mater. 2017, 151, 158–163. [Google Scholar] [CrossRef]
- Mhaya, A.M.; Huseien, G.F.; Abidin, A.R.Z.; Ismail, M. Long-term mechanical and durable properties of waste tires rubber crumbs replaced GBFS modified concretes. Constr. Build. Mater. 2020, 256, 119505. [Google Scholar] [CrossRef]
- Bisht, K.; Ramana, P. Sustainable production of concrete containing discarded beverage glass as fine aggregate. Constr. Build. Mater. 2018, 177, 116–124. [Google Scholar] [CrossRef]
- Prakash, R.; Thenmozhi, R.; Raman, S.N.; Subramanian, C.; Divyah, N. An investigation of key mechanical and durability properties of coconut shell concrete with partial replacement of fly ash. Struct. Concr. 2021, 22, E985–E996. [Google Scholar] [CrossRef]
- Palanisamy, M.; Kolandasamy, P.; Awoyera, P.; Gobinath, R.; Muthusamy, S.; Krishnasamy, T.R.; Viloria, A. Permeability properties of lightweight self-consolidating concrete made with coconut shell aggregate. J. Mater. Res. Technol. 2020, 9, 3547–3557. [Google Scholar] [CrossRef]
- Mathew, S.P.; Nadir, Y.; Arif, M.M. Experimental study of thermal properties of concrete with partial replacement of coarse aggregate by coconut shell. Mater. Today Proc. 2020, 27, 415–420. [Google Scholar] [CrossRef]
- Nadir, Y.; Sujatha, A. Durability properties of coconut shell aggregate concrete. KSCE J. Civ. Eng. 2018, 22, 1920–1926. [Google Scholar] [CrossRef]
- Liu, Q.-F.; Iqbal, M.F.; Yang, J.; Lu, X.Y.; Zhang, P.; Rauf, M. Prediction of chloride diffusivity in concrete using artificial neural network: Modelling and performance evaluation. Constr. Build. Mater. 2021, 268, 121082. [Google Scholar] [CrossRef]
- Liu, Q.-F.; Hu, Z.; Lu, X.Y.; Yang, J.; Azim, I.; Sun, W. Prediction of chloride distribution for offshore concrete based on statistical analysis. Materials 2020, 13, 174. [Google Scholar] [CrossRef]
- Boubekeur, T.; Boulekbache, B.; Aoudjane, K.; Ezziane, K.; Kadri, E.H. Prediction of the durability performance of ternary cement containing limestone powder and ground granulated blast furnace slag. Constr. Build. Mater. 2019, 209, 215–221. [Google Scholar] [CrossRef]
- Mhaya, A.M.; Huseien, G.F.; Faridmehr, I.; Abidin, A.R.Z.; Alyousef, R.; Ismail, M. Evaluating mechanical properties and impact resistance of modified concrete containing ground Blast Furnace slag and discarded rubber tire crumbs. Constr. Build. Mater. 2021, 295, 123603. [Google Scholar] [CrossRef]
- Abbas, Y. Simplex-lattice strength and permeability optimization of concrete incorporating silica fume and natural pozzolan. Constr. Build. Mater. 2018, 168, 199–208. [Google Scholar] [CrossRef]
- Güneyisi, E.; Gesoğlu, M.; Algın, Z.; Mermerdaş, K. Optimization of concrete mixture with hybrid blends of metakaolin and fly ash using response surface method. Compos. Part B Eng. 2014, 60, 707–715. [Google Scholar] [CrossRef]
- Kumar, S.; Rai, B.; Biswas, R.; Samui, P.; Kim, D. Prediction of rapid chloride permeability of self-compacting concrete using Multivariate Adaptive Regression Spline and Minimax Probability Machine Regression. J. Build. Eng. 2020, 32, 101490. [Google Scholar] [CrossRef]
- Sun, J.; Zhang, J.; Gu, Y.; Huang, Y.; Sun, Y.; Ma, G. Prediction of permeability and unconfined compressive strength of pervious concrete using evolved support vector regression. Constr. Build. Mater. 2019, 207, 440–449. [Google Scholar] [CrossRef]
- Mhaya, A.M.; Baharom, S.; Baghban, M.H.; Nehdi, M.L.; Faridmehr, I.; Huseien, G.F.; Algaifi, H.A.; Ismail, M. Systematic Experimental Assessment of POFA Concrete Incorporating Waste Tire Rubber Aggregate. Polymers 2022, 14, 2294. [Google Scholar] [CrossRef]
- Oyebisi, S.O.; Ede, A.N.; Olutoge, F.A. Optimization of design parameters of slag-corncob ash-based geopolymer concrete by the central composite design of the response surface methodology. Iran. J. Sci. Technol. Trans. Civ. Eng. 2021, 45, 27–42. [Google Scholar] [CrossRef]
- Habibi, A.; Ramezanianpour, A.M.; Mahdikhani, M.; Bamshad, O. RSM-based evaluation of mechanical and durability properties of recycled aggregate concrete containing GGBFS and silica fume. Constr. Build. Mater. 2021, 270, 121431. [Google Scholar] [CrossRef]
- Shahmansouri, A.A.; Nematzadeh, M.; Behnood, A. Mechanical properties of GGBFS-based geopolymer concrete incorporating natural zeolite and silica fume with an optimum design using response surface method. J. Build. Eng. 2021, 36, 102138. [Google Scholar] [CrossRef]
- Ferdosian, I.; Camões, A. Eco-efficient ultra-high performance concrete development by means of response surface methodology. Cem. Concr. Compos. 2017, 84, 146–156. [Google Scholar] [CrossRef]
- Foroughi, M.; Rahmani, A.R.; Asgari, G.; Nematollahi, D.; Yetilmezsoy, K.; Samarghandi, M.R. Optimization of a three-dimensional electrochemical system for tetracycline degradation using box-behnken design. Fresenius Environ. Bull. 2018, 27, 1914–1922. [Google Scholar]
- Mukhopadhyay, T.; Dey, T.K.; Chowdhury, R.; Chakrabarti, A. Structural damage identification using response surface-based multi-objective optimization: A comparative study. Arab. J. Sci. Eng. 2015, 40, 1027–1044. [Google Scholar] [CrossRef]
- Mohammed, B.S.; Yen, L.Y.; Haruna, S.; Seng Huat, M.L.; Abdulkadir, I.; Al-Fakih, A.; Liew, M.S.; Abdullah Zawawi, N.A.W. Effect of Elevated Temperature on the Compressive Strength and Durability Properties of Crumb Rubber Engineered Cementitious Composite. Materials 2020, 13, 3516. [Google Scholar] [CrossRef] [PubMed]
- Dan, S.; Banivaheb, S.; Hashemipour, H. Synthesis, characterization and absorption study of chitosan-g-poly (acrylamide-co-itaconic acid) hydrogel. Polym. Bull. 2021, 78, 1887–1907. [Google Scholar] [CrossRef]
- Oyebisi, S.; Ede, A.; Owamah, H.; Igba, T.; Mark, O.; Odetoyan, A. Optimising the Workability and Strength of Concrete Modified with Anacardium Occidentale Nutshell Ash. Fibers 2021, 9, 41. [Google Scholar] [CrossRef]
- Kwan, W.H.; Ramli, M.; Kam, K.J.; Sulieman, M.Z. Influence of the amount of recycled coarse aggregate in concrete design and durability properties. Constr. Build. Mater. 2012, 26, 565–573. [Google Scholar] [CrossRef]
- Gurumoorthy, N.; Arunachalam, K. Durability studies on concrete containing treated used foundry sand. Constr. Build. Mater. 2019, 201, 651–661. [Google Scholar] [CrossRef]
- Mo, K.H.; Thomas, B.S.; Yap, S.P.; Abutaha, F.; Tan, C.G. Viability of agricultural wastes as substitute of natural aggregate in concrete: A review on the durability-related properties. J. Clean. Prod. 2020, 275, 123062. [Google Scholar] [CrossRef]
- Shafigh, P.; Nomeli, M.A.; Alengaram, U.J.; Mahmud, H.B.; Jumaat, M.Z. Engineering properties of lightweight aggregate concrete containing limestone powder and high volume fly ash. J. Clean. Prod. 2016, 135, 148–157. [Google Scholar] [CrossRef]
- Bhardwaj, B.; Kumar, P. Waste foundry sand in concrete: A review. Constr. Build. Mater. 2017, 156, 661–674. [Google Scholar] [CrossRef]
- Banthia, N.; Biparva, A.; Mindess, S. Permeability of concrete under stress. Cem. Concr. Res. 2005, 35, 1651–1655. [Google Scholar] [CrossRef]
- Kumar, R.; Bhattacharjee, B. Porosity, pore size distribution and in situ strength of concrete. Cem. Concr. Res. 2003, 33, 155–164. [Google Scholar] [CrossRef]
- Sidiq, A.; Gravina, R.J.; Setunge, S.; Giustozzi, F. High-efficiency techniques and micro-structural parameters to evaluate concrete self-healing using X-ray tomography and Mercury Intrusion Porosimetry: A review. Constr. Build. Mater. 2020, 252, 119030. [Google Scholar] [CrossRef]
- Gallucci, E.; Scrivener, K.; Groso, A.; Stampanoni, M.; Margaritondo, G. 3D experimental investigation of the microstructure of cement pastes using synchrotron X-ray microtomography (μCT). Cem. Concr. Res. 2007, 37, 360–368. [Google Scholar] [CrossRef]
- Cuadrado-Rica, H.; Sebaibi, N.; Boutouil, M.; Boudart, B. Properties of ordinary concretes incorporating crushed queen scallop shells. Mater. Struct. 2016, 49, 1805–1816. [Google Scholar] [CrossRef]
- Amriou, A.; Bencheikh, M. New experimental method for evaluating the water permeability of concrete by a lateral flow procedure on a hollow cylindrical test piece. Constr. Build. Mater. 2017, 151, 642–649. [Google Scholar] [CrossRef]
- Hou, D.; Chen, D.; Wang, X.; Wu, D.; Ma, H.; Hu, X.; Zhang, Y.; Wang, P.; Yu, R. RSM-based modelling and optimization of magnesium phosphate cement-based rapid-repair materials. Constr. Build. Mater. 2020, 263, 120190. [Google Scholar] [CrossRef]
- Algaifi, H.A.; Alqarni, A.S.; Alyousef, R.; Bakar, S.A.; Ibrahim, M.W.; Shahidan, S.; Ibrahim, M.; Salami, B.A. Mathematical prediction of the compressive strength of bacterial concrete using gene expression programming. Ain Shams Eng. J. 2021, 12, 3629–3639. [Google Scholar] [CrossRef]
- Huseien, G.F.; Sam, A.R.M.; Algaifi, H.A.; Alyousef, R. Development of a sustainable concrete incorporated with effective microorganism and fly Ash: Characteristics and modeling studies. Constr. Build. Mater. 2021, 285, 122899. [Google Scholar] [CrossRef]
- Jitendra, K.; Khed, V.C. Optimization of concrete blocks with high volume fly ash and foundry sand. Mater. Today Proc. 2020, 27, 1172–1179. [Google Scholar] [CrossRef]
- Khoshkenari, A.G.; Shafigh, P.; Moghimi, M.; Mahmud, H.B. The role of 0–2 mm fine recycled concrete aggregate on the compressive and splitting tensile strengths of recycled concrete aggregate concrete. Mater. Des. 2014, 64, 345–354. [Google Scholar] [CrossRef]
- Nowak, A.; Rakoczy, A. Statistical model for compressive strength of lightweight concrete. Archit. Civ. Eng. Env. 2011, 4, 73–80. [Google Scholar]
- Ali Khan, M.; Zafar, A.; Akbar, A.; Javed, M.F.; Mosavi, A. Application of Gene Expression Programming (GEP) for the prediction of compressive strength of geopolymer concrete. Materials 2021, 14, 1106. [Google Scholar] [CrossRef]
- Alabduljabbar, H.; Huseien, G.F.; Sam, A.R.M.; Alyouef, R.; Algaifi, H.A.; Alaskar, A. Engineering Properties of Waste Sawdust-Based Lightweight Alkali-Activated Concrete: Experimental Assessment and Numerical Prediction. Materials 2020, 13, 5490. [Google Scholar] [CrossRef]




| Run NO. | Coded Value | Real Value | CCD Division | ||
|---|---|---|---|---|---|
| Replaced Coconut Shell (%) | Time (Days) | ||||
| 1 | −1 | −1 | 10 | 7 | Factorial points (2n) |
| 2 | 1 | −1 | 100 | 7 | |
| 3 | −1 | 1 | 10 | 28 | |
| 4 | 1 | 1 | 100 | 28 | |
| 5 | 1 | 0 | 100 | 17 | Axial points (2n) |
| 6 | −1 | 0 | 10 | 17 | |
| 7 | 0 | −1 | 55 | 7 | |
| 8 | 0 | 1 | 55 | 28 | |
| 9 | 0 | 0 | 55 | 17 | Centre points |
| 10 | 0 | 0 | 55 | 17 | |
| 11 | 0 | 0 | 55 | 17 | |
| 12 | 0 | 0 | 55 | 17 | |
| 13 | 0 | 0 | 55 | 17 | |
| Name of Specimens | Percentage of Fine Coconut Shell | Permeability m/s | Water Absorption % | ||
|---|---|---|---|---|---|
| 7 Days | 28 Days | 7 Days | 28 Days | ||
| FCSC10 | 10% | 8.92 × 10−12 | 1.2 × 10−11 | 4.65 | 5.21 |
| FCSC20 | 20% | 1.37 × 10−11 | 1.53 × 10−11 | 5.12 | 5.48 |
| FCSC30 | 30% | 1.48 × 10−11 | 1.73 × 10−11 | 5.34 | 5.53 |
| FCSC40 | 40% | 1.81 × 10−11 | 1.96 × 10−11 | 5.96 | 6.28 |
| FCSC50 | 50% | 2.02 × 10−11 | 2.3 × 10−11 | 5.99 | 6.63 |
| FCSC60 | 60% | 2.17 × 10−11 | 2.7 × 10−11 | 6.37 | 7.28 |
| FCSC70 | 70% | 2.58 × 10−11 | 3.38 × 10−11 | 7.78 | 8.17 |
| FCSC80 | 80% | 3.59 × 10−11 | 3.56 × 10−11 | 8.45 | 8.77 |
| FCSC90 | 90% | 4.52 × 10−11 | 4.69 × 10−11 | 9.54 | 10.23 |
| FCSC100 | 100% | 5.47 × 10−11 | 6.43 × 10−11 | 11.63 | 13.85 |
| Item | Second Polynomial Equations and the Involved Statistics Parameters | ||||
|---|---|---|---|---|---|
| Water Permeability (WP) | R2 = 0.999 | 0.9964 | 0.9813 | Adeq. Precision 44.47 | RMSE 8.2 × 10−13 |
| Water Absorption (WA) | R2 = 0.9987 | 0.9953 | 0.976 | Adeq. Precision 40.757 | RMSE 0.1492 |
| Model | Item | Mathematical Equation and Related Statistics Validation Parameters | ||||
|---|---|---|---|---|---|---|
| GEP | WA | Training | MAE = 0.817 | RMSE = 1.037 | R = 0.925 | R2 = 0.856 |
| Validation | MAE = 0.848 | RMSE = 0.959 | R = 0.982 | R2 = 0.964 | ||
| WP | Training | MAE = 2.73 × 10−12 | RMSE = 3.2 × 10−12 | R = 0.9756 | R2 = 0.9519 | |
| Validation | MAE = 2.6 × 10−12 | RMSE = 2.8 × 10−12 | R = 0.995 | R2 = 0.991 | ||
| ANN | WA | Training | MAE = 0.32 | RMSE = 0.4447 | R = 0.979 | R2 = 0.9598 |
| Validation | MAE = 0.123 | RMSE = 0.221 | R = 0.983 | R2 = 0.967 | ||
| WP | Training | MAE = 2.0 × 10−12 | RMSE = 2.4 × 10−12 | R = 0.985 | R2 = 0.9719 | |
| Validation | MAE = 6 × 10−13 | RMSE = 7 × 10−13 | R = 0.99 | R2 = 0.9981 | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mhaya, A.M.; Algaifi, H.A.; Shahidan, S.; Zuki, S.S.M.; Azmi, M.A.M.; Ibrahim, M.H.W.; Huseien, G.F. Systematic Evaluation of Permeability of Concrete Incorporating Coconut Shell as Replacement of Fine Aggregate. Materials 2022, 15, 7944. https://doi.org/10.3390/ma15227944
Mhaya AM, Algaifi HA, Shahidan S, Zuki SSM, Azmi MAM, Ibrahim MHW, Huseien GF. Systematic Evaluation of Permeability of Concrete Incorporating Coconut Shell as Replacement of Fine Aggregate. Materials. 2022; 15(22):7944. https://doi.org/10.3390/ma15227944
Chicago/Turabian StyleMhaya, Akram M., Hassan Amer Algaifi, Shahiron Shahidan, Sharifah Salwa Mohd Zuki, Mohamad Azim Mohammad Azmi, Mohd Haziman Wan Ibrahim, and Ghasan Fahim Huseien. 2022. "Systematic Evaluation of Permeability of Concrete Incorporating Coconut Shell as Replacement of Fine Aggregate" Materials 15, no. 22: 7944. https://doi.org/10.3390/ma15227944
APA StyleMhaya, A. M., Algaifi, H. A., Shahidan, S., Zuki, S. S. M., Azmi, M. A. M., Ibrahim, M. H. W., & Huseien, G. F. (2022). Systematic Evaluation of Permeability of Concrete Incorporating Coconut Shell as Replacement of Fine Aggregate. Materials, 15(22), 7944. https://doi.org/10.3390/ma15227944








