Corrosion Crack Morphology and Creep Analysis of Members Based on Meso-Scale Corrosion Penetration
Abstract
1. Introduction
2. Calculation of Creep and Corrosion Cracking
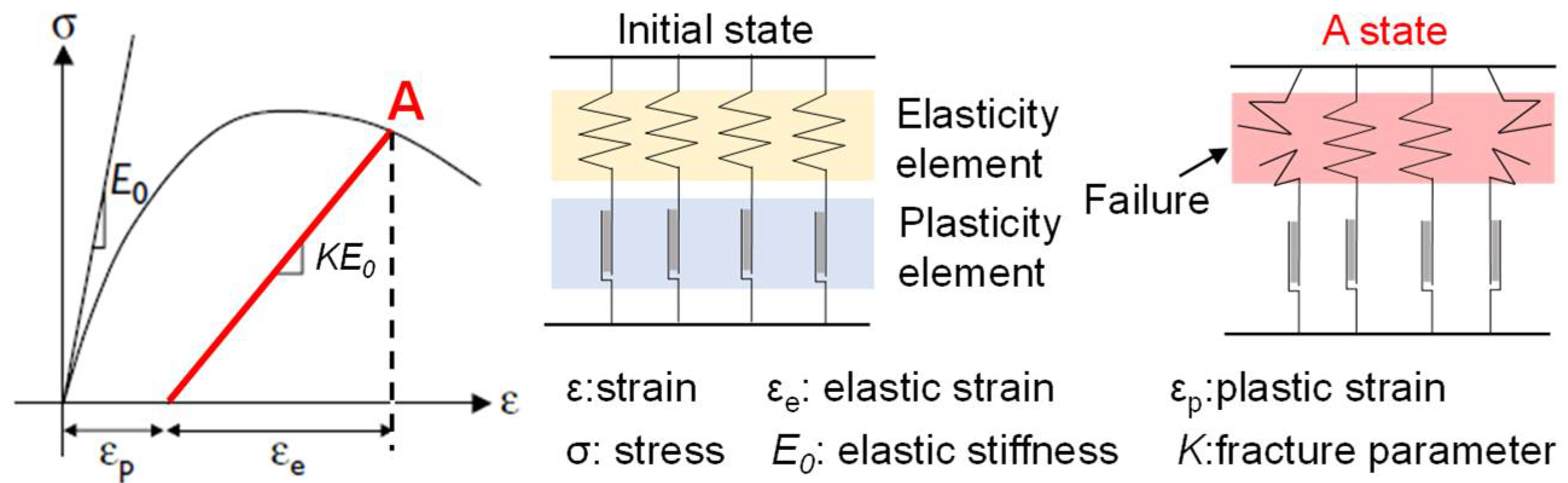
2.1. Creep Constitutive Model
2.2. Model of Corrosion Development
2.2.1. Solid Phase Corrosion Product
2.2.2. Liquid Phase Rust Product
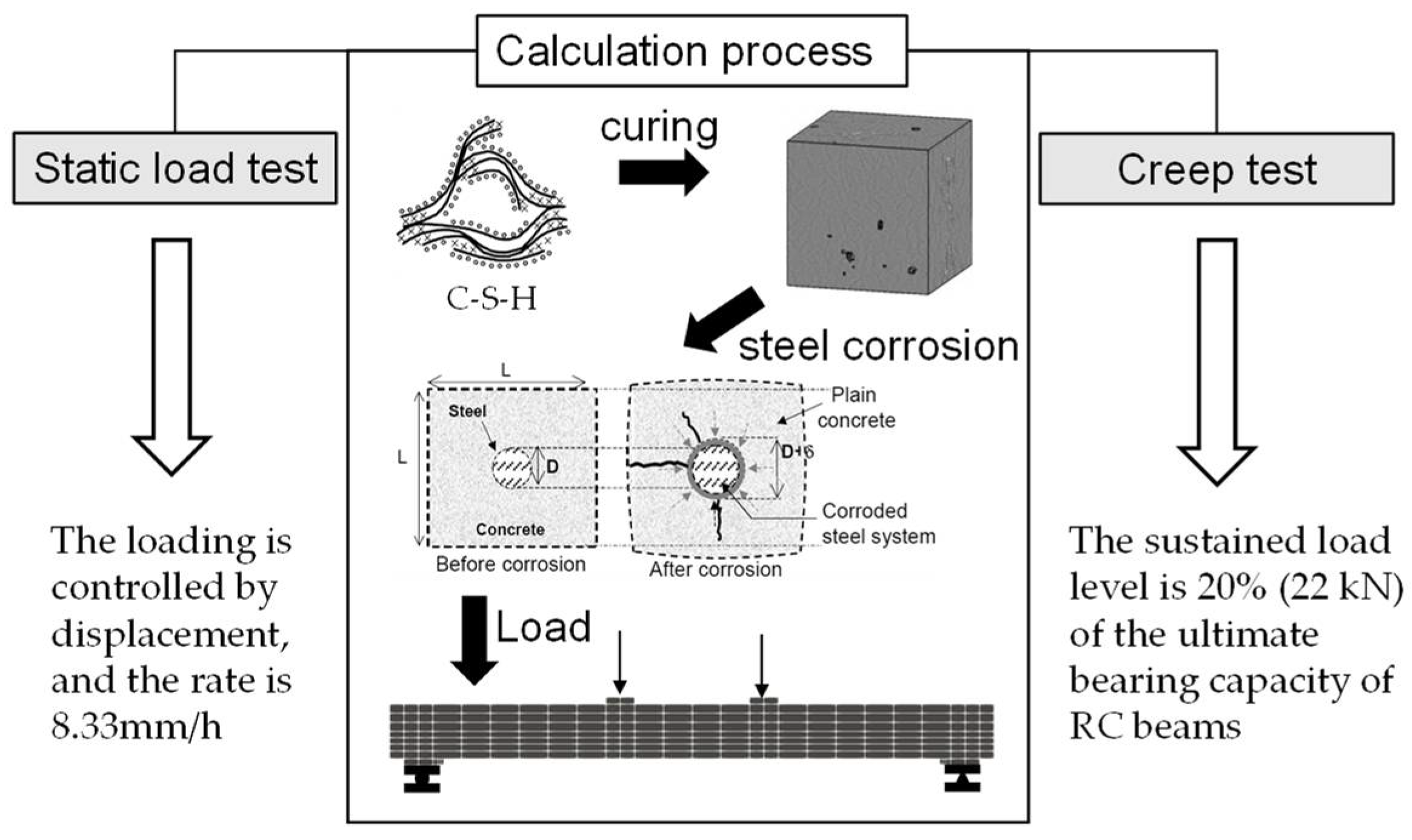
3. The Calculation Results and Analysis
3.1. Specimen Information
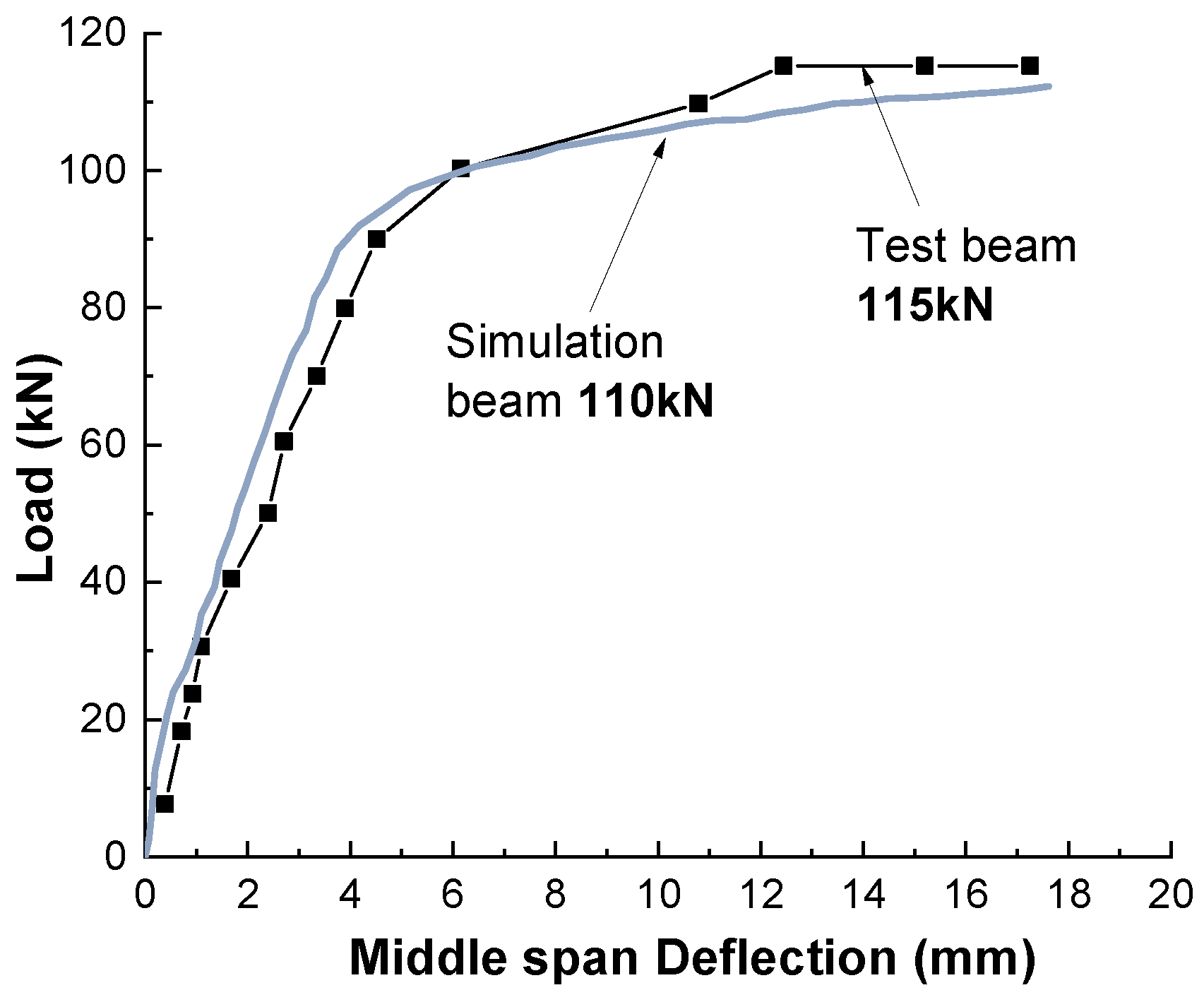
3.2. Flexural Capacity and Creep Bearing Capacity of Non-Corroded Beam
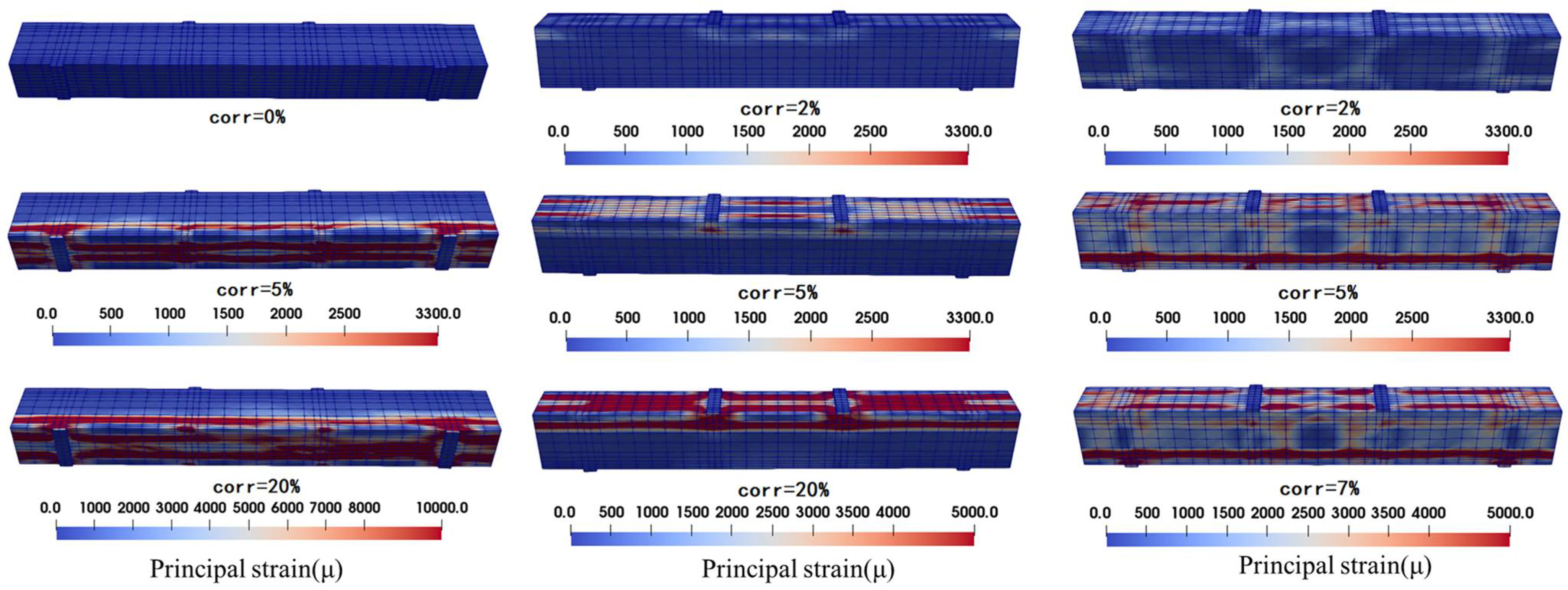
3.3. Corrosion Cracking Pattern
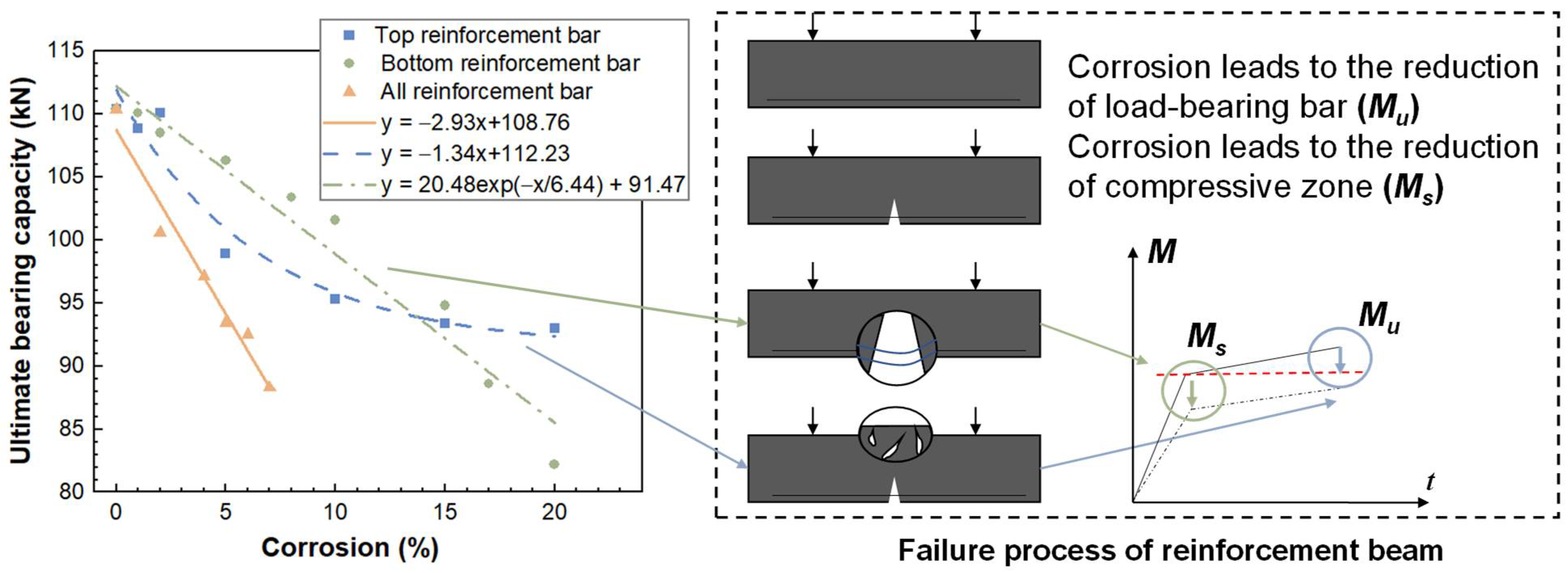
3.4. Flexural Capacity of Corroded Beams
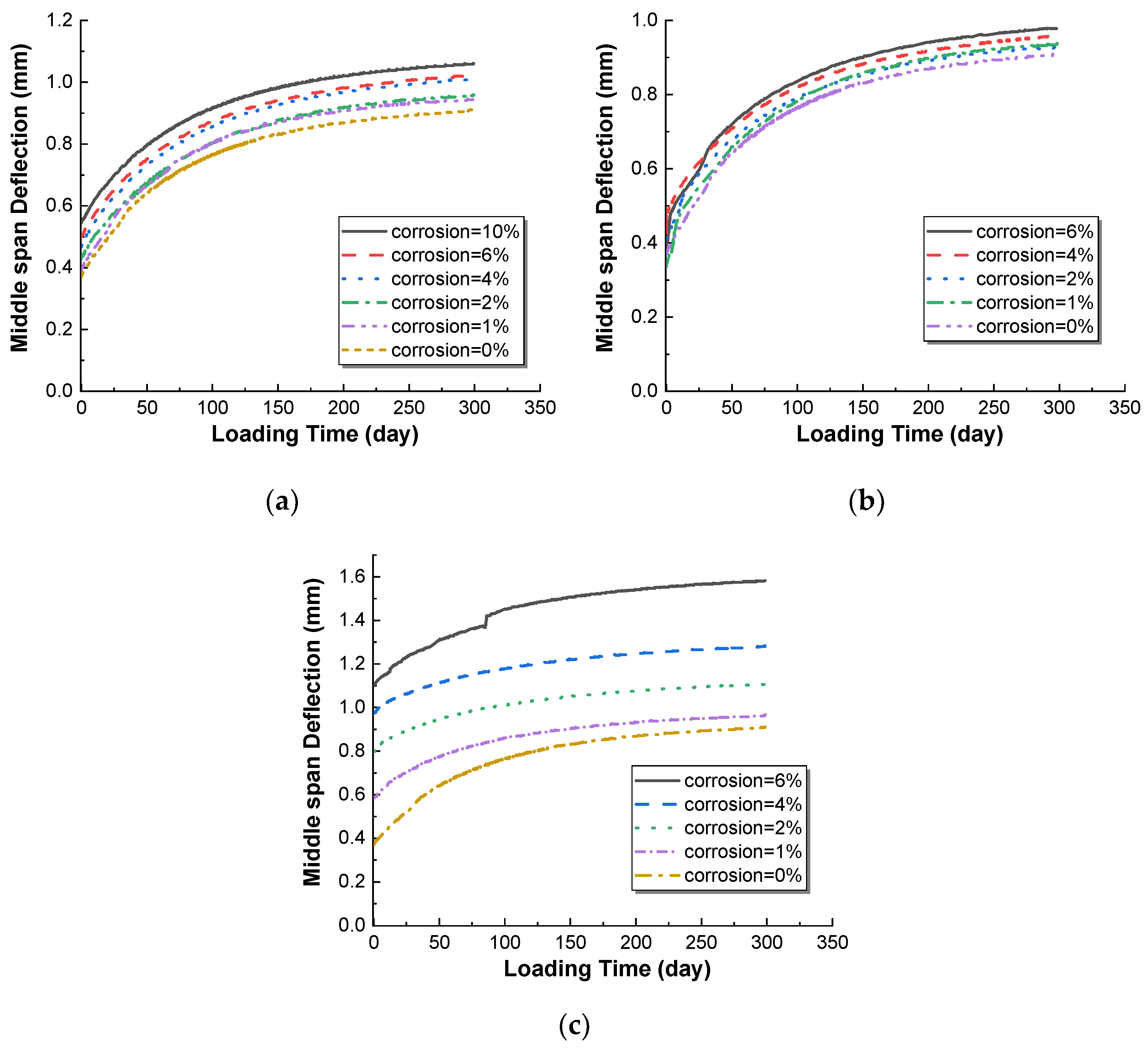
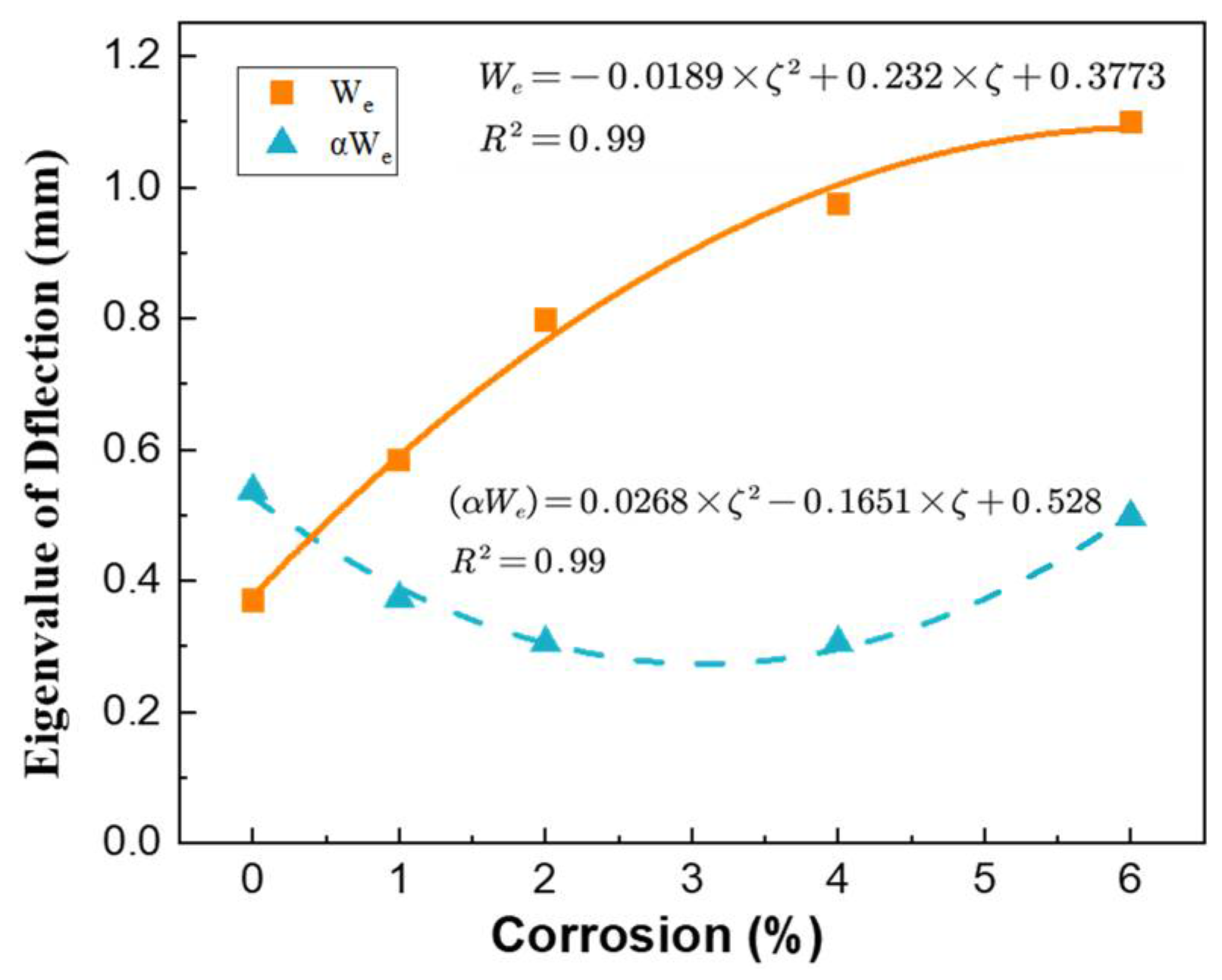
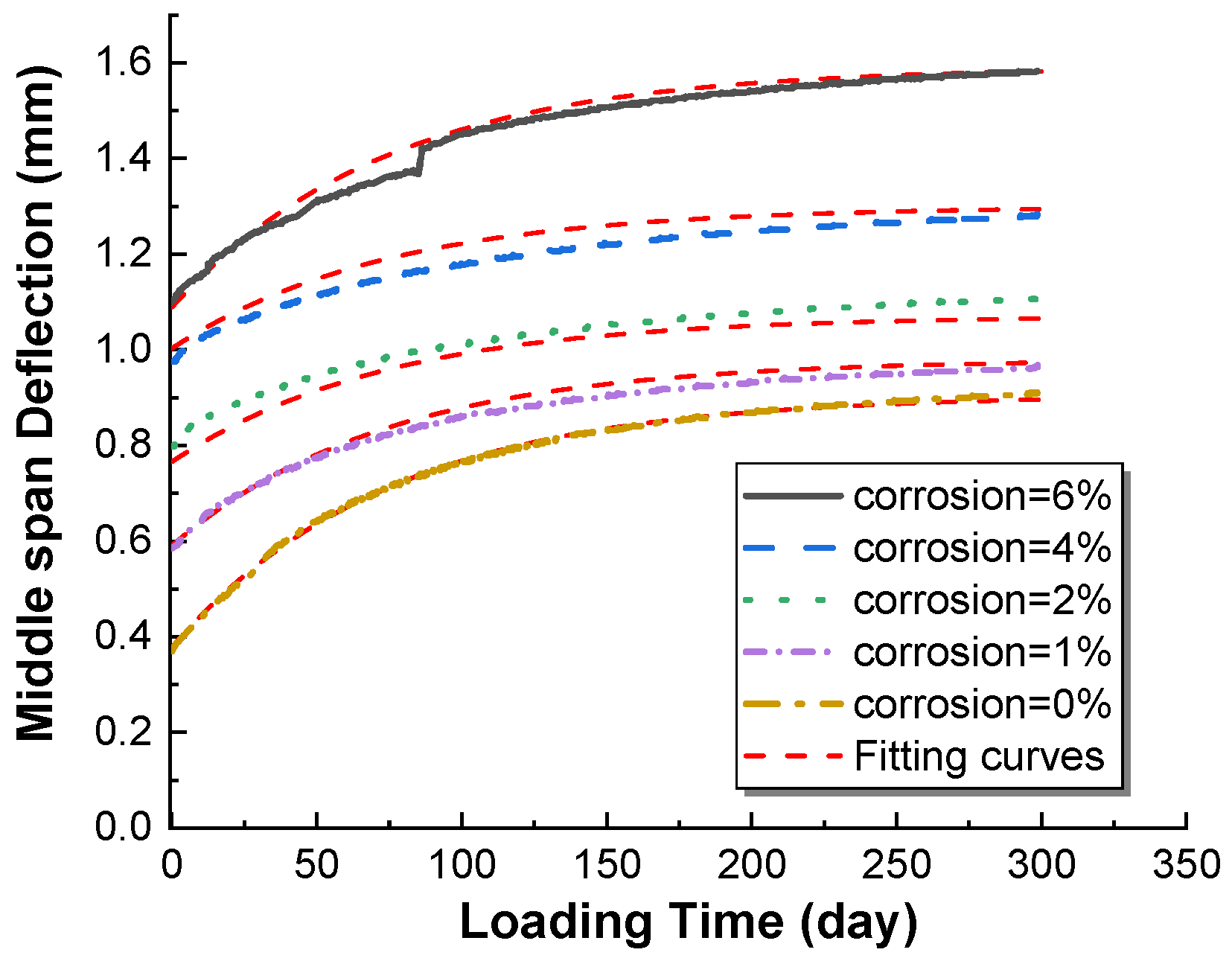
3.5. Creep Characteristics Analysis of Corroded Beams
4. Conclusions
- (1)
- In this paper, the time history effect of creep was considered based on the rate-type constitutive model, and the generation of rust expansion cracks and the penetration phenomenon of rust products were fully considered in the calculation of rust corrosion. The three-dimensional rust expansion and cracking modes caused by different rust corrosion conditions were simulated more realistically, and a logical connection was established with subsequent mechanical properties.
- (2)
- According to the calculation and observation of the ultimate bearing capacity of the beams with different corrosion forms, the corrosion of the longitudinal bars at the top reduces the strength of the concrete in the compression zone, which leads to a decrease in the strengthening section of the fracture curve of the members and causes the brittle failure mode of the reinforced concrete beams. The corrosion of the longitudinal bars at the bottom causes the reinforcement to work in advance and reduces the mechanical properties of the tensile bars. The ultimate bearing capacity of the beam decreases gradually with the increase in the corrosion rate of the longitudinal bars at the bottom.
- (3)
- By observing the load-bearing deformation of beams with different corrosion forms, it is found that the creep coefficient of RC beams decreases with the increase in corrosion rate. This phenomenon is mainly caused by the obvious increase in initial instantaneous deflection caused by corrosion, which does not mean that corrosion is conducive to the creep of beams. In addition, this phenomenon indicates that it is not easy to use the traditional concept of creep (deformation development/initial deformation) for the load-bearing deformation of corroded, damaged members, and it is more reasonable to use the global deflection to describe the long-term deformation of corroded, damaged members.
- (4)
- For future research on damaged members, this paper argues that the global deflection should be used reasonably to describe the performance degradation of members, where the damage study includes rust damage, fatigue damage, etc. The coefficient of creep was originally used in materials research and migrated to structural research. The creep coefficient is only suitable for the study of sustained load at undamaged times. For the creep of damaged members, it is recommended to directly use the absolute amount of plastic deformation at the sustained load for the description.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| ε | strain | the stress tensor in concrete | |
| εp | plastic strain | the stiffness matrix of corroded reinforcement | |
| εe | elastic strain | Density of the skeleton-pore system | |
| K | damage coefficient | density of skeleton | |
| E0 | elastic stiffness | density of pore medium | |
| σ | stress | n | the porosity in the skeleton-pore system |
| t | time | the stress tensor acting on the skeleton | |
| Φ | tendency of plastic deformation | p | the pore pressure |
| κ | linear creep | Kronecker symbol | |
| Cv | intrinsic creep time | ui | skeleton displacement (i-direction) |
| Clim | creep coefficient at infinite time | gi | gravitational acceleration (i-direction) |
| Vs | volume of solid phase corrosion production | wi | displacement of pore medium (i-direction) |
| Vl | volume of liquid phase corrosion production | ki | permeability of corrosion products |
| Vloss | volume of corrosion production | volume modulus of the skeleton-pore system | |
| Dcl | diameter of un-corroded steel | the volume of solid phase corrosion products retained on the reinforced concrete surface | |
| D | diameter of the original reinforcement | β | crystallization rate of rust products |
| γ | volume loss rate of reinforcement | icorr | the corrosion rate of reinforcement |
| α | volume expansion rate | k | transport rate |
| the natural corrosion expansion strain of reinforcement | Wp | creep deflection | |
| modulus of reinforcement | We | instantaneous elastic deflection | |
| G | the modulus of solid phase corrosion product | Wt | total deflection deformation |
References
- Cao, G.-H.; Han, C.-C.; Peng, P.; Zhang, W.; Tang, H. Creep Test and Analysis of Concrete Columns under Corrosion and Load Coupling. ACI Struct. J. 2019, 116, 121–130. [Google Scholar] [CrossRef]
- Yoon, S.; Wang, K.J.; Weiss, W.J.; Shah, S.P. Interaction between loading, corrosion, and serviceability of reinforced concrete. Aci. Mater. J. 2000, 97, 637–644. [Google Scholar]
- Shen, J.S.; Gao, X.; Li, B.; Du, K.; Jin, R.Y.; Chen, W.; Xu, Y.D. Damage Evolution of RC Beams Under Simultaneous Rein-forcement Corrosion and Sustained Load. Materials 2019, 12, 627. [Google Scholar] [CrossRef] [PubMed]
- Maekawa, K.; Okamura, H.; Pimanmas, A. Non-Linear Mechanics of Reinforced Concrete; Taylor & Francis Group: London, UK, 2003. [Google Scholar]
- Maekawa, K.; Ishida, T.; Kishi, T. Multi-Scale Modeling of Structural Concrete; Taylor & Francis Group: London, UK, 2008. [Google Scholar]
- Toongoenthong, K.; Maekawa, K. Simulation of Coupled Corrosive Product Formation, Migration into Crack and Propagation in Reinforced Concrete Sections. J. Adv. Concr. Technol. 2005, 3, 253–265. [Google Scholar] [CrossRef]
- Toongoenthong, K.; Maekawa, K. Multi-mechanical approach to structural performance assessment of corroded RC mem-bers in shear. J. Adv. Concr. Technol. 2005, 3, 107–122. [Google Scholar] [CrossRef]
- Li, C.Q.; Yang, S.T.; Saafi, M. Numerical Simulation of Behavior of Reinforced Concrete Structures considering Corrosion Effects on Bonding. J. Struct. Eng. 2014, 140, 04014092. [Google Scholar] [CrossRef]
- Xing, D. Study on the Deterioration of the Bearing Capacity of Rusty Reinforced Concrete Members. Master’s Thesis, Shandong University, Jinan, China, 2019. [Google Scholar]
- Bažant, Z.P.; Wu, S.T. Rate-type creep law of aging concrete based on maxwell chain. Mater. Struct. 1974, 7, 45–60. [Google Scholar] [CrossRef]
- Yu, Q.; Bazant, Z.P.; Wendner, R. Improved Algorithm for Efficient and Realistic Creep Analysis of Large Creep-Sensitive Concrete Structures. ACI Struct. J. 2012, 109, 665–675. [Google Scholar] [CrossRef]
- Di Luzio, G.; Cedolin, L.; Beltrami, C. Tridimensional Long-Term Finite Element Analysis of Reinforced Concrete Structures with Rate-Type Creep Approach. Appl. Sci. 2020, 10, 4772. [Google Scholar] [CrossRef]
- Lokhorst, S.; van Breugel, K. Simulation of the effect of geometrical changes of the microstructure on the deformational behaviour of hardening concrete. Cem. Concr. Res. 1997, 27, 1465–1479. [Google Scholar] [CrossRef]
- Zhu, Y.; Ishida, T.; Maekawa, K. Multi-scale constitutive model of concrete based on thermodynamic states of moisture in micro-pores. Doboku Gakkai Ronbunshu 2004, 2004, 241–260. [Google Scholar] [CrossRef][Green Version]
- Takaya, S.; Nakamura, S.; Yamamoto, T.; Miyagawa, T. Influence of steel corrosion products in concrete on crack opening weight loss of corrosion. J. Jpn. Soc. Civ. Eng. Ser. E2 2013, 69, 154–165. [Google Scholar]
- Gebreyouhannes, E.; Maekawa, K. Nonlinear Gel Migration in Cracked Concrete and Broken Symmetry of Corrosion Profiles. J. Adv. Concr. Technol. 2016, 14, 271–286. [Google Scholar] [CrossRef]
- Wong, H.S.; Zhao, Y.X.; Karimi, A.R.; Buenfeld, N.R.; Jin, W.L. On the penetration of corrosion products from reinforcing steel into concrete due to chloride-induced corrosion. Corros. Sci. 2010, 52, 2469–2480. [Google Scholar] [CrossRef]
- Caisheng, C. Experimental Research On Fatigue Performance Of Reinforced Concrete Beams Under Overload And Corrosion Damage. Master’s Thesis, Zhejiang University, Hangzhou, China, 2015. [Google Scholar]
- Malumbela, G.; Alexander, M.; Moyo, P. Interaction between corrosion crack width and steel loss in RC beams corroded un-der load. Cem. Concr. Res. 2010, 40, 1419–1428. [Google Scholar] [CrossRef]
- Du, Y.; Chan, A.; Clark, L.; Wang, X.; Gurkalo, F.; Bartos, S. Finite element analysis of cracking and delamination of concrete beam due to steel corrosion. Eng. Struct. 2013, 56, 8–21. [Google Scholar] [CrossRef]


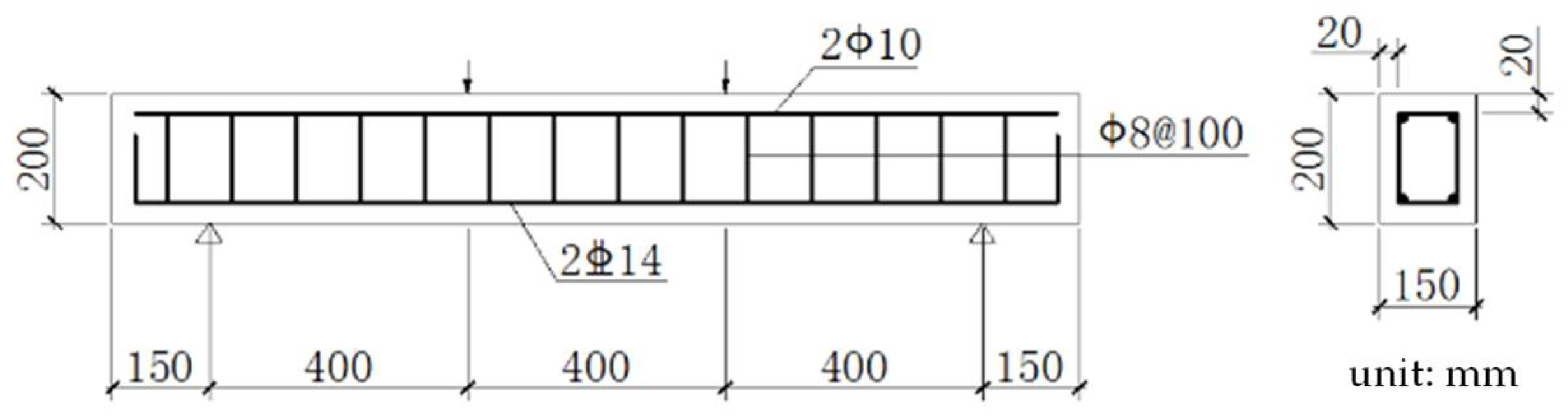

| Proportions of Concrete Mix | Water Cement Ratio | Water/kg·m−3 | Cement/kg·m−3 | Fine Aggregate/kg·m−3 | Coarse Aggregate/kg·m−3 |
| 0.49 | 220 | 449 | 1125 | 606 | |
| Reinforcement parameters | Steel grades | Diameter/mm | Yield strength/MPa | Ultimate strength/MPa | Ductility/% |
| HRB400 | 14 | 319.8 | 452.0 | 36.1 | |
| HPB300 | 8/10 | 424.8 | 585.4 | 31.9 |
| Corrosion (%) | 0 | 1 | 2 | 4 | 5 | 6 | 7 | 8 | 10 | 12 | 15 | 17 | 20 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Bearing Capacity (kN) | ||||||||||||||
| Top longitudinal reinforcement | 110.4 | 108.9 | 100.1 | - | 98.9 | - | - | - | - | - | 95.4 | - | 93 | |
| Bottom longitudinal reinforcement | 110.4 | 110.1 | 108.5 | - | 106.3 | - | - | 103.4 | 101.6 | 97.8 | 93.5 | 85.6 | 82.2 | |
| All reinforcement | 110.4 | - | 100.6 | 97.1 | 93.4 | 92.5 | 88.3 | - | - | - | - | - | - | |
| Corrosion Rate (%) | 0 | 1 | 2 | 4 | 6 | 10 | |
|---|---|---|---|---|---|---|---|
| Top longitudinal reinforcement | β | 74.36 | 64.79 | 69.22 | 69.13 | 59.30 | - |
| α | 1.45 | 1.75 | 1.25 | 1.15 | 1.46 | - | |
| Bottom longitudinal reinforcement | β | 74.36 | 73.38 | 84.12 | 77.58 | 78.94 | 77.06 |
| α | 1.45 | 1.43 | 1.25 | 1.17 | 1.05 | 0.95 | |
| All reinforcement | β | 74.36 | 73.11 | 79.17 | 87.12 | 90.24 | - |
| α | 1.45 | 0.64 | 0.38 | 0.31 | 0.45 | - | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zeng, B.; Yang, Y.; Gong, F.; Maekawa, K. Corrosion Crack Morphology and Creep Analysis of Members Based on Meso-Scale Corrosion Penetration. Materials 2022, 15, 7338. https://doi.org/10.3390/ma15207338
Zeng B, Yang Y, Gong F, Maekawa K. Corrosion Crack Morphology and Creep Analysis of Members Based on Meso-Scale Corrosion Penetration. Materials. 2022; 15(20):7338. https://doi.org/10.3390/ma15207338
Chicago/Turabian StyleZeng, Bin, Yiping Yang, Fuyuan Gong, and Koichi Maekawa. 2022. "Corrosion Crack Morphology and Creep Analysis of Members Based on Meso-Scale Corrosion Penetration" Materials 15, no. 20: 7338. https://doi.org/10.3390/ma15207338
APA StyleZeng, B., Yang, Y., Gong, F., & Maekawa, K. (2022). Corrosion Crack Morphology and Creep Analysis of Members Based on Meso-Scale Corrosion Penetration. Materials, 15(20), 7338. https://doi.org/10.3390/ma15207338






