Inverse Design of Valley-Like Edge States of Sound Degenerated Away from the High-Symmetry Points in a Square Lattice
Abstract
1. Introduction
2. Description of the Geometry of Scatters
3. Inverse Design
3.1. Quantitative Measures of Band Inversion
3.2. Common Bandgap Width
3.3. Problem Formulations
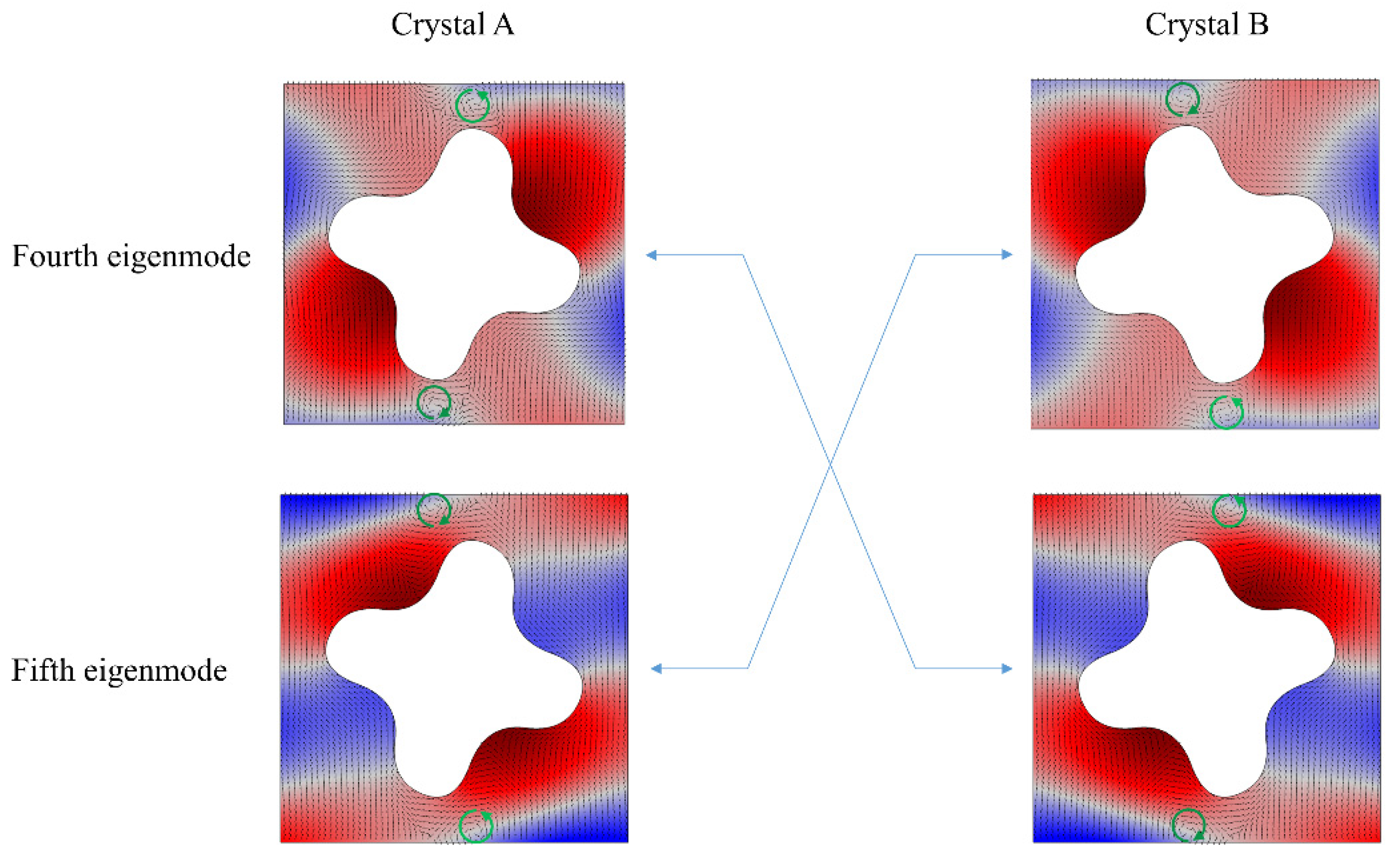
4. Valley-Like Vortex States
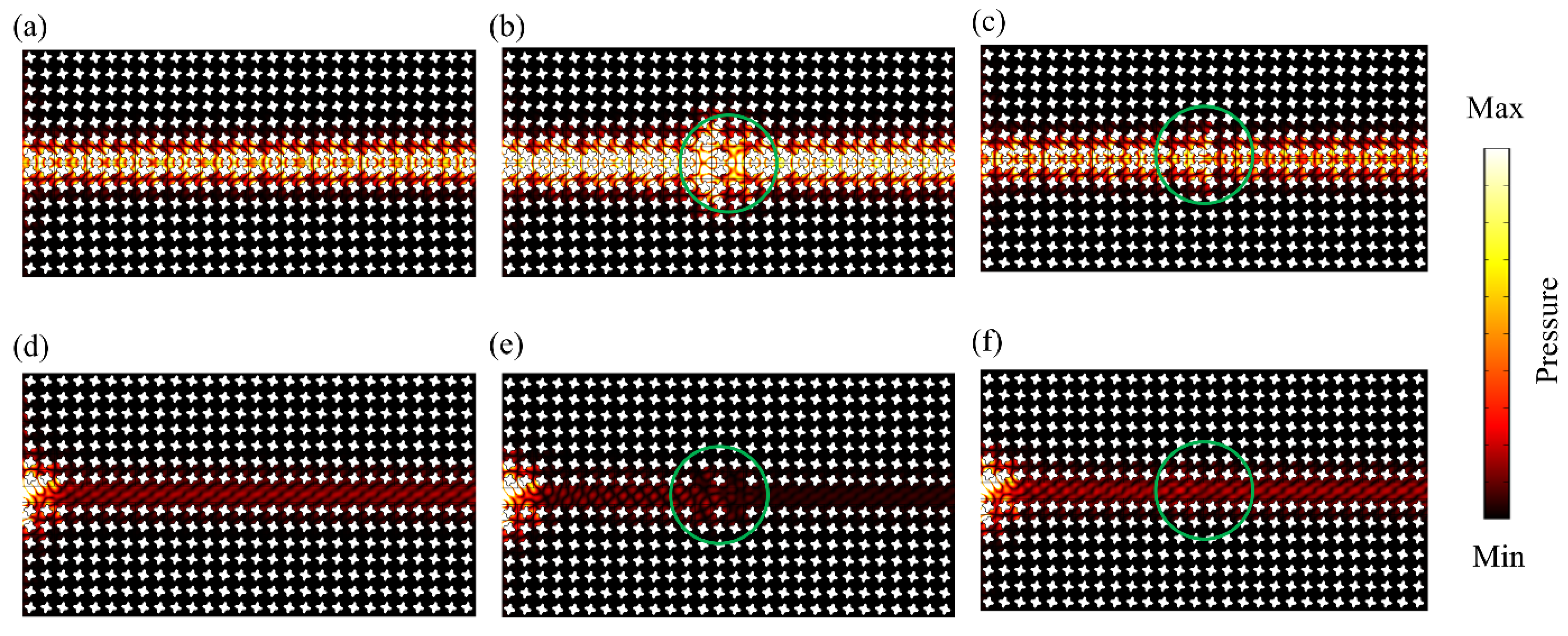
5. Valley-Like Edge States
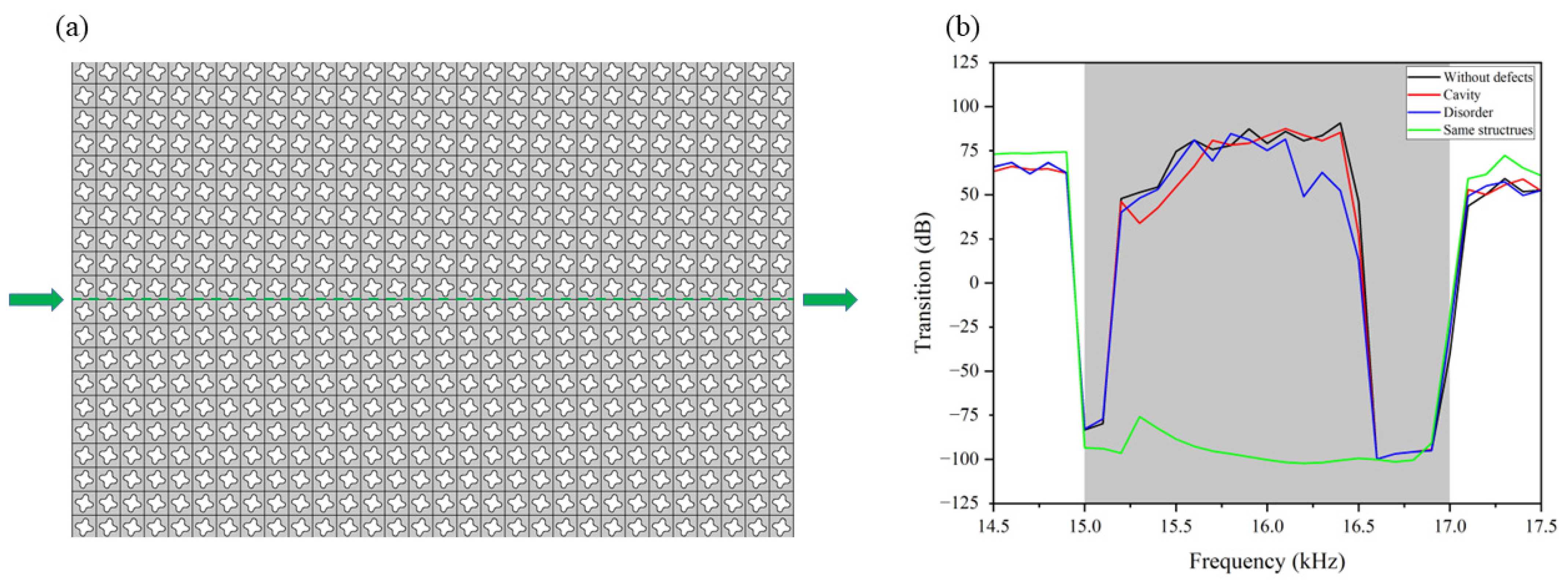
6. Observation of Energy Propagation against Defects
7. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Schaibley, J.R.; Yu, H.; Clark, G.; Rivera, P.; Ross, J.S.; Seyler, K.L.; Yao, W.; Xu, X. Valleytronics in 2D Materials. Nat. Rev. Mater. 2016, 1, 16055. [Google Scholar] [CrossRef]
- Ozawa, T.; Price, H.M.; Amo, A.; Goldman, N.; Hafezi, M.; Lu, L.; Rechtsman, M.C.; Schuster, D.; Simon, J.; Zilberberg, O. Topological Photonics. Rev. Mod. Phys. 2019, 91, 15006. [Google Scholar] [CrossRef]
- Zhang, X.; Xiao, M.; Cheng, Y.; Lu, M.; Christensen, J. Topological Sound. Commun. Phys. 2018, 1, 97. [Google Scholar] [CrossRef]
- Ma, G.; Xiao, M.; Chan, C.T. Topological Phases in Acoustic and Mechanical Systems. Nat. Rev. Phys. 2019, 1, 281–294. [Google Scholar] [CrossRef]
- Rycerz, A.; Tworzydło, J.; Beenakker, C. Valley Filter and Valley Valve in Graphene. Nat. Phys. 2007, 3, 172–175. [Google Scholar] [CrossRef]
- Xiao, D.; Yao, W.; Niu, Q. Valley-Contrasting Physics in Graphene: Magnetic Moment and Topological Transport Phys. Rev. Lett. 2007, 99, 236809. [Google Scholar] [CrossRef]
- Semenoff, G.W.; Semenoff, V.; Zhou, F. Domain Walls in Gapped Graphene. Phys. Rev. Lett. 2008, 101, 87204. [Google Scholar] [CrossRef]
- Gorbachev, R.V.; Song, J.; Yu, G.L.; Kretinin, A.V.; Withers, F.; Cao, Y.; Mishchenko, A.; Grigorieva, I.V.; Novoselov, K.S.; Levitov, L.S. Detecting Topological Currents in Graphene Superlattices. Science 2014, 346, 448–451. [Google Scholar] [CrossRef]
- Liu, Z.; Feng, W.; Xin, H.; Gao, Y.; Liu, P.; Yao, Y.; Weng, H.; Zhao, J. Two-Dimensional Spin–Valley-Coupled Dirac Semimetals in Functionalized Sbas Monolayers. Mater. Horiz. 2019, 6, 781–787. [Google Scholar] [CrossRef]
- Martin, I.; Blanter, Y.M.; Morpurgo, A.F. Topological Confinement in Bilayer Graphene. Phys. Rev. Lett. 2008, 100, 36804. [Google Scholar] [CrossRef]
- Qiao, Z.; Jung, J.; Niu, Q.; MacDonald, A.H. Electronic Highways in Bilayer Graphene. Nano Lett. 2011, 11, 3453–3459. [Google Scholar] [CrossRef] [PubMed]
- Zhang, F.; MacDonald, A.H.; Mele, E.J. Valley Chern Numbers and Boundary Modes in Gapped Bilayer Graphene. Proc. Natl. Acad. Sci. USA 2013, 110, 10546–10551. [Google Scholar] [CrossRef] [PubMed]
- Xu, X.; Yao, W.; Xiao, D.; Heinz, T.F. Spin and Pseudospins in Layered Transition Metal Dichalcogenides. Nat. Phys. 2014, 10, 343–350. [Google Scholar] [CrossRef]
- Mak, K.F.; McGill, K.L.; Park, J.; McEuen, P.L. The Valley Hall Effect in Mos2 Transistors. Science 2014, 344, 1489–1492. [Google Scholar] [CrossRef] [PubMed]
- Ju, L.; Shi, Z.; Nair, N.; Lv, Y.; Jin, C.; Velasco, J.; Ojeda-Aristizabal, C.; Bechtel, H.A.; Martin, M.C.; Zettl, A. Topological Valley Transport at Bilayer Graphene Domain Walls. Nature 2015, 520, 650–655. [Google Scholar] [CrossRef]
- Li, J.; Wang, K.; McFaul, K.J.; Zern, Z.; Ren, Y.; Watanabe, K.; Taniguchi, T.; Qiao, Z.; Zhu, J. Gate-Controlled Topological Conducting Channels in Bilayer Graphene. Nat. Nanotechnol. 2016, 11, 1060–1065. [Google Scholar] [CrossRef]
- Li, J.; Zhang, R.; Yin, Z.; Zhang, J.; Watanabe, K.; Taniguchi, T.; Liu, C.; Zhu, J. A Valley Valve and Electron Beam Splitter. Science 2018, 362, 1149–1152. [Google Scholar] [CrossRef]
- Deng, F.; Sun, Y.; Wang, X.; Xue, R.; Li, Y.; Jiang, H.; Shi, Y.; Chang, K.; Chen, H. Observation of Valley-Dependent Beams in Photonic Graphene. Opt. Express 2014, 22, 23605–23613. [Google Scholar] [CrossRef]
- Dong, J.; Chen, X.; Zhu, H.; Wang, Y.; Zhang, X. Valley Photonic Crystals for Control of Spin and Topology. Nat. Mater. 2017, 16, 298–302. [Google Scholar] [CrossRef]
- Bleu, O.; Solnyshkov, D.D.; Malpuech, G. Quantum Valley Hall Effect and Perfect Valley Filter Based On Photonic Analogs of Transitional Metal Dichalcogenides. Phys. Rev. B 2017, 95, 235431. [Google Scholar] [CrossRef]
- Ma, T.; Shvets, G. All-Si Valley-Hall Photonic Topological Insulator. New J. Phys. 2016, 18, 25012. [Google Scholar] [CrossRef]
- Noh, J.; Huang, S.; Chen, K.P.; Rechtsman, M.C. Observation of Photonic Topological Valley Hall Edge States. Phys. Rev. Lett. 2018, 120, 63902. [Google Scholar] [CrossRef]
- Gao, F.; Xue, H.; Yang, Z.; Lai, K.; Yu, Y.; Lin, X.; Chong, Y.; Shvets, G.; Zhang, B. Topologically Protected Refraction of Robust Kink States in Valley Photonic Crystals. Nat. Phys. 2018, 14, 140–144. [Google Scholar] [CrossRef]
- Chen, X.; Zhao, F.; Chen, M.; Dong, J. Valley-Contrasting Physics in All-Dielectric Photonic Crystals: Orbital Angular Momentum and Topological Propagation. Phys. Rev. B 2017, 96, 20202. [Google Scholar] [CrossRef]
- Xie, B.; Liu, H.; Cheng, H.; Liu, Z.; Chen, S.; Tian, J. Acoustic Topological Transport and Refraction in a Kekulé Lattice. Phys. Rev. Appl. 2019, 11, 44086. [Google Scholar] [CrossRef]
- Lu, J.; Qiu, C.; Ke, M.; Liu, Z. Valley Vortex States in Sonic Crystals. Phys. Rev. Lett. 2016, 116, 93901. [Google Scholar] [CrossRef] [PubMed]
- Ye, L.; Qiu, C.; Lu, J.; Wen, X.; Shen, Y.; Ke, M.; Zhang, F.; Liu, Z. Observation of Acoustic Valley Vortex States and Valley-Chirality Locked Beam Splitting. Phys. Rev. B 2017, 95, 174106. [Google Scholar] [CrossRef]
- Lu, J.; Qiu, C.; Ye, L.; Fan, X.; Ke, M.; Zhang, F.; Liu, Z. Observation of Topological Valley Transport of Sound in Sonic Crystals. Nat. Phys. 2017, 13, 369–374. [Google Scholar] [CrossRef]
- Lu, J.; Qiu, C.; Deng, W.; Huang, X.; Li, F.; Zhang, F.; Chen, S.; Liu, Z. Valley Topological Phases in Bilayer Sonic Crystals. Phys. Rev. Lett. 2018, 120, 116802. [Google Scholar] [CrossRef]
- Zhang, Z.; Tian, Y.; Wang, Y.; Gao, S.; Cheng, Y.; Liu, X.; Christensen, J. Directional Acoustic Antennas Based On Valley-Hall Topological Insulators. Adv. Mater. 2018, 30, 1803229. [Google Scholar] [CrossRef]
- Zhang, Z.; Tian, Y.; Cheng, Y.; Wei, Q.; Liu, X.; Christensen, J. Topological Acoustic Delay Line. Phys. Rev. Appl. 2018, 9, 34032. [Google Scholar] [CrossRef]
- Wang, M.; Ye, L.; Christensen, J.; Liu, Z. Valley Physics in Non-Hermitian Artificial Acoustic Boron Nitride. Phys. Rev. Lett. 2018, 120, 246601. [Google Scholar] [CrossRef] [PubMed]
- Ni, X.; Gorlach, M.A.; Alu, A.; Khanikaev, A.B. Topological Edge States in Acoustic Kagome Lattices. New J. Phys. 2017, 19, 55002. [Google Scholar] [CrossRef]
- Xia, B.; Zheng, S.; Liu, T.; Jiao, J.; Chen, N.; Dai, H.; Yu, D.; Liu, J. Observation of Valleylike Edge States of Sound at a Momentum Away from the High-Symmetry Points. Phys. Rev. B 2018, 97, 155124. [Google Scholar] [CrossRef]
- Vila, J.; Pal, R.K.; Ruzzene, M. Observation of Topological Valley Modes in an Elastic Hexagonal Lattice. Phys. Rev. B 2017, 96, 134307. [Google Scholar] [CrossRef]
- Chen, J.; Huo, S.; Geng, Z.; Huang, H.; Zhu, X. Topological Valley Transport of Plate-Mode Waves in a Homogenous Thin Plate with Periodic Stubbed Surface. Aip Adv. 2017, 7, 115215. [Google Scholar] [CrossRef]
- Huo, S.; Chen, J.; Huang, H.; Huang, G. Simultaneous Multi-Band Valley-Protected Topological Edge States of Shear Vertical Wave in Two-Dimensional Phononic Crystals with Veins. Sci. Rep. 2017, 7, 10335. [Google Scholar] [CrossRef]
- Liu, T.; Semperlotti, F. Tunable Acoustic Valley–Hall Edge States in Reconfigurable Phononic Elastic Waveguides. Phys. Rev. Appl. 2018, 9, 14001. [Google Scholar] [CrossRef]
- Pal, R.K.; Ruzzene, M. Edge Waves in Plates with Resonators: An Elastic Analogue of the Quantum Valley Hall Effect. New J. Phys. 2017, 19, 25001. [Google Scholar] [CrossRef]
- Yan, M.; Lu, J.; Li, F.; Deng, W.; Huang, X.; Ma, J.; Liu, Z. On-Chip Valley Topological Materials for Elastic Wave Manipulation. Nat. Mater. 2018, 17, 993–998. [Google Scholar] [CrossRef]
- Lera, N.; Torrent, D.; San-Jose, P.; Christensen, J.; Alvarez, J.V. Valley Hall Phases in Kagome Lattices. Phys. Rev. B 2019, 99, 134102. [Google Scholar] [CrossRef]
- Lu, J.; Qiu, C.; Xu, S.; Ye, Y.; Ke, M.; Liu, Z. Dirac Cones in Two-Dimensional Artificial Crystals for Classical Waves. Phys. Rev. B 2014, 89, 134302. [Google Scholar] [CrossRef]
- Chen, Z.; Ni, X.; Wu, Y.; He, C.; Sun, X.; Zheng, L.; Lu, M.; Chen, Y. Accidental Degeneracy of Double Dirac Cones in a Phononic Crystal. Sci. Rep. 2014, 4, 4613. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Wu, Y.; Mei, J. Double Dirac Cones in Phononic Crystals. Appl. Phys. Lett. 2014, 105, 14107. [Google Scholar] [CrossRef]
- Dai, H.; Liu, T.; Jiao, J.; Xia, B.; Yu, D. Double Dirac Cone in Two-Dimensional Phononic Crystals Beyond Circular Cells. J. Appl. Phys. 2017, 121, 135105. [Google Scholar] [CrossRef]
- Kong, X.; Zhou, Y.; Xiao, G.; Sievenpiper, D.F. Spin-Momentum Locked Modes on Anti-Phase Boundaries in Photonic Crystals. Opt. Express 2020, 28, 2070–2078. [Google Scholar] [CrossRef]
- Li, J.; Wang, J.; Wu, S.; Mei, J. Pseudospins and Topological Edge States in Elastic Shear Waves. Aip Adv. 2017, 7, 125030. [Google Scholar] [CrossRef]
- Nanthakumar, S.S.; Zhuang, X.; Park, H.S.; Nguyen, C.; Chen, Y.; Rabczuk, T. Inverse Design of Quantum Spin Hall-Based Phononic Topological Insulators. J. Mech. Phys. Solids 2019, 125, 550–571. [Google Scholar] [CrossRef]
- Chen, Y.; Meng, F.; Jia, B.; Li, G.; Huang, X. Inverse Design of Photonic Topological Insulators with Extra-Wide Bandgaps. Phys. Status Solidi Rapid Res. Lett. 2019, 13, 1900175. [Google Scholar] [CrossRef]
- Christiansen, R.E.; Wang, F.; Sigmund, O. Topological Insulators by Topology Optimization. Phys. Rev. Lett. 2019, 122, 234502. [Google Scholar] [CrossRef]
- Christiansen, R.E.; Wang, F.; Sigmund, O.; Stobbe, S. Designing Photonic Topological Insulators with Quantum-Spin-Hall Edge States Using Topology Optimization. Nanophotonics 2019, 8, 1363–1369. [Google Scholar] [CrossRef]
- Du, Z.; Chen, H.; Huang, G. Optimal Quantum Valley Hall Insulators by Rationally Engineering Berry Curvature and Band Structure. J. Mech. Phys. Solids 2020, 135, 103784. [Google Scholar] [CrossRef]
- Dong, H.; Zhao, S.; Zhu, R.; Wang, Y.; Cheng, L.; Zhang, C. Customizing Acoustic Dirac Cones and Topological Insulators in Square Lattices by Topology Optimization. J. Sound Vib. 2021, 493, 115687. [Google Scholar] [CrossRef]
- Xia, B.; Wang, G.; Zheng, S. Robust Edge States of Planar Phononic Crystals Beyond High-Symmetry Points of Brillouin Zones. J. Mech. Phys. Solids 2019, 124, 471–488. [Google Scholar] [CrossRef]
- He, W.; Chan, C.T. The Emergence of Dirac Points in Photonic Crystals with Mirror Symmetry. Sci. Rep. 2015, 5, 8186. [Google Scholar] [CrossRef] [PubMed]
- Mei, J.; Wu, Y.; Chan, C.T.; Zhang, Z. First-Principles Study of Dirac and Dirac-Like Cones in Phononic and Photonic Crystals. Phys. Rev. B 2012, 86, 35141. [Google Scholar] [CrossRef]
- Wang, Z.; Chong, Y.; Joannopoulos, J.D.; Soljačić, M. Observation of Unidirectional Backscattering-Immune Topological Electromagnetic States. Nature 2009, 461, 772–775. [Google Scholar] [CrossRef]
- He, C.; Sun, X.; Liu, X.; Lu, M.; Chen, Y.; Feng, L.; Chen, Y. Photonic Topological Insulator with Broken Time-Reversal Symmetry. Proc. Natl. Acad. Sci. USA 2016, 113, 4924–4928. [Google Scholar] [CrossRef]


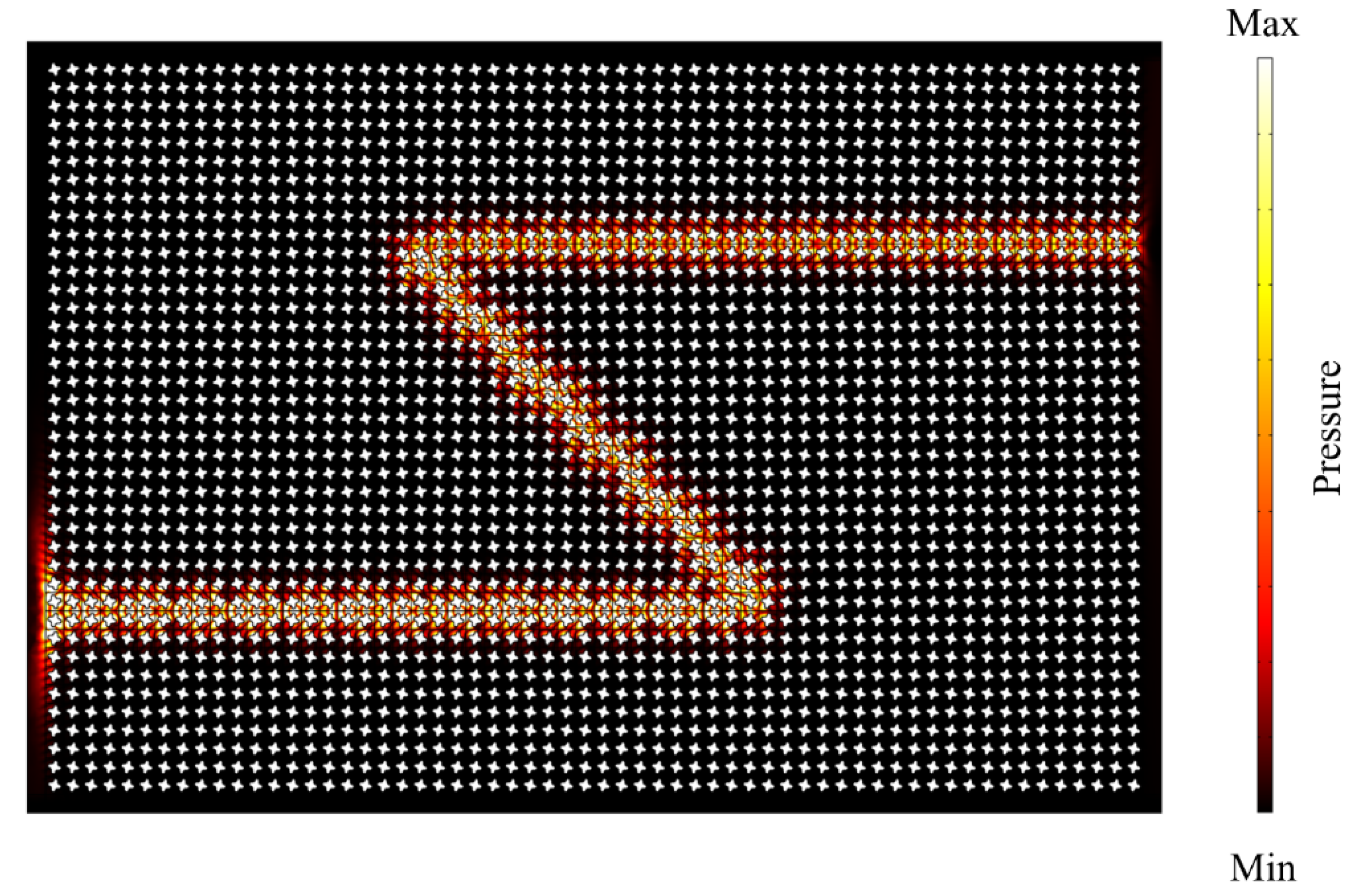
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, J.; Liu, Y.; Sun, D.; Hu, N.; Ning, H. Inverse Design of Valley-Like Edge States of Sound Degenerated Away from the High-Symmetry Points in a Square Lattice. Materials 2022, 15, 6697. https://doi.org/10.3390/ma15196697
Yang J, Liu Y, Sun D, Hu N, Ning H. Inverse Design of Valley-Like Edge States of Sound Degenerated Away from the High-Symmetry Points in a Square Lattice. Materials. 2022; 15(19):6697. https://doi.org/10.3390/ma15196697
Chicago/Turabian StyleYang, Jishi, Yaolu Liu, Dongyang Sun, Ning Hu, and Huiming Ning. 2022. "Inverse Design of Valley-Like Edge States of Sound Degenerated Away from the High-Symmetry Points in a Square Lattice" Materials 15, no. 19: 6697. https://doi.org/10.3390/ma15196697
APA StyleYang, J., Liu, Y., Sun, D., Hu, N., & Ning, H. (2022). Inverse Design of Valley-Like Edge States of Sound Degenerated Away from the High-Symmetry Points in a Square Lattice. Materials, 15(19), 6697. https://doi.org/10.3390/ma15196697




