Numerical Analysis of Microcrack Propagation Characteristics and Influencing Factors of Serrated Structural Plane
Abstract
:1. Introduction
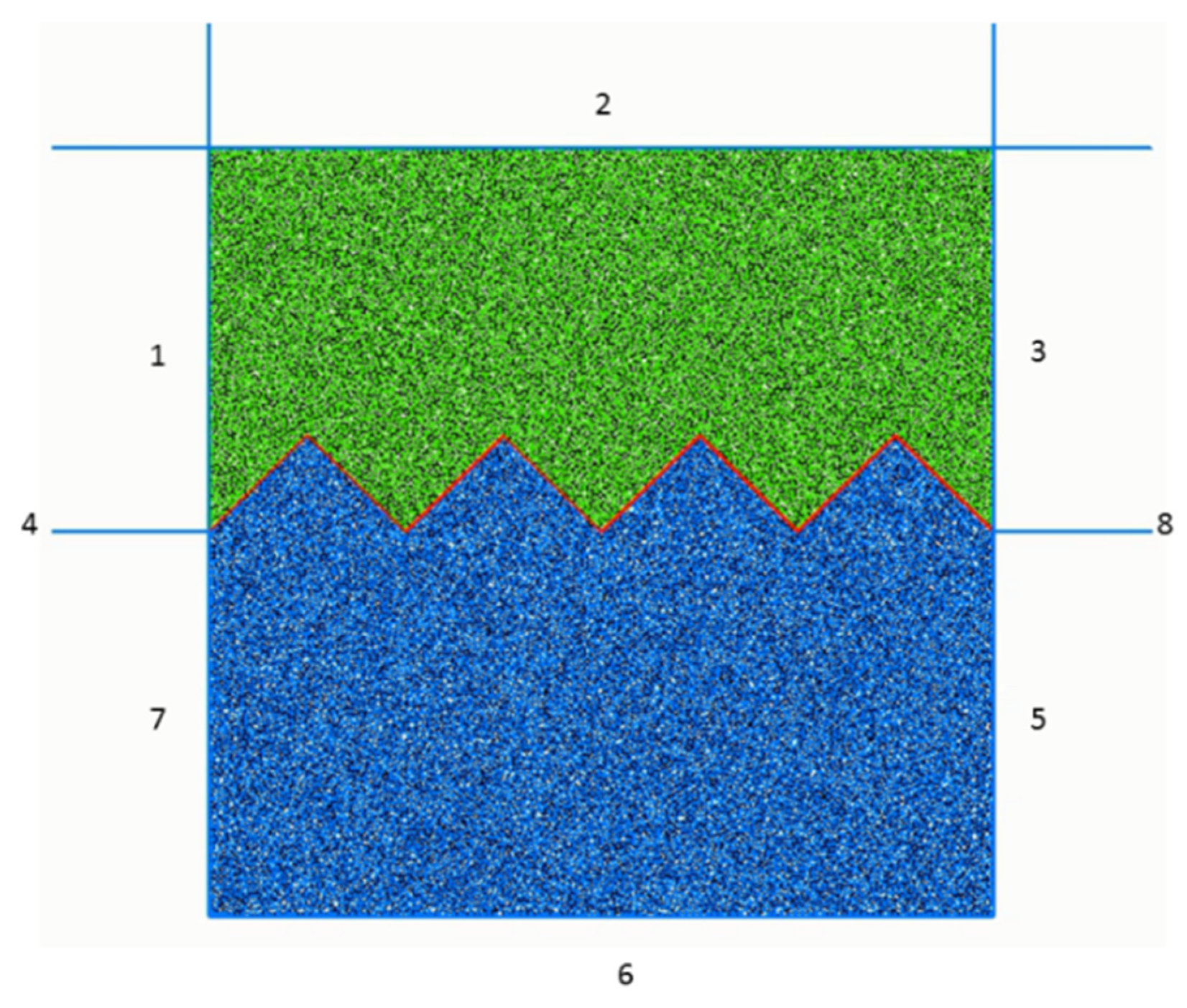
2. Establishment of the Numerical Calculation Model
3. Crack Evolution Rule in the Shear Process of the Structural Plane
3.1. The Crack Development Rule
3.2. The Evolution of Microcracks and Contact Force of Structure Plane
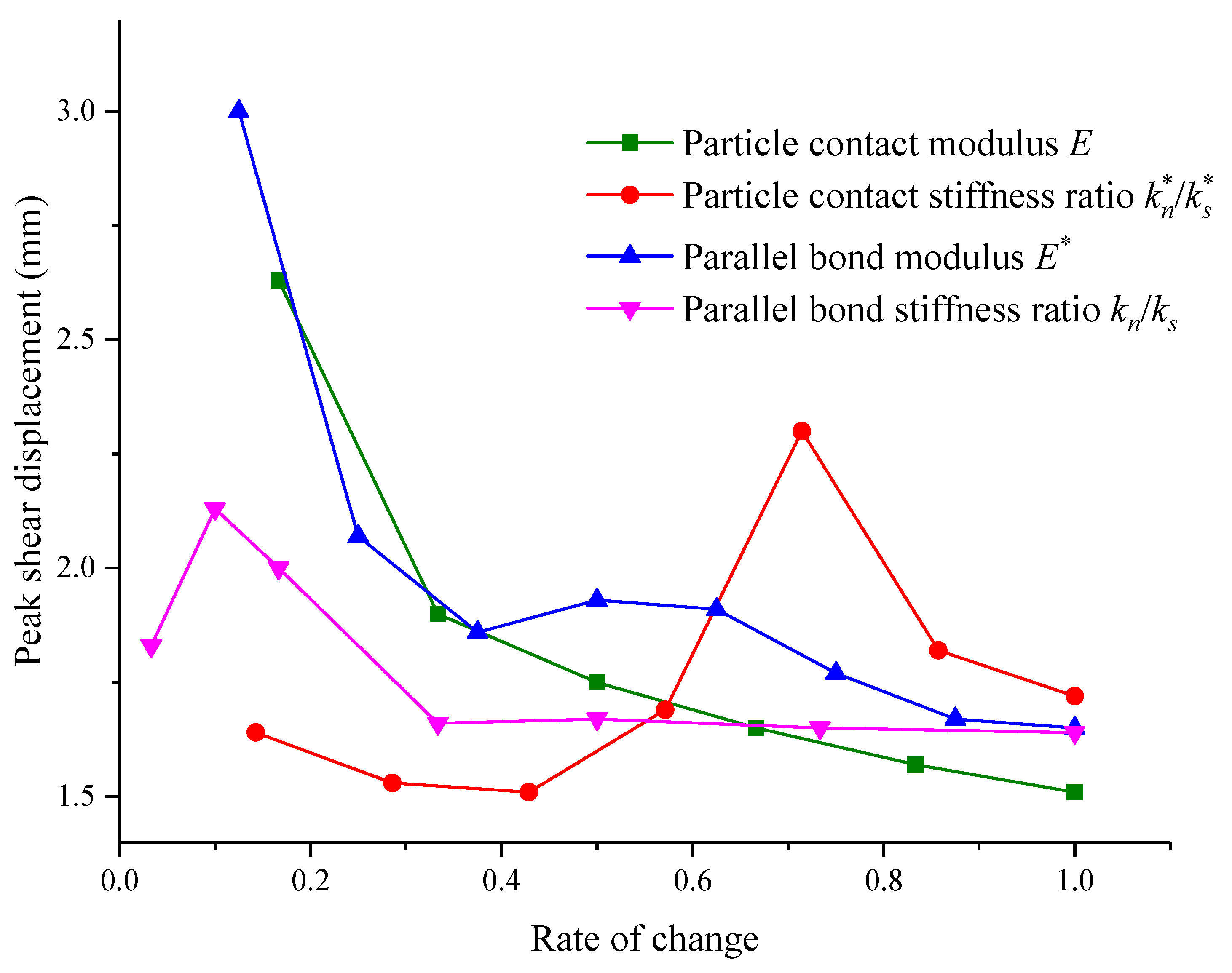
4. Effect of Micro-Parameters on Shear Mechanical Behavior
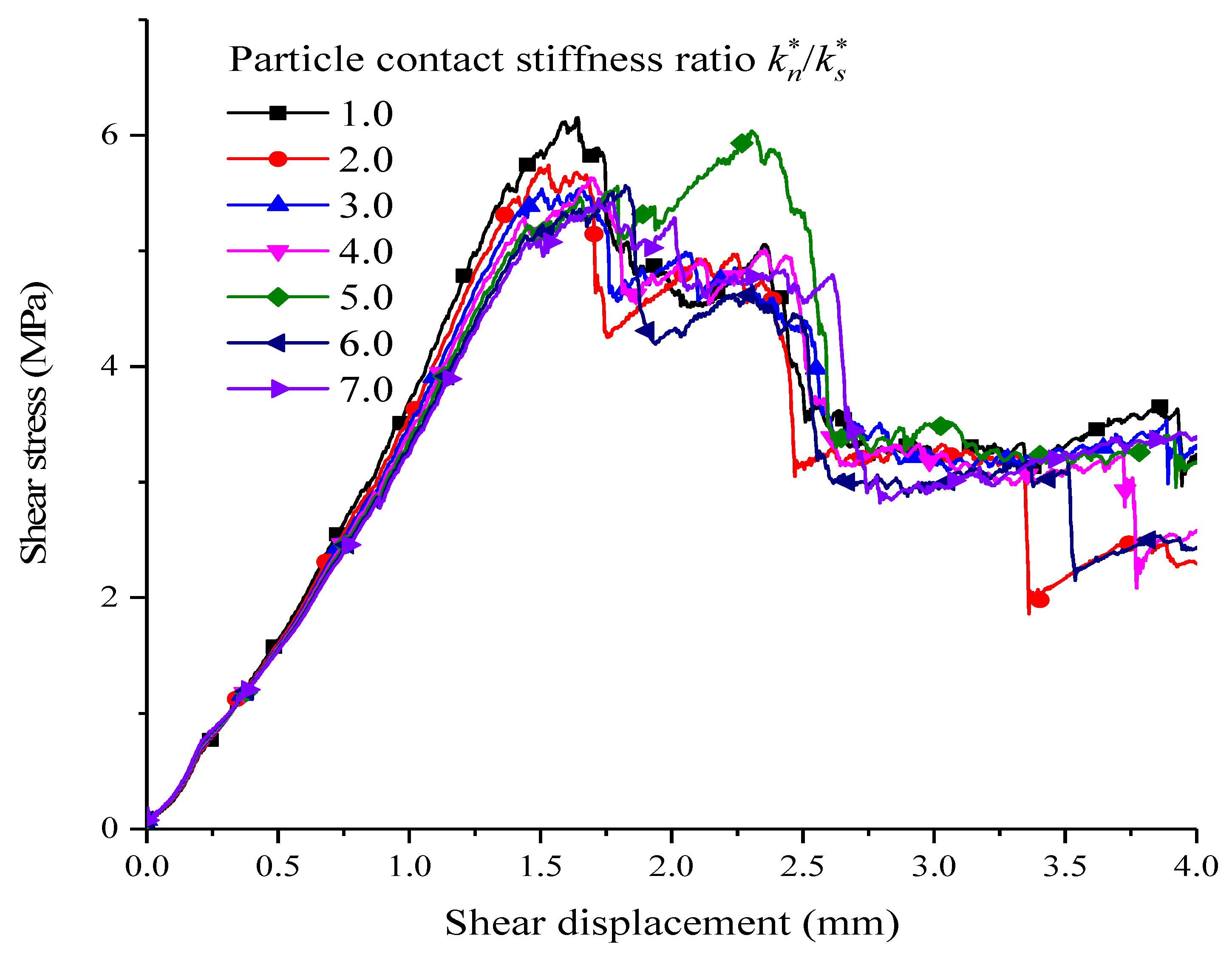
4.1. Parallel Bond Stiffness Ratio
4.2. Particle Contact Stiffness Ratio
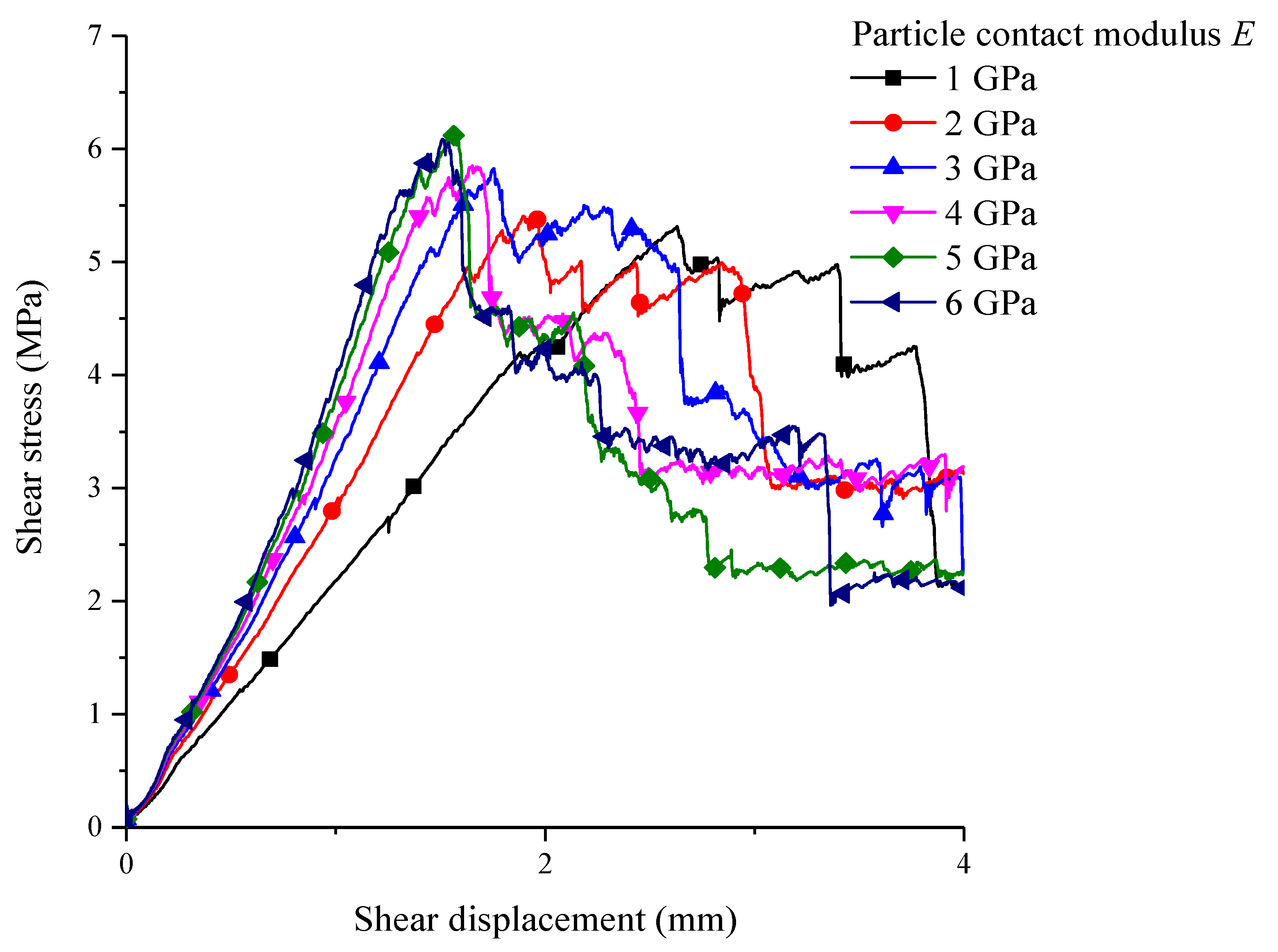
4.3. Particle Contact Modulus
4.4. Parallel Bond Modulus
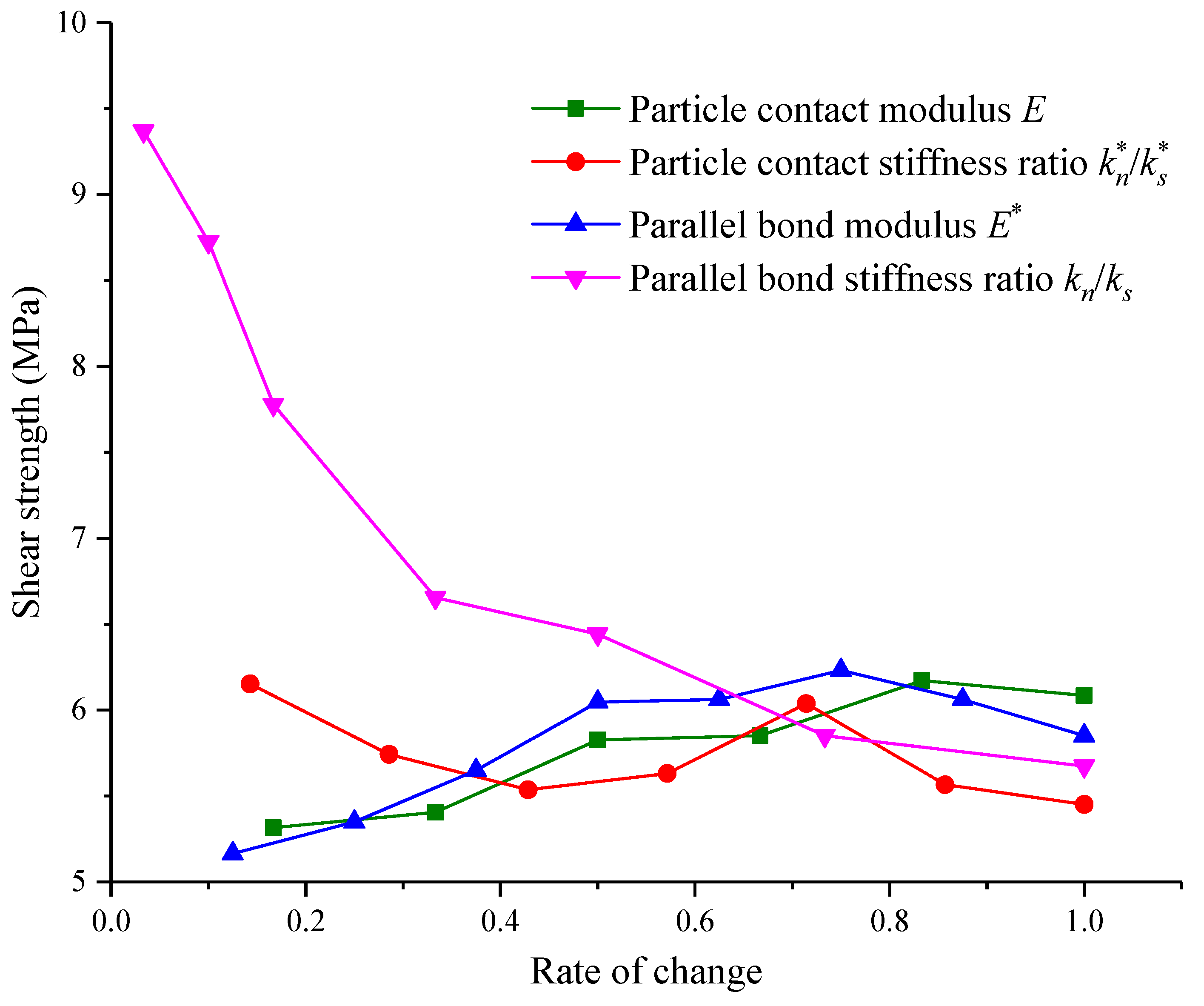
4.5. Effects of Micro-Parameters on Shear Strength of the Structural Plane
5. Quantitative Analysis of Micro-Parameters Based on Machine Learning
5.1. Extreme Gradient Boosting Decision Tree (XGBoost)
5.2. Particle Swarm Optimization (PSO) and Whale Optimization Algorithm (WOA)
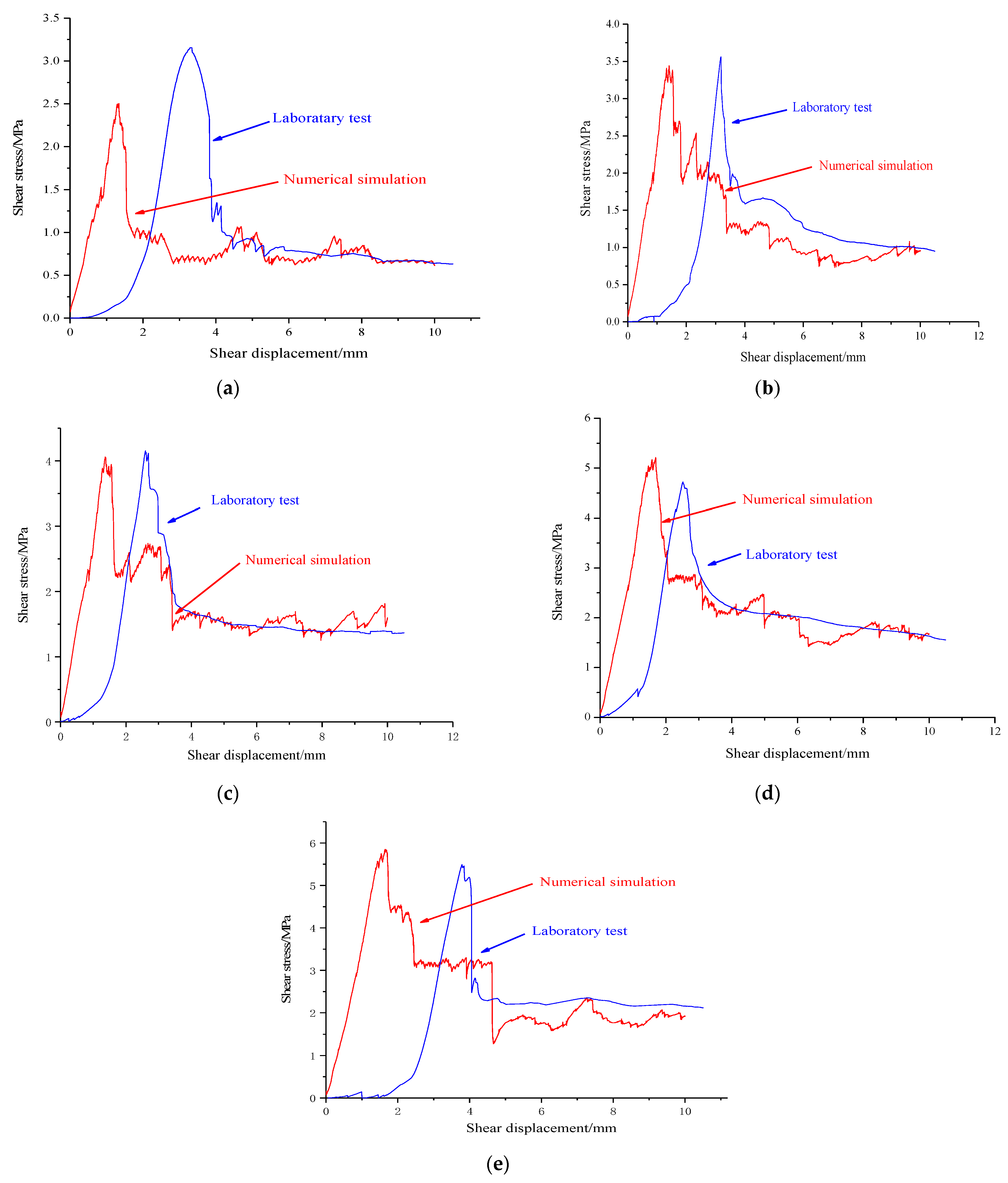
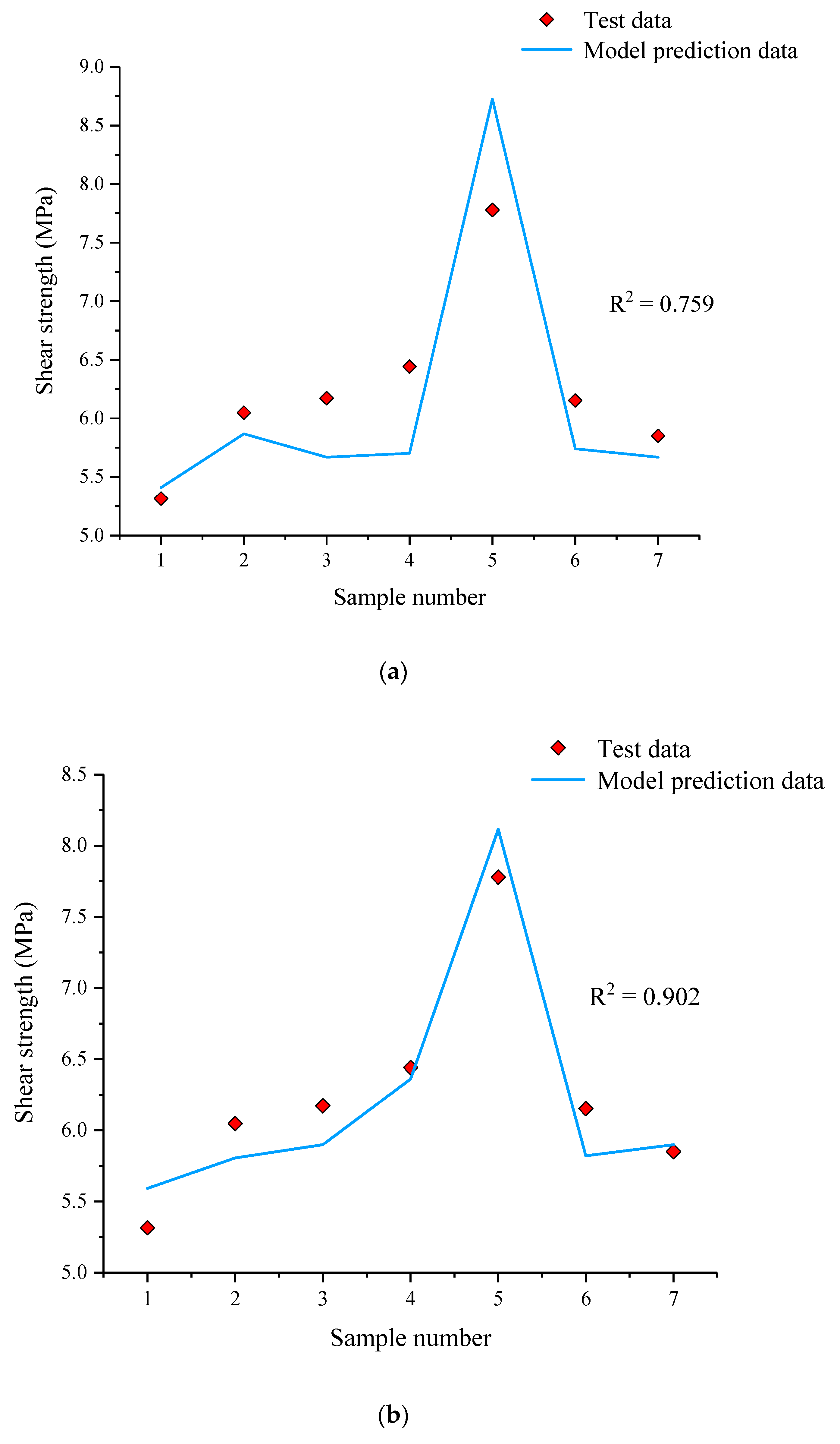
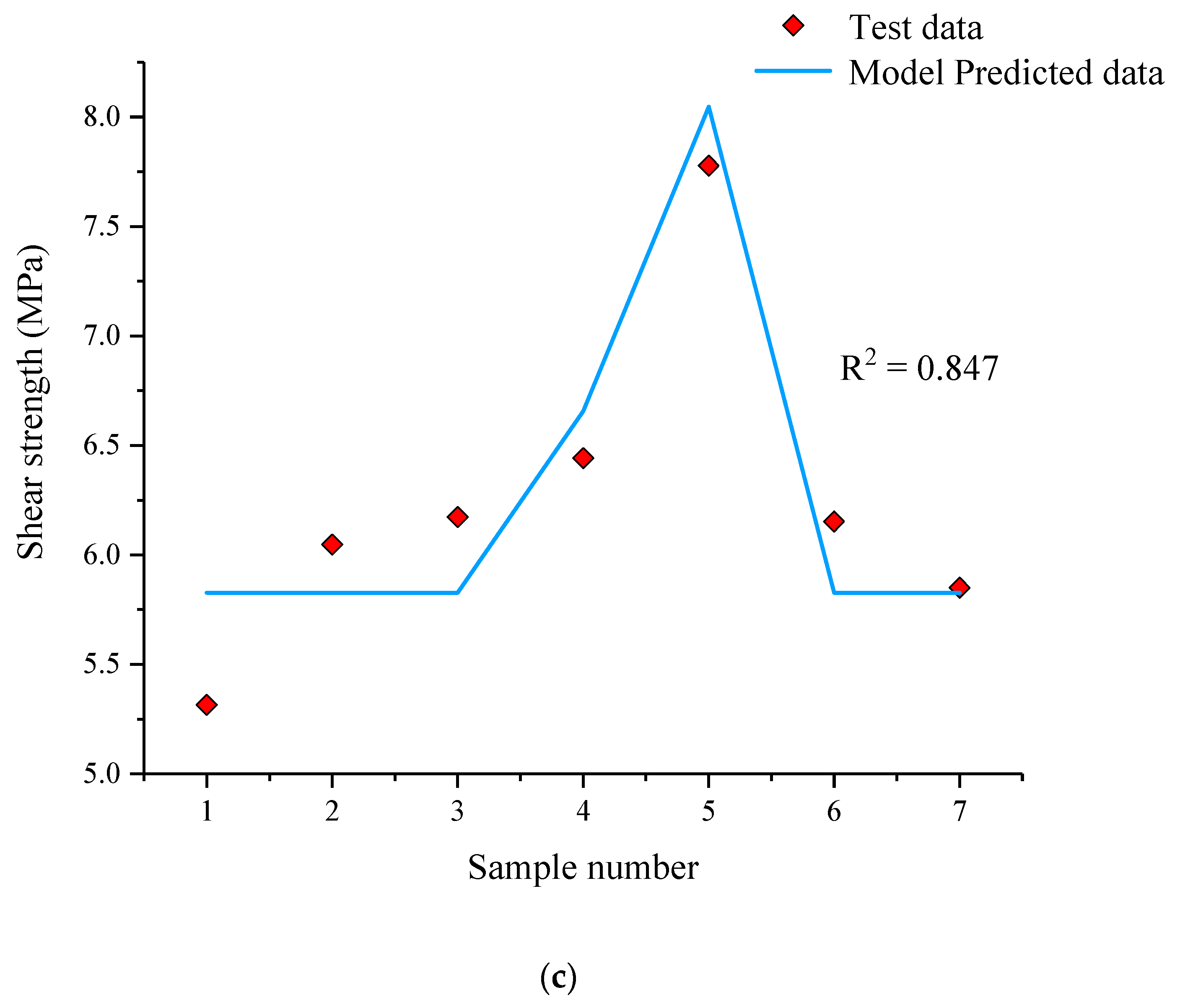
5.3. Model Validation and Analysis
6. Conclusions
- (1)
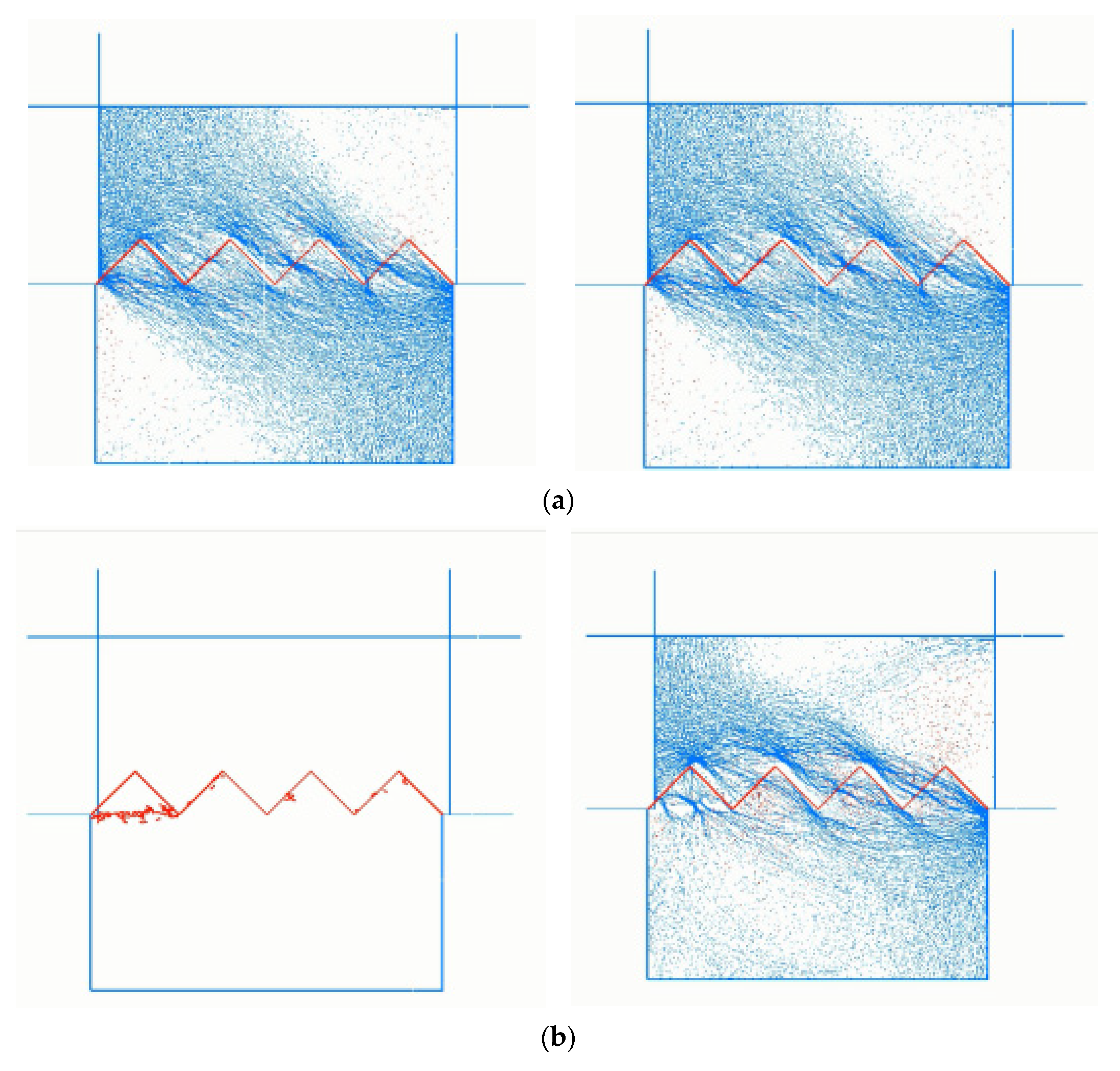
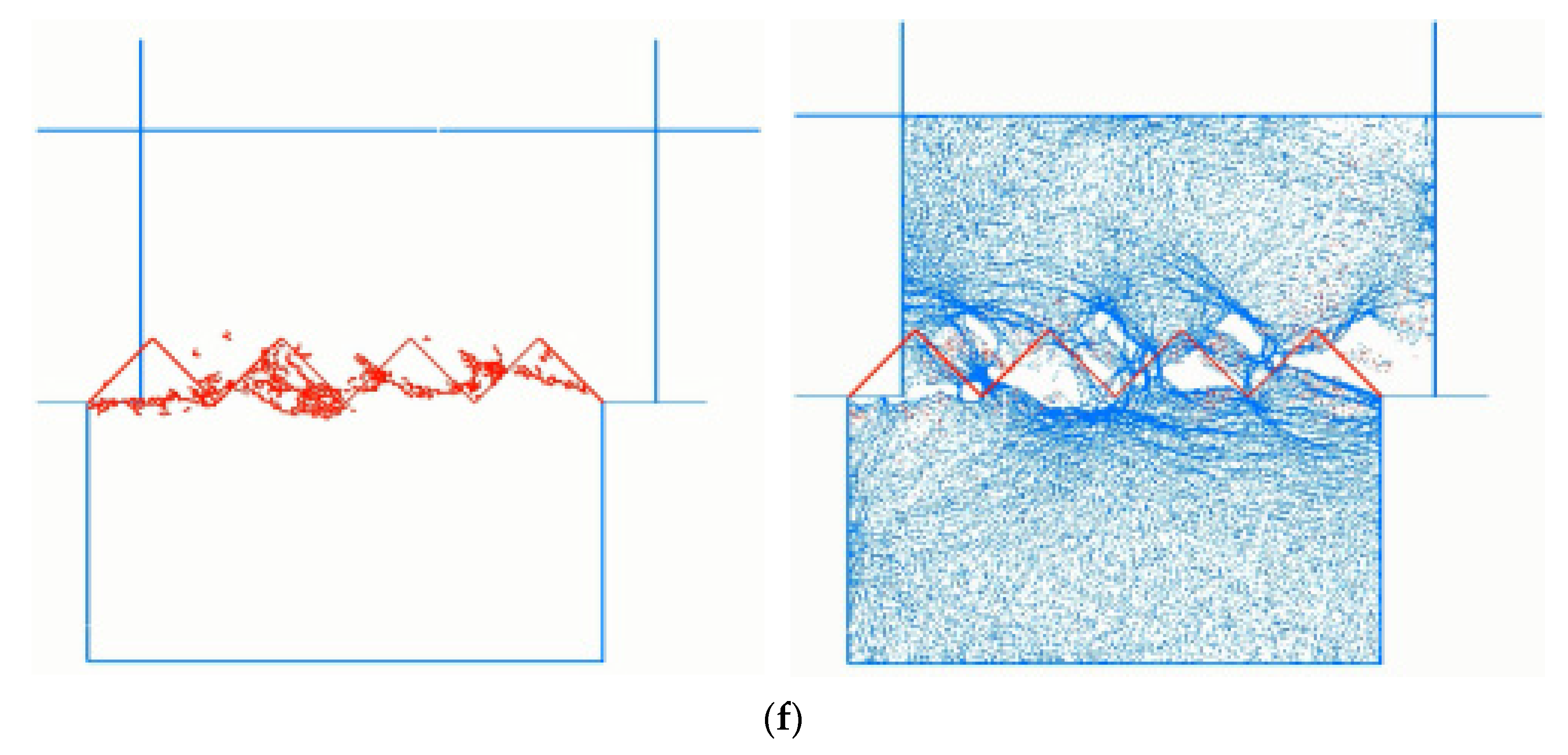
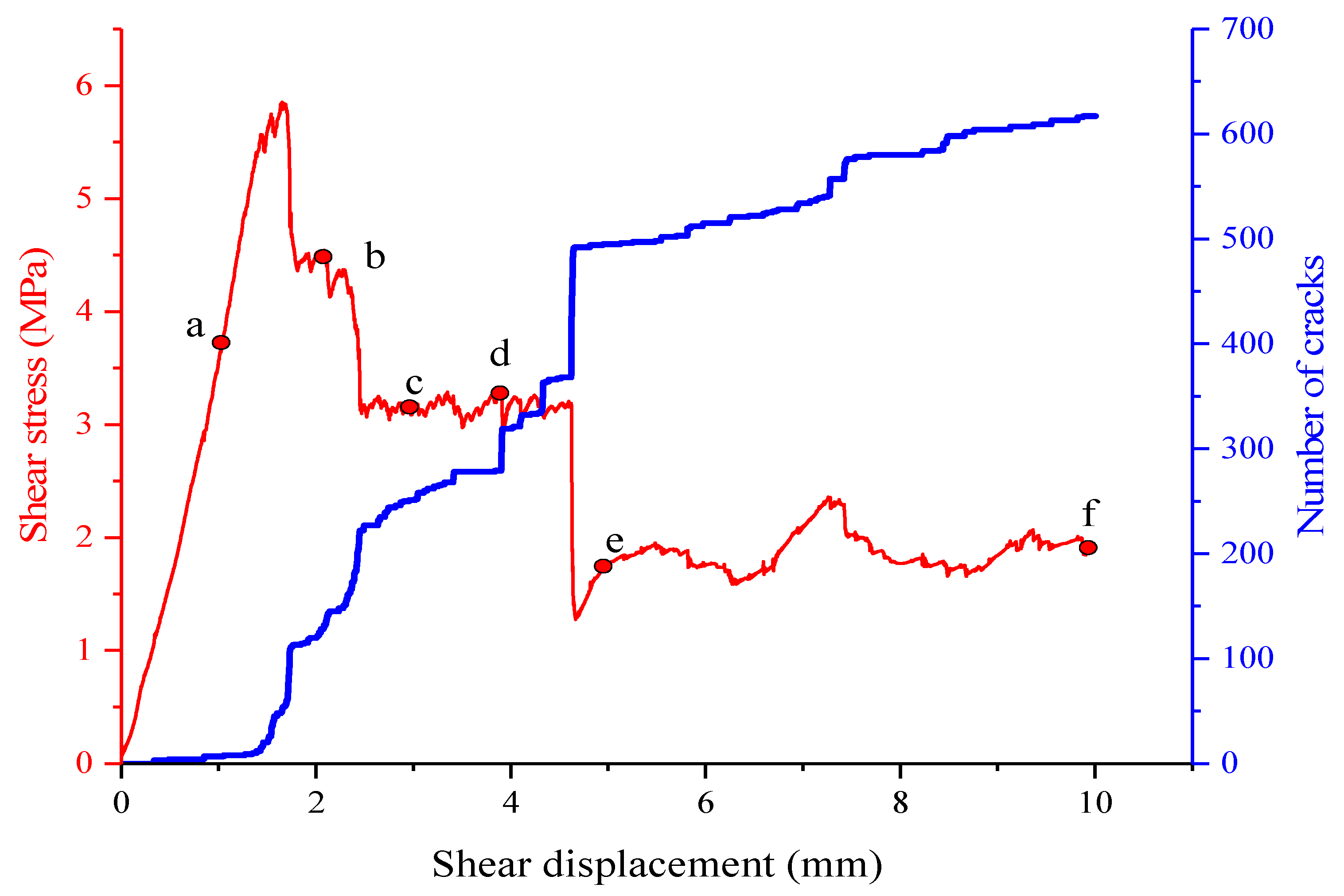
- The relationships among the number of microcracks, the normal stress and the shear stress during the shearing process of the serrated structural plane were analyzed. The results show that the greater the normal stress, the higher the number of microcracks. Before the shear stress reached its peak, the number of cracks increased slowly; after the shear stress reached its peak, the degrees of crack sprouting and expansion increased, the number of cracks kept increasing and the damage accumulated. Eventually, when the shear stress reached the residual shear strength, the number of microcracks tended to stabilize.
- (2)
- During the shearing process, the contact force distribution on the serrated surface has similarity with the evolutionary law of microcracks. In the initial stage of shearing, the contact force chain is mainly concentrated at the root of the sawtooth. As the shear progresses, the contact force chain becomes more and more concentrated, and the force on the root of the sawtooth becomes larger and larger. When the failure finally occurred, a large number of cracks appeared at the root of the sawtooth.
- (3)
- The four meso-parameters (particle contact modulus , particle contact stiffness ratio , parallel bond modulus , and parallel bond stiffness ratio ) have certain influences on the shear stress–shear displacement curve, shear strength and peak shear displacement. The particle contact stiffness ratio and parallel bond stiffness ratio are negatively correlated with the shear strength; and the particle contact modulus and parallel bond modulus are positively correlated with the shear strength. The peak shear displacement gradually decreases as the particle contact modulus and parallel bond modulus increase. The parallel bond stiffness ratio was negatively correlated with the peak shear displacement to some extent. The effect of parallel bond stiffness ratio on the peak shear displacement was not significant.
- (4)
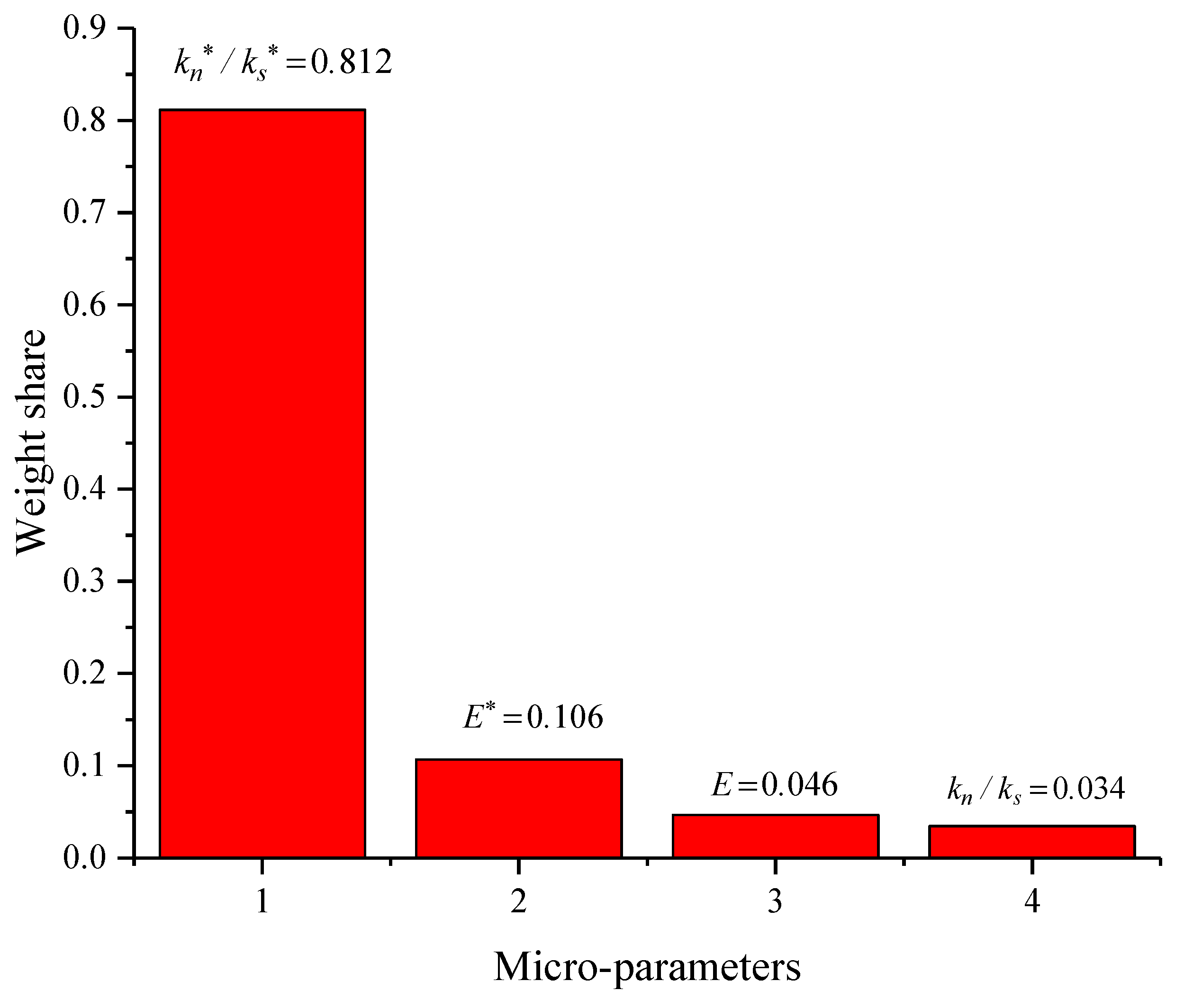
- To further quantitatively study the relationships between micro-parameters and shear strength, XGBoost, WOA-XGBoost, and PSO-XGBoost algorithms were introduced to construct the quantitative prediction model. It was found that WOA-XGBoost had the highest prediction accuracy and the best agreement between the predicted data and test data with an R2 of 0.902. Using this model to calculate the weight shares of micro-parameters, we realized the weight share of should be the highest, 0.812, followed by at 0.106, and the weight shares of and were the lowest, adding up to less than 0.1. This shows that is the factor that has the greatest influence on shear strength; has a small influence; and have almost no influence.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chen, Y.; Lin, H.; Li, S.; Cao, R.; Yong, W.; Wang, Y.; Zhao, Y. Shear expression derivation and parameter evaluation of Hoek–Brown criterion. Arch. Civ. Mech. Eng. 2022, 22, 77. [Google Scholar] [CrossRef]
- Tang, Y.; Lin, H.; Wang, Y.; Zhao, Y. Rock slope stability analysis considering the effect of locked section. Bull. Eng. Geol. Environ. 2021, 80, 7241–7251. [Google Scholar] [CrossRef]
- Tang, W.; Lin, H.; Chen, Y.; Feng, J.; Hu, H. Mechanical Characteristics and Acoustic Emission Characteristics of Mortar-Rock Binary Medium. Buildings 2022, 12, 665. [Google Scholar] [CrossRef]
- Beer, A.J.; Stead, D.; Coggan, J.S. Technical Note Estimation of the Joint Roughness Coefficient (JRC) by Visual Comparison. Rock Mech. Rock Eng. 2002, 35, 65–74. [Google Scholar] [CrossRef]
- Belem, T.; Souley, M.; Homand, F. Method for Quantification of Wear of Sheared Joint Walls Based on Surface Morphology. Rock Mech. Rock Eng. 2008, 42, 883–910. [Google Scholar] [CrossRef]
- Zhao, J. Joint surface matching and shear strength part B: JRC-JMC shear strength criterion. Int. J. Rock Mech. Min. Sci. 1997, 34, 179–185. [Google Scholar] [CrossRef]
- Park, J.-W.; Song, J.-J. Numerical simulation of a direct shear test on a rock joint using a bonded-particle model. Int. J. Rock Mech. Min. Sci. 2009, 46, 1315–1328. [Google Scholar] [CrossRef]
- Xie, S.; Lin, H.; Wang, Y.; Chen, Y.; Xiong, W.; Zhao, Y.; Du, S. A statistical damage constitutive model considering whole joint shear deformation. Int. J. Damage Mech. 2020, 29, 988–1008. [Google Scholar] [CrossRef]
- Lei, D.; Lin, H.; Wang, Y. Damage characteristics of shear strength of joints under freeze–thaw cycles. Arch. Appl. Mech. 2022, 92, 1615–1631. [Google Scholar] [CrossRef]
- Du, S.-G.; Lin, H.; Yong, R.; Liu, G.-J. Characterization of Joint Roughness Heterogeneity and Its Application in Representative Sample Investigations. Rock Mech. Rock Eng. 2022, 55, 3253–3277. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhang, C.; Wang, Y.; Lin, H. Shear-related roughness classification and strength model of natural rock joint based on fuzzy comprehensive evaluation. Int. J. Rock Mech. Min. Sci. 2020, 137, 104550. [Google Scholar] [CrossRef]
- Zhang, X.; Lin, H.; Wang, Y.; Yong, R.; Zhao, Y.; Du, S. Damage evolution characteristics of saw-tooth joint under shear creep condition. Int. J. Damage Mech. 2020, 30, 453–480. [Google Scholar] [CrossRef]
- Lin, H.; Sun, P.; Chen, Y.; Zhu, Y.; Fan, X.; Zhao, Y. Analytical and experimental analysis of the shear strength of bolted saw-tooth joints. Eur. J. Environ. Civ. Eng. 2020, 26, 1639–1653. [Google Scholar] [CrossRef]
- Xie, S.; Lin, H.; Cheng, C.; Chen, Y.; Wang, Y.; Zhao, Y.; Yong, W. Shear strength model of joints based on Gaussian smoothing method and macro-micro roughness. Comput. Geotech. 2021, 143, 104605. [Google Scholar] [CrossRef]
- Liu, B.; Lin, H.; Chen, Y. Deformation Characteristics of Bolted Rock Joints under Compression-Shear Load. Appl. Sci. 2022, 12, 5226. [Google Scholar] [CrossRef]
- Zhao, Y.; Chang, L.; Wang, Y.; Lin, H.; Liao, J.; Liu, Q. Dynamic response of cylindrical thick-walled granite specimen with clay infilling subjected to dynamic loading. Ingenieur-Archiv 2022, 92, 643–648. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhang, L.; Wang, W.; Liu, Q.; Tang, L.; Cheng, G. Experimental Study on Shear Behavior and a Revised Shear Strength Model for Infilled Rock Joints. Int. J. Géoméch. 2020, 20, 04020141. [Google Scholar] [CrossRef]
- Oh, J.; Li, Y.; Mitra, R.; Canbulat, I. A Numerical Study on Dilation of a Saw-Toothed Rock Joint Under Direct Shear. Rock Mech. Rock Eng. 2016, 50, 913–925. [Google Scholar] [CrossRef]
- Fan, X.; Yu, H.; Deng, Z.; He, Z.; Zhao, Y. Cracking and deformation of cuboidal sandstone with a single nonpenetrating flaw under uniaxial compression. Theor. Appl. Fract. Mech. 2022, 119, 103284. [Google Scholar] [CrossRef]
- Fan, X.; Yang, Z.; Li, K. Effects of the lining structure on mechanical and fracturing behaviors of four-arc shaped tunnels in a jointed rock mass under uniaxial compression. Theor. Appl. Fract. Mech. 2021, 112, 102887. [Google Scholar] [CrossRef]
- Li, X.; Li, Q.; Hu, Y.; Chen, Q.; Peng, J.; Xie, Y.; Wang, J. Study on Three-Dimensional Dynamic Stability of Open-Pit High Slope under Blasting Vibration. Lithosphere 2022, 2021. [Google Scholar] [CrossRef]
- Li, X.; Peng, J.; Xie, Y.; Li, Q.; Zhou, T.; Wang, J.; Zheng, W. Influence of High-Temperature Treatment on Strength and Failure Behaviors of a Quartz-Rich Sandstone under True Triaxial Condition. Lithosphere 2022, 2022. [Google Scholar] [CrossRef]
- Liu, J.; Zhao, Y.; Tan, T.; Zhang, L.; Zhu, S.; Xu, F. Evolution and modeling of mine water inflow and hazard characteristics in southern coalfields of China: A case of Meitanba mine. Int. J. Min. Sci. Technol. 2022, 32, 513–524. [Google Scholar] [CrossRef]
- Bahaaddini, M.; Hagan, P.; Mitra, R.; Khosravi, M. Experimental and numerical study of asperity degradation in the direct shear test. Eng. Geol. 2016, 204, 41–52. [Google Scholar] [CrossRef]
- Chen, Y.; Lin, H.; Xie, S.; Ding, X.; He, D.; Yong, W.; Gao, F. Effect of joint microcharacteristics on macroshear behavior of single-bolted rock joints by the numerical modelling with PFC. Environ. Earth Sci. 2022, 81, 1–12. [Google Scholar] [CrossRef]
- Zhou, K.; Liu, S.; Cheng, Y. Effect of Rheological Mesoparameters on Shear Mechanical Behavior of Joints. Geofluids 2022, 2022, 3512507. [Google Scholar] [CrossRef]
- Kong, W.; Li, Y.; Nie, L.; Dong, Z.; Cai, W.; Wang, Z.; Wang, K. Experimental and numerical investigations on crack propagation characteristics of rock-like specimens with preexisting flaws subjected to combined actions of internal hydraulic pressure and shear force. Arch. Appl. Mech. 2021, 92, 221–239. [Google Scholar] [CrossRef]
- Bahaaddini, M.; Sharrock, G.; Hebblewhite, B. Numerical direct shear tests to model the shear behaviour of rock joints. Comput. Geotech. 2013, 51, 101–115. [Google Scholar] [CrossRef]
- Jiang, M.; Liu, J.; Crosta, G.B.; Li, T. DEM analysis of the effect of joint geometry on the shear behavior of rocks. Comptes Rendus Mécanique 2017, 345, 779–796. [Google Scholar] [CrossRef]
- Yang, X.-X.; Qiao, W. Numerical investigation of the shear behavior of granite materials containing discontinuous joints by utilizing the flat-joint model. Comput. Geotech. 2018, 104, 69–80. [Google Scholar] [CrossRef]
- Xie, S.-J.; Lin, H.; Chen, Y.-F.; Wang, Y.-X. A new nonlinear empirical strength criterion for rocks under conventional triaxial compression. J. Central South Univ. 2021, 28, 1448–1458. [Google Scholar] [CrossRef]
- Zhang, C.; Wang, Y.; Ruan, H.; Ke, B.; Lin, H. The strain characteristics and corresponding model of rock materials under uniaxial cyclic load/unload compression and their deformation and fatigue damage analysis. Arch. Appl. Mech. 2021, 91, 2481–2496. [Google Scholar] [CrossRef]
- Zhang, C.; Lin, H.; Qiu, C.; Jiang, T.; Zhang, J. The effect of cross-section shape on deformation, damage and failure of rock-like materials under uniaxial compression from both a macro and micro viewpoint. Int. J. Damage Mech. 2020, 29, 1076–1099. [Google Scholar] [CrossRef]
- Yang, H.; Lin, H.; Chen, Y.; Wang, Y.; Zhao, Y.; Yong, W.; Gao, F. Influence of wing crack propagation on the failure process and strength of fractured specimens. Bull. Eng. Geol. Environ. 2022, 81, 1–19. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhang, L.; Liao, J.; Wang, W.; Liu, Q.; Tang, L. Experimental Study of Fracture Toughness and Subcritical Crack Growth of Three Rocks under Different Environments. Int. J. Géoméch. 2020, 20, 04020128. [Google Scholar] [CrossRef]
- Zhao, Y.; Liao, J.; Wang, Y.; Liu, Q.; Lin, H.; Chang, L. Crack coalescence patterns and local strain behaviors near flaw tip for rock-like material containing two flaws subjected to biaxial compression. Arab. J. Geosci. 2020, 13, 1–18. [Google Scholar] [CrossRef]
- Zhang, K.; Li, N.; Liu, W.; Xie, J. Experimental study of the mechanical, energy conversion and frictional heating characteristics of locking sections. Eng. Fract. Mech. 2020, 228, 106905. [Google Scholar] [CrossRef]
- Zhou, K.; Liu, C.; Li, S.; Cheng, Y. Size Effect on the Rheological Shear Mechanical Behaviors of Different Joints: A Numerical Study. Geofluids 2022, 2022, 1–9. [Google Scholar] [CrossRef]
- Cheng, Y.; He, D.; Qin, J.; Yang, J. Effect of Sawtooth Angle on Shear Mechanical Behavior of Structural Plane. Geotech. Geol. Eng. 2021, 39, 4169–4180. [Google Scholar] [CrossRef]
- Ruiyi, H.; Zhuwen, W.; Wenhua, W.; Fanghui, X.; Xinghua, Q.; Yitong, C. Lithology identification of igneous rocks based on XGboost and conventional logging curves, a case study of the eastern depression of Liaohe Basin. J. Appl. Geophys. 2021, 195, 104480. [Google Scholar] [CrossRef]
- Elbegue, A.A.; Allek, K.; Zeghouane, H. Geological mapping using extreme gradient boosting and the deep neural networks: Application to silet area, central Hoggar, Algeria. Acta Geophys. 2022, 70, 1581–1599. [Google Scholar] [CrossRef]
- Armaghani, D.J.; Mohamad, E.T.; Narayanasamy, M.S.; Narita, N.; Yagiz, S. Development of hybrid intelligent models for predicting TBM penetration rate in hard rock condition. Tunn. Undergr. Space Technol. 2017, 63, 29–43. [Google Scholar] [CrossRef]
- Murlidhar, B.R.; Nguyen, H.; Rostami, J.; Bui, X.; Armaghani, D.J.; Ragam, P.; Mohamad, E.T. Prediction of flyrock distance induced by mine blasting using a novel Harris Hawks optimization-based multi-layer perceptron neural network. J. Rock Mech. Geotech. Eng. 2021, 13, 1413–1427. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, D.X.; Zhang, X. Performance Analysis of Whale Optimization Algorithm. In Proceedings of the 7th International Conference on Communications, Signal Processing, and Systems (CSPS), Dalian, China, 14–16 July 2018; pp. 379–386. [Google Scholar]





| Min Particle Diameter (mm) | Particle Radius Ratio | Contact Modulus (GPa) | Contact Stiffness Ratio | Bond Modulus (GPa) | Bond Stiffness Ratio | Parallel Bond Normal Strength (MPa) | Parallel Bond Cohesion (MPa) | Internal Friction Angle of Parallel Bonding |
|---|---|---|---|---|---|---|---|---|
| 0.4 | 1.6 | 4 | 2.1 | 0.08 | 2.2 | 7.2 | 23.56 | 53.3 |
| Test Condition | Simulation Condition | |
|---|---|---|
| Cohesion (MPa) | 2.466 | 1.713 |
| Internal friction angle (°) | 55.55 | 64.21 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, X.; Lin, H.; Qin, J.; Cao, R.; Ma, S.; Hu, H. Numerical Analysis of Microcrack Propagation Characteristics and Influencing Factors of Serrated Structural Plane. Materials 2022, 15, 5287. https://doi.org/10.3390/ma15155287
Zhang X, Lin H, Qin J, Cao R, Ma S, Hu H. Numerical Analysis of Microcrack Propagation Characteristics and Influencing Factors of Serrated Structural Plane. Materials. 2022; 15(15):5287. https://doi.org/10.3390/ma15155287
Chicago/Turabian StyleZhang, Xing, Hang Lin, Jianxin Qin, Rihong Cao, Shaowei Ma, and Huihua Hu. 2022. "Numerical Analysis of Microcrack Propagation Characteristics and Influencing Factors of Serrated Structural Plane" Materials 15, no. 15: 5287. https://doi.org/10.3390/ma15155287
APA StyleZhang, X., Lin, H., Qin, J., Cao, R., Ma, S., & Hu, H. (2022). Numerical Analysis of Microcrack Propagation Characteristics and Influencing Factors of Serrated Structural Plane. Materials, 15(15), 5287. https://doi.org/10.3390/ma15155287








