Modified Micro-Mechanics Based Multiscale Model for Damage Analysis of Open-Hole Composite Laminates under Compression
Abstract
:1. Introduction
2. Multiscale Finite Element Modeling
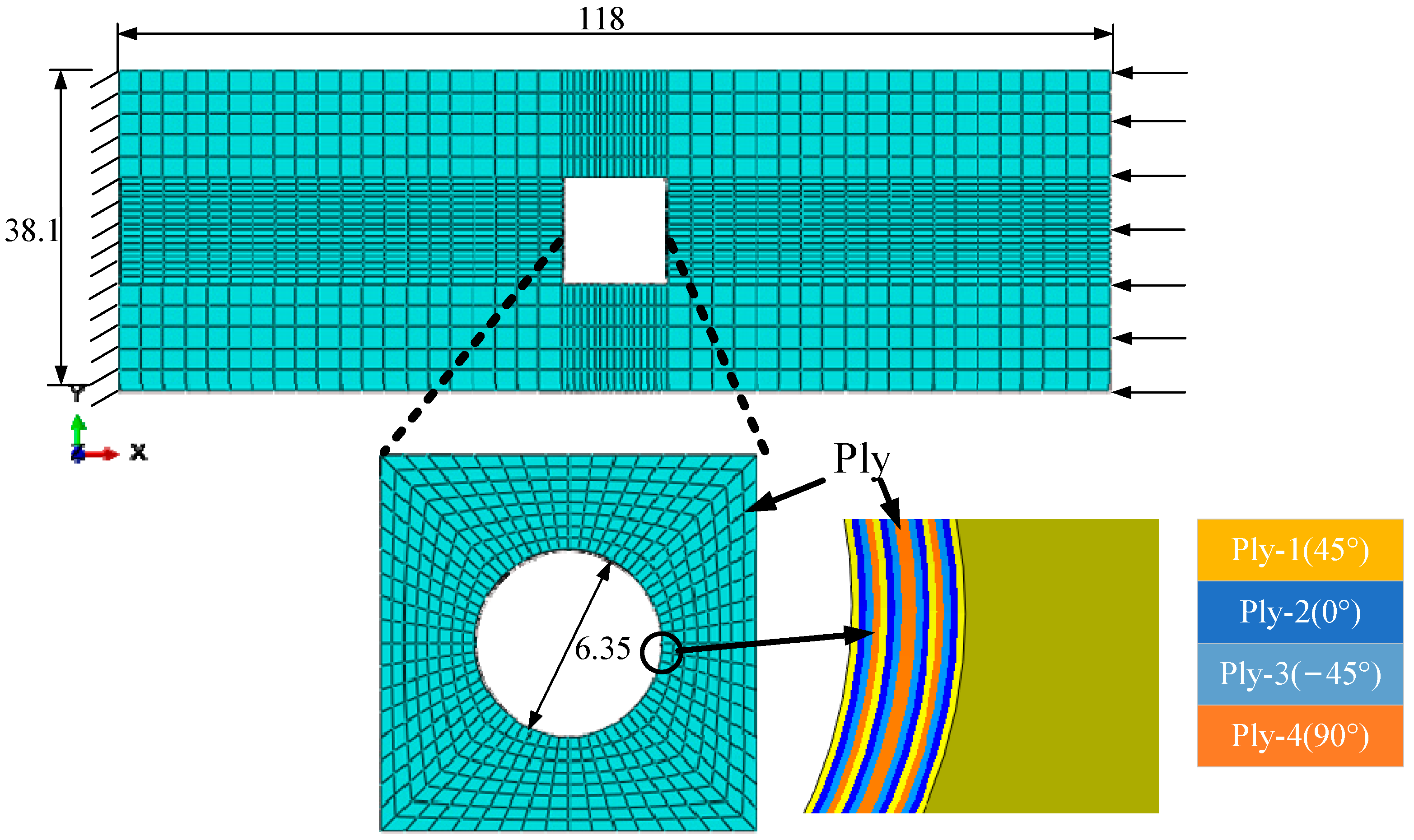
2.1. Macro Model
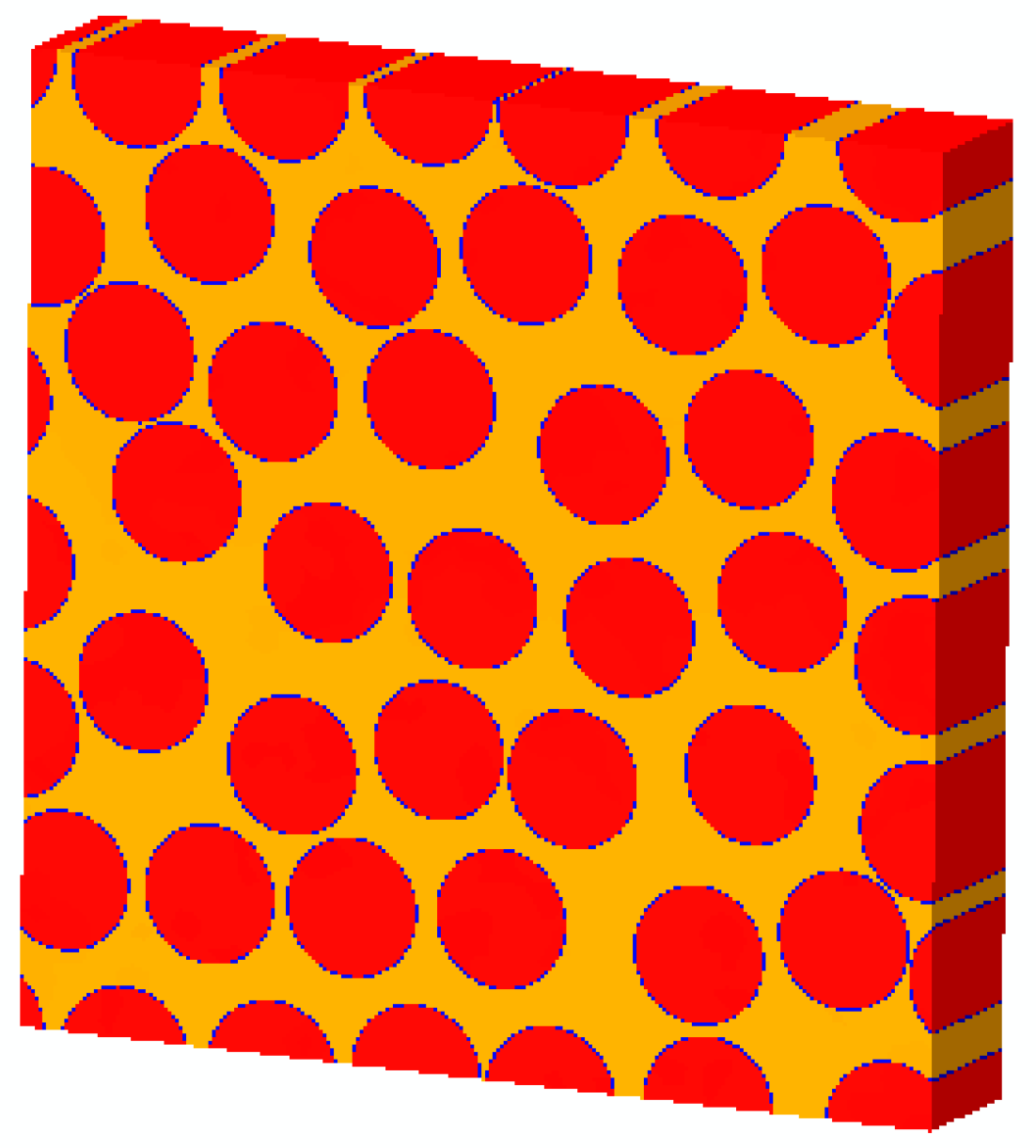
2.2. Micro Model Considering Interfaces
3. Multiscale Model Considering Interface
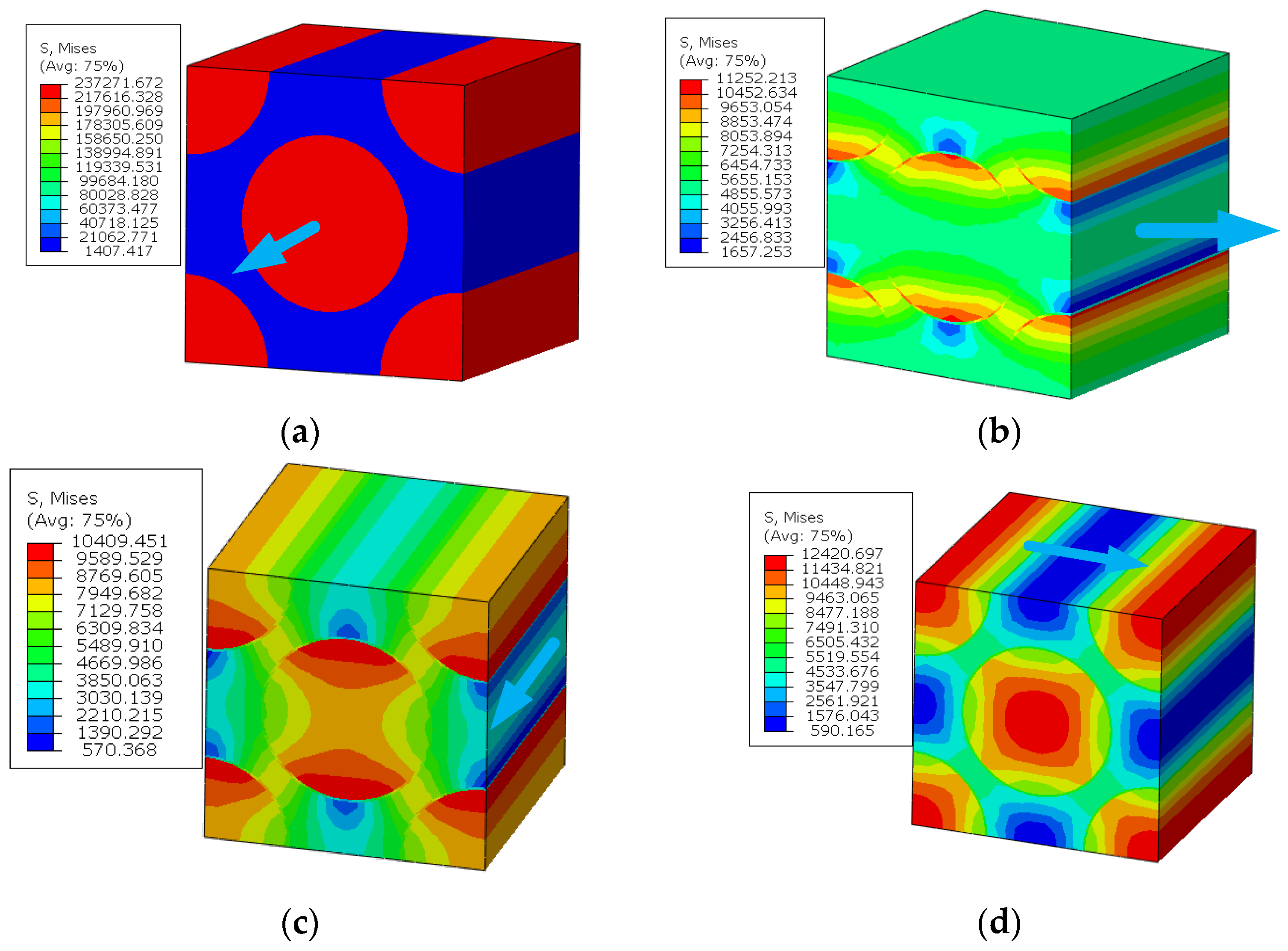
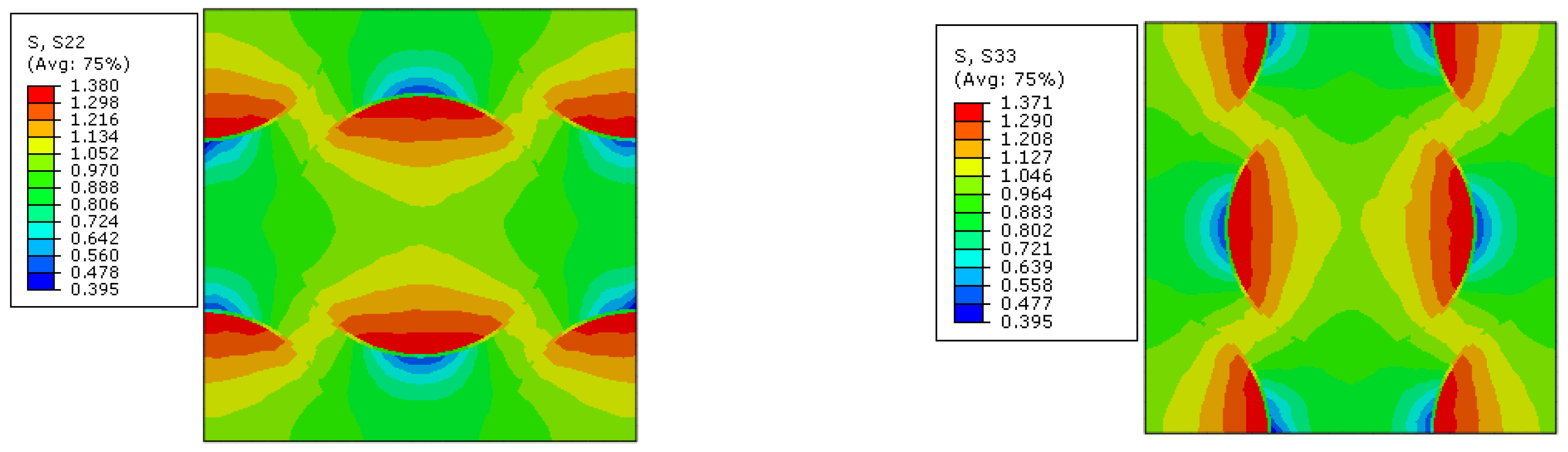
3.1. Calculation of Amplification Factors
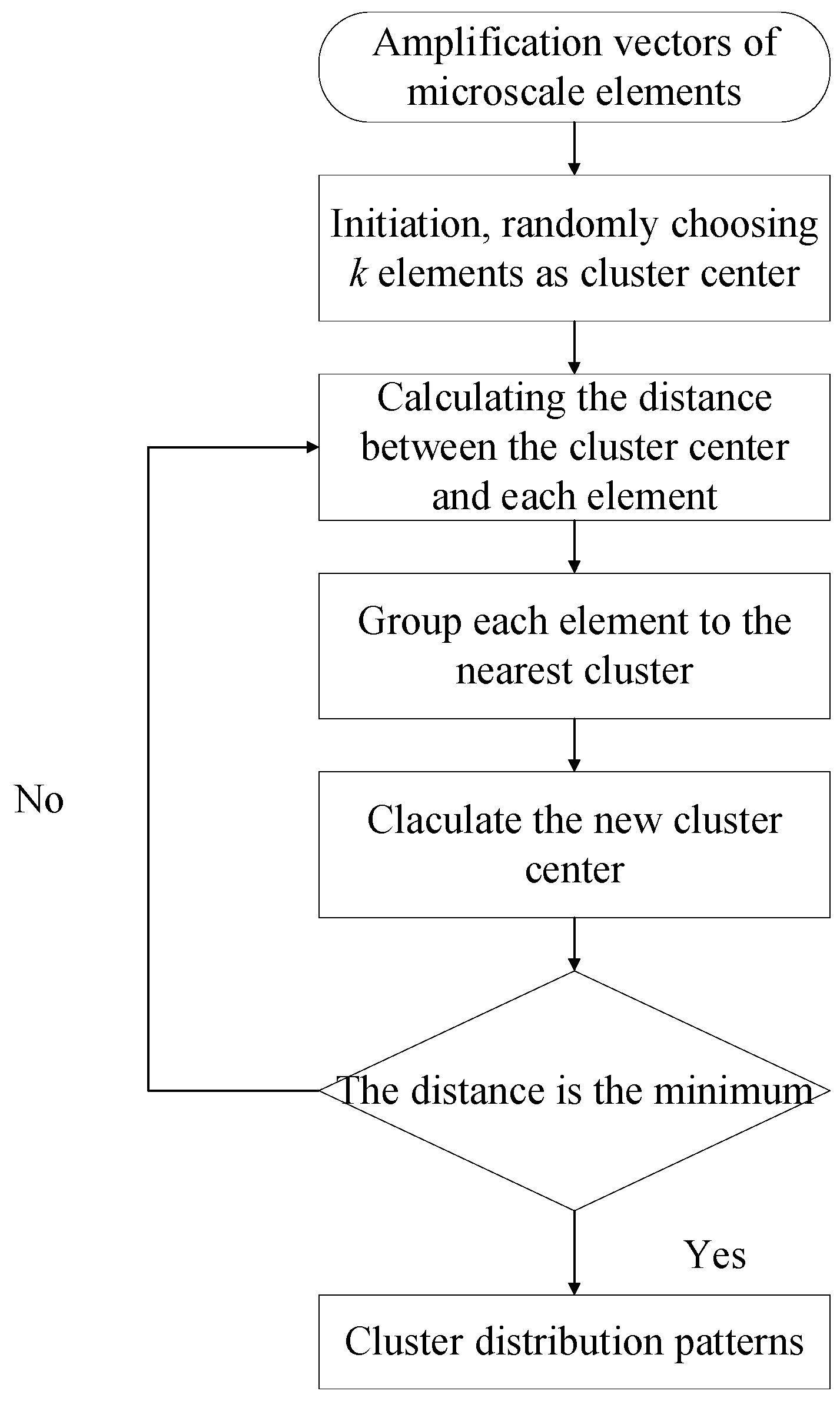
3.2. Extraction of Strain–Stress Amplification Factors with Clustering Analysis
3.3. Failure Criteria of the Constituents
3.4. Damage Model Derivation
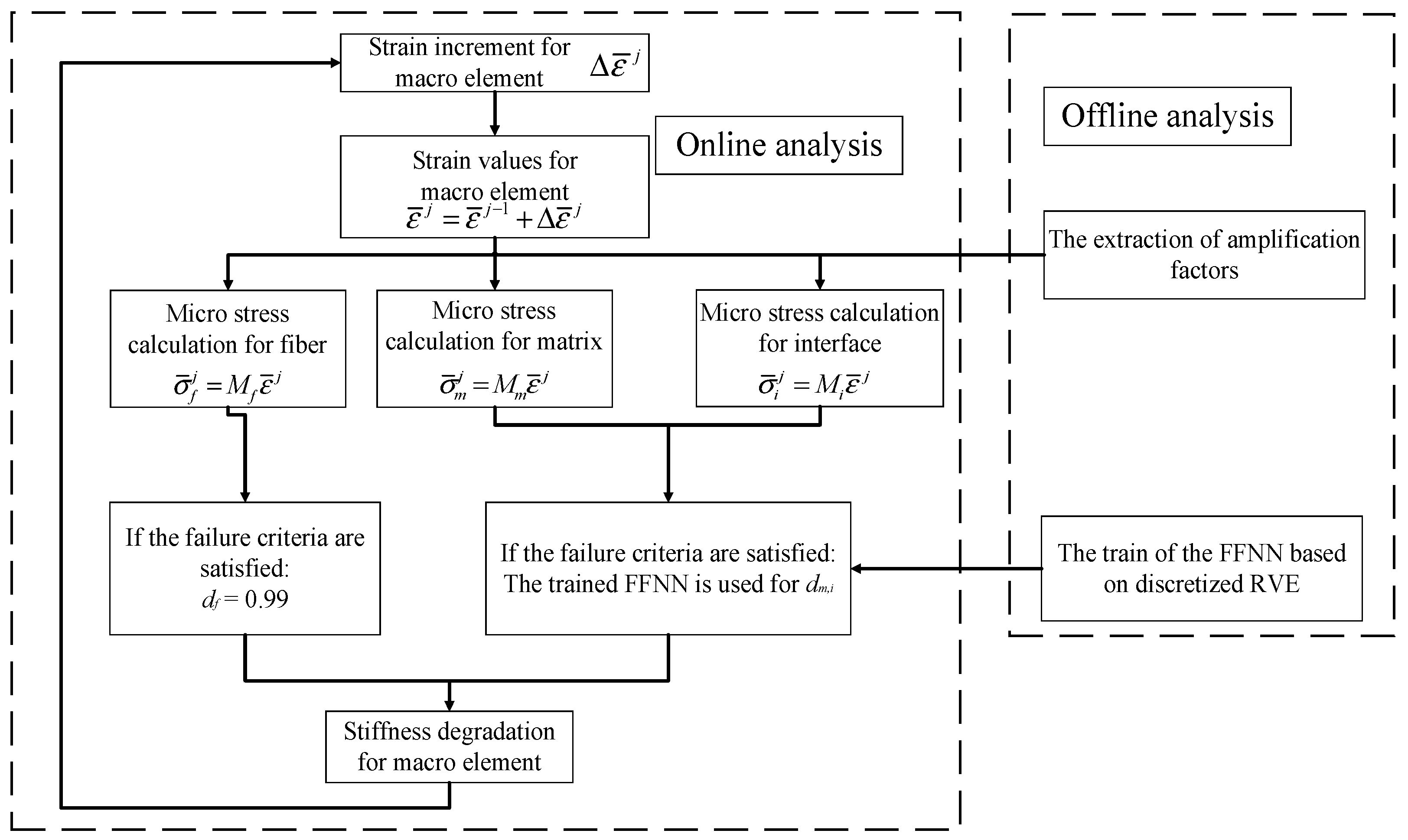
3.5. Modified Multiscale Numerical Implementation
4. Results and Discussion
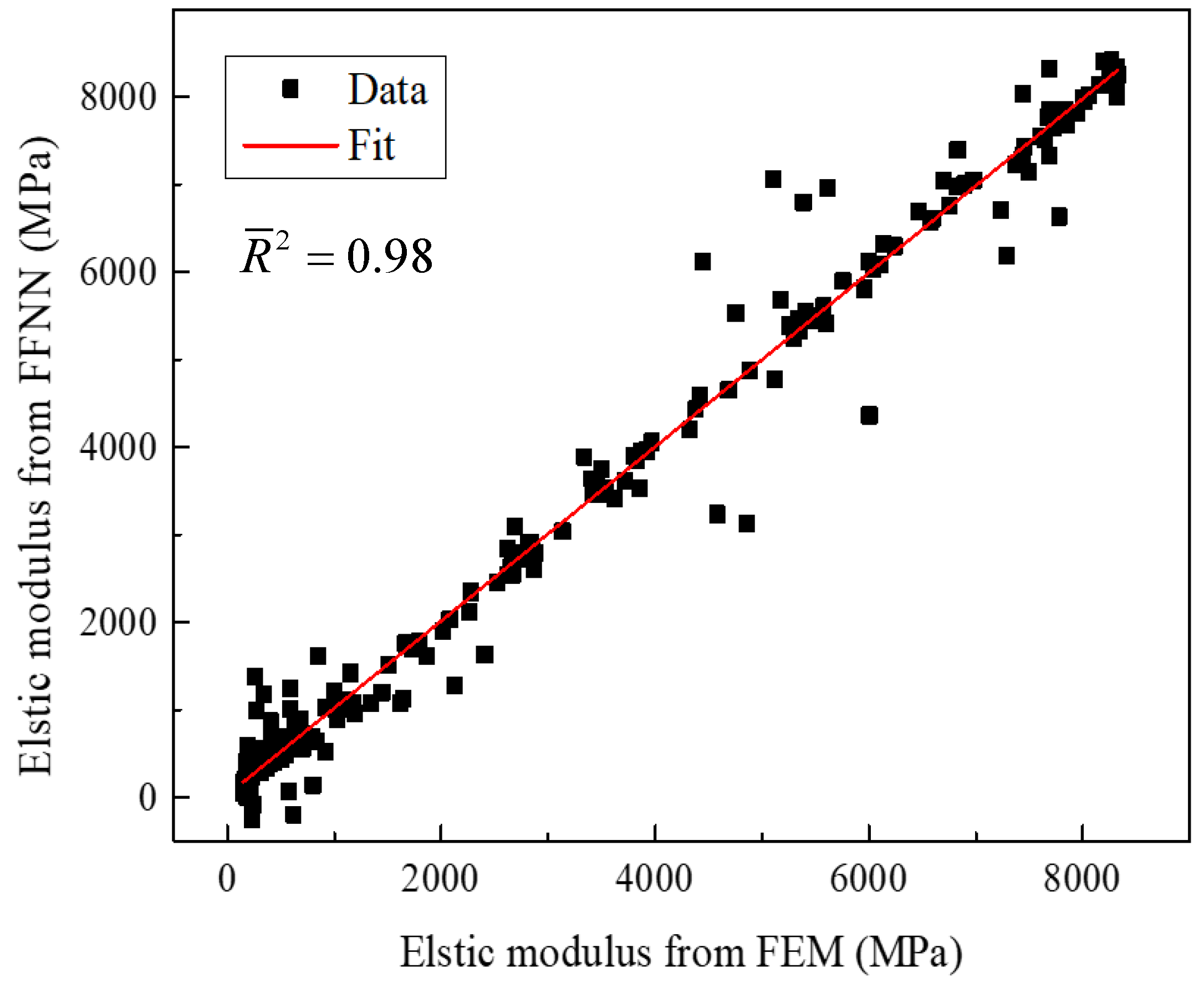
4.1. Offline Analysis
4.2. Online Analysis
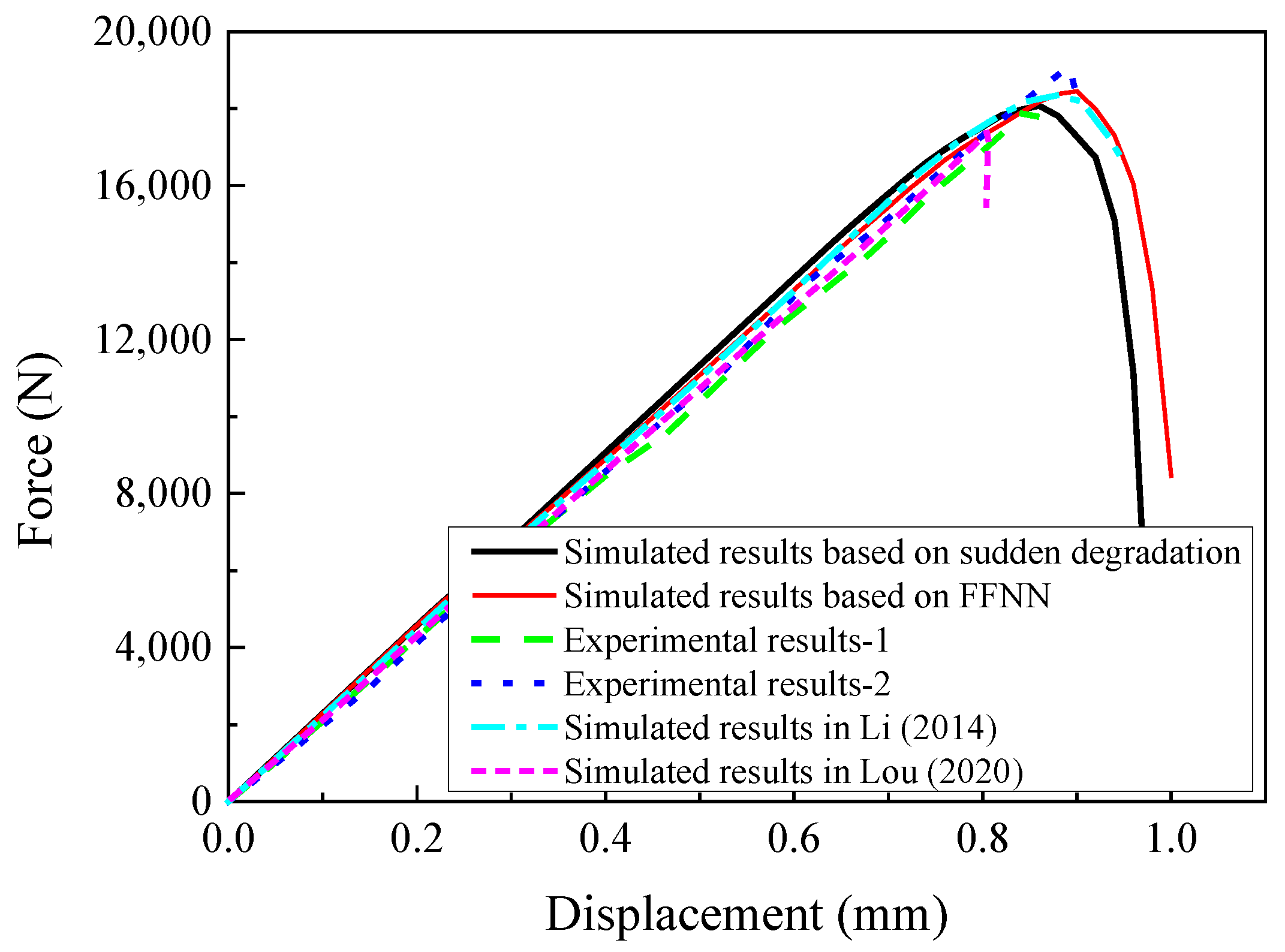
4.2.1. Model Validation
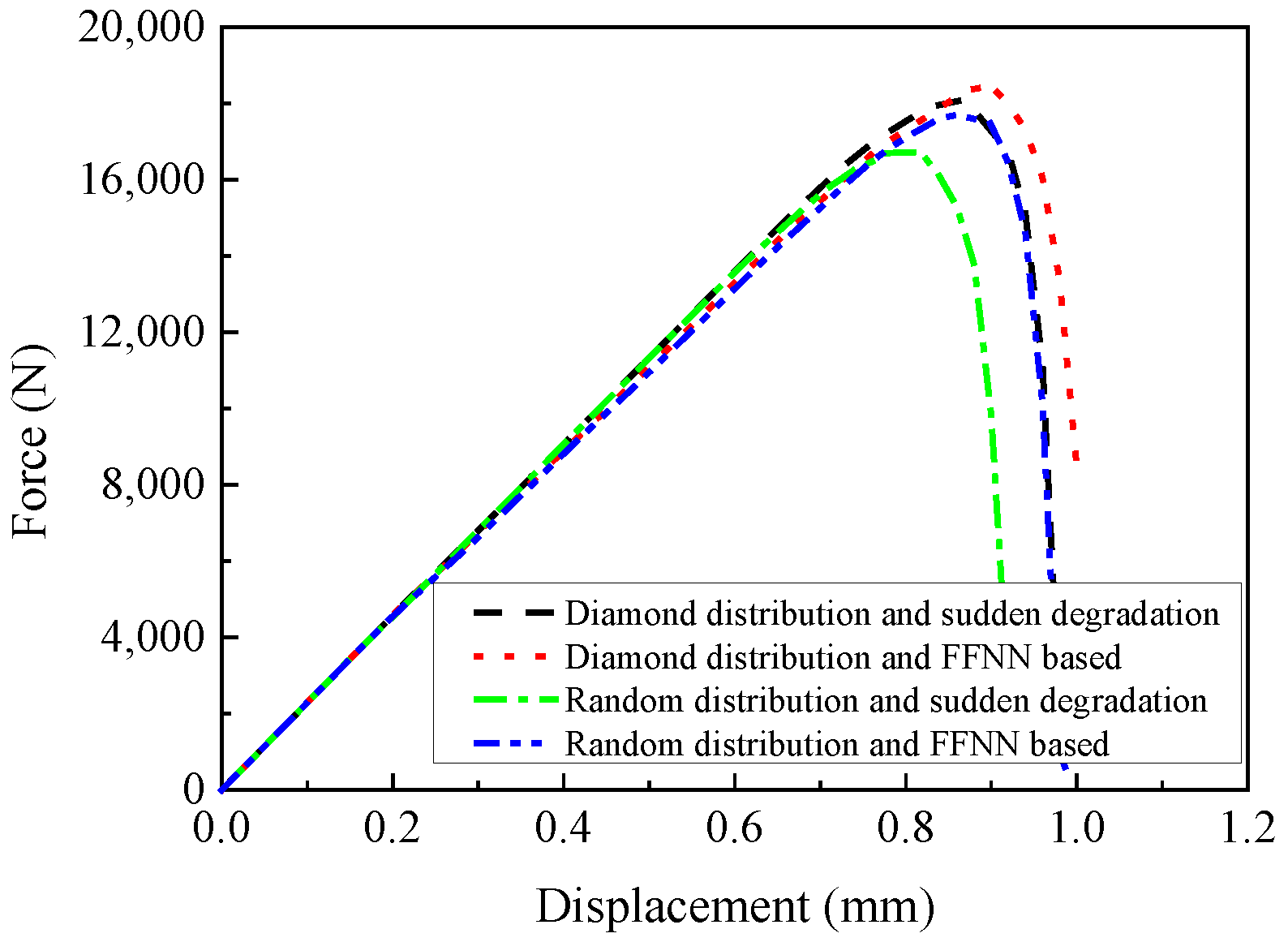
4.2.2. Effects of Degradation Models
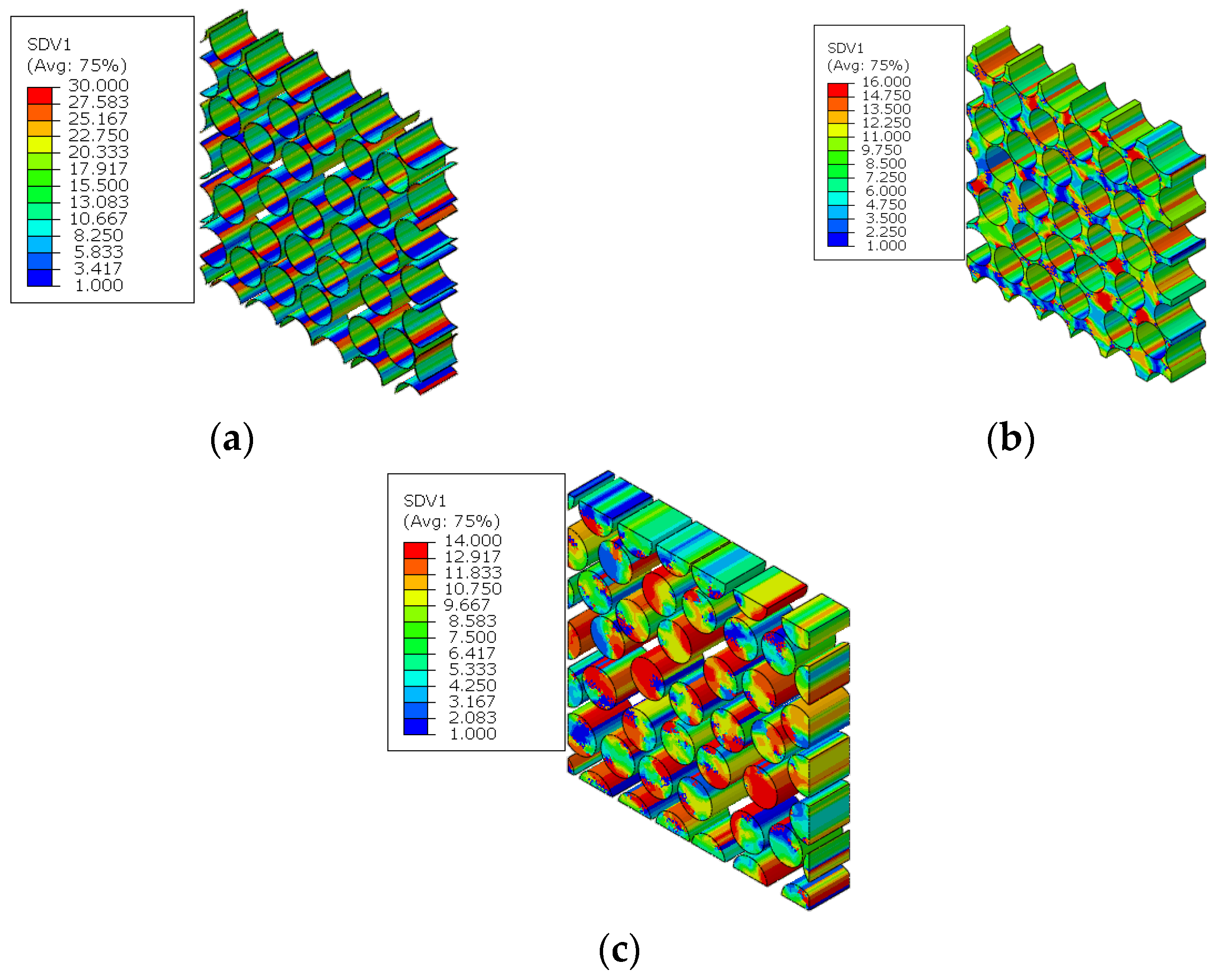
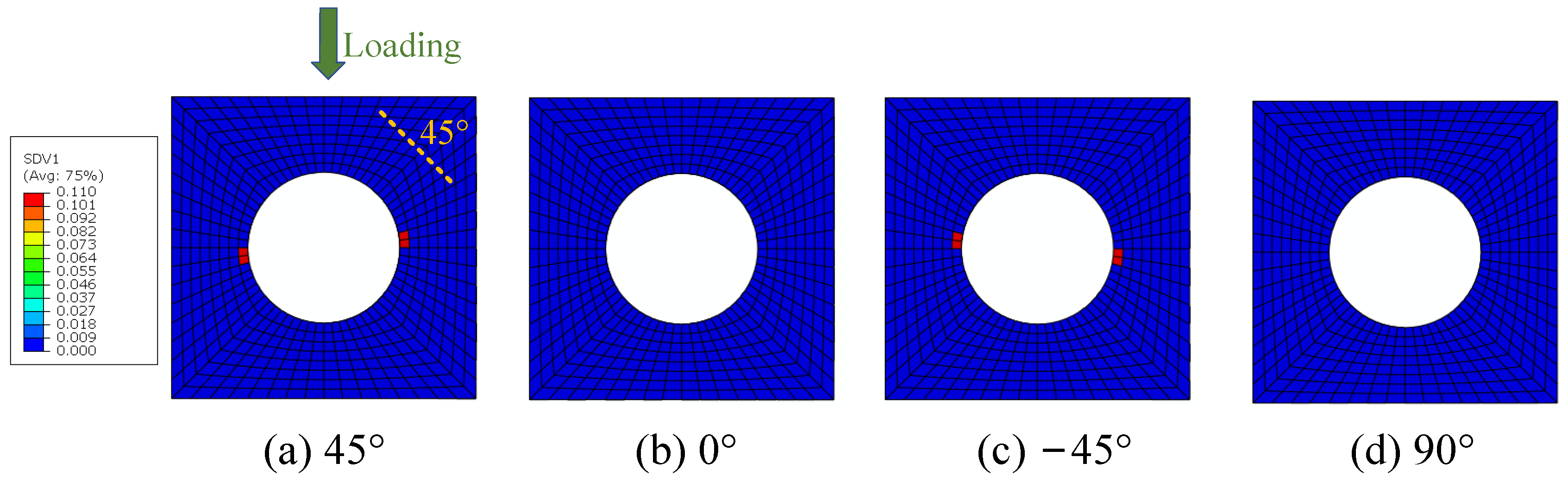
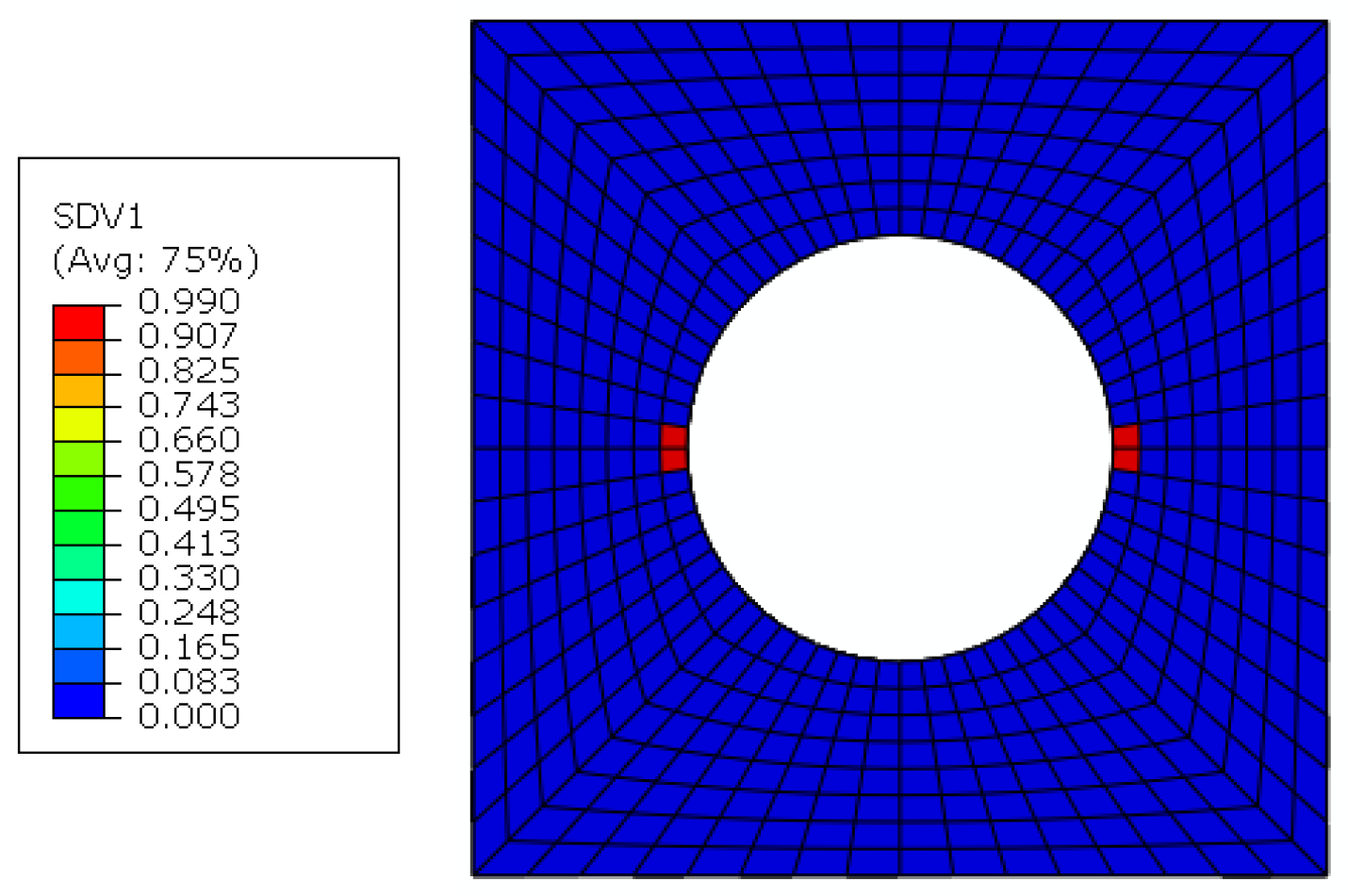
4.2.3. Effects of Fiber Distribution Patterns
5. Conclusions
- the predicted results are compared with the published experimental and numerical results first and the agreements between them demonstrate the effectiveness of the modified multiscale method.
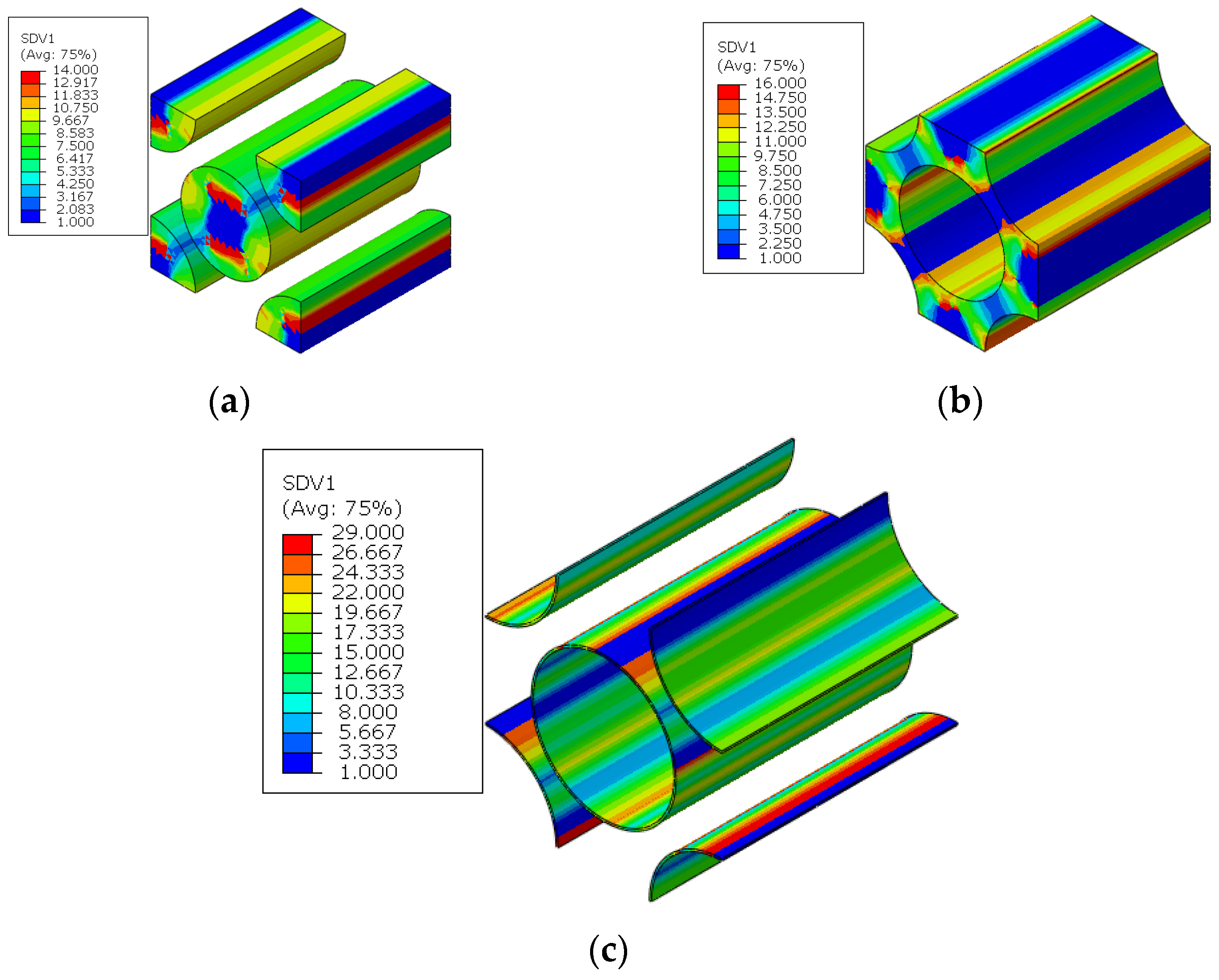
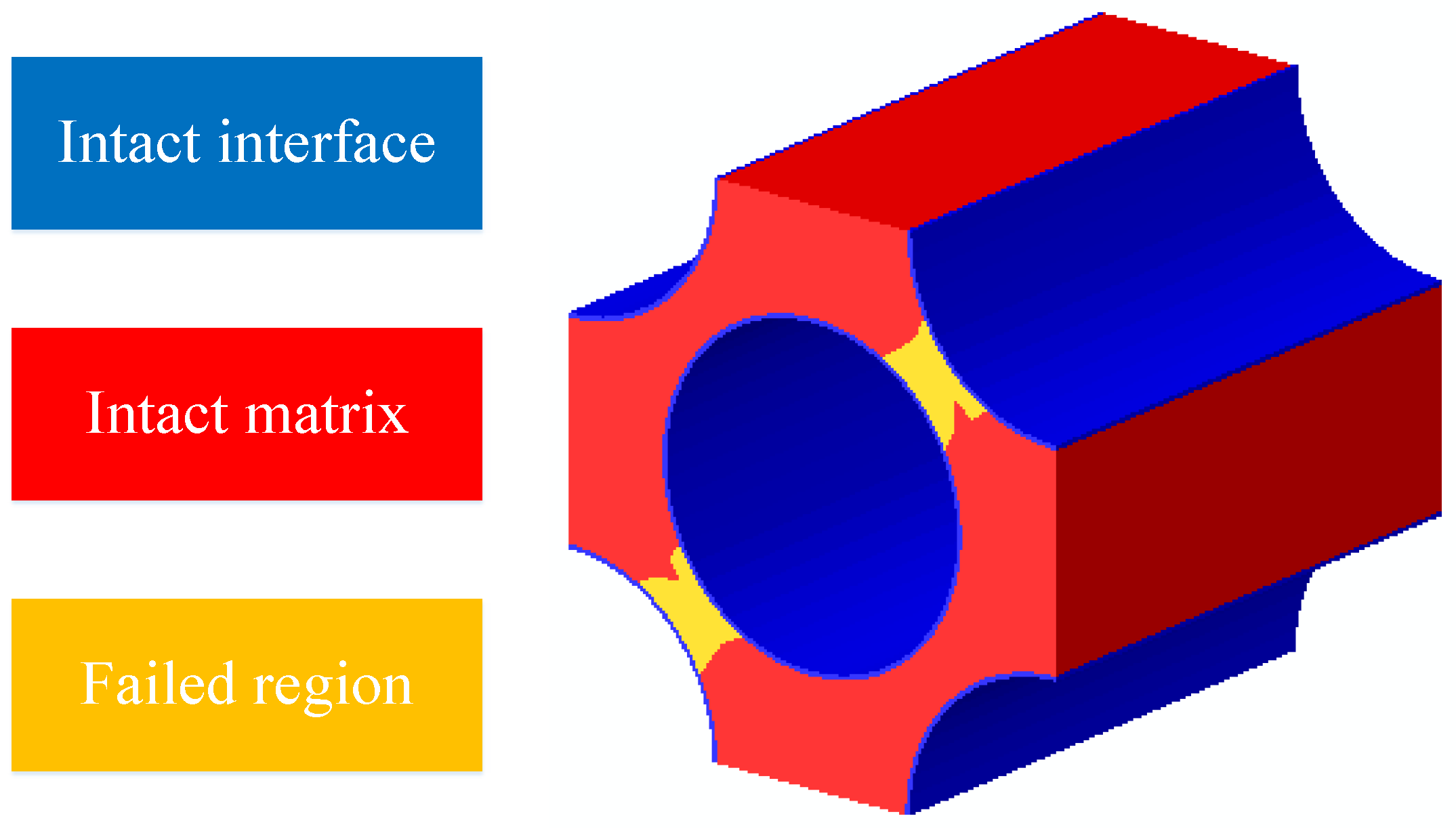
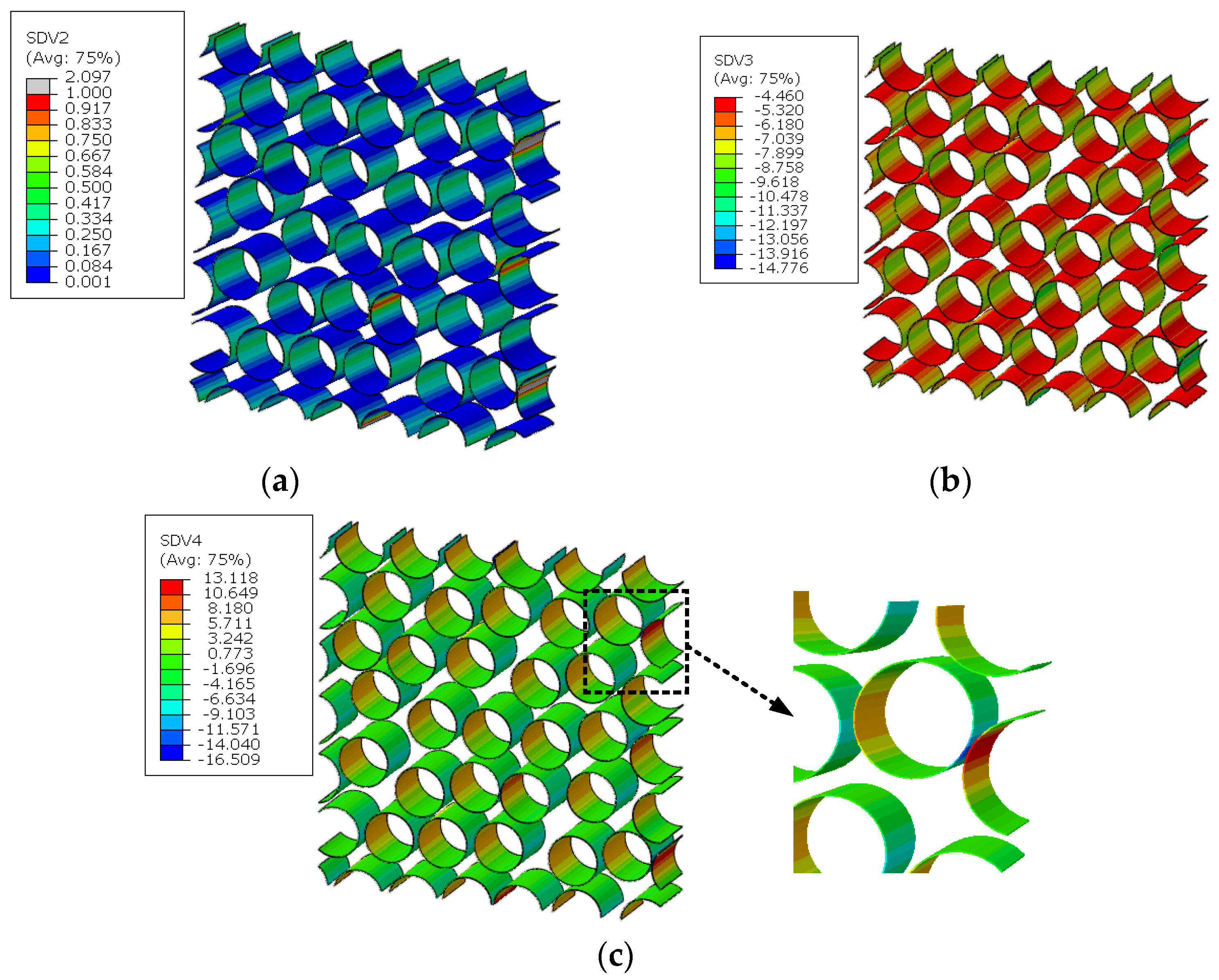
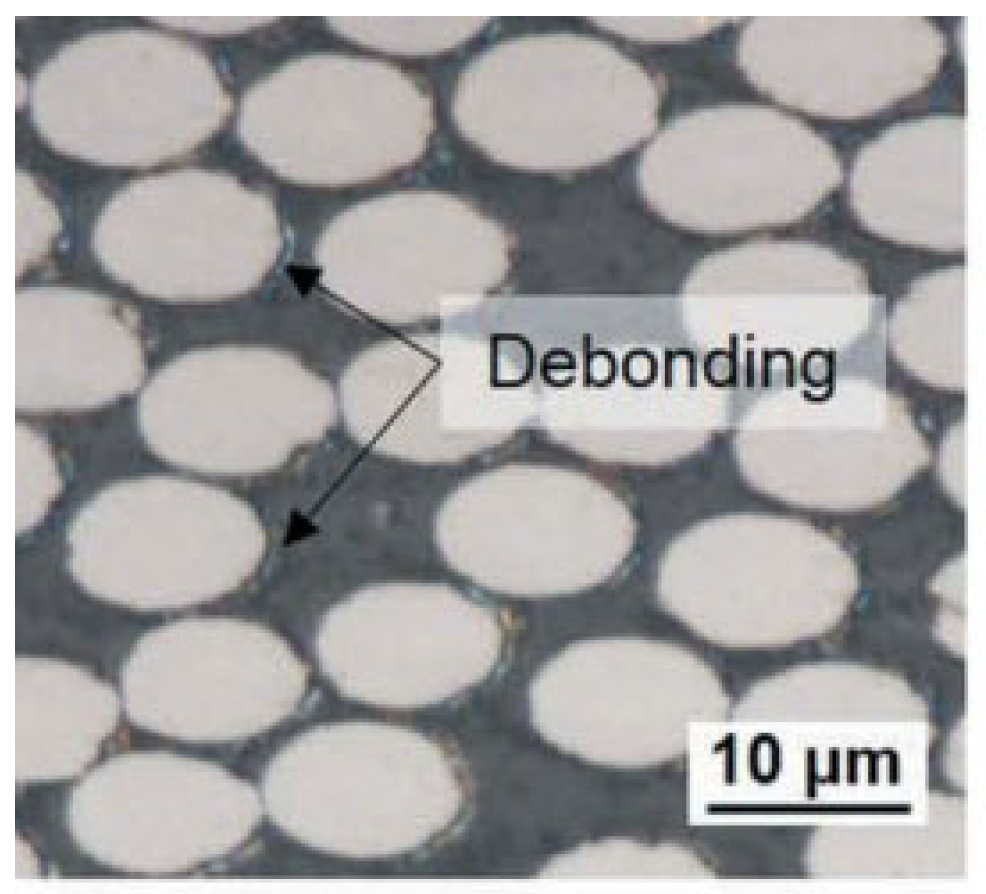
- through comparing the results obtained with and without considering interface debonding, it is found that considering interface results in the premature damage initiation in the laminates.
- from the results analyzed from the model considering both the fiber random distribution pattern and interface, it is found that, due to the smaller inter-fiber distance, the interface debonding and matrix damage initiate much earlier, which contributes to the decrease in the predicted compressive strength.
- with the multiscale method developed in this study, both the macroscale and the microscale damage process can be obtained. It is found that, at the microscale, the damage in the fiber random distribution model initiated at a local position rather than uniformly in the fiber diamond distribution model. Besides, the displacement loading increment for the formation of microscale crack in the fiber random distribution model is larger than that in the fiber diamond distribution model.
- as the progressive damage process of the microscale RVE is considered in the FFNN degradation method for determining the transverse damage value, the predicted strength values based on the FFNN are larger than those obtained based on the sudden degradation method.
- the difference induced by different degradation rules in the strength values from the models considering the fiber random distribution pattern is much larger than that from the models considering fiber diamond distribution pattern, which should be attributed to the non-uniform damage distribution at the microscale.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Suemasu, H.; Takahashi, H.; Ishikawa, T. On failure mechanisms of composite laminates with an open hole subjected to compressive load. Compos. Sci. Technol. 2006, 66, 634–641. [Google Scholar] [CrossRef]
- Suemasu, H.; Naito, Y.; Gozu, K.; Aoki, Y. Damage initiation and growth in composite laminates during open hole compression tests. Adv. Compos. Mater. 2012, 21, 209–220. [Google Scholar] [CrossRef]
- Zhou, S.; Sun, Y.; Chen, B.; Tay, T.E. Progressive damage simulation of open-hole composite laminates under compression based on different failure criteria. J. Compos. Mater. 2017, 51, 1239–1251. [Google Scholar] [CrossRef]
- Shimizu, S.; Sato, M.; Koyanagi, J.; Suemasu, H.; Kogo, Y. Numerical simulation of compressive failure of carbon-fiber-reinforced plastic laminates with various hole shapes. Adv. Compos. Mater. 2021, 30, 58–75. [Google Scholar] [CrossRef]
- Zhang, D.; Zheng, X.; Wu, T. Damage characteristics of open-hole laminated composites subjected to longitudinal loads. Compos. Struct. 2019, 230, 111474. [Google Scholar] [CrossRef]
- Zhao, L.; Li, Y.; Zhang, J.; Zhou, L.; Hu, N. A novel material degradation model for unidirectional CFRP composites. Compos. Part B. Eng. 2018, 135, 84–94. [Google Scholar] [CrossRef]
- Khedkar, S.; Chinthapenta, V.; Madhavan, M.; Ramji, M. Progressive failure analysis of CFRP laminate with interacting holes under compressive loading. J. Compos. Mater. 2015, 49, 3263–3283. [Google Scholar] [CrossRef]
- Zhang, H.; Shan, Y.; Guo, J.; Wen, W.; Cui, H. Experiments and simulations on the strength of open-hole composite laminates at different temperatures. Mech. Adv. Mater. Struct. 2019, 26, 1997–2008. [Google Scholar] [CrossRef]
- Su, Z.C.; Tay, T.E.; Ridha, M.; Chen, B.Y. Progressive damage modeling of open-hole composite laminates under compression. Compos. Struct. 2015, 122, 507–517. [Google Scholar] [CrossRef]
- Zhou, S.; Zhang, J.; Sun, Y.; Tian, K. Experimental and numerical investigation of open hole carbon fiber composite laminates under compression with three different stacking sequences. J. Mater. Res. Technol. 2019, 8, 2957–2968. [Google Scholar] [CrossRef]
- Zhou, S.; Sun, Y.; Muhammad, R.; Chen, B.; Tay, T.E. Progressive damage simulation of scaling effects on open-hole composite laminates under compression. J. Reinf. Plast. Compos. 2017, 36, 1369–1383. [Google Scholar] [CrossRef]
- Li, X.; Guan, Z.; Li, Z.; Liu, L. A new stress-based multi-scale failure criterion of composites and its validation in open hole tension tests. Chin. J. Aeronaut. 2014, 27, 1430–1441. [Google Scholar] [CrossRef] [Green Version]
- Xu, L.; Huang, Y.C.; Zhao, C.; Ha, S.K. Progressive failure prediction of woven fabric composites using a multi-scale approach. Int. J. Damage Mech. 2018, 27, 97–119. [Google Scholar] [CrossRef]
- Li, W.; Cai, H.; Li, C.; Wang, K.; Fang, L. Progressive failure of laminated composites with a hole under compressive loading based on micro-mechanics. Adv. Compos. Mater. 2014, 23, 477–490. [Google Scholar] [CrossRef]
- Liu, Z.; Guan, Z.; Tan, R.; Xu, J. Analysis of open-hole compressive CFRP laminates at various temperatures based on a multiscale strategy. Appl. Compos. Mater. 2019, 26, 923–944. [Google Scholar] [CrossRef]
- Lou, X.; Han, X.; Cai, H. A high-efficient model for interface debonding analysis of carbon fiber-reinforced polymer composite. Compos. Interfaces 2020, 27, 87–109. [Google Scholar] [CrossRef]
- Wang, M. A Multiscale Method Across Three Length Scales for Progressive Damage Analysis of Plain Woven Composites. Appl. Compos. Mater. 2021, 28, 1919–1944. [Google Scholar] [CrossRef]
- Wang, M.; Zhang, P.; Fei, Q.; Guo, F. Modified micro-mechanics based multiscale model for progressive failure prediction of 2D twill woven composites. Chin. J. Aeronaut. 2020, 33, 2070–2087. [Google Scholar] [CrossRef]
- Gu, Y.; Li, M.; Wang, J.; Zhang, Z. Characterization of the interphase in carbon fiber/polymer composites using a nanoscale dynamic mechanical imaging technique. Carbon 2010, 48, 3229–3235. [Google Scholar] [CrossRef]
- Wang, B.; Fang, G.; Liu, S.; Liang, J. Effect of heterogeneous interphase on the mechanical properties of unidirectional fiber composites studied by FFT-based method. Compos. Struct. 2019, 220, 642–651. [Google Scholar] [CrossRef]
- Elnekhaily, S.A.; Talreja, R. Damage initiation in unidirectional fiber composites with different degrees of nonuniform fiber distribution. Compos. Sci. Technol. 2018, 155, 22–32. [Google Scholar] [CrossRef]
- Borkowski, L.B.; Liu, K.C.; Chattopadhyay, A. From ordered to disordered: The effect of microstructure on composite mechanical performance. Comput. Mater. Contin. 2013, 37, 161–193. [Google Scholar]
- Wang, M.; Zhang, P.; Fei, Q.; Guo, F. Computational evaluation of the effects of void on the transverse tensile strengths of unidirectional composites considering thermal residual stress. Compos. Struct. 2019, 227, 111287. [Google Scholar] [CrossRef]
- Bheemreddy, V.; Chandrashekhara, K.; Dharani, L.R.; Hilmas, G.E. Computational study of micromechanical damage behavior in continuous fiber-reinforced ceramic composites. J. Mater. Sci. 2016, 51, 8610–8624. [Google Scholar] [CrossRef]
- Wang, Z.; Wang, X.; Zhang, J.; Liang, W.; Zhou, L. Automatic generation of random distribution of fibers in long-fiber-reinforced composites and mesomechanical simulation. Mater. Des. 2011, 32, 885–891. [Google Scholar] [CrossRef]
- Jin, K.K.; Huang, Y.; Lee, Y.H.; Ha, S.K. Distribution of micro stresses and interfacial tractions in unidirectional composites. J. Compos. Mater. 2008, 42, 1825–1849. [Google Scholar] [CrossRef]
- Liu, Z.; Bessa, M.A.; Liu, W.K. Self-consistent clustering analysis: An efficient multi-scale scheme for inelastic heterogeneous materials. Comput. Methods Appl. Mech. Eng. 2016, 306, 319–341. [Google Scholar] [CrossRef]
- Liu, Z.; Fleming, M.; Liu, W.K. Microstructural material database for self-consistent clustering analysis of elastoplastic strain softening materials. Comput. Methods Appl. Mech. Eng. 2018, 330, 547–577. [Google Scholar] [CrossRef]
- Han, X.; Gao, J.; Fleming, M.; Xu, C.; Xie, W.; Meng, S.; Liu, W.K. Efficient multiscale modeling for woven composites based on self-consistent clustering analysis. Comput. Methods Appl. Mech. Eng. 2020, 364, 112929. [Google Scholar] [CrossRef]
- Yan, S.; Zou, X.; Ilkhani, M.; Jones, A. An efficient multiscale surrogate modelling framework for composite materials considering progressive damage based on artificial neural networks. Compos. Part B. Eng. 2020, 194, 108014. [Google Scholar] [CrossRef]
- Zhang, Z.; Friedrich, K.; Velten, K. Prediction on tribological properties of short fibre composites using artificial neural networks. Wear 2002, 252, 668–675. [Google Scholar] [CrossRef]
- Zhang, Z.; Friedrich, K. Artificial neural networks applied to polymer composites: A review. Compos. Sci. Technol. 2003, 63, 2029–2044. [Google Scholar] [CrossRef]
- Lemaitre, J. A Course on Damage Mechanics; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Cybenko, G. Approximation by superpositions of a sigmoidal function. Math. Control. Signals Syst. 1989, 2, 303–314. [Google Scholar] [CrossRef]
- Hornik, K.; Stinchcombe, M.; White, H. Multilayer feedforward networks are universal approximators. Neural Netw. 1989, 2, 359–366. [Google Scholar] [CrossRef]
- Zhao, Z.; Wang, Z.; Yuan, J.; Ma, J.; He, Z.; Xu, Y.; Shen, X.; Zhu, L. Development of a novel feedforward neural network model based on controllable parameters for predicting effluent total nitrogen. Engineering 2021, 7, 195–202. [Google Scholar] [CrossRef]
- Li, W.; Cai, H.; Li, C. Static compressive strength prediction of open-hole structure based on non-linear shear behavior and micro-mechanics. Mech. Time-Depend. Mater. 2014, 18, 643–662. [Google Scholar] [CrossRef]





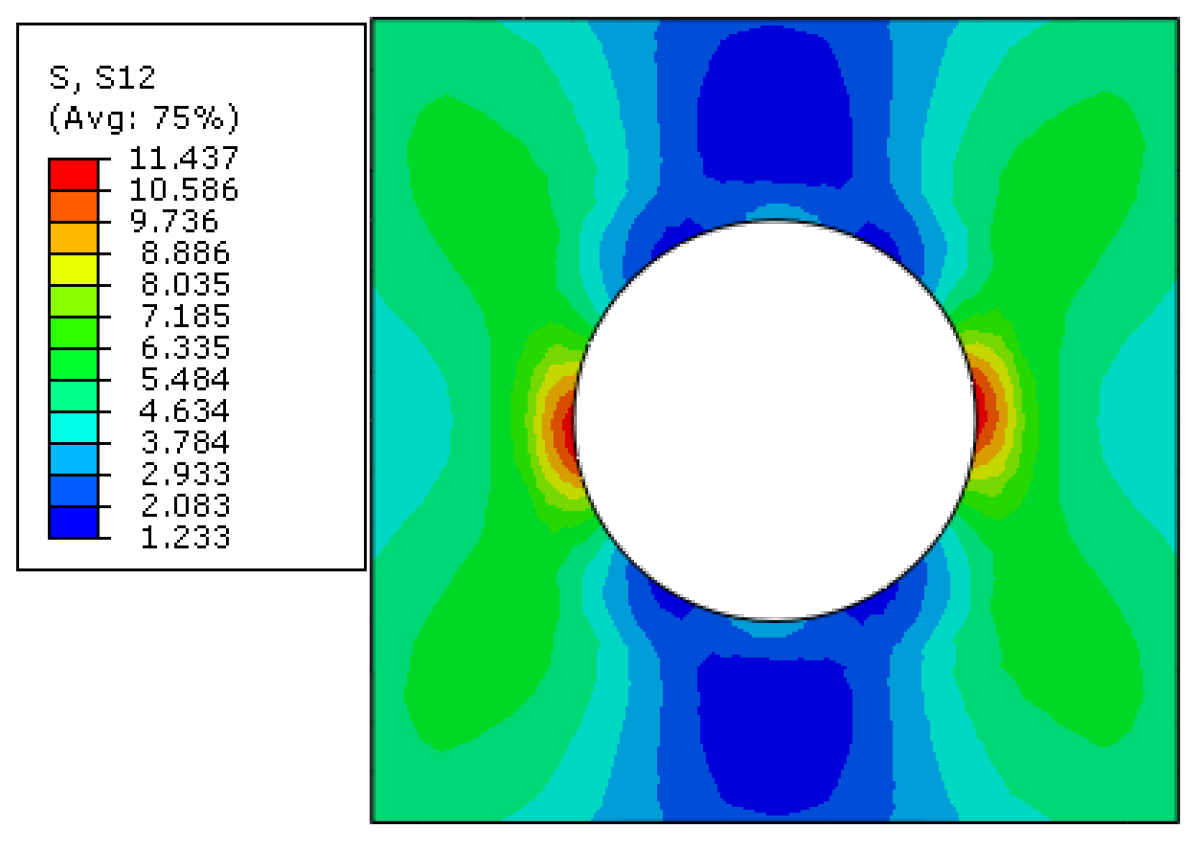
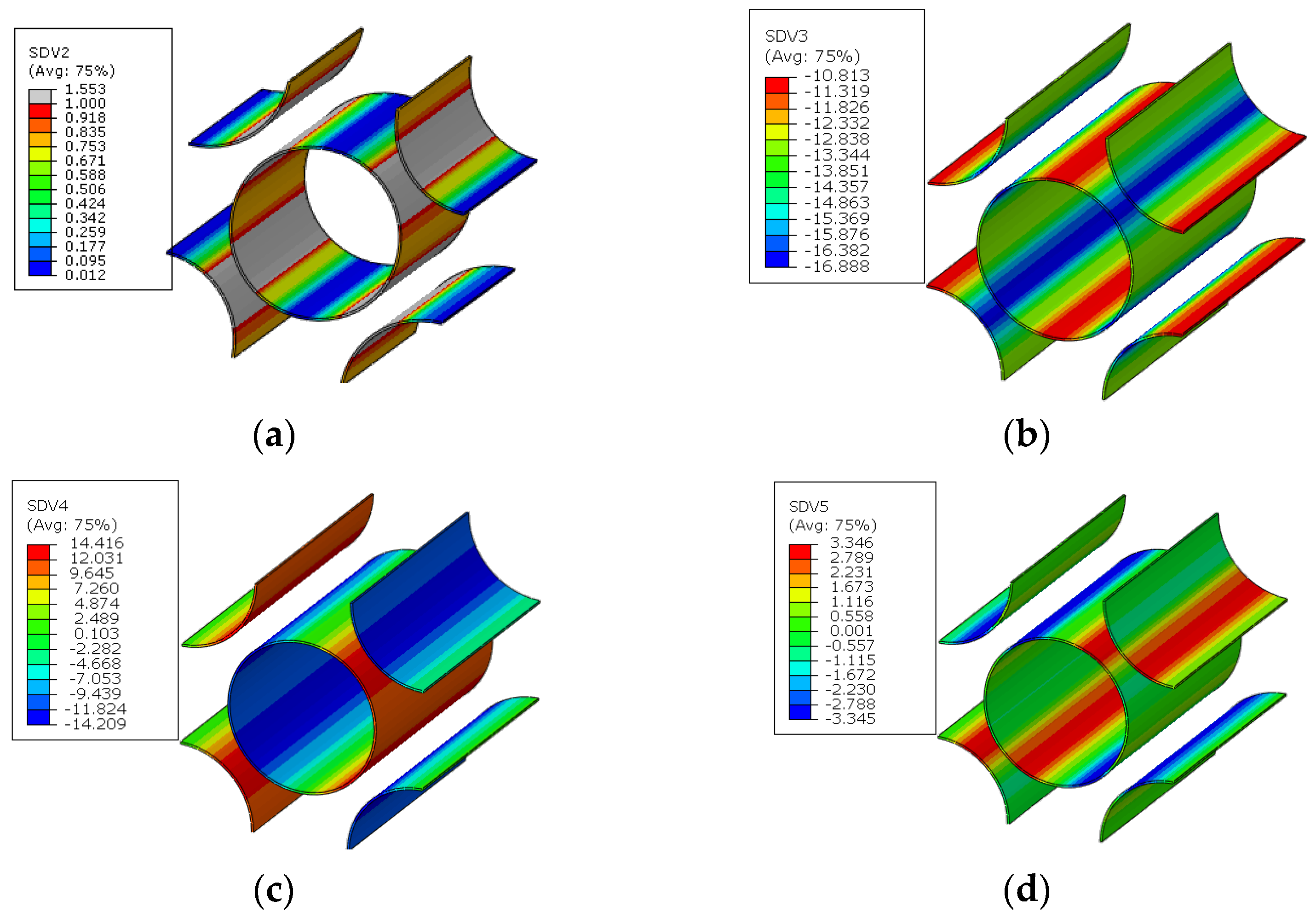

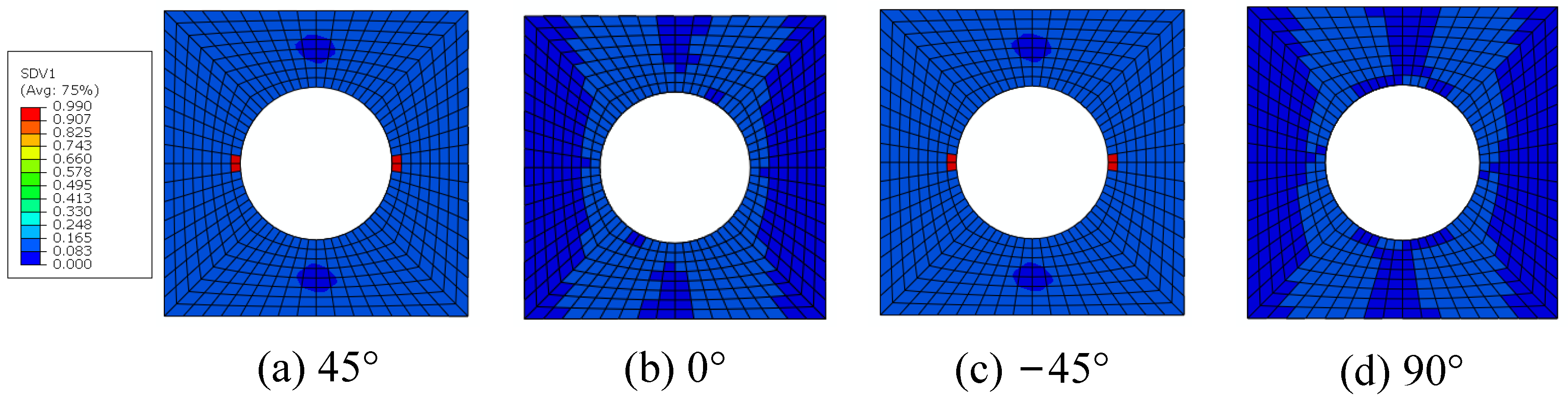

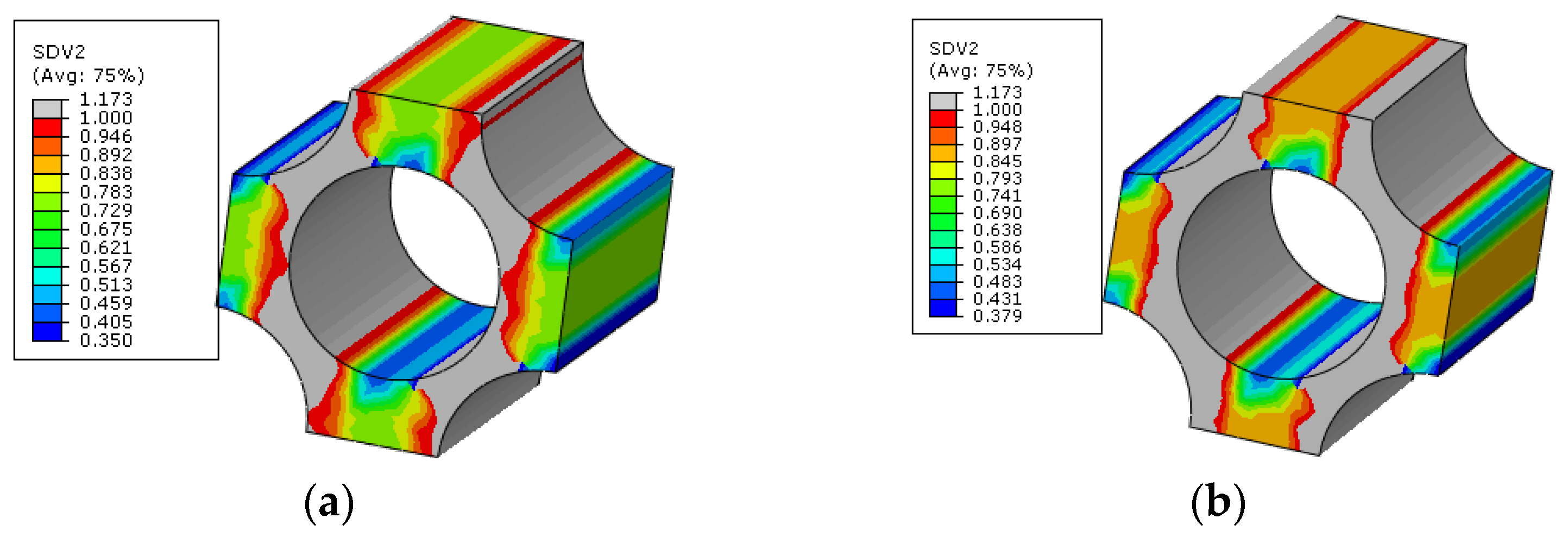
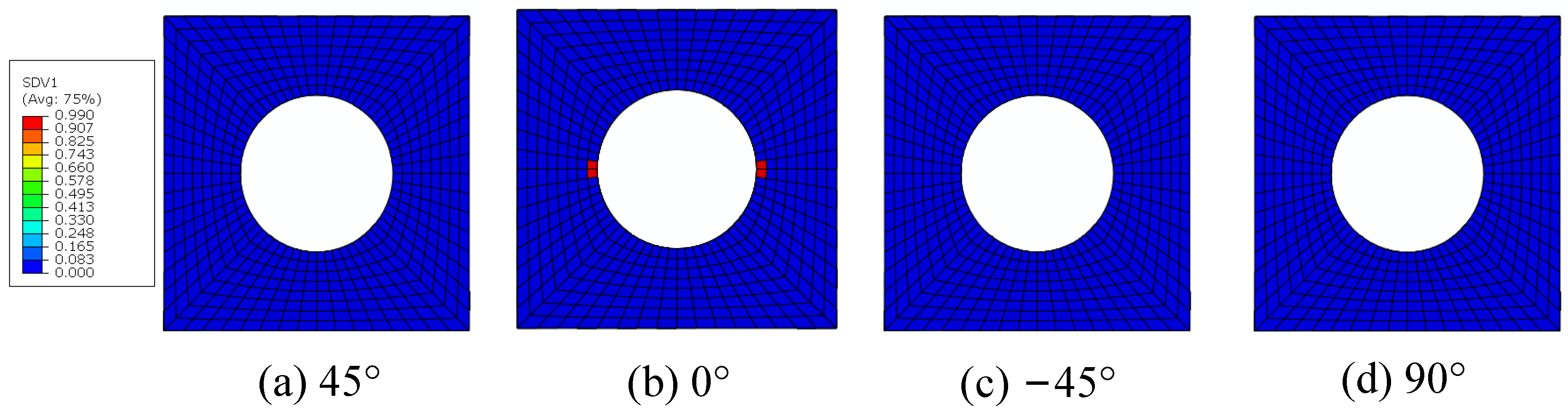
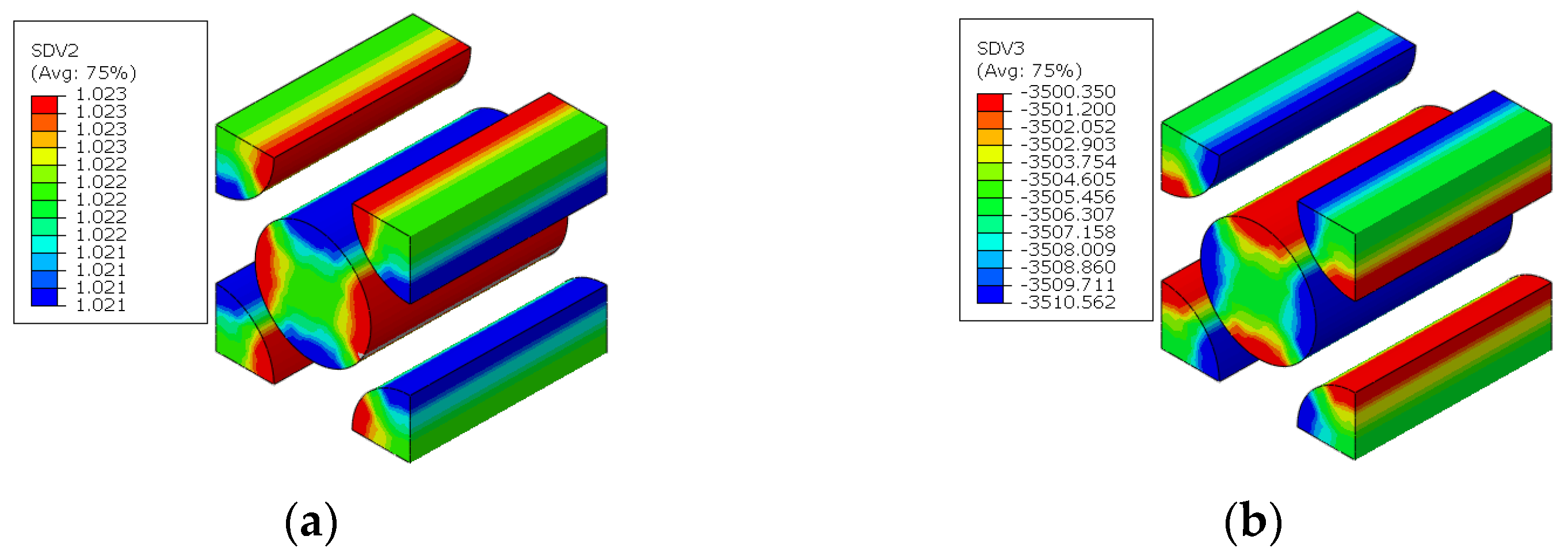
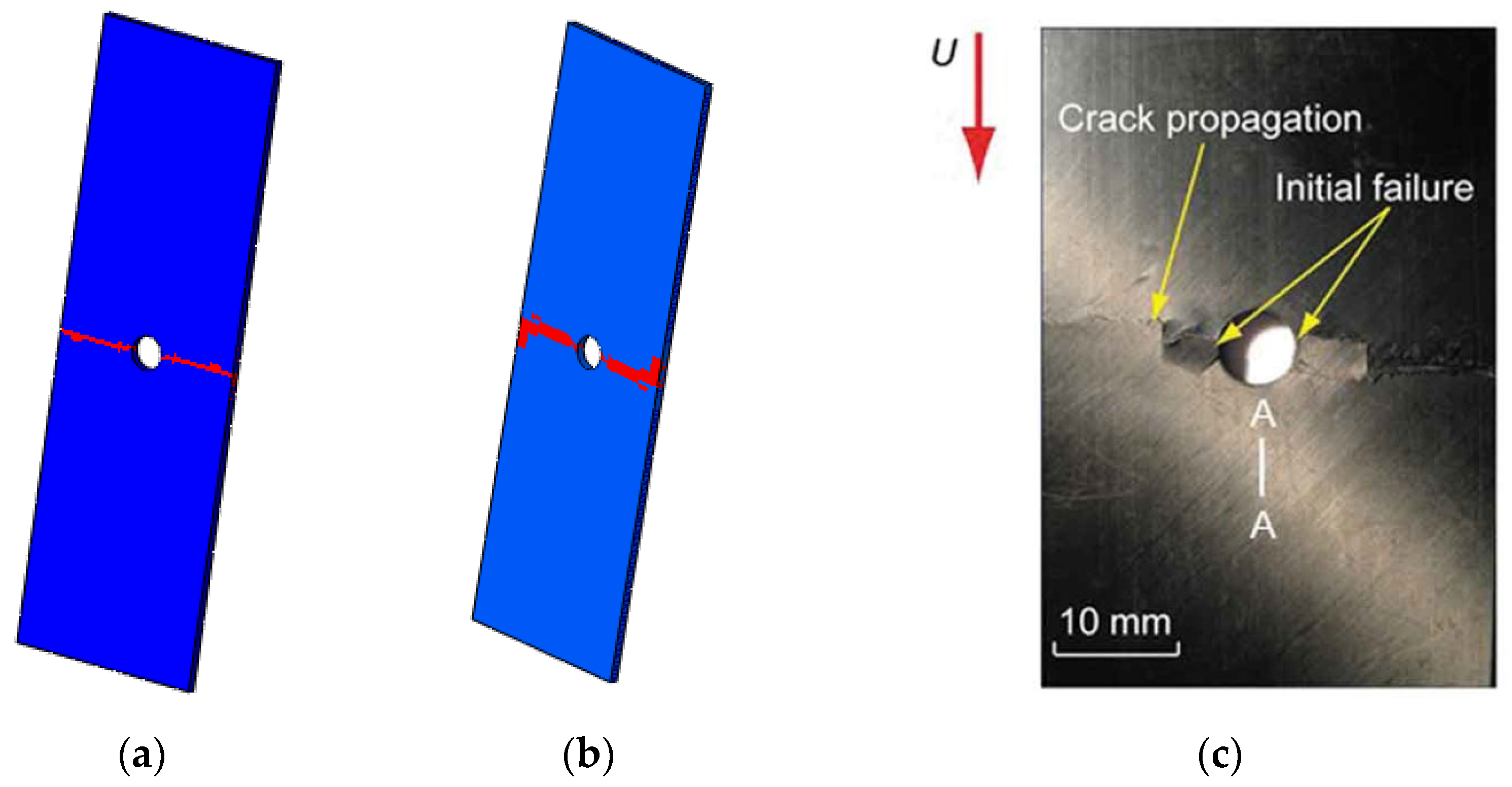

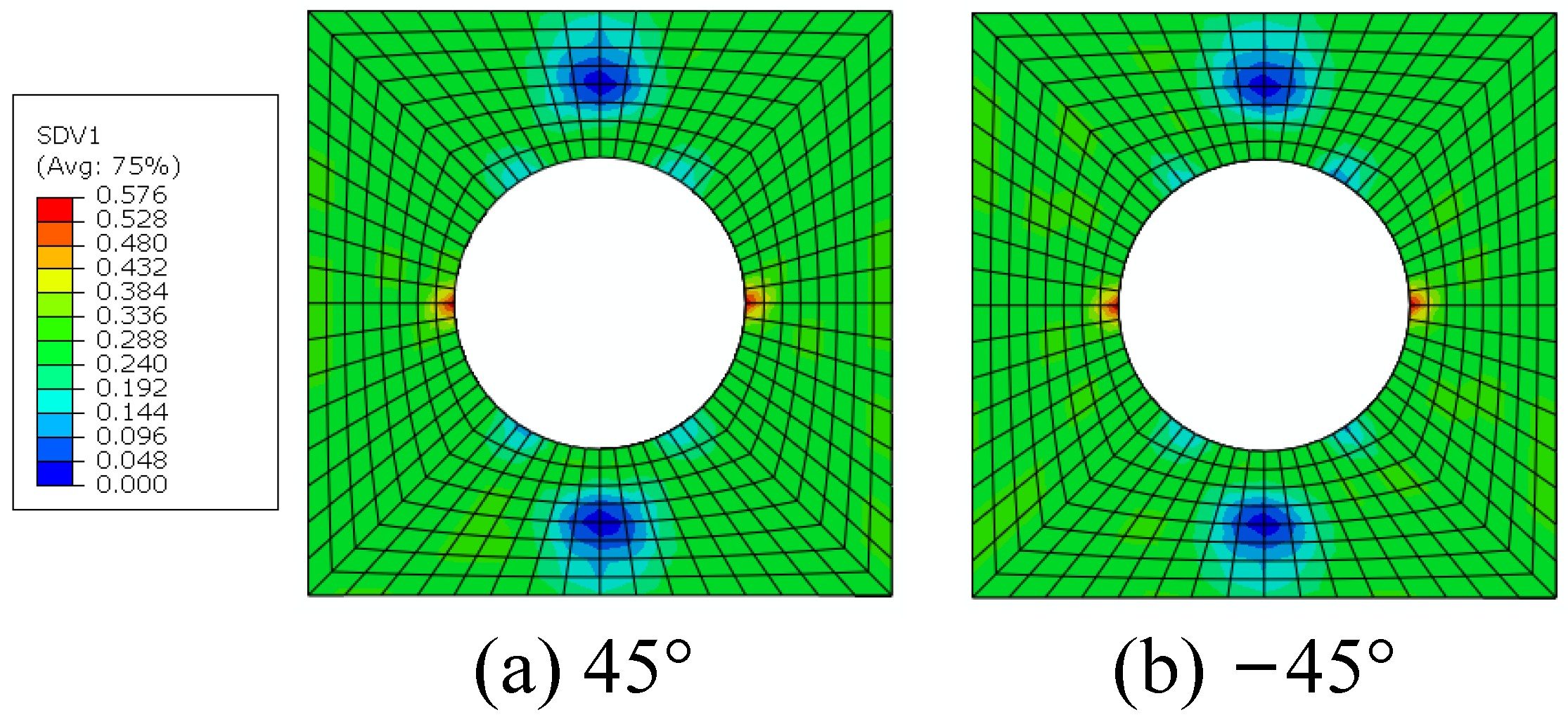
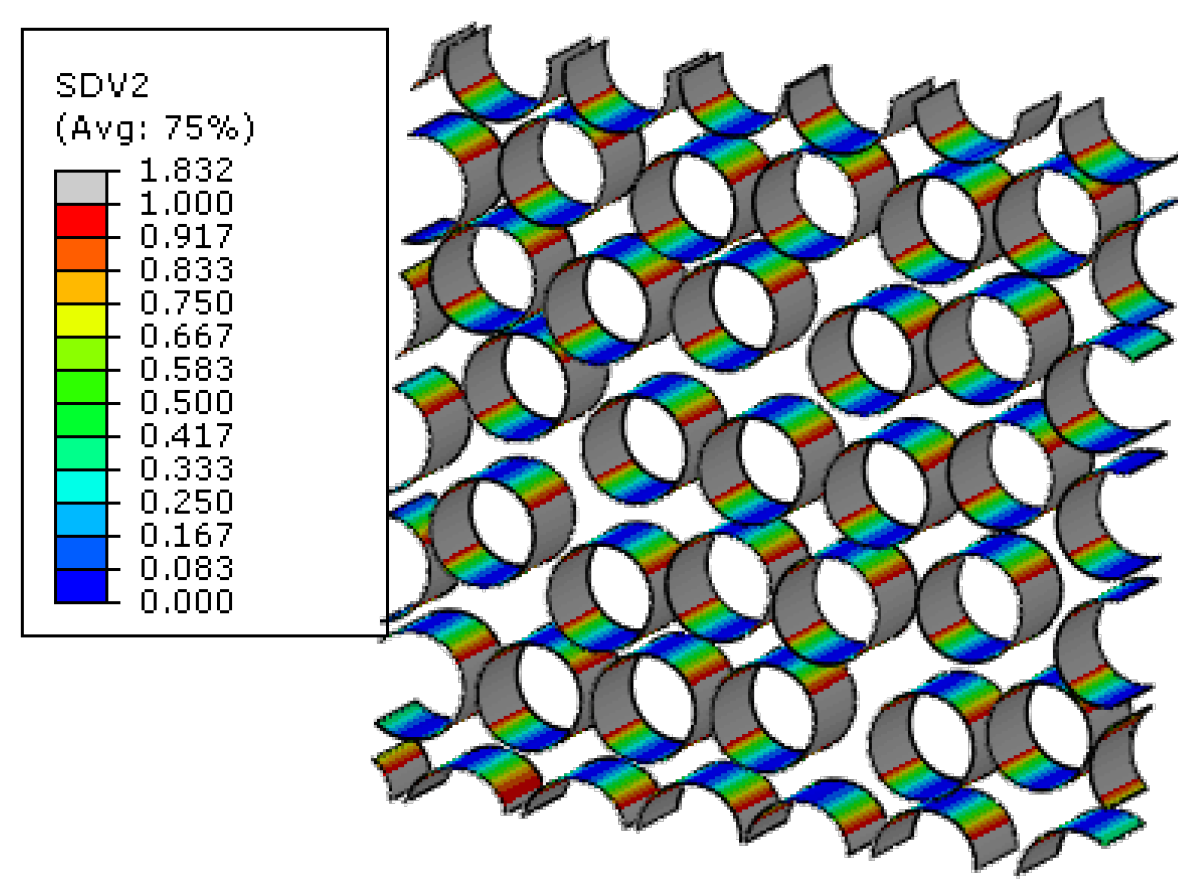


| Material Parameters | E11 (Gpa) | E22 = E33 (GPa) | G12 = G13 (GPa) | G23 (GPa) | v12 = v13 | v23 | Vf |
|---|---|---|---|---|---|---|---|
| Ply | 136 | 10 | 4.7 | 3.2 | 0.35 | 0.56 | 0.56 |
| Fiber | 240 | 42 | 23 | 12 | 0.33 | 0.71 | |
| Interface | 15.9 | 15.9 | 5.76 | 5.76 | 0.38 | 0.38 | |
| Matrix | 3 | 3 | 1.087 | 1.087 | 0.38 | 0.38 |
| Strength Parameters (MPa) | Tf | Cf | Tm | Cm | N | S |
|---|---|---|---|---|---|---|
| Values | 3710 | 3430 | 155 | 207 | 18 | 11.4 |
| Samples | RMSE (MPa) | R2 |
|---|---|---|
| Training | 131.9 | 0.999 |
| Validation | 623.9 | 0.977 |
| Testing | 808.5 | 0.964 |
| Samples | RMSE (MPa) | R2 |
|---|---|---|
| Training | 304.7 | 0.996 |
| Validation | 979.1 | 0.967 |
| Testing | 718.8 | 0.978 |
| Model | Average Experimental Value | In Ref. [16] | In Ref. [14] | With Sudden Degradation Model | With FFNN Model |
|---|---|---|---|---|---|
| Strength (KN) | 18.6 | 17.1 | 18.4 | 18.0 | 18.5 |
| Difference percentage (%) | — | −8.065 | −1.075 | −3.226 | −0.538 |
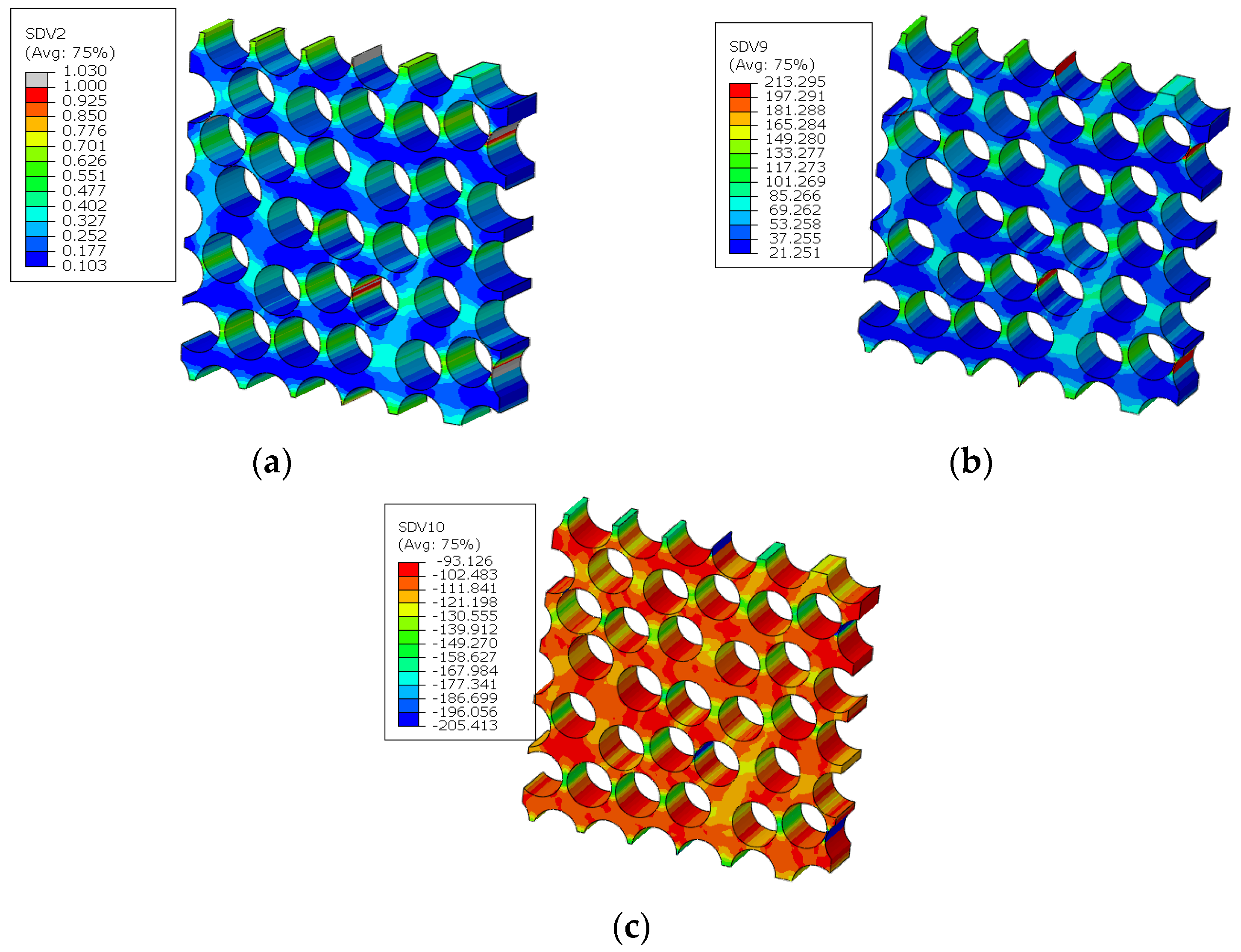
| Models | Interface Debonding | Matrix Damage | Fiber Breakage |
|---|---|---|---|
| Fiber diamond distribution | 0.06 mm | 0.64 mm | 0.62 mm |
| Fiber random distribution | 0.04 mm | 0.34 mm | 0.58 mm |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, M.; Hang, X. Modified Micro-Mechanics Based Multiscale Model for Damage Analysis of Open-Hole Composite Laminates under Compression. Materials 2022, 15, 5105. https://doi.org/10.3390/ma15155105
Wang M, Hang X. Modified Micro-Mechanics Based Multiscale Model for Damage Analysis of Open-Hole Composite Laminates under Compression. Materials. 2022; 15(15):5105. https://doi.org/10.3390/ma15155105
Chicago/Turabian StyleWang, Meng, and Xiaochen Hang. 2022. "Modified Micro-Mechanics Based Multiscale Model for Damage Analysis of Open-Hole Composite Laminates under Compression" Materials 15, no. 15: 5105. https://doi.org/10.3390/ma15155105
APA StyleWang, M., & Hang, X. (2022). Modified Micro-Mechanics Based Multiscale Model for Damage Analysis of Open-Hole Composite Laminates under Compression. Materials, 15(15), 5105. https://doi.org/10.3390/ma15155105





