Study of the Electron Beam Melting Process Parameters’ Influence on the Tensile Behavior of 3D Printed Ti6Al4V ELI Alloy in Static and Dynamic Conditions
Abstract
1. Introduction
2. Materials and Methods
3. Results and Discussion
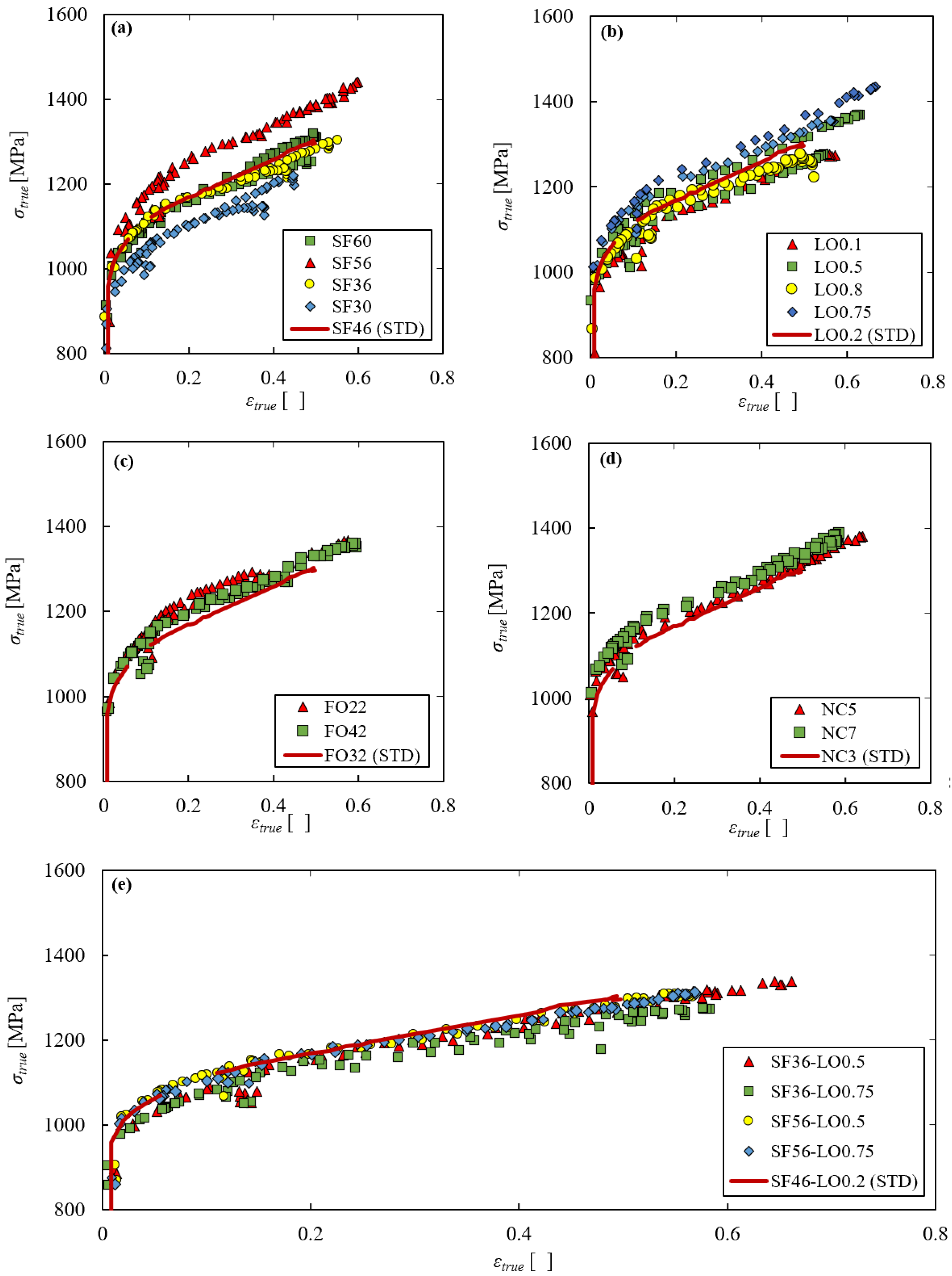
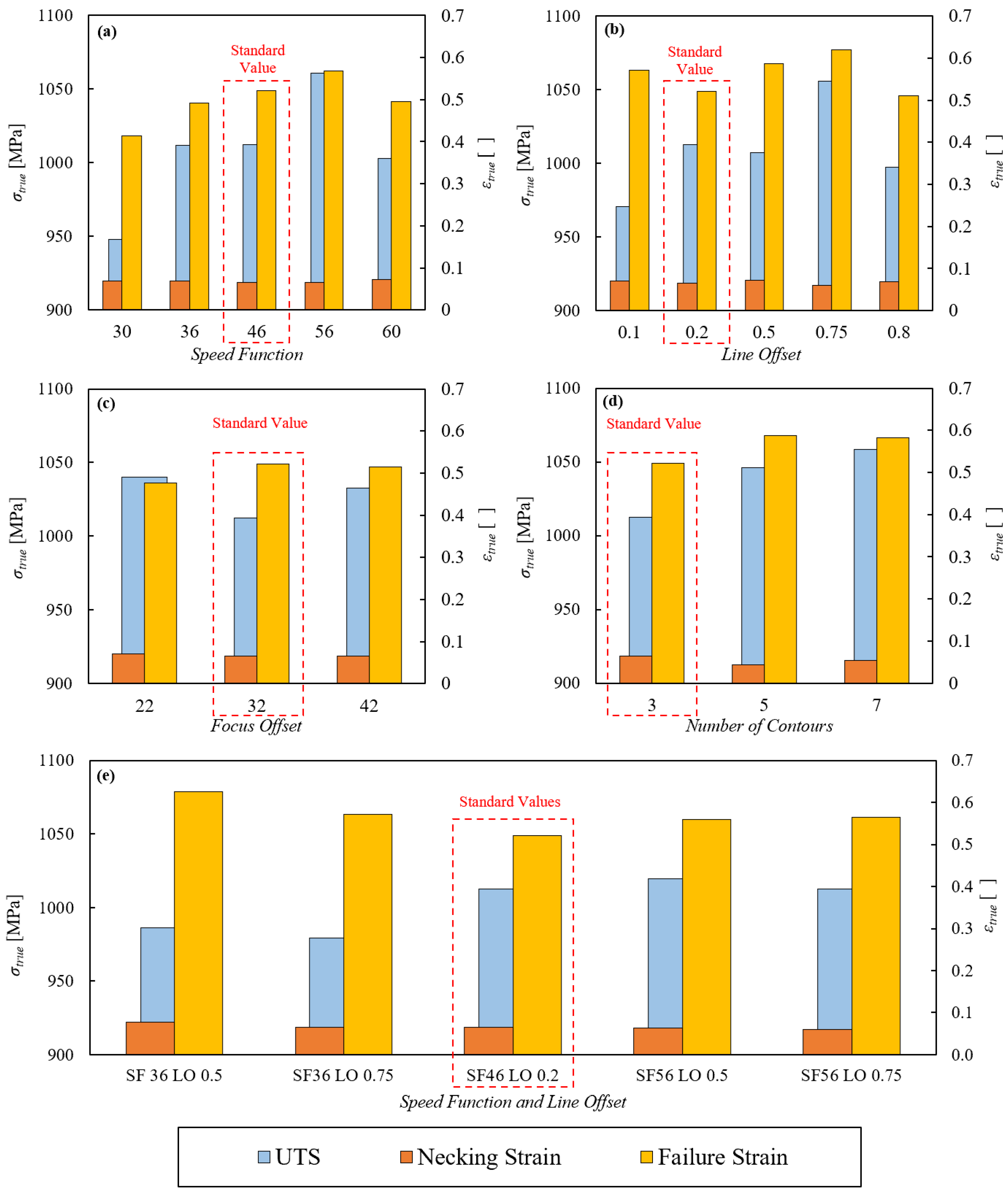
3.1. Static Experimental Series
3.2. Dynamic Experimental Series
3.3. Discussion
4. Conclusions
- At static rates, the tensile properties of the Ti alloy at hand are greatly affected by the speed function. Indeed, greater values than the default deliver better strength and ductility until an optimum value at SF = 56. Also, the line offset affects the performance of the material, with an optimum value of LO = 0.75. On the other hand, changing the focus offset and number of contours values has negligible effects. Considering also the results obtained changing the line offset and speed function values simultaneously, it was shown that the speed function is the most critical parameter regarding the tensile performance of the additive manufactured material. Then, the SF56 parameter set was chosen to be compared to the standard one.
- The standard and the SF56 parameter sets have been tested at 1, 15, and 700 s−1. Thanks to the adopted optical techniques, it was shown that the effective section-based strain and strain rate at fracture are approximately four and ten times higher than the elongation-based ones, respectively,
- The results have shown that the optimized SF56 parameter set has better performance compared to the standard one in terms of strength and ductility in both static and dynamic conditions, with higher maximum stress and fracture strain values.
- From the static and dynamic curves, the dynamic amplification obtained with the standard and SF56 parameter sets have been calculated. The results have shown that the optimized parameter set is also characterized by a higher strain rate sensitivity than the standard one.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Khorasani, M.; Ghasemi, A.; Rolfe, B.; Gibson, I. Additive manufacturing a powerful tool for the aerospace industry. Rapid Prototyp. J. 2021. [Google Scholar] [CrossRef]
- Yao, J.; Ding, R.; Li, K.; Du, B.; Zhao, L.; Yuan, Y. Study on the impact behavior of arch micro-strut (ARCH) lattice structure by selective laser melting (SLM). Rapid Prototyp. J. 2022. [Google Scholar] [CrossRef]
- Frazier, W.J. Metal additive manufacturing: A review. J. Mater. Eng. Perform. 2014, 23, 1917–1928. [Google Scholar] [CrossRef]
- Herzog, D.; Seyda, V.; Wycisk, E.; Emmelmann, C. Additive manufacturing of metals. Acta Mater. 2016, 117, 371–392. [Google Scholar] [CrossRef]
- Liu, S.; Shin, Y.C. Additive manufacturing of Ti6Al4V alloy: A review. Mater. Des. 2019, 164, 107552. [Google Scholar] [CrossRef]
- Silvestri, A.T.; Foglia, S.; Borrelli, R.; Franchitti, S.; Pirozzi, C.; Astarita, A. Electron beam melting of Ti6Al4V: Role of the process parameters under the same energy density. J. Manuf. Process. 2020, 60, 162–179. [Google Scholar] [CrossRef]
- Körner, C. Additive manufacturing of metallic components by selective electron beam melting—A review. Int. Mater. Rev. 2016, 61, 361–377. [Google Scholar] [CrossRef]
- Mirone, G.; Barbagallo, R.; Corallo, D.; Di Bella, S. Static and dynamic response of titanium alloy produced by electron beam melting. Procedia Struct. Integr. 2016, 2, 2355–2366. [Google Scholar] [CrossRef]
- Mirone, G.; Barbagallo, R.; Giudice, F.; Di Bella, S. Analysis and modelling of tensile and torsional behaviour at different strain rates of Ti6Al4V alloy additive manufactured by electron beam melting (EBM). Mater. Sci. Eng. A 2020, 793, 139916. [Google Scholar] [CrossRef]
- Chastand, V.; Quaegebeur, P.; Maia, W.; Charkaluk, E. Comparative study of fatigue properties of Ti-6Al-4V specimens built by electron beam melting (EBM) and selective laser melting (SLM). Mater. Charact. 2018, 143, 76–81. [Google Scholar] [CrossRef]
- Elias, C.N.; Lima JH, C.; Valiev, R.; Meyers, M.A. Biomedical applications of titanium and its alloys. JOM 2008, 60, 46–49. [Google Scholar] [CrossRef]
- Hao, Y.L.; Li, S.J.; Yang, R. Biomedical titanium alloys and their additive manufacturing. Rare Met. 2016, 35, 661–671. [Google Scholar] [CrossRef]
- Galati, M.; Minetola, P.; Rizza, G. Surface roughness characterisation and analysis of the Electron Beam Melting (EBM) process. Materials 2019, 12, 2211. [Google Scholar] [CrossRef] [PubMed]
- Prisco, U.; Astarita, A.; El Hassanin, A.; Franchitti, S. Influence of processing parameters on microstructure and roughness of electron beam melted Ti-6Al-4V titanium alloy. Mater. Manuf. Process. 2019, 34, 1753–1760. [Google Scholar] [CrossRef]
- Wang, X.; Gong, X.; Chou, K. Scanning speed effect on mechanical properties of Ti-6Al-4V alloy processed by electron beam additive manufacturing. Procedia Manuf. 2015, 1, 287–295. [Google Scholar] [CrossRef]
- Ge, W.; Guo, C.; Lin, F. Effect of process parameters on microstructure of TiAl alloy produced by electron beam selective melting. Procedia Eng. 2014, 81, 1192–1197. [Google Scholar] [CrossRef]
- Linares, J.M.; Chaves-Jacob, J.; Lopez, Q.; Sprauel, J.M. Fatigue life optimization for 17–4Ph steel produced by selective laser melting. Rapid Prototyp. J. 2022. [Google Scholar] [CrossRef]
- Shao, M.; Vijayan, S.; Nandwana, P.; Jinschek, J.R. The effect of beam scan strategies on microstructural variations in Ti-6Al-4V fabricated by electron beam powder bed fusion. Mater. Des. 2020, 196, 109165. [Google Scholar] [CrossRef]
- Mirone, G.; Barbagallo, R.; Di Bella, S. Effect of process parameters on the mechanical properties of a Titanium alloy fabricated by Electron Beam Melting (EBM). In IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2022; Volume 1214, p. 1. [Google Scholar]
- Mirone, G.; Verleysen, P.; Barbagallo, R. Tensile testing of metals: Relationship between macroscopic engineering data and hardening variables at the semi-local scale. Int. J. Mech. Sci. 2019, 150, 154–167. [Google Scholar] [CrossRef]
- Zhang, L.; Gour, G.; Petrinic, N.; Pellegrino, A. Rate dependent behaviour and dynamic strain localisation of three novel impact resilient titanium alloys: Experiments and modelling. Mater. Sci. Eng. A 2020, 771, 138552. [Google Scholar] [CrossRef]
- Mirone, G.; Barbagallo, R.; Giudice, F. Locking of the strain rate effect in Hopkinson bar testing of a mild steel. Int. J. Impact Eng. 2019, 130, 97–112. [Google Scholar] [CrossRef]
- Mirone, G.; Corallo, D.; Barbagallo, R. Interaction of strain rate and necking on the stress-strain response of uniaxial tension tests by Hopkinson bar. Procedia Struct. Integr. 2016, 2, 974–985. [Google Scholar] [CrossRef][Green Version]
- Mirone, G.; Barbagallo, R. How sensitivity of metals to strain, strain rate and temperature affects necking onset and hardening in dynamic tests. Int. J. Mech. Sci. 2021, 195, 106249. [Google Scholar] [CrossRef]
- Mirone, G.; Barbagallo, R.; Cadoni, E. Tensile test of a HSLA steel at high strain rates with two different SHTB facilities. Procedia Eng. 2017, 197, 89–98. [Google Scholar] [CrossRef]
- Beer, F.P.; Johnston, E.R.; DeWolf, J.T.; Mazurek, D.F. Mechanics of Materials, 7th ed.; MeGraw-Hill Education Ltd.: New York, NY, USA, 2015. [Google Scholar]
- Mirone, G. The dynamic effect of necking in Hopkinson bar tension tests. Mech. Mater. 2013, 58, 84–96. [Google Scholar] [CrossRef]
- Zhang, L.; Pellegrino, A.; Petrinic, N. Dynamic necking of a near α titanium alloy at high strain rates: Experiments and modelling. Def. Technol. 2021, 17, 1126–1134. [Google Scholar] [CrossRef]
- Jacques, N.; Rodríguez-Martínez, J.A. Influence on strain-rate history effects on the development of necking instabilities under dynamic loading conditions. Int. J. Solids Struct. 2021, 230, 111152. [Google Scholar] [CrossRef]
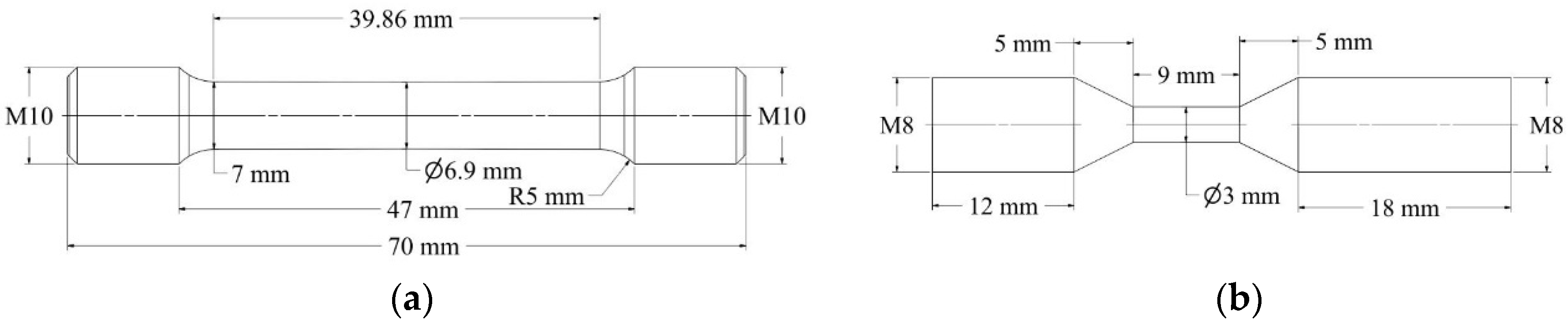
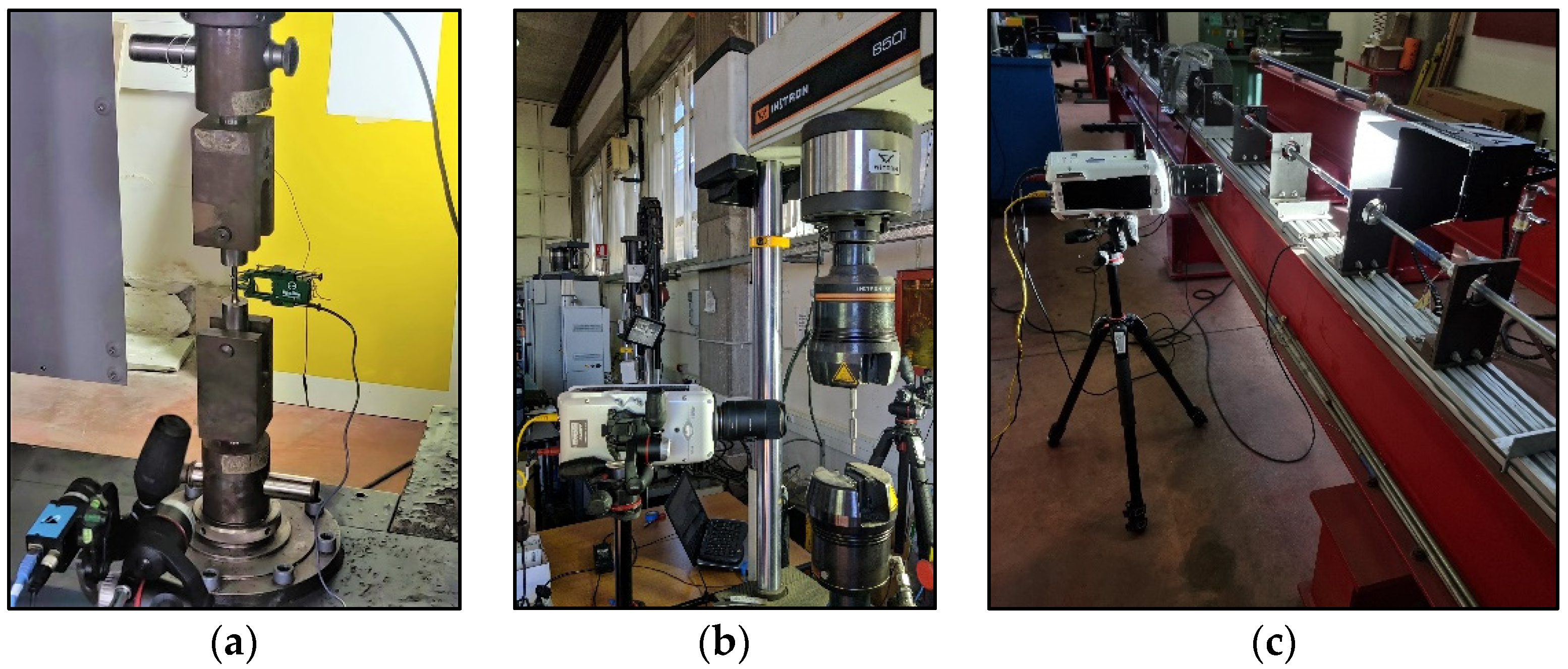



| Test Series | Experimental Setup | Nominal Strain Rate [s−1] |
|---|---|---|
| Static | Motor-driven machine Zwick Z100 | 0.003 |
| Dynamic—Intermediate Strain rates | Hydraulic machine Instron 8501 | 1 |
| 15 | ||
| Dynamic—High Strain Rates | SHTB | 700 |
| Series | Speed Function | Focus Offset [mA] | Line Offset [mm] | N. of Contours |
|---|---|---|---|---|
| STD | 46 | 32 | 0.2 | 3 |
| SF30 | 30 | 32 | 0.2 | 3 |
| SF36 | 36 | 32 | 0.2 | 3 |
| SF56 | 56 | 32 | 0.2 | 3 |
| SF60 | 60 | 32 | 0.2 | 3 |
| FO22 | 46 | 22 | 0.2 | 3 |
| FO42 | 46 | 42 | 0.2 | 3 |
| LO01 | 46 | 32 | 0.1 | 3 |
| LO05 | 46 | 32 | 0.5 | 3 |
| LO075 | 46 | 32 | 0.75 | 3 |
| LO08 | 46 | 32 | 0.8 | 3 |
| NC5 | 46 | 32 | 0.2 | 5 |
| NC7 | 46 | 32 | 0.2 | 7 |
| SF56_LO075 | 56 | 32 | 0.75 | 3 |
| SF56_LO05 | 56 | 32 | 0.5 | 3 |
| SF36_LO075 | 36 | 32 | 0.75 | 3 |
| SF36_LO05 | 36 | 32 | 0.5 | 3 |
| Test Series | R STD | R SF56 |
|---|---|---|
| Static | 1 | 1 |
| D1 | 1.02 | 1.03 |
| D15 | 1.07 | 1.10 |
| D700 | 1.09 | 1.14 |
| Performance Increase from STD to SF56 | |||
|---|---|---|---|
| Test Series | Stress Values | Failure Strain | Dynamic Amplification |
| Static | 7% | 9% | - |
| D1 | 5% | 13% | 50% |
| D15 | 5% | 14% | 43% |
| D700 | 4% | 9% | 56% |
| Average | 5% | 11% | 49% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Barbagallo, R.; Di Bella, S.; Mirone, G.; La Rosa, G. Study of the Electron Beam Melting Process Parameters’ Influence on the Tensile Behavior of 3D Printed Ti6Al4V ELI Alloy in Static and Dynamic Conditions. Materials 2022, 15, 4217. https://doi.org/10.3390/ma15124217
Barbagallo R, Di Bella S, Mirone G, La Rosa G. Study of the Electron Beam Melting Process Parameters’ Influence on the Tensile Behavior of 3D Printed Ti6Al4V ELI Alloy in Static and Dynamic Conditions. Materials. 2022; 15(12):4217. https://doi.org/10.3390/ma15124217
Chicago/Turabian StyleBarbagallo, Raffaele, Simone Di Bella, Giuseppe Mirone, and Guido La Rosa. 2022. "Study of the Electron Beam Melting Process Parameters’ Influence on the Tensile Behavior of 3D Printed Ti6Al4V ELI Alloy in Static and Dynamic Conditions" Materials 15, no. 12: 4217. https://doi.org/10.3390/ma15124217
APA StyleBarbagallo, R., Di Bella, S., Mirone, G., & La Rosa, G. (2022). Study of the Electron Beam Melting Process Parameters’ Influence on the Tensile Behavior of 3D Printed Ti6Al4V ELI Alloy in Static and Dynamic Conditions. Materials, 15(12), 4217. https://doi.org/10.3390/ma15124217






