LPBF Manufactured Functionally Graded Lattice Structures Obtained by Graded Density and Hybrid Poisson’s Ratio
Abstract
:1. Introduction
2. Materials and Methods
2.1. Material
2.2. Design and LPBF Manufacturing of Lattice Structures
2.3. Measurements and Characterizations
3. Results and Discussion
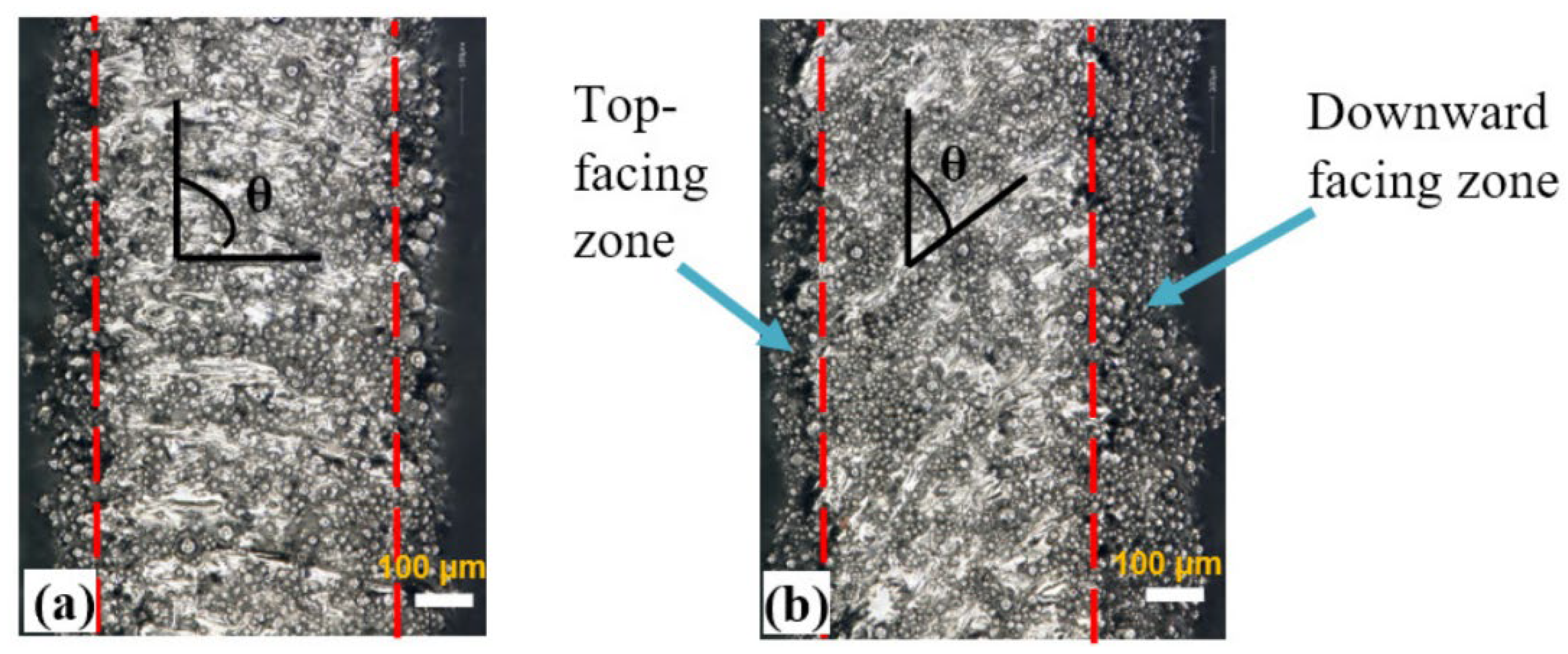
3.1. Lattice Manufacturability and Morphological Characterization
3.2. Relative Density and Surface Area
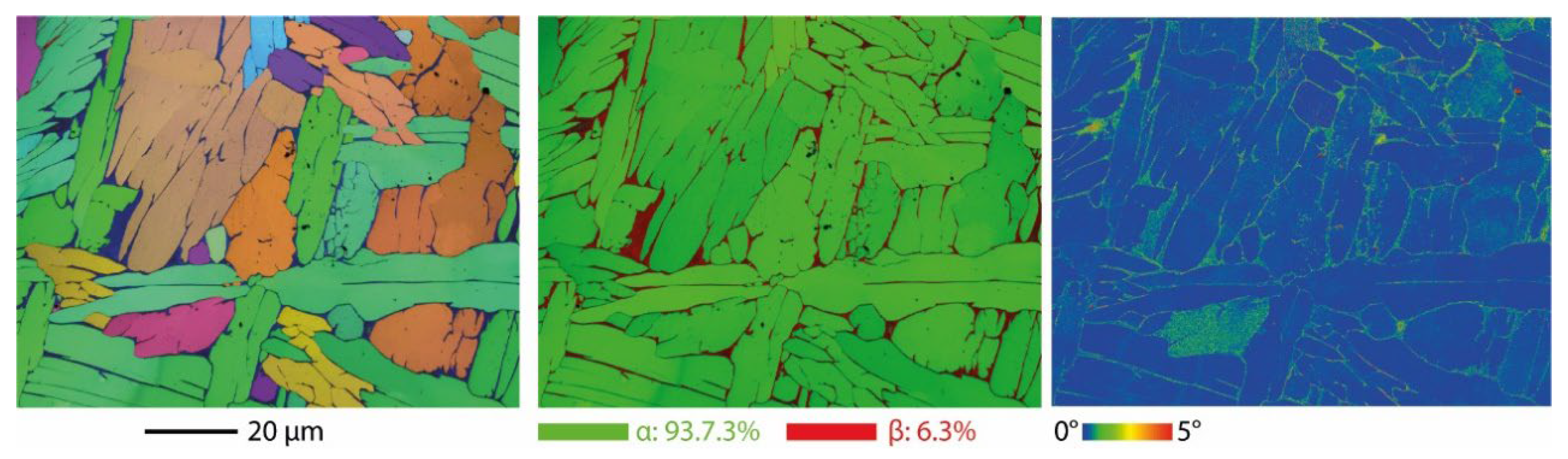
3.3. Microstructural Characterization
3.4. Micro-Hardness
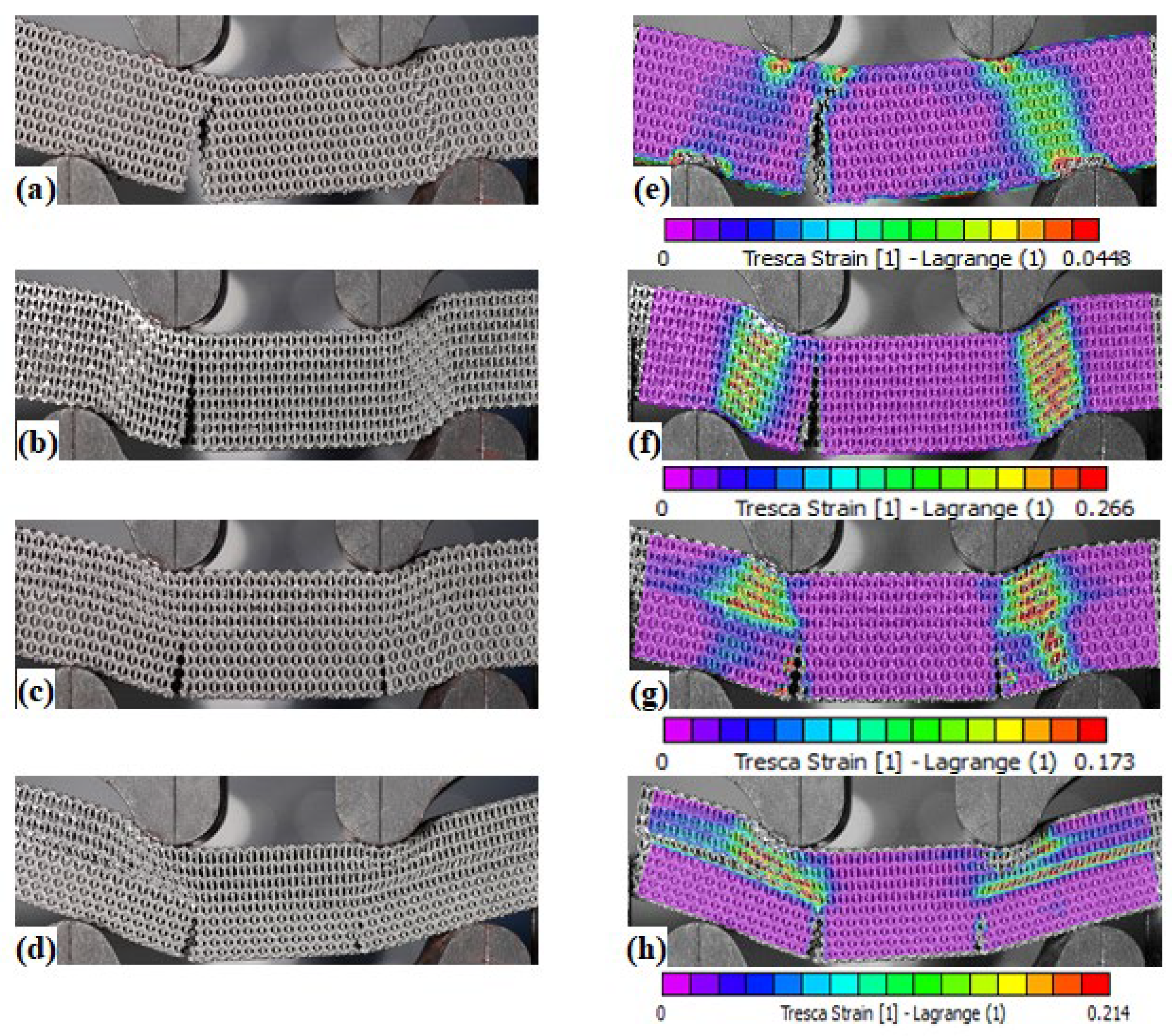
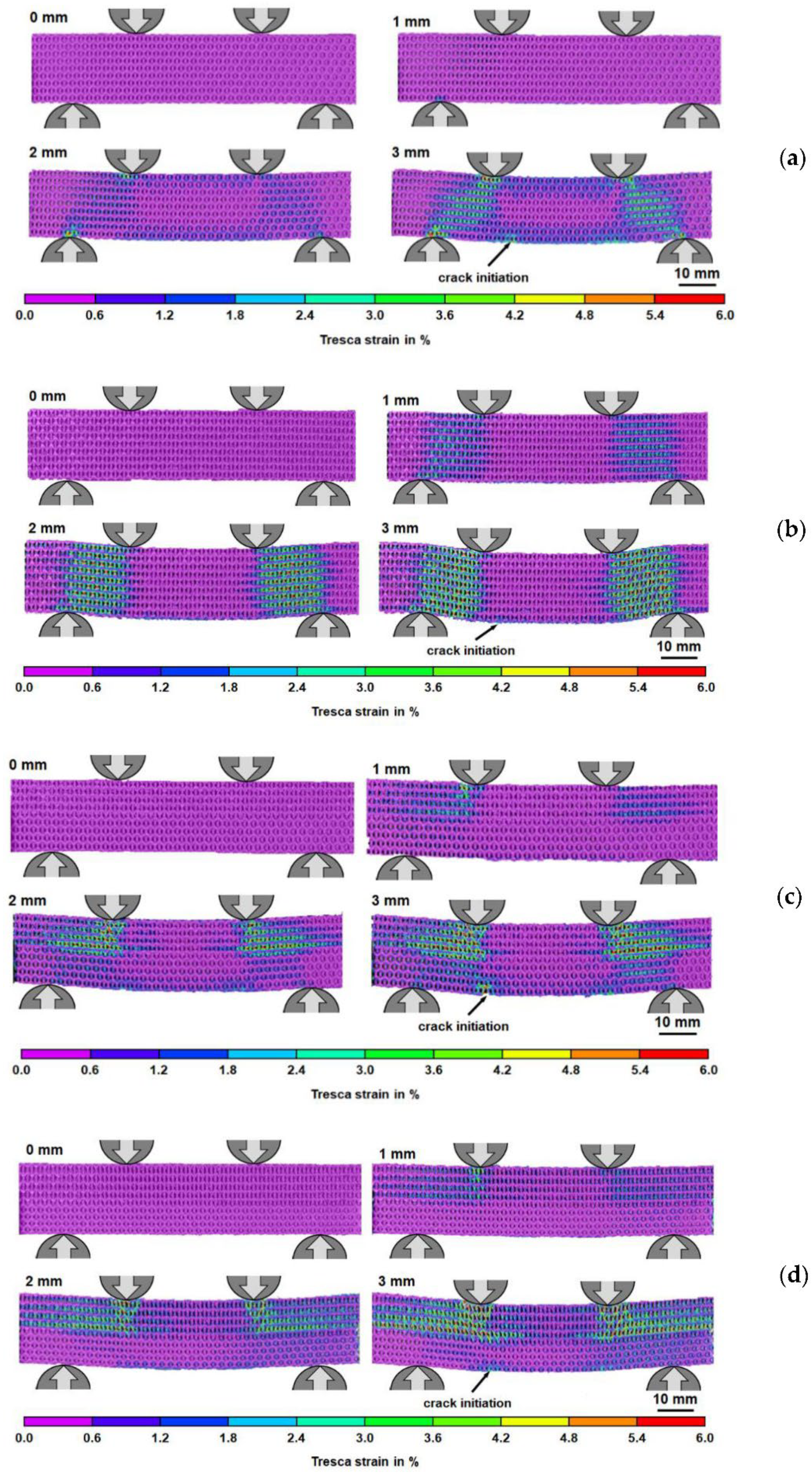
3.5. Flexural Properties
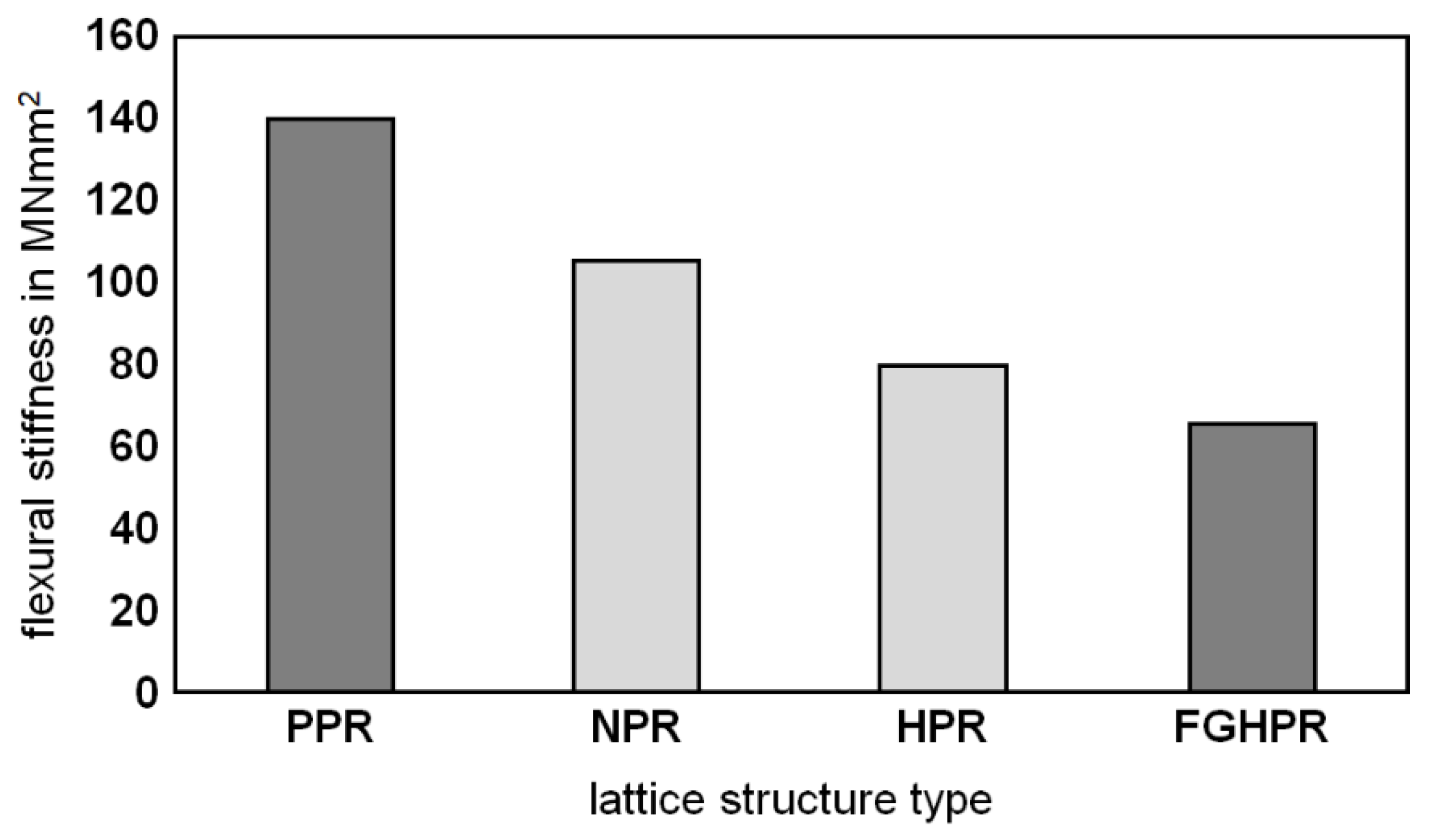
3.5.1. Flexural Stiffness
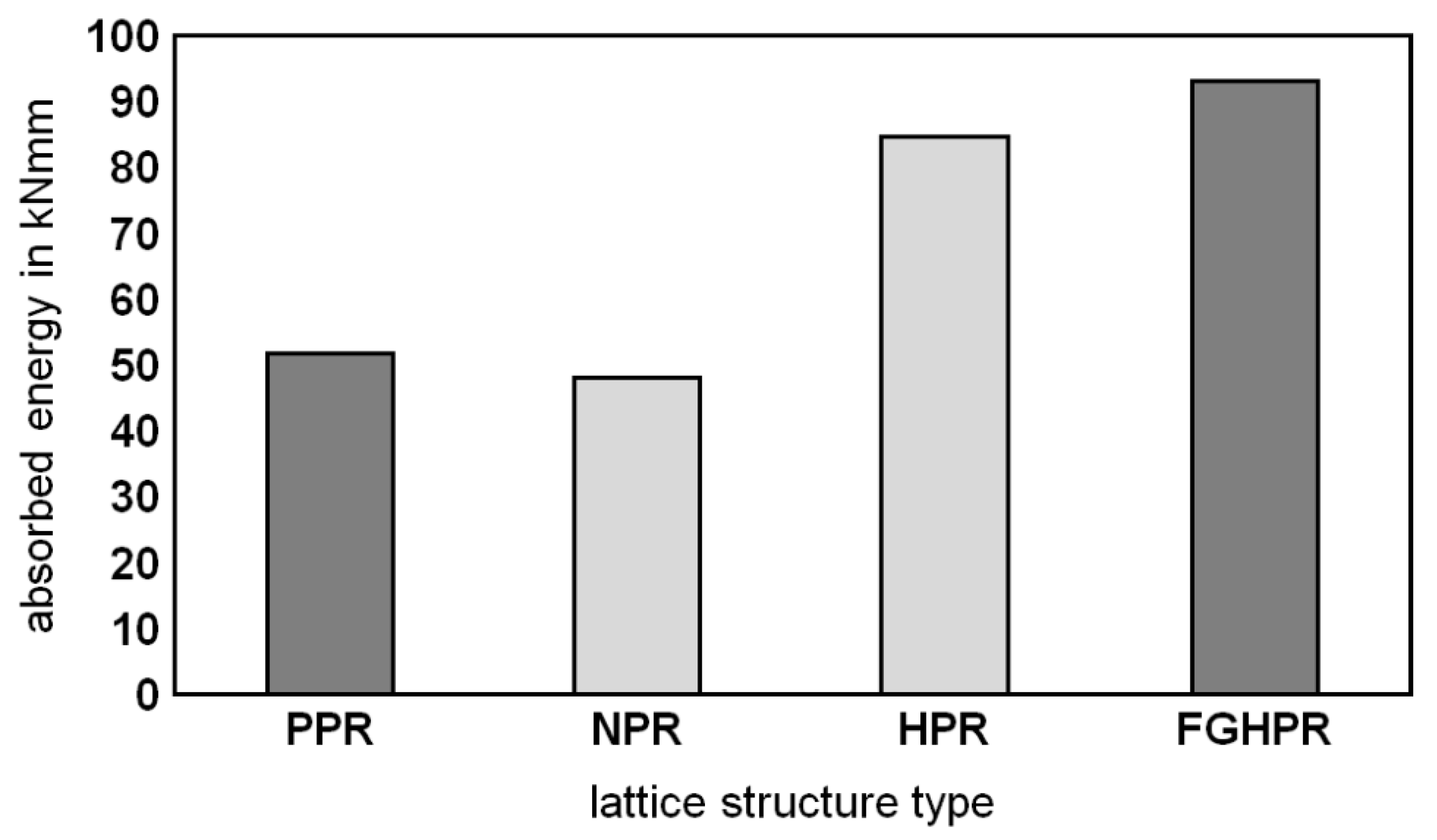
3.5.2. Absorbed Energy
4. Conclusions
- The four types of lattice structures were successfully manufactured, proving the capability of the LPBF technique to fabricate intricate functionally graded lattices.
- The hardness values obtained for the heat-treated LPBF-manufactured Ti-6Al-4V parts corresponded to those in the literature.
- This study also confirmed the formation of fine acicular α/α’ of the as-built Ti6Al4V samples due to the high cooling rate in the LPBF process and evolution of the β-phase fraction after heat treatment.
- The experimental investigation demonstrated that the PPR structure showed the highest strength.
- The PPR structure also showed high flexural stiffness (140 MN/mm2), followed by the NPR, HPR, then FGHPR structures with 105 MN/mm2, 80 MN/mm2, and 65 MN/mm2, respectively.
- The structural ductility of the PPR was significantly lower, which indicated that the PPR structures had a rather low energy absorption capacity. This result is consistent with the findings of Yang et al. [27].
- Enhanced stiffness and strength of HPR and FGHPR were expected to be obtained in the case of eliminating the shear flow (slipping) between the NPR portion and PPR portion. Eliminating the shear flow may be achieved by increasing the stiffness of the intermediate layer.
- The best response in terms of absorbed energy was obtained for the functionally graded hybrid PR (FGHPR) structure. Both the FGHPR and hybrid PR (HPR) structures showed a 78.7% and 62.9% increase in the absorbed energy, respectively, when compared to the PPR structure.
- The experimental results also revealed that the deformation and failure mechanisms evolved differently in the four structures.
Author Contributions
Funding
Conflicts of Interest
References
- Xiao, D.; Mu, L.; Zhao, G. Indentation response of sandwich panels with positive gradient metallic cellular core. J. Sandw. Struct. Mater. 2015, 17, 597–612. [Google Scholar] [CrossRef]
- Al-Saedi, D.S.J.; Masood, S.H.; Faizan-Ur-Rab, M.; Alomarah, A.; Ponnusamy, P. Mechanical properties and energy absorption capability of functionally graded F2BCC lattice fabricated by SLM. Mater. Des. 2018, 144, 32–44. [Google Scholar] [CrossRef]
- Ma, X.; Zhang, D.Z.; Zhao, M.; Jiang, J.; Luo, F.; Zhou, H. Mechanical and energy absorption properties of functionally graded lattice structures based on minimal curved surfaces. Int. J. Adv. Manuf. Technol. 2022, 118, 995–1008. [Google Scholar] [CrossRef]
- Yang, L.; Wu, S.; Yan, C.; Chen, P.; Zhang, L.; Han, C.; Cai, C. Fatigue properties of Ti-6Al-4V Gyroid graded lattice structures fabricated by laser powder bed fusion with lateral loading. Addit. Manuf. 2021, 46, 102214. [Google Scholar] [CrossRef]
- Chen, W.; Yang, J.; Kong, H.; Helou, M.; Zhang, D.; Zhao, J.; Jia, W.; Liu, Q.; He, P.; Li, X. Fatigue behaviour and biocompatibility of additively manufactured bioactive tantalum graded lattice structures for load-bearing orthopaedic applications. Mater. Sci. Eng. C 2021, 130, 112461. [Google Scholar] [CrossRef]
- Bai, L.; Gong, C.; Chen, X.; Zheng, J.; Xin, L.; Xiong, Y.; Wu, X.; Hu, M.; Li, K.; Sun, Y. Quasi-Static compressive responses and fatigue behaviour of Ti-6Al-4 V graded lattice structures fabricated by laser powder bed fusion. Mater. Des. 2021, 210, 110110. [Google Scholar] [CrossRef]
- Choy, S.Y.; Sun, C.-N.; Sin, W.J.; Leong, K.F.; Su, P.-C.; Wei, J.; Wang, P. Superior energy absorption of continuously graded microlattices by electron beam additive manufacturing. Virtual Phys. Prototyp. 2021, 16, 14–28. [Google Scholar] [CrossRef]
- Mahmoud, D.; Al-Rubaie, K.S.; Elbestawi, M.A. The influence of selective laser melting defects on the fatigue properties of Ti6Al4V porosity graded gyroids for bone implants. Int. J. Mech. Sci. 2021, 193, 106180. [Google Scholar] [CrossRef]
- Boldrin, L.; Hummel, S.; Scarpa, F.; Di Maio, D.; Lira, C.; Ruzzene, M.; Remillat, C.D.L.; Lim, T.C.; Rajasekaran, R.; Patsias, S. Dynamic behaviour of auxetic gradient composite hexagonal honeycombs. Compos. Struct. 2016, 149, 114–124. [Google Scholar] [CrossRef] [Green Version]
- Jiang, W.; Ren, X.; Wang, S.L.; Zhang, X.G.; Zhang, X.Y.; Luo, C.; Xie, Y.M.; Scarpa, F.; Alderson, A.; Evans, K.E. Manufacturing, characteristics and applications of auxetic foams: A state-of-the-art review. Compos. Part B Eng. 2022, 235, 109733. [Google Scholar] [CrossRef]
- Rezaei, S.; Kadkhodapour, J.; Hamzehei, R.; Taherkhani, B.; Anaraki, A.P.; Dariushi, S. Design and modeling of the 2D auxetic metamaterials with hyperelastic properties using topology optimization approach. Photonics Nanostructures-Fundam. Appl. 2021, 43, 100868. [Google Scholar] [CrossRef]
- Gao, J.; Xue, H.; Gao, L.; Luo, Z. Topology optimization for auxetic metamaterials based on isogeometric analysis. Comput. Methods Appl. Mech. Eng. 2019, 352, 211–236. [Google Scholar] [CrossRef]
- Zhang, G.; Khandelwal, K. Computational design of finite strain auxetic metamaterials via topology optimization and nonlinear homogenization. Comput. Methods Appl. Mech. Eng. 2019, 356, 490–527. [Google Scholar] [CrossRef] [Green Version]
- Albertini, F.; Dirrenberger, J.; Sollogoub, C.; Maconachie, T.; Leary, M.; Molotnikov, A. Experimental and computational analysis of the mechanical properties of composite auxetic lattice structures. Addit. Manuf. 2021, 47, 102351. [Google Scholar] [CrossRef]
- Valle, R.; Pincheira, G.; Tuninetti, V.; Fernandez, E.; Uribe-Lam, E. Design and Characterization of Asymmetric Cell Structure of Auxetic Material for Predictable Directional Mechanical Response. Materials 2022, 15, 1841. [Google Scholar] [CrossRef] [PubMed]
- Fu, M.-H.; Chen, Y.; Hu, L.-L. A novel auxetic honeycomb with enhanced in-plane stiffness and buckling strength. Compos. Struct. 2017, 160, 574–585. [Google Scholar] [CrossRef]
- Warmuth, F.; Osmanlic, F.; Adler, L.; Lodes, M.A.; Körner, C. Fabrication and characterisation of a fully auxetic 3D lattice structure via selective electron beam melting. Smart Mater. Struct. 2016, 26, 25013. [Google Scholar] [CrossRef]
- Shen, J.; Liu, K.; Zeng, Q.; Ge, J.; Dong, Z.; Liang, J. Design and mechanical property studies of 3D re-entrant lattice auxetic structure. Aerosp. Sci. Technol. 2021, 118, 106998. [Google Scholar] [CrossRef]
- Novak, N.; Vesenjak, M.; Krstulović-Opara, L.; Ren, Z. Mechanical characterisation of auxetic cellular structures built from inverted tetrapods. Compos. Struct. 2018, 196, 96–107. [Google Scholar] [CrossRef]
- Seetoh, I.P.; Liu, X.; Markandan, K.; Zhen, L.; Lai, C.Q. Strength and energy absorption characteristics of Ti6Al4V auxetic 3D anti-tetrachiral metamaterials. Mech. Mater. 2021, 156, 103811. [Google Scholar] [CrossRef]
- Kolken, H.M.A.; Lietaert, K.; van der Sloten, T.; Pouran, B.; Meynen, A.; Van Loock, G.; Weinans, H.; Scheys, L.; Zadpoor, A.A. Mechanical performance of auxetic meta-biomaterials. J. Mech. Behav. Biomed. Mater. 2020, 104, 103658. [Google Scholar] [CrossRef] [PubMed]
- Lim, T.C. Functionally graded beam for attaining Poisson-curving. J. Mater. Sci. Lett. 2002, 21, 1899–1901. [Google Scholar] [CrossRef]
- Guo, M.-F.; Yang, H.; Ma, L. Design and characterization of 3D AuxHex lattice structures. Int. J. Mech. Sci. 2020, 181, 105700. [Google Scholar] [CrossRef]
- Ingrole, A.; Hao, A.; Liang, R. Design and modeling of auxetic and hybrid honeycomb structures for in-plane property enhancement. Mater. Des. 2017, 117, 72–83. [Google Scholar] [CrossRef]
- Jin, Y.; Xie, C.; Gao, Q.; Zhou, X.; Li, G.; Du, J.; He, Y. Fabrication of multi-scale and tunable auxetic scaffolds for tissue engineering. Mater. Des. 2021, 197, 109277. [Google Scholar] [CrossRef]
- Wang, L.; Zhu, S.; Wang, B.; Tan, X.; Zou, Y.; Chen, S.; Li, S. Latitude-and-longitude-inspired three-dimensional auxetic metamaterials. Extrem. Mech. Lett. 2021, 42, 101142. [Google Scholar] [CrossRef]
- Yang, L.; Harrysson, O.; West, H.; Cormier, D. A Comparison of Bending Properties for Cellular Core Sandwich Panels. Mater. Sci. Appl. 2013, 4, 471–477. [Google Scholar] [CrossRef] [Green Version]
- Horn, T.J.; Harrysson, O.L.A.; Marcellin-Little, D.J.; West, H.A.; Lascelles, B.D.X.; Aman, R. Flexural properties of Ti6Al4V rhombic dodecahedron open cellular structures fabricated with electron beam melting. Addit. Manuf. 2014, 1–4, 2–11. [Google Scholar] [CrossRef]
- Rahman Rashid, R.A.; Mallavarapu, J.; Palanisamy, S.; Masood, S.H. A comparative study of flexural properties of additively manufactured aluminium lattice structures. Mater. Today Proc. 2017, 4, 8597–8604. [Google Scholar] [CrossRef]
- Korshunova, N.; Alaimo, G.; Hosseini, S.B.; Carraturo, M.; Reali, A.; Niiranen, J.; Auricchio, F.; Rank, E.; Kollmannsberger, S. Bending behavior of octet-truss lattice structures: Modelling options, numerical characterization and experimental validation. Mater. Des. 2021, 205, 109693. [Google Scholar] [CrossRef]
- Tao, Y.; Li, P.; Zhang, H.; Shi, S.Q.; Zhang, J.; Yin, Q. Compression and flexural properties of rigid polyurethane foam composites reinforced with 3D-printed polylactic acid lattice structures. Compos. Struct. 2022, 279, 114866. [Google Scholar] [CrossRef]
- Li, C.; Shen, H.-S.; Wang, H. Nonlinear bending of sandwich beams with functionally graded negative Poisson’s ratio honeycomb core. Compos. Struct. 2019, 212, 317–325. [Google Scholar] [CrossRef]
- AP&C. Data Sheet: Ti-6Al-4V-MC-16-539 Powder for Additive Manufacturing; AP&C: Boisbriand, QC, Canada, 2020. [Google Scholar]
- Shen, Y.; Cantwell, W.; Li, Y. Skin-core adhesion in high performance sandwich structures. J. Zhejiang Univ. Sci. A 2014, 15, 61–67. [Google Scholar] [CrossRef] [Green Version]
- Gorny, B.; Niendorf, T.; Lackmann, J.; Thoene, M.; Troester, T.; Maier, H.J. In situ characterization of the deformation and failure behavior of non-stochastic porous structures processed by selective laser melting. Mater. Sci. Eng. A 2011, 528, 7962–7967. [Google Scholar] [CrossRef]
- Brenne, F.; Niendorf, T.; Maier, H.J. Additively manufactured cellular structures: Impact of microstructure and local strains on the monotonic and cyclic behavior under uniaxial and bending load. J. Mater. Process. Technol. 2013, 213, 1558–1564. [Google Scholar] [CrossRef]
- Xu, W.; Lui, E.W.; Pateras, A.; Qian, M.; Brandt, M. In situ tailoring microstructure in additively manufactured Ti-6Al-4V for superior mechanical performance. Acta Mater. 2017, 125, 390–400. [Google Scholar] [CrossRef]
- Yan, C.; Hao, L.; Hussein, A.; Young, P. Ti-6Al-4V triply periodic minimal surface structures for bone implants fabricated via selective laser melting. J. Mech. Behav. Biomed. Mater. 2015, 51, 61–73. [Google Scholar] [CrossRef] [Green Version]
- Huang, Q.; Liu, X.; Yang, X.; Zhang, R.; Shen, Z.; Feng, Q. Specific heat treatment of selective laser melted Ti–6Al–4V for biomedical applications. Front. Mater. Sci. 2015, 9, 373–381. [Google Scholar] [CrossRef]
- Lancea, C.; Chicos, L.A.; Zaharia, S.M.; Pop, M.A. Microstructure and micro-hardness analyses of titanium alloy Ti-6Al-4V parts manufactured by selective laser melting. In Proceedings of the 4th International Conference on Computing and Solutions in Manufacturing Engineering 2016—CoSME’16, Brasnov, Romania, 3–4 November 2016; EDP Sciences: Les Ulis, France, 2017; pp. 1–9. [Google Scholar]
- Filetin, T. Bending Stiffness of Aluminium Foams. Rad Hrvat. Akad. Znan. Umjet. Teh. Znan. 2012, 15, 93–116. [Google Scholar]
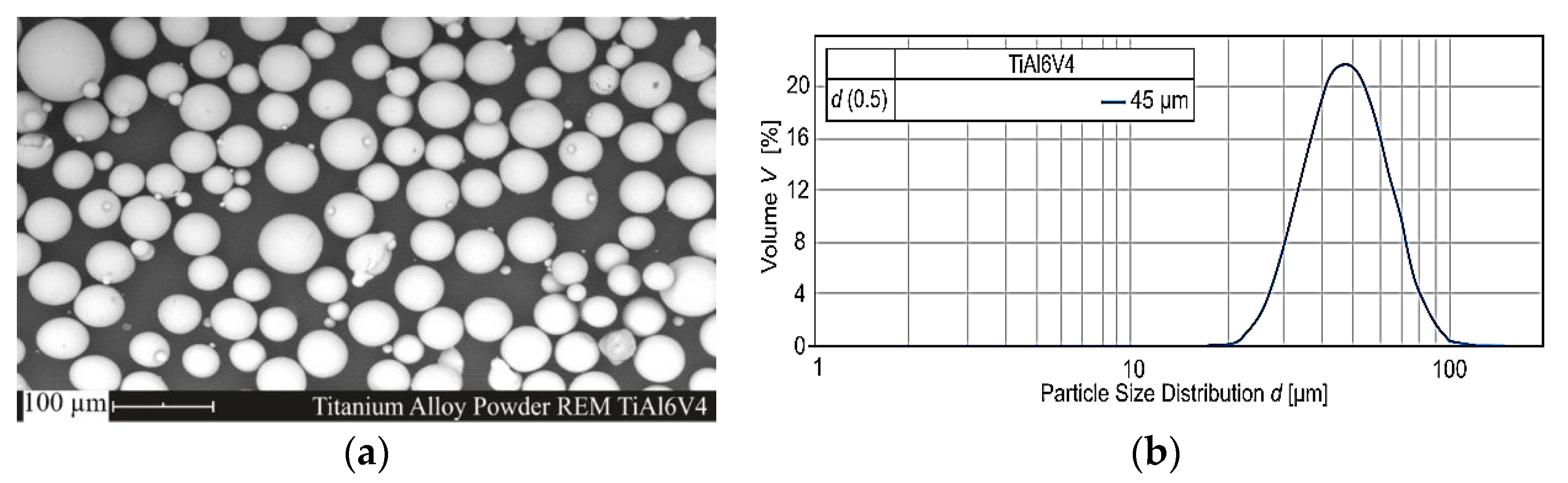
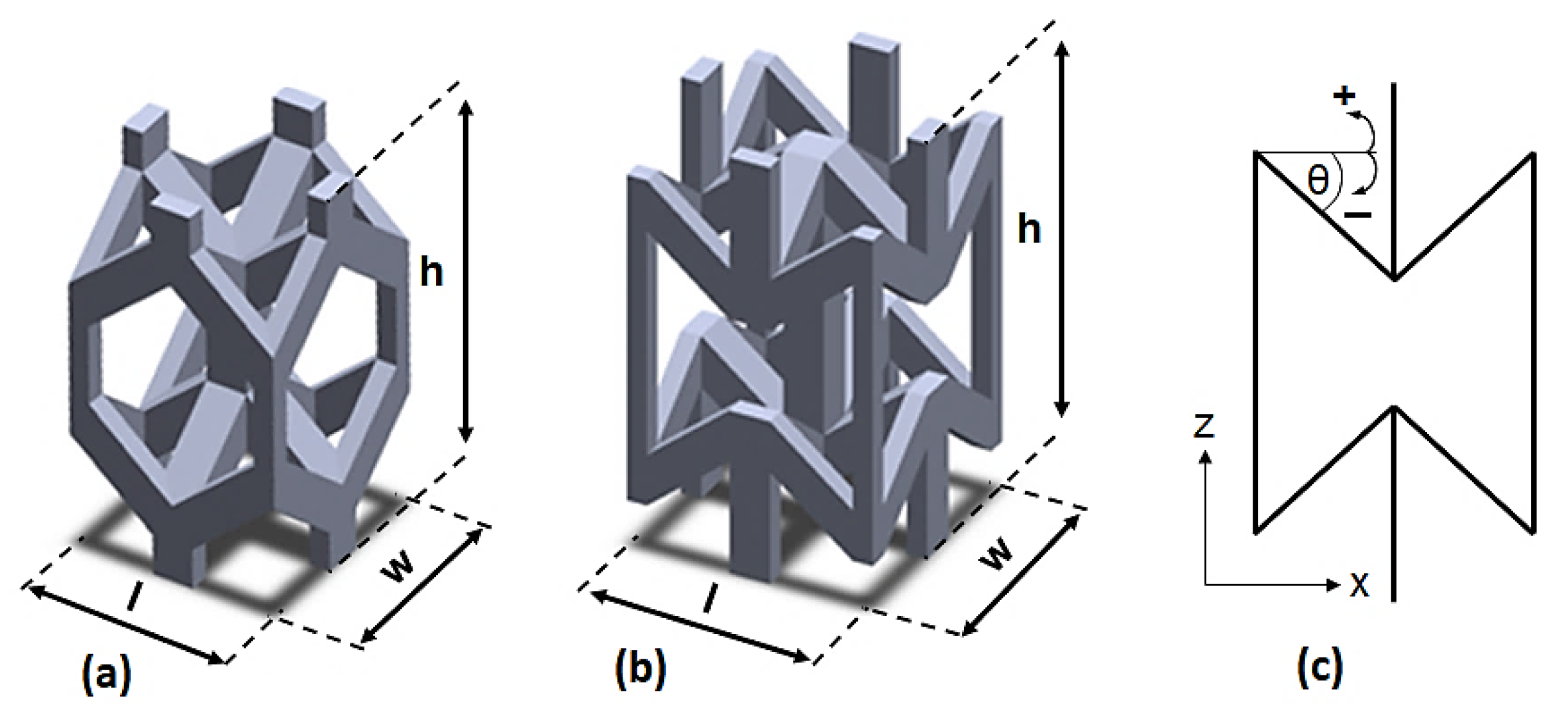
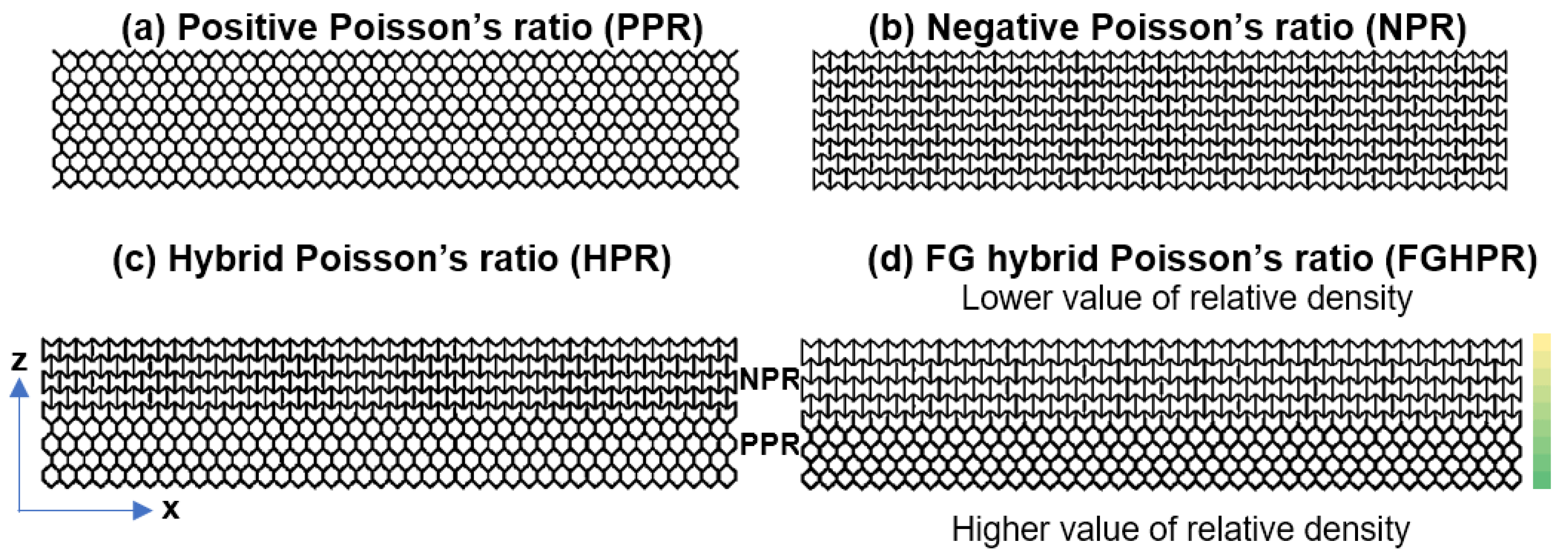
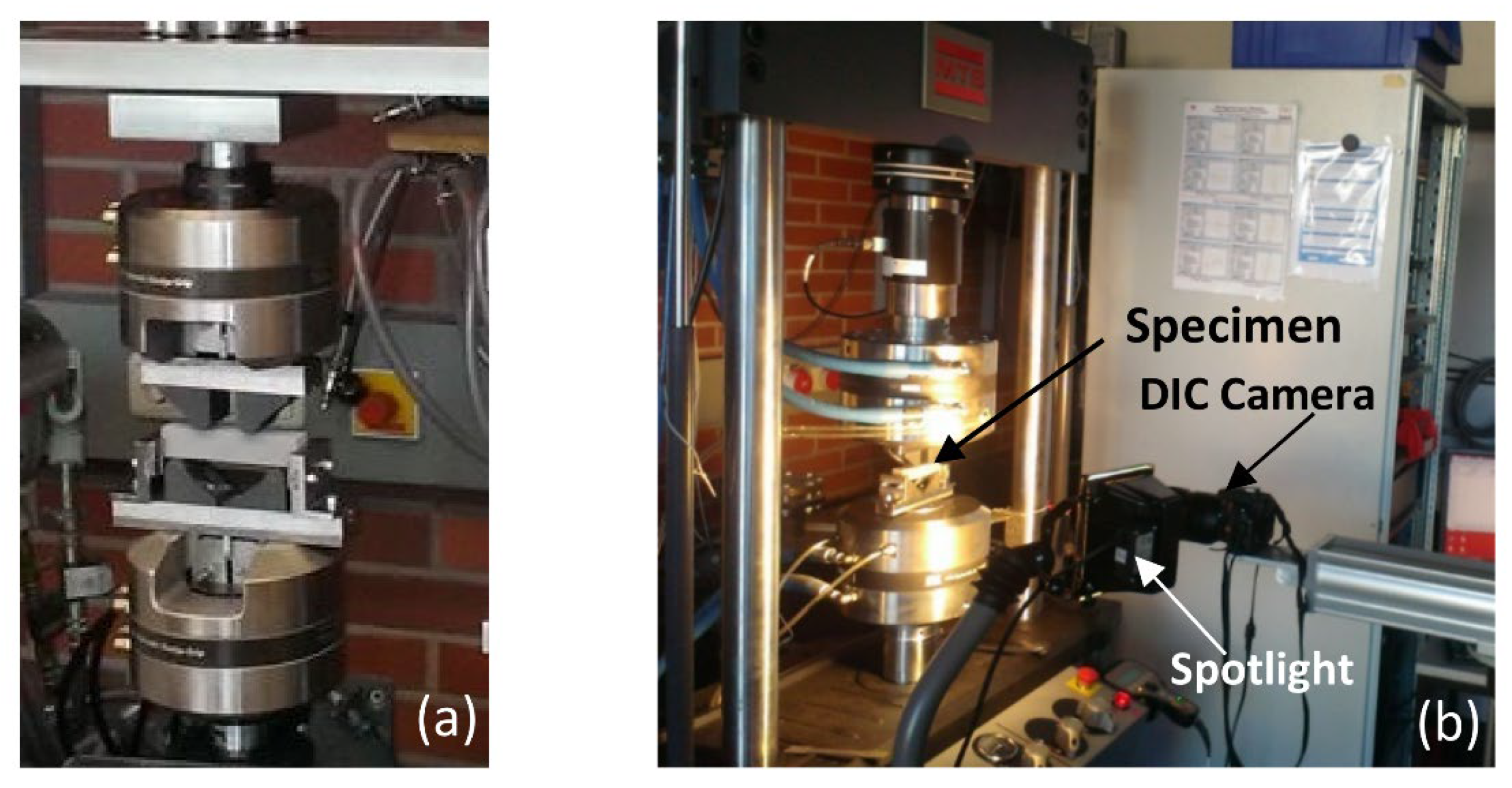





| Element | Al | V | Fe (Max.) | C | O | N | H | Ti |
|---|---|---|---|---|---|---|---|---|
| wt% | 6.4 | 4 | 0.2 | 0.01 | 0.13 | 0.02 | 0.002 | Bal. |
| Laser Power (W) | Layer Thickness (μm) | Hatch Space (μm) | Energy Density (J/mm3) | Scan Velocity mm/s | Scan Strategy | Building Plate Temperature, °C |
|---|---|---|---|---|---|---|
| 175 | 30 | 120 | 68,5 | 710 | Chessboard | 200 |
| Lattice Type | Theoretical Relative Density | Measured Relative Density | % Difference | Theoretical Surface Area (m2) |
|---|---|---|---|---|
| PPR | 0.28 | 0.3297 | +17.7 | 0.094 |
| NPR | 0.28 | 0.3457 | +23.4 | 0.111 |
| HPR | 0.28 | 0.3350 | +19.6 | 0.103 |
| FGHPR | 0.28 | 0.3322 | +18.6 | 0.102 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abdelaal, O.; Hengsbach, F.; Schaper, M.; Hoyer, K.-P. LPBF Manufactured Functionally Graded Lattice Structures Obtained by Graded Density and Hybrid Poisson’s Ratio. Materials 2022, 15, 4072. https://doi.org/10.3390/ma15124072
Abdelaal O, Hengsbach F, Schaper M, Hoyer K-P. LPBF Manufactured Functionally Graded Lattice Structures Obtained by Graded Density and Hybrid Poisson’s Ratio. Materials. 2022; 15(12):4072. https://doi.org/10.3390/ma15124072
Chicago/Turabian StyleAbdelaal, Osama, Florian Hengsbach, Mirko Schaper, and Kay-Peter Hoyer. 2022. "LPBF Manufactured Functionally Graded Lattice Structures Obtained by Graded Density and Hybrid Poisson’s Ratio" Materials 15, no. 12: 4072. https://doi.org/10.3390/ma15124072
APA StyleAbdelaal, O., Hengsbach, F., Schaper, M., & Hoyer, K.-P. (2022). LPBF Manufactured Functionally Graded Lattice Structures Obtained by Graded Density and Hybrid Poisson’s Ratio. Materials, 15(12), 4072. https://doi.org/10.3390/ma15124072







