The Influence of Density on the Value of Young’s Modulus for Dry Ice
Abstract
1. Introduction
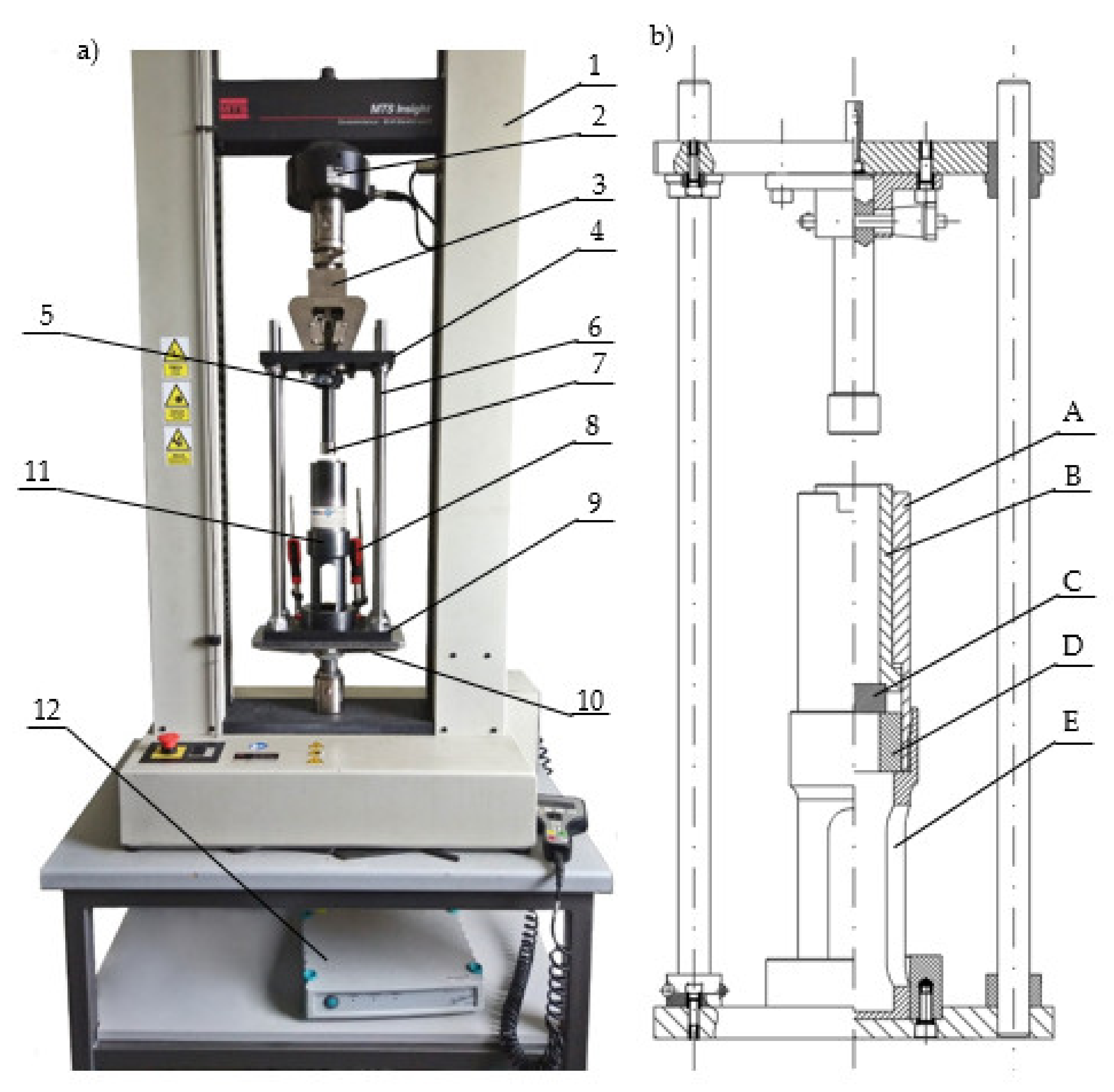
2. Materials and Methods
- The upper grip of the durometer together with the piston arrived at the reference position with the initial velocity.
- An initial downward motion was instigated with a travel speed of 1 mm/s until a resistance force value of 150 N was detected.
- The test was initialized. The machine grip moved downwards together with the installed upper plate and the piston at a test speed of 5 mm/s until the sample height value of 24 ± 0.05 mm was achieved.
- The assembly retracted with a speed of 5 mm/s until a force value of 0 N was achieved.
- The piston retracted with the end of the test speed to a height approximately 60 mm above the reference position to facilitate the removal of the sleeve and sample for weighing.
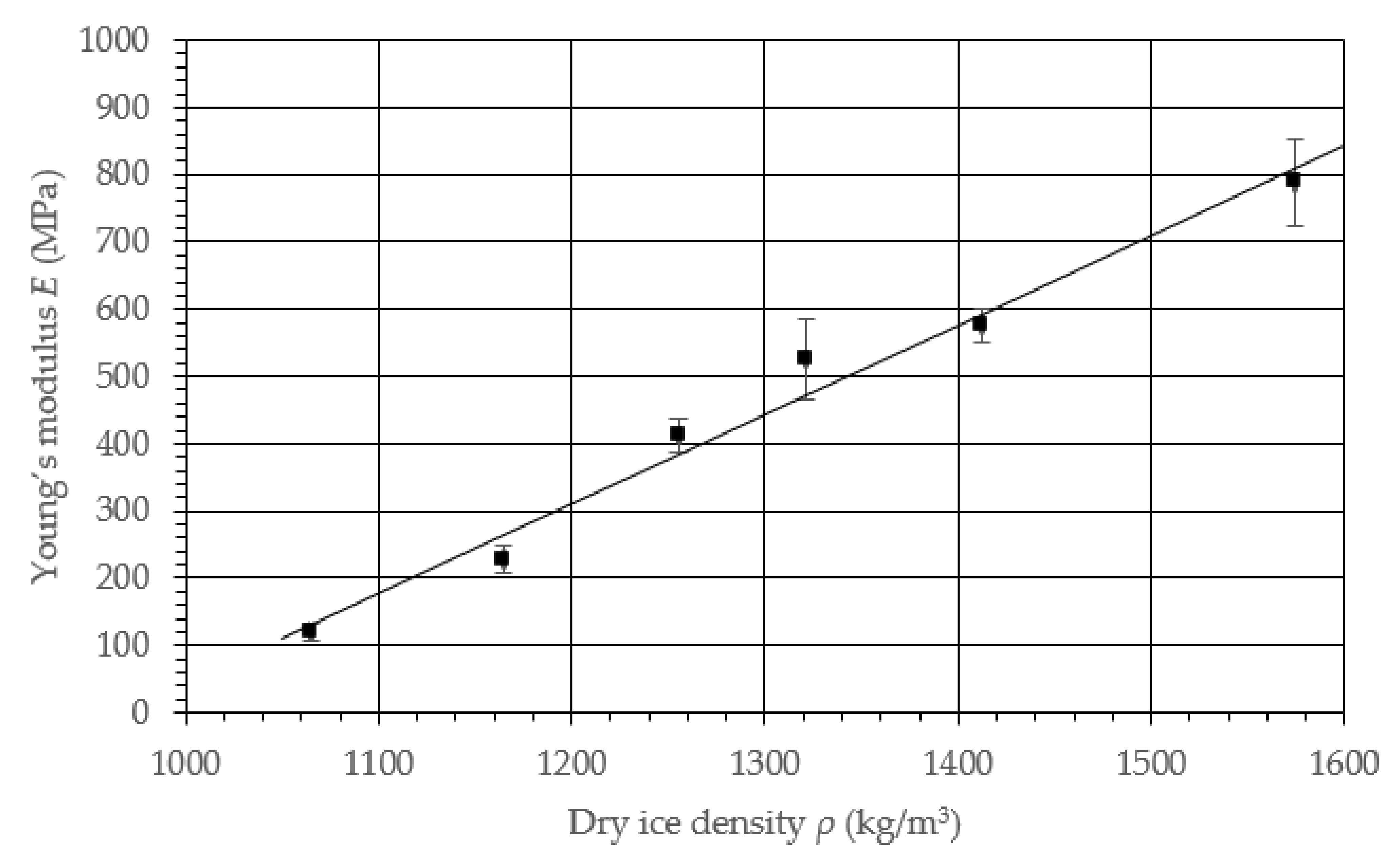
3. Results
4. Conclusions and Results Discussion
- -
- Numerical simulation of the compaction and extrusion processes using the DPC, CC and MC material models, for the purpose of estimating the working load;
- -
- Optimization of the geometric characteristics of the tools used in the processes of compaction and extrusion of dry ice, to increase the process efficiency;
- -
- Analysis of the energy consumption of the dry ice palletization process with the use of a gravity roller press.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Uhlmann, E.; Kretzschmar, M.; Elbing, F.; Mihotovic, V. Deburring with CO2 snow blasting. In Burrs—Analysis, Control and Removal; Aurich, J., Dornfeld, D., Eds.; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Gorecki, J.; Malujda, I.; Talaśka, K.; Wojtkowiak, D. Dry ice compaction in piston extrusion process. Acta Mech. Autom. 2017, 11, 313–316. [Google Scholar] [CrossRef][Green Version]
- Mikołajczak, A.; Krawczyk, P.; Kurkus-Gruszecka, M.; Badyda, K. Analysis of the Liquid Natural Gas Energy Storage basing on the mathematical model. Energy Procedia 2019, 159, 231–236. [Google Scholar] [CrossRef]
- Górecki, J.; Malujda, I.; Talaśka, K.; Kukla, M.; Tarkowski, P. Influence of the compression length on the ultimate stress in the process of mechanical agglomeration of dry ice. Procedia Eng. 2017, 177, 363–368. [Google Scholar] [CrossRef]
- Liu, Y.H.; Calvert, G.; Hare, C.; Ghadiri, M.; Matsusaka, S. Size measurement of dry ice particles produced form liquid carbon dioxide. J. Aerosol Sci. 2012, 48, 1–9. [Google Scholar] [CrossRef]
- Mazzoldi, A.; Hill, T.; Colls, J. CO2 transportation for carbon capture and storage: Sublimation of carbon dioxide from a dry ice bank. Int. J. Greenh. Gas Control 2008, 2, 210–218. [Google Scholar] [CrossRef]
- Górecki, J. Development of a testing station for empirical verification of the algebraic model of dry ice piston extrusion. Acta Mech. Autom. 2021, 15, 107–112. [Google Scholar] [CrossRef]
- Yamaguchi, H.; Niu, X.; Sekimoto, K.; Neksa, P. Investigation of dry ice blockage in an ultra-low temperature cascade refrigeration system using CO2 as a working fluid. Int. J. Refrig. 2011, 34, 466. [Google Scholar] [CrossRef]
- Liu, Y.; Maruyama, H.; Matsusaka, S. Agglomeration process of dry ice particles produced by expanding liquid carbon dioxide. Adv. Powder Technol. 2010, 21, 652–657. [Google Scholar] [CrossRef]
- Dong, S.; Song, B.; Hansz, B.; Liao, H.; Coddet, C. Combination Effect of Dry-Ice Blasting and Substrate Preheating on Plasma-Sprayed CoNiCrAlY Splats. J. Therm. Spray Technol. 2013, 22, 61–68. [Google Scholar] [CrossRef]
- Dzido, A.; Krawczyk, P.; Badyda, K.; Chondrokostas, P. Operational parameters impact on the performance of dry-ice blasting nozzle. Energy 2021, 214, 118847. [Google Scholar] [CrossRef]
- Li, M.-Z.; Liu, W.-W.; Qing, X.-C.; Yu, Y.; Liu, L.-H.; Tang, Z.-J.; Wang, H.-J.; Dong, Y.-Z.; Zhang, H.-C. Feasibility study of a new approach to removal of paint coatings in remanufacturing. J. Mater. Process. Technol. 2016, 234, 102. [Google Scholar] [CrossRef]
- Liu, Y.; Hirama, D.; Matusaka, S. Particle removal process during application of impinging dry ice jet. Powder Technol. 2017, 217, 607–613. [Google Scholar] [CrossRef]
- Masa, V.; Kuba, P.; Perilak, D.; Lokaj, J. Decrease in Consumption of Compressed Air in Dry Ice Blasting Machine. Chem. Eng. Trans. 2014, 39, 805–810. [Google Scholar] [CrossRef]
- Masa, V.; Kuba, P. Efficient use of compressed air for dry ice blasting. J. Clean. Prod. 2016, 111, 76–84. [Google Scholar] [CrossRef]
- Muckenhaupt, D.; Zutzmann, T.; Rudek, A.; Russ, G. An Experimental and Numerical Procedure for Energetic and Acoustic Optimization of Dry-ice Blasting Processes. Chem. Eng. Trans. 2019, 74, 967–972. [Google Scholar] [CrossRef]
- Otto, C.; Zahn, S.; Rost, F.; Zahn, P.; Jaros, D.; Rohm, H. Physical Methods of cleaning and Disinfection of Surfaces. Food Eng. Rev. 2011, 3, 171–188. [Google Scholar] [CrossRef]
- Spur, G.; Uhlmann, E.; Elbing, F. Dry-ice blasting for cleaning: Process, optimization and application. Wear 1999, 233–235, 402–411. [Google Scholar] [CrossRef]
- Witte, A.K.; Bobal, M.; David, R.; Blaettler, B.; Schoder, D.; Rossmanith, P. Investigation of the potential of dry ice blasting for cleaning and disinfection in the food production environment. LWT—Food Sci. Technol. 2017, 75, 735–741. [Google Scholar] [CrossRef]
- Górecki, J.; Talaśka, K.; Wałęsa, K.; Wilczyński, D.; Wojtkowiak, D. Mathematical Model Describing the Influence of Geometrical Parameters of Multichannel Dies on the Limit Force of Dry Ice Extrusion Process. Materials 2020, 13, 3317. [Google Scholar] [CrossRef]
- Ishiguro, M.; Kaneko, S.I.; Tajiri, T. Snow Consolidation Properties by using Mechanical Press Machine. J. Inst. Ind. Appl. Eng. 2020, 7, 83–90. [Google Scholar] [CrossRef]
- Kukla, M.; Górecki, J.; Malujda, I.; Talaśka, K.; Tarkowski, P. The determination of mechanical properties of magnetorheological elastomers (MREs). Procedia Eng. 2017, 177, 324–330. [Google Scholar] [CrossRef]
- Wojtkowiak, D.; Talaśka, K.; Wilczyński, D.; Górecki, J.; Wałęsa, K. Determining the Power Consumption of the Automatic Device for Belt Perforation Based on the Dynamic Model. Energies 2021, 14, 317. [Google Scholar] [CrossRef]
- Wilczyński, D.; Berdychowski, M.; Talaśka, K.; Wojtkowiak, D. Experimental and numerical analysis of the effect of compaction conditions on briquette properties. Fuel 2021, 288, 119613. [Google Scholar] [CrossRef]
- Berdychowski, M.; Talaśka, K.; Wilczyński, D. Evaluation of the possibility of using the Drucker-Prager-Cap model in simulations of the densification process of shredded natural materials. MATEC Web Conf. 2019, 254, 02018. [Google Scholar] [CrossRef][Green Version]
- Berdychowski, M.; Talaśka, K.; Malujda, I.; Kukla, M. Application of the Mohr-Coulomb model for simulating the biomass compaction process. IOP Conf. Ser. Mater. Sci. Eng. 2020, 776, 012066. [Google Scholar] [CrossRef]
- Berdychowski, M.; Wilczyński, D.; Talaśka, K.; Wojtkowiak, D. The Cam-Clay model in the application of technological process modeling. IOP Conf. Ser. Mater. Sci. Eng. 2020, 776, 012067. [Google Scholar] [CrossRef]
- Abaqus Documentation; Dassault Systemes: Paris, France, 2017.
- Johnson, P.; Cenkowski, S.; Paliwal, J. Compaction and relaxation characteristics of single compacts produced form distiller’s spent grain. J. Food Eng. 2013, 116, 260–266. [Google Scholar] [CrossRef]
- Górecki, J.; Malujda, I.; Talaśka, K.; Kukla, M.; Tarkowski, P. Static compression tests of concentrated crystallized carbon dioxide. Appl. Mech. Mater. 2015, 816, 490–495. [Google Scholar] [CrossRef]
- Buljak, V.; Baivier-Romero, S.; Kallel, A. Calibration of Drucker–Prager Cap Constitutive Model for Ceramic Powder Compaction through Inverse Analysis. Materials 2021, 14, 4044. [Google Scholar] [CrossRef]
- Sinha, T.; Curtis, J.; Hancock, B.; Wassgren, C. A study on the sensitivity of Drucker–Prager Cap model parameters during the decompression phase of powder compaction simulations. Powder Technol. 2010, 198, 315–324. [Google Scholar] [CrossRef]
- Prakash, M.; Cleary, P. Modelling highly deformable metal extrusion using SPH. Comput. Part. Mech. 2015, 2, 19–38. [Google Scholar] [CrossRef][Green Version]
- Zhang, B.; Jain, M.; Zhao, C.; Bruhis, M.; Lawcock, R.; Ly, K. Experimental calibration of density-dependent modified Drucker-Prager/Cap model using an instrumented cubic die for powder compact. Powder Technol. 2010, 204, 27–41. [Google Scholar] [CrossRef]
- Diarra, H.; Mazel, V.; Boillon, A.; Rehault, L.; Busignies, V.; Bereau, S.; Tchoreloff, P. Finite Element Method (FEM) modeling of the powder compaction of cosmetic products: Comparison between simulated and experimental result. Powder Technol. 2012, 224, 233–240. [Google Scholar] [CrossRef]

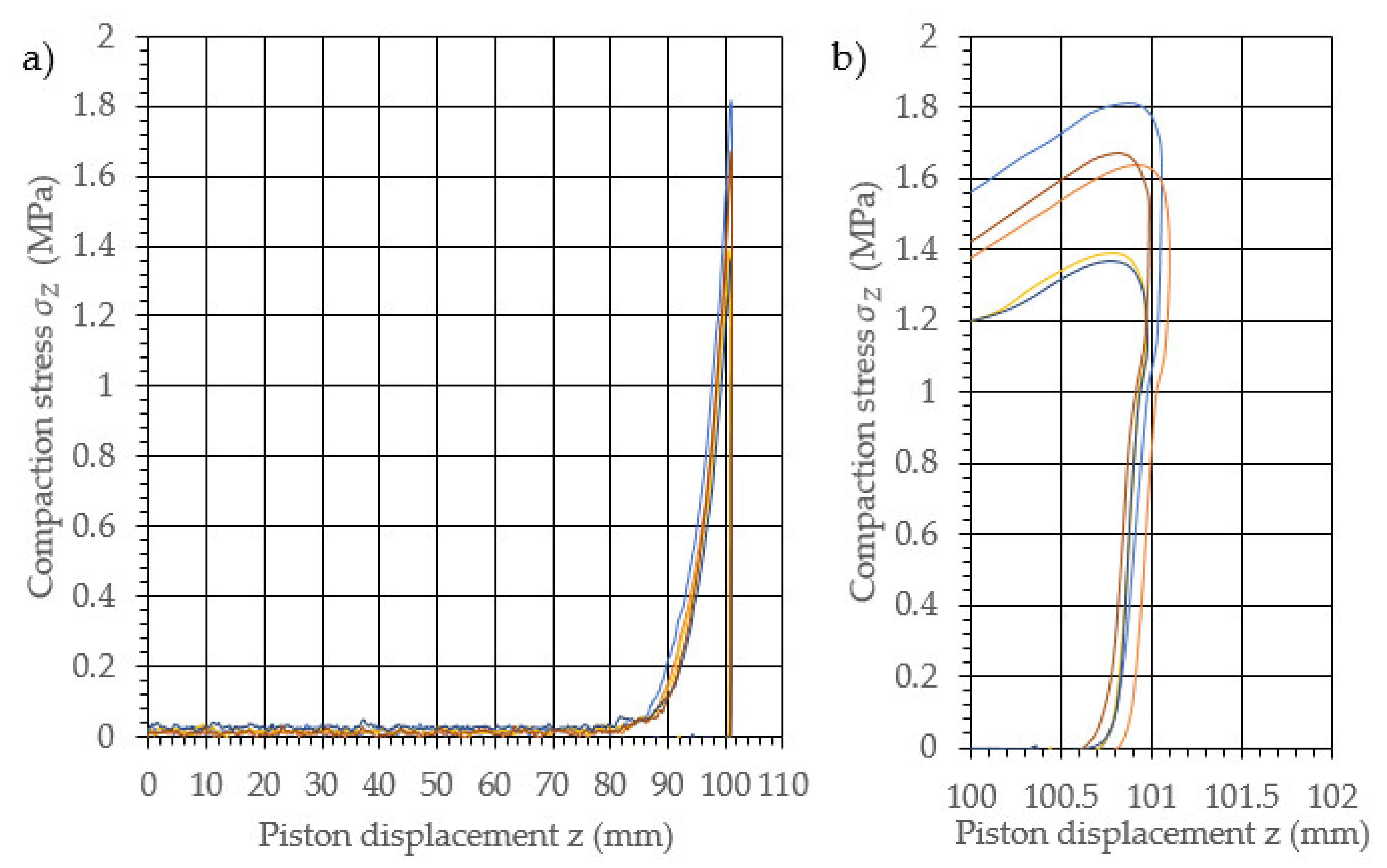
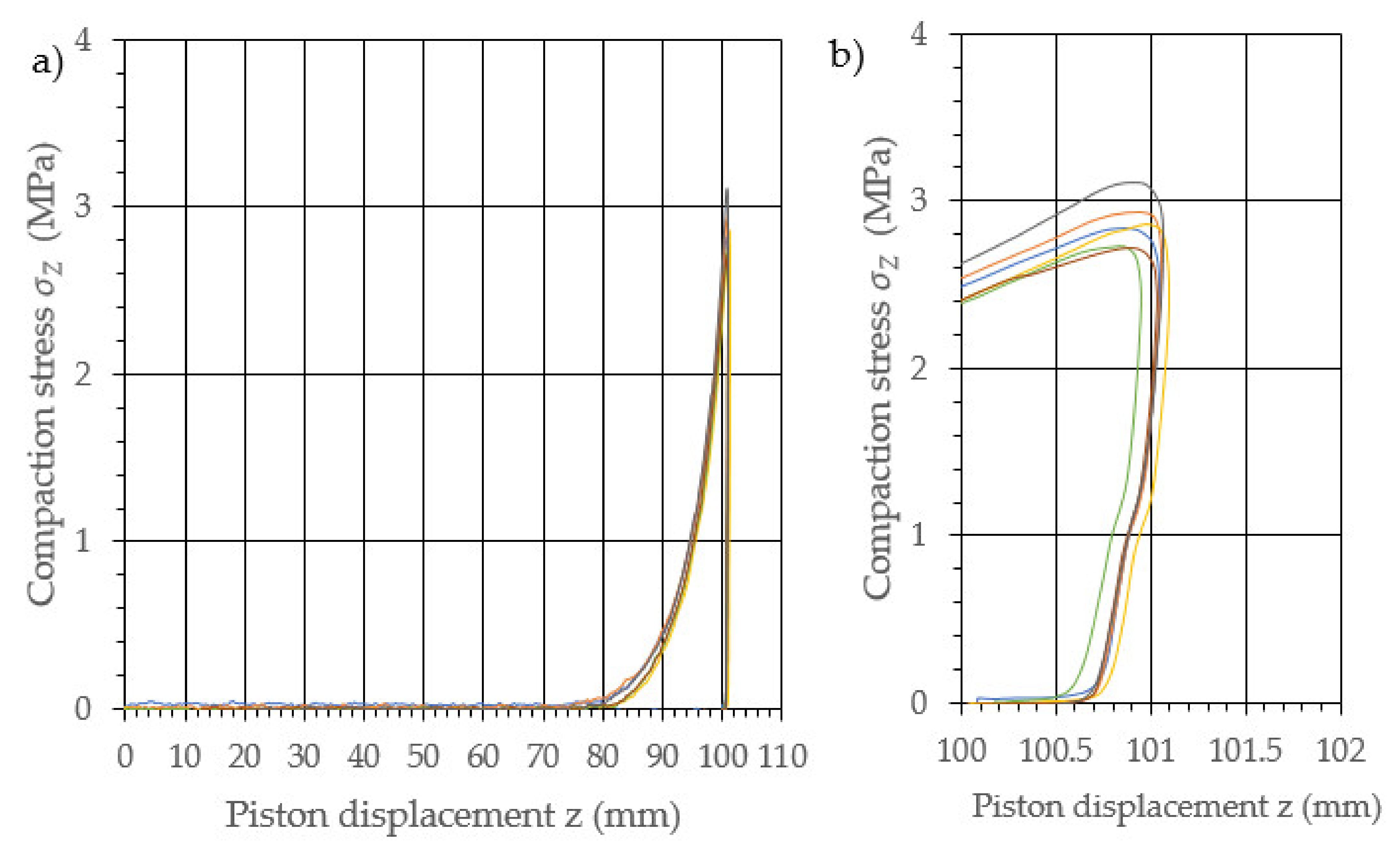

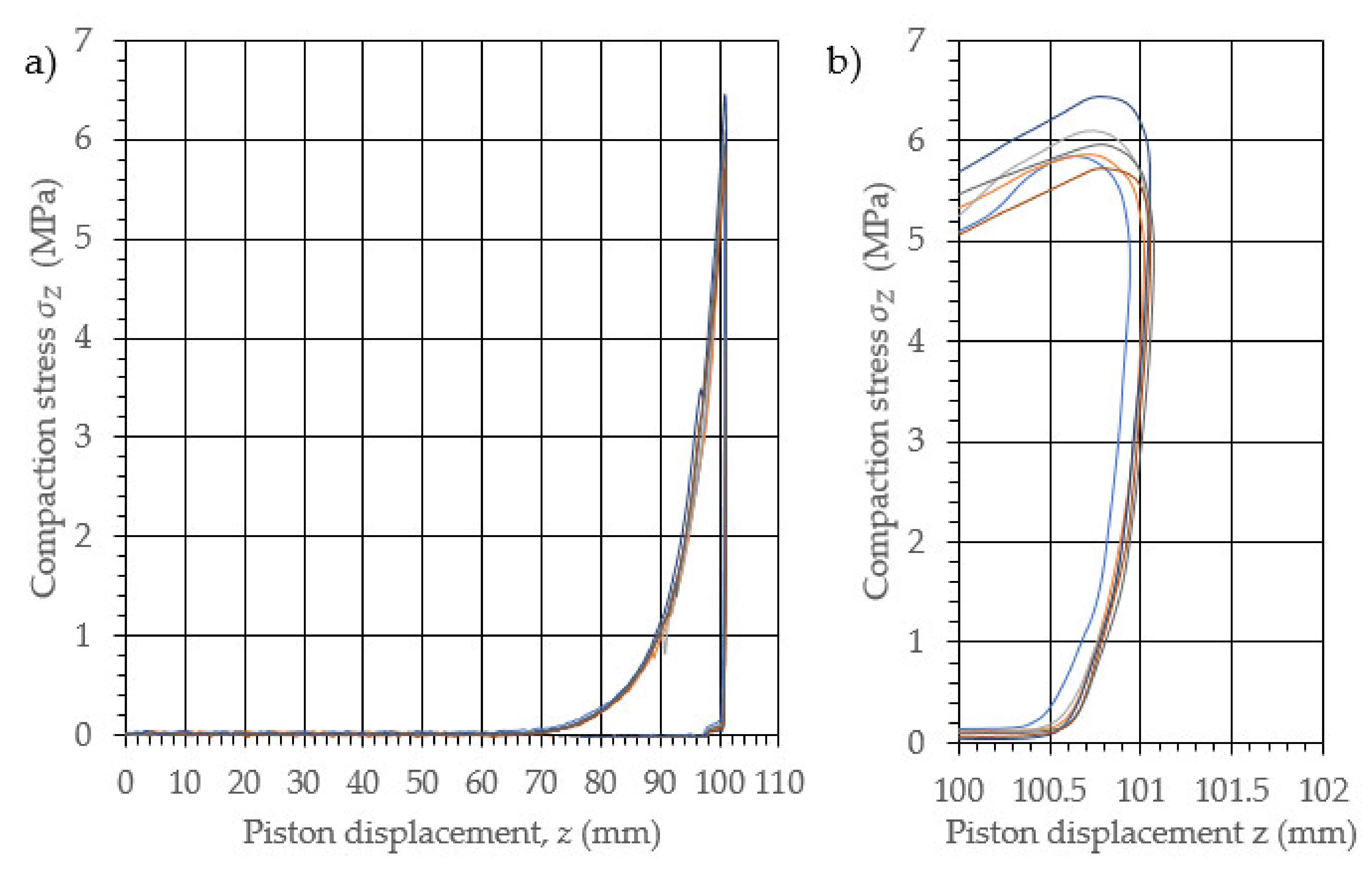
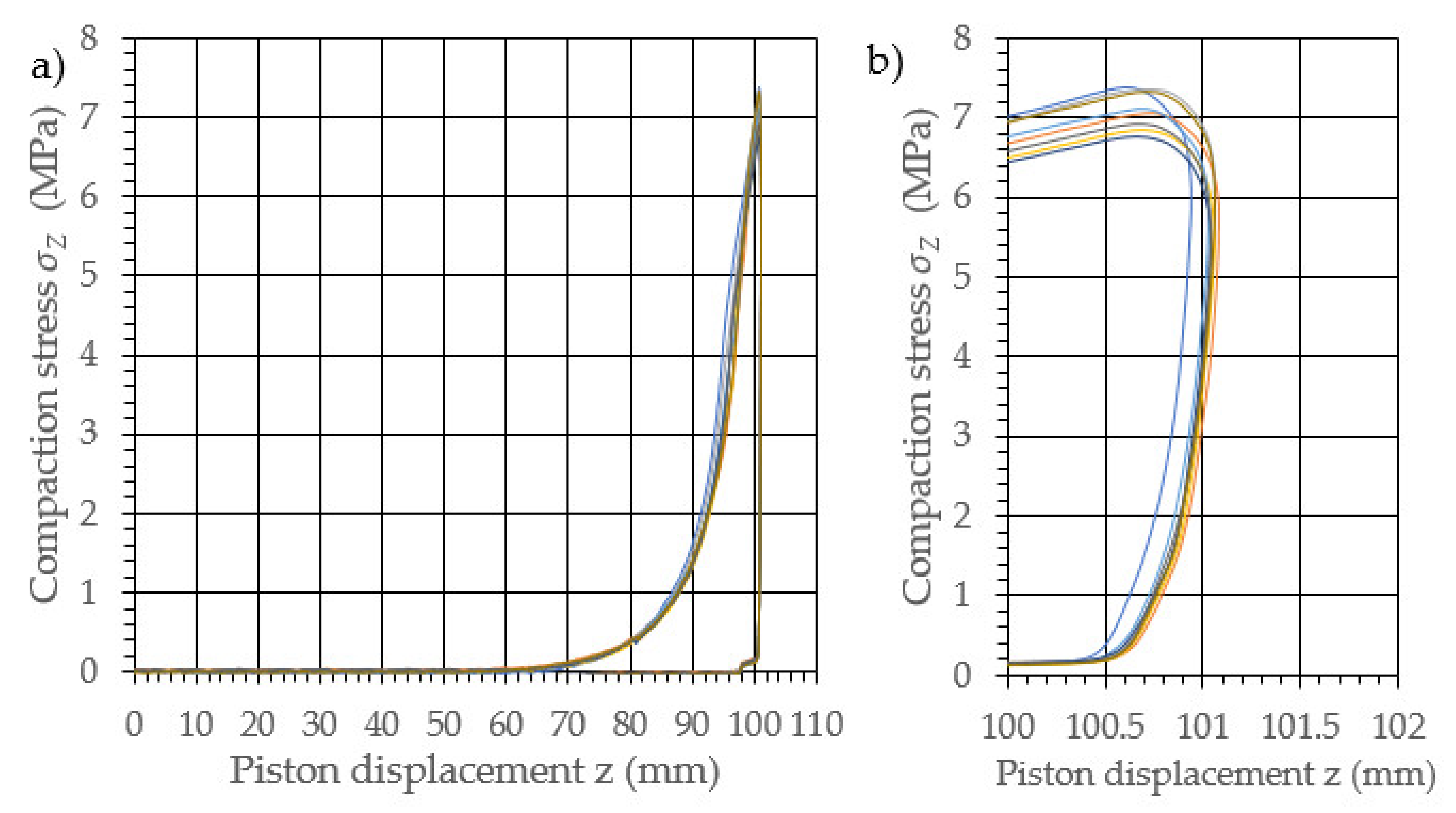
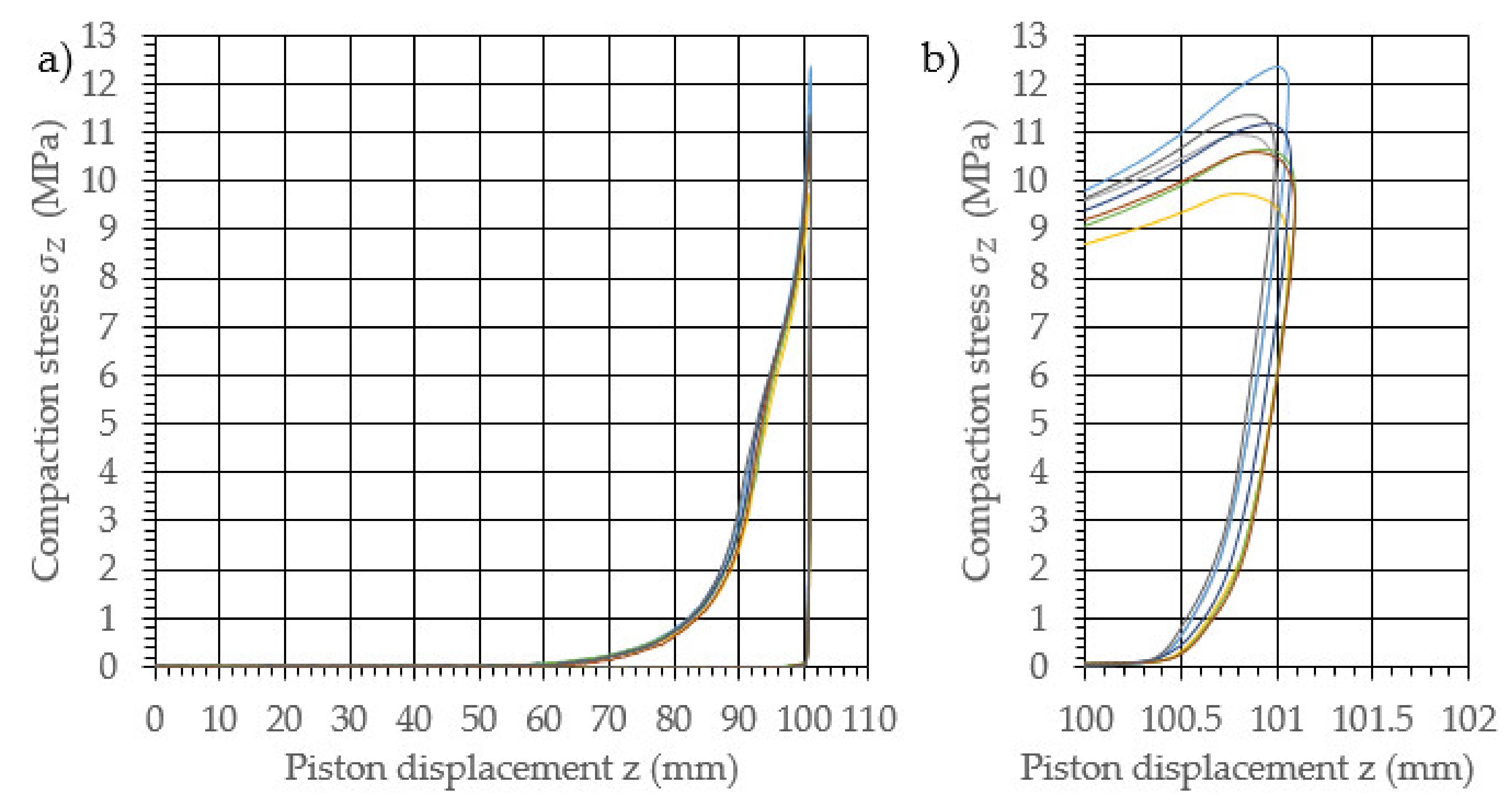
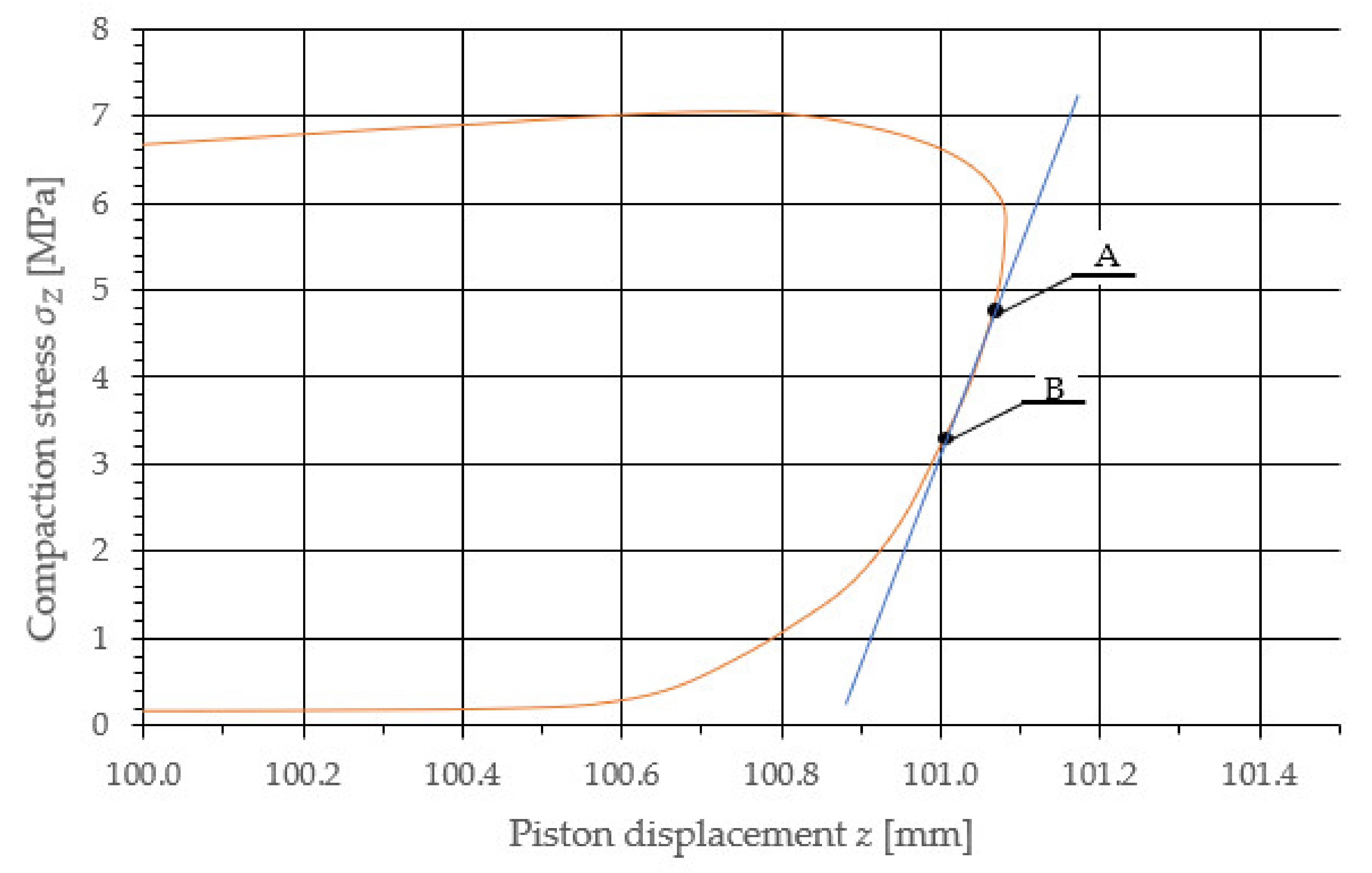
| m0 (g) | d (mm) | |||
|---|---|---|---|---|
| 20 | 18.06 (0.32) | 30 | 23.99 (0.04) | 1065.0 (20.69) |
| 22 | 19.73 (0.22) | 30 | 23.96 (0.04) | 1164.9 (12.78) |
| 24 | 21.27 (0.29) | 30 | 23.98 (0.06) | 1255.2 (17.79) |
| 26 | 22.39 (0.55) | 30 | 23.96 (0.04) | 1321.3 (33.26) |
| 28 | 23.94 (0.52) | 30 | 23.98 (0.05) | 1412.3 (28.97) |
| 30 | 26.66 (0.36) | 30 | 23.95 (0.03) | 1574.5 (20.66) |
| ρ (kg/m3) | (kg/m3) | (MPa) | (MPa) |
|---|---|---|---|
| 1065.0 | 20.69 | 120.82 | 12.9 |
| 1164.9 | 12.78 | 228.18 | 20.47 |
| 1255.2 | 17.79 | 411.84 | 24.23 |
| 1321.3 | 33.26 | 525.03 | 60.07 |
| 1412.3 | 28.97 | 576.73 | 25.55 |
| 1574.5 | 20.66 | 788.13 | 63.09 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Biszczanik, A.; Wałęsa, K.; Kukla, M.; Górecki, J. The Influence of Density on the Value of Young’s Modulus for Dry Ice. Materials 2021, 14, 7763. https://doi.org/10.3390/ma14247763
Biszczanik A, Wałęsa K, Kukla M, Górecki J. The Influence of Density on the Value of Young’s Modulus for Dry Ice. Materials. 2021; 14(24):7763. https://doi.org/10.3390/ma14247763
Chicago/Turabian StyleBiszczanik, Aleksandra, Krzysztof Wałęsa, Mateusz Kukla, and Jan Górecki. 2021. "The Influence of Density on the Value of Young’s Modulus for Dry Ice" Materials 14, no. 24: 7763. https://doi.org/10.3390/ma14247763
APA StyleBiszczanik, A., Wałęsa, K., Kukla, M., & Górecki, J. (2021). The Influence of Density on the Value of Young’s Modulus for Dry Ice. Materials, 14(24), 7763. https://doi.org/10.3390/ma14247763








