A Phase-Field Study of Microstructure Evolution in Tungsten Polycrystalline under He/D Irradiation
Abstract
1. Introduction
2. Materials and Methods
2.1. The Calculation of Fractal Dimension
2.2. Phase-Field Model
2.2.1. Chemical Free Energy Density
2.2.2. Numerical Implementation
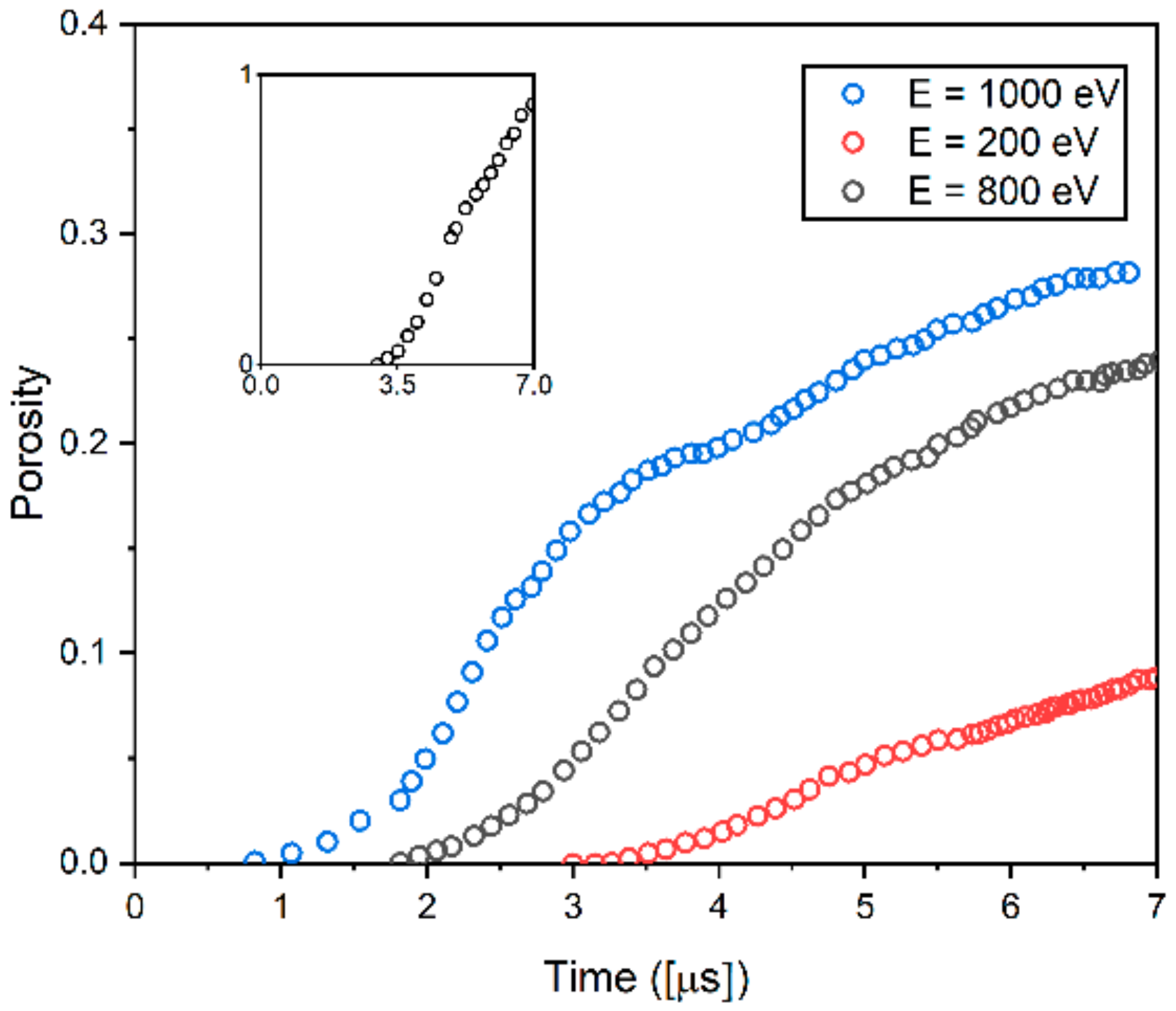
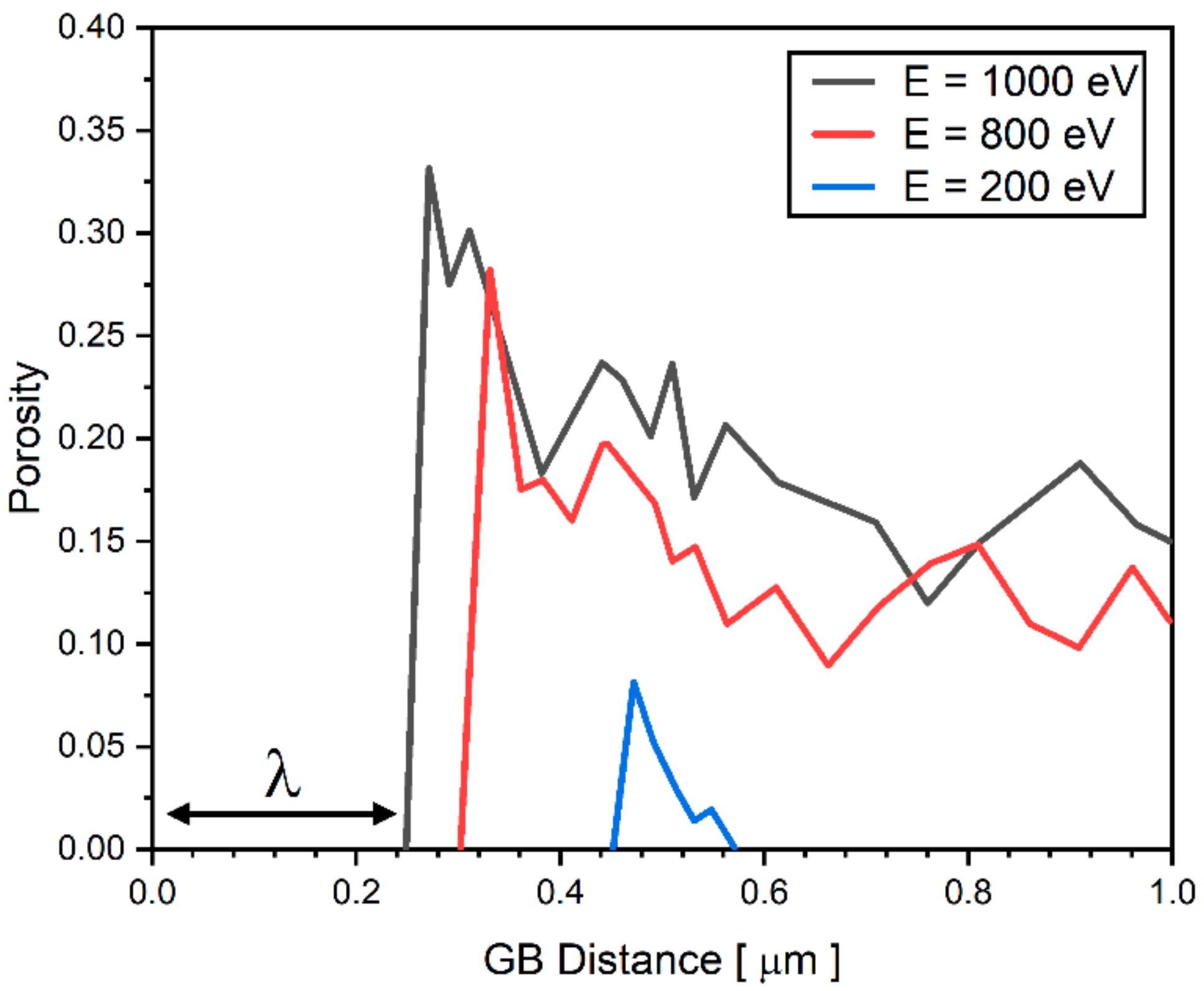
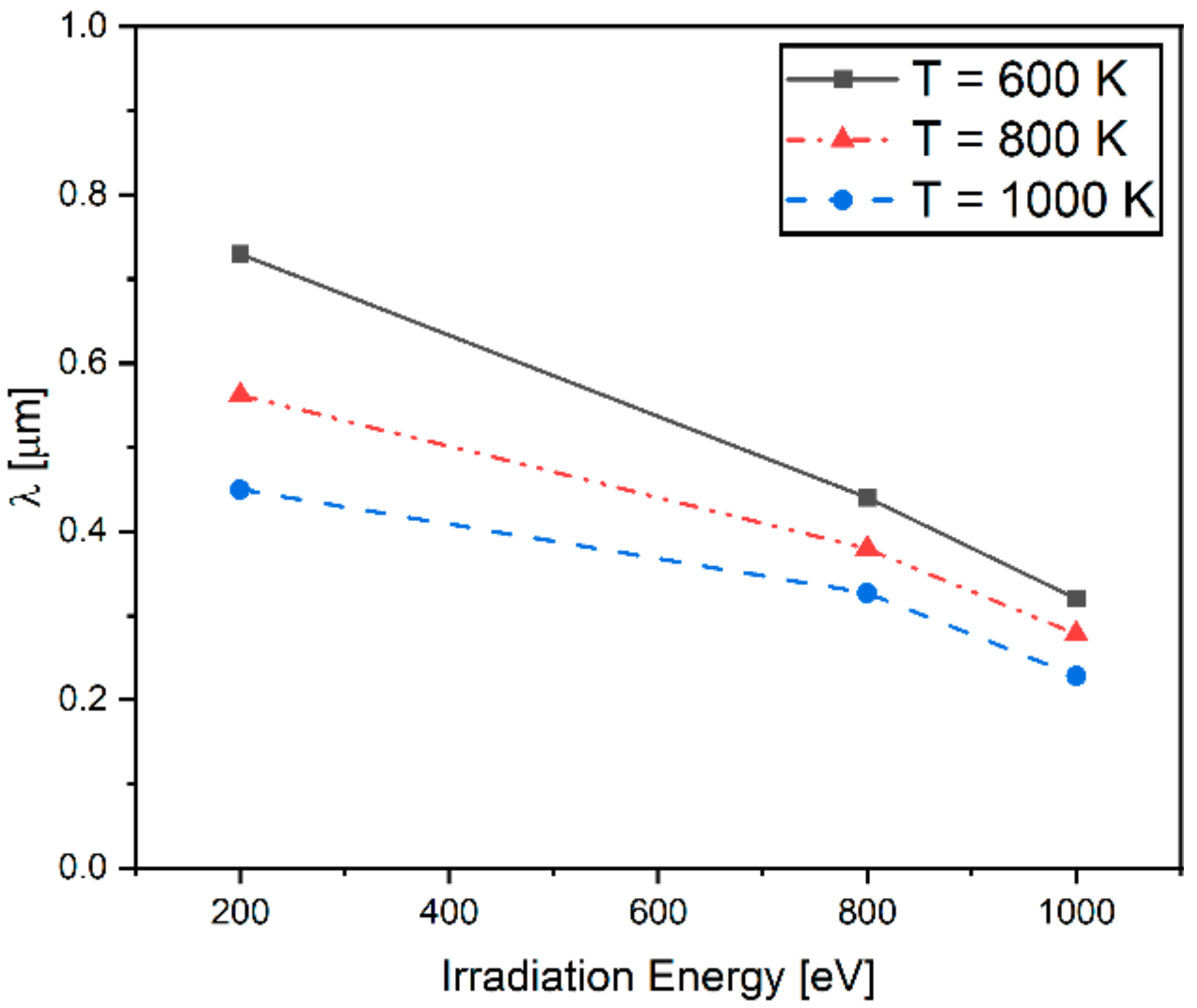
3. Results
4. Discussion
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Baluc, N.; Abe, K.; Boutard, J.L.; Chernov, V.M.; Diegele, E.; Jitsukawa, S.; Kimura, A.; Klueh, R.L.; Kohyama, A.; Kurtz, R.J.; et al. Status of R&D activities on materials for fusion power reactors. Nucl. Fusion 2007, 47, S696. [Google Scholar]
- Greenwood, L.R. Neutron interactions and atomic recoil spectra. J. Nucl. Mater. 1994, 216, 29–44. [Google Scholar] [CrossRef]
- Dubinko, V.I. The influence of dislocation structure and impurities on the void lattice formation in crystals under irradiation. J. Nucl. Mater. 1991, 178, 108–113. [Google Scholar] [CrossRef]
- Dubinko, V.I.; Tur, A.V.; Turkin, A.A.; Yanovskij, V.V. A mechanism of formation and properties of the void lattice in metals under irradiation. J. Nucl. Mater. 1989, 161, 57–71. [Google Scholar] [CrossRef]
- Abe, K.; Masuyama, T.; Satou, M.; Hamilton, M.L. Neutron irradiation damage and void lattice formation in a molybdenum alloy TZM. Mater. Trans. JIM 1993, 34, 1137–1142. [Google Scholar] [CrossRef]
- Jäger, W.; Trinkaus, H. Defect ordering in metals under irradiation. J. Nucl. Mater. 1993, 205, 394–410. [Google Scholar] [CrossRef]
- Dubinko, V.I.; Turkin, A.A. Self-organization of cavities under irradiation. Appl. Phys. A Solids Surf. 1994, 58, 21–34. [Google Scholar] [CrossRef]
- Ghoniem, N.M.; Walgraef, D.; Zinkle, S.J. Theory and experiment of nanostructure self-organization in irradiated materials. J. Comput. Aided Mater. Des. 2001, 8, 1–38. [Google Scholar] [CrossRef]
- Hu, S.Y.; Henager, C.H., Jr. Phase-field simulation of void migration in a temperature gradient. Acta Mater. 2010, 58, 3230–3237. [Google Scholar] [CrossRef]
- Aranson, I.S.; Kalatsky, V.A.; Vinokur, V.M. Continuum field description of crack propagation. Phys. Rev. Lett. 2000, 85, 118–121. [Google Scholar] [CrossRef] [PubMed]
- Karma, A.; Kessler, D.A.; Levine, H. Phase-field model of mode III dynamic fracture. Phys. Rev. Lett. 2001, 87, 045501. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Khachaturyan, A.G. Three-dimensional field model and computer modeling of martensitic transformations. Acta Mater. 1997, 45, 759–773. [Google Scholar] [CrossRef]
- Jin, Y.M.; Artemev, A.; Khachaturyan, A.G. Three-dimensional phase field model of low-symmetry martensitic transformation in polycrystal: Simulation of zeta ′(2) martensite in AuCd alloys. Acta Mater. 2001, 49, 2309–2320. [Google Scholar] [CrossRef]
- Kazaryan, A.; Wang, Y.; Dregia, S.A.; Patton, B.R. Generalized phase-field model for computer simulation of grain growth in anisotropic systems. Phys. Rev. B 2000, 61, 14275–14278. [Google Scholar] [CrossRef]
- Cahn, J.W.; Hilliard, J.E. Free Energy of a Nonuniform System. I. Interfacial Free Energy. J. Chem. Phys. 1958, 28, 258–267. [Google Scholar] [CrossRef]
- Hu, S.; Henager, C.H., Jr. Phase-field simulations of Te-precipitate morphology and evolution kinetics in Te-rich CdTe crystals. J. Cryst. Growth 2009, 311, 3184–3194. [Google Scholar] [CrossRef]
- Hu, S.; Henager, C.H., Jr. Phase-field modeling of void lattice formation under irradiation. J. Nucl. Mater. 2009, 394, 155–159. [Google Scholar] [CrossRef]
- Millett, P.C.; El-Azab, A.; Rokkam, S.; Tonks, M.; Wolf, D. Phase-field simulation of irradiated metals: Part I: Void kinetics. Comput. Mater. Sci. 2011, 50, 949–959. [Google Scholar] [CrossRef]
- Millett, P.C.; El-Azab, A.; Wolf, D. Phase-field simulation of irradiated metals: Part II: Gas bubble kinetics. Comput. Mater. Sci. 2011, 50, 960–970. [Google Scholar] [CrossRef]
- Crosby, T.; Ghoniem, N.M. Multiphysics model of thermomechanical and helium-induced damage of tungsten during plasma heat transients. J. Nucl. Mater. 2013, 442, S261–S266. [Google Scholar] [CrossRef]
- Crosby, T.; Ghoniem, N. Phase-field modeling of thermomechanical damage in tungsten under severe plasma transients. Comput. Mech. 2012, 50, 159–168. [Google Scholar] [CrossRef]
- Han, Y.S.; Tomar, V. An ab initio study of the peak tensile strength of tungsten with an account of helium point defects. Int. J. Plast. 2013, 48, 54–71. [Google Scholar] [CrossRef]
- Moisy, F. Matlab Codes for the Calculation of a Fractal Dimension; The MathWorks: Natick, MA, USA, 2008. [Google Scholar]
- Nishijima, D.; Ye, M.Y.; Ohno, N.; Takamura, S. Formation mechanism of bubbles and holes on tungsten surface with low-energy and high-flux helium plasma irradiation in NAGDIS-II. J. Nucl. Mater. 2004, 329, 1029–1033. [Google Scholar] [CrossRef]
- Ohno, N.; Kajita, S.; Nishijima, D.; Takamura, S. Surface modification at tungsten and tungsten coated graphite due to low energy and high fluence plasma and laser pulse irradiation. J. Nucl. Mater. 2007, 363, 1153–1159. [Google Scholar] [CrossRef]
- Kajita, S.; Wataru, S.; Noriyasu, O.; Naoaki, Y.; Tsubasa, S. Formation process of tungsten nanostructure by the exposure to helium plasma under fusion relevant plasma conditions. Nucl. Fusion 2009, 49, 095005. [Google Scholar] [CrossRef]
- Sakaguchi, W.; Kajita, S.; Ohno, N.; Takagi, M. In situ reflectivity of tungsten mirrors under helium plasma exposure. J. Nucl. Mater. 2009, 390, 1149–1152. [Google Scholar] [CrossRef]
- Nishijima, D.; Ye, M.Y.; Ohno, N.; Takamura, S. Incident ion energy dependence of bubble formation on tungsten surface with low energy and high flux helium plasma irradiation. J. Nucl. Mater. 2003, 313, 97–101. [Google Scholar] [CrossRef]
- Kajita, S.; Shuichi, T.; Noriyasu, O.; Dai, N.; Hirotomo, I.; Naoaki, Y. Sub-ms laser pulse irradiation on tungsten target damaged by exposure to helium plasma. Nucl. Fusion 2007, 47, 1358. [Google Scholar] [CrossRef]
- Fu, Z.; Yoshida, N.; Iwakiri, H.; Xu, Z. Thermal desorption and surface modification of He+ implanted into tungsten. J. Nucl. Mater. 2004, 329, 692–696. [Google Scholar] [CrossRef]
- Watanabe, Y.; Iwakiri, H.; Yoshida, N.; Morishita, K.; Kohyama, A. Formation of interstitial loops in tungsten under helium ion irradiation: Rate theory modeling and experiment. Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. At. 2007, 255, 32–36. [Google Scholar] [CrossRef]
- Tokitani, M.; Yoshida, N.; Tokunaga, K.; Sakakita, H.; Kiyama, S.; Koguchi, H.; Hirano, Y.; Masuzaki, S. Microscopic Deformation of Tungsten Surfaces by High Energy and High Flux Helium/Hydrogen Particle Bombardment with Short Pulses. Plasma Fusion Res. 2010, 5, 12. [Google Scholar] [CrossRef][Green Version]
- De Temmerman, G.; Bystrov, K.; Zielinski, J.J.; Balden, M.; Matern, G.; Arnas, C.; Marot, L. Nanostructuring of molybdenum and tungsten surfaces by low-energy helium ions. J. Vac. Sci. Technol. A Vac. Surf. Film. 2012, 30, 041306. [Google Scholar] [CrossRef]
- Cipiti, B.B.; Kulcinski, G.L. Helium and deuterium implantation in tungsten at elevated temperatures. J. Nucl. Mater. 2005, 347, 298–306. [Google Scholar] [CrossRef]
- Dathe, A.; Baveye, P. Dependence of the surface fractal dimension of soil pores on image resolution and magnification. Eur. J. Soil Sci. 2003, 54, 453–466. [Google Scholar] [CrossRef]
- Xu, Q.; Yoshiie, T.; Huang, H.C. Molecular dynamics simulation of vacancy diffusion in tungsten induced by irradiation. Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. At. 2003, 206, 123–126. [Google Scholar] [CrossRef]
- Materials, A.S.f.T.a. Standard Practice for Neutron Radiation Damage Simulation by Charge-Particle Irradiation. In Annual Book of ASTM Standards; ASTM: Philadelphia, PA, USA, 1996. [Google Scholar]
- Doherty, R.D.; Hughes, D.A.; Humphreys, F.J.; Jonas, J.J.; Jensen, D.J.; Kassner, M.E.; King, W.E.; McNelley, T.R.; McQueen, H.J.; Rollett, A.D. Current issues in recrystallization: A review. Mater. Sci. Eng. A 1997, 238, 219–274. [Google Scholar] [CrossRef]
- Hu, S.; Henager, C.H.; Heinisch, H.L.; Stan, M.; Baskes, M.I.; Valone, S.M. Phase-field modeling of gas bubbles and thermal conductivity evolution in nuclear fuels. J. Nucl. Mater. 2009, 392, 292–300. [Google Scholar] [CrossRef]
- Mills, R.L.; Liebenberg, D.H.; Bronson, J.C. Equation of state and melting properties of $^{4}\mathrm{He}$ from measurements to 20 kbar. Phys. Rev. B 1980, 21, 5137–5148. [Google Scholar] [CrossRef]
- Morishita, K.; Sugano, R. Mechanism map for nucleation and growth of helium bubbles in metals. J. Nucl. Mater. 2006, 353, 52–65. [Google Scholar] [CrossRef]
- Trinkaus, H. Energetics and formation kinetics of helium bubbles in metals. Radiat. Eff. 1983, 78, 189–211. [Google Scholar] [CrossRef]
- Liu, Y.-L.; Zhou, H.-B.; Jin, S.; Zhang, Y.; Lu, G.-H. Dissolution and diffusion properties of carbon in tungsten. J. Phys. Condens. Matter 2010, 22, 445504. [Google Scholar] [CrossRef]
- Gupta, V.K.; Yoon, D.-H.; Meyer Iii, H.M.; Luo, J. Thin intergranular films and solid-state activated sintering in nickel-doped tungsten. Acta Mater. 2007, 55, 3131–3142. [Google Scholar] [CrossRef]
- Paul, C.M.; Srujan, R.; Anter, E.-A.; Michael, T.; Dieter, W. Void nucleation and growth in irradiated polycrystalline metals: A phase-field model. Model. Simul. Mater. Sci. Eng. 2009, 17, 064003. [Google Scholar]
- Castro, M. Phase-field approach to heterogeneous nucleation. Phys. Rev. B 2003, 67, 035412. [Google Scholar] [CrossRef]
- Kolmogorov, A.N. On the statistical theory of the crystallization of metals. Bull. Acad. Sci. USSR Math. Ser 1937, 1, 355–359. [Google Scholar]
- Johnson, W.A.; Mehl, R.F. Reaction kinetics in processes of nucleation and growth. Trans. Aime 1939, 135, 396–415. [Google Scholar]
- Melvin, A. Kinetics of Phase Change. I General Theory. J. Chem. Phys. 1939, 7, 1103–1112. [Google Scholar]
- Jelčić, Ž.; Ranogajec, F. Radiation modified high impact polystyrene. Radiat. Phys. Chem. 2012, 81, 1366–1369. [Google Scholar] [CrossRef]
- Misra, A.; Demkowicz, M.; Zhang, X.; Hoagland, R. The radiation damage tolerance of ultra-high strength nanolayered composites. JOM J. Miner. Met. Mater. Soc. 2007, 59, 62–65. [Google Scholar] [CrossRef]
- Demkowicz, M.J.; Hoagland, R.G.; Uberuaga, B.P.; Misra, A. Influence of interface sink strength on the reduction of radiation-induced defect concentrations and fluxes in materials with large interface area per unit volume. Phys. Rev. B 2011, 84, 104102. [Google Scholar] [CrossRef]
- Han, W.Z.; Demkowicz, M.J.; Fu, E.G.; Wang, Y.Q.; Misra, A. Effect of grain boundary character on sink efficiency. Acta Mater. 2012, 60, 6341–6351. [Google Scholar] [CrossRef]
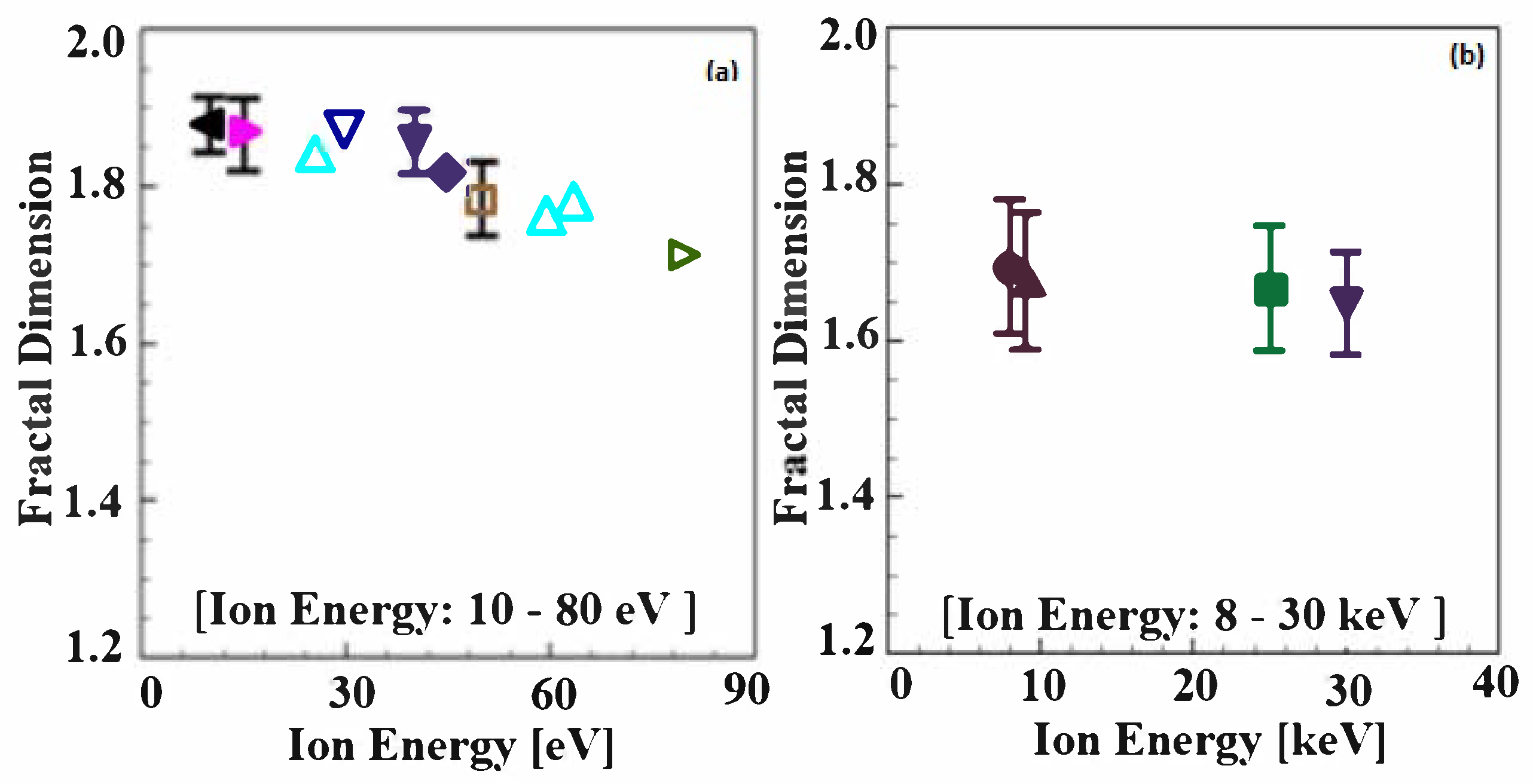
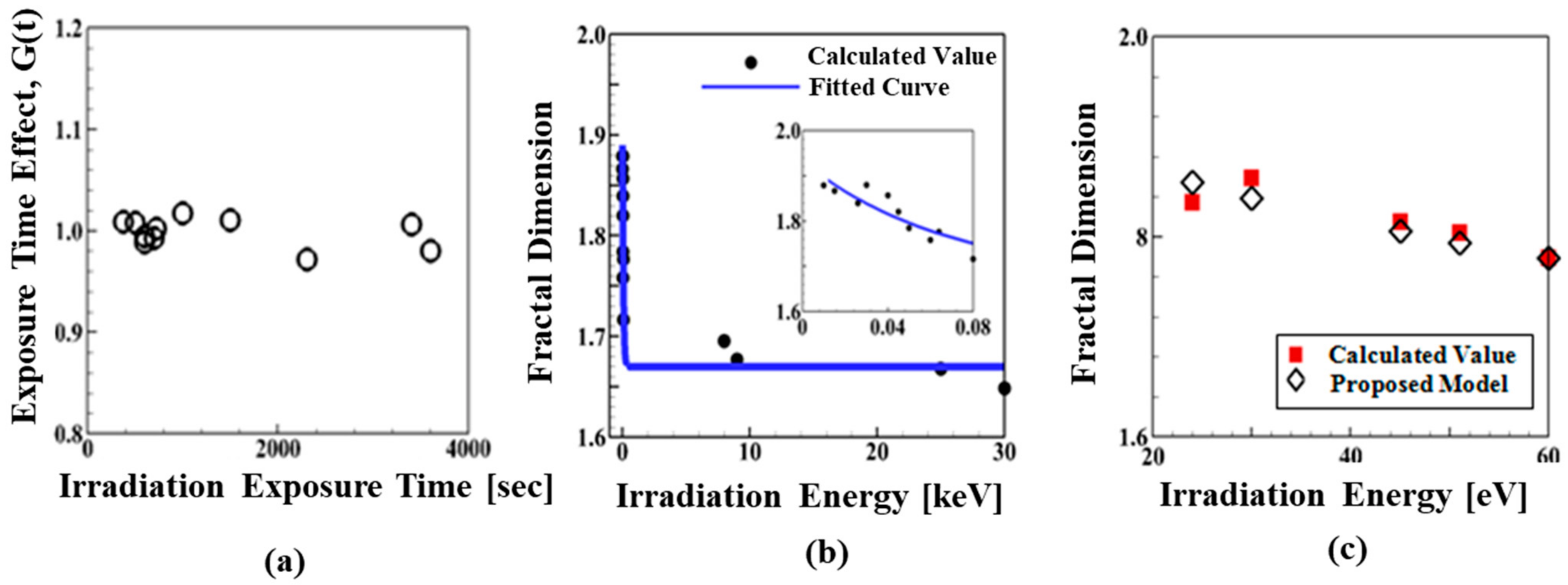
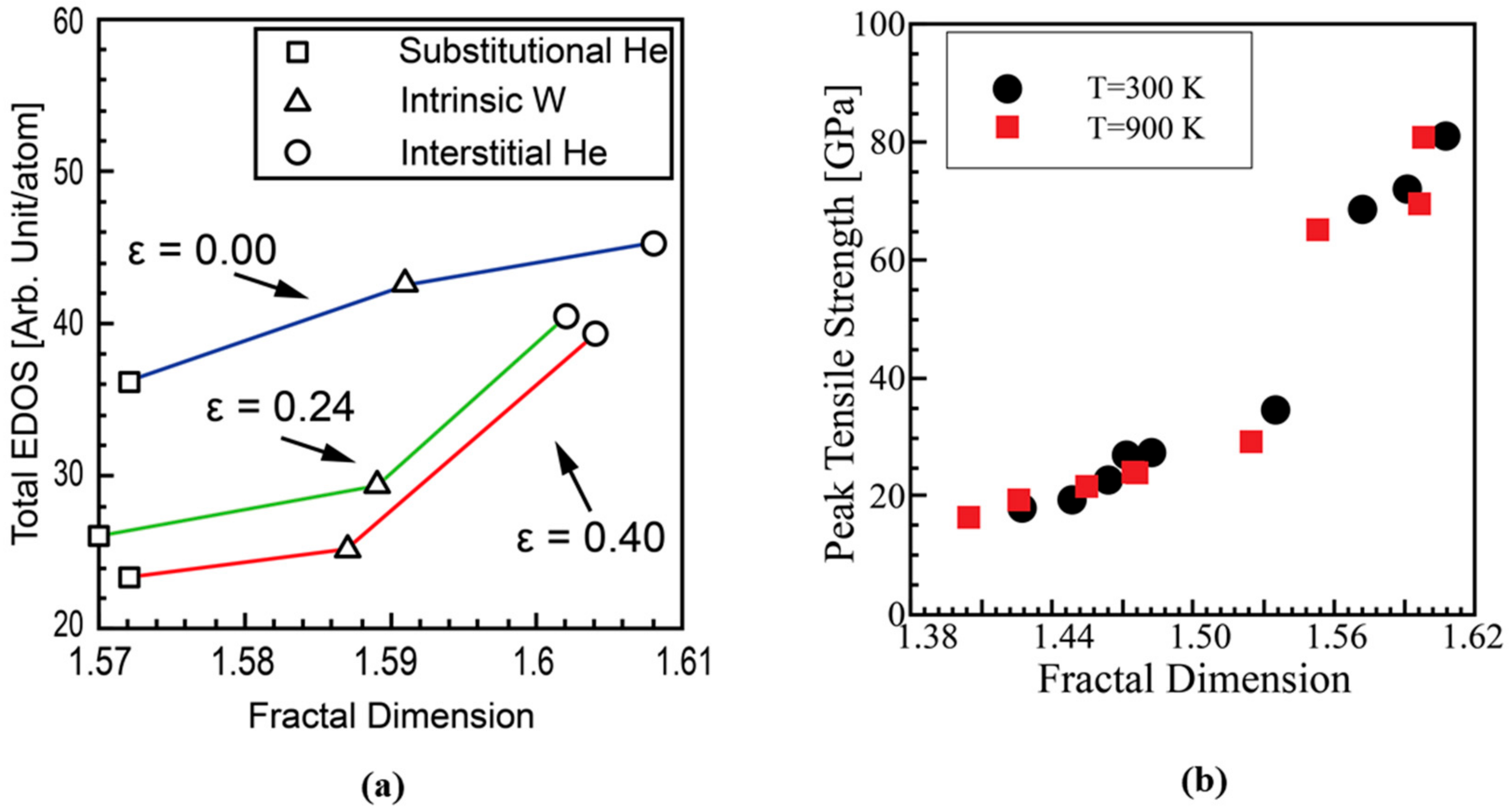

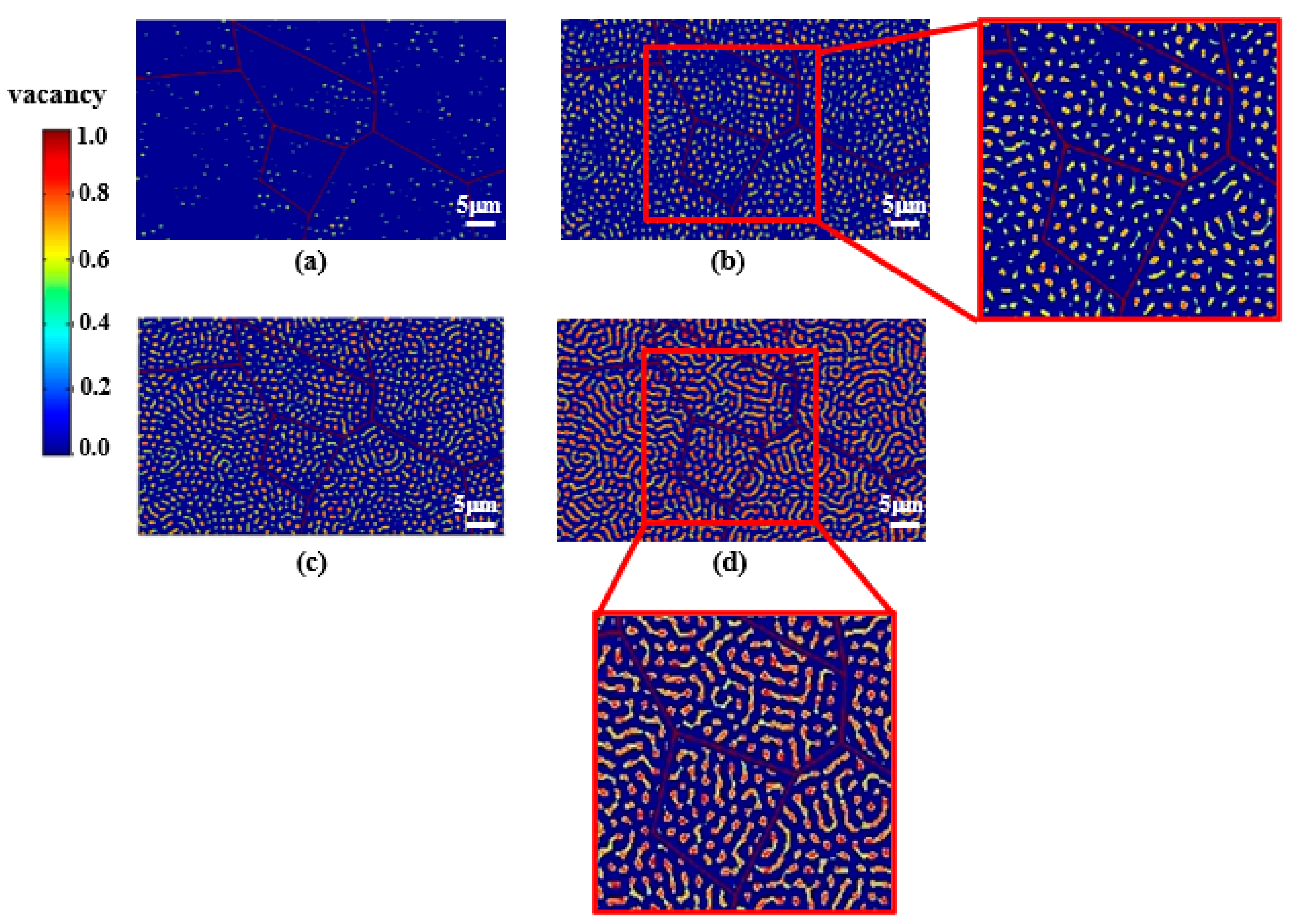
| Irradiation Ion Energy | Surface Temperature [K] | Fluence | Flux | Irradiation Time | Reference |
|---|---|---|---|---|---|
| 10 eV | 1600 | 2.3 × 1026/m2 | [29] | ||
| 10 eV | 2600 | 0.9 × 1027/m2 | 1.2 × 1023/m2 s | 7200 s | [24] |
| 15 eV | 1780 | 3600 s | [27] | ||
| 15 eV | 2200 | 1.0 × 1025/m2 | 8.3 × 1022/m2 s | 1000 s | [25] |
| 26 eV | 1950 | 1.7 × 1026/m2 | 2.6 × 1023/m2 s | 660 s | [28] |
| 30 eV | 2100 | 2.6 × 1027/m2 | 3.7 × 1023/m2 s | 7200 s | [24] |
| 45 eV | 1273 | 3.0 × 1026/m2 | 0.7–3 × 1024/m2 s | 1000 s | [33] |
| 50 eV | 1400 | 6.0 × 1024/m2 | 375 s | [26] | |
| 50 eV | 1890 | 1.7 × 1026/m2 | 7.5 × 1022/m2 s | 2300 s | [27] |
| 60 eV | 2300 | 1.5 × 1026/m2 | 2.6 × 1023/m2 s | 600 s | [28] |
| 64 eV | 2050 | 0.5 × 1026/m2 | 0.6 × 1023/m2 s | 720 s | [28] |
| 80 eV | 2100 | 3.3 × 1026/m2 | 9.2 × 1022/m2 s | 3600 s | [27] |
| 8 keV | 300 | 2.6 × 1019/m2 | 2.6 × 1017/m2 s | [31] | |
| 8 keV | 873 | 4.0 × 1021/m2 | 1.0 × 1021/m2 s | [30] | |
| 25 keV | 1800 | 4.0 × 1022/m2 | 8.8 × 1022/m2 s | [32] | |
| 30 keV | 1173 | 1.0 × 1021/m2 | [34] |
| Temperature [K] | f0 | f1 | f2 | f3 | f4 |
|---|---|---|---|---|---|
| 400 | 0.002 | 0.213 | −0.323 | 1.907 | −1.783 |
| 600 | −1.951 | 3.850 | −2.347 | 2.381 | −1.914 |
| 800 | 0 | 0.185 | −0.278 | 1.627 | −1.521 |
| 900 | 1.985 | −4.172 | 2.284 | 1.067 | −1.127 |
| 1000 | −0.784 | 1.669 | −1.766 | 1.548 | −0.668 |
| 1100 | 1.400 | −2.919 | 1.337 | 0.170 | 0.001 |
| T | 300 K | 400 K | 500 K | 600 K | 700 K | 800 K | 900 K | 1000 K |
|---|---|---|---|---|---|---|---|---|
| Dv | 3.46 × 10−37 | 3.41 × 10−30 | 5.01 × 10−26 | 3.11 × 10−23 | 3.08 × 10−21 | 9.95 × 10−20 | 1.42 × 10−18 | 1.21 × 10−17 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Han, Y.-S. A Phase-Field Study of Microstructure Evolution in Tungsten Polycrystalline under He/D Irradiation. Materials 2021, 14, 7433. https://doi.org/10.3390/ma14237433
Han Y-S. A Phase-Field Study of Microstructure Evolution in Tungsten Polycrystalline under He/D Irradiation. Materials. 2021; 14(23):7433. https://doi.org/10.3390/ma14237433
Chicago/Turabian StyleHan, You-Sung. 2021. "A Phase-Field Study of Microstructure Evolution in Tungsten Polycrystalline under He/D Irradiation" Materials 14, no. 23: 7433. https://doi.org/10.3390/ma14237433
APA StyleHan, Y.-S. (2021). A Phase-Field Study of Microstructure Evolution in Tungsten Polycrystalline under He/D Irradiation. Materials, 14(23), 7433. https://doi.org/10.3390/ma14237433





