Modelling of SMA Vibration Systems in an AVA Example
Abstract
:1. Introduction
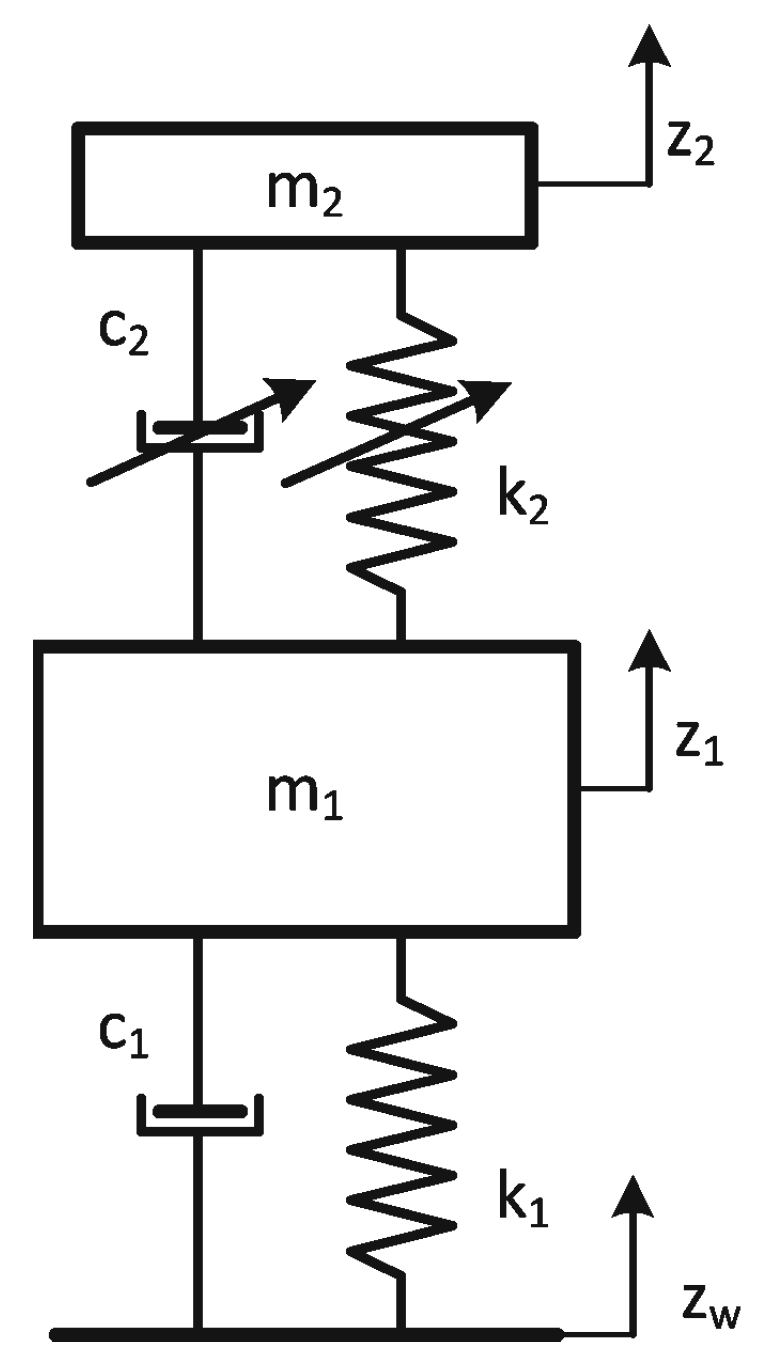
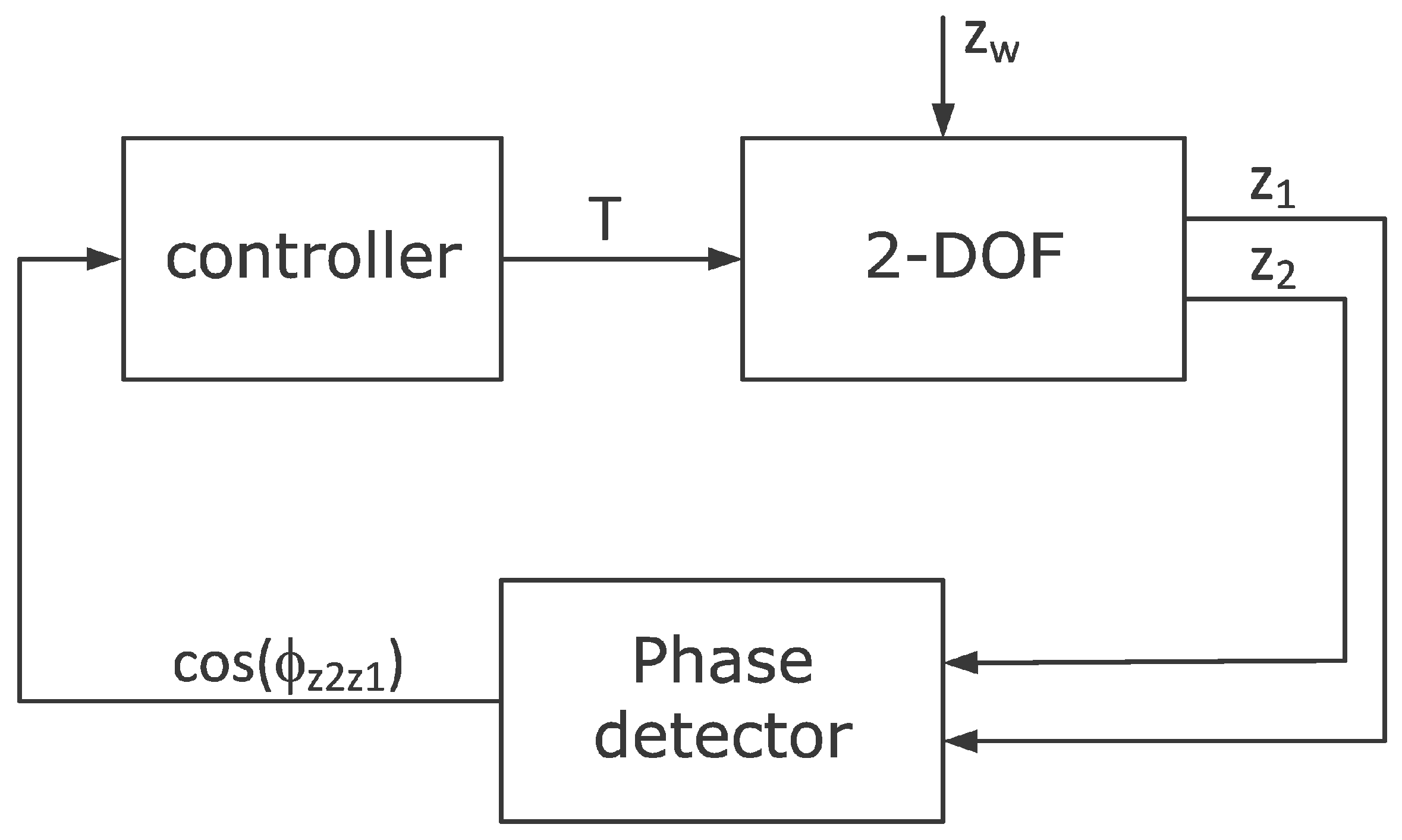
Vibration Systems with SMA Modelling
2. Materials and Methods
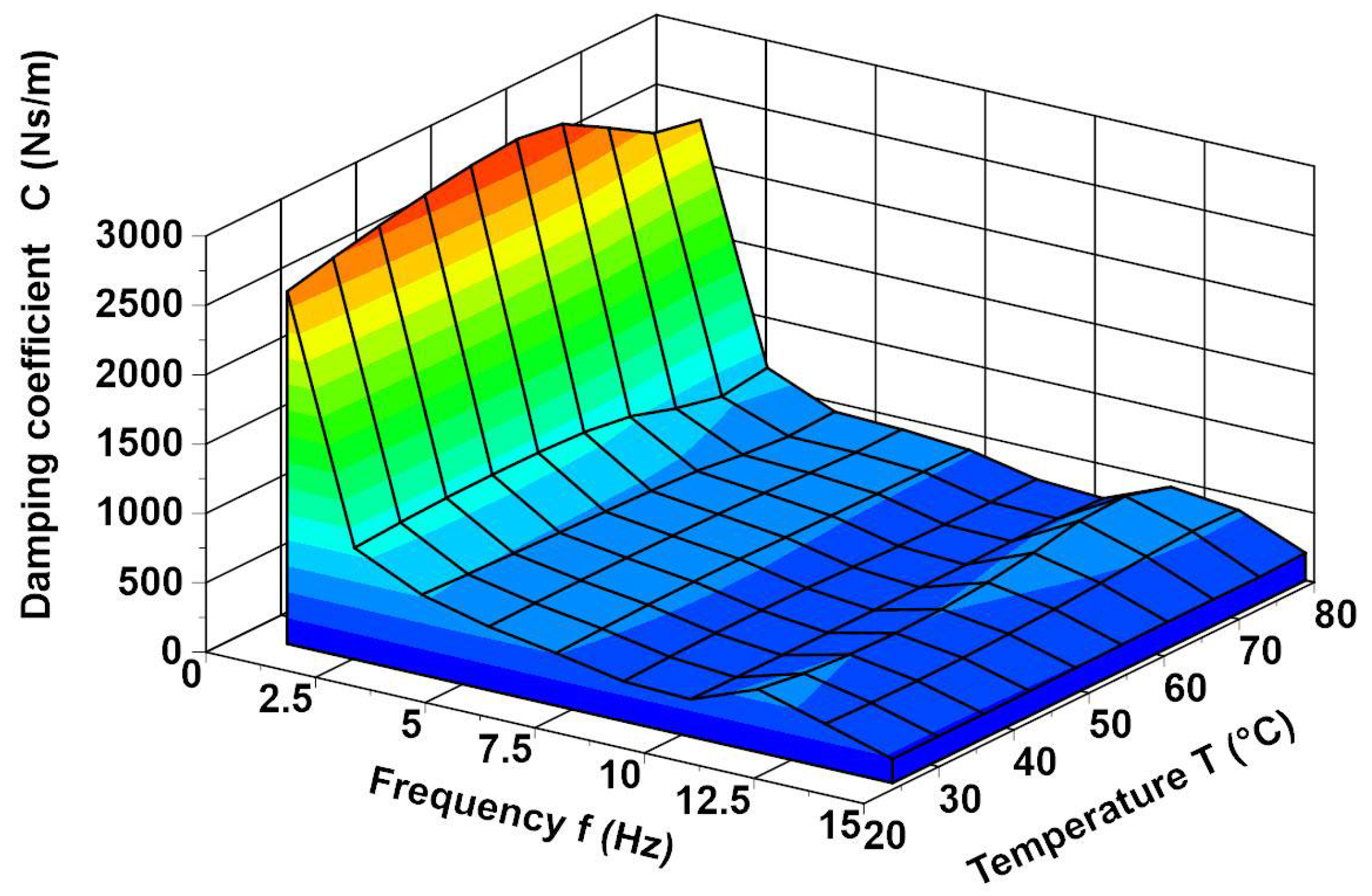
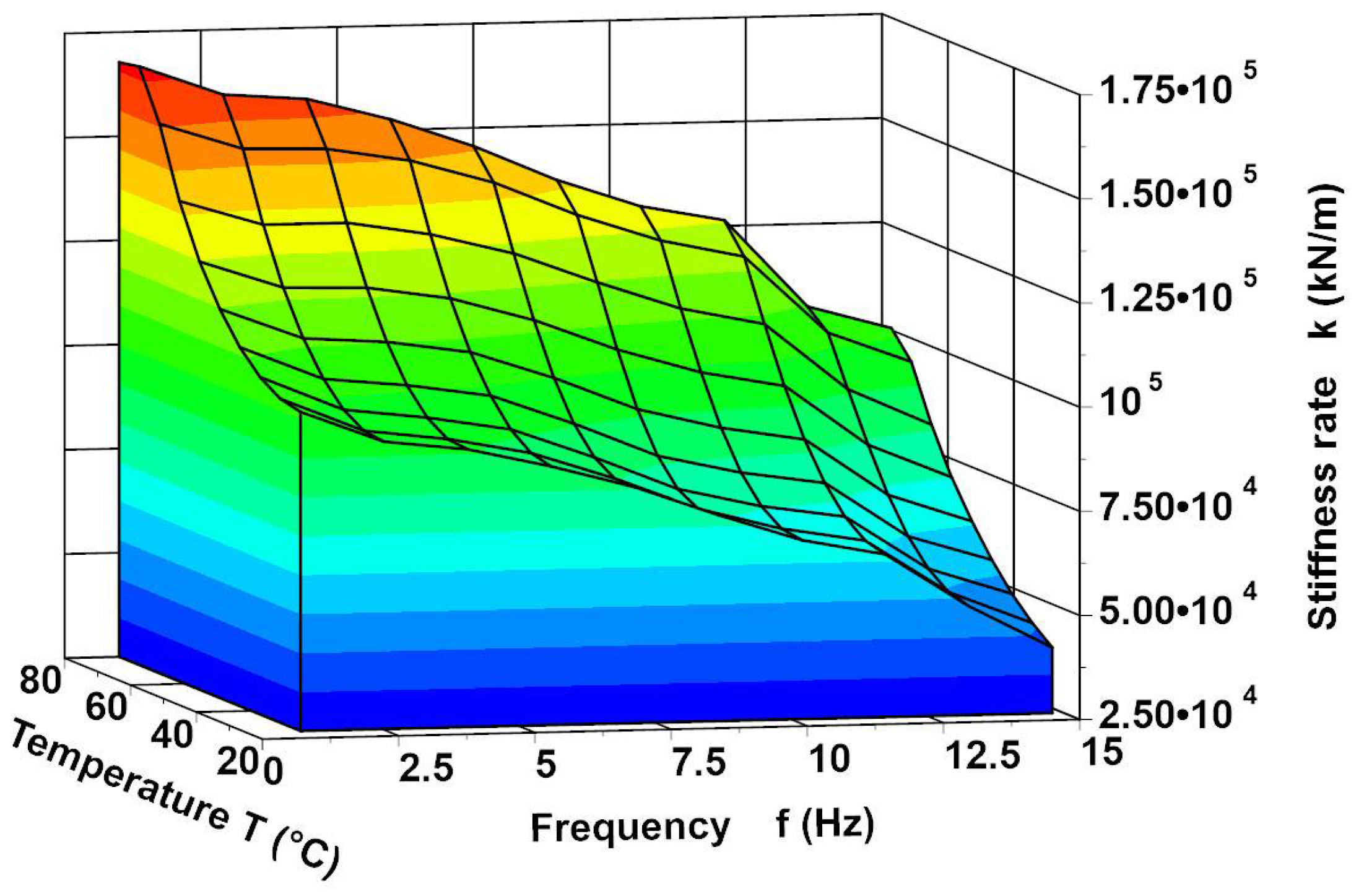
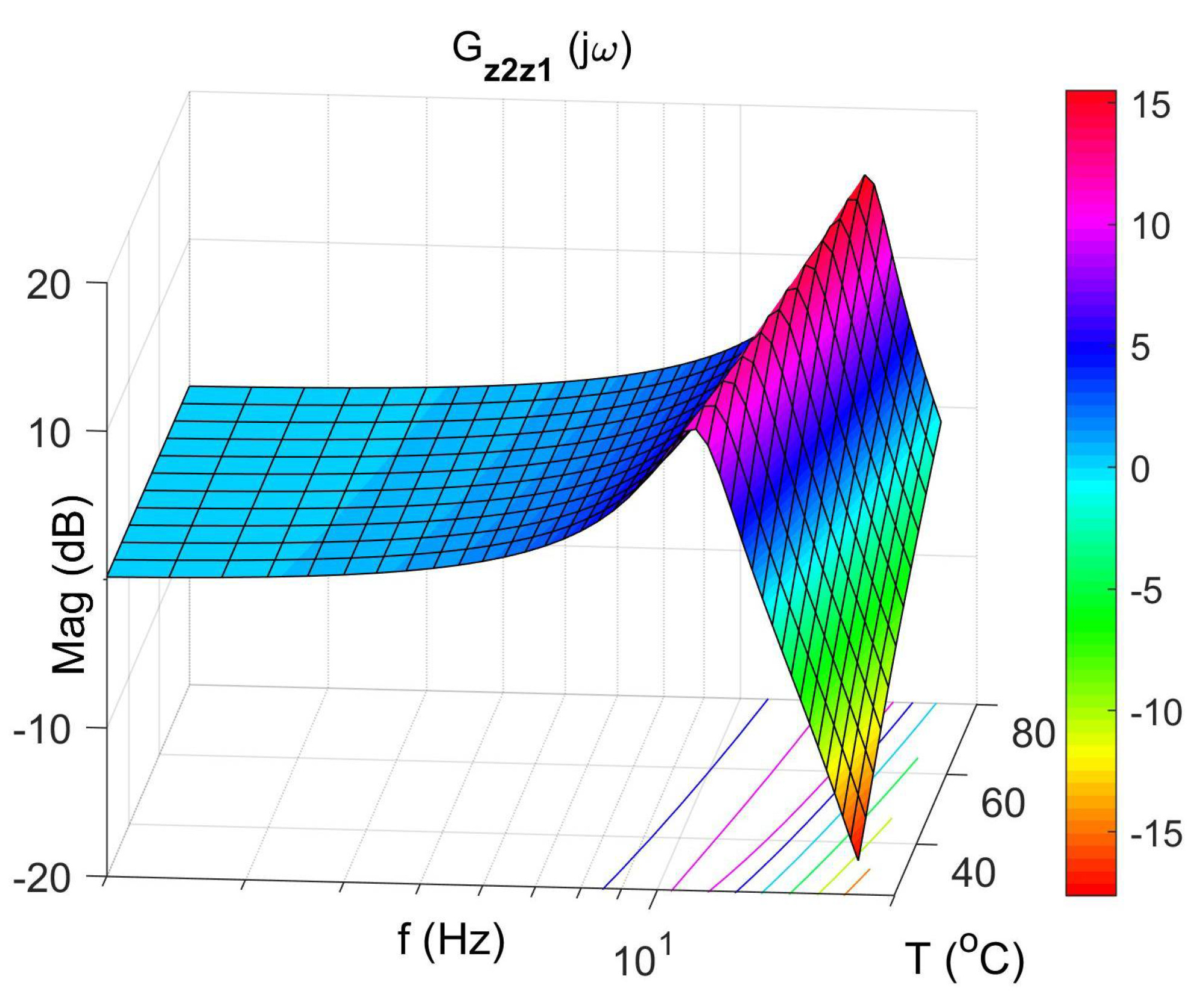
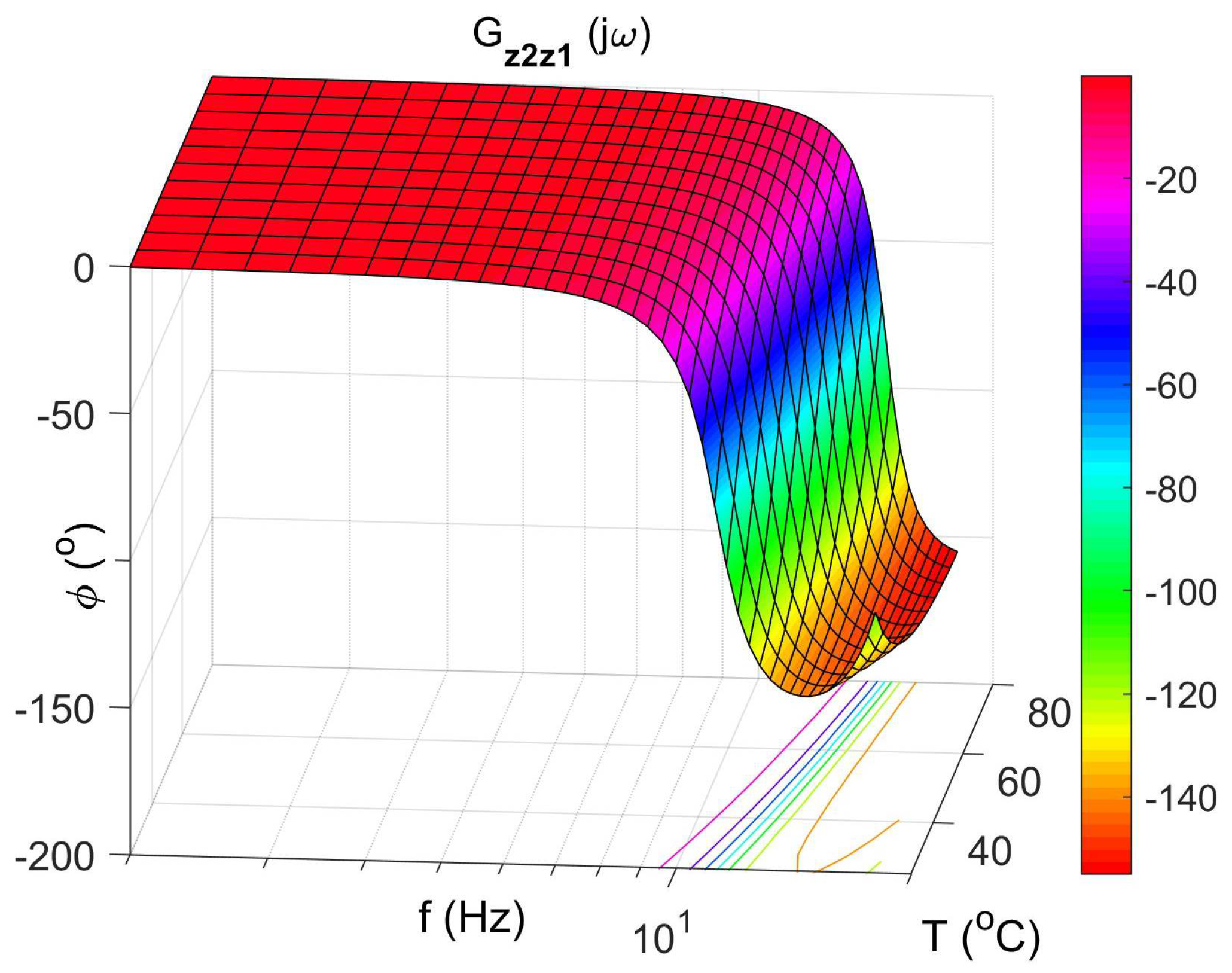
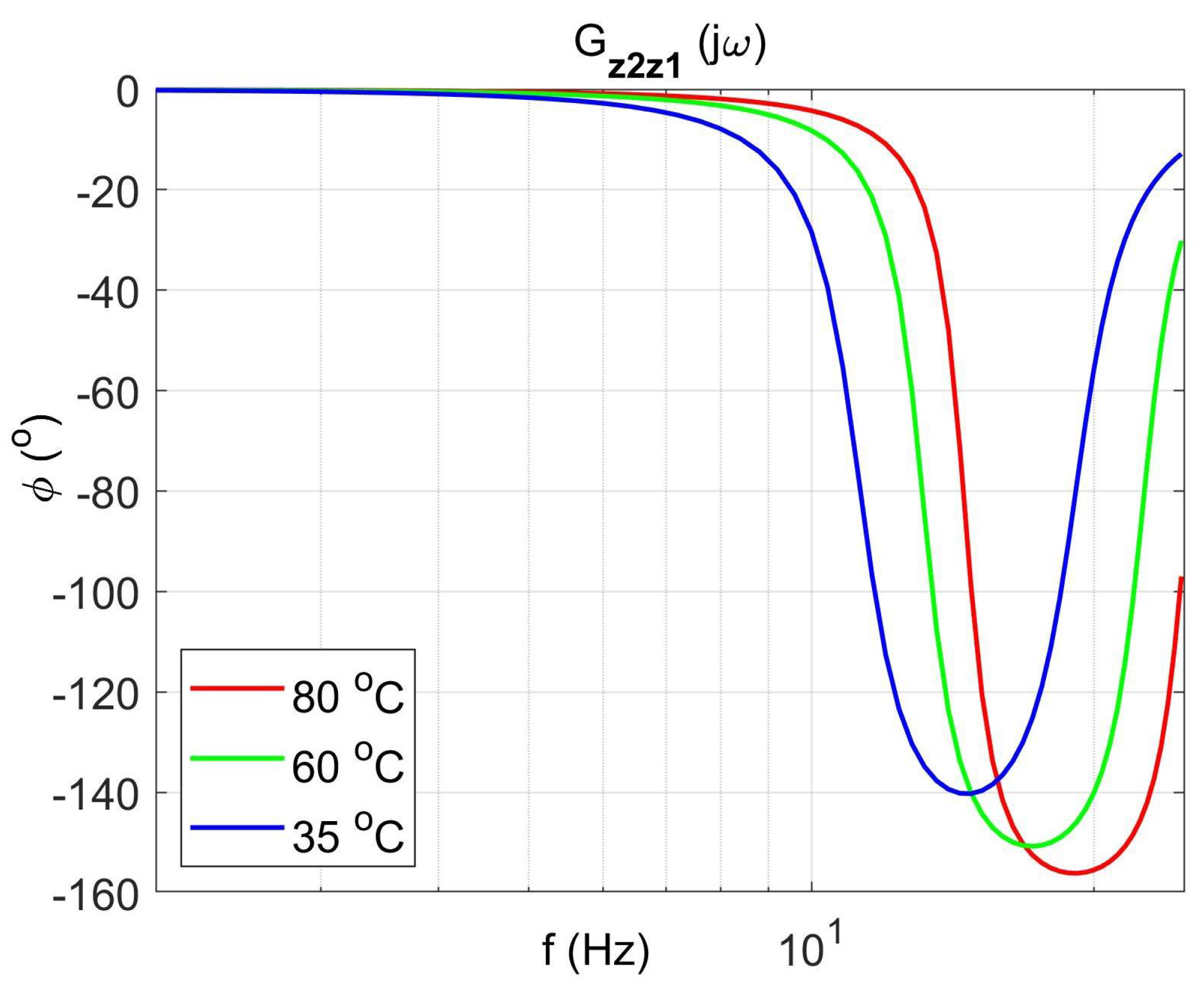
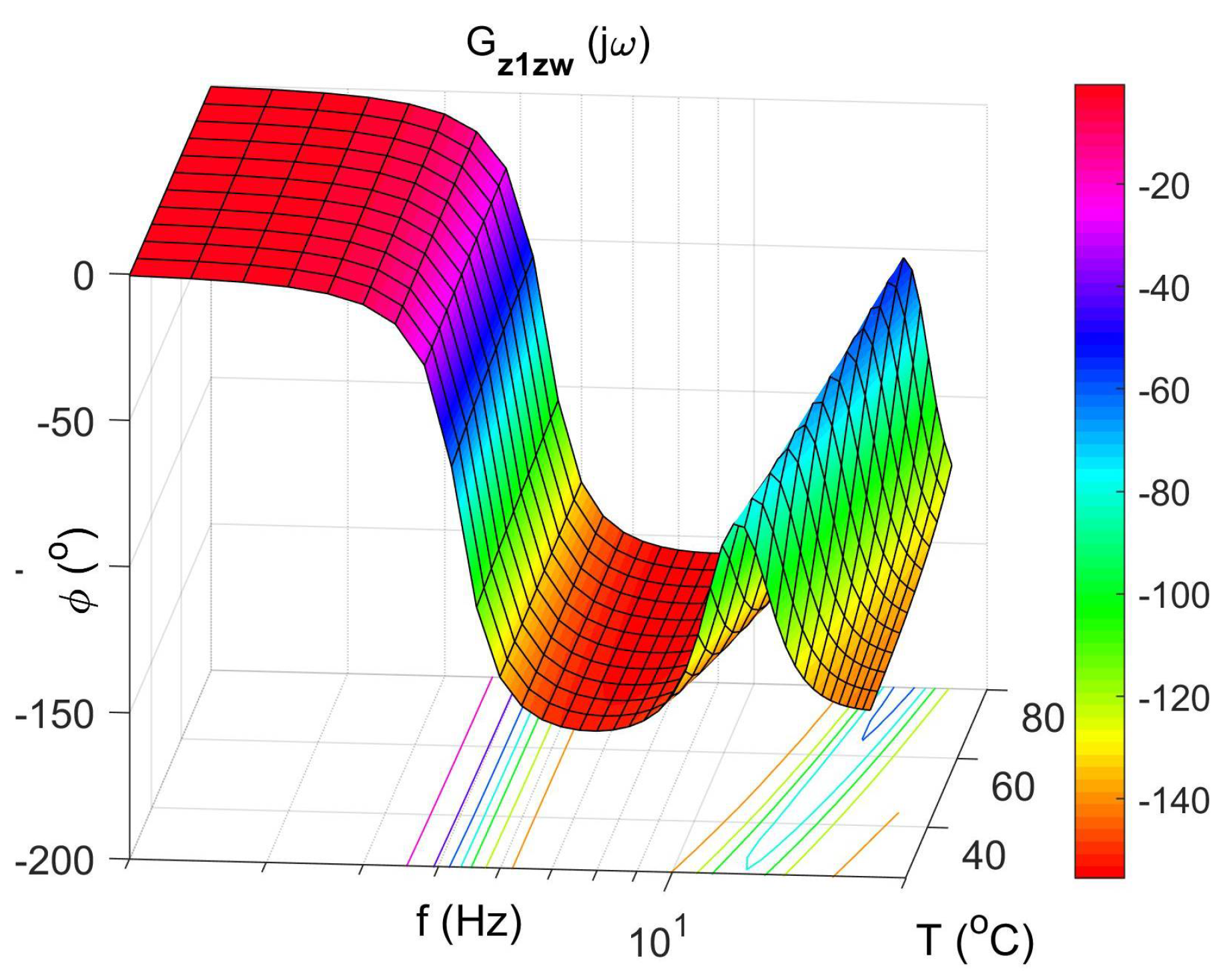
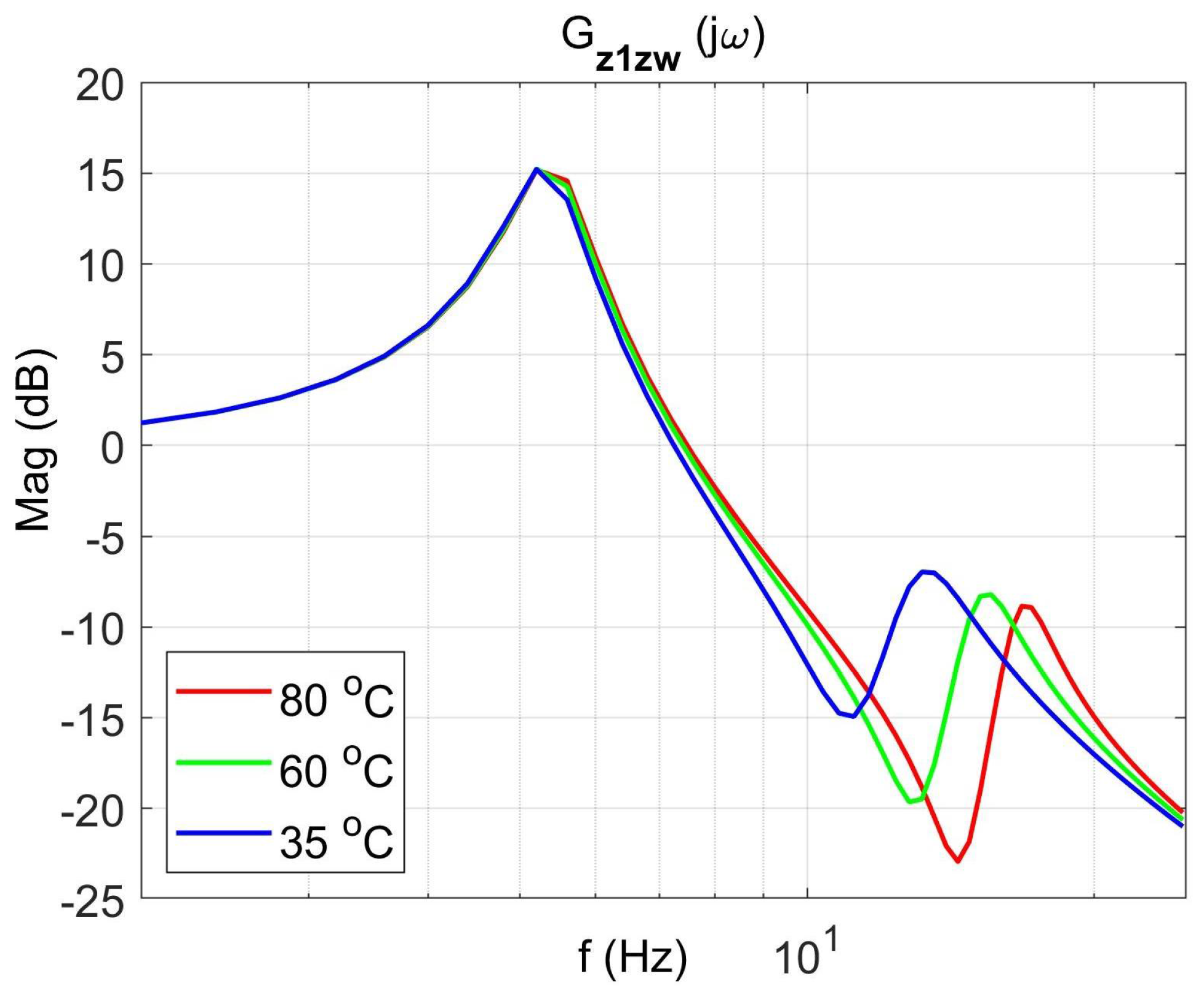
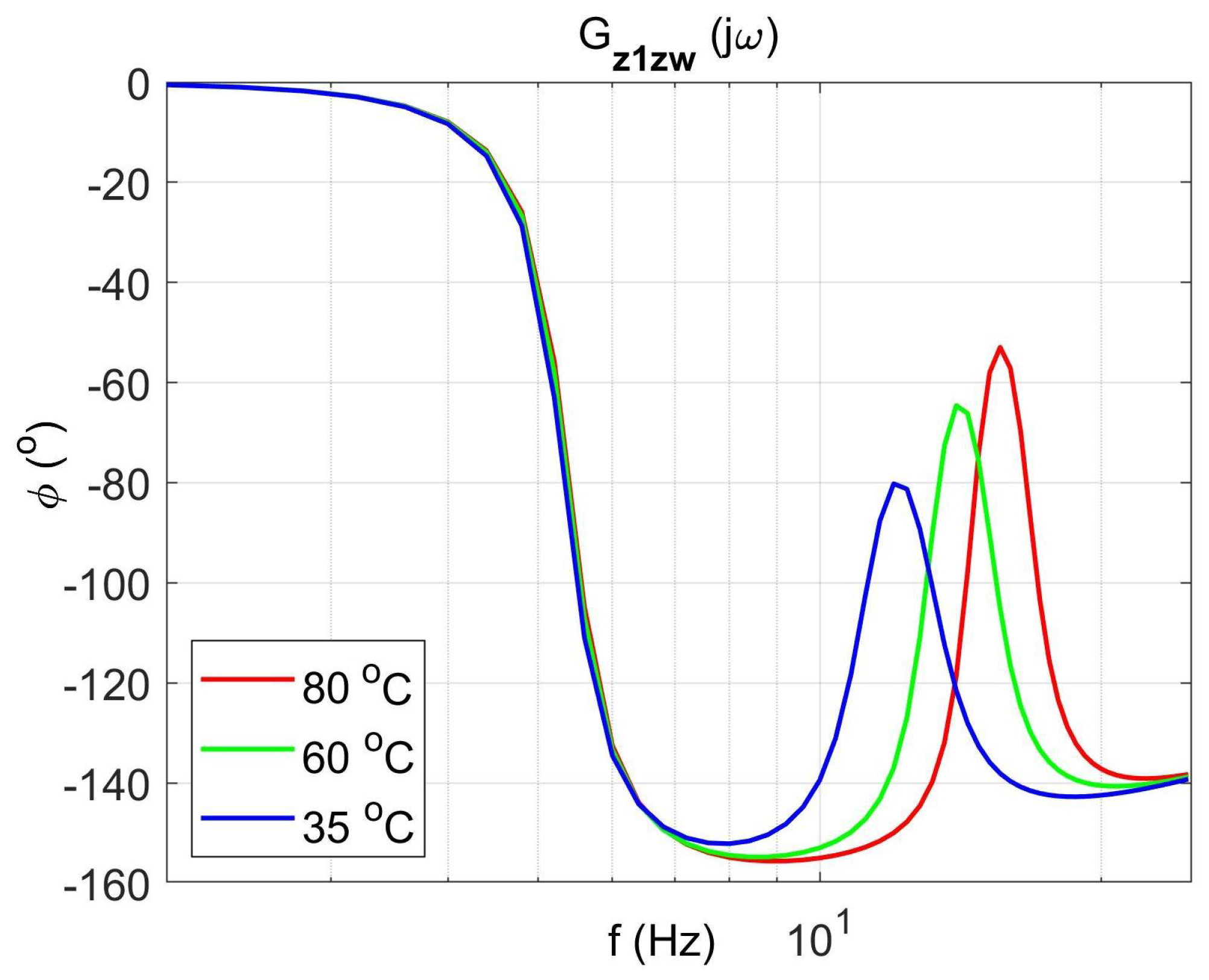
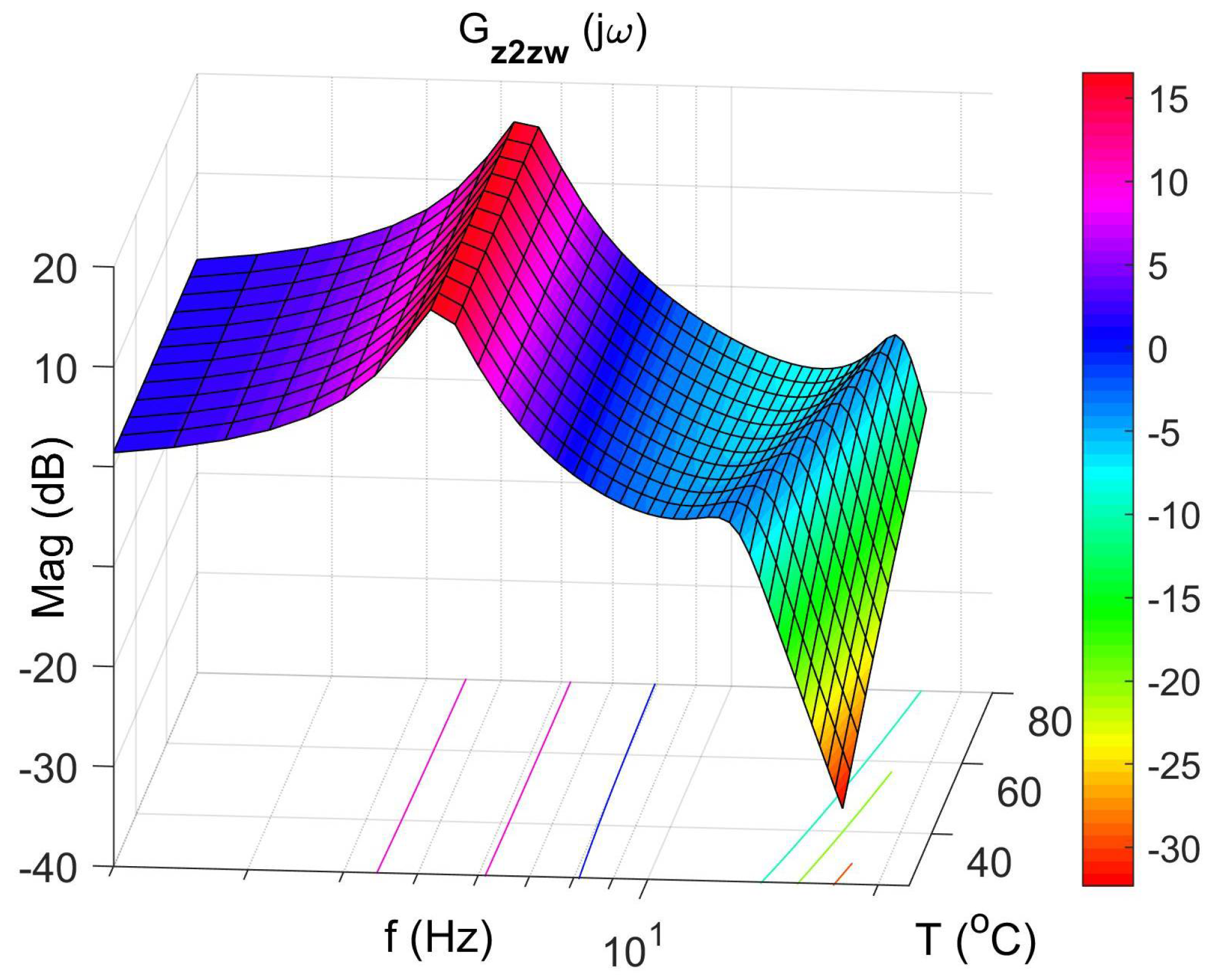
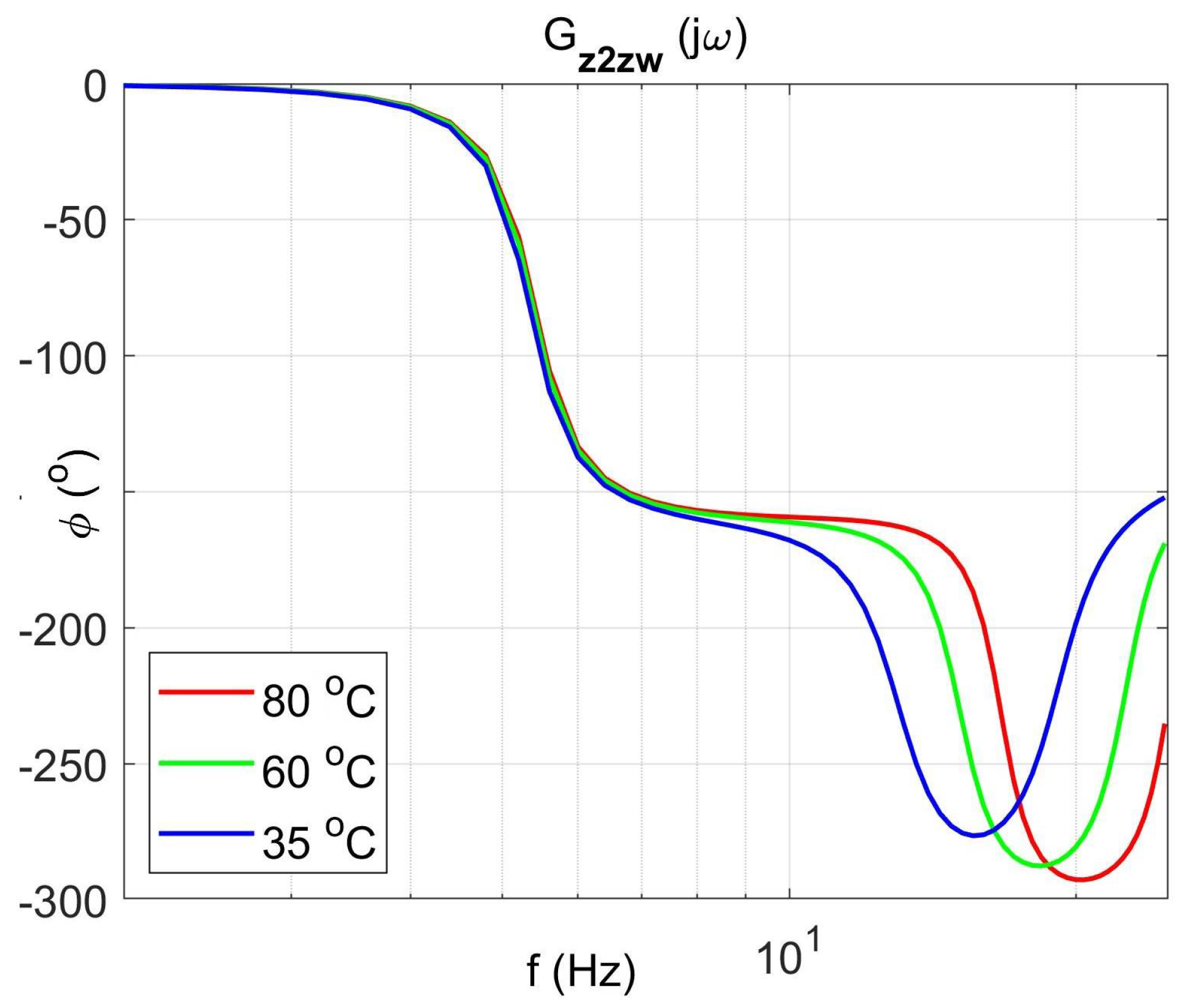
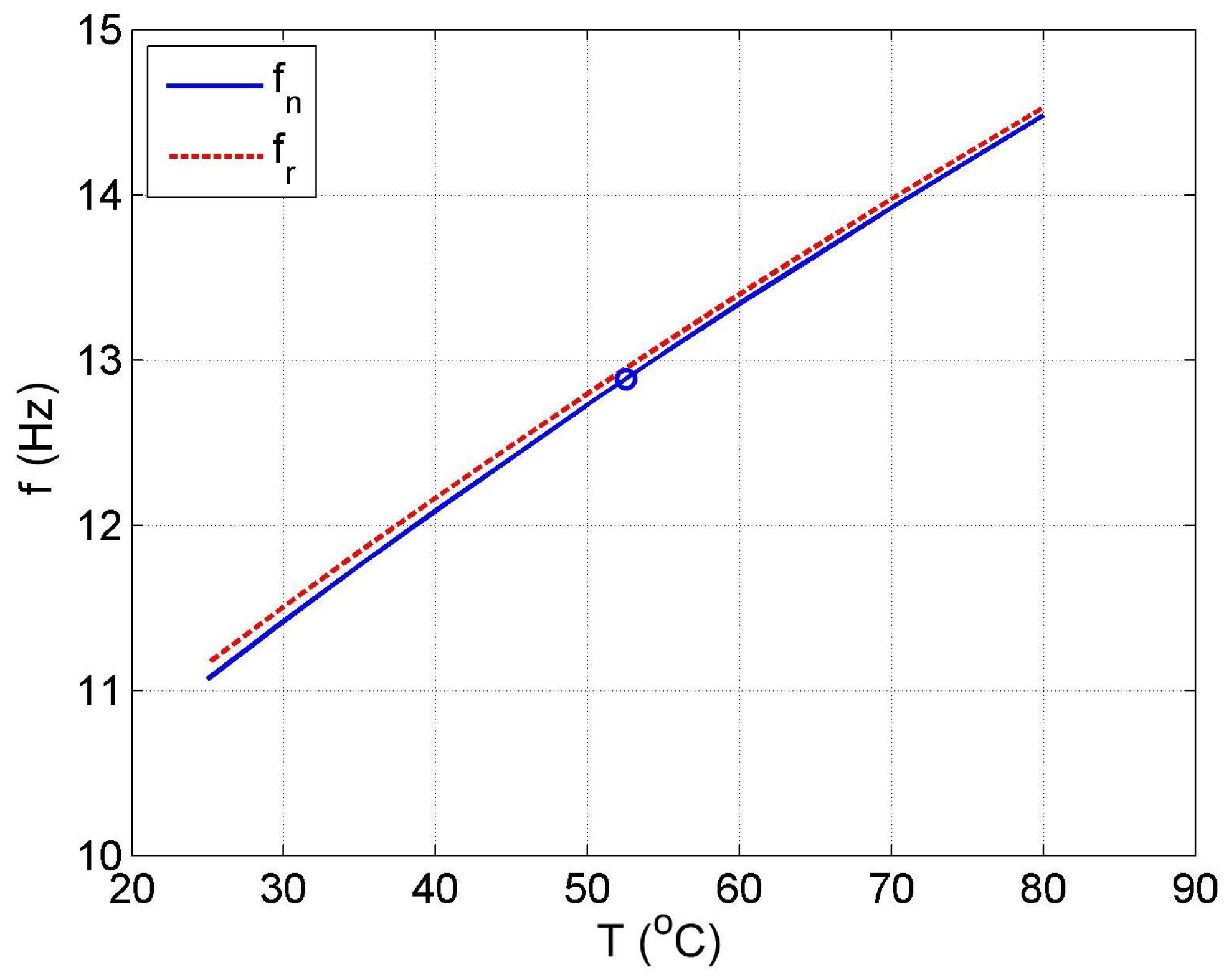
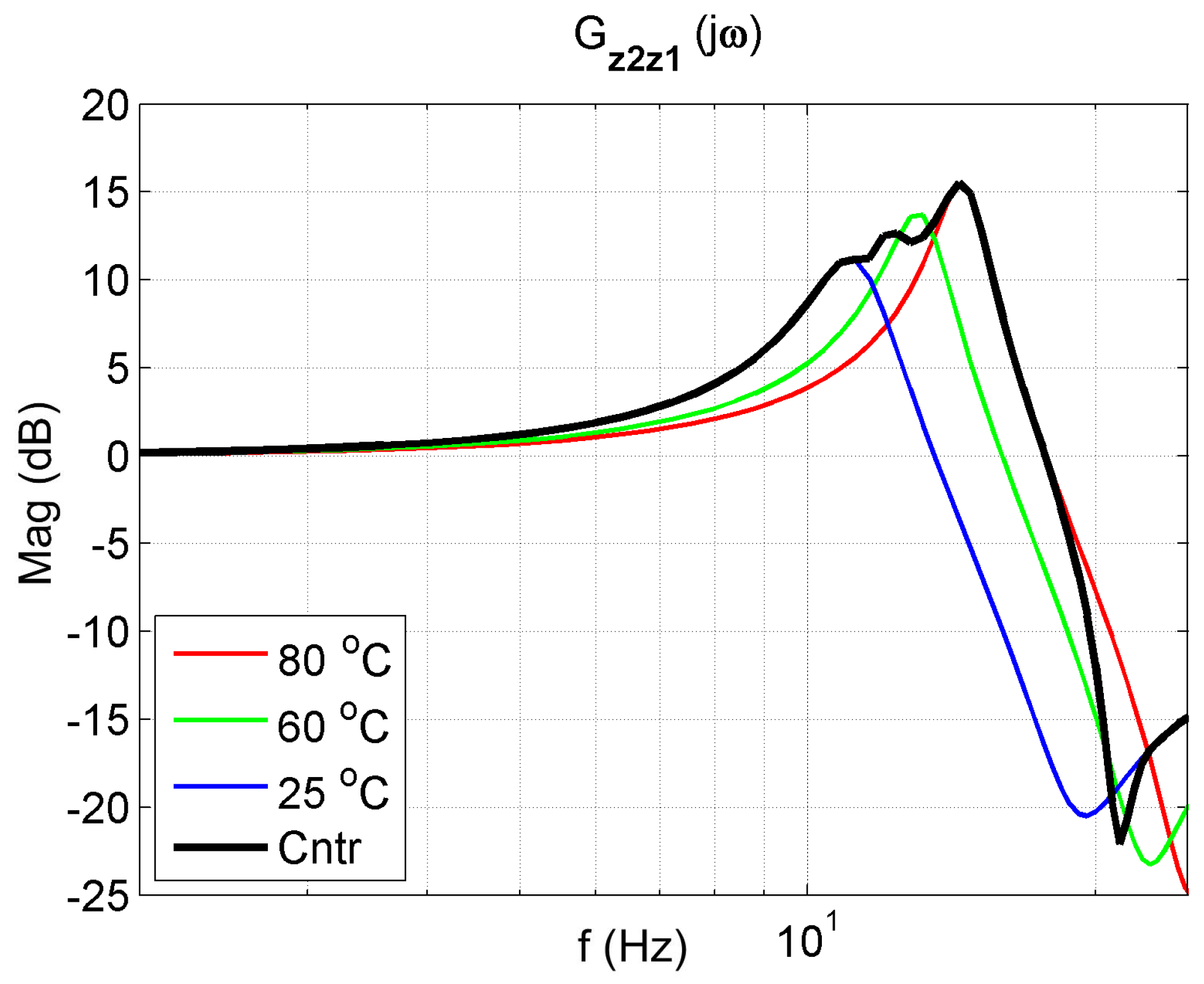
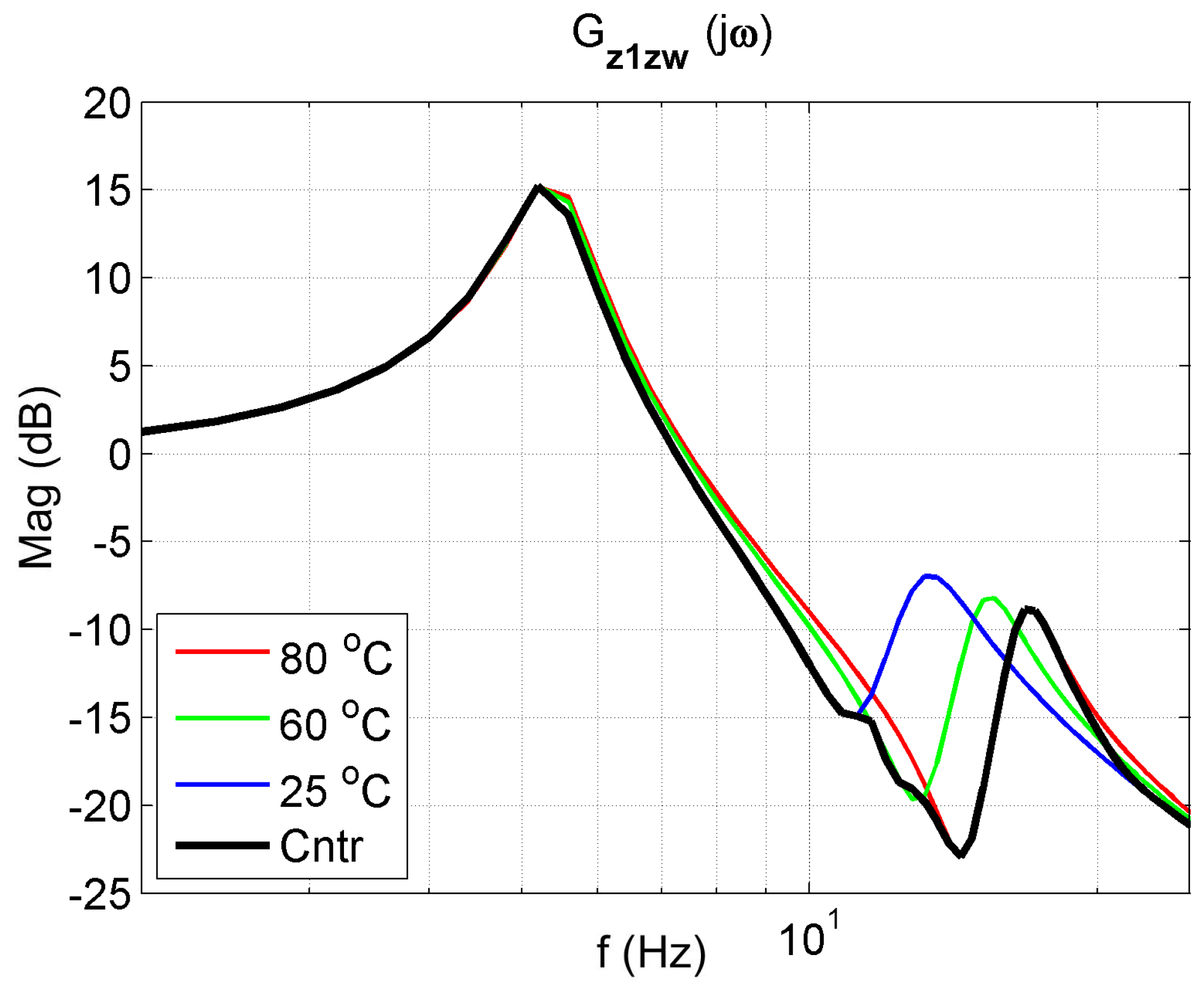
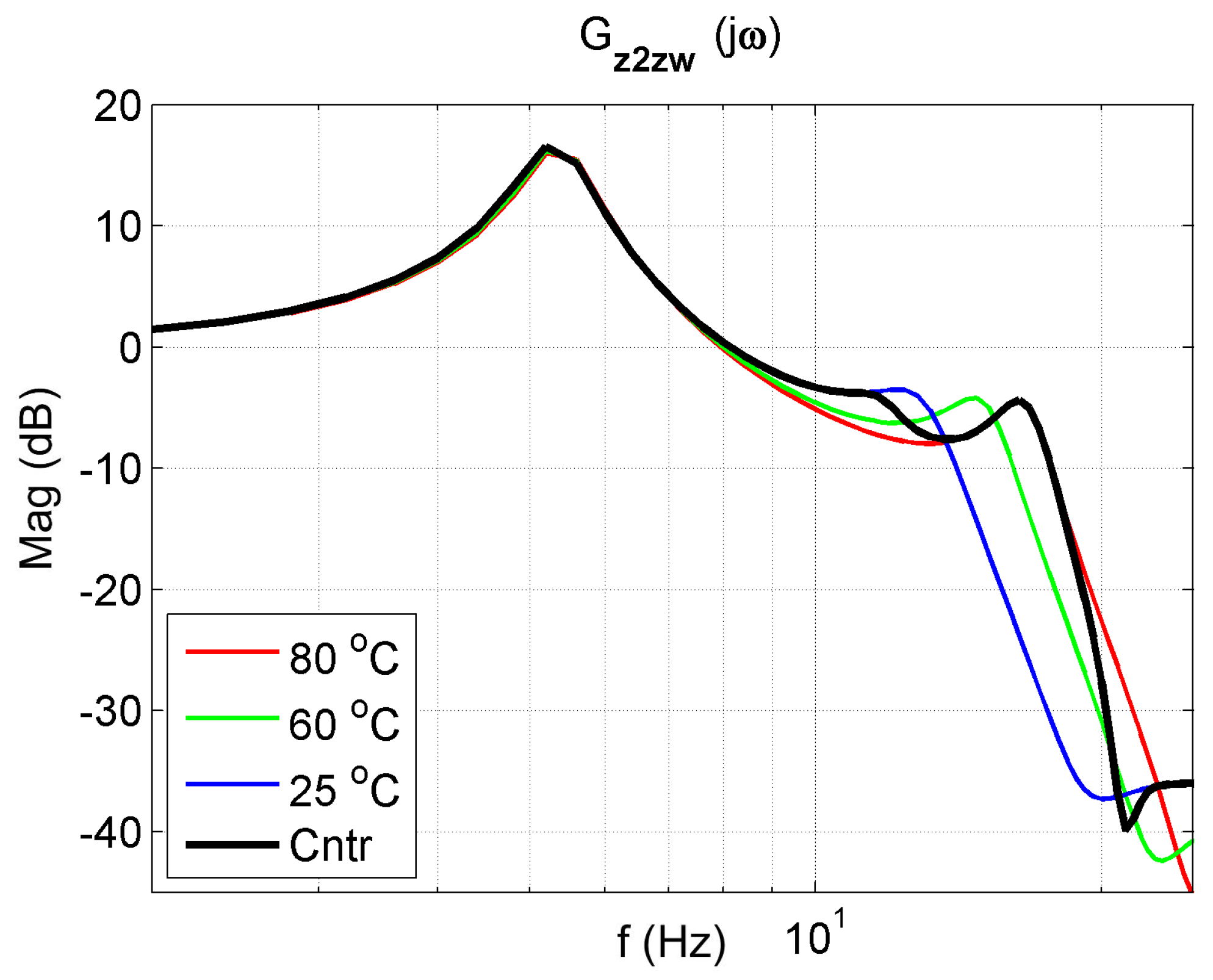
3. Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Khandelwal, A.; Buravalla, V. Models for Shape Memory Alloy Behavior: An overview of modelling approaches. Int. J. Struct. Chang. Solids 2009, 1, 111–148. [Google Scholar]
- Birman, V. Review of Mechanics of Shape Memory Alloy Structures. Appl. Mech. Rev. 1997, 50, 629–645. [Google Scholar] [CrossRef]
- Schwartz, M.; Wiley, J. Encyclopedia of Smart Materials Volume 1 and Volume 2; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2002; Volume 1, ISBN 0471177806. [Google Scholar]
- Paiva, A.; Savi, M.A. An overview of constitutive models for shape memory alloys. Math. Probl. Eng. 2006, 2006, 1–30. [Google Scholar] [CrossRef]
- Smith, R.C. Smart Material Systems; Society for Industrial and Applied Mathematics: Philadelphia, PA, USA, 2005; ISBN 978-0-89871-583-5. [Google Scholar]
- Lagoudas, D.C. Shape Memory Alloys. Modeling and Engineering Applications; Springer US: Boston, MA, USA, 2008; Volume 1, ISBN 978-0-387-47684-1. [Google Scholar]
- Achenbach, M.; Muller, I. Simulation of material behaviour, of alloys with shape memory. Arch. Mech. Mech. 1985, 37, 573–585. [Google Scholar]
- Achenbach, M.; Atanackovic, T.; Müller, I. A model for memory alloys in plane strain. Int. J. Solids Struct. 1986, 22, 171–193. [Google Scholar] [CrossRef]
- Seelecke, S. Modeling the dynamic behavior of shape memory alloys. Int. J. Non. Linear. Mech. 2002, 37, 1363–1374. [Google Scholar] [CrossRef]
- Heintze, O.; Seelecke, S. A coupled thermomechanical model for shape memory alloys—From single crystal to polycrystal. Mater. Sci. Eng. A 2008, 481–482, 389–394. [Google Scholar] [CrossRef]
- Naso, D.; Seelecke, S. Passivity Analysis and Port-Hamiltonian Formulation of the Müller-Achenbach-Seelecke Model for Shape Memory Alloys: The Isothermal Case. IFAC-PapersOnLine 2018, 51, 713–718. [Google Scholar] [CrossRef]
- Rączka, W.; Konieczny, J.; Sibielak, M.; Kowal, J. Discrete preisach model of a shape memory alloy actuator. Solid State Phenom. 2016, 248, 227–234. [Google Scholar] [CrossRef]
- Ahn, K.K.; Kha, N.B. Modeling and control of shape memory alloy actuators using Preisach model, genetic algorithm and fuzzy logic. Mechatronics 2008, 18, 141–152. [Google Scholar] [CrossRef]
- Mayergoyz, I.D. Mathematical Models of Hysteresis and Their Applications; Elsevier: Amsterdam, The Netherlands, 2003; ISBN 9780124808737. [Google Scholar]
- Duval, L.; Noori, M.; Hou, Z.; Davoodi, H.; Seelecke, S. Random vibration studies of an SDOF system with shape memory restoring force. Phys. B Condens. Matter 2000, 275, 138–141. [Google Scholar] [CrossRef] [Green Version]
- Ozga, A. Distribution of Random Pulses Forcing a Damped Oscillator Determined in a Finite Time Interval. Acta Phys. Pol. A 2014, 125, A159–A163. [Google Scholar] [CrossRef]
- Jabłoński, M.; Ozga, A. Determining the distribution of values of stochastic impulses acting on a discrete system in relation to their intensity. Acta Phys. Pol. A 2012, 121, 174–178. [Google Scholar] [CrossRef]
- Jabłoński, M.; Ozga, A. Statistical Characteristics of the Damped Vibrations of a String Excited by Stochastic Forces. Arch. Acoust. 2009, 612, 601–612. [Google Scholar]
- Kwaśniewski, J.; Dominik, I.; Konieczny, J.; Lalik, K.; Sakeb, A. Application of self-excited acoustical system for stress changes measurement in sandstone bar. J. Theor. Appl. Mech. 2011, 49, 1049–1058. [Google Scholar]
- Rzepecki, J.; Chraponska, A.; Mazur, K.; Wrona, S.; Pawelczyk, M. Semi-active reduction of device casing vibration using a set of piezoelectric elements. In Proceedings of the 20th International Carpathian Control Conference (ICCC), Kraków, Poland, 26–29 May 2019; pp. 648–652. [Google Scholar]
- Sibielak, M.; Raczka, W.; Konieczny, J.; Kowal, J. Optimal control based on a modified quadratic performance index for systems disturbed by sinusoidal signals. Mech. Syst. Signal Process. 2015, 64–65, 498–519. [Google Scholar] [CrossRef]
- Sibielak, M. Optimal controller for vibration isolation system with controlled hydraulic damper by piezoelectric stack. Mech. Syst. Signal Process. 2013, 36, 118–126. [Google Scholar] [CrossRef]
- Rączka, W.; Sibielak, M.; Konieczny, J. Active vehicle suspension with a weighted multitone optimal controller: Considerations of energy consumption. In Structural Health Monitoring, Photogrammetry & DIC; Society for Experimental Mechanics Series; Springer: Cham, Switzerland, 2018; Volume 6. [Google Scholar]
- Otsuka, K.; Wayman, C.M. Shape Memory Materials; Cambridge University Press: Cambridge, UK, 1998. [Google Scholar]
- Bojarski, Z.; Morawiec, H. Metale z Pamięcią Kształtu; Państwowe Wydawnictwo Naukowe: Warszawa, Poland, 1988. [Google Scholar]
- Kluszczyński, K.; Kciuk, M. Analytical Description of SMA Actuator Dynamics based on Fermi-Dirac Function. Acta Phys. Pol. A 2017, 131, 1274–1279. [Google Scholar] [CrossRef]
- Rączka, W.; Konieczny, J.; Sibielak, M. Mathematical Model of a Shape Memory Alloy Spring Intended for Vibration Reduction Systems. Solid State Phenom. 2011, 177, 65–75. [Google Scholar] [CrossRef]
- Tiseo, B.; Concilio, A.; Ameduri, S.; Gianvito, A. A Shape Memory Alloys Based Tuneable Dynamic Vibration Absorber For Vibration Tonal Control. J. Theor. Appl. Mech. 2010, 48, 135–153. [Google Scholar]
- Klein, W.; Mezyk, A.; Switonski, E. Modelling and tuning the SMA absorber. In Proceedings of the ISMA2006: International Conference on Noise and Vibration Engineering, Heverlee, Belgium, 18–20 September 2006; pp. 413–420. [Google Scholar]
- Rączka, W.; Sibielak, M.; Kowal, J.; Konieczny, J. Application of an SMA Spring for Vibration Screen Control. J. Low Freq. Noise Vib. Act. Control 2013, 32, 117–131. [Google Scholar] [CrossRef] [Green Version]
- Ostachowicz, W.M.; Kaczmarczyk, S. Vibrations of composite plates with SMA fibres in a gas stream with defects of the type of delamination. J. Compos. Struct. 2001, 54, 305–311. [Google Scholar] [CrossRef]
- Ni, Q.; Zhang, R.; Natsuki, T.; Iwamoto, M. Stiffness and vibration characteristics of SMA/ER3 composites with shape memory alloy short fibers. Compos. Struct. 2007, 79, 501–507. [Google Scholar] [CrossRef] [Green Version]
- Kurczyk, S.; Pawelczyk, M. Fuzzy Control for Semi-Active Vehicle Suspension. J. Low Freq. Noise Vib. Act. Control 2013, 32, 217–226. [Google Scholar] [CrossRef] [Green Version]
- Williams, K.; Chiu, G.; Bernhard, R. Adaptive-Passive Absorbers Using Shape-Memory Alloys. J. Sound Vib. 2002, 249, 835–848. [Google Scholar] [CrossRef]
- Williams, K.A.; Chiu, G.T.-C.; Bernhard, R.J. Dynamic modelling of a shape memory alloy adaptive tuned vibration absorber. J. Sound Vib. 2005, 280, 211–234. [Google Scholar] [CrossRef]
- Williams, K.A.; Chiu, G.T.-C.; Bernhard, R.J. Nonlinear control of a shape memory alloy adaptive tuned vibration absorber. J. Sound Vib. 2005, 288, 1131–1155. [Google Scholar] [CrossRef]
- Piedboeuf, M.C.; Gauvin, R.; Thomas, M. Damping Behaviour of Shape Memory Alloys: Strain Amplitude, Frequency and Temperature Effects. J. Sound Vib. 1998, 214, 885–901. [Google Scholar] [CrossRef]
- Toker, G.P.; Saedi, S.; Acar, E.; Ozbulut, O.E.; Karaca, H.E. Loading frequency and temperature-dependent damping capacity of NiTiHfPd shape memory alloy. Mech. Mater. 2020, 150, 103565. [Google Scholar] [CrossRef]
- Karakalas, A.A.; Machairas, T.T.; Lagoudas, D.C.; Saravanos, D.A. Quantification of Shape Memory Alloy Damping Capabilities Through the Prediction of Inherent Behavioral Aspects. Shape Mem. Superelasticity 2021, 7, 7–29. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rączka, W.; Konieczny, J.; Sibielak, M. Modelling of SMA Vibration Systems in an AVA Example. Materials 2021, 14, 5905. https://doi.org/10.3390/ma14195905
Rączka W, Konieczny J, Sibielak M. Modelling of SMA Vibration Systems in an AVA Example. Materials. 2021; 14(19):5905. https://doi.org/10.3390/ma14195905
Chicago/Turabian StyleRączka, Waldemar, Jarosław Konieczny, and Marek Sibielak. 2021. "Modelling of SMA Vibration Systems in an AVA Example" Materials 14, no. 19: 5905. https://doi.org/10.3390/ma14195905
APA StyleRączka, W., Konieczny, J., & Sibielak, M. (2021). Modelling of SMA Vibration Systems in an AVA Example. Materials, 14(19), 5905. https://doi.org/10.3390/ma14195905






