Numerical Modeling of Phase Transformations in Dual-Phase Steels Using Level Set and SSRVE Approaches
Abstract
:1. Introduction
2. Review of the Phase Transformation Models
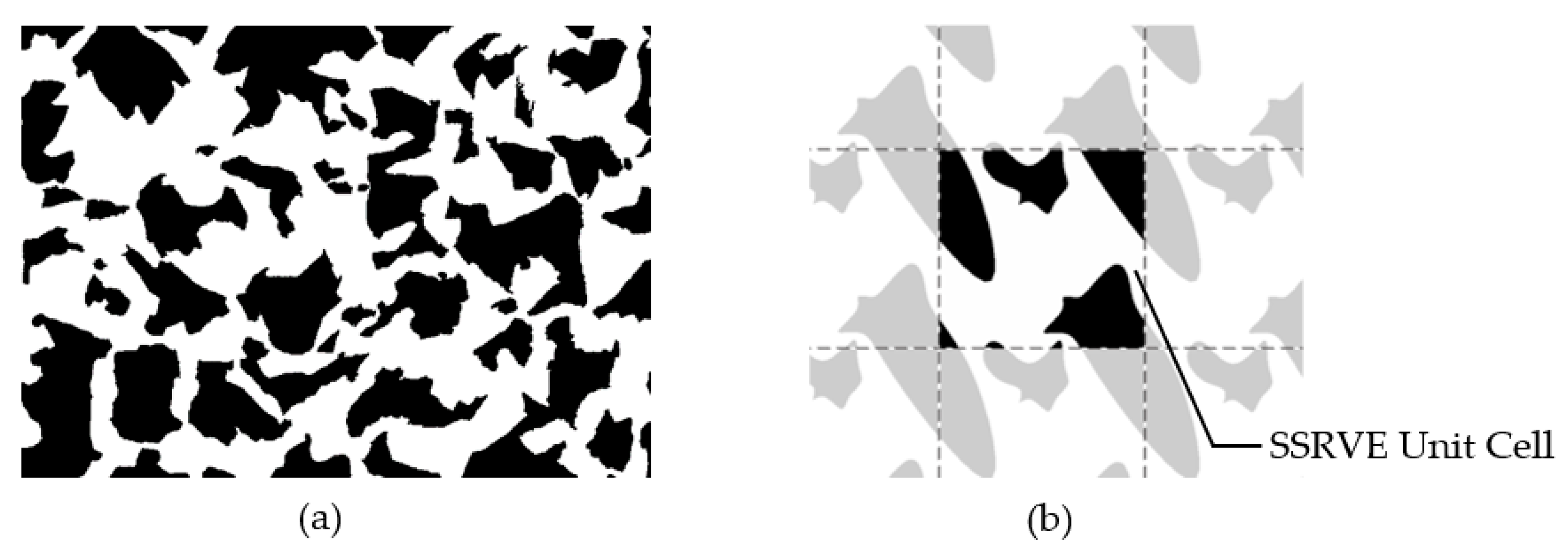
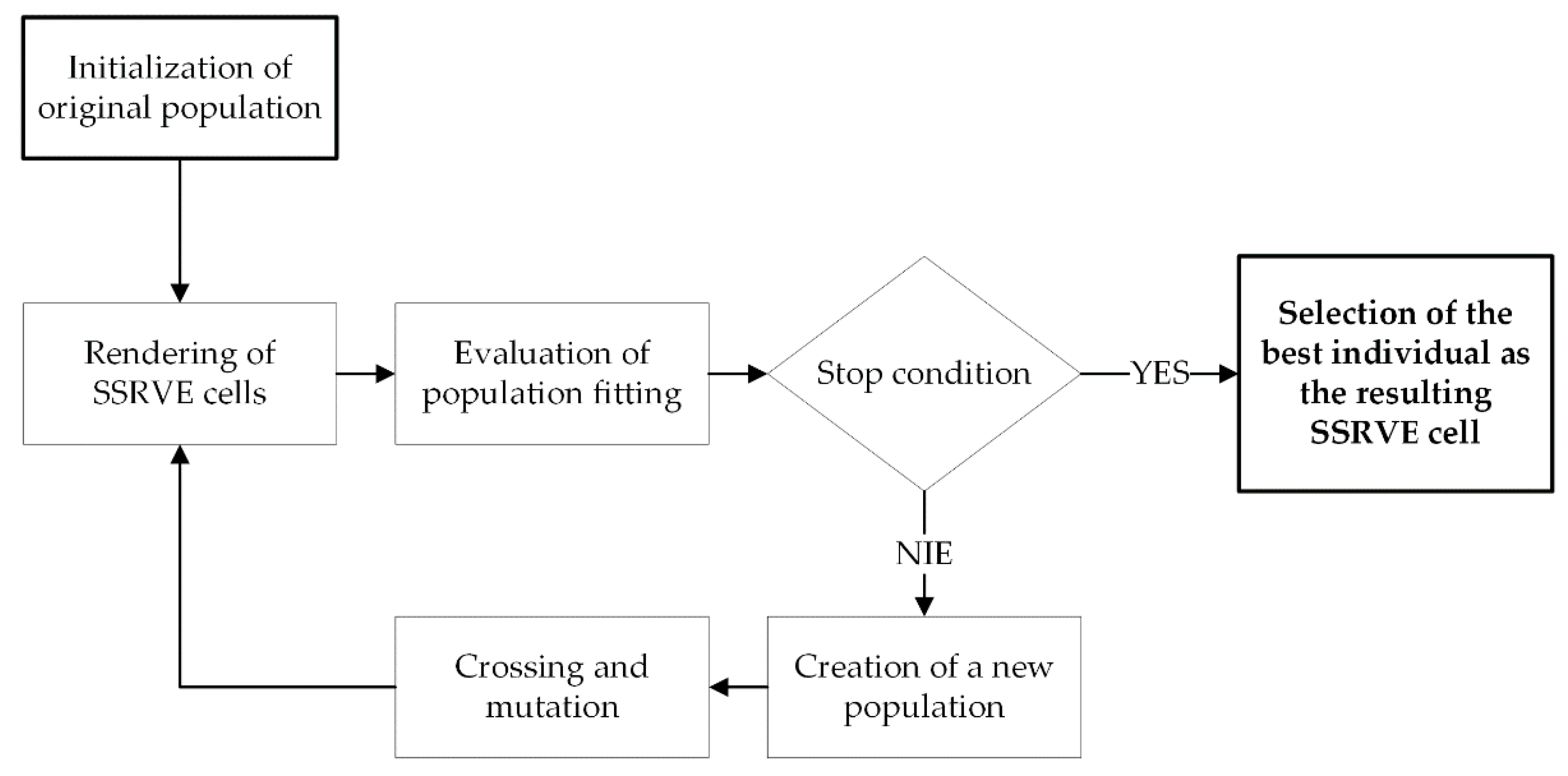
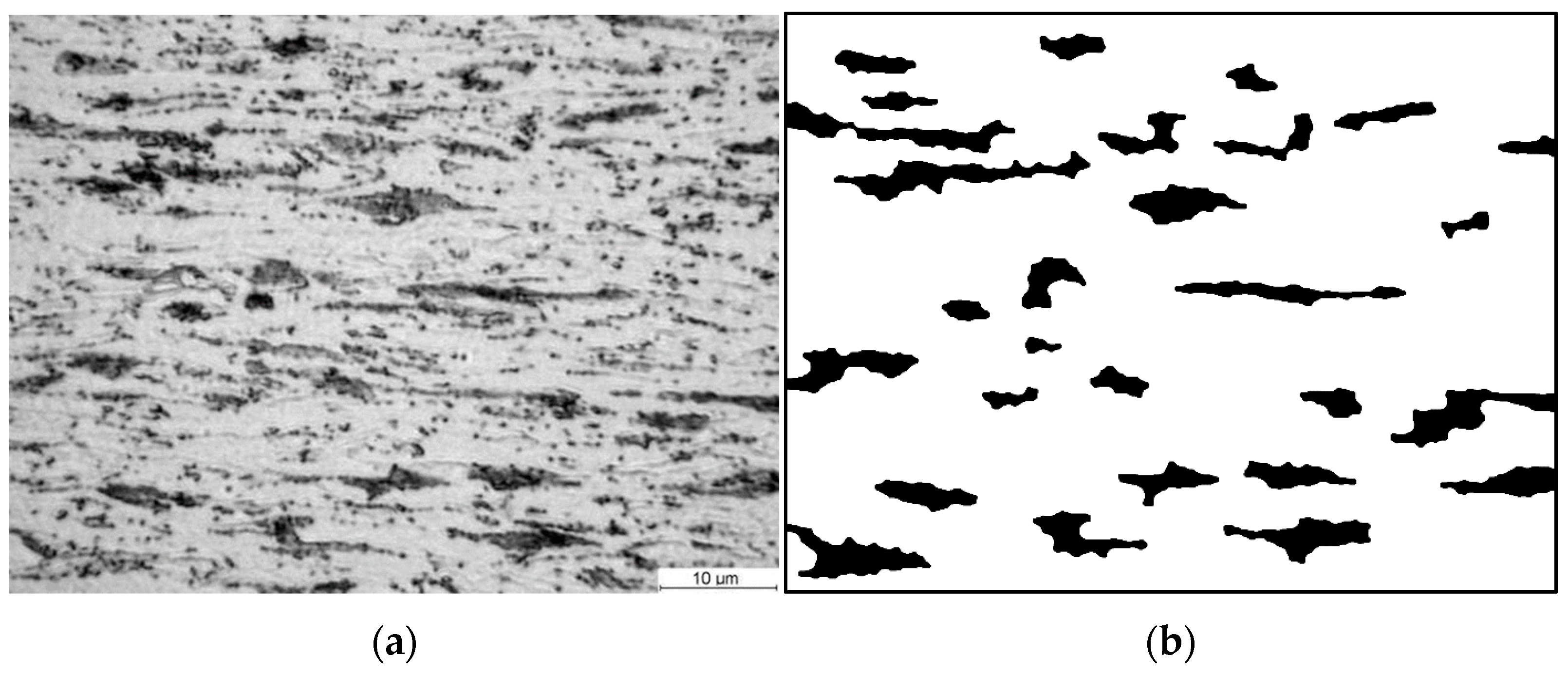
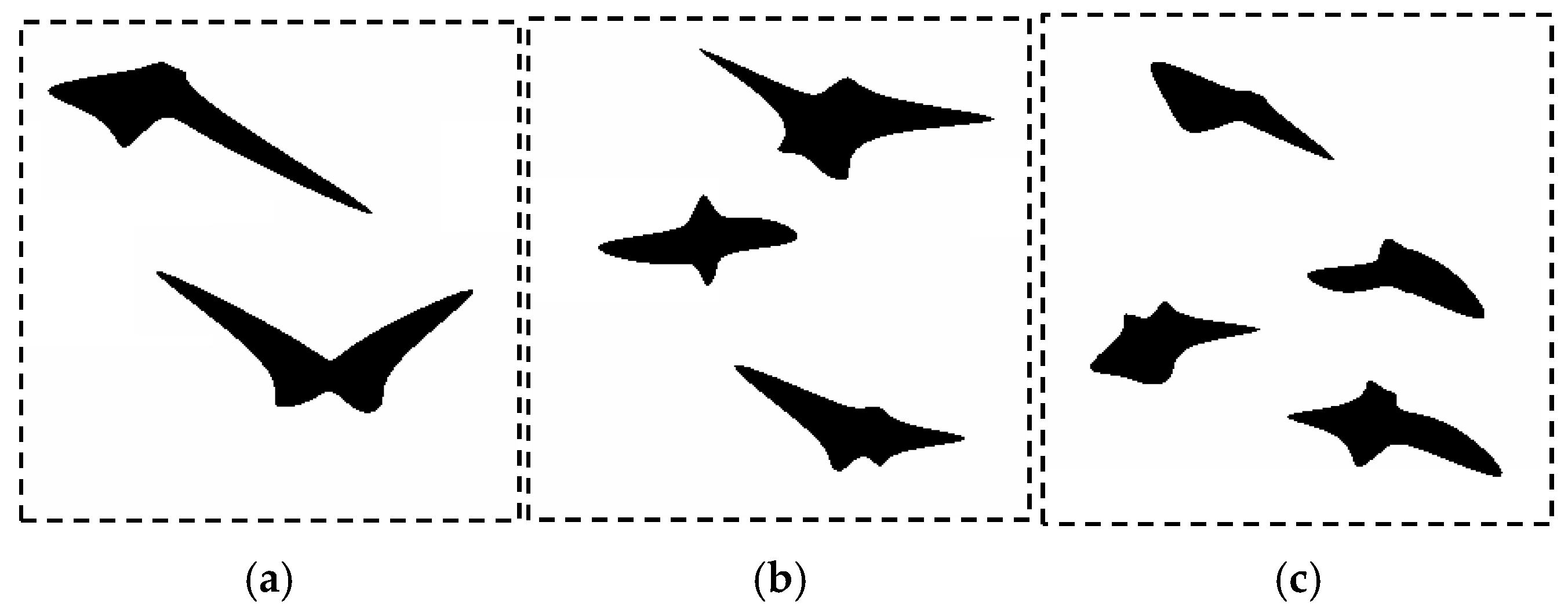
3. Methodology of Determining SSRVEs
4. Phase Transformation Model
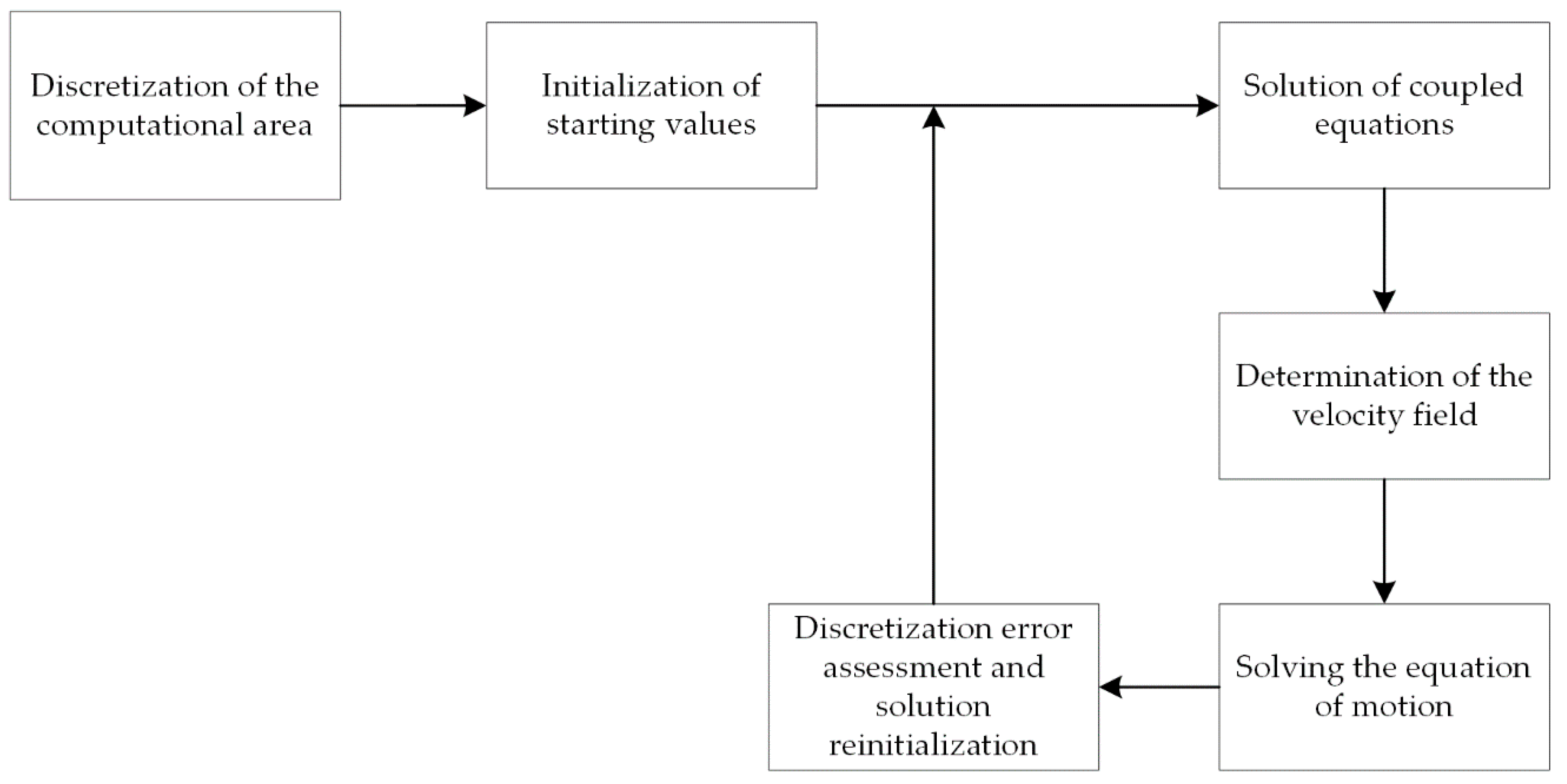
4.1. Level Set Method (LSM)
4.2. MLS-DIFF Model
4.2.1. Austenitic Transformation
4.2.2. Ferritic Transformation
5. Model Verification
5.1. Laboratory Verification
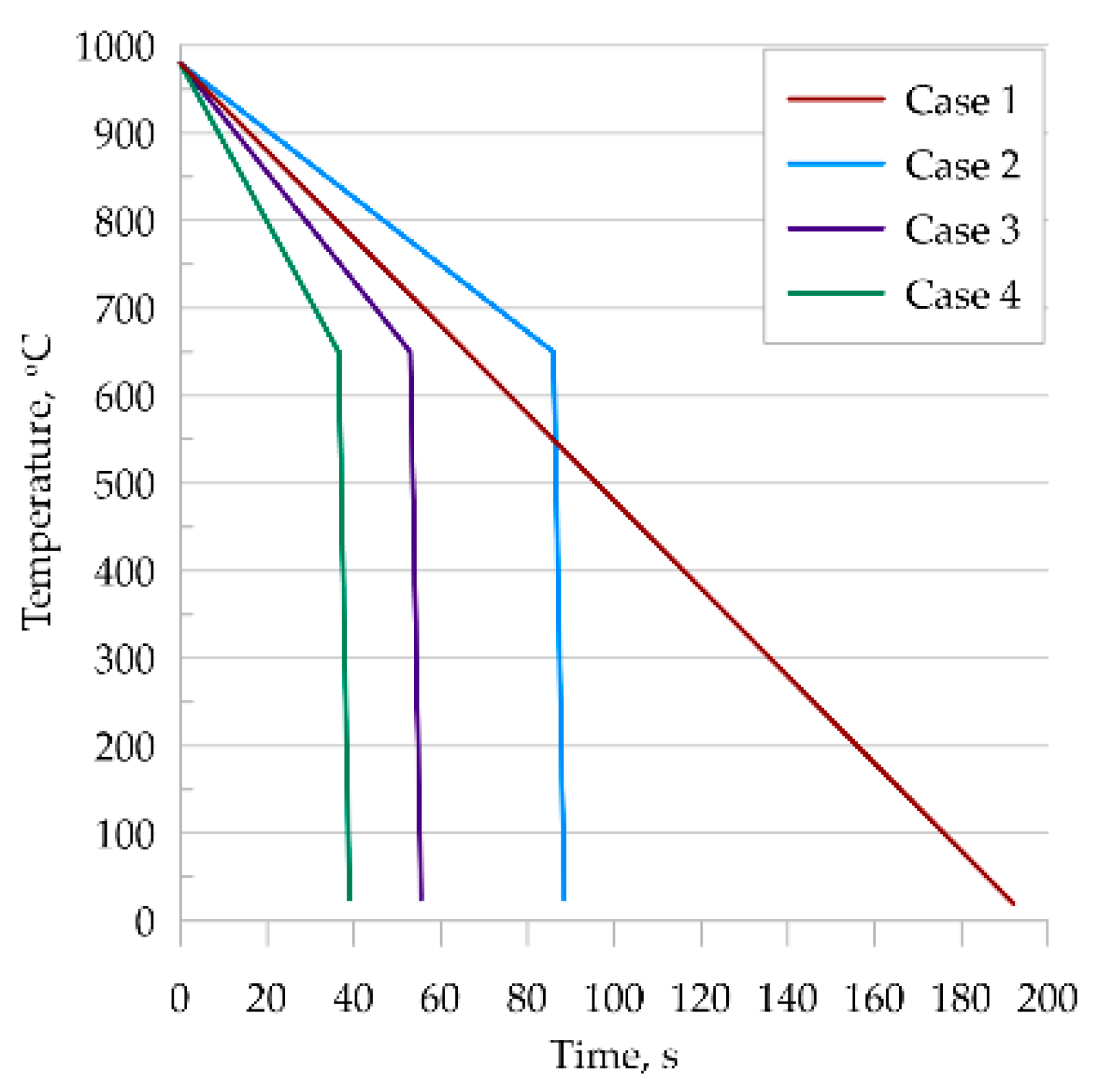
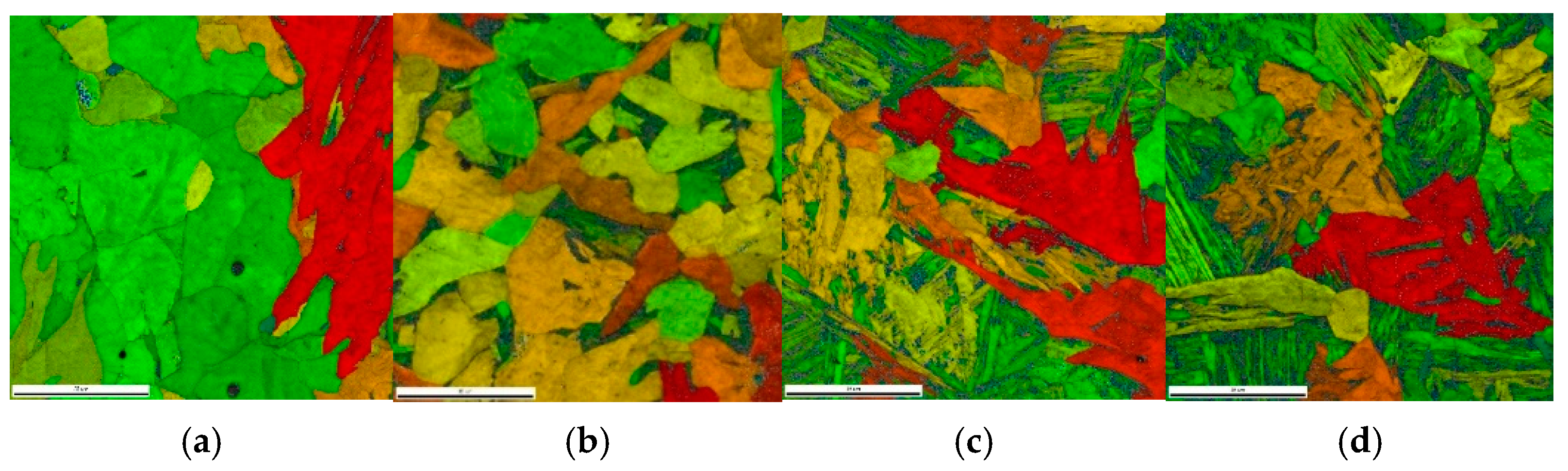
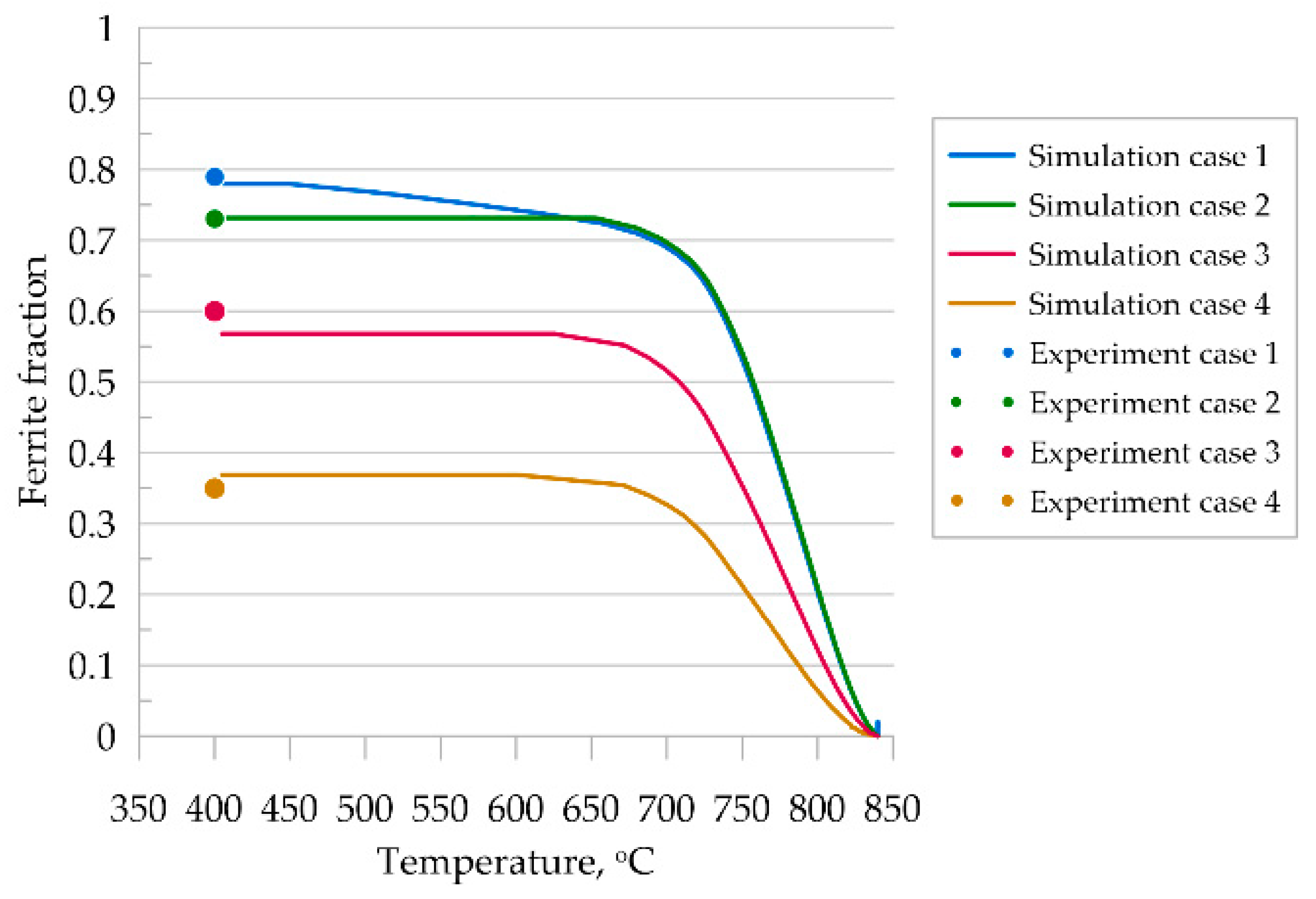
- Each of the selected temperature cycles was mapped using the MLS-DIFF model. For this purpose, a digital representation of a 100 × 100 μm-base microstructure with an average grain size of 30μm was prepared and simulations were performed on it. The initial microstructure is ferritic–perlitic. Obtained results from the EBSD analysis are presented in Figure 9. It can be seen that in the case of thermal cycle one and two, diffusive transformation products were obtained (with equiaxed ferrite morphologies), while in the case of thermal cycle three and four, acicular ferrite and bainite were produced, respectively.
5.2. Industrial Verification
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Conflicts of Interest
References
- Pietrzyk, M.; Madej, L.; Rauch, L.; Szeliga, D. Chapter Three—Conventional Modeling. In Achieving High Accuracy and Efficiency in Metals Processing Simulations; Computational Materials Engineering; Butterworth-Heinemann, Elsevier: Boston, MA, USA, 2015; pp. 69–151. [Google Scholar]
- Madej, L.; Rauch, L.; Perzynski, K.; Cybulka, P. Digital Material Representation as an efficient tool for strain inhomogeneities analysis at the micro scale level. Arch. Civ. Mech. Eng. 2011, 11, 661–679. [Google Scholar] [CrossRef]
- Bleck, W.; Prahl, U.; Hirt, G.; Bambach, M. Designing New Forging Steels by ICMPE. In Advances in Production Technology, Lecture Notes in Production Engineering; Brecher, C., Ed.; Springer: Cham, Switzerland, 2015; pp. 85–98. [Google Scholar]
- Balzani, D.; Schröder, J.; Brands, D. FE2-Simulation of Microheterogeneous Steels Based on Statistically Similar RVEs. In Proceedings of the IUTAM Symposium on Variational Concepts with Applications to the Mechanics of Materials; Springer: Dordrecht, The Netherlands, 2010; pp. 15–28. [Google Scholar]
- Avrami, M. Kinetics of Phase Change. I General Theory. J. Chem. Phys. 1939, 7, 1103–1112. [Google Scholar] [CrossRef]
- Johnson, W.A.; Mehl, R.F. Reaction kinetics in processes of nucleation and growth. Trans. Am. Inst. Min. Metall. Eng. 1939, 135, 416–442. [Google Scholar]
- Kolmogorov, A. On the Statistical Theory of Metal Crystallization. Bull. Acad. Sci. USSR Math. Ser. 1937, 1, 355–359. [Google Scholar]
- Leblond, J.B.; Devaux, J. A new kinetic model for anisothermal metallurgical transformations in steels including effect of austenite grain size. Acta Metall. 1984, 32, 137–146. [Google Scholar] [CrossRef]
- Milenin, I.; Pernach, M.; Pietrzyk, M. Application of the control theory to modelling austenite-ferrite phase transformation in steels. Comput. Methods Mater. Sci. 2015, 15, 327–335. [Google Scholar]
- Halder, C.; Madej, L.; Pietrzyk, M. Discrete micro-scale cellular automata model for modelling phase transformation during heating of dual phase steels. Arch. Civ. Mech. Eng. 2014, 14, 96–103. [Google Scholar] [CrossRef]
- Kuijing, S.; Wei, Y.; Dong, Z.; Wenjian, Z.; Fang, K.; Xiaoying, W. Cellular Automaton Modeling of Diffusion, Mixed and Interface Controlled Phase Transformation. J. Phase Equilibria Diffus. 2015, 36, 136–148. [Google Scholar] [CrossRef]
- Militzer, M. Phase field modeling of microstructure evolution in steels. Curr. Opin. Solid State Mater. Sci. 2011, 15, 106–115. [Google Scholar] [CrossRef]
- Yin, X.; Chen, W.; To, A.; McVeigh, C.; Liu, W.K. Statistical volume element method for predicting microstructure–constitutive property relations. Comput. Methods Appl. Mech. Eng. 2008, 197, 3516–3529. [Google Scholar] [CrossRef]
- Ostoja-Starzewski, M. Material spatial randomness: From statistical to representative volume element. Probabilistic Eng. Mech. 2006, 21, 112–132. [Google Scholar] [CrossRef]
- Rauch, Ł.; Bzowski, K.; Szeliga, D.; Pietrzyk, M. Development and Application of the Statistically Similar Representative Volume Element for Numerical Modelling of Multiphase Materials; Springer: Cham, Switzerland, 2020; pp. 389–402. [Google Scholar]
- Schröder, J.; Balzani, D.; Brands, D. Approximation of random microstructures by periodic statistically similar representative volume elements based on lineal-path functions. Arch. Appl. Mech. 2011, 81, 975–997. [Google Scholar] [CrossRef]
- Rauch, L.; Szeliga, D.; Bachniak, D.; Bzowski, K.; Słota, R.; Pietrzyk, M.; Kitowski, J. Identification of Multi-inclusion Statistically Similar Representative Volume Element for Advanced High Strength Steels by Using Data Farming Approach. Procedia Comput. Sci. 2015, 51, 924–933. [Google Scholar] [CrossRef] [Green Version]
- Osher, S.; Sethian, J.A. Fronts propagating with curvature-dependent speed: Algorithms based on Hamilton-Jacobi formulations. J. Comput. Phys. 1988, 79, 12–49. [Google Scholar] [CrossRef] [Green Version]
- Zhang, X.; Chen, J.-S.; Osher, S. A multiple level set method for modeling grain boundary evolution of polycrystalline materials. Interact. Multiscale Mech. 2008, 1, 191–209. [Google Scholar] [CrossRef]
- Bernacki, M.; Logé, R.E.; Coupez, T. Level set framework for the finite-element modelling of recrystallization and grain growth in polycrystalline materials. Scr. Mater. 2011, 64, 525–528. [Google Scholar] [CrossRef]
- Osher, S.; Fedkiw, R. Level Set Methods and Dynamic Implicit Surfaces; Springer: New York, NY, USA, 2003. [Google Scholar]
- Adalsteinsson, D.; Sethian, J.A. A Fast Level Set Method for Propagating Interfaces. J. Comput. Phys. 1995, 118, 269–277. [Google Scholar] [CrossRef] [Green Version]
- Roh, W.; Kikuchi, N. Analysis of Stefan Problem with Level Set Method. In Proceedings of the 8th AIAA/ASME Joint Thermophysics and Heat Transfer Conference, St. Louis, MO, USA, 23–25 June 2002. [Google Scholar]
- Sussman, M.; Smereka, P.; Osher, S. A Level Set Approach for Computing Solutions to Incompressible Two-Phase Flow. J. Comput. Phys. 1994, 114, 146–159. [Google Scholar] [CrossRef]
- Allaire, G.; Jouve, F.; Toader, A.-M. Structural optimization using sensitivity analysis and a level-set method. J. Comput. Phys. 2004, 194, 363–393. [Google Scholar] [CrossRef] [Green Version]
- Bzowski, K.; Rauch, L.; Pietrzyk, M. Application of statistical representation of the microstructure to modeling of phase transformations in DP steels by solution of the diffusion equation. Procedia Manuf. 2018, 15, 1847–1855. [Google Scholar] [CrossRef]
- Arndt, D.; Bangerth, W.; Blais, B.; Clevenger, T.C.; Fehling, M.; Grayver, A.V.; Heister, T.; Heltai, L.; Kronbichler, M.; Maier, M.; et al. The deal.II library, Version 9.2. J. Numer. Math. 2020, 28, 131–146. [Google Scholar] [CrossRef]
- Fang, H.; Mecozzi, M.G.; Brück, E.; van der Zwaag, S.; van Dijk, N.H. Analysis of the Grain Size Evolution for Ferrite Formation in Fe-C-Mn Steels Using a 3D Model Under a Mixed-Mode Interface Condition. Met. Mat. Trans. A 2018, 49, 41–53. [Google Scholar] [CrossRef] [Green Version]
- Bhadeshia, H.K.D.H. Diffusion of carbon in austenite. Met. Sci. 1981, 15, 477–480. [Google Scholar] [CrossRef]
- Thibaux, P.; Métenier, A.; Xhoffer, C. Carbon Diffusion Measurement in Austenite in the Temperature Range 500 °C to 900 °C. Met. Mat. Trans. A 2007, 38, 1169–1176. [Google Scholar] [CrossRef]
- Ågren, J. A revised expression for the diffusivity of carbon in binary Fe-C austenite. Scr. Metall. 1986, 20, 1507–1510. [Google Scholar] [CrossRef]
- Strangwood, M. 6—Fundamentals of ferrite formation in steels. In Phase Transformations in Steels; Pereloma, E., Edmonds, D.V., Eds.; Woodhead Publishing: Sawston, Cambridge, UK, 2012; Volume 1, pp. 187–224. [Google Scholar]
- Offerman, S.; van Dijk, N.; Sietsma, J.; Grigull, S.; Lauridsen, E.; Margulies, L.; Poulsen, H.F.; Rekveldt, M.; Zwaag, S. Grain Nucleation and Growth During Phase Transformations. Science 2002, 298, 1003–1005. [Google Scholar] [CrossRef] [Green Version]
- Cahn, J.W. The kinetics of grain boundary nucleated reactions. Acta Metall. 1956, 4, 449–459. [Google Scholar] [CrossRef]
- Collet, J.-L.; Kwiaton, N.; Madej, L.; Radwański, K.; Iung, T.; Halder, C.; Kuziak, R.; Hell, J.C.; Opara, J.; Zalecki, W.; et al. Property Oriented Design of Hard Constituent Hardness and Morphology in Continuously Annealed/Galvanised DP Sheets; European Commission: Luxembourg, 2015. [Google Scholar]






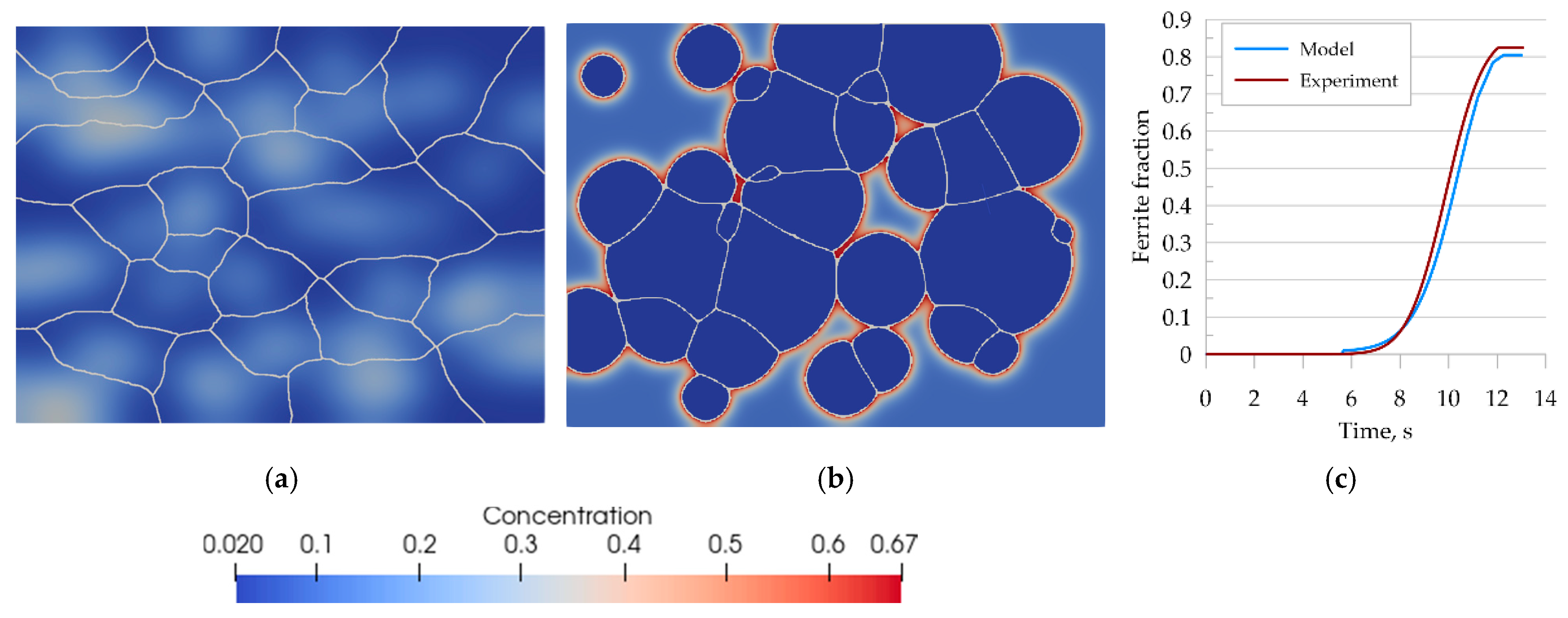

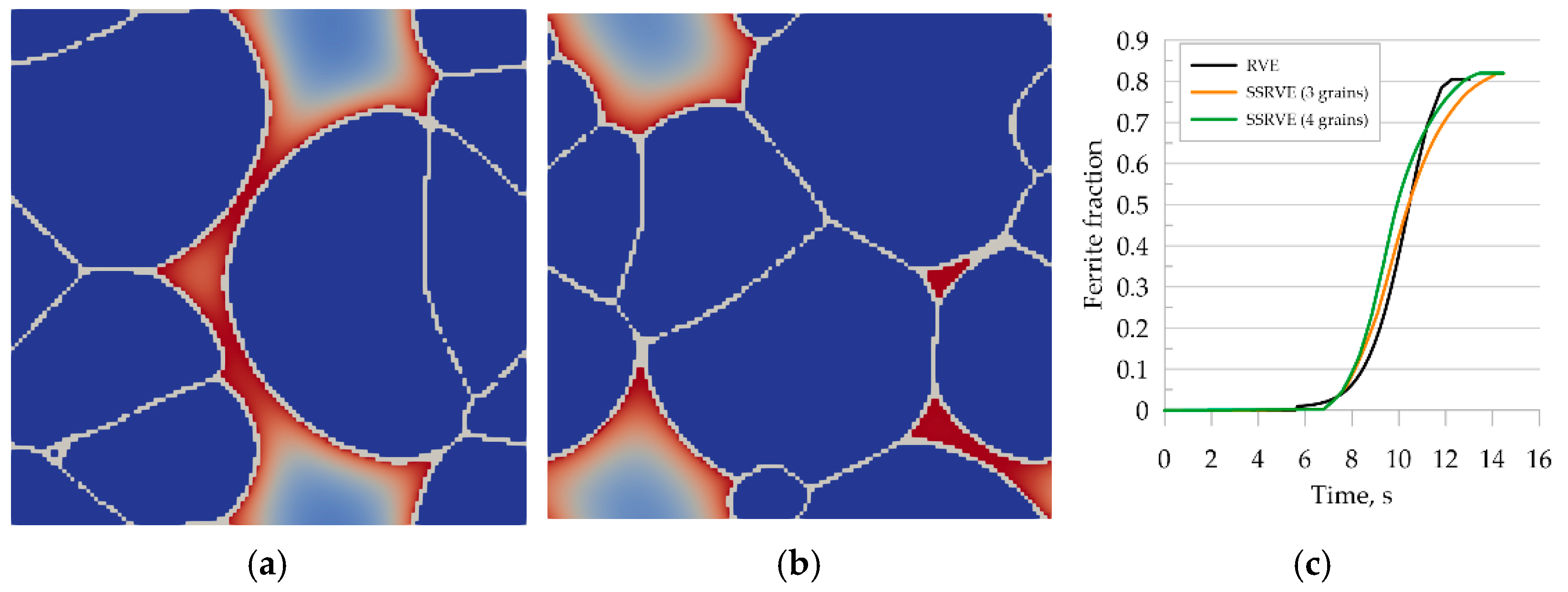

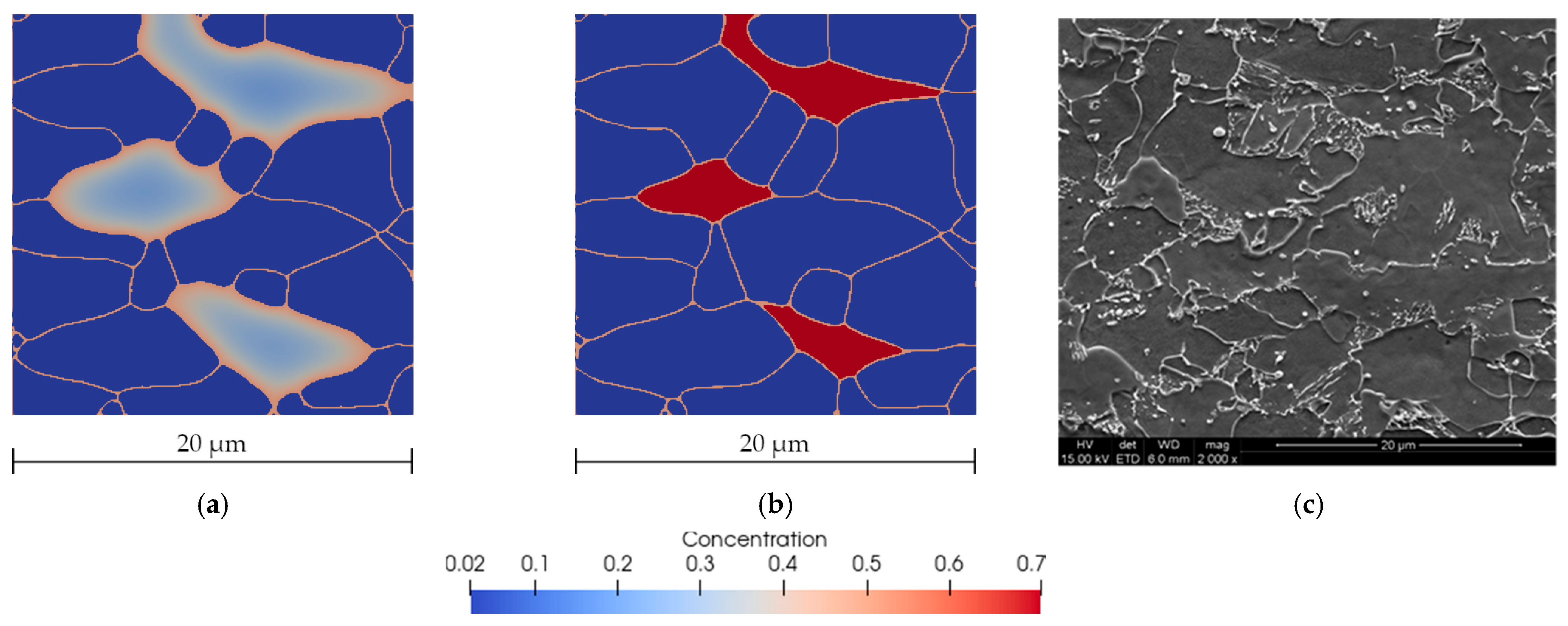
| Steel | C | Al | Cr | Cu | Mn | Mo | Ni | Si | N | P | S |
|---|---|---|---|---|---|---|---|---|---|---|---|
| A | 0.13 | 0.003 | 0.06 | 0.27 | 0.47 | 0.02 | 0.09 | 0.16 | 0.009 | 0.018 | 0.025 |
| B | 0.09 | 0.053 | 0.35 | 0.03 | 1.42 | 0.005 | 0.01 | 0.1 | 0.009 | 0.011 | 0.01 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bzowski, K.; Rauch, Ł.; Pietrzyk, M.; Kwiecień, M.; Muszka, K. Numerical Modeling of Phase Transformations in Dual-Phase Steels Using Level Set and SSRVE Approaches. Materials 2021, 14, 5363. https://doi.org/10.3390/ma14185363
Bzowski K, Rauch Ł, Pietrzyk M, Kwiecień M, Muszka K. Numerical Modeling of Phase Transformations in Dual-Phase Steels Using Level Set and SSRVE Approaches. Materials. 2021; 14(18):5363. https://doi.org/10.3390/ma14185363
Chicago/Turabian StyleBzowski, Krzysztof, Łukasz Rauch, Maciej Pietrzyk, Marcin Kwiecień, and Krzysztof Muszka. 2021. "Numerical Modeling of Phase Transformations in Dual-Phase Steels Using Level Set and SSRVE Approaches" Materials 14, no. 18: 5363. https://doi.org/10.3390/ma14185363
APA StyleBzowski, K., Rauch, Ł., Pietrzyk, M., Kwiecień, M., & Muszka, K. (2021). Numerical Modeling of Phase Transformations in Dual-Phase Steels Using Level Set and SSRVE Approaches. Materials, 14(18), 5363. https://doi.org/10.3390/ma14185363







