Effect of Loading Frequency Ratio on Multiaxial Asynchronous Fatigue Failure of 30CrMnSiA Steel
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Experimental Methods
3. Results and Discussion
3.1. Multiaxial Fatigue Life
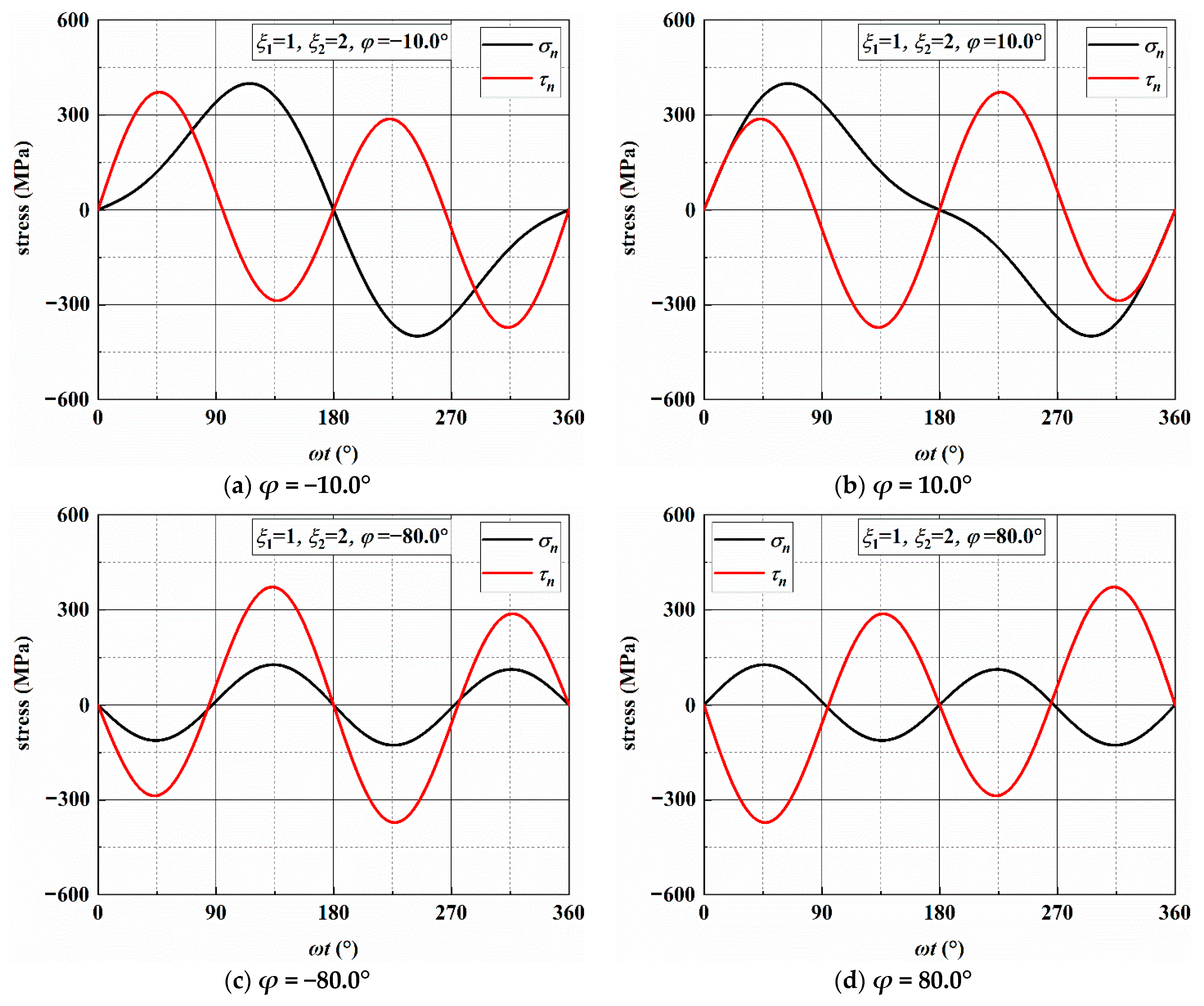
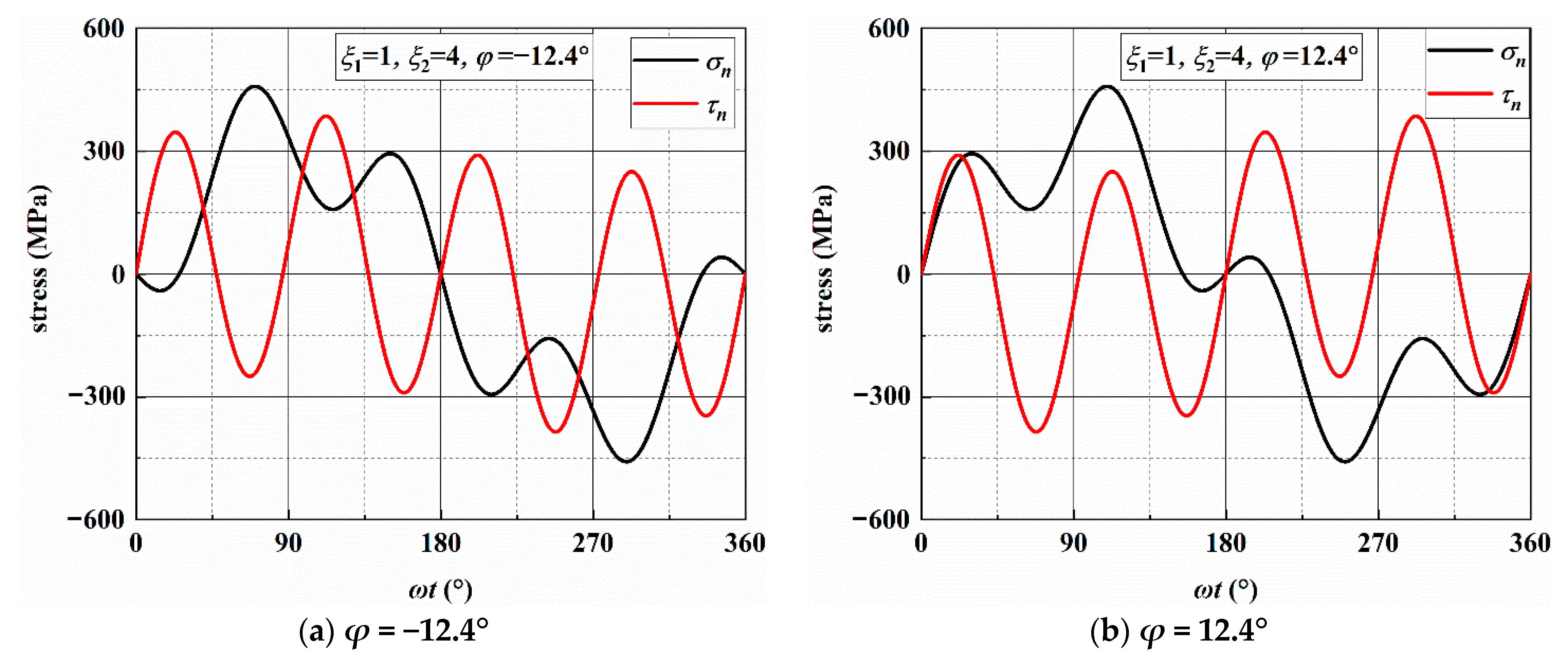
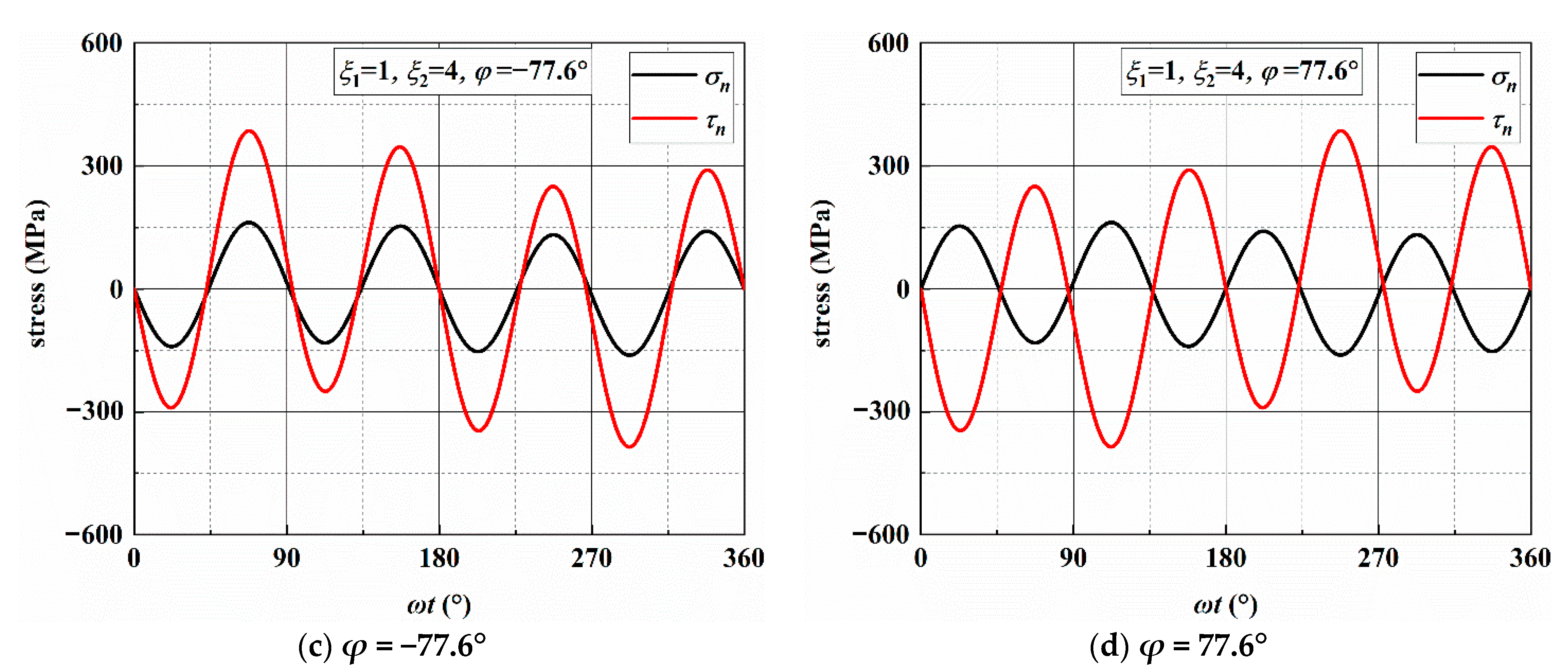
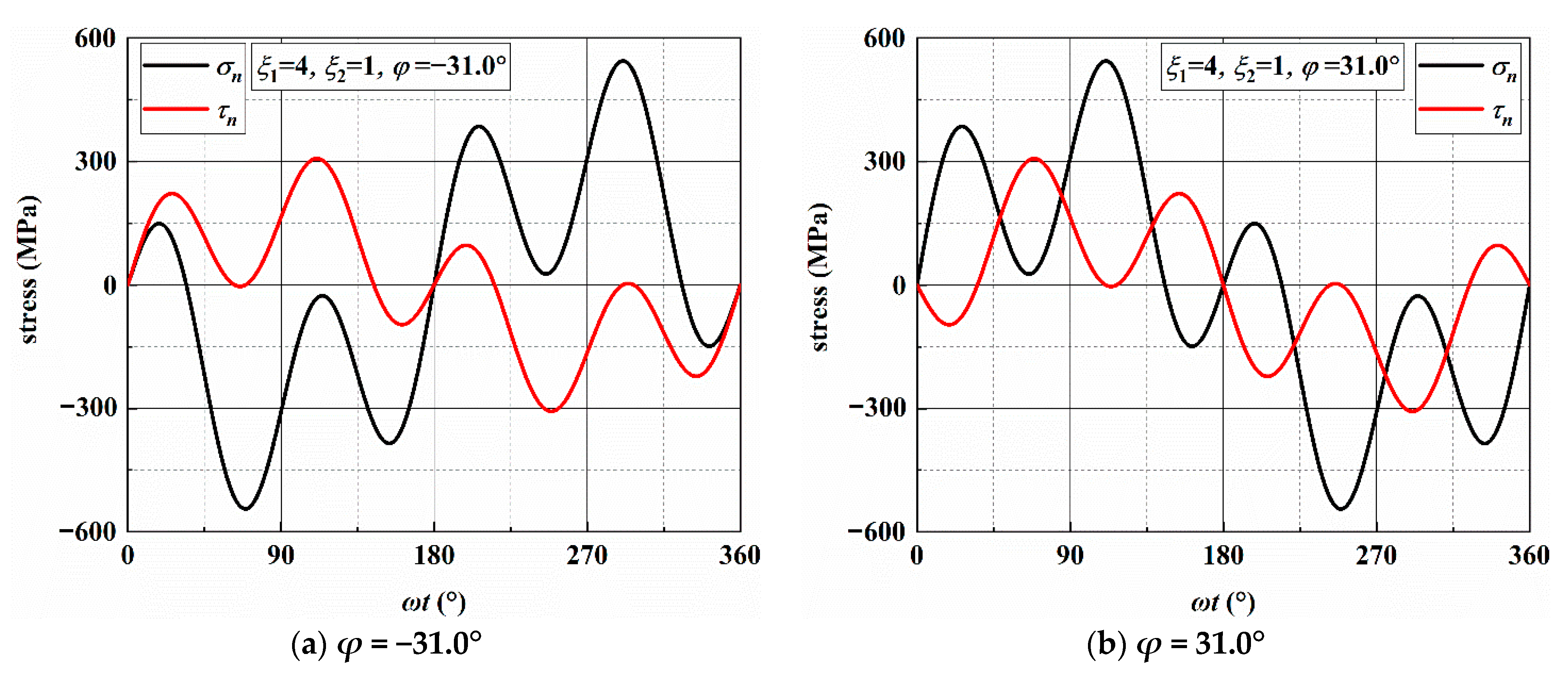
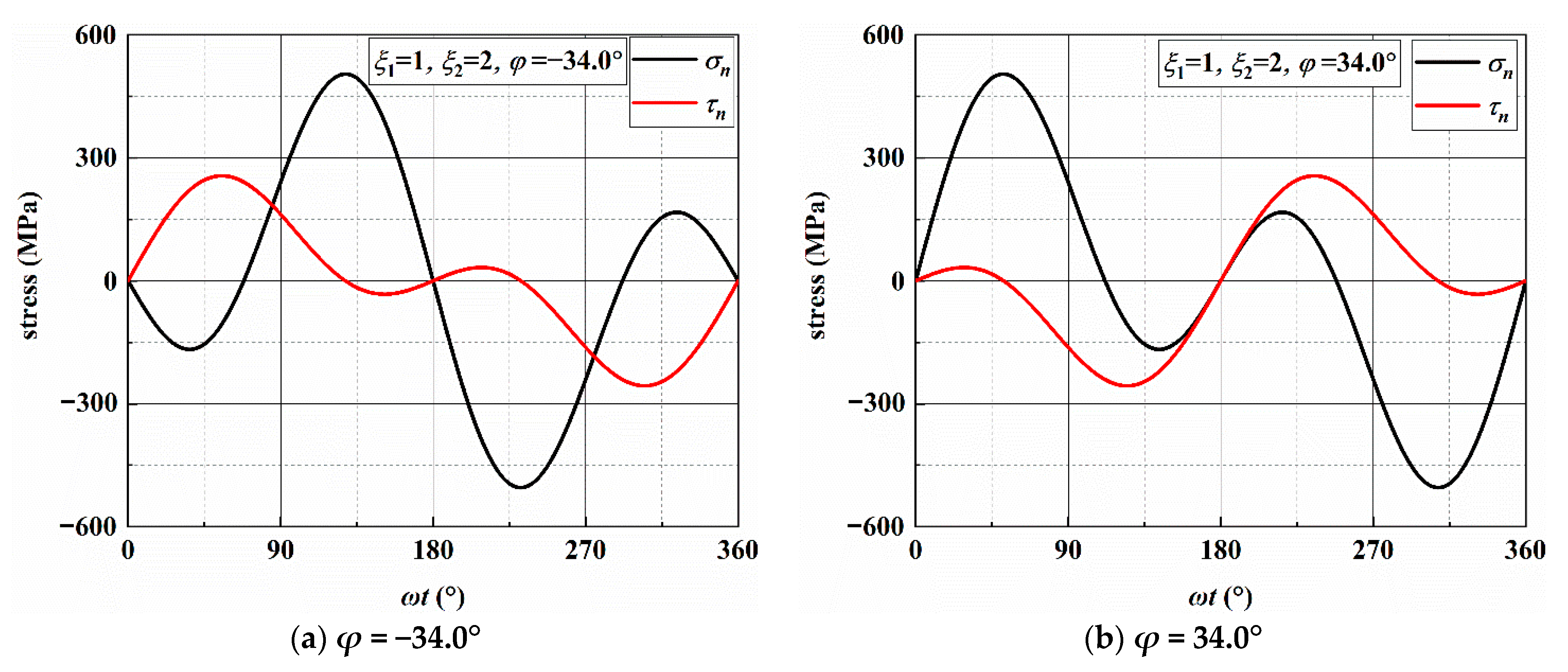
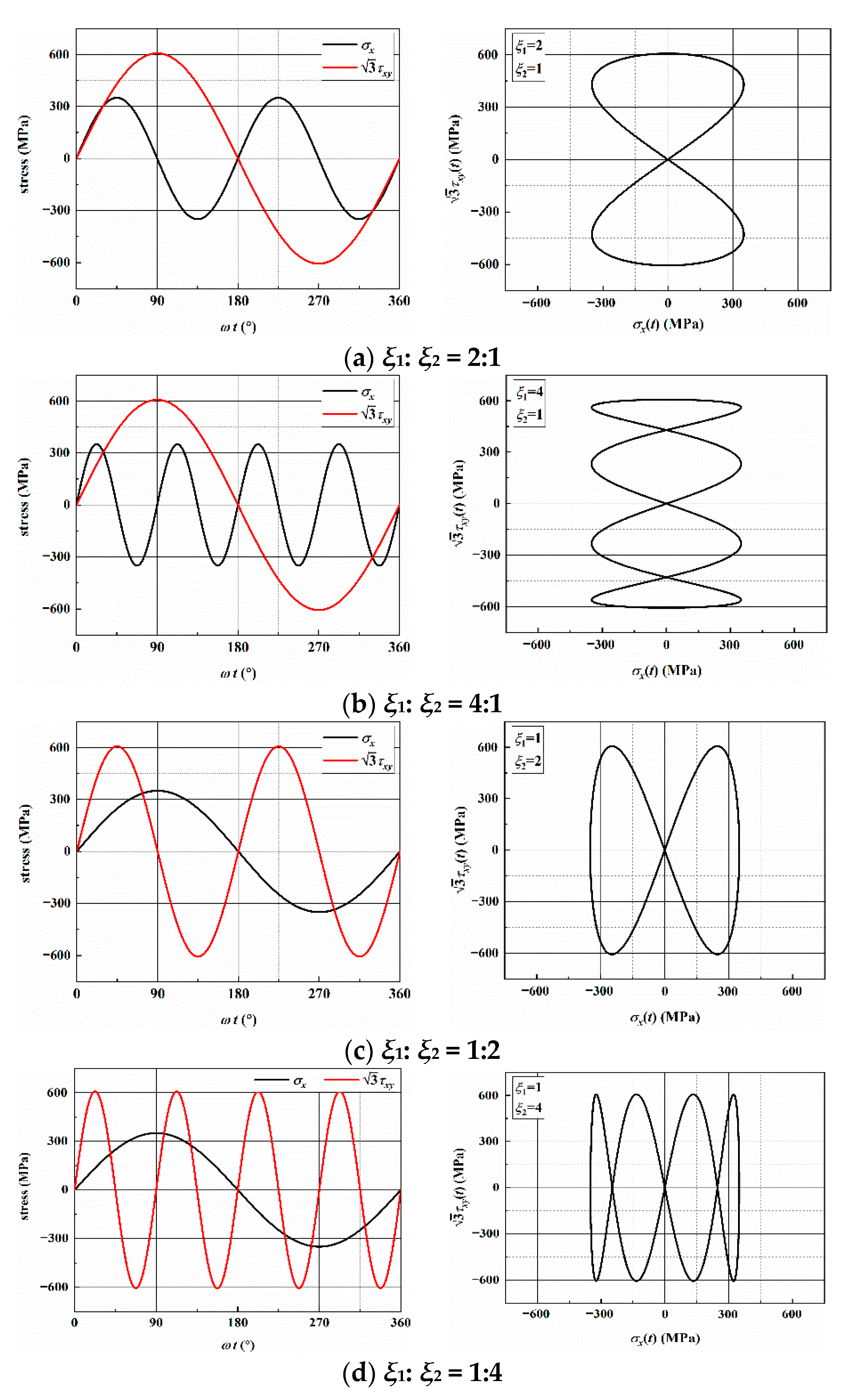
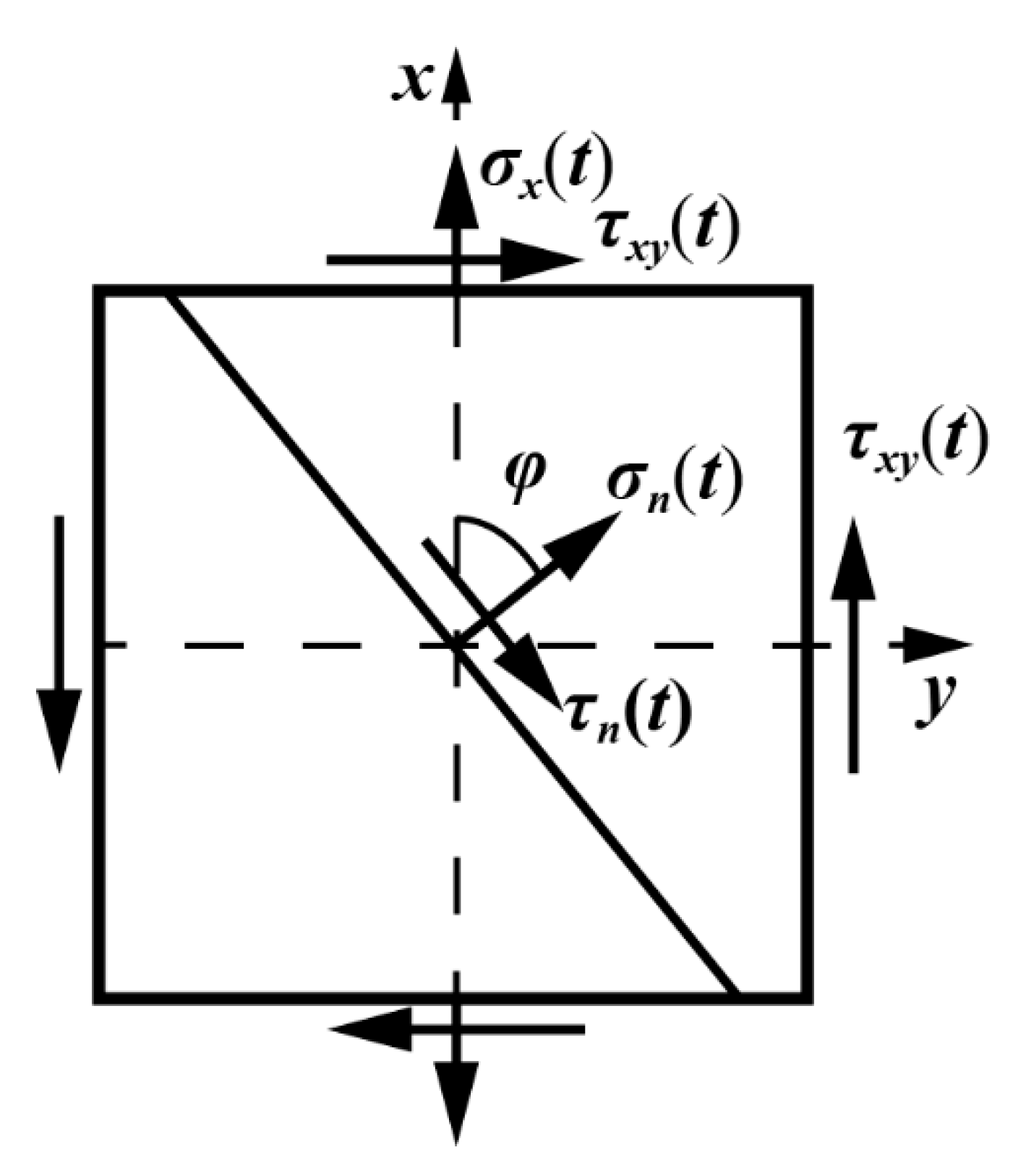
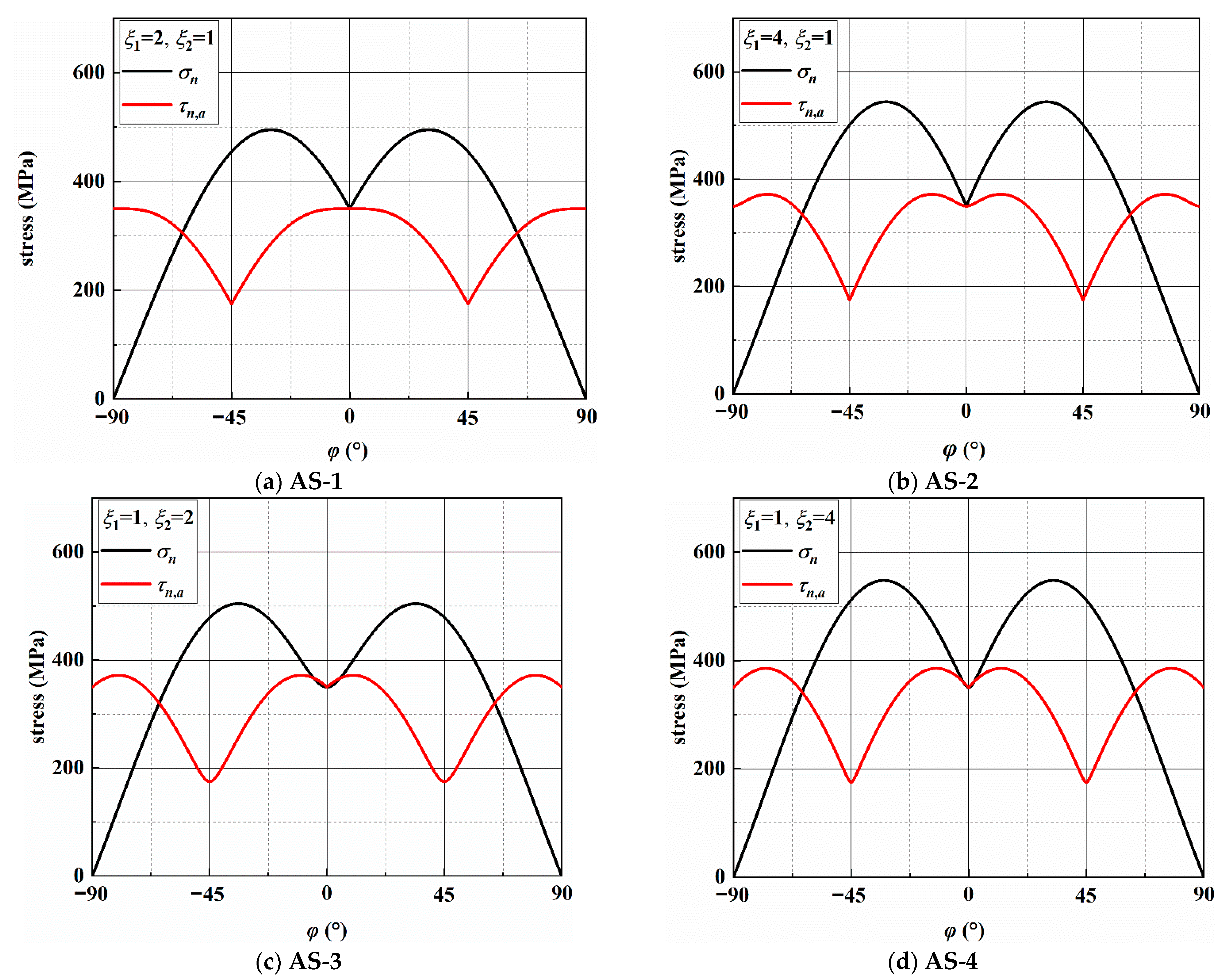
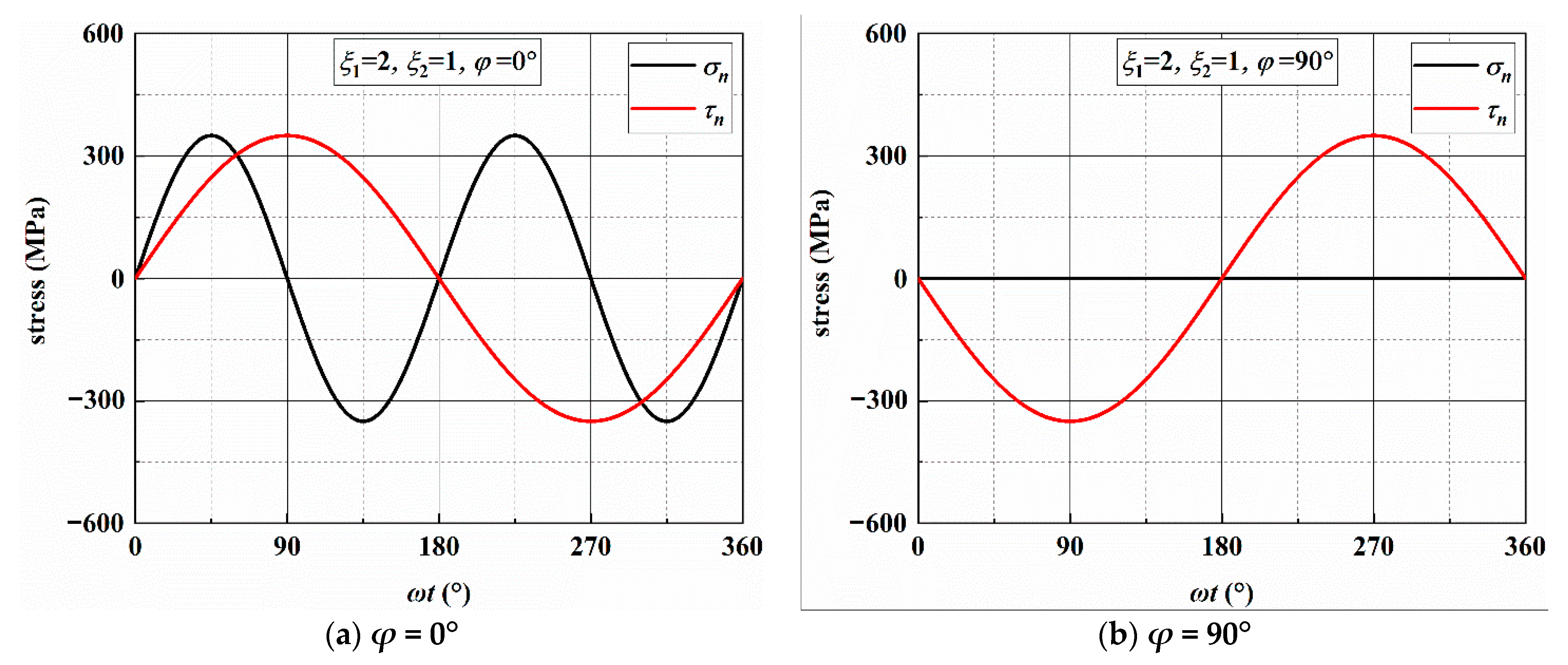
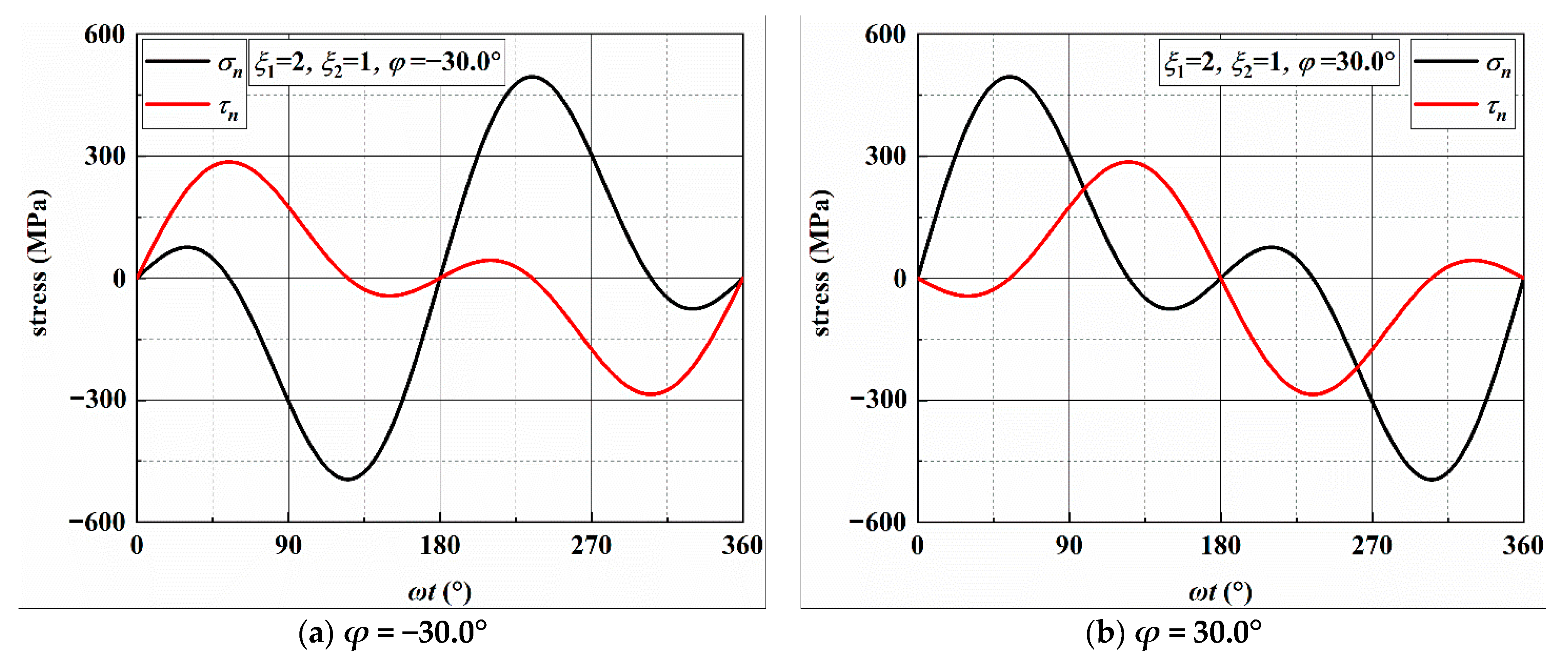
3.2. Stress Analysis of Individual Loading Blocks
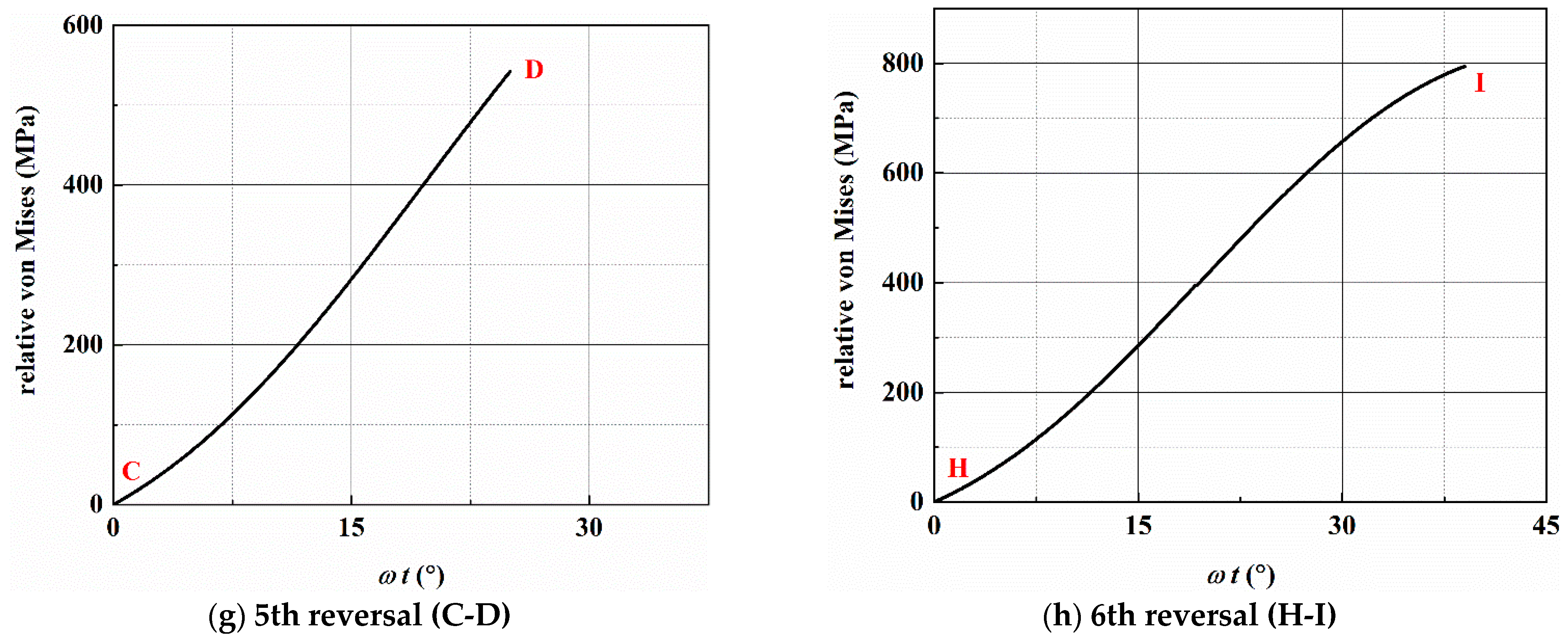
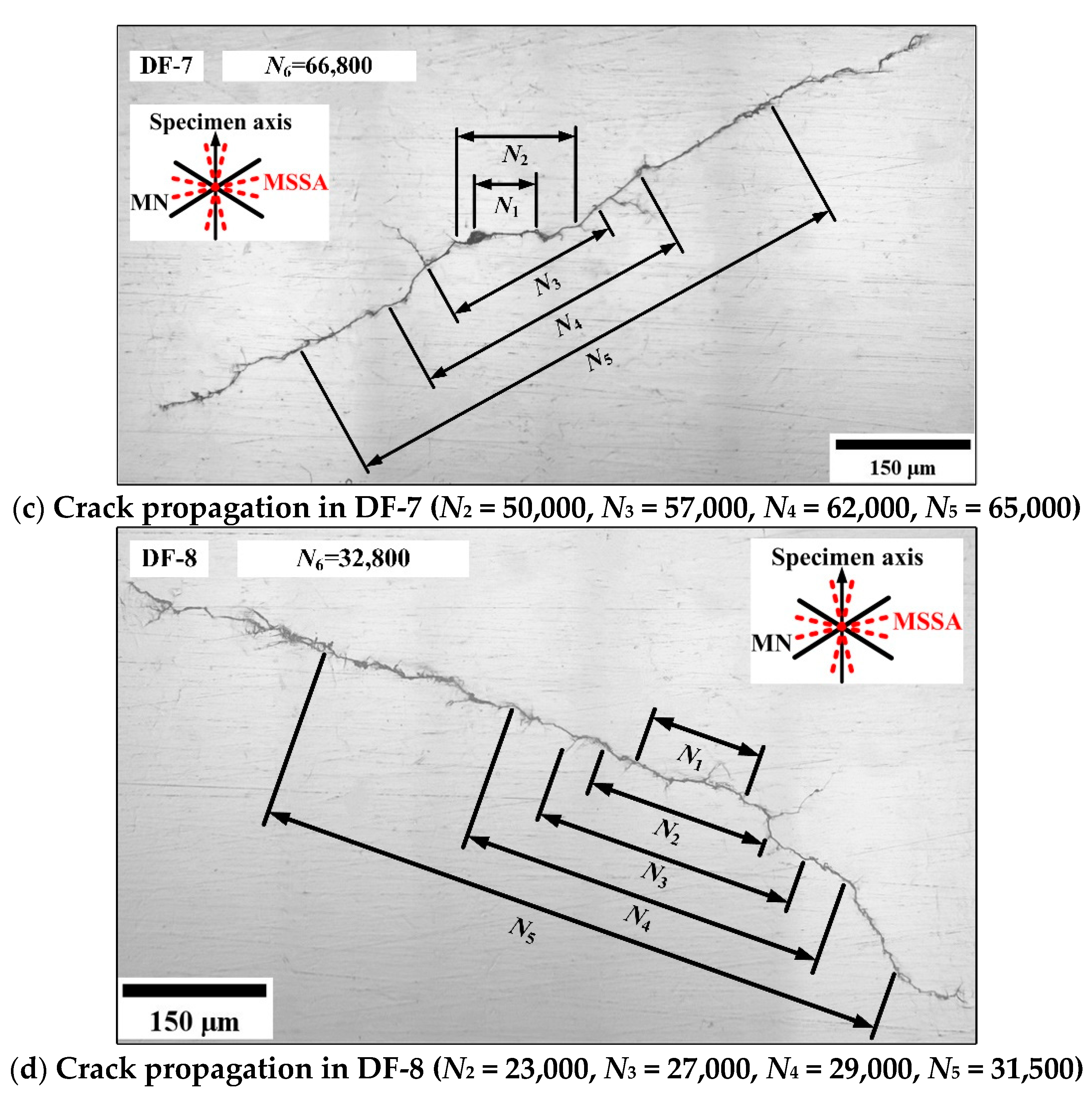
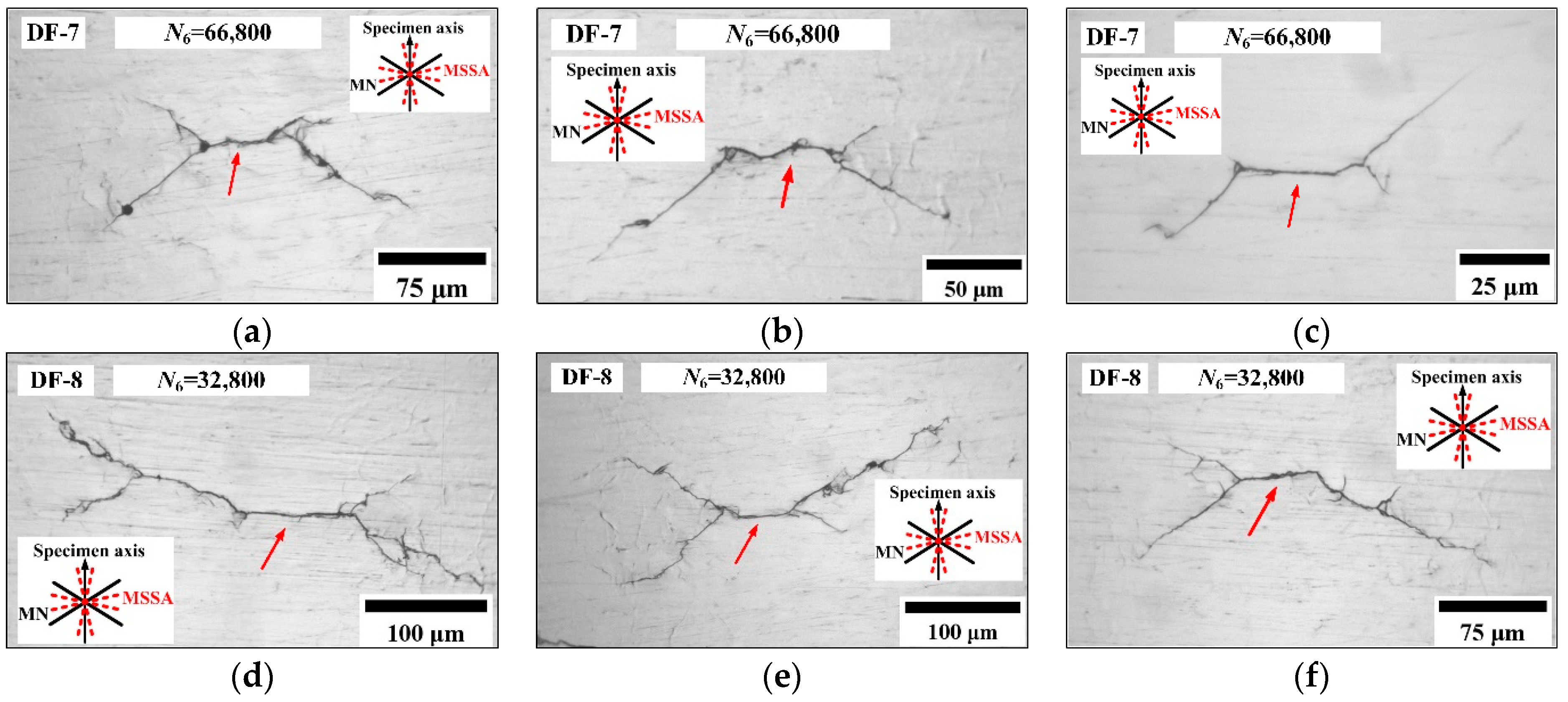
3.3. Crack Initiation and Propagation
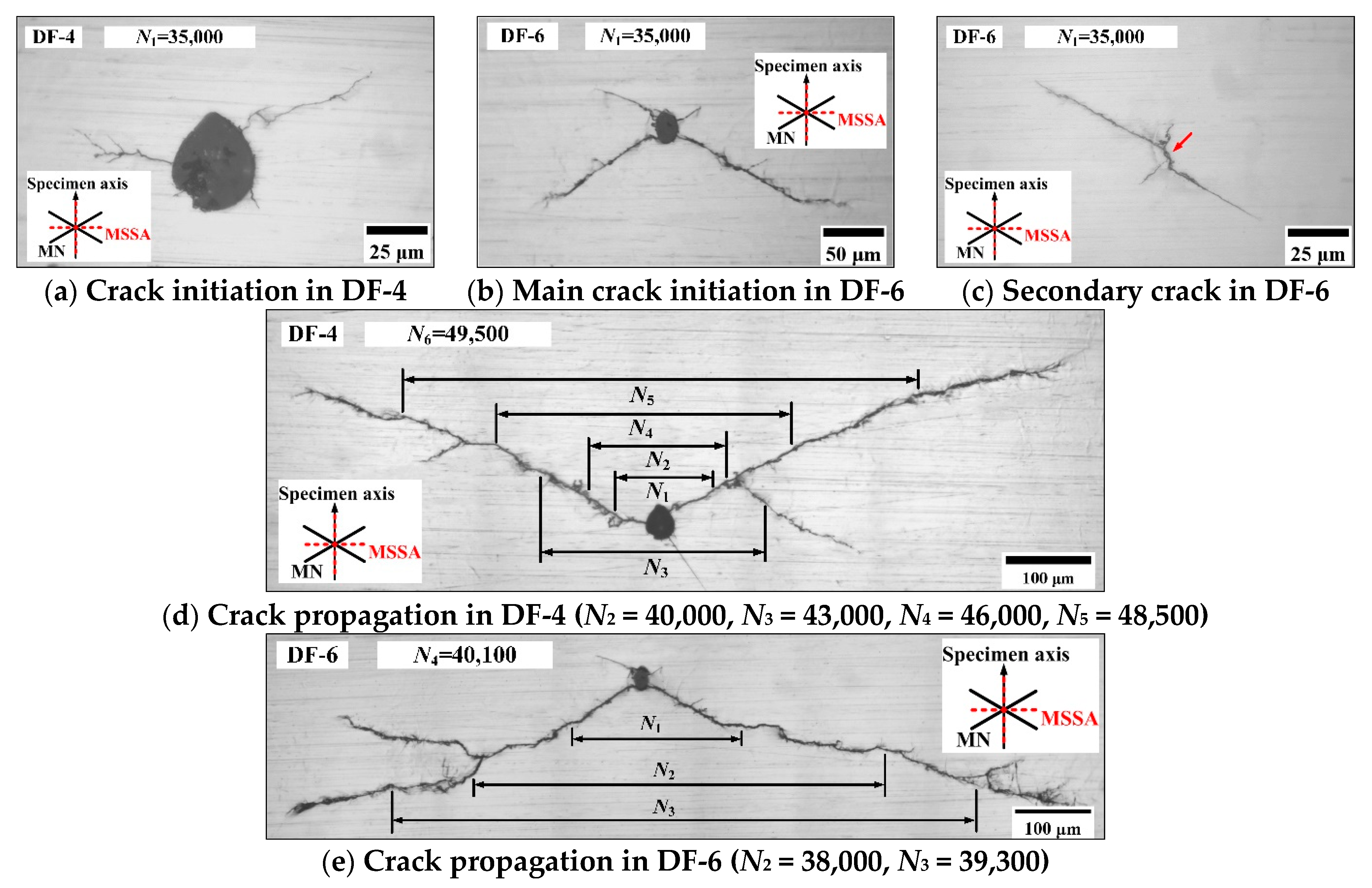
3.3.1. Crack Growth Paths of AS-1
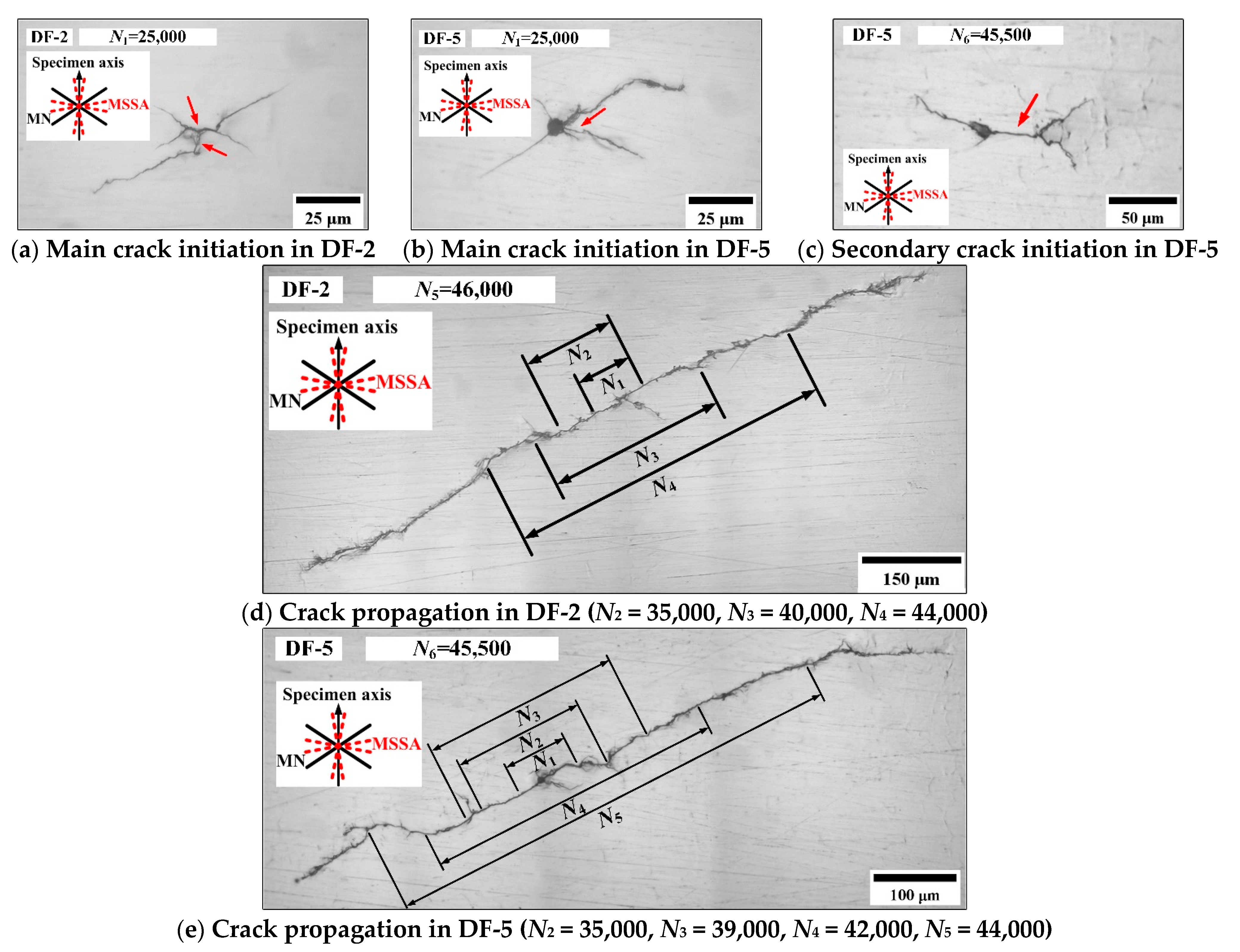
3.3.2. Crack Growth Paths of AS-2
3.3.3. Crack Growth Paths of AS-3
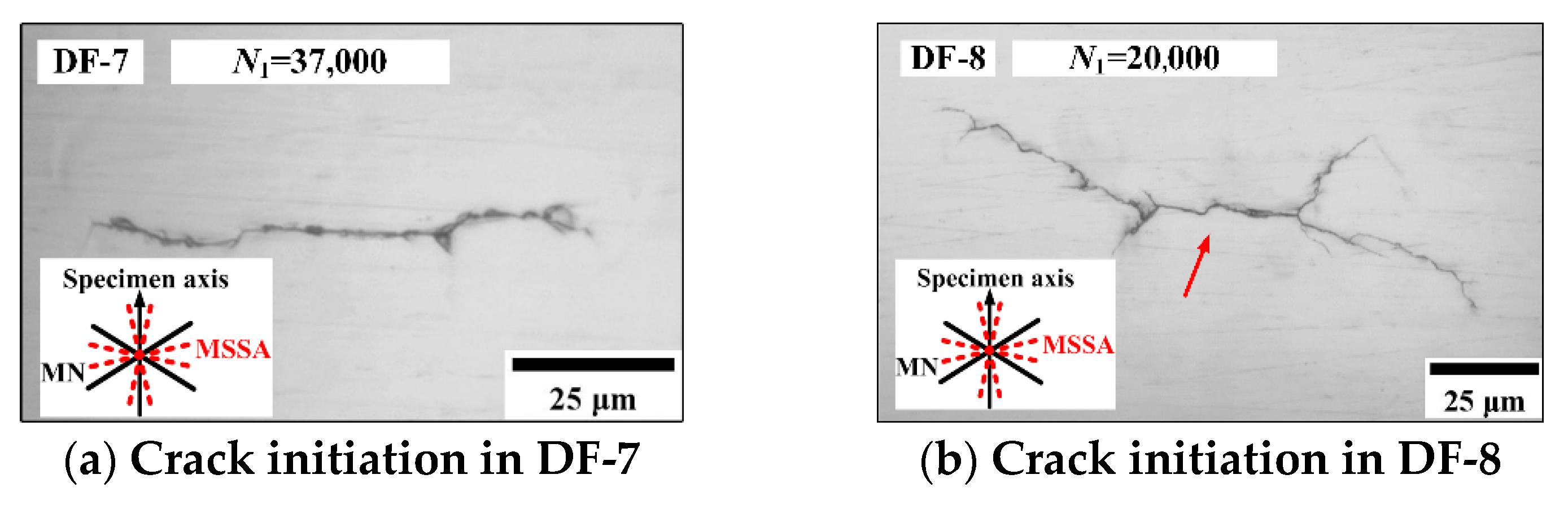
3.3.4. Crack Growth Paths of AS-4
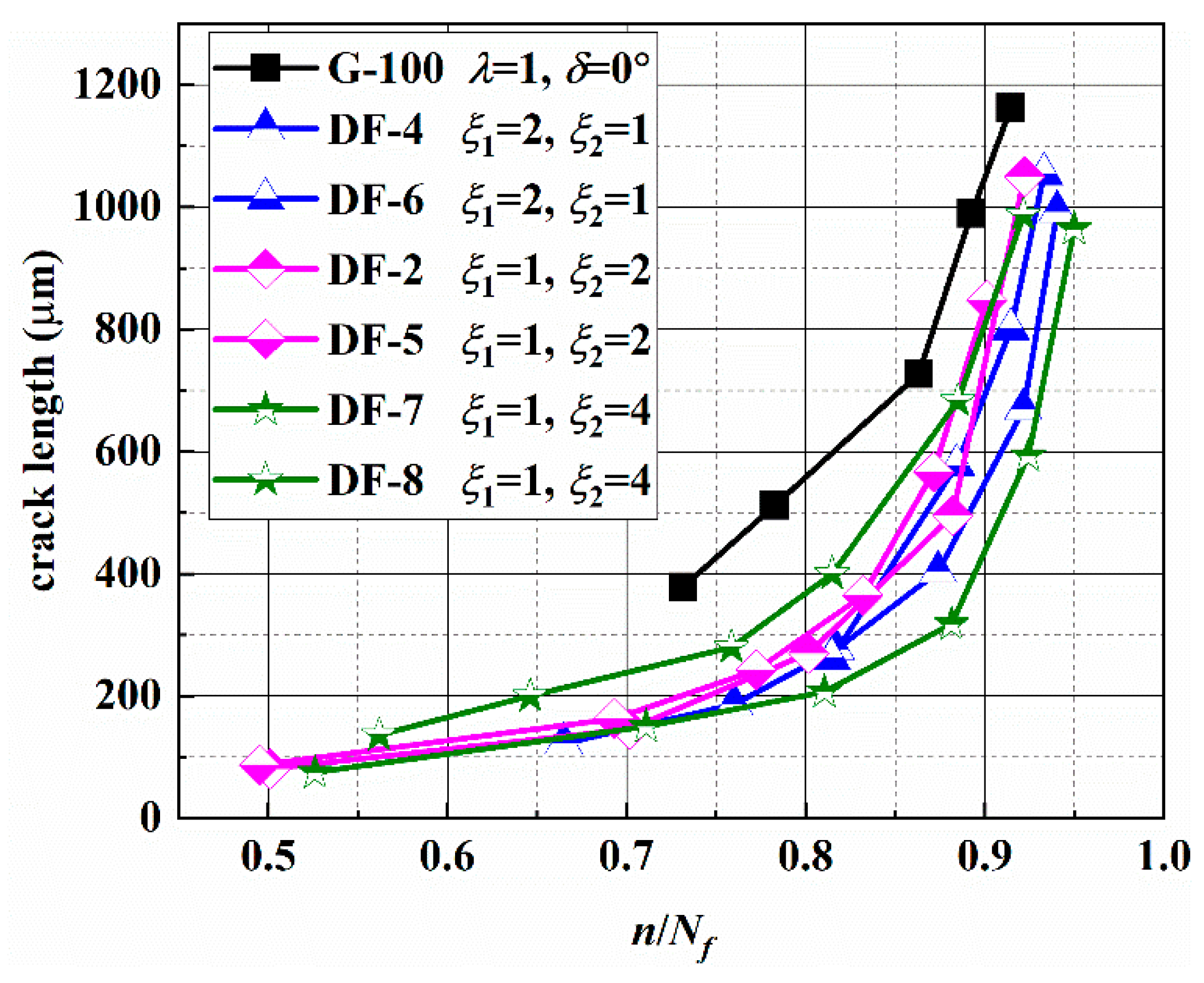
3.4. Crack Length versus Loading Blocks
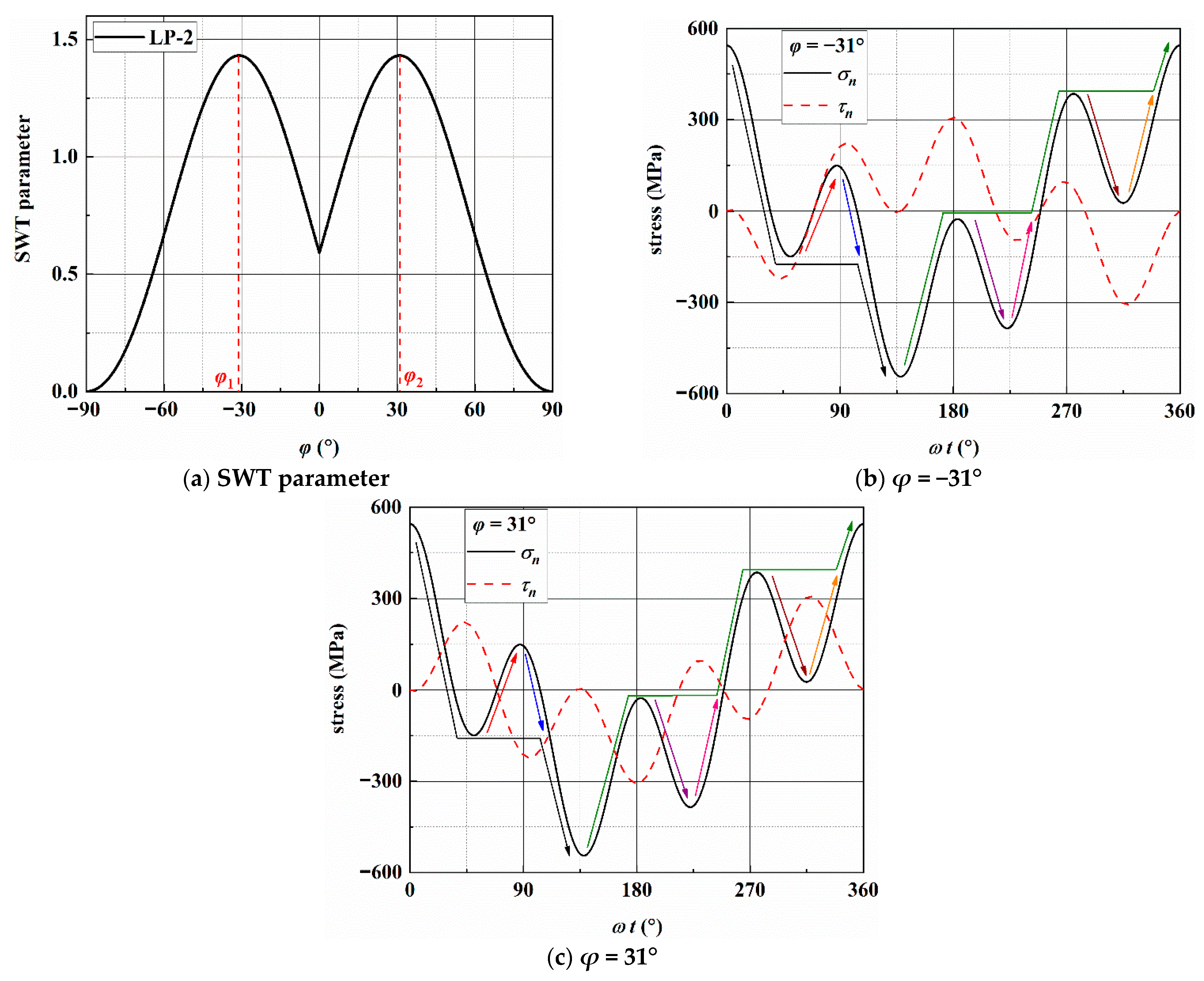
3.5. Fatigue Life Prediction
3.5.1. Existing Multiaxial Cycle Counting Method
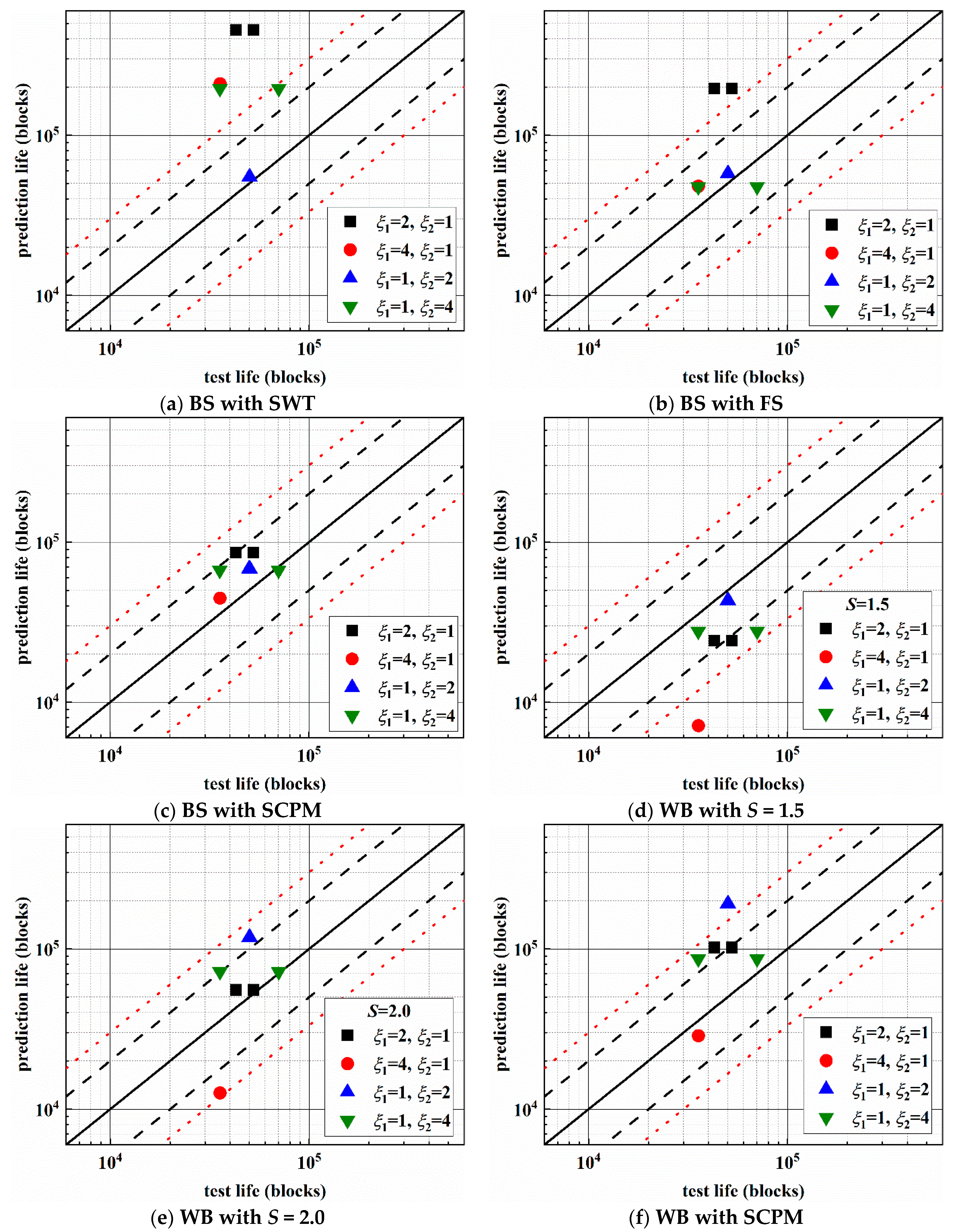
3.5.2. Fatigue Life Prediction Results
4. Conclusions
- (a)
- The experimental results show that fatigue failure life under asynchronous loadings decreases when the value of ξ1 or ξ2 increase from 1 to 2, and there is no significant change when the value of ξ1 or ξ2 increase from 2 to 4.
- (b)
- Based on the observation of the surface crack path, the crack initiates on the maximum shear stress amplitude plane with larger normal stress and propagates along the maximum normal stress planes. The proportion of the stage II crack propagation life is more than 50% of the fatigue failure life.
- (c)
- Under asynchronous loadings, the increasing of ξ1 or ξ2 results in more shear stress cycles and larger shear stress amplitude on the MSSA planes in a loading block, and this may be the reason for the increase of secondary cracks. Furthermore, the difference between the load cycle amplitudes on the MN plane causes the crack retardation and leads to the crack growth length being longer for the constant amplitude loading than that for the asynchronous loading under the same fatigue life ratio.
- (d)
- The applicability of Bannantine-Socie and Wang-Brown counting method is verified under multiaxial asynchronous fatigue loading with different fatigue failure criteria and Palmgren-Miner’s cumulative damage rule. The results indicate that the accuracy of the Bannantine-Socie model with the section critical plane method is higher than that of the others.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| E | Young’s modulus |
| G | shear modulus |
| Nf | fatigue failure life |
| N50 | logarithmic mean fatigue failure life |
| S | material parameter of Smith-Watson-Topper model |
| k | material parameter of Fatemi-Socie model |
| b | axial fatigue strength exponent |
| b0 | shear fatigue strength exponent |
| c | axial fatigue ductility exponent |
| c0 | shear fatigue ductility exponent |
| ξ1 | axial stress frequency |
| ξ2 | shear stress frequency |
| φ | direction of an arbitrary plane |
| ν’ | effective Poisson Ratio |
| σy | tensile yield strength |
| σu | tensile ultimate strength |
| τy | torsional yield strength |
| τu | torsional ultimate strength |
| σx(t) | axial stress |
| σx,a | axial stress amplitude |
| τxy(t) | shear stress |
| τxy,a | torsion stress amplitude |
| σn(t) | cyclic normal stress on the plane of φ |
| τn(t) | cyclic shear stress on the plane of φ |
| σn,max | maximum normal stress |
| σn | normal stress on the plane of φ |
| σn,a | normal stress amplitude on the plane of φ |
| τn,a | shear stress amplitude on the plane of φ |
| σn,m | mean normal stress |
| τna,max | maximum shear stress amplitude |
| γna,max | maximum shear strain amplitude |
| εn,a | principal strain amplitude |
| σ’f | axial fatigue strength coefficient |
| τ’f | shear fatigue strength coefficient |
| ε’f | axial fatigue ductility coefficient |
| γ’f | shear fatigue ductility coefficient |
Abbreviations
| AS | asynchronous loading path |
| MN | maximum normal |
| MSSA | maximum shear stress amplitude |
Appendix A
| Spec. ID | Contents | N1 | N2 | N3 | N4 | N5 | N6 |
|---|---|---|---|---|---|---|---|
| DF-4 | blocks | 35,000 | 40,000 | 43,000 | 46,000 | 48,500 | 49,500 |
| 2a (μm) | 122.5 | 186 | 276 | 403 | 669 | 993 | |
| DF-6 | blocks | 35,000 | 38,000 | 39,300 | 40,100 | — | — |
| 2a (μm) | 261 | 577 | 800 | 1054 | — | — | |
| DF-2 | blocks | 25,000 | 35,000 | 40,000 | 44,000 | 46,000 | — |
| 2a (μm) | 81 | 146 | 270 | 495 | 1050 | — | |
| DF-5 | blocks | 25,000 | 35,000 | 39,000 | 42,000 | 44,000 | 45,500 |
| 2a (μm) | 87 | 163 | 242 | 364 | 567 | 848 | |
| DF-9 | blocks | 32,000 | — | — | — | — | — |
| 2a (μm) | 920 | — | — | — | — | — | |
| DF-7 | blocks | 37,000 | 50,000 | 57,000 | 62,000 | 65,000 | 66,800 |
| 2a (μm) | 75 | 150 | 207 | 318 | 593 | 964 | |
| DF-8 | blocks | 20,000 | 23,000 | 27,000 | 29,000 | 31,500 | 32,800 |
| 2a (μm) | 136 | 200 | 280 | 400 | 683 | 987 |



References
- Luke, M.; Varfolomeev, I.; Lütkepohl, K.; Esderts, A. Fatigue crack growth in railway axles: Assessment concept and validation tests. Eng. Fract. Mech. 2011, 78, 714–730. [Google Scholar] [CrossRef]
- Tomaszewski, T. Fatigue life analysis of steel bicycle frame according to ISO 4210. Eng. Fail. Anal. 2021, 122, 105195. [Google Scholar] [CrossRef]
- Varfolomeev, I.; Luke, M.; Moroz, S. Experimental and numerical investigations of fatigue crack growth in various specimen geometries. Procedia Eng. 2010, 2, 1829–1837. [Google Scholar] [CrossRef]
- Berto, F.; Lazzarin, P. Fatigue strength of structural components under multi-axial loading in terms of local energy density averaged on a control volume. Int. J. Fatigue 2011, 33, 1055–1065. [Google Scholar] [CrossRef]
- Wu, S.C.; Luo, Y.; Shen, Z.; Zhou, L.C.; Zhang, W.H.; Kang, G.Z. Collaborative crack initiation mechanism of 25CrMo4 alloy steels subjected to foreign object damages. Eng. Fract. Mech. 2020, 225, 106844. [Google Scholar] [CrossRef]
- Liu, T.; Shi, X.; Zhang, J.; Fei, B. Crack initiation and propagation of 30CrMnSiA steel under uniaxial and multiaxial cyclic loading. Int. J. Fatigue 2019, 122, 240–255. [Google Scholar] [CrossRef]
- Liu, T.; Shi, X.; Zhang, J.; Fei, B. Multiaxial high-cycle fatigue failure of 30CrMnSiA steel with mean tension stress and mean shear stress. Int. J. Fatigue 2019, 129, 105219. [Google Scholar] [CrossRef]
- Wu, S.C.; Xu, Z.W.; Yu, C.; Kafka, O.L.; Liu, W.K. A physically short fatigue crack growth approach based on low cycle fatigue properties. Int. J. Fatigue 2017, 103, 185–195. [Google Scholar] [CrossRef]
- Lesiuk, G.; Duda, M.M.; Correia, J.; de Jesus, A.M.P.; Calçada, R. Fatigue crack growth of 42CrMo4 and 41Cr4 steels under different heat treatment conditions. Int. J. Struct. Integr. 2018, 9, 326–336. [Google Scholar] [CrossRef]
- Bartošák, M.; Horváth, J.; Španiel, M. Isothermal low-cycle fatigue and fatigue-creep of a 42CrMo4 steel. Int. J. Fatigue 2020, 135, 105538. [Google Scholar] [CrossRef]
- Pandiyarajan, R.; Arumugam, K.; Prabakaran, M.P.; Vetrivel Kumar, K. Fatigue life and fatigue crack growth rate analysis of high strength low alloy steel (42CrMo4). Mater. Today Proc. 2021, 37, 1957–1962. [Google Scholar] [CrossRef]
- Kvíčala, M.; Hendrych, A.; Životský, O.; Jandačka, P. The influence of Cr, Mn and Mo elements on cracks occurrence in low-alloyed Cr-Mo steels. Acta Metallurg. Slovaca 2010, 16, 122–126. [Google Scholar]
- Hou, X.-Q.; Li, Y.; Liu, C.-K.; He, Y.-H. Fracture Failure Analysis of a 30CrMnSiA Steel Shaft. J. Fail. Anal. Prev. 2012, 12, 485–489. [Google Scholar] [CrossRef]
- Zhang, W.; Gao, X.; Liu, X.; Liu, X. Fatigue life and stress retro estimation of 30CrMnSiA in laboratory environment. In Proceedings of the 4th International Seminar on Advances in Materials Science and Engineering, ISAMSE 2019, Shanghai, China, 16–18 August 2019; Feng, T., Ed.; Trans Tech Publications Ltd.: Baech, Switzerland, 2020; Volume 984, pp. 3–10. [Google Scholar]
- Hu, Z.; Gao, X.; Zhang, W.; Zhang, W.; Liu, X. Fracture Retro Estimation of Friction Plate Made of 30CrMnSiA in Laboratory Condition. J. Phys. Conf. Ser. 2020, 1653, 012061. [Google Scholar] [CrossRef]
- Chen, C.; Chen, Z.; Li, S.; Zhu, J.; Li, T.; Bian, H. Fracture failure analysis on swivel nut of 30CrMnSiA steel in aircraft. J. Phys. Conf. Ser. 2021, 1786, 012025. [Google Scholar] [CrossRef]
- Mei, J.; Dong, P. Modeling of path-dependent multi-axial fatigue damage in aluminum alloys. Int. J. Fatigue 2017, 95, 252–263. [Google Scholar] [CrossRef]
- Mei, J.; Dong, P. An equivalent stress parameter for multi-axial fatigue evaluation of welded components including non-proportional loading effects. Int. J. Fatigue 2017, 101, 297–311. [Google Scholar] [CrossRef]
- Mei, J.; Dong, P. A new path-dependent fatigue damage model for non-proportional multi-axial loading. Int. J. Fatigue 2016, 90, 210–221. [Google Scholar] [CrossRef]
- Jiang, Y.; Hertel, O.; Vormwald, M. An experimental evaluation of three critical plane multiaxial fatigue criteria. Int. J. Fatigue 2007, 29, 1490–1502. [Google Scholar] [CrossRef]
- Hoffmeyer, J.; Doring, R.; Seeger, T.; Vormwald, M. Deformation behaviour, short crack growth and fatigue livesunder multiaxial nonproportional loading. Int. J. Fatigue 2006, 28, 508–520. [Google Scholar] [CrossRef]
- Noban, M.; Jahed, H.; Winkler, S.; Ince, A. Fatigue characterization and modeling of 30CrNiMo8HH under multiaxial loading. Mater. Sci. Eng. A 2011, 528, 2484–2494. [Google Scholar] [CrossRef]
- Mielke, S. Untersuchungen zum Festigkeitsverhalten bei Mehrachsiger Randombeanspruchung unter Biegung und Torsion; Technische Universitat Clausthal: Clausthal Zellerfeld, Germany, 1980. [Google Scholar]
- Heidenreich, R.; Richter, I.; Zenner, H. Schubspannungsintensittshypothese—Weitere experimentelle und theoretische untersuchungen. Konstruktion 1984, 36, 99–104. [Google Scholar]
- McDiarmid, D.L. Fatigue under out-of-phase biaxial stresses of different frequencies. ASTM Spec. Tech. Publ. 1985, 606–621. [Google Scholar] [CrossRef]
- Mcdiarmid, D.L. Mean stress effects in biaxial fatigue where the stresses are out-of-phase and at different frequencies. In Proceedings of the 3rd International Conference on Biaxial/Multiaxial Fatigue, Stuttgart, FRG, Germany, 3–6 April 1989; Brown, M.W., Ed.; Mechanical Engineering Publication, Trans Tech Publications Ltd.: London, UK, 1989; Volume 53, pp. 1–18. [Google Scholar]
- McDiarmid, D.L. The effect of mean stress on biaxial fatigue where the stresses are out-of-phase and at different frequencies. In Proceedings of the 3rd International Conference on Biaxial/Multiaxial Fatigue, Stuttgart, FRG, Germany, 3–6 April 1989; Brown, M.W., Ed.; Mechanical Engineering Publication, Trans Tech Publications Ltd.: London, UK, 1989; Volume 53, pp. 605–619. [Google Scholar]
- Bernasconi, A.; Foletti, S.; Papadopoulos, I. A study on combined torsion and axial load fatigue limit tests with stresses of different frequencies. Int. J. Fatigue 2008, 30, 1430–1440. [Google Scholar] [CrossRef]
- Reis, L.; Li, B.; De Freitas, M. Multiaxial loadings with different frequencies between axial and torsional components in 42CrMo4 steel. Int. J. Struct. Integr. 2010, 1, 303–313. [Google Scholar] [CrossRef]
- Pejkowski, Ł.; Skibicki, D. Stress-strain response and fatigue life of four metallic materials under asynchronous loadings: Experimental observations. Int. J. Fatigue 2019, 128, 105202. [Google Scholar] [CrossRef]
- Pejkowski, Ł.; Seyda, J. Fatigue of four metallic materials under asynchronous loadings: Small cracks observation and fatigue life prediction. Int. J. Fatigue 2021, 142, 105904. [Google Scholar] [CrossRef]
- Varvani-Farahani, A. Critical plane-energy based approach for assessment of biaxial fatigue damage where the stress-time axes are at different frequencies. Eur. Struct. Integr. Soc. 2003, 31, 203–221. [Google Scholar]
- Endo, T.; Mitsunaga, K.; Takahashi, K.; Kobayashi, K.; Matsuishi, M. Damage evaluation of metals for random or varying loading-three aspects of rain flow method. Mech. Behav. Mater. 1974, 371–380. [Google Scholar]
- Lee, B. Fatigue analysis under variable amplitude loading using an energy parameter. Int. J. Fatigue 2003, 25, 621–631. [Google Scholar] [CrossRef]
- Bannantine, J.; Socie, D. A variable amplitude multiaxial fatigue life prediction method. In Proceedings of the 3rd International Conference on Biaxial/Multiaxial Fatigue, Stuttgart, FRG, Germany, 3–6 April 1989; Brown, M.W., Ed.; Mechanical Engineering Publication, Trans Tech Publications Ltd.: London, UK, 1989; Volume 53, pp. 35–51. [Google Scholar]
- Bannantine, J.; Socie, D. A Multiaxial Fatigue Life Estimation Technique; ASTM Special Technical Publication: West Conshohocken, PA, USA, 1992; pp. 245–275. [Google Scholar]
- Wang, C.H.; Brown, M.W. On plastic deformation and fatigue under multiaxial loading. Nucl. Eng. Des. 1996, 162, 75–84. [Google Scholar] [CrossRef]
- Wang, C.H.; Brown, M.W. Life prediction techniques for variable amplitude multiaxial fatigue—Part 2: Comparison with. J. Eng. Mater. Technol. 1996, 118, 371. [Google Scholar] [CrossRef]
- Wang, C.H.; Brown, M.W. Life prediction techniques for variable amplitude multiaxial fatigue—Part 1: Theories. J. Eng. Mater. Technol. 1996, 118, 367. [Google Scholar] [CrossRef]
- Meggiolaro, M.A.; De Castro, J.T.P. An improved multiaxial rainflow algorithm for non-proportional stress or strain histories—Part II: The Modified Wang–Brown method. Int. J. Fatigue 2012, 42, 194–206. [Google Scholar] [CrossRef]
- Meggiolaro, M.A.; De Castro, J.T.P. An improved multiaxial rainflow algorithm for non-proportional stress or strain histories—Part I: Enclosing surface methods. Int. J. Fatigue 2012, 42, 217–226. [Google Scholar] [CrossRef]
- Wei, Z.; Dong, P. A rapid path-length searching procedure for multi-axial fatigue cycle counting. Fatigue Fract. Eng. Mater. Struct. 2012, 35, 556–571. [Google Scholar] [CrossRef]
- Dong, P.; Wei, Z.; Hong, J.K. A path-dependent cycle counting method for variable-amplitude multi-axial loading. Int. J. Fatigue 2010, 32, 720–734. [Google Scholar] [CrossRef]
- Wei, Z.; Dong, P. Multiaxial fatigue life assessment of welded structures. Eng. Fract. Mech. 2010, 77, 3011–3021. [Google Scholar] [CrossRef]
- Shang, D.; Wang, D.; Xu, H.; Ping, A. A unified multiaxial fatigue damage parameter. Acta Mech. Solida Sin. 1998, 11, 261–274. [Google Scholar]
- Anes, V.; Reis, L.; Li, B.; De Freitas, M. New cycle counting method for multiaxial fatigue. Int. J. Fatigue 2014, 67, 78–94. [Google Scholar] [CrossRef]
- Anes, V.; Reis, L.; Freitas, M. Asynchronous multiaxial fatigue damage evaluation. Procedia Eng. 2015, 101, 421–429. [Google Scholar] [CrossRef]
- Anes, V.; Reis, L.; Li, B.; Fonte, M.; De Freitas, M. New approach for analysis of complex multiaxial loading paths. Int. J. Fatigue 2014, 62, 21–33. [Google Scholar] [CrossRef]
- Janssens, K.G.F. Universal cycle counting for non-proportional and random fatigue loading. Int. J. Fatigue 2020, 133, 105409. [Google Scholar] [CrossRef]
- Arora, P.; Gupta, S.K.; Samal, M.K.; Chattopadhyay, J. Multiaxial fatigue tests under variable strain paths and asynchronous loading and assessment of fatigue life using critical plane models. Int. J. Fatigue 2021, 145, 106049. [Google Scholar] [CrossRef]
- Smith, K.N.; Watson, P.; Topper, T.H. Stress-strain function for the fatigue of metals. J. Mater. 1970, 5, 767–778. [Google Scholar]
- Murakami, Y. Metal Fatigue: Effects of Small Defects and Nonmetallic Inclusion; Academic Press & Elsevier: London, UK, 2019. [Google Scholar]
- Fatemi, A.; Socie, D.F. A critical plane approach to multiaxial fatigue damage including out-of-phase loading. Fatigue Fract. Eng. Mater. Struct. 1988, 11, 149–165. [Google Scholar] [CrossRef]
- Wang, C.H.; Brown, M.W. A path-independent parameter for fatigue under proportional and non-proportional loading. Fatigue Fract. Eng. Mater. Struct. 1993, 16, 1285–1297. [Google Scholar] [CrossRef]
- Qi, X.; Liu, T.; Shi, X.; Wang, J.; Zhang, J.; Fei, B. A sectional critical plane model for multiaxial high-cycle fatigue life prediction. Fatigue Fract. Eng. Mater. Struct. 2021, 44, 689–704. [Google Scholar] [CrossRef]



| C | Mn | Si | P | Ni | Cr | W | Mo | V | Cu | Ti |
|---|---|---|---|---|---|---|---|---|---|---|
| 0.31 | 0.85 | 0.99 | 0.01 | 0.05 | 0.87 | 0.01 | 0.02 | 0.01 | 0.18 | 0.003 |
| E (GPa) | σy (MPa) | σu (MPa) | G (GPa) | τy (MPa) | τu (MPa) |
|---|---|---|---|---|---|
| 207 | 1196 | 1334 | 77.2 | 825 | 1040 |
| Load Path | σx,a (MPa) | τxy,a (MPa) | ξ1 | ξ2 | Spec. ID | Nf (blocks) | N50 (blocks) |
|---|---|---|---|---|---|---|---|
| — | 350 | 350 | 1 | 1 | G-10 | 185,261 | 141,984 |
| G-11 | 175,013 | ||||||
| G-12 | 119,687 | ||||||
| G-100 | 136,694 | ||||||
| G-104 | 108,778 | ||||||
| AS-1 | 350 | 350 | 2 | 1 | DF-4 | 52,632 | 47,560 |
| DF-6 | 42,976 | ||||||
| AS-2 | 350 | 350 | 4 | 1 | DF-9 | 35,702 | 35,710 |
| DF-10 | 35,717 | ||||||
| AS-3 | 350 | 350 | 1 | 2 | DF-2 | 49,892 | 50,195 |
| DF-5 | 50,500 | ||||||
| AS-4 | 350 | 350 | 1 | 4 | DF-7 | 70,330 | 50,033 |
| DF-8 | 35,593 |
| Load Path | ξ1 | ξ2 | MSSA | σn/MPa | τna,max (MPa) | MN | σn,max (MPa) | τn,a (MPa) |
|---|---|---|---|---|---|---|---|---|
| AS-1 | 2 | 1 | 0°/90° | 350/0 | 350.00 | ±30.0° | 494.98 | 285.77 |
| AS-2 | 4 | 1 | ±13.3°/±76.7° | 476.59/167.43 | 372.16 | ±31.0° | 544.26 | 307.08 |
| AS-3 | 1 | 2 | ±10.0°/±80.0° | 299.42/127.23 | 371.88 | ±34.0° | 504.46 | 256.13 |
| AS-4 | 1 | 4 | ±12.4°/±77.6° | 385.62/161.73 | 385.62 | ±32.5° | 548.07 | 295.18 |
| BS-SWT | BS-FS | BS-SCPM | WB-S = 1.5 | WB-S = 2.0 | WB-SCPM | |
|---|---|---|---|---|---|---|
| Ei ≤ 2 | 25.0 | 75.0 | 100.0 | 50.0 | 37.5 | 50.0 |
| Ei ≤ 3 | 37.5 | 75.0 | 100.0 | 75.0 | 75.0 | 75.0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, T.; Qi, X.; Shi, X.; Gao, L.; Zhang, T.; Zhang, J. Effect of Loading Frequency Ratio on Multiaxial Asynchronous Fatigue Failure of 30CrMnSiA Steel. Materials 2021, 14, 3968. https://doi.org/10.3390/ma14143968
Liu T, Qi X, Shi X, Gao L, Zhang T, Zhang J. Effect of Loading Frequency Ratio on Multiaxial Asynchronous Fatigue Failure of 30CrMnSiA Steel. Materials. 2021; 14(14):3968. https://doi.org/10.3390/ma14143968
Chicago/Turabian StyleLiu, Tianqi, Xinxin Qi, Xinhong Shi, Limin Gao, Tian Zhang, and Jianyu Zhang. 2021. "Effect of Loading Frequency Ratio on Multiaxial Asynchronous Fatigue Failure of 30CrMnSiA Steel" Materials 14, no. 14: 3968. https://doi.org/10.3390/ma14143968
APA StyleLiu, T., Qi, X., Shi, X., Gao, L., Zhang, T., & Zhang, J. (2021). Effect of Loading Frequency Ratio on Multiaxial Asynchronous Fatigue Failure of 30CrMnSiA Steel. Materials, 14(14), 3968. https://doi.org/10.3390/ma14143968







