Numerical Simulation of Vehicle–Lighting Pole Crash Tests: Parametric Study of Factors Influencing Predicted Occupant Safety Levels
Abstract
1. Introduction
2. Occupant Safety Levels Description
3. Problem Description
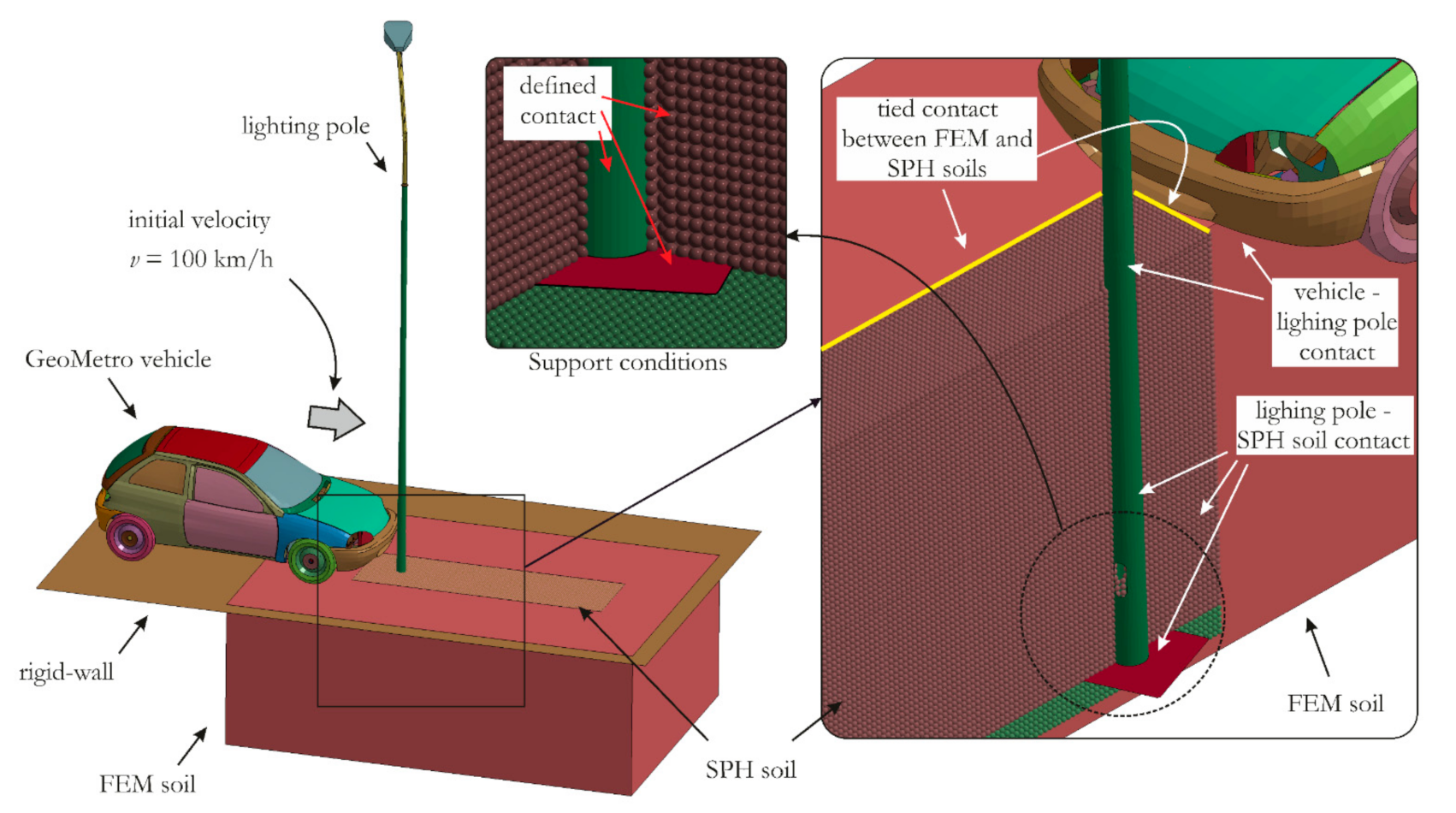
3.1. Model Definition
- FEA was conducted with implementation of massive parallel processing (MPP) LS-Dyna R10.1.0 explicit code.
- The soil was modeled using constant stress solid FEs with one integration point and with stiffness-based hourglass control. The average FE size was 30.0 mm. The elements were coupled with smoothed particle hydrodynamics (SPH) particles [30,31,32,33,34], which were used to represent the area within direct interaction with the lighting pole. The soil area in the present study was larger than the requirements presented in [1], with dimensions of 3.6 m, 5.3 m and 1.9 m for width, length and height, respectively. This choice was made due to the possible large deformation of the soil during lighting pole deflection depending on the constitutive model used. To couple the SPH particles within the area of direct interaction with the lighting pole, a kinematic constraint method in which the particles are tied to the Lagrangian surface was applied in order to maintain the continuity of the soil. The outer surfaces of the soil area were fixed.
- The SPH soil was modelled using the renormalization approximation, which is recommended for most applications [35]. A sensitivity study of particle density is not presented, since a very small influence on the results was observed. Ultimately, a regular grid of particles was used with a space between particles equal to 20.0 mm. Moreover, the recommended artificial bulk viscosity coefficients Q1 = 1.0 and Q2 = 1.0 were used for the SPH soil [35].
- The representative traffic pole with a height of 8.0 m and a diameter of 142.0 mm and 56.0 mm in the bottom and in the upper part of the pole, respectively, was adopted. The pole was mainly made of steel and was mounted into the ground at a depth of 1.6 m. To represent the ground–lighting pole interaction, a contact between the pole column and bottom plate was used. For the sake of a better presentation, the SPH soil was divided into two parts located above and below the bottom plate (Figure 1). The pole was discretized using fully integrated Lagrangian Belytschko-Tsay (BT) shell elements.
- For the vehicle, the widely used and deeply validated Suzuki Geo Metro FE model was adopted [20,25,26,27] as modified by the Department of Mechanics of Materials and Structure, Gdańsk University of Technology, Poland [26]. The model consists of 14,709 shell elements and 820 solids. Additionally, spring and discrete dampers are used to model shock absorbers. The majority of the parts in the model are modelled using piecewise linear plasticity material model with erosion criteria.
- The interactions between all parts in the model were simulated using a penalty function approach adopting Coulomb’s friction model [36,37,38]. In addition to friction properties between vehicle and lighting pole, the Coulomb’s friction coefficients of μ = 0.1 and μ = 0.4 for steel–steel and tire–ground pairs were used in the model, respectively.
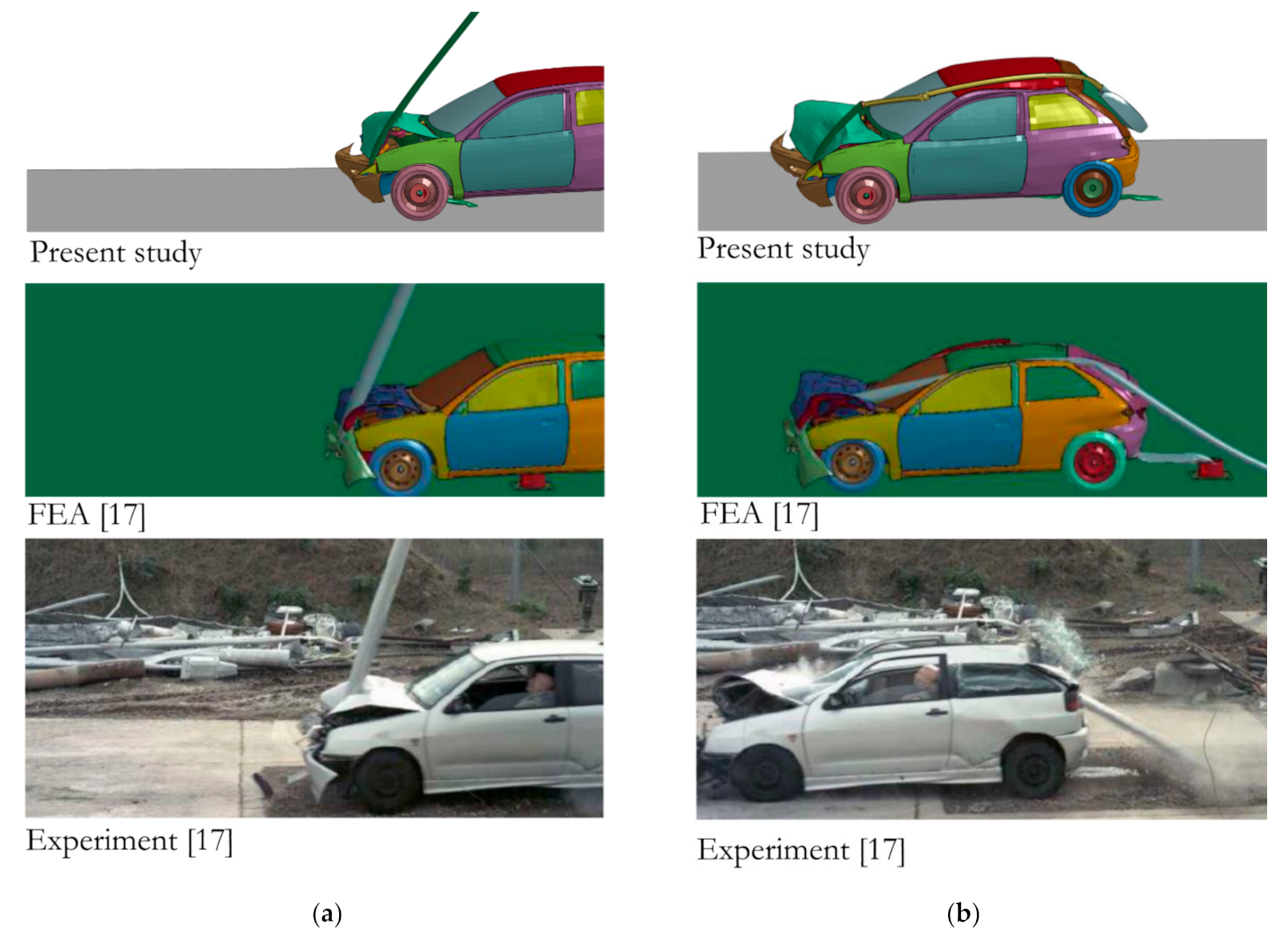
- To validate the model, the numerical simulations were compared with observations presented in [17], where similar testing conditions were presented.
3.2. Constitutive Modeling
4. Methodology of Numerical Simulations
4.1. Model Validation
4.2. Description of Crash Test Scenarios
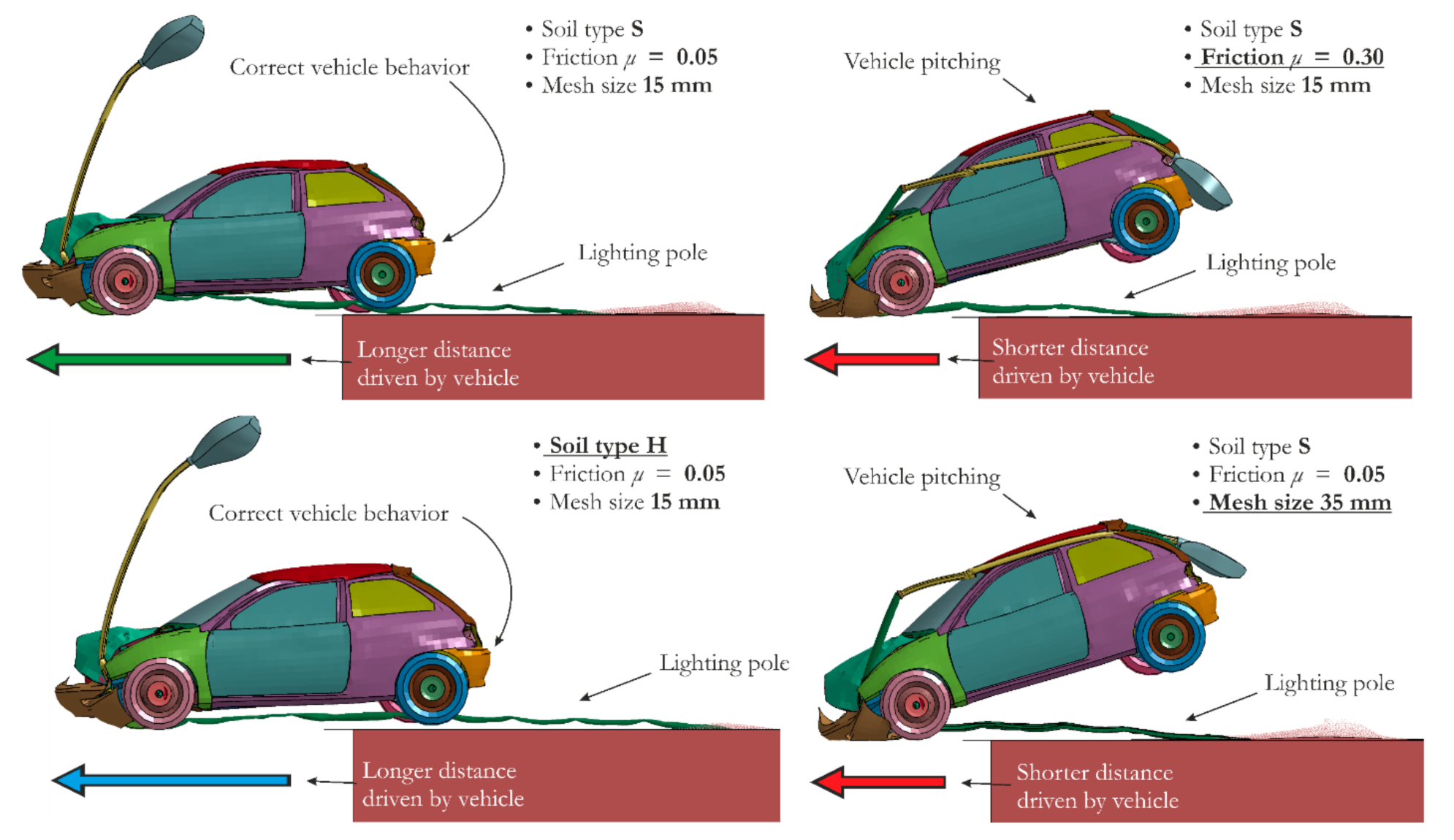
4.2.1. Mesh Size
4.2.2. Soil Properties
4.2.3. Friction Properties
5. Results and Discussion
5.1. Model Validation
5.2. Parametric Study
- history of velocity measured for the center of mass of the vehicle;
- history of ASI;
- vehicle behavior during crash impact;
- overall deformation of the lighting pole and soil;
- maximum values of ASI and THIV and minimum values of velocity.
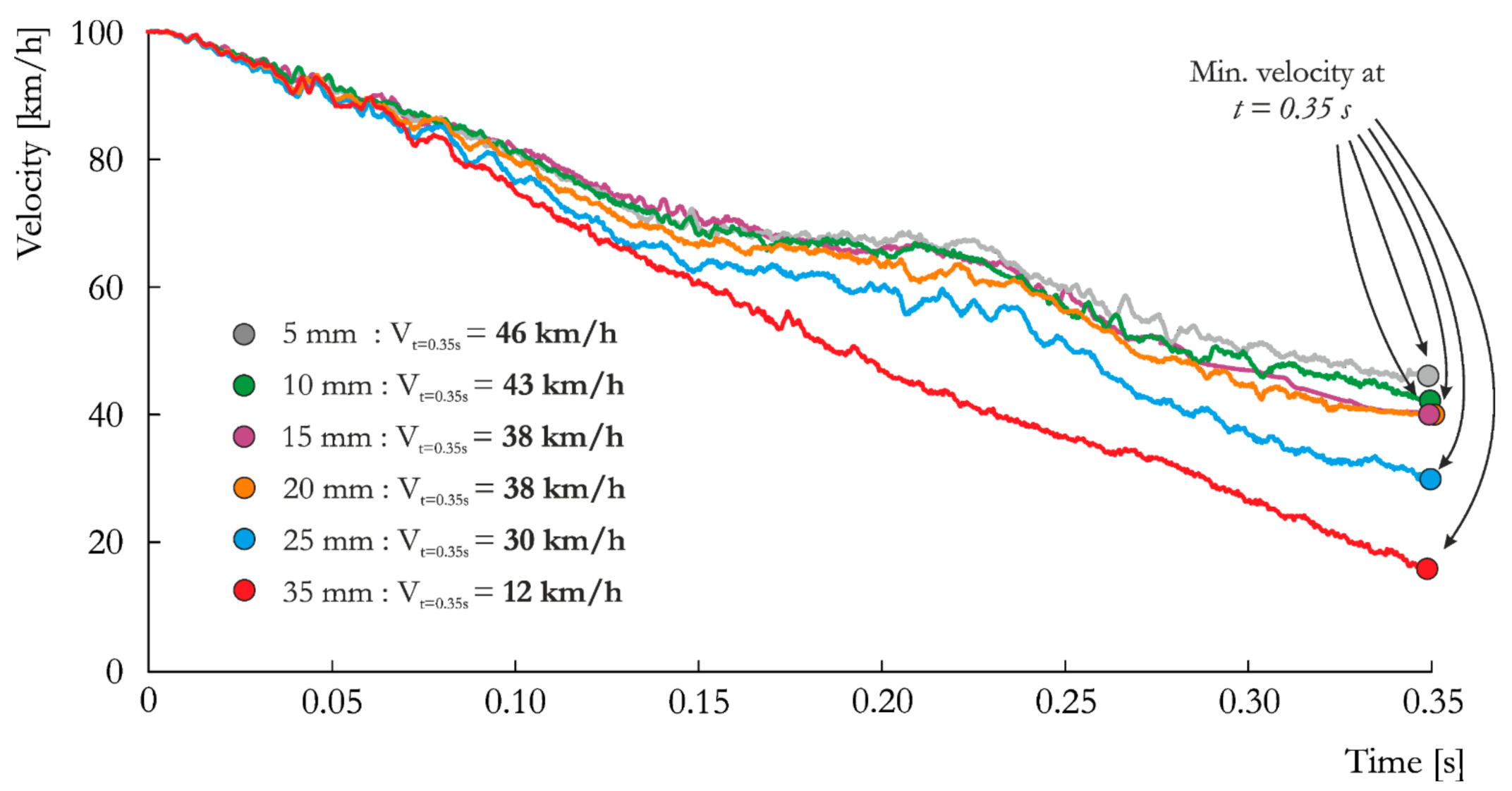
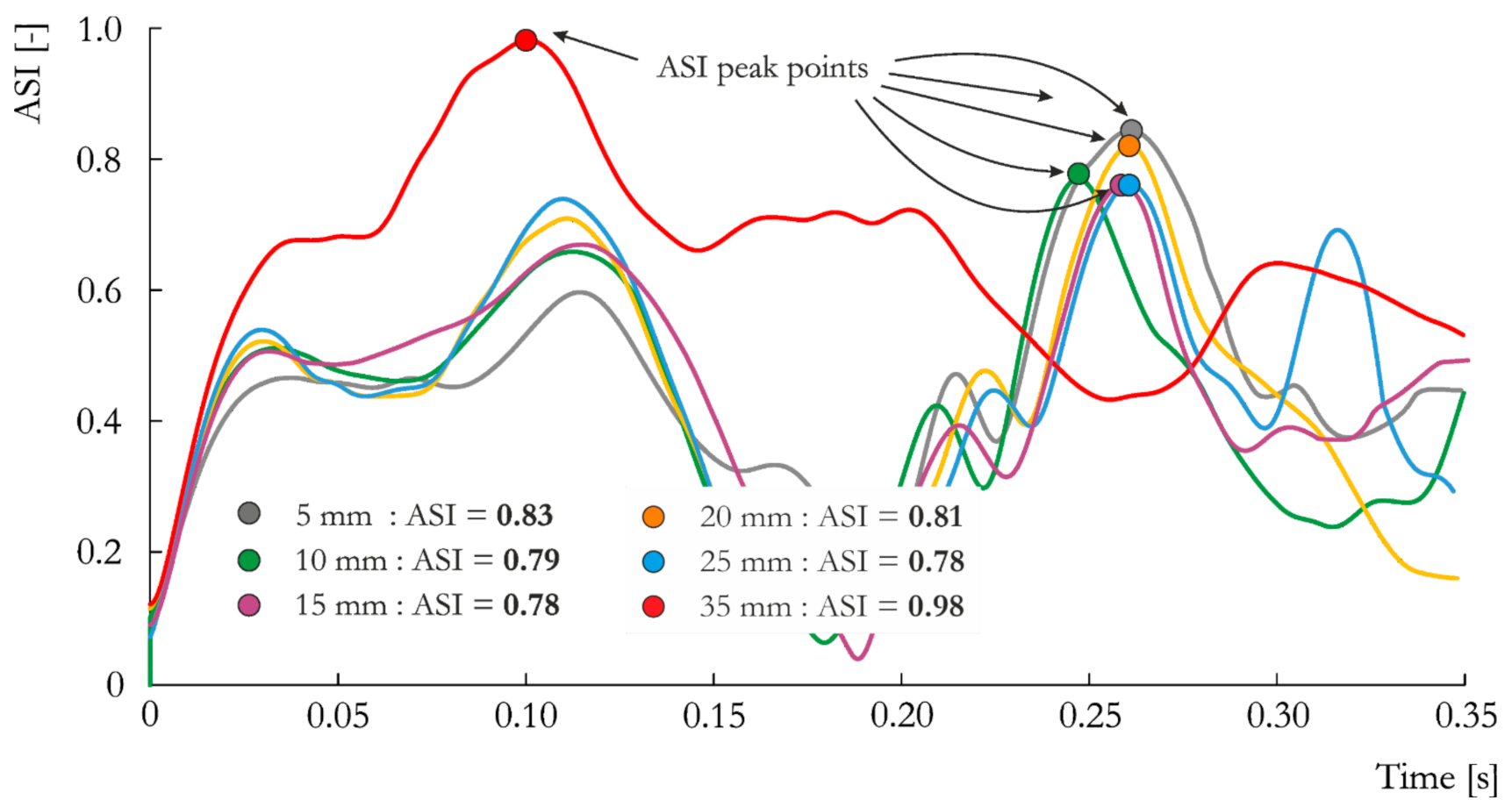
5.2.1. Influence of Mesh Size
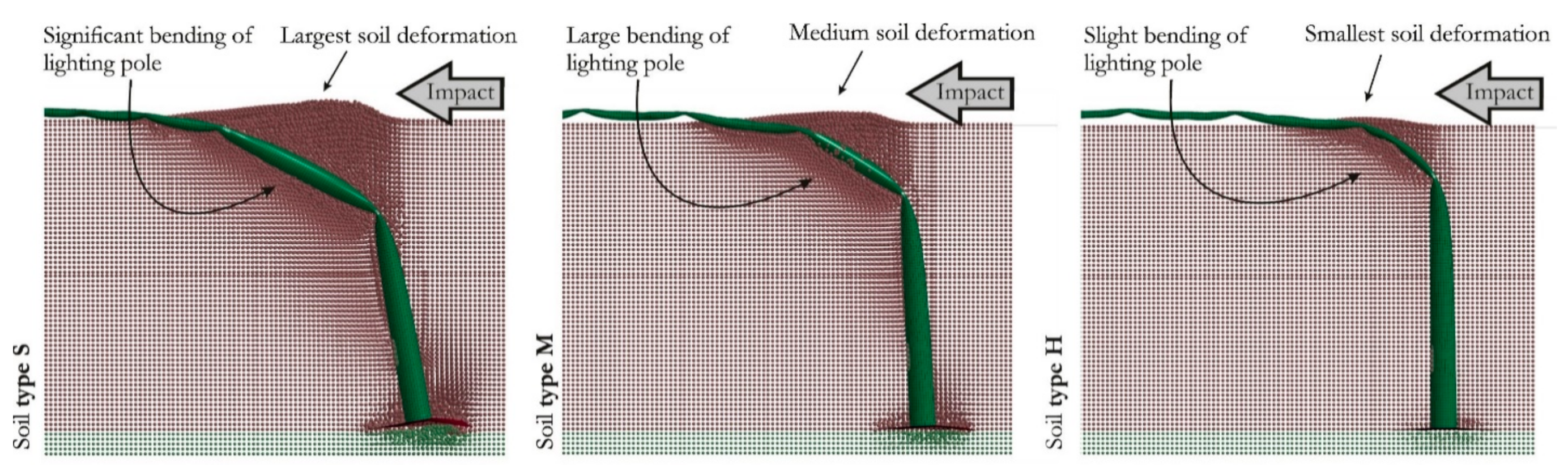
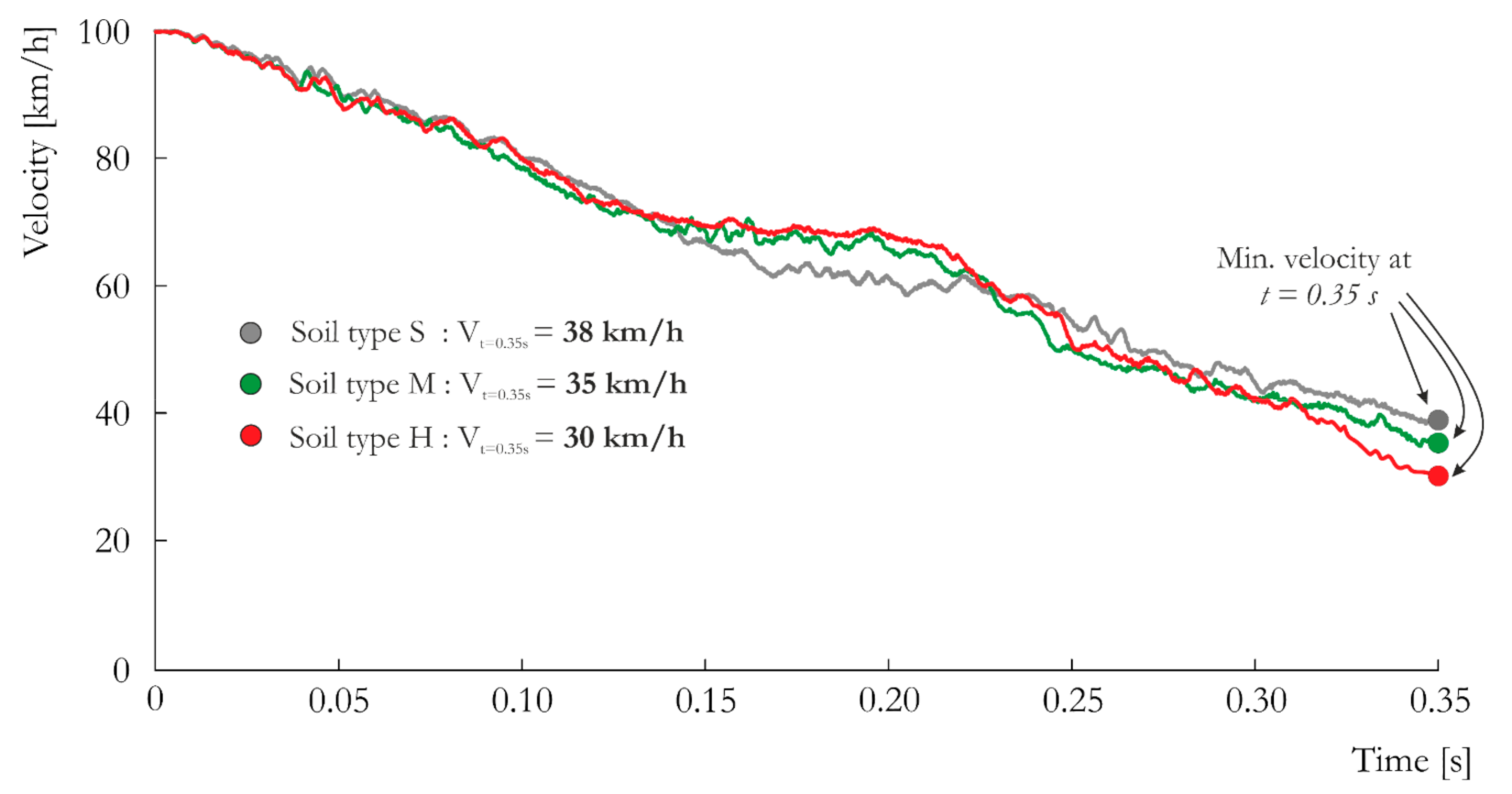
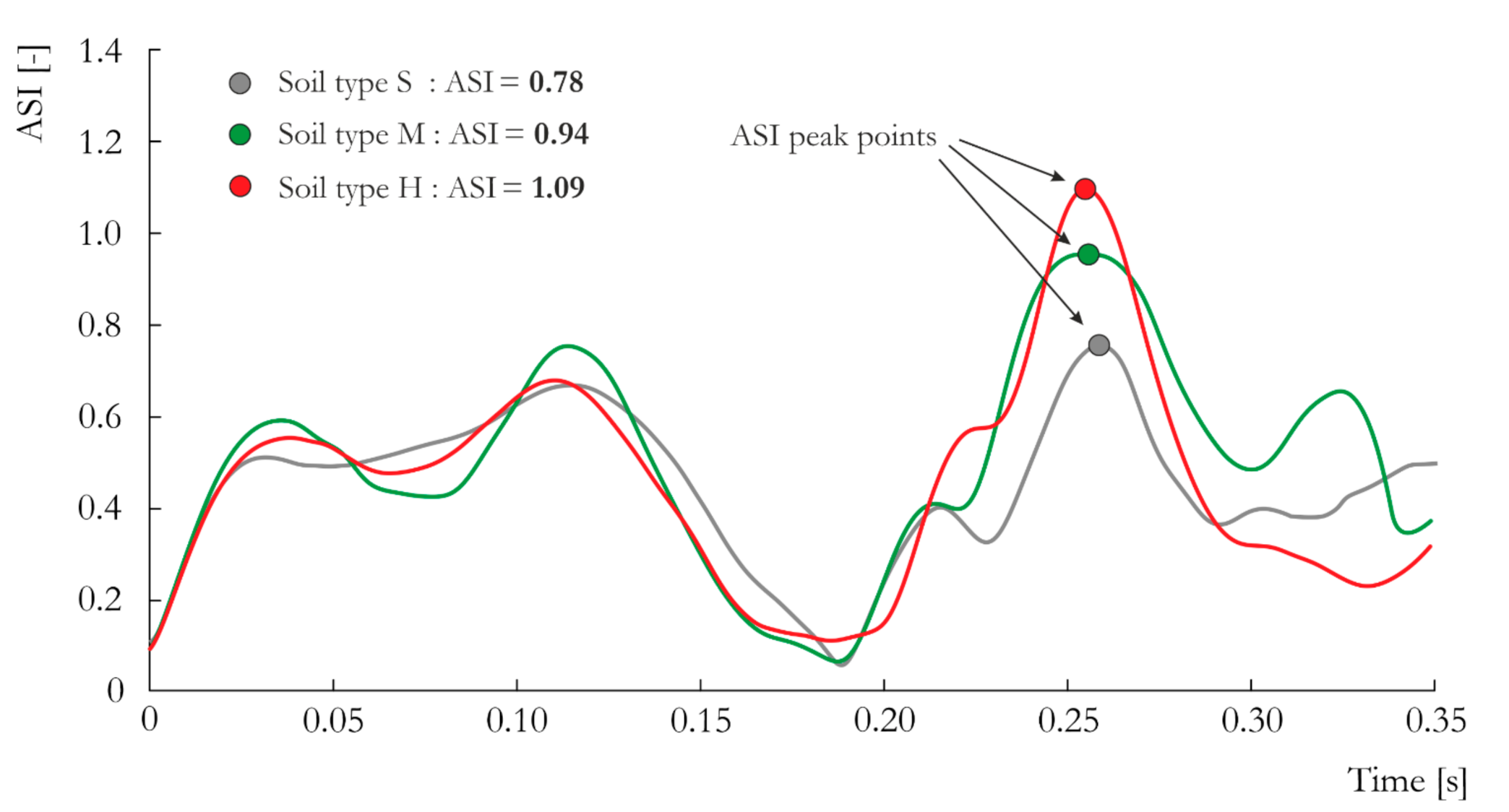
5.2.2. Influence of Soil Type
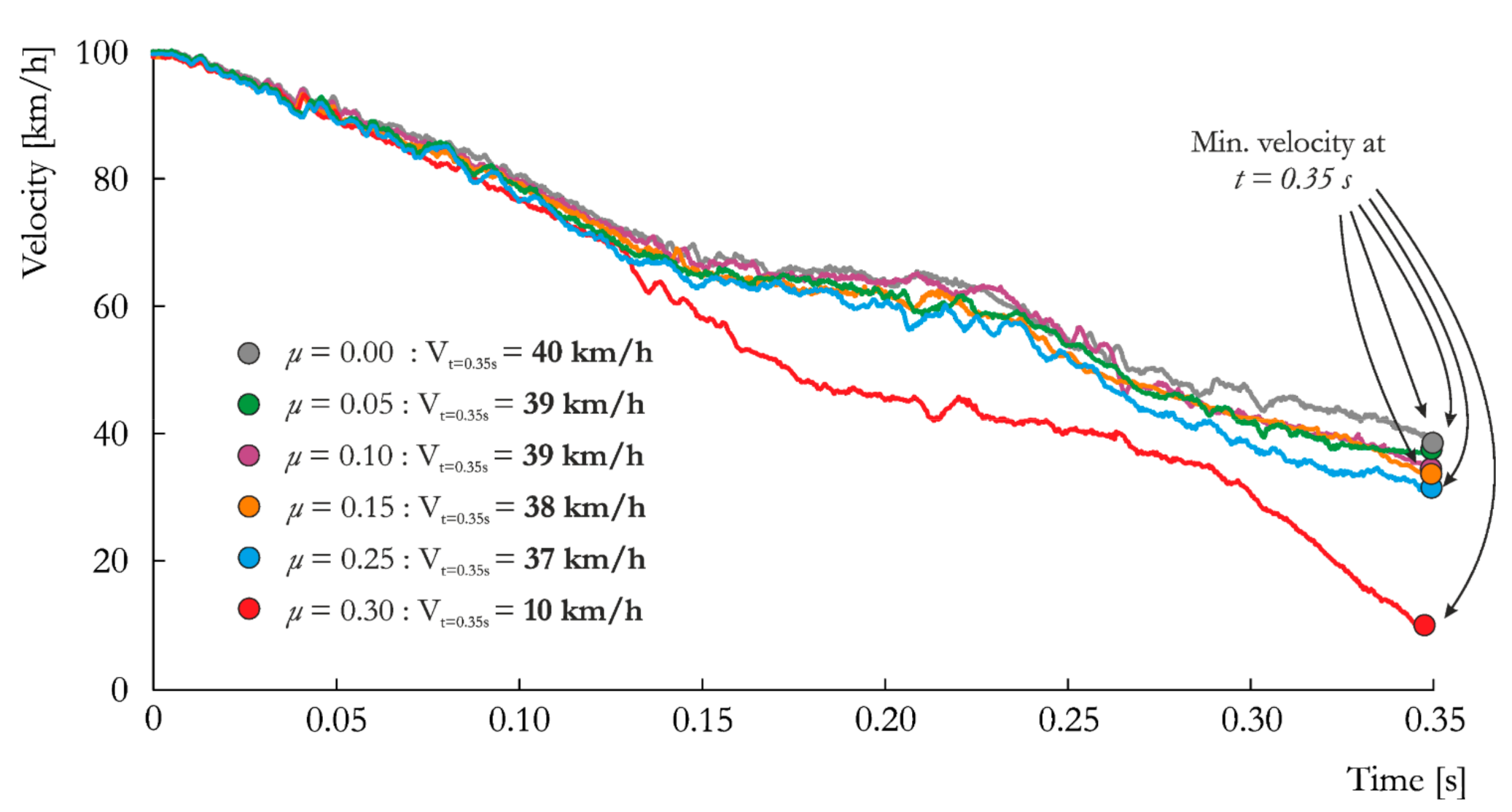
5.2.3. Influence of Friction
5.3. Comparisons between Crash Test Scenarios
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- European Committee for Standarization. Passive Safety of Support Structures for Road Equipment—Requirements and Test Methods 2005-06-02; E. 12767; European Committee for Standarization: Brussels, Belgium, 2019. [Google Scholar]
- Chell, J.; Brandani, C.E.; Fraschetti, S.; Chakraverty, J.; Camomilla, V. Limitations of the European barrier crash testing regulation relating to occupant safety. Accid. Anal. Prev. 2019, 133, 105239. [Google Scholar] [CrossRef]
- Sturt, R.; Fell, C. The relationship of injury risk to accident severity in impacts with roadside barriers. Int. J. Crashworthiness 2009, 14, 165–172. [Google Scholar] [CrossRef]
- Shojaati, M. Correlation between injury risk and impact severity index ASI. In Proceedings of the Swiss Transport Research Conference, Monte Verità, Ascona, Switzerland, 19–21 March 2003; pp. 1–10. [Google Scholar]
- Jamroziak, K.; Joszko, K.; Wolanski, W.; Gzik, M.; Burkacki, M.; Suchon, S.; Szarek, A.; Zielonka, K. Experimental and modelling research on coach passengers’ safety in frontal impacts. Arch. Civ. Mech. Eng. 2020, 4, 1–13. [Google Scholar] [CrossRef]
- Ptak, M. Method to Assess and Enhance Vulnerable Road User Safety during Impact Loading. Appl. Sci. 2019, 9, 1000. [Google Scholar] [CrossRef]
- Baranowski, P.; Damaziak, K.; Mazurkiewicz, L.; Malachowski, J.; Muszynski, A.; Vangi, D. Analysis of mechanics of side impact test defined in UN/ECE Regulation 129. Traffic Inj. Prev. 2018, 19, 256–263. [Google Scholar] [CrossRef] [PubMed]
- Ptak, M.; Kaczyński, P.; Fernandes, F.; de Sousa, R.A. Computer Simulations for Head Injuries Verification after Impact. In Proceedings of the 2nd Annual International Conference on Material, Machines and Methods for Sustainable Development (MMMS2020), Nha Trang, Vietnam, 12–15 November 2020; pp. 431–440. [Google Scholar]
- Burkacki, M.; Wolański, W.; Suchoń, S.; Joszko, K.; Gzik-Zroska, B.; Sybilski, K.; Gzik, M. Finite element head model for the crew injury assessment in a light armoured vehicle. Acta Bioeng. Biomech. 2020, 22, 174–183. [Google Scholar] [CrossRef]
- Sybilski, K.; Małachowski, J. Sensitivity study on seat belt system key factors in terms of disabled driver behavior during frontal crash. Acta Bioeng. Biomech. 2020, 21. [Google Scholar] [CrossRef]
- Abdel-Nasser, Y.A. Frontal crash simulation of vehicles against lighting columns using FEM. Alex. Eng. J. 2013, 52, 295–299. [Google Scholar] [CrossRef]
- Senthil, K.; Rupali, S. Crashworthiness of Highway Lamp Post against Vehicle Impact. J. Mater. Eng. Struct. 2019, 5, 371–385. [Google Scholar]
- Pachocki, Ł.; Bruski, D.; Burzyński, S.; Chróścielewski, J.; Wilde, K.; Witkowski, W. On the influence of the acceleration recording time on the calculation of impact severity indexes. MATEC Web Conf. 2018, 219, 1–7. [Google Scholar] [CrossRef]
- Elmarakbi, A.; Sennah, K.; Samaan, M.; Siriya, P. Crashworthiness of Motor Vehicle and Traffic Light Pole in Frontal Collisions. J. Transp. Eng. 2006, 132, 722–733. [Google Scholar] [CrossRef]
- Pawlak, M. The Acceleration Severity Index in the impact of a vehicle against permanent road equipment support structures. Mech. Res. Commun. 2016, 77, 21–28. [Google Scholar] [CrossRef]
- Klyavin, O.; Borovkov, A.; Michailov, A. Finite Element Modeling of the Crash-Tests for Energy Absorbing Lighting Columns. In Proceedings of the 6th EUROMECH Nonlinear Dynamics Conference ENOC-2008, Saint Petersburg, Russia, 30 June–4 July 2008; pp. 4–7. [Google Scholar]
- Jedliński, T.; Buśkiewicz, J.; Fritzkowski, P. Numerical and experimental analyses of a lighting pole in terms of passive safety of 100HE3 class. Vib. Phys. Syst. 2020, 31, 1–8. [Google Scholar] [CrossRef]
- Bruski, D.; Burzyński, S.; Chróścielewski, J.; Jamroz, K.; Pachocki, Ł.; Witkowski, W.; Wilde, K. Experimental and numerical analysis of the modified TB32 crash tests of the cable barrier system. Eng. Fail. Anal. 2019, 104, 227–246. [Google Scholar] [CrossRef]
- Budzynski, M.; Wilde, K.; Jamroz, K.; Chroscielewski, J.; Witkowski, W.; Burzynski, S.; Bruski, D.; Jelinski, L.; Pachocki, Ł. The effects of vehicle restraint systems on road safety. MATEC Web Conf. 2019, 262, 05003. [Google Scholar] [CrossRef]
- Bruski, D.; Burzyński, S.; Chróścielewski, J.; Pachocki, Ł.; Wilde, K.; Witkowski, W. The influence of position of the post or its absence on the performance of the cable barrier system. MATEC Web Conf. 2018, 219, 02012. [Google Scholar] [CrossRef]
- Ray, M.H. The use of finite element analysis in roadside hardware design. Int. J. Crashworthiness 1997, 2, 333–348. [Google Scholar] [CrossRef]
- Lee, A.-S. Validated Frontal LLNL Modified Honda Civic Ingrid Model for Three Rigid Pole and U-Channel Sign Post Impact Simulations; Task Report on Task D.2 of the FHWA-NHTSA-LLNL Cooperative Agreement; Lawrence Livermore National Laboratory: Livermore, CA, USA, 1994. [Google Scholar]
- Tabiei, A.; Wu, J. Roadmap for crashworthiness finite element simulation of roadside safety structures. Finite Elem. Anal. Des. 2000, 34, 145–157. [Google Scholar] [CrossRef]
- National Academies of Sciences, Engineering, and Medicine. Procedures for Verification and Validation of Computer Simulations Used for Roadside Safety Applications; The National Academies Press: Washington, WA, USA, 2011; ISBN 9780309431019. [Google Scholar]
- Niezgoda, T.; Barnat, W.; Dziewulski, P.; Kiczko, A. Numerical Modelling and Simulation of Road Crash Tests with the use of Advanced CAD/CAE Systems/Modelowanie Numeryczne I Symulacja Drogowych Testów Zderzeniowych Z Wykorzystaniem Zaawansowanych Systemów CAD/CAE. J. Konbin. 2012, 23, 95–108. [Google Scholar] [CrossRef]
- Bruski, D.; Burzyński, S.; Chróścielewski, J.; Pachocki, Ł.; Witkowski, W. On the validation of the LS-DYNA Geo Metro numerical model. MATEC Web Conf. 2019, 262, 10001. [Google Scholar] [CrossRef]
- Klasztorny, M.; Nycz, D.B.; Dziewulski, P.; Gieleta, R.; Stankiewicz, M.; Zielonka, K. Numerical modelling of post-ground subsystem in road safety barrier crash tests. Eng. Trans. 2019, 67, 513–534. [Google Scholar] [CrossRef]
- Krzeszowiec, M.; Małachowski, J. Badanie wpływu sformułowania elementu skończonego oraz schematu rozwiązywania równania ruchu na wyniki analizy MES na przykładzie niesymetrycznie obciążonej płyty. Bull. Mil. Univ. Technol. 2015, 64, 135–157. [Google Scholar] [CrossRef]
- European Committee for Standarization. European Committee for Standardization (CEN), European Standard EN 1317-1, Road Restraint Systems. Terminology and General Criteria for Test Methods; CEN: Brusseles, Belgium, 2019. [Google Scholar]
- Lucy, L.B. A numerical approach to the testing of the fission hypothesis. Astron. J. 1977, 82, 1013–1024. [Google Scholar] [CrossRef]
- Monaghan, J.; Lattanzio, J. A refined particle method for astrophysical problems. Astron. Astrophys. 1985, 149, 135–143. [Google Scholar]
- Liu, G.R.; Gu, Y.T. An Introduction to Meshfree Methods and Their Programming; Springer: Berlin, Germany, 2005; ISBN 1402032285. [Google Scholar]
- Liu, M.B.; Liu, G.R. Smoothed Particle Hydrodynamics (SPH): An Overview and Recent Developments. Arch. Comput. Methods Eng. 2010, 17, 25–76. [Google Scholar] [CrossRef]
- Baranowski, P.; Damaziak, K.; Malachowski, J.; Sergienko, V.P.; Bukharov, S.N. Modeling of abrasive wear by the meshless smoothed particle hydrodynamics method. J. Frict. Wear 2016, 37, 94–99. [Google Scholar] [CrossRef]
- Hallquist, J. LS-DYNA Theory Manual; Livermore Software Technology Corporation (LSTC): Livermore, LA, USA, 2019; Volume 19, p. 2507. ISBN 9254492507. [Google Scholar]
- Vulovic, S.; Zivkovic, M.; Grujovic, N.; Slavkovic, R.A. Comparative study of contact problems solution based on the penalty and Lagrange multiplier approaches. J. Serb. Soc. Comput. Mech. 2007, 1, 174–183. [Google Scholar]
- Wriggers, P.; Van, T.V.; Stein, E. Finite element formulation of large deformation impact-contact problems with friction. Comput. Struct. 1990, 37, 319–331. [Google Scholar] [CrossRef]
- Baranowski, P.; Małachowski, J.; Niezgoda, T.; Mazurkewicz, L. Dynamic behaviour of Various Fibre Systems During Impact Interaction—Numerical Approach. Fibres Text. East. Eur. 2015, 6, 72–81. [Google Scholar] [CrossRef]
- Kurzawa, A.; Pyka, D.; Jamroziak, K.; Bocian, M.; Kotowski, P.; Widomski, P. Analysis of ballistic resistance of composites based on EN AC-44200 aluminum alloy reinforced with Al2O3 particles. Compos. Struct. 2018, 201, 834–844. [Google Scholar] [CrossRef]
- Poplawski, A.; Kędzierski, P.; Morka, A. Identification of Armox 500T steel failure properties in the modeling of perforation problems. Mater. Des. 2020, 190, 108536. [Google Scholar] [CrossRef]
- Kędzierski, P.; Morka, A.; Stanisławek, S.; Surma, Z. Numerical modeling of the large strain problem in the case of mushrooming projectiles. Int. J. Impact Eng. 2020, 135, 103403. [Google Scholar] [CrossRef]
- Kucewicz, M.; Baranowski, P.; Małachowskii, J.; Trzciński, W.; Szymańczyk, L. Numerical Modelling of Cylindrical Test for Determining Jones—Wilkins—Lee Equation Parameters. In Proceedings of the 14th International Scientific Conference: Computer Aided Engineering, Polanica Zdrój, Poland, 20–23 June 2018; Rusiński, E., Pietrusiak, D., Eds.; Springer International Publishing: Cham, Switzerland, 2019; pp. 388–394. [Google Scholar]
- Baranowski, P.; Gieleta, R.; Malachowski, J.; Damaziak, K.; Mazurkiewicz, L. Split Hopkinson Pressure Bar impulse experimental measurement with numerical validation. Metrol. Meas. Syst. 2014, 21, 47–58. [Google Scholar] [CrossRef]
- Zhong, W.Z.; Mbarek, I.A.; Rusinek, A.; Bernier, R.; Jankowiak, T.; Sutter, G. Development of an experimental set-up for dynamic force measurements during impact and perforation, coupling to numerical simulations. Int. J. Impact Eng. 2016, 91, 102–115. [Google Scholar] [CrossRef]
- Baranowski, P.; Malachowski, J. Numerical study of selected military vehicle chassis subjected to blast loading in terms of tire strength improving. Bull. Pol. Acad. Sci. Tech. Sci. 2015, 63, 867–878. [Google Scholar] [CrossRef]
- Danek, W.; Gąsiorek, D. Numerical simulation of the car crash with aluminum, root mounted lighting column. Model. Inżynierskie 2018, 35, 25–30. [Google Scholar]
- Borovkov, A.; Klyavin, O.; Michailov, A. Finite Element Modeling and Analysis of Crash Safe Composite Lighting Columns, Contact-Impact Problem. In Proceedings of the 9th International LS-DYNA Users Conference, Detroit, MI, USA, 4–6 June 2006; pp. 11–20. [Google Scholar]
- Elmarakbi, A.; Sennah, K.; Siriya, P.; Emam, A. Parametric effects on the performance of traffic light poles in vehicle crashes. Int. J. Crashworthiness 2006, 11, 217–230. [Google Scholar] [CrossRef]
- Klasztorny, M.; Nycz, D.B.; Szurgott, P. Modelling and simulation of crash tests of N2-W4-A category safety road barrier in horizontal concave arc. Int. J. Crashworthiness 2016, 21, 644–659. [Google Scholar] [CrossRef]
- Stanislawek, S.; Dziewulski, P.; Kedzierski, P. Deterioration of Road Barrier Protection Ability Due to Variable Road Friction. Int. J. Simul. Model. 2019, 18, 432–440. [Google Scholar] [CrossRef]
- Ulker, M.B.C.; Rahman, M.S.; Zhen, R.; Mirmiran, A. Traffic Barriers under Vehicular Impact: From Computer Simulation to Design Guidelines. Comput. Civ. Infrastruct. Eng. 2008, 23, 465–480. [Google Scholar] [CrossRef]
- Neves, R.R.; Fransplass, H.; Langseth, M.; Driemeier, L.; Alves, M. Performance of some basic types of road barriers subjected to the collision of a light vehicle. J. Braz. Soc. Mech. Sci. Eng. 2018, 40, 274. [Google Scholar] [CrossRef]
- Vasenjak, M.; Ren, Z. Improving the roadside safety with computational simulations. In Proceedings of the 4th European LS-DYNA Users Conference, Ulm, Germany, 2–3 May 2003; pp. 21–32. [Google Scholar]
- Wilde, K.; Bruski, D.; Budzyński, M.; Burzyński, S.; Chróścielewski, J.; Jamroz, K.; Pachocki, Ł.; Witkowski, W. Numerical Analysis of TB32 Crash Tests for 4-cable Guardrail Barrier System Installed on the Horizontal Convex Curves of Road. Int. J. Nonlinear Sci. Numer. Simul. 2020, 21, 65–81. [Google Scholar] [CrossRef]
- Pin, T.; Pian, T.H.H. The convergence of finite element method in solving linear elastic problems. Int. J. Solids Struct. 1967, 3, 865–879. [Google Scholar] [CrossRef]
- Babuška, I. The Rate of Convergence for the Finite Element Method. SIAM J. Numer. Anal. 1971, 8, 304–315. [Google Scholar] [CrossRef]


| Impact Speed Vi (km/h) | Post-Impact Speed Ve (km/h) Measured at a Point 12 m beyond the Impact Point with the Support Structure | ||
|---|---|---|---|
| HE—Energy Absorption in the High Degree | LE—Energy Absorption in the Low Degree | NE—Non-Energy Absorption | |
| 50 | Ve = 0 | 0 ≤ Ve ≤ 5 | 5 < Ve ≤ 50 |
| 70 | 0 ≤ Ve ≤ 5 | 5 < Ve ≤ 30 | 30 < Ve ≤ 70 |
| 100 | 0 ≤ Ve ≤ 50 | 50 < Ve ≤ 70 | 70 < Ve ≤ 10 |
| Energy Absorption Category | Safety Level of Occupant | Speed | |||
|---|---|---|---|---|---|
| Crash Test at a Speed of 35 km/hMaximum Values | Crash Test at a Speed of 50 km/h, 70 km/h or 100 km/hMaximum Values | ||||
| ASI | THIV (km/h) | ASI | THIV (km/h) | ||
| HE | 3 | 1.0 | 27 | 1.0 | 27 |
| 2 | 1.0 | 27 | 1.2 | 33 | |
| 1 | 1.0 | 27 | 1.4 | 44 | |
| LE | 3 | 1.0 | 27 | 1.0 | 27 |
| 2 | 1.0 | 27 | 1.2 | 33 | |
| 1 | 1.0 | 27 | 1.4 | 44 | |
| NE | 3 | 0.6 | 11 | 0.6 | 77 |
| 2 | 1.0 | 27 | 1.0 | 27 | |
| 1 | 1.0 | 27 | 1.2 | 33 | |
| Parameter | Variable | Unit | Value |
|---|---|---|---|
| Density | ρ | kg/m3 | 7850.0 |
| Young’s Modulus | EJC | MPa | 210,000 |
| Poisson’s ratio | vJC | - | 0.29 |
| JC yield stress | AJC | MPa | 235.0 |
| Hardening parameter | BJC | MPa | 520.0 |
| Hardening parameter | n | - | 0.638 |
| Strain rate parameter | CJC | - | 0.046 |
| Failure strain | Psfail | - | 1.3 |
| Value | |||||
|---|---|---|---|---|---|
| Soil Type | |||||
| Parameter | Variable | Unit | S | M | H |
| Density | ρ | kg/m3 | 2100.0 | 2100.0 | 2100.0 |
| Shear Modulus | G | MPa | 2.75 | 10.0 | 27.5 |
| Bulk modulus for unloading | Bulk | MPa | 32.1 | 64.2 | 129.9 |
| Yield function constants for plastic yield function | a0 | MPa2 | 0.00058 | 0.0016 | 0.0024 |
| a1 | MPa | 0.010 | 0.019 | 0.037 | |
| a2 | - | 0.045 | 0.078 | 0.140 | |
| Pressure cut-off fortension fracture (<0) | PC | MPa | −2.0 | −2.0 | −2.0 |
| Bulk modulus for loading | K | MPa | 10.7 | 21.4 | 43.4 |
| Parameter | Referenced Study [17] | Present Study |
|---|---|---|
| Lighting pole height | 12.0 m | 8.0 m |
| Lighting pole thickness | 2.0 mm | 2.0 mm |
| Lighting pole material | S355 steel | S355 steel |
| Lighting pole mount type | concrete foundation | soil |
| Lighting pole mesh size | 10.0 mm | 10.0 mm |
| FEA code | LS-Dyna | LS-Dyna |
| Vehicle | Seat Ibiza (exp.) Geo Metro (sim.) | Geo Metro |
| Experiment type | EN 12767—100HE3 | EN 12767—100HE3 |
| Analyzed Factor | Test Name | Factor Values in Each Test | ||
|---|---|---|---|---|
| Soil Type | Mesh Size | Friction Properties | ||
| Mesh | S_05_0.05 | S | 5 mm | μ = 0.05 |
| S_10_0.05 | 10 mm | |||
| S_15_0.05 | 15 mm | |||
| S_20_0.05 | 20 mm | |||
| S_25_0.05 | 25 mm | |||
| S_35_0.05 | 35 mm | |||
| Soil | S_15_0.05 | S | 15 mm | μ = 0.05 |
| M_15_0.05 | M | |||
| H_15_0.05 | H | |||
| Friction | S_15_0.00 | S | 15 mm | μ = 0.05 |
| S_15_0.05 | 10 mm | μ = 0.00 | ||
| S_15_0.10 | 15 mm | μ = 0.10 | ||
| S_15_0.15 | 20 mm | μ = 0.15 | ||
| S_15_0.25 | 25 mm | μ = 0.25 | ||
| S_15_0.30 | 35 mm | μ = 0.30 | ||
| Test Name | Parameter | ||
|---|---|---|---|
| ASI (max. 1.0) | THIV (≤27 km/h) | Velocity (≤50 km/h) | |
| Mesh Size | |||
| S_05_0.05 | 0.83 + | 29.82 − | 46.0 + |
| S_10_0.05 | 0.79 + | 31.39 − | 43.0 + |
| S_15_0.05 | 0.78 + | 31.59 − | 38.0 + |
| S_20_0.05 | 0.81 + | 32.18 − | 38.0 + |
| S_25_0.05 | 0.78 + | 32.85 − | 30.0 + |
| S_35_0.05 | 0.98 + | 36.55 − | 12.0 + |
| Soil Type | |||
| S_15_0.05 | 0.78 + | 31.59 − | 38.0 + |
| M_15_0.05 | 0.94 + | 32.05 − | 35.0 + |
| H_15_0.05 | 1.09 - | 35.33 − | 30.0 + |
| Friction Coefficient | |||
| S_15_0.00 | 0.80 + | 30.29 − | 40.0 + |
| S_15_0.05 | 0.78 + | 31.59 − | 39.0 + |
| S_15_0.10 | 0.82 + | 32.32 − | 39.0 + |
| S_15_0.15 | 0.83 + | 33.31 − | 38.0 + |
| S_15_0.25 | 0.85 + | 34.26 − | 37.0 + |
| S_15_0.30 | 1.22 - | 36.78 − | 10.0 + |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Baranowski, P.; Damaziak, K. Numerical Simulation of Vehicle–Lighting Pole Crash Tests: Parametric Study of Factors Influencing Predicted Occupant Safety Levels. Materials 2021, 14, 2822. https://doi.org/10.3390/ma14112822
Baranowski P, Damaziak K. Numerical Simulation of Vehicle–Lighting Pole Crash Tests: Parametric Study of Factors Influencing Predicted Occupant Safety Levels. Materials. 2021; 14(11):2822. https://doi.org/10.3390/ma14112822
Chicago/Turabian StyleBaranowski, Paweł, and Krzysztof Damaziak. 2021. "Numerical Simulation of Vehicle–Lighting Pole Crash Tests: Parametric Study of Factors Influencing Predicted Occupant Safety Levels" Materials 14, no. 11: 2822. https://doi.org/10.3390/ma14112822
APA StyleBaranowski, P., & Damaziak, K. (2021). Numerical Simulation of Vehicle–Lighting Pole Crash Tests: Parametric Study of Factors Influencing Predicted Occupant Safety Levels. Materials, 14(11), 2822. https://doi.org/10.3390/ma14112822






