The Effect of Direct Electric Current on the Plastic Behavior of AA7075 Aluminum Alloy in Different States of Hardening
Abstract
:1. Introduction
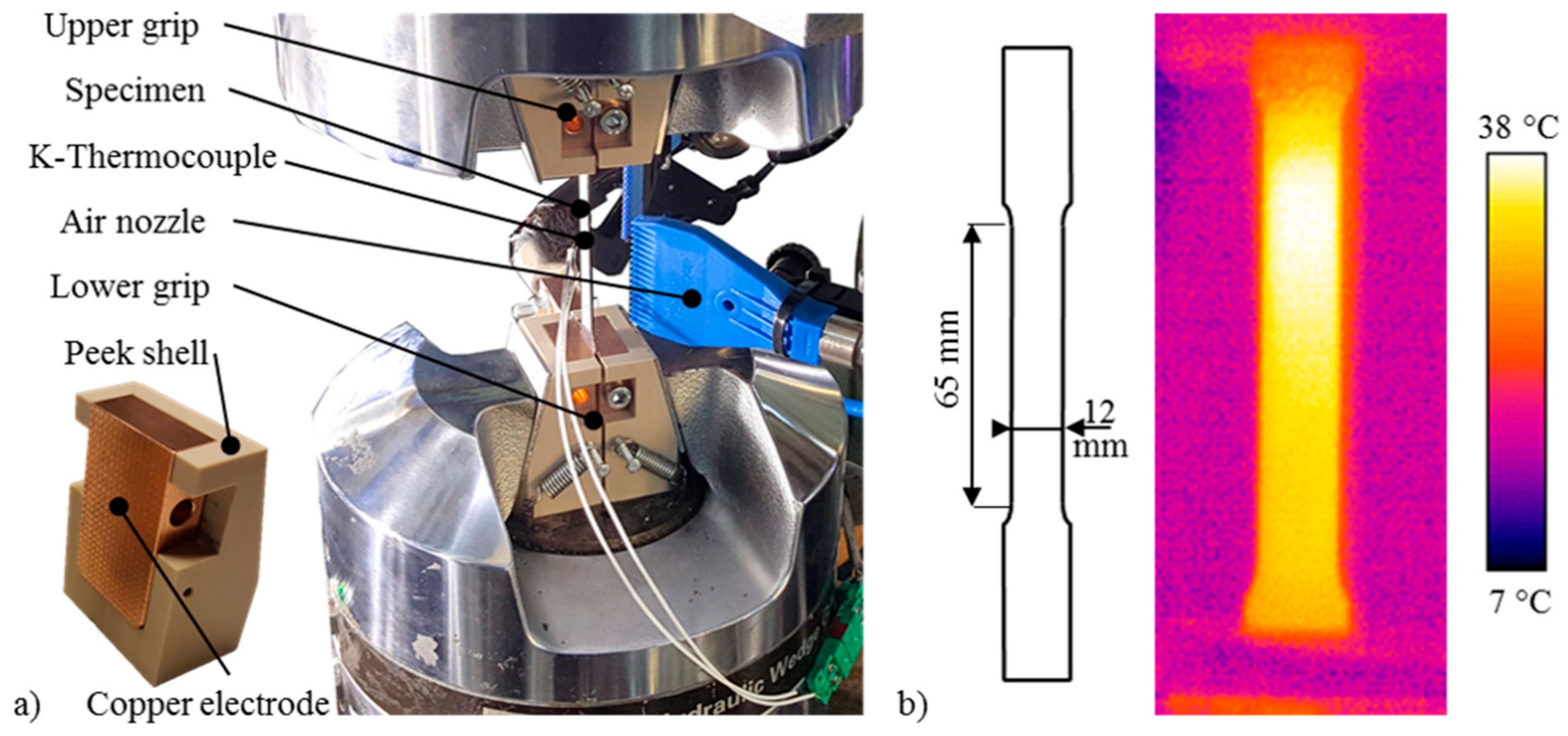
2. Materials and Methods
3. Results
3.1. Thermo-Electrical Results
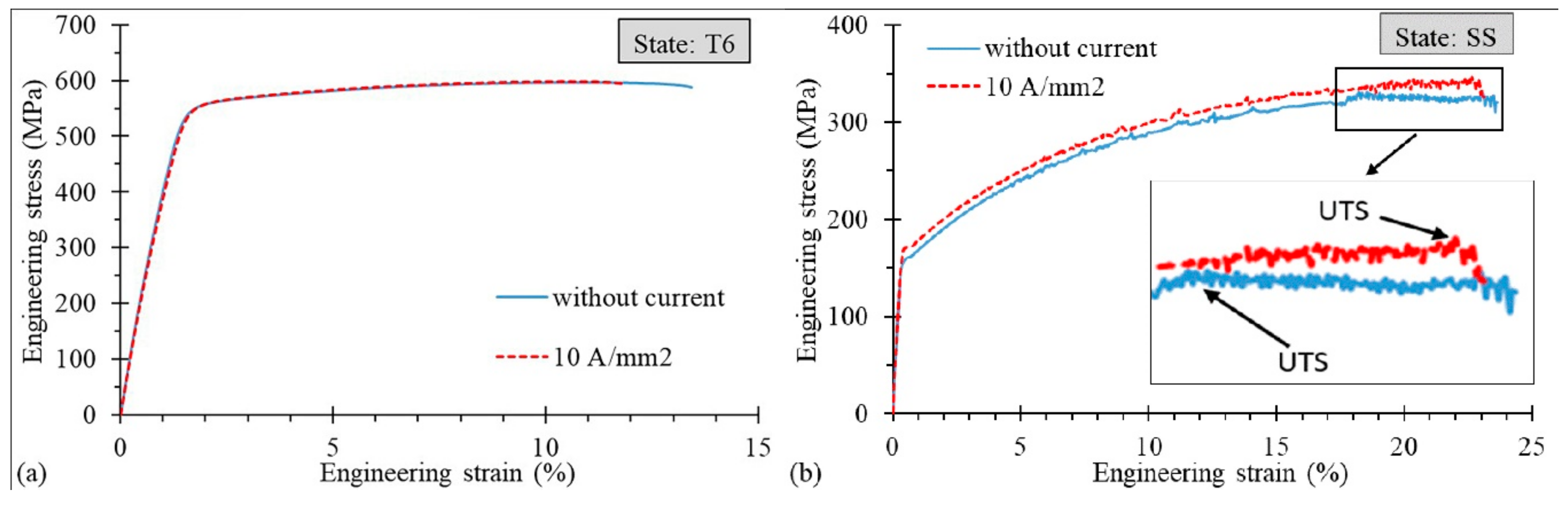
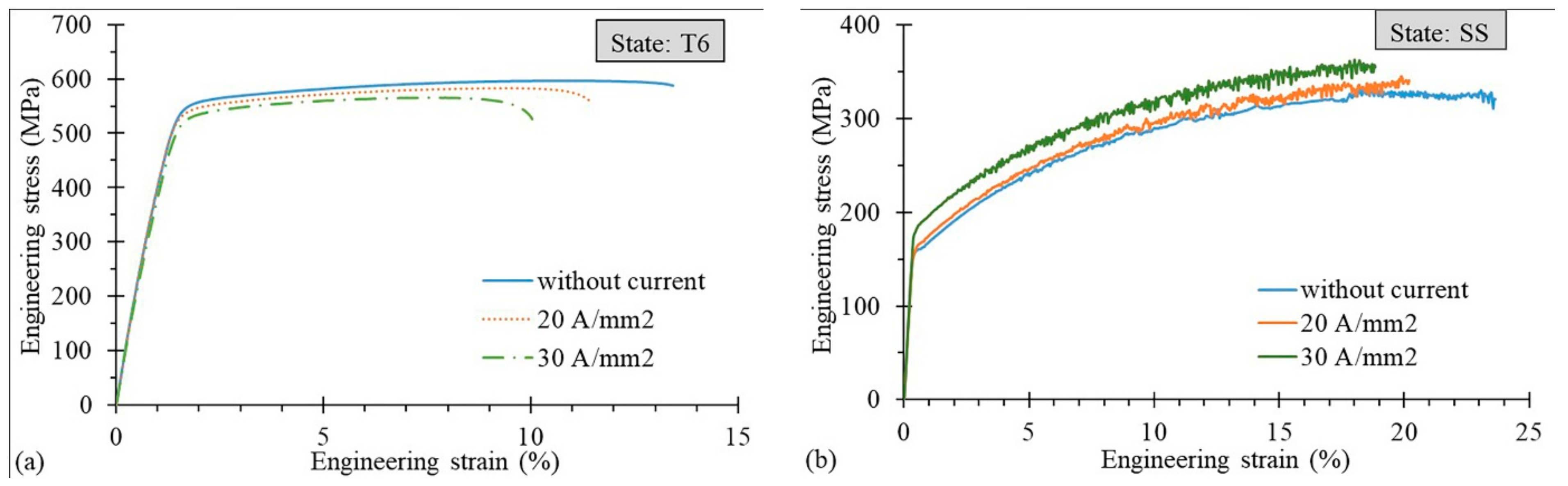
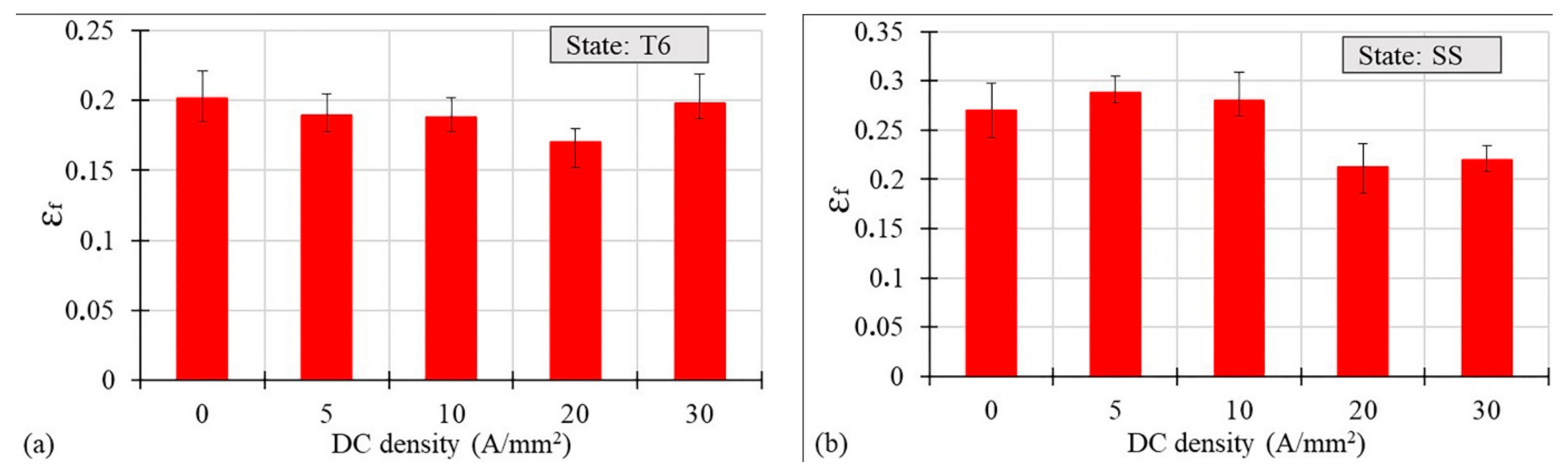
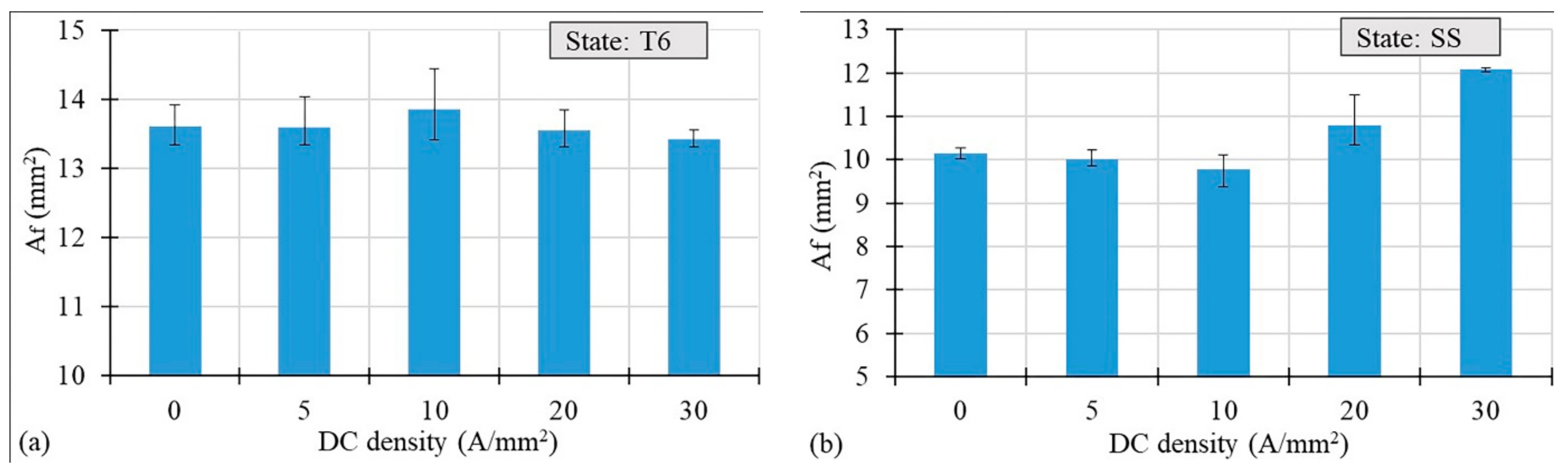
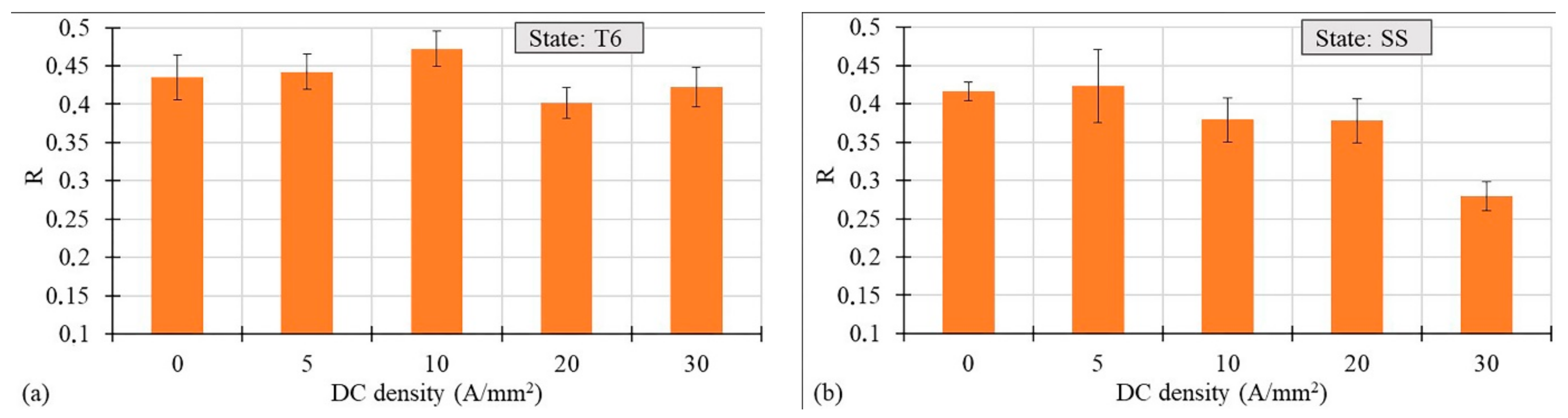
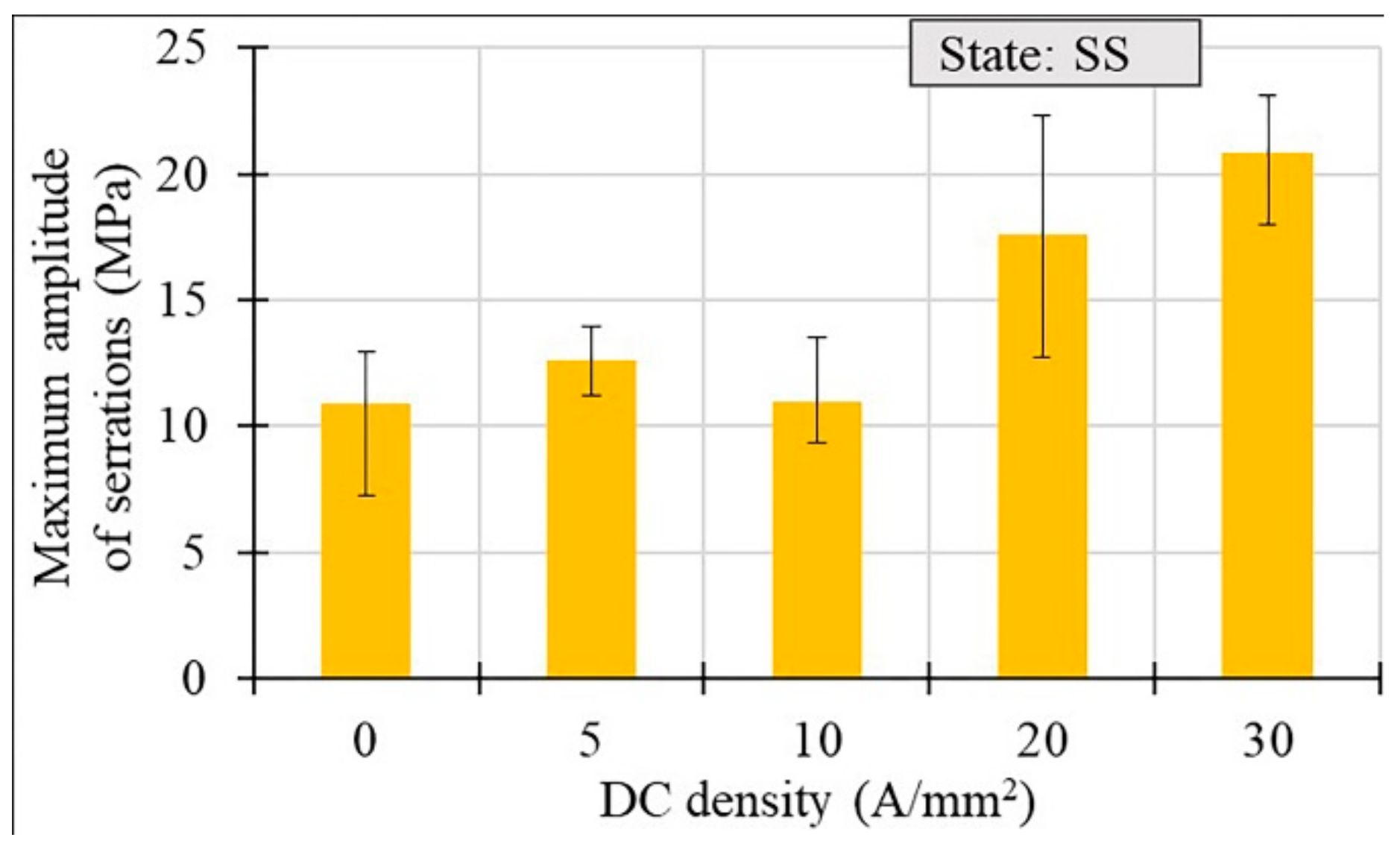
3.2. Mechanical Results
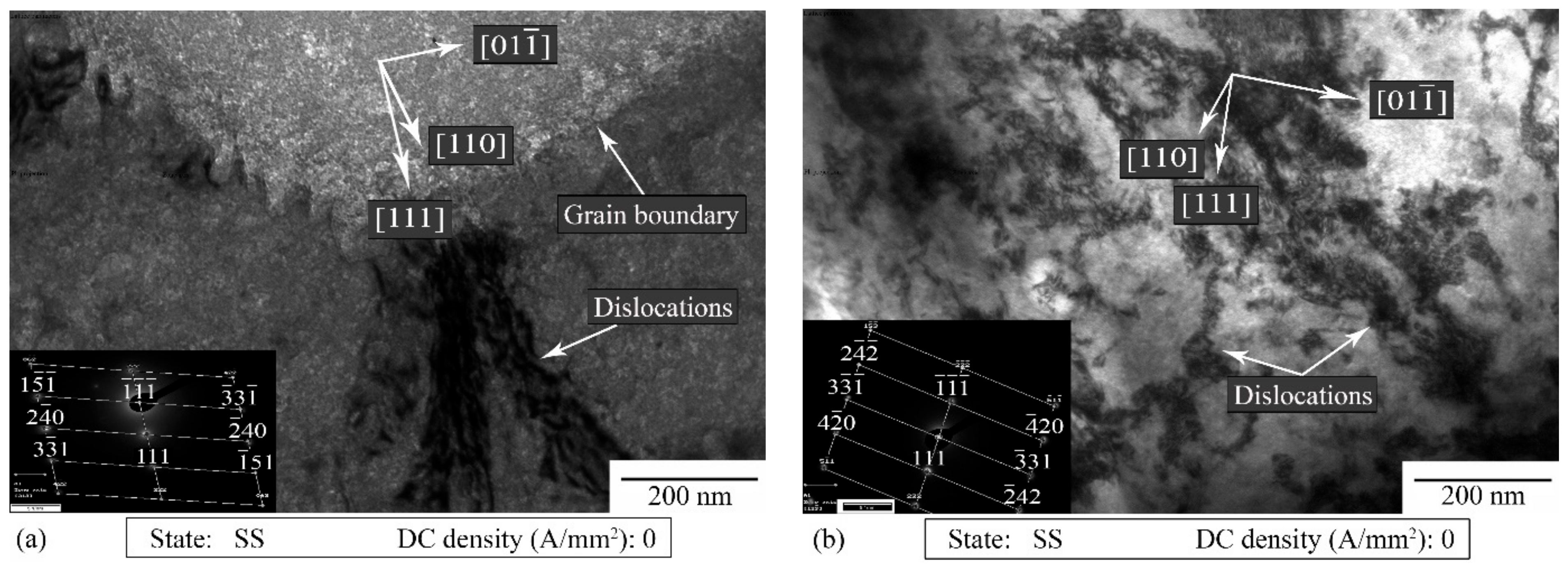
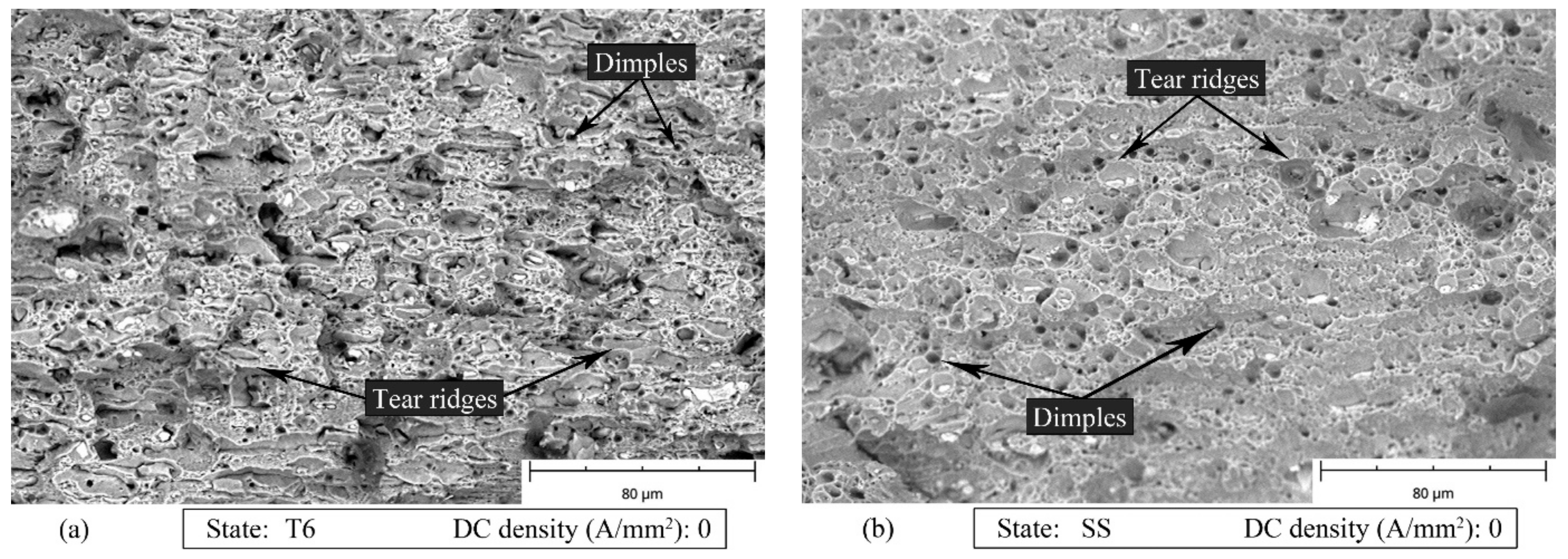
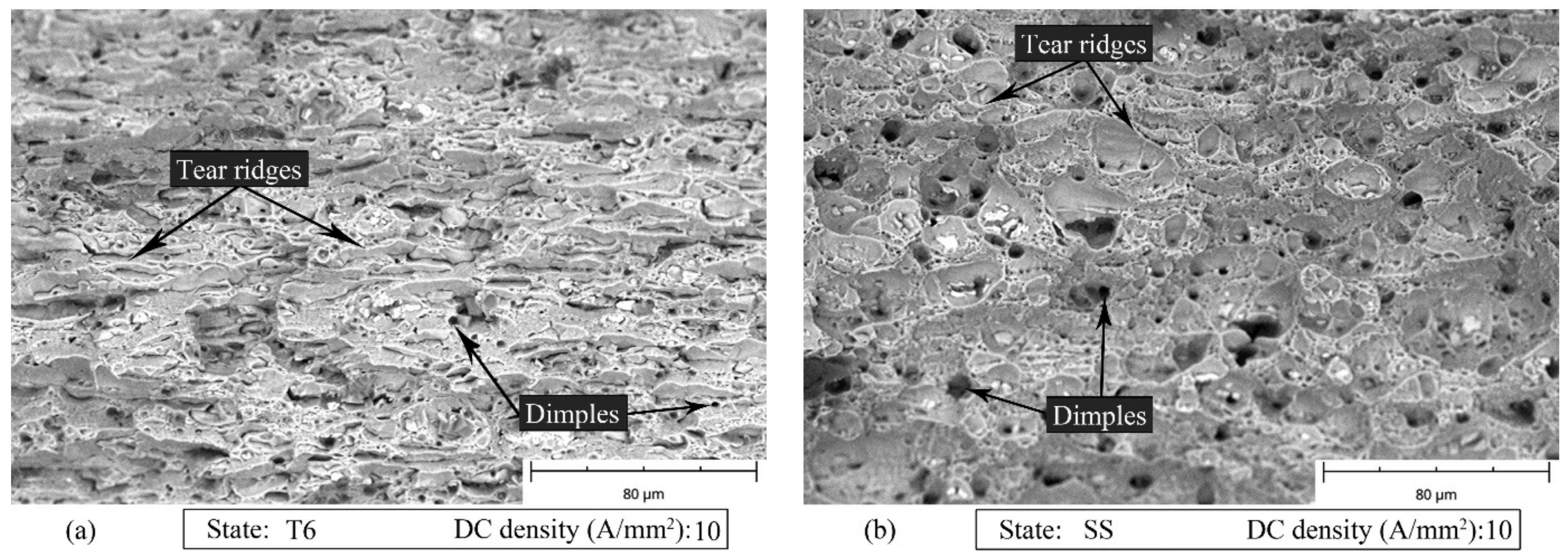
3.3. Microstructural Results
4. Discussion
4.1. Electron Wind Theory
4.2. Heterogeneous Microscale Joule Heating
4.3. Portevin–Le Chatelier Effect
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Polak, S.; Kaczyński, P.; Gronostajski, Z.; Jaskiewicz, K.; Krawczyk, J.; Skwarski, M.; Zwierzchowski, M.; Chorzȩpa, W. Warm forming of 7075 aluminum alloys. Procedia Eng. 2017, 207, 2399–2404. [Google Scholar] [CrossRef]
- Xiao, W.; Wang, B. Behaviors and modeling of thermal forming limits of AA7075 aluminum sheet. Arch. Civ. Mech. Eng. 2020, 20. [Google Scholar] [CrossRef] [Green Version]
- Liu, Y.; Zhu, Z.; Wang, Z.; Zhu, B.; Wang, Y.; Zhang, Y. Formability and lubrication of a B-pillar in hot stamping with 6061 and 7075 aluminum alloy sheets. Procedia Eng. 2017, 307, 723–728. [Google Scholar] [CrossRef]
- Gronostajski, Z.; Polak, S.; Jaśkiewicz, K.; Kaczyńskia, P.; Skwarski, M.; Krawczyk, J.; Chorzępa, W.; Śliz, K.; Uzar, S. Properties of B-pillar made of aluminum 7075 in warm forming process. Procedia Manuf. 2019. [Google Scholar] [CrossRef]
- Conrad, H. Some effects of an electric field on the plastic deformation of metals and ceramics. Mater. Res. Innov. 1998, 2, 1–8. [Google Scholar] [CrossRef]
- Roh, J.H.; Seo, J.J.; Hong, S.T.; Kim, M.J.; Han, H.N.; Roth, J.T. The mechanical behavior of 5052-H32 aluminum alloys under a pulsed electric current. Int. J. Plast. 2014, 58, 84–99. [Google Scholar] [CrossRef]
- Kim, M.; Song, J.; Huh, H. Effect of Pre-strain on Tensile Properties of Al5052-H32 under an Electropulsing Condition. Procedia Eng. 2017, 207, 371–376. [Google Scholar] [CrossRef]
- Salandro, W.A.; Jones, J.J.; McNeal, T.A.; Roth, J.T.; Hong, S.T.; Smith, M.T. Formability of Al 5xxx sheet metals using pulsed current for various heat treatments. J. Manuf. Sci. Eng. Trans. ASME 2010, 132, 051016. [Google Scholar] [CrossRef]
- Zhao, K.; Fan, R.; Wang, L. The Effect of Electric Current and Strain Rate on Serrated Flow of Sheet Aluminum Alloy 5754. J. Mater. Eng. Perform. 2016, 25, 781–789. [Google Scholar] [CrossRef]
- Kim, M.J.; Lee, K.; Oh, K.H.; Choi, I.S.; Yu, H.H.; Hong, S.T.; Han, H.N. Electric current-induced annealing during uniaxial tension of aluminum alloy. Scr. Mater. 2014, 75, 58–61. [Google Scholar] [CrossRef]
- Ross, C.D.; Irvin, D.B.; Roth, J.T. Manufacturing aspects relating to the effects of direct current on the tensile properties of metals. J. Eng. Mater. Technol. Trans. ASME 2007. [Google Scholar] [CrossRef]
- Breda, M.; Calliari, I.; Bruschi, S.; Forzan, M.; Ghiotti, A.; Michieletto, F.; Spezzapria, M.; Gennari, C. Influence of stacking fault energy in electrically assisted uniaxial tension of FCC metals. Mater. Sci. Technol. 2017, 33, 317–325. [Google Scholar] [CrossRef]
- Ghiotti, A.; Bruschi, S.; Simonetto, E.; Gennari, C.; Calliari, I.; Bariani, P. Electroplastic effect on AA1050 aluminum alloy formability. CIRP Ann. 2018, 67, 289–292. [Google Scholar] [CrossRef]
- Ruszkiewicz, B.J.; Mears, L.; Roth, J.T. Investigation of Heterogeneous Joule Heating as the Explanation for the Transient Electroplastic Stress Drop in Pulsed Tension of 7075-T6 Aluminum. J. Manuf. Sci. Eng. Trans. ASME 2018, 140, 091014. [Google Scholar] [CrossRef] [Green Version]
- Ruszkiewicz, B.J.; Mears, L. Investigation of the electroplastic effect through nominally equal energy deformation. In Proceedings of the ASME 2018 13th International Manufacturing Science Engineering Conference MSEC, College Station, TX, USA, 18–22 June 2018; Volume 4. [Google Scholar] [CrossRef]
- Hong, S.T.; Jeong, Y.H.; Chowdhury, M.N.; Chun, D.M.; Kim, M.J.; Han, H.N. Feasibility of electrically assisted progressive forging of aluminum 6061-T6 alloy. CIRP Ann. Manuf. Technol. 2015, 64, 277–280. [Google Scholar] [CrossRef]
- Zimniak, Z.; Radkiewicz, G. The electroplastic effect in the cold-drawing of copper wires for the automotive industry. Arch. Civ. Mech. Eng. 2008, 8, 173–179. [Google Scholar] [CrossRef]
- Bao, W.; Chu, X.; Lin, S.; Gao, J. Electro-plastic effect on tensile deformation behavior and microstructural mechanism of AZ31B alloy. Mater. Sci. Technol. 2017, 33, 836–845. [Google Scholar] [CrossRef]
- Zhao, K.; Fan, R. The effect of pulse electric current on the mechanical properties and fracture behaviors of aluminum alloy AA5754. J. Eng. Mater. Technol. Trans. ASME 2016, 138, 041009. [Google Scholar] [CrossRef]
- Yilmaz, A. The Portevin–Le Chatelier effect: A review of experimental findings. Sci. Technol. Adv. Mater. 2011, 12. [Google Scholar] [CrossRef]
- Chen, J.-Z.; Zhen, L.; Fan, L.-W.; Yang, S.-J.; Dai, S.-L.; Shao, W.-Z. Portevin–Le Chatelier effect in Al-Zn-Mg-Cu-Zr aluminum alloy. Trans. Nonferrous Met. Soc. China 2009, 19, 1071–1075. [Google Scholar] [CrossRef]
- Shibkov, A.A.; Denisov, A.A.; Zheltov, M.A.; Zolotov, A.E.; Gasanov, M.F. The electric current-induced suppression of the Portevin–Le Chatelier effect in Al-Mg alloys. Mater. Sci. Eng. A 2014, 610, 338–343. [Google Scholar] [CrossRef]
- Hariharan, K.; Lee, M.G.; Kim, M.J.; Han, H.N.; Kim, D.; Choi, S. Decoupling Thermal and Electrical Effect in an Electrically Assisted Uniaxial Tensile Test Using Finite Element Analysis. Metall. Mater. Trans. A Phys. Metall. Mater. Sci. 2015, 46, 3043–3051. [Google Scholar] [CrossRef]
- Xu, H.; Liu, X.; Zhang, D.; Zhang, X. Minimizing serrated flow in Al-Mg alloys by electroplasticity. J. Mater. Sci. Technol. 2019, 35, 1108–1112. [Google Scholar] [CrossRef]
- Xu, X.; Zhao, Y.; Ma, B.; Zhang, M. Rapid precipitation of T-phase in the 2024 aluminum alloy via cyclic electropulsing treatment. J. Alloys Compd. 2014, 610, 506–510. [Google Scholar] [CrossRef]
- Liu, Y.; Huang, M.; Ma, Z.; Zhan, L. Influence of the low-density pulse current on the ageing behavior of AA2219 aluminum alloy. J. Alloys Compd. 2016, 673, 358–363. [Google Scholar] [CrossRef]
- Thevenet, D.; Mliha-Touati, M.; Zeghloul, A. Characteristics of the propagating deformation bands associated with the Portevin–Le Chatelier effect in an Al-Zn-Mg-Cu alloy. Mater. Sci. Eng. A 2000, 291, 110–117. [Google Scholar] [CrossRef]
- Thevenet, D.; Mliha-Touati, M.; Zeghloul, A. The effect of precipitation on the Portevin–Le Chatelier effect in an Al-Zn-Mg-Cu alloy. Mater. Sci. Eng. A 1999, 266, 175–182. [Google Scholar] [CrossRef]




| Element | Zn | Mg | Cu | Cr | Fe | Ti | Mn | Al |
|---|---|---|---|---|---|---|---|---|
| % | 6.15 | 2.79 | 1.72 | 0.19 | 0.08 | 0.04 | 0.02 | residual |
| E (GPa) | Y0.2 (MPa) | UTS (MPa) | A (%) |
|---|---|---|---|
| 71.6 (±6) | 538 (±3) | 591.2 (±1.6) | 13.6 (±0.3) |
| Material State | T6 | SS |
|---|---|---|
| DC density (A/mm2) | 0, 5, 10, 20, 30 | 0, 5, 10, 20, 30 |
| Rolling direction (deg) | 0 | 0 |
| Strain rate (s−1) | 0.1 | 0.1 |
| Repeatability | 3 | 3 |
| DC Density (A/mm2) | 0 | 5 | 10 | 20 | 30 |
| Maximum Temperature in the T6 (°C) | 20 | 48 | 54 | 95 | 115 |
| Maximum Temperature in the SS (°C) | 20 | 36 | 42 | 70 | 106 |
| Strain Rate (s−1) | 0.1 | 0.1 | 0.1 | 0.1 | 0.1 |
| Repeatability | 3 | 3 | 3 | 3 | 3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dobras, D.; Bruschi, S.; Simonetto, E.; Rutkowska-Gorczyca, M.; Ghiotti, A. The Effect of Direct Electric Current on the Plastic Behavior of AA7075 Aluminum Alloy in Different States of Hardening. Materials 2021, 14, 73. https://doi.org/10.3390/ma14010073
Dobras D, Bruschi S, Simonetto E, Rutkowska-Gorczyca M, Ghiotti A. The Effect of Direct Electric Current on the Plastic Behavior of AA7075 Aluminum Alloy in Different States of Hardening. Materials. 2021; 14(1):73. https://doi.org/10.3390/ma14010073
Chicago/Turabian StyleDobras, Daniel, Stefania Bruschi, Enrico Simonetto, Małgorzata Rutkowska-Gorczyca, and Andrea Ghiotti. 2021. "The Effect of Direct Electric Current on the Plastic Behavior of AA7075 Aluminum Alloy in Different States of Hardening" Materials 14, no. 1: 73. https://doi.org/10.3390/ma14010073
APA StyleDobras, D., Bruschi, S., Simonetto, E., Rutkowska-Gorczyca, M., & Ghiotti, A. (2021). The Effect of Direct Electric Current on the Plastic Behavior of AA7075 Aluminum Alloy in Different States of Hardening. Materials, 14(1), 73. https://doi.org/10.3390/ma14010073






