Next Article in Journal
Long-Term Clinical Outcomes of Treatment with Dental Implants with Acid Etched Surface
Next Article in Special Issue
Following the Martensitic Configuration Footprints in the Transition Route of Ni-Mn-Ga Magnetic Shape Memory Films: Insight into the Role of Twin Boundaries and Interfaces
Previous Article in Journal
Effects of Volume Fraction and Surface Area of Aggregates on the Static Yield Stress and Structural Build-Up of Fresh Concrete
Previous Article in Special Issue
Structural and Magnetic Properties of FePd Thin Film Synthesized by Electrodeposition Method
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Materials
Volume 13
Issue 7
10.3390/ma13071552
materials-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Magnetic Properties of Electrospun Magnetic Nanofiber Mats after Stabilization and Carbonization

by
Nadine Fokin
1,
Timo Grothe
2,
Al Mamun
2,
Marah Trabelsi
2,3,
Michaela Klöcker
2,
Lilia Sabantina
2,
Christoph Döpke
2,
Tomasz Blachowicz
4,
Andreas Hütten
1 and
Andrea Ehrmann
2,*
1
Department of Physics, Center for Spinelectronic Materials and Devices, Bielefeld University, 33615 Bielefeld, Germany
2
Faculty of Engineering and Mathematics, Bielefeld University of Applied Sciences, 33619 Bielefeld, Germany
3
Ecole Nationale d’Ingénieurs de Sfax (ENIS), Sfax 3038, Tunisia
4
Institute of Physics–CSE, Silesian University of Technology, 44-100 Gliwice, Poland
*
Author to whom correspondence should be addressed.
Materials 2020, 13(7), 1552; https://doi.org/10.3390/ma13071552
Submission received: 5 March 2020 / Revised: 23 March 2020 / Accepted: 24 March 2020 / Published: 27 March 2020
(This article belongs to the Special Issue Magnetic Nanomaterials)
Browse Figures
Versions Notes

Abstract

:
Magnetic nanofibers are of great interest in basic research, as well as for possible applications in spintronics and neuromorphic computing. Here we report on the preparation of magnetic nanofiber mats by electrospinning polyacrylonitrile (PAN)/nanoparticle solutions, creating a network of arbitrarily oriented nanofibers with a high aspect ratio. Since PAN is a typical precursor for carbon, the magnetic nanofiber mats were stabilized and carbonized after electrospinning. The magnetic properties of nanofiber mats containing magnetite or nickel ferrite nanoparticles were found to depend on the nanoparticle diameters and the potential after-treatment, as compared with raw nanofiber mats. Micromagnetic simulations underlined the different properties of both magnetic materials. Atomic force microscopy and scanning electron microscopy images revealed nearly unchanged morphologies after stabilization without mechanical fixation, which is in strong contrast to pure PAN nanofiber mats. While carbonization at 500 °C left the morphology unaltered, as compared with the stabilized samples, stronger connections between adjacent fibers were formed during carbonization at 800 °C, which may be supportive of magnetic data transmission.

1. Introduction

Electrospinning makes it possible to create nanofiber mats from diverse materials, such as pure polymers [1,2,3], polymers blends, composite fibers from polymers and ceramics or metals [4,5], and cyclodextrins [6]. These can be used in diverse applications such as filters [7,8,9], biotechnology and tissue engineering [10,11,12], for energy harvesting and storage [13,14], or other “smart” functions [15]. Recently, a strong focus of diverse research groups has been related to electrospinning magnetic nanofibers, either as composites [16,17,18] or, after calcination of the composites to remove polymers, as pure metal nanofibers [19,20,21]. Such magnetic nanofiber mats can be used, e.g., as catalysts [22], for magnetic hyperthermia [23], or electromagnetic shielding [24]. In contrast to other methods, such as electrodeposition [25,26], seed-mediated growth [27], magnetic field patterning of magnetic precursor inks printed on a substrate [28], or electrochemical deposition [29], electrospinning has the advantage of enabling preparation of large-scale nanofiber networks in short times without the necessity to use a cleanroom, highly sophisticated equipment, or highly toxic material, and is thus often used to prepare magnetic nanofibers [30,31,32,33,34]. Notably, polyacrylonitrile (PAN) can be spun from the low-toxic solvent DMSO [3], making the whole process relatively easy to handle and avoiding unnecessary environmental pollution.
In contrast to other methods of producing magnetic nanofibers, electrospinning usually creates nanofibers with different bending radii and forms a nanofiber mat without or with only low-fiber orientation [35,36,37], resulting in much more complicated magnetic anisotropies than straight, even nanofibers [38,39,40]. This makes electrospun nanofiber mats challenging for some applications, e.g., in racetrack memory, which usually consists of an array of parallel arranged magnetic nanowires and can theoretically store data series in some ten- to hundred-domain walls per nanowire [41].
On the other hand, chaotic nanofiber mats or fiber networks with low orientation are interesting for other research areas; e.g., for bio-inspired neuromorphic computing [42]. This relatively new research area aims at reaching high-performance computing at low power consumption, avoiding the von Neumann bottleneck due to the separation of processor and memory in modern computers built according to the von Neumann architecture by integrating both parts, i.e., storing and calculating data in a structure inspired by the human brain. Recently, many attempts have been reported to implement neural networks, imitating the biological function of the brain, with conventional computers [43,44,45]. Much better performance in terms of speed and reduced power consumption, however, can be expected if physical networks of connected components are created to enable massive parallel computing [46,47,48,49].
Quasistatic and dynamic studies of the magnetic properties of bent nanofibers have been performed by different research groups, using simulations or experimental investigations which have underlined the aforementioned influence of diameter and curvature distributions [50,51,52]. For example, Alejos et al. showed that a local longitudinal field could be used to control the current-induced magnetization reversal in ferromagnetic strips. They used two bit-lines, above and below a ferromagnetic line sandwiched between a heavy metal and an oxidized layer. In such a heavy metal/ferromagnet/oxide triple layer, current-induced switching due to a global in-plane field is well-known [53,54,55], while the local field created domain walls at defined positions—which is necessary for racetrack applications [52].
For potential application in neuromorphic computing, memristors or other elements are often integrated in statistic fiber networks [56,57,58], an approach which cannot be realized with a single-step electrospinning process. Here, instead, we report on a combination of magnetic nanofibers with beads, tailored by a reduction of the polymer content in the spinning solution, as evaluated in detail in a former study [59]. This combination of long, thin nanofibers with beads along the fibers allows for combining data processing and storage, as shown in former studies [18,60,61,62,63].

2. Materials and Methods

2.1. Electrospinning

For the spinning solution, 14% polyacrylonitrile (PAN) (X-PAN, Dralon, Dormagen, Germany) was dissolved in dimethyl sulfoxide (DMSO, min 99.9%, purchased from S3 chemicals, Bad Oeynhausen, Germany) by stirring at room temperature for 2 h with a magnetic stirrer. PAN was chosen due to the possibility of stabilizing and carbonizing it after electrospinning, making it conductive [64].
To make the fibers magnetic, nanoparticles from Fe3O4 (magnetite, particle size 50–100 nm) and Fe2O3/NiO (diiron nickel tetraoxide or nickel ferrite, particle size <50 nm) were added (both purchased from Merck KGaA, Darmstadt, Germany) by stirring manually for 10 min and dispersing the nanoparticles in an ultrasonic bath for 40 min at 35 °C at a frequency of 37 kHz. Both materials are ferrimagnetic in bulk form and become superparamagnetic for very small nanoparticles [65,66]. The polymer: nanoparticle weight ratio used here was 1:1.8, identical to the highest nanoparticle concentration used in a previous study [18].
The needleless electrospinning machine Nanospider Lab (Elmarco, Liberec, Czech Republic) was used to prepare nanofiber mats on a polypropylene nonwoven substrate. Electrospinning was performed using a high voltage of 80 kV, a nozzle diameter of 0.9 mm, a carriage speed of 150 mm/s, a ground-substrate distance of 240 mm, and an electrode-substrate distance of 50 mm. The temperature in the chamber was held at 22 °C, while the relative humidity was set to 32%.

2.2. Stabilization and Carbonization

Parts of the samples were afterwards stabilized in a B150 muffle furnace (Nabertherm, Germany), approaching a temperature of 280 °C with a heating rate of 0.5 K/min, followed by isothermal treatment for 1 h. This step was necessary to enable carbonization, since pure PAN is not thermally stable at temperatures far above 300 °C, while carefully stabilized PAN is not degraded by high temperatures. Subsequent carbonization was performed in a CTF 12/TZF 12 furnace (Carbolite Gero Ltd., Neuhausen, Germany), approaching temperatures of 500 and 800 °C, respectively, with a heating rate of 10 K/min in a nitrogen flow of 150 mL/min (STP), again followed by isothermal treatment for 1 h.

2.3. Experimental Investigations

Magnetic measurements were performed with an alternating gradient magnetometer (AGM) Princeton MicroMag (LakeShore Cryotronics, Westerville, OH, USA). The surface morphology of the nanofiber mats was investigated using a scanning electron microscope (SEM), the Zeiss 1450VPSE, and an atomic force microscope (AFM), the FlexAFM Axiom (Nanosurf, Liestal, Switzerland). For the chemical investigations, a Fourier-transform infrared (FTIR) spectrometer, Excalibur 3100 (Varian, Inc., Palo Alto, CA, USA), was used. Fiber diameters were evaluated using the software ImageJ 1.51j8 (from National Institutes of Health, Bethesda, MD, USA).

2.4. Simulations

For comparison with the experimental investigations of the magnetic properties, the micromagnetic simulation software OOMMF (Object-Oriented MicroMagnetic Framework) was used [67], applying finite differences for meshing and solving the Landau–Lifshitz–Gilbert equation of motion [68]. The following material parameters were chosen for Fe3O4 (Fe2O3/NiO) according to typical literature values [69,70,71,72,73]: MS,magnetite = 500 × 103 A/m (MS,nickel-ferrite = 270 × 103 A/m), exchange constant Amagnetite = 12 × 10−12 J/m (Anickel-ferrite = 12 × 10−12 J/m), magneto-crystalline anisotropy constant K1,magnetite = 11 × 103 J/m3 (K1,nickel-ferrite = −6.9 × 103 J/m3). Since the electrospinning process can be expected to produce arbitrarily oriented crystallographic orientations of the nanoparticles, random orientations of the simulated grains of 5 nm diameter were chosen. While this approach would lead to strong modifications of the simulation results due to arbitrary crystallographic orientations in subsequent simulations, in case of cobalt or other materials with large magneto-crystalline anisotropy [74], here the influence of the shape anisotropy dominated the relatively small magneto-crystalline anisotropy, as usual in iron or permalloy samples of similar dimensions [75]. Setting the Gilbert damping constant to α = 0.5, a quasi-static case was modeled. Field sweeps were performed in the range of ±300 mT to reach saturation.
The simulated shapes—a branched and a single fiber, respectively—were taken arbitrarily from an SEM image as an example of possible parts of such nanofiber mats (Figure 1). Fiber thickness was assumed as 120 nm, as according to the chosen part of a real magnetic nanofiber mat. Generally, the fiber thickness and thus the shape of the cross-section can be modified by a hot-pressing treatment [76]; this possibility was not further investigated here.
The simulated hysteresis loops shown here were superposed of 7 hysteresis loops each, simulated for external magnetic field sweeps in the range of ±3000 Oe with the field axis applied along 0° (horizontal in Figure 1), 15°, … 90° (vertical in Figure 1). This was equivalent to averaging over different nanofiber orientations in a constant external magnetic field, as it was applied during the AGM measurements on the magnetic nanofiber mats.

3. Results and Discussion

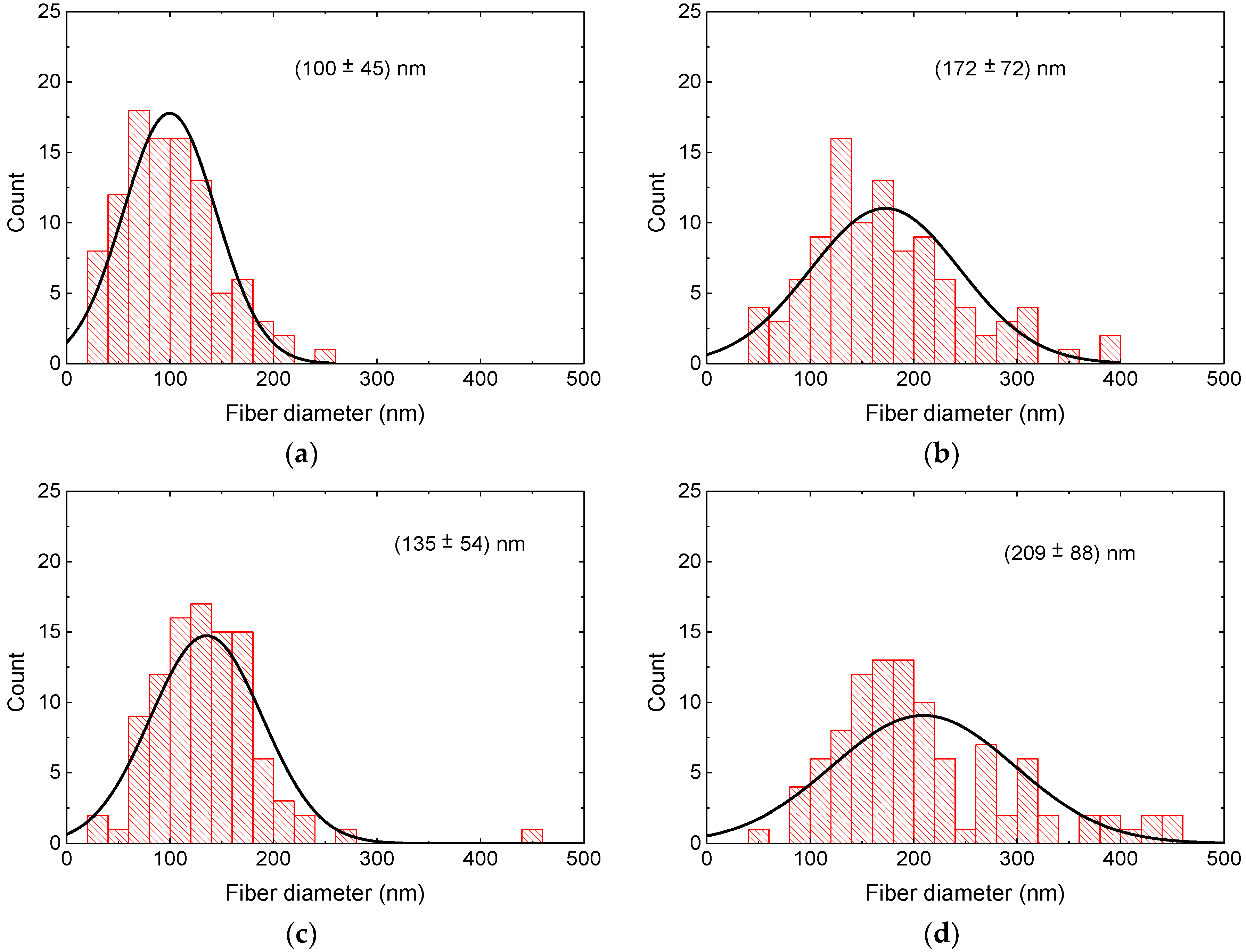
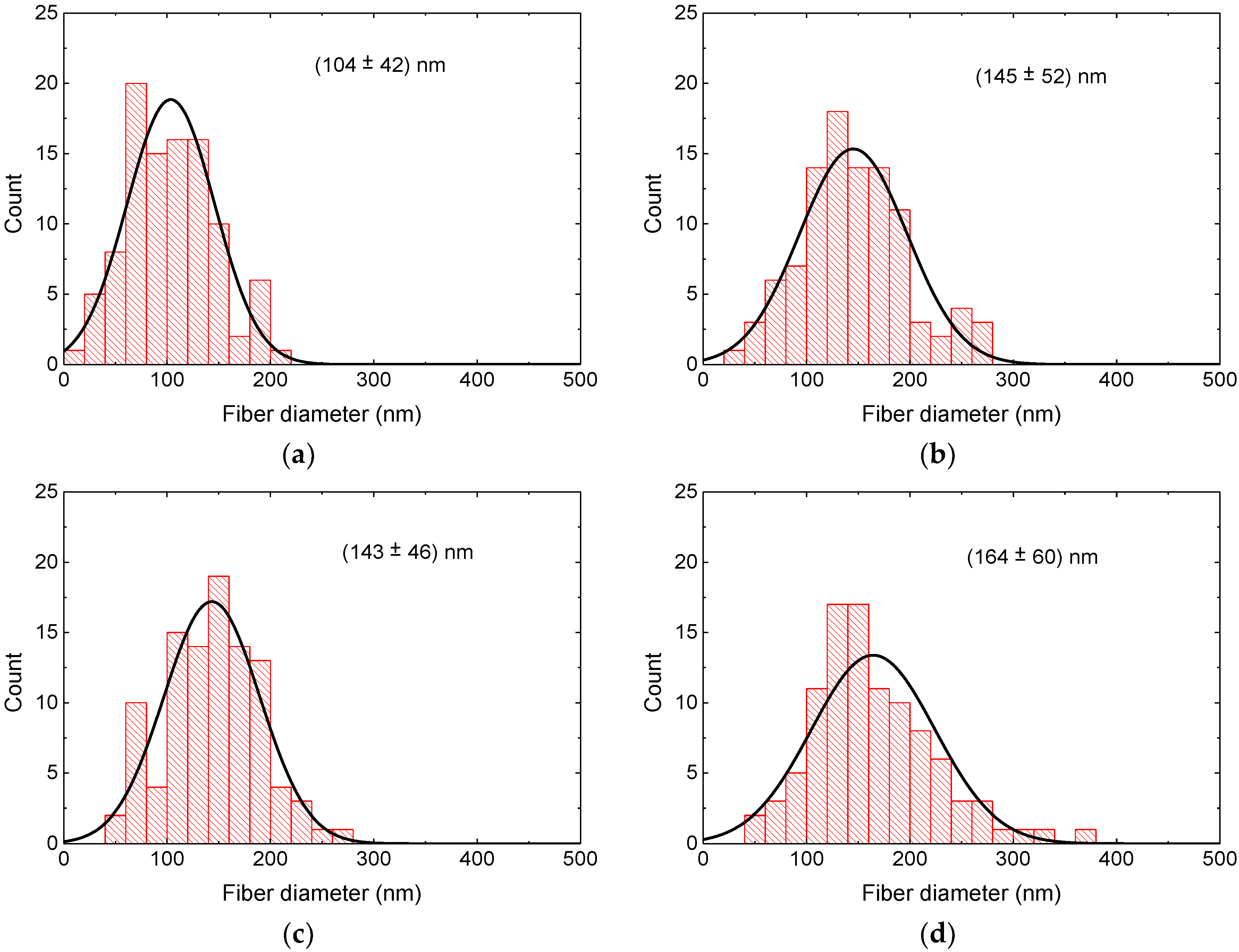
3.1. Morphological Investigations

Figure 2 depicts SEM images of the magnetite samples under examination; Figure 3 shows SEM images of the nickel-ferrite samples. As mentioned in Section 2, magnetic nanofiber mats with beads along the fibers were produced to test the combination of structures for data storage and transport for possible application in neuromorphic computing [18].
It should be mentioned that the aim of our recent study was not optimization of the numbers and dimensions of the beads, but investigation of the magnetic properties of nanofiber mats directly after electrospinning, as well as after stabilization and carbonization at different temperatures. Based on recent results and corresponding micromagnetic simulations, a further morphological optimization of the bead dimensions and quantities will be carried out.
In both nanofiber mats depicted in Figure 2 and Figure 3, the typical increase of the fiber diameter is visible due to relaxation of the internal stress in the nanofibers caused by the severe stretching during electrospinning [77]. Since the nanofiber mats were not mechanically fixed or even actively stretched during stabilization, this behavior can be expected, as discussed in detail in [77].
The diameter distributions of the nanofibers after the different treatments are depicted in Figure 4 and Figure 5. In PAN/magnetite as well as PAN/nickel-ferrite nanofiber mats, stabilization resulted in a larger average as well as in a broader distribution of the nanofiber diameters, the latter especially in case of PAN/magnetite. While the average diameter of PAN/magnetite nanofibers after carbonization at 500 °C was again smaller than after stabilization, both values were nearly identical for PAN/nickel-ferrite. Carbonization at 800 °C resulted in both material blends in the largest diameters.
It should be mentioned, nevertheless, that these differences are not statistically significant due to the broad distributions of the measured fiber diameters and may partly be based on the arbitrary choice of the sample areas under investigation.
Both magnetic nanofiber mats showed the desired beads after electrospinning, with a larger number of relatively large beads in the case of PAN/nickel-ferrite. Future micromagnetic simulations are necessary to investigate which bead sizes are advantageous for data storage or use as switches, as shown in [18]. The fibrous structure of the beads stayed similar after stabilization due to the slow heating process, which was optimized in former studies for pure PAN nanofibers and PAN with integrated metal-oxide nanoparticles [78,79]. After carbonization at 500 °C, however, the beads in the PAN/nickel-ferrite nanofiber mats became smaller, an effect which has to be investigated in detail in the future. At 800 °C, the fiber structure of the PAN/magnetite nanofiber mats changed unexpectedly, which was not the case for PAN/TiO2 nanofiber mats [79] or PAN/gelatin nanofiber mats [80]. While this pure nanofiber structure is of great interest for data transport in magnetic networks, it cannot be used any more for date storage. Similarly, the beads were nearly lost in the PAN/nickel-ferrite nanofiber mat carbonized at 800 °C.
Apparently, it is necessary to either find a compromise between conductivity—which usually increases with increasing carbonization temperature and would support current-driven domain-wall transport—and the beads which could be used for data storage and manipulation. On the other hand, the carbonization parameters used here, especially the heating rate, were optimized for PAN, PAN/gelatin, and PAN/TiO2, and can possibly be further optimized in the cases of PAN/magnetite, and PAN/nickel-ferrite, respectively, to maintain the desired structure.

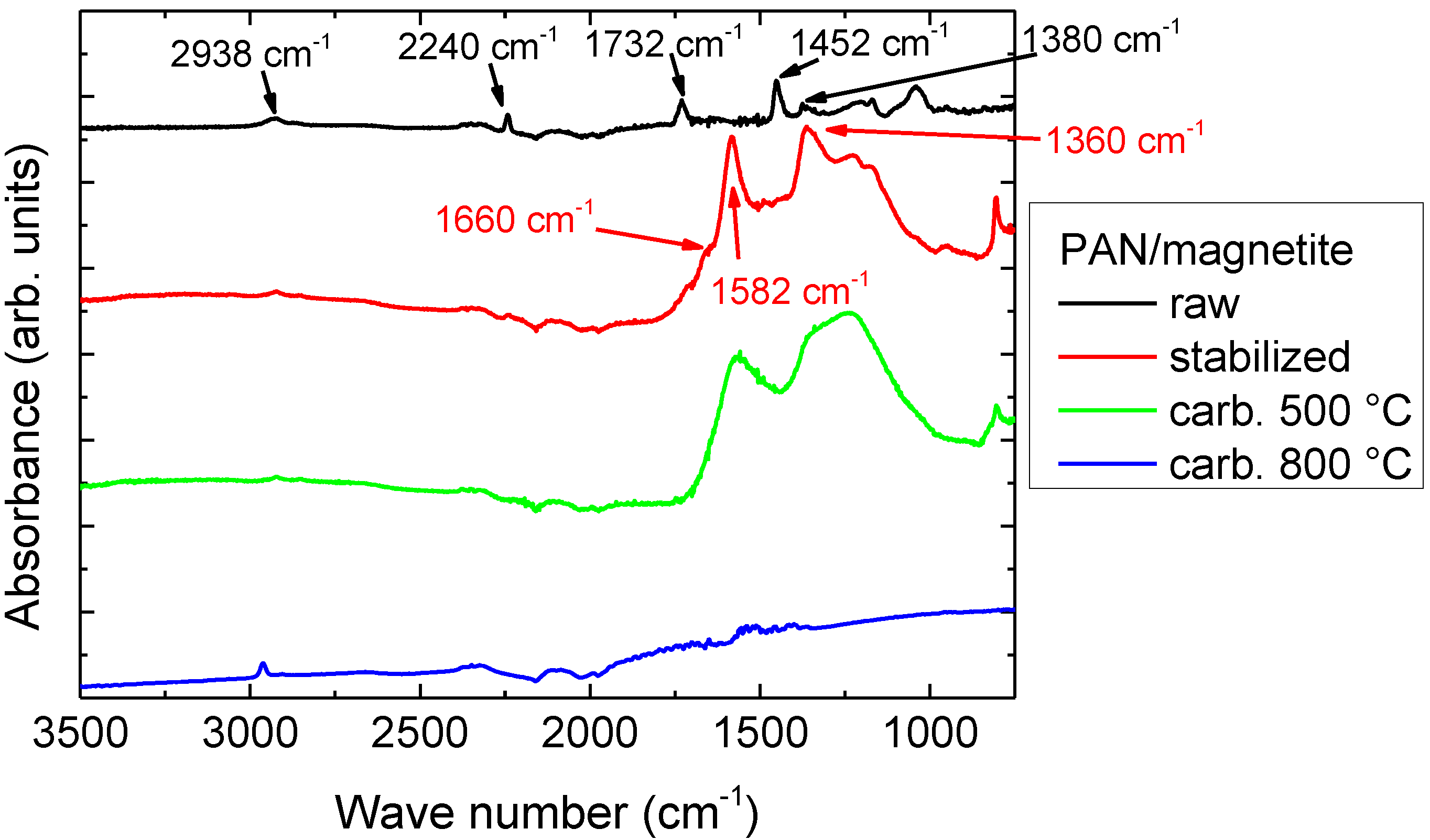
3.2. Chemical Investigations

Next, Figure 6 depicts FTIR spectra of PAN/magnetite samples; spectra measured for PAN/nickel-ferrite are approximately identical and are thus not shown here.
As described in detail in previous studies [78,79,80], the raw PAN/magnetite nanofiber mat was characterized by stretching vibrations of the C≡N nitrile functional group at 2240 cm−1, the carbonyl (C=O) stretching peak at 1732 cm−1, and bending and stretching vibrations of CH2 visible at 2938 cm−1, 1452 cm−1, and 1380 cm−1. In the raw nanofiber mat, no residues of the solvent DMSO were visible, underlining that only very small amounts of DMSO were left in these nanofiber mats.
In the stabilized nanofiber mats, the most prominent peaks were those of C=N stretching vibrations at 1582 cm−1, C=C stretching vibrations at 1660 cm−1, and C-H bending and C-H2 wagging around 1360 cm−1. After carbonization at 800 °C, the peaks nearly fully vanished since few functional groups were left after carbonization, resulting in the typical high absorbance of carbon. Temperature treatment at 500 °C was apparently not sufficient for a full carbonization process; instead, the FTIR spectrum still looked very similar to the stabilized sample.
Comparing the FTIR measurements with those performed on pure PAN nanofiber mats or PAN blends with gelatin, TiO2, etc. [78,79,80], no significant difference was visible due to the embedded magnetic nanoparticles.

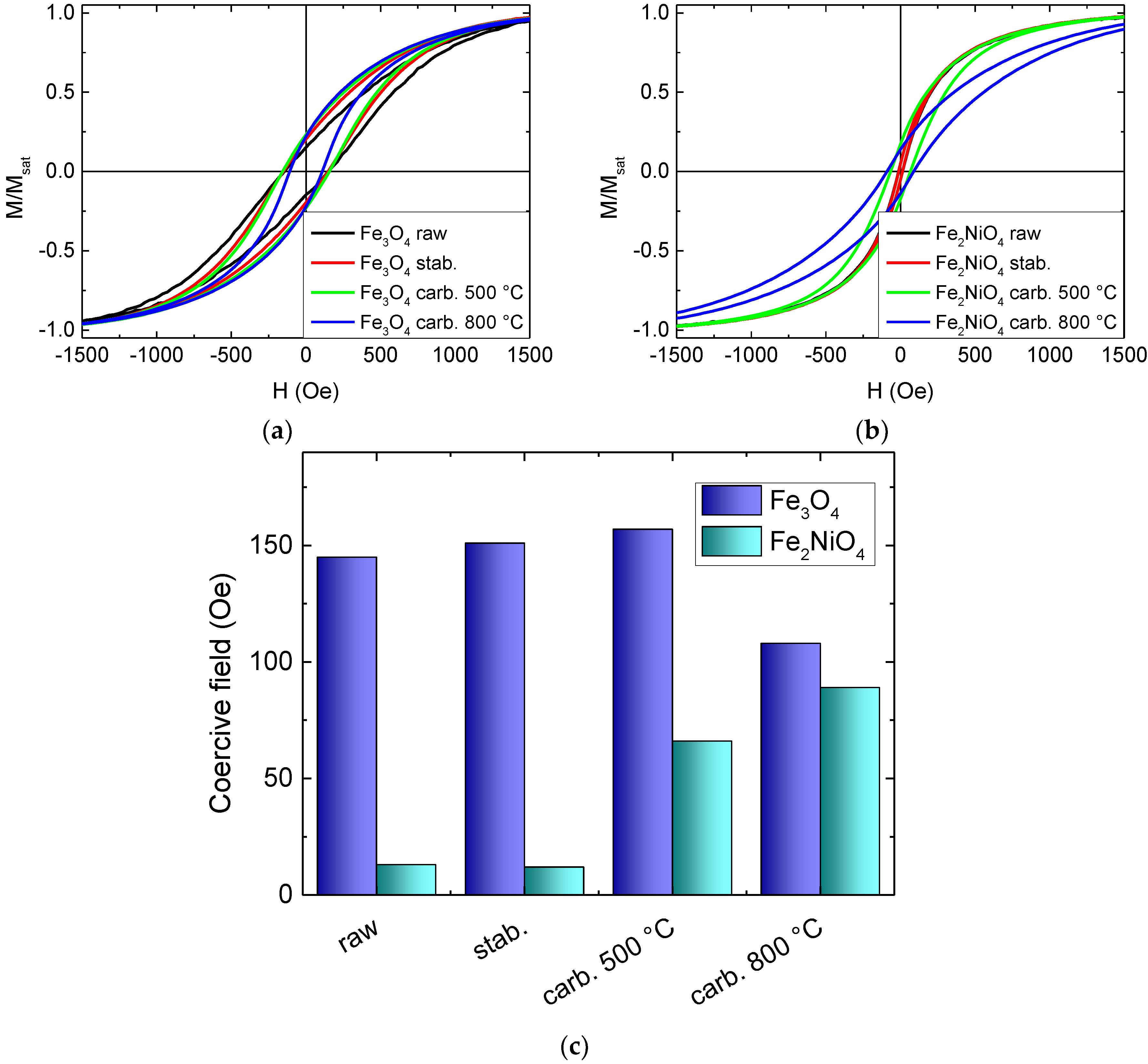
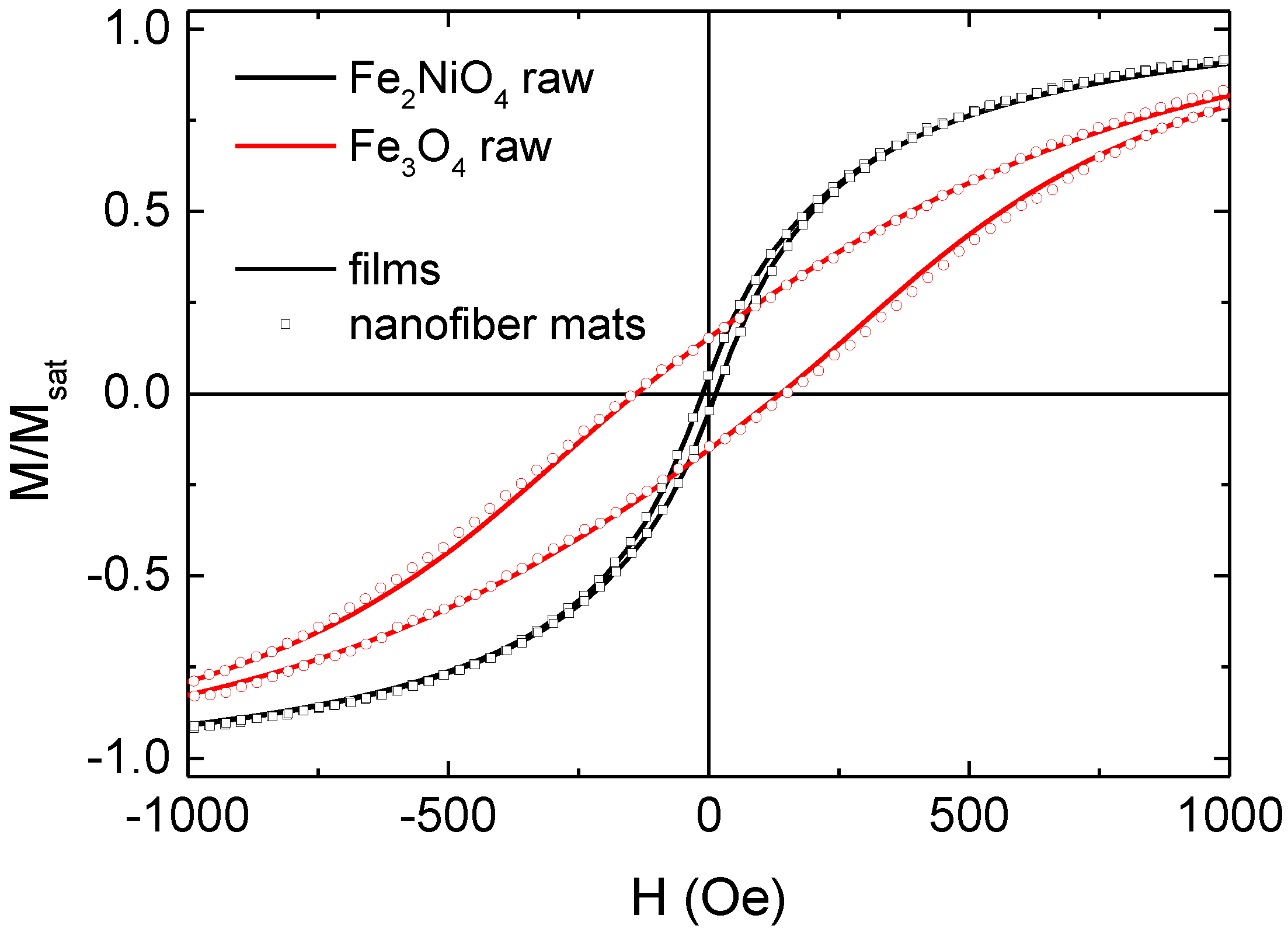
3.3. Magnetic Investigations

The magnetic characteristics of the magnetic nanofibers with magnetite and nickel-ferrite nanoparticles, respectively, are depicted in Figure 7.
Firstly, it is clearly visible that the coercive fields of the magnetite-based nanofibers were significantly larger than those of the nickel-ferrite-based nanofibers. For the PAN/magnetite nanofiber mats, the coercive fields did not change strongly with temperature treatments, while the PAN/nickel-ferrite nanofiber mats showed a soft magnetic behavior with only a very small coercive field at low temperature and much larger coercive fields after carbonization processes. Since both materials have similar magnetic properties, this difference can be attributed to the different nanoparticle dimensions—while the magnetite nanoparticles with diameters of 50–100 nm were large enough to show ferrimagnetic characteristics even without agglomerations, the nickel-ferrite nanoparticles with diameters below 50 nm were partly small enough for superparamagnetic behavior. Only after carbonization, when the nanofibers started forming conglutinations, were the neighboring nickel-ferrite nanoparticles near enough to overcome the superparamagnetic limit.
It must be mentioned that the highest carbonization temperature of 800 °C was above the Curie temperatures of both magnetic materials, which is approx. 570 °C for both bulk samples and 517 °C [81] or only 495 °C [82] for nanocrystalline nickel-ferrite. Similarly, in Fe3O4 nanocrystals, strongly reduced Curie temperatures down to 440 °C were reported [83]. In addition, a cation reordering was reported to occur in magnetite above 427 °C, corresponding to a gradual transformation from disordered to partially ordered configuration of magnetite [84]. These structural and magnetic effects may also have influenced the hysteresis loop shapes, in addition to the strong mass and volume loss; the latter resulting from an overproportioned shortening of the fibers as compared to the diameter increase, depicted in Figure 4 and Figure 5, of the fibers during stabilization and carbonization [78,79,80], which automatically reduced the distances between neighboring nanoparticles and thus influenced their collective magnetic characteristics [85].
Figure 8 shows a comparison of nanofiber mats and thin films, prepared from PAN with both magnetic nanoparticles used in this study. It is clearly visible that the magnetic properties were quite similar for both shapes, indicating that in both cases the nanoparticles were not near enough to each other to be subject to the magnetic shape anisotropy of the nanofibers, but were dominated by the single particle magnetic shape anisotropy. Whether there was any magnetic anisotropy of the particles inside the fibers, induced by electrospinning, has to be examined in future magnetic force microscope (MFM) investigations.

3.4. Micromagnetic Simulations

This finding is underlined by the micromagnetic simulations depicted in Figure 9, showing hysteresis loops averaged over simulations along 0°, 15°, … 90° for magnetite and nickel-ferrite branched and single fibers, in this way simulating the expected results of macroscopic measurements on stochastically distributed pure magnetic nanofibers with or without branches.
The finding that coercive fields of nickel-ferrite nanofibers were generally smaller than those of magnetite nanofibers corresponds to the experimental results. The absolute values, however, were significantly larger in the simulation, underlining that the magnetic nanofibers prepared by electrospinning contained distributed magnetic nanoparticles which showed different magnetization reversal processes than the completely magnetic simulated nanoparticles. Apparently, simulations of stochastically distributed nanoparticle networks in the nanofiber shells are necessary to investigate in more detail the influence of inter-particle distances and the overall fiber shell, building a matrix in which the magnetic nanoparticles are distributed, on static and dynamic magnetization characteristics of the nanofiber network.

4. Conclusions

Magnetic nanofibers were prepared by electrospinning PAN nanofibers filled with magnetite or nickel-ferrite nanoparticles. While raw and stabilized fibers were relatively even and straight, more and more conglutinations between neighboring fibers occurred with increasing carbonization temperature, in case of magnetite leading to a deformation of the fiber shape from round fibers into flat ribbons. The magnetic properties were, especially in case of the smaller nickel-ferrite nanoparticles, strongly influenced by the thermal post-treatments, indicating that magnetization reversal is dominated by single-particle characteristics rather than by clusters of strongly interacting nanoparticles. Comparison with micromagnetic simulations of full-metal oxide nanofibers underlined this finding. It should be mentioned that care must be taken in the evaluation of magnetic properties after heating the nanofiber mats above the Curie temperatures of the magnetic nanoparticles; these thermal influences may have caused modifications of the magnetic properties in addition to the well-known structural changes of the nanoparticles due to a high-temperature treatment.
Future simulations of randomly distributed nanoparticles of different sizes inside a nanofiber matrix are necessary to fully understand the magnetic behavior of electrospun, stabilized, and carbonized magnetic nanofibers. To tailor research according to the necessities of data storage and transport for neuromorphic computing and other novel applications, additional MFM investigations on magnetization reversal and dynamics in these random nanofiber networks are necessary to produce reliable neuromorphic computing systems.
Additionally, on the materials science side, tailoring of the dimensions and quantities of the beads according to the afore-simulated necessities should be carried out, as well as an investigation of the stabilization and carbonization process in more detail, including examination of high-resolution images of the inner structures of the fibers by transmission electron microscopy (TEM). In this study, the optimum stabilization and carbonization parameters for pure electrospun PAN nanofibers were applied; however, it cannot be excluded that due to the embedded metallic nanoparticles, a new optimization study is necessary to avoid the unexpected formation of ribbon-like structures in the PAN/magnetite nanofiber mats after carbonization at 800 °C.

Author Contributions

Conceptualization, A.E.; methodology, N.F., T.G., A.E., T.B., A.H.; validation, A.E., T.B., A.H.; formal analysis, A.E.; investigation, N.F., T.G., A.M., M.T., M.K., L.S., C.D.; writing—original draft preparation, T.B. and A.E.; writing—review and editing, all authors; visualization, A.E. All authors have read and agreed to the published version of the manuscript.

Funding

This research was funded by a Volkswagen Foundation grant “Adaptive Computing with Electrospun Nanofiber Networks” no. 93679, the Erasmus+ program of the European Union, the internal PhD funds of Bielefeld University of Applied Sciences and the internal HiF funds of Bielefeld University of Applied Sciences. The APC is funded by the Open Access Publication Fund of Bielefeld University of Applied Sciences and the Deutsche Forschungsgemeinschaft (DFG, German Research Foundation)–414001623.

Conflicts of Interest

The authors declare no conflict of interest. The funders had no role in the design of the study; in the collection, analyses, or interpretation of data; in the writing of the manuscript, or in the decision to publish the results.

References

  1. Greiner, A.; Wendorff, J.H. Electrospinning: A fascinating method for the preparation of ultrathin fibers. Angew. Chem. Int. Ed. 2007, 46, 5670–5703. [Google Scholar] [CrossRef] [PubMed]
  2. Klinkhammer, K.; Seiler, N.; Grafahrend, D.; Gerardo-Nava, J.; Mey, J.; Brook, G.A.; Möller, M.; Dalton, P.D.; Klee, D. Deposition of electrospun fibers on reactive substrates for in vitro investigations. Tissue Eng. Part C Methods 2009, 15, 77–85. [Google Scholar] [CrossRef] [PubMed]
  3. Grothe, T.; Wehlage, D.; Böhm, T.; Remche, A.; Ehrmann, A. Needleless electrospinning of PAN nanofibre mats. Tekstilec 2017, 60, 290–295. [Google Scholar] [CrossRef]
  4. Che Othman, F.E.; Yusof, N.; Hasbullah, H.; Jaafar, J.; Ismai, A.F.; Abdullah, N.; Nordin, N.A.H.M.; Aziz, F.; Salleh, W.N.W. Polyacrylonitrile/magnesium oxide-based activated carbon nanofibers with well-developed microporous structure and their adsorption performance for methane. J. Ind. Eng. Chem. 2017, 51, 281–287. [Google Scholar] [CrossRef]
  5. García-Mateo, F.J.; Cordero-Lanzac, T.; Berenguer, R.; Morallón, E.; Cazorla-Amorós, D.; Rodríguez-Mirasol, J.; Cordero, T. Lignin-derived Pt supported carbon (submicron)fiber electrocatalysts for alcohol electro-oxidation. Appl. Catal. B Environ. 2017, 211, 18–30. [Google Scholar] [CrossRef]
  6. Yoshida, H.; Sakuragi, K. Elicitation of crystallinity in cyclodextrin electrospinning. Bull. Chem. Soc. Jpn. 2019, 92, 927–929. [Google Scholar] [CrossRef]
  7. Yalcinkaya, F.; Boyraz, E.; Maryska, J.; Kucerova, K. A review on membrane technology and chemical surface modification for the oily wastewater treatment. Materials 2020, 13, 493. [Google Scholar] [CrossRef] [Green Version]
  8. Kozior, T.; Trabelsi, M.; Mamun, A.; Sabantina, L.; Ehrmann, A. Stabilization of electrospun nanofiber mats used for filters by 3D printing. Polymers 2019, 11, 1618. [Google Scholar] [CrossRef] [Green Version]
  9. Boyraz, E.; Yalcinkaya, F.; Hruza, J.; Maryska, J. Surface-modified nanofibrous PVDF membranes for liquid separation technology. Materials 2019, 12, 2702. [Google Scholar] [CrossRef] [Green Version]
  10. Mamun, A. Review of possible applications of nanofibrous mats for wound dressings. Tekstilec 2019, 62, 89–100. [Google Scholar] [CrossRef]
  11. Gao, S.T.; Tang, G.S.; Hua, D.W.; Xiong, R.; Han, J.; Jiang, S.; Zhang, Q.; Huang, C. Stimuli-responsive bio-based polymeric systems and their applications. J. Mater. Chem. B 2019, 7, 709–729. [Google Scholar] [CrossRef]
  12. Wehlage, D.; Blattner, H.; Mamun, A.; Kutzli, I.; Diestelhorst, E.; Rattenholl, A.; Gudermann, F.; Lütkemeyer, D.; Ehrmann, A. Cell growth on electrospun nanofiber mats from polyacrylonitrile (PAN) blends. Aims Bioeng. 2020, 7, 43–54. [Google Scholar] [CrossRef]
  13. Xue, Y.Y.; Guo, X.; Zhou, H.F.; Zhou, J. Influence of beads-on-string on Na-Ion storage behavior in electrospun carbon nanofibers. Carbon 2019, 154, 219–229. [Google Scholar] [CrossRef]
  14. Kohn, S.; Wehlage, D.; Juhász Junger, I.; Ehrmann, A. Electrospinning a dye-sensitized solar cell. Catalysts 2019, 9, 975. [Google Scholar] [CrossRef] [Green Version]
  15. Xue, J.J.; Wu, T.; Dai, Y.Q.; Xia, Y.N. Electrospinning and electrospun nanofibers: Methods, materials, and applications. Chem. Rev. 2019, 119, 5298–5415. [Google Scholar] [CrossRef]
  16. Peer, P.; Stenicka, M.; Filip, P.; Pizurova, N.; Babayan, V. Magnetorheological characterization and electrospinnability of ultrasound-treated polymer solutions containing magnetic nanoparticles. Colloid Polym. Sci. 2018, 296, 1849–1855. [Google Scholar] [CrossRef]
  17. Darwish, M.S.A.; Bakry, A.; Kolek, O.; Martinova, L.; Stibor, I. Electrospun functionalized magnetic polyamide 6 composite nanofiber: Fabrication and stabilization. Polym. Compos. 2019, 40, 296–303. [Google Scholar] [CrossRef]
  18. Döpke, C.; Grothe, T.; Steblinski, P.; Klöcker, M.; Sabantina, L.; Kosmalska, D.; Blachowicz, T.; Ehrmann, A. Magnetic nanofiber mats for data storage and transfer. Nanomaterials 2019, 9, 92. [Google Scholar] [CrossRef] [Green Version]
  19. Murillo-Ortíz, R.; Mirabal-García, M.; Martínez-Huerta, J.M.; Cabal Velarde, J.G.; Castaneda-Robles, I.E.; Lobo-Guerrero, A. Analysis of the magnetic properties in hard-magnetic nanofiber composite. J. Appl. Phys. 2018, 123, 105108. [Google Scholar] [CrossRef]
  20. Ghazi, N.; Chenari, H.M.; Ghodsi, F.E. Rietveld refinement, morphology analysis, optical and magnetic properties of magnesium-zinc ferrite nanofibers. J. Magn. Magn. Mater. 2018, 468, 132–140. [Google Scholar] [CrossRef]
  21. Blachowicz, T.; Ehrmann, A. Most recent developments in electrospun magnetic nanofibers: A review. J. Eng. Fibers Fabr. 2020, 15, 1558925019900843. [Google Scholar] [CrossRef]
  22. Lin, K.-Y.A.; Yang, M.-T.; Lin, J.-T.; Du, Y.C. Cobalt ferrite nanoparticles supported on electrospun carbon fiber as a magnetic heterogeneous catalyst for activating peroxymonosulfate. Chemosphere 2018, 208, 502–511. [Google Scholar] [CrossRef] [PubMed]
  23. Matos, R.J.R.; Chaparro, C.I.P.; Silva, J.C.; Valente, M.A.; Borges, J.P.; Soares, P.I.P. Electrospun composite cellulose acetate/iron oxide nanoparticles non-woven membranes for magnetic hyperthermia applications. Carbohydr. Polym. 2018, 198, 9–16. [Google Scholar] [CrossRef]
  24. Zhan, Y.Q.; Long, Z.H.; Wan, X.Y.; Zhang, J.M.; He, S.J.; He, Y. 3D carbon fiber mats/nano-Fe3O4 hybrid material with high electromagnetic shielding performance. Appl. Surf. Sci. 2018, 444, 710–720. [Google Scholar] [CrossRef]
  25. Li, X.R.; Song, G.J.; Ma, L.C.; Peng, Q.H.; Li, H.Y.; Ji, Z.J.; Cong, H.L. Structural and Magnetic Properties of Rare Earth Doped Multilayer Nanocable Arrays. J. Nanosci. Nanotechnol. 2020, 20, 1873–1877. [Google Scholar] [CrossRef] [PubMed]
  26. Agarwal, S.; Pohl, D.; Patra, A.K.; Nielsch, K.; Khatri, M.S. Preparation and nanoscale characterization of electrodeposited CoFe-Cu multilayer nanowires. Mater. Chem. Phys. 2019, 230, 231–238. [Google Scholar] [CrossRef]
  27. Yu, Q.L.; Zhang, Y.M.; Liu, Y.H.; Liu, Y. Magnetic supramolecular nanofibers of gold nanorods for photothermal therapy. Adv. Ther. 2019, 2, 1800137. [Google Scholar] [CrossRef]
  28. Mahajan, C.G.; Alfadhel, A.; Irving, M.; Kahn, B.E.; Borkholder, D.A.; Williams, S.A.; Cormier, D. Magnetic Field Patterning of Nickel Nanowire Film Realized by Printed Precursor Inks. Materials 2019, 12, 928. [Google Scholar] [CrossRef] [Green Version]
  29. Yu, Y.L.; Li, J.P.; Wang, J.; Wu, X.G.; Yu, C.Y.; Xu, T.; Chang, B.D.; Sun, H.Y.; Arandiyan, H. Orientation Growth and Magnetic Properties of Electrochemical Deposited Nickel Nanowire Arrays. Catalysts 2019, 9, 152. [Google Scholar] [CrossRef] [Green Version]
  30. Dolbashian, C.; Chavez, B.L.; Bauer, M.; Budi, M.; Andrew, J.S.; Crawford, T.M. Magnetic properties of aligned multiferroic Janus nanofiber agglomerates measured with the scattered magneto-optical Kerr effect. J. Phys. D Appl. Phys. 2020, 53, 195002. [Google Scholar] [CrossRef]
  31. Mei, L.Y.; Chen, H.Y.; Shao, Y.P.; Wang, J.Y.; Liu, Y.Q. Highly aligned magnetic composite nanofibers fabricated by magnetic-field-assisted electrospinning PAN/FeCo solution. High Perform. Polym. 2019, 31, 230–237. [Google Scholar] [CrossRef]
  32. Cai, N.; Chen, M.; Liu, M.M.; Wang, J.Z.; Shen, L.; Wang, J.Y.; Feng, X.J.; Yu, F.Q. Meso-microporous carbon nanofibers with in-situ embedded Co nanoparticles for catalytic oxidization of azo dyes. J. Mol. Liq. 2019, 289, 111060. [Google Scholar] [CrossRef]
  33. Erfan, N.A.; Barakat, N.; Müller-Borer, B.J. Preparation and characterization of beta-lactoglobulin/poly (ethylene oxide) magnetic nanofibers for biomedical applications. Colloids Surf. Physicochem. Eng. Asp. 2019, 576, 63–72. [Google Scholar] [CrossRef]
  34. Jiang, P.; Lu, J.F.; Li, K.; Chen, X.Q.; Dan, R.Q. Research on hydrophobicity of electrospun Fe3O4/PVDF nanofiber membranes under different preparation conditions. Fuller. Nanotub. Carbon Nanostructures 2020, 28, 381–386. [Google Scholar] [CrossRef]
  35. Roche, R.; Yalcinkaya, F. Incorporation of PVDF nanofibre multilayers into functional structure for filtration applications. Nanomaterials 2018, 8, 771. [Google Scholar] [CrossRef] [Green Version]
  36. Kurecic, M.; Smole, M.S. Electrospinning: Nanofibre production method. Tekstilec 2013, 56, 4–12. [Google Scholar] [CrossRef]
  37. Storck, J.L.; Grothe, T.; Mamun, A.; Sabantina, L.; Klöcker, M.; Blachowicz, T.; Ehrmann, A. Orientation of electrospun magnetic nanofibers near conductive areas. Materials 2020, 13, 47. [Google Scholar] [CrossRef] [Green Version]
  38. Blachowicz, T.; Ehrmann, A. Magnetization reversal in bent nanofibers of different cross sections. J. Appl. Phys. 2018, 124, 152112. [Google Scholar] [CrossRef] [Green Version]
  39. Gaididei, Y.; Goussev, A.; Kravchuk, V.P.; Pylypovskyi, O.V.; Robbins, J.M.; Sheka, D.D.; Slastikov, V.; Vasylkevych, S. Magnetization in narrow ribbons: Curvature effects. J. Phys. Math. Theor. 2017, 50, 385401. [Google Scholar] [CrossRef] [Green Version]
  40. Moreno, R.; Carvalho-Santos, V.L.; Espejo, A.P.; Laroze, D.; Chubykalo-Fesenko, O.; Altbir, D. Oscillatory behavior of the domain wall dynamics in a curved cylindrical magnetic nanowire. Phys. Rev. B 2017, 96, 184401. [Google Scholar] [CrossRef] [Green Version]
  41. Parkin, S.S.P.; Hayashi, M.; Thomas, L. Magnetic domain-wall racetrack memory. Science 2008, 320, 190–194. [Google Scholar] [CrossRef] [PubMed]
  42. Grollier, J.; Querlioz, D.; Stiles, M.D. Spintronic Nanodevices for Bioinspired Computing. Proc. IEEE 2016, 104, 2024–2039. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  43. LeCun, Y.; Bengio, Y.; Hinton, G. Deep learning. Nature 2015, 521, 7553. [Google Scholar] [CrossRef] [PubMed]
  44. Hinton, G.E.; Salakhutdinov, R.R. Reducing the dimensionality of data with neural networks. Science 2006, 313, 504–507. [Google Scholar] [CrossRef] [Green Version]
  45. Masquelier, T.; Thorpe, S.J. Unsupervised learning of visual features through spike timing dependent plasticity. PLoS Comput. Biol. 2007, 3, e31. [Google Scholar] [CrossRef]
  46. Indiveri, G.; Liu, S.C. Memory and information processing in neuromorphic systems. Proc. IEEE 2015, 103, 1379–1397. [Google Scholar] [CrossRef] [Green Version]
  47. Querlioz, D.; Bichler, O.; Vincent, A.F.; Gamrat, C. Bioinspired programming of memory devices for implementing an inference engine. Proc. IEEE 2015, 103, 1398–1416. [Google Scholar] [CrossRef] [Green Version]
  48. Meier, K. A mixed-signal universal neuromorphic computing system. In Proceedings of the 2015 IEEE International Electron Devices Meeting (IEDM), Washington, DC, USA, 7–9 December 2015. [Google Scholar]
  49. Merolla, P.A.; Arthur, J.V.; Alvarez-Icaza, R.; Cassidy, A.S.; Sawada, J.; Akopyan, F.; Jackson, B.L.; Imam, N.; Guo, C.; Nakamura, Y.; et al. A million spiking-neuron integrated circuit with a scalable communication network and interface. Science 2014, 345, 668–673. [Google Scholar] [CrossRef]
  50. Ryu, K.-S.; Thomas, L.; Yang, S.-H.; Parkin, S.S.P. Current induced tilting of domain walls in high velocity motion along perpendicularly magnetized micron-sized Co/Ni/Co racetracks. Appl. Phys. Express 2012, 5, 093006. [Google Scholar] [CrossRef]
  51. Yang, S.-H.; Ryu, K.-S.; Parkin, S.S.P. Domain-wall velocities of up to 750 m s−1 driven by exchange-coupling torque in synthetic antiferromagnets. Nat. Nanotechnol. 2015, 10, 221–226. [Google Scholar] [CrossRef]
  52. Alejos, O.; Raposo, V.; Tejerina, L.S.; Martinez, E. Efficient and controlled domain wall nucleation for magnetic shift registers. Sci. Rep. 2017, 7, 11909. [Google Scholar] [CrossRef] [Green Version]
  53. Liu, L.Q.; Lee, O.J.; Gudmundsen, T.J.; Ralph, D.C.; Buhrman, R.A. Current-Induced Switching of Perpendicularly Magnetized Magnetic Layers Using Spin Torque from the Spin Hall Effect. Phys. Rev. Lett. 2012, 109, 096602. [Google Scholar] [CrossRef] [Green Version]
  54. Liu, L.; Pai, C.-F.; Li, Y.; Tseng, H.W.; Ralph, D.C.; Buhrman, R.A. Spin-torque switching with the giant spin Hall effect of tantalum. Science 2012, 336, 555–558. [Google Scholar] [CrossRef] [Green Version]
  55. Martinez, E.; Torres, L.; Perez, N.; Hernandez, M.A.; Raposo, V.; Moretti, S. Universal chiral-triggered magnetization switching in confined nanodots. Sci. Rep. 2015, 5, 10156. [Google Scholar] [CrossRef]
  56. Allwood, D.A.; Xiong, G.; Cowburn, R.P. Domain wall cloning in magnetic nanowires. J. Appl. Phys. 2007, 101, 024308. [Google Scholar] [CrossRef]
  57. Yao, P.; Wu, H.; Gao, B.; Tang, J.S.; Zhang, Q.T.; Zhang, W.Q.; Yang, J.J.; Qian, H. Fully hardware-implemented memristor convolutional neural network. Nature 2020, 577, 641–646. [Google Scholar] [CrossRef]
  58. Lequeux, S.; Sampaio, J.; Cros, V.; Yakushiji, K.; Fukushima, A.; Matsumoto, R.; Kubota, H.; Yuasa, S.; Grollier, J. A magnetic synapse: Multilevel spin-torque memristor with perpendicular anisotropy. Sci. Rep. 2016, 6, 31510. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  59. Sabantina, L.; Rodríguez Mirasol, J.; Cordero, T.; Finsterbusch, K.; Ehrmann, A. Investigation of needleless electrospun PAN nanofiber mats. Secunderabad, India, Dec. 22–23, 2017. AIP Conf. Proc. 2018, 1952, 020085. [Google Scholar]
  60. Ehrmann, A.; Blachowicz, T. Vortex and double-vortex nucleation during magnetization reversal in Fe nanodots of different dimensions. J. Magn. Magn. Mater. 2019, 475, 727–733. [Google Scholar] [CrossRef]
  61. Kukuchi, N.; Okamoto, S.; Kitakami, O.; Shimada, Y.; Kim, S.G.; Otani, Y.; Fukamichi, K. Vertical bistable switching of spin vortex in a circular magnetic dot. J. Appl. Phys. 2001, 90, 6548. [Google Scholar] [CrossRef]
  62. Van Waeyenberge, B.; Puzic, A.; Stoll, H.; Chou, K.W.; Tyliszczak, T.; Hertel, R.; Fähnle, M.; Brückl, H.; Rott, K.; Reiss, G.; et al. Magnetic vortex core reversal by excitation with short bursts of an alternating field. Nature 2006, 444, 461–464. [Google Scholar] [CrossRef] [PubMed]
  63. Weigand, M.; van Waeyenberge, B.; Vanseenkiste, A.; Curcic, M.; Sackmann, V.; Stoll, H.; Tyliszczak, T.; Kaznatcheev, K.; Woltersdorf, G.; Back, C.H.; et al. Vortex Core Switching by Coherent Excitation with Single In-Plane Magnetic Field Pulses. Phys. Rev. Lett. 2009, 102, 077201. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  64. Arbab, S.; Teimoury, A.; Mirbaha, H.; Adolphe, D.C.; Noroozi, B.; Nourpanah, P. Optimum stabilization processing parameters for polyacrylonitrile-based carbon nanofibers and their difference with carbon (micro) fibers. Polym. Degrad. Stab. 2017, 142, 198–208. [Google Scholar] [CrossRef]
  65. Wei, Y.; Han, B.; Hu, X.Y.; Lin, Y.H.; Wang, X.Z.; Deng, X.L. Synthesis of Fe3O4 nanoparticles and their magnetic properties. Proc. Eng. 2012, 27, 632–637. [Google Scholar] [CrossRef] [Green Version]
  66. Duque, J.G.S.; Souza, E.A.; Meneses, C.T.; Kuobta, L. Magnetic properties of NiFe2O4 nanoparticles produced by a new chemical method. Phys. B 2007, 398, 287–290. [Google Scholar] [CrossRef]
  67. Donahue, M.J.; Porter, D.G. OOMMF User’s Guide, Version 1.0; Interagency Report NISTIR 6376; National Institute of Standards and Technology: Gaithersburg, MD, USA, 1999.
  68. Gilbert, T.L. A phenomenological theory of damping in ferromagnetic materials. IEEE Trans. Magn. 2004, 40, 3443. [Google Scholar] [CrossRef]
  69. Dantas, C.C.; Garna, A.M. Micromagnetic simulations of spinel ferrite particles. J. Magn. Magn. Mater. 2010, 322, 2824–2833. [Google Scholar] [CrossRef] [Green Version]
  70. Kamble, R.B.; Varade, V.; Ramesh, K.P.; Prasad, V. Domain size correlated magnetic properties and electrical impedance of size dependent nickel ferrite nanoparticles. AIP Adv. 2015, 5, 017119. [Google Scholar] [CrossRef]
  71. Mirgorod, Y.A.; Borshch, N.A.; Fedosyuk, V.M.; Yurkov, G.Y. Magnetic properties of nickel ferrite nanoparticles prepared using flotation extraction. Inorg. Mater. 2013, 49, 109–113. [Google Scholar] [CrossRef]
  72. Zhao, S.F.; Sun, Q.; Wang, R.M.; Han, Y.N. Growth and micromagnetic simulation of magnetite nanoparticles. Sci. China 2011, 54, 1208–1212. [Google Scholar] [CrossRef]
  73. Yani, A.; Kurniawan, C.; Djuhana, D. Investigation of the ground state domain structure transition on magnetite (Fe3O4). Univ. Indonesia, Bali, Indonesia. AIP Conf. Proc. 2018, 2023, 020020-1. [Google Scholar]
  74. Blachowicz, T.; Kosmalska, D.; Döpke, C.; Leiste, H.; Hahn, L.; Ehrmann, A. Varying steps in hysteresis loops of Co square nano-frames. J. Magn. Magn. Mater. 2019, 491, 165619. [Google Scholar] [CrossRef]
  75. Sudsom, D.; Juhász Junger, I.; Döpke, C.; Blachowicz, T.; Hahn, L.; Ehrmann, A. Micromagnetic simulation of vortex development in magnetic bi-material bow-tie structures. Condens. Matter 2020, 5, 5. [Google Scholar] [CrossRef] [Green Version]
  76. Blachowicz, T.; Döpke, C.; Ehrmann, A. Micromagnetic simulations of electrospun nanofiber networks. Phys. Rev. B 2020. submitted. [Google Scholar]
  77. Sabantina, L.; Rodríguez-Cano, M.Á.; Klöcker, M.; García-Mateos, F.J.; Ternero-Hidalgo, J.J.; Mamun, A.; Beermann, F.; Schwakenberg, M.; Voigt, A.-L.; Rodríguez Mirasol, J.; et al. Fixing PAN nanofiber mats during stabilization for carbonization and creating novel metal/carbon composites. Polymers 2018, 10, 735. [Google Scholar] [CrossRef] [Green Version]
  78. Sabantina, L.; Klöcker, M.; Wortmann, M.; Rodríguez-Mirasol, J.; Cordero, T.; Moritzer, E.; Finsterbusch, K.; Ehrmann, A. Stabilization of polyacrylonitrile nanofiber mats obtained by needleless electrospinning using dimethyl sulfoxide as solvent. J. Ind. Text. 2019. online first. [Google Scholar] [CrossRef]
  79. Sabantina, L.; Böttjer, R.; Wehlage, D.; Grothe, T.; Klöcker, M.; García-Mateos, F.J.; Rodríguez-Mirasol, J.; Cordero, T.; Ehrmann, A. Morphological study of stabilization and carbonization of polyacrylonitrile/TiO2 nanofiber mats. J. Eng. Fibers Fabr. 2019, 14, 1558925019862242. [Google Scholar] [CrossRef]
  80. Sabantina, L.; Wehlage, D.; Klöcker, M.; Mamun, A.; Grothe, T.; García-Mateos, F.J.; Rodríguez-Mirasol, J.; Cordero, T.; Finsterbusch, K.; Ehrmann, A. Stabilization of electrospun PAN/gelatin nanofiber mats for carbonization. J. Nanomater. 2018, 2018, 6131085. [Google Scholar] [CrossRef] [Green Version]
  81. Nabiyouni, G.; Fesharaki, M.J.; Mozafari, M.; Amighian, J. Characterization and magnetic properties of nickel ferrite nanoparticles prepared by ball milling technique. Chin. Phys. Lett. 2010, 27, 126401. [Google Scholar] [CrossRef]
  82. Iqbal, Y.; Bae, H.S.; Rhee, I.; Hong, S.W. Control of the saturation temperature in magnetic heating by using polyethylene-glycol-coated rod-shaped nickel-ferrite (NiFe2O4) nanoparticles. J. Korean Phys. Soc. 2016, 68, 587–592. [Google Scholar] [CrossRef]
  83. Manohar, A.; Krishnamoorthi, C. Low Curie-transition temperature and superparamagnetic nature of Fe3O4 nanoparticles prepared by colloidal nanocrystal synthesis. Mater. Chem. Phys. 2017, 192, 235–243. [Google Scholar]
  84. Levy, D.; Giustetto, R.; Hoser, A. Structure of magnetite (Fe3O4) above the Curie temperature: A cation ordering study. Phys. Chem. Miner. 2012, 39, 169–176. [Google Scholar] [CrossRef] [Green Version]
  85. Ehrmann, A.; Blachowicz, T. Influence of the distance between nanoparticles in clusters on the magnetization reversal process. J. Nanomater. 2017, 2017, 5046076. [Google Scholar] [CrossRef] [Green Version]
Materials 13 01552 g001 550
Figure 1. Simulated structures of dimensions 800 nm × 800 nm: (a) branched fiber; (b) single fiber.
Figure 1. Simulated structures of dimensions 800 nm × 800 nm: (a) branched fiber; (b) single fiber.
Materials 13 01552 g001
Materials 13 01552 g002 550
Figure 2. SEM images of polyacrylonitrile (PAN)/magnetite nanofiber mats: (a) after electrospinning; (b) after stabilization; (c) after carbonization at 500 °C; (d) after carbonization at 800 °C.
Figure 2. SEM images of polyacrylonitrile (PAN)/magnetite nanofiber mats: (a) after electrospinning; (b) after stabilization; (c) after carbonization at 500 °C; (d) after carbonization at 800 °C.
Materials 13 01552 g002
Materials 13 01552 g003 550
Figure 3. SEM images of PAN/nickel-ferrite nanofiber mats: (a) after electrospinning; (b) after stabilization; (c) after carbonization at 500 °C; (d) after carbonization at 800 °C.
Figure 3. SEM images of PAN/nickel-ferrite nanofiber mats: (a) after electrospinning; (b) after stabilization; (c) after carbonization at 500 °C; (d) after carbonization at 800 °C.
Materials 13 01552 g003
Materials 13 01552 g004 550
Figure 4. Distributions of the diameters of PAN/magnetite nanofibers: (a) after electrospinning; (b) after stabilization; (c) after carbonization at 500 °C; (d) after carbonization at 800 °C.
Figure 4. Distributions of the diameters of PAN/magnetite nanofibers: (a) after electrospinning; (b) after stabilization; (c) after carbonization at 500 °C; (d) after carbonization at 800 °C.
Materials 13 01552 g004
Materials 13 01552 g005 550
Figure 5. Distributions of the diameters of PAN/nickel-ferrite nanofibers: (a) after electrospinning; (b) after stabilization; (c) after carbonization at 500 °C; (d) after carbonization at 800 °C.
Figure 5. Distributions of the diameters of PAN/nickel-ferrite nanofibers: (a) after electrospinning; (b) after stabilization; (c) after carbonization at 500 °C; (d) after carbonization at 800 °C.
Materials 13 01552 g005
Materials 13 01552 g006 550
Figure 6. FTIR measurements of PAN/magnetite samples after electrospinning, stabilization, and carbonization at 500 and 800 °C. The lines are vertically offset for clarity.
Figure 6. FTIR measurements of PAN/magnetite samples after electrospinning, stabilization, and carbonization at 500 and 800 °C. The lines are vertically offset for clarity.
Materials 13 01552 g006
Materials 13 01552 g007 550
Figure 7. Alternating gradient magnetometer (AGM) measurements of hysteresis loops, performed on magnetic nanofibers including nanoparticles from (a) magnetite; (b) nickel-ferrite; and (c) coercive fields measured on these samples.
Figure 7. Alternating gradient magnetometer (AGM) measurements of hysteresis loops, performed on magnetic nanofibers including nanoparticles from (a) magnetite; (b) nickel-ferrite; and (c) coercive fields measured on these samples.
Materials 13 01552 g007
Materials 13 01552 g008 550
Figure 8. Hysteresis loops of as-prepared nanofiber mats and films from PAN with magnetite or nickel-ferrite nanoparticles, respectively.
Figure 8. Hysteresis loops of as-prepared nanofiber mats and films from PAN with magnetite or nickel-ferrite nanoparticles, respectively.
Materials 13 01552 g008
Materials 13 01552 g009 550
Figure 9. Simulated hysteresis loops of (a) magnetite branched fiber; (b) magnetite single fiber; (c) nickel-ferrite branched fiber; (d) nickel-ferrite single fiber.
Figure 9. Simulated hysteresis loops of (a) magnetite branched fiber; (b) magnetite single fiber; (c) nickel-ferrite branched fiber; (d) nickel-ferrite single fiber.
Materials 13 01552 g009

Share and Cite

MDPI and ACS Style

Fokin, N.; Grothe, T.; Mamun, A.; Trabelsi, M.; Klöcker, M.; Sabantina, L.; Döpke, C.; Blachowicz, T.; Hütten, A.; Ehrmann, A. Magnetic Properties of Electrospun Magnetic Nanofiber Mats after Stabilization and Carbonization. Materials 2020, 13, 1552. https://doi.org/10.3390/ma13071552

AMA Style

Fokin N, Grothe T, Mamun A, Trabelsi M, Klöcker M, Sabantina L, Döpke C, Blachowicz T, Hütten A, Ehrmann A. Magnetic Properties of Electrospun Magnetic Nanofiber Mats after Stabilization and Carbonization. Materials. 2020; 13(7):1552. https://doi.org/10.3390/ma13071552

Chicago/Turabian Style

Fokin, Nadine, Timo Grothe, Al Mamun, Marah Trabelsi, Michaela Klöcker, Lilia Sabantina, Christoph Döpke, Tomasz Blachowicz, Andreas Hütten, and Andrea Ehrmann. 2020. "Magnetic Properties of Electrospun Magnetic Nanofiber Mats after Stabilization and Carbonization" Materials 13, no. 7: 1552. https://doi.org/10.3390/ma13071552

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop