Pneumatically-Actuated Acoustic Metamaterials Based on Helmholtz Resonators
Abstract
1. Introduction
2. Materials and Methods
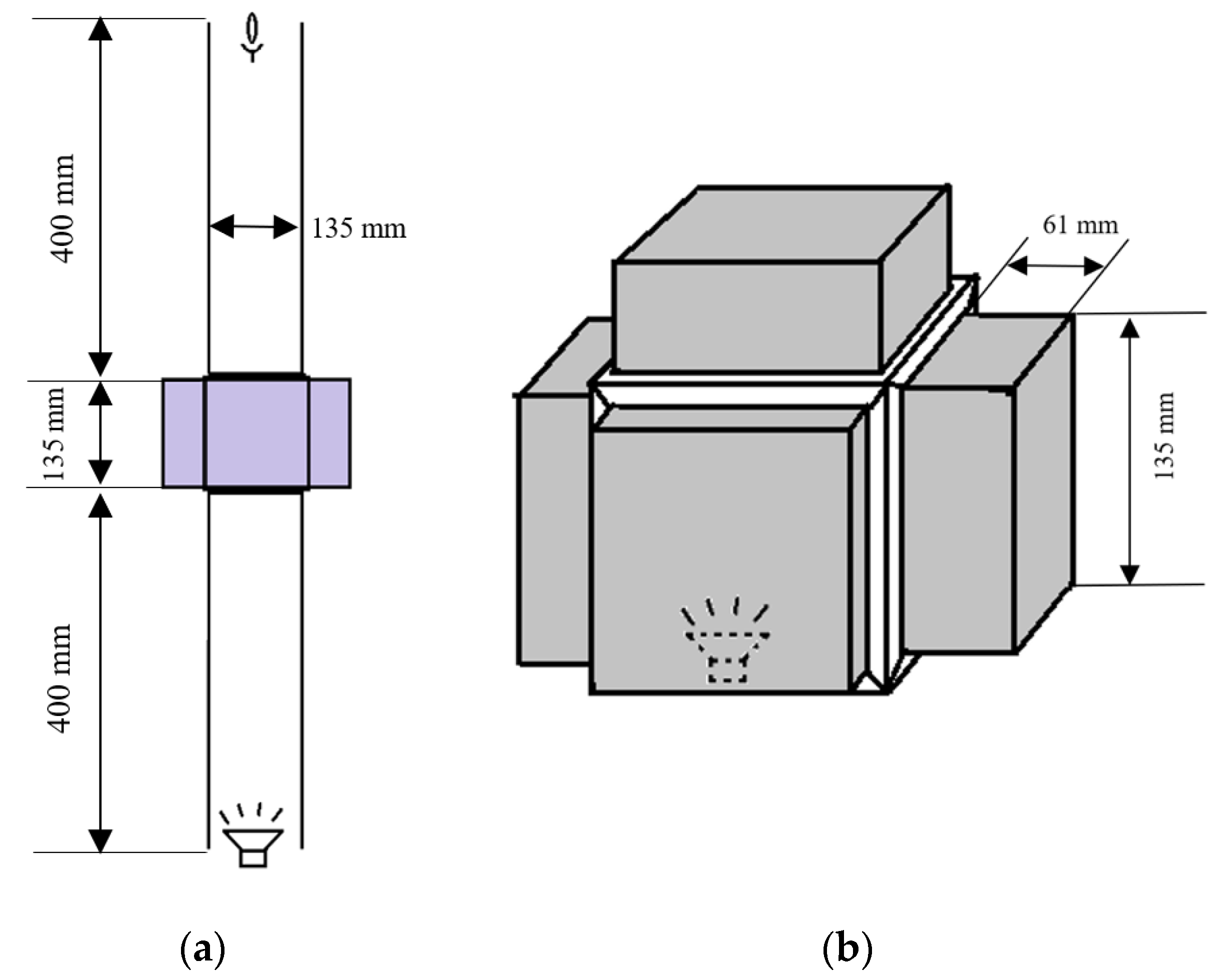
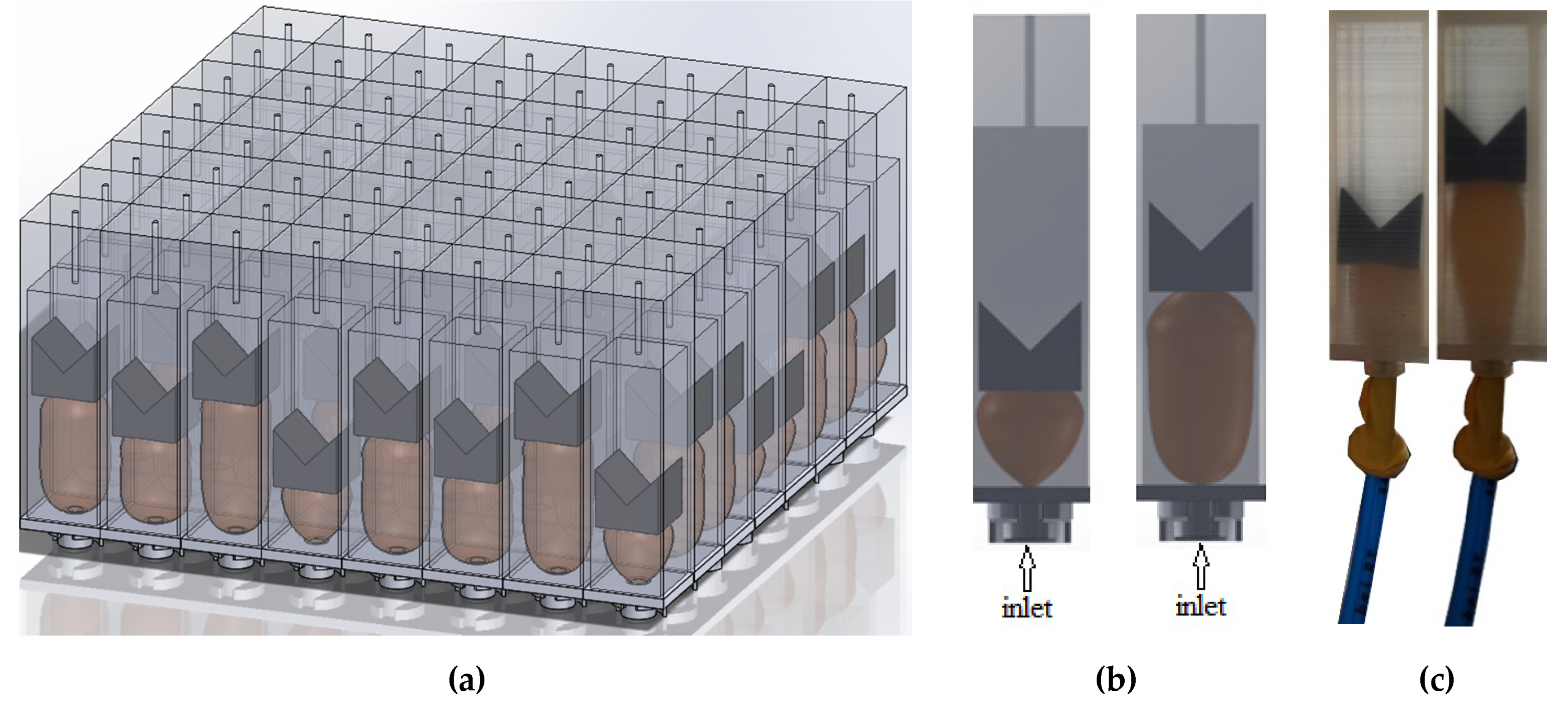
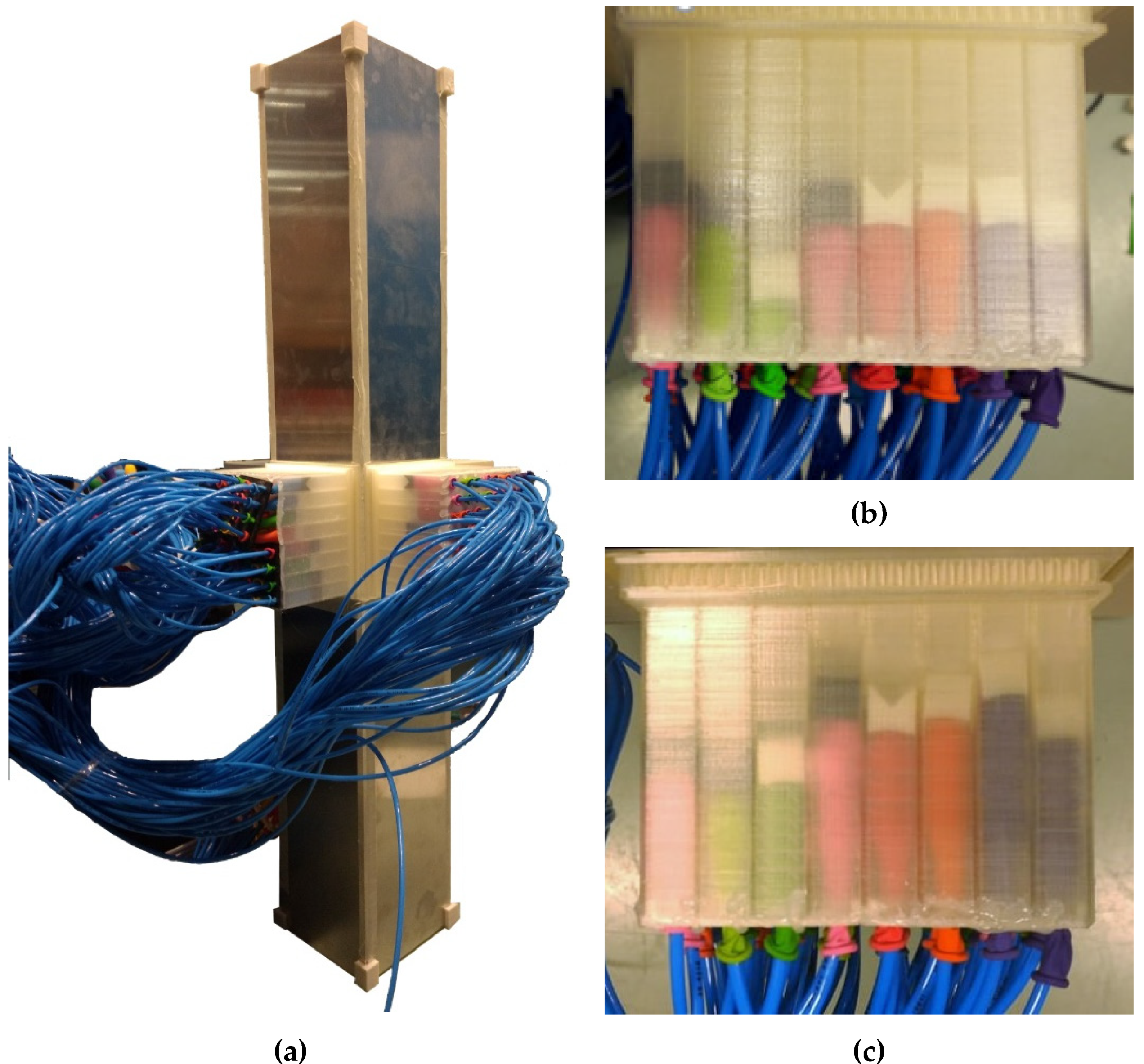
2.1. System Design
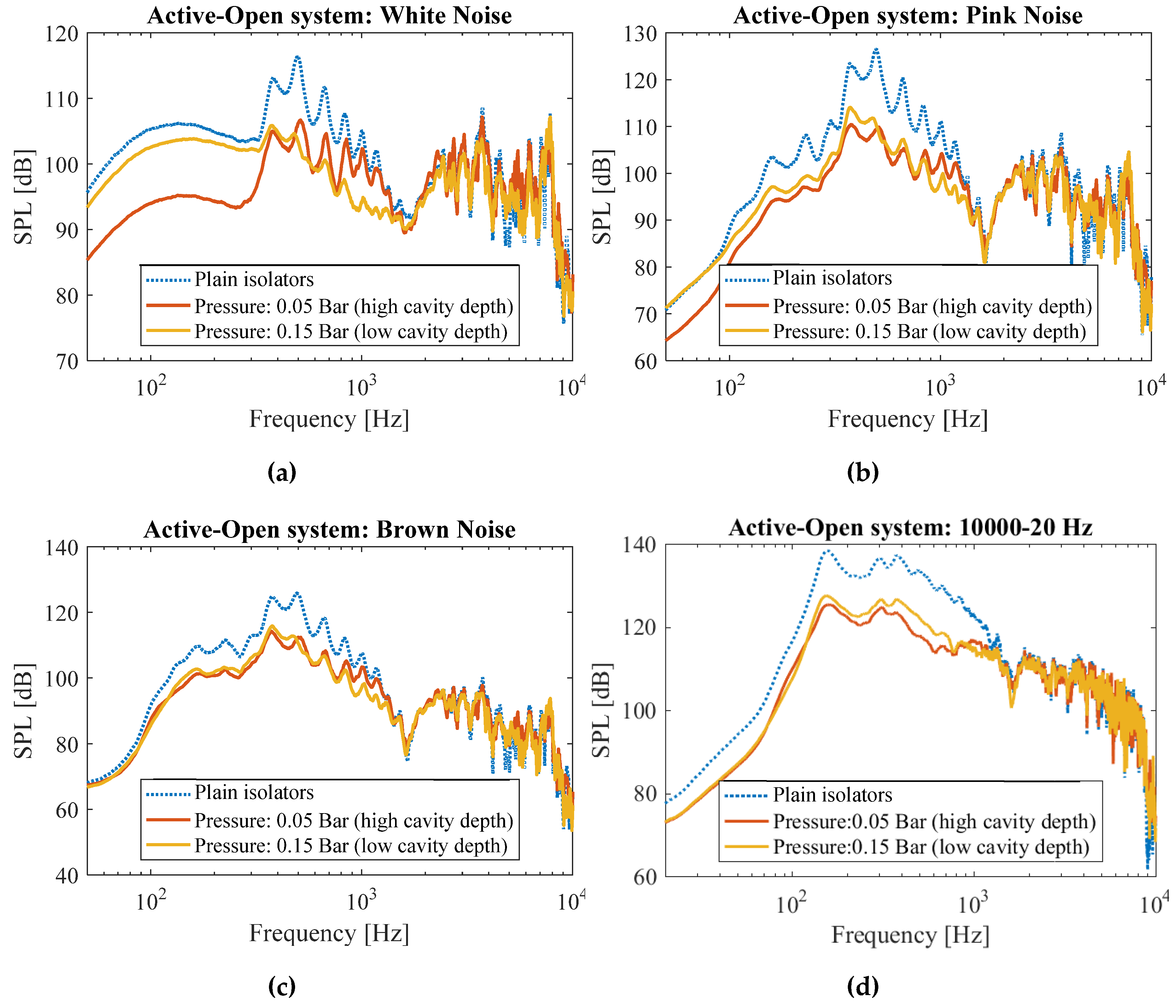
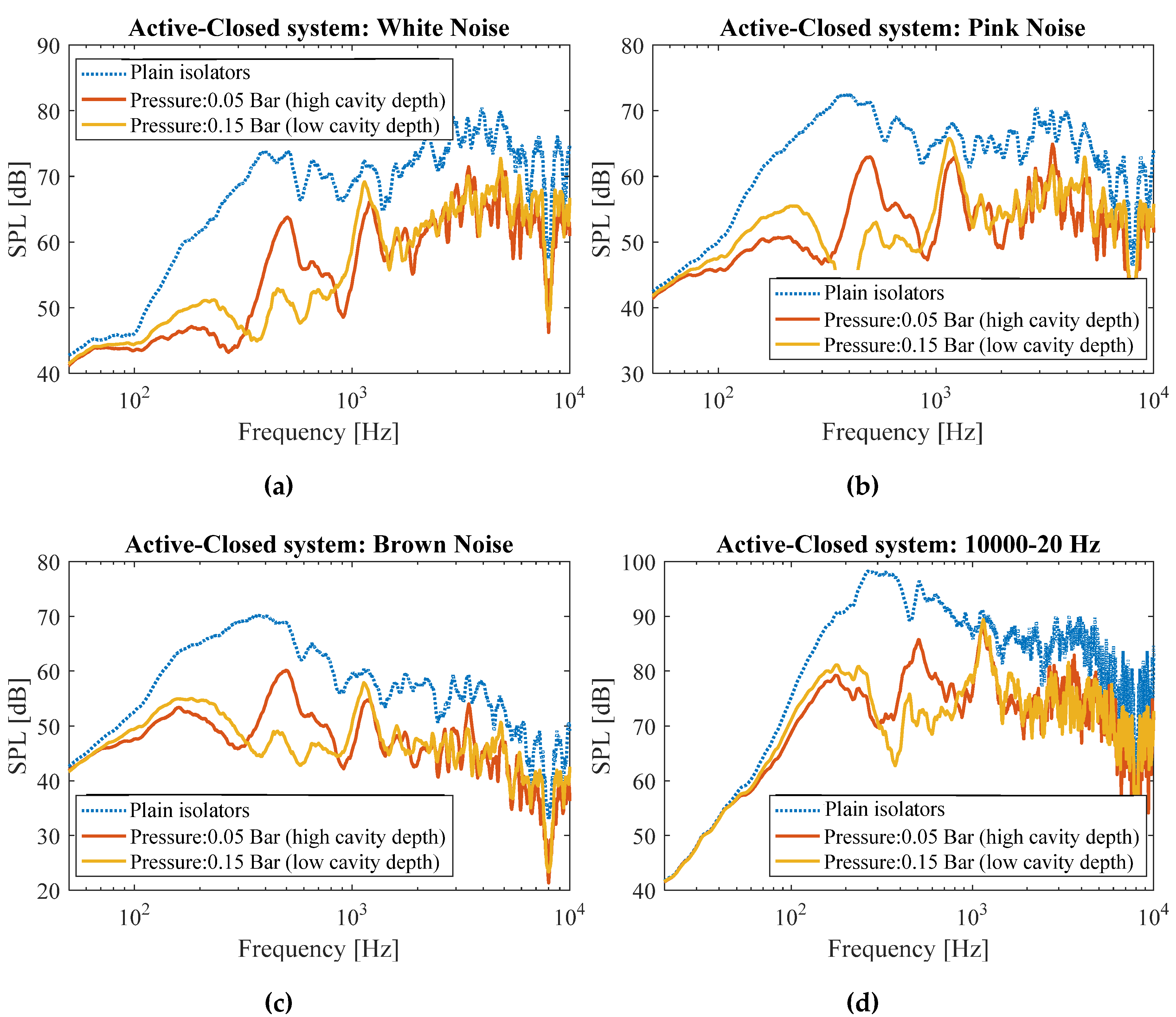
- White noise: white noise has a uniform signal power intensity distribution at all frequencies when frequency is plotted in Hz scale, giving a uniform power spectral density.
- Pink noise: In homogeny with white noise, the pink noise has a uniform power distribution if the bandwidth is plotted in a logarithmic scale. Consequently, if the frequency spectrum is plotted linearly, the sound magnitude is mainly concentrated at the lower end of the spectrum.
- Brown noise: In brown noise, the power amplitude decreases with a proportion of , with respect to frequency. In other words, in brown noise sample, the power amplitude decreases 6 dB per octave.
- A frequency sweep of 10,000→20 Hz to provide a diverse range of narrow-band frequency tones while measuring loudness in real time.
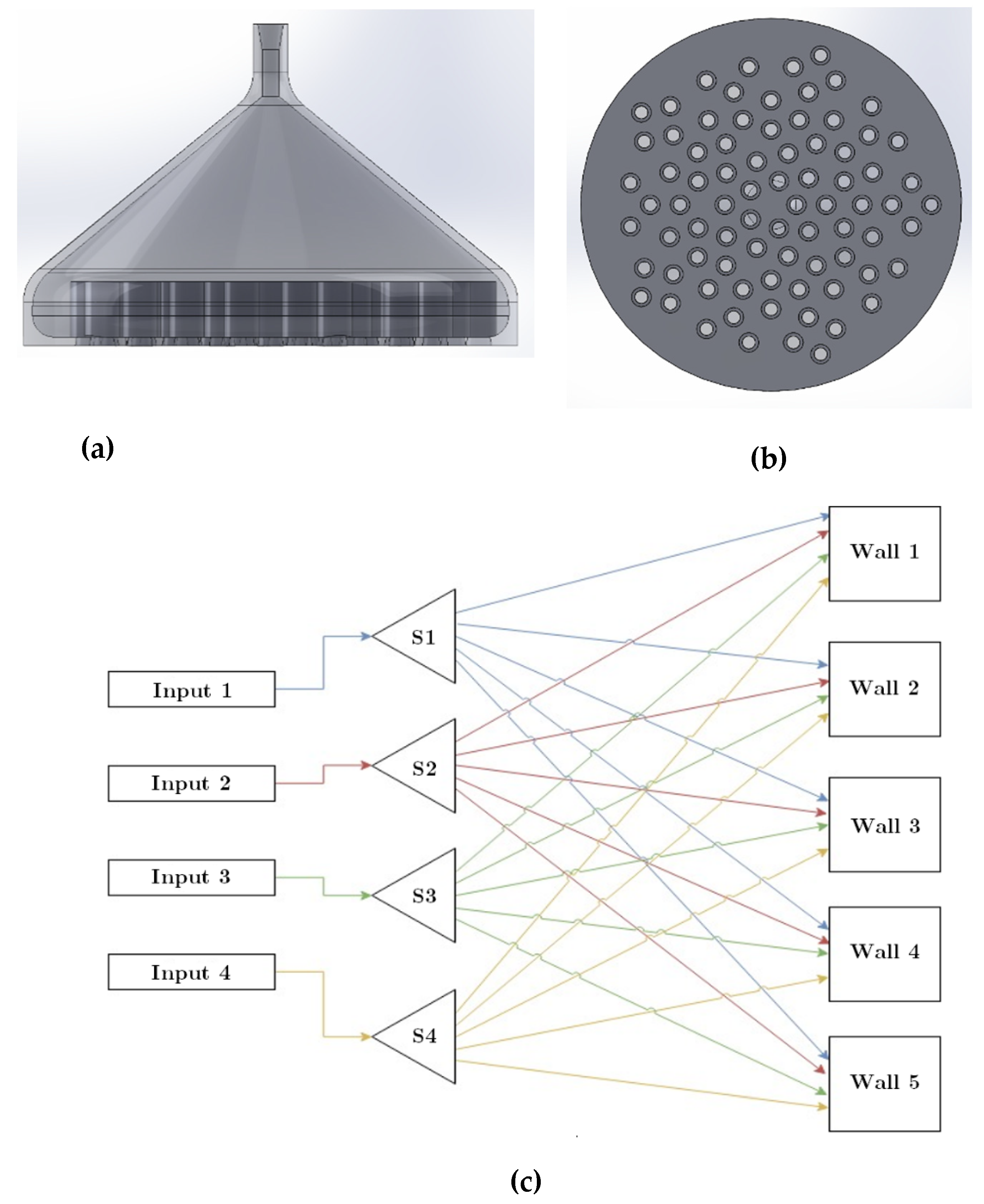
2.2. Manufacturing
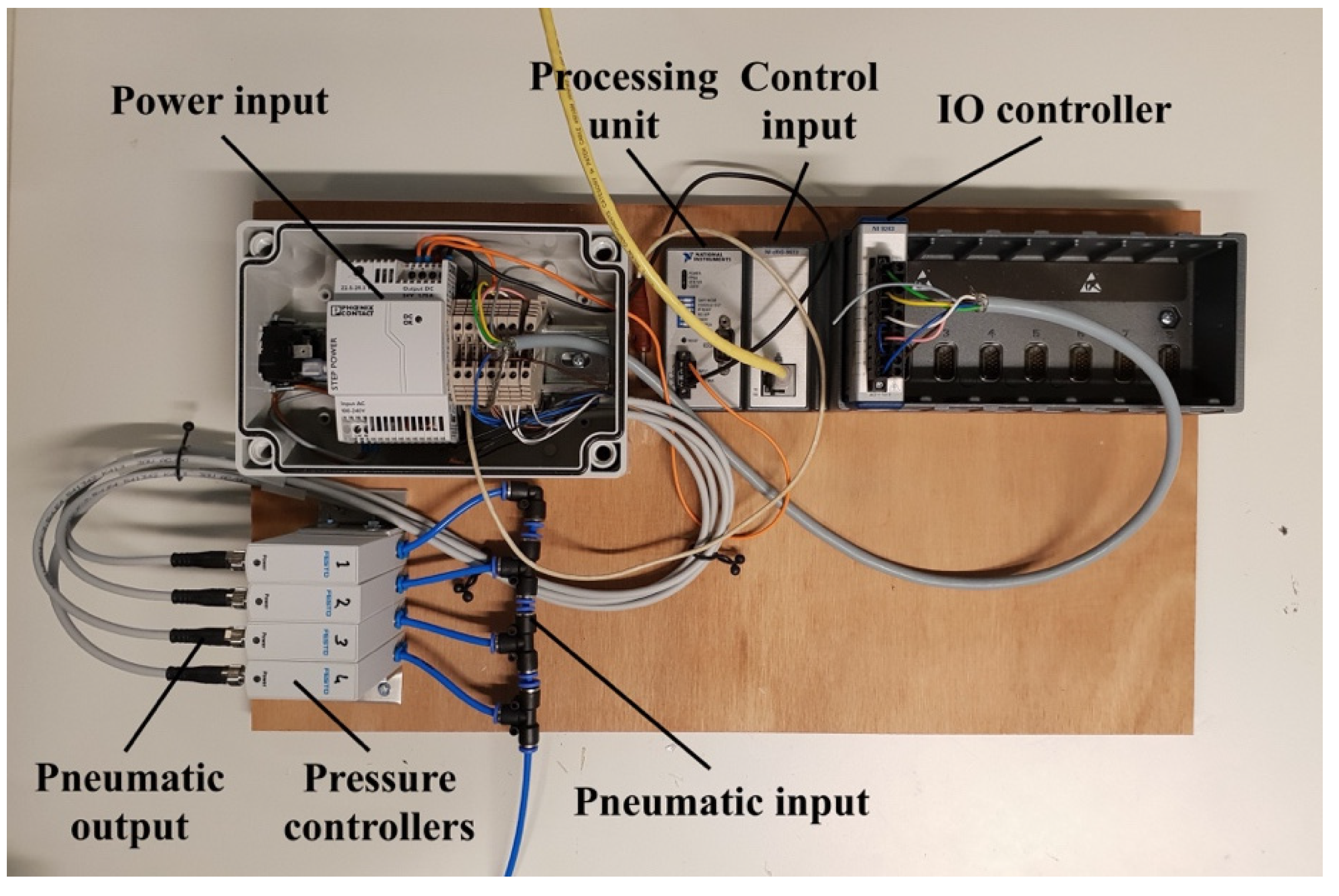
2.3. Actuation
3. Results and Discussions
3.1. Open System
3.2. Closed System
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Hedayati, R.; Mirzaali, M.; Vergani, L.; Zadpoor, A. Action-at-a-distance metamaterials: Distributed local actuation through far-field global forces. APL Mater. 2018, 6, 036101. [Google Scholar] [CrossRef]
- Celli, P.; Gonella, S. Tunable Directivity in Metamaterials with Reconfigurable Cell Symmetry. Appl. Phys. Lett. 2015, 106, 091905. [Google Scholar] [CrossRef]
- Zhou, X.; Assouar, M.B.; Oudich, M. Acoustic superfocusing by solid phononic crystals. Appl. Phys. Lett. 2014, 105, 233506. [Google Scholar] [CrossRef]
- Kaina, N.; Lemoult, F.; Fink, M.; Lerosey, G. Negative refractive index and acoustic superlens from multiple scattering in single negative metamaterials. Nature 2015, 525, 77–81. [Google Scholar] [CrossRef]
- Chen, X.; Xu, X.; Ai, S.; Chen, H.; Pei, Y.; Zhou, X. Active acoustic metamaterials with tunable effective mass density by gradient magnetic fields. Appl. Phys. Lett. 2014, 105, 071913. [Google Scholar] [CrossRef]
- Popa, B.-I.; Shinde, D.; Konneker, A.; Cummer, S.A. Active acoustic metamaterials reconfigurable in real time. Phys. Rev. B 2015, 91, 220303. [Google Scholar] [CrossRef]
- Chen, H.; Chan, C. Acoustic cloaking in three dimensions using acoustic metamaterials. Appl. Phys. Lett. 2007, 91, 183518. [Google Scholar] [CrossRef]
- Hedayati, R.; Jedari Salami, S.; Li, Y.; Sadighi, M.; Zadpoor, A.A. Semianalytical geometry-property relationships for some generalized classes of pentamodelike additively manufactured mechanical metamaterials. Phys. Rev. Appl. 2019, 11, 034057. [Google Scholar] [CrossRef]
- Casadei, F.; Delpero, T.; Bergamini, A.; Ermanni, P.; Ruzzene, M. Piezoelectric resonator arrays for tunable acoustic waveguides and metamaterials. J. Appl. Phys. 2012, 112, 064902. [Google Scholar] [CrossRef]
- Baz, A. The structure of an active acoustic metamaterial with tunable effective density. New J. Phys. 2009, 11, 123010. [Google Scholar] [CrossRef]
- Hedayati, R.; Lakshmanan, S.; Zwaag, S.V.D. Active Acoustic Metamaterial Based on Helmholtz Resonators to Absorb Broadband Low Frequency Noise. J. Sound Vib. 2020. Submitted. [Google Scholar]
- Hedayati, R.; Carpio, A.R.; Luesutthiviboon, S.; Ragni, D.; Avallone, F.; Casalino, D.; van der Zwaag, S. Role of polymeric coating on metallic foams to control the aeroacoustic noise reduction of airfoils with permeable trailing edges. Materials 2019, 12, 1087. [Google Scholar] [CrossRef] [PubMed]
- Ding, C.-L.; Zhao, X.-P. Multi-band and broadband acoustic metamaterial with resonant structures. J. Phys. D Appl. Phys. 2011, 44, 215402. [Google Scholar] [CrossRef]
- Cheer, J.; Daley, S.; McCormick, C. Feedforward control of sound transmission using an active acoustic metamaterial. Smart Mater. Struct. 2017, 26, 025032. [Google Scholar] [CrossRef]
- Reynolds, M.; Daley, S. An active viscoelastic metamaterial for isolation applications. Smart Mater. Struct. 2014, 23, 045030. [Google Scholar] [CrossRef]
- Zhang, X.; Liu, Z. Negative refraction of acoustic waves in two-dimensional phononic crystals. Appl. Phys. Lett. 2004, 85, 341–343. [Google Scholar] [CrossRef]
- Sanchez-Perez, J.V.; Rubio, C.; Martinez-Sala, R.; Sanchez-Grandia, R.; Gomez, V. Acoustic barriers based on periodic arrays of scatterers. Appl. Phys. Lett. 2002, 81, 5240–5242. [Google Scholar] [CrossRef]
- Shen, M.; Cao, W. Acoustic band-gap engineering using finite-size layered structures of multiple periodicity. Appl. Phys. Lett. 1999, 75, 3713–3715. [Google Scholar] [CrossRef]
- Popa, B.I.; Zigoneanu, L.; Cummer, S.A. Tunable active acoustic metamaterials. Phys. Rev. B 2013, 88, 024303. [Google Scholar] [CrossRef]
- Akl, W.; Baz, A. Multi-cell active acoustic metamaterial with programmable bulk modulus. J. Intell. Mater. Syst. Struct. 2010, 21, 541–556. [Google Scholar] [CrossRef]
- Xiao, S.; Ma, G.; Li, Y.; Yang, Z.; Sheng, P. Active control of membrane-type acoustic metamaterial by electric field. Appl. Phys. Lett. 2015, 106, 091904. [Google Scholar] [CrossRef]
- Chen, Z.; Xue, C.; Fan, L.; Zhang, S.Y.; Li, X.J.; Zhang, H.; Ding, J. A tunable acoustic metamaterial with double-negativity driven by electromagnets. Sci. Rep. 2016, 6, 30254. [Google Scholar] [CrossRef] [PubMed]
- Blackstock, D.T. Fundamentals of Physical Acoustics; John Wiley & Sons, Inc.: New York, NY, USA, 2000. [Google Scholar]
- Wolfe, J. Helmholtz Resonance; School of Physics at UNSW: Sydney, Australia, 2000; Available online: http://www.phys.unsw.edu.au/jw/Helmholtz.html (accessed on 20 January 2013).
- Sui, N.; Yan, X.; Huang, T.-Y.; Xu, J.; Yuan, F.-G.; Jing, Y. A lightweight yet sound-proof honeycomb acoustic metamaterial. Appl. Phys. Lett. 2015, 106, 171905. [Google Scholar] [CrossRef]
- Claeys, C.; Deckers, E.; Pluymers, B.; Desmet, W. A lightweight vibro-acoustic metamaterial demonstrator: Numerical and experimental investigation. Mech. Syst. Signal Process. 2016, 70, 853–880. [Google Scholar] [CrossRef]
- Esteve, S.J.; Johnson, M.E. Adaptive Helmholtz resonators and passive vibration absorbers for cylinder interior noise control. J. Sound Vib. 2005, 288, 1105–1130. [Google Scholar] [CrossRef]
- Birdsong, C.; Radcliffe, C.J. An electronically tunable resonator for noise control. Mech. Eng. 2001, 28, 2097–2103. [Google Scholar]
- De Bedout, J.M.; Franchek, M.; Bernhard, R.; Mongeau, L. Adaptive-passive noise control with self-tuning Helmholtz resonators. J. Sound Vib. 1997, 202, 109–123. [Google Scholar] [CrossRef]
- Nagaya, K.; Hano, Y.; Suda, A. Silencer consisting of two-stage Helmholtz resonator with auto-tuning control. J. Acoust. Soc. Am. 2001, 110, 289–295. [Google Scholar] [CrossRef]
- Kela, L. Adaptive Helmholtz resonator in a hydraulic system. World Acad. Sci. Eng. Technol. 2010, 44, 1002–1009. [Google Scholar]
- Yuan, J. Active Helmholtz resonator with positive real impedance. J. Vib. Acoust. 2007, 129, 94–100. [Google Scholar] [CrossRef]
- Singh, S.; Howard, C.; Hansen, C. Tuning a Semi-Active Helmholtz Resonator. In Proceedings of the 6th International Symposium on Active Noise and Vibration Control (ACTIVE 2006), Adelaide, Australia, 18–20 September 2006. [Google Scholar]
- Kela, L. Resonant frequency of an adjustable Helmholtz resonator in a hydraulic system. Arch. Appl. Mech. 2009, 79, 1115–1125. [Google Scholar] [CrossRef]
- Matsuhisa, H.; Ren, B.; Sato, S. Semiactive control of duct noise by a volume-variable resonator. JSME Int. J. Ser. Vib. Control Eng. Eng. Ind. 1992, 35, 223–228. [Google Scholar] [CrossRef]

| Type of Volume Change (neck/cell). | Decrease in Noise (dB) | Range of Frequency (Hz) | Actuation Type | Range of the Changing Parameter | Type (self-Tuning, Active, Adjustable) | Ref. |
|---|---|---|---|---|---|---|
| Cavity length | 100% | 80–170 | Pneumatic | N/A | Adjustable | [28] |
| Neck diameter | 4.2 dB | 75–115 | Rotary-actuated aperture | 9–58 mm | Adjustable | [27] |
| Volume (Sector angle change) | 29 dB | 65–150 | Rotary-actuated walls | 1491–14,093 cm3 | Self-tuning | [29] |
| Cavity length | 20 dB | - | Hydraulic | 43–243 mm | Active | [31] |
| Internal pressure | - | 50–500 (Max-160) | Electromagnetic diaphragm/piezoelectric | N/A | Active | [32] |
| Cavity length | 18 dB | 0–400 (Max-226) | - | 60–90 mm | Adjustable | [33] |
| Neck length | - | 3–75 | Hydraulic | 39–60 cm | Adjustable | [34] |
| Length of cavity | 30 dB | 80–140 | Pneumatic | 560–940 cm3 1.8–17 cm | Adjustable | [35] |
| Area of neck | 25 dB | 100–3000 | Rotary | - | Self-tuning | [30] |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hedayati, R.; Lakshmanan, S. Pneumatically-Actuated Acoustic Metamaterials Based on Helmholtz Resonators. Materials 2020, 13, 1456. https://doi.org/10.3390/ma13061456
Hedayati R, Lakshmanan S. Pneumatically-Actuated Acoustic Metamaterials Based on Helmholtz Resonators. Materials. 2020; 13(6):1456. https://doi.org/10.3390/ma13061456
Chicago/Turabian StyleHedayati, Reza, and Sandhya Lakshmanan. 2020. "Pneumatically-Actuated Acoustic Metamaterials Based on Helmholtz Resonators" Materials 13, no. 6: 1456. https://doi.org/10.3390/ma13061456
APA StyleHedayati, R., & Lakshmanan, S. (2020). Pneumatically-Actuated Acoustic Metamaterials Based on Helmholtz Resonators. Materials, 13(6), 1456. https://doi.org/10.3390/ma13061456






