Free Vibration Analysis of Curved Laminated Composite Beams with Different Shapes, Lamination Schemes, and Boundary Conditions
Abstract
:1. Introduction
2. Theoretical Formulations
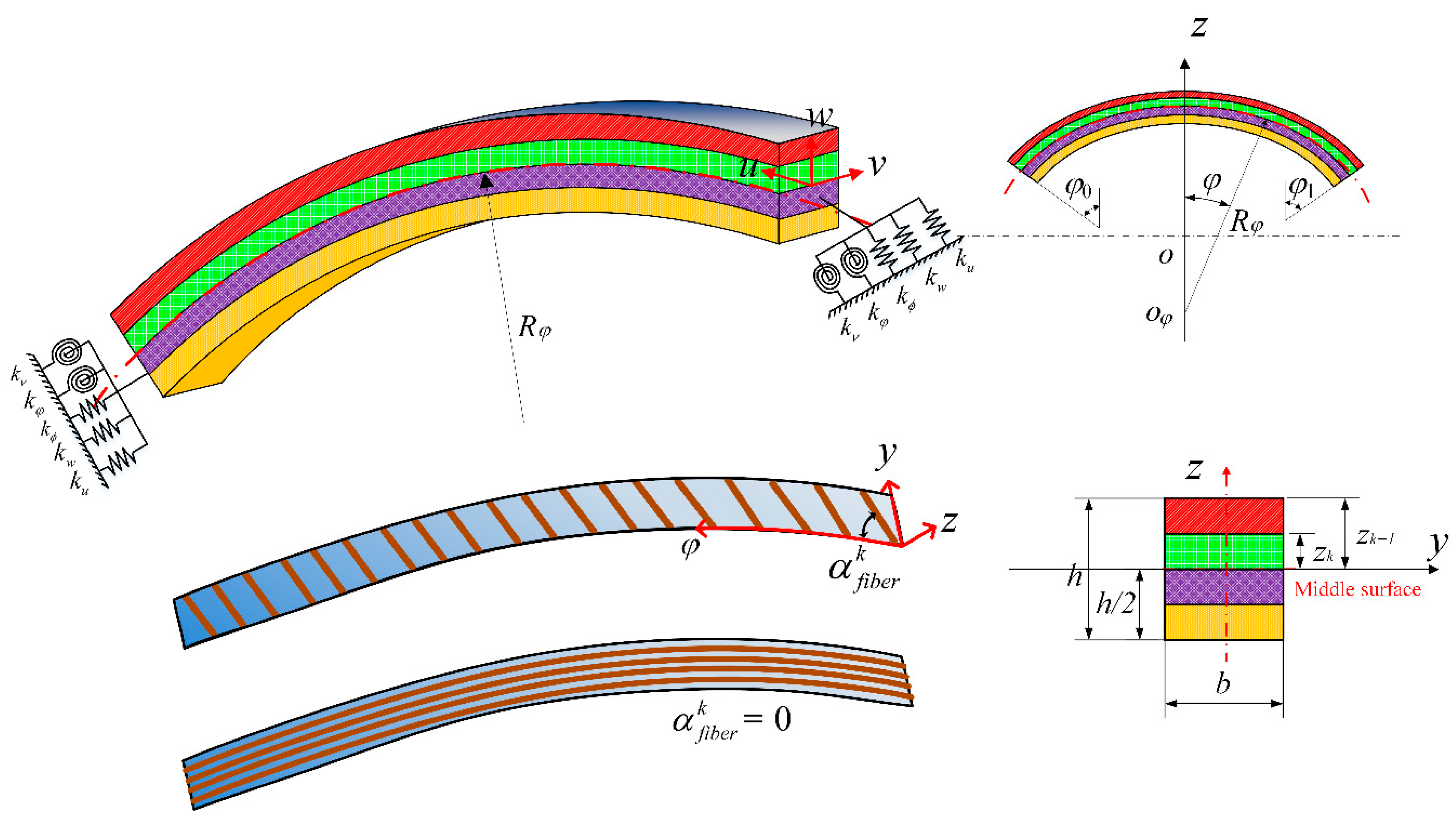
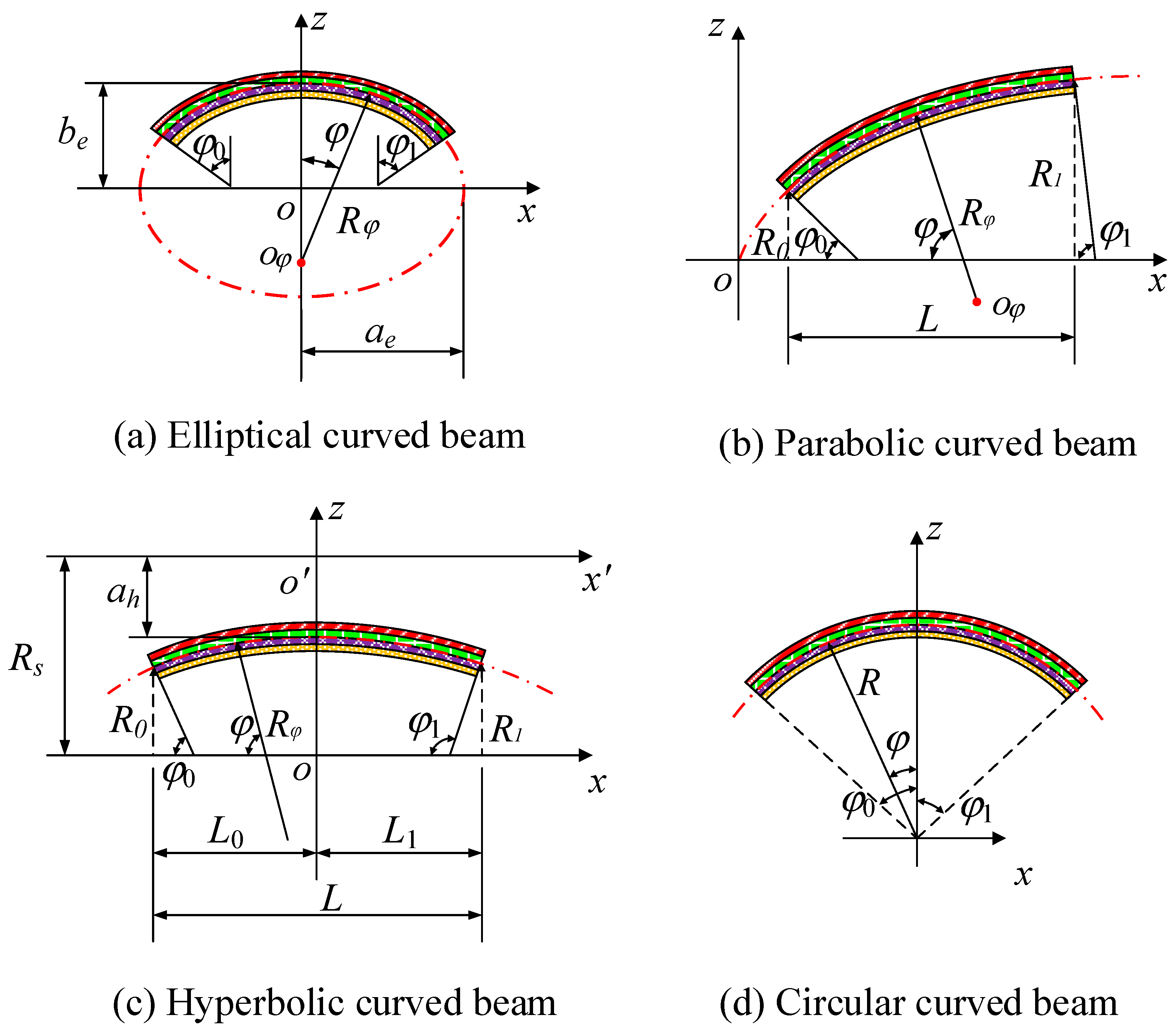
2.1. Description of the CLCB Model
2.2. Energy Expression of the CLCB
2.3. Variational Formulation for Curved Beam
2.4. Solution Procedure
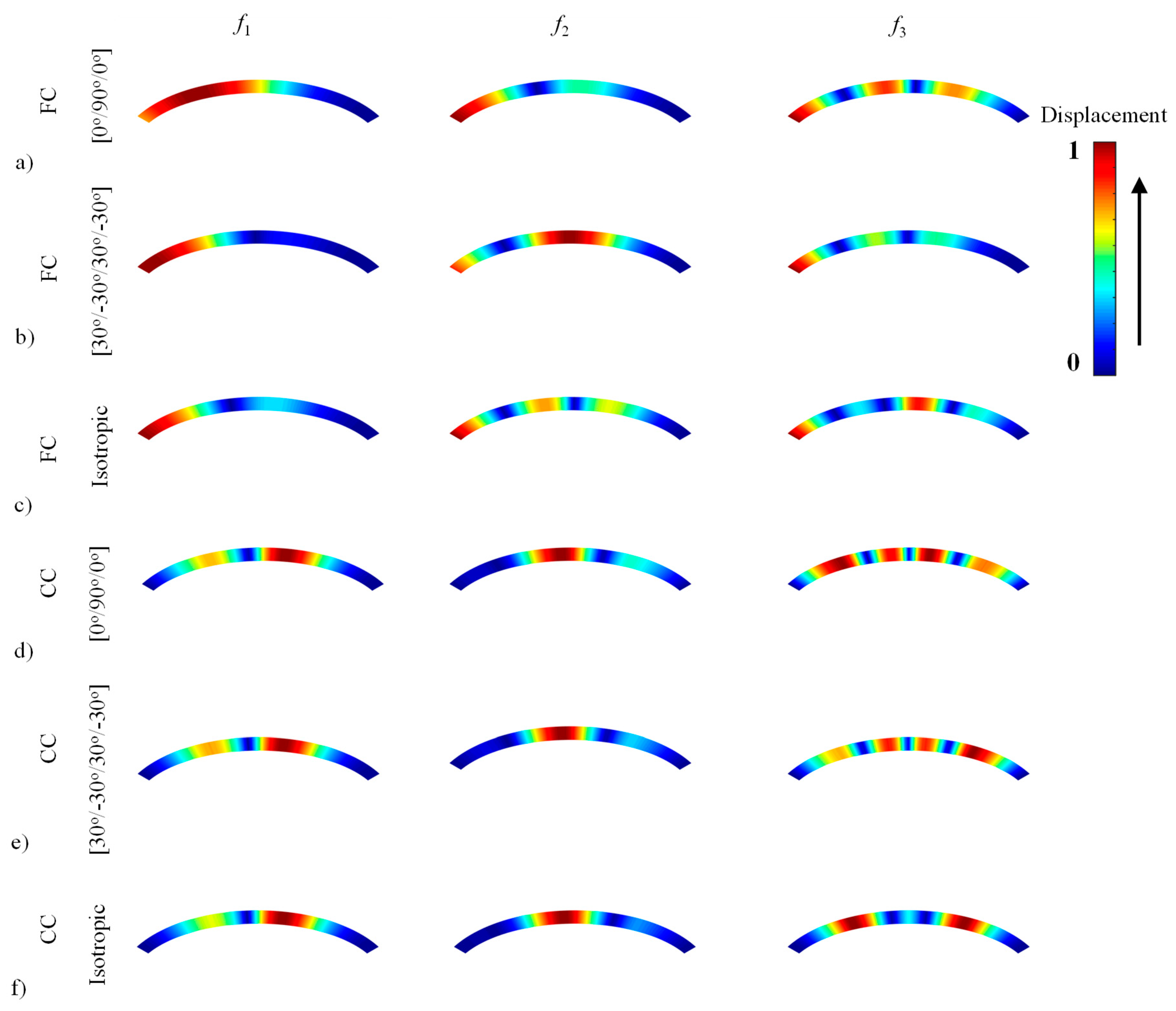
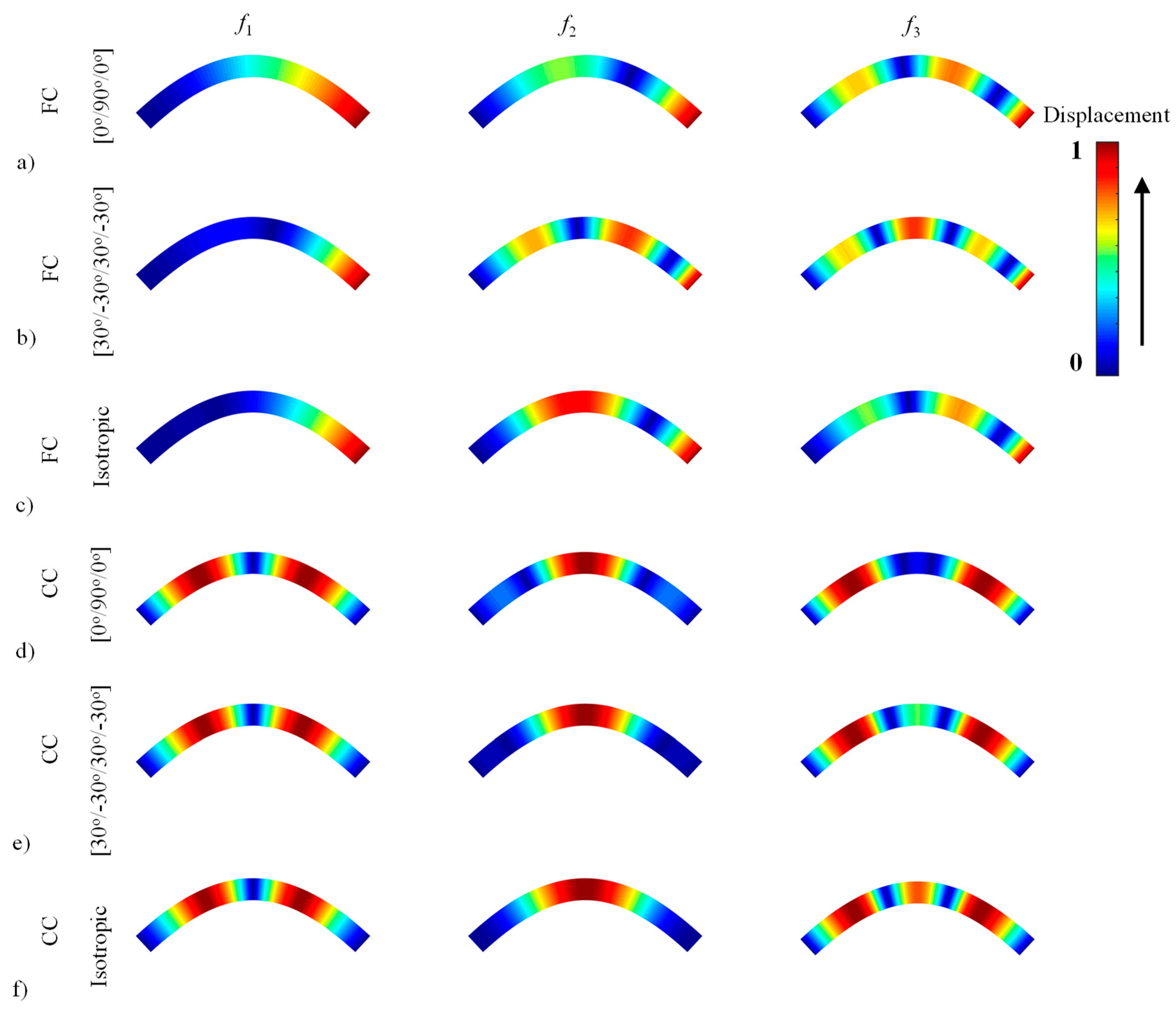
3. Results and Discussions
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A

References
- Huu Quoc, T.; Minh Tu, T.; Van Tham, V. Free vibration analysis of smart laminated functionally graded CNT reinforced composite plates via new four-variable refined plate theory. Materials 2019, 12, 3675. [Google Scholar] [CrossRef] [Green Version]
- Tornabene, F.; Fantuzzi, N.; Bacciocchi, M. Linear static behavior of damaged laminated composite plates and shells. Materials 2017, 10, 811. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Qatu, M.S. Theories and analyses of thin and moderately thick laminated composite curved beams. Int. J. Solids Struct. 1993, 30, 2743–2756. [Google Scholar] [CrossRef]
- Qatu, M.S. In-plane vibration of slightly curved laminated composite beams. J. Sound Vib. 1992, 159, 327–338. [Google Scholar] [CrossRef]
- Qatu, M.S.; Elsharkawy, A.A. Vibration of laminated composite arches with deep curvature and arbitrary boundaries. Comput. Struct. 1993, 47, 305–311. [Google Scholar] [CrossRef]
- Tseng, Y.P.; Huang, C.S.; Kao, M.S. In-plane vibration of laminated curved beams with variable curvature by dynamic stiffness analysis. Compos. Struct. 2000, 50, 103–114. [Google Scholar] [CrossRef]
- Carpentieri, G.; Tornabene, F.; Ascione, L.; Fraternali, F. An accurate one-dimensional theory for the dynamics of laminated composite curved beams. J. Sound Vib. 2015, 336, 96–105. [Google Scholar] [CrossRef]
- Khdeir, A.A.; Reddy, J.N. Free and forced vibration of cross-ply laminated composite shallow arches. Int. J. Solids Struct. 1997, 34, 1217–1234. [Google Scholar] [CrossRef]
- Surana, K.S.; Nguyen, S.H. Two-dimensional curved beam element with higher-order hierarchical transverse approximation for laminated composites. Comput. Struct. 1990, 36, 499–511. [Google Scholar] [CrossRef]
- Hajianmaleki, M.; Qatu, M.S. Static and vibration analyses of thick, generally laminated deep curved beams with different boundary conditions. Compos. Part B-Eng. 2012, 43, 1767–1775. [Google Scholar] [CrossRef]
- Jafari-Talookolaei, R.-A.; Abedi, M.; Hajianmaleki, M. Vibration characteristics of generally laminated composite curved beams with single through-the-width delamination. Compos. Struct. 2016, 138, 172–183. [Google Scholar] [CrossRef]
- Chen, W.Q.; Lv, C.F.; Bian, Z.G. Elasticity solution for free vibration of laminated beams. Compos. Struct. 2003, 62, 75–82. [Google Scholar] [CrossRef]
- Ascione, L.; Fraternali, F. A penalty model for the analysis of curved composite beams. Comput. Struct. 1992, 45, 985–999. [Google Scholar] [CrossRef]
- Ye, T.; Jin, G.; Ye, X.; Wang, X. A series solution for the vibrations of composite laminated deep curved beams with general boundaries. Compos. Struct. 2015, 127, 450–465. [Google Scholar] [CrossRef]
- Luu, A.-T.; Kim, N.-I.; Lee, J. NURBS-based isogeometric vibration analysis of generally laminated deep curved beams with variable curvature. Compos. Struct. 2015, 119, 150–165. [Google Scholar] [CrossRef]
- Shao, D.; Hu, S.; Wang, Q.; Pang, F. A unified analysis for the transient response of composite laminated curved beam with arbitrary lamination schemes and general boundary restraints. Compos. Struct. 2016, 154, 507–526. [Google Scholar] [CrossRef]
- Qu, Y.; Long, X.; Li, H.; Meng, G. A variational formulation for dynamic analysis of composite laminated beams based on a general higher-order shear deformation theory. Compos. Struct. 2013, 102, 175–192. [Google Scholar] [CrossRef]
- Garcia, C.; Trendafilova, I.; Zucchelli, A. The effect of polycaprolactone nanofibers on the dynamic and impact behavior of glass fibre reinforced polymer composites. J. Compos. Sci. 2018, 2, 43. [Google Scholar] [CrossRef] [Green Version]
- Garcia, C.; Wilson, J.; Trendafilova, I.; Yang, L. Vibratory behaviour of glass fibre reinforced polymer (GFRP) interleaved with nylon nanofibers. Compos. Struct. 2017, 176, 923–932. [Google Scholar] [CrossRef] [Green Version]
- Yagci, B.; Filiz, S.; Romero, L.L.; Ozdoganlar, O.B. A spectral-Tchebychev technique for solving linear and nonlinear beam equations. J. Sound Vib. 2009, 321, 375–404. [Google Scholar] [CrossRef]
- Bediz, B. Three-dimensional vibration behavior of bi-directional functionally graded curved parallelepipeds using spectral Tchebychev approach. Compos. Struct. 2018, 191, 100–112. [Google Scholar] [CrossRef]
- Bediz, B.; Aksoy, S. A spectral-Tchebychev solution for three-dimensional dynamics of curved beams under mixed boundary conditions. J. Sound Vib. 2018, 413, 26–40. [Google Scholar] [CrossRef]
- Tornabene, F.; Fantuzzi, N.; Bacciocchi, M.; Viola, E. Accurate inter-laminar recovery for plates and doubly-curved shells with variable radii of curvature using layer-wise theories. Compos. Struct. 2015, 124, 368–393. [Google Scholar] [CrossRef]
- Qu, Y.; Chen, Y.; Long, X.; Hua, H.; Meng, G. Free and forced vibration analysis of uniform and stepped circular cylindrical shells using a domain decomposition method. Appl. Acoust. 2013, 74, 425–439. [Google Scholar] [CrossRef]
- Qu, Y.; Hua, H.; Meng, G. A domain decomposition approach for vibration analysis of isotropic and composite cylindrical shells with arbitrary boundaries. Compos. Struct. 2013, 95, 307–321. [Google Scholar] [CrossRef]
- Qu, Y.; Long, X.; Yuan, G.; Meng, G. A unified formulation for vibration analysis of functionally graded shells of revolution with arbitrary boundary conditions. Compos. Part B-Eng. 2013, 50, 381–402. [Google Scholar] [CrossRef]
- Qu, Y.; Chen, Y.; Chen, Y.; Long, X.; Hua, H.; Meng, G. A Domain Decomposition Method for Vibration Analysis of Conical Shells With Uniform and Stepped Thickness. J. Vib. Acoust. 2013, 135, 011014. [Google Scholar] [CrossRef]
- Qin, B.; Zhong, R.; Wang, T.; Wang, Q.; Xu, Y.; Hu, Z. A unified Fourier series solution for vibration analysis of FG-CNTRC cylindrical, conical shells and annular plates with arbitrary boundary conditions. Compos. Struct. 2020, 232, 111549. [Google Scholar] [CrossRef]
- Zhang, H.; Zhu, R.; Shi, D.; Wang, Q.; Yu, H. Study on vibro-acoustic property of composite laminated rotary plate-cavity system based on a simplified plate theory and experimental method. Int. J. Mech. Sci. 2020, 167, 105264. [Google Scholar] [CrossRef]
- Ye, T.; Jin, G.; Su, Z. A spectral-sampling surface method for the vibration of 2-D laminated curved beams with variable curvatures and general restraints. Int. J. Mech. Sci. 2016, 110, 170–189. [Google Scholar] [CrossRef]
- Liu, T.; Wang, A.; Wang, Q.; Qin, B. (2020) Wave Based Method for Free Vibration Characteristics of Functionally Graded Cylindrical Shells with Arbitrary Boundary Conditions. Thin-Walled Struct. 2020, 148, 106580. [Google Scholar] [CrossRef]
- Choe, K.; Tang, J.; Shui, C.; Wang, A.; Wang, Q. Free vibration analysis of coupled functionally graded (FG) doubly-curved revolution shell structures with general boundary conditions. Compos. Struct. 2018, 194, 413–432. [Google Scholar] [CrossRef]
- Choe, K.; Wang, Q.; Tang, J.; Shui, C. Vibration analysis for coupled composite laminated axis-symmetric doubly-curved revolution shell structures by unified Jacobi-Ritz method. Compos. Struct. 2018, 194, 136–157. [Google Scholar] [CrossRef]
- Wang, Q.; Choe, K.; Shi, D.; Sin, K. Vibration analysis of the coupled doubly-curved revolution shell structures by using Jacobi-Ritz method. Int. J. Mech. Sci. 2018, 135, 517–531. [Google Scholar] [CrossRef]
- Shao, D.; Hu, S.; Wang, Q.; Pang, F. Free vibration of refined higher-order shear deformation composite laminated beams with general boundary conditions. Compos. Part B-Eng. 2017, 108, 75–90. [Google Scholar] [CrossRef]
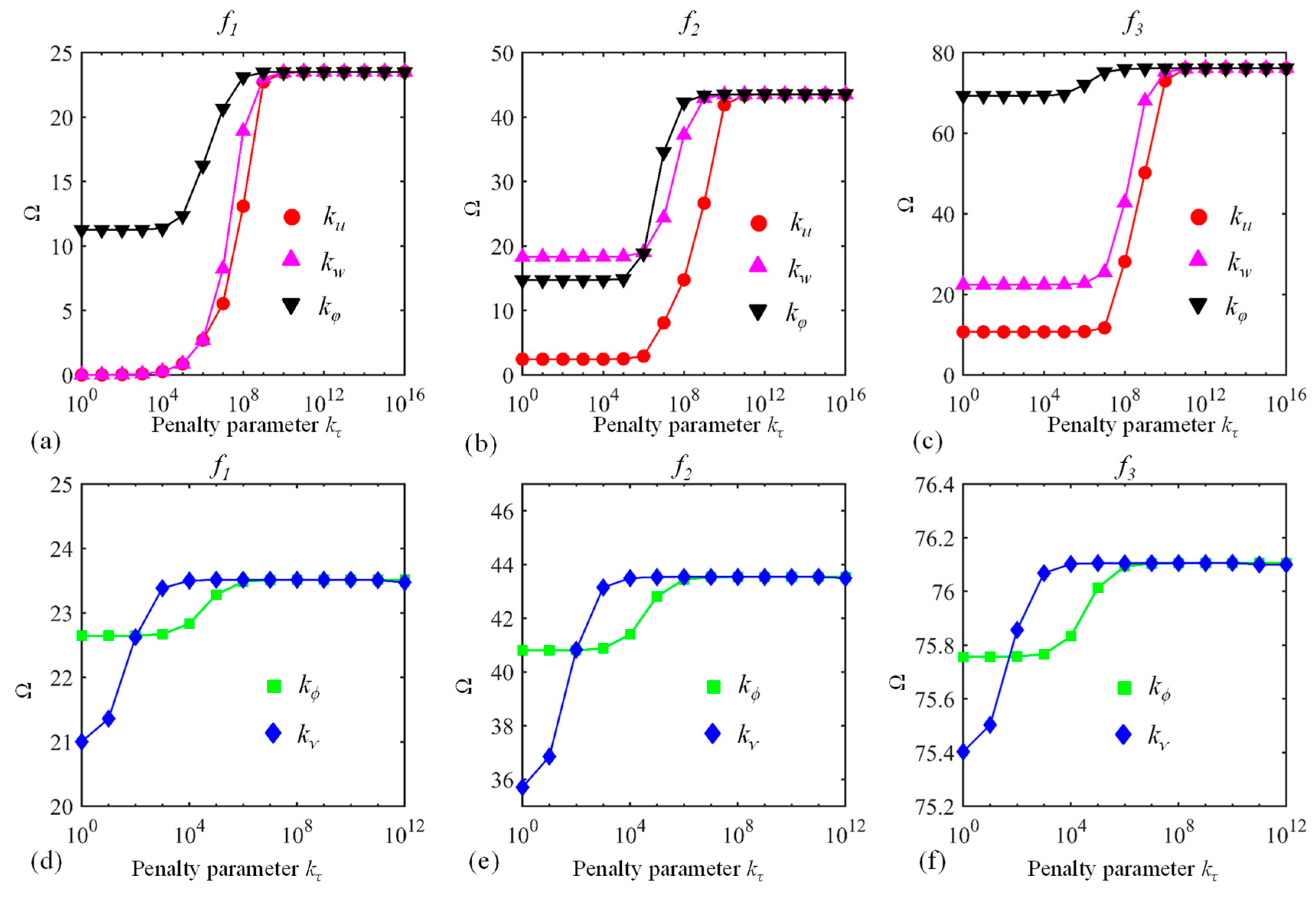
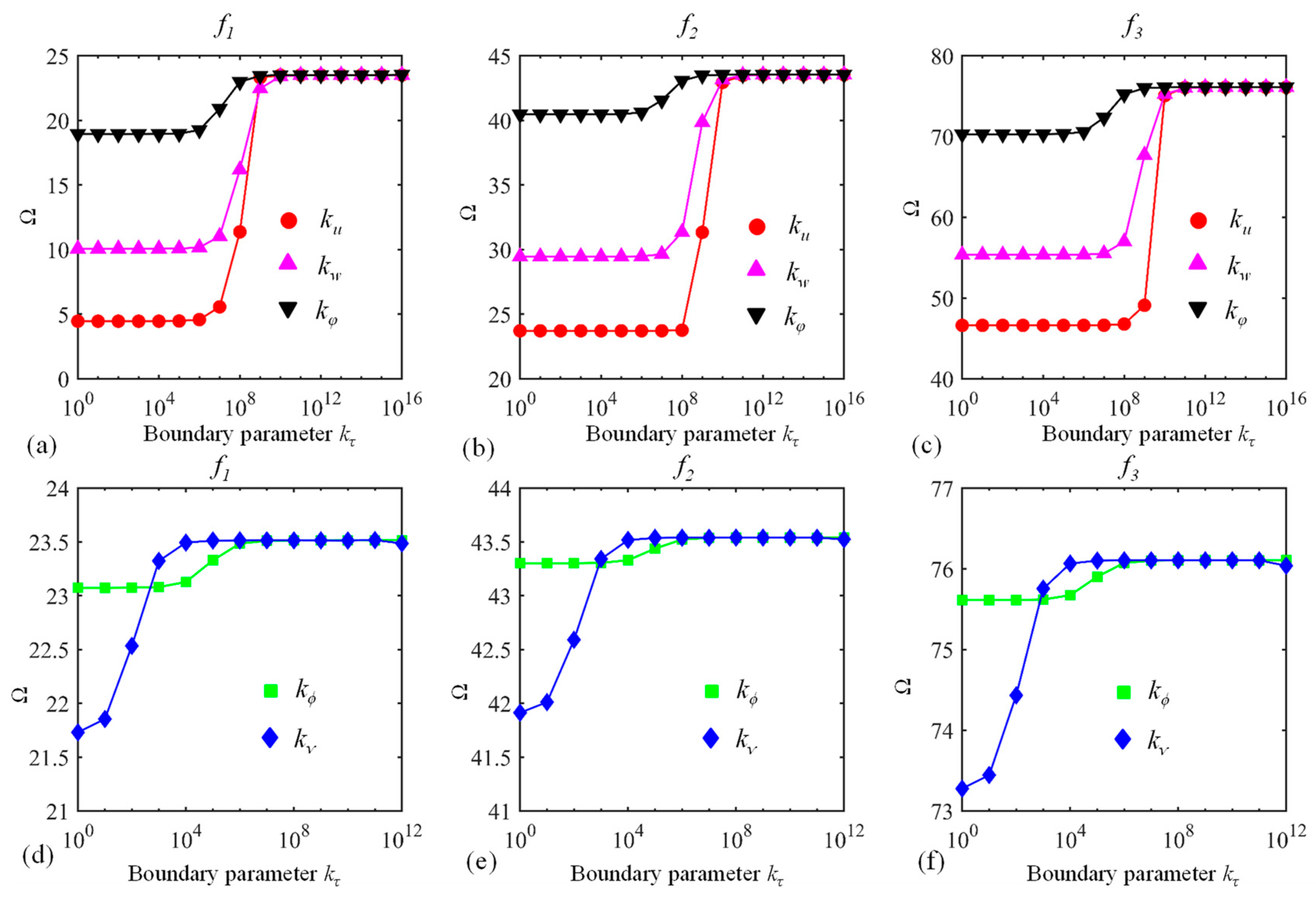
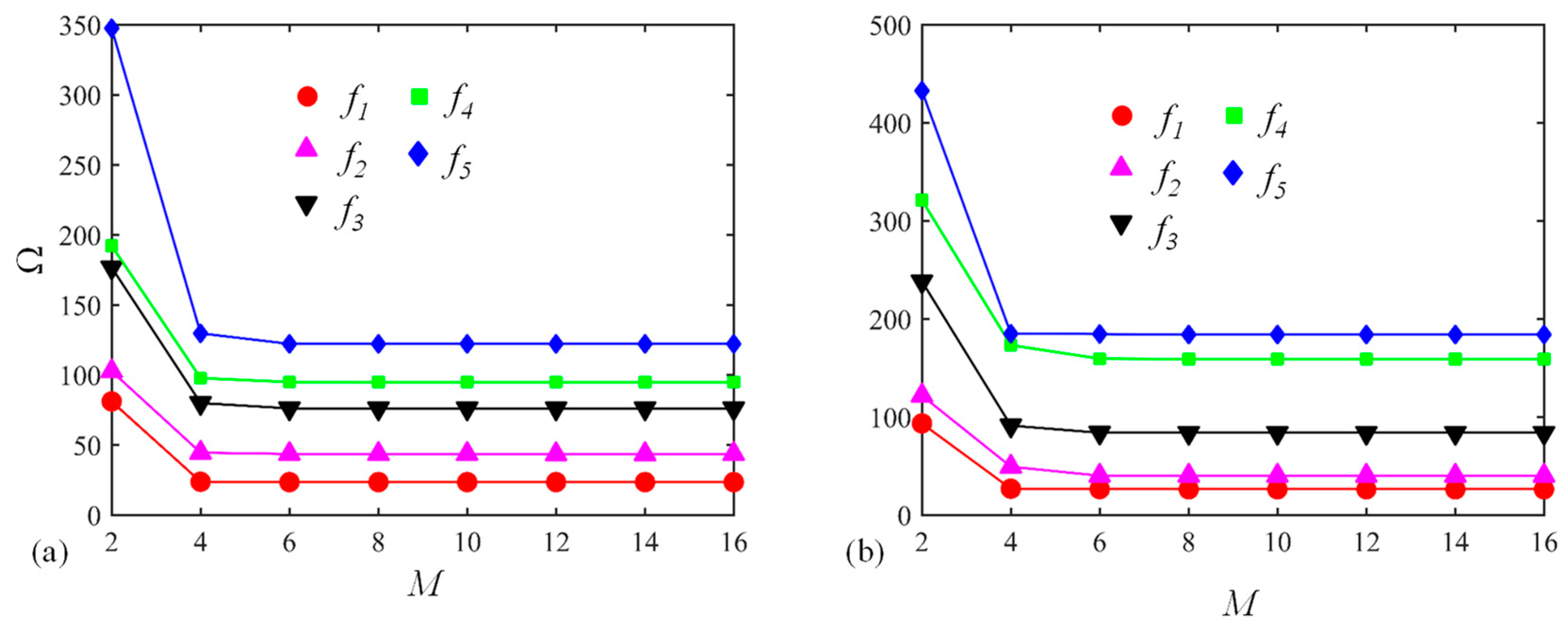


| Boundary Conditions | Boundary Coefficients | Penalty Parameters | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| ηu | ηw | ηφ | ηϕ | ην | ku | kw | kφ | kϕ | kν | |
| Free (F) | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| Simply supported (SS) | 1 | 1 | 0 | 0 | 0 | 1014 | 1014 | 0 | 0 | 0 |
| Slided (SD) | 0 | 1 | 0 | 0 | 0 | 0 | 1014 | 0 | 0 | 0 |
| Clamped (C) | 1 | 1 | 1 | 1 | 1 | 1014 | 1014 | 1014 | 108 | 108 |
| Elastic supported 1 (E1) | 1 | 1 | 1 | 1 | 1 | 108 | 108 | 1014 | 108 | 108 |
| Elastic supported 1 (E2) | 1 | 1 | 1 | 1 | 1 | 1014 | 1014 | 108 | 108 | 108 |
| Elastic supported 1 (E3) | 1 | 1 | 1 | 1 | 1 | 108 | 108 | 108 | 108 | 108 |
| h | Mode Number | Number of the Segment Nφ | Ref. [14] | Ref. [3] | ||||
|---|---|---|---|---|---|---|---|---|
| 2 | 4 | 6 | 8 | 10 | ||||
| 0.1 | 1 | 23.519 | 23.514 | 23.514 | 23.513 | 23.513 | 23.245 | 23.628 |
| 2 | 43.556 | 43.540 | 43.537 | 43.538 | 43.536 | 42.803 | 43.800 | |
| 3 | 76.148 | 76.105 | 76.101 | 76.099 | 76.099 | 74.476 | 76.687 | |
| 4 | 94.982 | 94.936 | 94.929 | 94.925 | 94.921 | 93.286 | 95.388 | |
| 5 | 122.318 | 122.192 | 122.186 | 122.183 | 122.180 | 120.064 | 123.059 | |
| 0.2 | 1 | 19.816 | 19.805 | 19.803 | 19.799 | 19.795 | 19.116 | 20.005 |
| 2 | 32.761 | 32.736 | 32.724 | 32.714 | 32.703 | 31.450 | 33.003 | |
| 3 | 55.356 | 55.340 | 55.304 | 55.264 | 55.224 | 52.850 | 55.849 | |
| 4 | 55.495 | 55.480 | 55.454 | 55.446 | 55.437 | 54.094 | 55.971 | |
| 5 | 79.636 | 79.568 | 79.550 | 79.544 | 79.533 | 75.675 | 80.422 | |
| Mode No. | Elliptical Beam | Paraboloidal Beam | Hyperbolical Beam | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Present | FEM | Error (%) | Present | FEM | Error (%) | Present | FEM | Error (%) | |
| 1 | 380.16 | 376.6 | 0.95 | 739.05 | 734 | 0.68 | 793.17 | 784.9 | 1.05 |
| 2 | 594.22 | 589.8 | 0.75 | 1162.5 | 1155.3 | 0.62 | 1207.4 | 1195.3 | 1.01 |
| 3 | 934.78 | 930.1 | 0.50 | 1826.5 | 1807.7 | 1.03 | 1809.0 | 1773.4 | 2.01 |
| 4 | 1116.0 | 1111.4 | 0.41 | 2481.2 | 2449.6 | 1.27 | 2433.8 | 2375.3 | 2.46 |
| 5 | 1503.5 | 1490.6 | 0.87 | 3161.2 | 3110.7 | 1.60 | 3107.4 | 3042 | 2.15 |
| 6 | 1819.4 | 1813.8 | 0.31 | 3409.7 | 3391 | 0.55 | 3451.1 | 3443.1 | 0.23 |
| 7 | 2169.6 | 2151.3 | 0.85 | 4011.5 | 3915.2 | 2.40 | 3979.0 | 3866 | 2.92 |
| 8 | 2375.5 | 2359.8 | 0.67 | 4655.6 | 4582 | 1.58 | 4565.8 | 4498.2 | 1.50 |
| Mode No. | F-F | F-C | C-SS | ||||||
|---|---|---|---|---|---|---|---|---|---|
| HBT[LST] | HBT[LMR] | Present | HBT[LST] | HBT[LMR] | Present | HBT[LST] | HBT[LMR] | Present | |
| 1 | 765.68 | 765.306 | 771.373 | 338.33 | 338.197 | 343.494 | 764.78 | 763.348 | 769.884 |
| 2 | 2100.96 | 2097.346 | 2115.244 | 1349.00 | 1346.992 | 1341.057 | 2097.01 | 2088.465 | 2108.493 |
| 3 | 4092.08 | 4077.308 | 4115.793 | 3019.38 | 3009.457 | 3093.753 | 4081.73 | 4053.791 | 4097.477 |
| 4 | 6707.87 | 6666.978 | 6744.373 | 5329.25 | 5298.837 | 5384.977 | 6686.94 | 6619.074 | 6700.256 |
| Lamination Schemes (°) | f (Hz) | Boundary Conditions | ||||||
|---|---|---|---|---|---|---|---|---|
| F-F | F-C | SS-SD | C-C | E1-E1 | E2-E2 | E3-E3 | ||
| [0] | 1 | 103.35 | 18.207 | 21.931 | 214.16 | 56.997 | 171.69 | 53.464 |
| 2 | 262.64 | 85.296 | 150.65 | 263.09 | 61.286 | 254.28 | 59.808 | |
| 3 | 460.62 | 226.56 | 300.36 | 539.35 | 97.939 | 512.11 | 97.190 | |
| 4 | 677.35 | 384.05 | 505.66 | 583.42 | 193.52 | 580.83 | 177.09 | |
| 5 | 898.48 | 581.31 | 685.43 | 886.62 | 359.43 | 860.50 | 320.60 | |
| [0/90] | 1 | 51.865 | 8.846 | 11.239 | 128.84 | 48.745 | 112.12 | 46.682 |
| 2 | 141.13 | 45.614 | 82.267 | 174.40 | 59.679 | 167.82 | 58.532 | |
| 3 | 267.66 | 129.77 | 179.62 | 371.09 | 83.032 | 348.96 | 82.089 | |
| 4 | 422.68 | 240.35 | 324.54 | 397.31 | 130.95 | 393.14 | 130.60 | |
| 5 | 597.93 | 378.64 | 469.09 | 633.42 | 220.11 | 586.10 | 213.05 | |
| [0/90/0] | 1 | 102.10 | 17.923 | 21.640 | 216.71 | 56.984 | 172.22 | 53.410 |
| 2 | 262.42 | 84.886 | 150.17 | 254.95 | 61.292 | 248.28 | 59.810 | |
| 3 | 465.29 | 227.31 | 300.04 | 547.94 | 97.685 | 518.58 | 97.038 | |
| 4 | 690.84 | 383.60 | 506.01 | 550.78 | 193.14 | 539.92 | 176.80 | |
| 5 | 920.99 | 576.64 | 653.50 | 867.54 | 362.43 | 833.45 | 322.23 | |
| [0/90/0/90] | 1 | 72.274 | 12.514 | 15.476 | 167.75 | 53.351 | 138.49 | 50.262 |
| 2 | 192.11 | 62.109 | 110.87 | 208.30 | 60.627 | 201.28 | 59.339 | |
| 3 | 353.63 | 172.04 | 232.27 | 450.34 | 89.398 | 423.16 | 89.277 | |
| 4 | 542.19 | 303.71 | 406.54 | 454.42 | 155.25 | 445.22 | 150.87 | |
| 5 | 744.91 | 464.35 | 547.68 | 729.13 | 280.43 | 687.52 | 260.79 | |
| Lamination Schemes (°) | f (Hz) | Boundary Conditions | ||||||
|---|---|---|---|---|---|---|---|---|
| F-F | F-C | SS-SD | C-C | E1-E1 | E2-E2 | E3-E3 | ||
| [0] | 1 | 373.52 | 64.623 | 164.28 | 371.90 | 85.164 | 322.36 | 82.579 |
| 2 | 800.98 | 312.45 | 507.90 | 657.91 | 87.915 | 607.76 | 87.789 | |
| 3 | 1253.6 | 707.78 | 881.86 | 1036.3 | 210.21 | 997.29 | 175.46 | |
| 4 | 1714.7 | 1110.7 | 1167.5 | 1424.0 | 552.63 | 1401.6 | 464.38 | |
| 5 | 2242.4 | 1181.7 | 1455.7 | 1859.1 | 964.69 | 1843.9 | 867.60 | |
| [0/90] | 1 | 197.02 | 32.159 | 87.238 | 245.62 | 81.521 | 197.89 | 77.791 |
| 2 | 491.20 | 185.02 | 320.31 | 469.30 | 87.631 | 420.62 | 87.597 | |
| 3 | 857.49 | 468.35 | 641.00 | 790.40 | 149.76 | 710.61 | 147.49 | |
| 4 | 1275.6 | 817.64 | 769.92 | 1149.3 | 349.65 | 1105.4 | 321.28 | |
| 5 | 1593.7 | 834.84 | 1048.4 | 1518.4 | 667.48 | 1415.6 | 612.78 | |
| [0/90/0] | 1 | 373.15 | 63.977 | 163.75 | 358.74 | 85.290 | 300.87 | 82.653 |
| 2 | 817.30 | 316.41 | 512.27 | 664.06 | 87.895 | 602.30 | 87.767 | |
| 3 | 1292.5 | 725.21 | 830.90 | 1058.7 | 209.82 | 1013.9 | 175.42 | |
| 4 | 1775.5 | 962.32 | 1061.6 | 1460.7 | 562.24 | 1437.0 | 468.36 | |
| 5 | 1921.2 | 1169.0 | 1464.0 | 1848.6 | 994.58 | 1841.3 | 888.14 | |
| [0/90/0/90] | 1 | 270.67 | 45.008 | 119.10 | 290.34 | 83.809 | 237.39 | 80.442 |
| 2 | 635.86 | 242.33 | 406.68 | 554.66 | 87.796 | 496.35 | 87.709 | |
| 3 | 1055.0 | 583.18 | 731.14 | 905.41 | 172.60 | 841.77 | 161.38 | |
| 4 | 1498.5 | 844.42 | 897.74 | 1277.4 | 442.01 | 1249.1 | 385.83 | |
| 5 | 1673.8 | 974.15 | 1237.1 | 1626.3 | 813.80 | 1588.1 | 733.36 | |
| Lamination Schemes (°) | f (Hz) | Boundary Conditions | ||||||
|---|---|---|---|---|---|---|---|---|
| F-F | F-C | SS-SD | C-C | E1-E1 | E2-E2 | E3-E3 | ||
| [0] | 1 | 304.37 | 63.190 | 84.575 | 516.70 | 83.783 | 454.97 | 82.037 |
| 2 | 706.30 | 215.16 | 437.29 | 896.56 | 84.652 | 867.29 | 82.866 | |
| 3 | 1133.2 | 599.33 | 851.70 | 1121.3 | 201.41 | 1098.4 | 169.28 | |
| 4 | 1564.2 | 1018.0 | 1265.3 | 1308.2 | 457.61 | 1284.3 | 390.81 | |
| 5 | 1987.4 | 1414.5 | 1411.8 | 1718.8 | 862.73 | 1701.3 | 772.01 | |
| [0/90] | 1 | 166.56 | 31.709 | 46.314 | 375.75 | 81.434 | 331.10 | 78.948 |
| 2 | 446.82 | 132.13 | 279.03 | 689.45 | 82.951 | 647.32 | 82.043 | |
| 3 | 776.96 | 399.38 | 585.56 | 834.51 | 143.64 | 739.01 | 141.75 | |
| 4 | 1162.2 | 743.98 | 878.91 | 1057.0 | 303.53 | 999.08 | 283.67 | |
| 5 | 1537.4 | 1058.2 | 988.53 | 1429.0 | 608.67 | 1378.6 | 561.35 | |
| [0/90/0] | 1 | 303.83 | 62.500 | 84.171 | 531.51 | 83.853 | 462.71 | 82.065 |
| 2 | 719.58 | 217.47 | 442.46 | 881.36 | 84.665 | 877.44 | 82.869 | |
| 3 | 1165.7 | 610.38 | 876.96 | 991.48 | 200.91 | 931.96 | 169.20 | |
| 4 | 1620.2 | 1050.2 | 1103.1 | 1356.6 | 464.23 | 1329.0 | 393.67 | |
| 5 | 2061.9 | 1277.5 | 1316.8 | 1782.3 | 887.96 | 1763.4 | 789.18 | |
| [0/90/0/90] | 1 | 225.23 | 44.300 | 62.334 | 446.17 | 82.850 | 388.20 | 80.604 |
| 2 | 570.08 | 169.93 | 352.15 | 766.94 | 83.946 | 757.90 | 82.550 | |
| 3 | 955.03 | 493.94 | 721.36 | 864.92 | 165.59 | 781.68 | 155.42 | |
| 4 | 1371.1 | 882.44 | 1018.6 | 1184.5 | 373.57 | 1146.2 | 332.75 | |
| 5 | 1760.7 | 1113.6 | 1118.4 | 1572.6 | 734.99 | 1541.4 | 663.31 | |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qin, B.; Zhao, X.; Liu, H.; Yu, Y.; Wang, Q. Free Vibration Analysis of Curved Laminated Composite Beams with Different Shapes, Lamination Schemes, and Boundary Conditions. Materials 2020, 13, 1010. https://doi.org/10.3390/ma13041010
Qin B, Zhao X, Liu H, Yu Y, Wang Q. Free Vibration Analysis of Curved Laminated Composite Beams with Different Shapes, Lamination Schemes, and Boundary Conditions. Materials. 2020; 13(4):1010. https://doi.org/10.3390/ma13041010
Chicago/Turabian StyleQin, Bin, Xing Zhao, Huifang Liu, Yongge Yu, and Qingshan Wang. 2020. "Free Vibration Analysis of Curved Laminated Composite Beams with Different Shapes, Lamination Schemes, and Boundary Conditions" Materials 13, no. 4: 1010. https://doi.org/10.3390/ma13041010
APA StyleQin, B., Zhao, X., Liu, H., Yu, Y., & Wang, Q. (2020). Free Vibration Analysis of Curved Laminated Composite Beams with Different Shapes, Lamination Schemes, and Boundary Conditions. Materials, 13(4), 1010. https://doi.org/10.3390/ma13041010







