Effect of the Drying Method of Pine and Beech Wood on Fracture Toughness and Shear Yield Stress
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
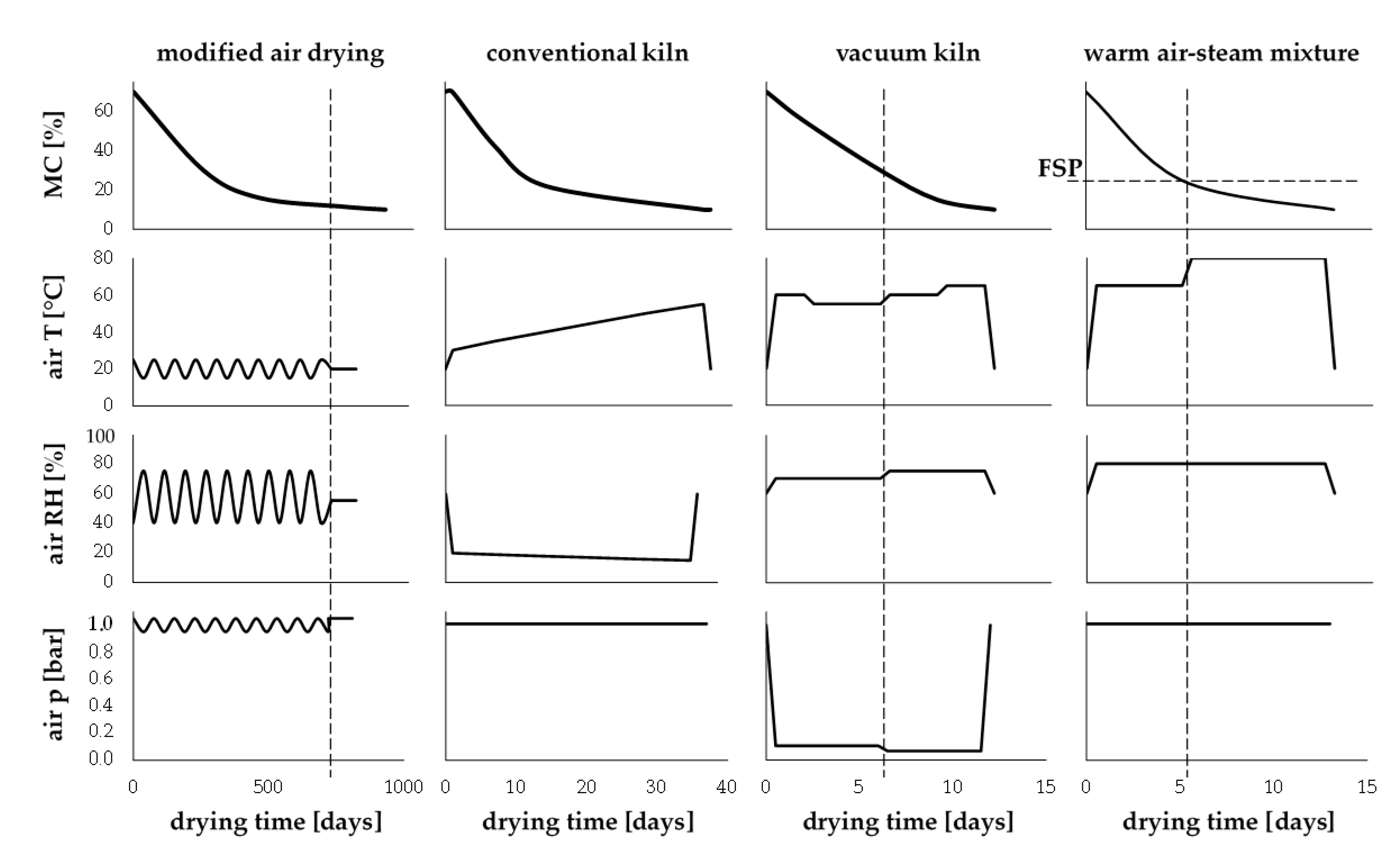
2.1.1. Modified Air-Drying Process
2.1.2. Conventional Kiln Drying Process
2.1.3. Vacuum Kiln Drying Process
2.1.4. Warm Air–Steam Mixture Experimental Drying Process
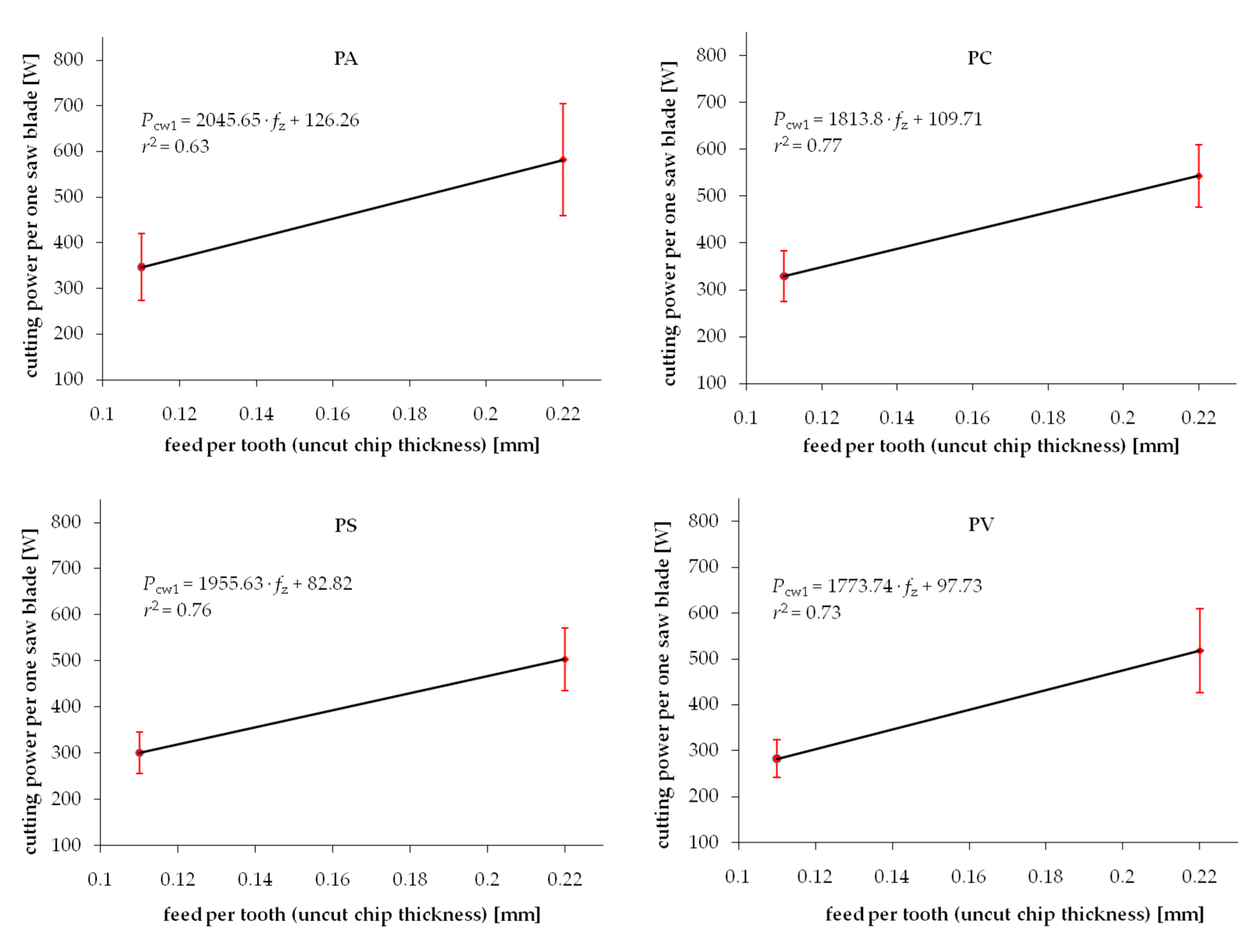
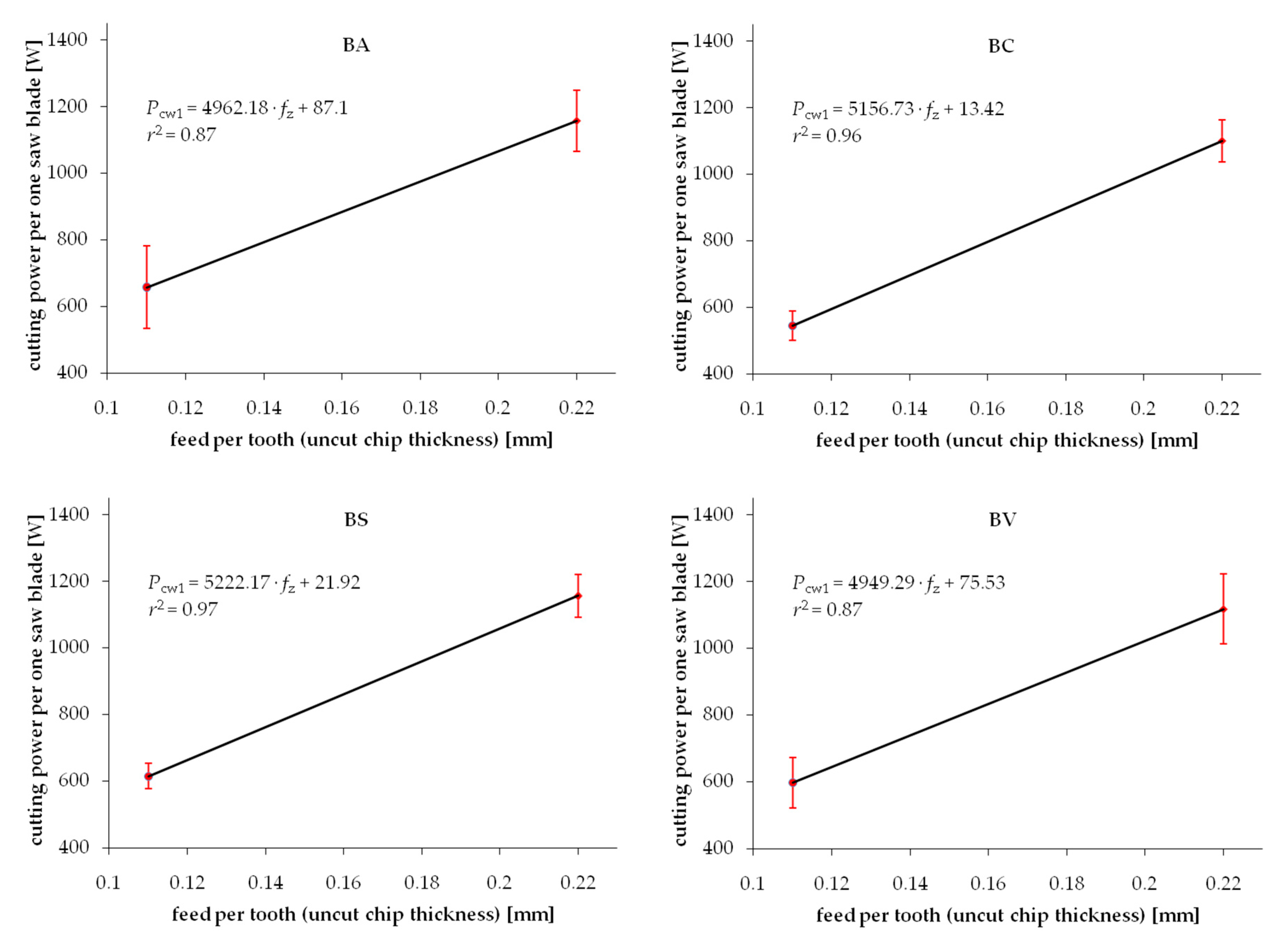
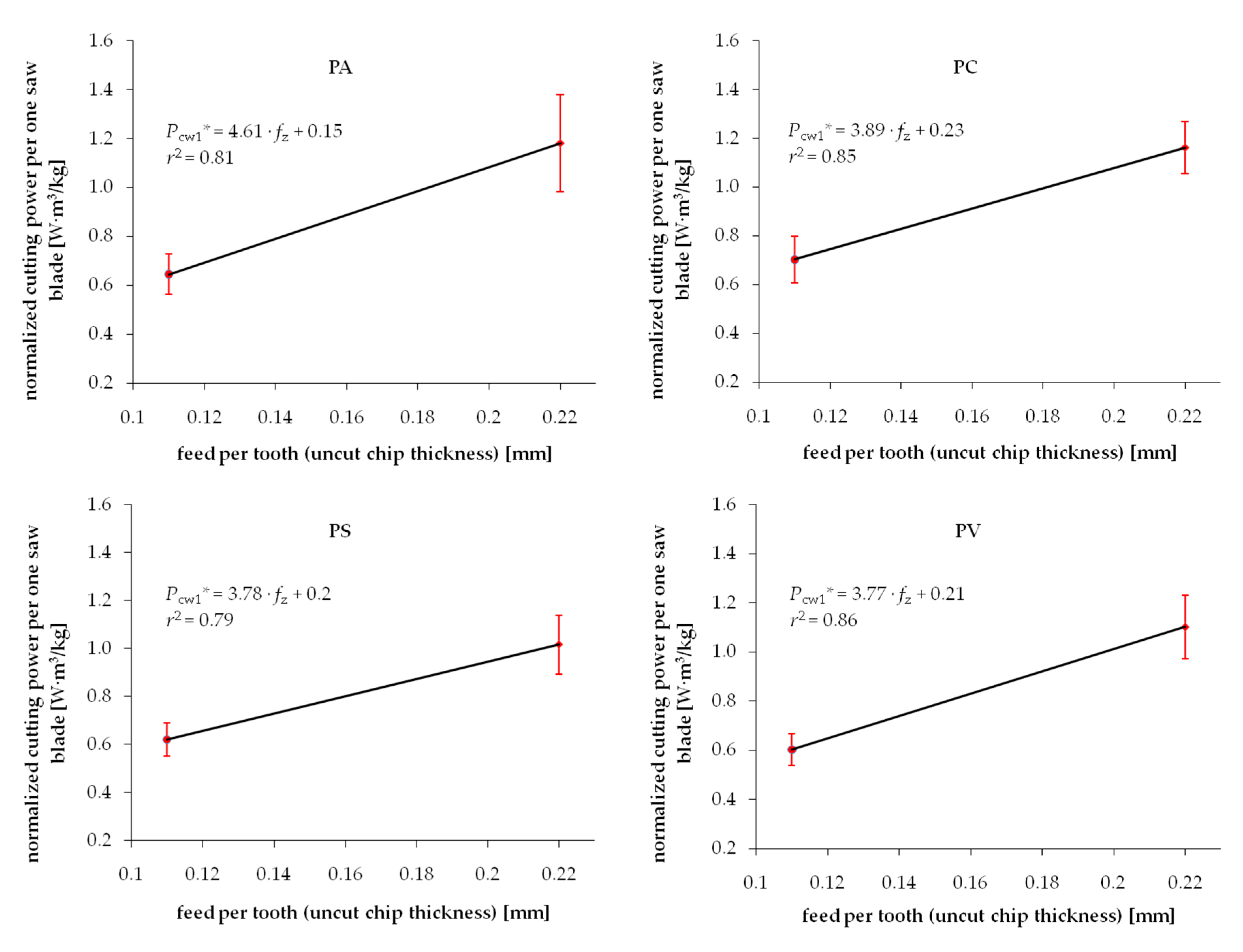
2.2. Machinability Tests
2.3. Methodology for Determination of Material Properties from the Cutting Test
- only freshly sharpened blades were used in cutting tests: component ❹ of Equation (5) = 0;
- chip acceleration power Pac is omitted: component ❸ of Equation (5) = 0;
- values of the shear angle Φc were calculated following the approach of Merchant [46], considering specific cutting zone geometry as well as coefficient of friction. This procedure is valid when deviations in the shear angle assigned to inherent material properties can be neglected for larger values of uncut chip thicknesses, as used in this experiment;
- the value of friction coefficient µ= 0.6 was adopted following the work of Glass and Zielinka [49];
- the effect of lateral forces on the power consumption can be omitted when cutting in straight direction and with minimal saw deviation [50].
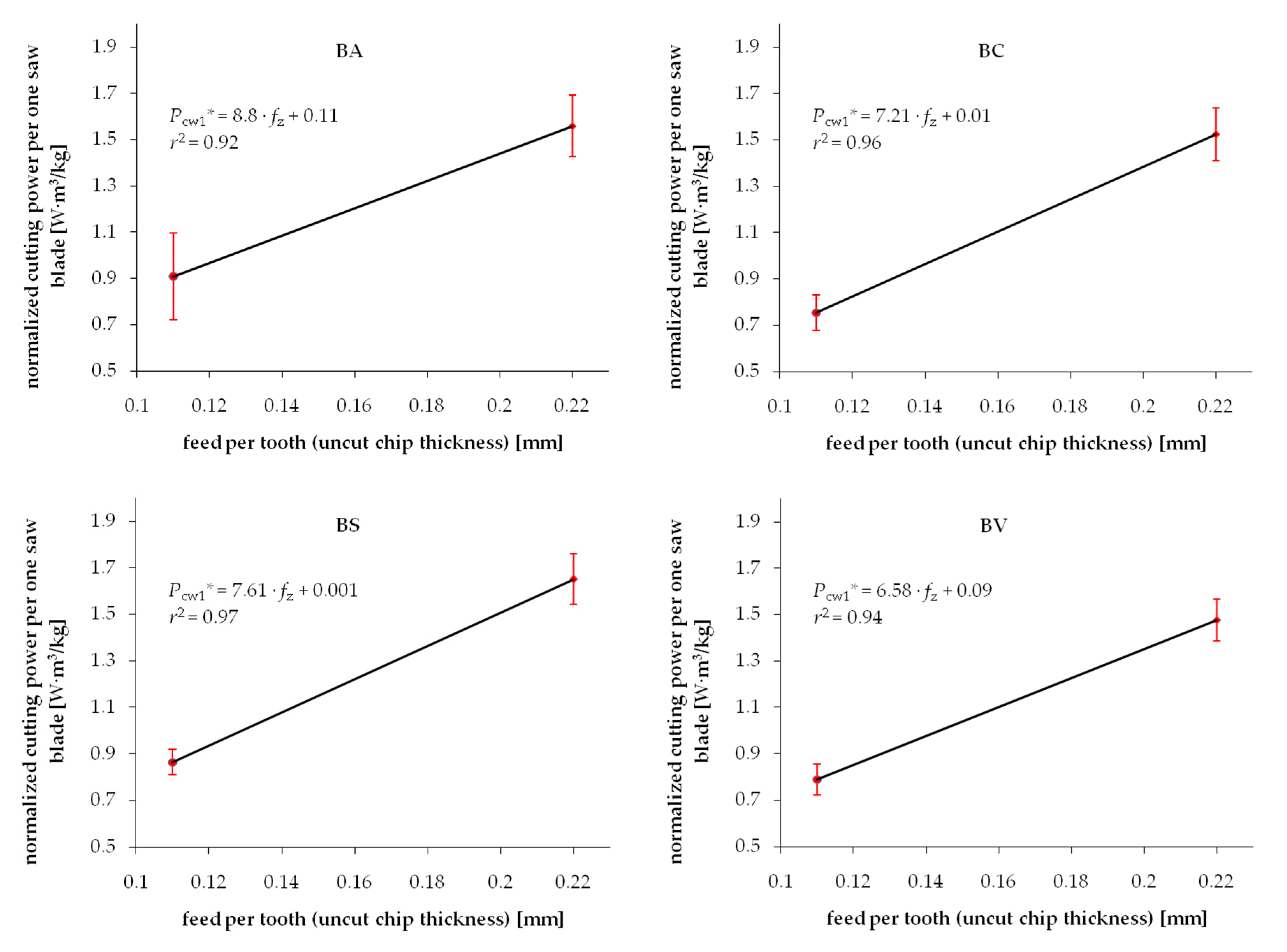
3. Results and Discussion
4. Conclusions
- The wood machining process on the frame saws can be adopted for straightforward determination of the fracture properties of materials by monitoring the power needed for cutting.
- Wood density is an important factor affecting the mechanism of the chip formation and should, therefore, be considered when interpreting cutting test results. A straightforward way for the results normalization was presented in this report.
- Technique of wood drying affects the physical-chemical properties that are revealed as a change of the fracture toughness and shear yield stress derived from the cutting tests.
- The observed trend of fracture properties changes was different for examined softwood (Scots pine) and hardwood (beech). Statistically significant differences (α = 0.05) between the determined mechanical properties of wood dried by four different methods were observed only for beech. It is related to differences in microstructure of both species and diverse deterioration mechanisms when exposing wood to elevated temperatures.
- The methodology presented here can be highly useful for accurate forecasting of the cutting power that is indispensable to optimize construction of woodworking machines and tools.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
List of Symbols
| BA BC BV BS | sample codes for beech wood | St | overall set (kerf width) |
| FSP | fiber saturation point | T | air temperature |
| HF | saw frame stroke | W | sample width |
| Hp | sample height | c0, c1 | coefficients of linear regression function |
| Lp | sample length | fz | feed per tooth |
| MC | moisture content | h | uncut chip thickness |
| MOE | modulus of elasticity | m | saws number |
| MOR | modulus of rupture | nF | number of strokes of saw frame per min |
| Pac | power needed for acceleration of chips | p | air pressure |
| Pc | average cutting power | tc | cutting time |
| PcT | overall cutting power | tp | tooth pitch |
| Pcw | average cutting power in a working stroke | vc | cutting speed |
| P*cw | average cutting power in a working stroke normalized by density | vf | feed speed |
| Pdull | power needed to chip formation while dullness of the cutting edge | za | number of teeth in contact with the kerf (average) |
| Pi | average idle power | βμ | friction angle |
| PT | mean total power | γ | shear strain along the shear plane |
| PA PC PV PS | sample codes for pine wood | γf | rake angle |
| Q | coefficient of friction correction | μ | friction coefficient |
| R | fracture toughness | ρ | density |
| R⊥ | fracture toughness in perpendicular direction | τγ | shear yield stress |
| R⊥* | fracture toughness in perpendicular direction normalized by density | τγ⊥ | shear yield stress in perpendicular direction |
| Rc | relative/compressive strength | τ*γ⊥ | shear yield stress in perpendicular direction normalized by density |
| RH | relative humidity | Φc | shear angle |
References
- Williamson, C. The energy sector: A hidden goliath. In Primary Wood Processing, 2nd ed.; Walker, J.C.F., Ed.; Springer Science and Business Media B.V.: Dordrecht, The Netherlands, 2006; p. 596. [Google Scholar]
- Bond, B.H.; Espinoza, O. A Decade of Improved Lumber Drying Technology. Curr. For. Rep. 2016, 2, 106–118. [Google Scholar] [CrossRef]
- Espinoza, O.; Bond, B.H. Vacuum Drying of Wood—State of the Art. Curr. For. Rep. 2016, 2, 223–235. [Google Scholar] [CrossRef]
- Allegretti, O.; Rémond, R.; Perré, P. Nonsymmetrical drying tests—Experimental and numerical results for free and constrained spruce samples. Dry. Technol. 2018, 36, 1554–1562. [Google Scholar] [CrossRef]
- He, Z.; Yang, F.; Peng, Y.; Yi, S. Ultrasound—Assisted vacuum drying of wood: Effects on drying time and product quality. BioResources 2013, 8, 855–863. [Google Scholar] [CrossRef]
- Zhang, J.-W.; Liu, H.-H.; Yang, H.; Yang, L. Drying Characteristics of Eucalyptus urophylla × E. grandis with Supercritical CO2. Materials 2020, 13, 3989. [Google Scholar] [CrossRef]
- Herrera-Díaz, R.; Sepúlveda-Villarroel, V.; Pérez-Peña, N.; Salvo-Sepúlveda, L.; Salinas-Lira, C.; Llano-Ponte, R.; Ananias, R.A. Effect of wood drying and heat modification on some physical and mechanical properties of radiata pine. Dry. Technol. 2017, 36, 537–544. [Google Scholar] [CrossRef]
- Oltean, L.; Teischinger, A.; Hansmann, C. Influence of low and moderate temperature kiln drying schedules on specific mechanical properties of Norway spruce wood. Holz Als Roh Werkst. 2010, 69, 451–457. [Google Scholar] [CrossRef]
- Roszyk, E.; Stachowska, E.; Majka, J.; Mania, P.; Broda, M. Moisture-Dependent Strength Properties of Thermally-Modified Fraxinus excelsior Wood in Compression. Materials 2020, 13, 1647. [Google Scholar] [CrossRef]
- Borrega, M.; Kärenlampi, P.P. Three mechanisms affecting the mechanical properties of spruce wood dried at high temperatures. J. Wood Sci. 2009, 56, 87–94. [Google Scholar] [CrossRef]
- Ayadi, R.; Koubaa, A.; Braghiroli, F.L.; Migneault, S.; Wang, H.; Bradai, C. Effect of the Pyro-Gasification Temperature of Wood on the Physical and Mechanical Properties of Biochar-Polymer Biocomposites. Materials 2020, 13, 1327. [Google Scholar] [CrossRef]
- Bekhta, P.; Sedliacik, J.; Bekhta, N. Effect of Veneer-Drying Temperature on Selected Properties and Formaldehyde Emission of Birch Plywood. Polymers 2020, 12, 593. [Google Scholar] [CrossRef]
- Bliem, P.; Konnerth, J.; Frybort, S.; Gartner, C.; Mauritz, R.; Van Herwijnen, H.W. Influence of drying and curing parameters on phenol-formaldehyde impregnated wood veneers. J. Adhes. 2019, 96, 253–271. [Google Scholar] [CrossRef]
- Klement, I.; Vilkovský, P.; Vilkovská, T. The Effect of Contact-Drying on Physical Properties of European Beech (Fagus sylvatica L.). Forests 2020, 11, 890. [Google Scholar] [CrossRef]
- Nakagawa, M.; Umemura, K.; Kawai, S.; Kanayama, K. Influence of drying temperature on NO2 sorption ability of cedar timber. J. Wood Sci. 2020, 66, 1–9. [Google Scholar] [CrossRef]
- Vitas, S.; Segmehl, J.S.; Burgert, I.; Cabane, E. Porosity and Pore Size Distribution of Native and Delignified Beech Wood Determined by Mercury Intrusion Porosimetry. Materials 2019, 12, 416. [Google Scholar] [CrossRef] [PubMed]
- Arantes, A.K.; De Conti, C.; Sturaro, S.M.E.; De Conti, A.C. Effect of Drying Methods in the Resistance Strength Compression Parallel Test for Bamboo. Adv. Mater. Res. 2015, 1088, 664–668. [Google Scholar] [CrossRef]
- Fonseca, E.; Barreira, L. Experimental and Numerical Method for Determining Wood Char-Layer at High Temperatures Due to An Anaerobic Heating. Int. J. Saf. Secur. Eng. 2011, 1, 65–76. [Google Scholar] [CrossRef]
- Fonseca, E.; Coelho, D.; Barreira, L. Structural safety in wooden beams under thermal and mechanical loading conditions. Int. J. Saf. Secur. Eng. 2012, 2, 242–255. [Google Scholar] [CrossRef][Green Version]
- Allegretti, O.; Brunetti, M.; Cuccui, I.; Ferrari, S.; Nocetti, M.; Terziev, N. Thermo—Vacuum modification of spruce (Picea abies Karst.) and fir (Abies alba Mill.) wood. BioResources 2012, 7, 3656–3669. [Google Scholar]
- Castro, G.; Rosso, L.; Allegretti, O.; Cuccui, I.; Cremonini, C.; Negro, F.; Zanuttini, R. Influence of thermo-vacuum treatment on bending properties of poplar rotary-cut veneer. iForest Biogeosci. For. 2017, 10, 161–163. [Google Scholar] [CrossRef]
- Blanchet, P.; Kaboorani, A.; Bustos, C. Understanding Effects of Drying Methods on Wood Mechanical Properties at Ultra and Cellular Levels. Wood Fiber Sci. 2016, 48, 1–12. [Google Scholar]
- Báder, M.; Németh, R.; Sandak, J.; Sandak, A. FTIR analysis of chemical changes in wood induced by steaming and longitudinal compression. Cellulose 2020, 27, 1–19. [Google Scholar] [CrossRef]
- Nge, T.T.; Tobimatsu, Y.; Yamamura, M.; Takahashi, S.; Takata, E.; Umezawa, T.; Yamada, T. Effect of Heat Treatment on the Chemical Structure and Thermal Properties of Softwood-Derived Glycol Lignin. Molecules 2020, 25, 1167. [Google Scholar] [CrossRef] [PubMed]
- Sandak, J.; Sandak, A.; Allegretti, O. Chemical Changes to Woody Polymers Due to High-Temperature Thermal Treatment Assessed with near Infrared Spectroscopy. J. Near Infrared Spectrosc. 2016, 24, 555–562. [Google Scholar] [CrossRef]
- Vidholdová, Z.; Sandak, A.; Sandak, J. Assessment of the chemical change in heat treated pine wood by near infrared spectroscopy. Acta Fac. Xylologiae 2019, 61, 31–42. [Google Scholar] [CrossRef]
- Sandak, A.; Allegretti, O.; Cuccui, I.; Rosso, L.; Castro, G.; Negro, F.; Cremonini, C.; Zanuttini, R. Thermo-Vacuum Modification of Poplar Veneers and its Quality Control. Bioresources 2016, 11, 10122–10139. [Google Scholar] [CrossRef]
- Očkajová, A.; Barcík, Š.; Kučerka, M.; Koleda, P.; Korčok, M.; Vyhnáliková, Z. Wood dust granular analysis in the sanding process of thermally modified wood versus its density. BioResources 2019, 14, 8559–8572. [Google Scholar] [CrossRef]
- Očkajová, A.; Kučerka, M.; Kminiak, R.; Krišťák, L.; Igaz, R.; Réh, R. Occupational Exposure to Dust Produced when Milling Thermally Modified Wood. Int. J. Environ. Res. Public Heal. 2020, 17, 1478. [Google Scholar] [CrossRef]
- Očkajová, A.; Kučerka, M.; Kminiak, R.; Rogoziński, T. Granulometric composition of chips and dust produced from the process of working thermally modified wood. Acta Fac. Xylologiae 2020, 62, 103–111. [Google Scholar] [CrossRef]
- Orłowski, K.A.; Chuchała, D.; Muziński, T.; Barański, J.; Banski, A.; Rogoziński, T. The effect of wood drying method on the granularity of sawdust obtained during the sawing process using the frame sawing machine. Acta Fac. Xylologiae 2019, 61, 83–92. [Google Scholar] [CrossRef]
- Nasir, V.; Cool, J. A review on wood machining: Characterization, optimization, and monitoring of the sawing process. Wood Mater. Sci. Eng. 2018, 15, 1–16. [Google Scholar] [CrossRef]
- Nasir, V.; Cool, J. Characterization, optimization, and acoustic emission monitoring of airborne dust emission during wood sawing. Int. J. Adv. Manuf. Technol. 2020, 109, 2365–2375. [Google Scholar] [CrossRef]
- Nadolny, K.; Kapłonek, W.; Sutowska, M.; Sutowski, P.; Myslinski, P.; Gilewicz, A. Experimental Studies on Durability of PVD-Based CrCN/CrN-Coated Cutting Blade of Planer Knives Used in the Pine Wood Planing Process. Materials 2020, 13, 2398. [Google Scholar] [CrossRef] [PubMed]
- Orlowski, K.A.; Ochrymiuk, T.; Atkins, A.; Chuchala, D. Application of fracture mechanics for energetic effects predictions while wood sawing. Wood Sci. Technol. 2013, 47, 949–963. [Google Scholar] [CrossRef]
- Orlowski, K.; Ochrymiuk, T.; Atkins, A. An Innovative Approach to the Forecasting of Energetic Effects While Wood Sawing. Drv. Ind. 2014, 65, 273–281. [Google Scholar] [CrossRef]
- Orlowski, K.A.; Ochrymiuk, T.; Sandak, J.; Sandak, J. Estimation of fracture toughness and shear yield stress of orthotropic materials in cutting with rotating tools. Eng. Fract. Mech. 2017, 178, 433–444. [Google Scholar] [CrossRef]
- Orlowski, K.A.; Ochrymiuk, T.; Hlásková, L.; Chuchala, D.; Kopecky, Z. Revisiting the estimation of cutting power with different energetic methods while sawing soft and hard woods on the circular sawing machine: A Central European case. Wood Sci. Technol. 2020, 54, 457–477. [Google Scholar] [CrossRef]
- Chuchala, D.; Ochrymiuk, T.; Orlowski, K.A.; Lackowski, M.; Taube, P. Predicting cutting power for band sawing process of pine and beech wood dried with the use of four different methods. Bioresources 2020, 15, 1844–1860. [Google Scholar] [CrossRef]
- Hlásková, L.; Orlowski, K.A.; Kopecký, Z.; Sviták, M.; Ochrymiuk, T. Fracture toughness and shear yield strength determination for two selected species of central European Provenance. Bioresources 2018, 13, 6171–6186. [Google Scholar] [CrossRef]
- Sinn, G.; Chuchała, D.; Orlowski, K.A.; Taube, P. Cutting model parameters from frame sawing of natural and impregnated Scots pine (Pinus sylvestris L.). Holz Als Roh Werkst. 2020, 78, 1–8. [Google Scholar] [CrossRef]
- Baranski, J. Moisture content during and after high- and normal-temperature drying processes of wood. Dry. Technol. 2017, 36, 751–761. [Google Scholar] [CrossRef]
- Barański, J.; Klement, I.; Vilkovská, T.; Konopka, A. High Temperature Drying Process of Beech Wood (Fagus sylvatica L.) with Different Zones of Sapwood and Red False Heartwood. Bioresources 2017, 12, 1861–1870. [Google Scholar] [CrossRef]
- Klement, I.; Vilkovská, T.; Baranski, J.; Konopka, A. The impact of drying and steaming processes on surface color changes of tension and normal beech wood. Dry. Technol. 2018, 37, 1490–1497. [Google Scholar] [CrossRef]
- Wasielewski, R.; Orlowski, K.A. Hybrid dynamically balanced saw frame drive. Holz Als Roh Werkst. 2002, 60, 202–206. [Google Scholar] [CrossRef]
- Orlowski, K.A.; Pałubicki, B. Recent progress in research on the cutting processes of wood. A review COST Action E35 2004–2008: Wood machining—Micromechanics and fracture. Holzforschung 2009, 63, 181–185. [Google Scholar] [CrossRef]
- Atkins, A. Modelling metal cutting using modern ductile fracture mechanics: Quantitative explanations for some longstanding problems. Int. J. Mech. Sci. 2003, 45, 373–396. [Google Scholar] [CrossRef]
- Atkins, A. Toughness and cutting: A new way of simultaneously determining ductile fracture toughness and strength. Eng. Fract. Mech. 2005, 72, 849–860. [Google Scholar] [CrossRef]
- Glass, S.V.; Zelinka, S.L. Moisture Relations and Physical Properties of Wood. In Wood Handbook—Wood as an Engineering Material; Centennial, Ed.; General Technical Report FPL-GTR-190; Department of Agriculture, Forest Service, Forest Products Laboratory: Madison, WI, USA, 2010; p. 508. [Google Scholar]
- Mohammadpanah, A.; Hutton, S.G. Dynamics Behavior of a Guided Spline Spinning Disk, Subjected to Conservative In-Plane Edge Loads, Analytical and Experimental Investigation. J. Vib. Acoust. 2016, 138, 041005. [Google Scholar] [CrossRef]
- Hlásková, L.; Kopecký, Z.; Solař, A.; Patočka, Z.; Mendel University. Cutting test as source of fracture toughness and shear yield strength for axial-perpendicular model of wood cutting. Wood Fiber Sci. 2019, 51, 58–68. [Google Scholar] [CrossRef]
- Kivimaa, E. Die Schnittkraft in der Holzbearbeitung. Holz Als Roh Werkst. 1952, 10, 94–108. [Google Scholar] [CrossRef]
- Orlicz, T. Obróbka Drewna Narzędziami Tnącymi, (in Polish: Wood Processing With Cutting Tools), 6th ed.; Skrypty SGGW-AR w Warszawie, Wydawnictwo SGGW-AR: Warszawa, Poland, 1988; p. 503. [Google Scholar]
- Kminiak, R.; Gaff, M. Roughness of Surface Created by Transversal Sawing of Spruce, Beech, and Oak Wood. Bioresources 2015, 10, 2873. [Google Scholar] [CrossRef]
- Stasiak, M.; Molenda, M.; Bańda, M.; Horabik, J.; Wiącek, J.; Parafiniuk, P.; Wajs, J.; Gancarz, M.; Gondek, E.; Lisowski, A.; et al. Friction and Shear Properties of Pine Biomass and Pellets. Materials 2020, 13, 3567. [Google Scholar] [CrossRef] [PubMed]
- Chuchala, D.; Orlowski, K.A.; Sandak, A.; Sandak, J.; Pauliny, D.; Barański, J. The Effect of Wood Provenance and Density on Cutting Forces While Sawing Scots Pine (Pinus sylvestris L.). Bioresources 2014, 9, 5349–5361. [Google Scholar] [CrossRef]
- Hermawan, A.; Sakagami, H.; Sobri, S.A.; Amini, M.H.M.; Ramle, S.F.M.; Rasid, S. The effects of drying temperatures on preservative retention and penetration of some Malaysian fast-growing species timbers. Dry. Technol. 2020, 1–10. [Google Scholar] [CrossRef]

| Sample Code | Drying Method | Wood Density ρ (Mean Value and Standard Deviation) [kg·m−3] |
|---|---|---|
| Pine Wood | ||
| PA | modified air drying | 515 ± 57.1 |
| PC | conventional kiln drying | 467 ± 26.4 |
| PS | heated-steam experimental drying | 490 ± 33.5 |
| PV | vacuum kiln drying | 474 ± 41.5 |
| Beech Wood | ||
| BA | modified air drying | 723 ± 52.0 |
| BC | conventional kiln drying | 722 ± 36.9 |
| BS | heated-steam experimental drying | 706 ± 25.4 |
| BV | vacuum kiln drying | 757 ± 38.5 |
| Parameter | Symbol | Value | Unit | |
|---|---|---|---|---|
| Machine Setting | ||||
| number of strokes of saw frame per min | nF | 685 | spm | |
| saw frame stroke | HF | 162 | mm | |
| number of saws in the gang | m | 5 | – | |
| average cutting speed | vc | 3.69 | m·s−1 | |
| feed speed | slow | vf1 | 0.9 | m·min−1 |
| fast | vf2 | 1.9 | m·min−1 | |
| feed per tooth | slow | fz1 | 0.11 | mm |
| fast | fz2 | 0.22 | mm | |
| Tool Setting | ||||
| the sharp saw blades with stellate tipped teeth | – | – | – | |
| overall set (kerf width) | St | 2 | mm | |
| saw blade thickness | s | 0.9 | mm | |
| free length of the saw blade | L0 | 318 | mm | |
| blade width | b | 30 | mm | |
| tooth pitch | tp | 13 | mm | |
| tool side rake angle | γf | 9 | ° | |
| tool side clearance | αf | 14 | ° | |
| tension stresses of saws in the gang | σN | 300 | MPa | |
| Sample Code | Drying Method | Normalized Fracture Toughness R*⊥ [J·m·kg−1] | Normalized Shear Yield Stress τ*γ⊥ [MPa·m3·kg−1] |
|---|---|---|---|
| Pine Wood | |||
| PA | air drying | 2.72 (±1.66) | 0.040 (±0.009) |
| PC | conventional kiln drying | 3.69 (±1.54) | 0.036 (±0.007) |
| PS | heated-steam experimental drying | 3.34 (±1.20) | 0.032 (±0.006) |
| PV | vacuum kiln drying | 3.59 (±1.08) | 0.034 (±0.006) |
| Beech Wood | |||
| BA | air drying | 2.42 (±0.65) | 0.062 (±0.003) |
| BC | conventional kiln drying | 1.08 (±0.40) | 0.066 (±0.003) |
| BS | heated-steam experimental drying | 0.39 (±0.21) | 0.068 (±0.002) |
| BV | vacuum kiln drying | 1.70 (±1.03) | 0.064 (±0.003) |
| Pine Wood | |||||||
|---|---|---|---|---|---|---|---|
| Sample Code | Source | DF | Adj SS | Adj MS | F-Value | p-Value | F-Critical |
| Fracture Toughness | |||||||
| PA | between groups | 3 | 4.1858 | 1.3953 | 0.7347 | 0.5404 | 2.9604 |
| PC | within groups | 27 | 51.2738 | 1.8990 | |||
| PS | total | 30 | 55.4596 | ||||
| PV | |||||||
| Shear Yield Stress | |||||||
| PA | between groups | 3 | 0.000285 | 9.51 × 10−5 | 1.9436 | 0.1464 | 2.9604 |
| PC | within groups | 27 | 0.001321 | 4.89 × 10−5 | |||
| PS | total | 30 | 0.001606 | ||||
| PV | |||||||
| Beech Wood | |||||||
| Fracture Toughness | |||||||
| BA | between groups | 3 | 14.2889 | 4.7630 | 9.6225 | 0.00026 | 3.0280 |
| BC | within groups | 23 | 11.3845 | 0.4950 | |||
| BS | total | 26 | 25.6734 | ||||
| BV | |||||||
| Shear Yield Stress | |||||||
| BA | between groups | 3 | 0.000134 | 4.46 × 10−5 | 3.1743 | 0.04333 | 3.0280 |
| BC | within groups | 23 | 0.000323 | 1.4 × 10−5 | |||
| BS | total | 26 | 0.000457 | ||||
| BV | |||||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chuchala, D.; Sandak, J.; Orlowski, K.A.; Muzinski, T.; Lackowski, M.; Ochrymiuk, T. Effect of the Drying Method of Pine and Beech Wood on Fracture Toughness and Shear Yield Stress. Materials 2020, 13, 4692. https://doi.org/10.3390/ma13204692
Chuchala D, Sandak J, Orlowski KA, Muzinski T, Lackowski M, Ochrymiuk T. Effect of the Drying Method of Pine and Beech Wood on Fracture Toughness and Shear Yield Stress. Materials. 2020; 13(20):4692. https://doi.org/10.3390/ma13204692
Chicago/Turabian StyleChuchala, Daniel, Jakub Sandak, Kazimierz A. Orlowski, Tomasz Muzinski, Marcin Lackowski, and Tomasz Ochrymiuk. 2020. "Effect of the Drying Method of Pine and Beech Wood on Fracture Toughness and Shear Yield Stress" Materials 13, no. 20: 4692. https://doi.org/10.3390/ma13204692
APA StyleChuchala, D., Sandak, J., Orlowski, K. A., Muzinski, T., Lackowski, M., & Ochrymiuk, T. (2020). Effect of the Drying Method of Pine and Beech Wood on Fracture Toughness and Shear Yield Stress. Materials, 13(20), 4692. https://doi.org/10.3390/ma13204692








