Addressing H-Material Interaction in Fast Diffusion Materials—A Feasibility Study on a Complex Phase Steel
Abstract
1. Introduction
2. Materials and Methods
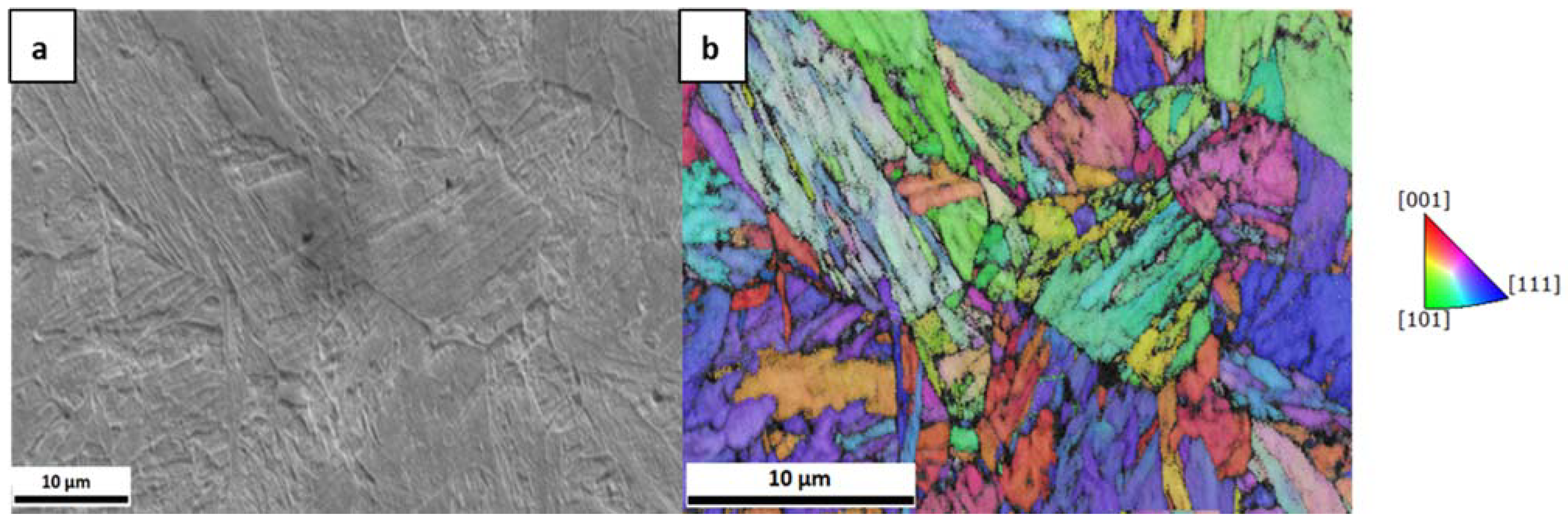
2.1. Material
2.2. Plasma H Charging
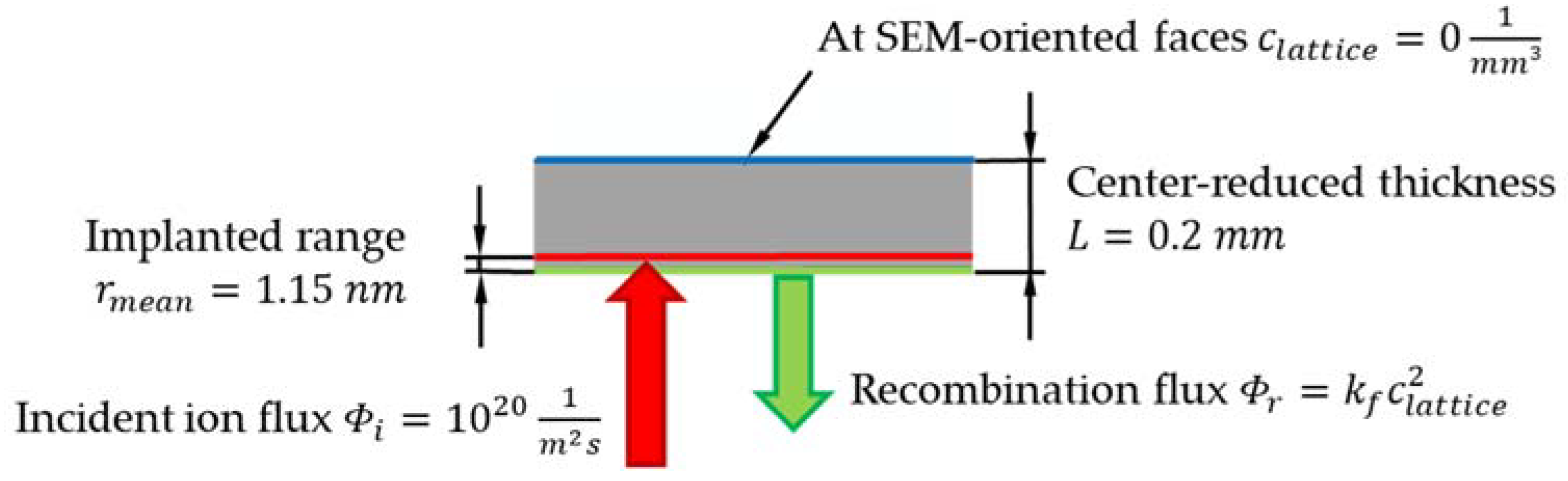
2.3. H Uptake and Diffusion Simulation
3. Results
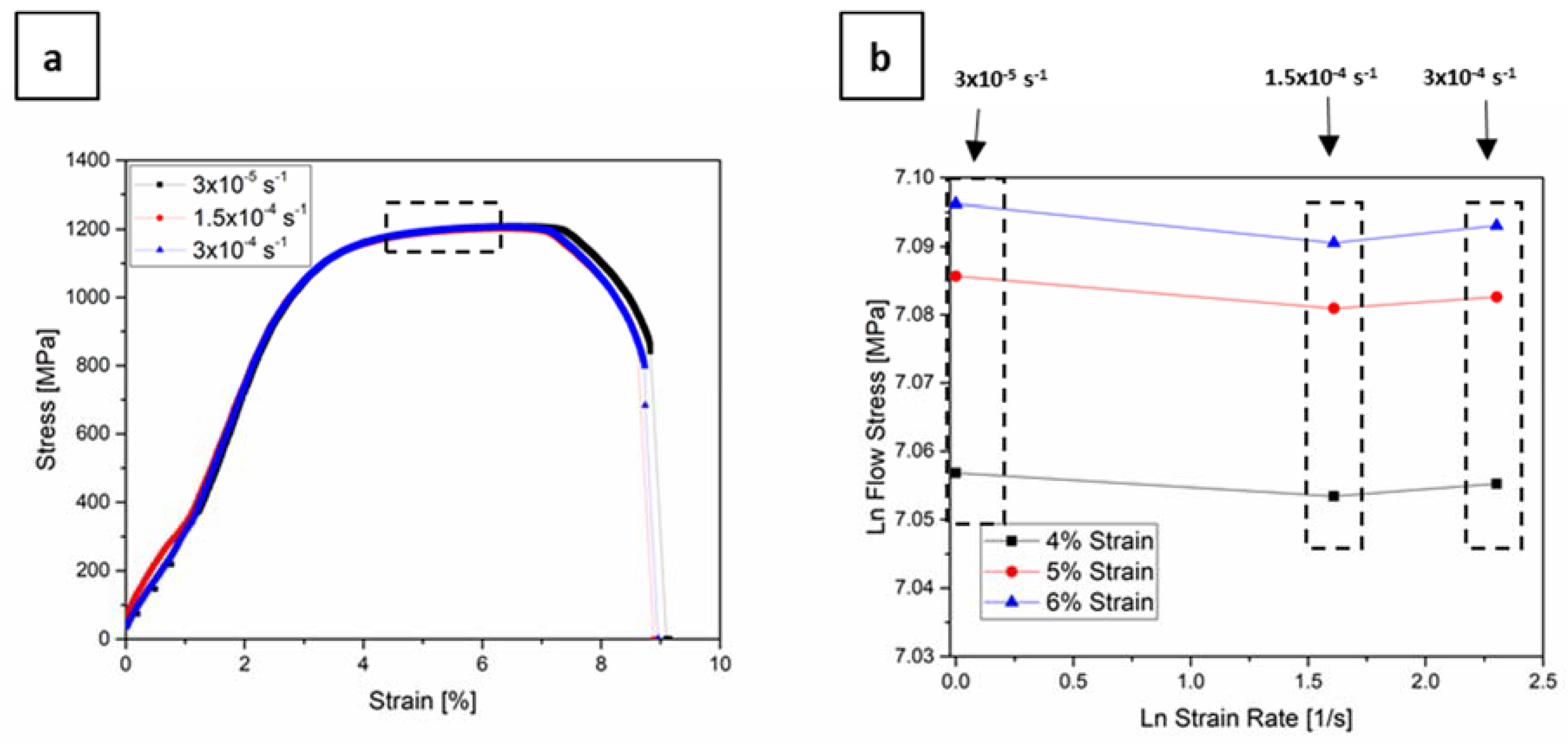
3.1. Rate Dependance of Flow Properties
3.2. Effect of H Charging
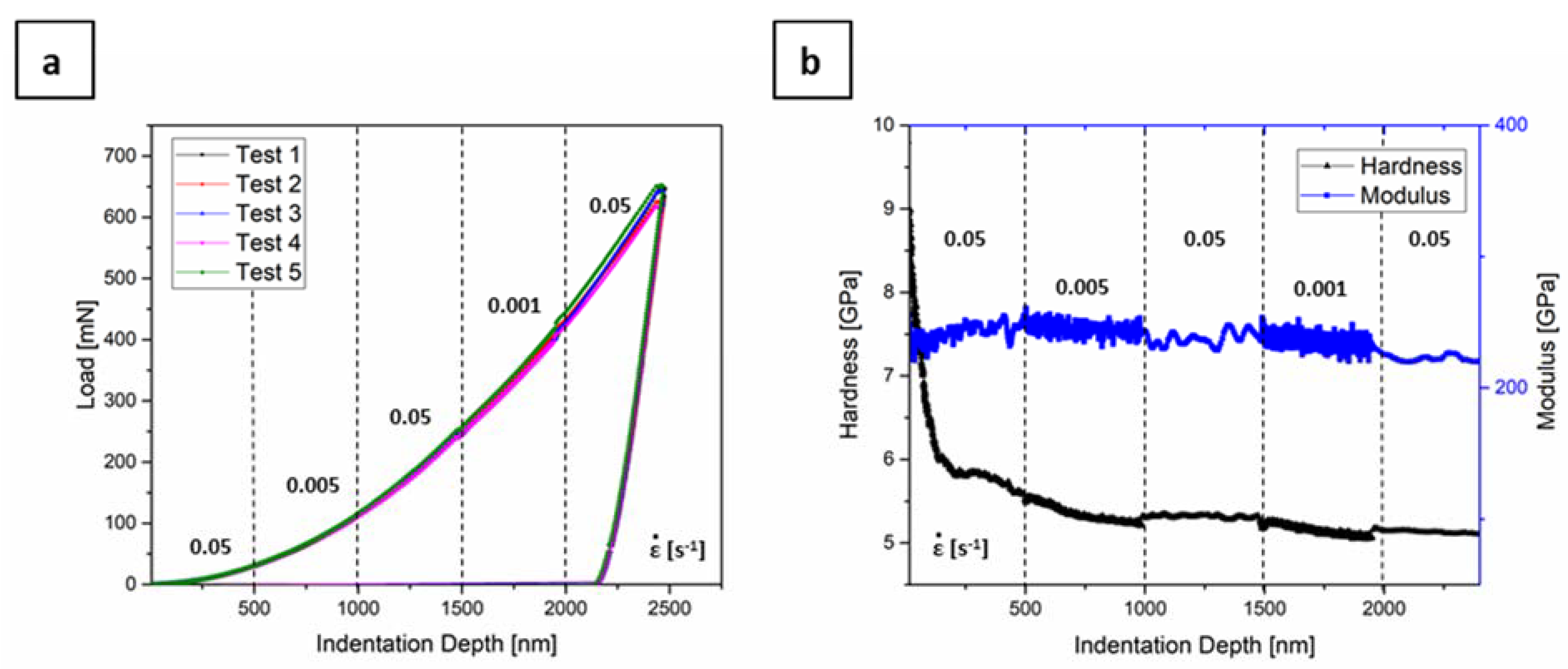
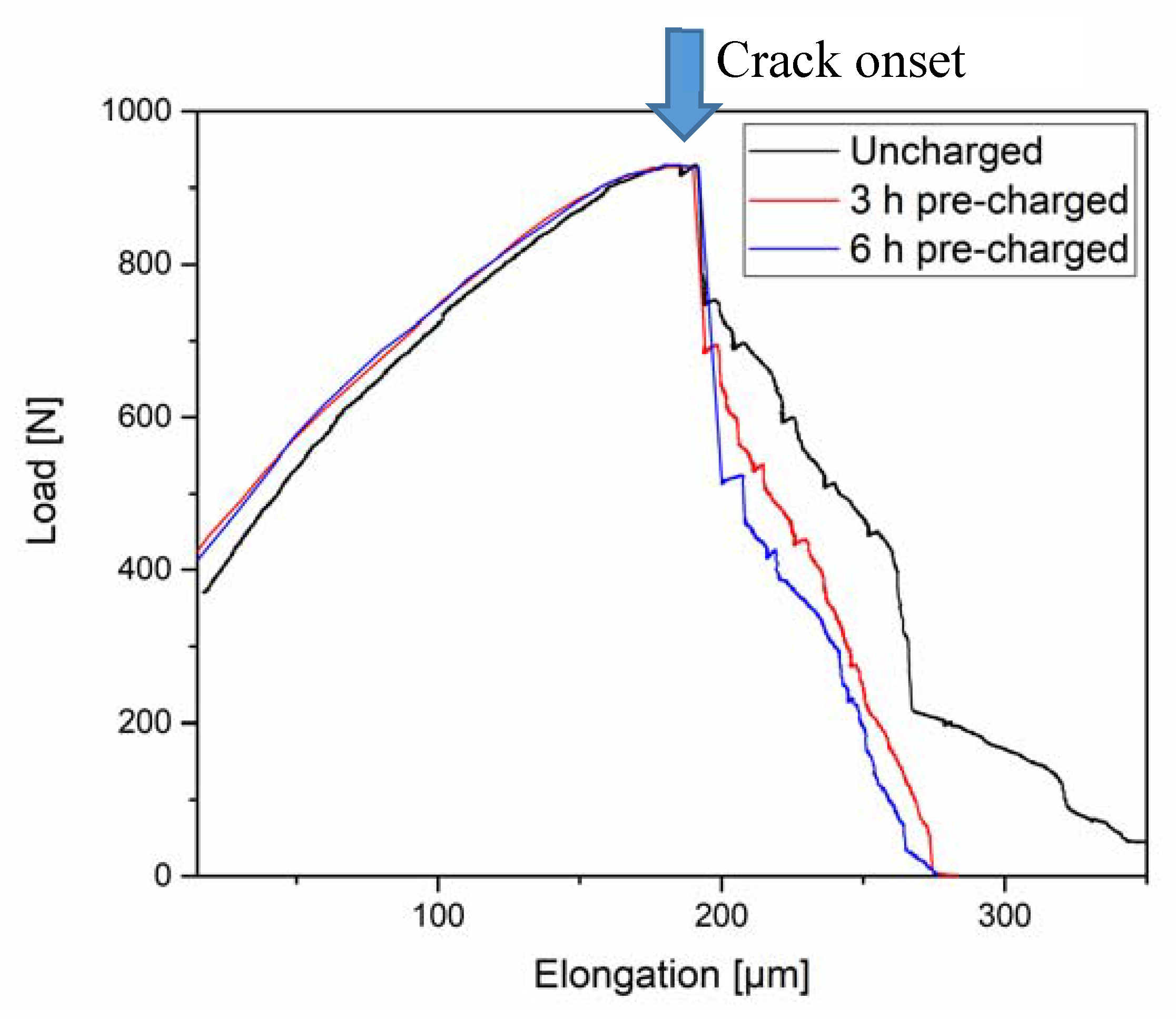
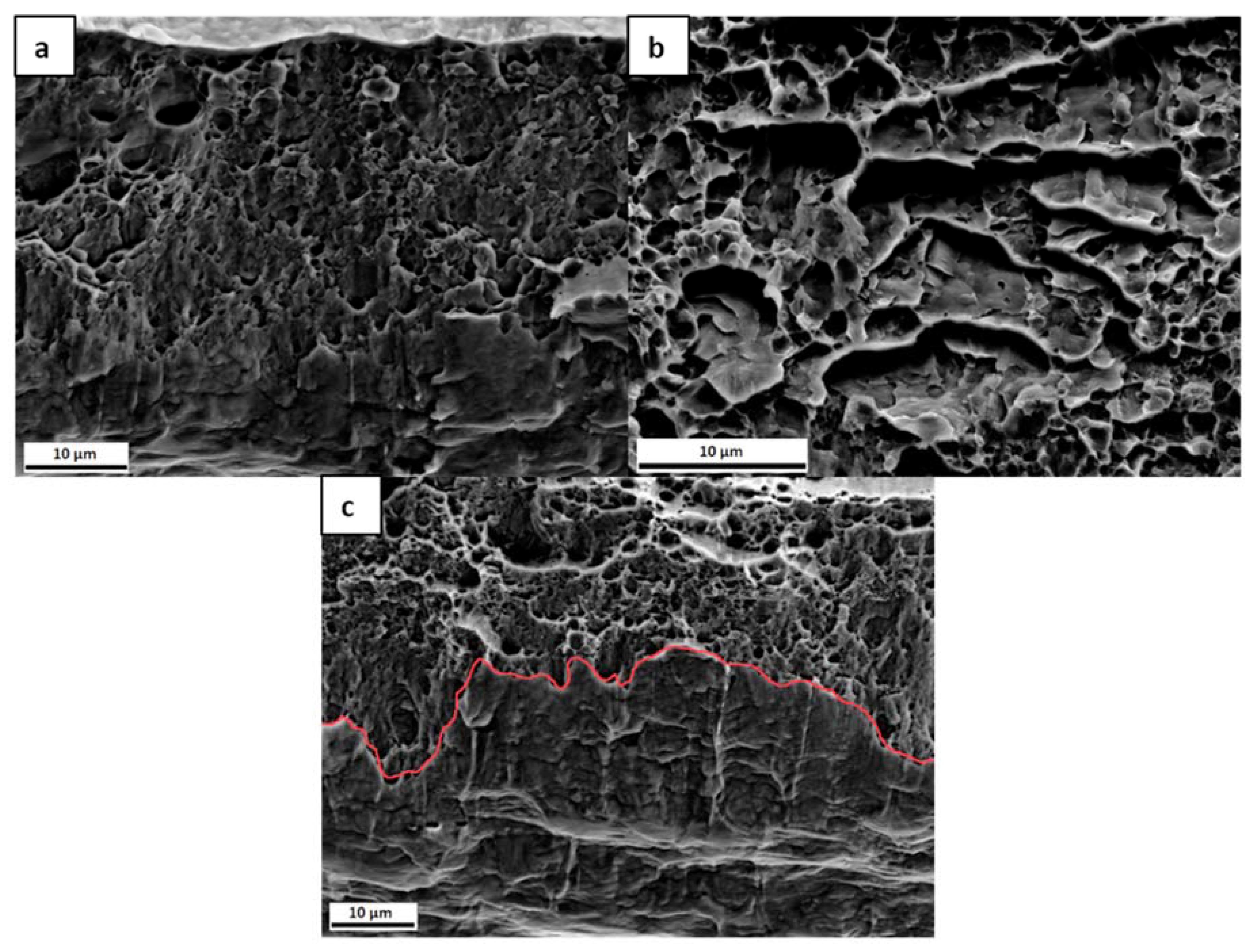
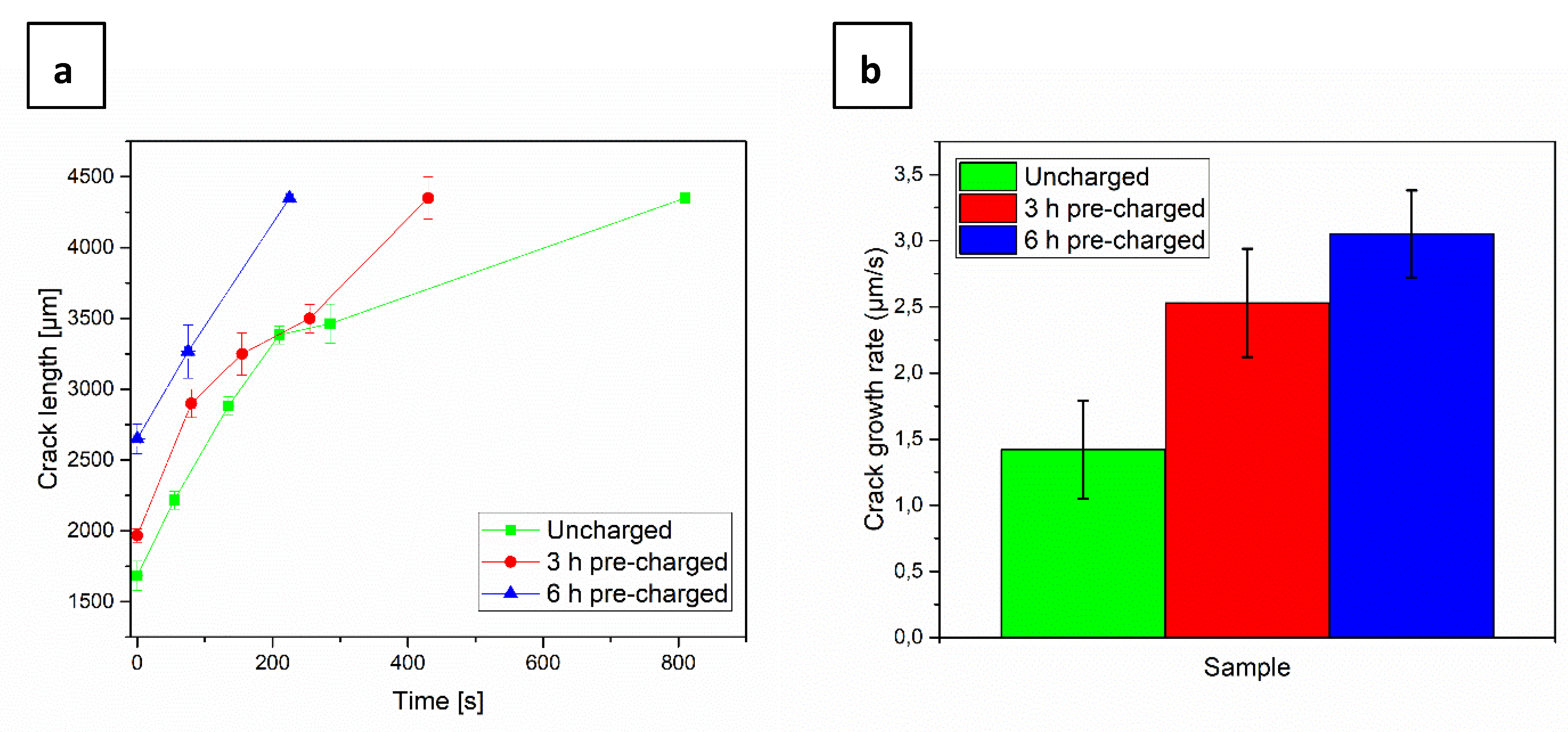
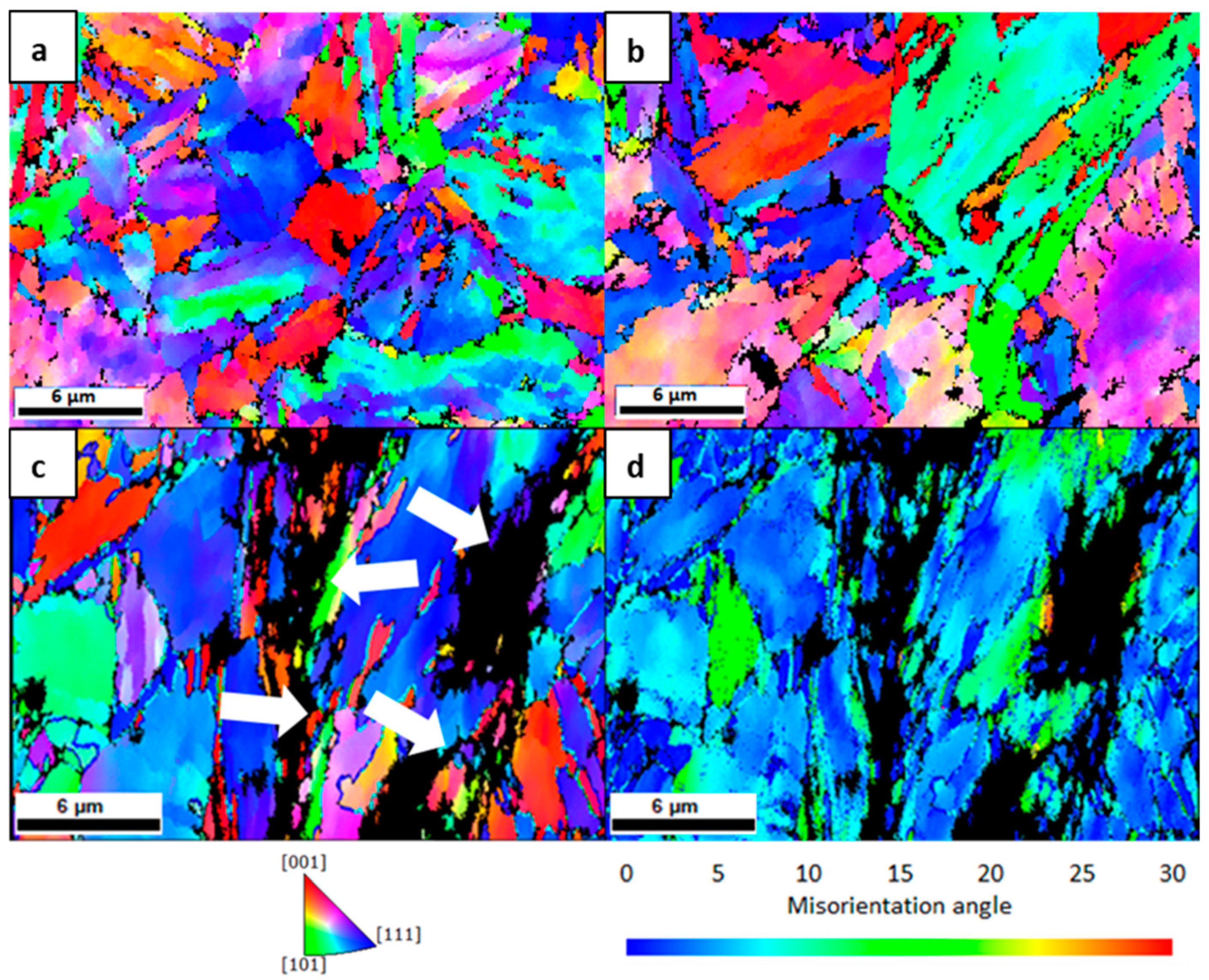
3.2.1. Effect of Pre-Charging Time
3.2.2. Effect of Plasma Parameters
3.2.3. Effect of H Charging-Discharging
3.3. H Concentration
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Matlock, D.K.; Speer, J.G.; De Moor, E.; Gibbs, P.J. Recent developments in advanced high strength sheet steels for automotive applications: An overview. Jestech. 2012, 15, 1–12. [Google Scholar]
- Bergmann, C.; Mraczek, K.; Kröger, B.; Sturel, T.; Jürgensen, J.; Yagodzinskyy, Y.; Guo, X.; Vucko, F.; Kuhlmann, M.; Veith, S.; et al. Hydrogen embrittlement resistance evaluation of advanced high strength steel for automotive applications. In Proceedings of the Steely Hydrogen, Ghent, Belgium, 29–31 May 2018; pp. 1–15. [Google Scholar]
- Eurostat. Available online: https://ec.europa.eu/eurostat/statistics-explained/index.php/Construction_production_(volume)_index_overview (accessed on 15 July 2020).
- Figueroa, D.; Robinson, M. The effects of sacrificial coatings on hydrogen embrittlement and re-embrittlement of ultra high strength steels. Corros. Sci. 2008, 50, 1066–1079. [Google Scholar] [CrossRef]
- Lovicu, G.; Bottazzi, M.; D’Aiuto, F.; De Sanctis, M.; DiMatteo, A.; Santus, C.; Valentini, R. Hydrogen Embrittlement of Automotive Advanced High-Strength Steels. Met. Mater. Trans. A 2012, 43, 4075–4087. [Google Scholar] [CrossRef]
- Louthan, M.R. Hydrogen Embrittlement of Metals: A Primer for the Failure Analyst. J. Fail. Anal. Prev. 2008, 8, 289–307. [Google Scholar] [CrossRef]
- Hilditch, T.B.; Lee, S.-B.; Speer, J.G.; Matlock, D.K. Response to Hydrogen Charging in High Strength Automotive Sheet Steel Products. Sae Tech. Pap. Ser. 2003, 47–56. [Google Scholar] [CrossRef]
- Gerberich, W.W.; Oriani, R.A.; Lji, M.-J.; Chen, X.; Foecke, T. The necessity of both plasticity and brittleness in the fracture thresholds of iron. Philos. Mag. A 1991, 63, 363–376. [Google Scholar] [CrossRef]
- Oriani, R.A.; Josephic, P.H. Equilibrium aspects of hydrogen-induced cracking in steels. Acta Metall. 1974, 22, 1065–1074. [Google Scholar] [CrossRef]
- Lynch, S. Environmentally assisted cracking: Overview of evidence for an adsorption-induced localised-slip process. Acta Met. 1988, 36, 2639–2661. [Google Scholar] [CrossRef]
- Lynch, S. Metallographic contributions to understanding mechanisms of environmentally assisted cracking. Metallography 1989, 23, 147–171. [Google Scholar] [CrossRef]
- Lynch, S.P. Comments on “A unified model of environment-assisted cracking. Scr. Mater. 2009, 61, 331–334. [Google Scholar] [CrossRef]
- Birnbaum, H.; Sofronis, P. Hydrogen-enhanced localized plasticity—A mechanism for hydrogen-related fracture. Mater. Sci. Eng. A 1994, 176, 191–202. [Google Scholar] [CrossRef]
- Robertson, I.; Birnbaum, H.; Sofronis, P. Chapter 91 Hydrogen Effects on Plasticity. Dislocat. Solids 2009, 15, 249–293. [Google Scholar]
- Birnbaum, H.K. Mechanisms of Hydrogen Related Fracture of Metals. In Mechanisms of Hydrogen Related Fracture of Metals; Defense Technical Information Center (DTIC): Urbana, IL, USA, 1989; pp. 639–658. [Google Scholar]
- Birnbaum, H.K.; Robertston, I.M.; Sofronis, P.; Teter, D. Mechanisms of hydrogen related fracture—A review. In Corrosion-Deformation Interactions; Trans Tech Publications: Zurich, Switzerland, 1996; pp. 172–195. [Google Scholar]
- Lynch, S. Hydrogen embrittlement phenomena and mechanisms. Corros. Rev. 2012, 30, 105–123. [Google Scholar] [CrossRef]
- Drexler, A.; Bergmann, C.; Manke, G.; Kokotin, V.; Mraczek, K.; Pohl, M.; Ecker, W. On the local evaluation of the hydrogen susceptibility of cold-formed and heat treated advanced high strength steel (AHSS) sheets. Mater. Sci. Eng. A. 2020, 800, 140276. [Google Scholar] [CrossRef]
- Drexler, A.; Ecker, W.; Winzer, N.; Mraczek, K.; Kokotin, V.; Manke, G.; Bergmann, C. A step towards numerical evaluation of the local hydrogen susceptibility of punched and cold-formed advanced high strength steel (AHSS) sheets. In Proceedings of the Steely Hydrogen, Gent, Belgium, 29–31 May 2018. [Google Scholar]
- Drexler, A.; Bergmann, C.; Manke, G.; Kokotin, V.; Mraczek, K.; Pohl, M.; Ecker, W. Local hydrogen accumulation after cold forming and heat treatment in punched advanced high strength steel sheets. J. Alloys Compd. 2020, in press. [Google Scholar]
- Murakami, Y.; Yokoyama, N.N.; Takai, K. Fatigue. Effect of Hydrogen Trapped by Inclusions on Ultra-Long Life Fatigue Failure of Bearing Steel. J. Soc. Mater. Sci. Jpn. 2001, 50, 1068–1073. [Google Scholar] [CrossRef]
- Todoshchenko, O.; Yagodzinskyy, Y.; Yagodzinska, V.; Saukkonen, T.; Hänninen, H. Hydrogen effects on fracture of high-strength steels with different micro-alloying. Corros. Rev. 2015, 33, 515–527. [Google Scholar] [CrossRef]
- Ravichandran, K.; Dwarakadasa, E. Kishore Some considerations on the occurrence of intergranular fracture during fatigue crack growth in steels. Mater. Sci. Eng. 1986, 83, L11–L16. [Google Scholar] [CrossRef]
- Venezuela, J.; Liu, Q.; Zhang, M.; Zhou, Q.; Atrens, A. A review of hydrogen embrittlement of martensitic advanced high-strength steels. Corros. Rev. 2016, 34, 153–186. [Google Scholar] [CrossRef]
- DePover, T.; Escobar, D.M.P.; Wallaert, E.; Zermout, Z.; Verbeken, K. Effect of hydrogen charging on the mechanical properties of advanced high strength steels. Int. J. Hydrogen Energy 2014, 39, 4647–4656. [Google Scholar] [CrossRef]
- DePover, T.; Wallaert, E.; Verbeken, K. Fractographic analysis of the role of hydrogen diffusion on the hydrogen embrittlement susceptibility of DP steel. Mater. Sci. Eng. A 2016, 649, 201–208. [Google Scholar] [CrossRef]
- McCoy, R.A.; Gerberich, W.W.; Zackay, V.F. On the resistance of TRIP steel to hydrogen embrittlement. Met. Mater. Trans. A 1970, 1, 2031–2034. [Google Scholar] [CrossRef][Green Version]
- Davies, R.G. Hydrogen embrittlement of dual-phase steels. Met. Mater. Trans. A 1981, 12, 1667–1672. [Google Scholar] [CrossRef]
- Duprez, L.; Verbeken, K.; Verhaege, M. Effect of hydrogen on the mechanical properties of multiphase high strength steels. In Proceedings of the 2008 International Hydrogen Conference: Effects of hydrogen on materials, Jackson, WY, USA, 7–10 September 2008; pp. 62–69. [Google Scholar]
- Ronevich, J.A.; Speer, J.G.; Matlock, D.K. Hydrogen Embrittlement of Commercially Produced Advanced High Strength Sheet Steels. Sae Int. J. Mater. Manuf. 2010, 3, 255–267. [Google Scholar] [CrossRef]
- DePover, T.; Laureys, A.; Escobar, D.P.; Eeckhout, E.V.D.; Wallaert, E.; Verbeken, K. Understanding the Interaction between a Steel Microstructure and Hydrogen. Materials 2018, 11, 698. [Google Scholar] [CrossRef] [PubMed]
- Malitckii, E.; Yagodzinskyy, Y.; Vilaҫa, P. Role of retained austenite in hydrogen trapping and hydrogen-assisted fatigue fracture of high-strength steels. Mater. Sci. Eng. A 2019, 760, 68–75. [Google Scholar] [CrossRef]
- Loidl, M.; Kolk, O.; Veith, S.; Gobel, T. Characterization of hydrogen embrittlement in automotive advanced high strength steels. Mater. Werkst. 2011, 42, 1105–1110. [Google Scholar] [CrossRef]
- Rehrl, J.; Mraczek, K.; Pichler, A.; Werner, E. Mechanical properties and fracture behavior of hydrogen charged AHSS/UHSS grades at high- and low strain rate tests. Mater. Sci. Eng. A 2014, 590, 360–367. [Google Scholar] [CrossRef]
- Bhadeshia, H.K.D.H. Prevention of Hydrogen Embrittlement in Steels. Isij Int. 2016, 56, 24–36. [Google Scholar] [CrossRef]
- Ebner, A.S.; Brinckmann, S.; Plesiutschnig, E.; Clemens, H.; Pippan, R.; Maier-Kiener, V. A Modified Electrochemical Nanoindentation Setup for Probing Hydrogen-Material Interaction Demonstrated on a Nickel-Based Alloy. JOM 2020, 72, 1–10. [Google Scholar] [CrossRef]
- Wan, D.; Deng, Y.; Meling, J.I.H.; Alvaro, A.; Barnoush, A. Hydrogen-enhanced fatigue crack growth in a single-edge notched tensile specimen under in-situ hydrogen charging inside an environmental scanning electron microscope. Acta Mater. 2019, 170, 87–99. [Google Scholar] [CrossRef]
- DePover, T.; Hajilou, T.; Wan, D.; Wang, D.; Barnoush, A.; Verbeken, K. Assessment of the potential of hydrogen plasma charging as compared to conventional electrochemical hydrogen charging on dual phase steel. Mater. Sci. Eng. A 2019, 754, 613–621. [Google Scholar] [CrossRef]
- Kim, J.; Tasan, C.C. Microstructural and micro-mechanical characterization during hydrogen charging: An in situ scanning electron microscopy study. Int. J. Hydrogen Energy 2019, 44, 6333–6343. [Google Scholar] [CrossRef]
- Massone, A.; Manhard, A.; Jacob, W.; Drexler, A.; Ecker, W.; Hohenwarter, A.; Wurster, S.; Kiener, D. An SEM compatible plasma cell for in situ studies of hydrogen-material interaction. Rev. Sci. Instrum. 2020, 91, 043705. [Google Scholar] [CrossRef] [PubMed]
- Maier, V.; Durst, K.; Mueller, J.; Backes, B.; Höppel, H.W.; Göken, M. Nanoindentation strain-rate jump tests for determining the local strain-rate sensitivity in nanocrystalline Ni and ultrafine-grained Al. J. Mater. Res. 2011, 26, 1421–1430. [Google Scholar] [CrossRef]
- Toh, T.; Baldwin, W.M. Ductility of steel with varying concentrations of hydrogen. In Stress Corrosion Cracking and Embrittlement; Robertson, W.D., Ed.; Wiley: New York, NY, USA, 1956; pp. 176–186. [Google Scholar]
- Shu, W.; Okuno, K.; Hayashi, Y. Ion-driven permeation of deuterium in metals. Jaeri-M. 1993, 43, 1–62. [Google Scholar]
- Mutzke, A.; Schneider, R.; Eckstein, W.; Dohmen, R.; Schmid, K.; von Toussaint, U.; Badelow, G. SDTrimSP Version 6.00 (IPP 2019-2); Max-Planck-Institut für Plasmaphysik: Garching, Germany, 2019; pp. 1–92. [Google Scholar]
- Zhou, H.; Hirooka, Y.; Ashikawa, N.; Muroga, T.; Sagara, A. Gas- and plasma-driven hydrogen permeation through a reduced activation ferritic steel alloy F82H. J. Nucl. Mater. 2014, 455, 470–474. [Google Scholar] [CrossRef]
- Baskes, M. A calculation of the surface recombination rate constant for hydrogen isotopes on metals. J. Nucl. Mater. 1980, 92, 318–324. [Google Scholar] [CrossRef]
- Barrera, O.; Tarleton, E.; Tang, H.; Cocks, A. Modelling the coupling between hydrogen diffusion and the mechanical behaviour of metals. Comput. Mater. Sci. 2016, 122, 219–228. [Google Scholar] [CrossRef]
- Fischer, F.; Mori, G.; Svoboda, J. Modelling the influence of trapping on hydrogen permeation in metals. Corros. Sci. 2013, 76, 382–389. [Google Scholar] [CrossRef]
- Dassault Systemes. ABAQUS User’s Manual; Dassault Systemes: Velizy-Villacoublay, France, 2016. [Google Scholar]
- Svoboda, J.; Fischer, F. Modelling for hydrogen diffusion in metals with traps revisited. Acta Mater. 2012, 60, 1211–1220. [Google Scholar] [CrossRef]
- Drexler, A.; Siegl, W.; Tkadletz, M.; Ecker, W.; Mori, G.; Svoboda, J.; Fischer, F.D. Cycled hydrogen permeation through Armco iron—A joint experimental and modeling approach. Corros. Sci. 2020, 176, 109017. [Google Scholar] [CrossRef]
- Svoboda, J.; Mori, G.; Prethaler, A.; Fischer, F. Determination of trapping parameters and the chemical diffusion coefficient from hydrogen permeation experiments. Corros. Sci. 2014, 82, 93–100. [Google Scholar] [CrossRef]
- Drexler, A.; DePover, T.; Verbeken, K.; Ecker, W. Model-based interpretation of thermal desorption spectra of Fe-C-Ti alloys. J. Alloys Compd. 2019, 789, 647–657. [Google Scholar] [CrossRef]
- Drexler, A.; DePover, T.; Leitner, S.; Verbeken, K.; Ecker, W. Microstructural based hydrogen diffusion and trapping models applied to Fe–C X alloys. J. Alloys Compd. 2020, 826, 154057. [Google Scholar] [CrossRef]
- Alturk, R.; Mates, S.; Xu, Z.; Abu-Farha, F. Effects of Microstructure on the Strain Rate Sensitivity of Advanced Steels. In Proceedings of the 3rd Pan American Materials Congress, San Diego, CA, USA, 26 February–2 March 2017; Springer Science and Business Media LLC: San Diego, CA, USA, 2017; pp. 243–254. [Google Scholar]
- Canale, L.C.; Yao, X.; Gu, J.; Totten, G. A historical overview of steel tempering parameters. Int. J. Microstruct. Mater. Prop. 2008, 3, 474. [Google Scholar] [CrossRef]
- Grange, R.A.; Hribal, C.R.; Porter, L.F. Hardness of tempered martensite in carbon and low-alloy steels. Met. Mater. Trans. A 1977, 8, 1775–1785. [Google Scholar] [CrossRef]
- Pharr, G.M.; Herbert, E.G.; Gao, Y. The Indentation Size Effect: A Critical Examination of Experimental Observations and Mechanistic Interpretations. Annu. Rev. Mater. Res. 2010, 40, 271–292. [Google Scholar] [CrossRef]
- Zakroczymski, T.; Glowacka, A.; Swiatnicki, W. Effect of hydrogen concentration on the embrittlement of a duplex stainless steel. Corros. Sci. 2005, 47, 1403–1414. [Google Scholar] [CrossRef]
- Selwyn, G.S. Optical diagnostic techniques for rie. In Proceedings of the Sixth Symposium on Plasma Processing, San Diego, CA, USA, 29–31 October 1986; Mathad, G.S., Schwartz, G.C., Gottscho, R.A., Eds.; Electrochemical Society: Pennington, NJ, USA, 1987; pp. 220–253. [Google Scholar]
- Lynch, S. Mechanistic and fractographic aspects of stress corrosion cracking. Corros. Rev. 2012, 30, 105–123. [Google Scholar] [CrossRef]
- Thompson, A.W. The behavior of sensitized 309S stainless steel in hydrogen. Mater. Sci. Eng. 1974, 14, 253–264. [Google Scholar] [CrossRef]
- Stenerud, G.; Wenner, S.; Olsen, J.S.; Johnsen, R. Effect of different microstructural features on the hydrogen embrittlement susceptibility of alloy 718. Int. J. Hydrogen Energy 2018, 43, 6765–6776. [Google Scholar] [CrossRef]
- Tiwari, G.; Bose, A.; Chakravartty, J.; Wadekar, S.; Totlani, M.; Arya, R.; Fotedar, R. A study of internal hydrogen embrittlement of steels. Mater. Sci. Eng. A 2000, 286, 269–281. [Google Scholar] [CrossRef]
- Marchi, C.S.; Somerday, B.; Tang, X.; Schiroky, G. Effects of alloy composition and strain hardening on tensile fracture of hydrogen-precharged type 316 stainless steels. Int. J. Hydrogen Energy 2008, 33, 889–904. [Google Scholar] [CrossRef]
- Matsuo, T.; Yamabe, J.; Matsuoka, S.; Murakami, Y. Influence of Hydrogen and Prestrain on Tensile Properties of Type 316L Austenitic Stainless Steel. In Proceedings of the 2008 International Hydrogen Conference: Effects of Hydrogen on Materials, Jackson, WY, USA, 7–10 September 2008; pp. 105–112. [Google Scholar]
- Thompson, A.W. Ductile fracture topography: Geometrical contributions and effects of hydrogen. Met. Mater. Trans. A 1979, 10, 727–731. [Google Scholar] [CrossRef]
- Takai, K.; Watanuki, R. Hydrogen in Trapping States Innocuous to Environmental Degradation of High-strength Steels. Isij Int. 2003, 43, 520–526. [Google Scholar] [CrossRef]
- Robertson, I.M.; Sofronis, P.; Nagao, A.; Martin, M.L.; Wang, S.; Gross, D.W.; Nygren, K.E. Hydrogen Embrittlement Understood. Met. Mater. Trans. A 2015, 46, 1085–1103. [Google Scholar] [CrossRef]
- Brewer, L.; Othon, M.; Young, L.; Angeliu, T. Misorientation Mapping for Visualization of Plastic Deformation via Electron Back-Scattered Diffraction. Microsc. Microanal. 2006, 12, 85–91. [Google Scholar] [CrossRef]
- Gao, L.; Manhard, A.; Jacob, W.; Von Toussaint, U.; Balden, M.; Schmid, K. High-flux hydrogen irradiation-induced cracking of tungsten reproduced by low-flux plasma exposure. Nucl. Fusion 2019, 59, 056023. [Google Scholar] [CrossRef]


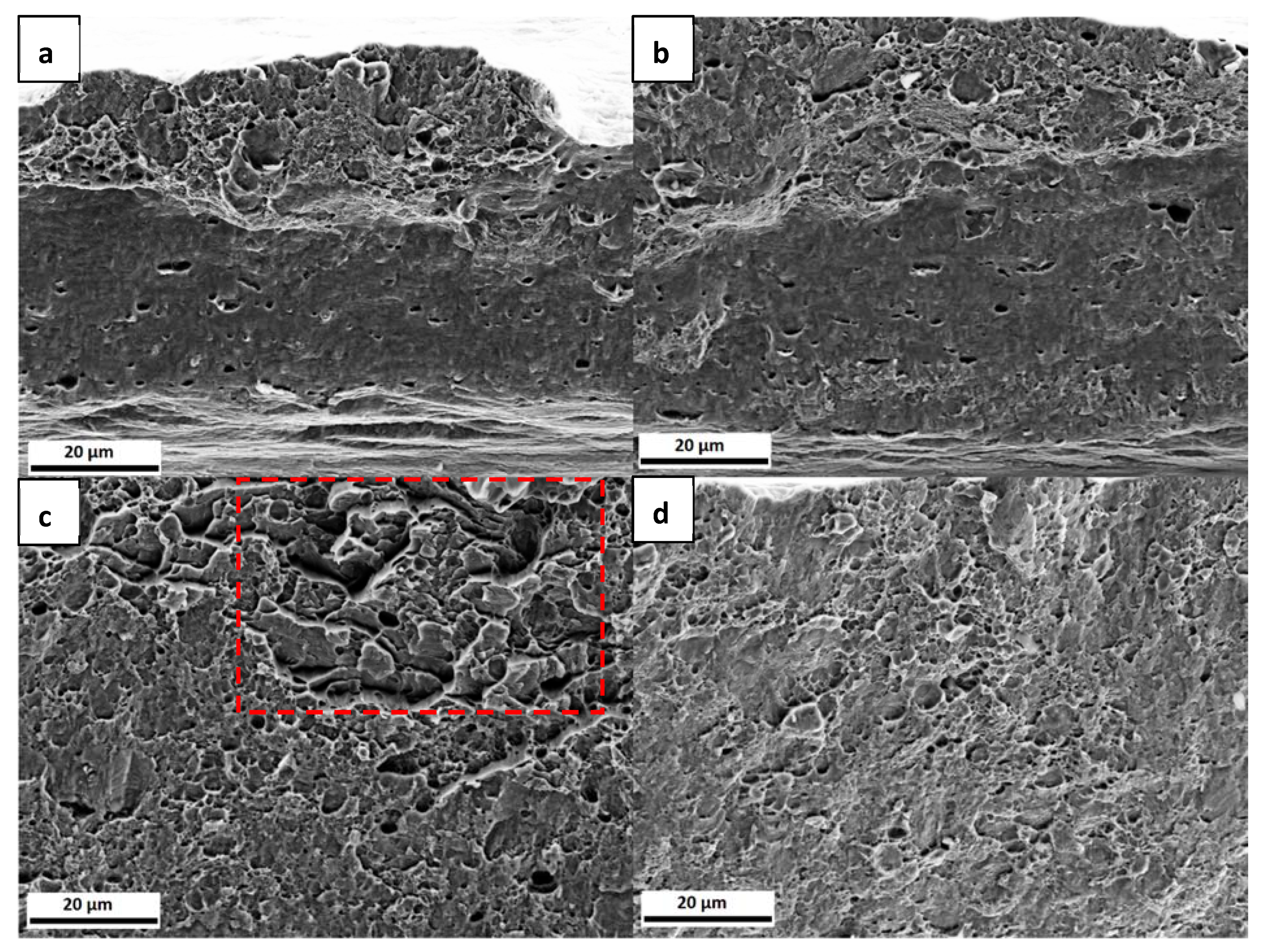
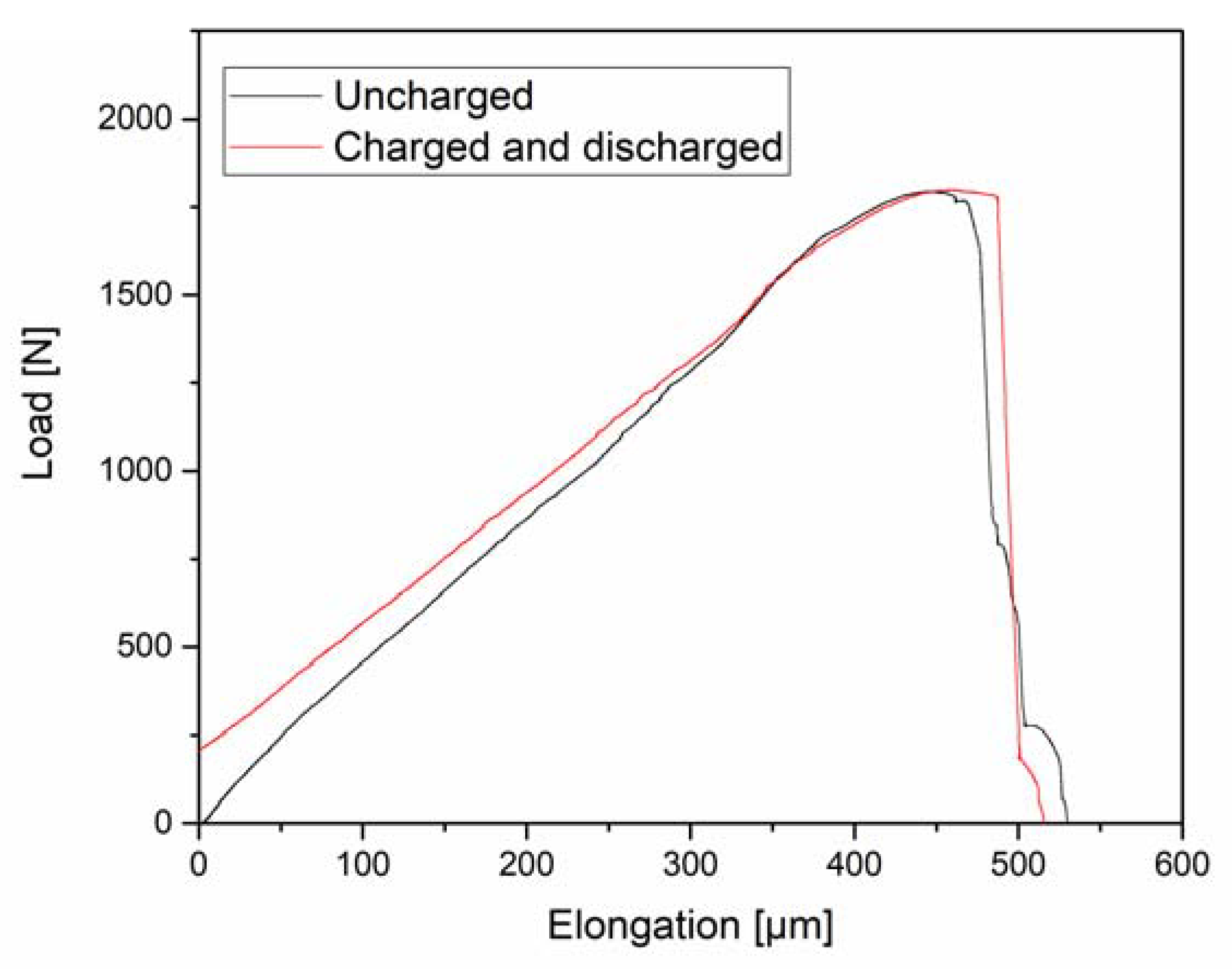

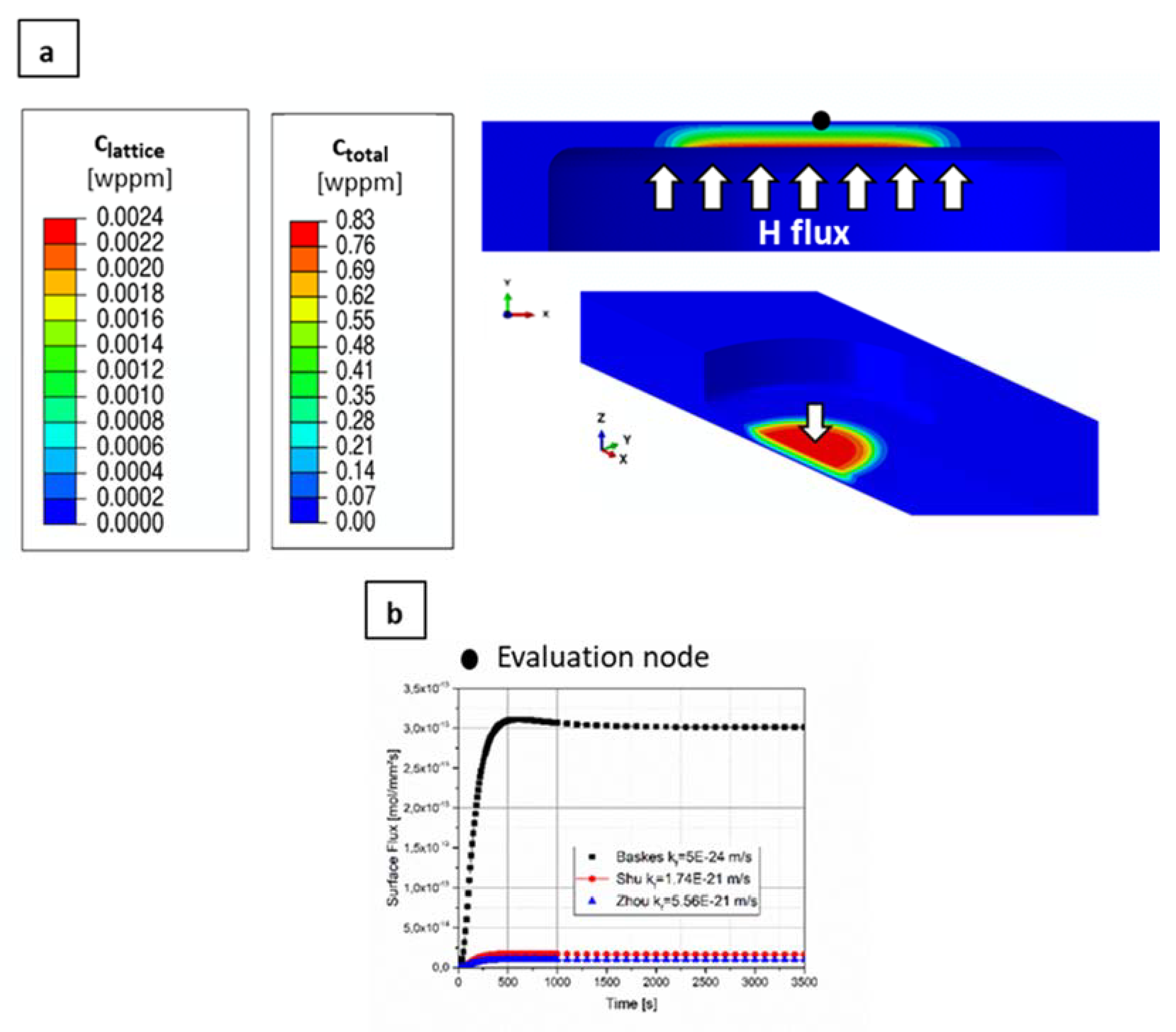
| C | Mn | Si | Cr | S | Nb | Ti | Al | |
|---|---|---|---|---|---|---|---|---|
| wt% | <0.20 | <2.6 | <0.8 | <1.00 | <0.010 | <0.05 | <0.15 | 0.015–1.0 |
| Case Study | Overall Thickness (mm) | Center-Reduced Thickness (mm) |
|---|---|---|
| Rate Dependence of Flow Properties (Tensile Samples) | 1.06 ± 0.01 | – |
| Effect of H Pre-Charging Time | 0.5 ± 0.01 | 0.12 ± 0.01 |
| Effect of Plasma Parameters | 1.10 ± 0.01 | 0.18 ± 0.01 |
| Effect of H Charging-Discharging | 1.10 ± 0.01 | 0.20 ± 0.01 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Massone, A.; Manhard, A.; Drexler, A.; Posch, C.; Ecker, W.; Maier-Kiener, V.; Kiener, D. Addressing H-Material Interaction in Fast Diffusion Materials—A Feasibility Study on a Complex Phase Steel. Materials 2020, 13, 4677. https://doi.org/10.3390/ma13204677
Massone A, Manhard A, Drexler A, Posch C, Ecker W, Maier-Kiener V, Kiener D. Addressing H-Material Interaction in Fast Diffusion Materials—A Feasibility Study on a Complex Phase Steel. Materials. 2020; 13(20):4677. https://doi.org/10.3390/ma13204677
Chicago/Turabian StyleMassone, Agustina, Armin Manhard, Andreas Drexler, Christian Posch, Werner Ecker, Verena Maier-Kiener, and Daniel Kiener. 2020. "Addressing H-Material Interaction in Fast Diffusion Materials—A Feasibility Study on a Complex Phase Steel" Materials 13, no. 20: 4677. https://doi.org/10.3390/ma13204677
APA StyleMassone, A., Manhard, A., Drexler, A., Posch, C., Ecker, W., Maier-Kiener, V., & Kiener, D. (2020). Addressing H-Material Interaction in Fast Diffusion Materials—A Feasibility Study on a Complex Phase Steel. Materials, 13(20), 4677. https://doi.org/10.3390/ma13204677







