Research on Temperature Field Distribution in a Frame Mold during Autoclave Process
Abstract
1. Introduction
2. Experiment
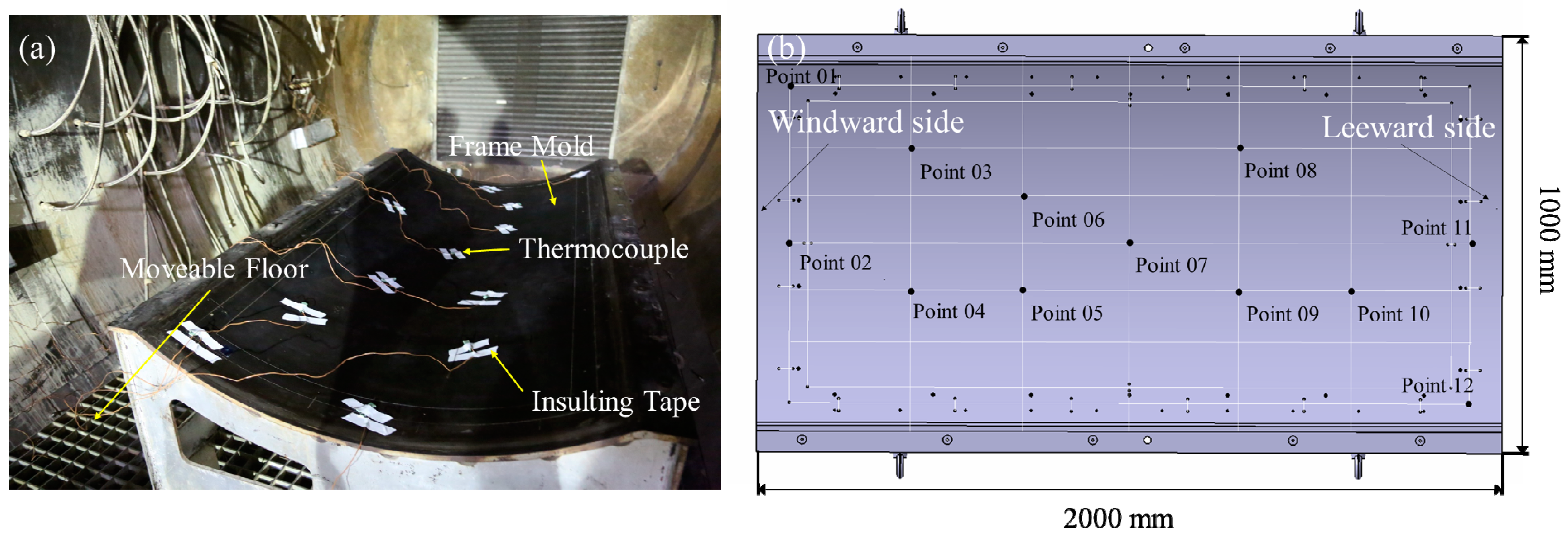
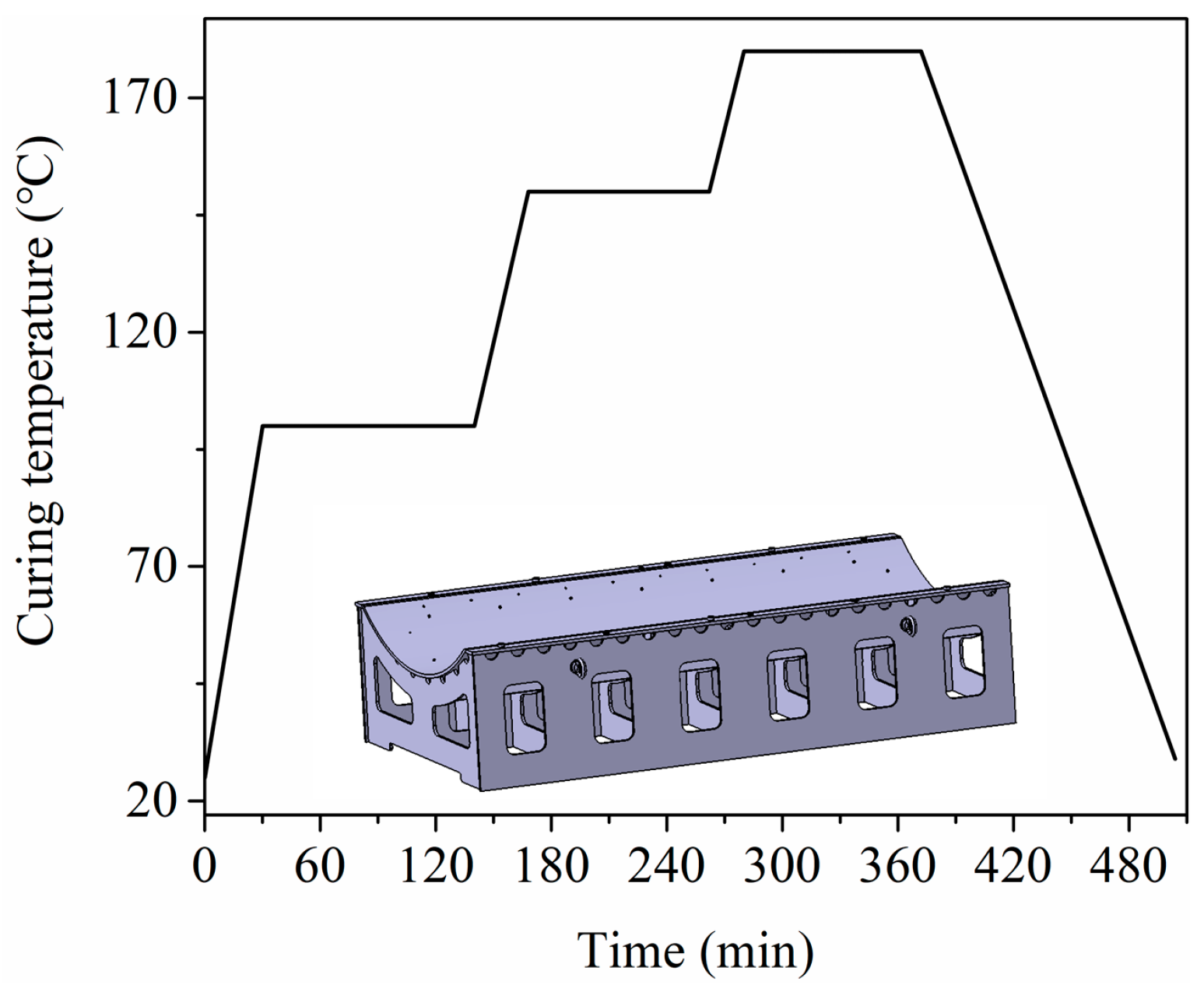
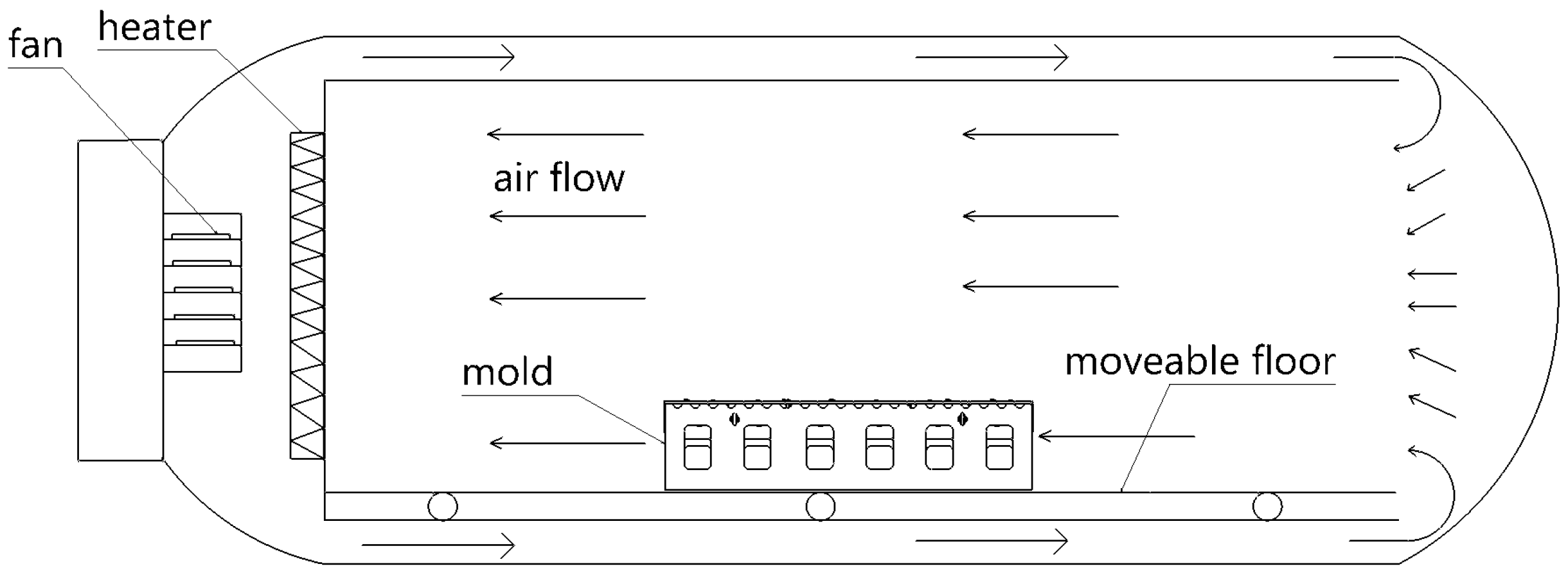
2.1. Experimental Setup
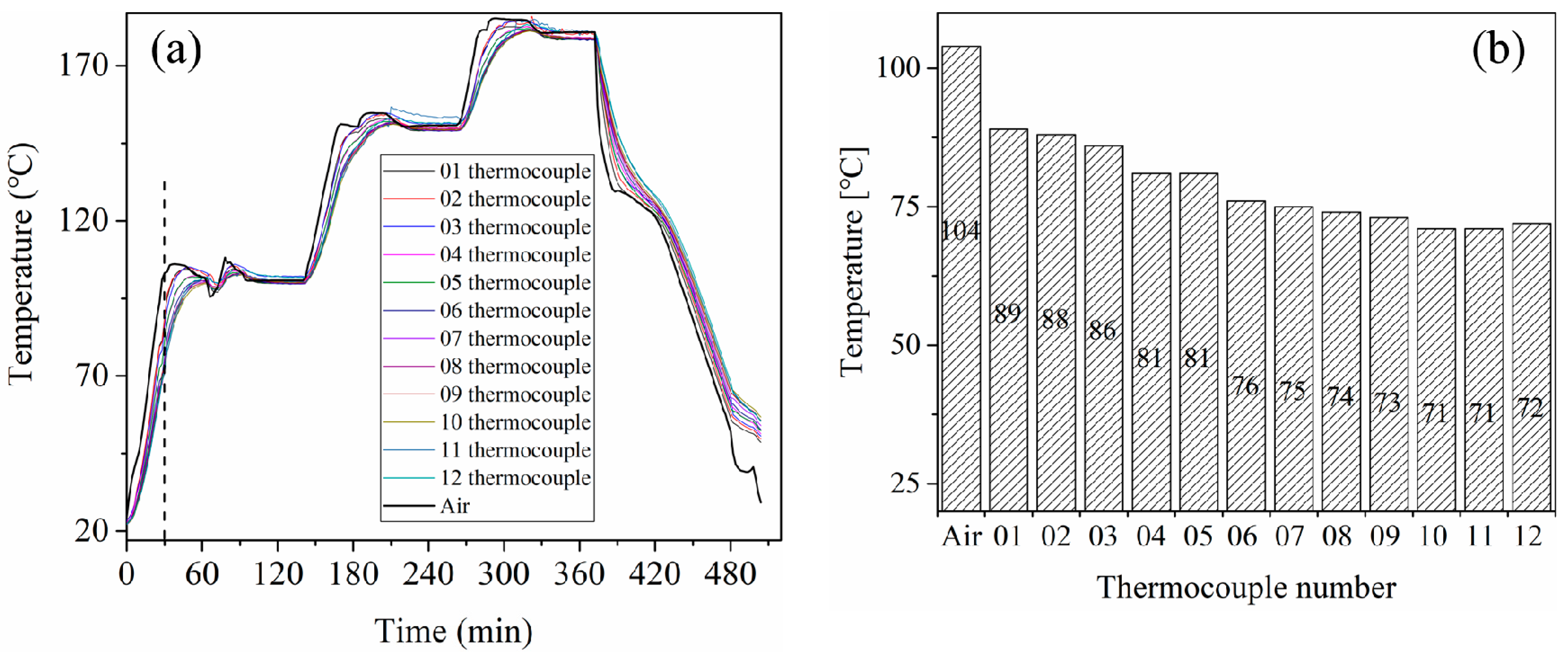
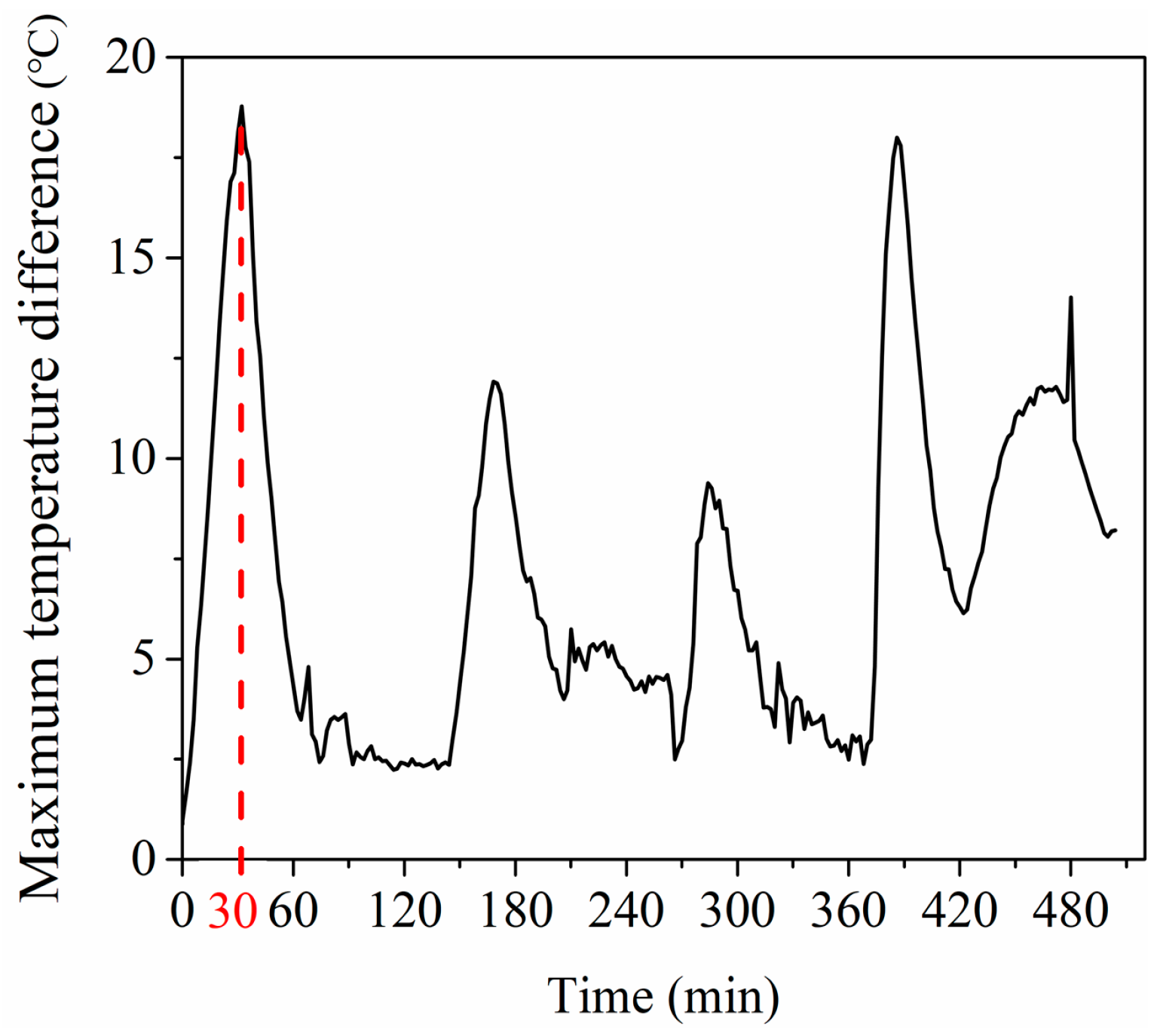
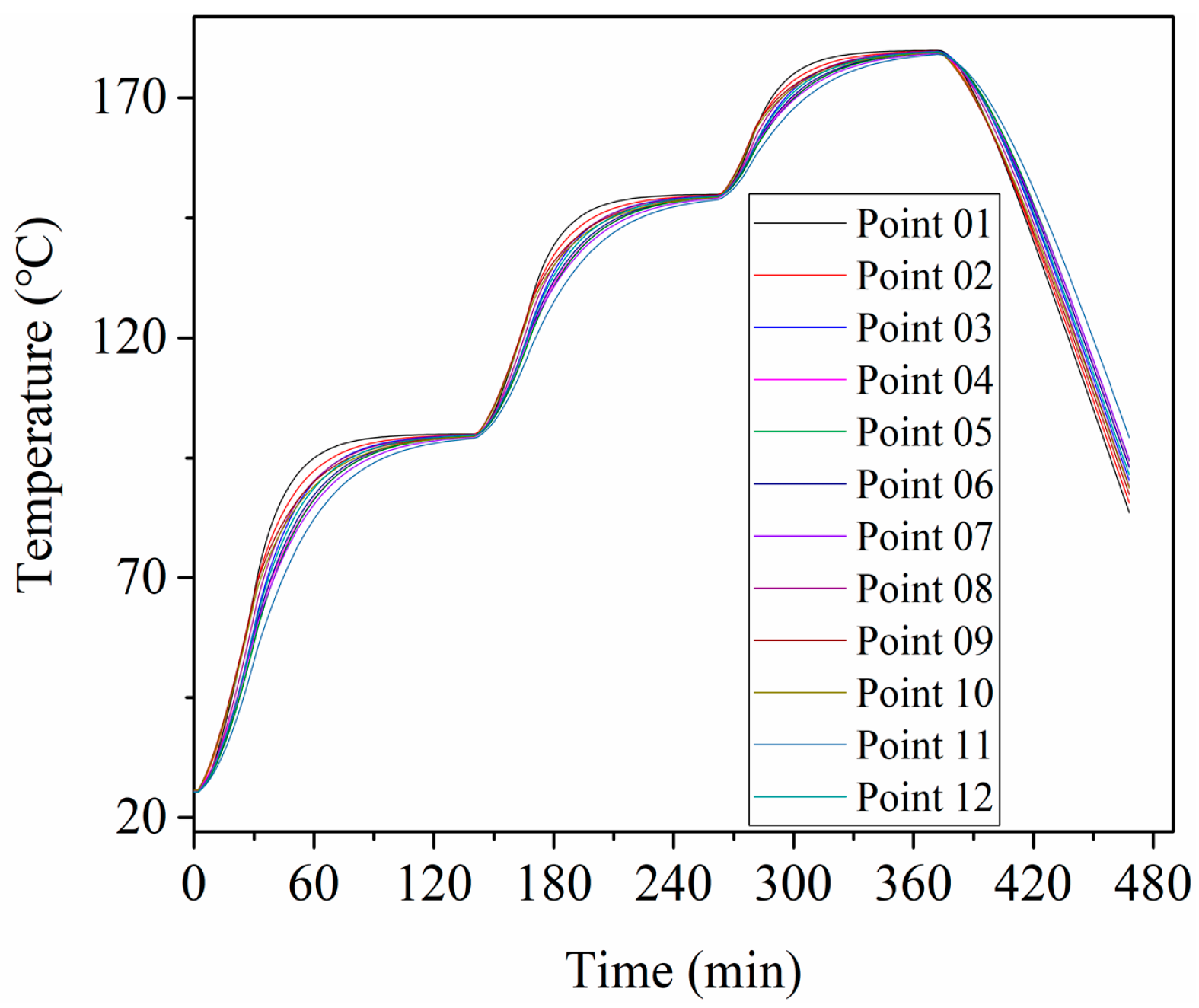
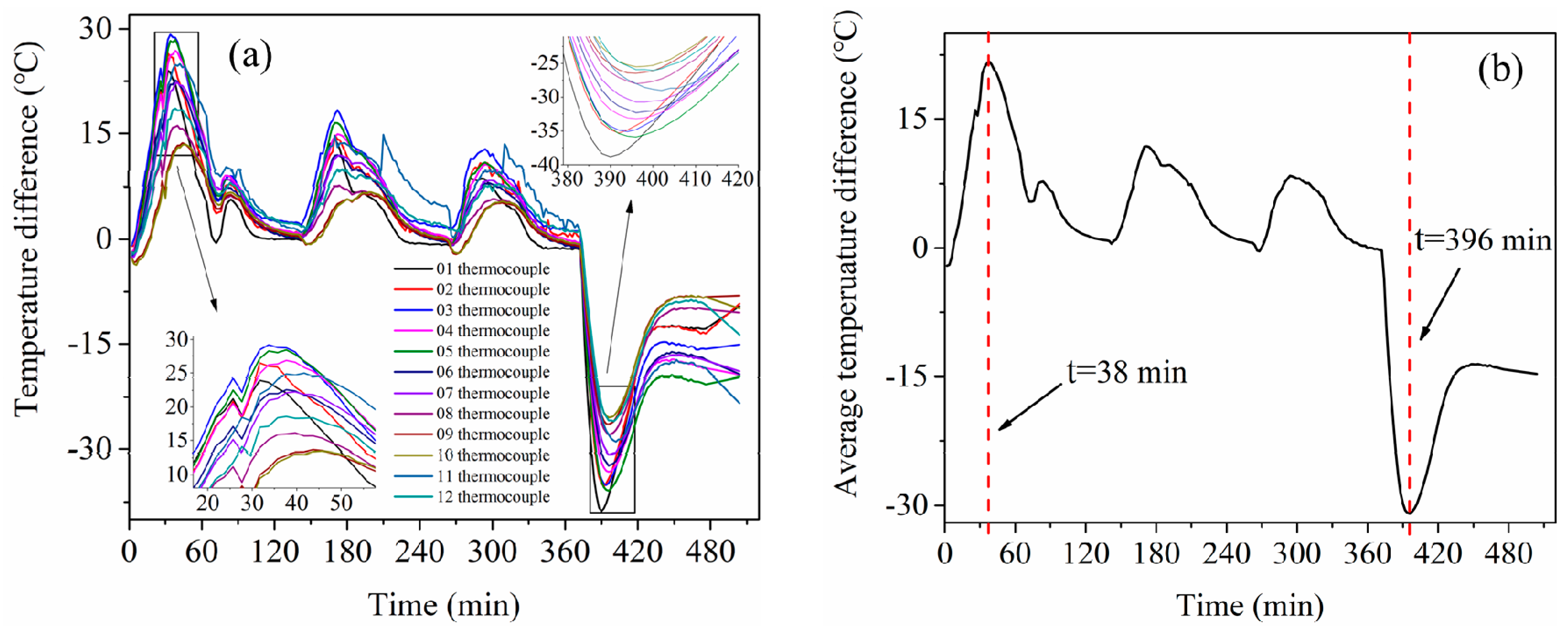
2.2. Experimental Results
3. Numerical Model
3.1. Convective Heat Transfer Model
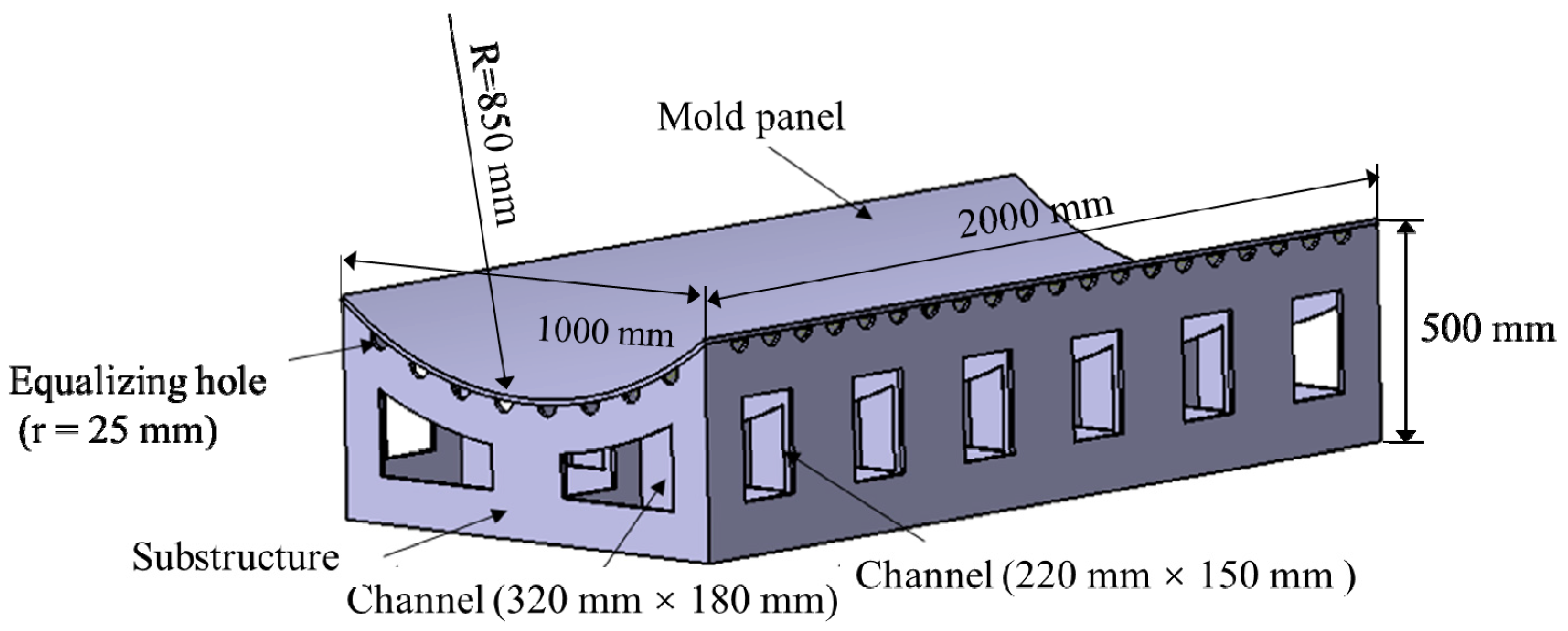
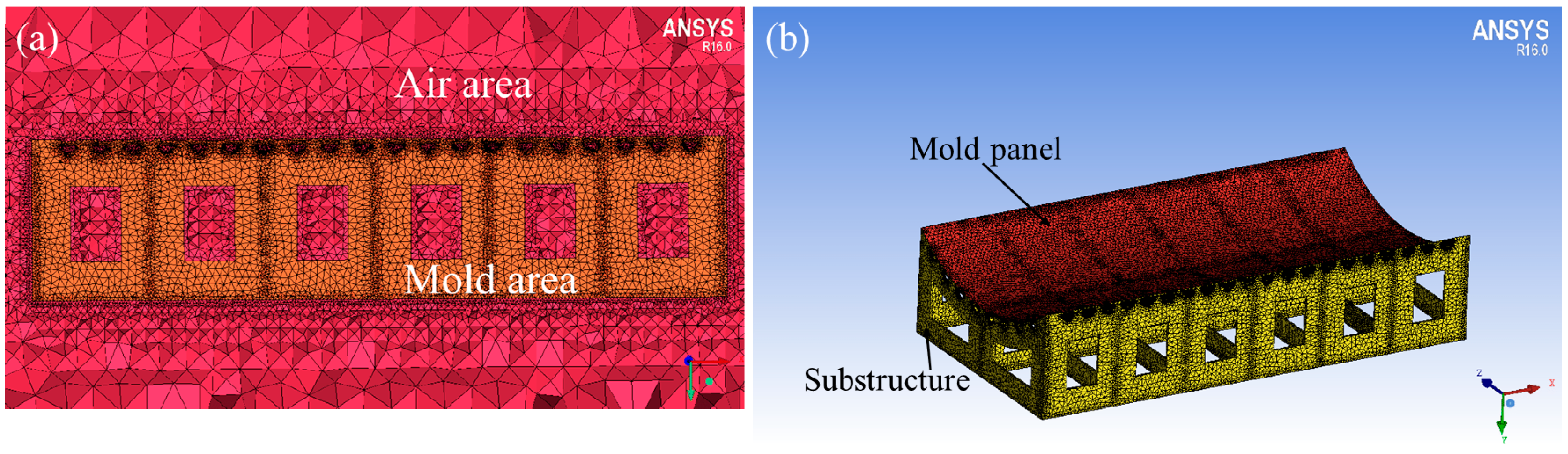
3.2. Geometric Model and Mesh
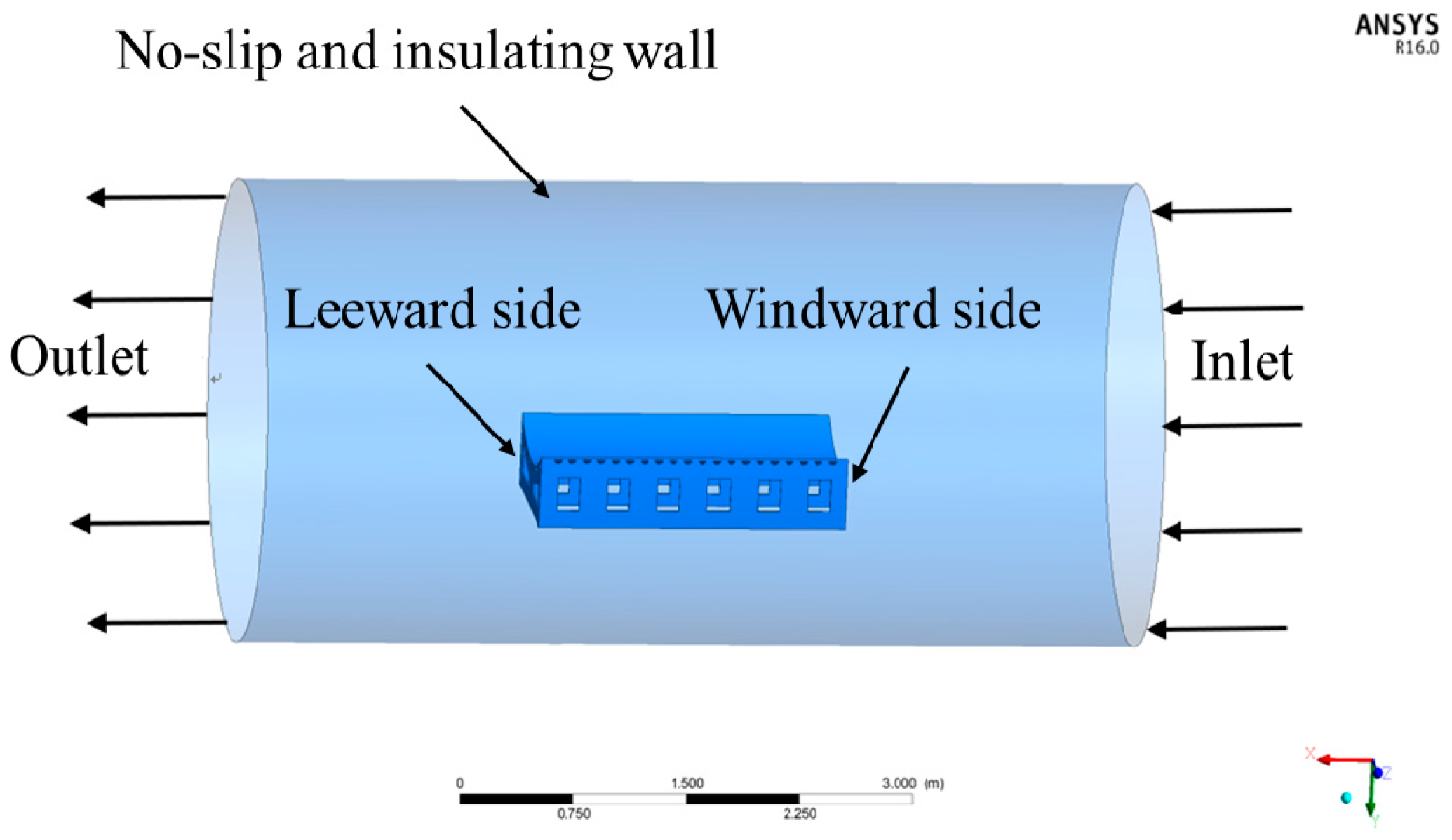
3.3. Boundary Conditions and Solvers
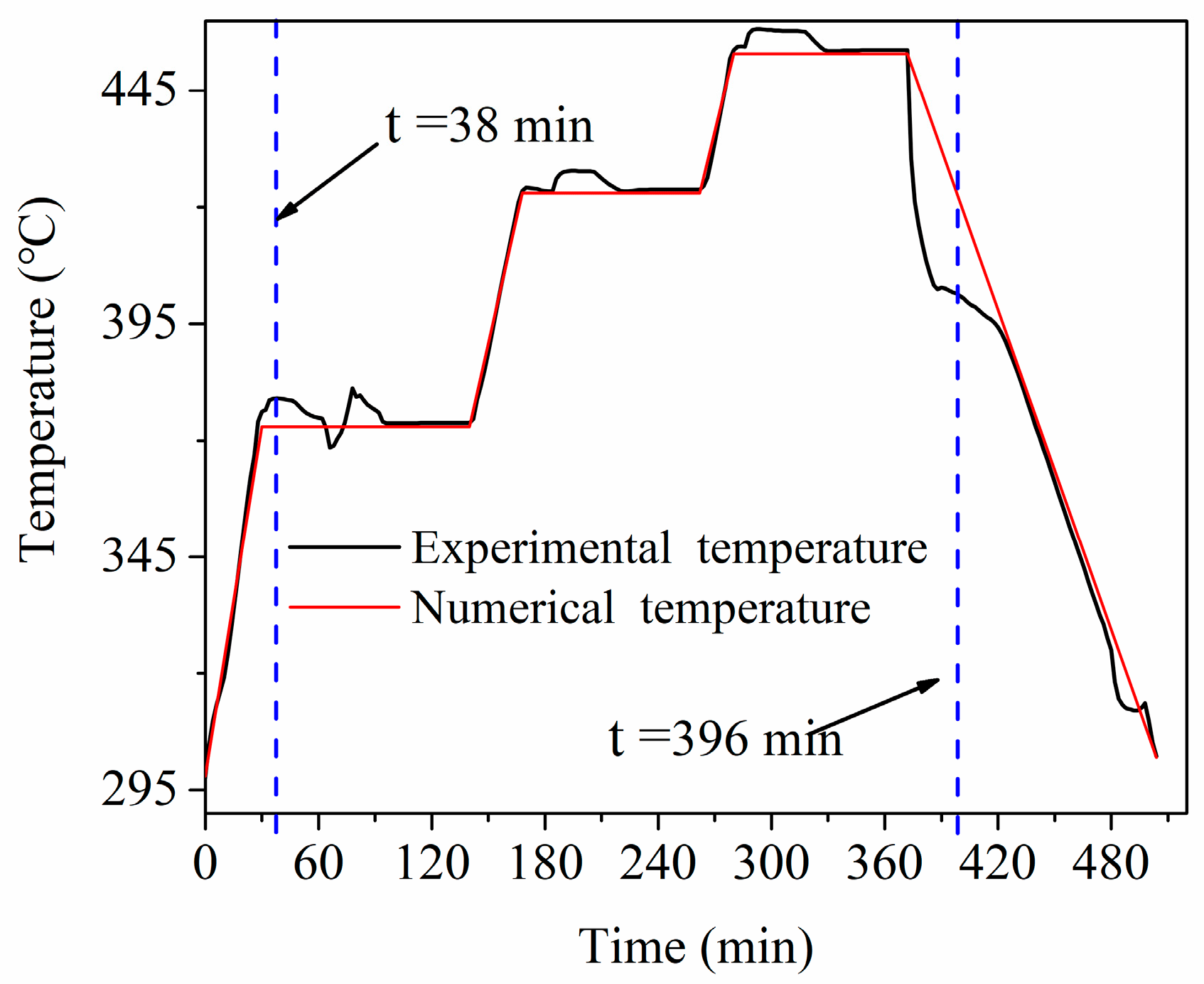
3.4. Validation of the Numerical Model
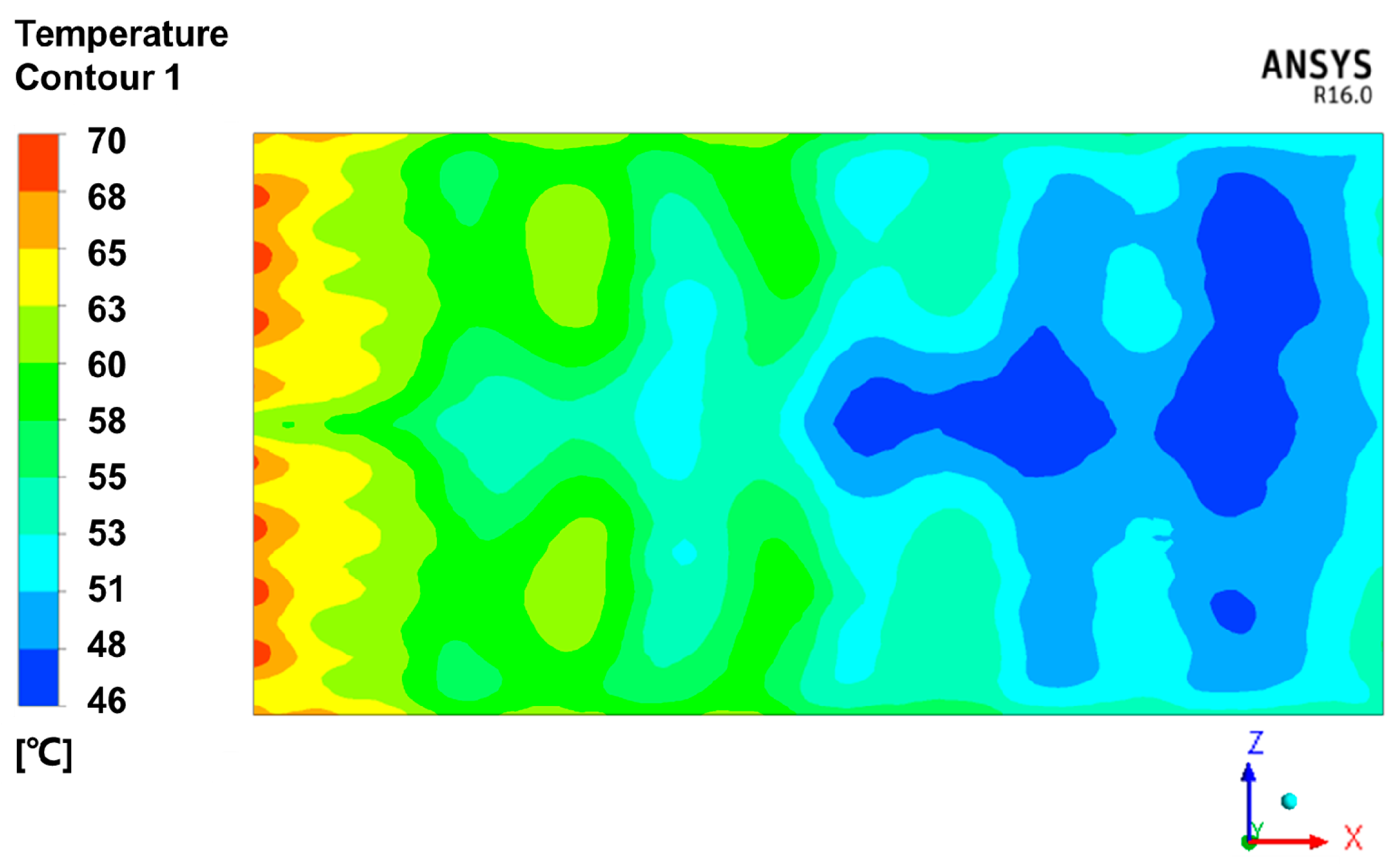
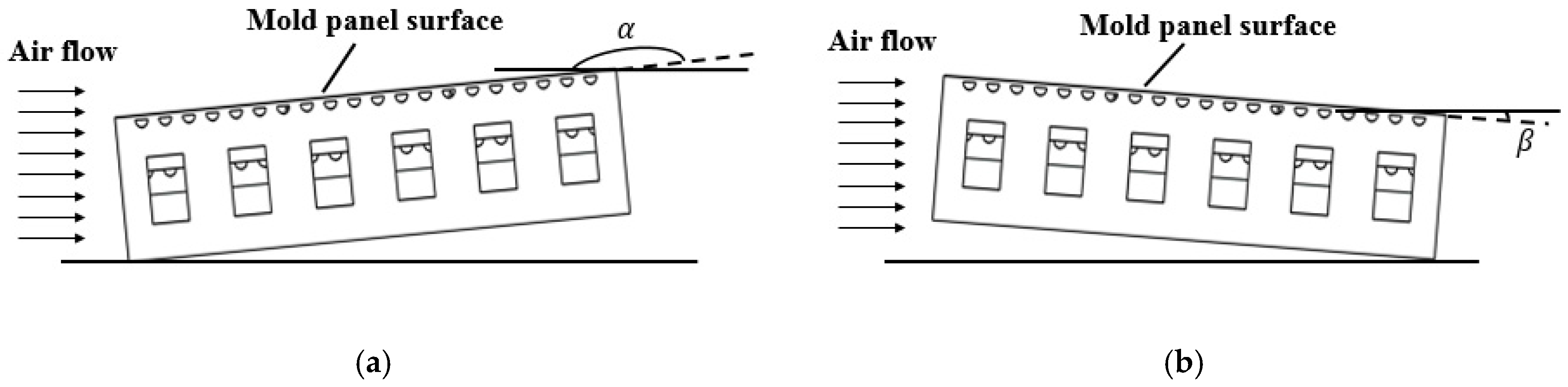
4. Control of Temperature Distribution in the Mold Panel
4.1. Research Concept
4.2. Methodology of Temperature Distribution Control
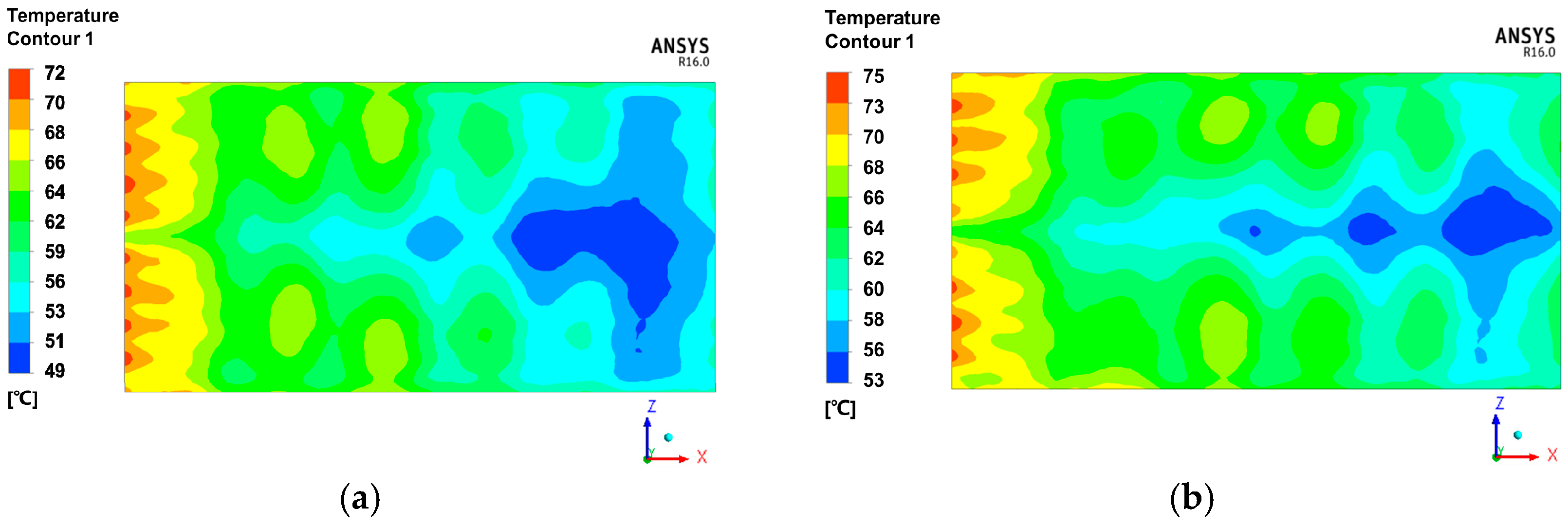
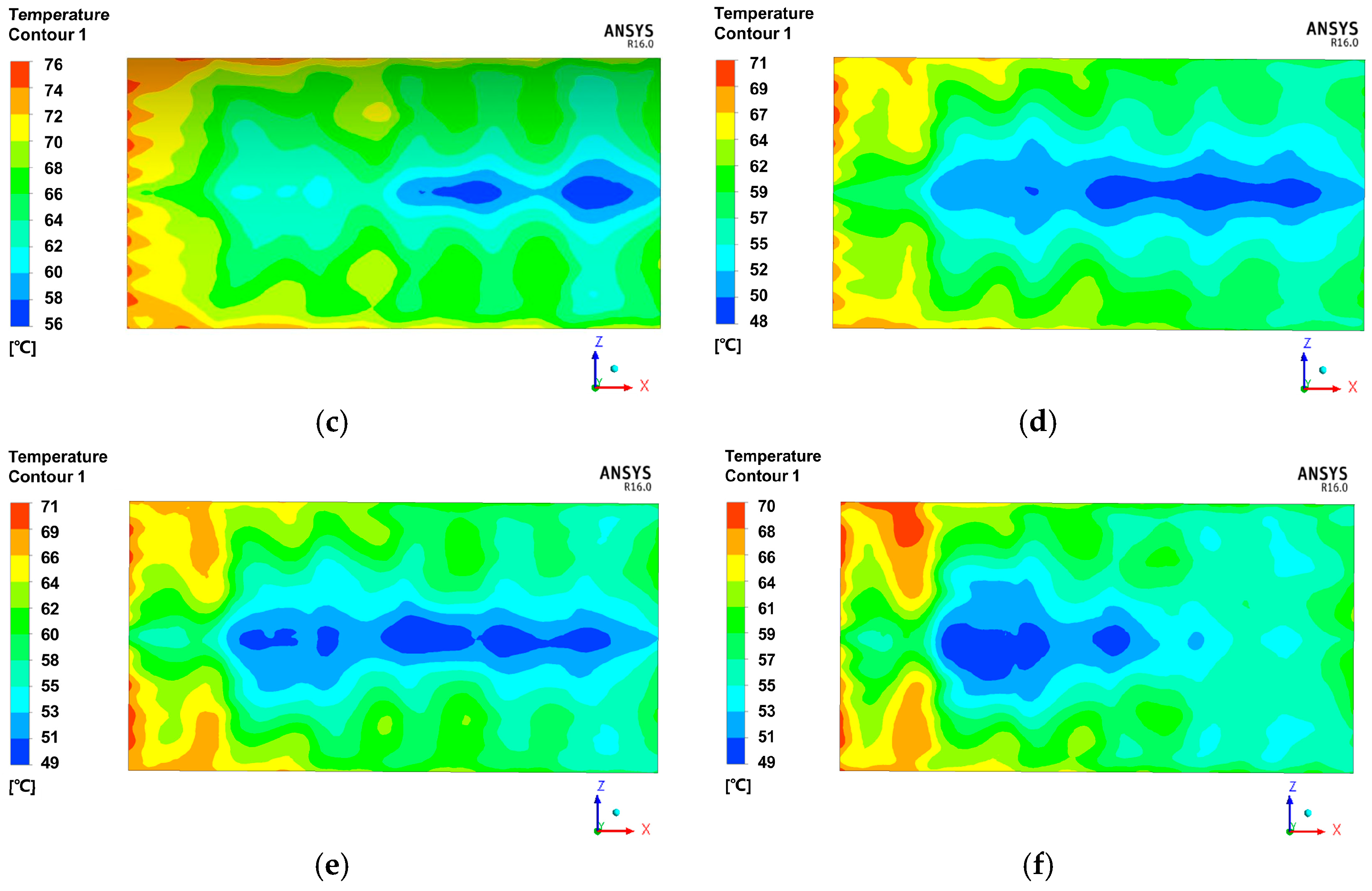
4.3. Results and Discussion
5. Conclusions
- The maximum temperature difference of 18 °C for the 12 measurement points appeared at the timing of 30 min.
- The difference between the predicted temperature and the experimental temperature was 5.92%, which shows a good agreement.
- The proposed controlling method can significantly improve the temperature uniformity in the mold. The standard deviation decreased by 13.3% as the placement angle α changed from 180 to 168°.
Author Contributions
Funding
Conflicts of Interest
References
- Srinivasa, I.L.; Rajan, S. Advanced Composites Materials in Civil Engineering Structures; American Society of Civil Engineers: New York, NY, USA, 1991. [Google Scholar]
- Upadhya, A.R.; Dayananda, G.N.; Kamalakannan, G.M.; Setty, J.R.; Daniel, C. Autoclaves for Aerospace Applications: Issues and Challenges. Int. J. Aerosp. Eng. 2011, 2011, 1–11. [Google Scholar] [CrossRef]
- Baran, I.; Cinar, K.; Ersoy, N.; Akkerman, R.; Hattel, J.H. A Review on the Mechanical Modeling of Composite Manufacturing Processes. Arch. Comput. Methods Eng. 2016, 24, 365–395. [Google Scholar] [CrossRef] [PubMed]
- Sun, J.; Gu, Y.; Li, Y.; Li, M.; Zhang, Z. Role of Tool-Part Interaction in Consolidation of L-Shaped Laminates during Autoclave Process. Appl. Compos. Mater. 2011, 19, 583–597. [Google Scholar] [CrossRef]
- Fernlund, G.; Rahman, N.; Courdji, R.; Bresslauer, M.; Poursartip, A.; Willden, K.; Nelson, K. Experimental and numerical study of the effect of cure cycle, tool surface, geometry, and lay-up on the dimensional fidelity of autoclave-processed composite parts. Compos. Part A Appl. Sci. Manuf. 2002, 33, 341–351. [Google Scholar] [CrossRef]
- Khoun, L.; Centea, T.; Hubert, P. Characterization Methodology of Thermoset Resins for the Processing of Composite Materials—Case Study: CYCOM 890RTM Epoxy Resin. J. Compos. Mater. 2009, 44, 1397–1415. [Google Scholar] [CrossRef]
- Khoun, L. Process-Induced Stresses and Deformations in Woven Composites Manufactured by Resin Transfer Moulding. Ph.D. Thesis, McGill University, Montreal, QC, Canada, July 2009. [Google Scholar]
- Gao, Y.; Qu, C.H. Numerical simulation about heat-fluid coupling in autoclaves. Ind. Furn. 2012, 4, 37–39. [Google Scholar]
- Zengyuan, G. Mechanism and control of convective heat transfer Coordination of velocity and heat flow fields. Sci. Bull. Eng. 2001, 46, 596–599. [Google Scholar]
- Maffezzoli, A.; Grieco, A.D. Optimization of Parts Placement in Autoclave Processing of Composites. Appl. Compos. Mater. 2012, 20, 233–248. [Google Scholar] [CrossRef]
- Twigg, G.; Poursartip, A.; Fernlund, G. Tool–part interaction in composites processing. Part II: Numerical modelling. Compos. Part A Appl. Sci. Manuf. 2004, 35, 135–141. [Google Scholar] [CrossRef]
- Vafayan, M.; Ghoreishy, M.H.R.; Abedini, H.; Beheshty, M.H. Development of an optimized thermal cure cycle for a complex-shape composite part using a coupled finite element/genetic algorithm technique. Iran. Polym. J. 2015, 24, 459–469. [Google Scholar] [CrossRef]
- Sarrazin, H.; Kim, B.; Ahn, S.-H.; Springer, G.S. Effects of Processing Temperature and Layup on Springback. J. Compos. Mater. 1995, 29, 1278–1294. [Google Scholar] [CrossRef]
- Adolf, D.; Martin, J.E. Calculation of Stresses in Crosslinking Polymers. J. Compos. Mater. 1996, 30, 13–34. [Google Scholar] [CrossRef]
- Tarshakurdi, K.; Olivier, P. Thermoviscoelastic analysis of residual curing stresses and the influence of autoclave pressure on these stresses in carbon/epoxy laminates. Compos. Sci. Technol. 2002, 62, 559–565. [Google Scholar] [CrossRef]
- Dong, A.; Zhao, Y.; Zhao, X.; Yu, Q. Cure Cycle Optimization of Rapidly Cured Out-Of-Autoclave Composites. Materials 2018, 11, 421. [Google Scholar] [CrossRef]
- Bogetti, T.A.; Gillespie, J.W. Two-Dimensional Cure Simulation of Thick Thermosetting Composites. J. Compos. Mater. 1991, 25, 239–273. [Google Scholar] [CrossRef]
- Struzziero, G.; Skordos, A. Multi-objective optimisation of the cure of thick components. Compos. Part A Appl. Sci. Manuf. 2017, 93, 126–136. [Google Scholar] [CrossRef]
- White, S.R.; Kim, Y. Staged curing of composite materials. Compos. Part A Appl. Sci. Manuf. 1996, 27, 219–227. [Google Scholar] [CrossRef]
- Wang, Q.; Wang, L.; Zhu, W.; Xu, Q.; Ke, Y. Design optimization of molds for autoclave process of composite manufacturing. J. Reinf. Plast. Compos. 2017, 36, 1564–1576. [Google Scholar] [CrossRef]
- Xie, G.N.; Liu, J.; Zang, W.H.; Lorenzini, G.; Biserni, C. Simulation and improvement of temperature distributions of a framed mould during the autoclave composite curing process. J. Eng. Thermophys. 2013, 22, 43–61. [Google Scholar] [CrossRef]
- Weber, T.A.; Arent, J.-C.; Münch, L.; Duhovic, M.; Balvers, J.M. A fast method for the generation of boundary conditions for thermal autoclave simulation. Compos. Part A Appl. Sci. Manuf. 2016, 88, 216–225. [Google Scholar] [CrossRef]
- Chen, F.; Zhan, L.; Li, S. Refined simulation of temperature distribution in molds during autoclave process. Iran. Polym. J. 2016, 25, 775–785. [Google Scholar] [CrossRef]
- Kluge, N.; Lundström, T.; Ljung, A.-L.; Westerberg, L.; Nyman, T. An experimental study of temperature distribution in an autoclave. J. Reinf. Plast. Compos. 2016, 35, 566–578. [Google Scholar] [CrossRef]
- Dios, M.; Gonzalez-R, P.L.; Maffezzoli, A. A mathematical modeling approach to optimize composite parts placement in autoclave. Int. Trans. Oper. Res. 2016, 24, 115–141. [Google Scholar] [CrossRef]
- Hudek, M. Examination of Heat Transfer During Autoclave Processing of Polymer Composites. Master’s Thesis, University of Manitoba, Winnipeg, MB, Canada, 2001. [Google Scholar]
- Ramezanizadeh, M.; Nazari, M.A.; Ahmadi, M.H.; Chau, K.-W. Experimental and numerical analysis of a nanofluidic thermosyphon heat exchanger. Eng. Appl. Comput. Fluid Mech. 2018, 13, 40–47. [Google Scholar] [CrossRef]
- Wang, L.; Zhu, W.; Wang, Q.; Xu, Q.; Ke, Y. A heat-balance method for autoclave process of composite manufacturing. J. Compos. Mater. 2018, 53, 641–652. [Google Scholar] [CrossRef]
- Ghalandari, M.; Koohshahi, E.M.; Mohamadian, F.; Shamshirband, S.; Chau, K.W. Numerical simulation of nanofluid flow inside a root canal. Eng. Appl. Comput. Fluid Mech. 2019, 13, 254–264. [Google Scholar] [CrossRef]
- Guo, Z.; Tao, W.; Shah, R. The field synergy (coordination) principle and its applications in enhancing single phase convective heat transfer. Int. J. Heat Mass Transf. 2005, 48, 1797–1807. [Google Scholar] [CrossRef]
| α (°) | The Maximum Difference (°C) | SD (°C) |
|---|---|---|
| 180 | 24.1 | 5.71 |
| 175 | 22.8 | 5.21 |
| 170 | 22.2 | 5.01 |
| 168 | 19.9 | 4.95 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Han, N.; An, L.; Fan, L.; Hua, L.; Gao, G. Research on Temperature Field Distribution in a Frame Mold during Autoclave Process. Materials 2020, 13, 4020. https://doi.org/10.3390/ma13184020
Han N, An L, Fan L, Hua L, Gao G. Research on Temperature Field Distribution in a Frame Mold during Autoclave Process. Materials. 2020; 13(18):4020. https://doi.org/10.3390/ma13184020
Chicago/Turabian StyleHan, Ning, Luling An, Longxin Fan, Leilei Hua, and Guoqiang Gao. 2020. "Research on Temperature Field Distribution in a Frame Mold during Autoclave Process" Materials 13, no. 18: 4020. https://doi.org/10.3390/ma13184020
APA StyleHan, N., An, L., Fan, L., Hua, L., & Gao, G. (2020). Research on Temperature Field Distribution in a Frame Mold during Autoclave Process. Materials, 13(18), 4020. https://doi.org/10.3390/ma13184020




