Study on Properties Prediction and Braiding Optimization of Axial Braided Carbon/Carbon Composite
Abstract
1. Introduction
2. A Method for Predicting the Macroscopic Properties of Axial Braided C/C Composite
2.1. Homogenization Process and Stiffness Prediction Method
2.2. A Method for Predicting CTE of Composites Based on Energy Method
2.3. The Boundary Conditions
3. The Introduction of the Experiment Carried out in the Early Stage
3.1. Properties Test of Fiber Rod
3.2. Macroscopic Properties Test
4. RVE Establishment and Properties of Component
4.1. RVE Establishment
4.2. Study on the Properties of Components
4.2.1. Mechanical Properties of Matrix and Interface
4.2.2. Study on Mechanical Properties of Fiber Rod and Fiber Bundle
5. Calculation Results and Analysis
5.1. The Calculation Results of Elastic Properties
5.2. The Calculation Result of CTE
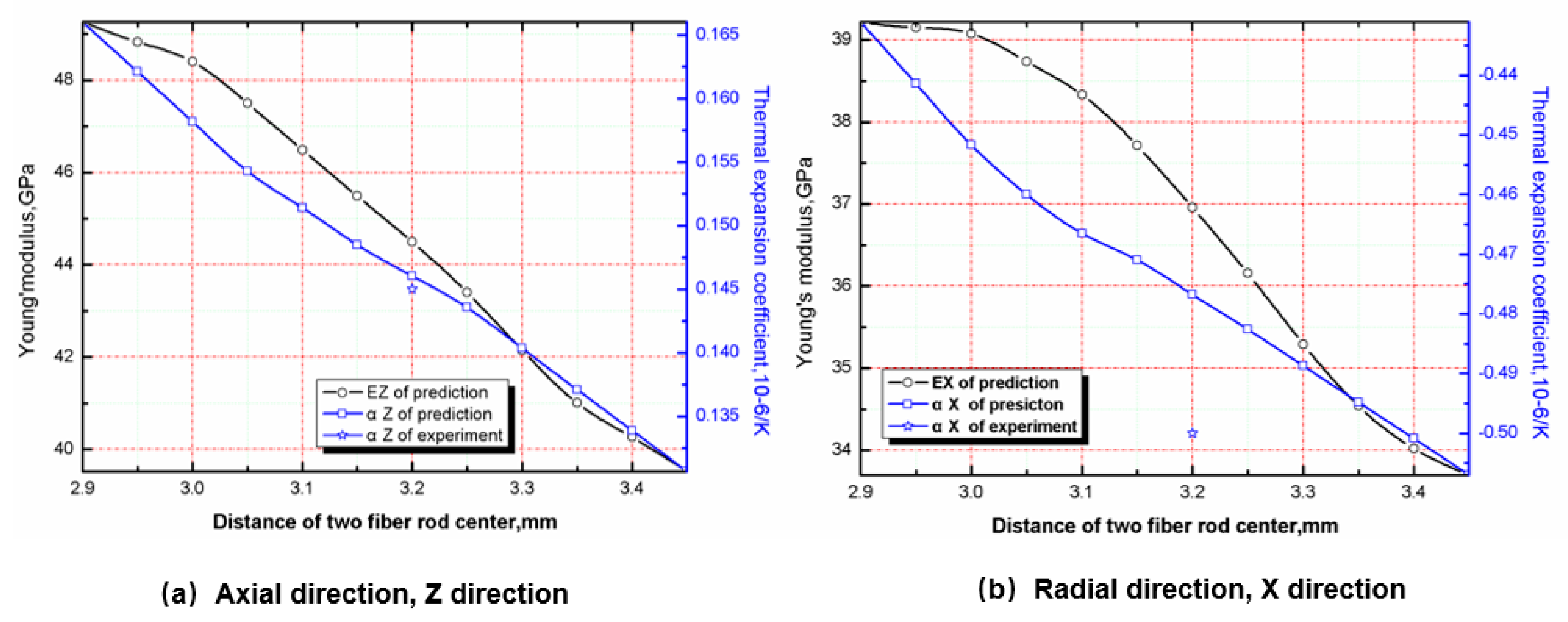
5.3. Effect of Braiding Spacing on Stiffness and CTE
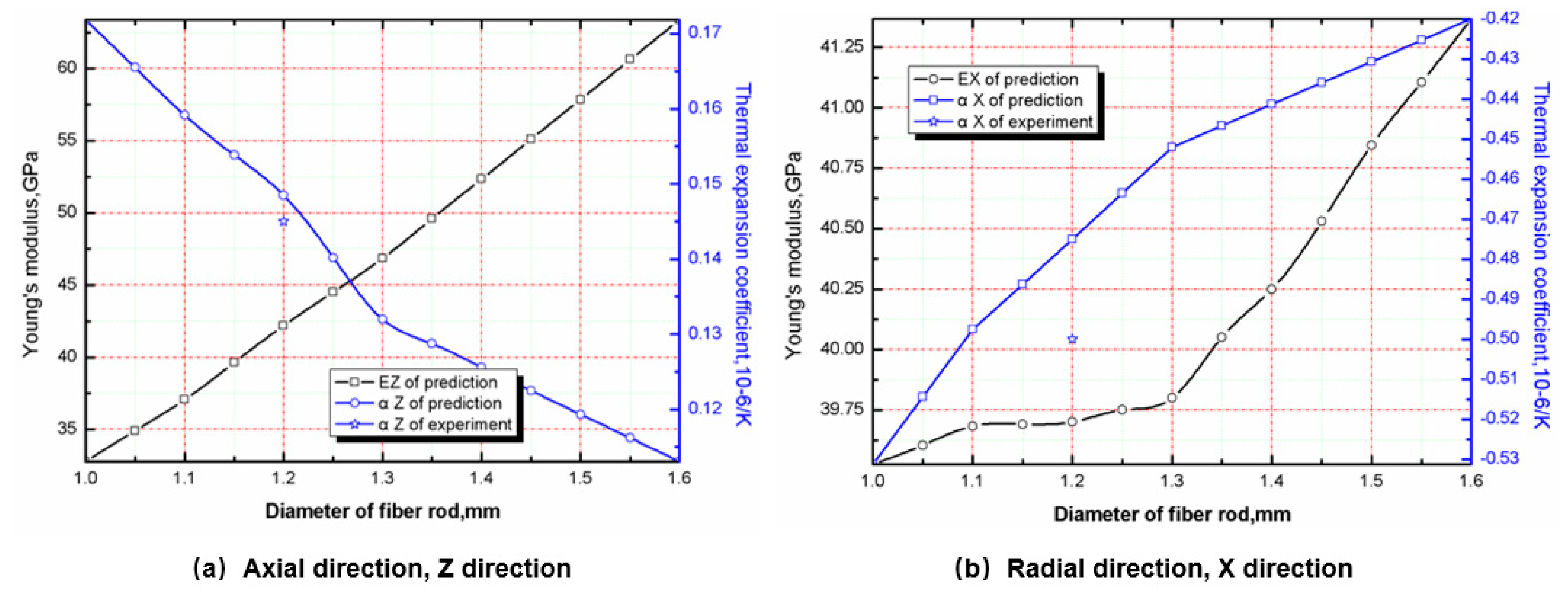
5.4. Effect of Fiber Rod Diameter on Stiffness and CTE
6. Conclusions
- (1)
- The FEM was utilized for the calculation of the stiffness properties of the matrix and interface, and the results showed that the porosity was important to determine the elastic properties of the matrix and interface. It can be seen that the elastic properties of the matrix and interface change linearly with the porosity, which increases, leading to decreases of both the elastic modulus and Poisson’s ratio.
- (2)
- The stiffness performance of the reinforcing phase was calculated by FEM. The calculation results showed that the reinforcing phase manifested transversely isotropic characteristic even if there was a few of pores. It was found that the prediction precision of tensile modulus, compression modulus and shear modulus was reliable, being 13.4%, 13.7% and 10.4%, respectively.
- (3)
- Based on the homogenization theory and energy method combined with the RVE of axial braided C/C composite material, the macroscopic stiffness and CTE of the material were also predicted. By comparing the predicted results with the experimental results in different states, it can be concluded that the numerical predicted results are basically consistent with the experimental results, thus proving the effectiveness of the prediction method.
- (4)
- By calculating the macroscopic stiffness performance and CTE under the conditions with different braiding spacing and fiber rod diameter, the relationship between the microstructure and macroscopic properties was evaluated. The results show that the best diameter of the axial braided C/C composite fiber rod studied in this paper is 1.26 mm, and the optimization of braiding spacing needs to be carried out in combination with the ablative property.
- (5)
- The advantages of the prediction method in this paper are as follows: by combining the homogenization method with FEM, the pore characteristics of the fiber rod (bundle), matrix and interface can be considered, so as to obtain the properties of mesoscopic materials accurately. As the input parameters, the stiffness prediction of the material has higher precision. The disadvantage is that the pore characteristics cannot be added into the finite element model of RVE for the joint calculation of macroscopy-mesoscopic and microscopic, which is also the focus of the follow-up research of this project.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Hui, W.-H.; Bao, F.-T.; Wei, X.-G.; Liu, Y. Ablation performance of a 4D-braided C/C composite in a parameter-variable channel of a Laval nozzle in a solid rocket motor. New Carbon Mater. 2017, 32, 365–373. [Google Scholar] [CrossRef]
- Wang, C.; Tang, M.; Liu, W.; Zhu, T. Study on microstructure characteristics of axially braided carbon/carbon composites based on SEM and Micro-CT. Materials 2020, 13, 1414. [Google Scholar] [CrossRef] [PubMed]
- Gao, B.; Tang, M.; Yang, Y.; Shi, H. Mechanical experiment of 4D in-plain C/C composites. Acta Mater. Compos. Sin. 2011, 28, 245–250. [Google Scholar]
- Rajneesh, S.; Ramesh, B.A.; Puneet, M. Finite element analysis for mechanical characterization of 4D inplane carbon/carbon composite with imperfect microstructure. Lat. Am. J. Solids Struct. 2014, 11, 170–184. [Google Scholar]
- Li, D.S.; Yao, Q.Q.; Jiang, N.; Jiang, L. Bend properties and failure mechanism of a carbon/carbon composite with a 3D needle-punched preform at room and high temperatures. New Carbon Mater. 2016, 31, 437–444. [Google Scholar] [CrossRef]
- Ren, J.; Zhang, Y.; Fu, Y.; Zhang, P.; Tian, S.; Zhang, L. Effects of the second phase on the microstructure and ablation resistance of HfC coating on C/C composites. Surf. Coat. Technol. 2018, 344, 250–258. [Google Scholar] [CrossRef]
- Shan, Z.; Kang, H.; Zang, Y.; Liu, F. Microscopic structure model and finite element simulation of mechanical properties of fiber-bar composites reinforced by three dimensional weaving. Acta Mater. Compos. Sin. 2015, 32, 138–149. [Google Scholar]
- Tan, H.; Huang, X.; Liu, L.; Guan, Y.; Chen, W. Dynamic compressive behavior of four-step three-dimensional braided composites along three directions. Int. J. Impact Eng. 2019, 134, 103366. [Google Scholar] [CrossRef]
- Castaneda, N.; Wisner, B.; Cuadra, J.; Amini, S.; Kontsos, A. Investigation of the Z-binder role in progressive damage of 3D woven composites. Compos. Part A Appl. Sci. Manuf. 2017, 98, 76–89. [Google Scholar] [CrossRef]
- Gerlach, R.; Siviour, R.C.; Wiegand, J.; Petrinic, N. In-plane and through-thickness properties, failure modes, damage and delamination in 3D woven carbon fibre composites subjected to impact loading. Compos. Sci. Technol. 2011, 72, 397–411. [Google Scholar] [CrossRef]
- Lu, Z.; Xia, B.; Yang, Z. Investigation on the tensile properties of three-dimensional full five-directional braided composites. Comput. Mater. Sci. USA 2013, 77, 445–455. [Google Scholar] [CrossRef]
- Zhang, P. Microstructure Modeling and Mechanical Properties Prediction of 3d Needled C/C Composites; Northwestern Polytechnical University: Xi’an, China, 2016. [Google Scholar]
- Yan, S.; Zhang, J.; Sun, B.; Guo, B. In situ measurement of strains at different locations in 3-D braided composites with FBG sensors. Compos. Struct. 2019, 230, 111527. [Google Scholar] [CrossRef]
- Dang, H.; Zhao, Z.; Liu, P.; Zhang, C.; Tong, L.; Li, Y. A new analytical method for progressive failure analysis of two-dimensional triaxially braided composites. Compos. Sci. Technol. 2020, 186, 107936. [Google Scholar] [CrossRef]
- Hao, W.; Huang, Z.; Zhang, L.; Zhao, G.; Luo, Y. Study on the torsion behavior of 3-D braided composite shafts. Compos. Struct. 2019, 229, 111384. [Google Scholar] [CrossRef]
- Xu, C.-H.; Xu, D.-S.; Song, L.-Y.; Xu, K. Microstructure characterization from X-ray micro-tomography and tensile failure mechanism of 4D in-plane carbon/carbon composites. J. Solid Rocket Technol. 2013, 36, 811–815. [Google Scholar]
- Tang, M.; Gao, B.; Yang, Y.-C.; Shi, H.-B. Mechanical properties prediction of 4D-in plane carbon/carbon composites based on homogenization method. J. Solid Rocket Technol. 2011, 34, 109–112. [Google Scholar]
- Zhu, Z.; Qiang, H. Forecast research on micromechanical properties of 4D in-plane C/C composites basedon homogenization theory. J. Solid Rocket Technol. 2019, 42, 92–96. [Google Scholar]
- Shokrieh, M.M.; Mazloomi, M.S. A new analytical model for calculation of stiffness of three-dimensional four-directional braided composites. Compos. Struct. 2012, 94, 1005–1015. [Google Scholar] [CrossRef]
- Zhang, W.; Wang, F.; Dai, G. Topology optimal design of material microstructures using strain energy-based method. Chin. J. Aeronaut. 2007, 20, 321–328. [Google Scholar] [CrossRef]
- Yevtushenko, A.A.; Grzes, P.; Adamowicz, A. The temperature mode of the carbon-carbon multi-disc brake in the view of the interrelations of its operating characteristics. Materials 2020, 13, 1878. [Google Scholar] [CrossRef]
- Vignoles, G.L.; Aspa, Y.; Quintard, M. Modelling of carbon-carbon composite ablation in rocket nozzles. Compos. Sci. Technol. 2010, 70, 1303–1311. [Google Scholar] [CrossRef]
- Liu, F.; Guan, Z.; Bian, T. Damage model for predicting shear strength of carbon/carbon composite fastener based on post-failure behavior. Compos. Struct. 2019, 221, 110864.1–110864.17. [Google Scholar] [CrossRef]
- Mohammed, A.S.K.; Sehitoglu, H.; Rateick, R. Interface graphitization of carbon-carbon composites by nanoindentation. Carbon 2019, 150, 425–435. [Google Scholar] [CrossRef]
- Pimazzoni, A.; Cescon, E.; Dalla, P.M. Thermo-mechanical analysis of unidirectional carbon-carbon composite for thermal imaging diagnostic of a particle beam. Fusion Eng. Des. 2019, 146, 2457–2461. [Google Scholar] [CrossRef]
- Li, L.; Aliabadi, M.H. Elastic property prediction and damage mechanics analysis of 3D braided composite. Theor. Appl. Fract. Mech. 2019, 104, 102338. [Google Scholar] [CrossRef]
- Li, W.; Fang, G.; Li, W.; Liang, J.; Li, M. Role of mesoscopic features on thermochemical ablative behavior of 3D C/C braided composites. Int. J. Heat Mass Transf. 2019, 144, 118602. [Google Scholar] [CrossRef]
- Lachaud, J.; Aspa, Y.; Vignoles, L.G. Analytical modeling of the steady state ablation of a 3D C/C composite. Int. J. Heat Mass Transf. 2008, 51, 2614–2627. [Google Scholar] [CrossRef]
- Wang, C.G.; Xu, G.; Gong, J.L. Buckling instability of flexible joint under high pressure in solid rocket motor. Int. J. Aerosp. Eng. 2020, 2020, 10. [Google Scholar]
- Chinnaraj, R.K.; Hong, S.M.; Kim, H.S.; Oh, P.Y. Ablation experiments of ultra-high-temperature ceramic coating on carbon-carbon composite using ICP plasma wind tunnel. Int. J. Aeronaut. Space Sci. USA 2020, in press. [Google Scholar] [CrossRef]
- Wang, C.G.; Xu, G. The Analytical method to compute the strain on the soft PSD in double-pulse SRM. Int. J. Aerosp. Eng. 2019, 2019, 7. [Google Scholar] [CrossRef]
- Wang, C.; Chen, C.; Ren, Q.; Chen, K. Investigation on metal diaphragm of pulse separation device in dual-pulse solid rocket motor. Proc. Inst. Mech. Eng. 2017, 231, 444–451. [Google Scholar]
- Chowdhury, P.; Sehitoglu, H.; Rateick, R. Damage tolerance of carbon-carbon composites in aerospace application. Carbon 2018, 126, 382–393. [Google Scholar] [CrossRef]



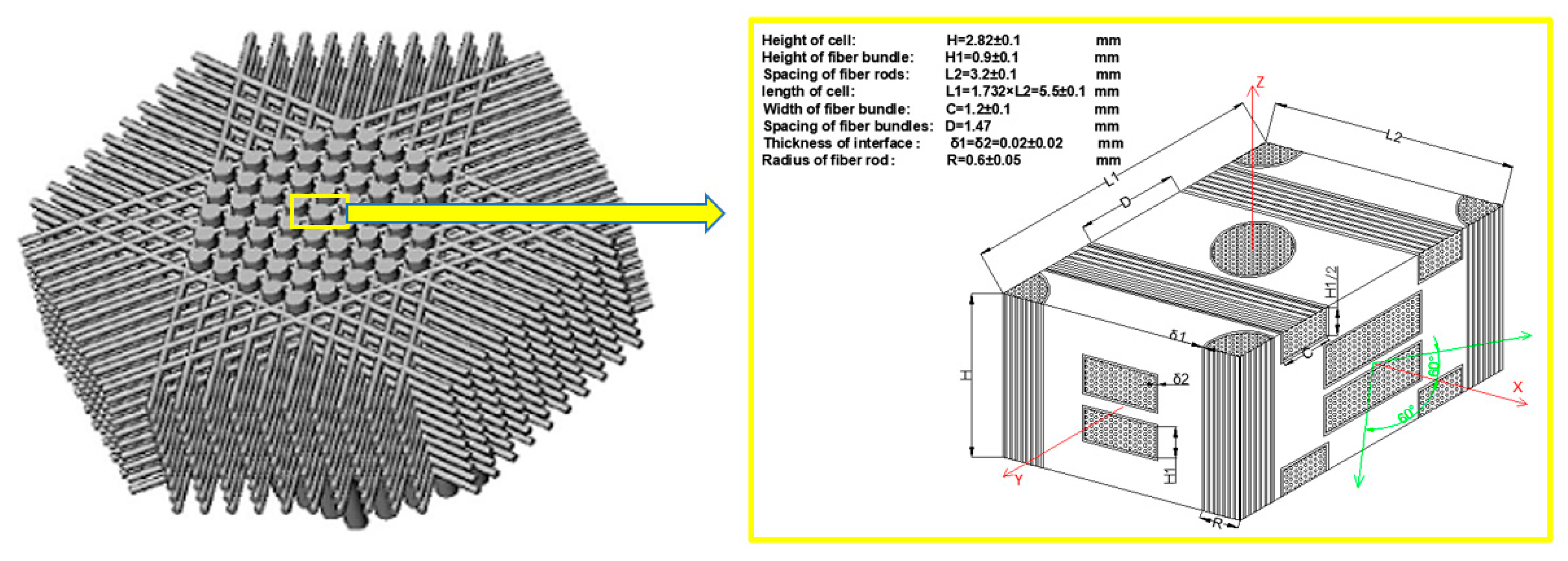
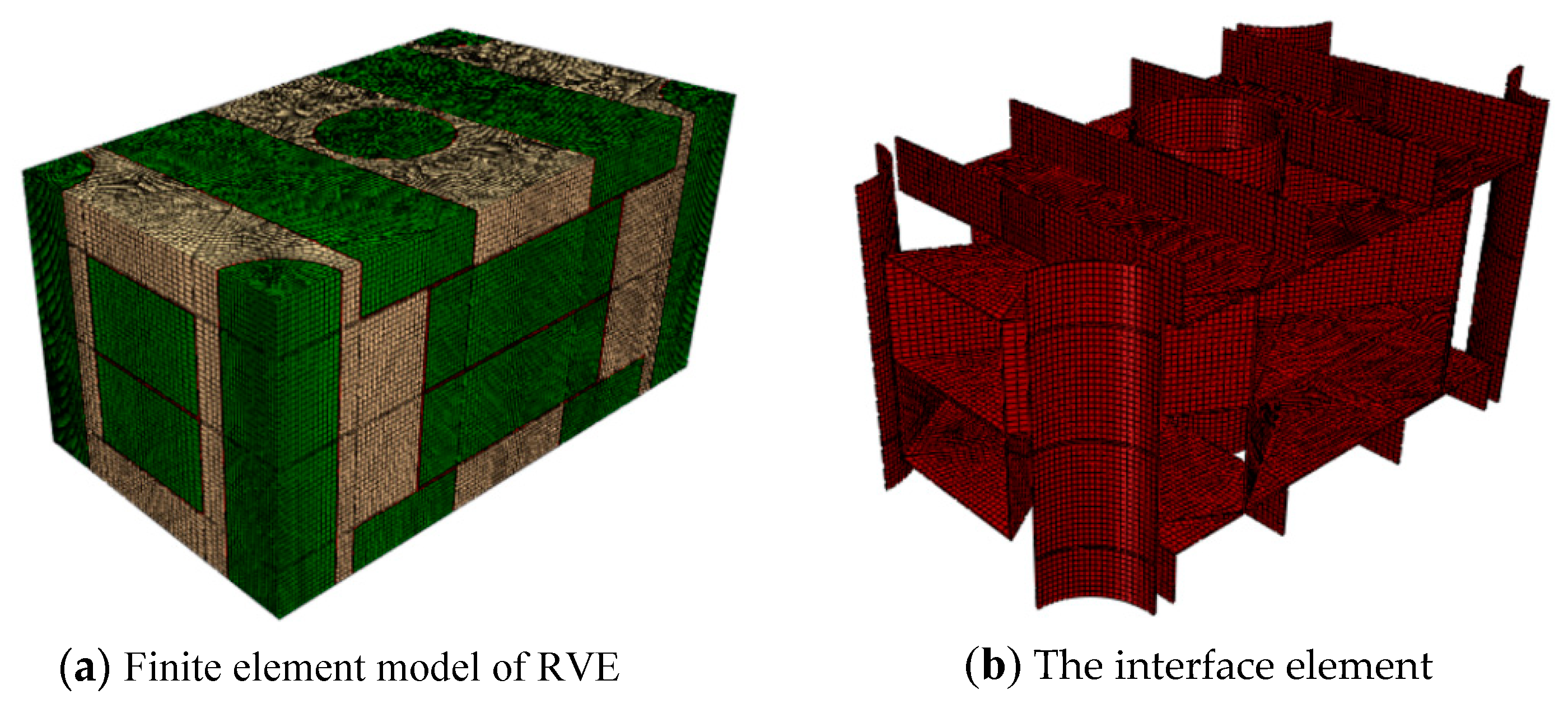


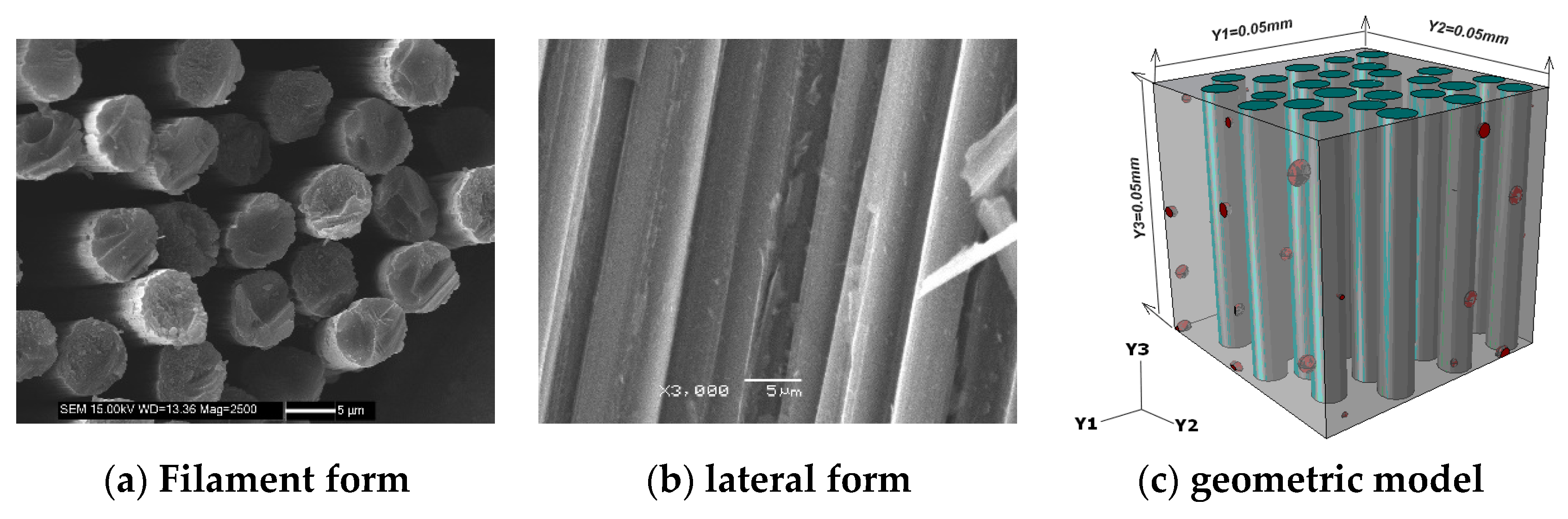
| Item | Ex, GPa | |||
|---|---|---|---|---|
| Mean Value | Standard Deviation | Mean Value | Standard Deviation | |
| Tension | 211.1 | 10.3 | 0.06 | 0.02 |
| Compression | 99.4 | 8.47 | 0.15 | 0.03 |
| Item | () | () | ||||||
|---|---|---|---|---|---|---|---|---|
| Mean Value | Standard Deviation | Mean Value | Standard Deviation | Mean Value | Standard Deviation | Mean Value | Standard Deviation | |
| Tension | 38.3 | 2.3 | 0.28 | 0.41 | 40.5 | 4.2 | 0.09 | 0.05 |
| Compression | 20.9 | 1.9 | 0.28 | 0.04 | 22.1 | 2.1 | 0.09 | 0.05 |
| Shear | 12.6 | 1.3 | 3.4 | 0.5 | ||||
| Item | αx, 10−6/K | αy = αz, 10−6/K | |||||
|---|---|---|---|---|---|---|---|
| T300 fiber | 345.1☆120.0★ | 19.0 | 26.7 | 0.2 | 0.35 | −0.78 | 8.14 |
| Matrix | 12.34 | - | - | 0.23 | - | −1.3 | −1.3 |
| Item | αx, 10−6/K | αy = αz, 10−6/K | |||||
|---|---|---|---|---|---|---|---|
| The predictive value of the fiber rod | 239.5☆85.8★ | 17.37 | 20.25 | 0.11 | 0.45 | −0.94 | 5.1 |
| Test value of fiber rod | 211.1☆99.4★ | 22.6 | 0.06 | - | - | - | |
| The predictive value of fiber bundle | 220.8☆79.1★ | 17.11 | 18.96 | 0.12 | 0.40 | −0.99 | 4.52 |
| Item | Elastic Modulus GPa | Poisson’s Ratio | Shear Modulus GPa | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Predicted value (tension) | 37.0 | 33.6 | 44.6 | 0.37 | 0.09 | 0.11 | 12.2 | 3.3 | 2.5 |
| Experimental value (tension) | 38.3 | - | 40.5 | 0.28 | 0.09 | - | 12.6 | 3.4 | - |
| Predicted value (compression) | 22.3 | 20.5 | 21.1 | 0.38 | 0.12 | 0.15 | - | - | - |
| Experimental value (compression) | 20.9 | - | 22.1 | 0.28 | 0.09 | - | - | - | - |
| Item | Axial Coefficient of Thermal Expansion, 10−6/K | Radial Coefficient of Thermal Expansion, 10−6/K |
|---|---|---|
| Predicted value | 0.147 | −0.477 |
| Experimental value | 0.145 | −0.50 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, C.; Cao, P.; Tang, M.; Tian, W.; Liu, K.; Liu, B. Study on Properties Prediction and Braiding Optimization of Axial Braided Carbon/Carbon Composite. Materials 2020, 13, 2588. https://doi.org/10.3390/ma13112588
Wang C, Cao P, Tang M, Tian W, Liu K, Liu B. Study on Properties Prediction and Braiding Optimization of Axial Braided Carbon/Carbon Composite. Materials. 2020; 13(11):2588. https://doi.org/10.3390/ma13112588
Chicago/Turabian StyleWang, Chunguang, Peng Cao, Min Tang, Weiping Tian, Ketong Liu, and Baorui Liu. 2020. "Study on Properties Prediction and Braiding Optimization of Axial Braided Carbon/Carbon Composite" Materials 13, no. 11: 2588. https://doi.org/10.3390/ma13112588
APA StyleWang, C., Cao, P., Tang, M., Tian, W., Liu, K., & Liu, B. (2020). Study on Properties Prediction and Braiding Optimization of Axial Braided Carbon/Carbon Composite. Materials, 13(11), 2588. https://doi.org/10.3390/ma13112588





