Dealing with the Effect of Air in Fluid Structure Interaction by Coupled SPH-FEM Methods
Abstract
1. Introduction
2. Materials and Methods
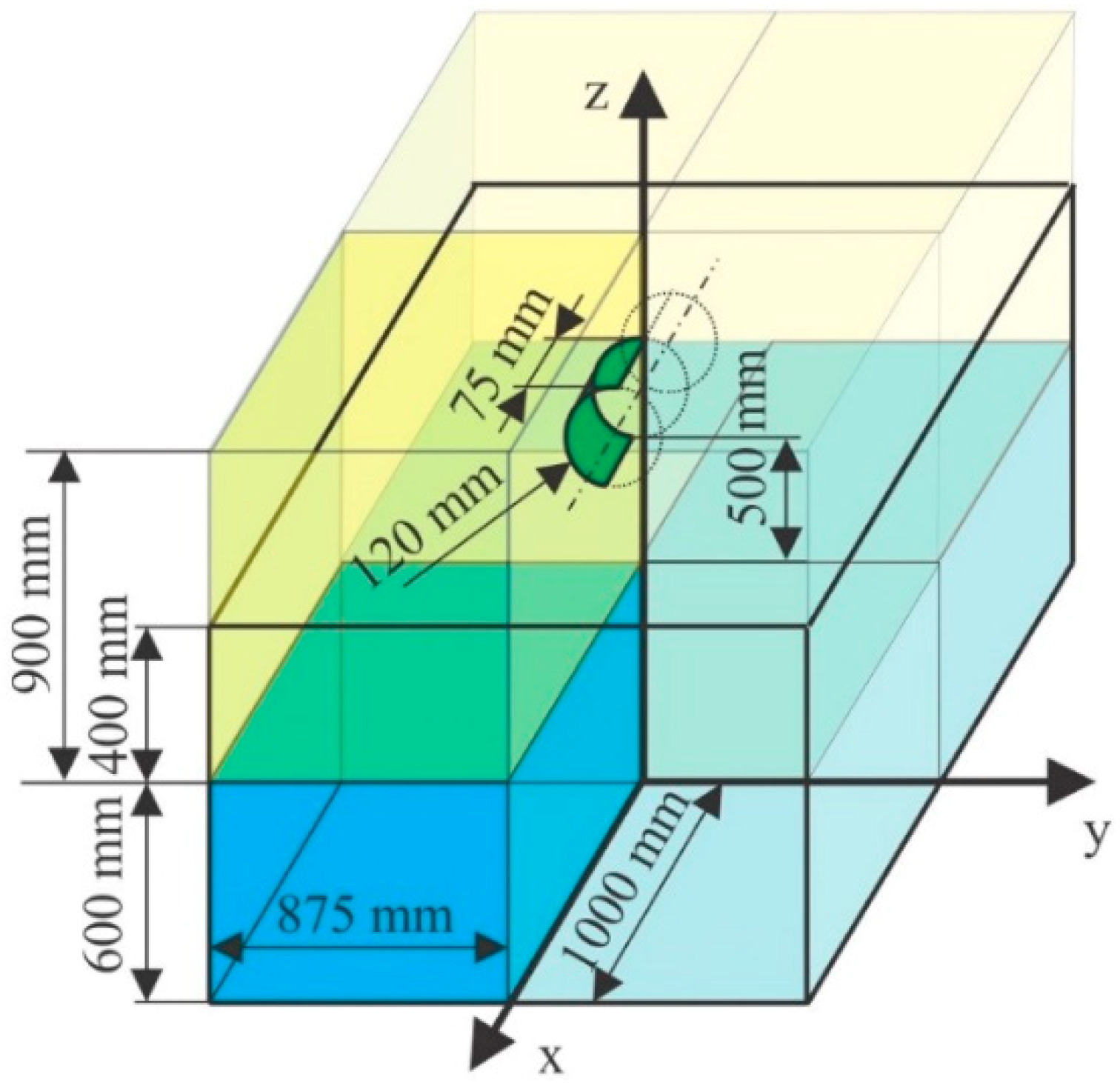
2.1. Geometry
2.2. Materials Properties
2.3. Kernel Approximation
2.4. Particle Approximation
2.5. SPH for Viscous Fluids
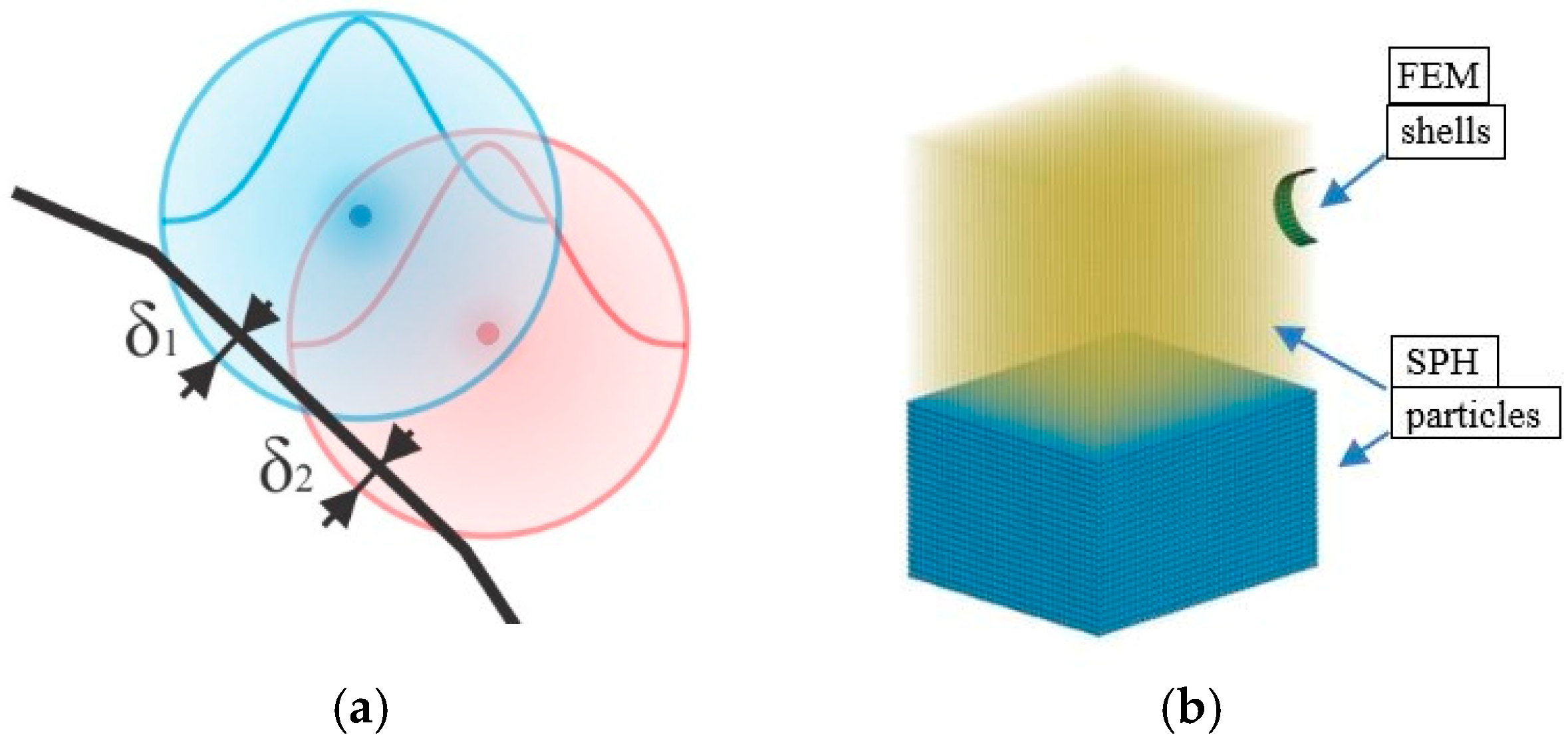
2.6. Contacts in a Coupled FEM-SPH
2.7. System Discretization
- -
- 76 shell elements, 1.8 mm thick, with a total amount of 100 nodes, for the flexible cylinder;
- -
- 33,600 particles for the water;
- -
- 50,500 articles for air, when considered.
3. Numerical Results
3.1. Free Fall
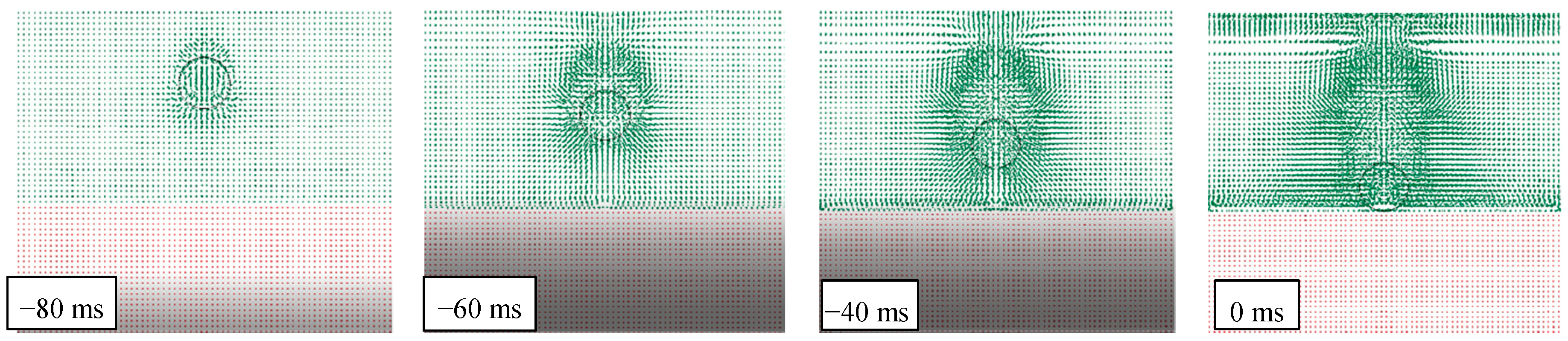
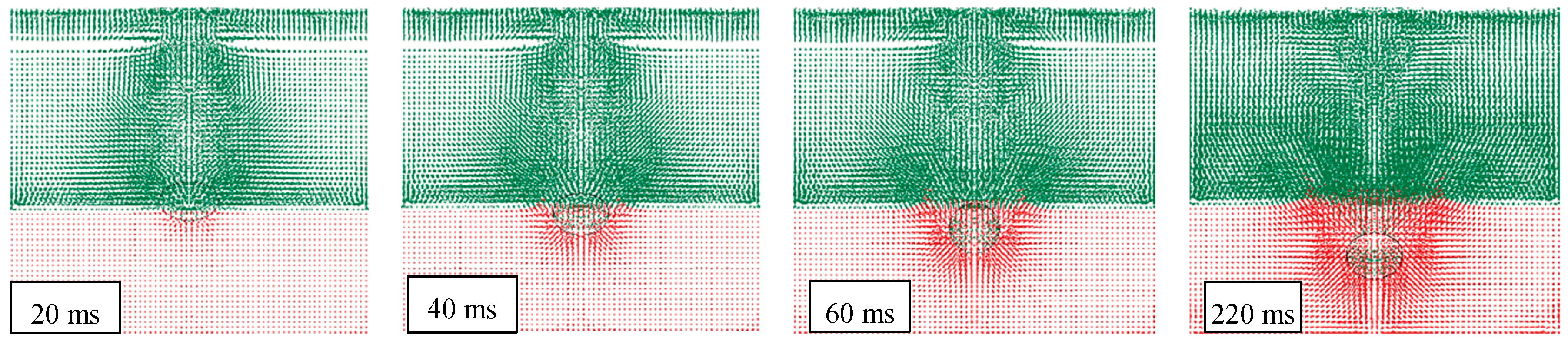
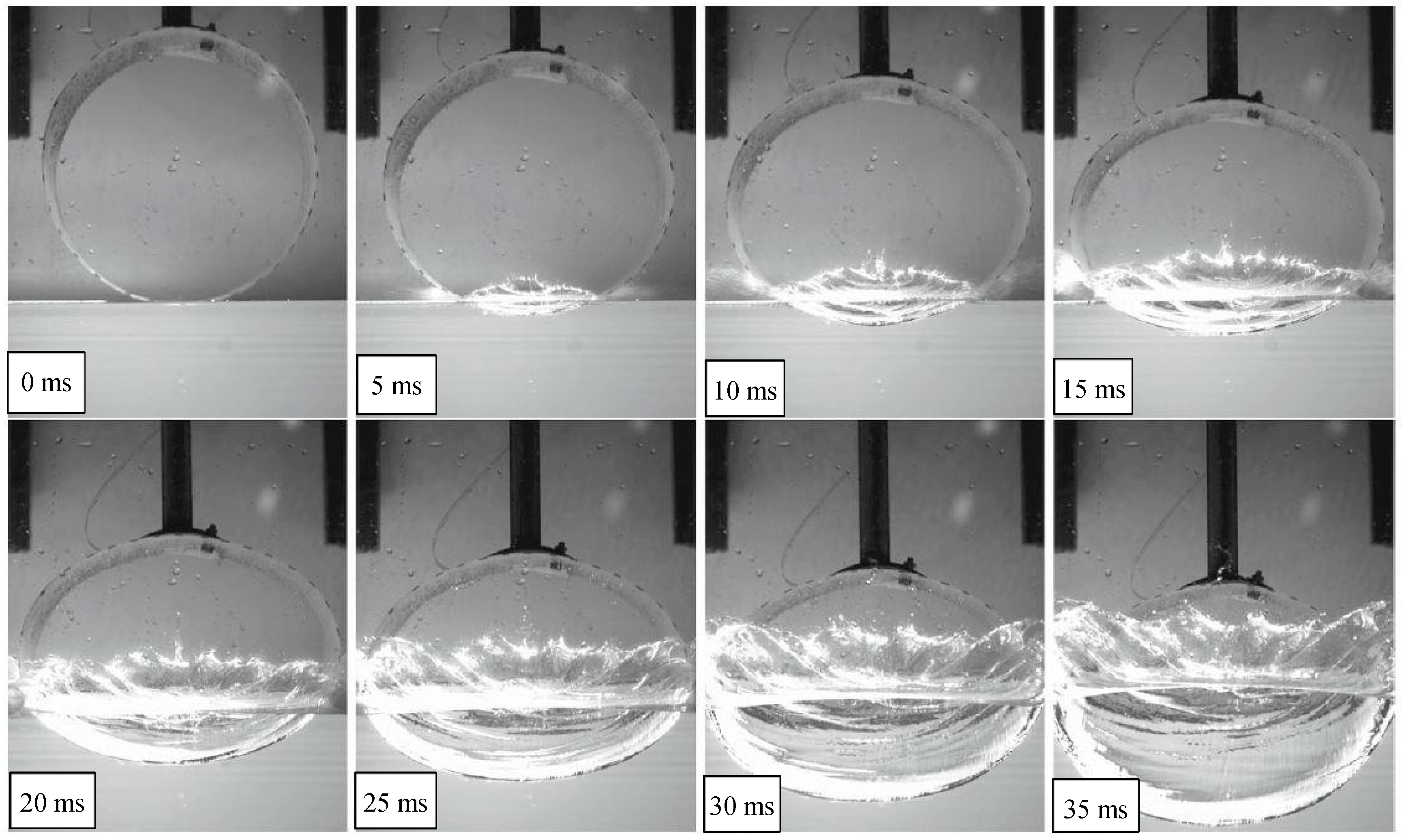
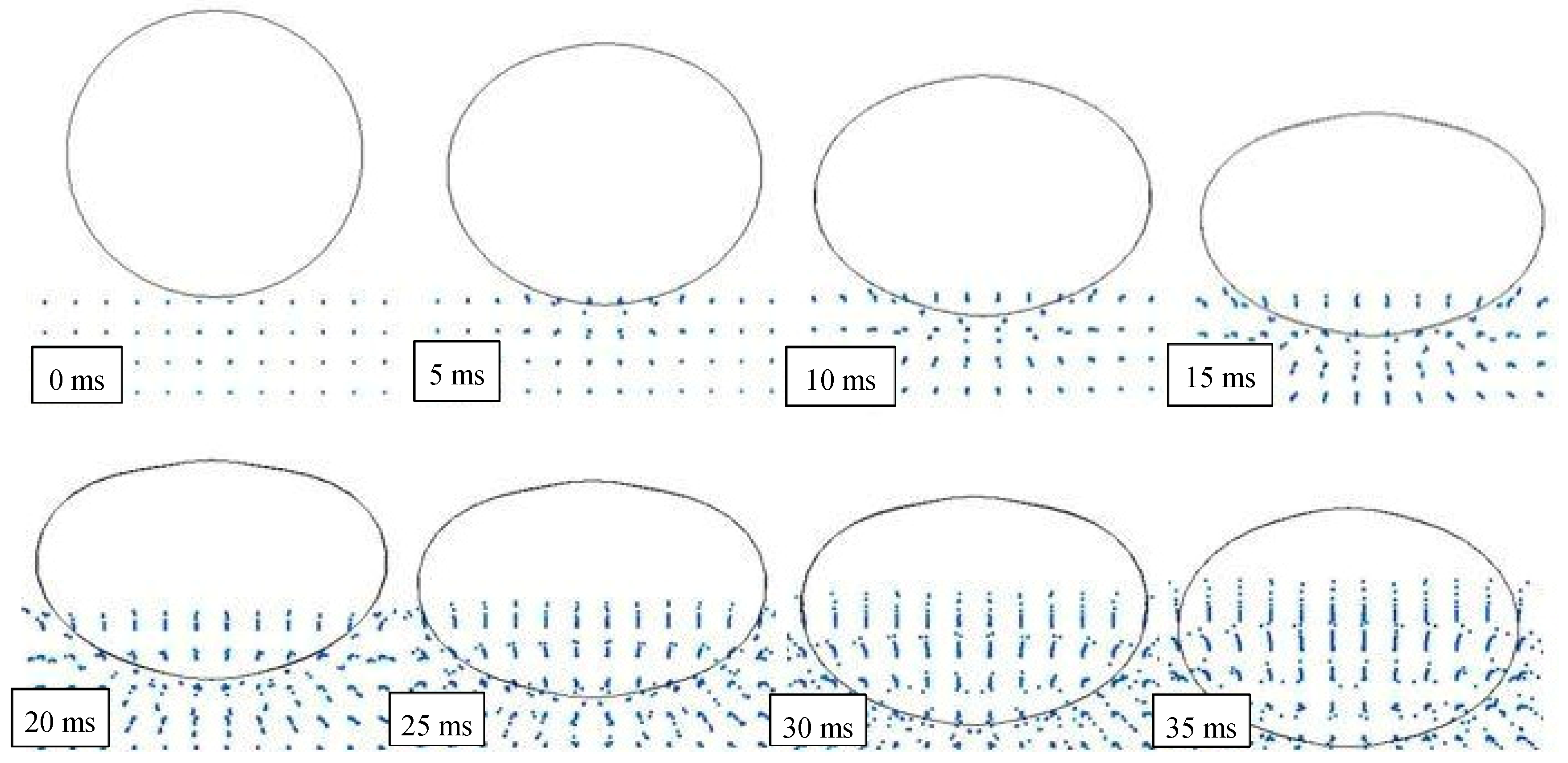
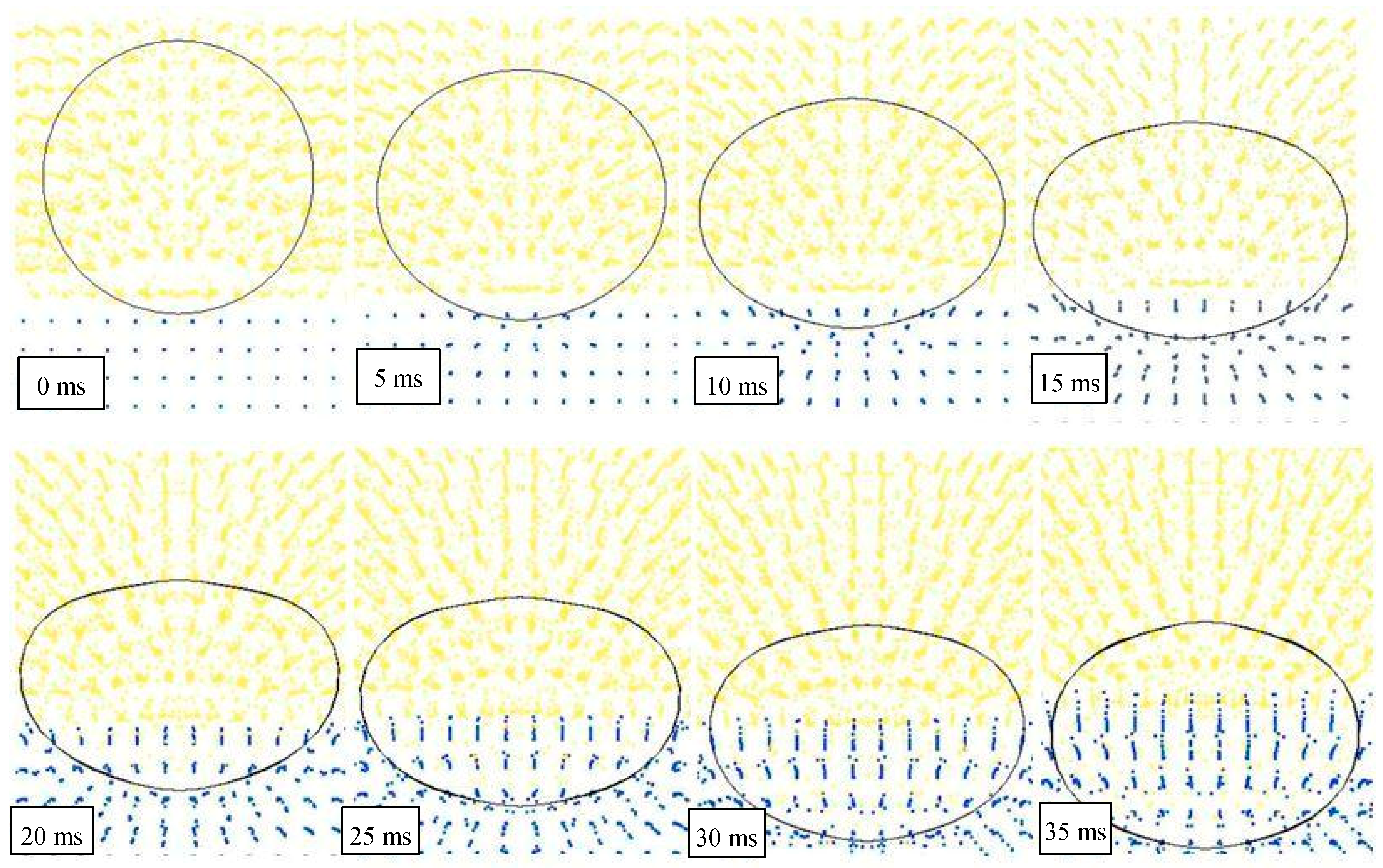
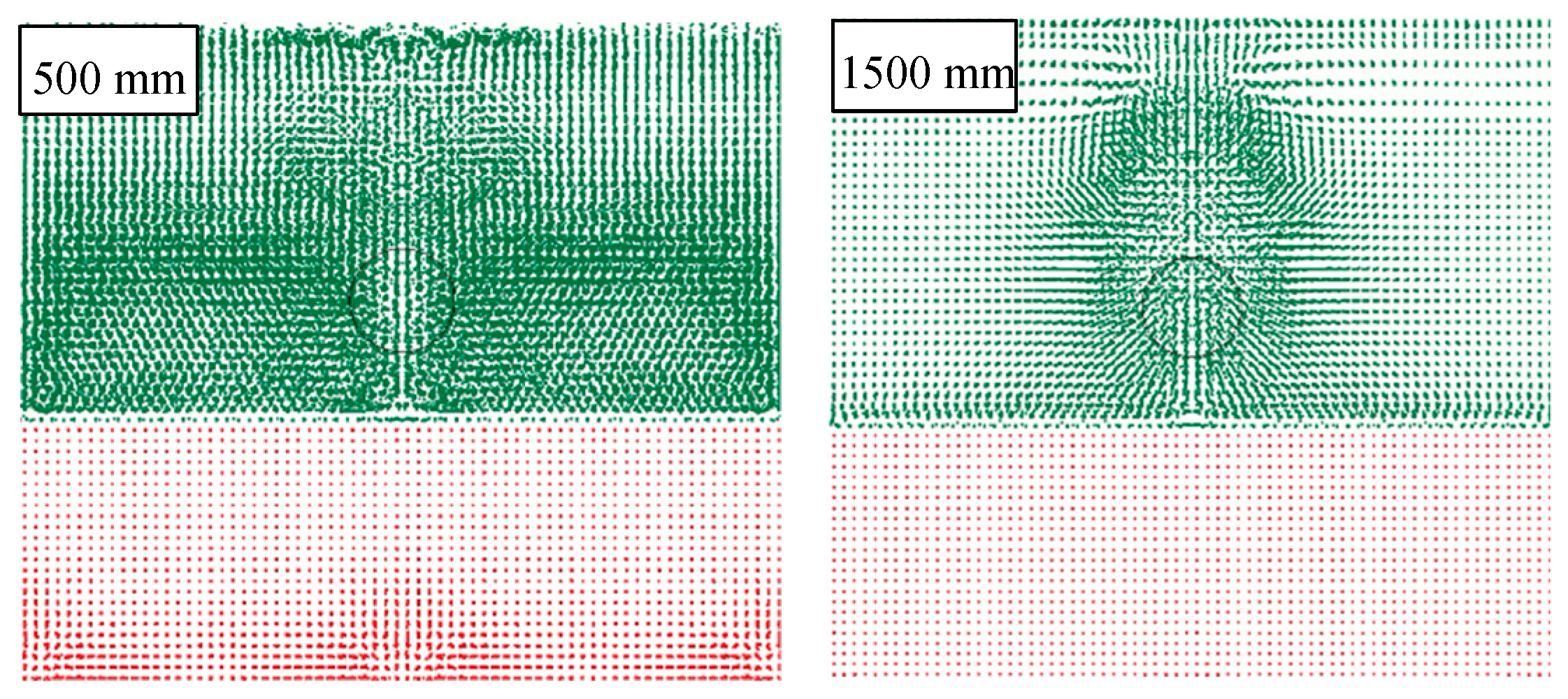
3.2. Water Penetration
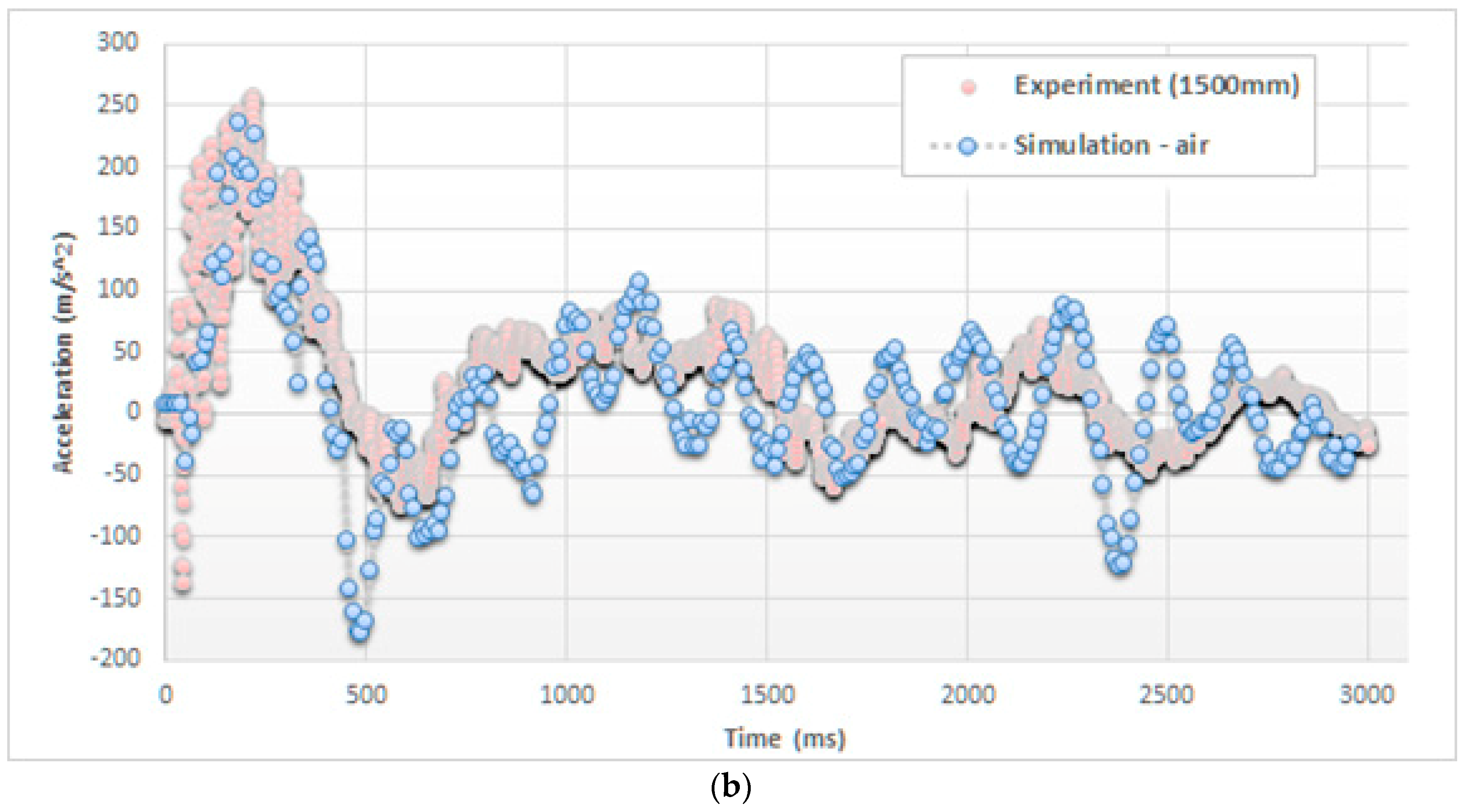
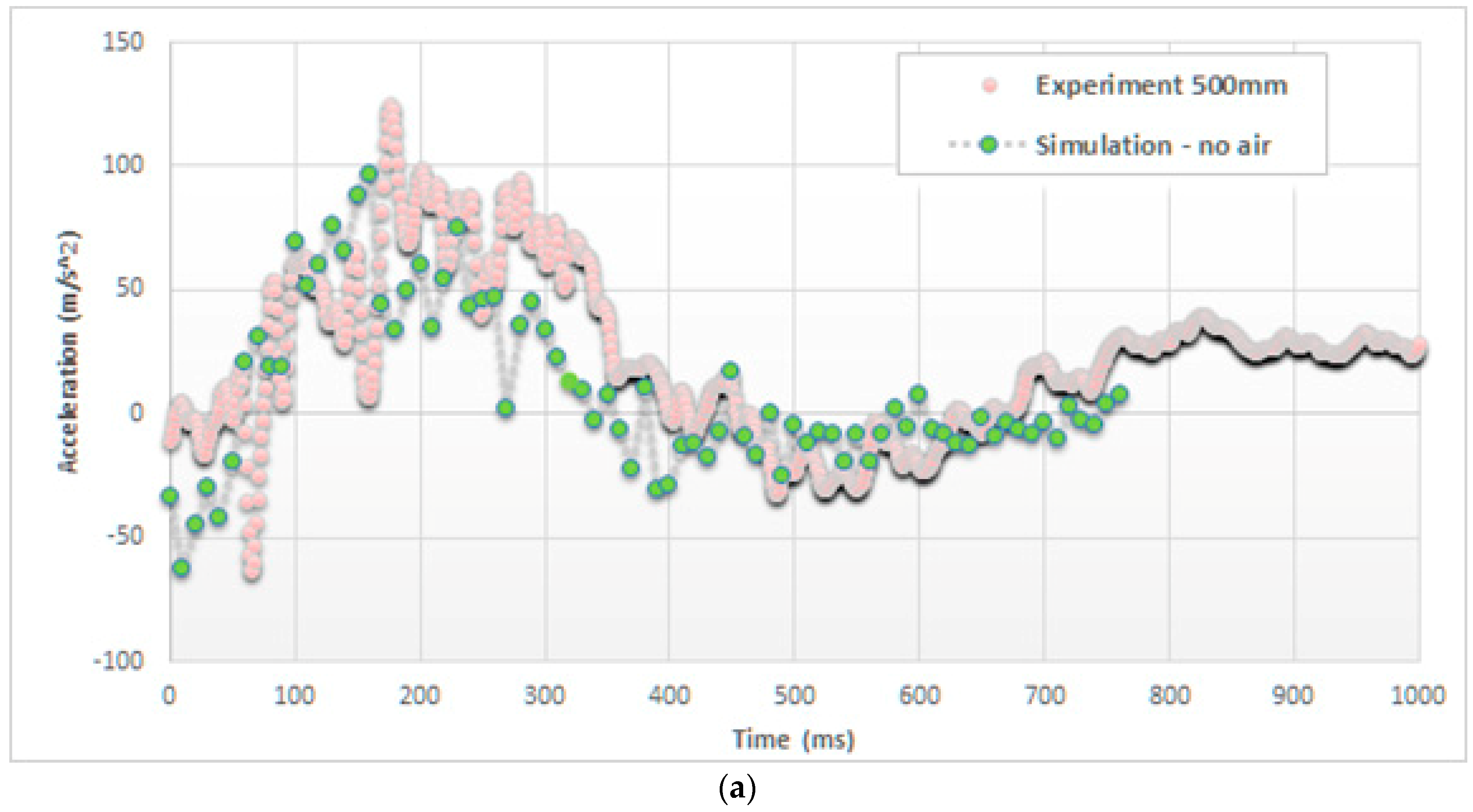
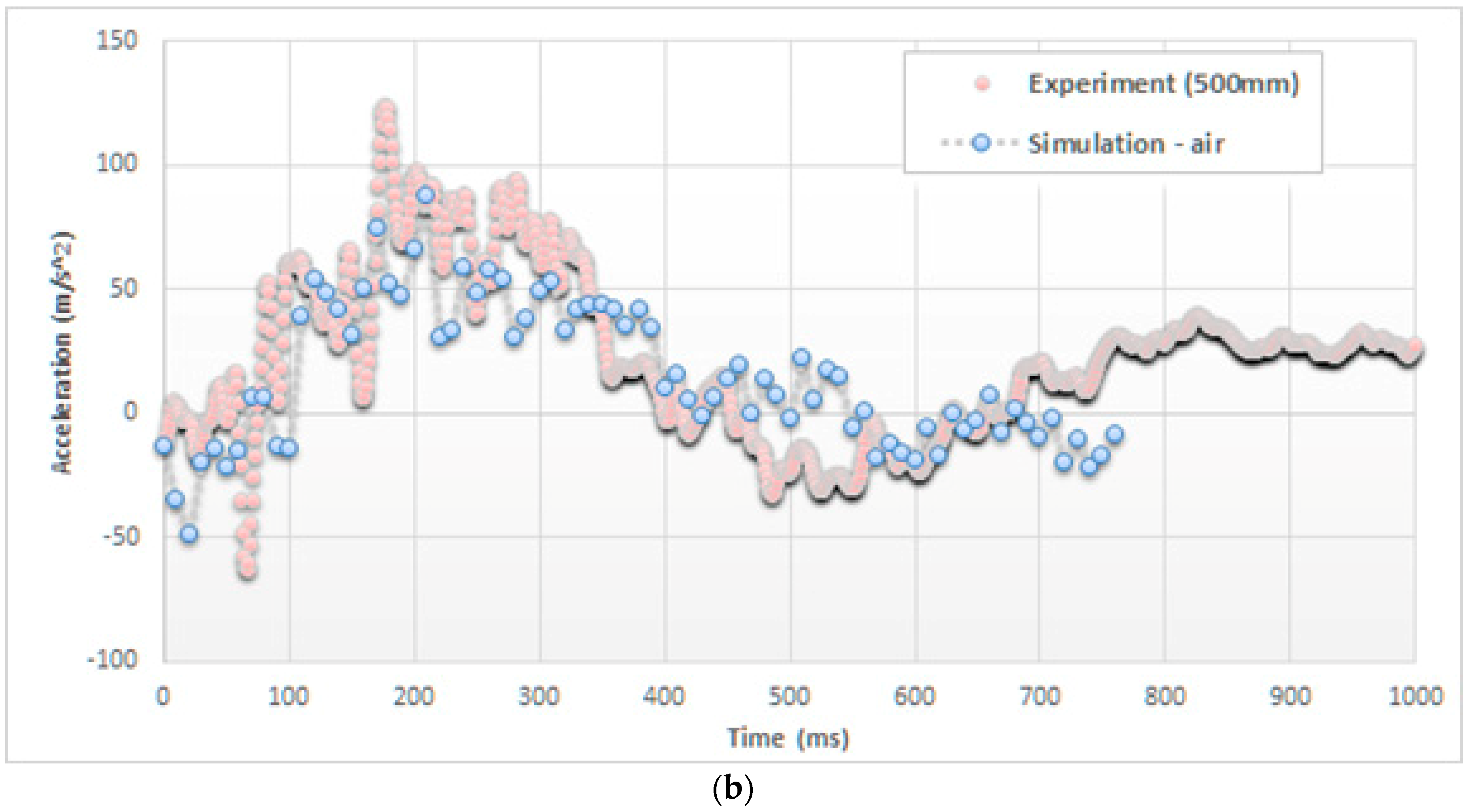
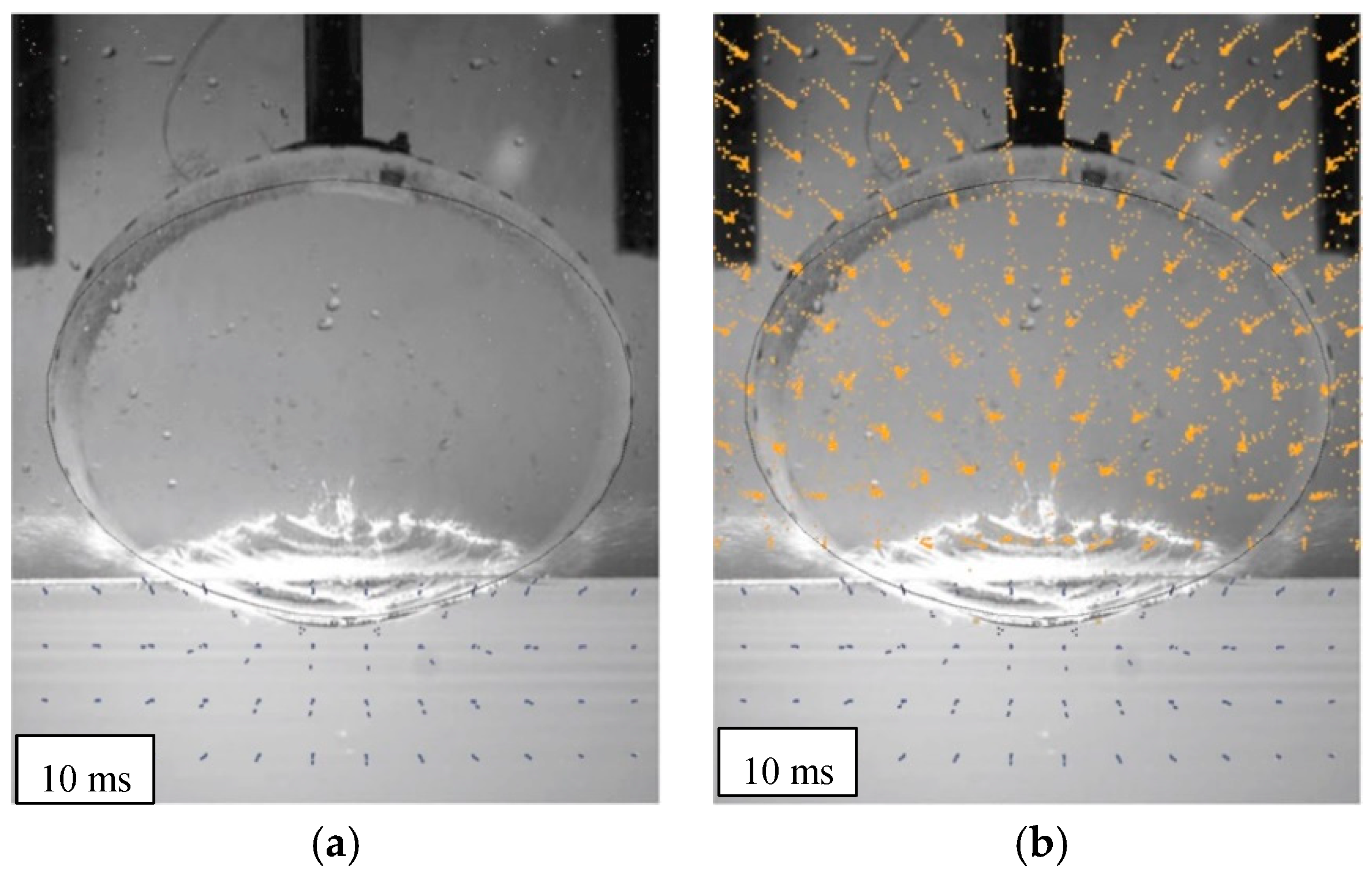
4. Simulation vs. Experiment
4.1. Accelerations
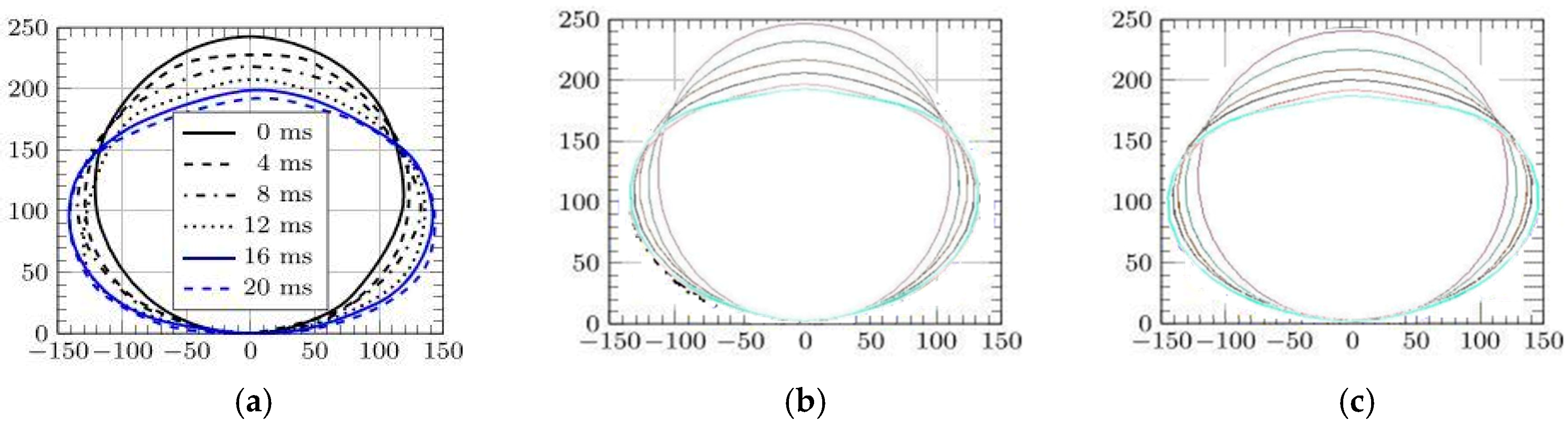
4.2. Deformations
4.3. Algorithm Efficacy
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Bathe, K.J. Finite Element Procedures; Prentice Hall: Upper Saddle River, NJ, USA, 2007. [Google Scholar]
- Wittenberg, T.C.; Van Baten, T.J.; De Boer, A. Design of fiber metal laminate shear panels for ultra-high capacity aircraft. Aircr. Des. 2011, 4, 99–113. [Google Scholar] [CrossRef]
- Fragassa, C. Engineering Design Driven by Models and Measures: The Case of a Rigid Inflatable Boat. J. Mar. Sci. Eng. 2019, 7, 6. [Google Scholar] [CrossRef]
- Javid, I.; Zhu, S. Modeling and Simulation of Ship Structures Using Finite Element Method. Int. J. Ind. Manuf. Eng. 2018, 12, 941–947. [Google Scholar]
- Lee, H.; Song, M.C.; Suh, J.C.; Chang, B.J. Hydro-elastic analysis of marine propellers based on a BEM-FEM coupled FSI algorithm. Int. J. Nav. Archit. Ocean Eng. 2014, 6, 562–577. [Google Scholar] [CrossRef]
- Jiapeng, J.; Ping, X.; Baoyuan, Z.; Bifu, H. A finite element parametric modeling technique of aircraft wing structures. Chin. J. Aeronaut. 2013, 5, 1202–1210. [Google Scholar]
- Nguyen, V.; Zehn, M.; Marinkovic, D. An efficient co-rotational FEM formulation using a projector matrix. Facta Univ. Ser. Mech. Eng. 2016, 14, 227–240. [Google Scholar] [CrossRef]
- Felippa, C.A.; Haugen, B. Unifield Formulation of Small-Strain Corotational Finite Elements: I. Theory; Center for Aerospace Structures, College of Engineering, University of Colorado: Boulder, CO, USA, 2005. [Google Scholar]
- Marinkovic, D.; Zehn, M.; Rama, G. Towards real-time simulation of deformable structures by means of co-rotational finite element formulation. Meccanica 2018, 53, 3123–3136. [Google Scholar] [CrossRef]
- Randolph, M.; Wang, D.; Zhou, H.; Hossain, M.S.; Hu, Y. Large Deformation Finite Element Analysis for Offshore Applications. In Proceedings of the 12th International Conference of International Association for Computer Methods and Advances in Geomechanics, Goa, India, 1–6 October 2008. [Google Scholar]
- Qu, Q.; Wang, R.; Guo, H.; Liu, P. Numerical Study of Water Impact of an Elastic Cylindrical Shell. AIAA J. 2016, 10, 3296–3303. [Google Scholar] [CrossRef]
- Vignjevic, R.; Djordjevic, N.; Gemkow, S.; De Vuyst, T.; Campbell, J. SPH as a nonlocal regularisation method: Solution for instabilities due to strain-softening. Comput. Methods Appl. Mech. Eng. 2014, 277, 281–304. [Google Scholar] [CrossRef]
- Herreros, M.I.; Mabssout, M. A two-steps time discretization scheme using the SPH method for shock wave propagation. Comput. Methods Appl. Mech. Eng. 2011, 200, 1833–1845. [Google Scholar] [CrossRef]
- Jackson, K.E.; Fuchs, Y.T. Comparison of ALE and SPH simulations of vertical drop tests of a composite fuselage section into water. In Proceedings of the 10th International LS-DYNA Conference, Dearborn, MI, USA, 8–10 June 2008. [Google Scholar]
- Wagner, H. Über Stoß-und Gleitvorgänge an der Oberfläche von Flüssigkeiten. ZAMM J. Appl. Math. Mech. 1932, 12, 193–215. [Google Scholar] [CrossRef]
- Von Karman, T. The Impact on Seaplane Floats During Landing; National Advisory Committee for Aeronautics: Washington, DC, USA, 1929.
- Kvalsvold, J.; Faltinsen, O.M. Hydroelastic modelling of wet deck slamming on multihull vessels. J. Ship Res. 1995, 39, 225–239. [Google Scholar]
- Faltinsen, O.M. The effect of hydroelasticity on ship slamming. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 1997, 355, 575–591. [Google Scholar] [CrossRef]
- Faltinsen, O.M.; Kvålsvold, J.; Aarsnes, J.V. Wave impact on a horizontal elastic plate. J. Mar. Sci. Technol. 1997, 2, 87–100. [Google Scholar] [CrossRef]
- Faltinsen, O.M. Water entry of a wedge by hydroelastic orthotropic plate theory. J. Ship Res. 1999, 43, 180–193. [Google Scholar]
- Korobkin, A.; Guéret, R.; Malenica, S. Hydroelastic coupling of beam finite element model with Wagner theory of water impact. J. Fluids Struct. 2006, 22, 493–504. [Google Scholar] [CrossRef]
- Lu, C.; He, Y.; Wu, G. Coupled analysis of nonlinear interaction between fluid and structure during impact. J. Fluids Struct. 2000, 14, 127–146. [Google Scholar] [CrossRef]
- Zhao, R.; Faltinsen, O.M. Water entry of two-dimensional bodies. J. Fluid Mech. 1993, 246, 593–612. [Google Scholar] [CrossRef]
- Bereznitski, A. Slamming: The role of hydroelasticity. Int. Shipbuild. Prog. 2001, 4, 333–351. [Google Scholar]
- Le Sourne, H.; County, N.; Besnier, F.; Kammerer, C.; Legavre, H. LS-DYNA Applications in Shipbuilding. In Proceedings of the 4th European LS-DYNA Users Conference, Ulm, Germany, 22–23 May 2003; pp. 1–16. [Google Scholar]
- Stenius, I. Finite Element Modelling of Hydroelasticity Hull-Water Impacts. Ph.D. Thesis, Royal Institute of Technology, KTH, Stockholm, Sweden, 2006. [Google Scholar]
- Liu, G.-R. Mesh Free Methods: Moving Beyond the Finite Element Method; CRC Press: Boca Raton, FL, USA, 2002. [Google Scholar]
- Campbell, J.C.; Vignjevic, R. Simulating structural response to water impact. Int. J. Impact Eng. 2012, 49, 1–10. [Google Scholar] [CrossRef]
- Lucy, L.B. A numerical approach to the testing of the fission hypothesis. Astron. J. 1977, 82, 1013–1024. [Google Scholar] [CrossRef]
- Gingold, R.A.; Monaghan, J.J. Smoothed particle hydrodynamics: Theory and application to non-spherical stars. Mon. Not. R. Astron. Soc. 1977, 181, 375–389. [Google Scholar] [CrossRef]
- Monaghan, J.J.; Pongracic, H. Artificial viscosity for particle methods. Appl. Numer. Math. 1985, 1, 187–194. [Google Scholar] [CrossRef]
- La Rocca, G.; Van Tooren, M.J. Knowledge-based engineering approach to support aircraft multidisciplinary design and optimization. J. Aircr. 2009, 46, 1875–1885. [Google Scholar] [CrossRef]
- Liu, M.B.; Liu, G.R. Smoothed particle hydrodynamics (SPH): An overview and recent developments. Arch. Comput. Methods Eng. 2010, 17, 25–76. [Google Scholar] [CrossRef]
- Liu, M.B.; Liu, G.R.; Lam, K.Y.; Zong, Z. Smoothed particle hydrodynamics for numerical simulation of underwater explosion. Comput. Mech. 2003, 30, 106–118. [Google Scholar] [CrossRef]
- Fourtakas, G.; Stansby, P.K.; Rogers, B.D.; Lind, S.J. An Eulerian-Lagrangian incompressible SPH formulation (ELI-SPH) connected with a sharp interface. Comput. Methods Appl. Mech. Eng. 2008, 329, 532–552. [Google Scholar] [CrossRef]
- Hu, D.; Long, T.; Xiao, Y.; Han, X.; Gu, Y. Fluid-structure interaction analysis by coupled FE-SPH model based on a novel searching algorithm. Comput. Methods Appl. Mech. Eng. 2014, 276, 266–286. [Google Scholar] [CrossRef]
- Groenenboom, P.; Siemann, M. Fluid-structure interaction by the mixed SPH-FE method with application to aircraft ditching. Int. J. Multiphys. 2016, 9, 249–265. [Google Scholar] [CrossRef]
- Xiao, Y.; Dong, H.; Zhan, H.; Gu, Y. A new particle generation method for arbitrary 2D geometries in SPH modeling. Int. J. Comput. Methods 2017, 14, 1750023. [Google Scholar] [CrossRef]
- Xu, J.; Wang, J. Interaction methods for the SPH parts (multiphase flows, solid bodies) in LS-DYNA. In Proceedings of the 13th International LS-DYNA Users Conference, Detroit, MI, USA, 8–10 June 2014. [Google Scholar]
- Cercos-Pita, J.L.; Antuono, M.; Colagrossi, A.; Souto-Iglesias, A. SPH energy conservation for fluid–solid interactions. Comput. Methods Appl. Mech. Eng. 2017, 317, 771–791. [Google Scholar] [CrossRef]
- Khabakhpasheva, T.I. Fluid-Structure Interaction During the Impact of a Cylindrical Shell on a Thin Layer of Water. J. Fluids Struct. 2009, 25, 431–444. [Google Scholar] [CrossRef]
- Panciroli, R.; Ubertini, S.; Minak, G.; Jannelli, E. Experiments on the dynamics of flexible cylindrical shells impacting on a water surface. Exp. Mech. 2015, 55, 1537–1550. [Google Scholar] [CrossRef]
- Panciroli, R.; Abrate, S.; Minak, G.; Zucchelli, A. Hydroelasticity in water-entry problems: Comparison between experimental and SPH results. Compos. Struct. 2012, 94, 532–539. [Google Scholar] [CrossRef]
- Climent, H.; Benitez, L.; Rosich, F.; Rueda, F.; Pentecote, N. Aircraft ditching numerical simulation. In Proceedings of the 25th International Congress of the Aeronautical Sciences, Hamburg, Germany, 3–8 September 2006. [Google Scholar]
- Siemann, M.H.; Langrand, B. Coupled fluid-structure computational methods for aircraft ditching simulations: Comparison of ALE-FE and SPH-FE approaches. Comput. Struct. 2017, 188, 95–108. [Google Scholar] [CrossRef]
- Groenenboom, P.H.; Campbell, J.; Benítez Montañés, L.; Siemann, M.H. Innovative SPH methods for aircraft ditching. In Proceedings of the 11th WCCM/5th ECCM, Barcelona, Spain, 20–25 July 2014. [Google Scholar]
- Panciroli, R.; Abrate, S.; Minak, G. Dynamic response of flexible wedges entering the water. Compos. Struct. 2013, 99, 163–171. [Google Scholar] [CrossRef]
- De Camargo, F.V. Survey on Experimental and Numerical Approaches to Model Underwater Explosions. J. Mar. Sci. Eng. 2019, 7, 15. [Google Scholar] [CrossRef]
- Gong, K.; Shao, S.; Liu, H.; Lin, P.; Gui, Q. Cylindrical Smoothed Particle Hydrodynamics Simulations of Water Entry. J. Fluids Eng. 2019, 141, 071303. [Google Scholar] [CrossRef]
- Antoci, C.; Gallati, M.; Sibilla, S. Numerical simulation of fluid-structure interaction by SPH. Comput. Struct. 2007, 85, 879–890. [Google Scholar] [CrossRef]
- Adami, S.; Hu, X.Y.; Adam, N.A. Simulating three-dimensional turbulence with SPH. Cent. Turbul. Res. Proc. Summer Progr. 2012, 2012, 177–185. [Google Scholar]
- Panciroli, R.; Pagliaroli, T.; Minak, G. On Air-Cavity Formation during Water Entry of Flexible Wedges. J. Mar. Sci. Eng. 2018, 6, 155. [Google Scholar] [CrossRef]
- Fragassa, C.; de Camargo, F.V.; Pavlovic, A.; Minak, G. Explicit numerical modeling assessment of basalt reinforced composites for low-velocity impact. Compos. Part B Eng. 2019, 163, 522–535. [Google Scholar] [CrossRef]
- Shao, S.; Gotoh, H. Turbulence particle models for tracking free surfaces. J. Hydraul. Res. 2005, 43, 276–289. [Google Scholar] [CrossRef]
- Gotoh, H.; Shibahara, T.; Sakai, T. Sub-Particle-Scale Turbulence Model for the MPS Method—Lagrangian Flow Model for Hydraulic Engineering. Comp. Fluid Dyn. J. 2001, 9, 339–347. [Google Scholar]
- Gong, K.; Shao, S.; Liu, H.; Wang, B.; Tan, S.K. Two-phase SPH simulation of fluid-structure interactions. J. Fluids Struct. 2016, 65, 155–179. [Google Scholar] [CrossRef]


| *CONTACT_AUTOMATIC_NODES_TO_SURFACE | |||||
|---|---|---|---|---|---|
| FS | Static Coefficient of Friction | 0 | SFST | Scale factor for slave surface | 1 |
| FD | Dynamic Coefficient of Friction | 0 | SFMT | Scale factor for master surface | 1 |
| DC | Exponential decay coefficient | 0 | FSF | Coulomb frictional scale factor | 1 |
| VC | Coefficient for viscous friction | 0 | VSF | Viscous frictional Scale factor | 1 |
| VDC | Viscous damping Coefficient | 0 | SOFT | Soft constraint option (Penalty) | 0 |
| PENCHK | Small penetration in Contact | 0 | SOFSCL | Scale factor for constraint forces | 0.1 |
| BT | Birth time | 0 | LCIDAB | Load curve ID defining airbag | 0 |
| DT | Death time | 1020 | MAXPAR | Maximum parametric coordinate | 1.025 |
| SFS | Scale factor on slave | 1–0.5 | SBOPT | Segment-based contact options | 2 |
| SFM | Scale factor on master | 1–0.5 | DEPTH | Search depth in automatic contact | 2 |
| SST | Optional thickness for slave | - | BSORT | Number of cycle between bucket sorts | - |
| MST | Optional thickness for master | - | FRCFRQ | Number of cycle between contact force | 1 |
| *DEFINE_SPH_TO_SPH_COUPLING | |||||
|---|---|---|---|---|---|
| PFACT | Penalty scale factor | 0.7 | DFACT | Penalty scale factor for contact damping coefficient | 1 |
| SRAD | Scale factor nodes to nodes contact | 1.0 | |||
| Smoothing Length | Drop Height | ||||
|---|---|---|---|---|---|
| Air | 1.0 | 1.1 | 1.2 | Δ | |
| without | 1 | 1.12 | 1.43 | 43% | 500 mm |
| with | 2.68 | 3.22 | 4.06 | 51% | |
| Δ | 168% | 187% | 184% | ||
| without | 0.97 | 1.13 | 1.50 | 54% | 1500 mm |
| with | 2.76 | 3.30 | 4.95 | 79% | |
| Δ | 183% | 192% | 230% | ||
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fragassa, C.; Topalovic, M.; Pavlovic, A.; Vulovic, S. Dealing with the Effect of Air in Fluid Structure Interaction by Coupled SPH-FEM Methods. Materials 2019, 12, 1162. https://doi.org/10.3390/ma12071162
Fragassa C, Topalovic M, Pavlovic A, Vulovic S. Dealing with the Effect of Air in Fluid Structure Interaction by Coupled SPH-FEM Methods. Materials. 2019; 12(7):1162. https://doi.org/10.3390/ma12071162
Chicago/Turabian StyleFragassa, Cristiano, Marko Topalovic, Ana Pavlovic, and Snezana Vulovic. 2019. "Dealing with the Effect of Air in Fluid Structure Interaction by Coupled SPH-FEM Methods" Materials 12, no. 7: 1162. https://doi.org/10.3390/ma12071162
APA StyleFragassa, C., Topalovic, M., Pavlovic, A., & Vulovic, S. (2019). Dealing with the Effect of Air in Fluid Structure Interaction by Coupled SPH-FEM Methods. Materials, 12(7), 1162. https://doi.org/10.3390/ma12071162







