Equivalent Method of Joint Interface Based on Persson Contact Theory: Virtual Material Method
Abstract
1. Introduction
2. Materials and Methods
2.1. Virtual Material Method Based on Persson Contact Theory
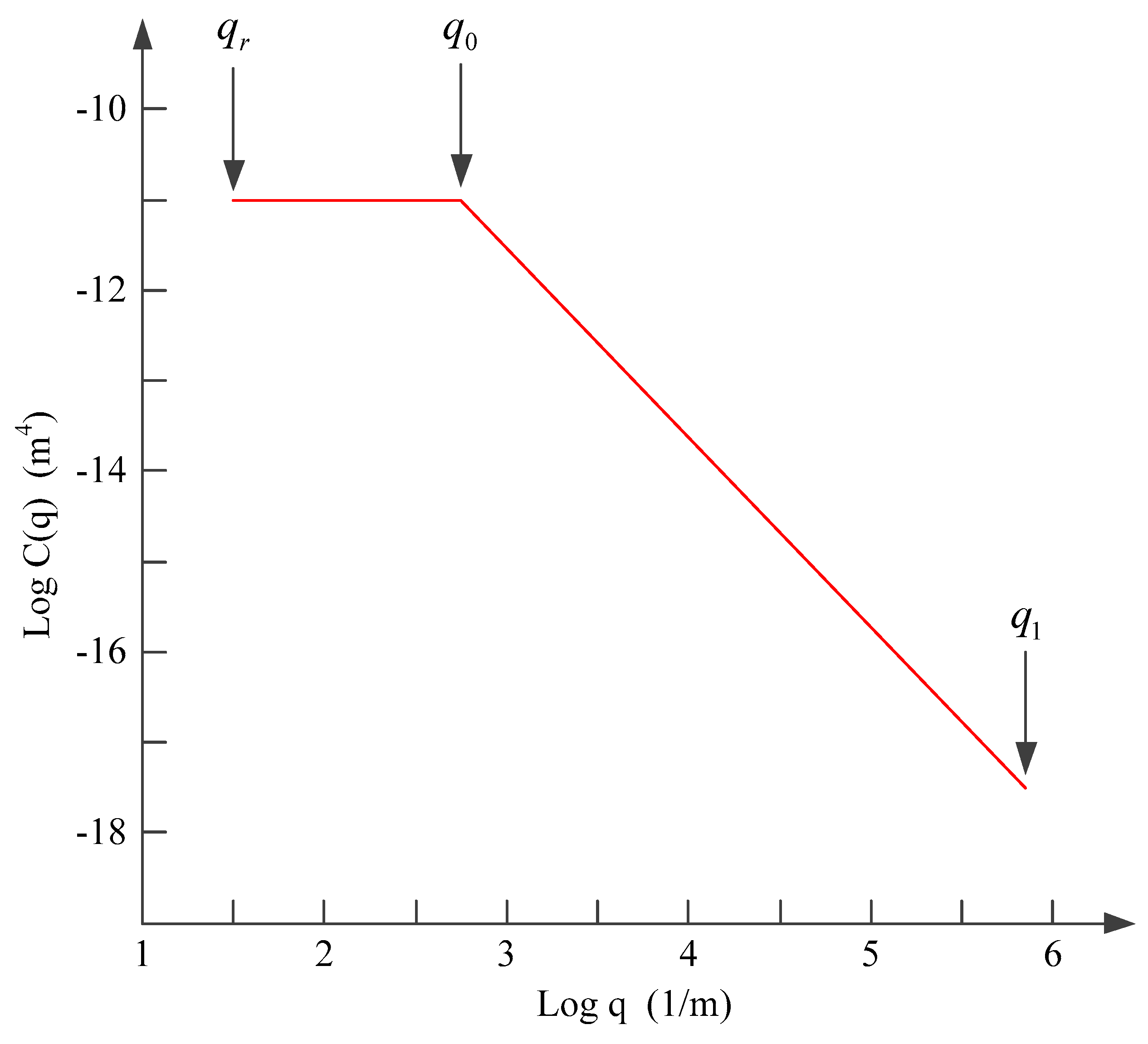
2.1.1. The Surface Roughness Power Spectrum
2.1.2. The Normal Stiffness Model of Interface
2.2. Virtual Material Method Based on M–B Fractal Contact Theory
3. Modal Simulation, Modal Experiment and Results
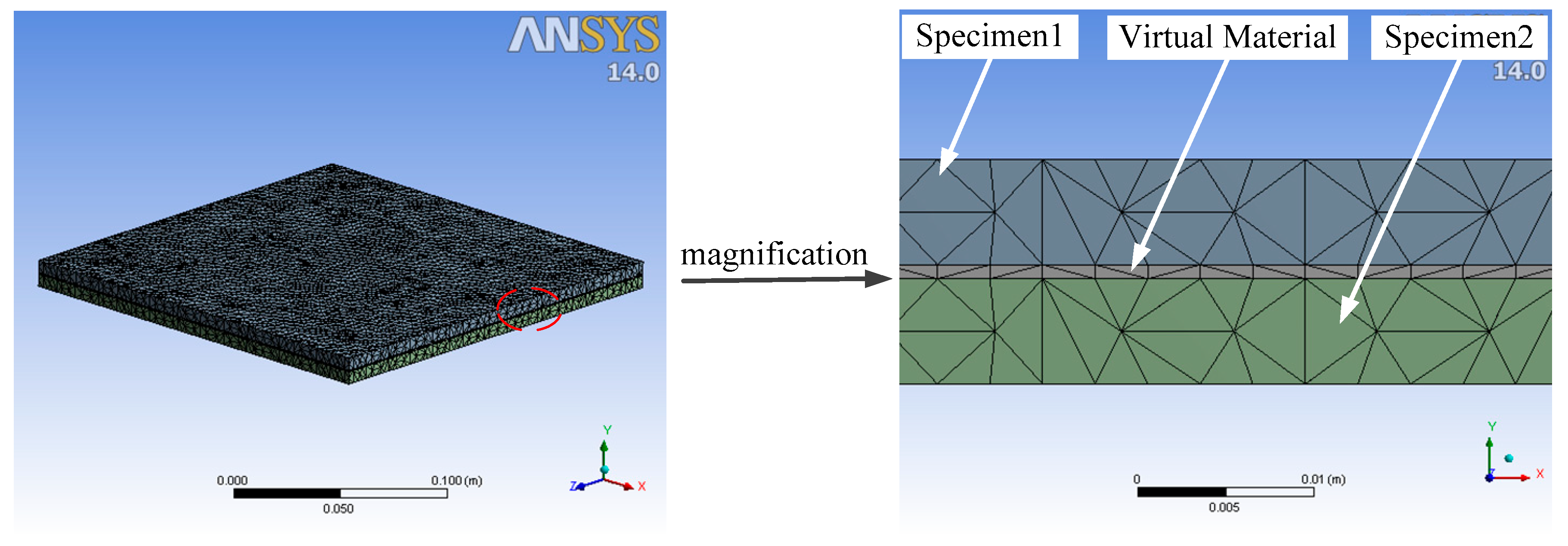
3.1. Modal Simulation
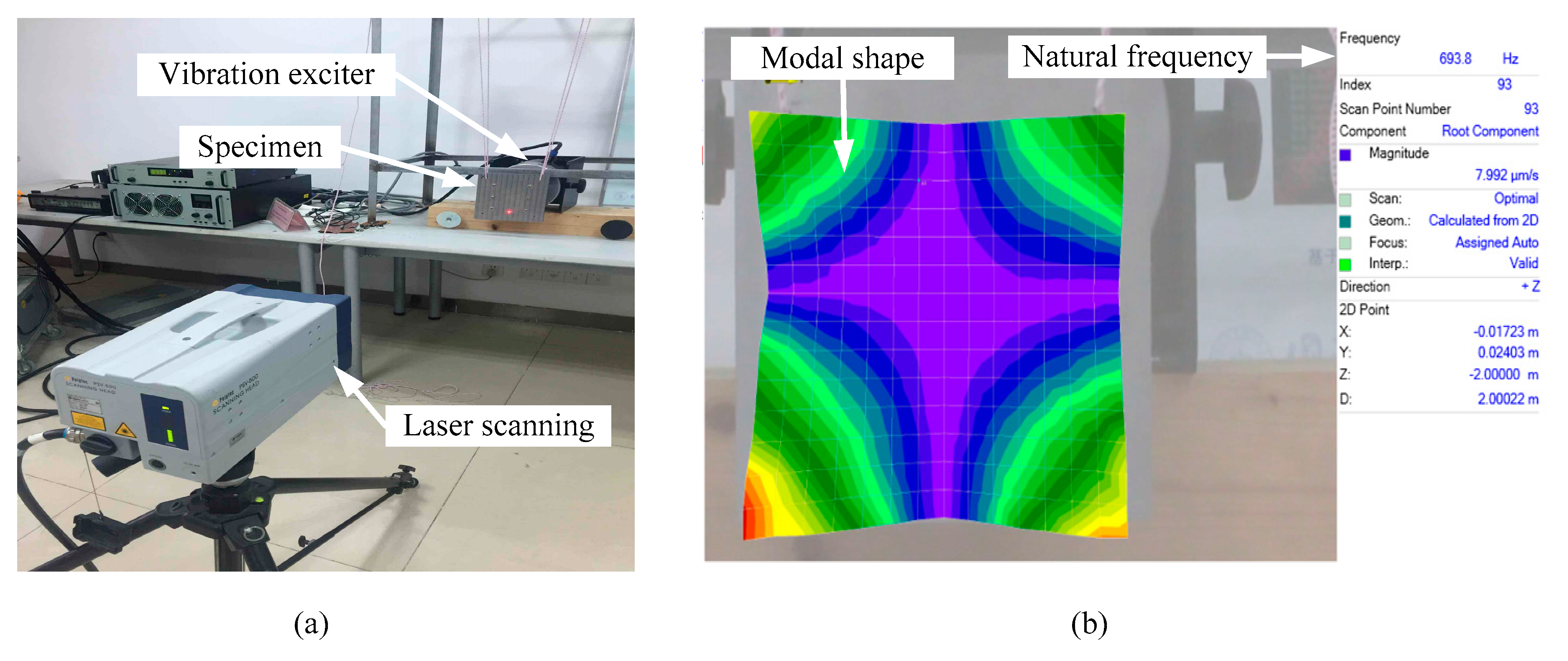
3.2. Modal Experiment
3.3. Results
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Ibrahim, R.A.; Pettit, C.L. Uncertainties and dynamic problems of bolted joints and other fasteners. J. Sound Vib. 2005, 279, 857–936. [Google Scholar] [CrossRef]
- Tian, H.; Li, B.; Liu, H.; Mao, K.; Peng, F.; Huang, X. A new method of virtual material hypothesis-based dynamic modeling on fixed joint interface in machine tools. Int. J. Mach. Tool Manuf. 2011, 51, 239–249. [Google Scholar] [CrossRef]
- Huang, K.; Jin, J. Research on bolt preload simulation based on virtual material method. Mach. Des. Manuf. 2012, 8, 148–150. [Google Scholar]
- Zhao, Y.; Yang, C.; Cai, L.; Shi, W.; Liu, Z. Surface contact stress-based nonlinear virtual material method for dynamic analysis of bolted joint of machine tool. Precis. Eng. 2016, 43, 230–240. [Google Scholar] [CrossRef]
- Han, R.; Li, G.; Gong, J.; Zhang, M.; Zhang, K. Experimental verification and comparative analysis of equivalent methods on metal’s fixed joint interface. Materials 2019, 12, 2381. [Google Scholar] [CrossRef] [PubMed]
- Jana, T.; Anirban, M.; Prasanta, S. Dynamic contact interactions of fractal surfaces. Appl. Surf. Sci. 2017, 392, 872–882. [Google Scholar] [CrossRef]
- Majumdar, A.; Tien, C.L. Fractal characterization and simulation of rough surfaces. Wear 1990, 136, 313–327. [Google Scholar] [CrossRef]
- Gagnepin, J.J.; Roques-Carmes, C. Fractal approach to two-dimensional and three-dimensional surface roughness. Wear 1990, 109, 114–119. [Google Scholar] [CrossRef]
- Lopez, J.; Hansali, G.; Zahouani, H. 3D fractal-based characterization for engineered surface topography. Int. J. Mach. Tools Manuf. 1995, 35, 211–217. [Google Scholar] [CrossRef]
- Marotta, E.E.; Fletcher, L.S.; Dietz, T.A. Thermal contact resistance modeling of non-flat, roughened surfaces with non-metallic coatings. J. Heat Transf. 2001, 123, 11–23. [Google Scholar] [CrossRef]
- Panagouli, O.K.; Mastrodimou, K. Dependence of friction coefficient on the resolution of asperities in metallic rough surfaces under cyclic loading. Int. J. Solids Struct. 2017, 108, 85–97. [Google Scholar] [CrossRef]
- Panagouli, O.K.; Iordanidou, K. Dependence of friction coefficient on the resolution and fractal dimension of metallic fracture surfaces. Int. J. Solids Struct. 2013, 50, 3106–3118. [Google Scholar] [CrossRef]
- Panagouli, O.K.; Mistakidis, E. Dependence of contact area on the resolution of fractal interfaces in elastic and inelastic problems. Eng. Comput. 2011, 28, 717–746. [Google Scholar] [CrossRef]
- Panagiotopoulos, P.D.; Mistakidis, E.S.; Panagouli, O.K. Fractal interfaces with unilateral contact and friction conditions. Comput. Method Appl. Mech. Eng. 1992, 99, 395–412. [Google Scholar] [CrossRef]
- Yuan, Y.; Gan, L.; Liu, K.; Yang, X. Elastoplastic contact mechanics model of rough surface based on fractal theory. Chin. J. Mech. Eng. 2017, 30, 207–215. [Google Scholar] [CrossRef]
- Majumdar, A.; Bhushan, B. Role of fractal geometry in roughness characterization and contact mechanics of surfaces. J. Tribol. 1990, 112, 205–216. [Google Scholar] [CrossRef]
- Persson, B.N.J. On the fractal dimension of rough surfaces. Tribol. Lett. 2014, 54, 99–106. [Google Scholar] [CrossRef]
- Persson, B.N.J. Theory of rubber friction and contact mechanics. J. Chem. Phys. 2001, 115, 3840–3861. [Google Scholar] [CrossRef]
- Yang, C.; Persson, B.N.J. Molecular dynamics study of contact mechanics: Contact area and interfacial separation from small to full contact. Phys. Rev. Lett. 2008, 100, 024303. [Google Scholar] [CrossRef]
- Yang, C.; Persson, B.N.J. Contact mechanics: Contact area and interfacial separation from small contact to full contact. J. Phys. 2008, 20, 215214. [Google Scholar] [CrossRef]
- Persson, B.N.J. On the elastic energy and stress correlation in the contact between elastic solids with randomly rough surfaces. J. Phys. 2008, 20, 312001. [Google Scholar] [CrossRef]
- Almqvist, A.; Campana, C.; Prodanov, N.; Persson, B.N.J. Interfacial separation between elastic solids with randomly rough surfaces: Comparison between theory and numerical techniques. J. Mech. Phys. Solids 2011, 59, 2355–2369. [Google Scholar] [CrossRef]
- Persson, B.N.J. Contact mechanics for randomly rough surfaces. Surf. Sci. Rep. 2006, 61, 201–227. [Google Scholar] [CrossRef]
- Persson, B.N.J.; Bucher, F.; Chiaia, B. Elastic contact between randomly rough surfaces: Comparison of theory with numerical results. Phys. Rev. 2002, 65, 184106. [Google Scholar] [CrossRef]
- Persson, B.N.J. Relation between interfacial separation and load: A general theory of contact mechanics. Phys. Rev. Lett. 2007, 99, 125502. [Google Scholar] [CrossRef] [PubMed]
- Pastewka, L.; Prodanov, N.; Lorenz, B.; Müser, M.H.; Robbins, M.O.; Persson, B.N.J. Finite-size scaling in the interfacial stiffness of rough elastic contacts. Phys. Rev. 2013, 87, 062809. [Google Scholar] [CrossRef] [PubMed]
- Persson, B.N.J.; Albohr, O.; Tartaglino, U.; Volokitin, A.I.; Tosatti, E. On the nature of surface roughness with application to contact mechanics, sealing, rubber friction and adhesion. J. Phys.-Condens. Matter 2005, 17, R1–R62. [Google Scholar] [CrossRef] [PubMed]
- Pan, W.; Li, X.; Wang, L.; Guo, N.; Mu, J. A normal contact stiffness fractal prediction model of dry-friction rough surface and experimental verification. Eur. J. Mech. Solid 2017, 66, 94–102. [Google Scholar] [CrossRef]
- Mayer, M.H.; Gaul, L. Segment-to-segment contact elements for modelling joint interfaces in finite element analysis. Mech. Syst. Signal Proc. 2007, 21, 724–734. [Google Scholar] [CrossRef]
- Ye, H.; Huang, Y.; Li, P.; Li, Y.; Bai, L. Virtual material parameter acquisition based on the basic characteristics of the bolt joint interfaces. Tribol. Int. 2016, 95, 109–117. [Google Scholar] [CrossRef]
- Wang, S.; Komvopoulos, K. A fractal theory of the interfacial temperature distribution in the slow sliding regime: Part I—Elastic contact and heat transfer analysis. J. Tribol. 1994, 116, 812–823. [Google Scholar] [CrossRef]
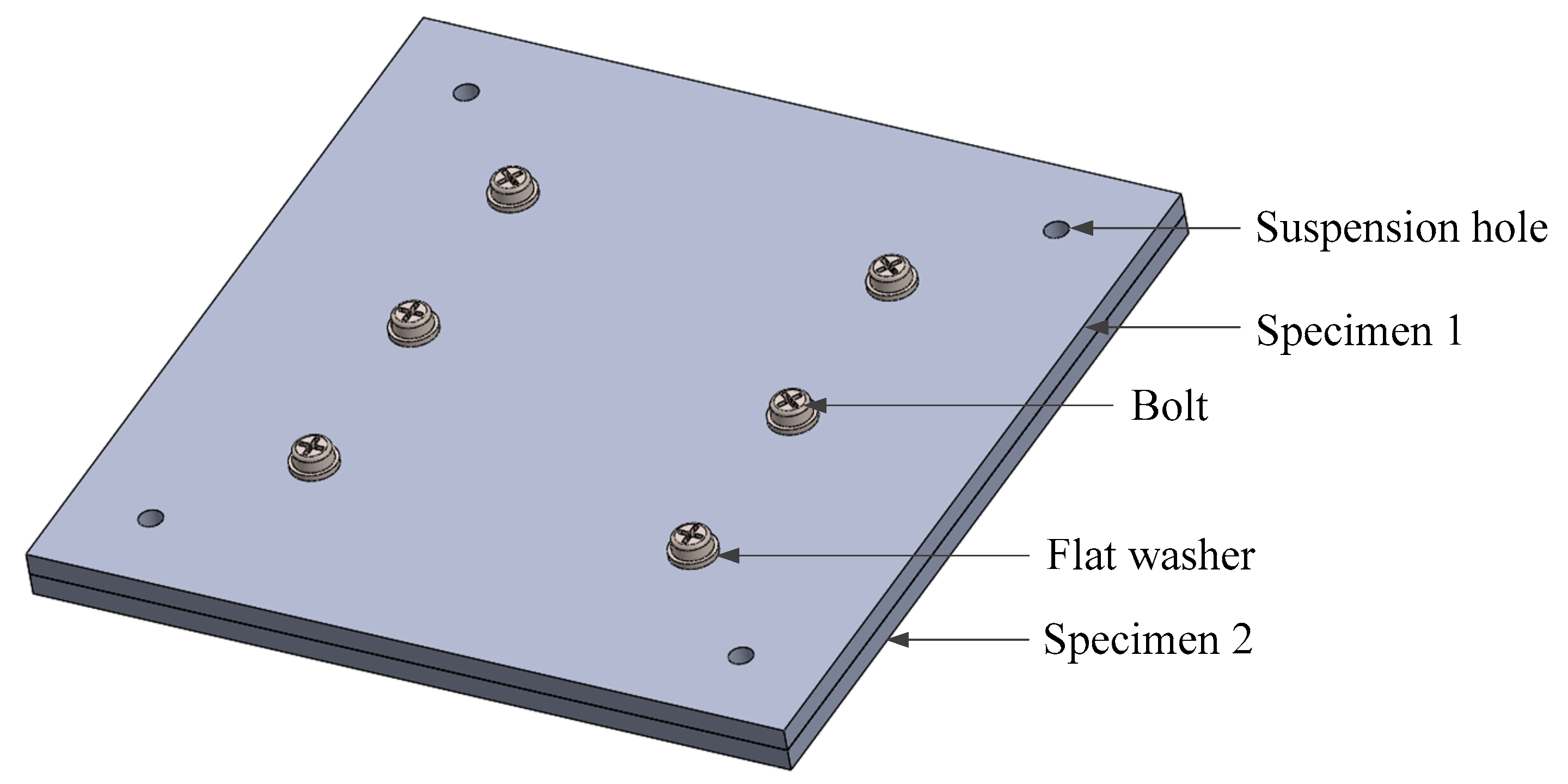


| Parameter | Specimens |
|---|---|
| Elastic modulus: | 209 |
| Poisson‘s ratio: | 0.3 |
| Density: | 7850 |
| Roughness: | 3.2 |
| Yield strength: | 355 |
| Hardness: | 190 |
| Frequency | |||||
|---|---|---|---|---|---|
| Persson contact theory | 720.4 | 1141.3 | 1355.0 | 1524.1 | 1635.1 |
| M–B contact theory | 745.5 | 1201.8 | 1403.8 | 1600.1 | 1725.2 |
| Frequency | |||||
|---|---|---|---|---|---|
| Modal experiment | 693.8 | 1093.8 | 1292.2 | 1459.4 | 1567.2 |
| Order | 1 | 2 | 3 | 4 | 5 |
|---|---|---|---|---|---|
| Error of Persson | 3.78% | 4.34% | 4.86% | 4.43% | 4.34% |
| Error of M–B | 7.45% | 9.87% | 8.57% | 9.63% | 10.0% |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Han, R.; Li, G.; Gong, J.; Zhang, M.; Zhang, K. Equivalent Method of Joint Interface Based on Persson Contact Theory: Virtual Material Method. Materials 2019, 12, 3150. https://doi.org/10.3390/ma12193150
Han R, Li G, Gong J, Zhang M, Zhang K. Equivalent Method of Joint Interface Based on Persson Contact Theory: Virtual Material Method. Materials. 2019; 12(19):3150. https://doi.org/10.3390/ma12193150
Chicago/Turabian StyleHan, Renxiu, Guoxi Li, Jingzhong Gong, Meng Zhang, and Kai Zhang. 2019. "Equivalent Method of Joint Interface Based on Persson Contact Theory: Virtual Material Method" Materials 12, no. 19: 3150. https://doi.org/10.3390/ma12193150
APA StyleHan, R., Li, G., Gong, J., Zhang, M., & Zhang, K. (2019). Equivalent Method of Joint Interface Based on Persson Contact Theory: Virtual Material Method. Materials, 12(19), 3150. https://doi.org/10.3390/ma12193150





