1. Introduction
Ceramic matrix composites are lightweight and exhibit excellent heat resistance and oxidation resistance. Hence, they are utilized in various high-temperature members/components. In recent years, attempts have even been made to implement ceramics in structural components that require higher safety, including next-generation aircraft engine components. However, as ceramics are brittle materials and exhibit a probabilistic fracture behavior, they are still not highly reliable.
The probabilistic fracture behavior of ceramics is due to brittle fractures originating from inherent “defects (flaws, scratches)” [
1,
2,
3,
4,
5,
6]. Ceramics contain various defects distributed inside the bulk material and on the surface. These defects are caused by sintering processes or subsequent processing, and their distribution characteristics may be different even for products of the same lot. For this reason, the scatter in fracture strength emerges for each specimen or structural components. In addition, ceramic strength depends on size [
7]. This is known as the “size effect” and is a direct consequence of statistical distributions and the weakest link theory. The size effect of strength is an important and relevant consequence of the stochastic behavior of the strength of brittle materials [
2,
3,
4,
5,
6,
7,
8]. Therefore, it is difficult to follow directly the procedure from “standardized material tests” to “structure design at actual size,” which is used for general metal-like materials.
Accordingly, statistical methods based on the Weibull distribution have been adopted until now for the strength evaluation of ceramics as brittle materials [
2,
3,
4,
5,
6,
7]. The Weibull distribution is generally understood as the following theory: While pulling a plurality of connected chains, entire chains can be destroyed by destroying the weakest link (referred to as the weakest link theory). The most prominent effect of the weakest link theory is the size effect on strength, which results from the simple fact that it is more probable to find a larger and hence weaker pore critically located in a large specimen than in a small specimen under similar loading conditions. Thus, it is possible to grasp the relationship between the volume and strength of a component using the Weibull distribution, in a certain range. However, numerous tests are required to obtain the Weibull distribution, which are expensive and time consuming. Therefore, a numerical method of predicting the scatter in strength, which reflects the distribution of the defects generated in materials during manufacturing processes, is required.
Several methods that consider the stochastic distribution of flaws and use Monte Carlo simulations, etc., have been reported to predict the scatter in the strength of ceramics [
4,
5,
8,
9,
10]. Furthermore, a reliability evaluation method that combines the detailed structural analysis result obtained using the finite element method (FEM) and the Weibull statistical results of a standardized specimen has been proposed to examine the case where an arbitrary load acts on a component with an arbitrary shape [
11,
12,
13,
14].
Meanwhile, the authors proposed a finite element analysis (FEA) methodology that predicts the scatter in ceramic strength based on microstructure data (relative density, defect distribution, grain size distribution, etc.) [
15]. Specifically, the information of the microstructure distribution obtained through image observation is represented by various probability density functions. This allows for strength analysis based on the FEM by indirectly reflecting the parameters of a continuum damage model via a fracture mechanics model. In addition, it is possible to reproduce the stochastic distribution of a microstructure in a number of analysis targets (for example, tens to hundreds of specimens) by assuming the same lot using random numbers. As a result, we succeeded in directly creating a Weibull distribution from the results of FEA, in which different fracture strengths were predicted for each analysis target.
In this study, we show that not only the scatter in the strength of same-size specimens, but also the size effect can be considered by the previously proposed FEA methodology [
15]. Ceramics have different material strengths depending on the test specimen size and test method by size dependency. Therefore, it is important to be able to evaluate the size dependence of ceramics by the proposed FEA methodology [
15]. Specifically, we prepared four kinds of finite element (FE) models and analyzed them under three kinds of loading conditions, that is three-point bending, four-point bending, and simple tension. We analyzed
N = 30 specimens under each condition. Then, we created Weibull distributions and investigated the relationship between fracture strength and effective volume. Herein, the effective volume of a component corresponds to the volume of a hypothetical tensile specimen loaded with the maximum equivalent stress amplitude of the component and having the same failure probability.
The ceramic material we examined was Al
2O
3/30 vol% SiC, which is the same as that used in the previous study [
15]. The processing defects on its surfaces are repaired in advance through heat treatment because the Al
2O
3/30 vol% SiC particle composite has a self-healing property [
16,
17,
18,
19]. Thus, it is possible to consider only the fractures that start from internal flaws [
16,
17].
3. FE Model and Boundary Condition
Figure 3 shows the FE analysis models used in this study.
Figure 3a,b shows the three-point bending tests with different sizes of specimens, and
Figure 3c shows the four-point bending test, while
Figure 3d shows the tensile test. The thickness and width of the specimens were 3 and 4 mm, respectively. These were the same for all models. In addition, four values (10, 22, 40, and 60 mm) of the lengths of specimens were considered for the tensile test (
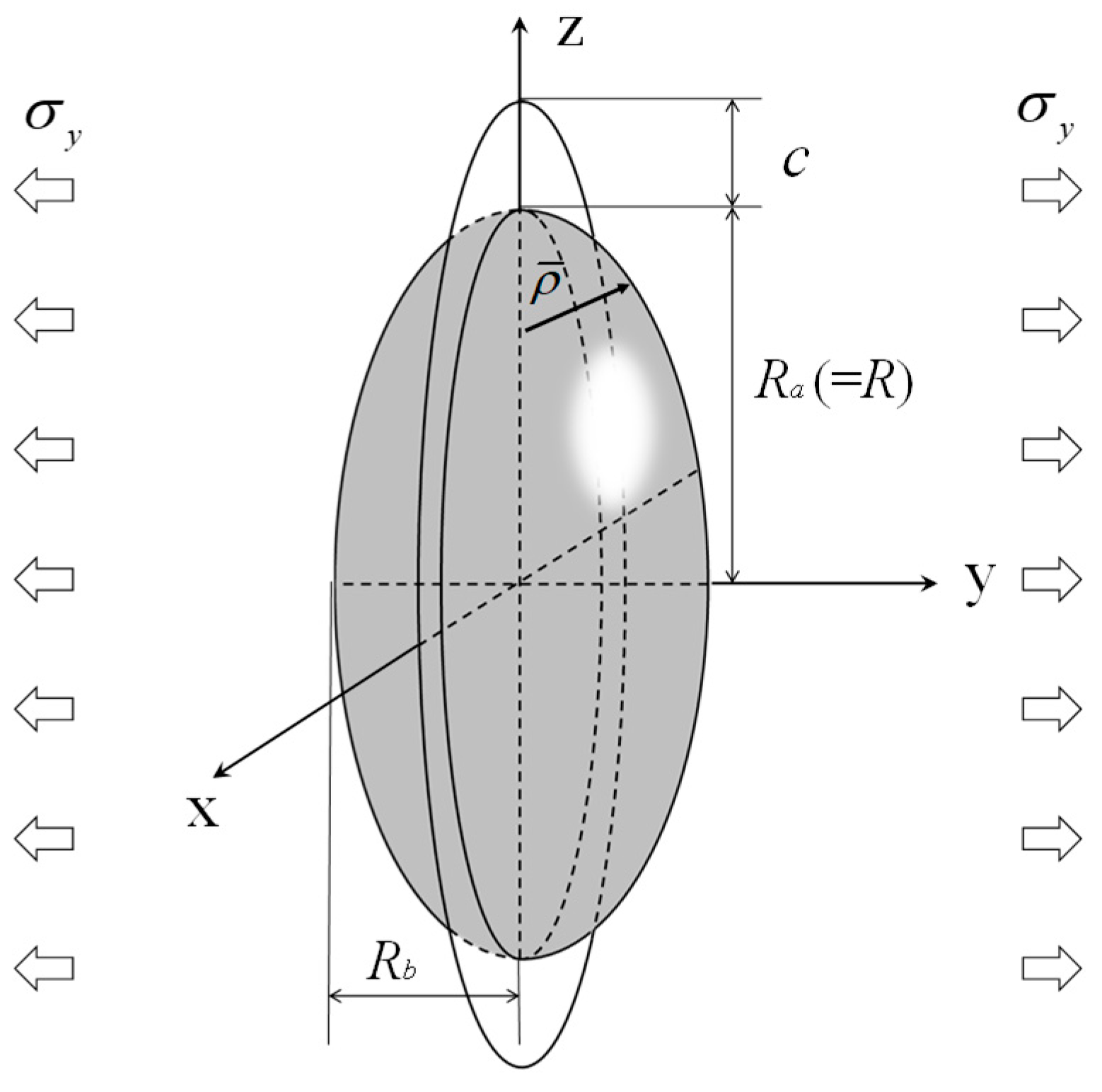
Figure 3d) to simply change the effective volume. The jigs of the three-point bending test and four-point bending test were set as rigid bodies. In the calculations of the bending tests, a constant forced velocity (constant crosshead speed of 5 mm/s) in the vertical direction was imposed on the upper jig, while the displacements of the lower jigs were fixed. A friction coefficient of 0.3 was set at the contact boundary between the specimen and jigs. In the calculations of the tensile test, tensile load was applied by completely fixing one end of the specimen and applying forced displacement (constant velocity of 3 mm/s) to the other end. Note that the major axis orientation of pores corresponds to the y-axis direction in
Figure 3.
In this study, the commercial FEM software package LS−DYNA (r7.1.2, Livermore Software Technology Corporation, California, CA, America) and its material user subroutine, umat XX [
29], were utilized. A dynamic explicit method was adopted to perform a numerical integration in the time domain. All specimens shown in
Figure 3 were discretized by an eight-node solid element with dimensions of 0.5 mm × 0.5 mm × 0.5 mm. Moreover, one-point Gaussian integration was adopted.
The material properties and the condition of the microstructure distribution are shown in
Table 1 and
Table 2, respectively. In the present FEA methodology, the statistical dispersion of the material parameters for each element was set based on the material properties given in
Table 1 and the average and standard deviation of the microstructure distribution given in
Table 2.
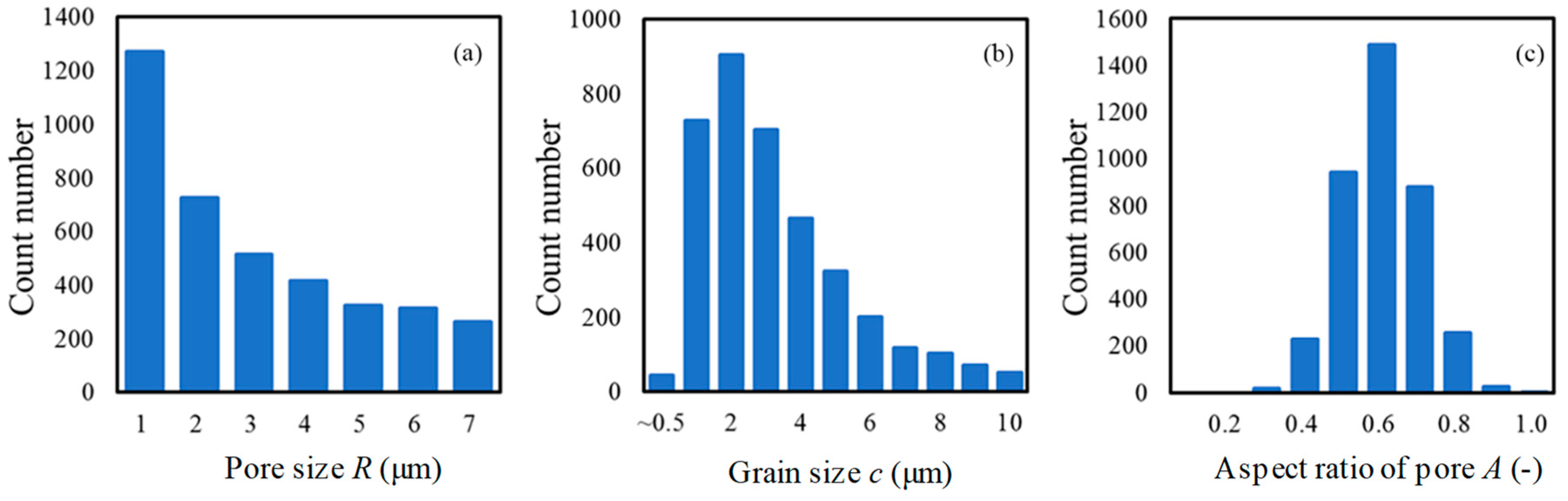
Figure 4 shows an example of the histograms of microstructure data obtained under the conditions listed in
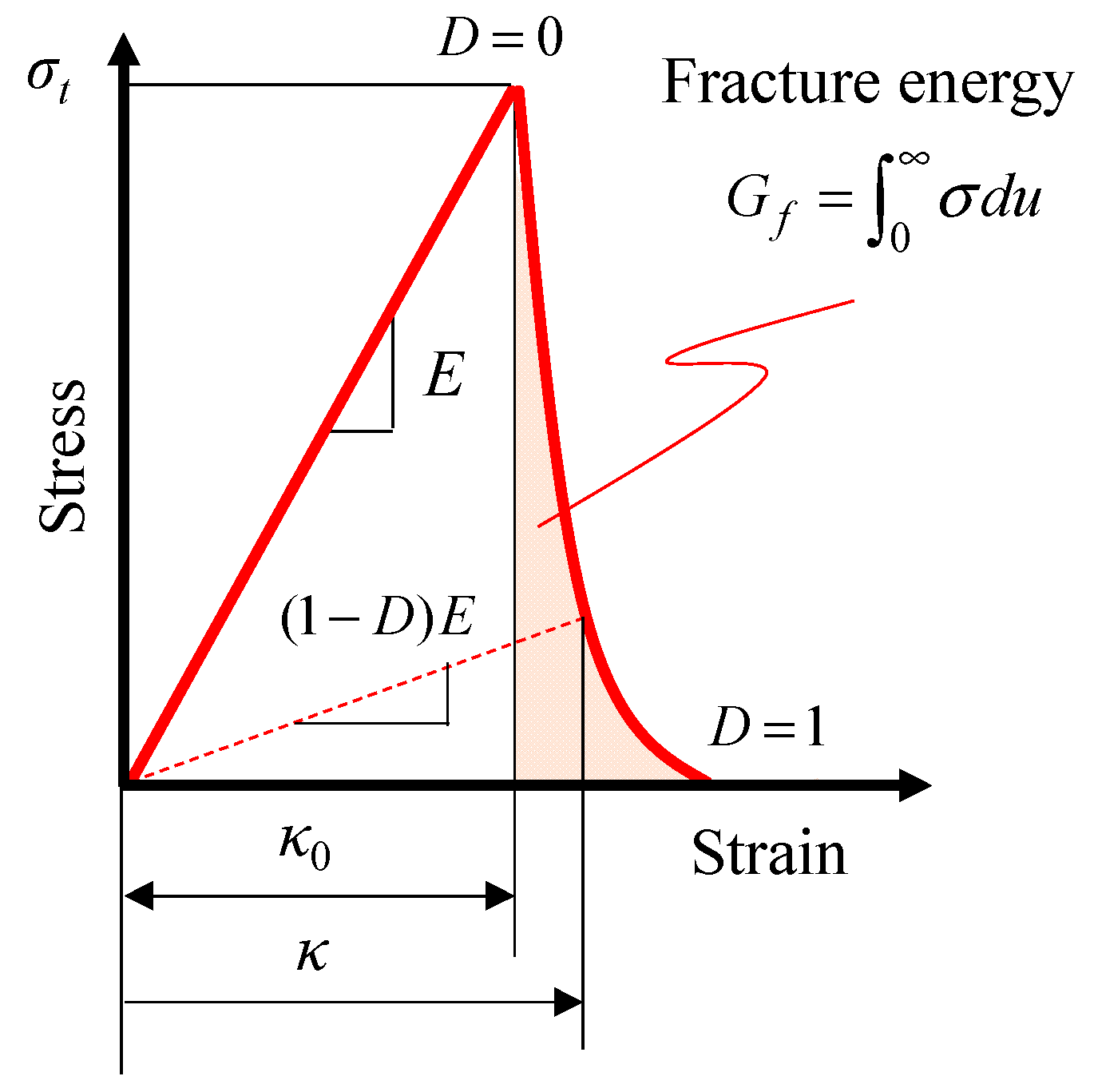
Table 2. Here, the class (abscissa) and frequency (ordinate) of the histograms correspond to the values of each element and the number of elements, respectively. The data in the histograms were obtained for an arbitrary specimen with a length of 40 mm of the four-point bending test model. Based on the statistically-distributed microstructure data and using Equations (4), (8) and (9), the fracture stress
σt, fracture energy
Gf, and strain
κ0 at damage initiation were evaluated for each element (Gaussian point). The above-mentioned parameter setting operation for the damage model was performed only at the Time 0 step.
Figure 4a shows that the existence probability of the likelihood of a pore being present within a specified amount of volume decreases as pore size increases, according to the inverse power law distribution. The minimum value of the histogram corresponds to the maximum pore size that would exist in the volume per element. Note that, even though pores of several sizes were found in one element, we neglected the presence of micro pores and the interference between pores (the overlap of stress fields). Moreover, we only considered the largest pore, which might become a fracture origin in each element.
4. Results and Discussion
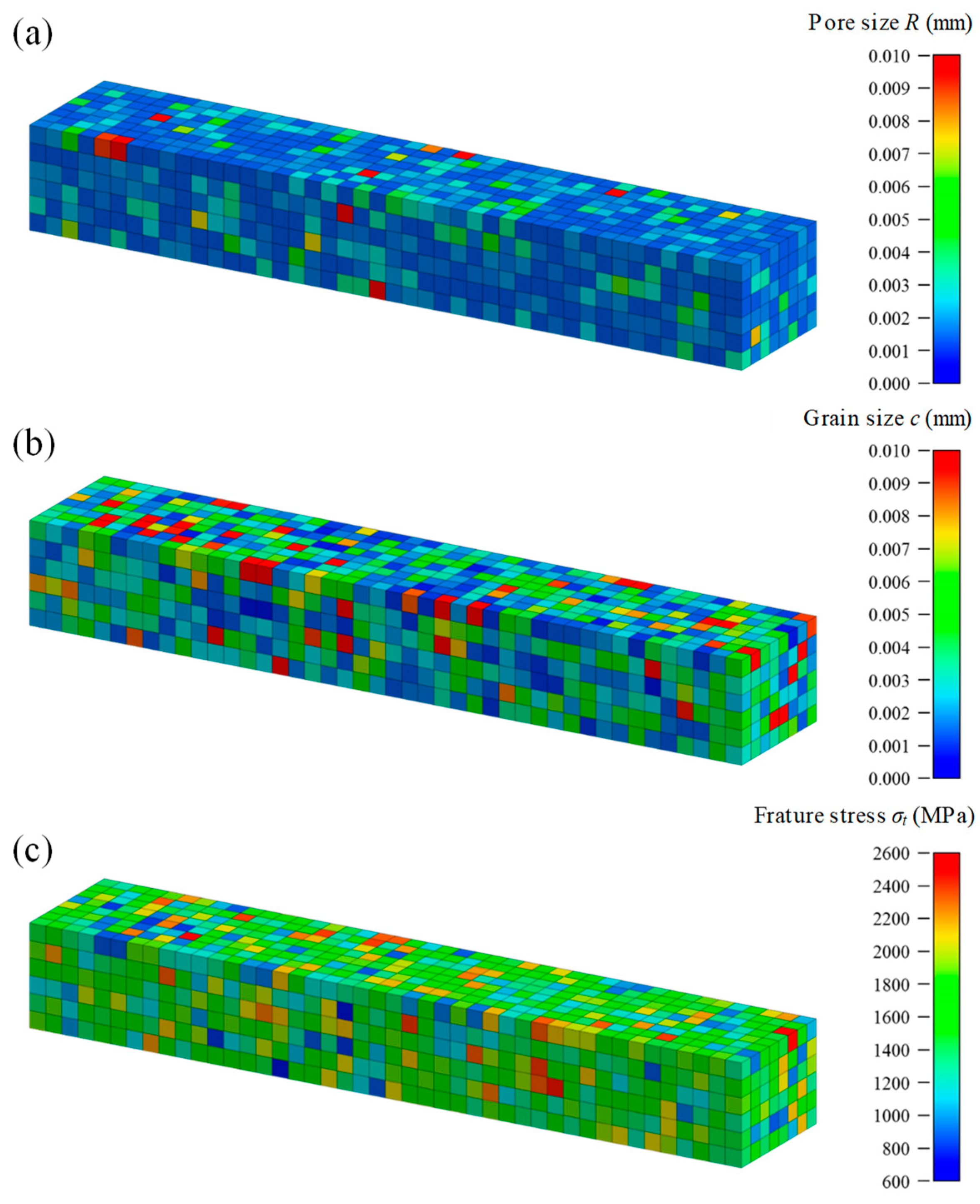
Figure 5a–c shows the contour maps of pore size, grain size, and fracture stress, respectively. It can be confirmed from the figure that fracture stress is distributed stochastically based on the different pore size and grain size of each element. Moreover, we confirmed that each specimen represents a different distribution by random numbers.
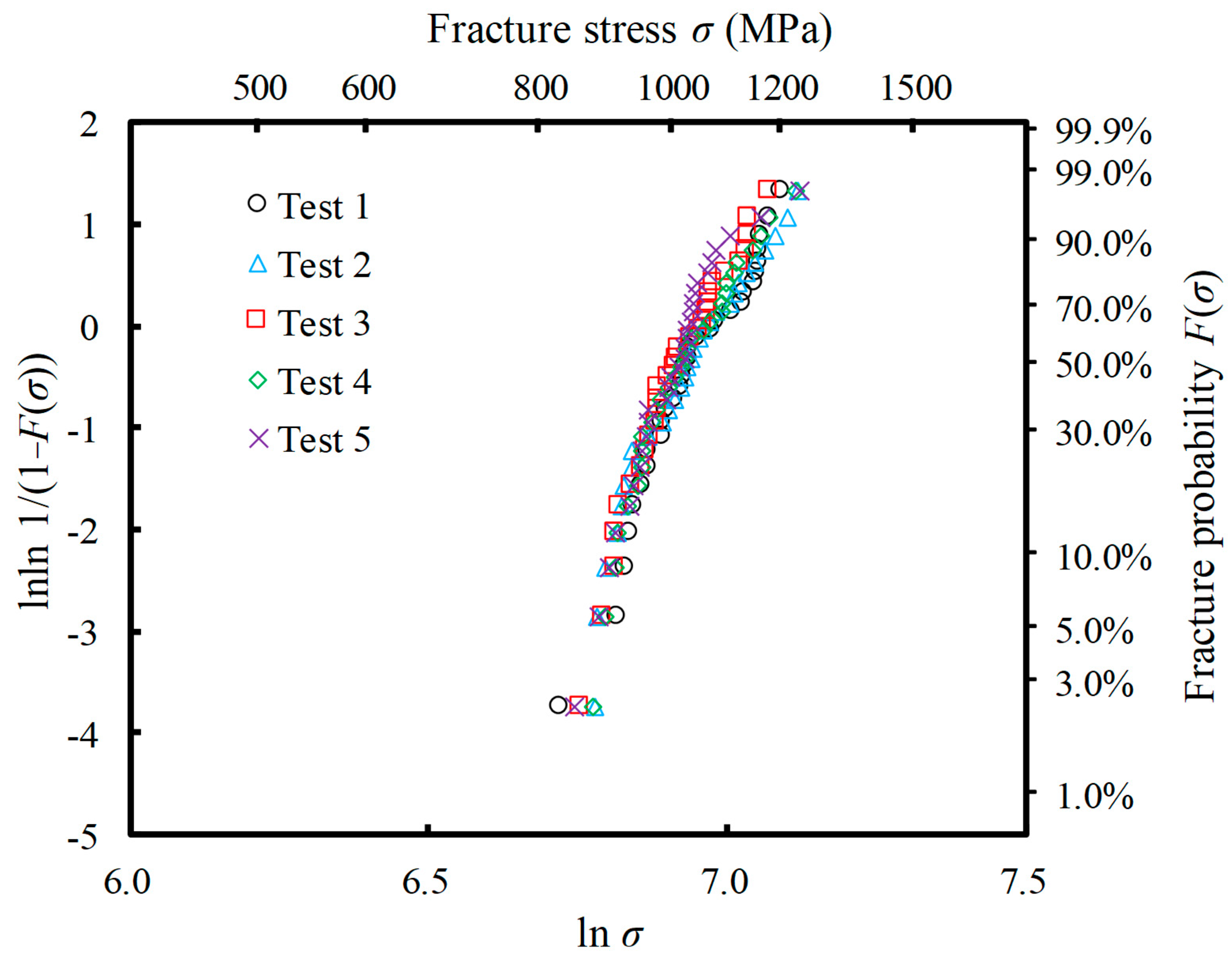
Figure 6 shows the Weibull distribution obtained by the FEA of the four-point bending test. The graph includes the results of five tests. The number of specimens in each dataset was set as
N = 30. Even if the same microstructure distribution condition shown in
Table 2 were used for each specimen, the parameters of the damage model set for each element were random, and thus, different Weibull distributions were obtained. However, note that the Weibull modulus and scale parameter were almost the same in the results of the five tests because we assumed the same lots of the Al
2O
3/30 vol% SiC particulate composite.
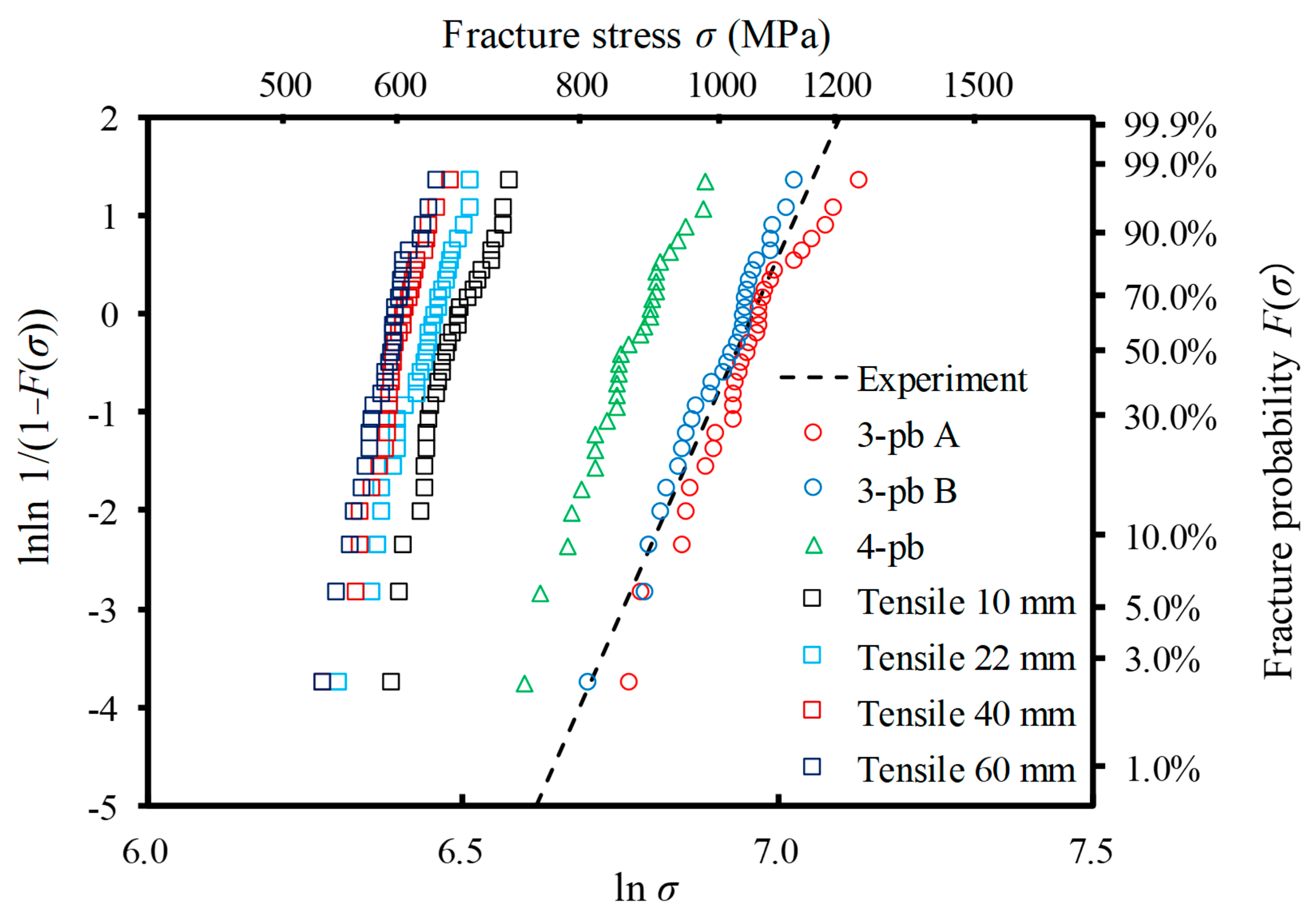
Figure 7 shows the Weibull distribution obtained by each analysis model shown in
Figure 3. Here, the number of specimens in each dataset was set to
N = 30. For the sake of reference, the experimental result [
16] of the three-point bending test with the same specimen size as the three-point bending Test A is shown as represented by the broken line. As the effective volume increased, the scatter of strength decreased, and the Weibull modulus
m increased, in the range of the examined condition. Moreover, it can also be confirmed that the scale parameter
β representing the mean strength decreased with the increase in effective volume. It is thought that these tendencies depend on the distribution characteristics of the microstructure. The present methodology can analyze the fact that the fracture of ceramics is caused by the probability of the existence of large flaws in the part subjected to a tensile stress. Hence, the predicted strength depends on the effective volume.
Table 3 lists the Weibull modulus
m and the scale parameter
β obtained by FEA for each test model.
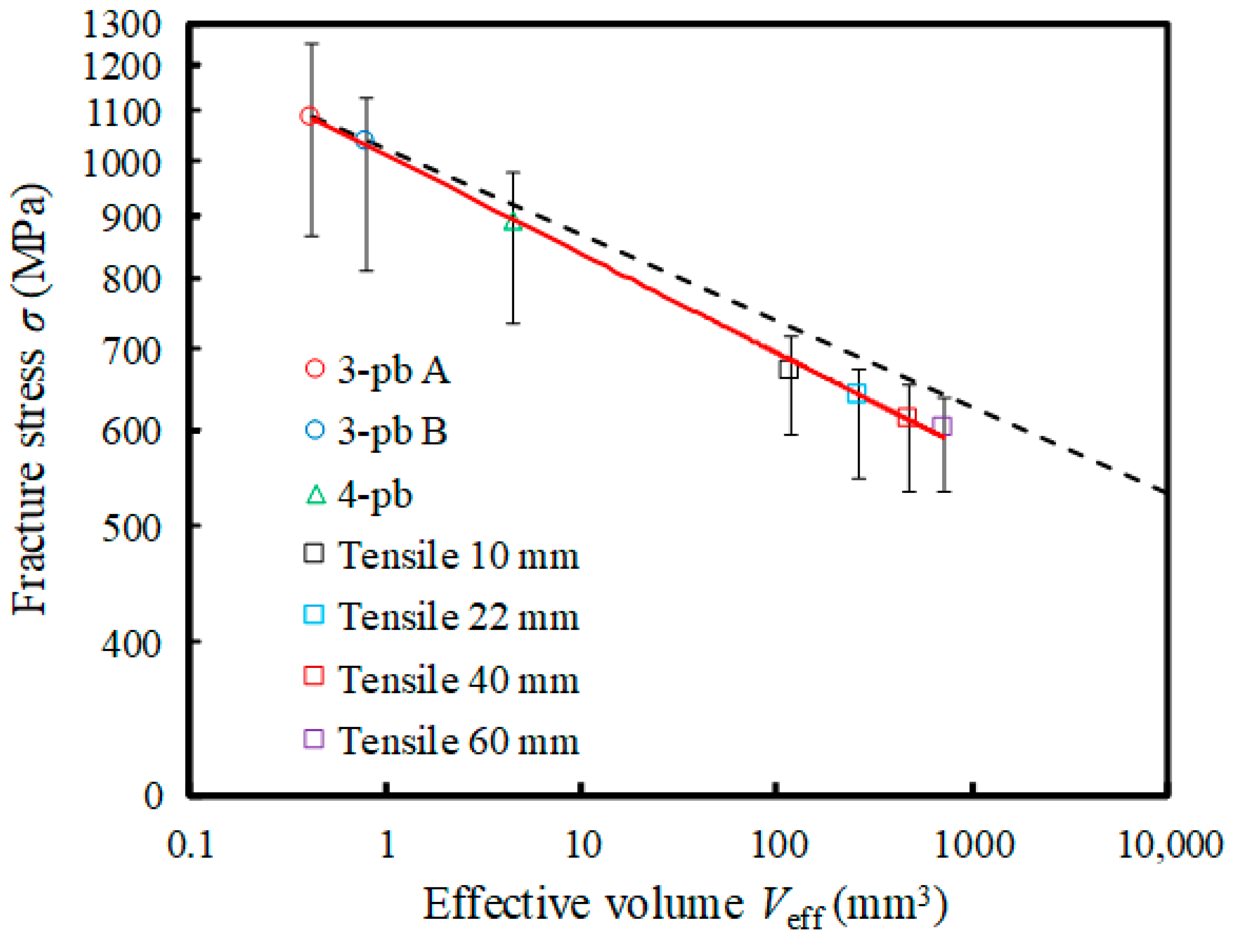
Figure 8 shows the relationship between the mean strength (scale parameter
β) and effective volume
Veff in log-log scale. Here, in the bending tests, the effective volumes of each specimen were evaluated using the Weibull modulus
m3A of three-point bending Test A, as shown in
Table 3. Each error bar on the plots indicates the maximum strength (fracture probability:
P(
σ) = 97.7%) and minimum strength (fracture probability:
P(
σ) = 2.3%). As in previous reports [
4,
5,
6,
7,
8], the scale parameter decreased with increasing effective volume in the logarithmic scales. The solid line in the graph indicates the approximate line obtained by the least squares method. It was confirmed that the results obtained by the present FEA exhibited a trend similar to that predicted by the Weibull theory.
In general, in ceramics, if the effective volume and the scale parameter obtained by tests are
V0 and
σ0, respectively, the fracture strength
σt in different tests having effective volume
Veff is regarded as following the relation:
Herein, the Weibull modulus
m was assumed to be equal in certain size ranges in that relation. The broken line in
Figure 8 shows the estimated mean strength using the Weibull modulus
m3A of three-point bending Test A, which was the smallest specimen. From the comparison of the broken line with the plots, it can be seen that the conventional estimation based on Equation (11) predicted dangerous side strength. In particular, when the effective volume became 100-times or more, the estimated strength became a higher value. In the case of the tensile test, it is rational to think that the existence probability of large pores drastically increases, and the scatter of strength becomes small as the effective volume increases. It is thought that the present FEA methodology can deal with such situations.
Meanwhile, the present FEA methodology can naturally predict both the Weibull modulus and mean strength for specimens having arbitrary sizes because an effective volume does not need to be evaluated in advance. This enables the evaluation of the safety side strength (see the approximate solid line in the graph in
Figure 8). Moreover, the present methodology can predict the lower limit of strength from the fracture probability (e.g.,
P(
σ) = 2.3% in the case of
N = 30), which is the lower limit of the error bar, and enables further reliability evaluation. Therefore, even with brittle materials, it is possible to design components/members in accordance with safety requirements considering the “lower limit of strength.” It is also thought that the present methodology can be used for optimization of the microstructure distribution, sintering processes, and subsequent processing, according to the shapes of components/members.
5. Conclusions
In this study, we investigated the size effect of ceramics by using the FEA methodology [
15], which can predict the scatter of the strength of ceramics based on microstructure information. Four kinds of specimen sizes were prepared, and three kinds of loading tests were carried out. We then analyzed
N = 30 specimens for each condition to create Weibull distributions. The results in the examined condition showed that the Weibull modulus increased and the scatter of strength decreased as the effective volume increased, since the probability of existence of large pores (defects) increased. Furthermore, it was also shown that the scale parameter decreased with increases in the effective volume in log-log scale, and the data obtained by FEA followed the trend of the Weibull theory. It was possible to analyze the size effect on strength, which is an important and relevant consequence of the statistical behavior of brittle ceramics, by using the present FEA methodology.
Moreover, unlike the conventional method, in which the Weibull modulus is assumed to be a constant value, the present methodology can enable us to evaluate the safety side strength because it can naturally analyze the fracture behavior under any given shape and boundary conditions.