Quasi-Periodic and Fractal Polymers: Energy Structure and Carrier Transfer
Abstract
1. Introduction
2. Sequences and Notation
2.1. Fibonacci
2.2. Thue–Morse
2.3. Double-Period
2.4. Rudin–Shapiro
2.5. Cantor Set
2.6. Asymmetric Cantor Set
3. Theory
3.1. Stationary States—Time-Independent Problem
3.2. Time-Dependent Problem
4. Results
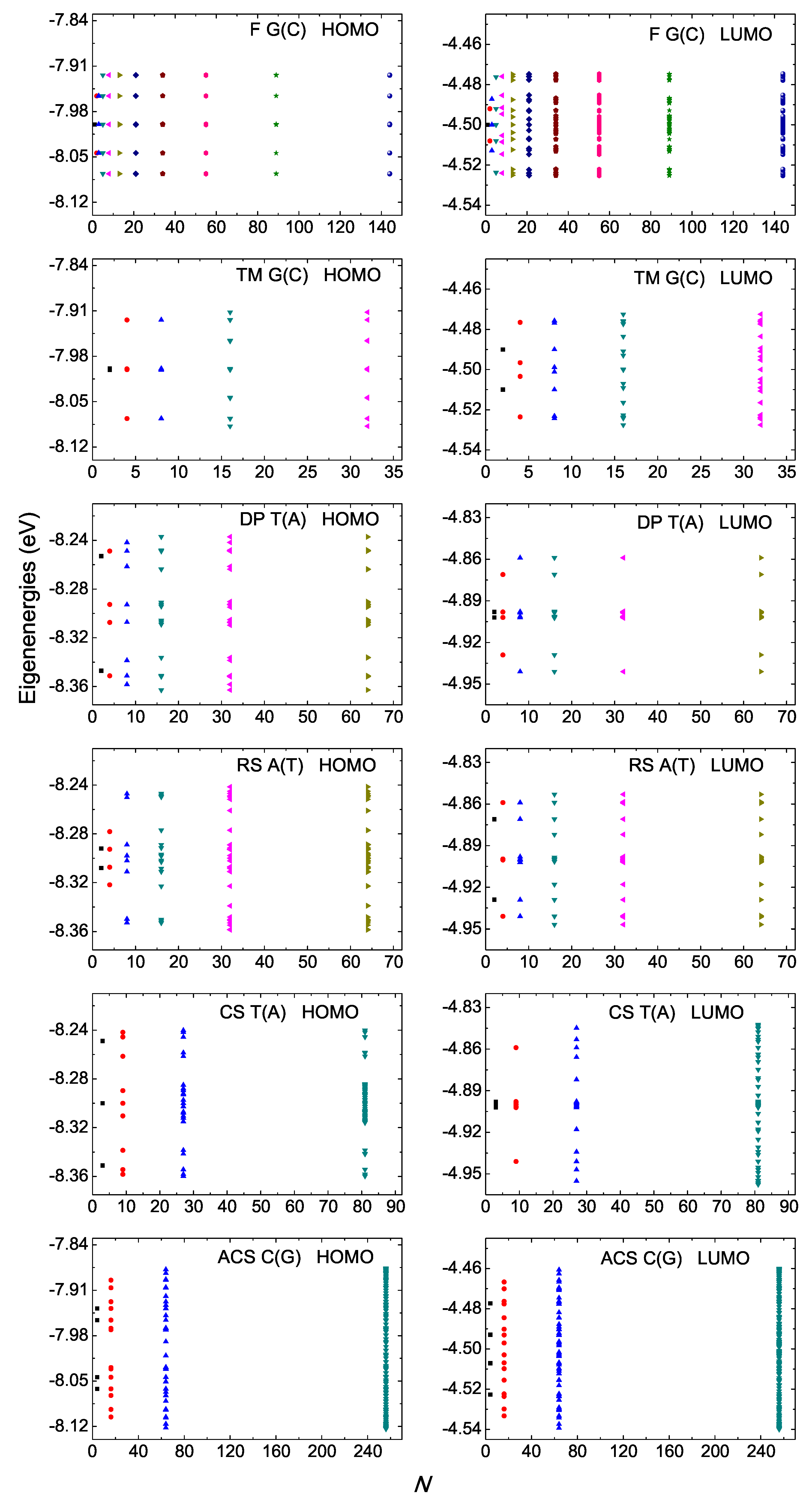
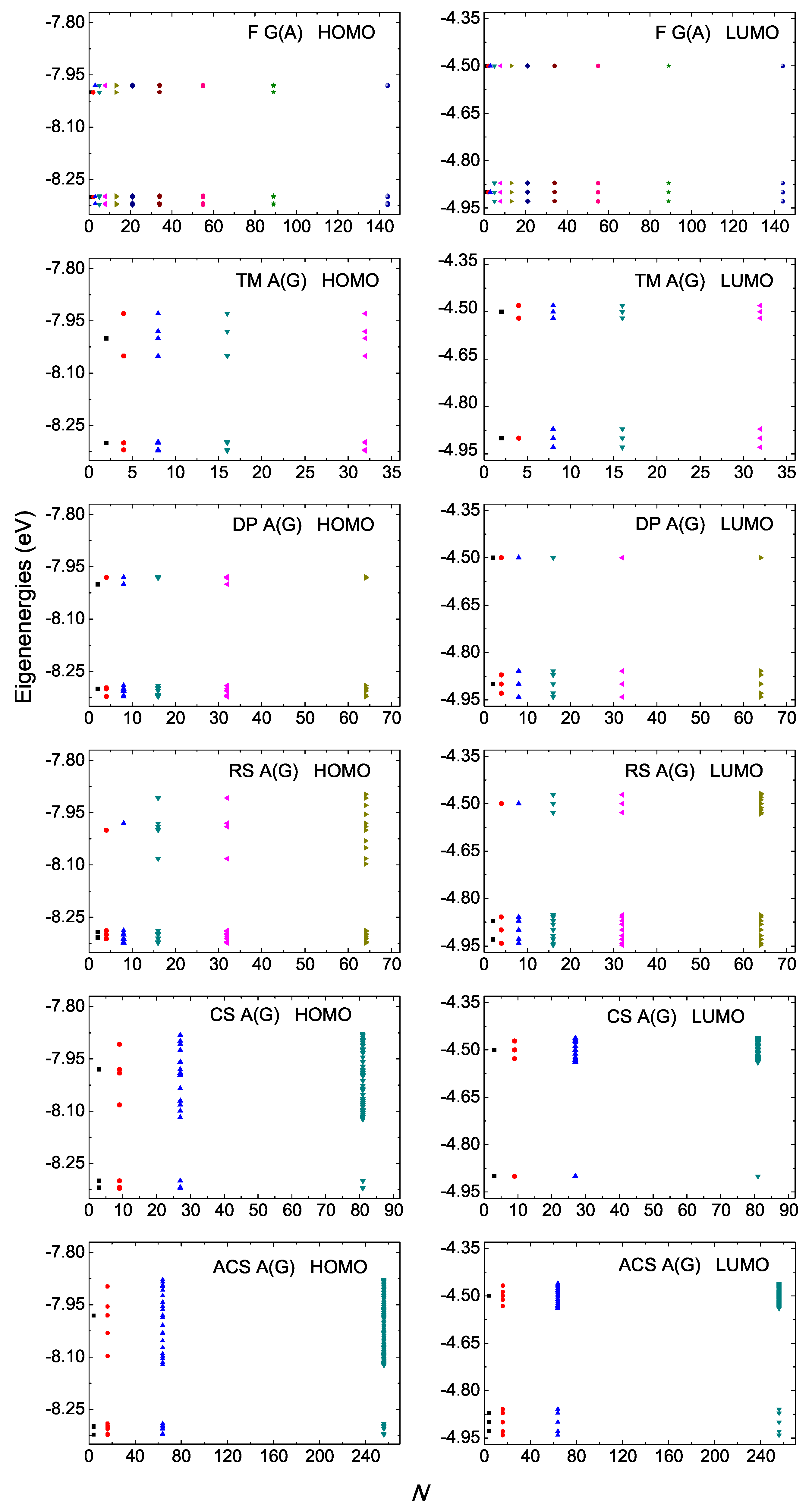
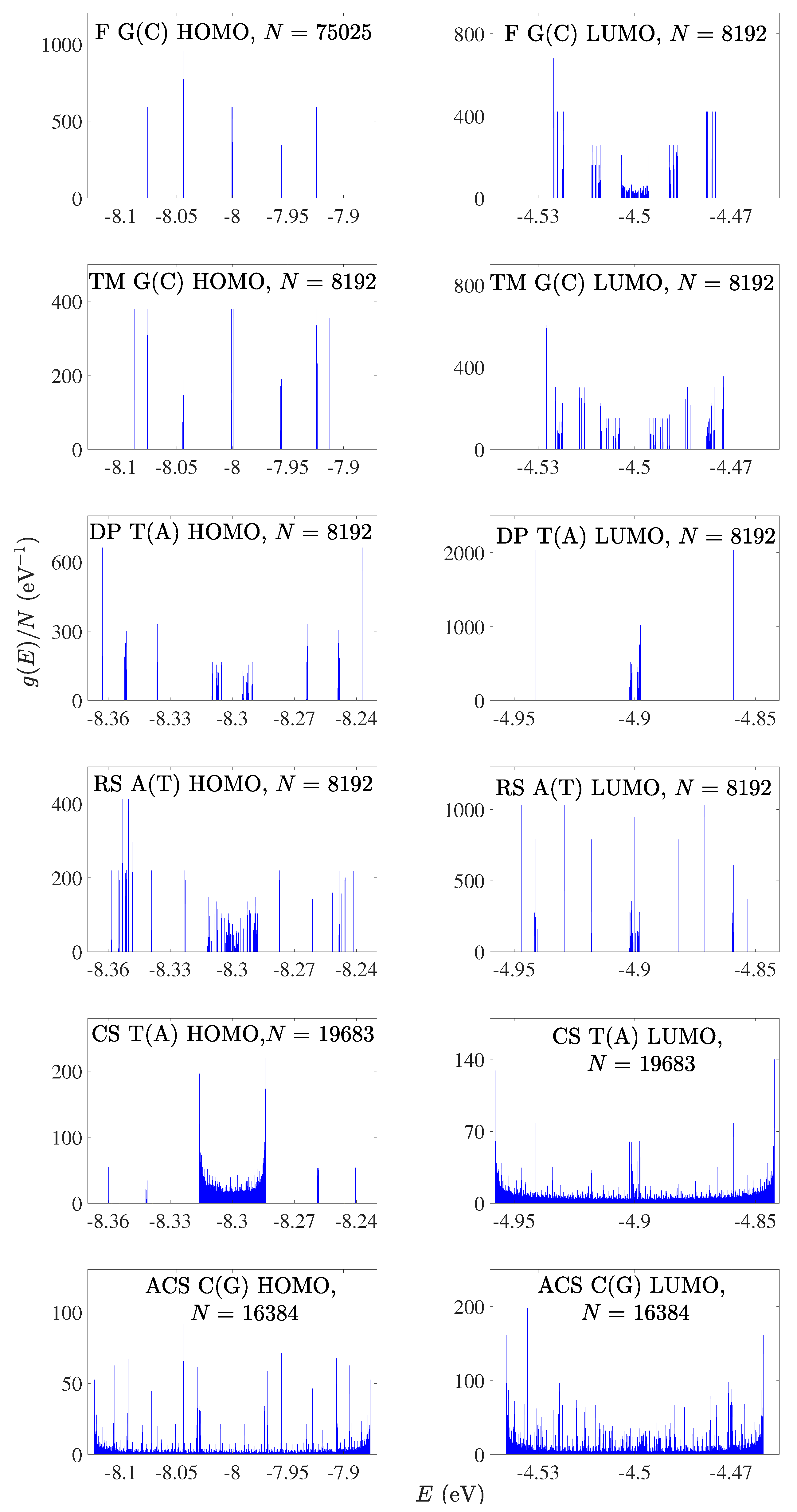
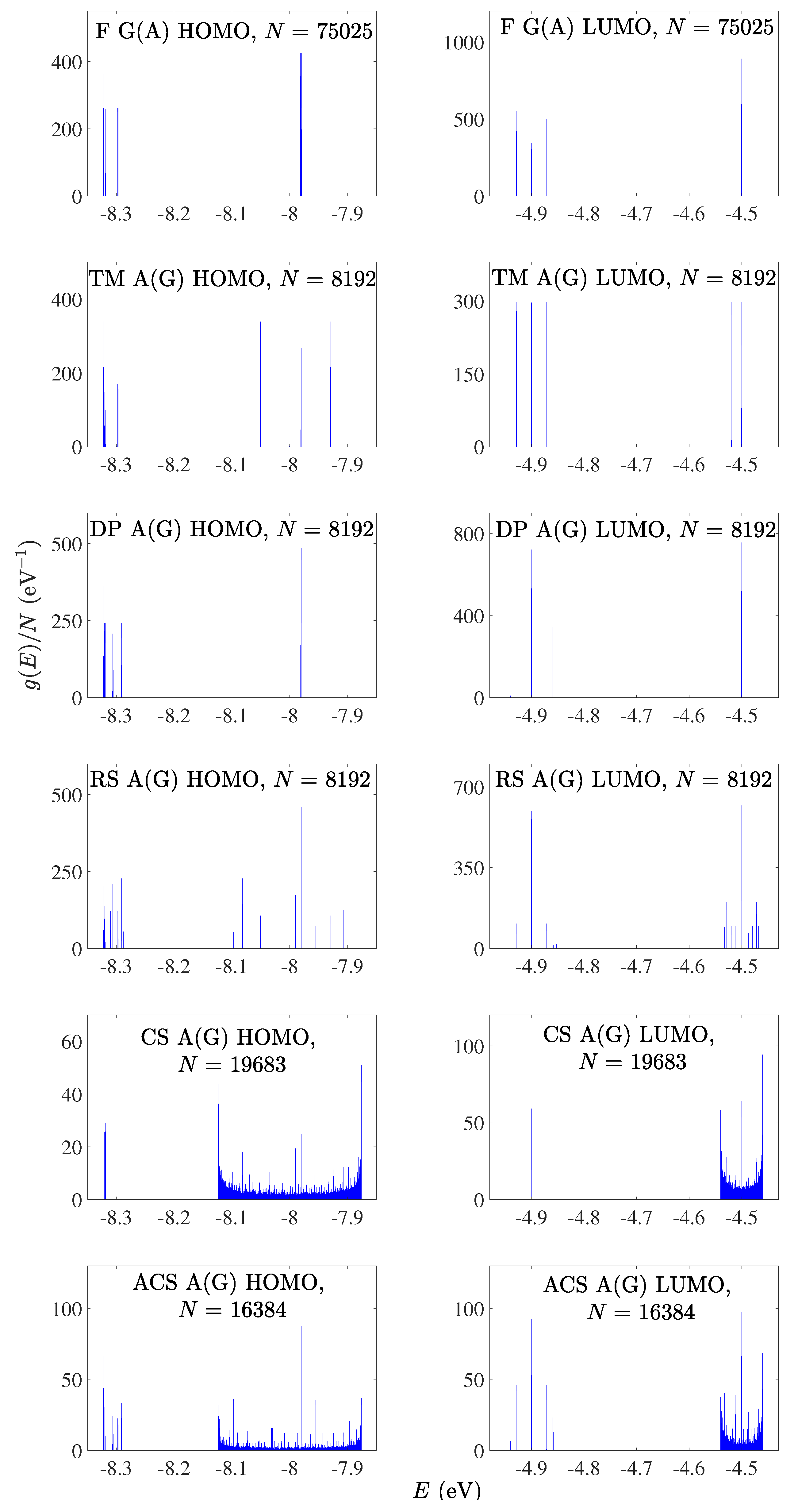
4.1. Eigenspectra, Density of States, Energy Gaps
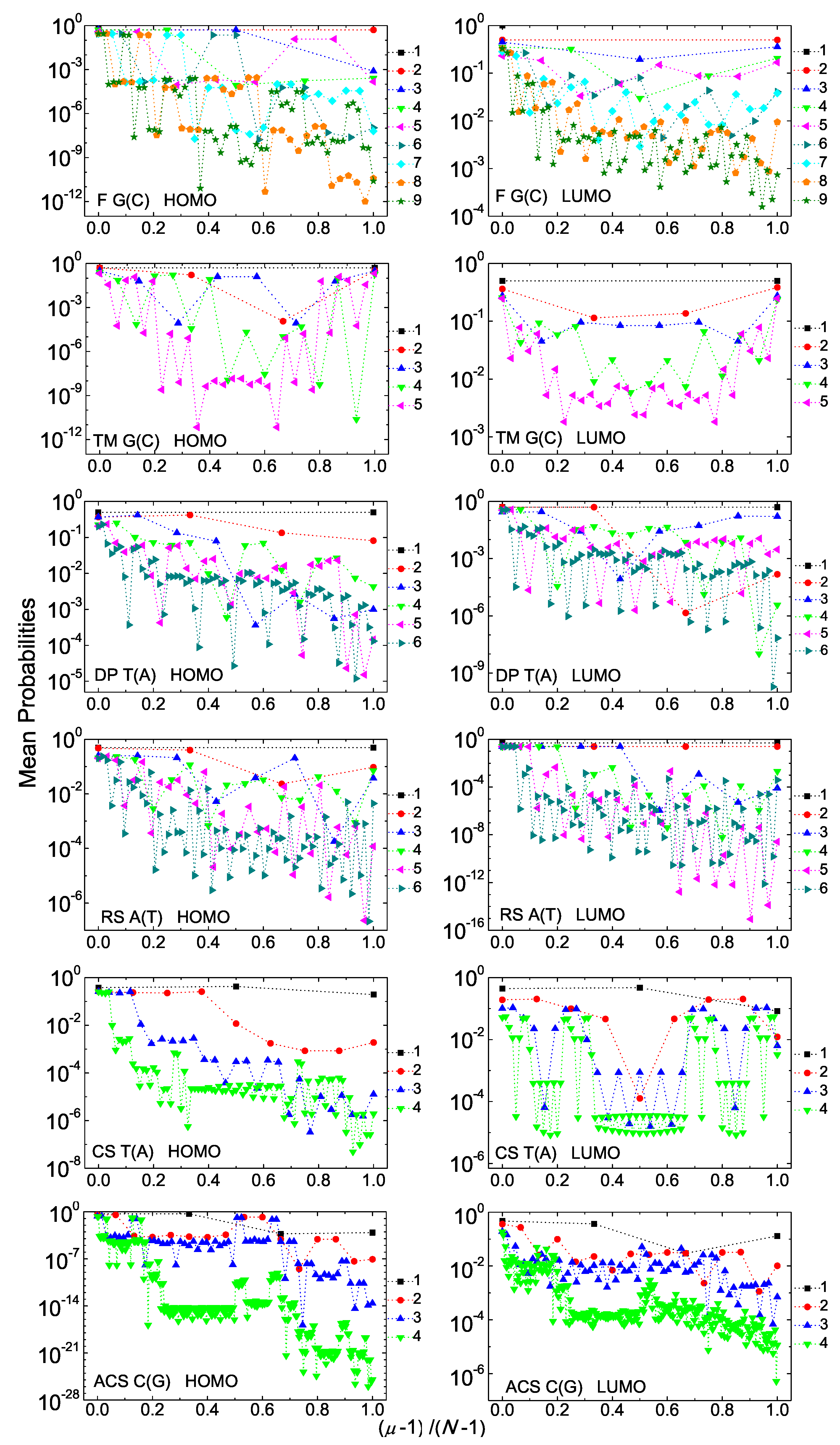
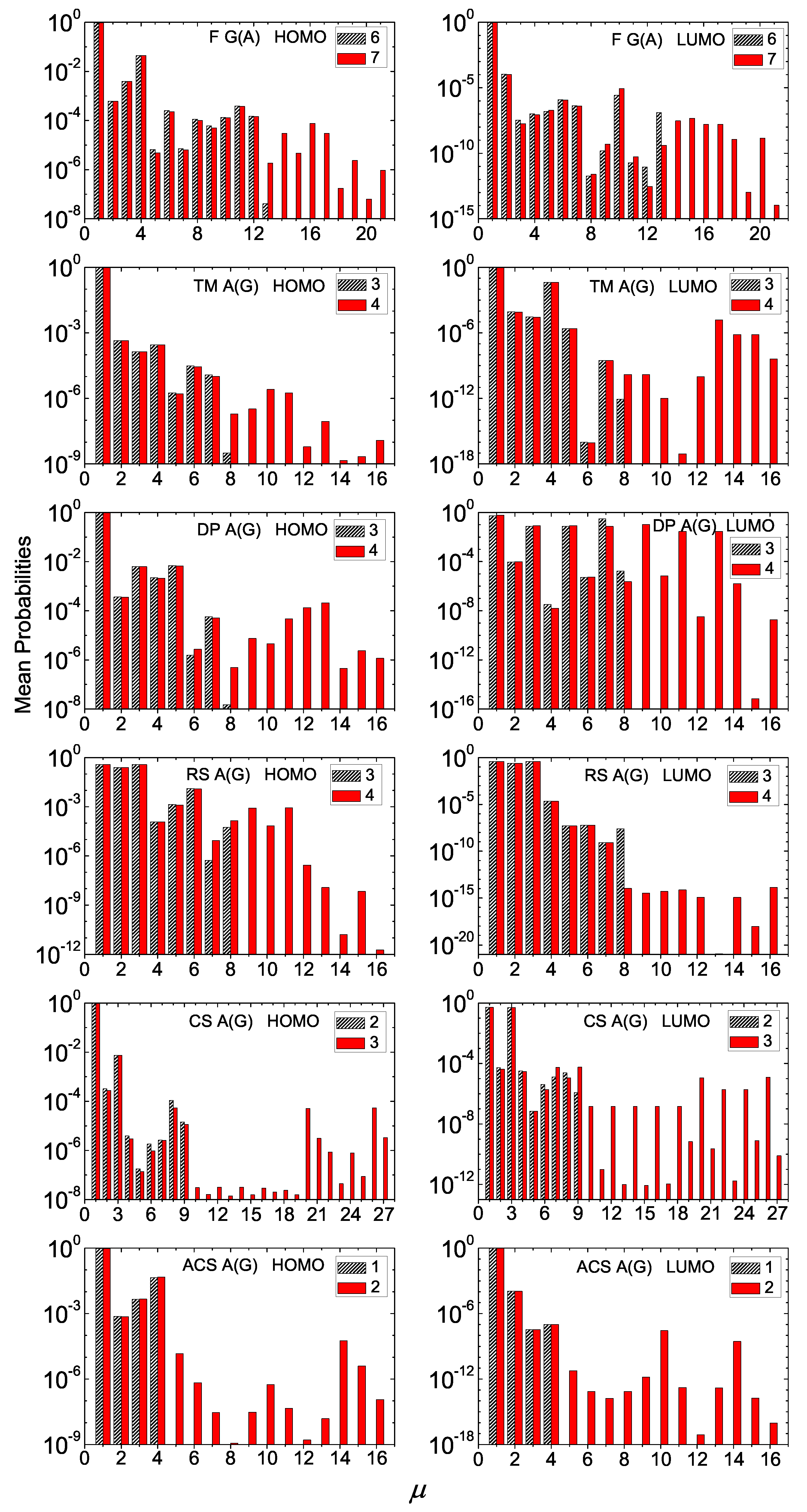
4.2. Mean over Time Probabilities
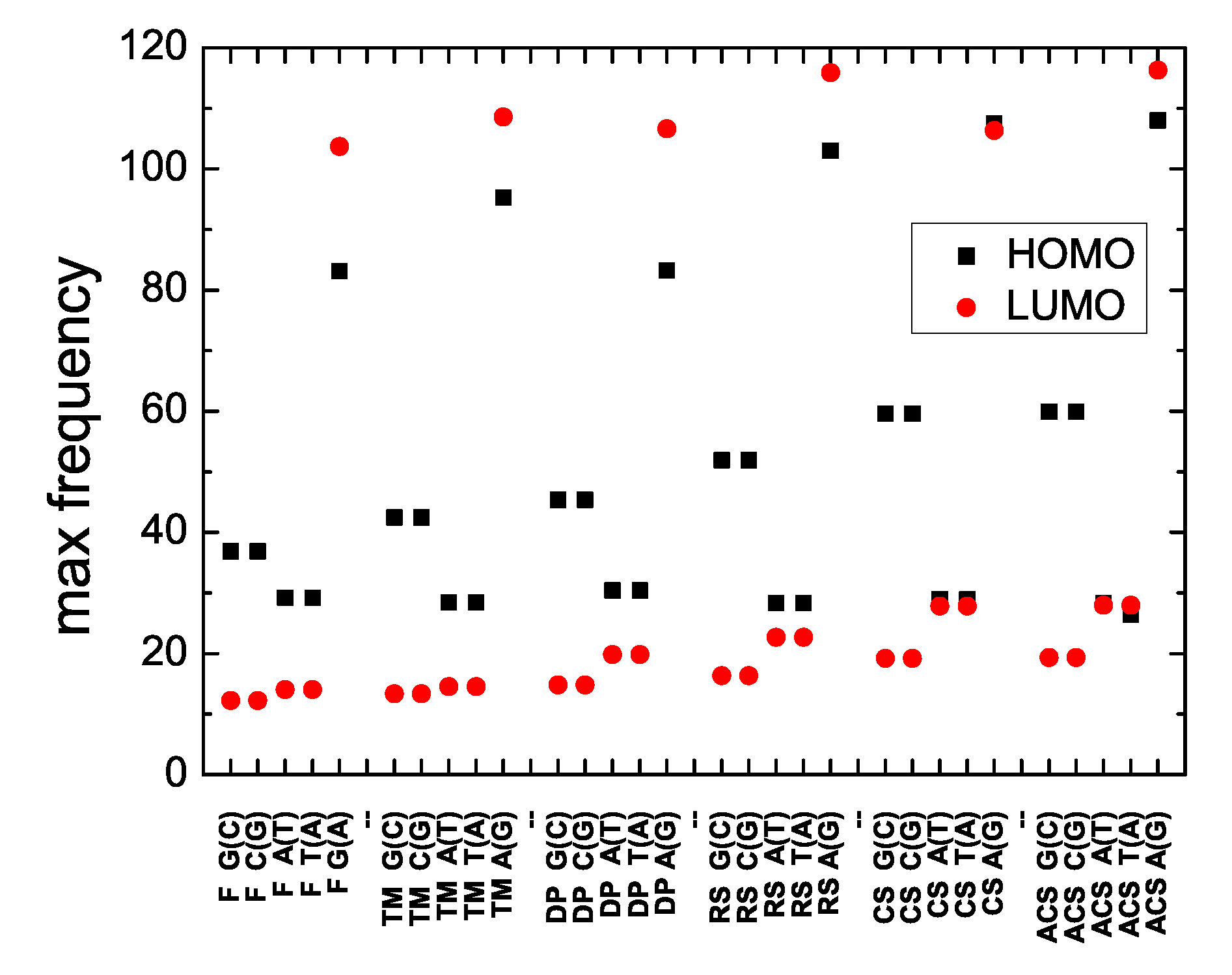
4.3. Frequency Content
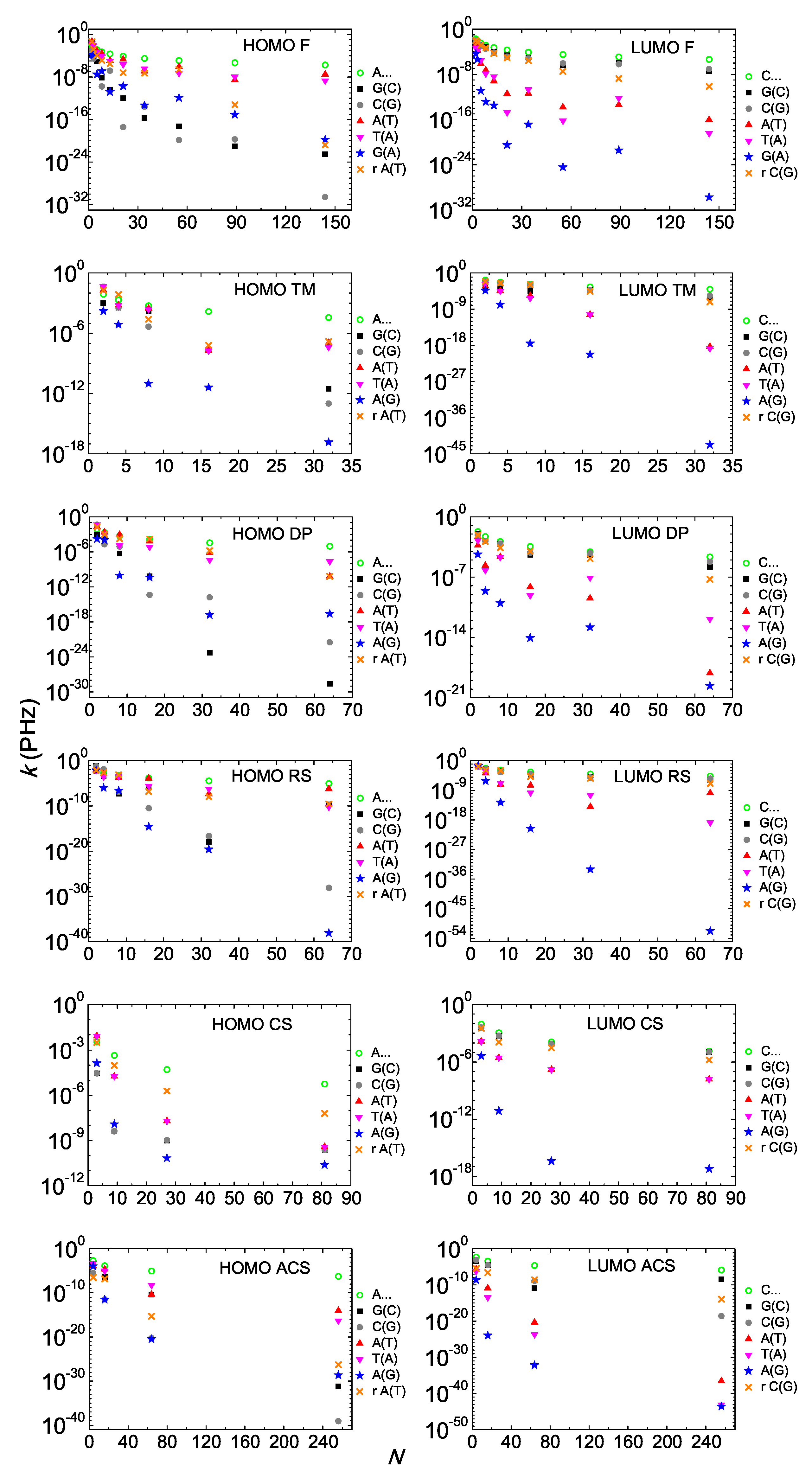
4.4. Pure Mean Transfer Rates
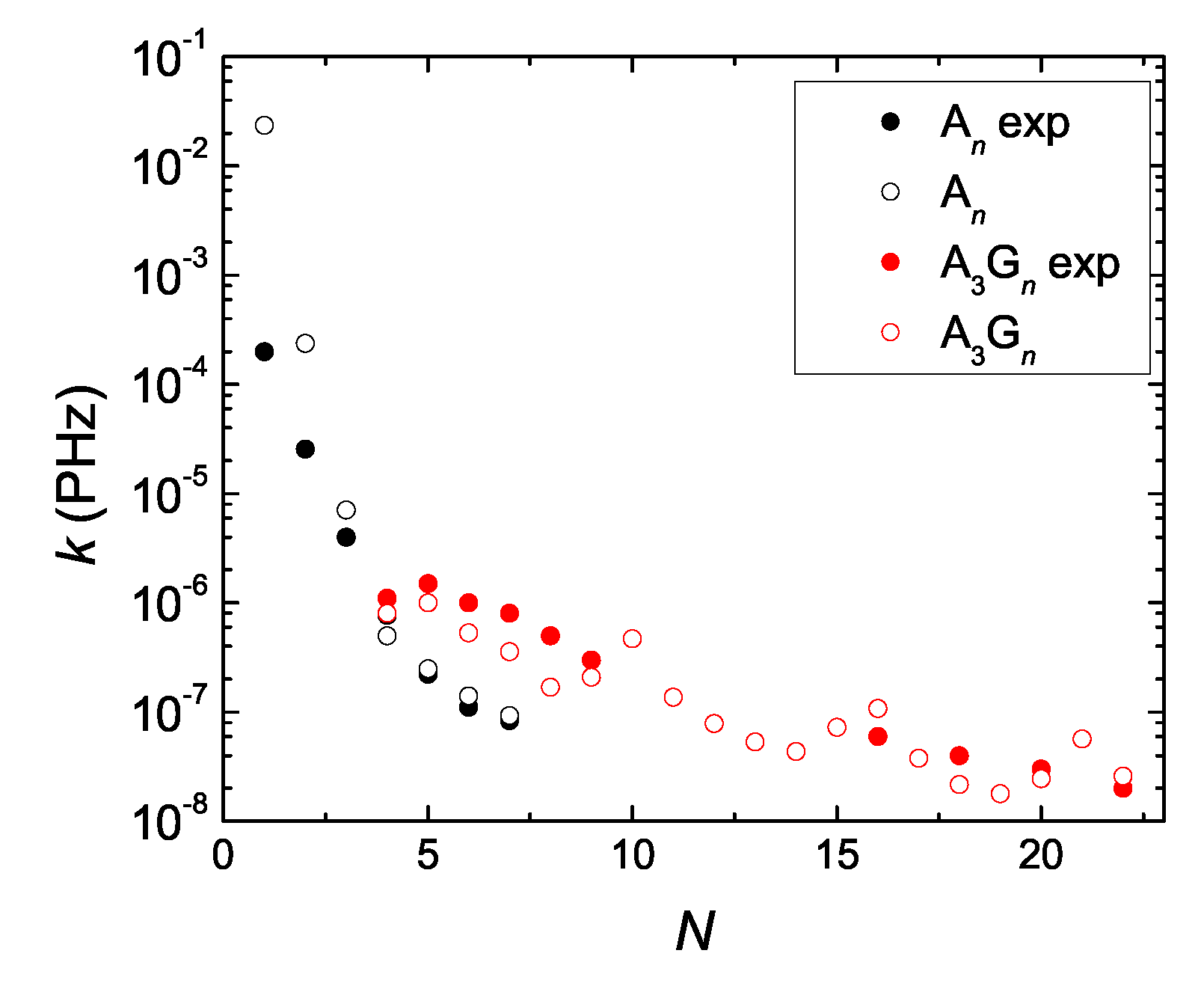
4.5. Transfer Rates in Experiments
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| DNA | Deoxyribonucleic acid |
| RNA | Ribonucleic acid |
| B-DNA | B form of DNA |
| A | Adenine |
| G | Guanine |
| C | Cytosine |
| T | Thymine |
| TB | Tight-Binding |
| I polymers | polymers made of the same monomer |
| D polymers | polymers made of different monomers |
| TM | Thue–Morse |
| F | Fibonacci |
| DP | Double-Period |
| RS | Rudin–Shapiro |
| CS | Cantor Set |
| ACS | Asymmetric Cantor Set |
| DOS | Density of states |
| HOMO | Highest Occupied Molecular Orbital |
| LUMO | Lowest Unoccupied Molecular Orbital |
| WMF | weighted mean frequency |
| TWMF | total weighted mean frequency |
| FIR | far infrared |
| MIR | middle infrared |
Appendix A

References
- Page, C.C.; Moser, C.C.; Dutton, P.L. Mechanism for electron transfer within and between proteins. Curr. Opin. Chem. Biol. 2003, 7, 551–556. [Google Scholar] [CrossRef] [PubMed]
- Giese, B. Electron transfer through DNA and peptides. Bioorg. Med. Chem. 2006, 14, 6139–6143. [Google Scholar] [CrossRef] [PubMed]
- Kannan, A.M.; Renugopalakrishnan, V.; Filipek, S.; Li, P.; Audette, G.F.; Munukutla, L. Bio-Batteries and Bio-Fuel Cells: Leveraging on Electronic Charge Transfer Proteins. J. Nanosci. Nanotechnol. 2009, 9, 1665–1678. [Google Scholar] [CrossRef] [PubMed]
- Moser, C.C.; Ross Anderson, J.L.; Dutton, P.L. Guidelines for tunneling in enzymes. Biochim. Biophys. Acta 2010, 1797, 1573–1586. [Google Scholar] [CrossRef] [PubMed]
- Gray, H.B.; Winkler, J.R. Electron flow through metalloproteins. Biochim. Biophys. Acta 2010, 1797, 1563–1572. [Google Scholar] [CrossRef] [PubMed]
- Artés, J.M.; López-Martínez, M.; Díez-Pérez, I.; Sanz, F.; Gorostiza, P. Nanoscale charge transfer in redox proteins and DNA: Towards biomolecular electronics. Electrochim. Acta 2014, 140, 83–95. [Google Scholar] [CrossRef]
- Dandliker, P.J.; Holmlin, R.E.; Barton, J.K. Oxidative Thymine Dimer Repair in the DNA Helix. Science 1997, 275, 1465–1468. [Google Scholar] [CrossRef] [PubMed]
- Rajski, S.R.; Jackson, B.A.; Barton, J.K. DNA repair: models for damage and mismatch recognition. Mutat. Res. 2000, 447, 49–72. [Google Scholar] [CrossRef]
- Burrows, C.J.; Muller, J.G. Oxidative Nucleobase Modifications Leading to Strand Scission. Chem. Rev. 1998, 98, 1109–1152. [Google Scholar] [CrossRef]
- Cadet, J. DNA damage caused by oxidation, deamination, ultraviolet radiation and photoexcited psoralens. In DNA Adducts: Identification and Biological Significance; Hemminki, K., Dipple, A., Shuker, D.E.G., Kadlubar, F.F., Segerback, D., Bartsch, H., Eds.; Scientific Publication No 125; International Agency for Research on Cancer: Lyon, France, 1994; pp. 245–276. [Google Scholar]
- Shih, C.T.; Cheng, Y.Y.; Wells, S.A.; Hsu, C.L.; Römer, R.A. Charge transport in cancer-related genes and early carcinogenesis. Comput. Phys. Commun. 2011, 182, 36–38. [Google Scholar] [CrossRef]
- Maciá, E.; Triozon, F.; Roche, S. Contact-dependent effects and tunneling currents in DNA molecules. Phys. Rev. B 2005, 71, 113106. [Google Scholar] [CrossRef]
- Rawtani, D.; Kuntmal, B.; Agrawal, Y. Charge transfer in DNA and its diverse modelling approaches. Front. Life Sci. 2016, 9, 214–225. [Google Scholar] [CrossRef][Green Version]
- Simserides, C. A systematic study of electron or hole transfer along DNA dimers, trimers and polymers. Chem. Phys. 2014, 440, 31–41. [Google Scholar] [CrossRef]
- Lambropoulos, K.; Chatzieleftheriou, M.; Morphis, A.; Kaklamanis, K.; Theodorakou, M.; Simserides, C. Unbiased charge oscillations in B-DNA: Monomer polymers and dimer polymers. Phys. Rev. E 2015, 92, 032725. [Google Scholar] [CrossRef] [PubMed]
- Lambropoulos, K.; Chatzieleftheriou, M.; Morphis, A.; Kaklamanis, K.; Lopp, R.; Theodorakou, M.; Tassi, M.; Simserides, C. Electronic structure and carrier transfer in B-DNA monomer polymers and dimer polymers: Stationary and time-dependent aspects of a wire model versus an extended ladder model. Phys. Rev. E 2016, 94, 062403. [Google Scholar] [CrossRef] [PubMed]
- Lambropoulos, K.; Kaklamanis, K.; Morphis, A.; Tassi, M.; Lopp, R.; Georgiadis, G.; Theodorakou, M.; Chatzieleftheriou, M.; Simserides, C. Wire and extended ladder model predict THz oscillations in DNA monomers, dimers and trimers. J. Phys. Condens. Matter 2016, 28, 495101. [Google Scholar] [CrossRef] [PubMed]
- Lambropoulos, K.; Vantaraki, C.; Bilia, P.; Mantela, M.; Simserides, C. Periodic polymers with increasing repetition unit: Energy structure and carrier transfer. Phys. Rev. E 2018, 98, 032412. [Google Scholar] [CrossRef]
- Hawke, L.G.D.; Kalosakas, G.; Simserides, C. Electronic parameters for charge transfer along DNA. Eur. Phys. J. E 2010, 32, 291–305. [Google Scholar] [CrossRef] [PubMed]
- Marcus, R.A. Nonadiabatic processes involving quantumlike and classical-like coordinates with applications to nonadiabatic electron transfers. J. Chem. Phys. 1984, 81, 4494–4500. [Google Scholar] [CrossRef]
- Marcus, R.A. Electron transfer reactions in chemistry. Theory and experiment. Rev. Mod. Phys. 1993, 65, 599–610. [Google Scholar] [CrossRef]
- Miller, A.; Abrahams, E. Impurity Conduction at Low Concentrations. Phys. Rev. 1960, 120, 745–755. [Google Scholar] [CrossRef]
- Fishchuk, I.I.; Kadashchuk, A.; Hoffmann, S.T.; Athanasopoulos, S.; Genoe, J.; Bässler, H.; Köhler, A. Unified description for hopping transport in organic semiconductors including both energetic disorder and polaronic contributions. Phys. Rev. B 2013, 88, 125202. [Google Scholar] [CrossRef]
- Fishchuk, I.I.; Kadashchuk, A.; Hoffmann, S.T.; Athanasopoulos, S.; Genoe, J.; Bässler, H.; Köhler, A. Analytic model of hopping transport in organic semiconductors including both energetic disorder and polaronic contributions. AIP Conf. Proc. 2014, 1610, 47–52. [Google Scholar] [CrossRef]
- Vukmirović, N.; Wang, L.W. Carrier hopping in disordered semiconducting polymers: How accurate is the Miller–Abrahams model? Appl. Phys. Lett. 2010, 97, 043305. [Google Scholar] [CrossRef]
- Oelerich, J.O.; Jansson, F.; Nenashev, A.V.; Gebhard, F.; Baranovskii, S.D. Energy position of the transport path in disordered organic semiconductors. J. Phys. Condens. Matter 2014, 26, 255801. [Google Scholar] [CrossRef] [PubMed]
- Van der Kaap, N.J.; Katsouras, I.; Asadi, K.; Blom, P.W.M.; Koster, L.J.A.; de Leeuw, D.M. Charge transport in disordered semiconducting polymers driven by nuclear tunneling. Phys. Rev. B 2016, 93, 140206(R). [Google Scholar] [CrossRef]
- Marcus, R.A.; Sutin, N. Electron transfers in chemistry and biology. Biochim. Biophys. Acta 1985, 811, 265–322. [Google Scholar] [CrossRef]
- Tessler, N.; Preezant, Y.; Rappaport, N.; Roichman, Y. Charge Transport in Disordered Organic Materials and Its Relevance to Thin-Film Devices: A Tutorial Review. Adv. Mater. 2009, 21, 2741–2761. [Google Scholar] [CrossRef]
- Segal, D.; Nitzan, A.; Davis, W.B.; Wasielewski, M.R.; Ratner, M.A. Electron Transfer Rates in Bridged Molecular Systems 2. A Steady-State Analysis of Coherent Tunneling and Thermal Transitions. J. Phys. Chem. B 2000, 104, 3817–3829. [Google Scholar] [CrossRef]
- Shimazaki, T.; Asai, Y.; Yamashita, K. Theoretical Rate Constants of Super-Exchange Hole Transfer and Thermally Induced Hopping in DNA. J. Phys. Chem. B 2005, 109, 1295–1303. [Google Scholar] [CrossRef]
- Manning, G.S. The persistence length of DNA is reached from the persistence length of its null isomer through an internal electrostatic stretching force. Biophys. J. 2006, 91, 3607–3616. [Google Scholar] [CrossRef] [PubMed]
- Koslowski, T.; Jurjiu, A.; Blumen, A. Polaron Formation and Hopping Conduction in Hyperbranched Polymers: A Theoretical Approach. J. Phys. Chem. B 2004, 108, 3283–3288. [Google Scholar] [CrossRef]
- Koslowski, T.; Jurjiu, A.; Blumen, A. Models of Irregular Hyperbranched Polymers: Topological Disorder and Mechanical Response. Macromol. Theory Simul. 2006, 15, 538–545. [Google Scholar] [CrossRef]
- Jurjiu, A.; Turcu, F.; Galiceanu, M. Dynamics of a Complex Multilayer Polymer Network: Mechanical Relaxation and Energy Transfer. Polymers 2018, 10, 164. [Google Scholar] [CrossRef] [PubMed]
- Wohlgamuth, C.H.; McWilliams, M.A.; Slinker, J.D. DNA as a Molecular Wire: Distance and Sequence Dependence. Anal. Chem. 2013, 85, 8634–8640. [Google Scholar] [CrossRef] [PubMed]
- Lewis, F.D.; Wasielewski, M.R. Dynamics and efficiency of photoinduced charge transport in DNA: Toward the elusive molecular wire. Pure Appl. Chem. 2013, 85, 1379–1387. [Google Scholar] [CrossRef]
- Kawai, K.; Majima, T. Increasing the hole transfer rate through DNA by chemical modification. In Chemical Science of π-Electron Systems; Akasaka, T., Fukuzumi, A.O.S., Kandori, H., Aso, Y., Eds.; Springer: Tokyo, Japan, 2015. [Google Scholar] [CrossRef]
- Gutiérrez, R.; Caetano, R.; Woiczikowski, P.B.; Kubař, T.; Elstner, M.; Cuniberti, G. Structural fluctuations and quantum transport through DNA molecular wires: A combined molecular dynamics and model Hamiltonian approach. New J. Phys. 2010, 12, 023022. [Google Scholar] [CrossRef]
- Ye, Y.J.; Shen, L.L. DFT approach to calculate electronic transfer through a segment of DNA double helix. J. Comput. Chem. 2000, 21, 1109–1117. [Google Scholar] [CrossRef]
- Ye, Y.J.; Jiang, Y. Electronic structures and long-range electron transfer through DNA molecules. Int. J. Quantum Chem. 2000, 78, 112–130. [Google Scholar] [CrossRef]
- Barnett, R.N.; Cleveland, C.L.; Landman, U.; Boone, E.; Kanvah, S.; Schuster, G.B. Effect of base sequence and hydration on the electronic and hole transport properties of duplex DNA: Theory and Experiment. J. Phys. Chem. A 2003, 107, 3525–3537. [Google Scholar] [CrossRef]
- Artacho, E.; Machado, M.; Sánchez-Portal, D.; Ordejón, P.; Soler, J.M. Electrons in dry DNA from density functional calculations. Mol. Phys. 2003, 101, 1587–1594. [Google Scholar] [CrossRef][Green Version]
- Adessi, C.; Walch, S.; Anantram, M.P. Environment and structure influence on DNA conduction. Phys. Rev. B 2003, 67, 081405. [Google Scholar] [CrossRef]
- Mehrez, H.; Anantram, M.P. Interbase electronic coupling for transport through DNA. Phys. Rev. B 2005, 71, 115405. [Google Scholar] [CrossRef]
- Voityuk, A.A. Electronic couplings and on-site energies for hole transfer in DNA: Systematic quantum mechanical/molecular dynamic study. J. Chem. Phys. 2008, 128, 115101. [Google Scholar] [CrossRef] [PubMed]
- Kubař, T.; Woiczikowski, P.B.; Cuniberti, G.; Elstner, M. Efficient Calculation of Charge-Transfer Matrix Elements for Hole Transfer in DNA. J. Phys. Chem. B 2008, 112, 7937–7947. [Google Scholar] [CrossRef] [PubMed]
- Tassi, M.; Morphis, A.; Lambropoulos, K.; Simserides, C. RT-TDDFT study of hole oscillations in B-DNA monomers and dimers. Cogent Phys. 2017, 4, 1361077. [Google Scholar] [CrossRef]
- Cuniberti, G.; Craco, L.; Porath, D.; Dekker, C. Backbone-induced semiconducting behavior in short DNA wires. Phys. Rev. B 2002, 65, 241314. [Google Scholar] [CrossRef]
- Roche, S.; Bicout, D.; Maciá, E.; Kats, E. Long Range Correlations in DNA: Scaling Properties and Charge Transfer Efficiency. Phys. Rev. Lett. 2003, 91, 228101. [Google Scholar] [CrossRef] [PubMed]
- Roche, S. Sequence Dependent DNA-Mediated Conduction. Phys. Rev. Lett. 2003, 91, 108101. [Google Scholar] [CrossRef]
- Palmero, F.; Archilla, J.F.R.; Hennig, D.; Romero, F.R. Effect of base-pair inhomogeneities on charge transport along the DNA molecule, mediated by twist and radial polarons. New J. Phys. 2004, 6, 13. [Google Scholar] [CrossRef]
- Yamada, H. Localization of electronic states in chain models based on real DNA sequence. Phys. Lett. A 2004, 332, 65–73. [Google Scholar] [CrossRef][Green Version]
- Apalkov, V.M.; Chakraborty, T. Electron dynamics in a DNA molecule. Phys. Rev. B 2005, 71, 033102. [Google Scholar] [CrossRef]
- Klotsa, D.; Römer, R.A.; Turner, M.S. Electronic Transport in DNA. Biophys. J. 2005, 89, 2187–2198. [Google Scholar] [CrossRef] [PubMed]
- Shih, C.T.; Roche, S.; Römer, R.A. Point-Mutation Effects on Charge-Transport Properties of the Tumor-Suppressor Gene p53. Phys. Rev. Lett. 2008, 100, 018105. [Google Scholar] [CrossRef] [PubMed]
- Joe, Y.S.; Lee, S.H.; Hedin, E.R. Electron transport through asymmetric DNA molecules. Phys. Lett. A 2010, 374, 2367–2373. [Google Scholar] [CrossRef]
- Yi, J. Conduction of DNA molecules: A charge-ladder model. Phys. Rev. B 2003, 68, 193103. [Google Scholar] [CrossRef]
- Caetano, R.A.; Schulz, P.A. Sequencing-Independent Delocalization in a DNA-Like Double Chain with Base Pairing. Phys. Rev. Lett. 2005, 95, 126601. [Google Scholar] [CrossRef]
- Wang, X.F.; Chakraborty, T. Charge Transfer via a Two-Strand Superexchange Bridge in DNA. Phys. Rev. Lett. 2006, 97, 106602. [Google Scholar] [CrossRef]
- Albuquerque, E.L.; Fulco, U.L.; Freire, V.N.; Caetano, E.W.S.; Lyra, M.L.; de Moura, F.A.B.F. DNA-based nanobiostructured devices: The role of quasiperiodicity and correlation effects. Phys. Rep. 2014, 535, 139–209. [Google Scholar] [CrossRef]
- Sarmento, R.G.; Mendes, G.A.; Albuquerque, E.L.; Fulco, U.L.; Vasconcelos, M.S.; Ujsághy, O.; Freire, V.N.; Caetano, E.W.S. The DNA electronic specific heat at low temperature: The role of aperiodicity. Phys. Lett. A 2012, 376, 2413–2417. [Google Scholar] [CrossRef]
- Sarmento, R.G.; Albuquerque, E.L.; Sesion, P.D.; Fulco, U.L.; de Oliveira, B.P.W. Electronic transport in double-strand poly(dG)–poly(dC) DNA segments. Phys. Lett. A 2009, 373, 1486–1491. [Google Scholar] [CrossRef]
- Albuquerque, E.L.; Vasconcelos, M.S.; Lyra, M.L.; de Moura, F.A.B.F. Nucleotide correlations and electronic transport of DNA sequences. Phys. Rev. E 2005, 71, 021910. [Google Scholar] [CrossRef] [PubMed]
- Cuniberti, G.; Maciá, E.; Rodríguez, A.; Römer, R.A. Tight-Binding Modeling of Charge Migration in DNA Devices. In Charge Migration in DNA: Perspectives from Physics, Chemistry, and Biology; Chakraborty, T., Ed.; Springer: Berlin/Heidelberg, Germany, 2007; pp. 1–20. [Google Scholar] [CrossRef]
- Maciá, E. Electronic structure and transport properties of double-stranded Fibonacci DNA. Phys. Rev. B 2006, 74, 245105. [Google Scholar] [CrossRef]
- Páez, C.J.; Schulz, P.A.; Wilson, N.R.; Römer, R.A. Robust signatures in the current–voltage characteristics of DNA molecules oriented between two graphene nanoribbon electrodes. New J. Phys. 2012, 14, 093049. [Google Scholar] [CrossRef]
- Kundu, S.; Karmakar, S.N. Electronic specific heat of DNA: Effects of backbones and disorder. Phys. Lett. A 2015, 379, 1377–1383. [Google Scholar] [CrossRef]
- Fathizadeh, S.; Behnia, S.; Ziaei, J. Engineering DNA Molecule Bridge between Metal Electrodes for High-Performance Molecular Transistor: An Environmental Dependent Approach. J. Phys. Chem. B 2018, 122, 2487–2494. [Google Scholar] [CrossRef] [PubMed]
- Lambropoulos, K.; Simserides, C. Periodic, quasiperiodic, fractal, Kolakoski, and random binary polymers: Energy structure and carrier transport. Phys. Rev. E 2019, 99, 032415. [Google Scholar] [CrossRef]
- Sigler, L. Fibonacci’s Liber Abaci: A Translation into Modern English of Leonardo Pisano’s Book of Calculation; Springer: New York, NY, USA, 2003. [Google Scholar] [CrossRef]
- Singh, P. The so-called fibonacci numbers in ancient and medieval India. Hist. Math. 1985, 12, 229–244. [Google Scholar] [CrossRef]
- Prouhet, E. Mémoire sur les relations entre les puissances des nombres. C. R. Acad. Sci. Paris 1851, 33, 225. (In French) [Google Scholar]
- Nagell, T.; Selberg, A.; Selberg, S.; Thalberg, K. (Eds.) Selected Mathematical Papers of Axel Thue; Universitetsforlaget: Oslo, Norway, 1977. [Google Scholar]
- Morse, H.M. Recurrent Geodesics on a Surface of Negative Curvature. Trans. Am. Math. Soc. 1921, 22, 84–100. [Google Scholar] [CrossRef]
- Rahimi, H. Analysis of photonic spectra in Thue–Morse, double-period and Rudin–Shapiro quasiregular structures made of high temperature superconductors in visible range. Opt. Mater. 2016, 57, 264–271. [Google Scholar] [CrossRef]
- Janot, C. Quasicrystals: A Primer; Clarendon Press: Oxford, UK, 1955. [Google Scholar]
- Brillhart, J.; Morton, P. A Case Study in Mathematical Research: The Golay-Rudin–Shapiro Sequence. Am. Math. Mon. 1996, 103, 854–869. [Google Scholar] [CrossRef]
- Shapiro, H.S. Extremal Problems for Polynomials and Power Series. Master’s Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 1951. [Google Scholar]
- Rudin, W. Some theorems on Fourier coefficients. Proc. Am. Math. Soc. 1959, 10, 855. [Google Scholar] [CrossRef]
- Cantor, G. Über unendliche, lineare Punktmannigfaltigkeiten. Math. Ann. 1883, 21, 545. (In German) [Google Scholar] [CrossRef]
- Cullum, J.K.; Willoughby, R.A. Tridiagonal Matrices. In Lanczos Algorithms for Large Symmetric Eigenvalue Computations; Society for Industrial and Applied Mathematics: Philadelphia, PA, USA, 2002; pp. 76–91. [Google Scholar] [CrossRef]
- Marques, M.A.L.; Ullrich, C.A.; Nogueira, F.; Rubio, A.; Burke, K.; Gross, E.K.U. (Eds.) Time-Dependent Density Functional Theory; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar] [CrossRef]
- Ralha, R. Perturbation Splitting for More Accurate Eigenvalues. SIAM J. Matrix Anal. Appl. 2009, 31, 75–91. [Google Scholar] [CrossRef]
- Mantela, M. Charge Transfer in Aperiodic B-DNA Segments: Tight Binding Description at the Base-Pair Level. Master’s Thesis, National and Kapodistrian University of Athens, Athens, Greece, 2017. (In Greek). [Google Scholar]
- Theodorakou, M. Charge Transfer in Aperiodic B-DNA Segments, Made of Different Base Pairs: Tight Binding Description at the Base-Pair Level. Master’s Thesis, National and Kapodistrian University of Athens, Athens, Greece, 2018. (In Greek). [Google Scholar]
- Lambropoulos, K.; Mantela, M.; Simserides, C. Frequency content of carrier oscillations along B-DNA polymers. In Proceedings of the 2017 Progress in Electromagnetics Research Symposium—Fall (PIERS— FALL), Singapore, 19–22 November 2017; pp. 186–193. [Google Scholar] [CrossRef]
- Vantaraki, C. Carrier Transfer in Periodic Polymer B-DNA Segments Based on the G-C Monomer: Base-Pair-Level Description within the Tight-Binding Approach. Bachelor’s Thesis, National and Kapodistrian University of Athens, Athens, Greece, 2017. (In Greek). [Google Scholar]
- Bilia, P. Carrier Transfer in Periodic Polymer B-DNA Segments Based on the G-C and a-T Monomers with Purine on Purine: Base-Pair-Level Description within the Tight-Binding Approach. Bachelor’s Thesis, National and Kapodistrian University of Athens, Athens, Greece, 2019. (In Greek). [Google Scholar]
- Meggers, E.; Michel-Beyerle, M.E.; Giese, B. Sequence Dependent Long Range Hole Transport in DNA. J. Am. Chem. Soc. 1998, 120, 12950–12955. [Google Scholar] [CrossRef]
- Giese, B.; Amaudrut, J.; Köhler, A.K.; Spormann, M.; Wessely, S. Direct observation of hole transfer through DNA by hopping between adenine bases and by tunnelling. Nature 2001, 412, 318–320. [Google Scholar] [CrossRef]
- Kawai, K.; Majima, T. Hole Transfer Kinetics of DNA. Acc. Chem. Res. 2013, 46, 2616–2625. [Google Scholar] [CrossRef]
- Lewis, F.D.; Wu, T.; Zhang, Y.; Letsinger, R.L.; Greenfield, S.R.; Wasielewski, M.R. Distance-Dependent Electron Transfer in DNA Hairpins. Science 1997, 277, 673–676. [Google Scholar] [CrossRef]
- Wan, C.; Fiebig, T.; Schiemann, O.; Barton, J.K.; Zewail, A.H. Femtosecond direct observation of charge transfer between bases in DNA. Proc. Natl. Acad. Sci. USA 2000, 97, 14052–14055. [Google Scholar] [CrossRef]
- Takada, T.; Kawai, K.; Fujitsuka, M.; Majima, T. Direct observation of hole transfer through double-helical DNA over 100 A. Proc. Natl. Acad. Sci. USA 2004, 101, 14002–14006. [Google Scholar] [CrossRef] [PubMed]
- Mickley Conron, S.M.; Thazhathveetil, A.K.; Wasielewski, M.R.; Burin, A.L.; Lewis, F.D. Direct Measurement of the Dynamics of Hole Hopping in Extended DNA G-Tracts. An Unbiased Random Walk. J. Am. Chem. Soc. 2010, 132, 14388–14390. [Google Scholar] [CrossRef] [PubMed]
- Vura-Weis, J.; Wasielewski, M.R.; Thazhathveetil, A.K.; Lewis, F.D. Efficient Charge Transport in DNA Diblock Oligomers. J. Am. Chem. Soc. 2009, 131, 9722–9727. [Google Scholar] [CrossRef] [PubMed]



| Type | Sequence Example | Notation |
|---|---|---|
| Fibonacci I | G, C, CG, CGC, CGCCG, … | F G(C) |
| Fibonacci D | G, A, AG, AGA, AGAAG, … | F G(A) |
| Thue–Morse I | G, GC, GCCG, GCCGCGGC, … | TM G(C) |
| Thue–Morse D | A, AG, AGGA, AGGAGAAG, … | TM A(G) |
| Double Period I | T, TA, TATT, TATTTATA, … | DP T(A) |
| Double Period D | A, AG, AGAA, AGAAAGAG, … | DP A(G) |
| Rudin–Shapiro I | AA, AAAT, AAATAATA, … | RS A(T) |
| Rudin–Shapiro D | AA, AAAG, AAAGAAGA, … | RS A(G) |
| Cantor Set I | T, TAT, TATAAATAT, … | CS T(A) |
| Cantor Set D | A, AGA, AGAGGGAGA, … | CS A(G) |
| Asymmetric Cantor Set I | C, CGCC, CGCCGGGGCGCCCGCC, … | ACS C(G) |
| Asymmetric Cantor Set D | A, AGAA, AGAAGGGGAGAAAGAA, … | ACS A(G) |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mantela, M.; Lambropoulos, K.; Theodorakou, M.; Simserides, C. Quasi-Periodic and Fractal Polymers: Energy Structure and Carrier Transfer. Materials 2019, 12, 2177. https://doi.org/10.3390/ma12132177
Mantela M, Lambropoulos K, Theodorakou M, Simserides C. Quasi-Periodic and Fractal Polymers: Energy Structure and Carrier Transfer. Materials. 2019; 12(13):2177. https://doi.org/10.3390/ma12132177
Chicago/Turabian StyleMantela, Marilena, Konstantinos Lambropoulos, Marina Theodorakou, and Constantinos Simserides. 2019. "Quasi-Periodic and Fractal Polymers: Energy Structure and Carrier Transfer" Materials 12, no. 13: 2177. https://doi.org/10.3390/ma12132177
APA StyleMantela, M., Lambropoulos, K., Theodorakou, M., & Simserides, C. (2019). Quasi-Periodic and Fractal Polymers: Energy Structure and Carrier Transfer. Materials, 12(13), 2177. https://doi.org/10.3390/ma12132177






