Theoretical and Experimental Study on the Effective Piezoelectric Properties of 1-3 Type Cement-Based Piezoelectric Composites
Abstract
1. Introduction
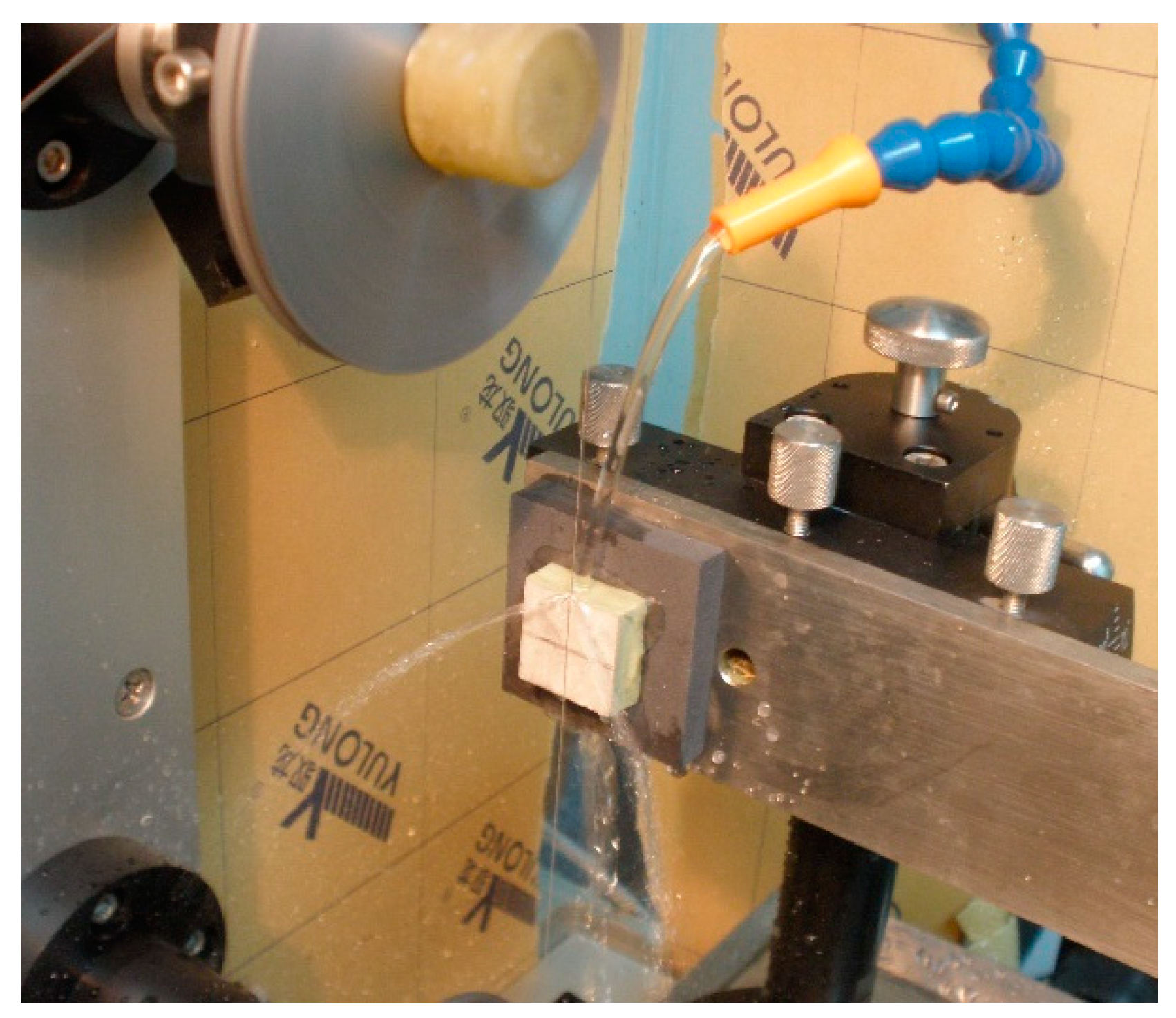
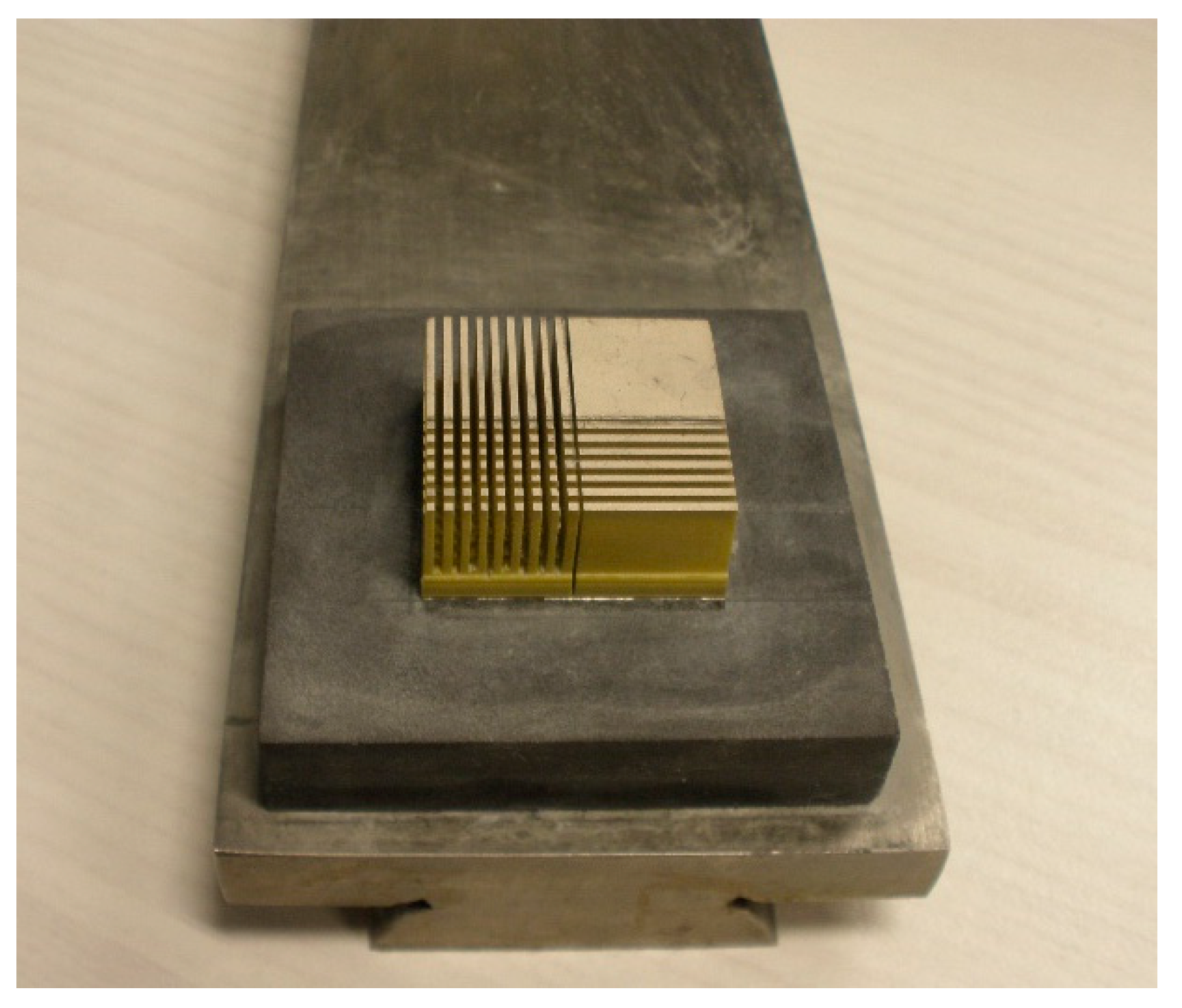
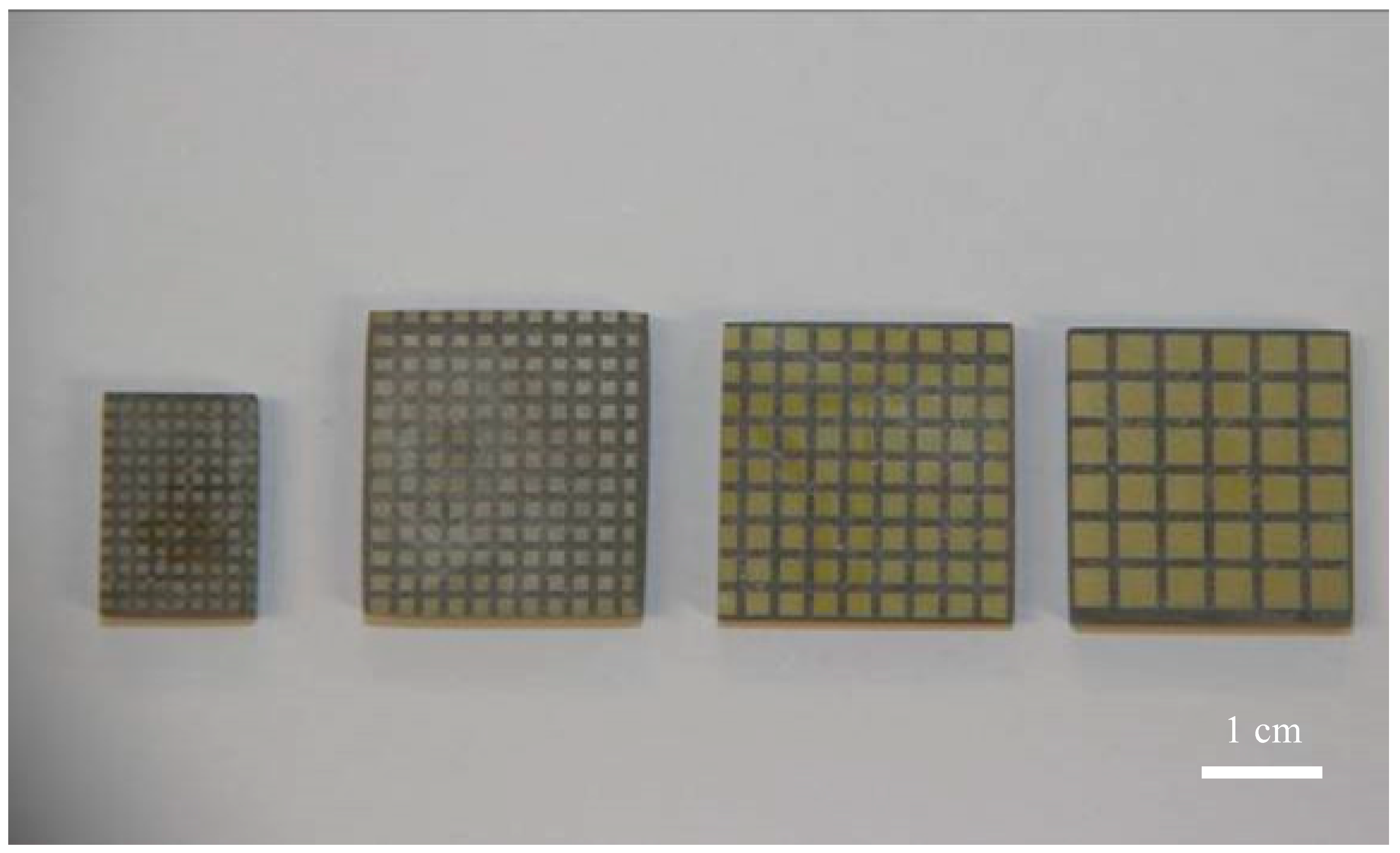
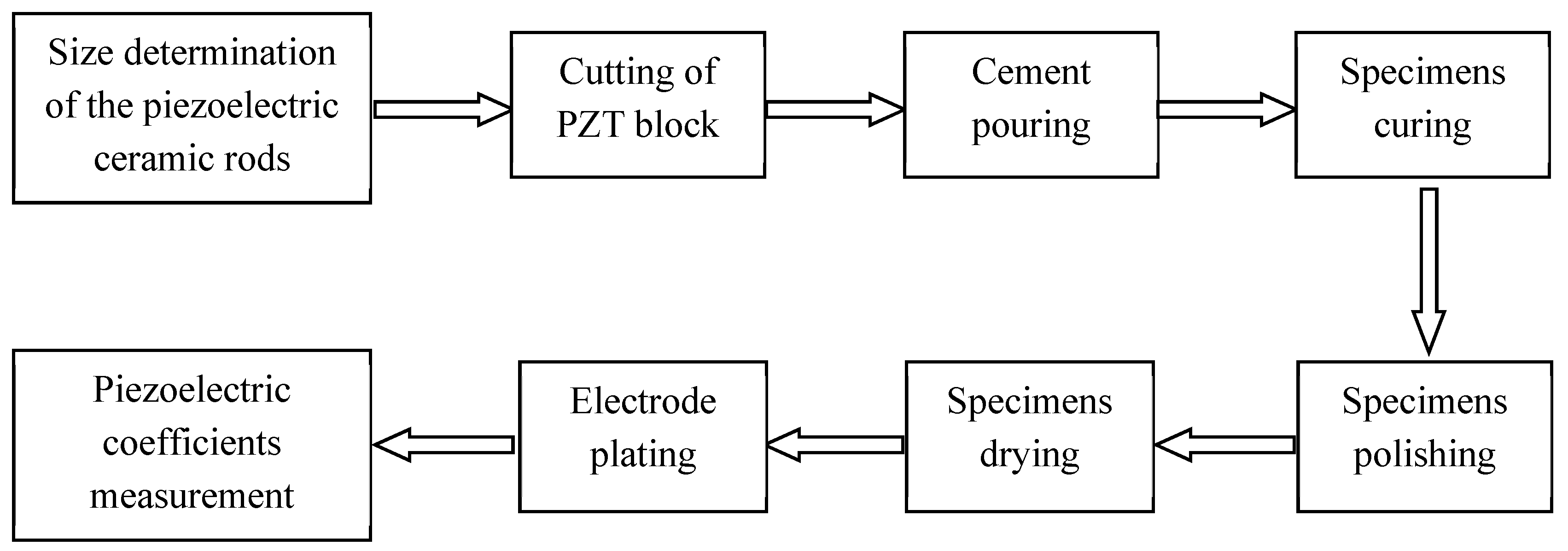
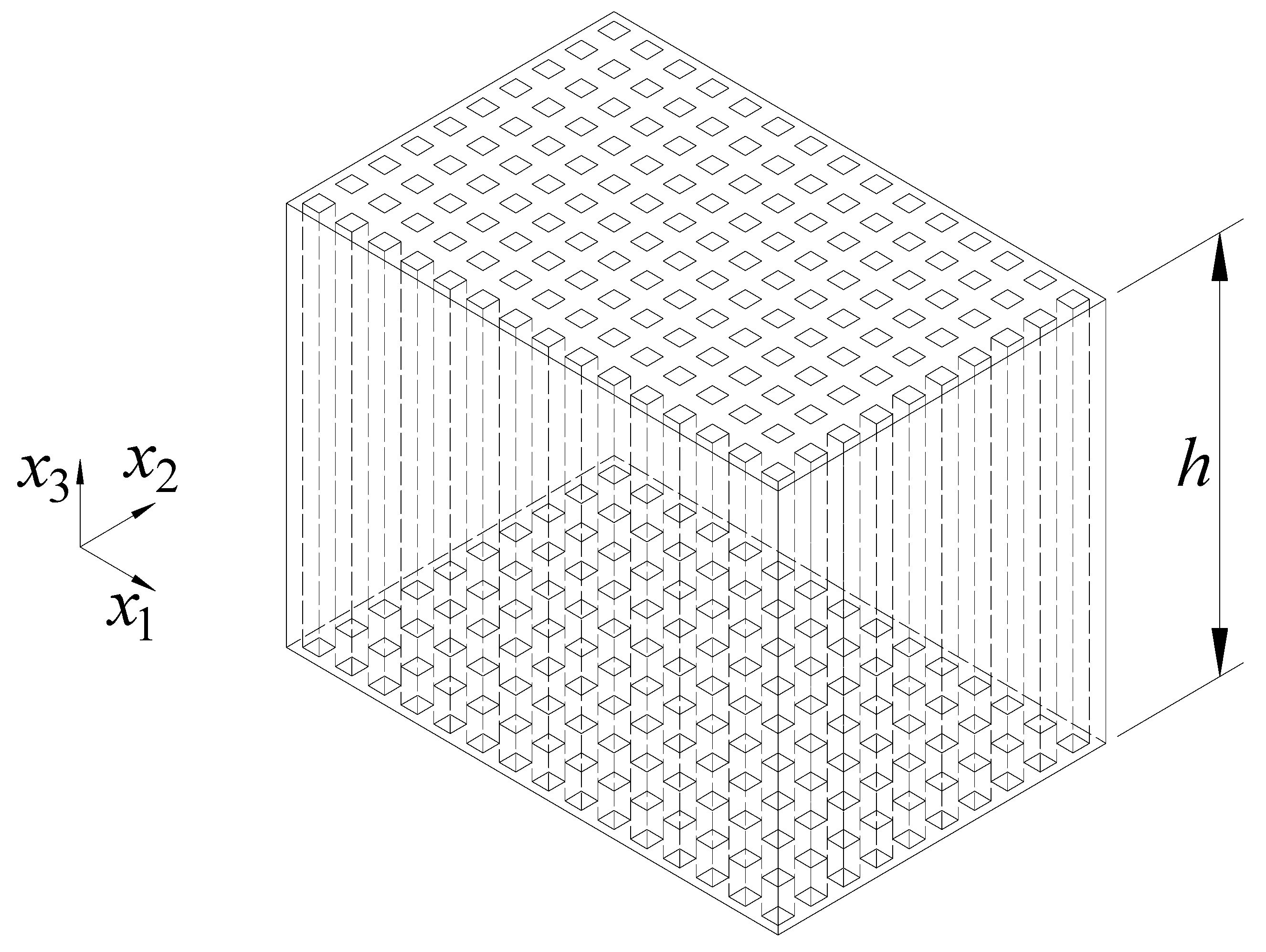
2. Experiment
3. Multiscale Homogenization Model
3.1. Basic Equations
3.2. Basic Theory of Multiscale Homogenization
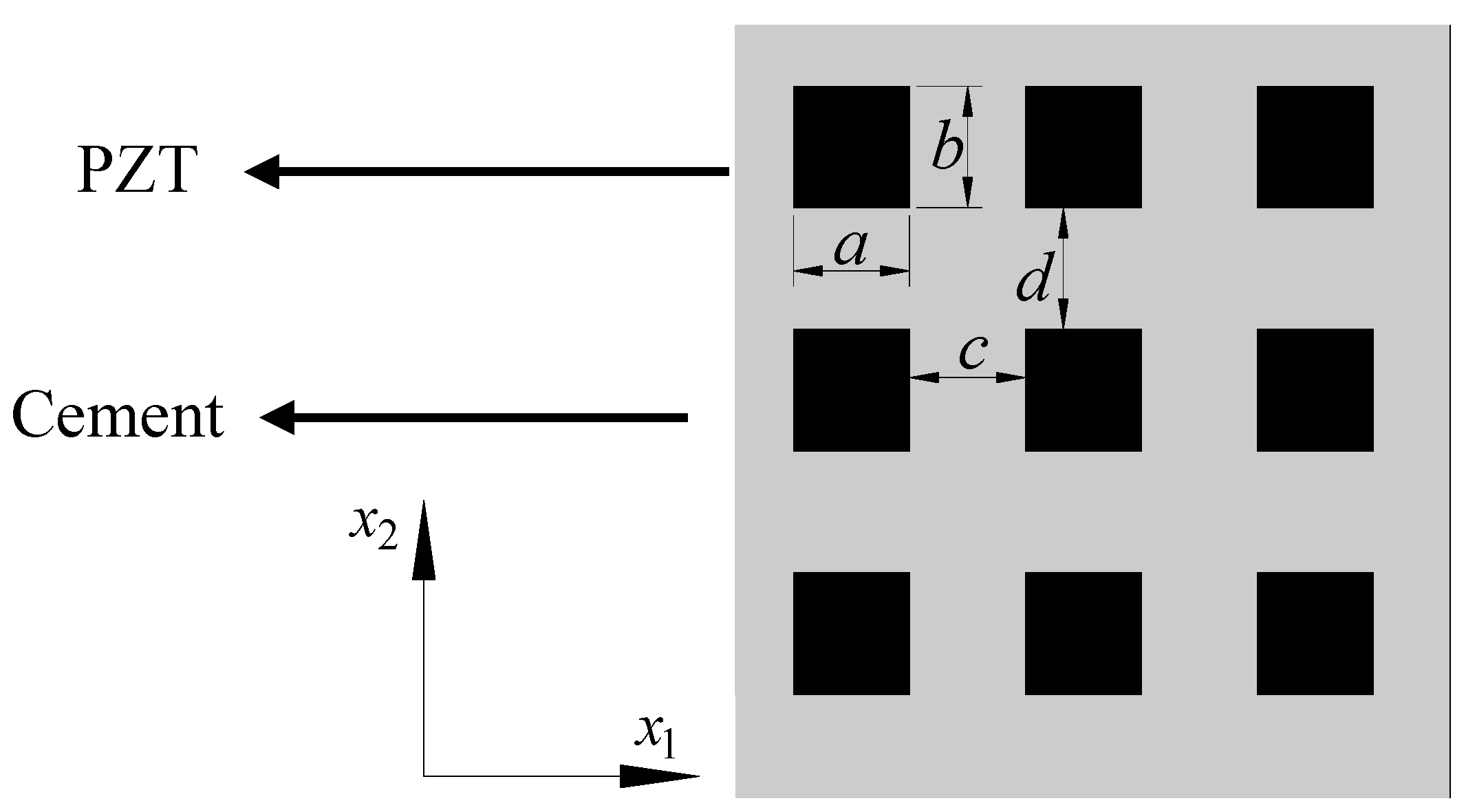
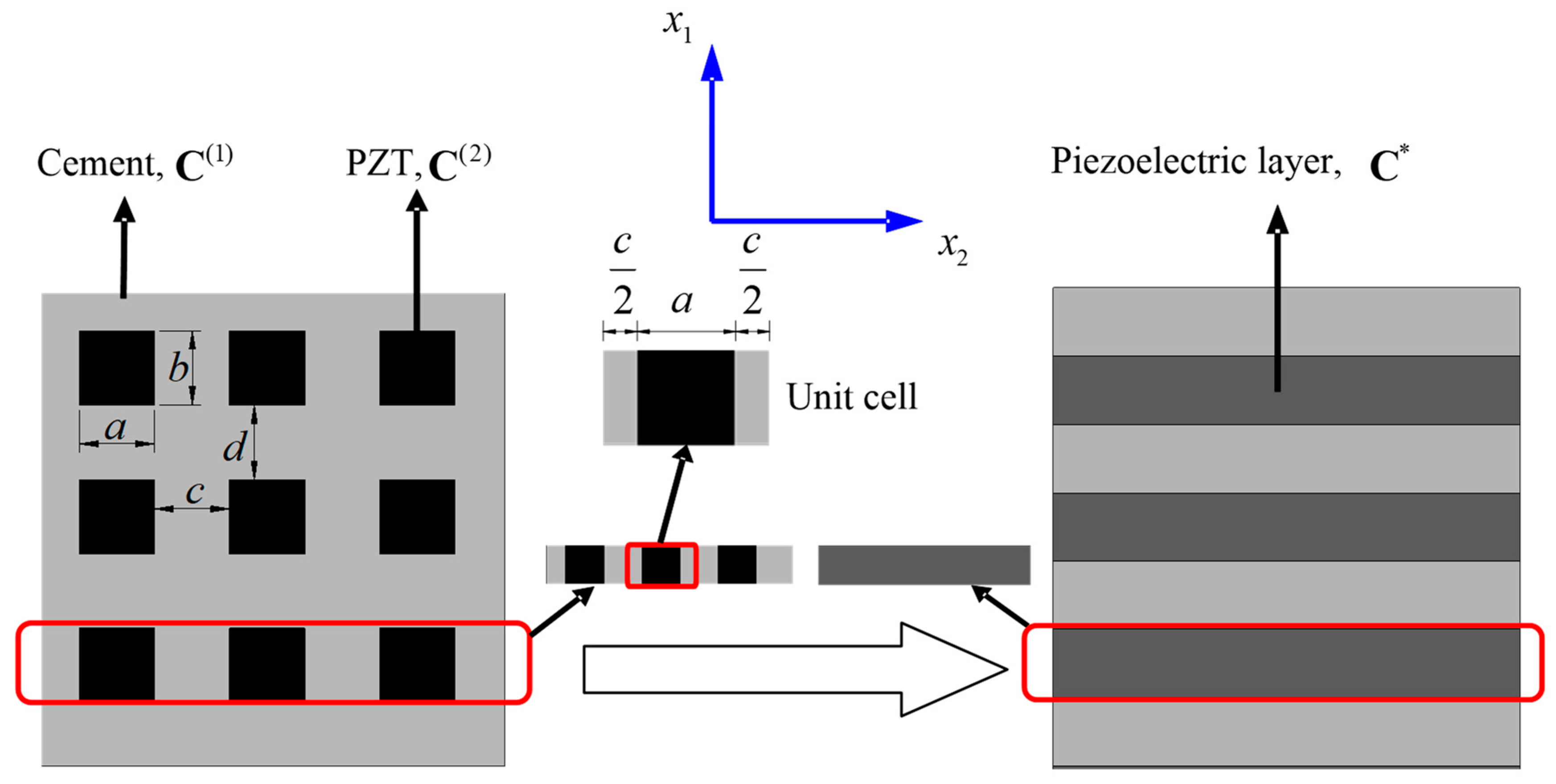
3.3. First Homogenization for the 1-3 Type Cement Based Piezoelectric Composite
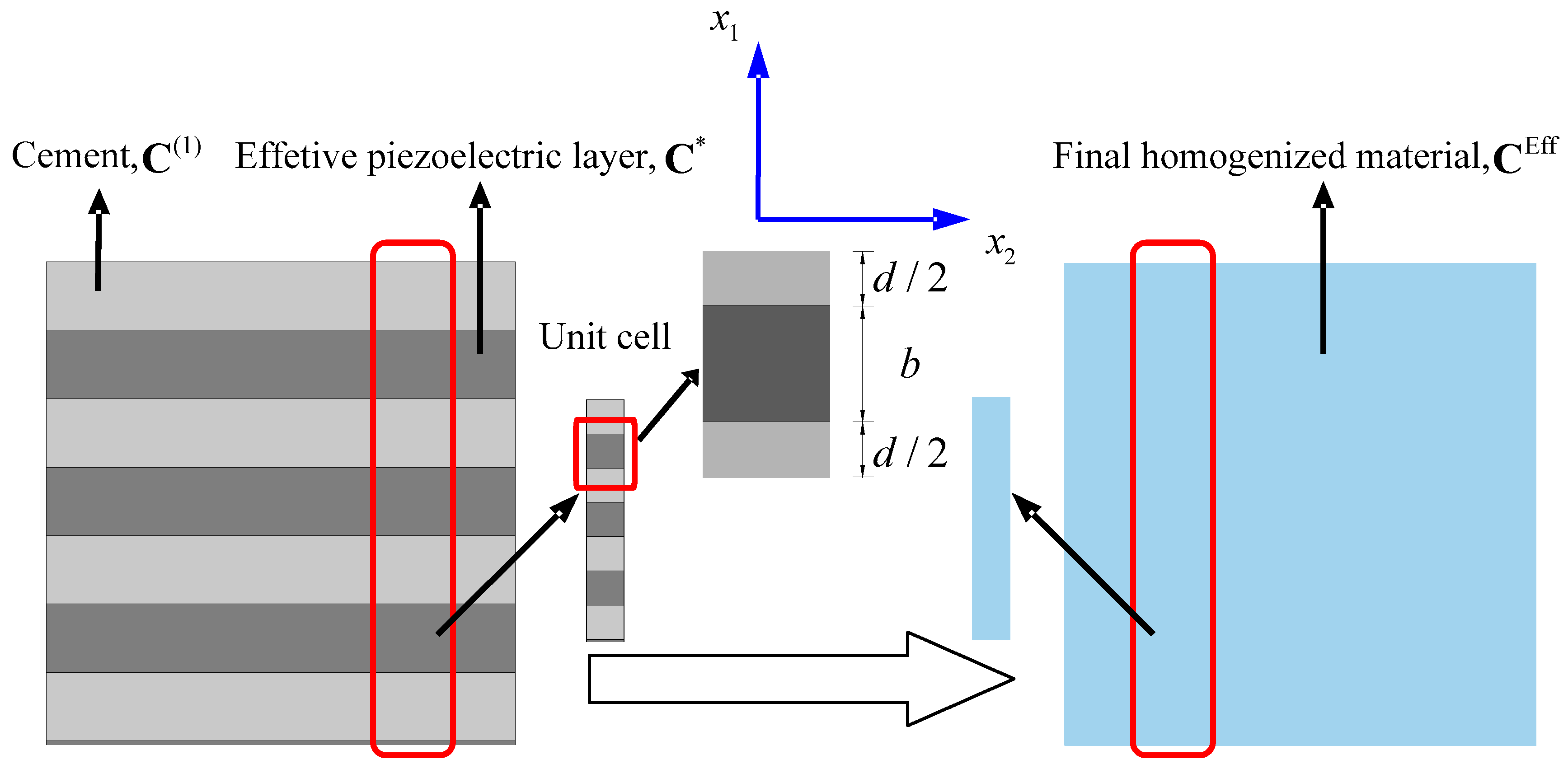
3.4. Second Homogenization for the 1-3 Type Cement Based Piezoelectric Composite
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A. Non-Zero Components of the Generalized Elasticity Tensors after the First Homogenization
Appendix B. Non-Zero Components of the Generalized Elasticity Tensors after the Second Homogenization
References
- Farrar, C.R.; Worden, K. An introduction to structural health monitoring. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2007, 365, 303–315. [Google Scholar] [CrossRef] [PubMed]
- Huston, D.R.; Fuhr, P.L.; Ambrose, T.P.; Barker, D.A. Intelligent civil structures-activities in vermont. Smart Mater. Struct. 1994, 3, 129–139. [Google Scholar] [CrossRef]
- Ansari, F.; Maji, A.; Leung, C. Intelligent Civil Engineering Materials and Structure; American Society of Civil Engineers: New York, NY, USA, 1997. [Google Scholar]
- Aizawa, S.; Kakizawa, T.; Higasino, M. Case studies of smart materials for civil structures. Smart Mater. Struct. 1998, 7, 617–626. [Google Scholar] [CrossRef]
- Ko, J.; Ni, Y. Technology developments in structural health monitoring of large-scale bridges. Eng. Struct. 2005, 27, 1715–1725. [Google Scholar] [CrossRef]
- Park, S.H.; Yun, C.B.; Roh, Y.R.; Lee, J.J. Health monitoring of steel structures using impedance of thickness modes at PZT patches. Smart. Struct. Syst. 2005, 1, 339–353. [Google Scholar] [CrossRef]
- Park, S.H.; Ahmad, S.; Yun, C.B.; Roh, Y.R. Experimental and analytical studies for impedance-based smart health monitoring of concrete structures. Key Eng. Mater. 2006, 321, 170–173. [Google Scholar] [CrossRef]
- Bhalla, S.K.; Moharana, S. Modelling of piezo-bond structure system for structural health monitoring using emi technique. Key Eng. Mater. 2013, 569, 1234–1240. [Google Scholar] [CrossRef]
- Zhu, X.; Hao, H.; Fan, K. Detection of delamination between steel bars and concrete using embedded piezoelectric actuators/sensors. J. Civ. Struct. Health Monit. 2013, 3, 105–115. [Google Scholar] [CrossRef]
- Wu, H.P.; Li, L.; Chai, G.Z.; Song, F.; Kitamura, T. Three-dimensional thermal weight function method for the interface crack problems in bimaterial structures under a transient thermal loading. J. Therm. Stresses 2016, 39, 371–385. [Google Scholar] [CrossRef]
- Li, Z.J.; Zhang, D.; Wu, K.R. Cement-based 0-3 piezoelectric composites. J. Am. Ceram. Soc. 2002, 85, 305–313. [Google Scholar] [CrossRef]
- Huang, S.F.; Chang, J.; Xu, R.H.; Liu, F.T.; Lu, L.C.; Ye, Z.M.; Cheng, X. Piezoelectric properties of 0-3 PZT/sulfoaluminate cement composites. Smart Mater. Struct. 2004, 13, 270–274. [Google Scholar] [CrossRef]
- Chaipanich, A.; Jaitanong, N.; Tunkasiri, T. Fabrication and properties of PZT-ordinary Portland cement composites. Mater. Lett. 2007, 61, 5206–5208. [Google Scholar] [CrossRef]
- Chaipanich, A.; Jaitanong, N.; Yimnirun, R. Effect of compressive stress on the ferroelectric hysteresis behavior in 0-3 PZT-cement composites. Mater. Lett. 2010, 64, 562–564. [Google Scholar] [CrossRef]
- Li, Z.J.; Zhang, D.; Wu, K.R. Cement matrix 2-2 piezoelectric composite—Part 1. Sensory effect. Mater. Struct. 2001, 34, 506–512. [Google Scholar]
- Zhang, D.; Li, Z.J.; Wu, K.R. 2-2 piezoelectric cement matrix composite: Part II. Actuator effect. Cem. Concr. Res. 2002, 32, 825–830. [Google Scholar] [CrossRef]
- Han, R.; Shi, Z.F.; Mo, Y.L. Static analysis of 2-2 cement-based piezoelectric composites. Arch. Appl. Mech. 2011, 81, 839–851. [Google Scholar] [CrossRef]
- Zhang, T.T.; Shi, Z.F. Exact analysis of the dynamic properties of a 2-2 cement based piezoelectric transducer. Smart Mater. Struct. 2011, 20. [Google Scholar] [CrossRef]
- Shi, Z.F.; Wang, J.J. Dynamic analysis of 2-2 cement-based piezoelectric transducers. J. Intell. Mater. Syst. Struct. 2013, 24, 99–107. [Google Scholar] [CrossRef]
- Lam, K.H.; Chan, H.L.W. Piezoelectric cement-based 1-3 composites. Appl. Phys. A Mater. Sci. Process. 2005, 81, 1451–1454. [Google Scholar] [CrossRef]
- Li, Z.J.; Huang, S.F.; Qin, L.; Chen, X. An investigation on 1-3 cement based piezoelectric composites. Smart Mater. Struct. 2007, 16, 999–1005. [Google Scholar] [CrossRef]
- Qin, L.; Huang, S.F.; Cheng, X.; Lu, Y.Y.; Li, Z.J. The application of 1-3 cement-based piezoelectric transducers in active and passive health monitoring for concrete structures. Smart Mater. Struct. 2009, 18. [Google Scholar] [CrossRef]
- Cheng, X.; Xu, D.; Lu, L.; Huang, S.F.; Jiang, M.H. Performance investigation of 1-3 piezoelectric ceramic cement composite. Mater. Chem. Phys. 2010, 121, 63–69. [Google Scholar] [CrossRef]
- Potong, R.; Rianyoi, R.; Jaitanong, N.; Yimnirun, R.; Chaipanich, A. Ferroelectric hysteresis behavior and dielectric properties of 1-3 lead zirconate titanate-cement composites. Ceram. Int. 2012, 38, 267–270. [Google Scholar] [CrossRef]
- Potong, R.; Rianyoi, R.; Ngamjarurojana, A.; Chaipanich, A. Dielectric and piezoelectric properties of 1-3 non-lead barium zirconate titanate-portland cement composites. Ceram. Int. 2013, 39, 53–57. [Google Scholar] [CrossRef]
- Li, Z.J.; Dong, B.Q.; Zhang, D. Influence of polarization on properties of 0-3 cementbased PZT composites. Cem. Concr. Compos. 2005, 27, 27–32. [Google Scholar] [CrossRef]
- Xing, F.; Dong, B.Q.; Li, Z.J. Dielectric, piezoelectric, and elastic properties of cement based piezoelectric ceramic composites. J. Am. Ceram. Soc. 2008, 91, 2886–2891. [Google Scholar] [CrossRef]
- Eshelby, J.D. The determination of the elastic field of an ellipsoidal inclusion, and related problems. Proc. R. Soc. A Math. Phys. Eng. Sci. 1957, 241, 376–396. [Google Scholar] [CrossRef]
- Dunn, M.L.; Taya, M. Micromechanics predictions of the effective electroelastic moduli of piezoelectric composites. Int. J. Solids Struct. 1993, 30, 161–175. [Google Scholar]
- Mori, T.; Tanaka, K. Average stress in matrix and average elastic energy of materials with misfitting inclusions. Acta Metall. 1973, 21, 571–574. [Google Scholar] [CrossRef]
- McLaughlin, R. A study of the differential scheme for composite materials. Int. J. Eng. Sci. 1977, 15, 237–244. [Google Scholar] [CrossRef]
- Hill, R. A self-consistent mechanics of composite materials. J. Mech. Phys. Solids 1965, 13, 213–222. [Google Scholar] [CrossRef]
- Christensen, R.M.; Lo, K.H. Solutions for effective shear properties in three phase sphere and cylinder models. J. Mech. Phys. Solids 1979, 27, 315–330. [Google Scholar] [CrossRef]
- Odegard, G.M. Constitutive Modeling of Piezoelectric Polymer Composites. Acta Mater. 2004, 52, 5315–5330. [Google Scholar] [CrossRef]
- Grekov, A.A.; Kramarov, S.O.; Kuprienko, A.A. Anomalous behavior of the two-phase lamellar piezoelectric texture. Ferroelectrics 1987, 76, 43–48. [Google Scholar] [CrossRef]
- Benveniste, Y.; Dvorak, G.J. Uniform fields and universal relations in piezoelectric composites. J. Mech. Phys. Solids 1992, 40, 1295–1312. [Google Scholar] [CrossRef]
- Ray, M.C.; Batra, R.C. Effective properties of carbon nanotube and piezoelectric fiber reinforced hybrid smart composites. J. Appl. Mech. ASME 2009, 76. [Google Scholar] [CrossRef]
- Bennett, J.; Hayward, G. Design of 1-3 piezocomposite hydrophones using finite element analysis. IEEE Trans. Ultrason. Ferroelect. Freq. Contr. 1997, 44, 565–574. [Google Scholar] [CrossRef]
- Poizat, C.; Sester, M. Effective properties of composites with embedded piezoelectric fibres. Comput. Mater. Sci. 1999, 16, 89–97. [Google Scholar] [CrossRef]
- Pettermann, H.E.; Suresh, S. A comprehensive unit cell model: A study of coupled effects in piezoelectric 1-3 composites. Int. J. Solids Struct. 2000, 37, 5447–5464. [Google Scholar] [CrossRef]
- Kar-Gupta, R.; Venkatesh, T.A. Electromechanical response of 1-3 piezoelectric composites: Effect of poling characteristics. J. Appl. Phys. 2005, 98. [Google Scholar] [CrossRef]
- Benssousan, A.; Lions, J.L.; Papanicoulau, G. Asymptotic Analysis for Periodic Structures; North Holland Publishing Company: Amsterdam, The Netherlands, 1978. [Google Scholar]
- Bravo-Castillero, J.; Guinovart-Díaz, R.; Sabina, F.J.; Rodríguez-Ramos, R. Closed-form expressions for the effective coefficients of a fiber-reinforced composite with transversely isotropic constituents—II. Piezoelectric and square symmetry. Mech. Mater. 2001, 33, 237–248. [Google Scholar] [CrossRef]
- Sabina, F.J.; Rodríguez-Ramos, R.; Bravo-Castillero, J.; Guinovart-Díaz, R. Closed-form expressions for the effective coefficients of a fibre-reinforced composite with transversely isotropic constituents. II: Piezoelectric and hexagonal symmetry. J. Mech. Phys. Solids 2001, 49, 1463–1479. [Google Scholar] [CrossRef]
- Barnett, D.M.; Lothe, J. Dislocations and line charges in anisotropic piezoelectric insulators. Phys. Status Solidi B Basic Res. 1975, 67, 105–111. [Google Scholar] [CrossRef]
- Babuška, I. Solution of interface problems by homogenization. I. SIAM J. Math. Anal. 1976, 7, 603–634. [Google Scholar] [CrossRef]
- Babuška, I. Solution of interface problems by homogenization. II. SIAM J. Math. Anal. 1976, 7, 635–645. [Google Scholar] [CrossRef]
- Qin, Q.; Yang, Q. Macro-Micro Theory on Multifield Coupling Behavior of Heterogeneous Materials; Higher Education Press & Springer: Beijing, China, 2008. [Google Scholar]
- Bravo-Castillero, J.; Otero, J.A.; Rodríguez-Ramos, R. Asymptotic homogenization of laminated piezocomposite materials. Int. J. Solids Struct. 1998, 35, 527–541. [Google Scholar] [CrossRef]
- Fu, C.Q.; Wang, Z.; Jin, X.Y.; Zhu, X.Y.; Zhu, J. Effective piezoelectric coefficients of cement-based 2-2 type piezoelectric composites based on a multiscale homogenization model. Compos. Struct. 2018, 194, 170–177. [Google Scholar] [CrossRef]
- Guinovart-Diaz, R.; Bravo-Castillero, J.; Rodríguez-Ramos, R.; Guinovart-Díaz, R. Closed-form expressions for the effective coefficients of fibre-reinforced composite with transversely isotropic constituents. I: Elastic and hexagonal symmetry. J. Mech. Phys. Solids 2001, 49, 1445–1462. [Google Scholar] [CrossRef]
- Fang, Z.B.; Sun, W.; Tzeng, J.T. Asymptotic homogenization and numerical implementation to predict the effective mechanical properties for electromagnetic composite conductor. J. Compos. Mater. 2004, 38, 1371–1385. [Google Scholar] [CrossRef]
- Banno, H. Theoretical equations for dielectric and piezoelectric properties of ferroelectric composites based on modified cubes model. Jpn. J. Appl. Phys. 1985, 24, 445–447. [Google Scholar] [CrossRef]
- Li, L.L.; Zhang, S.J.; Xu, Z.; Geng, X.C.; Wen, F.; Luo, J.; Shrout, T.R. Hydrostatic piezoelectric properties of [011] poled Pb(Mg1/3Nb2/3)O3-PbTiO3 single crystals and 2-2 lamellar composites. Appl. Phys. Lett. 2014, 104. [Google Scholar] [CrossRef] [PubMed]



| Material | Elastic Constant | Piezoelectric Coefficient | Relative Dielectric Constant c | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| PZT-5H | 127 | 80.2 | 84.7 | 117 | 23 | −6.6 | 23.2 | 17 | 3131 | 3400 | 4551 | 5366 |
| Cement d | 15.4 | 3.9 | 3.9 | 15.4 | 5.8 | 0 | 0 | 0 | 19 | 19 | 19 | 19 |
| Effective Piezoelectric Parameters | Sample Number | ||||
|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | ||
| Size parameter (mm) | a | 1.24 | 0.95 | 2 | 3 |
| b | 1.24 | 0.95 | 2 | 3 | |
| c | 0.74 | 0.49 | 0.65 | 0.75 | |
| d | 0.74 | 0.49 | 0.65 | 0.75 | |
| h e | 6.67 | 6.47 | 5.3 | 5.8 | |
| Volume fraction of PZT f | 0.39 | 0.435 | 0.57 | 0.64 | |
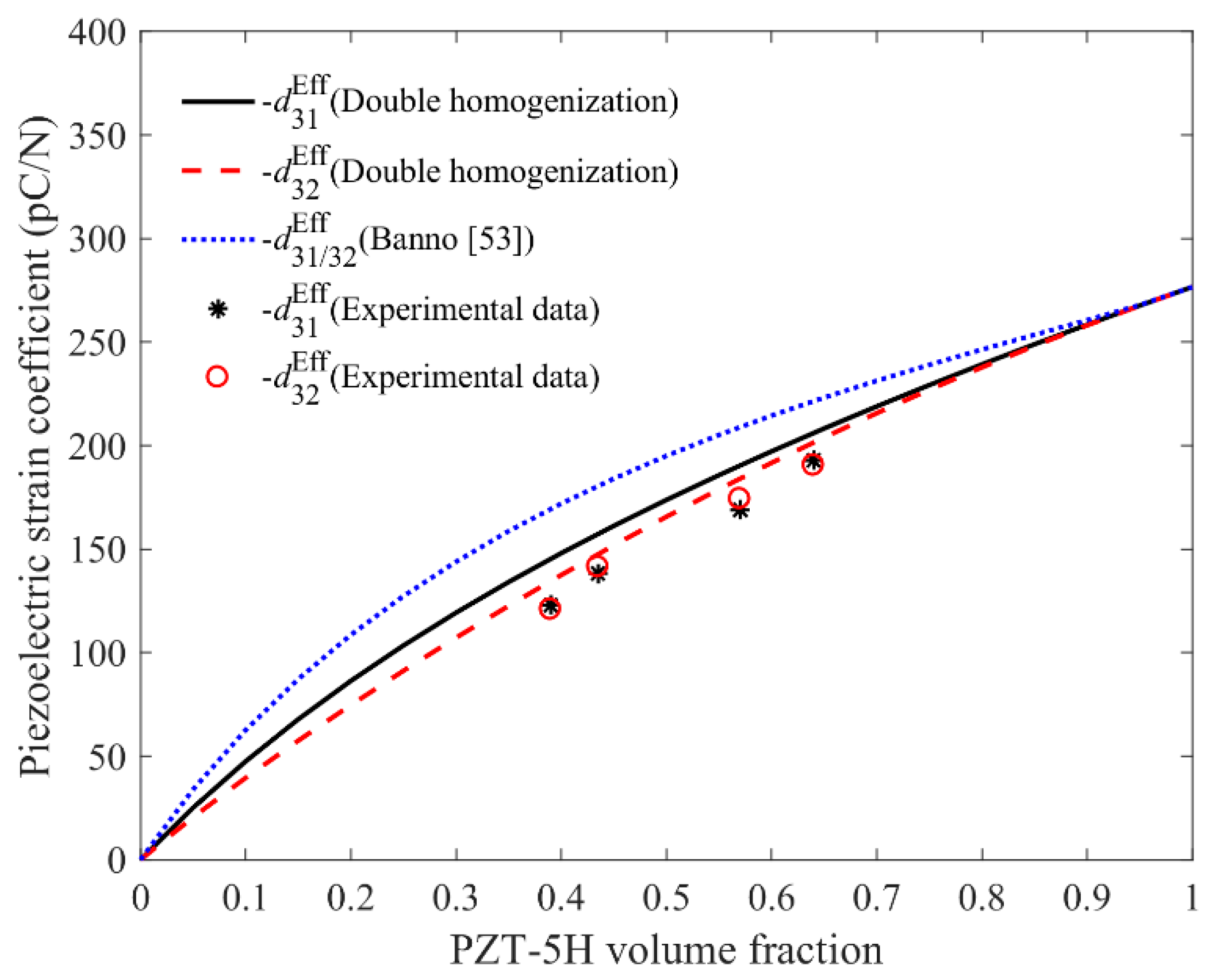
(pC/N) | Present model | 145 | 157 | 190 | 206 |
| Experimental | 123 | 138 | 169 | 193 | |
| Relative error f | 0.15 | 0.12 | 0.11 | 0.06 | |
(pC/N) | Present model | 135 | 148 | 184 | 202 |
| Experimental | 121 | 141 | 174 | 190 | |
| Relative error | 0.10 | 0.04 | 0.05 | 0.06 | |
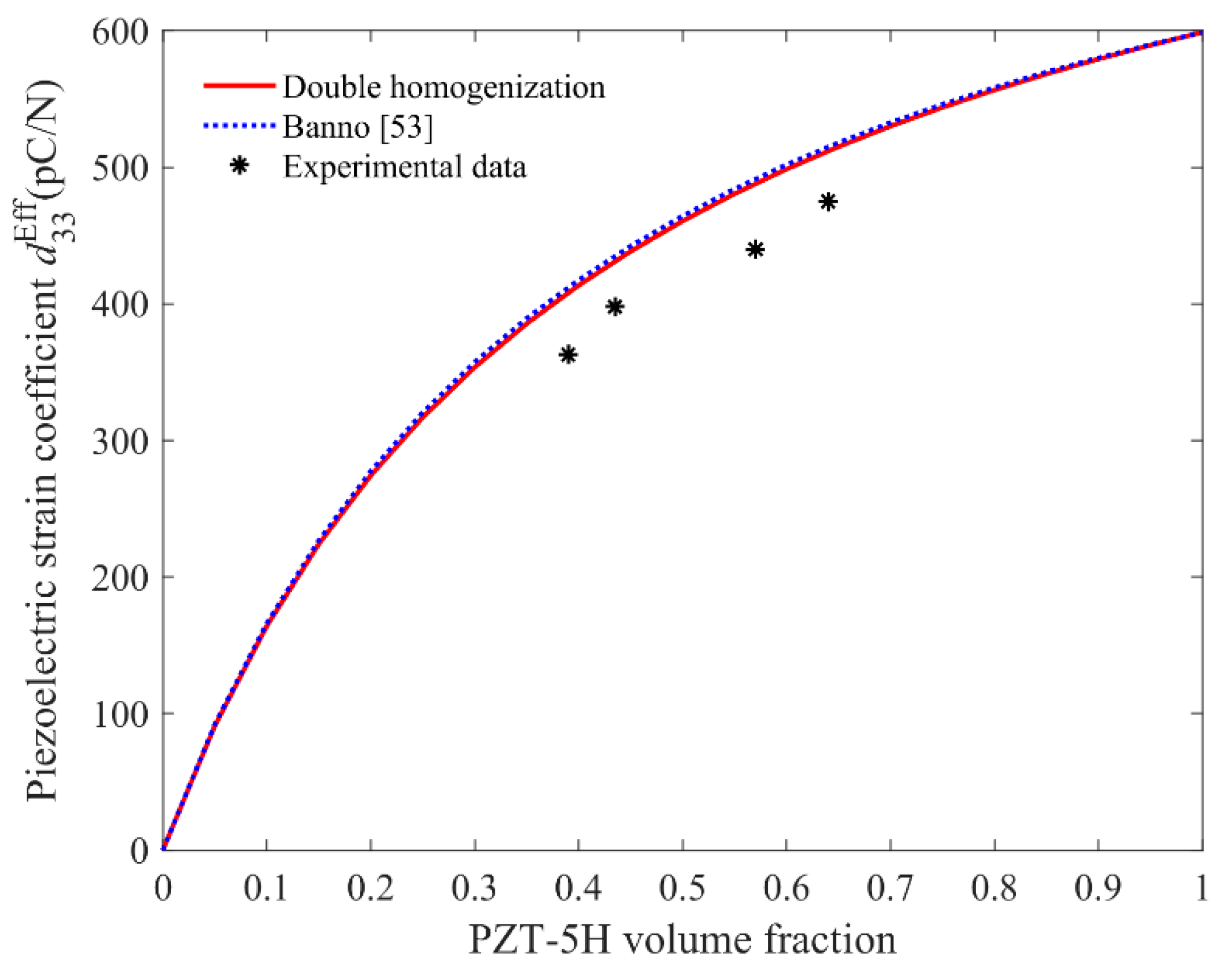
(pC/N) | Present model | 408 | 431 | 488 | 512 |
| Experimental | 363 | 398 | 440 | 475 | |
| Relative error | 0.11 | 0.08 | 0.10 | 0.07 | |
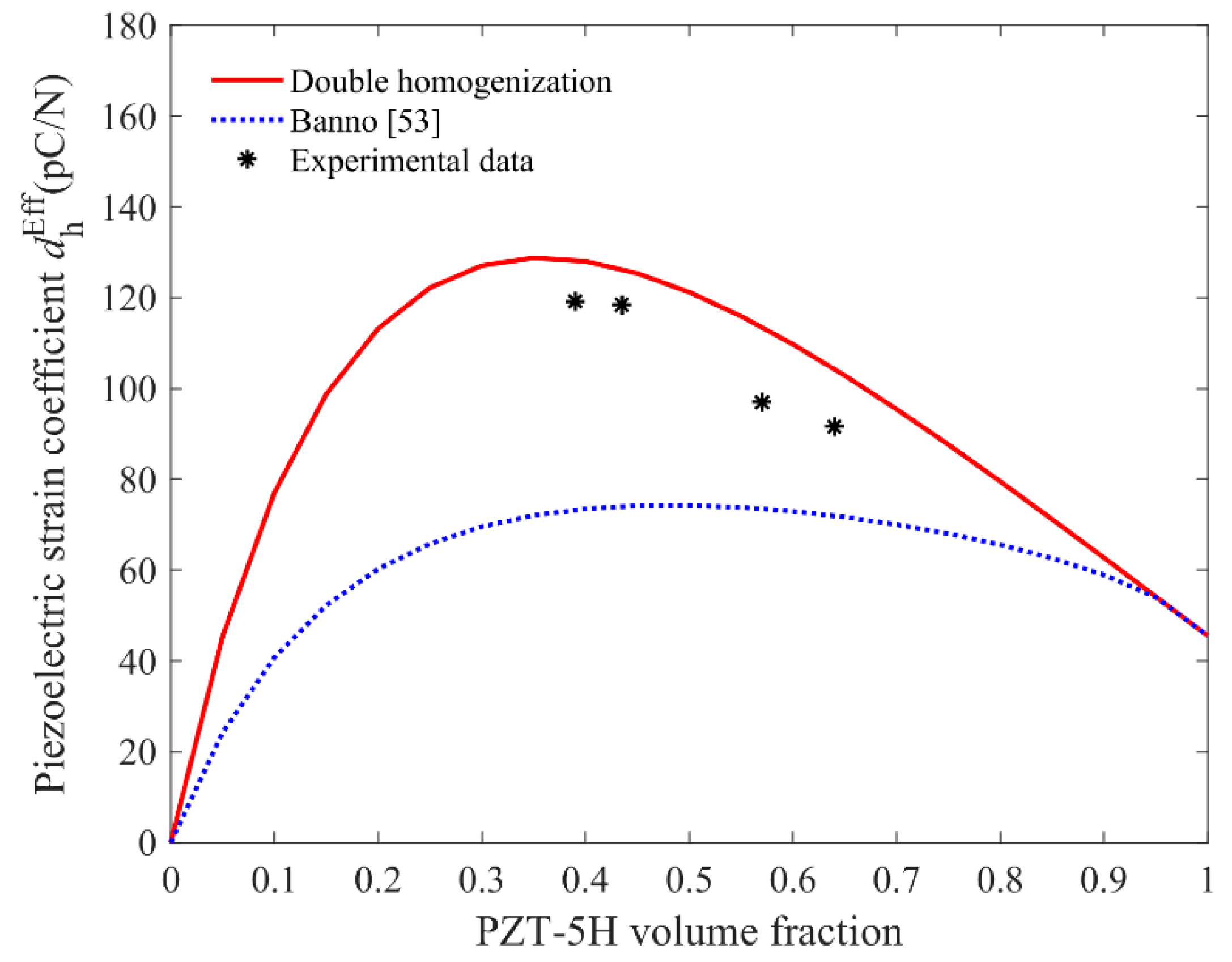
(pC/N) | Present model | 128 | 126 | 114 | 104 |
| Experimental | 119 | 119 | 97 | 92 | |
| Relative error | 0.07 | 0.06 | 0.15 | 0.12 | |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, J.; Wang, Z.; Zhu, X.; Yang, B.; Fu, C. Theoretical and Experimental Study on the Effective Piezoelectric Properties of 1-3 Type Cement-Based Piezoelectric Composites. Materials 2018, 11, 1698. https://doi.org/10.3390/ma11091698
Zhu J, Wang Z, Zhu X, Yang B, Fu C. Theoretical and Experimental Study on the Effective Piezoelectric Properties of 1-3 Type Cement-Based Piezoelectric Composites. Materials. 2018; 11(9):1698. https://doi.org/10.3390/ma11091698
Chicago/Turabian StyleZhu, Jun, Zhi Wang, Xingyi Zhu, Bo Yang, and Chuanqing Fu. 2018. "Theoretical and Experimental Study on the Effective Piezoelectric Properties of 1-3 Type Cement-Based Piezoelectric Composites" Materials 11, no. 9: 1698. https://doi.org/10.3390/ma11091698
APA StyleZhu, J., Wang, Z., Zhu, X., Yang, B., & Fu, C. (2018). Theoretical and Experimental Study on the Effective Piezoelectric Properties of 1-3 Type Cement-Based Piezoelectric Composites. Materials, 11(9), 1698. https://doi.org/10.3390/ma11091698






