First-Principles Studies on the Structural and Electronic Properties of As Clusters

Abstract

Based on the genetic algorithm (GA) incorporated with density functional theory (DFT) calculations, the structural and electronic properties of neutral and charged arsenic clusters As_n (n = 2–24) are investigated. The size-dependent physical properties of neutral clusters, such as the binding energy, HOMO-LUMO gap, and second difference of cluster energies, are discussed. The supercluster structures based on the As₈ unit and As₂ bridge are found to be dominant for the larger cluster As_n (n ≥ 8). Furthermore, the possible geometric structures of As₂₈, As₃₈, and As₁₈₀ are predicted based on the growth pattern.

1. Introduction

In recent years, due to the fast development of nanotechnology, people are more interested in atomic clusters, which are composed of several to thousands of atoms, molecules, or ions through a physical or chemical bonding force [1]. Clusters can also be regarded as the transitional forms between atoms and bulk, and their fundamental properties depend vitally on the cluster size. Therefore, it is quite meaningful to study the structural and electronic properties of clusters using theoretical research, identifying their potential capacities for numerous applications. Arsenic has been widely applied in many fields such as semiconductors, optoelectronics, and biopharmaceutics [2,3,4,5,6,7,8,9,10,11]. Besides, vanadium doped phosphorus clusters, and pure and doped arsenic clusters have received a large amount of attention from both experimental and theoretical fields in recent years [12,13,14,15,16,17,18,19,20,21,22,23].

Experimentally, the study of As_n clusters has focused on small-sized clusters with n ≤ 5 [12,13,14,15,16,17]. For example, Wang et al. [12] utilized high-resolution photoelectron spectroscopy to study the electronic vibration and spin orbit of As₄. Bennett et al. [13] have measured the appearance potentials and ion translational energies for the As₁, As₂, and As₃ ions formed by the dissociative resonance capture of As₄. Lippa et al. [14] have probed the electron affinities of As_n (n = 1–5) using photoelectron spectra in 1998. Brumbach and Rosenblatt [15] have investigated the vibrational modes of As₄ with Raman spectroscopy. Yonezo [16] designed a high-temperature nozzle assembly for gas-electron diffraction to determine the structure of As₄. Jeffrey et al. [17] measured the ionization potentials (IPs) for As_n (n = 1–5) using gas-phase charge-transfer reactions. No experimental data are available for As_n with n ≥ 6 right now.

In the theoretical aspect, Zhao et al. [18] and Bai et al. [21] studied the structures, thermochemistry, and electron affinities of As_n (n = 1–16) and their anions. Their results showed that the even-numbered neutral As_n species are more stable than the odd-numbered clusters, but the even-numbered anionic As_n species are less stable than the odd-numbered species. Liang et al. [19] probed the electronic structure and property of neutral and charged arsenic clusters As_n^(+1,0,−1) (n = 2–8). At the B3LYP/6-311+G(d) theoretical level, Guo [20] investigated the geometries and energies for neutral and charged As_n (n = 2–15) clusters, and reported their relative stability, ionization potential, and electron affinity. Baruah et al. [23] using a generalized gradient approximation (GGA) to explore the geometry, vibrational modes, and polarizabilities, as well as the infrared and Raman spectra of fullerene-like arsenic cages with n = 4, 8, 20, 28, 32, 36, and 60. Zhao et al. [22] investigated the structures and electronic properties of As_n clusters with even-numbered As_n (n = 6–28) using density functional theory (DFT) with the Perdew–Burke–Ernzerhof functional and a doubled numerical basis set with d-polarization functions(PBE/DND) scheme and found that the supercluster structures based on As₄, As₆, and As₈ units, and the As₂ bridge were dominant for the larger As_n with n ≥ 14.

Although many theoretical works have been performed on the As_n clusters, all the results are always most of the artificial speculation studies to investigate the structure of clusters in certain symmetries. In this work, we have performed a genetic algorithm (GA) incorporated with density functional theory (DFT) calculations to explore the structures and electronic properties of As_n (n = 2–24) neutral and charged clusters. After we have determined the growth pattern of As_n clusters, the possible geometric structures of As₂₈, As₃₈, and As₁₈₀ are predicted based on the growth pattern. We also discussed the size-dependent physical properties of neutral clusters such as the binding energy, HOMO-LUMO gap and second difference of cluster energies.

2. Computational Methodology

In order to search the global minimum structures of As_n clusters, we combined a genetic algorithm (GA) simulation with local optimization at the Dmol³ level [24,25,26,27,28,29]. The fundamental aim in GA is to divide the potential-energy surface (PES) into a number of regions and find the locally stable isomers in each region. In the GA program, we generated 15 As_n (n = 3–12) and 20 As_n (n = 13–24) initial populations to ensure that we could find the local minimum. Any population can be chosen as parents to generate their child cluster according to a crossover operation. In addition, there was a possibility of a 30% mutation rate for a single parent to produce child alone. The child cluster was optimized with Dmol³, and then compared with its parent in energy. The child with lower energy replaced its high-energy parent. The whole process of genetic algorithm with 2000 iterations was to ensure we got the lowest energy structure.

The optimization of As_n (n = 2–24) clusters was performed using DFT with the Perdew–Burke–Ernzerhof (PBE) [24] exchange-correlation functional and an all-electron basis set of the double-numerical-plus-d-polarization (DND) type, as implemented in the Dmol³ [25] package. A self-consistent field calculation kept the accuracy with an energy convergence for 10⁻⁶ a.u., and the forces for 2 × 10⁻³ a.u. There was no symmetry restriction for geometry optimization.

For each As_n (n = 2–24) cluster, we saved ten energetically lower isomer structures for further electronic structure calculations, which were performed using the Vienna Ab-initio Simulation Package (VASP) codes. The Kohn–Sham equations were solved variationally in a plane wave basis set using the projector-augmented-wave (PAW) method. The exchange-correlation energy was described by the functional of Perdew, Burke, and Ernzerhof (PBE) based on the generalized gradient approximation (GGA). The energy cutoff was set to be 400 eV and the vacuum space was set to be at least 14 Å to separate the interactions between the neighboring slabs. Only the Gamma k-point was used to sample the Brillouin zone for the geometry and electronic structure calculations. All structures were fully relaxed by Gaussian smearing and electronic structure calculations by tetrahedron smearing method until the convergence criteria (with the force less than 0.02 eV/Å and the energy less than 10⁻⁵ eV). Although each structure of As_n (n = 2–24) cluster was further optimized by VASP, the energy sequencing of each cluster was basically unchanged.

3. Results and Discussions

3.1. As_n (n = 2–8) Clusters

The bond length of an As₂ cluster (2a in Figure 1) is 2.103 Å through experimental measurement [30]. In our calculation the distance between the As atoms was 2.118 Å, which is closer to the experimental data compared with the 2.142 Å calculated using PBE/DND methods [22].

For the As₃ cluster, the energy of the structure with C_2v symmetry (3a in Figure 1) was the global minimum. It was an isosceles triangle structure with a top angle of 65.14° and side length of 2.325 Å. It was energetically lower than the linear chain structure with D∞h symmetry (3b in Figure 1), in which structure, the bond length was 2.204 Å.

The ground-state structure of As₄ with T_d symmetry (4a in Figure 1) was a regular tetrahedron, which was consistent with the previous reports [18,19,20]. Its energy was much lower than other isomorphic configurations. The energy of the rectangular structure with D_2h symmetry (4b in Figure 1) was 0.605 eV/atom higher than that of the ground state and the chain structure with C₂ symmetry (4c in Figure 1) was energetically higher than the rectangular structure.

The lowest energy structure of As₅ had C_2v symmetry (5a in Figure 1) and it may be considered as adding an atom in the cross section of a dihedral formed by four atoms. It had 0.07 eV/atom less energy than the rectangular pyramid structure with D_4h symmetry (5b in Figure 1) and 0.142 eV/atom less than the planar structure with D_5h symmetry (5c in Figure 1).

The trigonal prism with D_3h symmetry (6a in Figure 1) was the ground-state structure for As₆ and was only 0.005 eV/atom lower than the benzvalene type with C_2v symmetry (6b in Figure 1) and 0.117 eV/atom lower than the dihedral angle structure of six atoms with C_2v symmetry (6c in Figure 1). The side length was 2.522 Å and the edge length was 2.559 Å. Our ground state was consistent with the result calculated by B3LYP/6-311+G(d) [20] or PBE/DND methods [22]. However, the structure 6b in Figure 1 was found to be the lowest energy by Liang [19] using MP2(full)/g-31G(d) methods and Bai [21] using B3LYP/DZP++ methods. As the outer shell structure of As is 3s²3p³, we think our results are reasonable as the completely three-coordination structure (6a in Figure 1) must be more stable than the structure with two two-coordinations (6b in Figure 1).

In the case of As₇, the ground-state structure with C₂v symmetry (7a in Figure 1) could be derived from the trigonal prism of As₆ by edge-capping with an additional As atom. This low-energy structure is also predicted in Refs. [19,20,21]. Its energy was lower than the structure with Cs symmetry (7b in Figure 1) by 0.008 eV/atom and the structure with Cs symmetry (7c in Figure 1) by 0.053 eV/atom.

The wedge-like structure that looks like a cage with C_2v symmetry was obtained as the lowest energy structure for As₈, as seen from 8a in Figure 1. It was energetically lower than the structure with C_2v symmetry (8b in Figure 1) by 0.038 eV/atom and the structure with Cs symmetry (8c in Figure 1) by 0.048 eV/atom. We also checked the cage structure with O_h symmetry cut from the bulk phase reported by Baruah [23], and we found that it was 0.64 eV energy higher than our ground state structure.

After analysis of the ground structures of As_n (n = 2–8) clusters, we find that As₂ was a one-dimensional bridge, As₃ was a two-dimensional isosceles triangle and As₄ became a three-dimensional tetrahedron. When n was larger than 3, the two-dimensional cluster structure, such as 4b and 5c in Figure 1, sorts more and more backward energetically. We can conclude that the structure of small As clusters tends towards a three-dimensional cage structure and it was not stable for a 2-D planar structure.

3.2. As_n (n = 9–18) Clusters

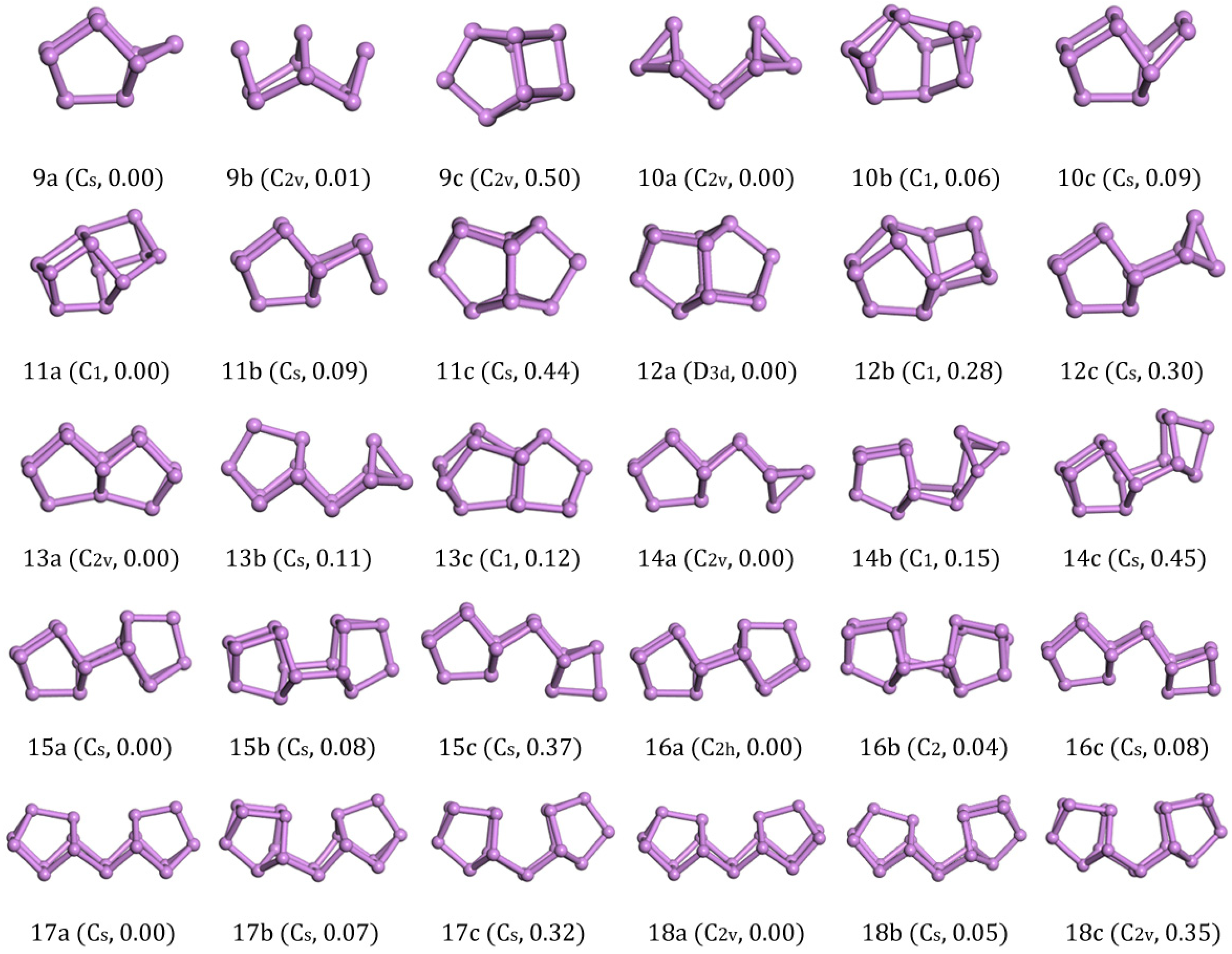
The ground-state structure of As₉ with C_s symmetry (9a in Figure 2) could be regarded as being derived from a cage-like As₈ structure by attaching an As atom at one side. The isomers (C_2v) with a higher symmetry (9b,c in Figure 2) were less stable based on our VASP calculations. It also hinted to us that the ground structure of As₈ might be a stable cluster with a magic number. From further calculations, we found that the cage-like As₈ structures unit served as the primary building unit for forming the As clusters with larger sizes.

The lowest-energy structure (C_2v) of As₁₀ consistd of the cage structure of 8a in Figure 1 that was edge-capped by each As atom. After relaxation, the upper bond broke to form two four-atom cages and an As₂ bridge. The structure 10b in Figure 2 was a new structure discovered by our GA global searching. The structure obtained by Zhao [22] is structure 10c in Figure 2, whose energy was higher than 10b in Figure 2 by 0.006 eV/atom and 10a in Figure 2 by 0.009 eV/atom.

The ground structure of As₁₁ was 11a in Figure 2 which was formed on the base of As₁₀ with an As atom added above the As₂ dimer and linked with an As₈ cage. It was more stable than the 11b in Figure 2 isomer with C_s symmetry by 0.008 eV/atom and 11c in Figure 2 isomer by 0.04 eV/atom with C_s symmetry in energy.

For As₁₂, the structure with D_3d symmetry was confirmed to be the lowest energy structure among all the structural candidates considered. The highly-symmetric structure was shaped of two As₈ cages that share a four-atom plane. From another point of view, the ground-state structure of As₁₂ was a layered structure of three layers of atoms (3 + 6 + 3). Such a nice structure was energetically lower than 12b in Figure 2 with C₁ symmetry by 0.023 eV/atom and 12c in Figure 2 with C_s symmetry by 0.025 eV/atom.

Viewing the ground-state structure of As₁₃ carefully, we also found two As₈ cages. Different from As₁₂, the two cages jointly owned a three-atom plane. It had 0.008 eV/atom less energy than the structural 13b in Figure 2 (C₁) and 0.009 eV/atom less than the structure 13c in Figure 2 (C_s).

As shown in the picture, As₁₄ with Cs symmetry could be considered to be composed of an As atom link to the three-atom plane on one side of the As₁₃ (13a in Figure 2). The less stable isomer 14b was a distorted structure of 14a, which was energetically higher by 0.011eV/atom. The 14c was a structure without an As₂ dimer bridge, it was linked by As₈ cage with an As₆ cage and had 0.032 eV/atom more energy than 14a in Figure 2.

The ground-state structure of As₁₅ with C_s symmetry was composed of two connected cages (As₈ and As₇). The other two candidates 15b in Figure 2 with C_s symmetry and 15c in Figure 2 with C_s symmetry were less stable than the ground-state structure by 0.005 eV/atom and 0.025 eV/atom in energy, respectively.

An upward As₈ cage and a downward As₈ cage connected to form a new structure as the ground state of As₁₆ (16a in Figure 2 with C_2h symmetry). It was more stable than the C₂-symmetry isomer 16b in Figure 2 and the C_s-symmetry isomer 16c in Figure 2 by 0.05 eV and 0.08 eV in energy. Although structural 16c in Figure 2 contained an As₈ cage and an As₂ bridge, the As₆ cage in the structure led to the overall energy as being higher than other two isomers.

The lowest energy structure of As₁₇ (17a in Figure 2) with C_s symmetry was built by As₈ and As₇ units with an As₂ bridge in the middle. The two C_s-symmetry isomorphic structure 17b,c in Figure 2 were also formed by the As₈ and As₇ units with different orientation connections.

The ground-state of As₁₈ (18a in Figure 2) with C_2v symmetry was formed by two identical As₈ units and an As₂ bridge in the middle. Our structure was exactly the same as that in Ref. [22]. Two slightly higher energy isomers (18b in Figure 2) with C_2h symmetry and 18c in Figure 2 with C_2v symmetry were combined by the same units as 18a in Figure 2 with different orientations, and they were energetically higher than 18a in Figure 2 by 0.05 eV and 0.35 eV energy. The calculations showed that the structure with As₈ unit and As₂ bridge in the middle was more stable than other cage structures. We also generated one As₁₈ structure cut from bulk phase and the energy was 3.17 eV higher than the ground state. So, we think the chain structure with As₈ units and an As₂ bridge is much more important for middle-sized As_n clusters.

3.3. As_n (n = 19–24) Clusters

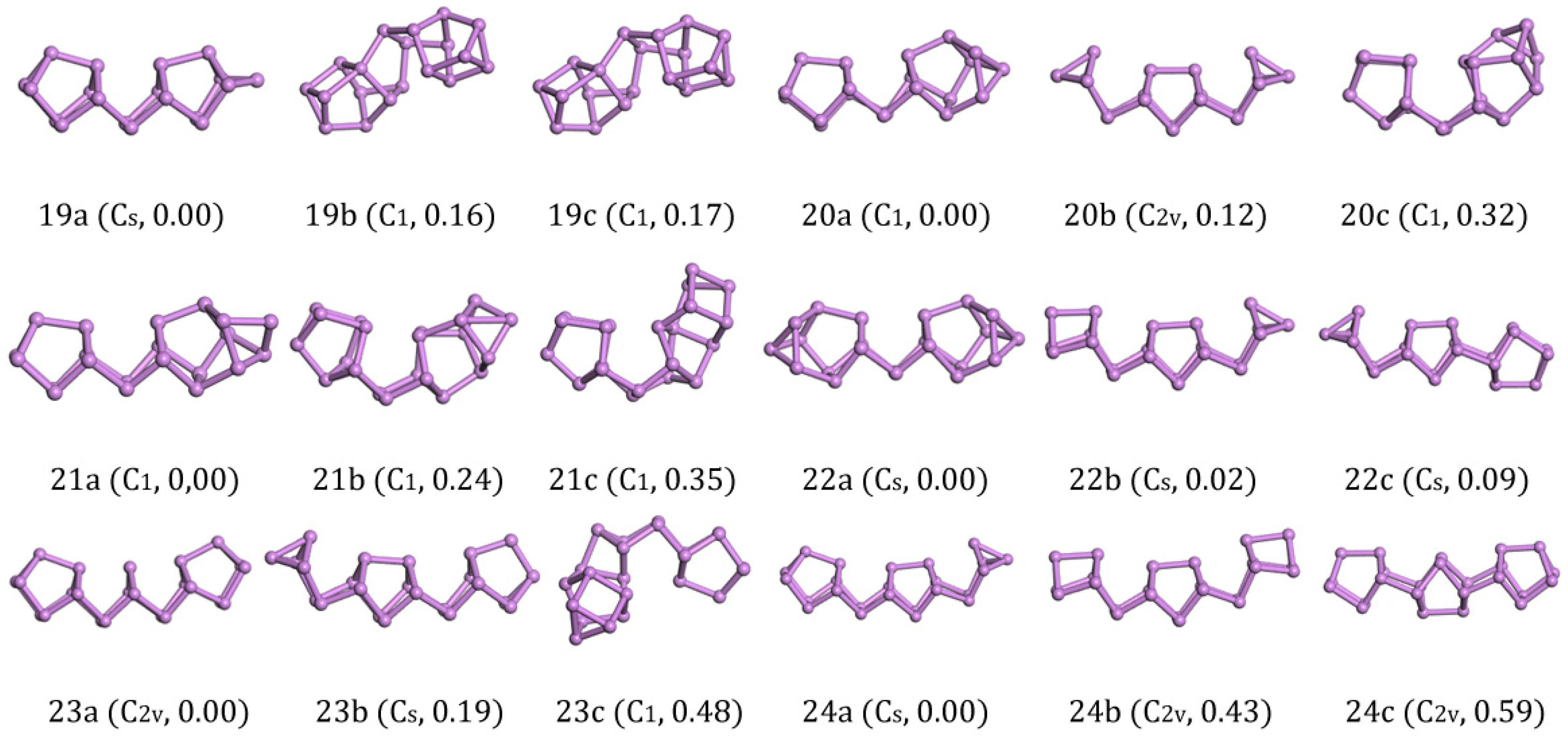
The ground-state structure of As₁₉ (19a in Figure 3) with C_s symmetry could be regarded as an As atom added into one side of As₁₈. Therefore, As₁₉ (19a in Figure 3) could be considered as the combination of As₈-As₂-As₈-As₁. The isomers of As₁₉ (19b,c in Figure 3) with only C₁ symmetry were both built up by two identical As₈ units and an As₃ bridge. Due to the distortion of the structures, they had 0.008 eV/atom and 0.009 eV/atom higher energy than 19a in Figure 3.

The ground-state structures of As₂₀ was predicted to be C₁ symmetry, as shown in 20a in Figure 3. It could be regarded as an As₈ cage link with an As₁₀ cage joined by an As₂ bridge. As₂₀ (20a in Figure 3) could be considered as the combination of As₈-As₂-As₁₀. Zhao [22] predicted the optimal combinations for the As₂₀ is super-clusters of As₄-As₂-As₈-As₂-As₄. The energy of As₂₀ (20a in Figure 3) we got based on the genetic algorithm was energetically lower than the structure 20b in Figure 3 with C_2v symmetry. A distorted structure 20c in Figure 3 originated from 20a in Figure 3 also appeared in our calculations. After the calculations with VASP, two isomers showed 0.006 eV/atom and 0.053 eV/atom higher energy than 20a in Figure 3. This result shows the super-clusters of As₄-As₂-As₈-As₂-As₄ did not have much of an advantage.

For As₂₁, the most stable structure (21a in Figure 3) could be regarded as an As atom link to the As₁₀ cage of As₂₀ (20a in Figure 3). Two other isomers (21b,c in Figure 3) were constituted by an As₈ cage and an irregular As₁₁ structure linked with an As₂ bridge. They were energetically higher than 21a in Figure 3 by 0.011 eV/atom and 0.017 eV/atom.

Rather than simply increasing the number of atoms on the edge, the ground-state structure of As₂₂ with C_s symmetry are formed with two symmetrical As₁₀ cages in the As₂₀ units and an As₂ bridge in the middle. The isomers were two kinds of super-clusters (22b in Figure 3, As₆-As₂-As₈-As₂-As₄ and 22c in Figure 3, As₄-As₂-As₈-As₈), and they were stretched by more units compared to the ground-state structures (22a in Figure 3). Although we could find stable As₈ and As₄ units in isomers, they each had 0.02 eV and 0.09 eV higher energy than 22a in Figure 3. According to previous findings, it can be found that the second lower energy As₁₀ cage 10b in Figure 2 will be favorable if the structure is composed by an As₈ unit connected with an As₂ bridge.

The ground-state structures of As₂₃ (23a in Figure 3) with C_2v symmetry seemed to be four As₈ cages linked to each other to share the three-atom plane, and the bottom edge of the middle two cages were broken. At the same time, we could also regard 23a in Figure 3 as a super-cluster of As₈-As₂-As₃-As₂-As₈. The other two candidates, 23b in Figure 3 with C_s symmetry and 23c in Figure 3 with C₁ symmetry, were less stable than the structure 23a in Figure 3 by 0.008 eV/atom and 0.021 eV/atom in energy, respectively.

The lowest energy structure of As₂₄ with C_s symmetry (24a in Figure 3) was built by three units (an As₄ cage and two identical As₈ cages), connecting the neighboring structure with an As₂ bridge. Zhao [22] considered that the optimal combinations of the super-clusters As₂₄ are As₆-As₂-As₈-As₂-As₆ (24b in Figure 3) with C_2v symmetry. DFT calculations show that the ground-state structure of As₂₄ we get based on the genetic algorithm weare the combinations of As₈-As₂-As₈-As₂-As₄, which was energetically lower than the structure 24b in Figure 3 by 0.018 eV/atom. Besides, we also gained another high symmetric structure with C_2v symmetry (24c in Figure 3) that was constituted by three As₈ units. However, its energy was 0.025 eV/atom higher than the ground state structure (24a in Figure 3). We could realize from this result that the lowest energy structures of larger As clusters not only have the combination of As₈ units but also needed an As₂ bridge in the middle of adjacent units.

3.4. As_n (n = 2–24) Charged Clusters

We also studied the ground structures of As_n (n = 2–24) charged clusters. Theoretically, it was easy to simulate a cationic or anionic cluster by adjusting the total electrons from the neutral cluster. From Figures S2 and S3, we could know the lowest energy structures of charged clusters (n = 2–4) were almost the same as with neutral cases. For As₈ clusters, 8a in Figure 1 structures were quite stable even in cationic or anionic cases and it was the cluster with the magic number. For other As_n (n < 16) clusters, the isomers changed the energy sequence as the system changed the electron numbers. It was interesting to find that the structures for As_n (15 < n < 24) clusters were quite stable whatever attachment of extra electron to the neutral or losing of an electron from the neutral cluster.

3.5. As_n (n = 28, 38, 40, 180) Clusters

With the increase of cluster size, it was more and more difficult to exhaust all possible local minimum structures. We tried to study the larger clusters As₂₈, As₃₈, and As₄₀ based on the above findings. The structural size evolution and electronic properties of arsenic clusters indicated that the clusters combined by an As₂ bridge and an As₈ cage had lower energy than their isomers and showed more stability in each local size-dependent range. Here we have to emphasize that As₄ and As₆ units were not dominant for the larger As_n cluster, which was different from Zhao’s result [22]. Furthermore, different sizes of fullerene cage structure isomers were also calculated to compare with our ground state structures in energy, and their energies were far more than units-linked one-dimensional structures. Given this understanding, we constructed As₂₈ as As₈-As₂-As₈-As₂-As₈ and As₃₈ as As₈-As₂-As₈-As₂-As₈-As₂-As₈ in all possible ways. 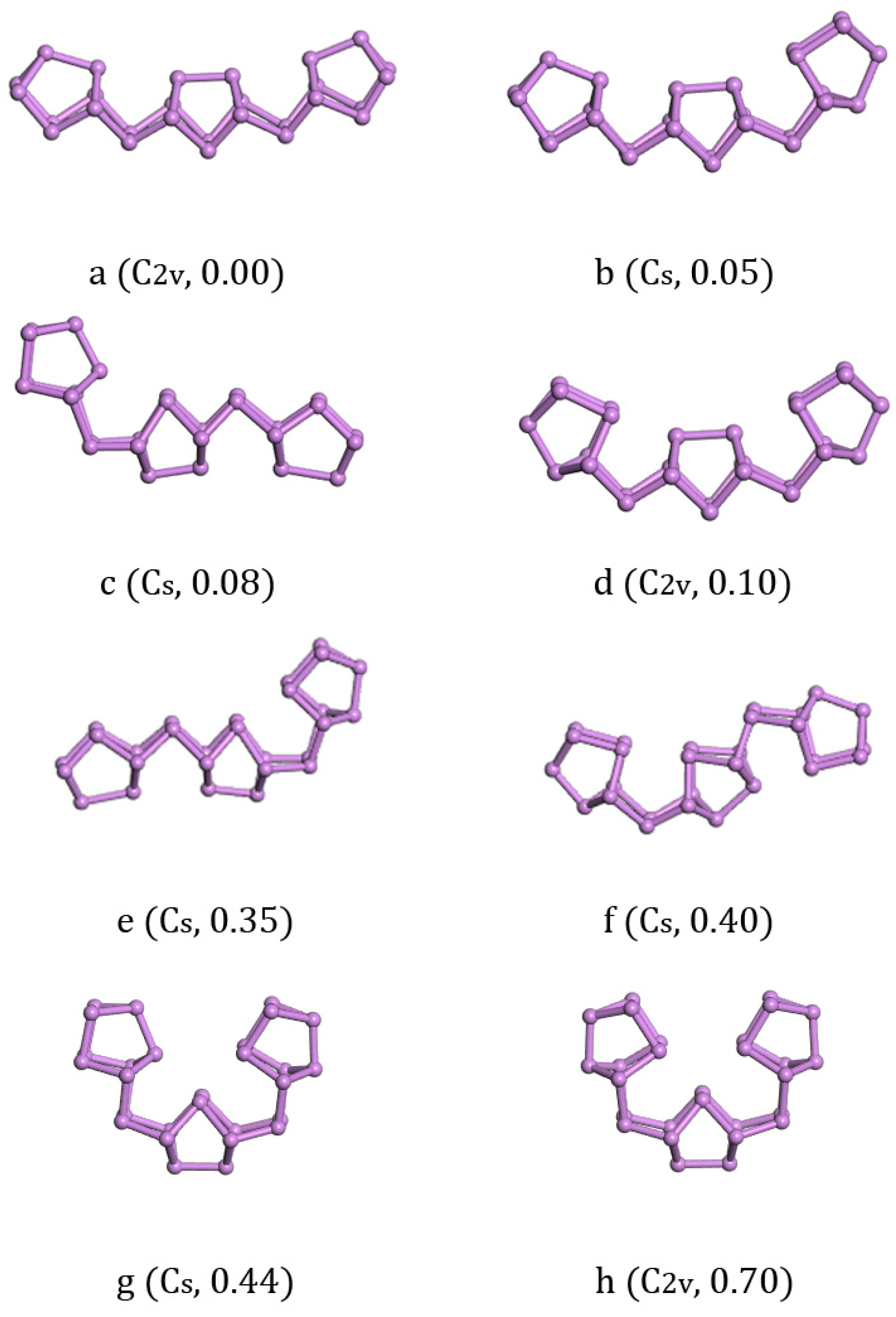The structures we calculated of As₂₈ are listed along with the increase of energy in Figure 4. The lowest energy structure of As₂₈ with C_2v symmetry (Figure 4a) had 0.05 eV less energy than the structure with C_s symmetry (Figure 4b) and 0.10 eV less than the structure with C_2v symmetry (Figure 4c). In addition to considering structure growth in the one-dimensional direction, we also calculated the longitudinal growth mode. Three isomers Figure 4c,e,f were all with C_s symmetry and energetically higher than the lowest energy structure a with 0.08 eV, 0.35 eV, and 0.40 eV, respectively. Besides, the other two semi-ring isomers Figure 4g,h had 0.44 eV and 0.70 eV energy higher than the ground state structure. Compared with the bulk truncated structure of As₂₈, the lowest energy structure of As₂₈ was 0.16 eV/atom lower.

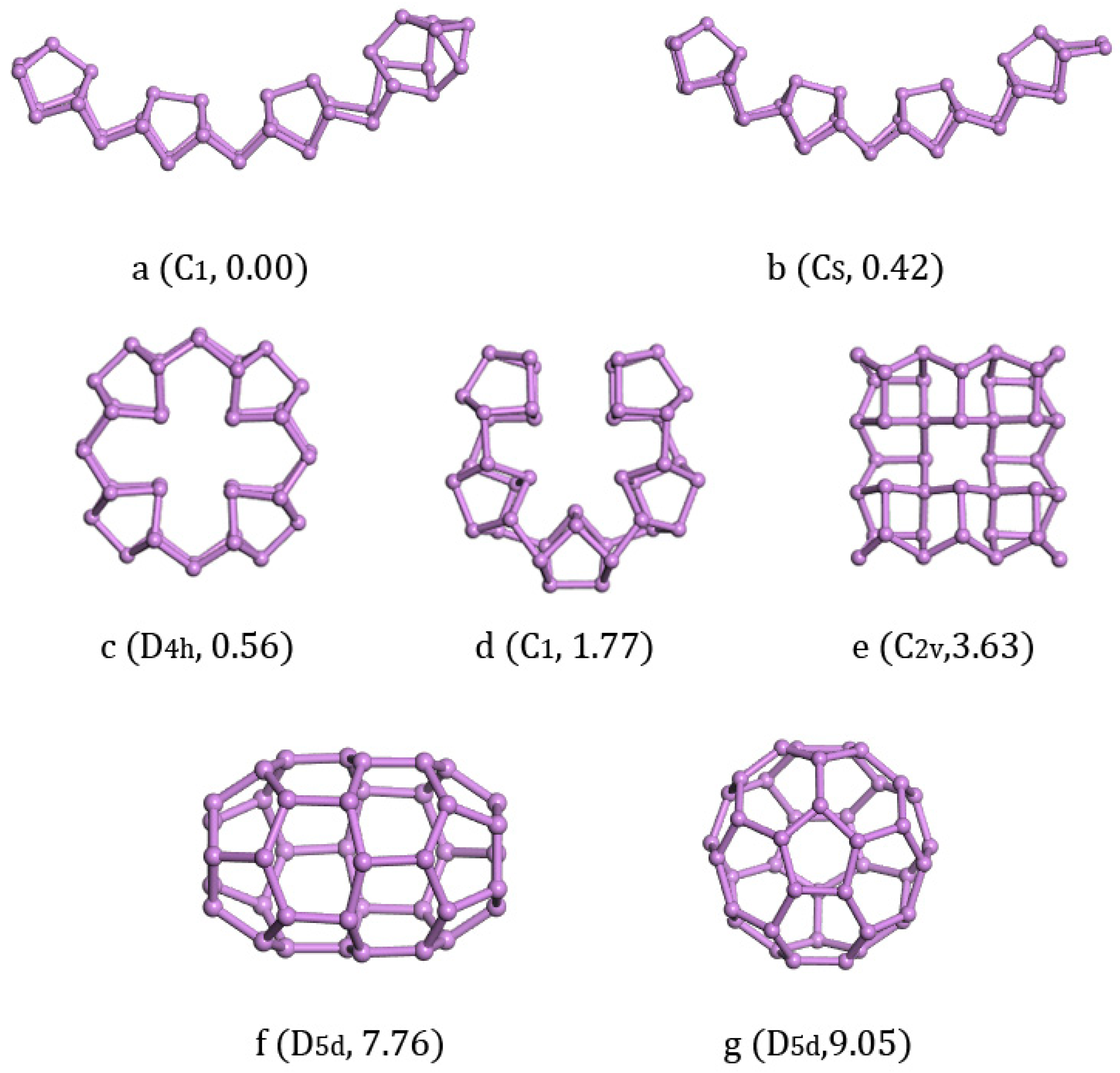
The lowest energy structure of As₃₈ with C_2v symmetry (Figure 5a) and its isomers are listed in Figure 5. We could find that the structure of Figure 5a was a continuation of As₈, As₁₈, and As₂₈ clusters, and all of them were constructed with As₈ cages in the same direction with As₂ bridges. The structural isomers Figure 5b with C_2v symmetry and Figure 5c with C_2h symmetry could be regarded as one-dimensional chain structures, the same as Figure 5a. Their respective energies were 0.10 eV and 0.22 eV higher than Figure 5a. The C_2v isomer Figure 5d could be regarded as a two-dimensional structure that has four As₈ cages held in four directions and all of them point to the center. The energy of this structure was highest in our calculation with 1.11 eV higher energy than a. Compared with the bulk truncated structure of As₃₈, the lowest energy structure of As₃₈ was 0.156 eV/atom lower. We could find that the structures did not change previous growth tendencies even with increasing the size of the clusters.

Considering that As₄₀ can form ring and fullerene cage structures, we also studied the structures of As₄₀. The structures we calculated of As₄₀ are listed along with the increase of energy in Figure 6. We found that the lowest energy structure of As₄₀ with C₁ symmetry (Figure 6a) and its isomers Figure 6b (0.42 eV energy higher) with C_s symmetry both were one-dimensional chain structures. Three isomers Figure 6c,d,e could all be regarded as two-dimensional structures and energetically higher than the lowest energy structure a with 0.014 eV/atom, 0.044 eV/atom, and 0.09 eV/atom, respectively. Seemingly stable three-dimensional fullerene cage isomers Figure 6f with D_5d symmetry and Figure 6g with D_5d symmetry were 0.194 eV/atom and 0.226 eV/atom higher in energy than the lowest energy structure.

Based on the finding above, we could construct the ring structure of an As₁₈₀ cluster based on the As₈ units and As₂ bridge, which is shown in Figure 7. The HOMO-LUMO gap of As₁₈₀ was 1.868 eV and the binding energy per atom was −2.901 eV.

3.6. Electronic Properties of As_n Clusters

The binding energy per atom for the ground states of As_n (n = 2–24) clusters are shown in Figure 8a. In the size range of n = 12–24, the binding energy increased smoothly with weak odd-even oscillation properties. This result can be related to the evolution of the ground-state from cage-like structure to cage-link structure at n = 12. Besides, the binding energy of As₈ was a peak value in the small size of n = 3–11, and this suggests the ground structure of As₈ would be a vital growth unit in larger structures. Our next calculation also proved the conjecture.

In Figure 8b, we present the energy gaps between the highest occupied molecular orbital (HOMO) and lowest unoccupied molecular orbital (LUMO) for the lowest-energy state of As_n (n = 2–24) clusters. As is known, the cluster with the larger energy gap is more stable and easier to prepare. As the largest energy gap of As₄ is 4.06 eV, it is the most prominent species in arsenic vapor, leading to a number of experimental and theoretical studies on As₄ clusters. Although the gaps of As_n clusters from n = 5–10 change smoothly, we also observe the gap of As₈ is highest locally, which points out that the stability of As₈ was higher than the neighboring cluster. The HOMO-LUMO gap was higher for As_n (n = 4, 6, 8, 12, 14, 16, 18, 20, 22, and 24) than their adjacent structures. The atoms in these even-numbered sequences were all three-coordination and eight-electron structure. The odd-numbered clusters were unable to achieve this condition, so they were less stable than the odd-numbered species.

In clusters physics, the second-order difference of cluster energy is a more sensitive datum to reflect the stability of clusters. We plotted the second-order difference of cluster energies defined by Δ²E = E(n + 1) + E(n − 1) − 2E(n). Figure 8c describes how the second-order differential energy changed with the increase of atom number and it shows good odd–even oscillation properties. The second-order difference of cluster energies of even-numbered clusters were all higher than their adjacent odd-numbered clusters. Therefore, we could draw the conclusion that even-numbered clusters were more stable than their neighboring odd-numbered clusters. Above all peaks for Δ²E, three local maximum peaks were found at n = 4, 8, and 18, where As_n (n = 4, 8, and 18) clusters were chemically stable.

4. Conclusions

We have adopted the genetic algorithm and all-electron DFT calculations to systematically study the structures and electronic properties of As_n (n = 2–24). The ground-state structures of As_n clusters change from two to three dimensional after n = 3. Arsenic clusters followed a structural growth pattern starting from n = 8 and the structures of As_n (n = 9–24) clusters could all be regarded as evolving from the ground state structure of an As₈ unit and an As₂ bridge. The binding energy of As_n (n = 2–24) clusters had a periodic step-like behavior, and the size-dependent HOMO-LUMO gap and second-order difference of cluster energies exhibited obvious even–odd alternations with several magic numbers. Based on the growth pattern concluded from small As_n clusters, the possible superstructures of As₂₈, As_38, As₄₀, and As₁₈₀ were discussed.