Design Method of Lightweight Metamaterials with Arbitrary Poisson’s Ratio
Abstract
1. Introduction
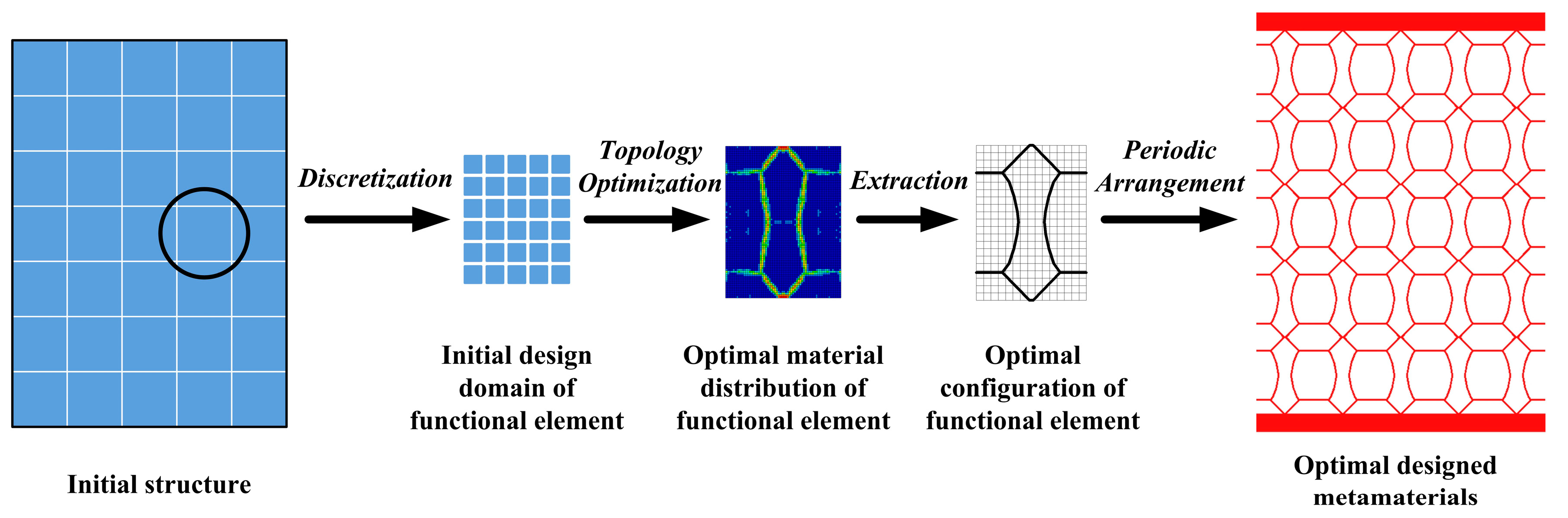
2. Functional Element Topology Optimization Method
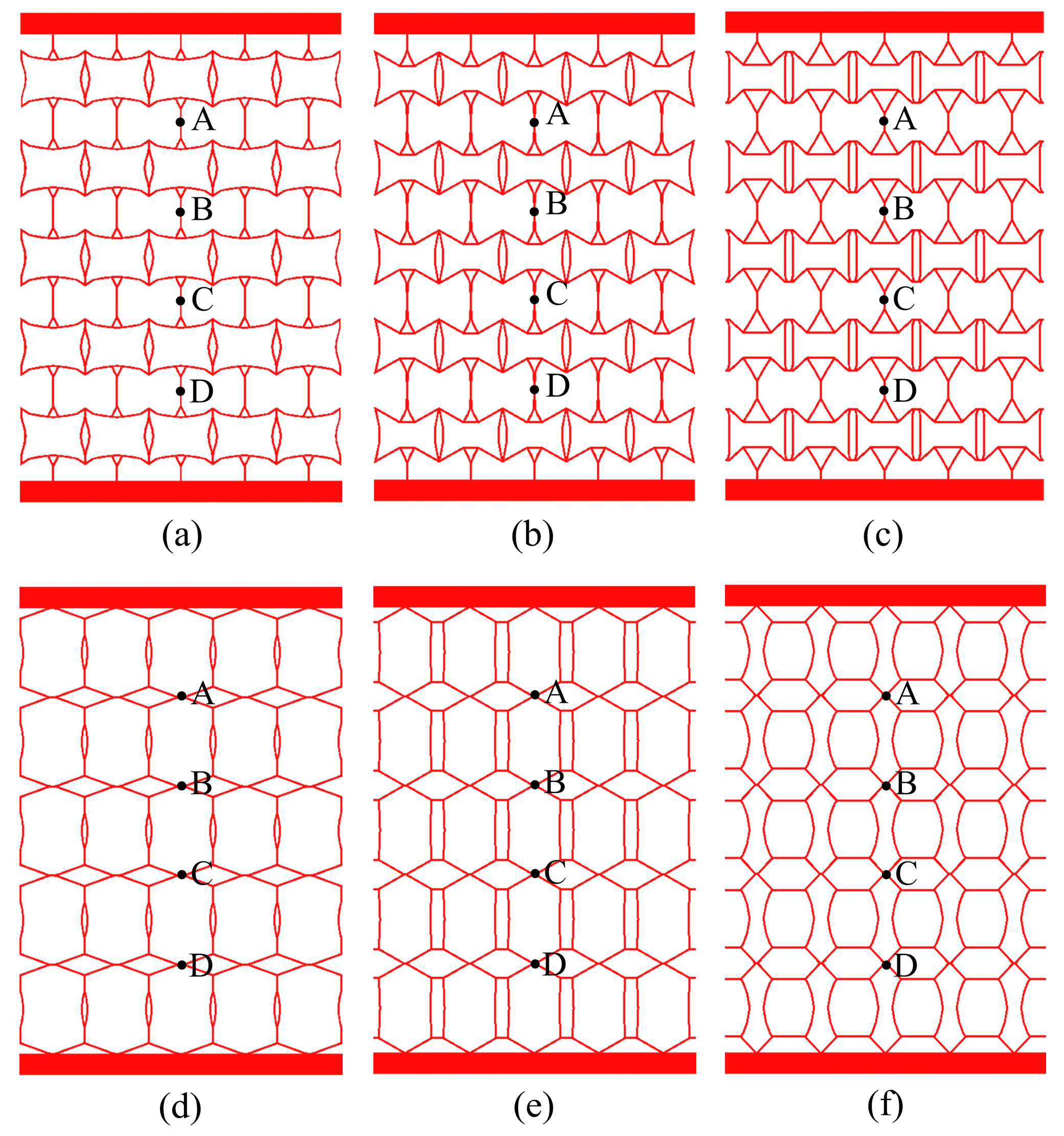
2.1. Definition of Functional Elements and Metamaterials
2.2. Macroscopic Poisson’s Ratio Effect of Functional Elements
3. Design Method of Lightweight Metamaterials
3.1. Topology Optimization Theory Used in FETO Method
3.2. Mathematical Model of Metamaterials Lightweight Design
3.3. Optimization Solutions of Lightweight Metamaterials
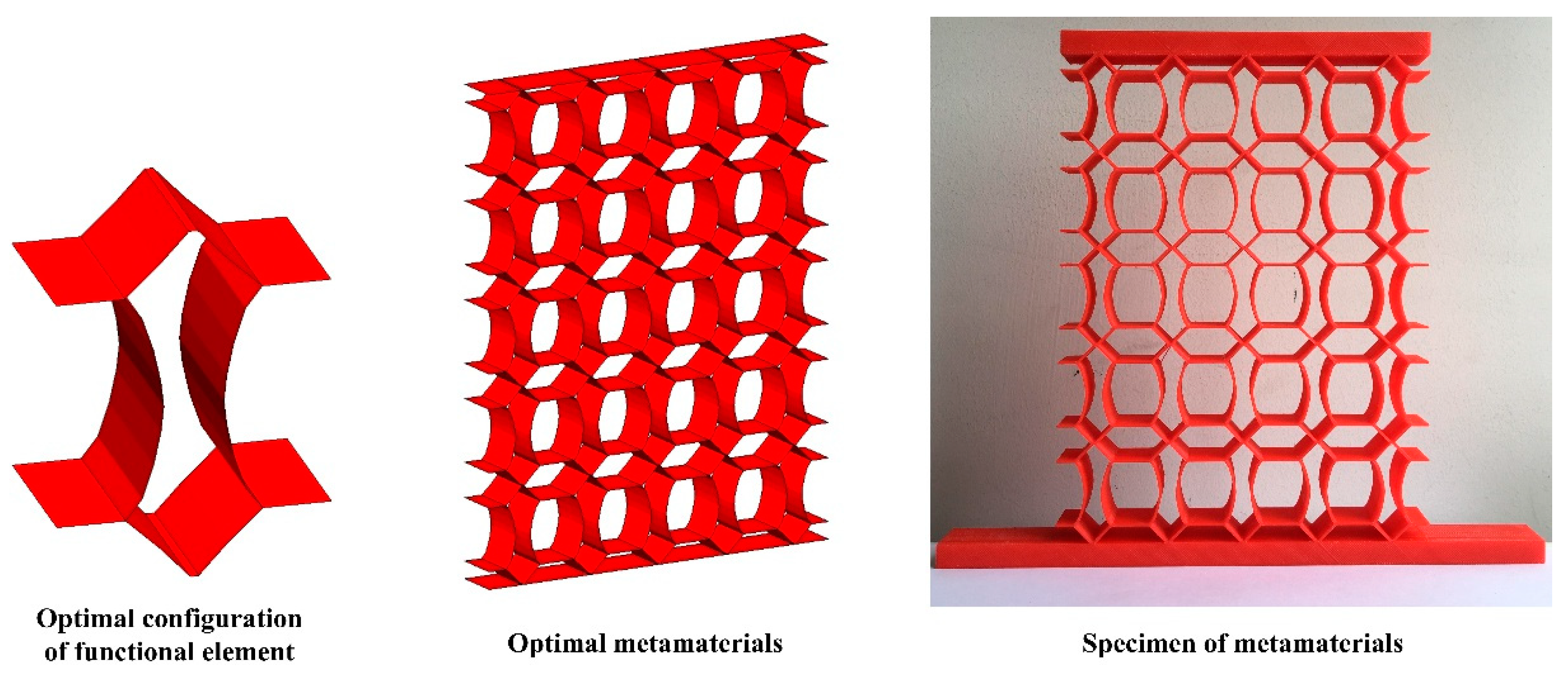
3.4. Extraction of the Optimal Configurations
3.5. Metamaterials Formation Based on Periodic Arrangement of Functional Elements
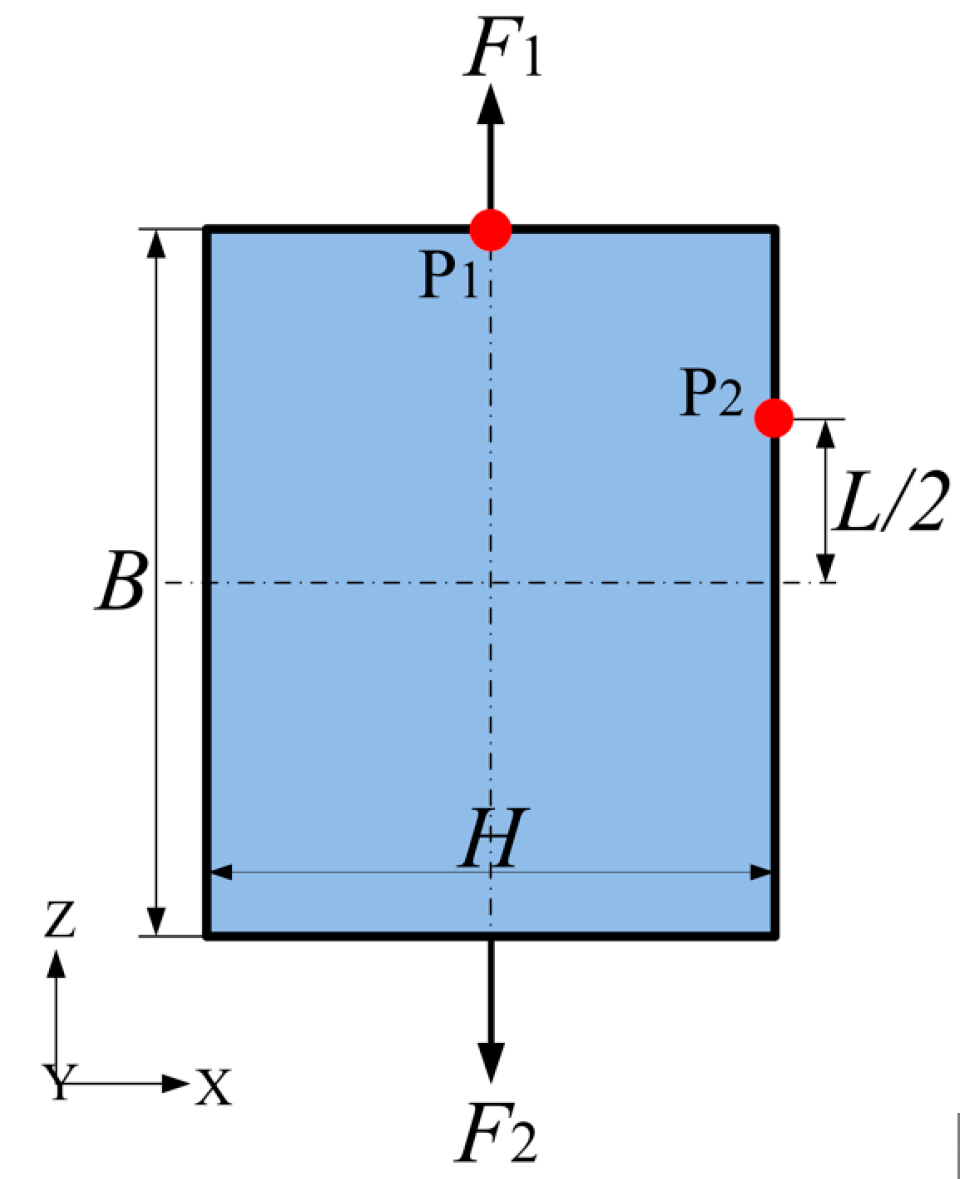
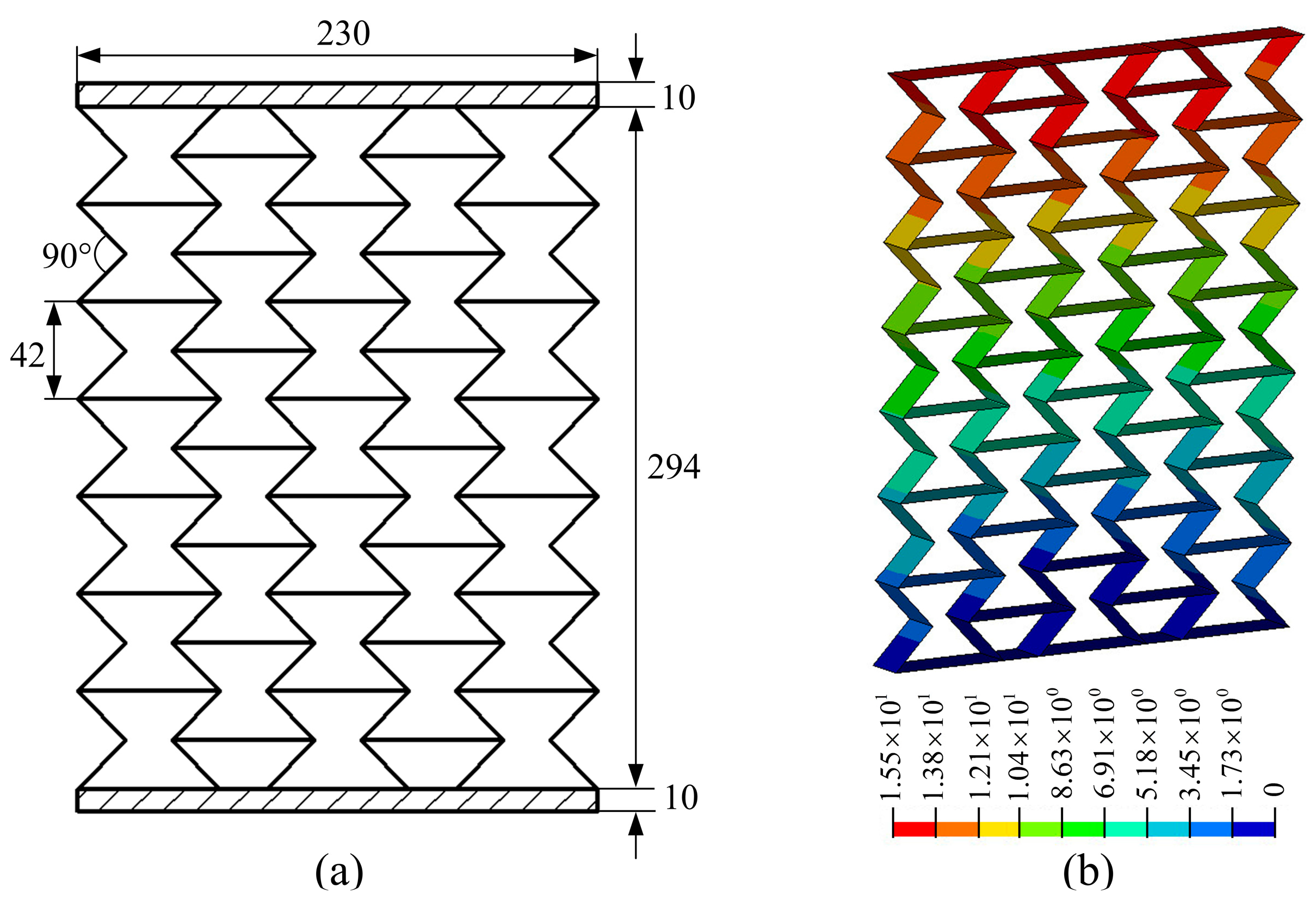
4. Evaluation Method of Static Properties of Metamaterials
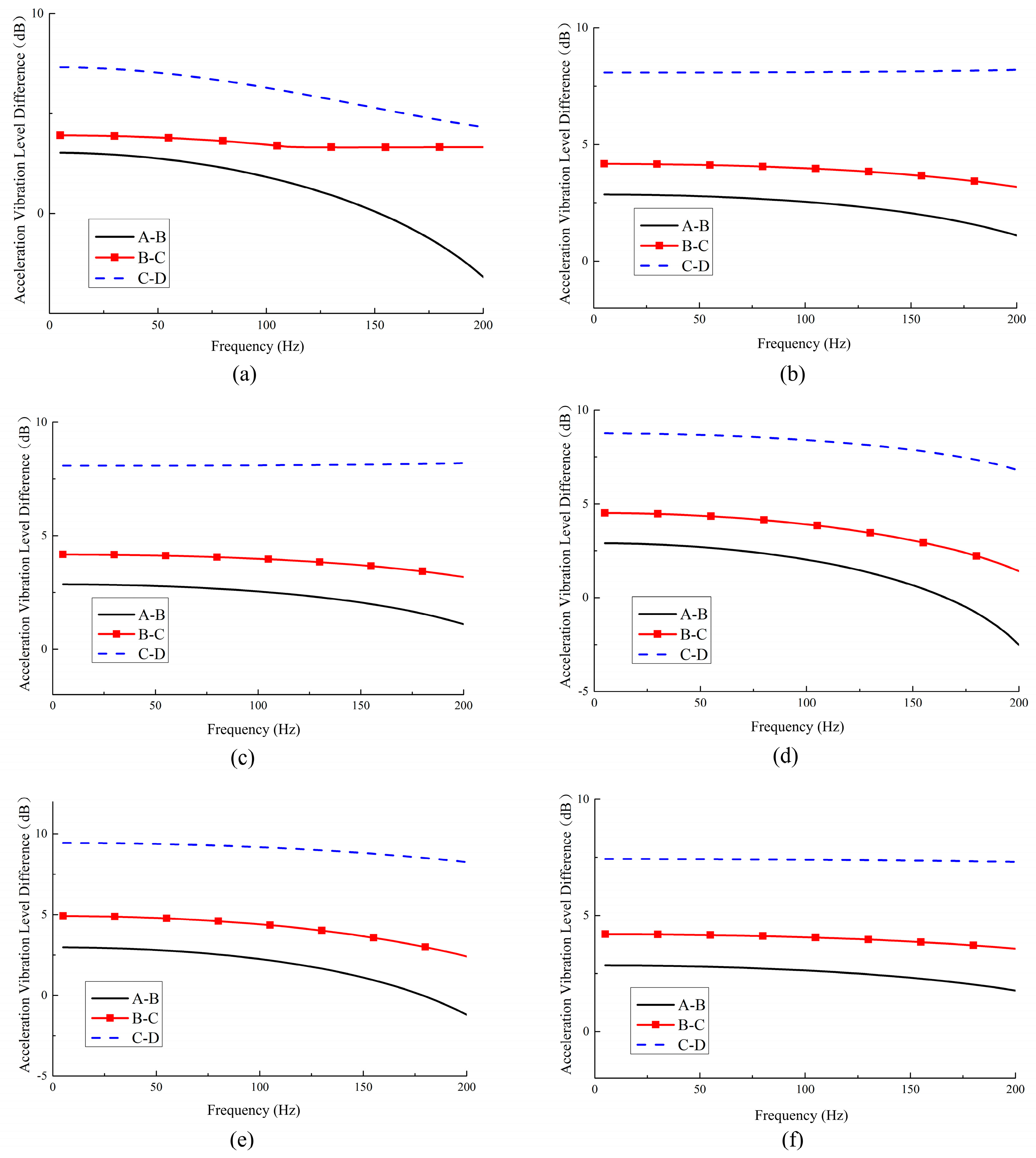
5. Evaluation Method of Vibration Reduction of Metamaterials
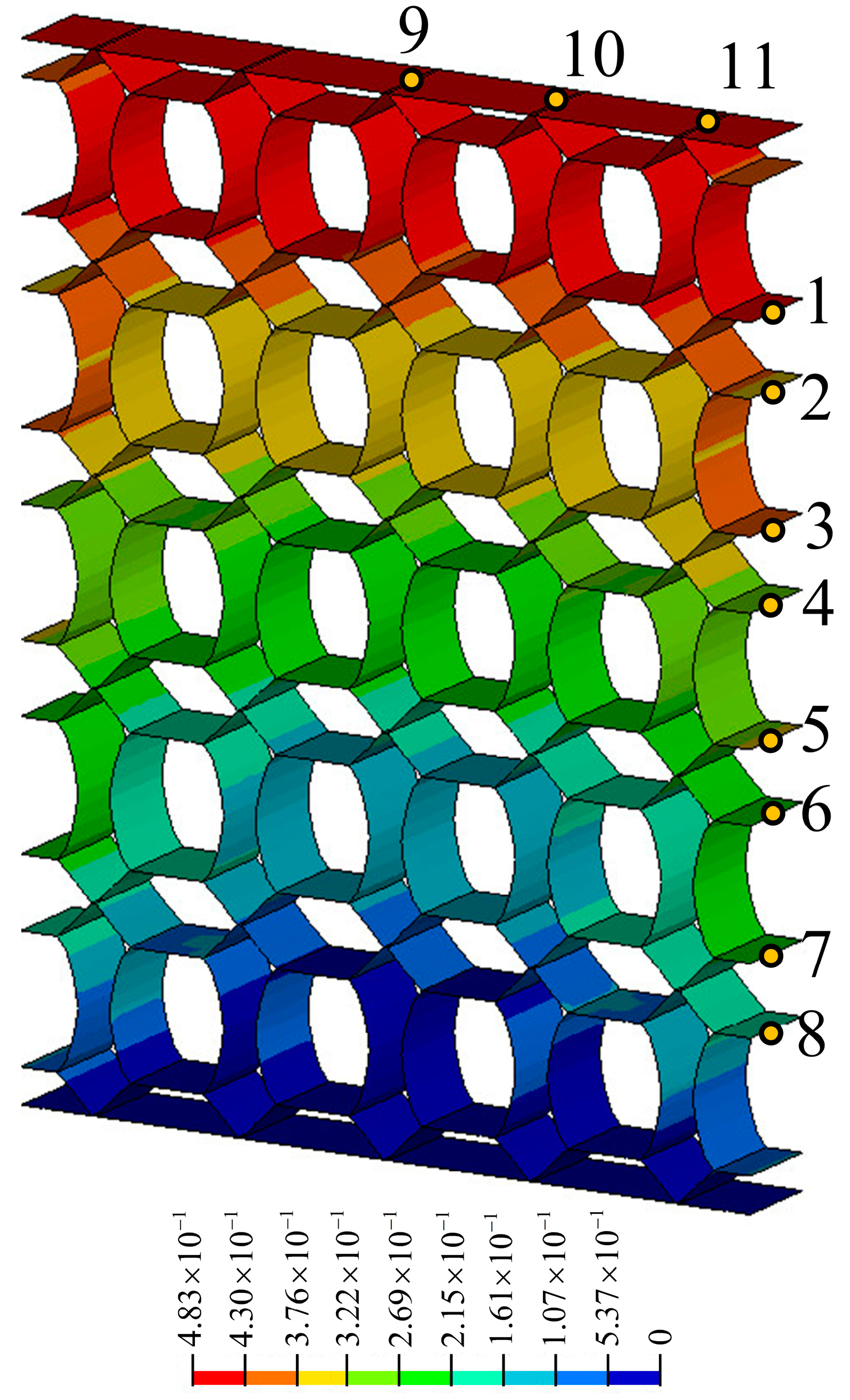
5.1. Frequency Response Analysis
5.2. Vibration Reduction Performance
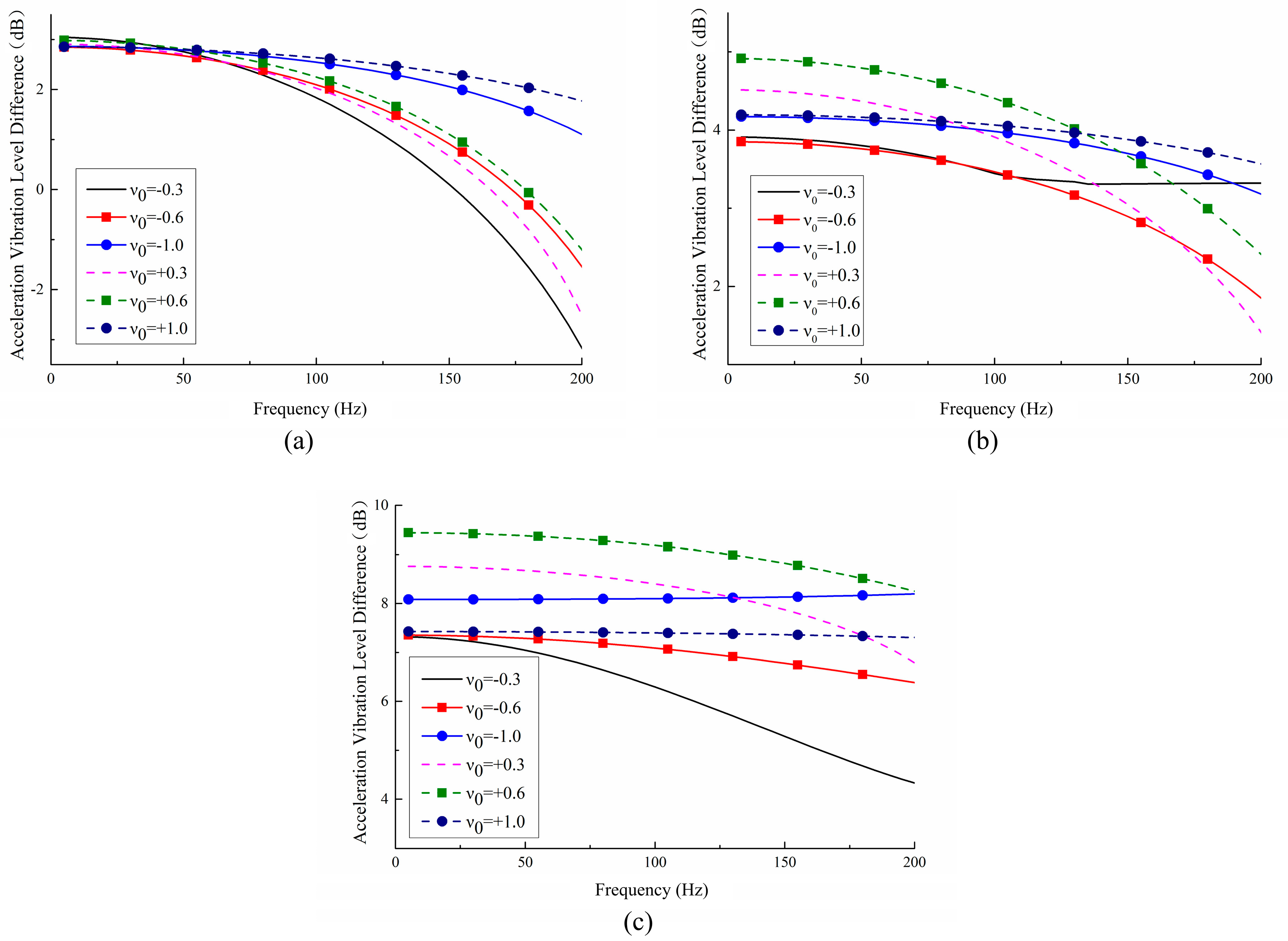
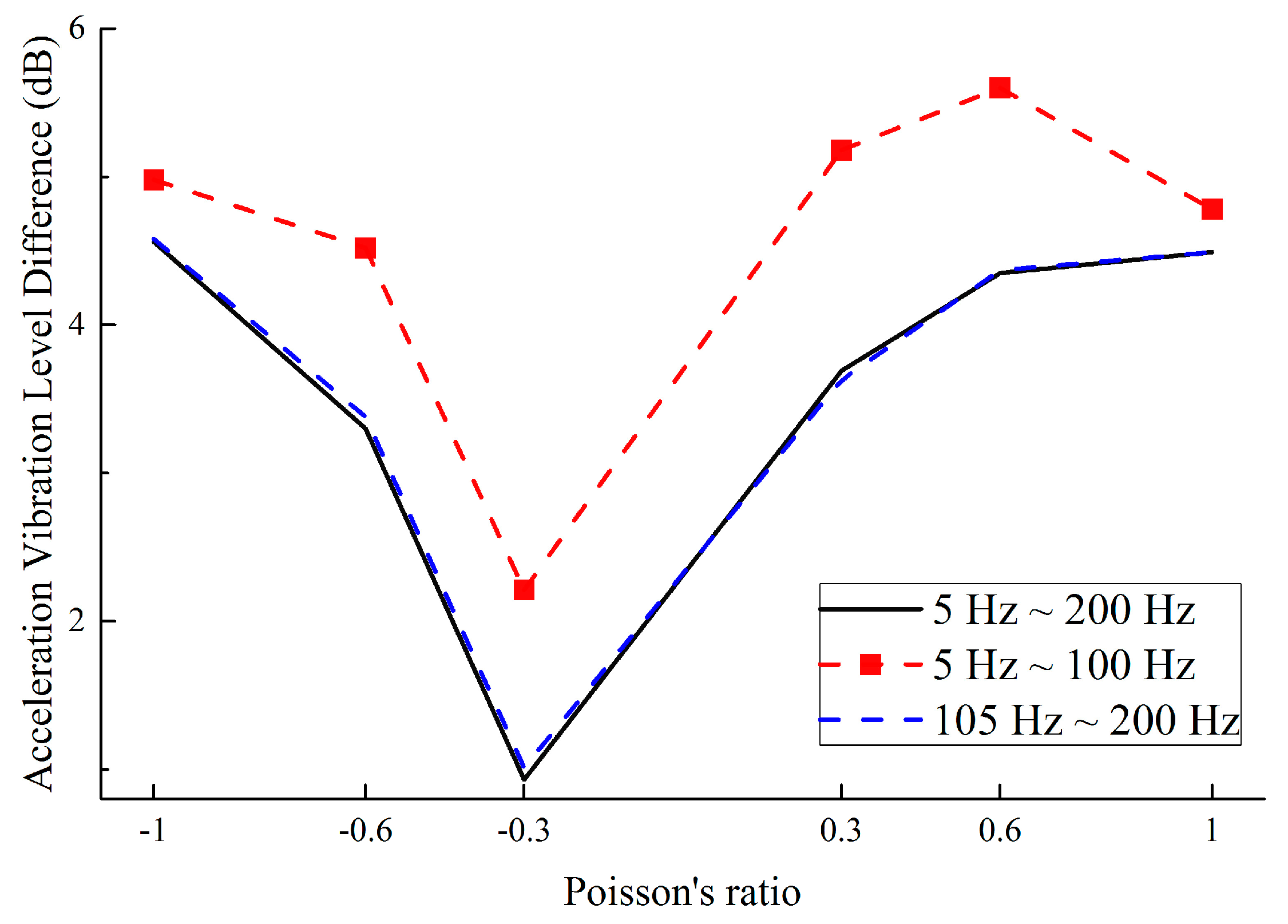
5.3. Relationship between PR and Vibration Reduction Performance
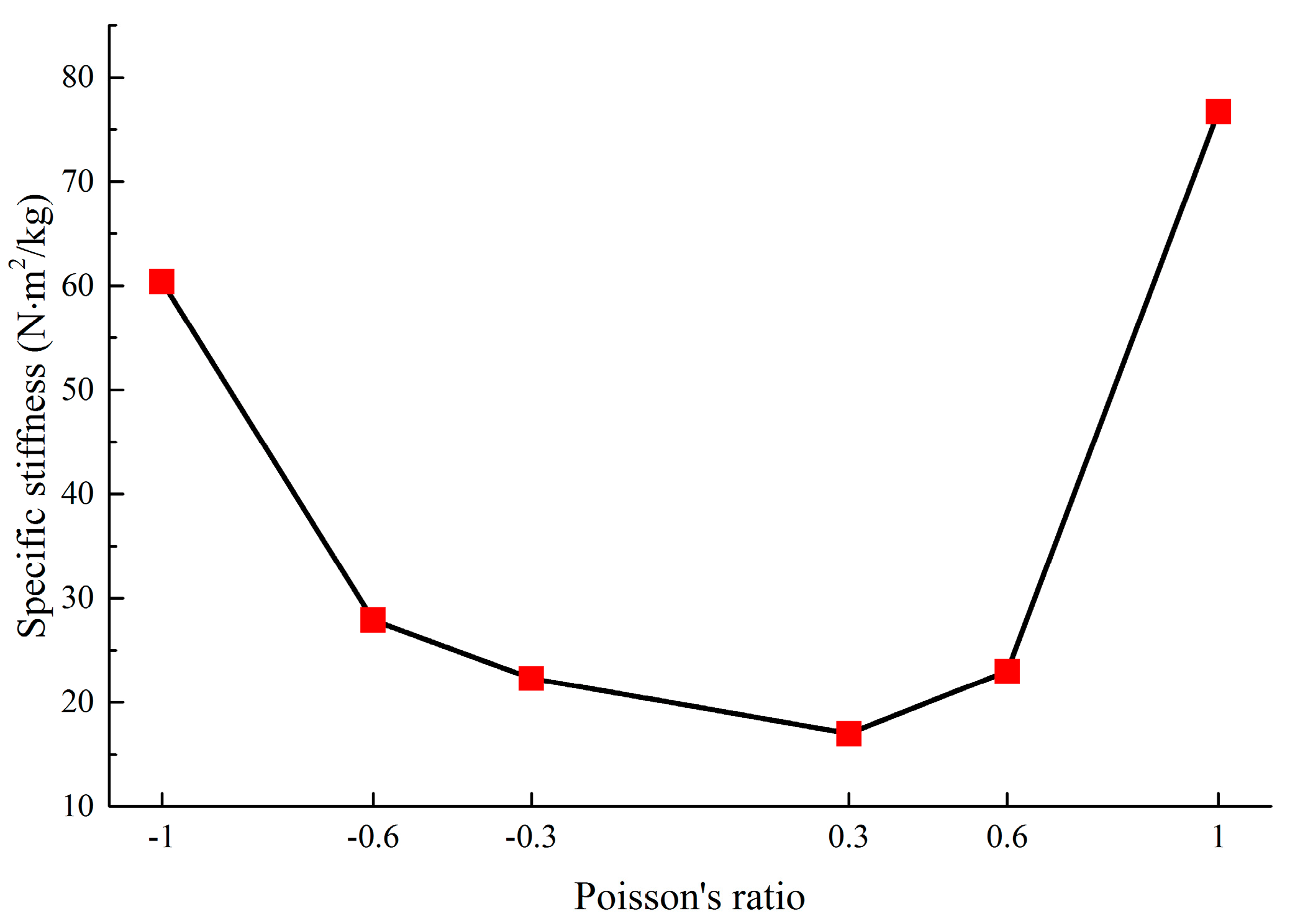
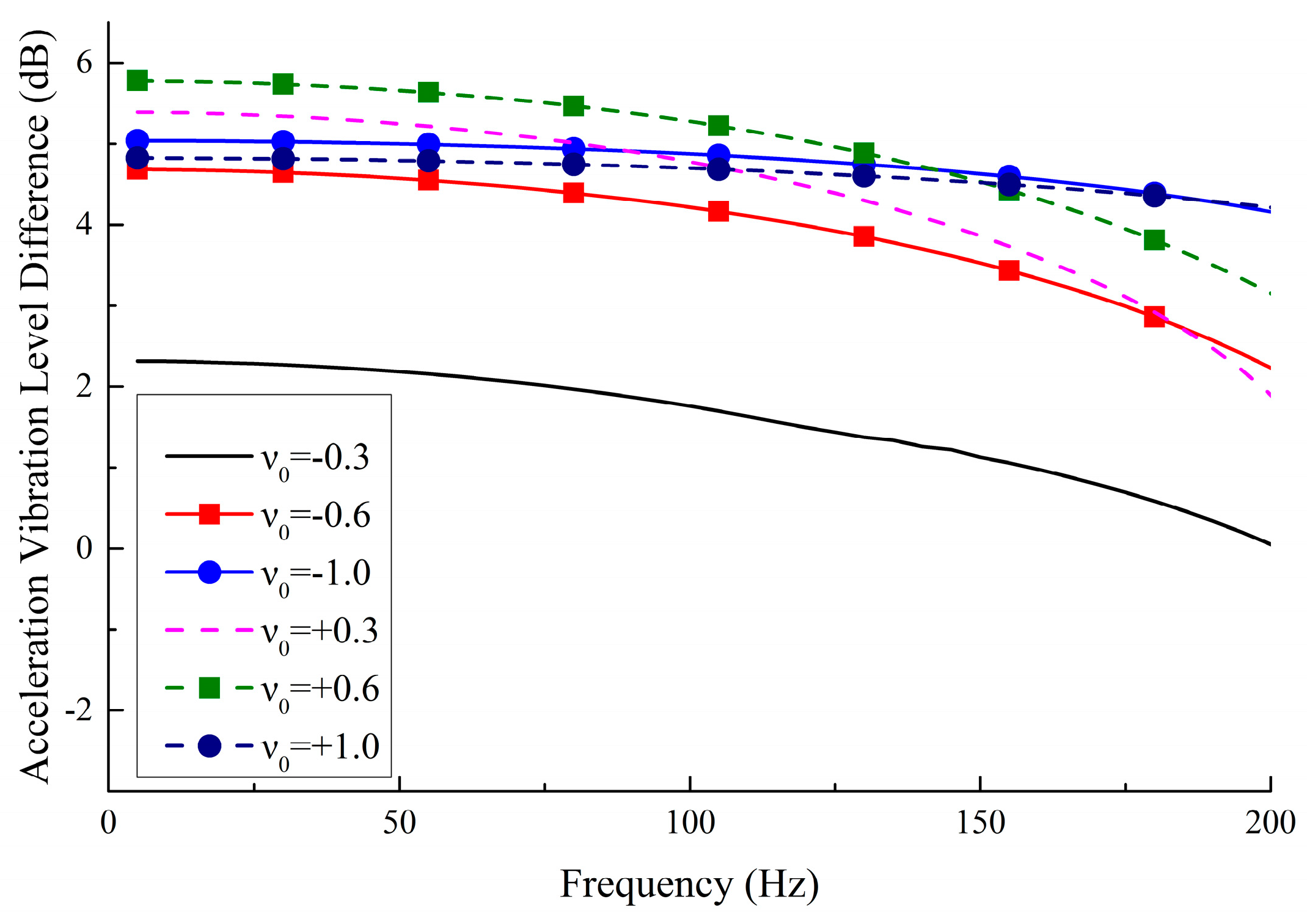
6. Lightweight Effects on Deformation Resistance and Vibration Reduction
7. Conclusions
- This work not only provides several new configurations of metamaterials, but the core innovation is to propose a design method of lightweight metamaterials by establishing a mathematical optimization model. Moreover, the design method enables the design of lightweight metamaterials with a specified Poisson’s ratio value.
- Comparison with honeycomb materials shows that the designed novel metamaterials exhibits outstanding deformation resistance and vibration reduction performance.
- The concepts of specific stiffness, , and specific vibration reduction, , are defined to evaluate the lightweight effects of novel metamaterials on deformation resistance and vibration reduction, respectively.
Author Contributions
Funding
Conflicts of Interest
References
- Nia, A.A.; Razavi, S.B.; Majzoobi, G.H. Ballistic limit determination of aluminum honeycombs-Experimental study. Mater. Sci. Eng. A 2008, 488, 273–280. [Google Scholar]
- Yungwirth, C.J.; Wadley, H.N.; O’Connor, J.H.; Zakraysek, A.J.; Deshpande, V.S. Impact response of sandwich plates with a pyramidal lattice core. Int. J. Eng. Sci. 2008, 35, 920–936. [Google Scholar] [CrossRef]
- Compton, B.G.; Lewis, J. A 3D-printing of lightweight cellular composites. Adv. Mater. 2014, 26, 5930–5935. [Google Scholar] [CrossRef] [PubMed]
- Jiang, L.; Hu, H. Finite element modeling of multilayer orthogonal auxetic composites under low-velocity impact. Materials. 2017, 10, 908. [Google Scholar] [CrossRef] [PubMed]
- Duc, N.D.; Seung-Eock, K.; Cong, P.H.; Cong, P.H.; Anh, N.T.; Khoa, N.D. Dynamic response and vibration of composite double curved shallow shells with negative Poisson’s ratio in auxetic honeycombs core layer on elastic foundations subjected to blast and damping loads. Int. J. Mech. Sci. 2017, 133, 504–512. [Google Scholar] [CrossRef]
- Wang, X.T.; Wang, B.; Wen, Z.H.; Li, M. Fabrication and mechanical properties of CFRP composite three-dimensional double-arrow-head auxetic structures. Compos. Sci. Technol. 2018, 164, 92–102. [Google Scholar] [CrossRef]
- Alderson, A.; Alderson, K.L. Auxetic materials. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2007, 221, 565–575. [Google Scholar] [CrossRef]
- Heng, L.; Wang, B.; Li, M.; Zhang, Y.; Jiang, L. Advances in Fabrication Materials of Honeycomb Structure Films by the Breath-Figure Method. Materials 2013, 6, 460–482. [Google Scholar] [CrossRef] [PubMed]
- Bückmann, T.; Stenger, N.; Kadic, M.; Kaschke, J.; Frölich, A.; Kennerknecht, T.; Eberl, C.; Thiel, M.; Wegner, M. Tailored 3D mechanical metamaterials made by dip in direct laser writing optical lithography. Adv. Mater. 2012, 24, 2710–2714. [Google Scholar] [CrossRef] [PubMed]
- Ren, X.; Das, R.; Tran, P.; Ngo, T.D.; Xie, Y.M. Auxetic metamaterials and structures: A review. Smart Mater. Struct. 2018, 27, 023001. [Google Scholar] [CrossRef]
- Lekesiz, H.; Bhullar, S.K.; Karaca, A.A.; Jun, M.B.G. Mechanical characterization of auxetic stainless steel thin sheets with reentrant structure. Smart Mater. Struct. 2017, 26, 085022. [Google Scholar] [CrossRef]
- Wan, H.; Ohtaki, H.; Kotosaka, S.; Hu, G. A study of negative Poisson’s ratios in auxetic honeycombs based on a large deflection model. Eur. J. Mech. A Solids 2004, 23, 95–106. [Google Scholar] [CrossRef]
- Findeisen, C.; Hohe, J.; Kadic, M.; Gumbsch, P. Characteristics of mechanical metamaterials based on buckling elements. J. Mech. Phys. Solids 2017, 102, 151–164. [Google Scholar] [CrossRef]
- Strek, T.; Jopek, H.; Idczak, E.; Wojciechowski, K. Computational Modelling of structures with non-intuitive behaviour. Materials 2017, 10, 1386. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; Fu, M.H. A novel three-dimensional auxetic lattice meta-material with enhanced stiffness. Smart Mater. Struct. 2017, 26, 105029. [Google Scholar] [CrossRef]
- Catapano, A.; Montemurro, M. A multi-scale approach for the optimum design of sandwich plates with honeycomb core. Part II: The optimization strategy. Compos. Struct. 2014, 118, 677–690. [Google Scholar] [CrossRef]
- Seepersad, C.C.; Kumar, R.S.; Allen, J.K.; Mistree, F.; Mcdowell, D.L. Multifunctional design of prismatic cellular materials. J. Comput. Aided Mater. Des. 2004, 11, 163–181. [Google Scholar] [CrossRef]
- Ju, J.; Summers, J.D.; Ziegert, J.; Fadel, G. Design of honeycomb meta-materials for high shear flexure. In Proceedings of the International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, American Society of Mechanical Engineers, San Diego, CA, USA, 30 August–2 September 2009; pp. 805–813. [Google Scholar]
- Boucher, M.A.; Smith, C.W.; Scarpa, F.; Rajasekaran, R.; Evans, K.E. Effective topologies for vibration damping inserts in honeycomb structures. Compos. Struct. 2013, 106, 1–14. [Google Scholar] [CrossRef]
- Strek, T.; Jopek, H.; Idczak, E. Computational design of two-phase auxetic structures. Phys. Status Solidi B 2016, 253, 1387–1394. [Google Scholar] [CrossRef]
- Czarnecki, S.; Łukasiak, T.; Lewiński, T. The isotropic and cubic material designs. Recovery of the underlying microstructures appearing in the least compliant continuum bodies. Materials 2017, 10, 1137. [Google Scholar] [CrossRef] [PubMed]
- Awrejcewicz, J.; Pavlov, S.P.; Bodyagina, K.S.; Zhigalov, M.V.; Krysko, V.A. Design of composite structures with extremal elastic properties in the presence of technological constraints. Compos. Struct. 2017, 174, 19–25. [Google Scholar] [CrossRef]
- Wang, B.; Cheng, G.D. Design of cellular structures for optimum efficiency of heat dissipation. Struct. Multidiscip. Optim. 2005, 30, 447–458. [Google Scholar] [CrossRef]
- Qin, H.; Yang, D.; Ren, C. Modelling theory of functional element design for metamaterials with arbitrary negative Poisson’s ratio. Comput. Mater. Sci. 2018, 150, 121–133. [Google Scholar] [CrossRef]
- Chen, S.; Ryu, S.C. Design and characterization of rounded re-entrant honeycomb patterns for lightweight and rigid auxetic structures. Smart Mater. Struct. 2017, 26, 115026. [Google Scholar] [CrossRef]
- Ingrole, A.; Hao, A.; Liang, R. Design and modeling of auxetic and hybrid honeycomb structures for in-plane property enhancement. Mater. Des. 2017, 117, 72–83. [Google Scholar] [CrossRef]
- Evans, K.E.; Nkansah, M.A.; Hutchinson, I.J.; Rogers, S.C. Molecular network design. Nature 1991, 353, 124–125. [Google Scholar] [CrossRef]
- Carta, G.; Brun, M.; Baldi, A. Design of a porous material with isotropic negative Poisson’s ratio. Mech. Mater. 2016, 97, 67–75. [Google Scholar] [CrossRef]
- Li, D.; Yin, J.; Dong, L.; Lakes, R.S. Numerical analysis on mechanical behaviors of hierarchical cellular structures with negative Poisson’s ratio. Smart Mater. Struct. 2016, 26, 025014. [Google Scholar] [CrossRef]
- Yang, W.; Li, Z.M.; Shi, W.; Xie, B.H.; Yang, M.B. Review on auxetic materials. J. Mater. Sci. 2004, 39, 3269–3279. [Google Scholar] [CrossRef]
- Prawoto, Y. Seeing auxetic materials from the mechanics point of view: A structural review on the negative Poisson’s ratio. Comput. Mater. Sci. 2012, 58, 140–153. [Google Scholar] [CrossRef]
- Scarpa, F.; Tomlinson, G. Theoretical characteristics of the vibration of sandwich plates with in-plane negative Poisson’s ratio values. J. Sound Vib. 2000, 230, 45–67. [Google Scholar] [CrossRef]
- Grima, J.N.; Gatt, R.; Ellul, B.; Chetcuti, E. Auxetic behaviour in non-crystalline materials having star or triangular shaped perforations. J. Non Cryst. Solids 2010, 356, 1980–1987. [Google Scholar] [CrossRef]
- Gibson, L.J.; Ashby, M.F. Cellular Solids: Structure and Properties; Cambridge University Press: Cambridge, UK, 1988. [Google Scholar]
- Schwerdtfeger, J.; Wein, F.; Leugering, G.; Singer, R.F.; Körner, C.; Stingl, M.; Schury, F. Design of auxetic structures via mathematical optimization. Adv. Mater. 2011, 23, 2650–2654. [Google Scholar] [CrossRef] [PubMed]
- Guan, G.Y.; Jiao, G.Q.; Zhang, Z.G. Wiley Online Library, Uniaxial Macro-Mechanical Property and Failure Analysis of a 2D-Woven SiC/SiC Composite. Available online: https://onlinelibrary.wiley.com/doi/abs/10.1002/9781118932995.ch30 (accessed on 24 August 2018).
- Carneiro, V.H.; Puga, H.; Meireles, J. Analysis of the geometrical dependence of auxetic behavior in reentrant structures by finite elements. Acta Mech. Sin. 2016, 32, 295–300. [Google Scholar] [CrossRef]
- Kato, J.; Yachi, D.; Terada, K.; Kyoya, T. Topology optimization of micro-structure for composites applying a decoupling multi-scale analysis. Struct. Multidiscip. Optim. 2014, 49, 595–608. [Google Scholar] [CrossRef]
- Sigmund, O.; Maute, K. Topology optimization approaches. Struct. Multidiscip. Optim. 2013, 48, 1031–1055. [Google Scholar] [CrossRef]
- Huang, X.; Zhou, S.W.; Xie, Y.M.; Li, Q. Topology optimization of microstructures of cellular materials and composites for macrostructure. Comput. Mater. Sci. 2013, 67, 397–407. [Google Scholar] [CrossRef]
- Van, D.N.P.; Maute, K.; Langelaar, M.; Van, K.F. Level-set methods for structural topology optimization: A review. Struct. Multidiscip. Optim. 2013, 48, 437–472. [Google Scholar]
- Huang, J.; Zhang, Q.; Scarpa, F.; Liu, Y.; Leng, J. In-plane elasticity of a novel auxetic honeycomb design. Compos. Part. B Eng. 2017, 110, 72–82. [Google Scholar] [CrossRef]
- Zhang, X.; Yang, D. Mechanical properties of auxetic cellular material consisting of re-entrant hexagonal honeycombs. Materials 2016, 9, 900. [Google Scholar] [CrossRef] [PubMed]
- Grima, J.N.; Attard, D.; Gatt, R.; Cassar, R.N. A novel process for the manufacture of auxetic foams and for their re-conversion to conventional form. Adv. Eng. Mater. 2009, 11, 533–535. [Google Scholar] [CrossRef]
- Lanczos, C. An Iteration Method for the Solution of the Eigenvalue Problem of Linear Differential and Integral Operators; United States Governm Press Office: Los Angeles, CA, USA, 1950.
- Rossikhin, Y.A.; Shitikova, M.V. New approach for the analysis of damped vibrations of fractional oscillators. Shock Vib. 2009, 16, 365–3878. [Google Scholar] [CrossRef]

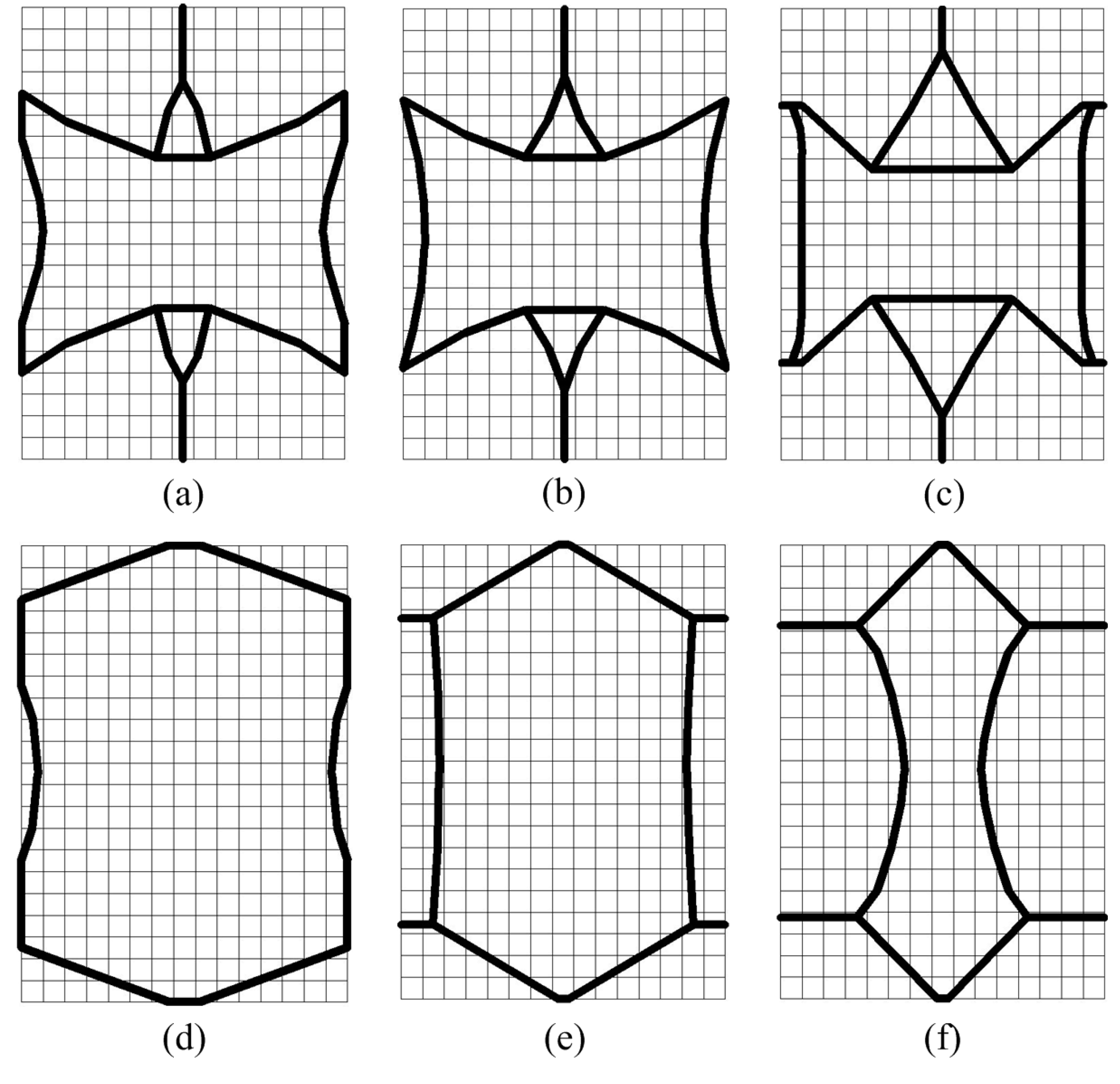




| Design Requirements | ||||||
| After Extraction | ||||||
| Relative Error Ratio | +5.3% | −2.8% | +2.7% | +1.0% | −3.7% | +8.0% |
| Metamaterials | P/N | W/kg | ||||
|---|---|---|---|---|---|---|
| Honeycomb with | 33 | 15.07 | 2.2 | 1.092 × 10−1 | 294 × 230 × 20 | 27.2 |
| 31 | 1.39 | 22.3 | 9.13 × 10−2 | 210 × 150 × 20 | 153.9 | |
| 19 | 0.68 | 27.9 | 9.81 × 10−2 | 210 × 150 × 20 | 179.4 | |
| 25 | 0.41 | 60.4 | 1.071 × 10−1 | 210 × 150 × 20 | 355.3 | |
| 36 | 2.12 | 17.0 | 8.18 × 10−2 | 210 × 150 × 20 | 130.9 | |
| 50 | 2.17 | 23.0 | 7.77 × 10−2 | 210 × 150 × 20 | 186.7 | |
| 36 | 0.47 | 76.7 | 8.06 × 10−2 | 210 × 150 × 20 | 599.5 |
| Metamaterials with Various PR | ||||||
| Natural Frequency | 89.5 Hz | 97.5 Hz | 136.2 Hz | 78.1 Hz | 90.9 Hz | 163.1 Hz |
| Poisson’s Ratio | ||||||
| Mass (g) | 91.3 | 98.1 | 107.1 | 81.8 | 77.7 | 80.6 |
| (dB) | 1.56 | 3.95 | 4.78 | 4.40 | 4.99 | 4.63 |
| Specific Vibration Reduction(dB/kg) | 17 | 40 | 45 | 54 | 64 | 57 |
| Normalized | 0.27 | 0.63 | 0.70 | 0.84 | 1.00 | 0.90 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qin, H.; Yang, D.; Ren, C. Design Method of Lightweight Metamaterials with Arbitrary Poisson’s Ratio. Materials 2018, 11, 1574. https://doi.org/10.3390/ma11091574
Qin H, Yang D, Ren C. Design Method of Lightweight Metamaterials with Arbitrary Poisson’s Ratio. Materials. 2018; 11(9):1574. https://doi.org/10.3390/ma11091574
Chicago/Turabian StyleQin, Haoxing, Deqing Yang, and Chenhui Ren. 2018. "Design Method of Lightweight Metamaterials with Arbitrary Poisson’s Ratio" Materials 11, no. 9: 1574. https://doi.org/10.3390/ma11091574
APA StyleQin, H., Yang, D., & Ren, C. (2018). Design Method of Lightweight Metamaterials with Arbitrary Poisson’s Ratio. Materials, 11(9), 1574. https://doi.org/10.3390/ma11091574





