Preparation of Fibrillated Cellulose Nanofiber from Lyocell Fiber and Its Application in Air Filtration
Abstract
:1. Introduction
2. Materials and Methods
2.1. Nanofibrillation of Lyocell Fiber
2.2. Characterization of Lyocell Nanofiber
2.3. Preparation of Nanofiber Composite Filter Paper
2.4. Filtration Test of Nanofiber Composite Filter Media
3. Results and Discussion
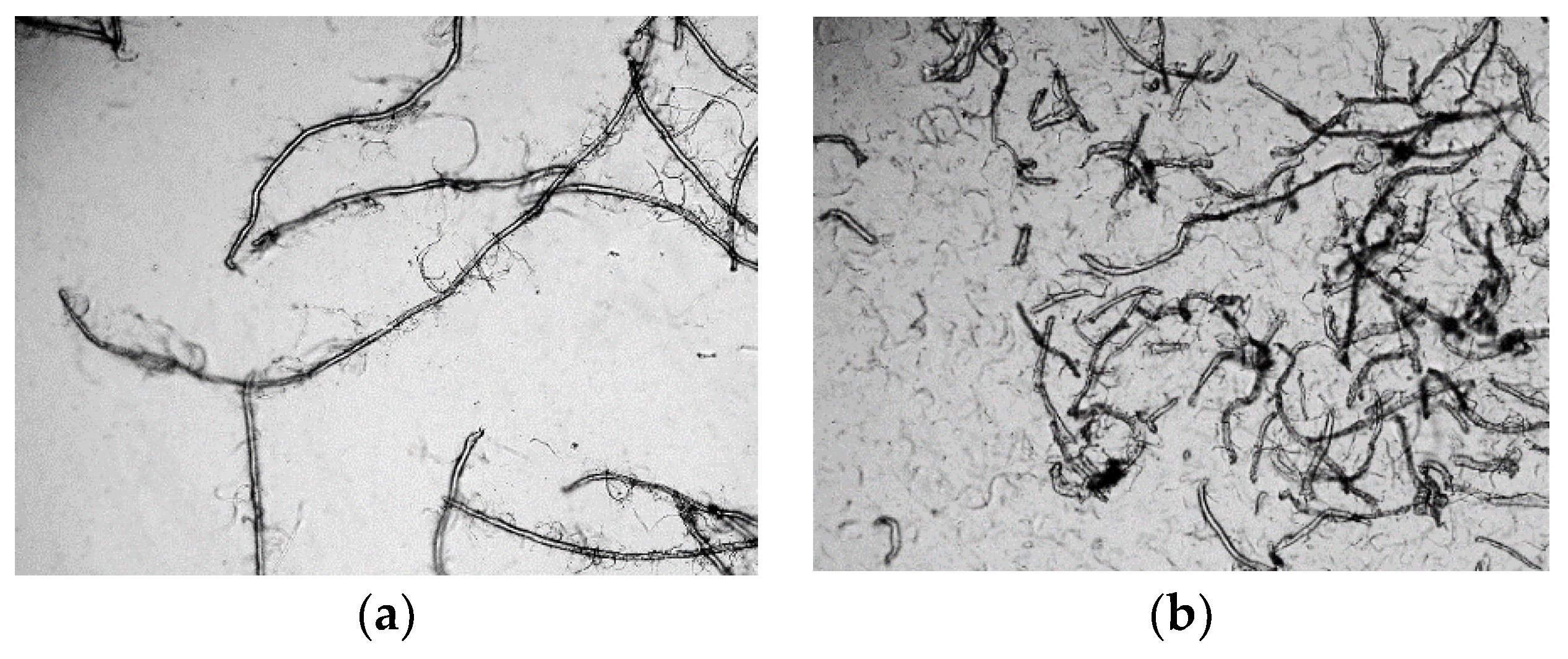
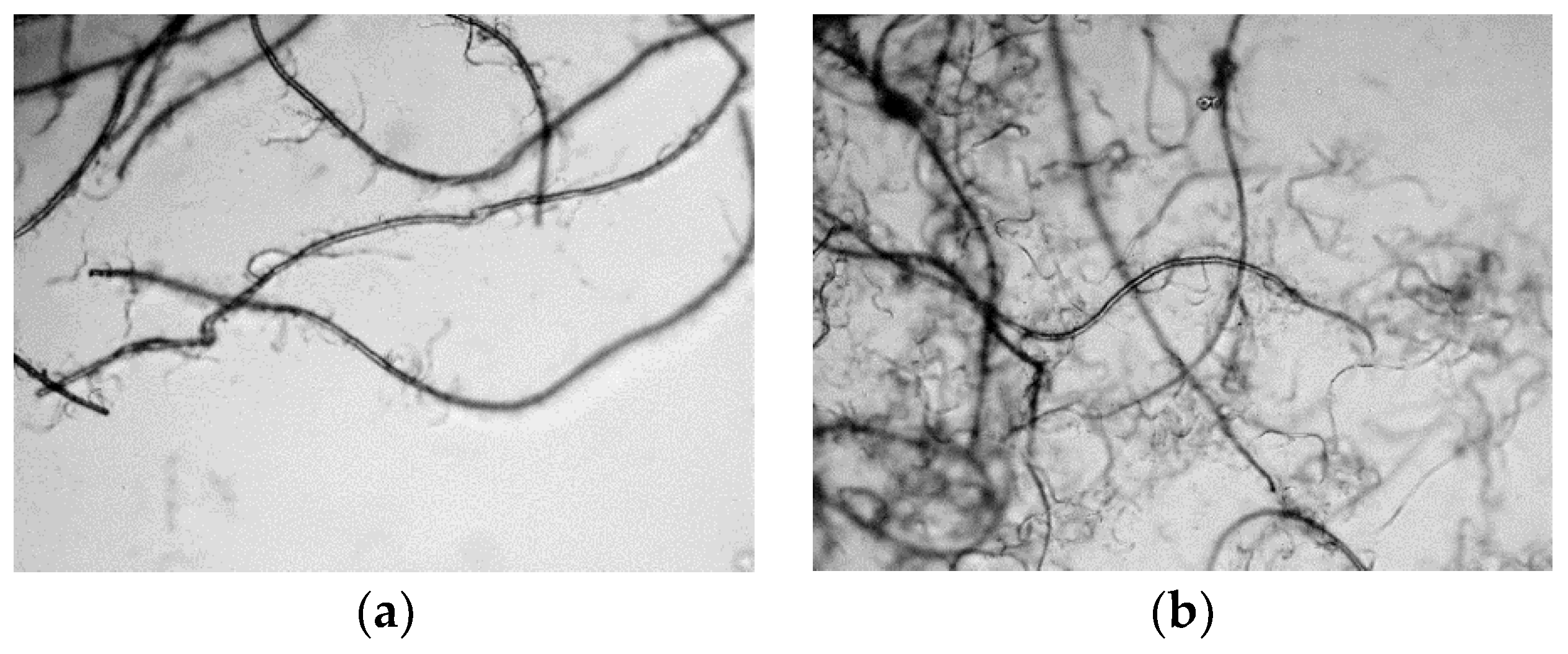
3.1. Effect of Refining Pressure on Fibrillation of Lyocell Fiber
3.2. Effect of Number of Revolutions on Fibrillation of Lyocell Fiber
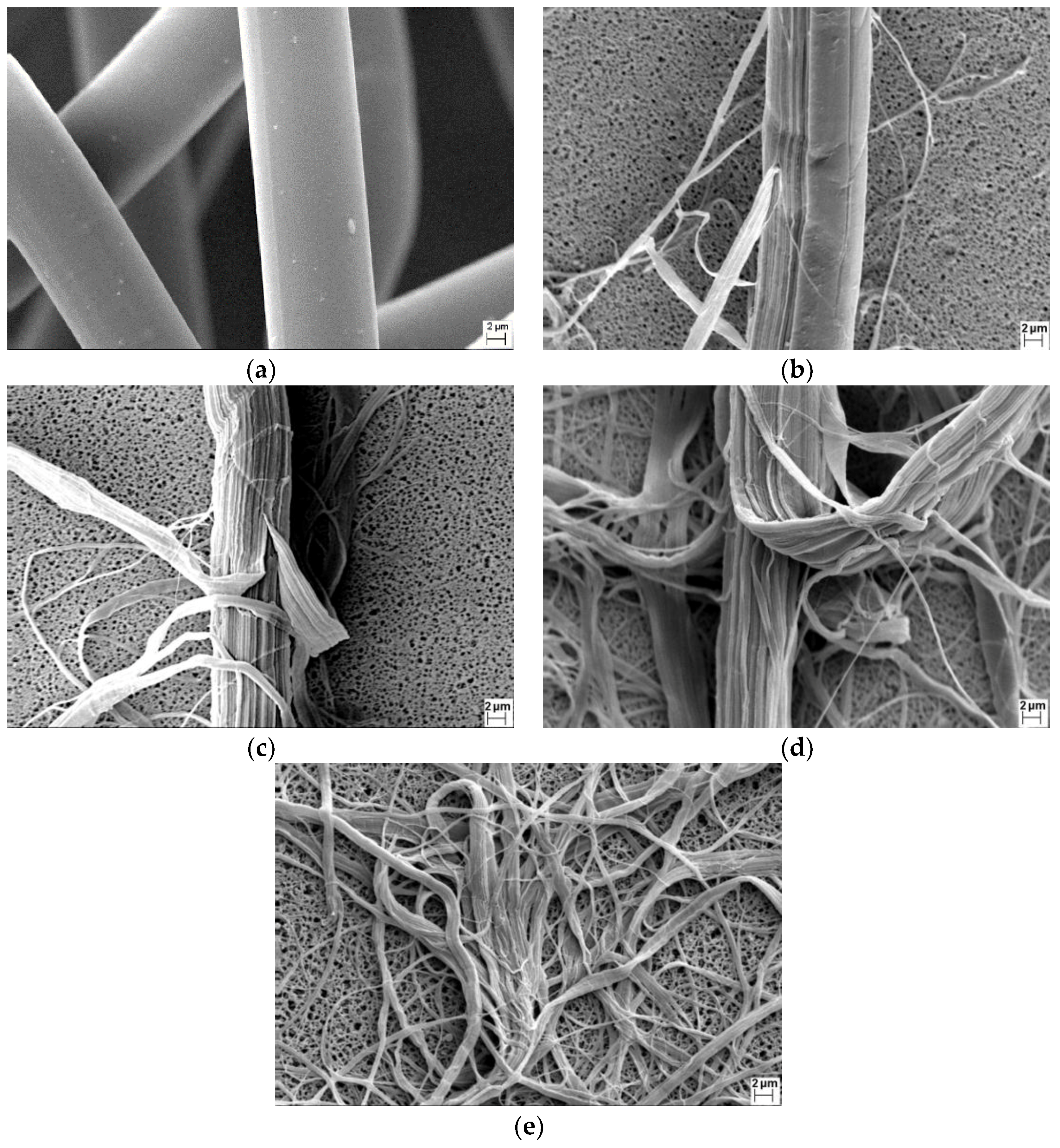
3.3. Physical Properties of Filter Paper Containing Fibrillated Nanofiber
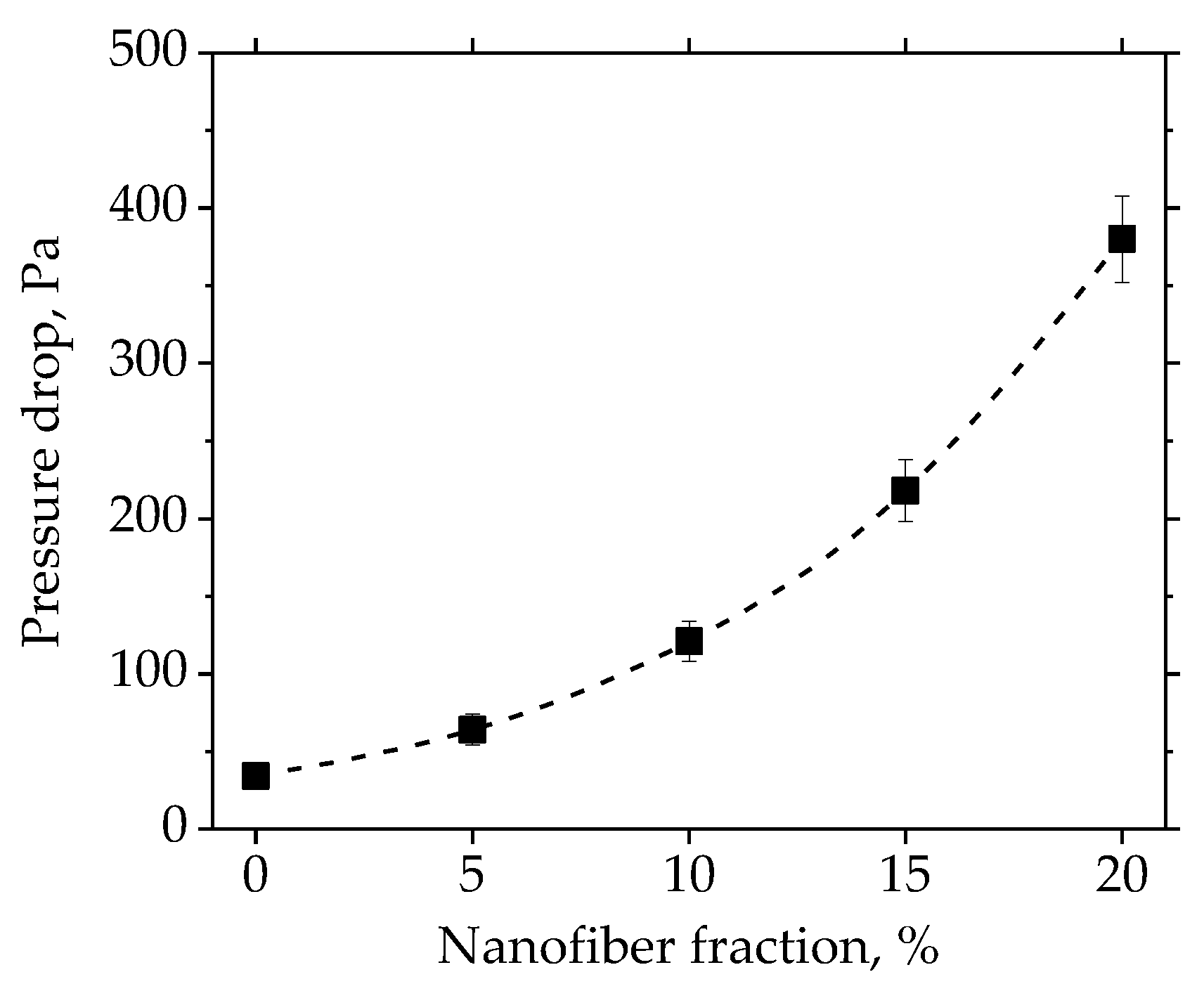
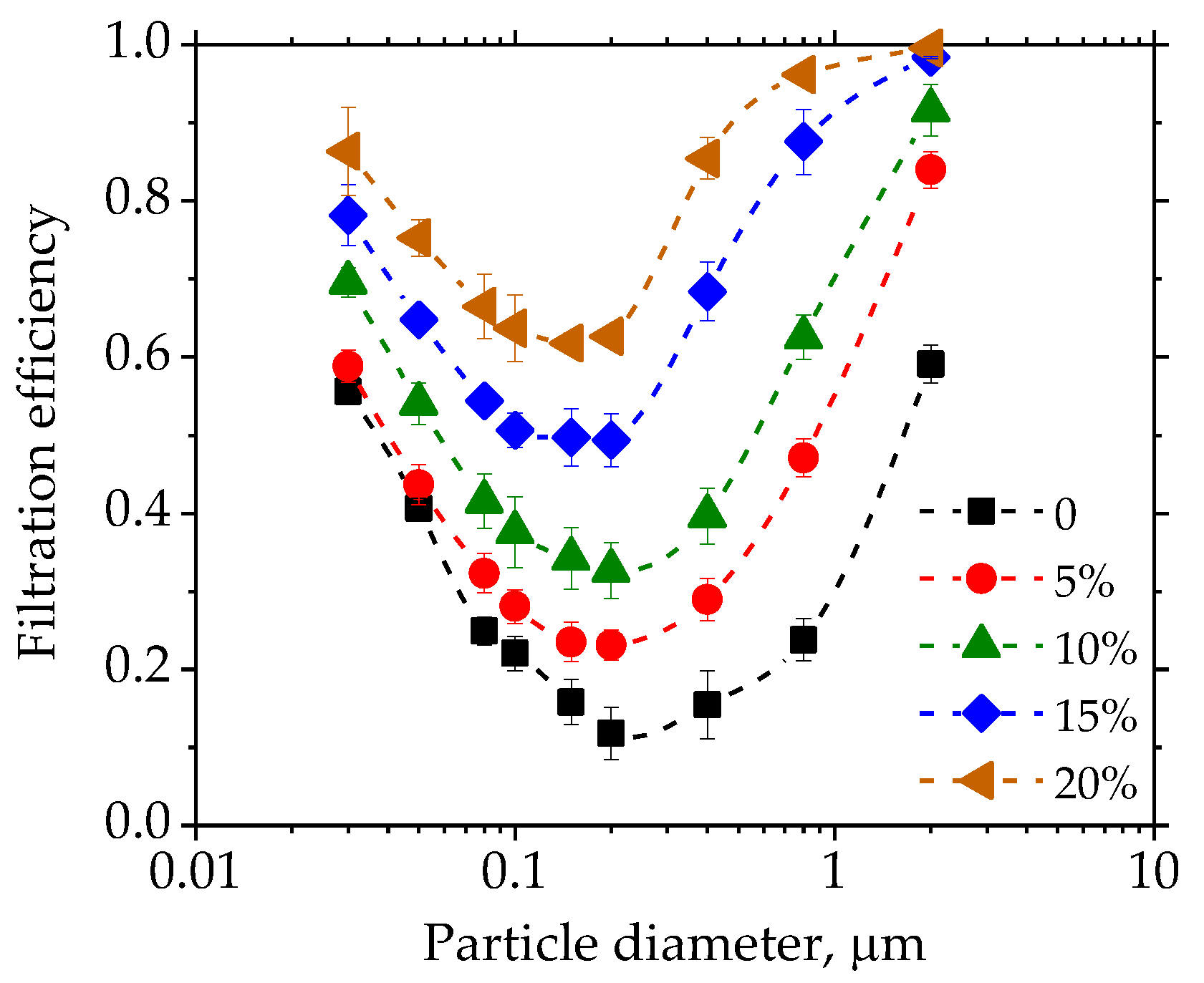
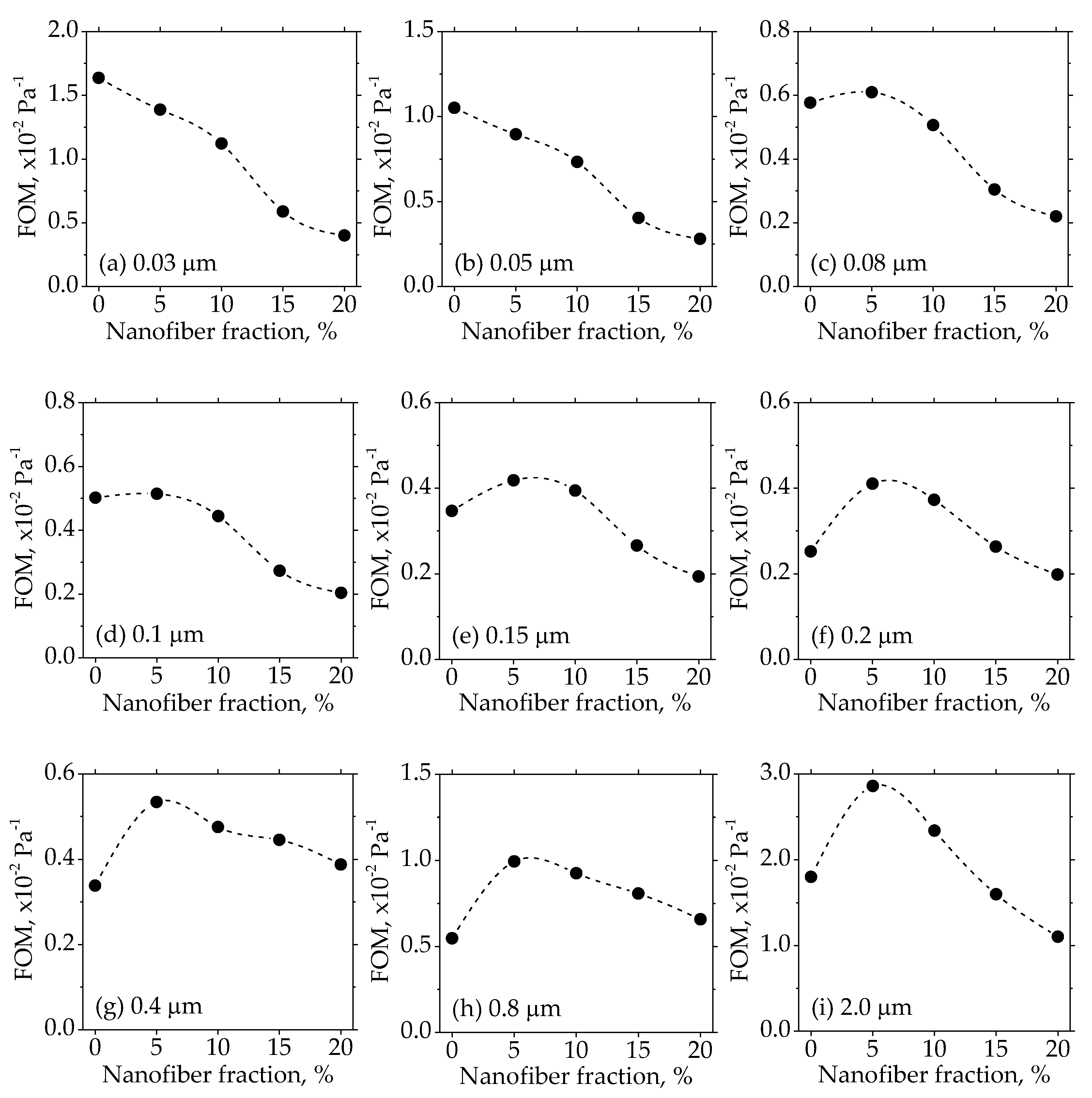
3.4. Filtration Performance of Filter Paper Containing Fibrillated Nanofiber
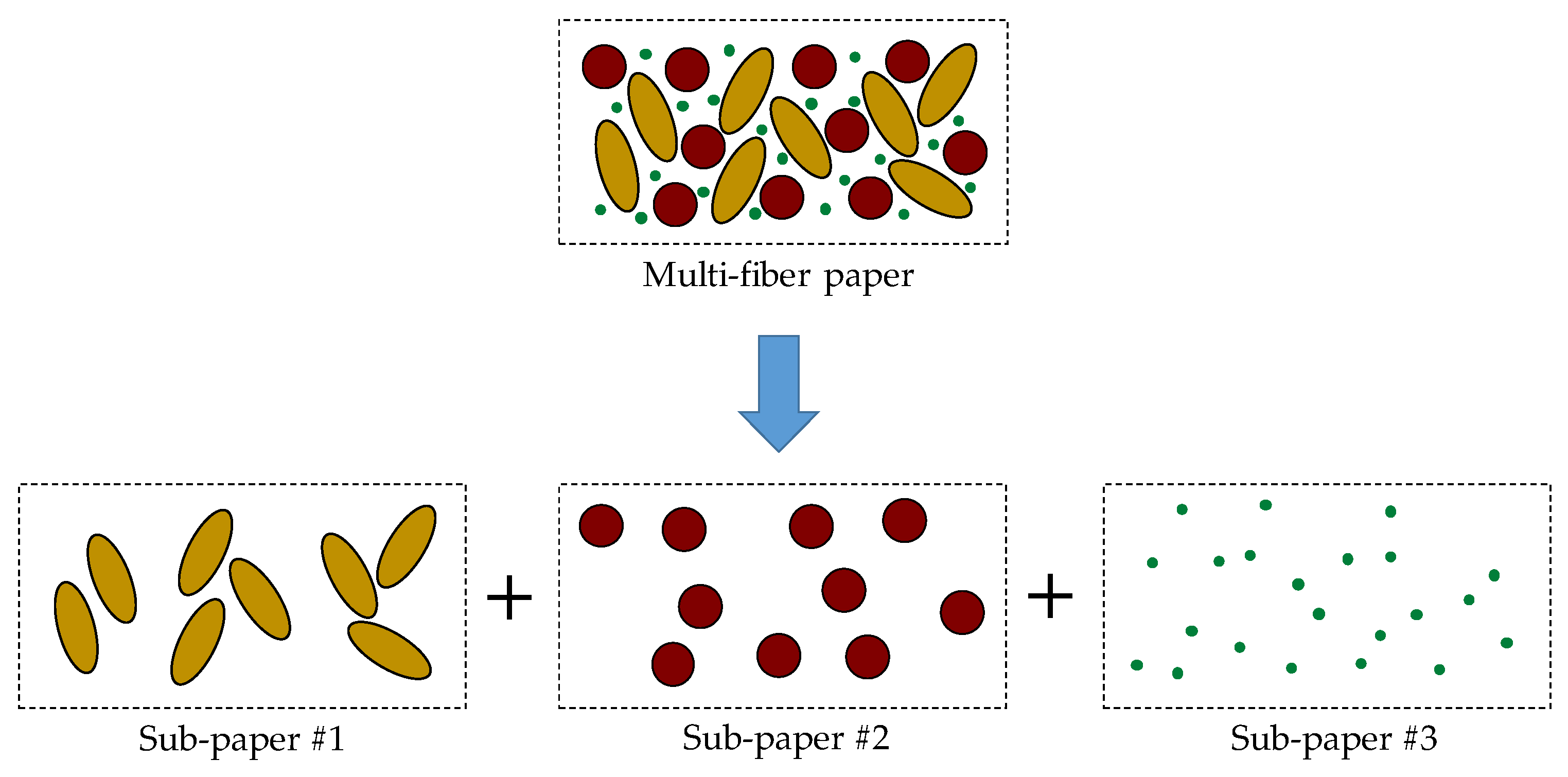
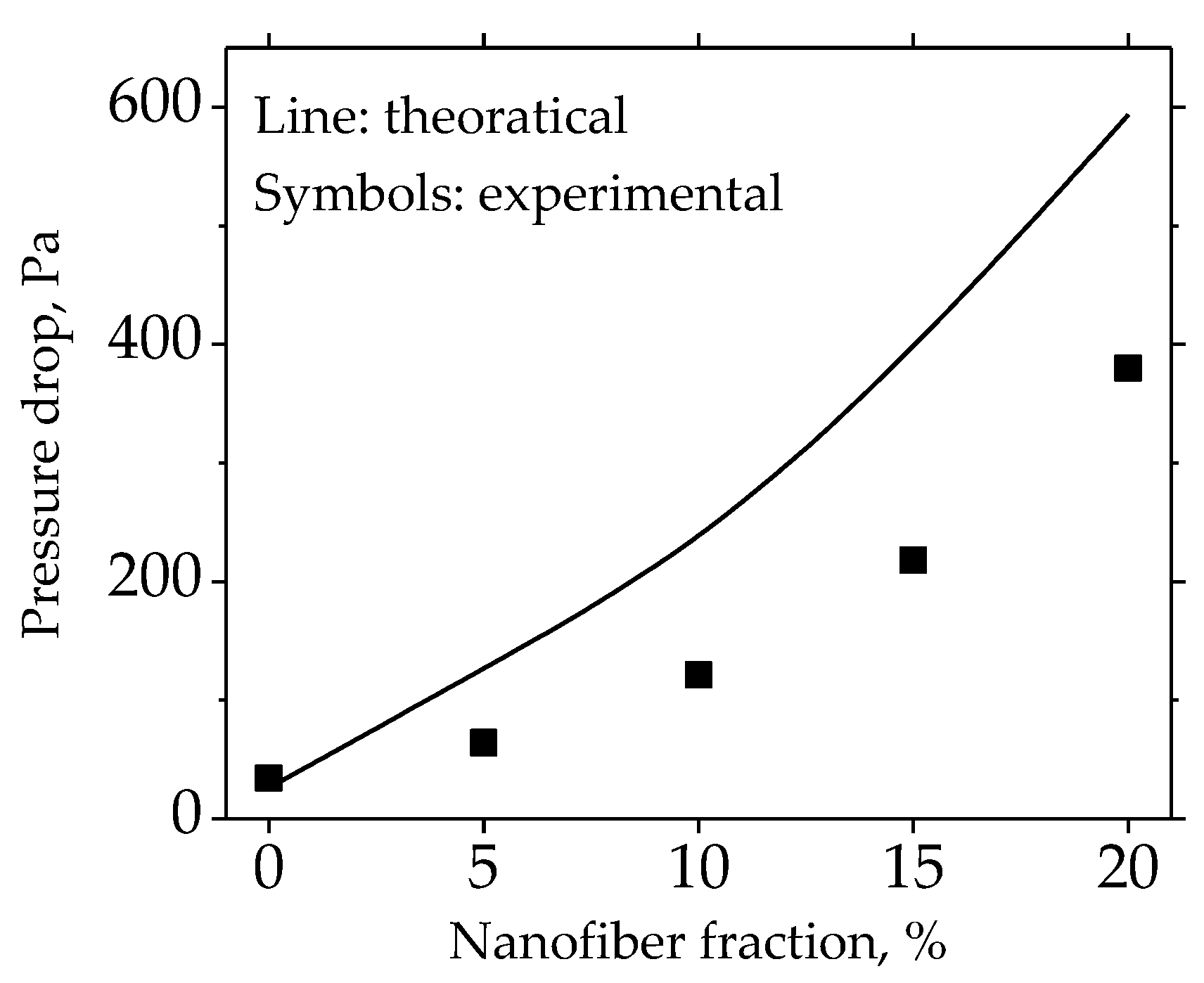
3.5. Theoretical Analysis of Nanofiber Inhomogeneity
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Pui, D.Y.H.; Chen, S.C.; Zuo, Z. PM2.5 in China: Measurements, sources, visibility and health effects, and mitigation. Particuology 2014, 13, 1–26. [Google Scholar] [CrossRef]
- Gupta, A.; Novick, V.J.; Biswas, P.; Monson, P.R. Effect of humidity and particle hygroscopicity on the mass loading capacity of high efficiency particulate air (HEPA) filters. Aerosol Sci. Technol. 1993, 19, 94–107. [Google Scholar] [CrossRef]
- Joubert, A.; Laborde, J.C.; Bouilloux, L.; Calle-Chazelet, S.; Thomas, D. Influence of humidity on clogging of flat and pleated HEPA filters. Aerosol Sci. Technol. 2010, 44, 1065–1076. [Google Scholar] [CrossRef]
- Poon, W.S.; Liu, B.Y.H. A bimodal loading test for engine and general purpose air cleaning filters. SAE Tech. Pap. 1997, 970674. [Google Scholar] [CrossRef]
- Wang, C.; Otani, Y. Removal of nanoparticles from gas streams by fibrous filters: A review. Ind. Eng. Chem. Re. 2012, 52, 5–17. [Google Scholar] [CrossRef]
- Thomas, D.; Penicot, P.; Contal, P.; Leclerc, D.; Vendel, J. Clogging of fibrous filters by solid aerosol particles experimental and modelling study. Chem. Eng. Sci. 2001, 56, 3549–3561. [Google Scholar] [CrossRef]
- Hutten, I.M. Handbook of Nonwoven Filter Media, 2nd ed.; Elsevier Butterworth Heinemann: Oxford, UK, 2012; pp. 520–523. [Google Scholar]
- Chen, D.R.; Pui, D.Y.H.; Liu, B.Y.H. Optimization of pleated filter designs using a finite-element numerical model. Aerosol Sci. Technol. 1995, 23, 579–590. [Google Scholar] [CrossRef]
- Wang, J.; Kim, S.C.; Pui, D.Y.H. Investigation of the figure of merit for filters with a single nanofiber layer on a substrate. J. Aerosol Sci. 2008, 39, 323–334. [Google Scholar] [CrossRef]
- Podgórski, A.; Bałazy, A.; Gradoń, L. Application of nanofibers to improve the filtration efficiency of the most penetrating aerosol particles in fibrous filters. Chem. Eng. Sci. 2006, 61, 6804–6815. [Google Scholar] [CrossRef]
- Wang, J.; Kim, S.C.; Pui, D.Y.H. Figure of merit of composite filters with micrometer and nanometer fibers. Aerosol Sci. Technol. 2008, 42, 722–728. [Google Scholar] [CrossRef]
- Bao, L.; Seki, K.; Niinuma, H.; Otani, Y.; Balgis, R.; Ogi, T.; Gradon, L.; Okuyama, K. Verification of slip flow in nanofiber filter media through pressure drop measurement at low-pressure conditions. Sep. Purif. Technol. 2016, 159, 100–107. [Google Scholar] [CrossRef]
- Leung, W.W.F.; Hung, C.H.; Yuen, P.T. Experimental investigation on continuous filtration of sub-micron aerosol by filter composed of dual-layers including a nanofiber layer. Aerosol Sci. Technol. 2009, 43, 1174–1183. [Google Scholar] [CrossRef]
- Lavoine, N.; Desloges, I.; Dufresne, A.; Bras, J. Microfibrillated cellulose—its barrier properties and applications in cellulosic materials: A review. Carbohydr. Polym. 2012, 90, 735–764. [Google Scholar] [CrossRef] [PubMed]
- Huang, P.; Zhao, Y.; Kuga, S.; Wu, M.; Huang, Y. A versatile method for producing functionalized cellulose nanofibers and their application. Nanoscale 2016, 8, 3753–3759. [Google Scholar] [CrossRef] [PubMed]
- Fan, B.; Chen, S.; Yao, Q.; Sun, Q.; Jin, C. Fabrication of cellulose nanofiber/ALOOH aerogel for flame retardant and thermal insulation. Materials 2017, 10, 311. [Google Scholar] [CrossRef] [PubMed]
- Ma, H.; Burger, C.; Hsiao, B.S.; Chu, B. Fabrication and characterization of cellulose nanofiber based thin-film nanofibrous composite membranes. J. Memb. Sci. 2014, 454, 272–282. [Google Scholar] [CrossRef]
- Choo, K.; Ching, Y.C.; Chuah, C.H.; Julai, S.; Liou, N.S. Preparation and characterization of polyvinyl alcohol-chitosan composite films reinforced with cellulose nanofiber. Materials 2016, 9, 644. [Google Scholar] [CrossRef] [PubMed]
- Abe, K.; Iwamoto, S.; Yano, H. Obtaining cellulose nanofibers with a uniform width of 15 nm from wood. Biomacromolecules 2007, 8, 3276–3278. [Google Scholar] [CrossRef] [PubMed]
- Zhang, L.; Tsuzuki, T.; Wang, X. Preparation of cellulose nanofiber from softwood pulp by ball milling. Cellulose 2015, 22, 1729–1741. [Google Scholar] [CrossRef]
- Jonoobi, M.; Mathew, A.P.; Oksman, K. Producing low-cost cellulose nanofiber from sludge as new source of raw materials. Ind. Crops. Prod. 2012, 40, 232–238. [Google Scholar] [CrossRef]
- Halib, N.; Perrone, F.; Cemazar, M.; Dapas, B.; Farra, R.; Abrami, M.; Chiarappa, G.; Forte, G.; Zanconati, F.; Pozzato, G.; et al. Potential applications of nanocellulose—Containing materials in the biomedical field. Materials 2017, 10, 977. [Google Scholar] [CrossRef] [PubMed]
- Iwamoto, S.; Lee, S.H.; Endo, T. Relationship between aspect ratio and suspension viscosity of wood cellulose nanofibers. Polym. J. 2014, 46, 73. [Google Scholar] [CrossRef]
- Siró, I.; Plackett, D. Microfibrillated cellulose and new nanocomposite materials: A review. Cellulose 2010, 17, 459–494. [Google Scholar] [CrossRef]
- Khalil, H.A.; Davoudpour, Y.; Islam, M.N.; Mustapha, A.; Sudesh, K.; Dungani, R.; Jawaid, M. Production and modification of nanofibrillated cellulose using various mechanical processes: A review. Carbohydr. Polym. 2014, 99, 649–665. [Google Scholar] [CrossRef] [PubMed]
- Chakraborty, A.; Sain, M.; Kortschot, M. Cellulose microfibrils: A novel method of preparation using high shear refining and cryocrushing. Holzforschung 2005, 59, 102–107. [Google Scholar] [CrossRef]
- Hubbe, M.A.; Rojas, O.J.; Lucia, L.A.; Sain, M. Cellulosic nanocomposites: A review. BioResources 2008, 3, 929–980. [Google Scholar]
- Schurz, J.; Lenz, J. Investigations on the structure of regenerated cellulose fibers. Macromol. Symp. 1994, 83, 273–389. [Google Scholar] [CrossRef]
- Crawshaw, J.; Bras, W.; Mant, G.R.; Cameron, R.E. Simultaneous SAXS and WAXS investigations of changes in native cellulose fiber microstructure on swelling in aqueous sodium hydroxide. J. Appl. Polym. Sci. 2002, 83, 1209–1218. [Google Scholar] [CrossRef]
- Crawshaw, J.; Cameron, R.E. A small angle X-ray scattering study of the pore structure in tencel cellulose fibres and effects on physical treatments. Polymer 2000, 41, 4691–4698. [Google Scholar] [CrossRef]
- Shibata, M.; Oyamada, S.; Kobayashi, S.I.; Yaginuma, D. Mechanical properties and biodegradability of green composites based on biodegradable polyesters and Lyocell fabric. J. Appl. Polym. Sci. 2004, 92, 3857–3863. [Google Scholar] [CrossRef]
- Lenz, J.; Schurz, J.; Wrentschur, E. Properties and structure of solvent-spun and viscose-type fibres in the swollen state. Colloid Polym. Sci. 1993, 271, 460–468. [Google Scholar] [CrossRef]
- Zhang, W.; Okubayashi, S.; Bechtold, T. Fibrillation tendency of cellulosic fibers—Part 3. effects of alkali pretreatment of Lyocell fiber. Carbohydr. Polym. 2005, 59, 173–179. [Google Scholar] [CrossRef]
- Öztürk, H.B.; Okubayashi, S.; Bechtold, T. Splitting tendency of cellulosic fibers—Part 1. the effect of shear force on mechanical stability of swollen Lyocell fibers. Cellulose 2006, 13, 393–402. [Google Scholar] [CrossRef]
- Yasumura, P.K.; DAlmeida, M.L.O.; Park, S.W. Multivariate statistical evaluation of physical properties of pulps refined in a PFI mill. O Papel. 2012, 73, 59–65. [Google Scholar]
- Tang, M.; Hu, J.; Liang, Y.; Pui, D.Y.H. Pressure drop, penetration and quality factor of filter paper containing nanofibers. Text. Res. J. 2017, 87, 498–508. [Google Scholar] [CrossRef]
- Choi, H.J.; Kumita, M.; Hayashi, S.; Yuasa, H.; Kamiyama, M.; Seto, T.; Tsai, C.J.; Otani, Y. Filtration properties of nanofiber/microfiber mixed filter and prediction of its performance. Aerosol Air Qual. Res. 2017, 17, 1052–1062. [Google Scholar] [CrossRef]
- Laboratory Beating of Pulp (PFI Mill Method), TAPPI T248 sp-15; TAPPI Press: Atlanta, GA, USA, 2015.
- Pulps-Determination of Drainability-Part 1: Schopper-Riegler Method, ISO 5267-1; International Organization for Standardization: Geneva, Switzerland, 1999.
- Pulps-Preparation of Laboratory Sheets for Physical Testing-Part 1: Conventional Sheet-Former Method, ISO 5269-1; International Organization for Standardization: Geneva, Switzerland, 2005.
- Thickness (Caliper) of Paper, Paperboard, and Combined Board, TAPPI T411 om-15; TAPPI Press: Atlanta, GA, USA, 2015.
- Standard Test Methods for Pore Size Characteristics of Membrane Filters by Bubble Point and Mean Flow Pore Test, ASTM F316-03; ASTM International: West Conshohocken, PA, USA, 2011.
- Thielke, J.F.; Charlson, R.J.; Winter, J.W.; Ahlquist, N.C.; Whitby, K.T.; Husar, R.B.; Liu, B.Y.H. Multiwavelength nephelometer measurements in los angeles smog aerosols. ii. correlation with size distributions, volume concentrations. J. Colloid Interface Sci. 1972, 39, 252–259. [Google Scholar] [CrossRef]
- Tang, M.; Chen, S.C.; Chang, D.Q.; Xie, X.; Sun, J.; Pui, D.Y.H. Filtration efficiency and loading characteristics of PM2.5 through composite filter media consisting of commercial HVAC electret media and nanofiber layer. Sep. Purif. Technol. 2017, 198, 137–145. [Google Scholar] [CrossRef]
- Road Vehicles-Inlet Air Cleaning Equipment for Internal Combustion Engines and Compressors-Part 1: Fractional Efficiency Testing with Fine Particles (0.3 µm to 5 µm Optical Diameter), ISO/TS 19713-1; International Organization for Standardization: Geneva, Switzerland, 2010.
- Schuster, K.C.; Aldred, P.; Villa, M.; Baron, M.; Loidl, R.; Biganska, O.; Patlazhan, S.; Navard, P.; Rüf, H.; Jerich, E. Characterising the emerging Lyocell fibres structures by ultra small angle neutron scattering (USANS). Lenzinger Berichte 2003, 82, 107–117. [Google Scholar]
- Ducos, F.; Biganska, O.; Schuster, K.C.; Navard, P. Influence of the Lyocell fibers structure on their fibrillation. Cellul. Chem. Technol. 2006, 40, 299–311. [Google Scholar]
- Hinds, W. Aerosol Technology: Properties, Behavior, and Measurement of Airborne Particles, 2nd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2012; pp. 187–188. [Google Scholar]
- Brown, R.C. Air Filtration: An Integrated Approach to the Theory and Applications of Fibrous Filters; Pergamon Press: Oxford, UK, 1993; pp. 73–116. [Google Scholar]
- Kirsch, A.A.; Stechkina, I.B. The theory of aerosol filtration with fibrous filters. In Fundamentals of Aerosol Science; Shaw, D.T., Ed.; John Wiley and Sons: Hoboken, NJ, USA, 1978; p. 165. [Google Scholar]
- Pich, J. Pressure drop of fibrous filters at small Knudsen numbers. Ann. Occup. Hyg. 1966, 9, 23–27. [Google Scholar] [CrossRef] [PubMed]


| Sample No. | Softwood Fiber | PET Fiber | Lyocell Nanofiber |
|---|---|---|---|
| #1 | 75% | 25% | 0 |
| #2 | 70% | 25% | 5% |
| #3 | 65% | 25% | 10% |
| #4 | 60% | 25% | 15% |
| #5 | 55% | 25% | 20% |
| Number of Revolutions | Beating Degree, °SR | Average Fiber Length, mm | Fine Fibers Content, % | Specific Surface Area, m2/g | Average Fiber Diameter df, µm |
|---|---|---|---|---|---|
| 0 | 13 | 4.00 ± 0.01 | 0 | - | - |
| 5000 | 18 | 3.16 ± 0.06 | 15.0 ± 0.2 | - | - |
| 10,000 | 31 | 2.66 ± 0.08 | 29.8 ± 1.1 | - | - |
| 20,000 | 58 | 1.47 ± 0.11 | 38.3 ± 1.2 | 2.42 ± 0.04 | 1.1 ± 0.09 |
| 40,000 | 79 | 1.31 ± 0.09 | 43.7 ± 1.5 | 3.33 ± 0.02 | 0.799 ± 0.06 |
| Filter Paper No. | Weight Fraction of Nanofibers | Basic Weight, g/m2 | Thickness, mm | Porosity | Mean Pore Size, µm |
|---|---|---|---|---|---|
| #1 | 0 | 104.3 ± 1.0 | 0.452 ± 0.012 | 0.843 ± 0.020 | 22.6 ± 0.5 |
| #2 | 5% | 104 ± 1.5 | 0.408 ± 0.010 | 0.827 ± 0.013 | 13.5 ± 0.3 |
| #3 | 10% | 103.6 ± 1.3 | 0.415 ± 0.009 | 0.830 ± 0.023 | 10.2 ± 0.3 |
| #4 | 15% | 103.6 ± 1.6 | 0.400 ± 0.010 | 0.824 ± 0.031 | 6.1 ± 0.2 |
| #5 | 20% | 104 ± 0.9 | 0.390 ± 0.011 | 0.819 ± 0.021 | 5.0 ± 0.2 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Long, J.; Tang, M.; Liang, Y.; Hu, J. Preparation of Fibrillated Cellulose Nanofiber from Lyocell Fiber and Its Application in Air Filtration. Materials 2018, 11, 1313. https://doi.org/10.3390/ma11081313
Long J, Tang M, Liang Y, Hu J. Preparation of Fibrillated Cellulose Nanofiber from Lyocell Fiber and Its Application in Air Filtration. Materials. 2018; 11(8):1313. https://doi.org/10.3390/ma11081313
Chicago/Turabian StyleLong, Jin, Min Tang, Yun Liang, and Jian Hu. 2018. "Preparation of Fibrillated Cellulose Nanofiber from Lyocell Fiber and Its Application in Air Filtration" Materials 11, no. 8: 1313. https://doi.org/10.3390/ma11081313
APA StyleLong, J., Tang, M., Liang, Y., & Hu, J. (2018). Preparation of Fibrillated Cellulose Nanofiber from Lyocell Fiber and Its Application in Air Filtration. Materials, 11(8), 1313. https://doi.org/10.3390/ma11081313




