A Review of Mechanoluminescence in Inorganic Solids: Compounds, Mechanisms, Models and Applications
Abstract
1. Introduction
2. Basic Concepts
2.1. Symmetry and Tensor Properties in Crystals
2.2. Dynamic Deformation and Its Consequences
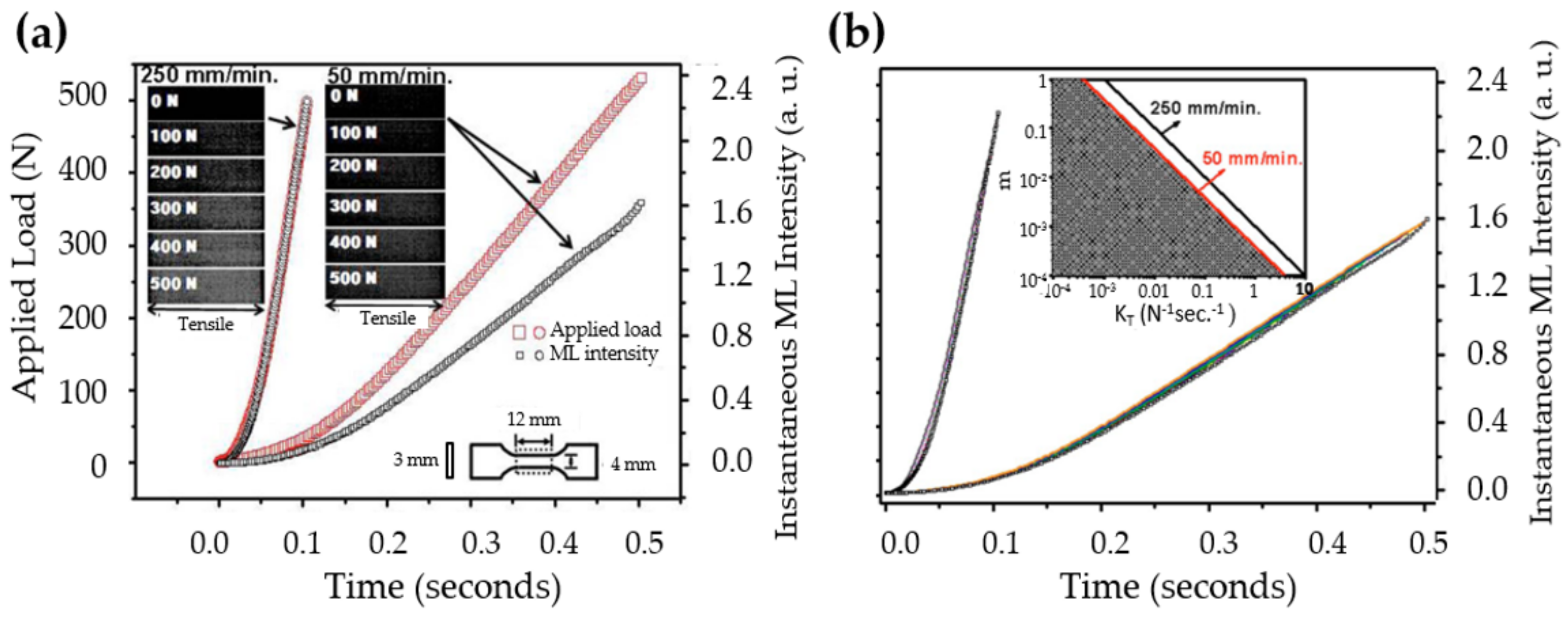
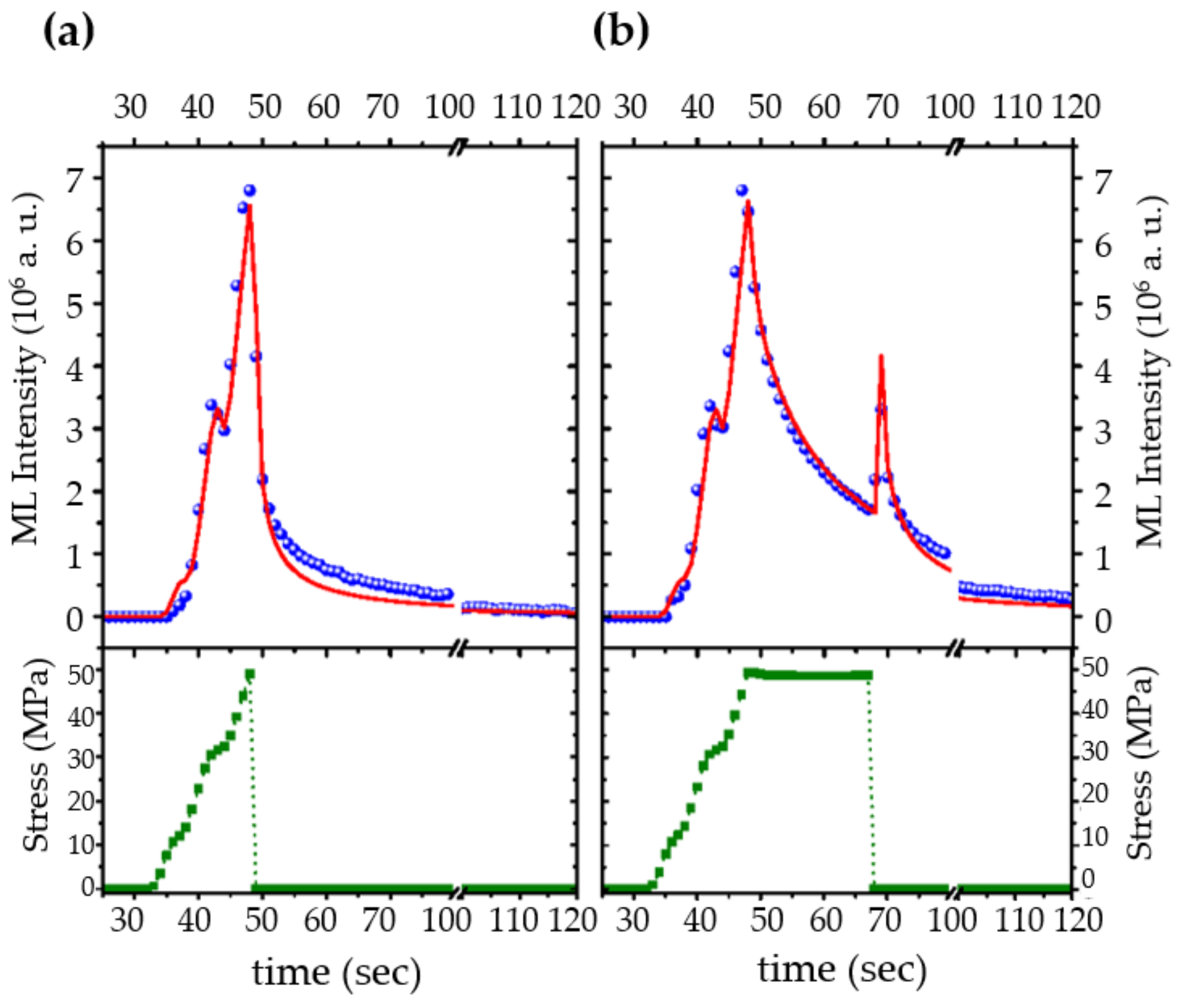
2.3. Measurement and Performance of ML Materials
3. Mechanoluminescent Compounds
3.1. Known ML Compounds
3.2. Crystal Structures and Their Relation to ML
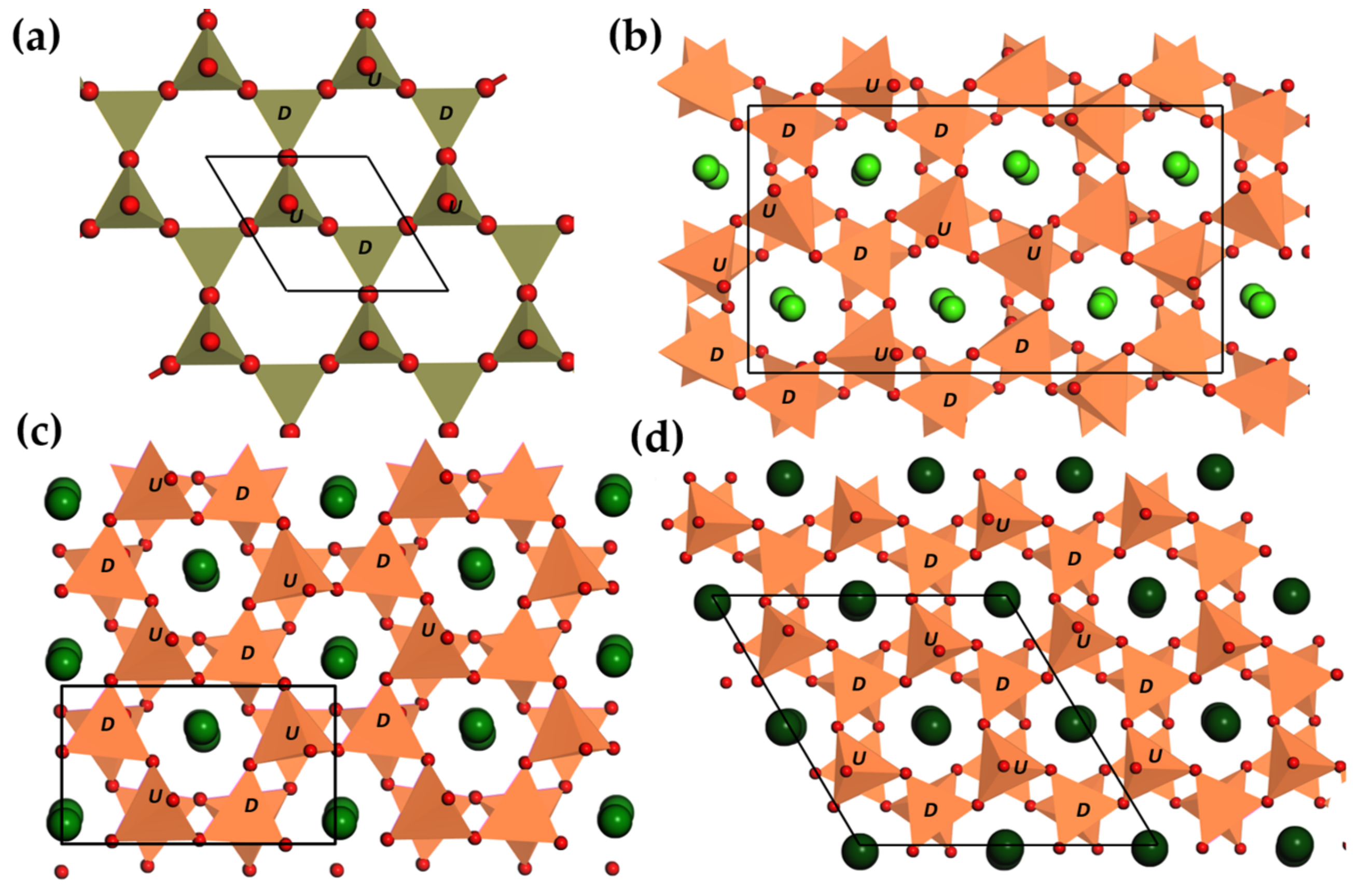
3.2.1. Rock Salt and Wurtzite-Related Compounds
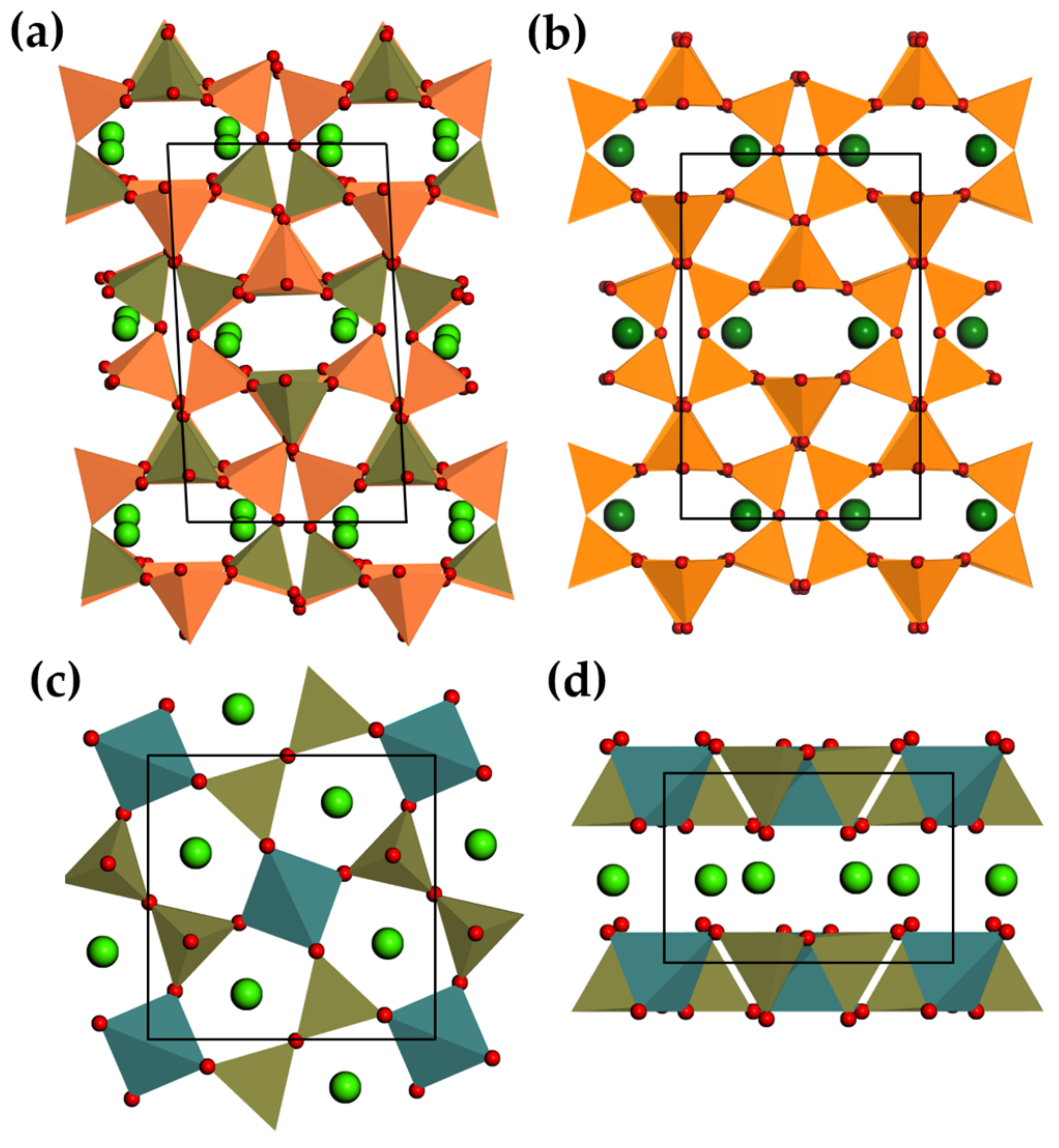
3.2.2. Tridymites
3.2.3. Anorthite and Melilites
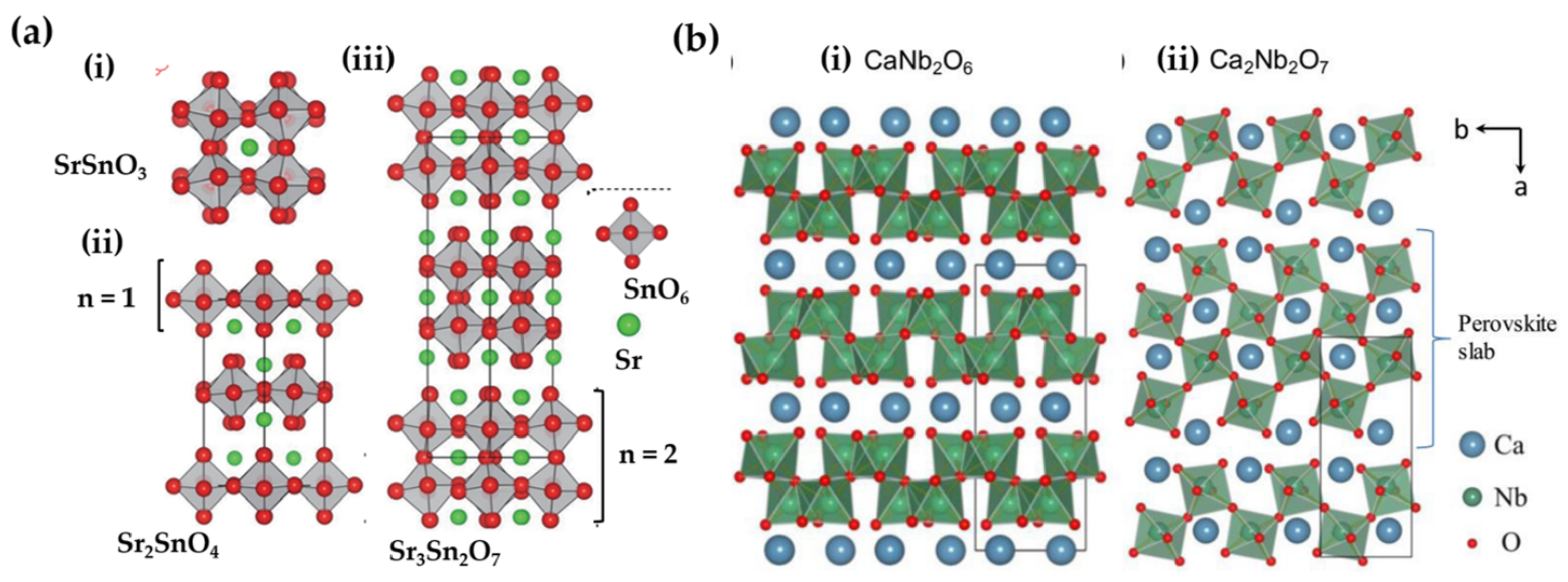
3.2.4. Perovskite Related Compounds
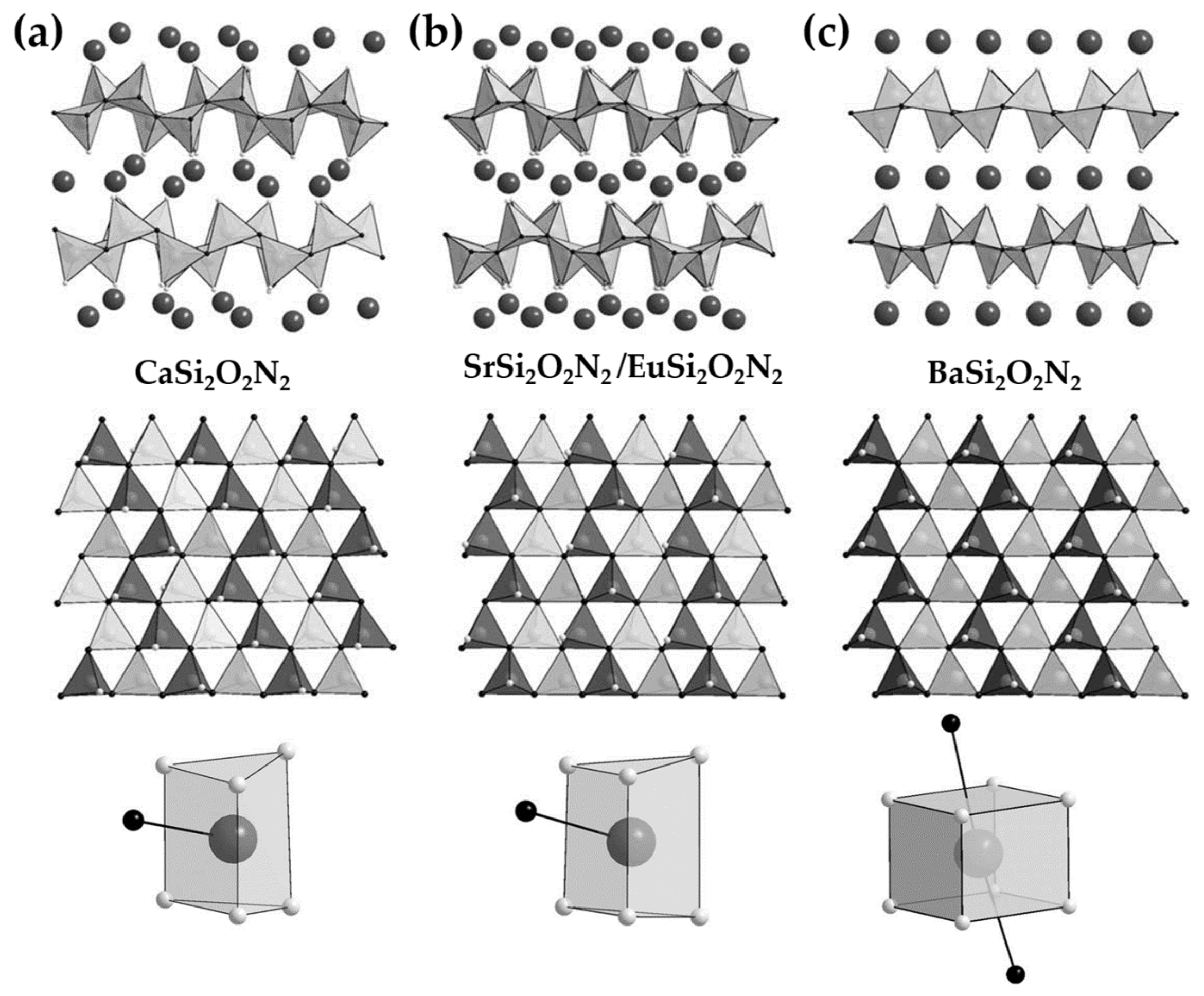
3.2.5. MSiON: Eu (M = Ba, Sr, Ca) and Other Compounds
3.3. Microstructures
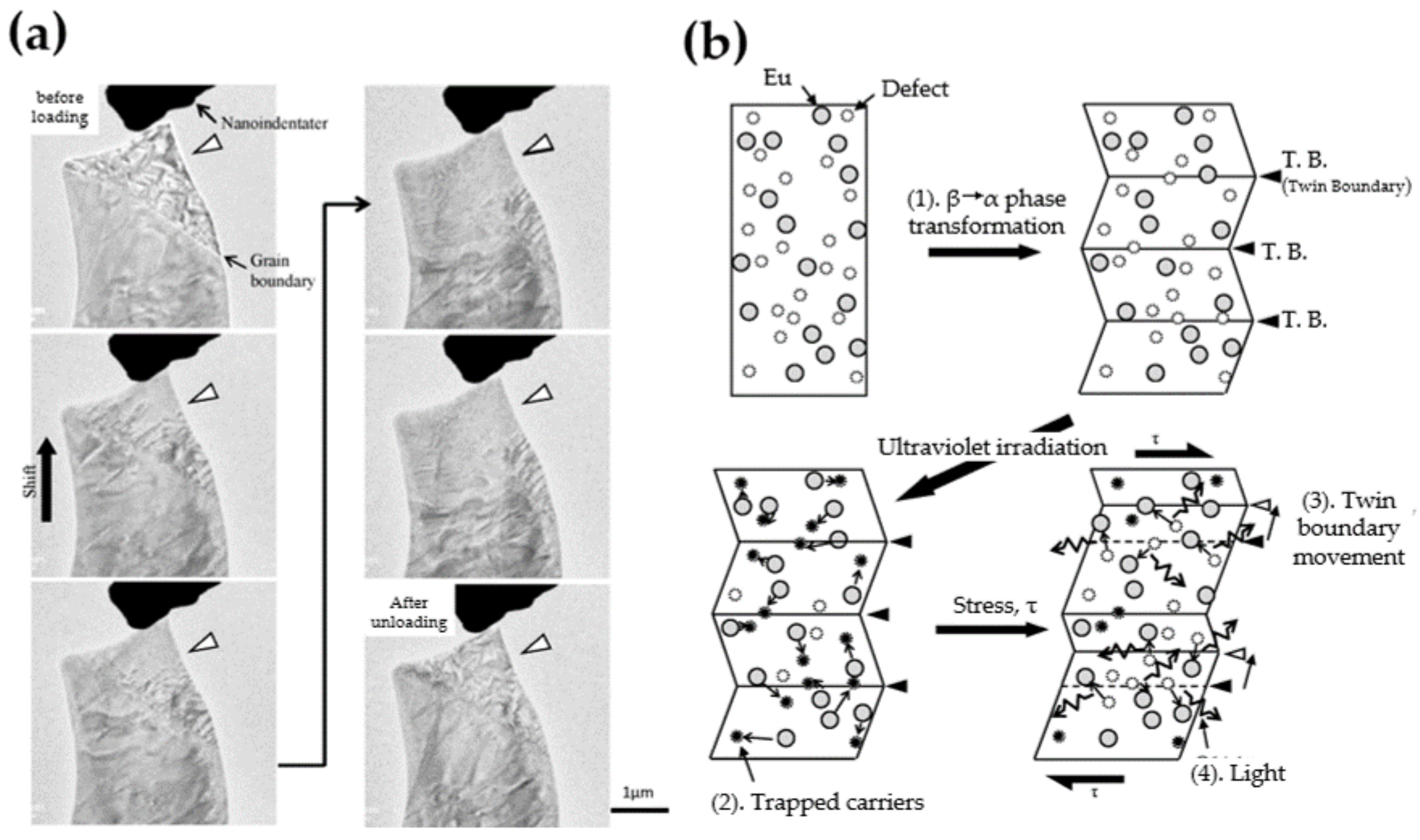
3.3.1. Structural Phase Transitions and Their Consequences
3.3.2. Modulated Structure and Chemical Gradients
3.4. General Remarks
4. Proposed Mechanism and Models
4.1. Mechanism 1: Piezoelectrically Induced Detrapping by Reducing Trap Depth
- i
- upon photo-excitation, 4f electrons of Eu ions are lifted to 5d levels and subsequently escape to the conduction band, leading to Eu ions being oxidized to Eu;
- ii
- the created electrons in the conduction band are trapped at defect centres, e.g., vacancies , co-dopants R, or other defects;
- iii
- when stress is loaded, the depth of traps is reduced due to the piezoelectric field, leading to the detrapping of electrons (to the conduction band);
- iv
- the released electron is captured by Eu, which in turn reduces to an excited ion Eu;
- v
- de-excitation of the excited Eu ions provides emission of light.
4.1.1. The Rate Equation Method
4.1.2. Viscoelasticity Method
4.2. Mechanism 2: Carrier Release by the Electric Field Produced by Domain Structures
- i
- electrons are photo-ionized from Eu ions and trapped at defects in SrAlO:Eu.
- ii
- upon the mechanical load, the twin boundaries show a pseudo-elastic deformation and creates an electric field around the boundary to release the trapped electrons.
- iii
- the electrons are captured by Eu, which turn into Eu, and the de-excitation to the ground state of Eu yields the emission of light.
4.3. Mechanism 3: Carrier Release by Movement of Dislocation
4.4. Remarks
5. Proven and Potential Applications
5.1. Visualization of Stress Distribution
5.2. Visualization of Ultrasonic Fields
5.3. Light Sources and Displays
5.4. Sensing Other Fields
5.5. Design Consideration for ML Phosphors
6. Conclusions and Outlooks
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A. Stress Distribution of a Disc Under a Pair of Diametrical Compressive Load


Appendix B. Influence of Point Group on the Components of Piezoelectric Tensor and Piezo-Optical Tensor
Appendix C. An Estimation of Piezoelectric Field in ZnS Crystallite
References
- Bacon, F. Fourth Book. In The Advancement of Learning, 1st ed.; Devey, J., Ed.; Press of P. F Collier & Son: New York, NY, USA, 1901; pp. 208–209. [Google Scholar]
- Harvey, K.N. Triboluminescence, Piezoluminescence, Crystalloluminescence and Lyoluminescence. In A History of Luminescence—From Earliest Times Until 1900, 1st ed.; Harvey, K.-N., Ed.; The American Philosophical Society: Philadelphia, PA, USA, 1957; pp. 378–379. [Google Scholar]
- Chandra, B.P. Mechanoluminescence. In Luminescence of Solids, 1st ed.; Vlj, O.-R., Ed.; Springer Science+Business Media, LLC: New York, NY, USA, 1998; pp. 361–387. ISBN 978-1-4613-7446-6. [Google Scholar]
- Sweeting, L.M. Triboluminescence with and without air. Chem. Mater. 2001, 13, 854–870. [Google Scholar] [CrossRef]
- Jha, P.; Chandra, B.P. Survey of the literature on mechanoluminescence from 1605 to 2013. Luminescence 2014, 29, 977–993. [Google Scholar] [CrossRef] [PubMed]
- Kricka, L.J.; Stroebe, l.J.; Stanley, P.E. Triboluminescence: 1968–1998. Luminescence 1999, 14, 215–220. [Google Scholar] [CrossRef]
- Walton, A.J. Triboluminescence. Adv. Phys. 1977, 26, 887–948. [Google Scholar] [CrossRef]
- Sage, I.; Bourhill, G. Triboluminescent materials for structural damage monitoring. J. Mater. Chem. 2001, 11, 231–245. [Google Scholar] [CrossRef]
- Xu, C.-N.; Watanabe, T.; Akiyama, M.; Zheng, X.-G. Direct view of stress distribution in solid by mechanoluminescence. Appl. Phys. Lett. 1999, 74, 2414–2416. [Google Scholar] [CrossRef]
- Xu, C.-N.; Watanabe, T.; Akiyama, M.; Zheng, X.-G. Artificial skin to sense mechanical stress by visible light emission. Appl. Phys. Lett. 1999, 74, 1236–1238. [Google Scholar] [CrossRef]
- Zhang, H.; Terasaki, N.; Yamada, H.; Xu, C.-N. Mechanoluminescence of Europium-doped SrAMgSi2O7 (A = Ca, Sr, Ba). Jpn. J. Appl. Phys. 2009, 48, 04C109. [Google Scholar] [CrossRef]
- Xu, C.-N.; Zheng, X.-G.; Akiyama, M.; Nonaka, K.; Watanabe, T. Dynamic visualization of stress distribution by mechanoluminescence image. Appl. Phys. Lett. 2000, 76, 179–181. [Google Scholar] [CrossRef]
- Chen, L.; Wong, M.-C.; Bai, G.; Jie, W.; Hao, J. White and green light emissions of flexible polymer composites under electric field and multiple strains. Nano Energy 2015, 14, 372–381. [Google Scholar] [CrossRef]
- Kim, J.S.; Kim, G.-W. New non-contacting torque sensor based on the mechanoluminescence of ZnS:Cu microparticles. Sens. Actuators A Phys. 2014, 218, 125–131. [Google Scholar] [CrossRef]
- Kim, G.-W.; Kim, J.-S. Dynamic torsional response analysis of mechanoluminescent paint and its application to non-contacting automotive torque transducers. Meas. Sci. Technol. 2014, 25, 015009. [Google Scholar] [CrossRef]
- Kersemans, M.; Smet, P.F.; Lammens, N.; Degrieck, J.; Van Paepegem, W. Fast reconstruction of a bounded ultrasonic beam using acoustically induced piezoluminescence. Appl. Phys. Lett. 2015, 107, 234102. [Google Scholar] [CrossRef]
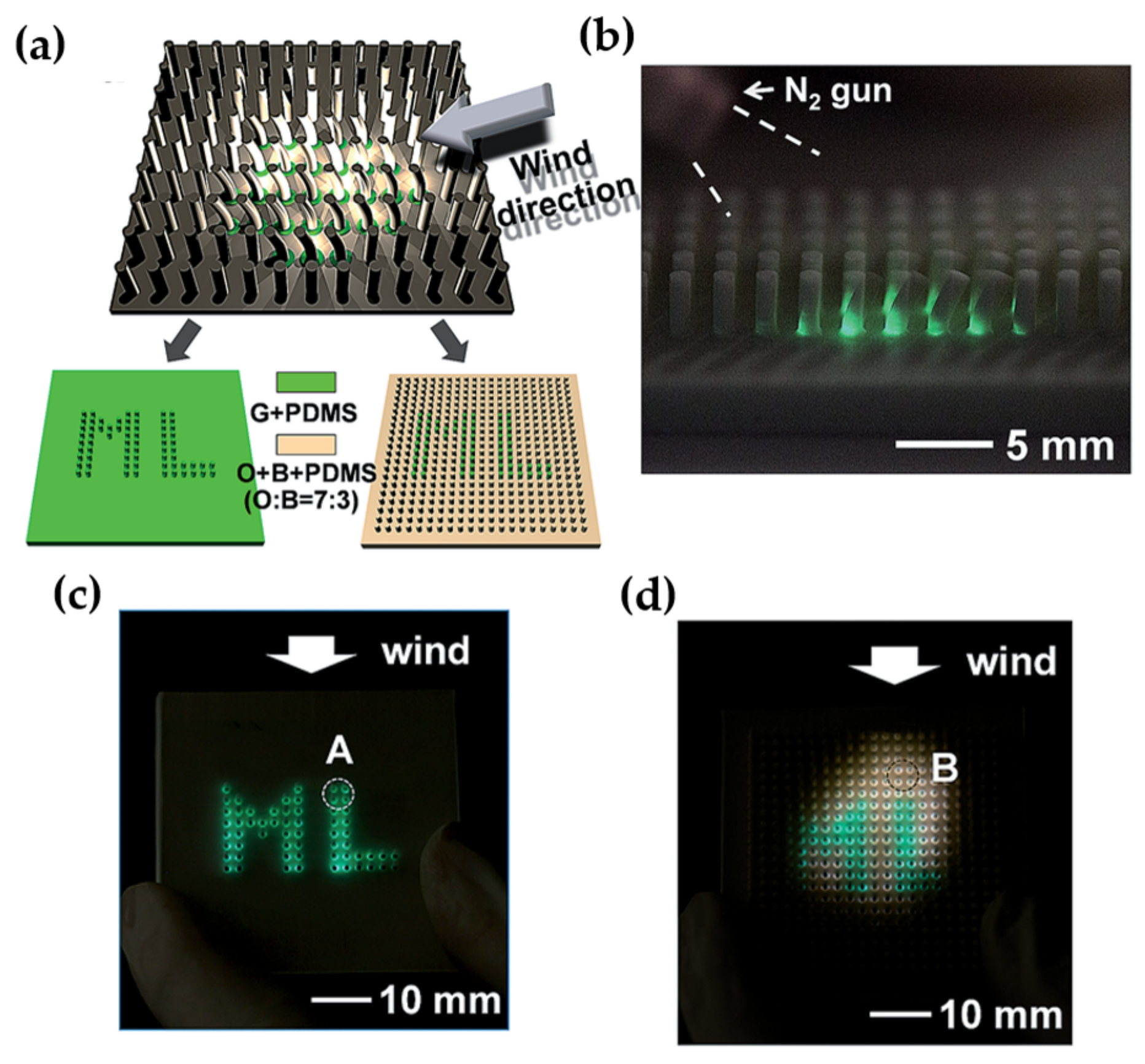
- Jeong, S.M.; Song, S.; Joo, K.-I.; Kim, J.; Hwang, S.-H.; Jeong, J.; Kim, H. Bright, Wind-driven white mechanoluminescence from zinc sulphide microparticles embedded in a polydimethylsiloxane elastomer. Energy. Environ. Sci. 2014, 7, 3338–3346. [Google Scholar] [CrossRef]
- Kim, J.S.; Koh, H.J.; Lee, W.D.; Shin, N.; Kim, J.G.; Lee, K.H.; Sohn, K.S. Quasi-dynamic visualization of crack propagation and wake evolution in Y-TZP ceramic by mechano-luminescence. Met. Mater. Int. 2008, 14, 165–169. [Google Scholar] [CrossRef]
- Timilsina, S.; Lee, K.H.; Jang, I.Y.; Kim, J.S. Mechanoluminescent determination of the mode I stress intensity factor in SrAl2O4:Eu2+, Dy3+. Acta Mater. 2013, 61, 7197–7206. [Google Scholar] [CrossRef]
- Timilsina, S.; Lee, K.H.; Kwon, Y.N.; Kim, J.S. Optical evaluation of in situ crack propagation by using mechanoluminescence of SrAl2O4:Eu2+, Dy3+. J. Am. Ceram. Soc. 2015, 98, 2197–2204. [Google Scholar] [CrossRef]
- Kim, J.S.; Kwon, Y.-N.; Shin, N.; Sohn, K.-S. Mechanoluminescent SrAl2O4:Eu, Dy phosphor for use in visualization of quasidynamic crack propagation. Appl. Phys. Lett. 2007, 90, 241916. [Google Scholar] [CrossRef]
- Wong, M.-C.; Chen, L.; Tsang, M.-K.; Zhang, Y.; Hao, J. Magnetic-induced luminescence from flexible composite laminates by coupling magnetic field to piezophotonic effect. Adv. Mater. 2015, 27, 4488–4495. [Google Scholar] [CrossRef] [PubMed]
- Akiyama, M.; Xu, C.-N.; Matsui, H.; Nonaka, K.; Watanabe, T. Recovery phenomenon of mechanoluminescence from Ca2Al2SiO7:Ce by irradiation with ultraviolet light. Appl. Phys. Lett. 1999, 75, 2548–2550. [Google Scholar] [CrossRef]
- Carpenter, M.A.; Howard, C.J.; Andrew, M.J.; McKnight, R.E.A.; Liu, Y.; Withers, R.L. Elastic anomalies due to structural phase transitions in mechanoluminescent SrAl2O4:Eu. J. Appl. Phys. 2010, 107, 013505. [Google Scholar] [CrossRef]
- Van den Eeckhout, K.; Smet, P.F.; Poelman, D. Persistent luminescence in Eu2+-doped compounds: A review. Materials 2010, 3, 2536–2566. [Google Scholar] [CrossRef]
- Van den Eeckhout, K.; Poelman, D.; Smet, P.F. Persistent luminescence in non-Eu2+-doped compounds: A review. Materials 2013, 6, 2789–2818. [Google Scholar] [CrossRef] [PubMed]
- Smet, P.F.; Viana, B.; Tanabe, S.; Peng, M.; Hölsä, J.; Chen, W. Feature issue introduction: persistent and photostimulable phosphors—An established research field with clear challenges ahead. Opt. Mater. Express 2016, 6, 1414–1419. [Google Scholar] [CrossRef]
- Chandra, V.K.; Chandra, B.P.; Jha, P. Models for intrinsic and extrinsic elastico and plastico-mechanoluminescence of solids. J. Lumin. 2013, 138, 267–280. [Google Scholar] [CrossRef]
- Zhang, J.-C.; Long, Y.-Z.; Yan, X.; Wang, X.; Wang, F. Creating recoverable mechanoluminescence in piezoelectric calcium niobates through Pr3+ doping. Chem. Mater. 2016, 28, 4052–4057. [Google Scholar] [CrossRef]
- Matsuo, H.; Ikeda, K.; Hata, S.; Nakashima, H.; Yamada, H.; Xu, C.-N. Phase transformation behavior and pseudoelastic deformation in SrAl2O4. J. Alloys Compd. 2013, 577, S507–S516. [Google Scholar] [CrossRef]
- Peng, D.; Chen, B.; Wang, F. Recent advances in doped mechanoluminescent phosphors. ChemplusChem 2015, 80, 1029–1215. [Google Scholar] [CrossRef]
- Timilsina, S.; Kim, J.S.; Kim, J.; Kim, G.-W. Review of state-of-the-art sensor applications using mechanoluminescence microparticles. Int. J. Precis. Eng. Man. 2016, 17, 1237–1247. [Google Scholar] [CrossRef]
- Bünzli, J.-C.G.; Wong, K.-L. Lanthanide mechanoluminescence. J. Rare Earth. 2018, 36, 1–41. [Google Scholar] [CrossRef]
- Janssen, T.; Janner, A.; Looijenga-Vos, A.; De Wolff, P.M. Incommensurate and commensurate modulated structures. In International Tables for Crystallography, 3rd ed.; Prince, E., Ed.; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2006; Volume C, pp. 907–955. ISBN 1-4020-1900-9. [Google Scholar]
- Neumann, F.E. Vorlesungen über die Theorie der Elastizität der festen Körper und des Lichtähers, 1st ed.; B. G. Teubner-Verlag: Leipzig, Germany, 1885. [Google Scholar]
- Jeevanjee, N. An Introduction to Tensors and Group Theory for Physicists, 1st ed.; Springer Science+Business Media, LLC: New York, NY, USA, 2011; pp. 3–81. ISBN 978-0-8176-4714-8. [Google Scholar]
- Authier, A. Introduction to the properties of tensors. In International Tables for Crystallography, 1st ed.; Authier, A., Ed.; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2003; Volume D, pp. 3–32. ISBN 1-4020-0714-0. [Google Scholar]
- Fumi, F.G. Physical properties of crystals: The direct-inspection method. Acta Crystallogr. 1952, 5, 44–48. [Google Scholar] [CrossRef]
- Dresselhaus, M.S.; Dresselhaus, G.; Jorio, A. Group Theory: Application to the Physics of Condensed Matter, 1st ed.; Springer-Verlag: Berlin/Heidelberg, Germany, 2008; pp. 455–479. ISBN 978-3-540-32897-1. [Google Scholar]
- Vanderbilt, D.; King-Smith, R.D. Electric polarization as a bulk quantity and its relations to surface charge. Phys. Rev. B 1993, 48, 4442–4455. [Google Scholar] [CrossRef]
- Nye, J.F. Physical Properties of Crystals: Their Representation by Tensors and Matrices, 1st ed.; Clarendon Press: Oxford, UK, 1985; pp. 68–81. ISBN 0-19-851165-5. [Google Scholar]
- Lines, M.E.; Glass, A.M. Principles and Applications of Ferroelectrics and Related Materials, 1st ed.; Clarendon Press: Clarendon, UK, 1977; pp. 8–15. ISBN 0-19-851286-4. [Google Scholar]
- Jiang, X.; Huang, W.; Zhang, S. Flexoelectric nano-generator: Materials structures and devices. Nano Energy 2013, 2, 1079–1092. [Google Scholar] [CrossRef]
- Salje, E.K.H. Phase Transitions in Ferroelastic and Co-elastic Crystals; Cambridge University Press: Cambridge, UK, 1993; pp. 1–12. ISBN 978-0-521-42936-8. [Google Scholar]
- Nye, J.F. Physical Properties of Crystals: Their Representation by Tensors and Matrices, 1st ed.; Clarendon Press: Oxford, UK, 1985; pp. 235–273. ISBN 0-19-851165-5. [Google Scholar]
- Green, A.E.; Zerna, W. Theoretical Elasticity, 2nd ed.; Dover Publications, Inc.: New York, NY, USA, 1968; pp. 53–76. ISBN 0-486-67076-7. [Google Scholar]
- Arfken, G.B.; Weber, H.J.; Harris, F.E. Mathematical Methods for Physicists, 7th ed.; Academic Press: Waltham, MA, USA, 2013; pp. 205–249. ISBN 78-0-12-384654-9. [Google Scholar]
- Chen, Z.-D. Geometric field theory of finite deformation in continuum mechanics. Chin. J. Theor. Appl. Mech. 1979, 15, 107–117. [Google Scholar] [CrossRef]
- Noll, W. A mathematical theory of the mechanical behavior of continuous media. Arch. Ration. Mech. Anal. 1958, 2, 197–226. [Google Scholar] [CrossRef]
- Xiao, J. Geometric Field Theory of Strain, 1st ed.; Science Press: Beijing, China, 2017; pp. 136–160. ISBN 978-7-03-050711-2. [Google Scholar]
- Xiao, J. Evolution of Continuum from Elastic Deformation to Flow. arXiv, 2005; arXiv:physics/0511170. [Google Scholar]
- Lakes, R. Viscoelastic Materials, 1st ed.; Cambridge University Press: Cambridge, UK, 2009; pp. 14–52. ISBN 978-0-521-88568-3. [Google Scholar]
- Boltzmann, L. Zur Theorie der elastischen Nachwirkung. Wiener Berichte 1874, 70, 275–306. [Google Scholar]
- Xiao, J. Theoretic discussion on rate-dependent constitutive equations. In Advance in Rheology Research in China, 1st ed.; Zhejiang University Press: Hangzhou, China, 2010; pp. 26–30. ISBN 9787308080545. [Google Scholar]
- Smith, R.C.; Seelecke, S.; Dapino, M.; Ounaies, Z. A unified framework for modelling hysteresis in ferroic materials. J. Mech. Phys. Solids 2006, 45, 46–85. [Google Scholar] [CrossRef]
- Lakes, R. Viscoelastic Materials, 1st ed.; Cambridge University Press: New York, NY, USA, 2009; pp. 3–5. ISBN 978-0-521-88568-3. [Google Scholar]
- Kamimura, S.; Yamada, H.; Xu, C.-N. Development of new elasticoluminescent material SrMg2(PO4)2:Eu. J. Lumin. 2012, 132, 526–530. [Google Scholar] [CrossRef]
- Sakai, K.; Koga, T.; Imai, Y.; Maehara, S.; Xu, C.-N. Observation of mechanically induced luminescence from microparticles. Phys. Chem. Chem. Phys. 2006, 8, 2819–2822. [Google Scholar] [CrossRef] [PubMed]
- Persits, N.; Aharoni, A.; Tur, M. Quantitative characterization of ZnS:Mn embedded polyurethane optical emission in three mechanoluminescent regimes. J. Lumin. 2017, 181, 467–476. [Google Scholar] [CrossRef]
- Van der Heggen, D.; Joos, J.J.; Burbano, D.C.R.; Capobianco, J.A.; Smet, P.F. Counting the photons: Determining the absolute storage capacity of persistent phosphors. Materials 2017, 10, 867. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Gecevicius, M.; Qiu, J. Long persistent phosphors—From fundamentals to applications. Chem. Soc. Rev. 2016, 45, 2090–2136. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Q.Y.; Huang, X.Y. Recent progress in quantum cutting phosphors. Prog. Mater Sci. 2010, 55, 352–427. [Google Scholar] [CrossRef]
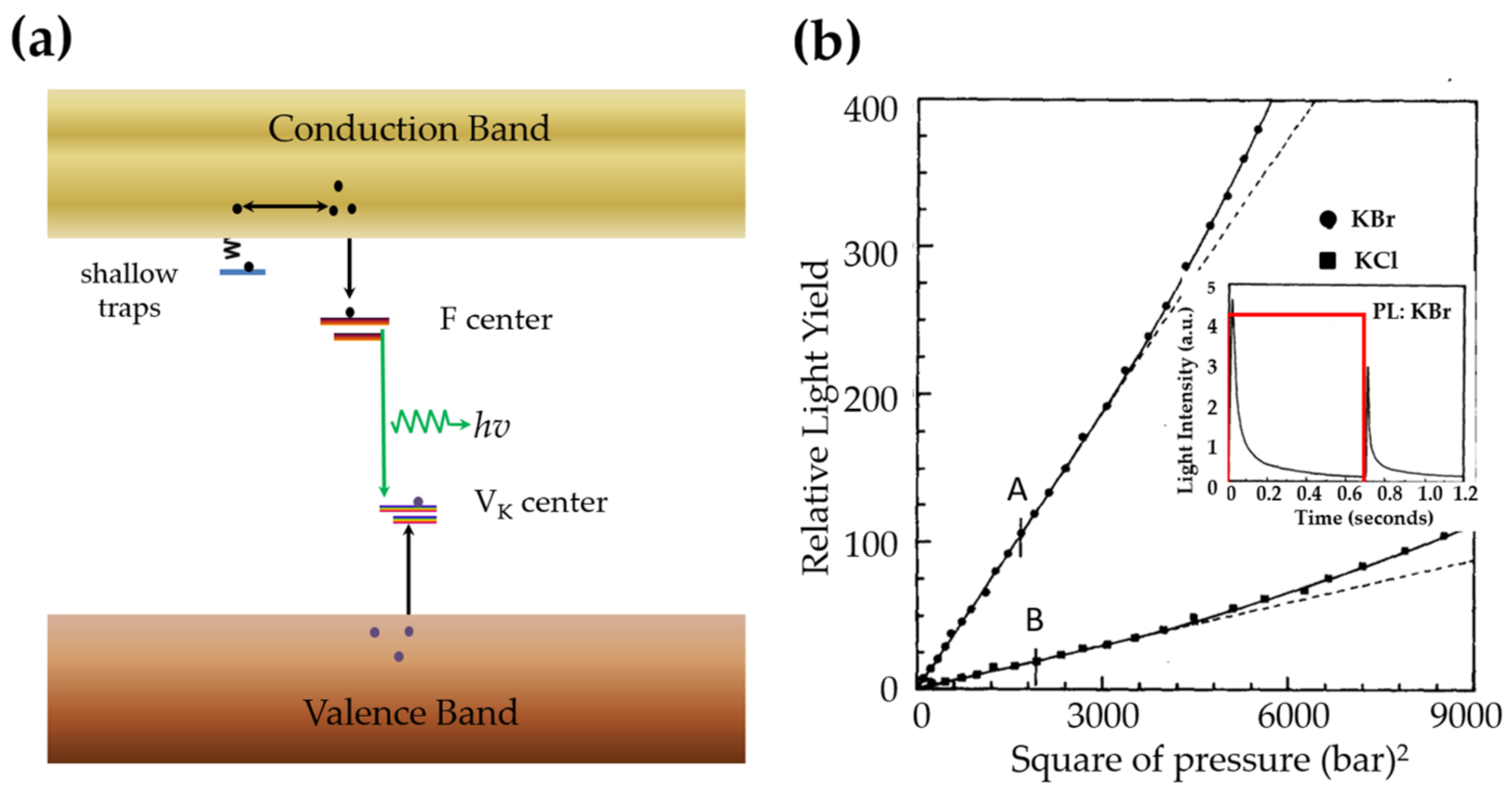
- Chandra, B.P.; Baghel, R.N.; Singh, P.K.; Luka, A.K. Deformation-induced excitation of the luminescence centres in coloured alkali halide crystals. Radiat Eff. Defects Solids 2009, 164, 500–507. [Google Scholar] [CrossRef]
- Butler, C.T. Room-temperature deformation luminescence in alkali halides. Phys. Rev. 1966, 141, 750–757. [Google Scholar] [CrossRef]
- Bose, H.N. Thermoluminescence of alkali halide phosphors. Proc. Phys. Soc. B 1954, 68, 249–252. [Google Scholar] [CrossRef]
- Atari, N.A. Piezoluminescence phenomenon. Phys. Lett. 1982, 90, 93–96. [Google Scholar] [CrossRef]
- Hagihara, T.; Hayashiuchi, Y.; Kojima, Y.; Yamamoto, Y.; Ohwaki, S.; Okada, T. Deformation luminescence in gamma-irradiated alkali halides. Phys. Lett. A 1989, 137, 213–216. [Google Scholar] [CrossRef]
- Alvarez-García, S.; Piters, T.M. Participation of Eu-aggregates in the photoluminescence, afterglow and thermoluminescence of UV-irradiated KCl:Eu at 20 K. J. Phys. Condens. Matter 2005, 17, 181–188. [Google Scholar] [CrossRef] [PubMed]
- Nakamura, S.; Kawaguchi, K.; Ohgaku, T. Deformation luminescence of X-irradiated KCl:Eu2+ by bending test. IOP Conf. Ser. Mater. Sci. Eng. 2009, 3, 012022. [Google Scholar] [CrossRef]
- Sharma, J. Thermoluminescence properties of some alkali halides. Phys. Rev. 1956, 101, 1295–1297. [Google Scholar] [CrossRef]
- Gartia, R.K.; Rey, L.; Singh, T.T.; Singh, T.B. Thermoluminescence of alkali halides and its implications. Nucl. Instrum. Methods Phys. Res. B 2012, 274, 129–134. [Google Scholar] [CrossRef]
- Molotskii, M.I.; Shmurak, S.Z. Elementary acts of deformation luminescence. Phys. Lett. A 1992, 166, 286–291. [Google Scholar] [CrossRef]
- Sastry, S.B.S.; Balasubramany, K. Thermoluminescence of irradiated RbCl and RbCI:Sn crystals. Phys. Status Solidi B 1978, 90, 375. [Google Scholar] [CrossRef]
- Sastry, S.B.S.; Sapru, S. Thermoluminescence glow and emission studies on suprapure rubidium bromide irradiated at room temperature. Phys. Status Solidi B 1979, 94, K149–K154. [Google Scholar] [CrossRef]
- Shao, Q.; Tolner, H.; Li, H.; Kuang, Y.; Li, Q.; Dong, Y.; Jiang, J. P-146: Thermoluminescence spectra of MgO poly-crystals exposed to vacuum ultraviolet (VUV) radiation. SID Symp. Dig. Tech. 2012, 39, 1756–1758. [Google Scholar] [CrossRef]
- Dickinson, J.T.; Scudiero, L.; Yasuda, K.; Kim, M.-W.; Langford, S.C. Dynamic tribological probes: Particle emission and transient electrical measurements. Tribol. Lett. 1997, 3, 53–67. [Google Scholar] [CrossRef]
- Matsuzawa, T.; Aoki, Y.; Takeuchi, N.; Murayama, Y. A new long phosphorescent phosphor with high brightness SrAl2O4:Eu2+, Dy3+. J. Electrochem. Soc. 1996, 143. [Google Scholar] [CrossRef]
- Katsumata, T.; Nabae, T.; Sasajima, K.; Komuro, S.; Morikawa, T. Effects of composition on the long phosphorescent SrAl2O4:Eu2+, Dy3+ phosphor crystals. J. Electrochem. Soc. 1997, 144, L243–L245. [Google Scholar] [CrossRef]
- Fu, X.; Yamada, H.; Xu, C.-N. Triboluminescence properties of highly oriented SrAl2O4:Eu2+ films on Inconel 600 substrate. Electrochem. Solid-State Lett. 2008, 11, J27–J30. [Google Scholar] [CrossRef]
- Xu, C.-N.; Yamada, H.; Wang, X.S.; Zheng, X.-G. Strong elasticoluminescence from monoclinic-structure SrAl2O4. Appl. Phys. Lett. 2004, 84, 3040–3042. [Google Scholar] [CrossRef]
- Yun, G.J.; Rahimi, M.R.; Gandomi, A.H.; Lim, G.-C.; Choi, J.-S. Stress sensing performance using mechanoluminescence of SrAl2O4:Eu (SAOE) and SrAl2O4:Eu, Dy (SAOED) under mechanical loadings. Smart Mater. Struct. 2013, 22, 055006. [Google Scholar] [CrossRef]
- Jia, Y.; Yei, M.; Jia, W.Y. Stress-induced mechanoluminescence in SrAl2O4:Eu2+, Dy3+. Opt. Mater. 2006, 28, 974–979. [Google Scholar] [CrossRef]
- Katsumata, T.; Toyomane, S.; Sakai, R.; Komuro, S.; Morikawa, T. Trap levels in Eu-doped SrAl2O4 phosphor crystals co-doped with rare earth elements. J. Am. Ceram. Soc. 2006, 89, 932–936. [Google Scholar] [CrossRef]
- Terasawa, Y.; Xu, C.-N.; Yamada, H.; Kubo, M. Near infra-red mechanoluminescence from strontium aluminate doped with rare-earth ions. IOP Conf. Ser. Mater. Sci. Eng. 2011, 18, 212013. [Google Scholar] [CrossRef]
- Jia, D. Relocalization of Ce3+ 5d electrons from host conduction band. J. Lumin. 2006, 117, 170–178. [Google Scholar] [CrossRef]
- Jia, D.; Wang, X.-J.; Jia, W.; Yen, W.M. Trapping processes of 5d electrons in Ce3+ doped SrAl2O4. J. Lumin. 2007, 122, 311–314. [Google Scholar] [CrossRef]
- Zhang, H.; Yamada, H.; Terasaki, N.; Xu, C.-N. Ultraviolet mechanoluminescence from SrAl2O4:Ce and SrAl2O4:Ce, Ho. Appl. Phys. Lett. 2007, 91, 081905. [Google Scholar] [CrossRef]
- Sohn, K.-S.; Seo, S.Y.; Park, H.D. Search for long phosphorescence materials by combinatorial chemistry method. Electrochem. Solid-State Lett. 2001, 4, H26–H29. [Google Scholar] [CrossRef]
- Sohn, K.-S.; Seo, S.Y.; Kwon, Y.N.; Park, H.D. Direct observation of crack tip stress field using the mechanoluminescence of SrAl2O4:(Eu, Dy, Nd). J. Am. Ceram. Soc. 2002, 85, 712–714. [Google Scholar] [CrossRef]
- Kim, J.S.; Kwon, Y.-N.; Sohn, K.-S. Dynamic visualization of crack propagation and bridging stress using the mechano-luminescence of SrAl2O4:(Eu, Dy, Nd). Acta Mater. 2003, 51, 6437–6442. [Google Scholar] [CrossRef]
- Katsumata, T.; Nabae, T.; Matsuzawa, K.S.T. Growth and characteristics of long persistent SrAl2O4-and CaAl2O4-based phosphor crystals by a floating zone technique. J. Cryst. Growth 1998, 183, 361–365. [Google Scholar] [CrossRef]
- Aitasalo, T.; Hölsä, J.; Jungner, H.; Lastusaari, M.; Niittykoski, J. Thermoluminescence study of persistent luminescence materials: Eu2+- and R3+-doped calcium aluminates, CaAl2O4:Eu2+, R3+. J. Phys. Chem. B 2006, 110, 4589–4598. [Google Scholar] [CrossRef] [PubMed]
- Wei, Y.; Wu, Z.; Jia, Y.; Liu, Y. Piezoelectrically-induced stress-luminescence phenomenon in CaAl2O4:Eu2+. J. Alloys Compd. 2015, 646, 86–89. [Google Scholar] [CrossRef]
- Jia, D.; Wang, X.-J.; Yen, W.M. Electron traps in Tb3+-doped CaAl2O4. Chem. Phys. Lett. 2002, 363, 241–244. [Google Scholar] [CrossRef]
- Satapathy, K.K.; Mishra, G.C.; Kher, R.S.; Dhoble, S.J. Mechanoluminescence and thermoluminescence characterization of Tb3+ doped CaAl2O4: A theoretical and experimental study. RSC Adv. 2015, 5, 79391–79396. [Google Scholar] [CrossRef]
- Tanaka, K.; Fujita, K.; Taniguchi, T.; Hirao, K.; Ishihara, T. Triboluminescence of alkaline earth aluminate polycrystals doped with Dy3+. J. Appl. Phys. 2000, 88, 4069–4074. [Google Scholar] [CrossRef]
- Katsumata, T.; Sakai, R.; Komuro, S.; Morikawa, T.; Kimura, H. Growth and characteristics of long duration phosphor crystals. J. Cryst. Growth 1999, 188, 869–871. [Google Scholar] [CrossRef]
- Sakaihara, I.; Tanaka, K.; Wakasugi, T.; Ota, R.; Fujita, K.; Hirao, K.; Ishihara, T. Triboluminescence of (Sr, Ba)Al2O4 polycrystals doped with Eu3+ and Eu2+. Jpn. J. Appl. Phys. 2002, 41, 1419–1423. [Google Scholar] [CrossRef]
- Qiu, J.; Igarashi, H.; Makishima, A. Long-lasting phosphorescence in Mn2+:Zn2GeO4 crystallites precipitated in transparent GeO2-B2O3-ZnO glass-ceramics. Sci. Technol. Adv. Mater. 2005, 6, 431–434. [Google Scholar] [CrossRef]
- Zhao, H.; Wang, X.; Li, J.; Li, Y.; Yao, X. Strong mechanoluminescence of Zn2(Ge0.9Si0.1)O2: Mn with weak persistent luminescence. Appl. Phys. Express 2016, 9, 012104. [Google Scholar] [CrossRef]
- Marsui, H.; Xu, C.-N.; Akiyama, M.; Watanabe, T. Strong mechanoluminescence from UV-irradiated spinels of ZnGa2O4: Mn and MgGa2O4: Mn. Jpn. J. Appl. Phys. 2000, 39, 6582–6586. [Google Scholar] [CrossRef]
- Uheda, K.; Maruyama, T.; Takizawa, H.; Endo, T. Synthesis and long-period phosphorescence of ZnGa2O4:Mn2+ spinel. J. Alloys Compd. 1997, 262, 60–64. [Google Scholar] [CrossRef]
- Matsui, H.; Xu, C.-N.; Tateyama, H. Stress-stimulated luminescence from ZnAl2O4:Mn. Appl. Phys. Lett. 2001, 78, 1068–1070. [Google Scholar] [CrossRef]
- Satapathy, K.K.; Mishra, G.C.; Khan, F. ZnAl2O4:Eu novel phosphor: SEM and mechanoluminescence characterization synthesized by solution combustion technique. Luminescence 2015, 30, 564–567. [Google Scholar] [CrossRef] [PubMed]
- Matsui, H.; Xu, C.N.; Liu, Y.; Tateyama, H. Origin of mechanoluminescence from Mn-activated ZnAl2O4: Triboelectricity-induced electroluminescence. Phys. Rev. B 2004, 69. [Google Scholar] [CrossRef]
- Garlick, G.F.J.; Wells, A.F.; Wilkins, M.H.F. Zinc sulfide phosphor constitution and its effect on electron traps. J. Chem. Phys. 1949, 17, 399–404. [Google Scholar] [CrossRef]
- Xu, C.-N.; Zheng, X.-G.; Watanabe, T.; Akiyama, M.; Usui, I. Enhancement of adhesion and triboluminescence of ZnS:Mn films by annealing technique. Thin Solid Films 1999, 352, 273–277. [Google Scholar] [CrossRef]
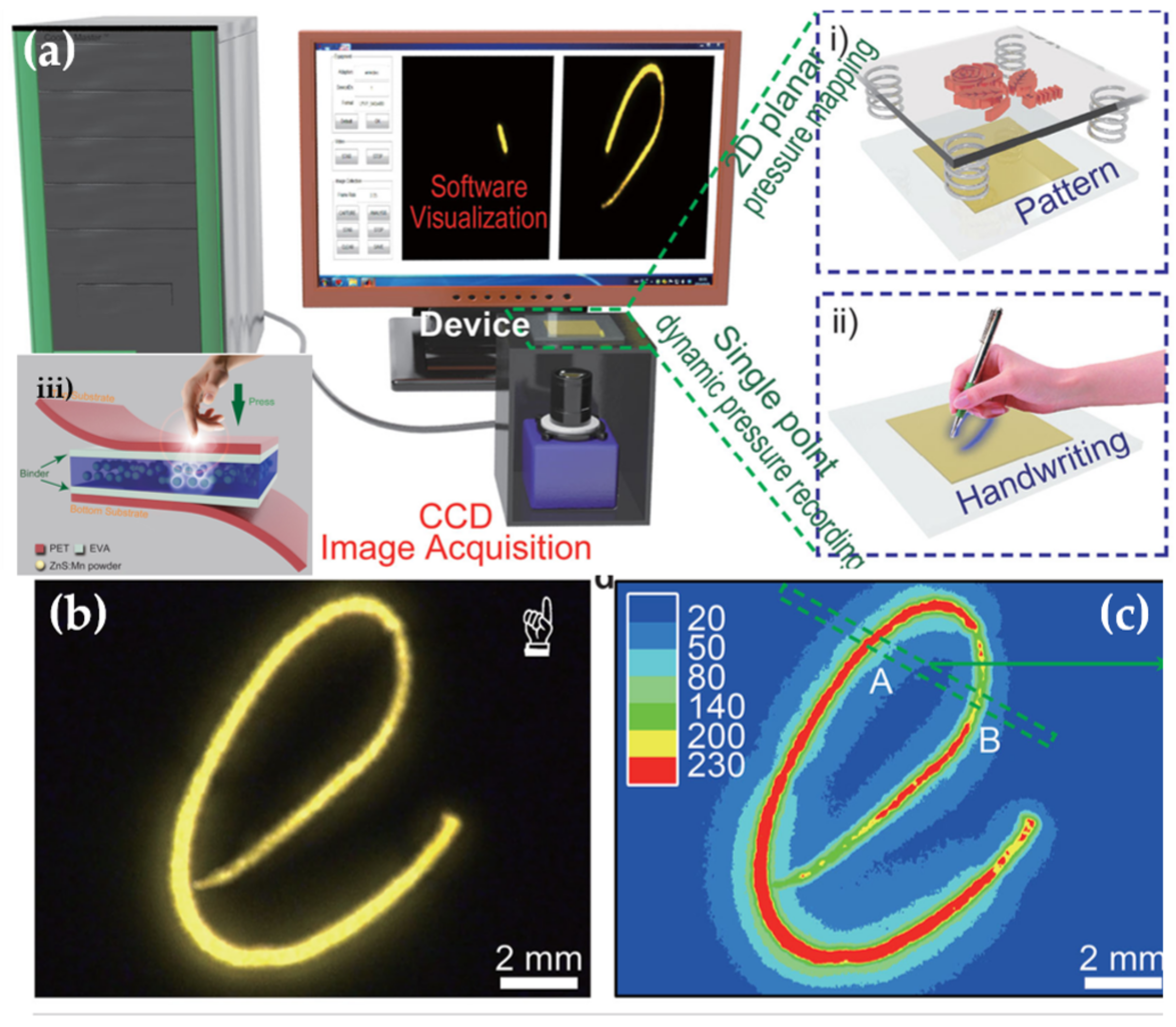
- Wang, X.; Zhang, H.; Yu, R.; Dong, L.; Peng, D.; Zhang, A.; Zhang, Y.; Liu, H.; Pan, C.; Wang, Z.L. Dynamic pressure mapping of personalized handwriting by a flexible sensor matrix based on the mechanoluminescence process. Adv. Mater. 2015, 27, 2324–2331. [Google Scholar] [CrossRef] [PubMed]
- Agyeman, O.; Xu, C.-N.; Usui, I.; Zheng, X.-G.; Suzuki, M. Thermal annealing effects on the triboluminescence intensity of sputtered ZnS:Mn thin films. In Advanced Photonic Sensors: Technology and Applications, Proceedings of the Optics and Optoelectronic Inspection and Control: Techniques, Applications, and Instrumentation, Beijing, China, 8–10 November 2000; Tang, J., Xu, C.-N., Li, H.H., Eds.; Spie: Washington, DC, USA, 2001; Volume 4220, pp. 350–354. [Google Scholar]
- Agyeman, O.; Xu, C.-N.; Shi, S.W.; Zheng, X.-G.; Suzuki, M. Enhancement in ZnS:Mn triboluminescent film intensity using ZnO films as buffer layers. In Advanced Environmental Sensing Technology II, Proceedings of the Environmental and Industrial Sensing, Boston, MA, USA, 28–31 October 2001; Vo-Dinh, T., Buttgenbach, S., Eds.; SPIE: Washington, DC, USA, 2002; Volume 4576, pp. 181–187. [Google Scholar]
- Agyeman, O.; Xu, C.-N.; Suzuki, M.; Zheng, X.-G. Upgrading the triboluminescence of ZnS:Mn film by optimization of sputtering and thermal annealing conditions. J. Mater. Res. 2002, 17, 959–963. [Google Scholar] [CrossRef]
- Onwona-Agyeman, B.; Xu, C.-N.; Shi, W.S.; Suzuki, M.; Zheng, X.-G. Triboluminescence of ZnS:Mn films deposited on quartz substrates with ZnO buffer layers. Jpn. J. Appl. Phys. 2002, 41, 5259–5261. [Google Scholar] [CrossRef]
- Chandra, B.P.; Xu, C.-N.; Yamada, H.; Zheng, X.-G. Luminescence induced by elastic deformation of ZnS:Mn nanoparticles. J. Lumin. 2010, 130, 442–450. [Google Scholar] [CrossRef]
- Fontenot, R.S.; Hollerman, W.A. Measuring triboluminescence from ZnS:Mn produced by ballistic impacts. J. Instrum. 2011, 6. [Google Scholar] [CrossRef]
- Kobakhidze, L.; Guidry, C.J.; Hollerman, W.A.; Fontenot, R.S. Detecting mechanoluminescence from ZnS:Mn powder using a high speed camera. IEEE Sens. J. 2013, 13, 3053–3059. [Google Scholar] [CrossRef]
- Chandra, V.K.; Chandra, B.P.; Jha, P. Mechanoluminescence of ZnS:Mn phosphors excited by hydrostatic pressure steps and pressure pulses. Physica B 2014, 452, 23–30. [Google Scholar] [CrossRef]
- Zhang, Y.; Gao, G.; Chan, H.L.W.; Dai, J.; Wang, Y.; Hao, J. Piezo-phototronic effect-induced dual-mode light and ultrasound emission from ZnS:Mn/PMN-PT thin film strucutres. Adv. Mater. 2012, 24, 1729–1735. [Google Scholar] [CrossRef] [PubMed]
- Garlick, G.F.J.; Gibson, A.F. The electron trap mechanism of luminescence in sulphide and silicate phosphors. Proc. Phys. Soc. 1948, 60, 574–590. [Google Scholar] [CrossRef]
- Hoogenstraaten, W. The chemistry of traps in zinc sulfide phophors. J. Electrochem. Soc. 1953, 100, 356–365. [Google Scholar] [CrossRef]
- Smith, A.W.; Turkevich, J. A study of the electron traps in zinc sulfide phosphor. Phys. Rev. 1952, 87, 306–308. [Google Scholar] [CrossRef]
- Jeong, S.M.; Song, S.; Lee, S.-K.; Choi, B. Mechanically driven light-generator with high durability. Appl. Phys. Lett. 2013, 102, 051110. [Google Scholar] [CrossRef]
- Sohn, K.S.; Timilsina, S.; Singh, S.P.; Lee, J.W.; Kim, J.S. A mechanoluminescent ZnS:Cu/Rhodamine/ SiO2/PDMS and piezoresistive CNT/PDMS hybrid sensor: Red-light emission and a standardized strain quantification. ACS Appl. Mater. Interfaces 2016, 8, 34777–34783. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; Zhang, Y.; Karnaushenko, D.; Chen, L.; Hao, J.; Ding, F.; Schmidt, O.G. Addressable and color-tunable piezophotonic light-emitting stripes. Adv. Mater. 2017, 29, 1605165. [Google Scholar] [CrossRef] [PubMed]
- Cho, M.Y.; Kim, J.S.; Kim, G.W. Hysteresis compensation of photoluminescence in ZnS:Cu for noncontact shaft torque sensing. Appl. Opt. 2016, 55, 1670–1674. [Google Scholar] [CrossRef] [PubMed]
- Wong, M.C.; Chen, L.; Bai, G.; Huang, L.B.; Hao, J. Temporal and remote tuning of piezophotonic-effect-induced luminescence and color gamut via modulating magnetic field. Adv. Mater. 2017, 29. [Google Scholar] [CrossRef] [PubMed]
- Jeong, S.M.; Song, S.; Kim, H.; Joo, K.-I.; Takezoe, H. Mechanoluminescence color conversion by spontaneous fluorescent-dye-diffusion in elastomeric zinc sulfide composite. Adv. Funct. Mater. 2016, 26, 4848–4858. [Google Scholar] [CrossRef]
- Jeong, S.M.; Song, S.; Lee, S.-K.; Ha, N.Y. Color manipulation of mechanoluminescence from stress-activated composite films. Adv. Mater. 2013, 25, 6194–6200. [Google Scholar] [CrossRef] [PubMed]
- Jeong, S.M.; Song, S.; Joo, K.-I.; Jeong, J.; Chung, S.-H. Bidirectional two colored light emission from stress-activated ZnS-microparticles-embedded polydimethylsiloxane elastomer films. Opt. Mater. Express 2013, 3, 1600–1607. [Google Scholar] [CrossRef]
- Reddy, D.R.; Reddy, B.K. Laser-like mechanoluminescence in ZnMnTe-diluted magnetic semiconductor. Appl. Phys. Lett. 2002, 81, 460–462. [Google Scholar] [CrossRef]
- Pateria, D.; Baghel, R.N.; Bisen, D.P.; Jha, P.; Chandra, B.P. Mechanoluminescence of (ZnS)1−x(MnTe)x nanophosphors excited by impact of a load. J. Lumin. 2015, 166, 335–345. [Google Scholar] [CrossRef]
- Rao, N.M.; Krishnaiah, G.; Sambasivam, S.; Reddy, K.R.; Reddy, D.R.; Reddy, B.K.; Xu, C.-N. Structural, optical and electrical properties of luminescent (ZnS)1−x(MnTe)x powders. J. Alloys Compd. 2009, 468, 360–364. [Google Scholar] [CrossRef]
- Rao, N.M.; Reddy, D.R.; Reddy, B.K.; Xu, C.-N. Intense red mechanoluminescence from (ZnS)1−x(MnTe)x. Phys. Lett. A 2008, 372, 4122–4126. [Google Scholar] [CrossRef]
- Pan, C.; Zhang, J.-C.; Zhang, M.; Yan, X.; Long, Y.-Z.; Wang, X. Intrinsic oxygen vacancies mediated multi-mechano-responsive piezoluminescence in undoped zinc calcium oxysulfide. Appl. Phys. Lett. 2017, 110, 233904. [Google Scholar] [CrossRef]
- Zhang, J.-C.; Xu, C.-N.; Kamimura, S.; Terasawa, Y.; Yamada, H.; Wang, X. An intense elastico-mechanoluminescence material CaZnOS:Mn2+ for sensing and imaging multiple mechanical stresses. Opt. Express 2013, 21, 12976–12986. [Google Scholar] [CrossRef] [PubMed]
- Tu, D.; Xu, C.-N.; Fujio, Y. Intense red emitting mechanoluminescence from CaZnOS:Mn, Li with c-axis preferred orientation. J. Adv. Dielectr. 2014, 04, 1450017. [Google Scholar] [CrossRef]
- Tu, D.; Xu, C.-N.; Fujio, Y.; Kamimura, S.; Sakata, Y.; Ueno, N. Phosphorescence quenching by mechanical stimulus in CaZnOS:Cu. Appl. Phys. Lett. 2014, 105, 011908. [Google Scholar] [CrossRef]
- Tu, D.; Xu, C.-N.; Fujio, Y.; Yoshida, A. Mechanism of mechanical quenching and mechanoluminescence in phosphorescent CaZnOS:Cu. Light Sci. Appl. 2015, 4, e356. [Google Scholar] [CrossRef]
- Tu, D.; Xu, C.-N.; Fujio, Y.; Yoshida, A. Tuning the mechano-optical conversion in CaZnOS with Cu ion concentration. J. Phys. D Appl. Phys. 2015, 48, 475105. [Google Scholar] [CrossRef]
- Zhang, H.; Peng, D.; Wang, W.; Dong, L.; Pan, C. Mechanically induced light emission and infrared-laser-induced upconversion in the Er-doped CaZnOS multifunctional piezoelectric semiconductor for optical pressure and temperature sensing. J. Phy. Chem. C 2015, 119, 28136–28142. [Google Scholar] [CrossRef]
- Wang, W.; Peng, D.; Zhang, H.; Yang, X.; Pan, C. Mechanically induced strong red emission in samarium ions doped piezoelectric semiconductor CaZnOS for dynamic pressure sensing and imaging. Opt. Commun. 2016, 395, 24–28. [Google Scholar] [CrossRef]
- Tu, D.; Peng, D.; Xu, C.-N.; Yoshida, A. Mechanoluminescence properties of red-emitting piezoelectric semiconductor MZnOS:Mn2+ (M = Ca, Ba) with layered structure. J. Cerm. Soc. Jpn. 2016, 124, 702–705. [Google Scholar] [CrossRef][Green Version]
- Li, L.; Wong, K.-L.; Li, P.; Peng, M. Mechanoluminescence properties of Mn2+-doped BaZnOS phosphor. J. Mater. Chem. C 2016, 4, 8166–8170. [Google Scholar] [CrossRef]
- Aitasalo, T.; Hölsä, J.; Kirm, M.; Laamanen, T.; Lastusaari, M.; Niittykoski, J.; Raud, J.; Valtonen, R. Persistent luminescence and synchrotron radiation study of Ca2MgSi2O7:Eu2+, R3+ materials. Radiat. Meas. 2007, 42, 644–647. [Google Scholar] [CrossRef]
- Gong, Y.; Wang, Y.; Jiang, Z.; Xu, X.; Li, Y. Luminescent properties of long lasting phosphor Ca2MgSi2O7:Eu2+. Mater. Res. Bull. 2009, 44, 1916–1919. [Google Scholar] [CrossRef]
- Zhang, H.; Yamada, H.; Terasaki, N.; Xu, C.-N. Green mechanoluminescence of Ca2MgSi2O7:Eu2+ and Ca2MgSi2O7:Eu2+, Dy. J. Electrochem. Soc. 2008, 155, J55–J57. [Google Scholar] [CrossRef]
- Jiang, L.; Chang, C.; Mao, D. Luminescent properties of CaMgSi2O6 and Ca2MgSi2O7 phosphors activated by Eu2+, Dy3+, and Nd3+. J. Alloys Compd. 2003, 360, 193–197. [Google Scholar] [CrossRef]
- Lin, Y.; Nan, C.-W.; Zhou, X.; Wu, J.; Wang, H.; Chen, D.; Xu, S. Preparation and characterization of long afterglow M2MgSi2O7-based (M = Ca, Sr, Ba) photoluminescent phosphors. Mater. Chem. Phys. 2003, 82, 860–863. [Google Scholar] [CrossRef]
- Zhang, H.; Terasaki, N.; Yamada, H.; Xu, C.-N. Development of mechanoluminescent micro-particles Ca2MgSi2O7:Eu, Dy and their application in sensors. Thin Solid Films 2009, 518, 610–613. [Google Scholar] [CrossRef]
- Zhang, H.; Xu, C.-N.; Terasaki, N.; Yamada, H. Detection of stress distribution using Ca2MgSi2O7:Eu, Dy microparticles. Physica E 2010, 42, 2872–2875. [Google Scholar] [CrossRef]
- Shrivastava, R.; Kaur, J. Mechanoluminescence of Ca2MgSi2O7:Eu2+, Dy3+ phosphor by impulsive deformation. Integr. Ferroelectr. 2015, 159, 49–56. [Google Scholar] [CrossRef]
- Sahu, I.P.; Bisen, D.P.; Brahme, N.; Tamrakar, R.K. Enhanced luminescence performance of Sr2MgSi2O7:Eu2+ blue long persistence phosphor by co-doping with Ce3+ ions. J. Mater. Sci. Mater. Electron. 2016, 27, 554–569. [Google Scholar] [CrossRef]
- Liua, B.; Shi, C.; Yin, M.; Dong, L.; Xiao, Z. The trap states in the Sr2MgSi2O7 and (Sr, Ca)2MgSi2O7 long afterglow phosphor activated by Eu2+ and Dy3+. J. Alloys Compd. 2005, 387, 65–69. [Google Scholar] [CrossRef]
- Lin, Y.; Tang, Z.; Zhang, Z.; Wang, X.; Zhang, J. Preparation of a new long afterglow blue-emitting Sr2MgSi2O7 photoluminescence phosphors. J. Mater. Sci. Lett. 2001, 20, 1505–1506. [Google Scholar] [CrossRef]
- Sahu, I.P.; Bisen, D.P.; Brahme, N.; Sharma, R. Luminescence properties of Eu2+, Dy3+-doped Sr2MgSi2O7, and Ca2MgSi2O7 phosphors by solid-state reaction method. Res. Chem. Intermed. 2015, 41, 6649–6664. [Google Scholar] [CrossRef]
- Aitasalo, T.; Hreniak, D.; Hölsä, J.; Laamanen, T.; Lastusaari, M.; Niittykoski, J.; Pellé, F.; Strȩk, W. Persistent luminescence of Ba2MgSi2O7:Eu2+. J. Lumin. 2007, 122, 110–112. [Google Scholar] [CrossRef]
- Shrivastava, R.; Kaura, J.; Chandra, B.P. Mechanoluminescence of Ba2MgSi2O7 doped with Eu2+ and Dy3+ phosphor by impulsive deformation. Luminescence 2015, 30, 1207–1211. [Google Scholar] [CrossRef] [PubMed]
- Zhang, H.; Yamada, H.; Terasaki, N.; Xu, C.-N. Stress-induced mechanoluminescence in SrCaMgSi2O7:Eu. Electrochem. Solid-State Lett. 2007, 10, J129–J131. [Google Scholar] [CrossRef]
- Fu, X.; Zheng, S.; Shi, J.; Zhang, H. Enhanced blue mechanoluminescence of SrnMgSi2O5+n:Eu alkali-earth silicate induced by defective phase. J. Lumin. 2017, 192, 117–122. [Google Scholar] [CrossRef]
- Kodama, N.; Takahashi, T.; Yamaga, M.; Tanii, Y.; Qiu, J.; Hirao, K. Long-lasting phosphorescence in Ce3+ doped Ca2Al2SiO7 and CaYAl3O7 crystals. Appl. Phys. Lett. 1999, 75, 1715–1717. [Google Scholar] [CrossRef]
- Akiyama, M.; Xu, C.-N.; Nonaka, K. Improvement in mechanoluminescence intensity of Ca2Al2SiO7:Ce by the statistical approach. J. Electrochem. Soc. 2003, 150, H115–H118. [Google Scholar] [CrossRef]
- Ding, Y.; Zhang, Y.; Wang, Z.; Li, W.; Mao, D.; Han, H.; Chang, C. Photoluminescence of Eu single doped and Eu/Dy codoped Sr2Al2SiO7 phosphors with long persistent. J. Lumin. 2009, 129. [Google Scholar] [CrossRef]
- Sahu, I.P.; Bisen, D.P.; Brahme, N.; Tamrakar, R.K. Luminescence enhancement of bluish-green Sr2Al2SiO7:Eu2+ phosphor by dysprosium co-doping. J. Lumin. 2015, 167, 278–288. [Google Scholar] [CrossRef]
- Sahu, I.P. The role of europium and dysprosium in the bluish-green long lasting Sr2Al2SiO7:Eu2+, Dy3+ phosphor by solid state reaction method. J. Mater. Sci. Mater. Electron. 2015, 26, 7059–7072. [Google Scholar] [CrossRef]
- Kodama, N.; Sasaki, N.; Yamaga, M.; Masui, Y. Long-lasting phosphorescence of Eu2+ in melilite. J. Lumin. 2001, 94–95, 19–22. [Google Scholar] [CrossRef]
- Zhang, H.; Yamada, H.; Terasaki, N.; Xu, C.-N. Blue light emission from stress-activated CaYAl3O7:Eu. J. Electrochem. Soc. 2008, 155, J128–J131. [Google Scholar] [CrossRef]
- Zhang, H.; Xu, C.-N.; Terasaki, N.; Yamada, H. Electro-mechano-optical luminescence from CaYAl3O7:Ce. Electrochem. Solid-State Lett. 2011, 14, J76–J80. [Google Scholar] [CrossRef]
- Ju, G.; Hu, Y.; Chen, L.; Wang, X.; Mu, Z. Persistent luminescence in CaAl2Si2O8:Eu2+-R3+ (R = Pr, Nd, Dy, Ho, Er). J. Lumin. 2014, 146, 102–108. [Google Scholar] [CrossRef]
- Zhang, L.; Yamada, H.; Imai, Y.; Xu, C.-N. Observation of elasticoluminescence from CaAl2Si2O8:Eu2+ and its water resistance behavior. J. Electrochem. Soc. 2008, 155, J63–J65. [Google Scholar] [CrossRef]
- Wang, Z.; Wang, Y.; Zhang, P.; Fan, X.; Qian, G. Tunable afterglow color in Eu2+ and Dy3+ co-activated alkaline earth feldspar solid solutions phophors. J. Lumin. 2007, 124, 140–142. [Google Scholar] [CrossRef]
- Zhang, L.; Xu, C.-N.; Yamada, H.; Bu, N. Enhancement of mechanoluminescence in CaAl2Si2O8:Eu2+ by partial Sr2+ substitution for Ca2+. J. Electrochem. Soc. 2010, 157, J50–J53. [Google Scholar] [CrossRef]
- Zhan, T.Z.; Xu, C.-N.; Yamada, H.; Terasawa, Y.; Zhang, L.; Iwase, H.; Kawai, M. Enhancement of impact-induced mechanoluminescence by swift heavy ion irradiation. Appl. Phys. Lett. 2012, 100, 0141019. [Google Scholar] [CrossRef]
- Liu, L.; Li, C.; Wang, S.; Su, Q. Redshift phenomenon of the excitation light of long life emission phosphor. Appl. Phys. Lett. 2006, 88, 241107. [Google Scholar] [CrossRef]
- Jia, W.; Jia, D.; Rodriguez, T.; Evans, D.R.; Meltzer, R.S.; Yen, W.M. UV excitation and trapping centers in CaTiO3:Pr3+. J. Lumin. 2006, 119, 13–18. [Google Scholar] [CrossRef]
- Zhang, J.-C.; Long, Y.-Z.; Wang, X.; Xu, C.-N. Controlling elastico-mechanoluminescence in diphase (Ba, Ca)TiO3:Pr3+ by co-doping different rare earth ions. RSC Adv. 2014, 4, 40665–40675. [Google Scholar] [CrossRef]
- Wang, X.; Xu, C.-N.; Yamada, H.; Nishikubo, K.; Zheng, X.-G. Electro-mechano-optical conversions in Pr3+-doped BaTiO3-CaTiO3 ceramics. Adv. Mater. 2005, 17, 1254–1258. [Google Scholar] [CrossRef]
- Zhang, J.-C.; Tang, M.; Wang, X.; Li, Y.; Yao, X. Elastico-mechanoluminescence properties of Pr3+-doped BaTiO3-CaTiO3 diphase ceramics with water resistance behavior. Ceram. Int. 2012, 38, S581–S584. [Google Scholar] [CrossRef]
- Zhang, J.-C.; Wang, X.; Yao, X.; Xu, C.-N.; Yamada, H. Strong elastico-mechanoluminescence in diphase (Ba, Ca)TiO3:Pr3+ with self-assembled sandwich architectures. J. Electrochem. Soc. 2010, 157, G269. [Google Scholar] [CrossRef]
- Zhang, J.-C.; Wang, X.; Yao, X.; Xu, C.-N.; Yamada, H. Studies on AC electroluminescence device made of BaTiO3-CaTiO3:Pr3+ diphase ceramics. Appl. Phys. Express 2010, 3, 022601. [Google Scholar] [CrossRef]
- Zhang, J.-C.; Wan, Y.; Xin, X.; Han, W.-P.; Zhang, H.-D.; Sun, B.; Long, Y.-Z.; Wang, X. Elastico-mechanoluminescent enhancement with Gd3+ codoping in diphase (Ba, Ca)TiO3:Pr3+. Opt. Mater. Express 2014, 4, 2300. [Google Scholar] [CrossRef]
- Tu, D.; Xu, C.-N.; Yoshida, A.; Fujihala, M.; Hirotsu, J.; Zheng, X.-G. LiNbO3:Pr3+: A multipiezo material with simultaneous piezoelectricity and sensitive piezoluminescence. Adv. Mater. 2017, 29, 1606914. [Google Scholar] [CrossRef] [PubMed]
- Lei, B.-F.; Yue, S.; Zhang, Y.-Z.; Liu, Y.-L. Luminescence properties of Sr2SnO4:Sm3+ afterglow phosphors. Chin. Phys. Lett. 2010, 27, 037201. [Google Scholar] [CrossRef]
- Xu, X.; Wang, Y.; Zeng, W.; Gong, Y. Luminescence and storage properties of Sm-doped alkaline-earth atannates. J. Electrochem. Soc. 2011, 158, J305–J309. [Google Scholar] [CrossRef]
- Kamimura, S.; Yamada, H.; Xu, C.-N. Strong reddish-orange light emission from stress-activated Srn+1SnnO3n+1:Sm3+ (n = 1, 2, ∞) with perovskite-related structures. Appl. Phys. Lett. 2012, 101, 091113. [Google Scholar] [CrossRef]
- Lei, B.; Manb, S.-Q.; Liu, Y.; Yue, S. Luminescence properties of Sm3+-doped Sr3Sn2O7 phosphor. Mater. Chem. Phys. 2014, 124, 912–915. [Google Scholar] [CrossRef]
- Ju, Z.; Wei, R.; Zheng, J.; Gao, X.; Zhang, S.; Liu, W. Synthesis and phosphorescence mechanism of a reddish orange emissive long afterglow phosphor Sm3+-doped Ca2SnO4. Appl. Phys. Lett. 2011, 98, 121906. [Google Scholar] [CrossRef]
- Zhao, H.; Chai, X.; Wang, X.; Li, Y.; Yao, X. Mechanoluminescence in (Sr, Ca, Ba)2SnO4:Sm3+, La3+ ceramics. J. Alloys Compd. 2016, 656, 94–97. [Google Scholar] [CrossRef]
- Li, J.; Xu, C.-N.; Tu, D.; Chai, X.; Wang, X.; Liu, L.; Kawasaki, E. Tailoring bandgap and trap distribution via Si or Ge substitution for Sn to improve mechanoluminescence in Sr3Sn2O7:Sm3+ layered perovskite oxide. Acta Mater. 2018, 145, 464–469. [Google Scholar] [CrossRef]
- Wang, B.; Lin, H.; Xu, J.; Chen, H.; Lin, Z.; Huang, F.; Wang, Y. Design, preparation, and characterization of a novel red long-persistent perovskite phosphor: Ca3Ti2O7:Pr3+. Inorg. Chem. 2015, 54, 11299–11306. [Google Scholar] [CrossRef] [PubMed]
- Fan, X.-H.; Zhang, J.-C.; Zhang, M.; Pan, C.; Yan, X.; Han, W.-P.; Zhang, H.-D.; Long, Y.-Z.; Wang, X. Piezoluminescence from ferroelectric Ca3Ti2O7:Pr3+ long-persistent phosphor. Opt. Express 2017, 25, 14238–14246. [Google Scholar] [CrossRef] [PubMed]
- Botterman, J.; Van den Eeckhout, K.; Bos, A.J.J.; Dorenbos, P.; Smet, P.F. Persistent luminescence of MSi2O2N2:Eu phosphor. Opt. Mater. Express 2012, 2, 341–349. [Google Scholar] [CrossRef]
- Qin, J.; Zhang, H.; Lei, B.; Hu, C.; Li, J.; Liu, Y.; Meng, J.; Wang, J.; Zheng, M.; Xiao, Y. Thermoluminescence and temperature-dependent afterglow properties in BaSi2O2N2:Eu2+. J. Am. Ceram. Soc. 2013, 96, 3149–3154. [Google Scholar] [CrossRef]
- Botterman, J.; Van den Eeckhout, K.; De Baere, I.; Poelman, D.; Smet, P.F. Mechanoluminescence in BaSi2O2N2:Eu. Acta Mater. 2012, 60, 5494–5500. [Google Scholar] [CrossRef]
- Bregiroux, D.; Wallez, G.; Popa, K. Structural study of polyporphism and thermal behavior of CaZr(PO4)2. Solid State Sci. 2015, 41, 43–47. [Google Scholar] [CrossRef]
- Zhang, J.-C.; Xu, C.-N.; Long, Y.-Z. Elastico-mechanoluminescence in CaZr(PO4)2:Eu2+ with multiple trap levels. Opt. Express 2013, 21, 13699–13709. [Google Scholar] [CrossRef] [PubMed]
- Cong, Y.; Li, B.; Wang, X.-J.; Lei, B.; Li, W. Synthesis and optical property study of nanocrystalline ZrO2:Ti long-lasting phosphors. J. Electrochem. Soc. 2008, 15, K195–K198. [Google Scholar] [CrossRef]
- Carvalho, J.M.; Rodrigues, L.C.V.; Hölsä, J.; Lastusaari, M.; Nunes, L.A.O.; Felinto, M.C.F.C.; Malta, O.L.; Brito, H.F. Influence of titanium and lutetium on the persistent luminescence of ZrO2. Opt. Mater. Express 2012, 2, 331–340. [Google Scholar] [CrossRef]
- Tiwari, N.; Kuraria, R.K.; Kuraria, S.R. Mechanoluminescence induced by impulsive deformation in zirconium:Ti phosphor. Radiat Eff. Defects Solids 2015, 170, 679–689. [Google Scholar] [CrossRef]
- Akiyama, M.; Xu, C.-N.; Nonaka, K. Intense visible light emission from stress-activated Zr2:Ti. Appl. Phys. Lett. 2002, 81, 457–459. [Google Scholar] [CrossRef]
- Tyler, W.W. Plastic flow in alkali halide crystals. Phys. Rev. 1952, 86, 801–802. [Google Scholar] [CrossRef]
- Kruglov, A.S.; Ei-shanshoury, I.A.; Matta, M.K. On the Luminescence of γ-rayed KCl crystals induced by plastic deformation. J. Phys. Soc. Jpn. 1966, 21, 2147–2153. [Google Scholar] [CrossRef]
- Alzetta, G.; Chudacek, I.; Scarmozzino, R. Excitation of triboluminescence by deformation of single crystals. Phys. Status Solidi A 1970, 1, 775–785. [Google Scholar] [CrossRef]
- Catti, M.; Noel, Y.; Dovesi, R. Full piezoelectric tensors of wurtzite and zinc blende ZnO and ZnS by first principle calculations. J. Phys. Chem. Solids 2003, 64, 2183–2190. [Google Scholar] [CrossRef]
- Meyer, B.K.; Stadler, W. Native defect identification in II–VI materials. J. Cryst. Growth 1996, 161, 119–127. [Google Scholar] [CrossRef]
- Krishnan, S.; Van der Walt, H.; Venkatesh, V.; Sundaresan, V.B. Dynamic characterization of elastico-mechanoluminescence towards structural health monitoring. J. Intell. Mater. Syst. Sturct. 2017, 28, 2458–2464. [Google Scholar] [CrossRef]
- Chandra, V.K.; Chandra, B.P.; Jha, P. Self-recovery of mechanoluminescence in ZnS:Cu and ZnS:Mn phosphors by trapping of drifting charge carriers. Appl. Phys. Lett. 2013, 103, 161113. [Google Scholar] [CrossRef]
- Sambrook, T.; Smura, C.F.; Clarke, S.J.; Ok, K.M.; Halasyamani, P.S. Structure and physical properties of the polar oxysulfide CaZnOS. Inorg. Chem. 2007, 46, 2571–2574. [Google Scholar] [CrossRef] [PubMed]
- Broadley, S.; Gál, Z.A.; Corà, F.; Smura, C.F.; Clarke, S.J. Vertex-linked ZnO2S2 tetrahedra in the oxysulfide BaZnOS: A new coordination environment for zinc in a condensed solid. Inorg. Chem. 2005, 44, 9092–9096. [Google Scholar] [CrossRef] [PubMed]
- Müller-Buschbaum, H. The crystal chemistry of AM2O4 oxometalates. J. Alloys Compd. 2003, 349, 49–104. [Google Scholar] [CrossRef]
- Prodjosantoson, A.K.; Kennedyn, B.J. Synthesis and evolution of the crystalline phases in Ca1−xSrxAl2O4. J. Solid State Chem. 2002, 168, 229–236. [Google Scholar] [CrossRef]
- Schulze, A.-R.; Buschbaum, M. Zur Verbindungsbildung von MeO: M2O3. IV. Zur Struktur von monoklinem SrAl2O4. Z. Anorg. Allg. Chem. 1981, 475, 205–210. [Google Scholar] [CrossRef]
- Henderson, C.M.B.; Taylor, D. The Structural behaviour of the nepheline family: (1) Sr and Ba aluminates (MAl2O4). Mineral. Mag. 1982, 45, 111–127. [Google Scholar] [CrossRef]
- Yamada, H.; Kusaba, H.; Xu, C.-N. Anisotropic lattice behavior in elasticoluminescent material SrAl2O4: Eu2+. Appl. Phys. Lett. 2008, 92, 101909. [Google Scholar] [CrossRef]
- Ishii, Y.; Tsukasaki, H.; Tanaka, E.; Mori, S. A fluctuating state in framework compounds (Ba, Sr)Al2O4. Sci. Rep. 2016, 6, 19154. [Google Scholar] [CrossRef] [PubMed]
- Rodehorst, U.; Carpenter, M.A.; Marion, S.; Henderson, C.M.B. Structural phase transitions and mixing behaviour of the Ba-aluminate (BaAl2O4)–Sr-aluminate (SrAl2O4) solid solution. Mineral. Mag. 2003, 67, 989–1013. [Google Scholar] [CrossRef]
- Hang, C.; Simonov, M.A.; Belov, N.V. Crystal strucutre of williemite Zn2[SiO4] amd its germanium analog Zn2[GeO4]. Sov. Phys. Crystallogr. 1970, 15, 387–390. [Google Scholar]
- McGuinn, M.D.; Redfern, S.A.T. Ferroelastic phase transition along the join CaAl2Si2O8-SrAl2Si2O8. Am. Mineral. 1994, 79, 24–30. [Google Scholar]
- Hemon, A.; Courbion, G. The crystal structure of α-SrZn2(PO4)2: A hurlbutite type. J. Solid State Chem. 1990, 85, 164–168. [Google Scholar] [CrossRef]
- Shannon, R.D. Revised effective ionic radii and systematic studies of interatomic distances in halides and chaleogenides. Acta Crystallogr. A 1976, 32, 751–767. [Google Scholar] [CrossRef]
- Greenwood, N.N.; Earnshaw, A. Chemistry of the Elements, 2nd ed.; Butterworth-Heinemann: Oxford, UK, 1998; pp. 112–115, 1027–1028. ISBN 0-7506-3365-4. [Google Scholar]
- Schaper, A.K.; Schosnig, M.; Kutoglu, A.; Treutmann, W.; Rager, H. Transition from the incommensurately modulated structure to the lock-in phase in Co-åkermanite. Acta Crystallogr. B 2001, 57, 443–448. [Google Scholar] [CrossRef] [PubMed]
- Tamura, T.; Yoshiasa, A.; Iishi, K.; Takeno, S.; Maeda, H.; Emura, S.; Koto, K. Local structure of (Ca, Sr)2(Mg, Co, Zn)Si2O7 melilite solid-solution with modulated structure. Phys. Chem. Miner. 1996, 23, 81–88. [Google Scholar] [CrossRef]
- Van Heurck, C.; Van Tendeloo, G.; Amelinckx, S. The modulated structure in the melilite Ca2ZnGe2O7. Phys. Chem. Miner. 1992, 18, 441–452. [Google Scholar] [CrossRef]
- Seifert, F.; Czank, M.; Simons, B.; Schmahl, W. A commensurate-incommensurate phase transition in iron-bearing åkermanites. Phys. Chem. Miner. 1987, 14, 26–35. [Google Scholar] [CrossRef]
- Kusaka, K.; Haga, N.; Ohmasa, M.; Hagiya, K.; Iishi, K.; Haga, N. On variety of the Ca coordination in the incommensurate structure of synthetic iron-bearing åkermanite, Ca2(Mg0.55, Fe0.45)Si2O7. Mineral. J. 1998, 20, 47–58. [Google Scholar] [CrossRef][Green Version]
- Jiang, J.C.; Schosnig, M.; Schaper, A.K.; Ganster, K.; Rager, H.; Tóth, L. Modulations in incommensurate (Ca1−xSrx)2MgSi2O7 single crystals. Phys. Chem. Miner. 1998, 26, 128–134. [Google Scholar] [CrossRef]
- Louisnathan, S.J. Refinement of the crystal structure of a natural gehlenite Ca2Al(Al, Si)2O7. Can. Mineral. 1971, 10, 822–837. [Google Scholar]
- Loewenstein, W. The distribution of aluminum in the tetrahedra of silicates and aluminates. Am. Mineral. 1954, 39, 92–96. [Google Scholar]
- Gemmi, M.; Merlini, M.; Cruciani, G.; Artioli, G. Non-ideality and defectivity of the åkermanite-gehlenite solid solution: An X-ray diffraction and TEM study. Am. Mineral. 2007, 92, 1685–1694. [Google Scholar] [CrossRef]
- Yang, Y.; Lu, H.; Yu, C.; Chen, J.-M. First-principles calculations of structural, electronic and elastic properties of Ca2MgSi2O7. Compt. Mater. Sci. 2009, 47, 35–40. [Google Scholar] [CrossRef]
- Haussühl, S.; Liebertz, J. Elastic and thermoelastic properties of synthetic Ca2MgSi2O7 (åkermanite) and Ca2ZnSi2O7 (hardystonite). Phys. Chem. Miner. 2004, 31, 565–567. [Google Scholar] [CrossRef]
- Brown, J.M.; Ange, R.J.; Ross, N.L. Elasticity of plagioclase feldspars. J. Geophys. Res. B Solid Earth 2016, 121, 663–675. [Google Scholar] [CrossRef]
- Kaercher, P.; Militzer, B.; Wenk, H.-R. Ab initio calculations of elastic constants of plagioclase feldspars. Am. Mineral. 2014, 99, 2344–2352. [Google Scholar] [CrossRef]
- Damjanovic, D. Lead-based piezoelectric materials. In Piezoelectric and Acoustic Materials for Transducer Applications, 1st ed.; Safari, A., Akdoğan, E.K., Eds.; Springer: New York, NY, USA, 2008; pp. 59–79. ISBN 978-0-387-76538-9. [Google Scholar]
- Fisk, Z.; Sarrao, J.L. The new generation high-temperature superconductors. Annu. Rev. Mater. Sci. 1997, 27, 35–67. [Google Scholar] [CrossRef]
- Zhu, J.; Thomas, A. Perovskite-type mixed oxides as catalytic material for NO removal. Appl. Catal. B 2009, 92, 225–233. [Google Scholar] [CrossRef]
- Yamamoto, H.; Okamoto, S.; Kobayashi, H. Luminescence of rare-earth ions in perovskite-type oxides: From basic research to applications. J. Lumin. 2002, 100, 325–332. [Google Scholar] [CrossRef]
- Wang, X.; Yamada, H.; Xu, C.-N. Large electrostriction near the solubility limit in BaTiO3-CaTiO3 ceramics. Appl. Phys. Lett. 2005, 86, 022905. [Google Scholar] [CrossRef]
- Smith, R.T.; Welsh, F.S. Temperature dependence of the elastic, piezoelectric, and dielectric constants of lithium tantalate and lithium niobate. J. Appl. Phys. 1971, 42, 2219–2230. [Google Scholar] [CrossRef]
- Megaw, H.D. A Note on the strueture of lithium niobate, LiNbO3. Acta Crystallogr. A 1968, 24, 583–588. [Google Scholar] [CrossRef]
- Green, M.A.; Prasssides, K.; Day, P.; Neumann, D.A. Structure of the n = 2 and n = ∞ member of the Ruddlesden-Popper series, Srn+1SnnO3n+1. Int. J. Inorg. Mater. 2000, 2, 35–41. [Google Scholar] [CrossRef]
- Kechele, J.A.; Oeckler, O.; Stadler, F.; Schnick, W. Structure elucidation of BaSi2O2N2–A host lattice for rare-earth doped luminescent materials in phosphor-converted (pc)-LEDs. Solid State Sci. 2009, 11, 537–543. [Google Scholar] [CrossRef]
- Bachmann, V.; Ronda, C.; Oeckler, O.; Schnick, W.; Meijerink, A. Color point tuning for (Sr, Ca, Ba)Si2O2N2:Eu2+ for white light LEDs. Chem. Mater. 2009, 21, 316–325. [Google Scholar] [CrossRef]
- Seibald, M.; Rosenthal, T.; Oeckler, O.; Fahrnbauer, F.; Tucks, A.; Schmidt, P.J.; Schnick, W. Unexpected luminescence properties of Sr0.25Ba0.75Si2O2N2:Eu2+—A narrow blue emitting oxonitridosilicate with cation ordering. Chem. Eur. J. 2012, 18, 13446–13452. [Google Scholar] [CrossRef] [PubMed]
- Lazarowska, A.; Mahlik, S.; Grinberg, M.; Li, G.; Liu, R.S. Structural phase transitions and photoluminescence properties of oxonitridosilicate phosphors under high hydrostatic pressure. Sci. Rep. 2016, 6, 34010. [Google Scholar] [CrossRef] [PubMed]
- Shimakawa, K.; Singh, J.; O’Leary, S.K. Optical properties of disordered condensed matter. In Optical Properties of Condensed Matter and Applications, 1st ed.; Singh, J., Ed.; John Wiley & Sons, Ltd.: West Sussex, UK, 2006; pp. 47–62. ISBN 978-0-470-02192-7. [Google Scholar]
- Salje, E.K.H. Modulated minerals as potential ferroic materials. J. Phys. Condens. Matter 2015, 27, 305901. [Google Scholar] [CrossRef] [PubMed]
- Tsukasaki, H.; Ishii, Y.; Tanaka, E.; Kurushima, K.; Mori, S. Modulated structures and associated microstructures in the ferroelectric phase of Ba1−xSrxAl2O4 (0.7 < x < 1.0). Jpn. J. Appl. Phys. 2016, 55, 011502. [Google Scholar] [CrossRef]
- Avdeev, M.; Yakovlev, S.; Yaremchenko, A.A.; Kharton, V.V. Transitions between P21, P63 (A) and P6322 modifications of SrAl2O4 by in situ high-temperature X-ray and neutron diffraction. J. Solid State Chem. 2007, 180, 3535–3544. [Google Scholar] [CrossRef]
- Abakumov, A.M.; Lebedev, O.I.; Nistor, L.; Van Tendeloo, G.; Amelinckx, S. The ferroelectric phase transition in tridymite type BaAl2O4 studied by electron microscopy. Phase Transit. 2000, 71, 143–160. [Google Scholar] [CrossRef]
- Larsson, A.-K.; Withers, R.L.; Perez-Mato, J.M.; Gerald, J.D.F.; Saines, P.J.; Kennedy, B.J.; Liu, Y. On the microstructure and symmetry of apparently hexagonal BaAl2O4. J. Solid State Chem. 2008, 181, 1816–1823. [Google Scholar] [CrossRef]
- Stokes, H.T.; Sadate, C.; Hatch, D.M.; Boyer, L.L.; Mehl, M.J. Analysis of the ferroelectric phase transition in BaAl2O4 by group theoretical methods and first principles calculations. Phys. Rev. B 2002, 65, 064105. [Google Scholar] [CrossRef]
- Mori, S.; Katsumura, S.; Ozaki, T.; Tanaka, E.; Ishii, Y.; Kurushima, K.; Kubota, Y.; Taniguchi, H. Structural phase transition and microstructures in stuffed tridymite-type compounds: Ba(Al, Fe)2O4. Ferroelectrics 2014, 464, 116–121. [Google Scholar] [CrossRef]
- Tanaka, E.; Ishii, Y.; Tsukasaki, H.; Taniguchi, H.; Mori, S. Structural changes and microstructures in stuffed tridymite-type compounds Ba1−xSrxAl2O4. Jpn. J. Appl. Phys. 2014, 53, 09PB01. [Google Scholar] [CrossRef]
- Fukuda, K.; Iwata, T.; Orito, T. Structural disorder in Ba0.6Sr0.4Al2O4. J. Solid State Chem. 2005, 178, 3662–3666. [Google Scholar] [CrossRef]
- Ghose, S.; McMullan, P.K.; Weber, H.-P. Neutron diffraction studies of the P ⇔ I transition in anorthite, CaAl2Si2O8, and the crystal structure of the body-centered phase at 514 K. Z. Kristallogr. 1993, 204, 215–237. [Google Scholar] [CrossRef]
- Angel, R.J.; Carpenter, M.A.; Finger, L.W. Structural variation associated with compositional variation and order-disorder behaviour in anorthite-rich feldspars. Am. Mineral. 1990, 75, 150–162. [Google Scholar]
- Ghose, S.; Van Tendeloo, G.; Amelinckx, S. Dynamics of a second-order phase transition: P to I phase transition in anorthite, CaAl2Si2O8. Science 1988, 242, 1539–1541. [Google Scholar] [CrossRef] [PubMed]
- Xu, H.; Buseck, P.R.; Carpenter, M.A. Twinning in synthetic anorthite: A transmission electron microscopy investigation. Am. Mineral. 1997, 82, 125–130. [Google Scholar] [CrossRef]
- Töepel-Schadt, J.; Müeller, W.F.; Pentinghaus, H. Transmission electron microscopy of SrAl2Si2O8—Feldspar and hexacelsian polymorphs. J. Mater. Sci. 1978, 13, 1809–1816. [Google Scholar] [CrossRef]
- Müller, W.F. Phase transitions and associated domains in hexacelsian (BaAl2Si2O8). Phys. Chem. Miner. 1977, 1, 71–82. [Google Scholar] [CrossRef]
- McGuinn, M.D.; Redfern, S.A.T. Lattice simulation studies of the ferroelastic phase transitions in (Na, K)AISi3O8 and (Sr, Ca)Al2Si2O8 feldspar solid solutions. Am. Mineral. 1997, 82, 8–15. [Google Scholar] [CrossRef]
- McGuinn, M.D.; Redfern, S.A.T. High temperature ferroelastic strain below the I-I transition in Ca1−xSrxAl2Si2O8 feldspars. Eur. J. Mineral. 1997, 9, 1159–1172. [Google Scholar] [CrossRef]
- Tribaudino, M.; Zhang, M.; Salje, E.K.H. Cation ordering and phase transitions in feldspars along the join CaAl2Si2O8-SrAl2Si2O8: A TEM, IR and XRD investigation. Am. Mineral. 2009, 73, 119–130. [Google Scholar] [CrossRef]
- Benna, P.; Bruno, E. Single-crystal in-situ high-temperature structural investigation of the I-I phse transition in the Ca0.2Sr0.8Al2Si2O8 feldspar. Am. Mineral. 2003, 88, 1532–1541. [Google Scholar] [CrossRef]
- Ma̧czka, M.; Hanuza, J.; Kaminskii, A.; Kojima, S. High-resolution Brillouin scattering studies of phase transitions in Ca2MgSi2O7 and Ca2ZnSi2O7 silicates. J. Alloys Compd. 2015, 638, 34–37. [Google Scholar] [CrossRef]
- Kusz, J.; Bohm, H. X-ray studies of phase transitions of (Ca,Sr)-åkermanite solid solutions. Z. Kristallogr. 2001, 216, 509–512. [Google Scholar] [CrossRef]
- Van Aert, S.; Turner, S.; Delville, R.; Schryvers, D.; Van Tendeloo, G.; Ding, X.; Salje, E.K.H. Functional twin boundaries. Phase Transit. 2013, 86, 1052–1059. [Google Scholar] [CrossRef]
- Van Aert, S.; Turner, S.; Delville, R.; Schryvers, D.; Van Tendeloo, G.; Salje, E.K.H. Direct observation of ferroelectricity at ferroelastic domain boundaries in CaTiO3 by electron microscopy. Adv. Mater. 2012, 24, 523–527. [Google Scholar] [CrossRef] [PubMed]
- Von Hippel, A. Ferroelectricity, domain structure, and phase transitions of barium titanate. Rev. Mod. Phys. 1950, 22, 221–237. [Google Scholar] [CrossRef]
- Fu, W.T.; Visser, D.; IJdo, D.J.W. Neutron powder diffraction study of phase transitions in Sr2SnO4. J. Solid State Chem. 2004, 177, 4081–4086. [Google Scholar] [CrossRef]
- Oeckler, O.; Stadler, F.; Rosenthal, T.; Schnick, W. Real structure of SrSi2O2N2. Solid State Sci. 2007, 9, 205–212. [Google Scholar] [CrossRef]
- Trolliard, G.; Mercurio, D.L.; Perez-Mato, J.M. Martensitic phase transition in pure zirconia: A crystal chemistry viewpoint. Z. Kristallogr. 2011, 226, 264–290. [Google Scholar] [CrossRef]
- Tolédano, J.-C.; Janovec, V.; Kopsky, V.; Scott, J.F.; Bocěk, P. Structural phase transitions. In International Tables for Crystallography, 1st ed.; Authier, A., Ed.; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2003; Volume D, pp. 338–376. ISBN 1-4020-0714-0. [Google Scholar]
- Guymont, M. Domain structures arising from transitions between two crystals whose space groups are group-subgroup related. Phys. Rev. B 1978, 18, 5385–5393. [Google Scholar] [CrossRef]
- Janovec, V.; Přívratská, J. Domain structures. In International Table of Crystallography, 1st ed.; Authier, A., Ed.; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2003; Volume D, pp. 449–502. ISBN 1-4020-0714-0. [Google Scholar]
- Dove, M.T. Theory of displacive phase transitions in minerals. Am. Mineral. 1997, 82, 213–244. [Google Scholar] [CrossRef]
- Carpenter, M.A. Elastic properties of minerals and the influence of phase transitions. Am. Mineral. 2006, 91, 229–246. [Google Scholar] [CrossRef]
- Barandiaran, Z.; Seijo, L. The abinitio model potential representation of the crystalline environment. theoretical study of the local distortion on NaCl:Cu+. J. Chem. Phys. 1988, 89, 5739–5746. [Google Scholar] [CrossRef]
- Davis, M.; Budimir, M.; Damjanovic, D.; Setter, N. Rotator and extender ferroelectrics: Importance of the shear coefficient to the piezoelectric properties of domain-engineered crystals and ceramics. J. Appl. Phys. 2007, 101, 054112. [Google Scholar] [CrossRef]
- Budimir, M.; Damjanovic, D.; Setter, N. Piezoelectric anisotropy-phase transition in perovskite single crystals. J. Appl. Phys. 2003, 94, 6753–6761. [Google Scholar] [CrossRef]
- Pinheiroa, C.B.L.; Abakumov, A.M. Superspace crystallography: A key to the chemistry and properties. IUCrJ 2015, 2, 137–154. [Google Scholar] [CrossRef] [PubMed]
- Yang, H.; Hazen, R.M.; Downs, R.T.; Finger, L.W. Structural change associated with the incommensurate-normal phase transition in åkermanite, Ca2MgSi2O7 , at high pressure. Phys. Chem. Miner. 1997, 24, 510–519. [Google Scholar] [CrossRef]
- Arakcheeva, A.; Logvinovich, D.; Chapuis, G.; Morozov, V.; Eliseeva, S.V.; Bünzli, J.-C.G.; Pattison, P. The luminescence of NaxEu3+2/3−x/3MoO4 scheelites depends on the number of Eu-clusters occurring in their incommensurately modulated structure. Chem. Sci. 2012, 3, 384–390. [Google Scholar] [CrossRef]
- Zhai, B.-G.; Yang, L.; Ma, Q.-L.; Liu, X.; Huang, Y.M. Mechanism of the prolongation of the green afterglow of SrAl2O4:Dy3+ caused by the use of H3BO3 flux. J. Lumin. 2017, 181, 78–87. [Google Scholar] [CrossRef]
- Su, Q.; Li, C.; Wang, J. Some interesting phenomena in the study of rare earth long lasting phosphors. Opt. Mater. 2014, 36, 1894–1900. [Google Scholar] [CrossRef]
- Korthout, K.; Van den Eeckhout, K.; Botterman, J.; Nikitenko, S.; Poelman, D.; Smet, P.F. Luminescence and X-ray absorption measurements of persistent SrAl2O4:Eu,Dy powders: Evidence for valence state changes. Phys. Rev. B 2011, 84, 085140. [Google Scholar] [CrossRef]
- Joos, J.J.; Poelman, D.; Smet, P.F. Energy level modelling of lanthanide materials: Review and uncertainty analysis. Phys. Chem. Chem. Phys. 2015, 17, 19058–19078. [Google Scholar] [CrossRef] [PubMed]
- Botterman, J.; Joos, J.J.; Smet, P.F. Trapping and detrapping in SrAl2O4:Eu,Dy persistent phosphors: Influence of excitation wavelength and temperature. Phys. Rev. B 2014, 90, 085147. [Google Scholar] [CrossRef]
- Clabau, F.; Rocquefelte, X.; Jobic, S.; Deniard, P.; Whangbo, M.-H.; Garcia, A.; Mercier, T.L. Mechanism of Phosphorescence Appropriate for the Long-Lasting Phosphors Eu2+-Doped SrAl2O4 with Codopants Dy3+ and B3+. Chem. Mater. 2005, 17, 3904–3912. [Google Scholar] [CrossRef]
- Jain, M.; Guralnik, B.; Andersen, M.T. Stimulated luminescence emission from localized recombination in randomly distribution defects. J. Phys. Condens. Matter 2012, 24, 385402. [Google Scholar] [CrossRef] [PubMed]
- Pagonis, V.; Kulp, C.; Chaney, C.-G.; Tchiya, M. Quantum tunneling recombination in a system of randomly distributed trapped electrons and positive ions. J. Phy. Condens. Matter 2017, 29, 365701. [Google Scholar] [CrossRef] [PubMed]
- Azad, A.I.; Rahimi, M.R.; Yun, G.J. Quantitative full-field strain measurements by SAOED (SrAl2O4:Eu2+, Dy3+) mechanoluminescent materials. Smart Mater. Struct. 2016, 25, 095032. [Google Scholar] [CrossRef]
- Adachi, S. Properties of Group-IV, II-V and II-VI Semiconductors, 1st ed.; John Wiley & Sons, Ltd.: West Sussex, UK, 2005; pp. 103–143. ISBN 0-470-09032-4. [Google Scholar]
- Keldish, L.V. The effect of strong electric field on the optical properties of insulating crystals. Sov. Phys. JETP 1958, 34, 788–790. [Google Scholar]
- Franz, V.W. Einflußeines elektrischen Feldes auf eine optische Absorptionskante. Z. Naturforschg. 1958, 13, 484–489. [Google Scholar] [CrossRef]
- Miller, D.A.B.; Chemla, D.S.; Damen, T.C.; Gossard, A.C.; Wiegmann, W.; Wood, T.H.; Burrus, C.A. Band-edge electroabsorption in quantum well structures: The quantum-confined stark effect. Phys. Rev. Lett. 1984, 53, 2173–2176. [Google Scholar] [CrossRef]
- Yacobi, B.G.; Brada, Y. Franz-Keldysh effect and internal fields in ZnS crystals. Phys. Rev. B 1974, 10, 665–670. [Google Scholar] [CrossRef]
- Peiner, E.; Guttzeit, A.; Wehmann, H.-H. The effect of threading dislocations on optical absorption and electron scattering in strongly mismatched heteroepitaxial III-V compound semiconductors on silicon. J. Phys. Condens. Matter 2002, 14, 13195–13201. [Google Scholar] [CrossRef]
- Yacobi, B.G.; Brada, Y. Defect-induced electric field in ZnS. Solid State Commun. 1976, 18, 135–138. [Google Scholar] [CrossRef]
- Chandra, B.P.; Chandra, V.K.; Jha, P. Elastico-mechanoluminescence and crystal-structure relationships in persistent luminescent materials and II–VI semiconductor phosphors. Physica B 2015, 463, 62–67. [Google Scholar] [CrossRef]
- Chandra, V.K.; Chandra, B.P. Dynamics of the mechanoluminescence induced by elastic deformation of persistent luminescent crystals. J. Lumin. 2012, 132, 858–869. [Google Scholar] [CrossRef]
- Chandra, V.K.; Chandra, B.P. Suitable materials for elastico mechanoluminescence-based stress sensors. Opt. Mater. 2011, 34, 194–200. [Google Scholar] [CrossRef]
- Chandra, B.P.; Baghel, R.N.; Luka, A.K.; Sanodiya, T.R.; Kuraria, R.K.; Kuraria, S.R. Strong mechanoluminescence induced by elastic deformation of rare-earth-doped strontium aluminate phosphors. J. Lumin. 2009, 129, 760–766. [Google Scholar] [CrossRef]
- Sasakura, H.; Kobayashi, H.; Tanaka, S.; Mita, J. The dependence of electroluminescent characteristics of ZnS:Mn thin films unpon their device parameters. J. Appl. Phys. 1981, 52, 6901–6906. [Google Scholar] [CrossRef]
- Chandra, V.K.; Chandra, B.P.; Jha, P. Strong luminescence induced by elastic deformation of piezoelectric crystals. Appl. Phys. Lett. 2013, 102, 241105. [Google Scholar] [CrossRef]
- Joos, J.J.; Lejaeghere, K.; Korthout, K.; Feng, A.; Poelman, D.; Smet, P.F. Charge transfer induced energy storage in CaZnOS:Mn—Insight from experimental and computational spectroscopy. Phys. Chem. Chem. Phys. 2017, 19, 9075–9085. [Google Scholar] [CrossRef] [PubMed]
- Huang, B.; Peng, D.; Pan, C. “Energy Relay Center” for doped mechanoluminescence materials: A case study on Cu-doped and Mn-doped CaZnOS. Phys. Chem. Chem. Phys. 2017, 19, 1190–1208. [Google Scholar] [CrossRef] [PubMed]
- Kim, J.S.; Kibble, K.; Kwon, Y.N.; Sohn, K.-S. Rate-equation model for the loading-rate-dependent mechanoluminescence of SrAl2O4:Eu2+, Dy3+. Opt. Lett. 2009, 34, 1915–1917. [Google Scholar] [CrossRef] [PubMed]
- Press, W.H.; Teukolsky, S.A.; Vetterling, W.T.; Flannery, B.P. Numerical Recipes—The art of Scientific Computing, 3rd ed.; Cambride University Press: New York, NY, USA, 2007; pp. 899–954. ISBN 978-0-521-88068-8. [Google Scholar]
- Chandra, B.P.; Chandra, V.K.; Jha, P. Microscopic theory of elastico-mechanoluminescent smart materials. Appl. Phys. Lett. 2014, 104, 031102. [Google Scholar] [CrossRef]
- Chandra, B.P.; Chandra, V.K.; Jha, P. Piezoelectrically-induced trap-depth reduction model of elastico-mechanoluminescent materials. Physica B 2015, 461, 38–48. [Google Scholar] [CrossRef]
- Van den Eeckhout, K.; Bos, A.J.J.; Poelman, D.; Smet, P.F. Revealing trap depth distributions in persistent phosphors. Phys. Rev. B 2013, 87, 0465126. [Google Scholar] [CrossRef]
- Chen, R.; Pagonis, V. Thermally and Optically Stimulated Luminescence: A Simulation Approach, 1st ed.; John Wiley & Sons Ltd.: West Sussex, UK, 2011; pp. 39–63. ISBN 9780470749272. [Google Scholar]
- Sohn, K.-S.; Park, W.B.; Timilsina, S.; Kim, J.S. Mechanoluminescence of SrAl2O4:Eu2+, Dy3+ under cyclic loading. Opt. Lett. 2014, 39, 1410–1413. [Google Scholar] [CrossRef] [PubMed]
- Dubernet, M.; Gueguen, Y.; Houizot, P.; Célarié, F.; Sangleboeuf, J.-C.; Orain, H.; Rouxel, T. Evidence and modelling of mechanoluminescne in a transparent glass particulate composite. Appl. Phys. Lett. 2015, 107, 151906. [Google Scholar] [CrossRef]
- Palmer, R.G.; Stein, D.L.; Abrahams, E.; Anderson, P.W. Hierarchically constrained dynamics for glassy relaxation. Phys. Rev. Lett. 1984, 53, 958–961. [Google Scholar] [CrossRef]
- Chen, R. Apparent stretched-exponential luminescence decay in crystalline solids. J. Lumin. 2003, 102, 510–518. [Google Scholar] [CrossRef]
- Sohn, K.-S.; Cho, M.Y.; Kim, M.; Kim, J.S. A smart load-sensing system using standardized mechano-luminescence measurement. Opt. Express 2015, 23, 6073–6082. [Google Scholar] [CrossRef] [PubMed]
- Stoneham, A.M. Theory in Solids: Electronic Structure of Defects in Insulators and Semiconductors; Clarendon Press: Oxford, UK, 2001; pp. 652–680. ISBN 978-0198507802. [Google Scholar]
- Seitz, F. Colour centers in alkali halide crystals II. Rev. Mod. Phys. 1954, 26, 7–94. [Google Scholar] [CrossRef]
- Seitz, F. Colour centers in alkali halide crystals. Rev. Mod. Phys. 1946, 18, 384–408. [Google Scholar] [CrossRef]
- Emtage, P.R. Binding of electrons, holes, and excitons to dislocations in insulators. Phys. Rev. 1967, 163, 865–872. [Google Scholar] [CrossRef]
- Hirth, J.P.; Lothe, J. Theory of Dislocations, 2nd ed.; John Wiley & Sons: New York, NY, USA, 1982; pp. 487–639. ISBN 0-471-09125-1. [Google Scholar]
- Whitworth, R.W. Charged dislocations in ionic crystals. Adv. Phys. 1975, 24, 203–304. [Google Scholar] [CrossRef]
- Metz, F.I.; Schweiger, R.N.; Leidler, H.R.; Girifalco, L.A. Stress activated luminescence in X-irradiated alkali halide crystals. J. Phys. Chem. 1957, 61, 86–89. [Google Scholar] [CrossRef]
- Chandra, B.P. Mechanoluminescence induced by elastic deformation of coloured alkali halide crystals using pressure steps. J. Lumin. 2008, 128, 1217–1224. [Google Scholar] [CrossRef]
- Senchukov, F.D.; Shmurak, S.Z. Interaction of dislocations with hole centers in colored alkali halide crystals. Zh. Eksp. Teor. Fiz. 1973, 65, 2356–2363. [Google Scholar]
- Timoshenko, Y.K.; Shunina, V.A. Electronic structure of the KCl and AgCl nanocrystals with edge dislocations. Phys. Status Solidi C 2005, 2, 1788–1791. [Google Scholar] [CrossRef]
- Shmurak, S.Z. Dislocation spectroscopy of crystals. Phys. Solid State 1999, 41, 1963–1969. [Google Scholar] [CrossRef]
- Chandra, B.P.; Bagri, A.K.; Chandra, V.K. Mechanoluminescence response to the plastic flow of coloured alkali halide crystals. J. Lumin. 2010, 130, 309–314. [Google Scholar] [CrossRef]
- Chandra, B.P.; Goutam, R.K.; Chandra, V.K.; Raghuwanshi, D.S.; Luka, A.K.; Baghel, R.N. Mechanoluminescence induced by elastic and plastic deformation of coloured alkali halide crystals at fixed strain rates. Radiat. Eff. Defects Solids 2010, 165, 907–919. [Google Scholar] [CrossRef]
- Rajput, N.; Tiwari, S.; Chandra, B.P. Temperature dependence of pulse-induced mechanoluminescence excitation in coloured alkali halide crystals. Bull. Mater. Sci. 2004, 27, 505–509. [Google Scholar] [CrossRef]
- Hayashiuchi, Y.; Hagihara, T.; Okada, T. Theory of deformation luminescence in KCl crystals. Phys. Lett. A 1990, 147, 245–249. [Google Scholar] [CrossRef]
- Sohn, K.-S.; Timilsina, S.; Singh, S.P.; Choi, T.; Kim, J.S. Mechanically driven luminescence in a ZnS:Cu-PDMS composite. APL Mater. 2016, 4, 106102. [Google Scholar] [CrossRef]
- Gan, J.; Kang, M.G.; Meeker, M.A.; Khodaparast, G.A.; Bodnar, R.J.; Mahaney, J.E.; Maurya, D.; Priya, S. Enhanced piezoluminescence in non-stoichiometric ZnS:Cu microparticle based light emitting elastomers. J. Mater. Chem. C 2017, 5, 5387–5394. [Google Scholar] [CrossRef]
- Basnet, R.; Timilsina, S.; Lee, K.H.; Kim, J.S. Evaluation of the elasto-plastic crack tip singularities via mechano-luminescent effects. Int. J. Eng. Sci. 2018, 123, 127–142. [Google Scholar] [CrossRef]
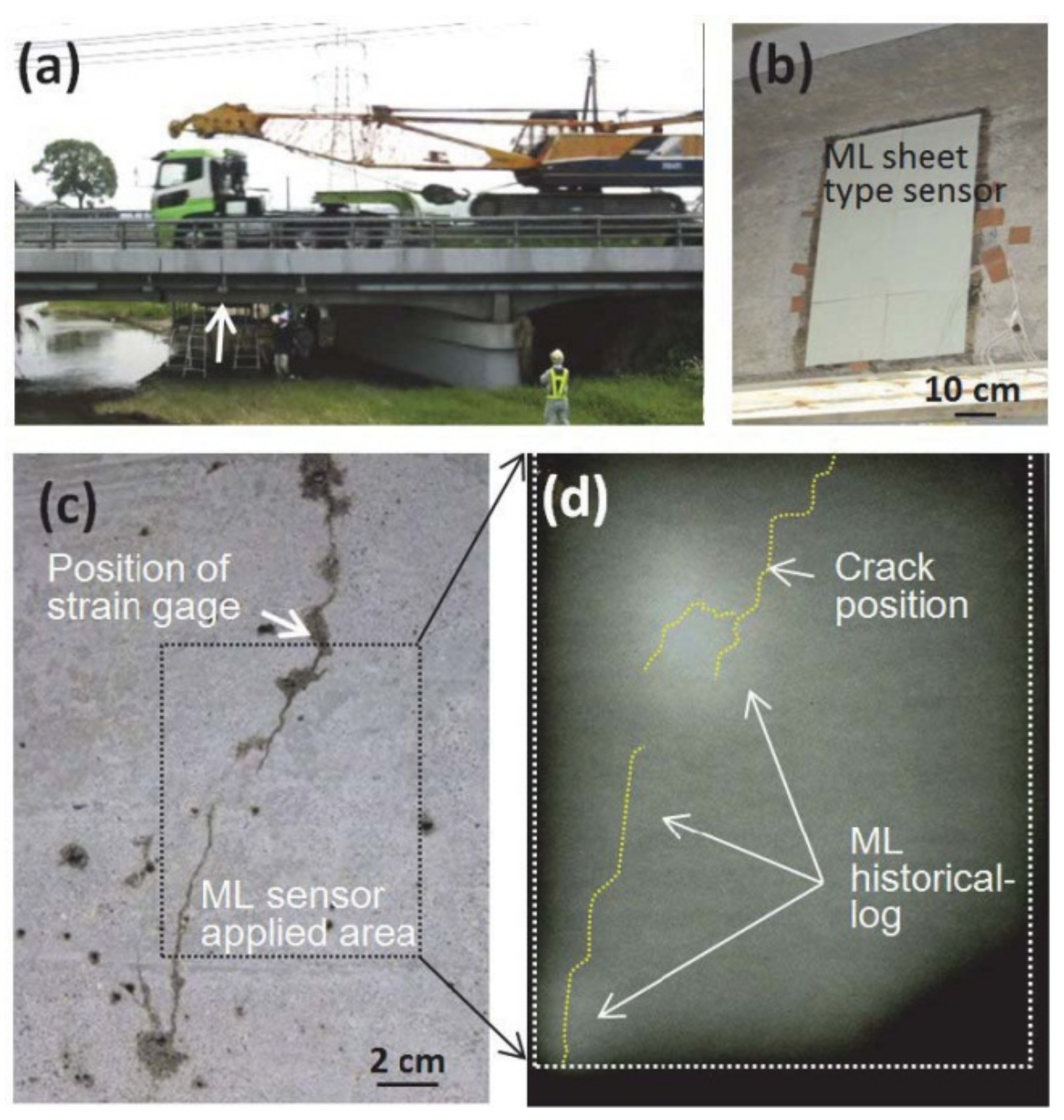
- Terasaki, N.; Xu, C.-N. Historical-log recording system for crack opening and growth based on mechanoluminescent flexible sensor. IEEE Sens. J. 2013, 13, 3999–4004. [Google Scholar] [CrossRef]
- Ueno, N.; Xu, C.-N.; Watanabe, S. Fatigue crack detection of CFRP composite pressure vessel using mechanoluminescent Sensor. In Proceedings of the 2013 IEEE Sensor, Baltimore, MD, USA, 3–6 November 2013; IEEE: New York, NY, USA, 2013; pp. 1–4. [Google Scholar]
- Fujio, Y.; Xu, C.-N.; Terasawa, Y.; Sakata, Y.; Yamabe, J.; Ueno, N.; Terasaki, N.; Yoshida, A.; Watanabe, S.; Murakami, Y. Sheet sensor using SrAl2O4:Eu mechanoluminescent material for visualizing inner crack of high-pressure hydrogen vessel. Int. J. Hydrog. Energy 2016, 41, 1333–1340. [Google Scholar] [CrossRef]
- Wang, X.; Que, M.; Chen, M.; Han, X.; Li, X.; Pan, C.; Wang, Z.L. Full Dynamic-range pressure sensor matrix based on optical and electrical dual-mode sensing. Adv. Mater. 2017, 29, 1605817. [Google Scholar] [CrossRef] [PubMed]
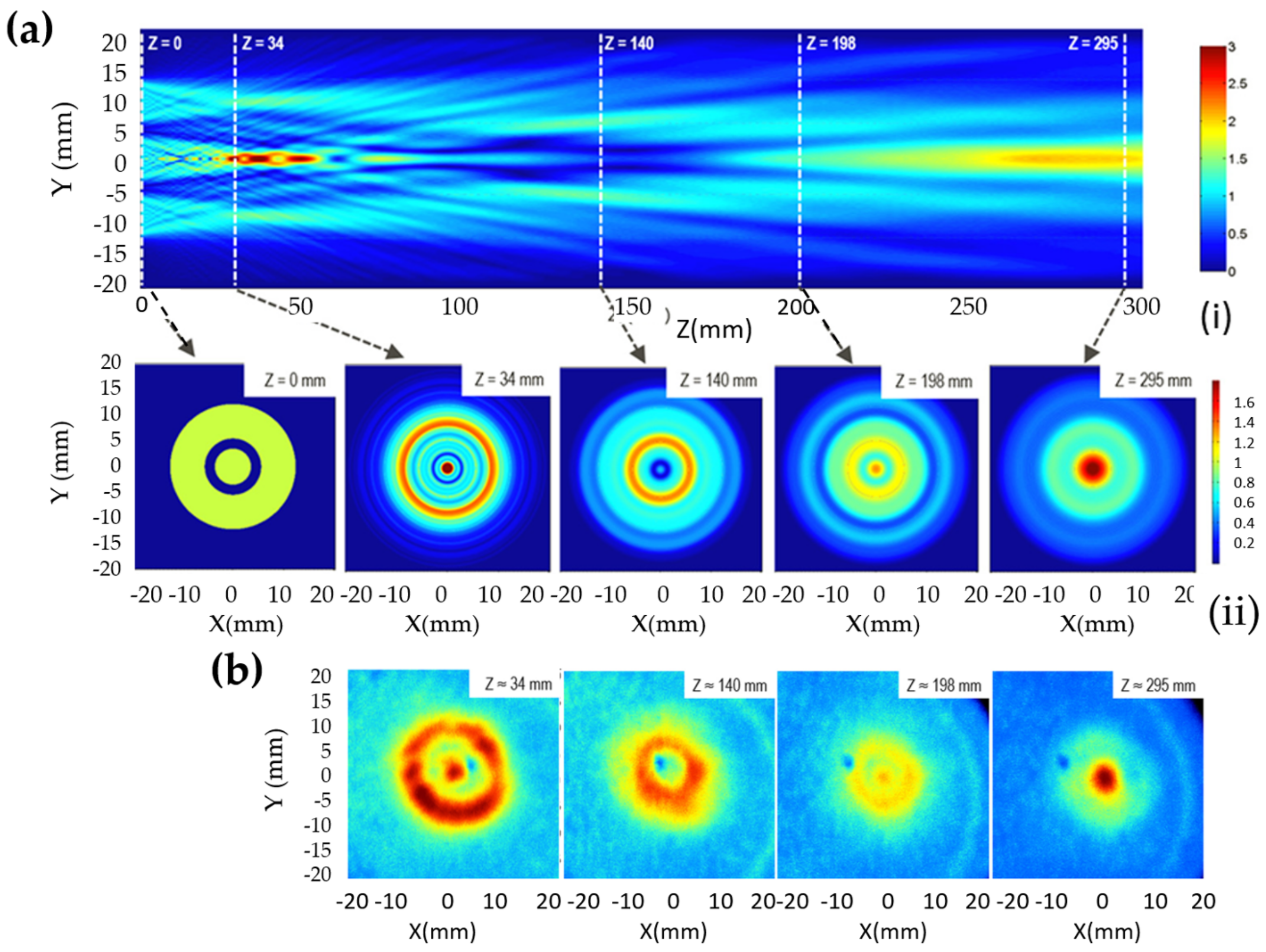
- Terasaki, N.; Yamada, H.; Xu, C.-N. Ultrasonic wave induced mechanoluminescence and its application for photocatalysis as ubiquitous light source. Catal. Today 2013, 201, 203–208. [Google Scholar] [CrossRef]
- Terasaki, N.; Xu, C.-N. Performance of single mechanoluminescent particle as ubiquitous light source. J. Colloid Interface Sci. 2014, 427, 62–66. [Google Scholar] [CrossRef] [PubMed]
- Sadd, M.H. Elasticity: Theory, Application, and Numerics, 1st ed.; Elsevier Butterworth-Heinemann: Oxford, UK, 2005; pp. 186–189. ISBN 0-12-605811-3. [Google Scholar]
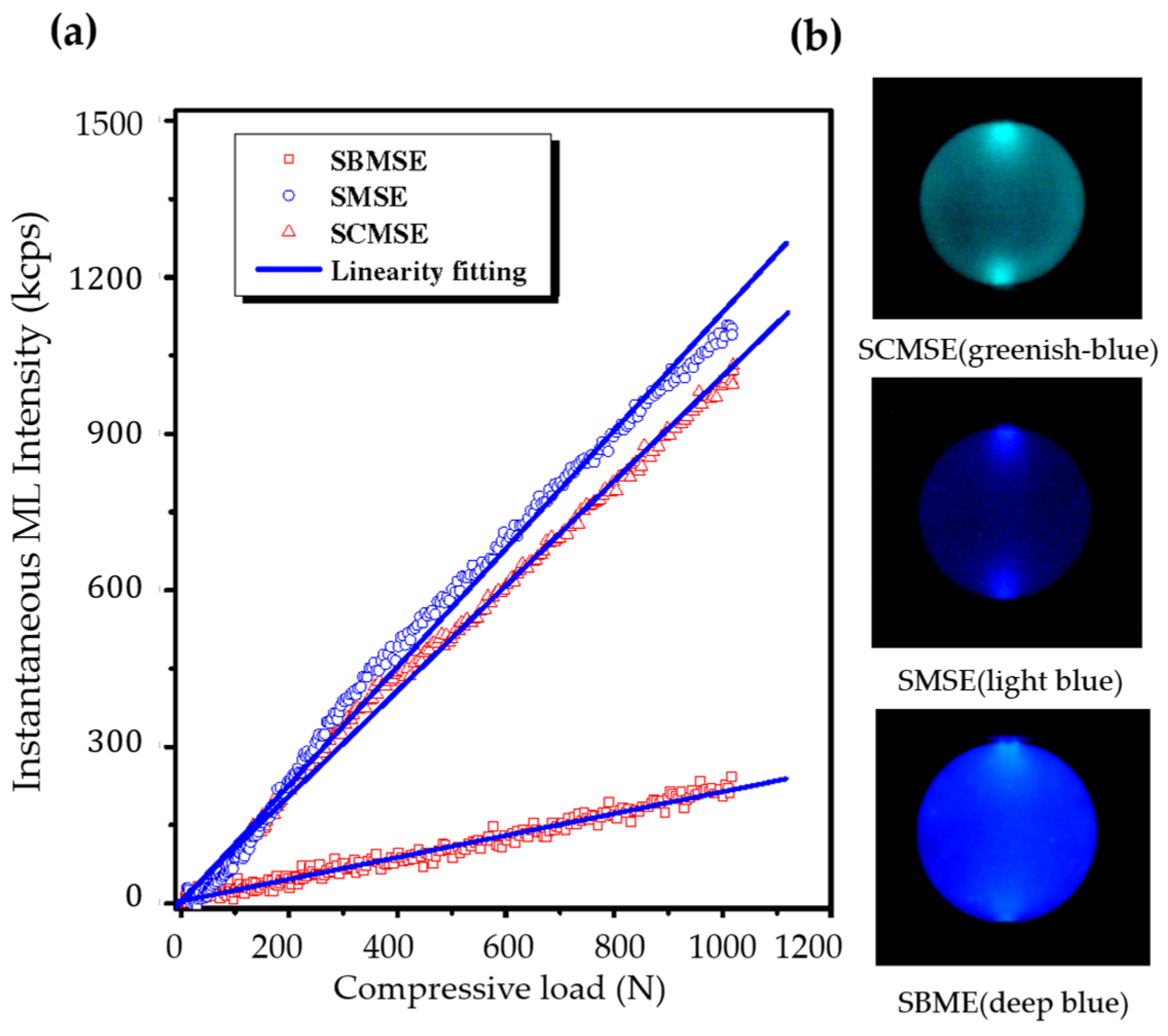
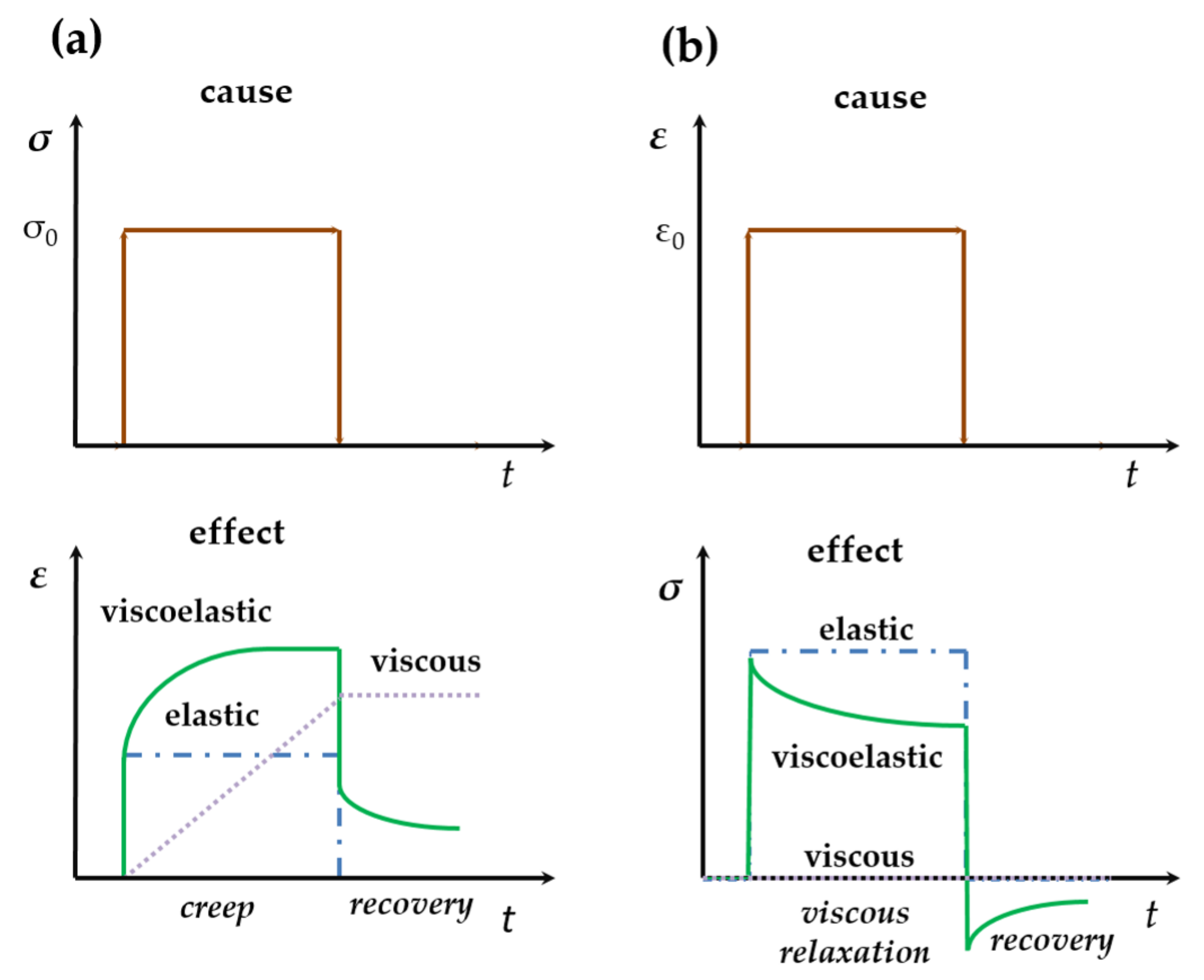
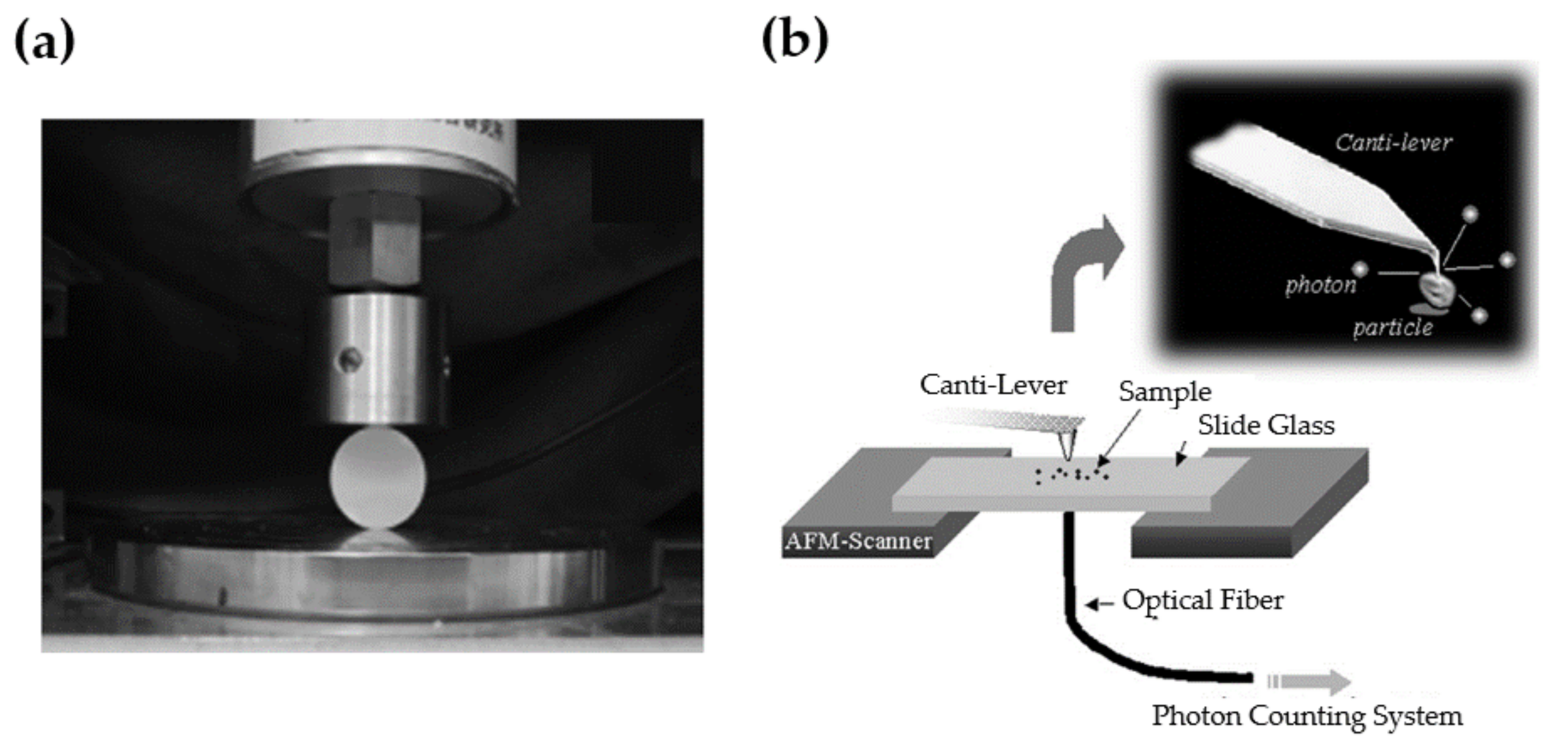
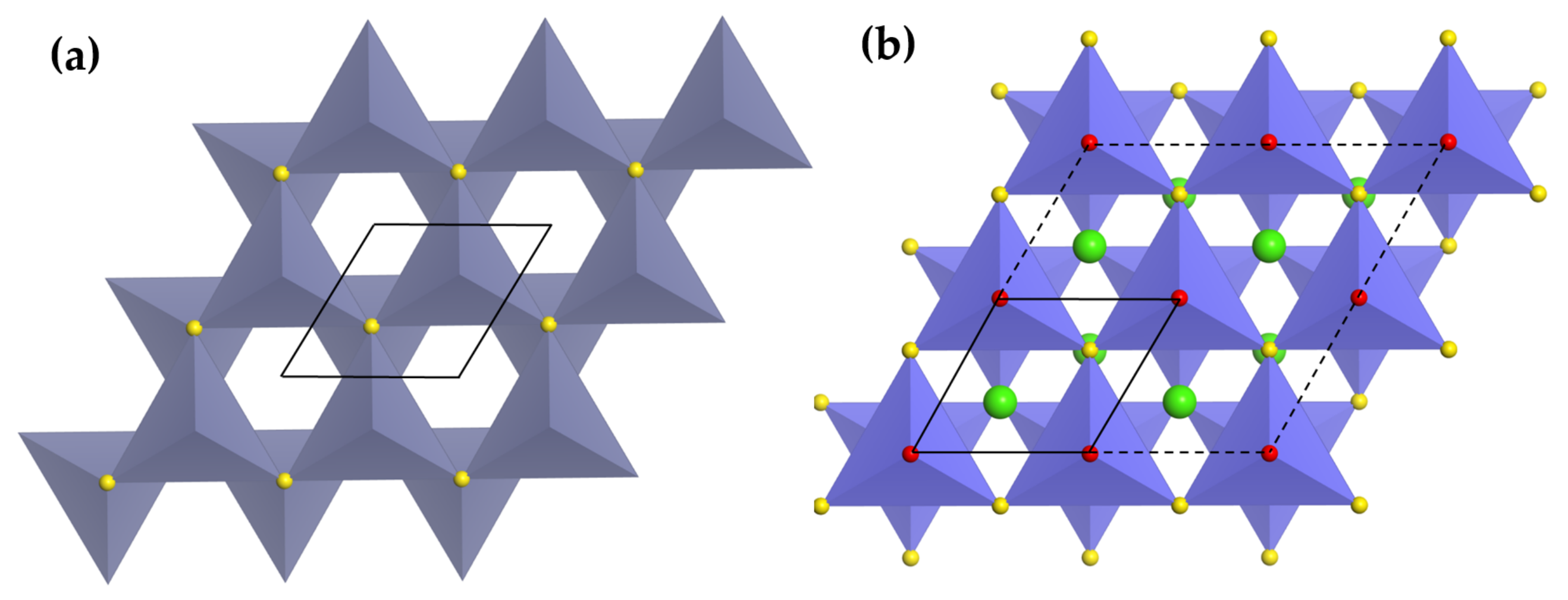





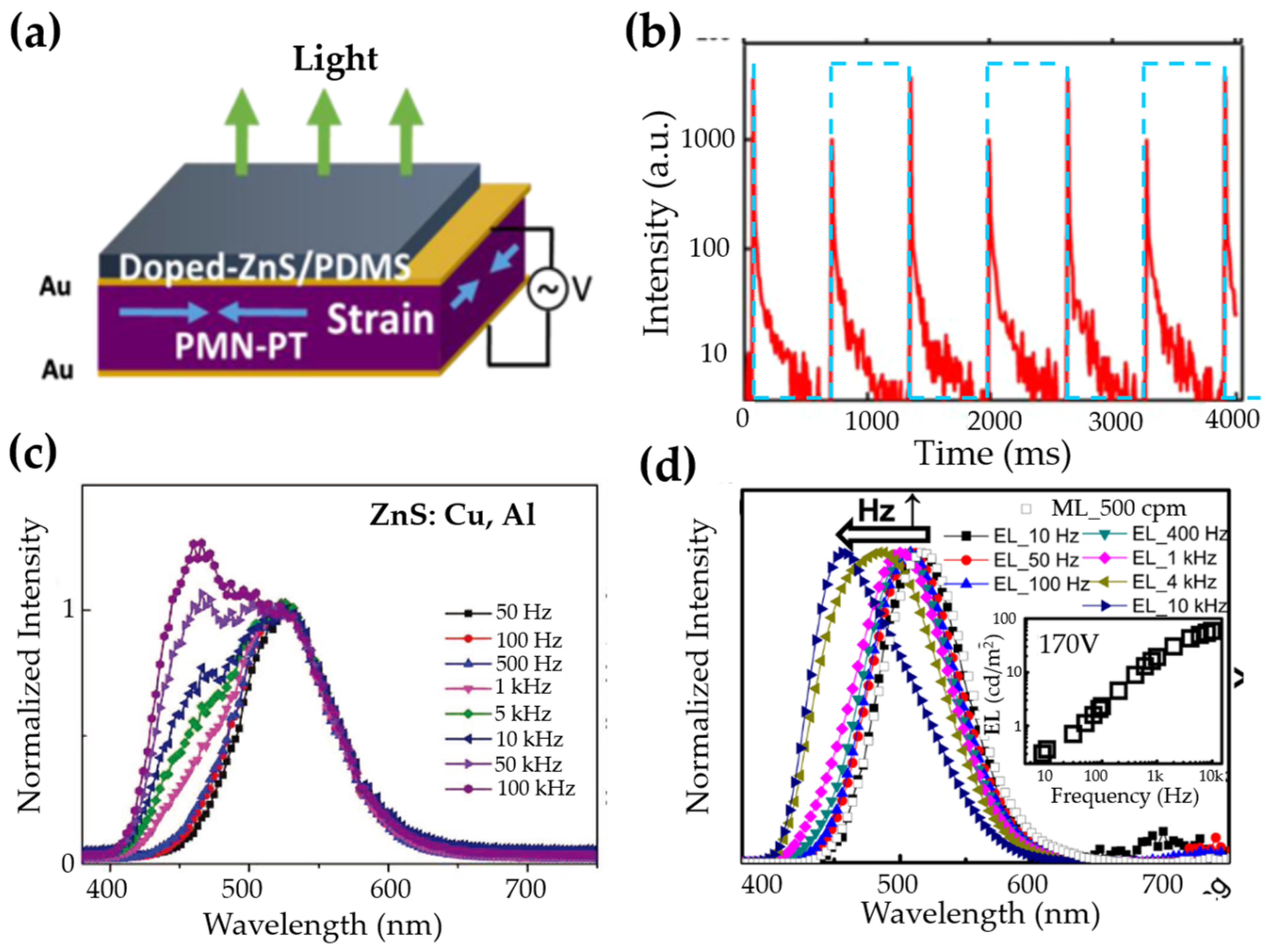
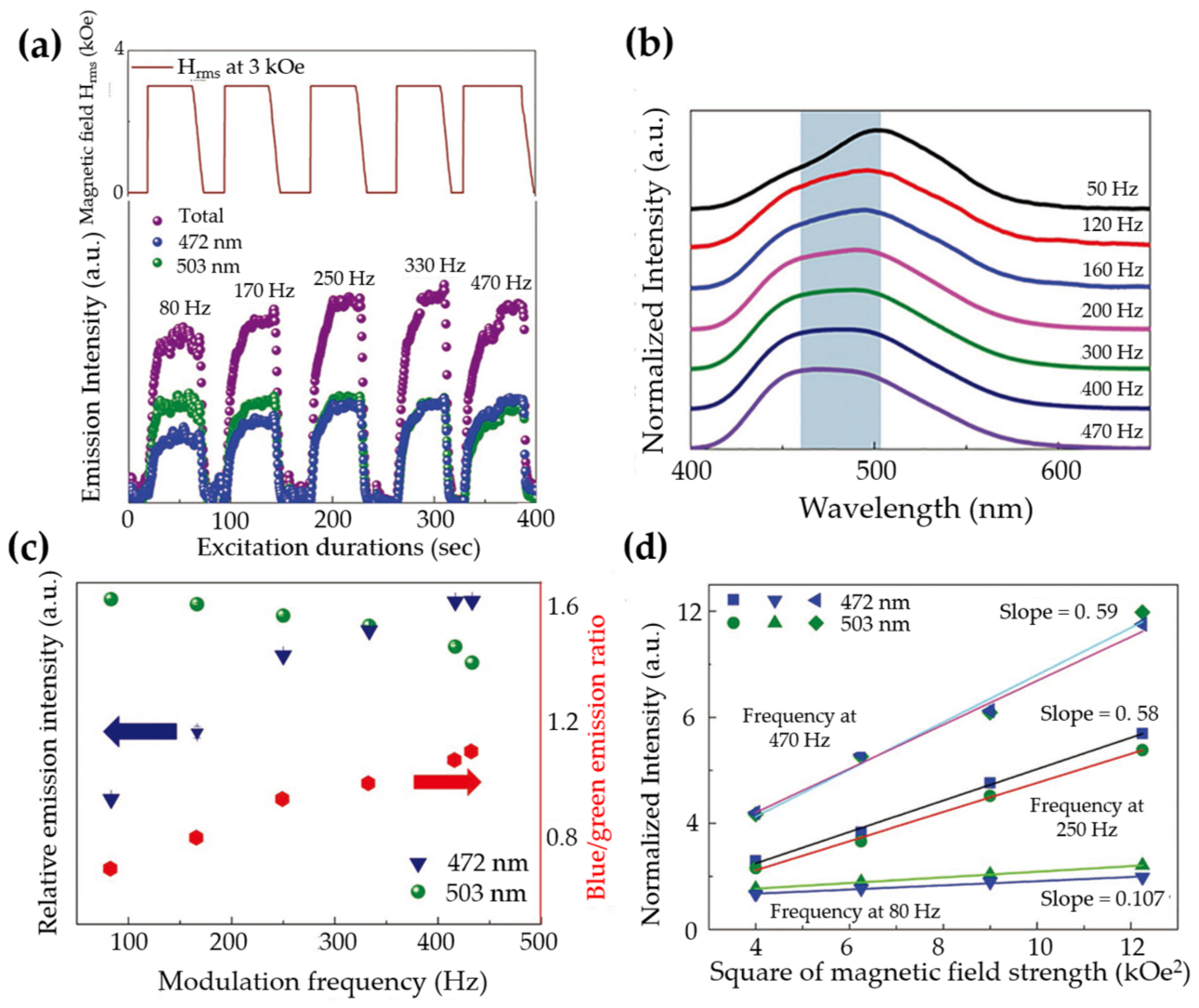
| Structure | Host | Space Group | Piezo | Dopants | (nm) | PerL, Trap Depth (eV) | Load | ML Indicators |
|---|---|---|---|---|---|---|---|---|
| Rock Salt | KCl | Fmm | − | - | 430, 520 | + [63,64], 0.22, 0.37, 0.51, 1.12 [65] | E, P [63,64,66,67] | ?, ? |
| KCl | Fmm | − | Eu | 420 | + [68] | E, P [69] | ?, ? | |
| KI | Fmm | − | - | 515 | + [63,64], 0.20, 0.21, 0.22, 0.29, 0.32, 0.43 [70] | E, P [63,64] | ?, ? | |
| KBr | Fmm | − | - | 465 | + [63,64], 0.27, 0.28, 0.29, 0.35, 0.36, 0.44 [71] | E, P [63,64] | ?, ? | |
| NaF | Fmm | − | - | 270, 306, 334, 410, 610 | + [64], 0.31, 0.57, 0.67, 0.84, 1.0, 1.22 [70] | E, P [64] | ?, ? | |
| NaCl | Fmm | − | - | 282, 318, 362, 610 | + [63,64], 0.74, 0.86, 0.99, 1.20, 1.47 [71] | E, P [63,64] | ?, ? | |
| LiF | Fmm | − | - | 290, 560, 650 | +, 0.25, 0.26, 0.36, 0.46, 0.53, 0.78 [70] | E, P [63,64,72] | ||
| RbCl | Fmm | − | - | 510, 430 | + [64], 1.65 [73] | E, P [64] | ?, ? | |
| RbBr | Fmm | − | - | 465, 495 | + [64], 1.55, 1.78 [74] | E, P [64] | ?, ? | |
| RbI | Fmm | − | - | 525, 630 | + [64], ? | E, P [64] | ?, ? | |
| MgO | Fmm | − | - | 420, 520 | ?, 0.31 [75] | E, P [76] | (0.1, ?), ? | |
| Tridymite | SrAlO | P2 | + | Eu | 520 | +, 0.77 [77,78] | E, T [79] | (40, 900) [19], - |
| SrAlO | P2 | + | Eu/Dy | 520 | +, 0.65 [77,78] | E [80,81,82] | (0, >1.0 k), - | |
| SrAlO | P2 | + | Eu/Er | 524, 1530 | + [77,78], 0.75 [83] | E [84] | (30, 750)(IR), - | |
| SrAlO | P2 | + | Ce | 381, 450 | + [85], 0.75 [86] | E [87] | (∼0, 500), 0.005 | |
| SrAlO | P2 | + | Ce/Ho | 381, 450 | + [85], ? | E [87] | (30, 700), 0.052 | |
| SrAlO | P2 | + | Eu/Dy/Nd | 520 | + [88] , ? | E, P [89,90] | -, - | |
| SrAlO | P2 | + | Eu/B | 520 | +, 0.77 | E, T | ?, - | |
| CaAlO | P2/n | − | Eu | 440 | + [91], 0.42, 0.53, 0.58 [92] | I [93] | -, - | |
| CaAlO | P2/n | − | Tb | 495,545 | +, 0.66, 1.08 [94] | I [95] | -, - | |
| SrBaAlO (x = 0, 0.1, 0.2, 0.4) | P6(x = 0.4) P2(others) | + | Dy | 480,575 | ?, ? | T [96] | -, - | |
| SrBaAlO (x = 0, 0.1, 0.2, 0.4) | P6(x = 0.4) P2(others) | + | Eu/Eu | 520, 613, 700 | + [97], ? | T [98] | -, - | |
| SrCaAlO | P2 | + | Dy | 480, 575 | +, ? | T [96] | -, - | |
| ZnGeSiO | R3 | + | Mn | 535 | +, 40 C [99] | E [100] | (600, 2.8k), ∼10 | |
| Spinels | MgGaO | Fdm | − | Mn | 506 | +, 60 C [101] | T [101] | ?, ? |
| ZnGaO | Fdm | − | Mn | 505 | +, 70 C [102] | T [101] | ?, ? | |
| ZnAlO | Fdm | − | Mn | 560 | +, 125 C [103] | T, I [103,104,105] | ?, ? | |
| Wurtzite | ZnS | P6mc | + | Mn | 585 | −, 0.3 [106] | E, P, T [107,108,109,110,111,112,113,114,115,116,117] | (0.6, 50), 0.7|2.2 |
| ZnS | P6mc | + | Cu | 520 | + [118], 0.20–0.30, 0.42–0.46, 0.54–0.60 [119,120] | E [14,121,122,123,124,125,126] | ?, ? | |
| ZnS | P6mc | + | Mn/Cu(Al) | 517, 587 | +, ? | E [13,17,22,127,128] | ?, ? | |
| ZnS | P6mc | + | Cu/Al | (475), 525 | +, ? | E [22,123] | ?, ? | |
| ZnTe | P6mc | + | Mn | ? | −, ? | T [129] | ∼0, ? | |
| (ZnS)(MnTe) (x < 1/4) | P6mc | + | Mn | 650 | ?, ? | I [130,131,132] | ?, ? | |
| CaZnOS | P6mc | + | - | 438, 515, 614 | −, 0.17, 0.58 [133] | T, E, I [133] | ?, ? | |
| CaZnOS | P6mc | + | Mn | 580 | −, 0.18, 0.58, 0.67 [134] | T, E [134,135] | (0, 1.0 k), ∼0.325 | |
| CaZnOS | P6mc | + | Cu | 450, 530 | +, 0.42, 0.71 [136] | E [136,137,138] | (0, 350), ? | |
| CaZnOS | P6mc | + | Er | 525, 540, 560 | − [139], ? | E [139] | ?, ? | |
| CaZnOS | P6mc | + | Sm | 566, 618, 655 | −, ? | E, T [140] | (13, 65), 0.48 | |
| BaZnOS | Cmcm | − | Mn | 634 | + [141], 0.83 [142] | E [141,142] | (∼100, 5.0 k), 1.0 | |
| Melilite | CaMgSiO | P2m | + | Eu | 530 | + [143], 90 C [144] | E [145] | (0, 1.0 k), < |
| CaMgSiO | P2m | + | Eu/Dy | 530 | +, 0.66 [143,146,147] | E [148,149,150] | (∼70, 1.0 k), 1.433 | |
| SrMgSiO | P2m | + | Eu | 499 | + [151], 0.50 [11] | E [11] | (∼70, ?), 1.134 | |
| SrMgSiO | P2m | + | Eu/Dy | 460 | +, 0.75 [147,152,153] | I [148,154] | ?, ? | |
| BaMgSiO | C2/c | + | Eu/Dy | 495 | + [155], ? | I [156] | ?, ? | |
| SrCaMgSiO | P2m | + | Eu | 496 | +, 0.47 [11] | E [157] | (∼70, 1.0 k), 1.02 | |
| SrBaMgSiO | P2m | + | Eu | 440 | +, 0.55 [11] | E [11] | (∼0, 1.0 k), 0.213 | |
| SrMgSiO () | P2m | + | Eu | 468 | +, 0.45, 0.78 [158] | E [158] | ?, ? | |
| CaAlSiO | P2m | + | Ce | 417 | +, 90 C [159] | E [23,160] | (220, 1.0 k), - | |
| SrAlSiO | P2m | + | Eu | 484 | +, 0.88 [161] | I [162,163] | -, - | |
| SrAlSiO | P2m | + | Eu/Dy | 484 | +, 0.52 [161] | I [162,163] | -, - | |
| CaYAlO | P2m | + | Eu | 440 | + [164], ? | E [165] | (30, 1.0 k), 0.03 | |
| CaYAlO | P2m | + | Ce | 425 | +, 80 C [159] | E [166] | (10, 1.0 k), - | |
| Anorthite | CaAlSiO | P | − | Eu | 430 | +, 0.68, 0.82 [167] | E [168] | (33, >1.0 k), 0.748 |
| CaSrAlSiO () | I | − | Eu | 406–440 | + [169], ? | E [168,170,171] | (44, 400), 1.12 (x = 0.4) | |
| SrMg(PO) | Pm | + | Eu | 412 | +, 0.77 [172] | E [57] | (∼0, >1.0 k), - | |
| Perovskite | BaCaTiO () | P4mm+Pbnm | + | Pr | 612 | + [173], 0.356 [174] | E [175,176,177,178] | (70, >1.0 k), 0.006 |
| BaCaTiO | P4mm+Pbnm | + | Pr/Dy | 612 | +, 0.35 [173] | E [174,179] | (∼0, >900), 0.003 | |
| BaCaTiO | P4mm+Pbnm | + | Pr/La-Lu | 612 | +, 0.31–0.38 [173], 0.00 for Ce [173] | E [174] | ?, ? | |
| LiNbO | R3c | + | Pr | 612 | ?, ? | E [180] | (30, 1.0 k)?, - | |
| SrSnO | Pccn | − | Sm | 575, 625, 660 | + [181], 117 C, 187 C [182] | E [183] | (15, >250), - | |
| (Ca, Sr, Ba)SnO | Pccn | − | Sm/La | 575, 625, 660 | +, 0.56, 0.84, 1.35 [185,186] | E [186] | (20, 100), - | |
| SrSnO | Amam | − | Sm | 575, 625, 660 | + [184], ? | E [183] | (15, 250)?, - | |
| Sr(Sn, Si)O | Amam | − | Sm | 575, 625, 660 | +, 113 C [187] | E [187] | (?, ∼2350), ∼1.3 | |
| Sr(Sn, Ge)O | Amam | − | Sm | 575, 625, 660 | +, 117 C [187] | E [187] | (?, ∼2350), ∼2.1 | |
| CaTiO | Ccm2 | + | Pr | 615 | +, 0.69–0.92 [188] | E,T [189] | (∼10, 200), - | |
| CaNbO | Pbcn | − | Pr | 613 | − [29], 0.71, 0.81, 1.04 [29] | E [29] | (∼30, 1.0 k), 0.001 | |
| CaNbO | P2 | + | Pr | 613 | + [29], 0.60, 0.89, 1.04, 1.27 [29] | E [29] | (∼0, 1.0 k), 0.016 | |
| CaNbO | Fm3m or P4/nnc | + | Pr | 613 | − [29], 0.74 [29] | E [29] | (∼50, 1.0 k), 0.004 | |
| MSiON (M = Ca, Sr, Ba) | BaSiON | Cmcm | + | Eu | 498 | + [190], 0.46, 0.48 [191] | E [192] | -, - |
| SrSiON | P1 | + | Eu | 498 | +, 100 C [190] | E [192] | -, - | |
| Others | CaZr(PO) | Pna2 [193] | + | Eu | 474 | +, 0.69, 0.83, 1.17, 1.87 [194] | E [194] | (5, >2.0 k), - |
| ZrO | P2/c | − | Ti | 470 | + [195], 0.81, 1.00, 1.16 [196] | E [197,198] | (∼0, 100), - |
| Host | HT Phase | Atomic Order | Domains | Twins |
|---|---|---|---|---|
| SrAlO | P6 (680–860 C) P622 (>860 C) | Al displacement, modulation q = (0, 0.5, 0) [30,247,248] | translational, ferroelastic, ferroelectric [30,247,248] | 3 types [30,247,248] |
| BaAlO | P622 [249,250,251] | modulation q = (0.5, 0, 0) (<250 K) [252] | translational, orientational, nano, APBs [249,250] | 180 rotational twins [249,250] |
| SrCaAlO | P622, C2 [253] | ? | ferroelectric | ? |
| SrBaAlO () | P622, C2 [253] | Al displacement, modulation q = (0, 0.5, 0) [253] | ferroelastic ferroelectric | twins [247] |
| SrBaAlO | P622 (>500 K) [214] | [AlO] fluctuation, O disorder [253,254] | ferroelectric [253,254] | ? |
| MgGaO | ? | Mg disorder, inverse spinel [101] | ? | ? |
| CaAlSiO | I [255] (>514 K) | ∼8% Al, Si disorder [256] | APBs [257] | twins [258]//(010) |
| SrAlSiO | Immm [259] | Al, Si disorder | APBs [259] | twins [259] |
| BaAlSiO | Immm [260] | ? | APBs [260] | ? |
| CaSrAlSiO () | I [261] | ? | ? | ? |
| CaSrAlSiO ( 0.60–0.85) | I [262,263] | O disorder [264] | APBs [264] | albite twins [264] |
| CaSrAlSiO () | I | Al-Si disorder [256] | ? | ? |
| CaMgSiO | P [265] (>355 K) | modulation [223] | micro [225,266] | ? |
| CaAlSiO | P | Al-Si order@T, Al partly order@T | ? | ? |
| CaSrMgSiO () | P 350–295 K | ‘Titan’ modulation, Sr order [225,266] | micro [225,266] | ? |
| Ca(AlMg)-(AlSi)O | ? | Al, Si order [228] | twins [228] | |
| CaTiO | ? | Ti displacement | ? | twins [267,268] |
| BaTiO | Pmm | Ti displacement | ferroelectric ferroelastic | twins [269] |
| LiNbO | Rc (>1470 K) | Li [180] vacancies | ? | ? |
| SrSnO | Bmab [270] (400–600 K) Imm [270] (>600 K) | vacancies | ? | ? |
| -CaZr(PO) | Pnma [193] (>1000 C) | ? | ? | ? |
| BaSiON | ? | disorder [241] | domains [192,241] | twins [271] |
| SrSiON | ? | ? | domains [271] | ? |
| ZrO | Pmc [272] (>1500 K) | ? | ? | ? |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Feng, A.; Smet, P.F. A Review of Mechanoluminescence in Inorganic Solids: Compounds, Mechanisms, Models and Applications. Materials 2018, 11, 484. https://doi.org/10.3390/ma11040484
Feng A, Smet PF. A Review of Mechanoluminescence in Inorganic Solids: Compounds, Mechanisms, Models and Applications. Materials. 2018; 11(4):484. https://doi.org/10.3390/ma11040484
Chicago/Turabian StyleFeng, Ang, and Philippe F. Smet. 2018. "A Review of Mechanoluminescence in Inorganic Solids: Compounds, Mechanisms, Models and Applications" Materials 11, no. 4: 484. https://doi.org/10.3390/ma11040484
APA StyleFeng, A., & Smet, P. F. (2018). A Review of Mechanoluminescence in Inorganic Solids: Compounds, Mechanisms, Models and Applications. Materials, 11(4), 484. https://doi.org/10.3390/ma11040484





