Design and 4D Printing of Cross-Folded Origami Structures: A Preliminary Investigation
Abstract
:1. Introduction
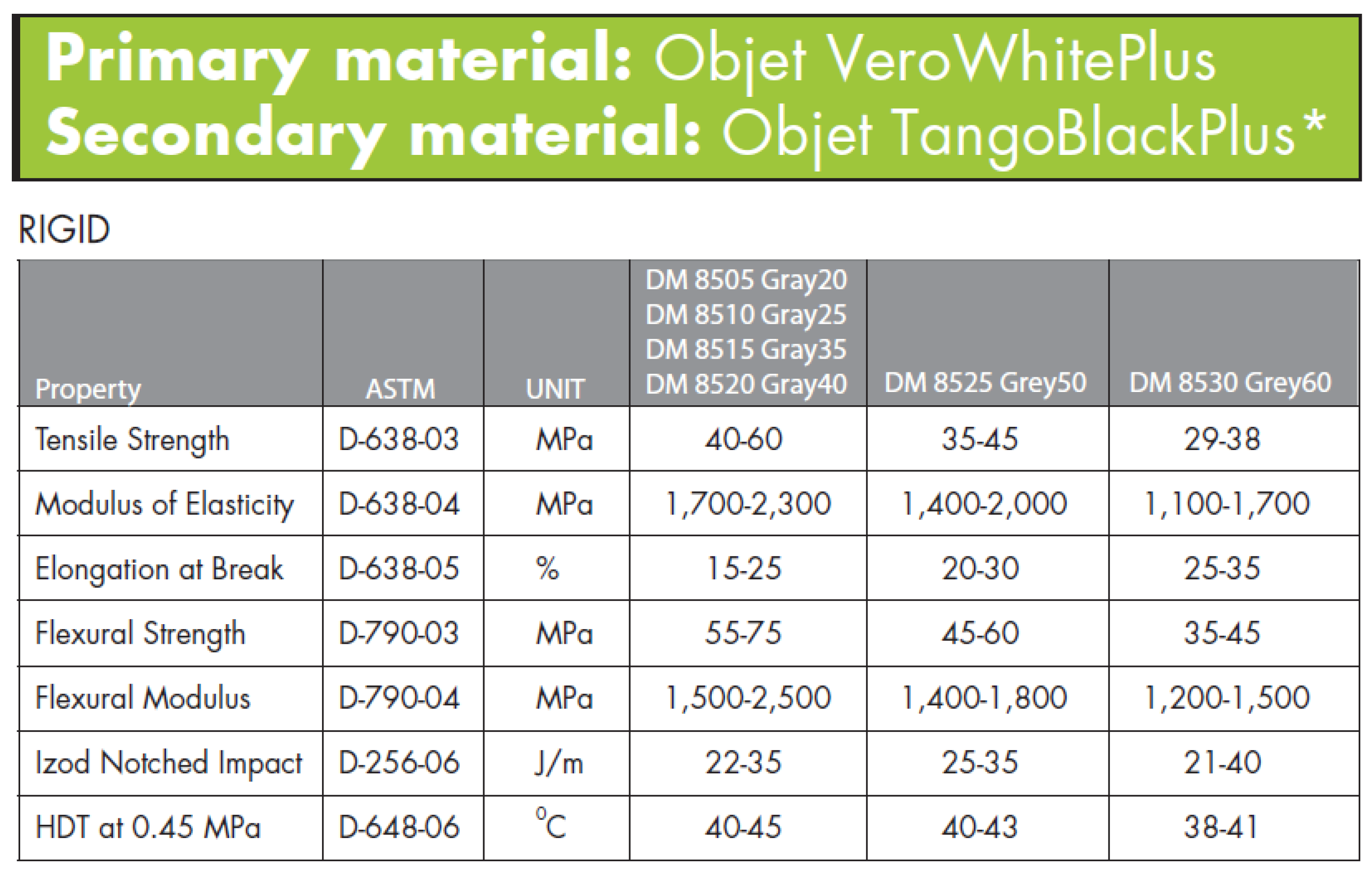
2. Materials and Methods
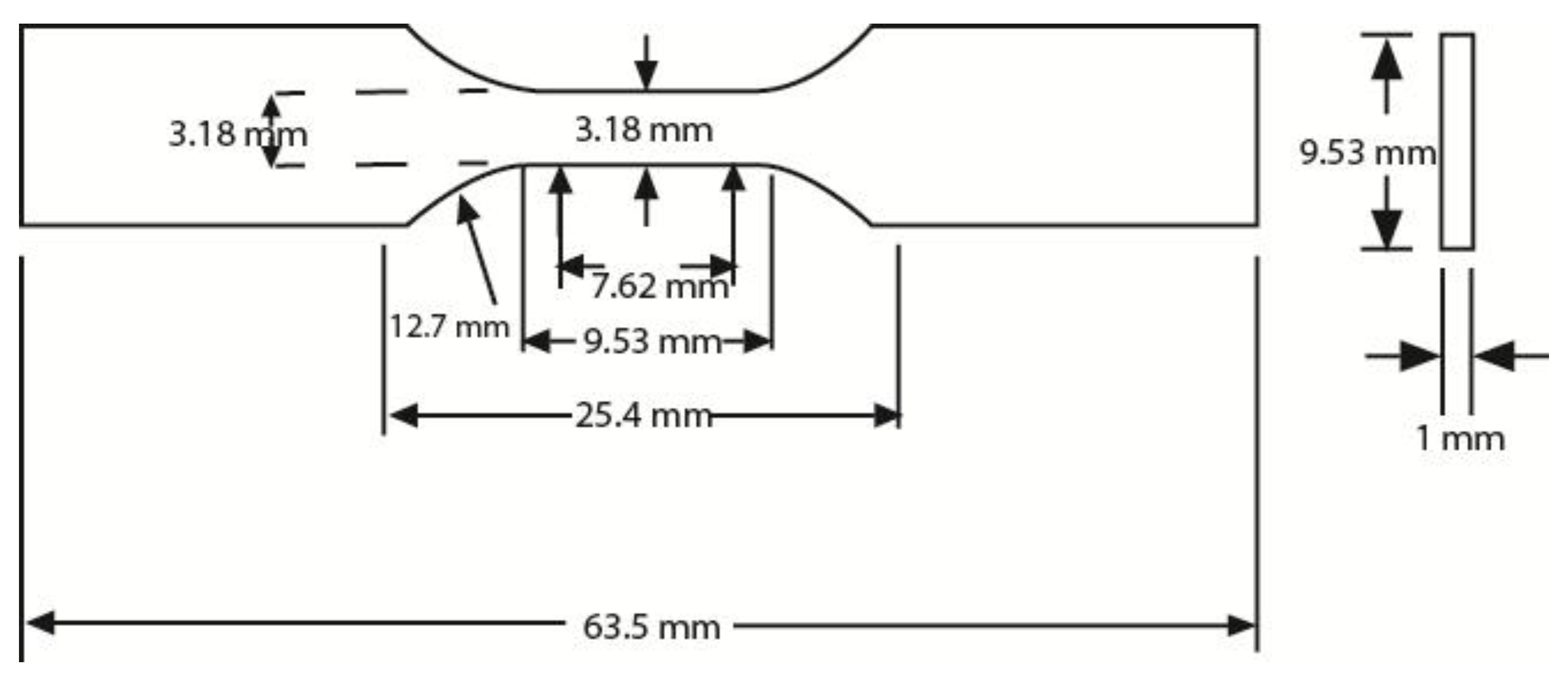
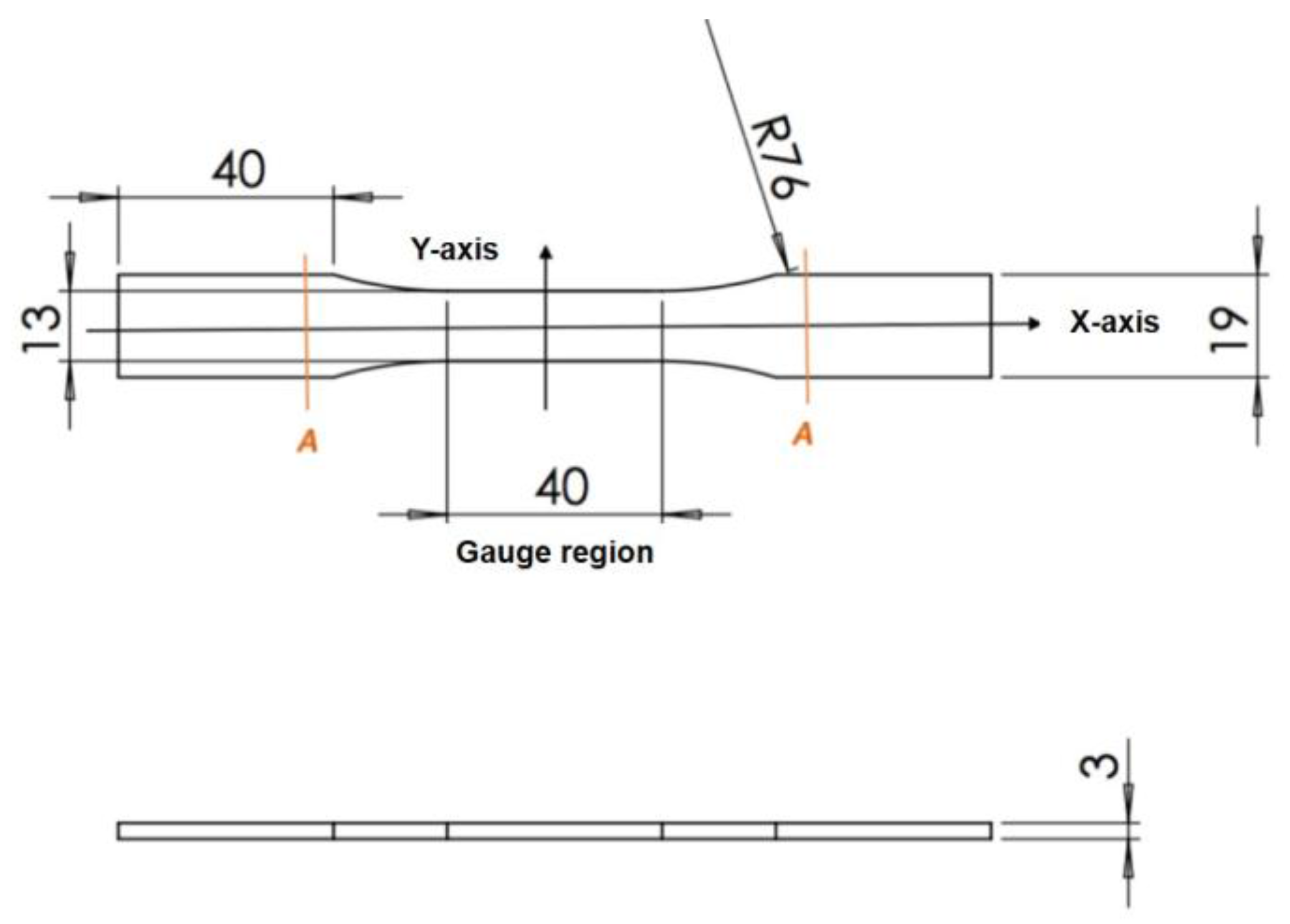
2.1. Preparation of Single Material Specimens and Tensile Test
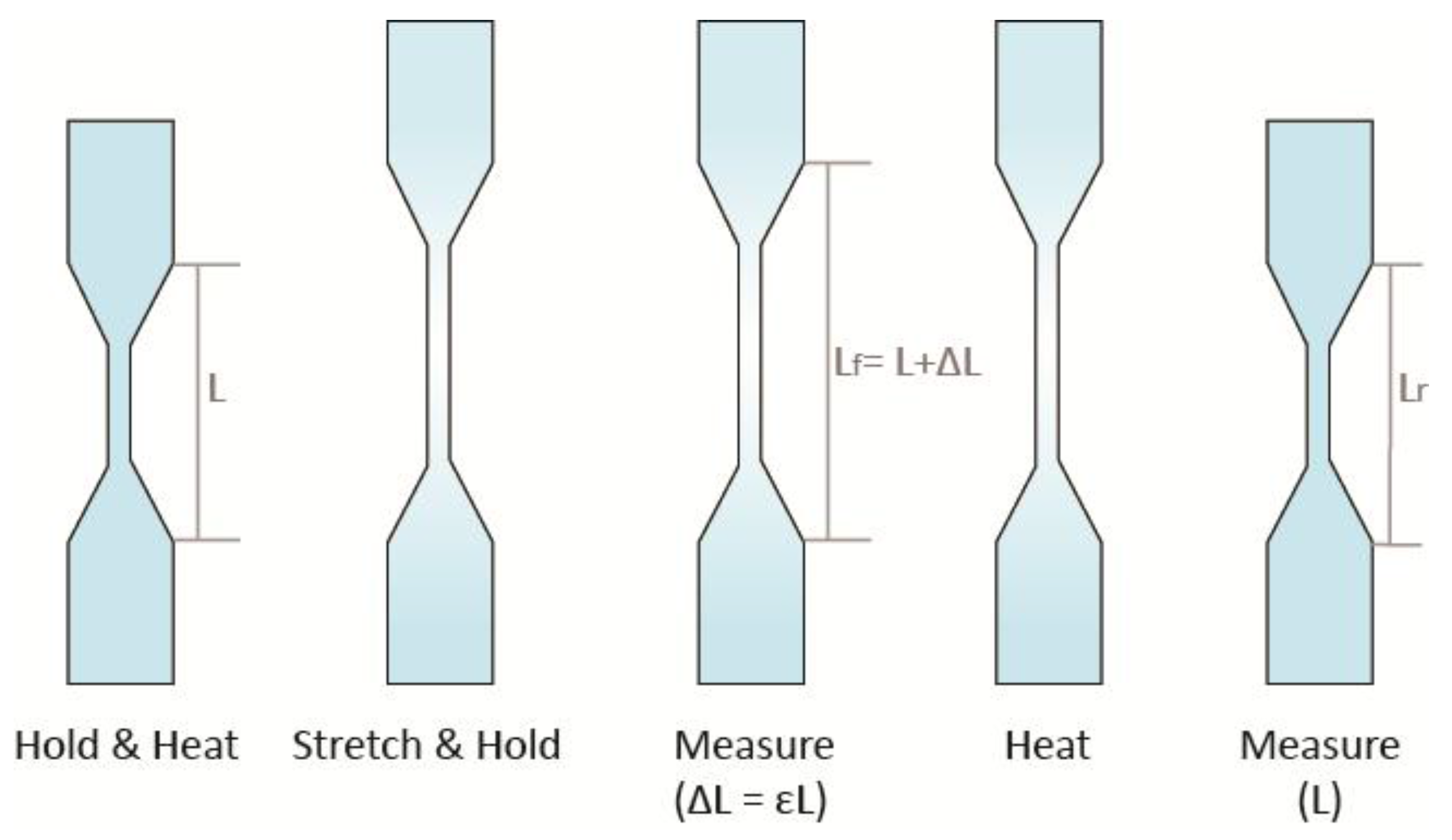
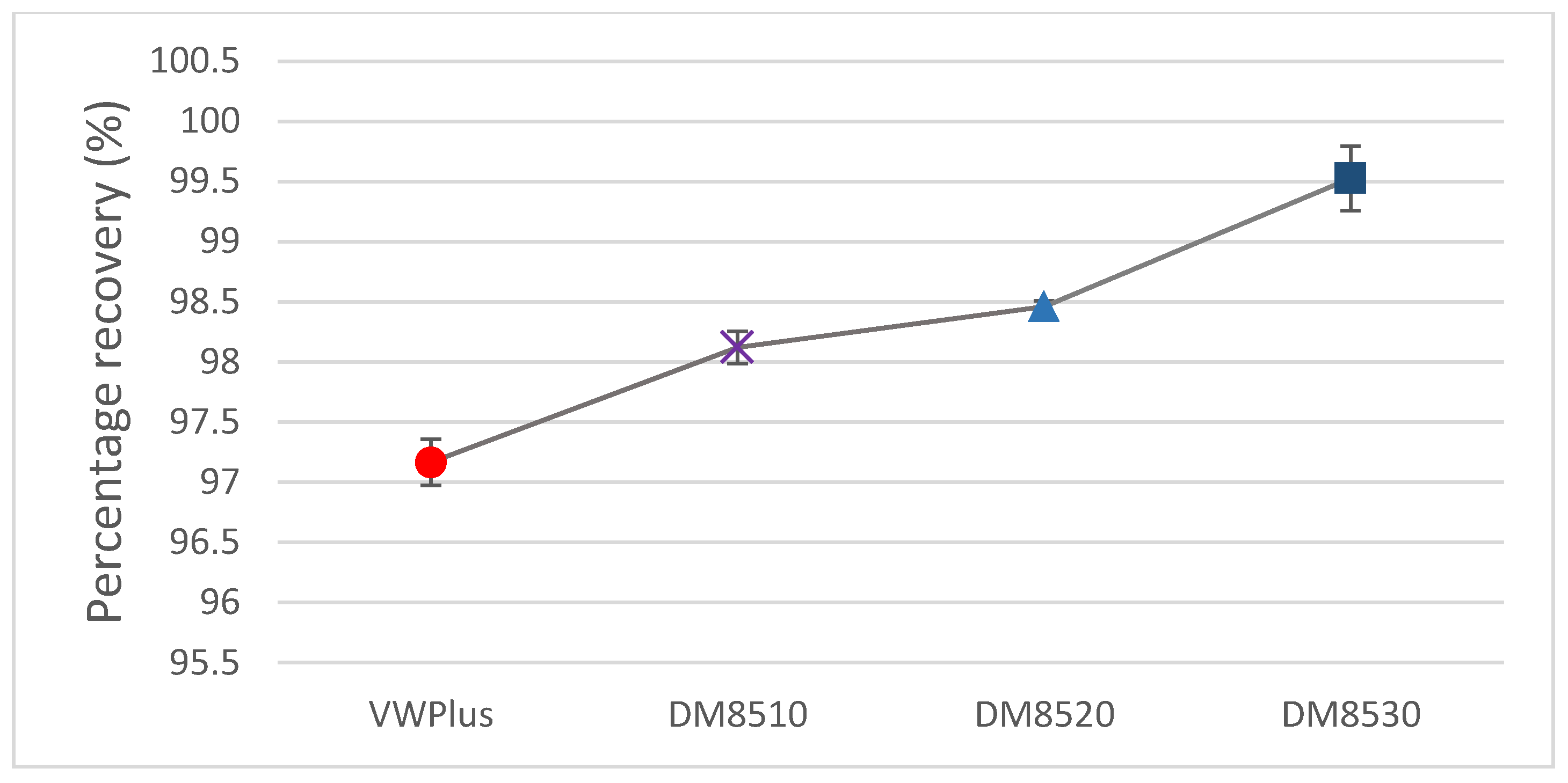
2.2. Calculation of Shape Recovery for Single Material Specimens
- For every specimen of four different materials, the specimen was held using tensile grips at a room temperature of 25 °C.
- The displacement of the crosshead was set to 3.5 mm and the load calibration was applied.
- The chamber was heated to an equilibrium temperature of 70 °C and tensile testing was performed.
- After the tensile test was completed, the chamber temperature was reduced to 25 °C with the specimen still being held by tensile grips and the change in length was measured.
- The specimen was placed in a hot water batch of 70 °C for shape recovery and the change in length was measured again.
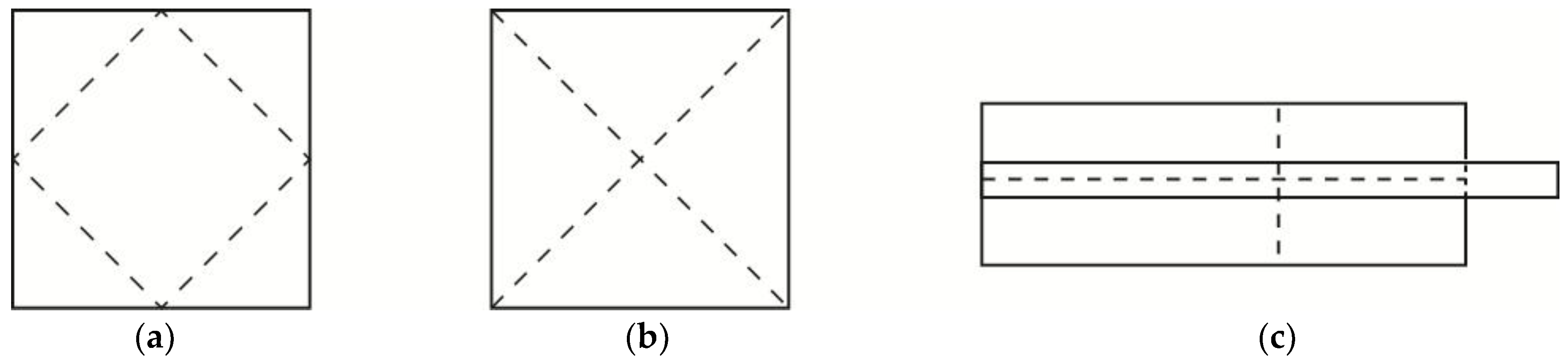
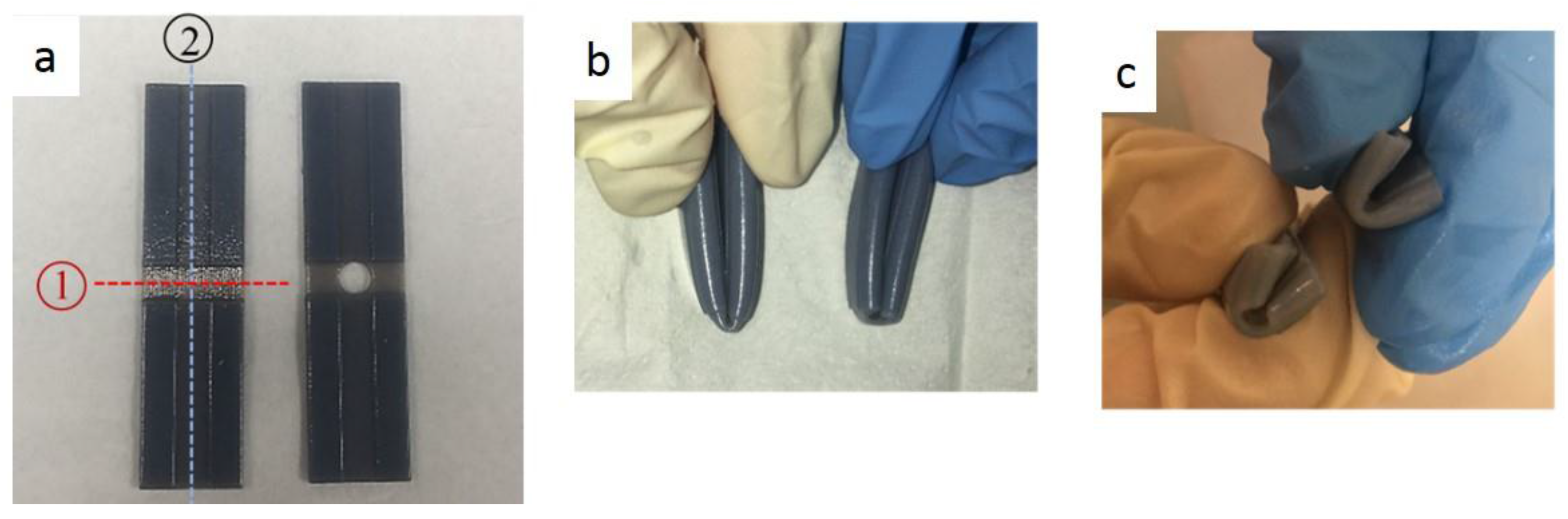
2.3. Design and Crossfolding of Single Material Smart Structures
2.4. Preparation of Multimaterial Specimens for Tensile Test
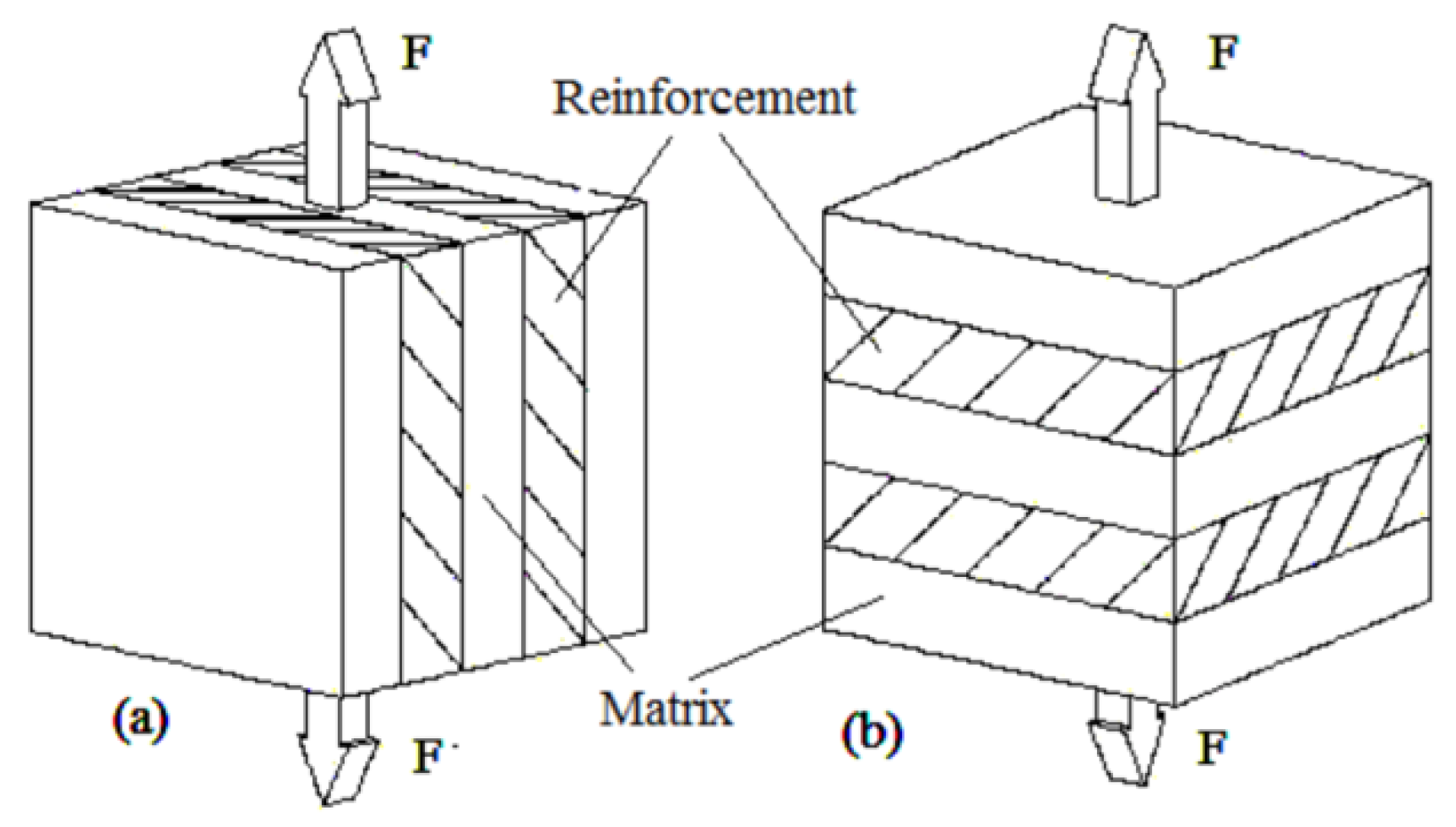
2.5. Rule of Mixtures for Multimaterial Specimens
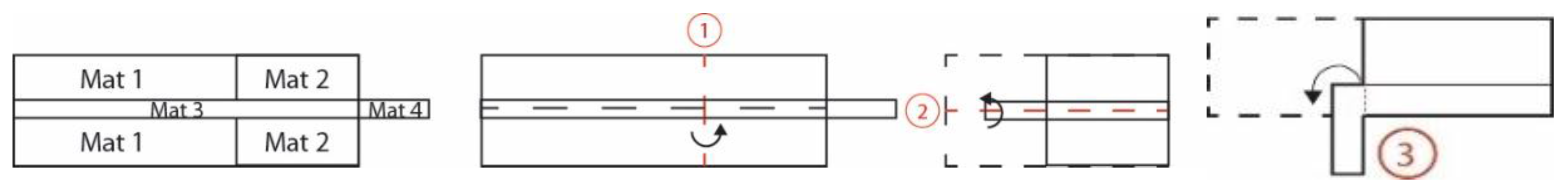
2.6. Design and Crossfolding of Multimaterial Smart Structures
3. Results and Discussion
3.1. Shape Recovery of Single Material Specimens
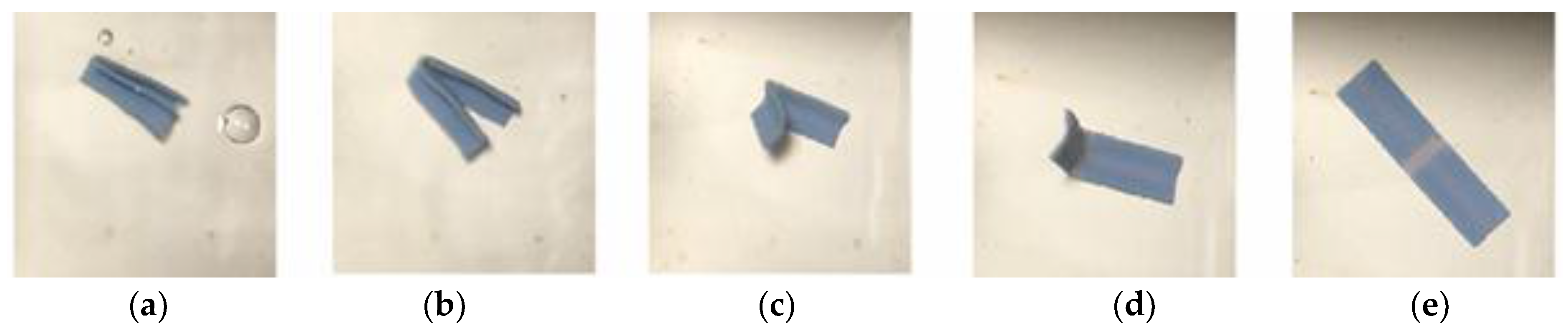
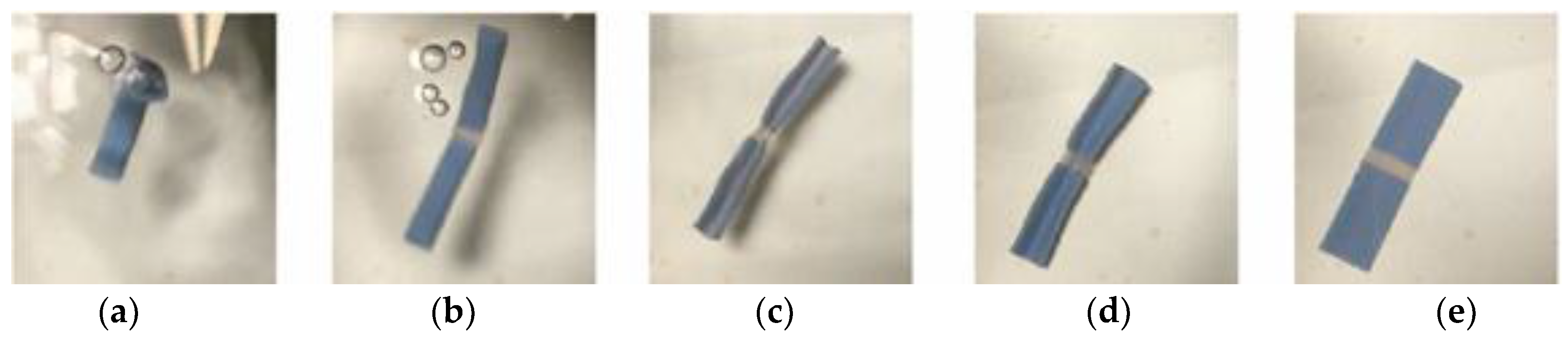
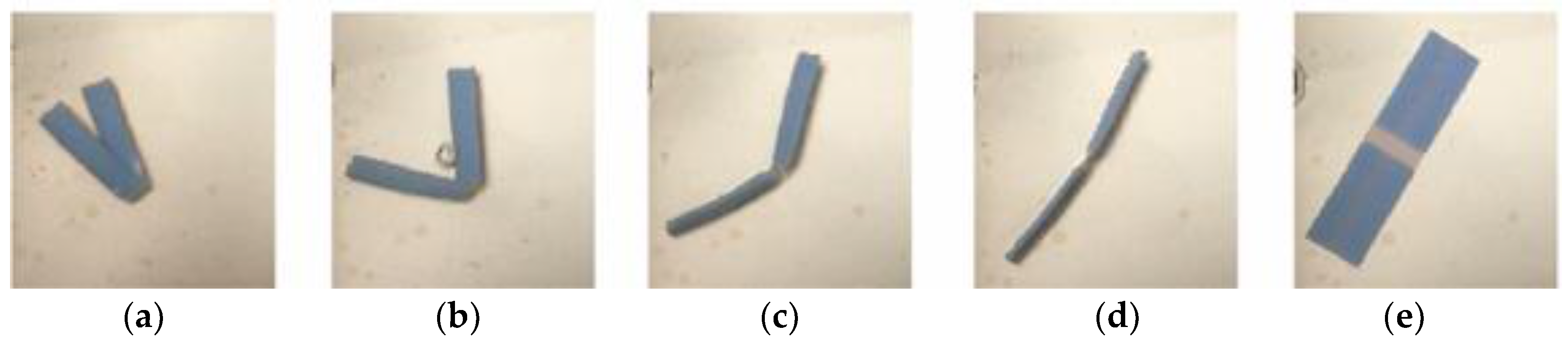
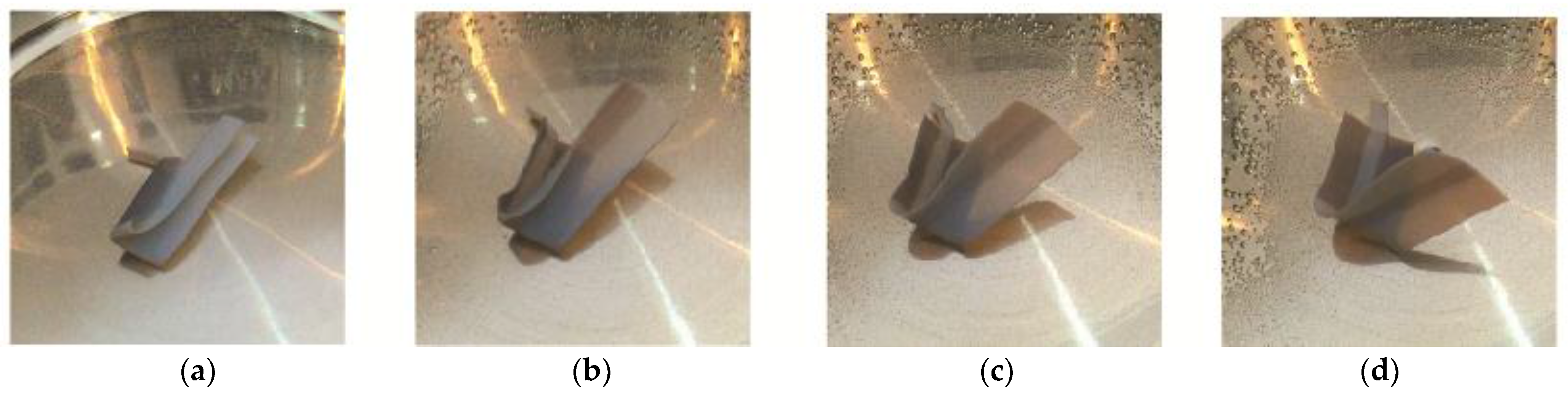
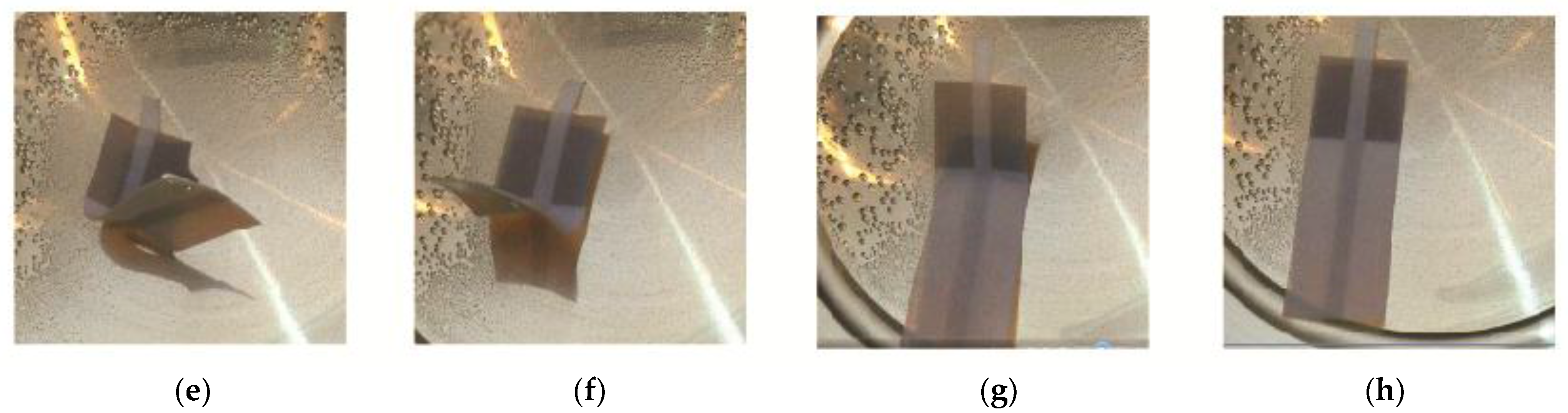
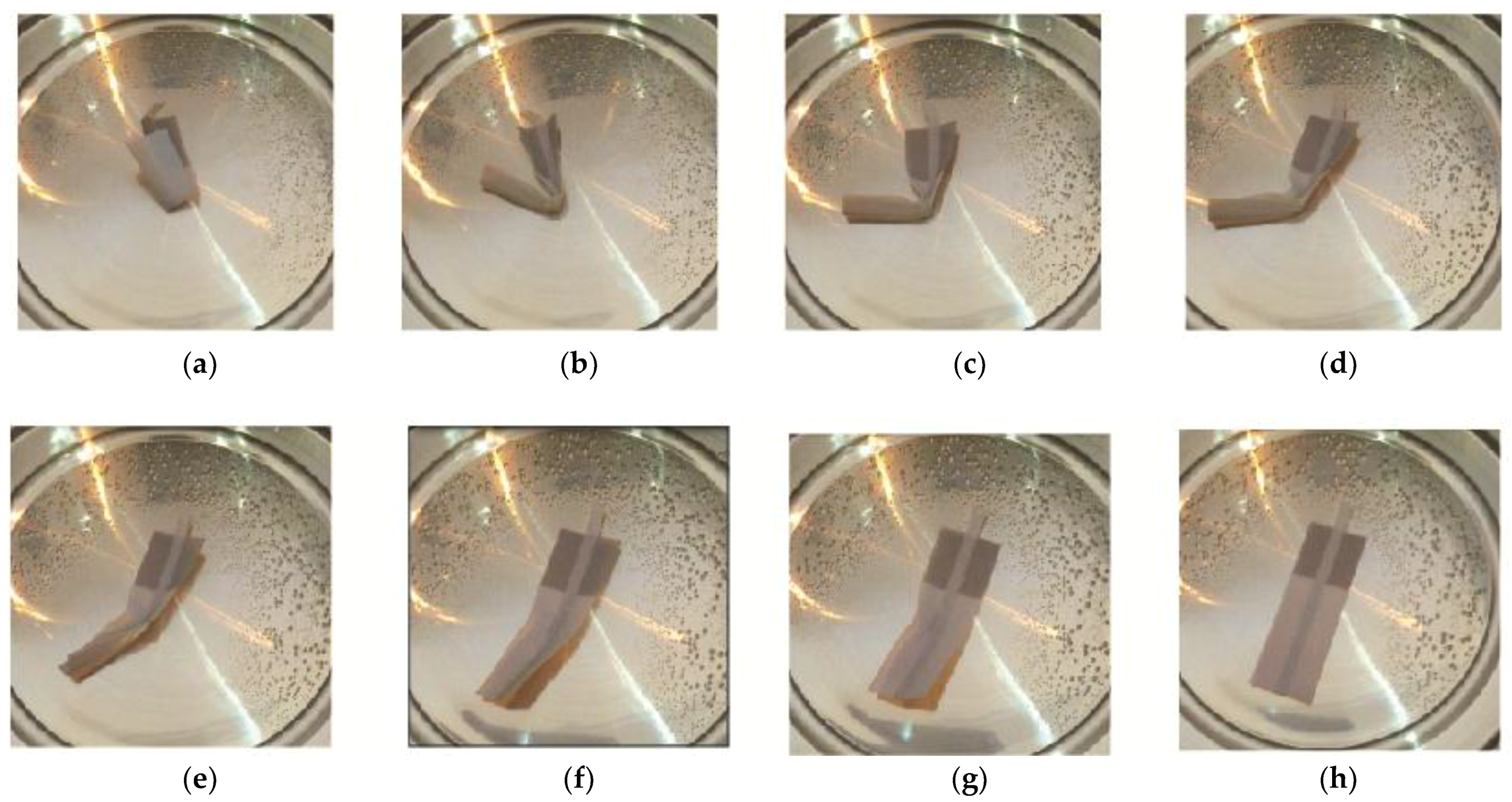
3.2. Recovery of Crossfolded Single Material Smart Structures
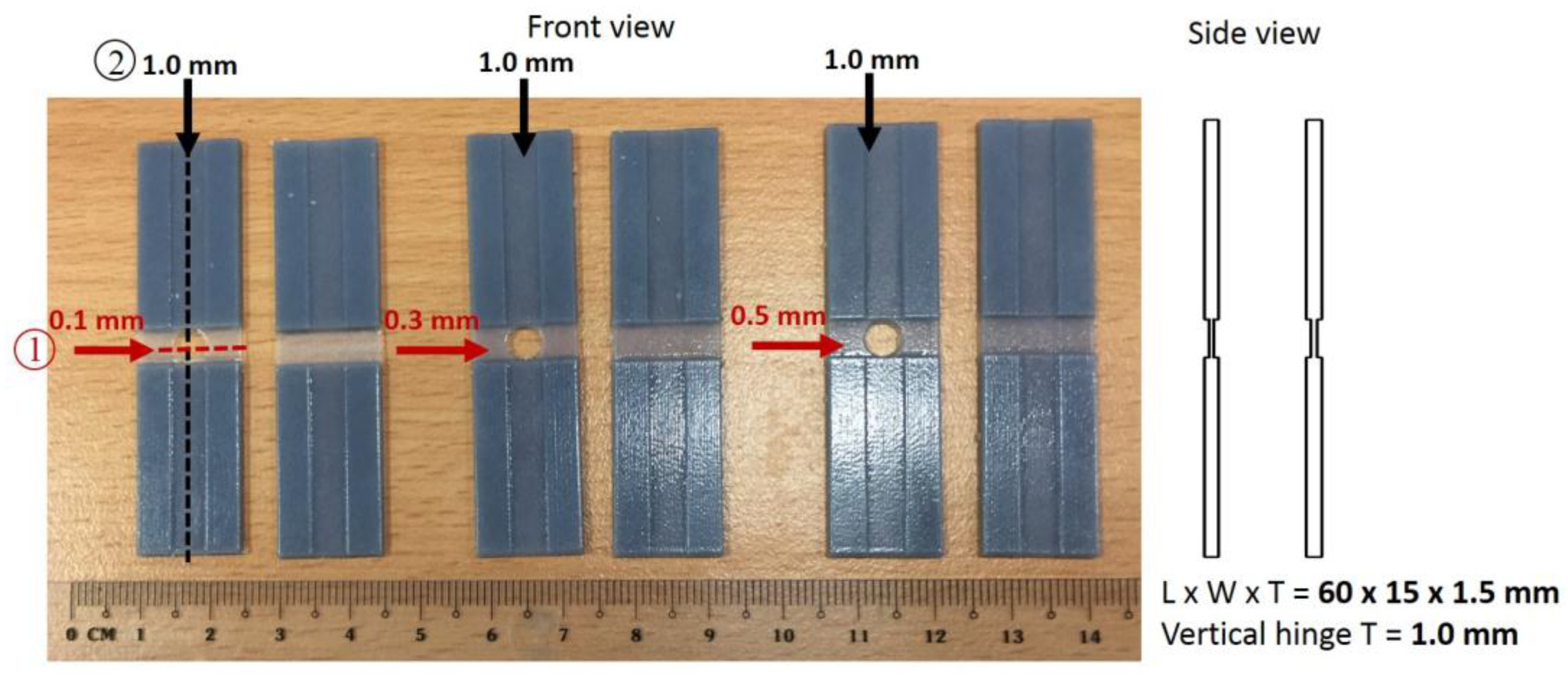
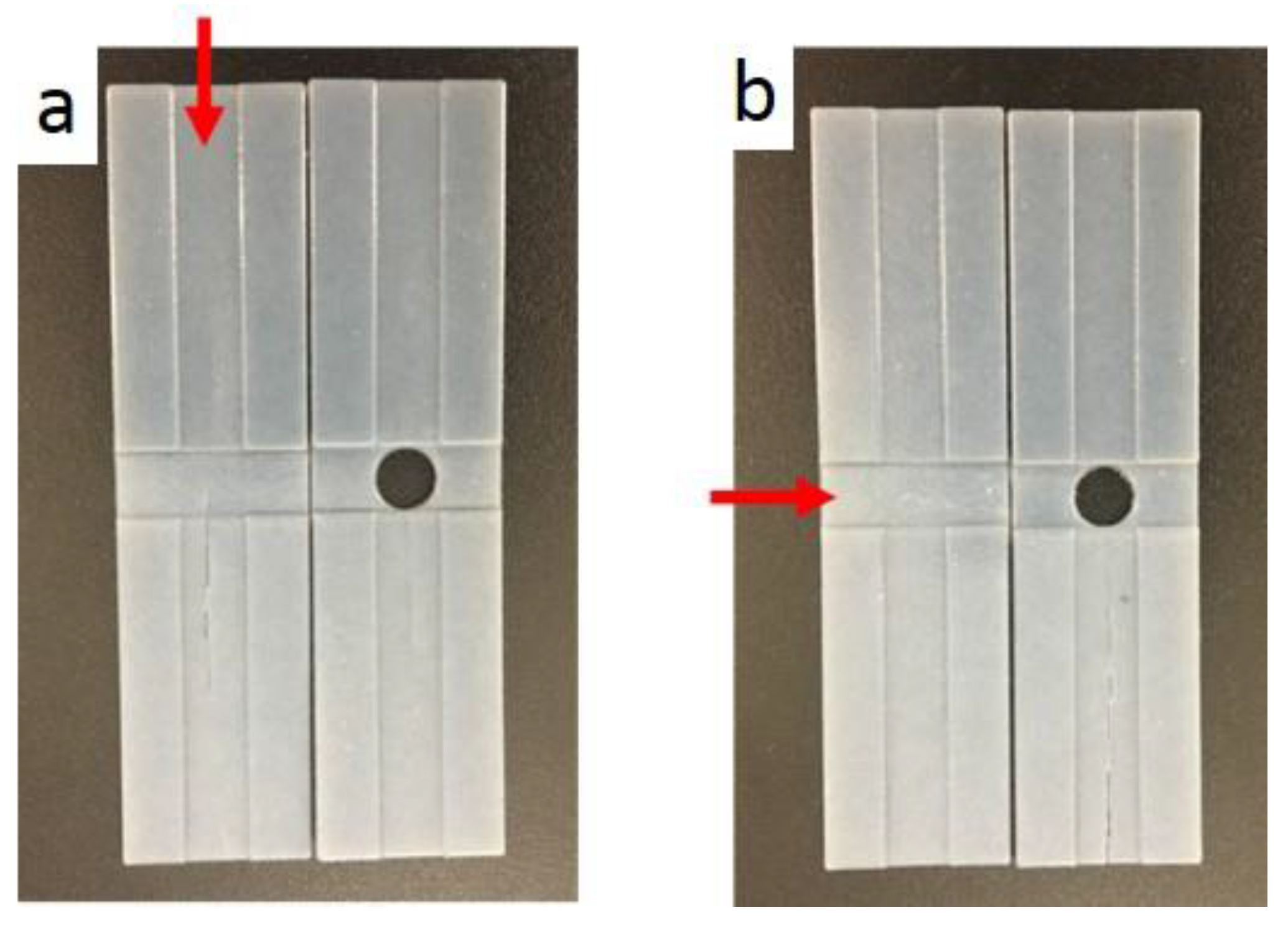
3.2.1. Thickness of Folding Line 1 = 0.5 mm, Folding Line 2 = 1 mm
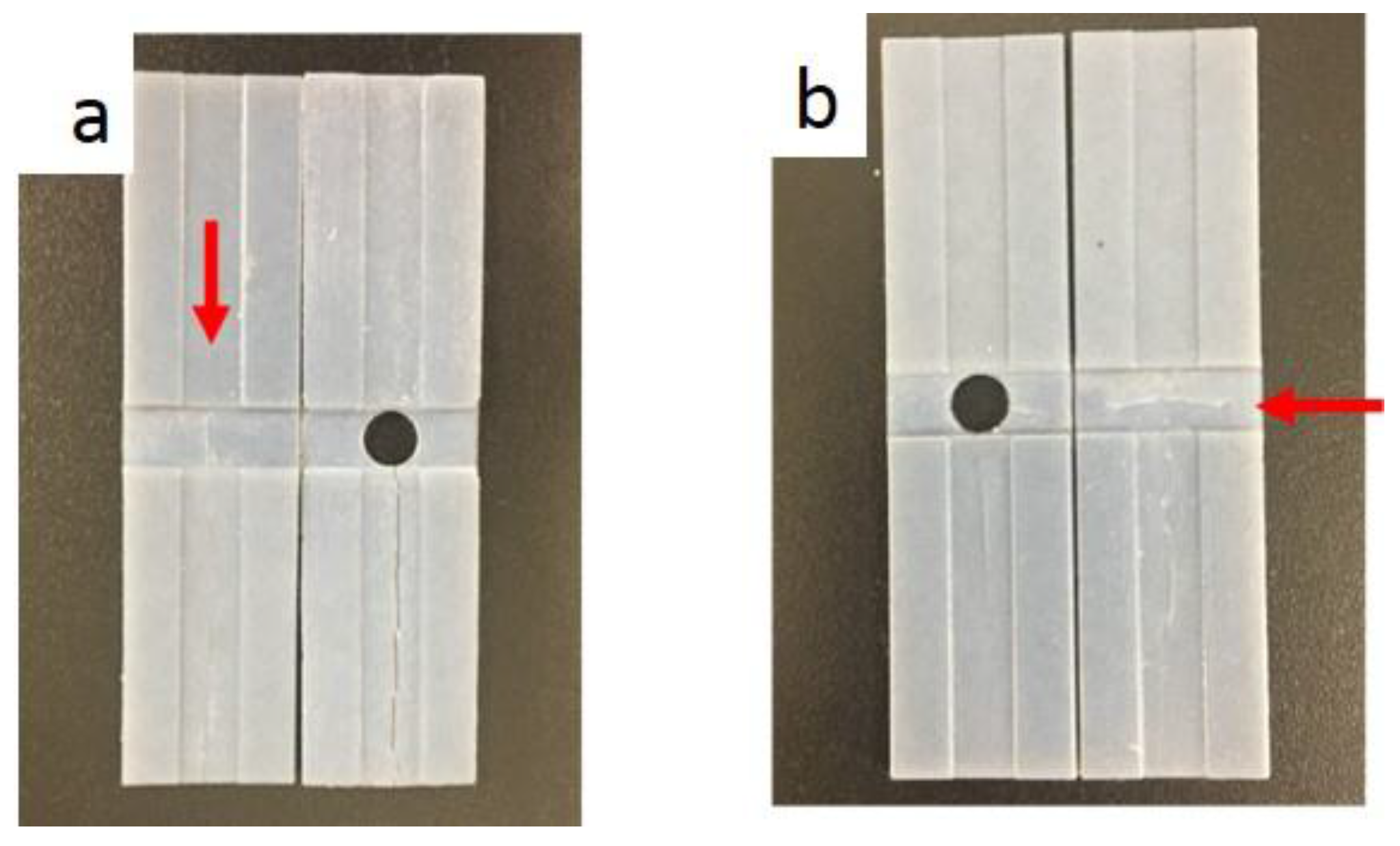
3.2.2. Thickness of Folding Line 1 = 0.3 mm, Folding Line 2 = 1 mm
3.2.3. Thickness of Folding Line 1 = 0.1 mm, Folding Line 2 = 1 mm
3.3. Analysis of Tensile Test Results for Multimaterial Specimens
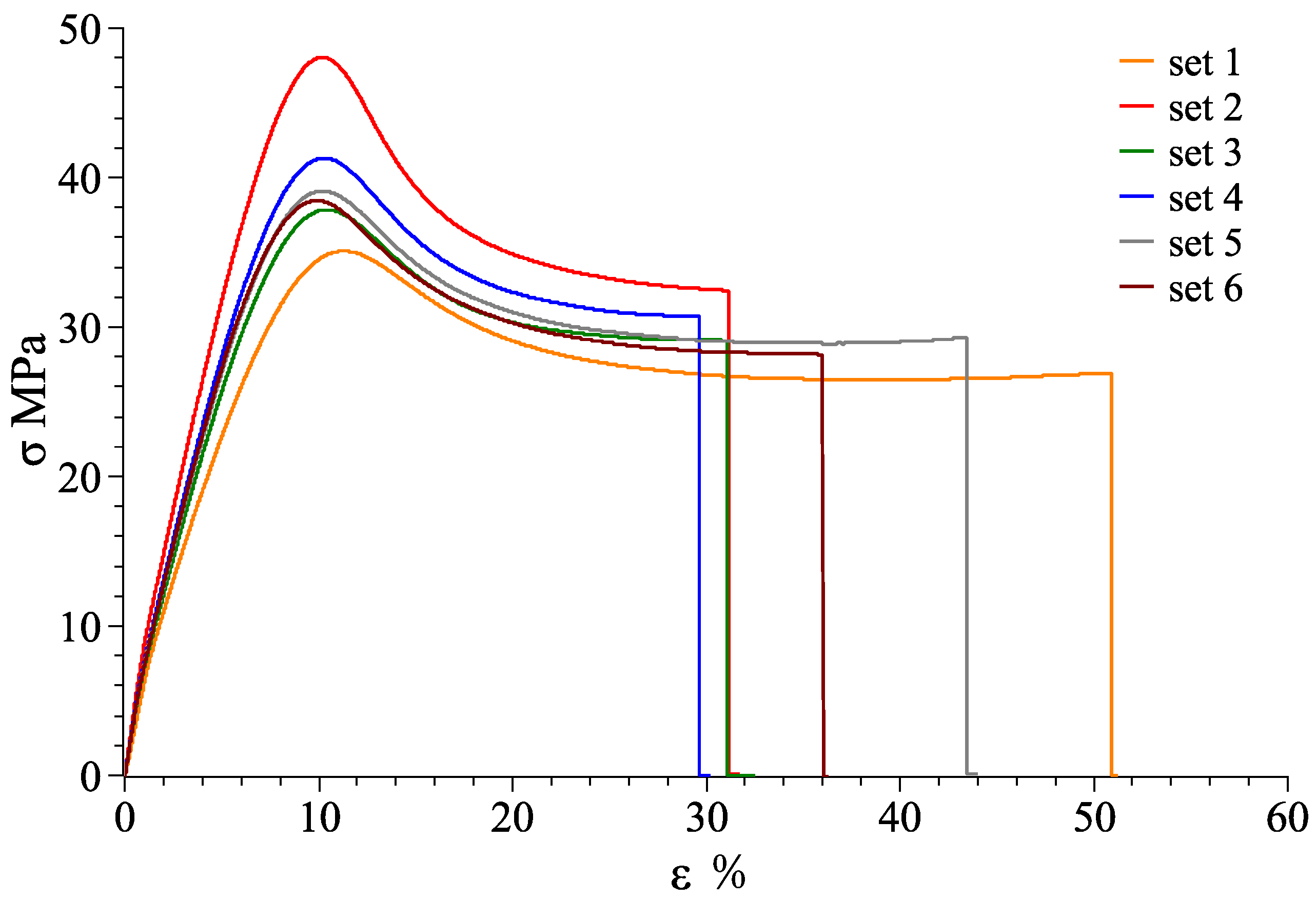
3.3.1. Stress-Strain Curves
3.3.2. Theoretical Calculation versus Experimental Data
3.3.3. Rupture Location and Interfacial Bonding Strength
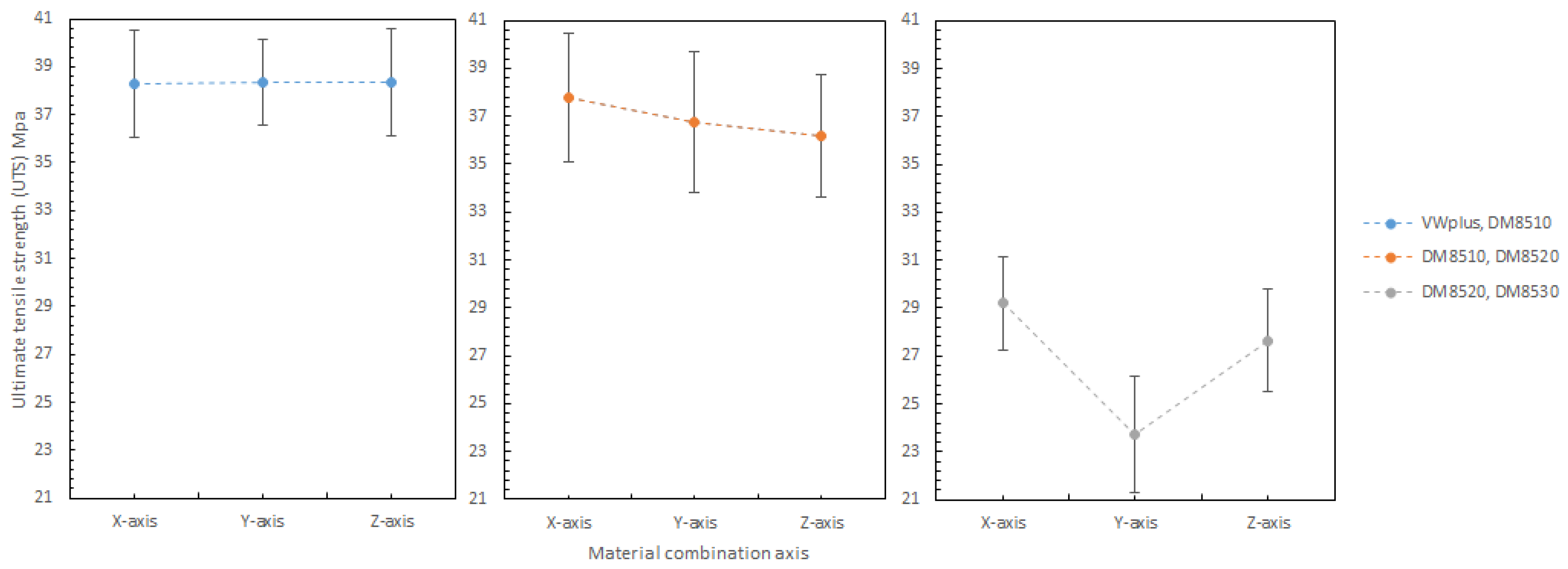
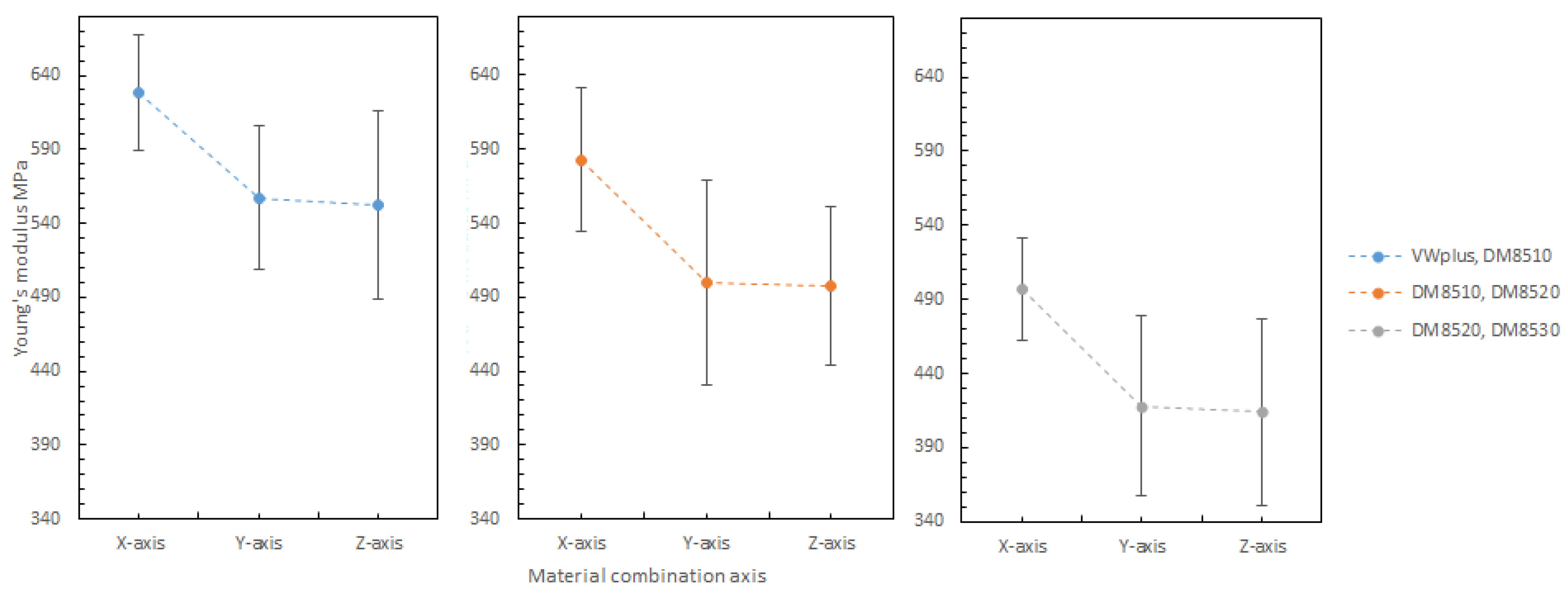
3.3.4. Effect of the Material Combination Axis
3.4. Recovery of Crossfolded Multimaterial Smart Structures
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A
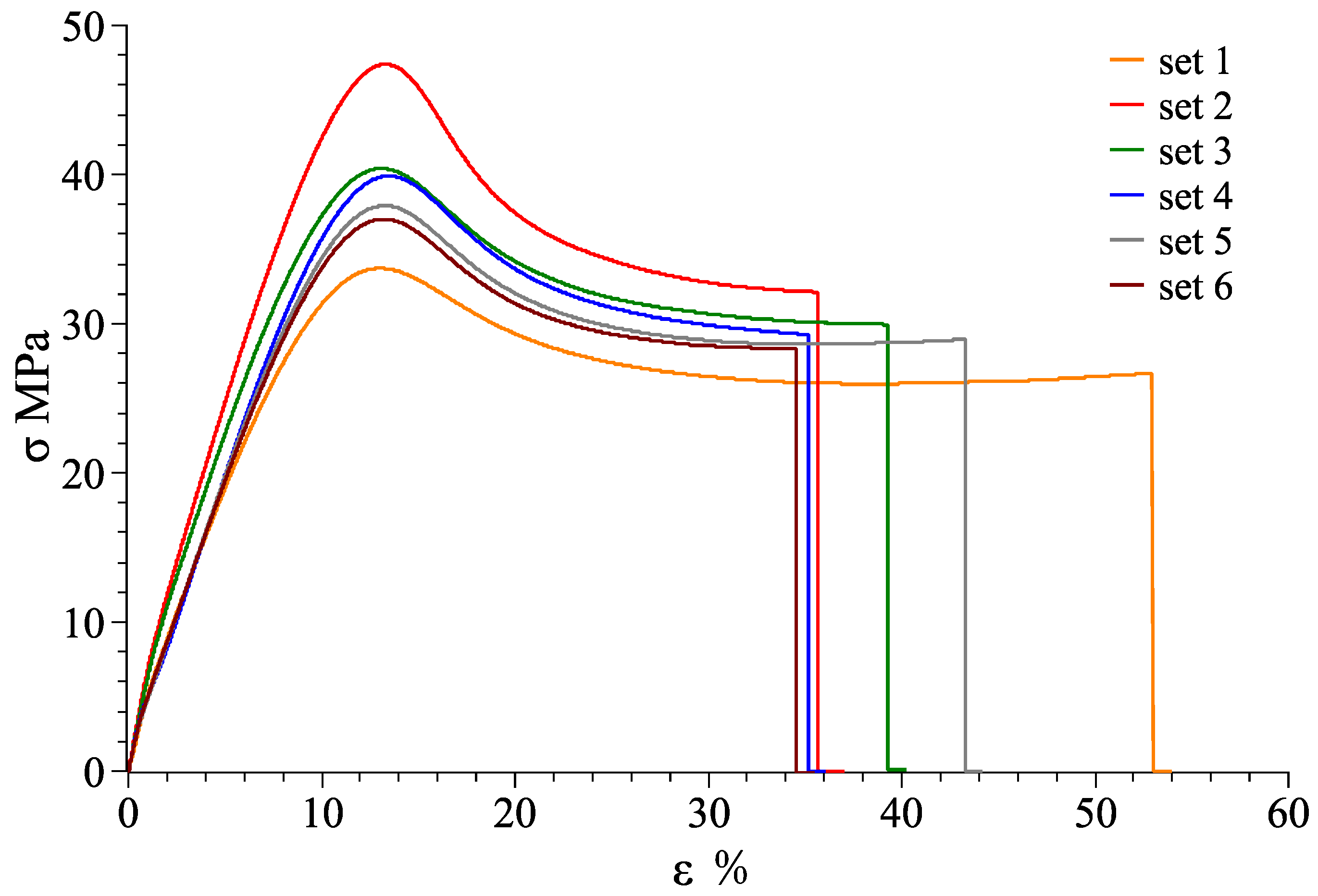
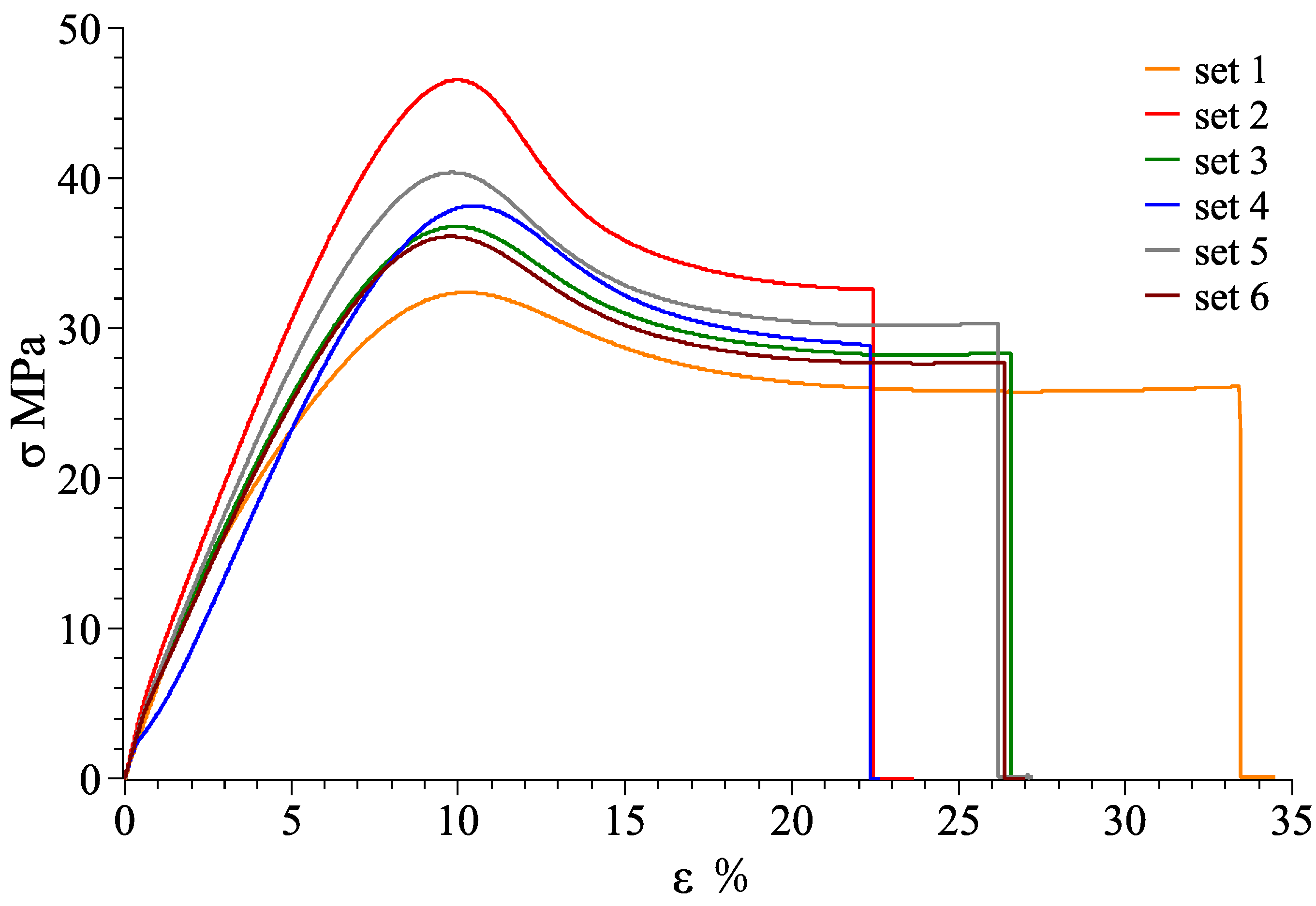


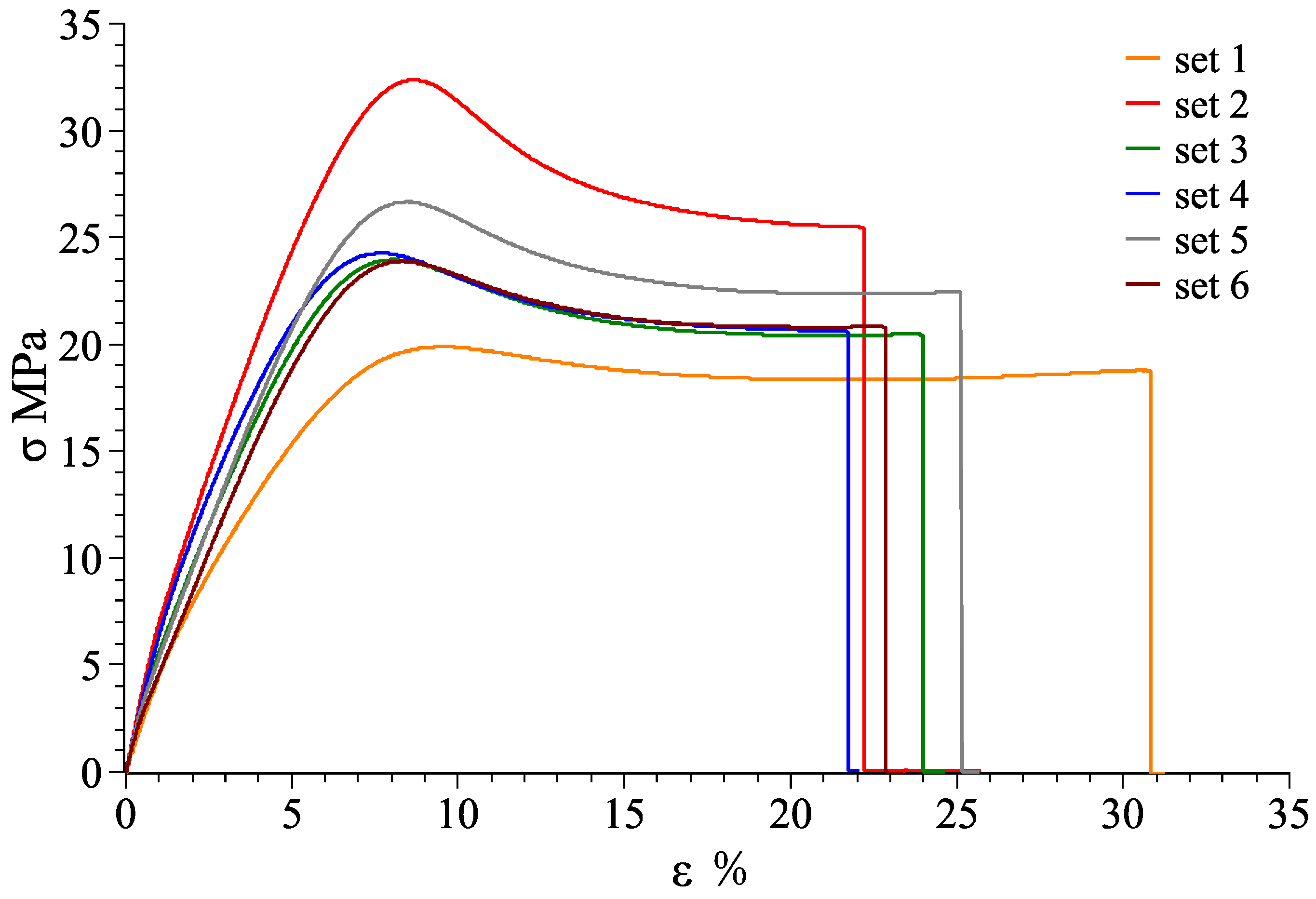
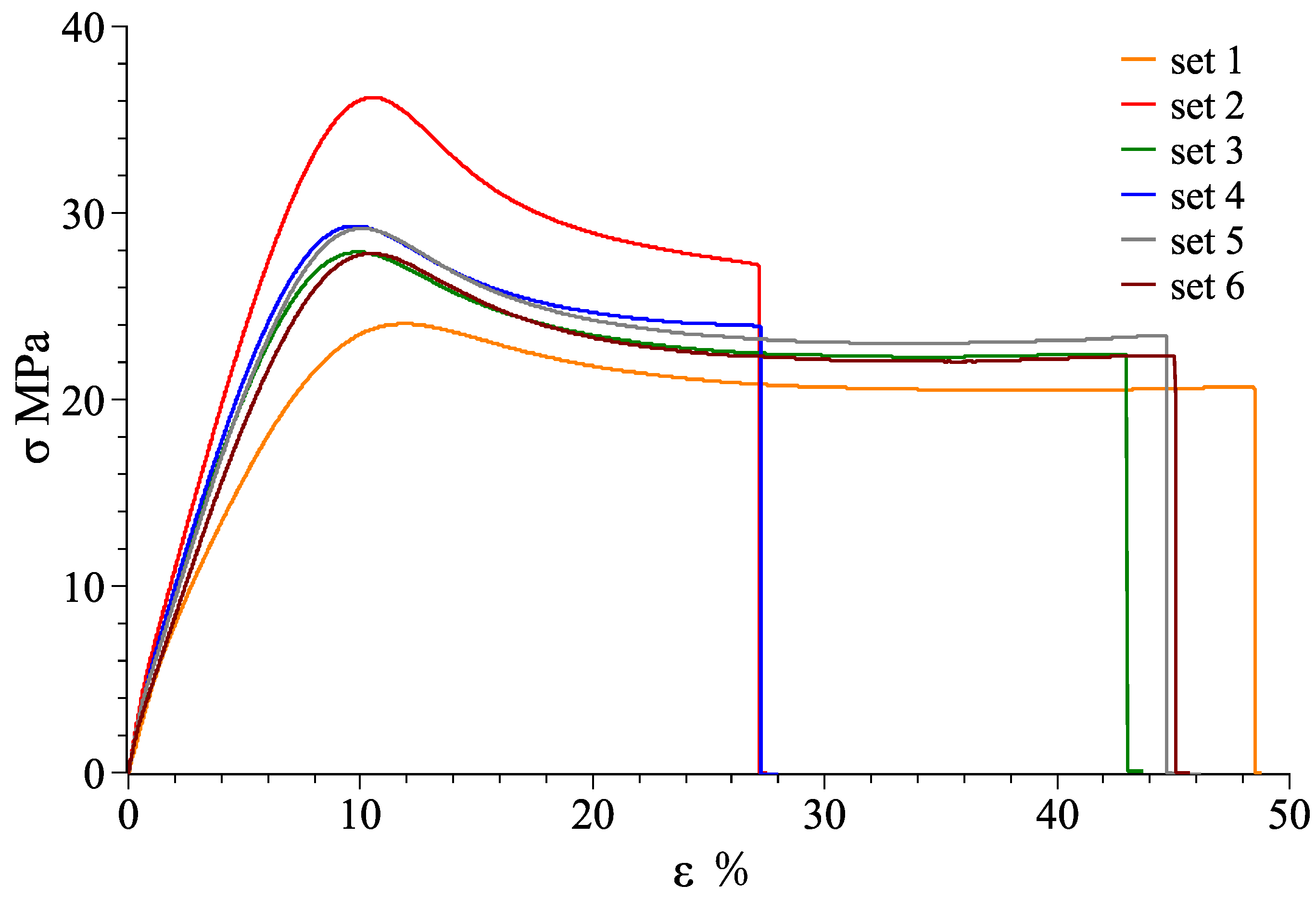
| Parameter | X-Axis | Y-Axis | X-Axis | |||
|---|---|---|---|---|---|---|
| Mean | Standard Deviation | Mean | Standard Deviation | Mean | Standard Deviation | |
| Ultimate Tensile Stress (MPa) | 37.791 | ± 2.7 | 36.753 | ± 2.9 | 36.202 | ± 2.6 |
| Maximum Strain (%) | 41.910 | ± 7.5 | 39.341 | ± 6.1 | 53.617 | ± 12.5 |
| Young’s Modulus (MPa) | 582.590 | ± 48.5 | 499.329 | ± 69.3 | 497.307 | ± 53.6 |
| Parameter | X-Axis | Y-Axis | Z-Axis | |||
|---|---|---|---|---|---|---|
| Mean | Standard Deviation | Mean | Standard Deviation | Mean | Standard Deviation | |
| Ultimate Tensile Stress (MPa) | 29.202 | ± 1.9 | 23.727 | ± 2.4 | 27.650 | ± 2.1 |
| Maximum Strain (%) | 43.882 | ± 9.1 | 36.114 | ± 5.1 | 60.440 | ± 11.8 |
| Young’s Modulus (MPa) | 496.813 | ± 35.0 | 417.935 | ± 60.8 | 413.767 | ± 63.5 |
Appendix B

Appendix C
| Sample No. | After Tension (mm) | Percent Elongation (%) | After Recovery (mm) | Percent Recovery (%) |
|---|---|---|---|---|
| 1 | 28.06 | 10.7777 | 25.98 | 97.4339 |
| 2 | 28.00 | 10.5409 | 26.09 | 96.9996 |
| 3 | 28.12 | 11.0146 | 26.10 | 96.9601 |
| 4 | 28.25 | 11.5278 | 26.04 | 97.1970 |
| 5 | 28.20 | 11.3304 | 26.03 | 97.2365 |
| Average | 28.13 | 11.0383 | 26.05 | 97.1654 |
| Sample No. | After Tension (mm) | Percent Elongation (%) | After Recovery (mm) | Percent Recovery (%) |
|---|---|---|---|---|
| 1 | 28.10 | 10.9356 | 25.75 | 98.3419 |
| 2 | 28.22 | 11.4094 | 25.82 | 98.0655 |
| 3 | 28.40 | 12.1200 | 25.83 | 98.0261 |
| 4 | 28.46 | 12.3569 | 25.83 | 98.0261 |
| 5 | 28.09 | 10.8962 | 25.80 | 98.1445 |
| Average | 28.25 | 11.5436 | 25.81 | 98.1208 |
| Sample No. | After Tension (mm) | Percent Elongation (%) | After Recovery (mm) | Percent Recovery (%) |
|---|---|---|---|---|
| 1 | 28.36 | 11.9621 | 25.70 | 98.5393 |
| 2 | 28.29 | 11.6857 | 25.72 | 98.4603 |
| 3 | 28.20 | 11.3304 | 25.73 | 98.4208 |
| 4 | 28.15 | 11.1330 | 25.72 | 98.4603 |
| 5 | 28.35 | 11.9226 | 25.73 | 98.4208 |
| Average | 28.27 | 11.6068 | 25.72 | 98.4603 |
| Sample No. | After Tension (mm) | Percent Elongation (%) | After Recovery (mm) | Percent Recovery (%) |
|---|---|---|---|---|
| 1 | 28.10 | 10.9356 | 25.34 | 99.9605 |
| 2 | 28.57 | 12.7912 | 25.43 | 99.6052 |
| 3 | 28.16 | 11.1725 | 25.48 | 99.4078 |
| 4 | 28.38 | 12.0411 | 25.50 | 99.3289 |
| 5 | 28.36 | 11.9621 | 25.50 | 99.3289 |
| Average | 28.31 | 11.7805 | 25.45 | 99.5263 |
| Material | Transition Temperature (Tg) |
|---|---|
| VeroWhitePlus | 55.6 °C |
| DM 8510 | 53.5 °C |
| DM 8520 | 51.6 °C |
| DM 8530 | 47.4 °C |
References
- Ge, Q.; Dunn, C.K.; Qi, H.J.; Dunn, M.L. Active origami by 4D printing. Smart Mater. Struct. 2014, 23, 094007. [Google Scholar] [CrossRef]
- Campbell, T.A.; Tibbits, S.; Garrett, B. The programmable world. Sci. Am. 2014, 311, 60–65. [Google Scholar] [CrossRef] [PubMed]
- Pei, E. 4D printing–revolution or fad? Assem. Autom. 2014, 34, 123–127. [Google Scholar] [CrossRef]
- Leist, S.K.; Gao, D.; Chiou, R.; Zhou, J. Investigating the shape memory properties of 4D printed polylactic acid (PLA) and the concept of 4D printing onto nylon fabrics for the creation of smart textiles. Virtual Phys. Prototyp. 2017, 12, 290–300. [Google Scholar] [CrossRef]
- Yang, C.; Wang, B.; Li, D.; Tian, X. Modelling and characterisation for the responsive performance of CF/PLA and CF/PEEK smart materials fabricated by 4D printing. Virtual Phys. Prototyp. 2017, 12, 69–76. [Google Scholar] [CrossRef]
- Choong, Y.Y.C.; Maleksaeedi, S.; Eng, H.; Su, P.-C.; Wei, J. Curing characteristics of shape memory polymers in 3D projection and laser stereolithography. Virtual Phys. Prototyp. 2017, 12, 77–84. [Google Scholar] [CrossRef]
- Zarek, M.; Layani, M.; Eliazar, S.; Mansour, N.; Cooperstein, I.; Shukrun, E.; Szlar, A.; Cohn, D.; Magdassi, S. 4D printing shape memory polymers for dynamic jewellery and fashionwear. Virtual Phys. Prototyp. 2016, 11, 263–270. [Google Scholar] [CrossRef]
- Ge, Q.; Sakhaei, A.H.; Lee, H.; Dunn, C.K.; Fang, N.X.; Dunn, M.L. Multimaterial 4D printing with tailorable shape memory polymers. Sci. Rep. 2016, 6, 31110. [Google Scholar] [CrossRef] [PubMed]
- Wu, Y.-J. Four-dimensional (4D) Printing and Its Applications. In Proceedings of the NIP & Digital Fabrication Conference; Society for Imaging Science and Technology: Springfield, VA, USA; Denver, CO, USA, 2017; pp. 160–164. [Google Scholar]
- Leist, S.K.; Zhou, J. Current status of 4D printing technology and the potential of light-reactive smart materials as 4D printable materials. Virtual Phys. Prototyp. 2016, 11, 249–262. [Google Scholar] [CrossRef]
- Khoo, Z.X.; Liu, Y.; Low, Z.H.; An, J.; Chua, C.K.; Leong, K.F. Fabrication of SLM NiTi Shape Memory Alloy via Repetitive Laser Scanning. Shap. Mem. Superelasticity 2018, 1–9. [Google Scholar] [CrossRef]
- Khoo, Z.X.; Teoh, J.E.M.; Liu, Y.; Chua, C.K.; Yang, S.; An, J.; Leong, K.F.; Yeong, W.Y. 3D printing of smart materials: A review on recent progresses in 4D printing. Virtual Phys. Prototyp. 2015, 10, 103–122. [Google Scholar] [CrossRef]
- Chua, C.K.; Leong, K.F. 3D Printing and Additive Manufacturing: Principles and Applications, Fifth Edition of Rapid Prototyping Fivth Edition ed; World Scientific Publishing Company: Singapore, 2016. [Google Scholar]
- Ge, Q.; Qi, H.J.; Dunn, M.L. Active materials by four-dimension printing. Appl. Phys. Lett. 2013, 103, 131901. [Google Scholar] [CrossRef]
- Lee, A.Y.; An, J.; Chua, C.K. Two-Way 4D Printing: A Review on the Reversibility of 3D-Printed Shape Memory Materials. Engineering 2017, 3, 663–674. [Google Scholar] [CrossRef]
- Yuan, C.; Wang, T.; Dunn, M.L.; Qi, H.J. 3D printed active origami with complicated folding patterns. Int. J. Precis. Eng. Manuf. Green Technol. 2017, 4, 281–289. [Google Scholar] [CrossRef]
- Peraza-Hernandez, E.A.; Hartl, D.J.; Malak, R.J., Jr.; Lagoudas, D.C. Origami-inspired active structures: A synthesis and review. Smart Mater. Struct. 2014, 23, 094001. [Google Scholar] [CrossRef]
- Ahmed, S.; Ounaies, Z.; Frecker, M. Investigating the performance and properties of dielectric elastomer actuators as a potential means to actuate origami structures. Smart Mater. Struct. 2014, 23, 094003. [Google Scholar] [CrossRef]
- Ahmed, S.; Lauff, C.; Crivaro, A.; McGough, K.; Sheridan, R.; Frecker, M.; von Lockette, P.; Ounaies, Z.; Simpson, T.; Lien, J.-M. Multi-field responsive origami structures: Preliminary modeling and experiments. In Proceedings of the ASME 2013 International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Portland, OR, USA, 4–7 August 2013; American Society of Mechanical Engineers: New York, NY, USA, 2013. [Google Scholar]
- Teoh, J.; An, J.; Chua, C.; Lv, M.; Krishnasamy, V.; Liu, Y. Hierarchically self-morphing structure through 4D printing. Virtual Phys. Prototyp. 2017, 12, 61–68. [Google Scholar] [CrossRef]
- Teoh, J.E.M.; Zhao, Y.; An, J.; Chua, C.K.; Liu, Y. Multi-stage responsive 4D printed smart structure through varying geometric thickness of shape memory polymer. Smart Mater. Struct. 2017, 26, 125001. [Google Scholar] [CrossRef]
- ASTM D638-02a Standard. Standard Test Method for Tensile Properties of Plastics; ASTM International: West Conshohocken, PA, USA, 2002. [Google Scholar]
- Askeland, D.; Fulay, P.; Wright, W. The Science and Engineering of Materials; Nelson Education: Toronto, ON, Canada, 2011. [Google Scholar]
- Roylance, D. Introduction to Composite Materials; Department of Materials Science and Engineering, Massachusetts Institute of Technology: Cambridge, MA, USA, 2000. [Google Scholar]
- Gooch, J.W. Law of Mixtures. In Encyclopedic Dictionary of Polymers; Springer: New York, NY, USA, 2011; p. 421. [Google Scholar]
- Kim, H.S. On the rule of mixtures for the hardness of particle reinforced composites. Mater. Sci. Eng. A 2000, 289, 30–33. [Google Scholar] [CrossRef]
- Mechanical Properties. Available online: http://www.materials.unsw.edu.au/tutorials/online-tutorials/1-mechanical-properties (accessed on 20 November 2017).
- Callister, W.D.; Rethwisch, D.G. Fundamentals of Materials Science and Engineering; Wiley: London, UK, 2000. [Google Scholar]
- Johnston, B. Mechanics of Materials, 5th ed.; McGraw Hill: New York, NY, USA, 2006. [Google Scholar]
- Kalpakjian, S.; Schmid, S.R. Manufacturing Engineering and Technology, International edition (4th ed.); Prentice Hall Inc.: Upper Saddle River, NJ, USA, 2001. [Google Scholar]




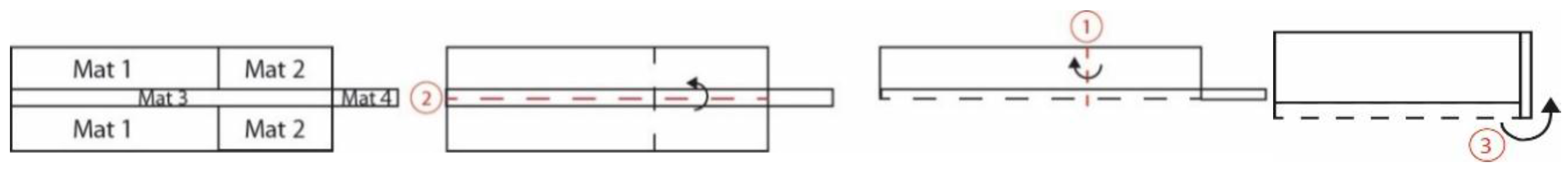



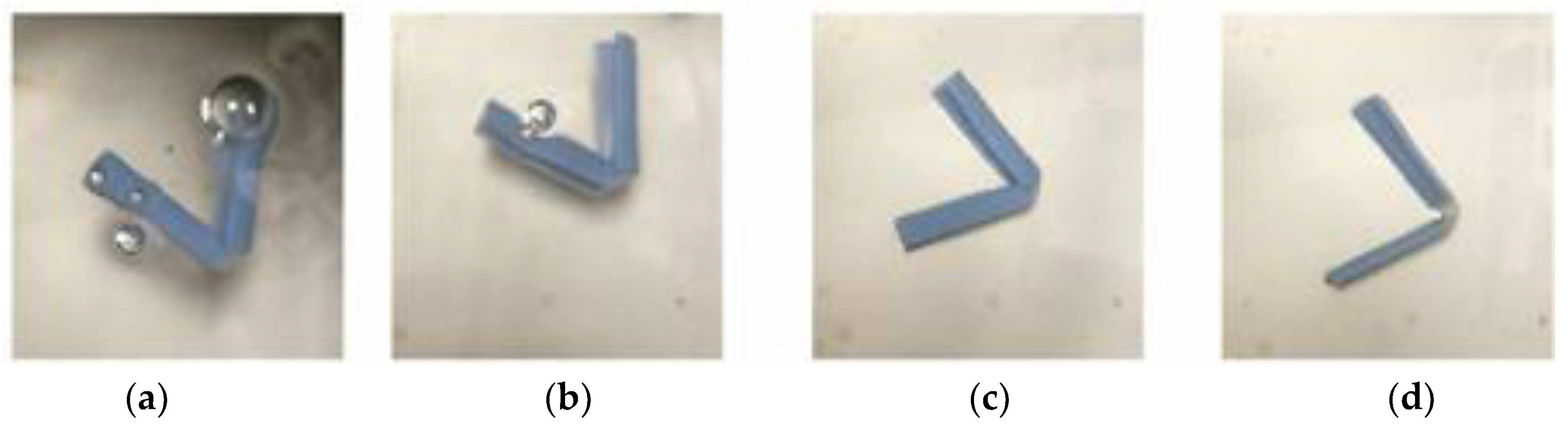
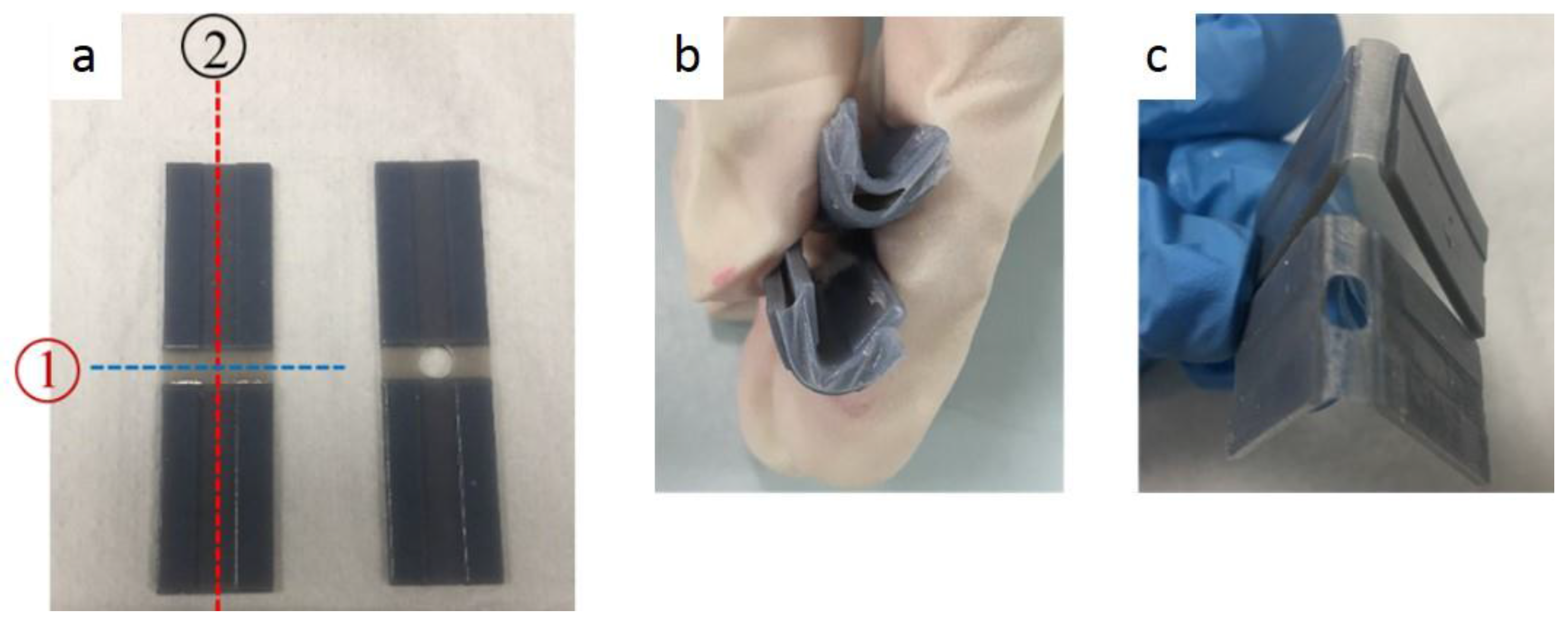
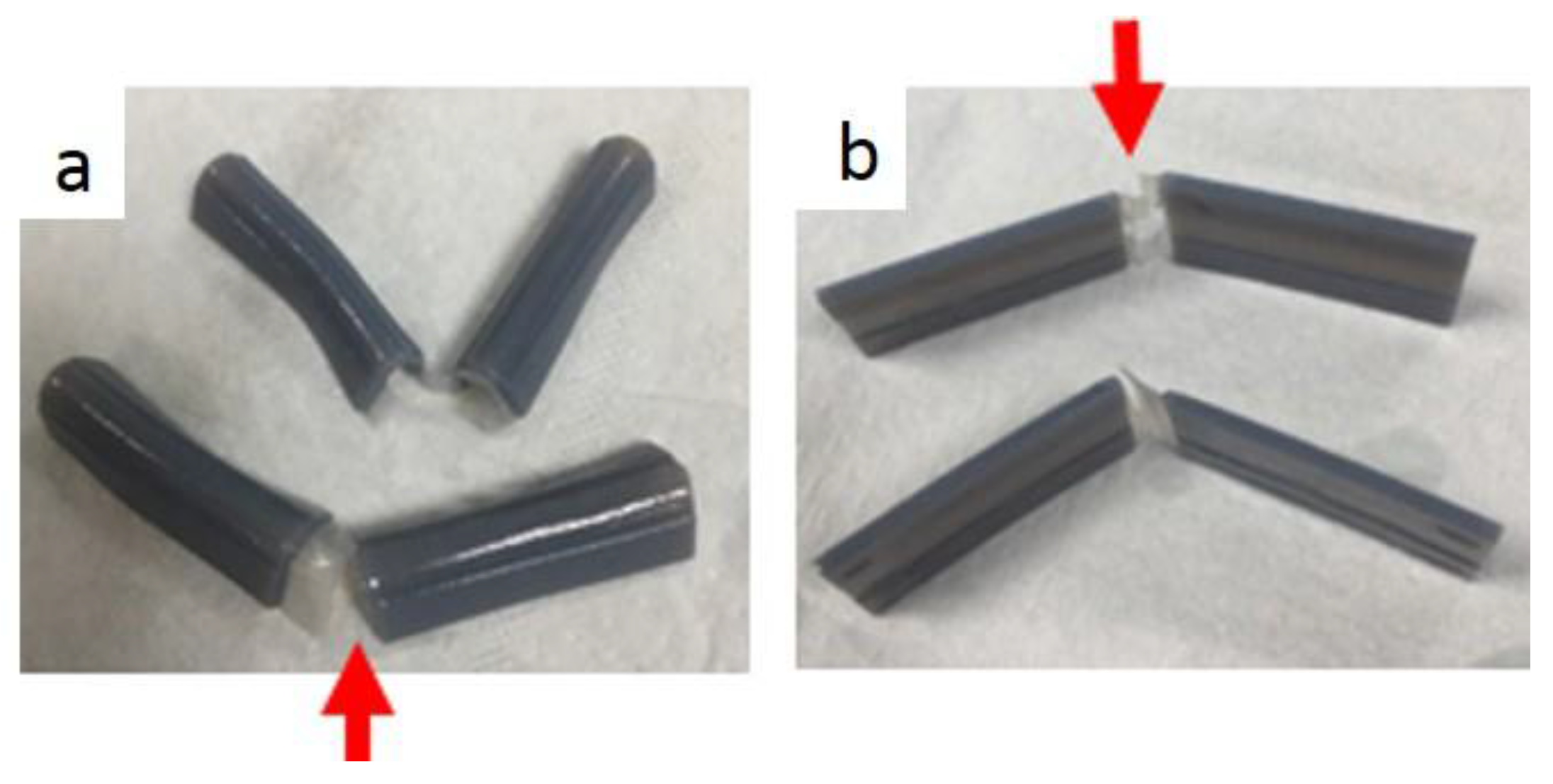

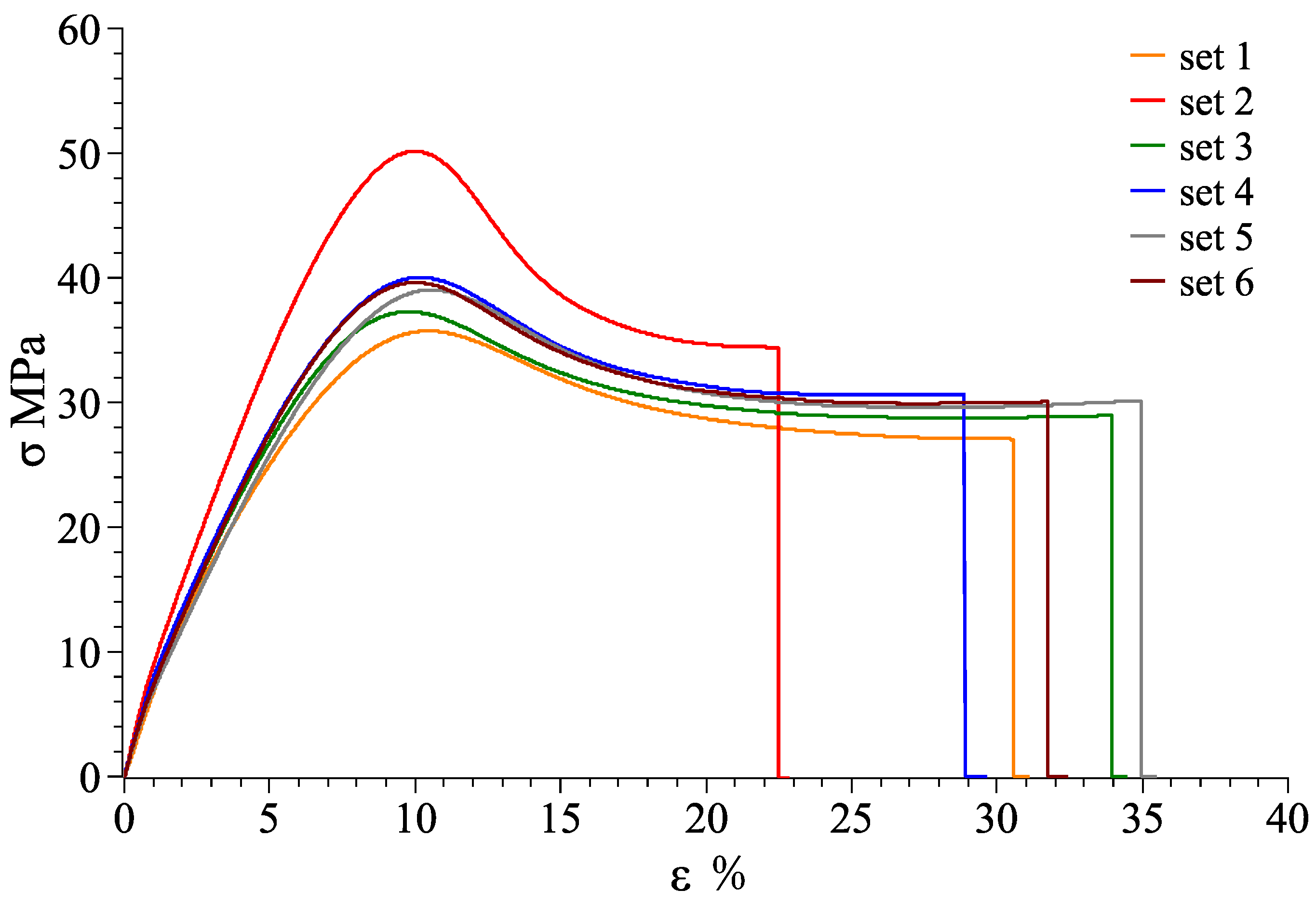




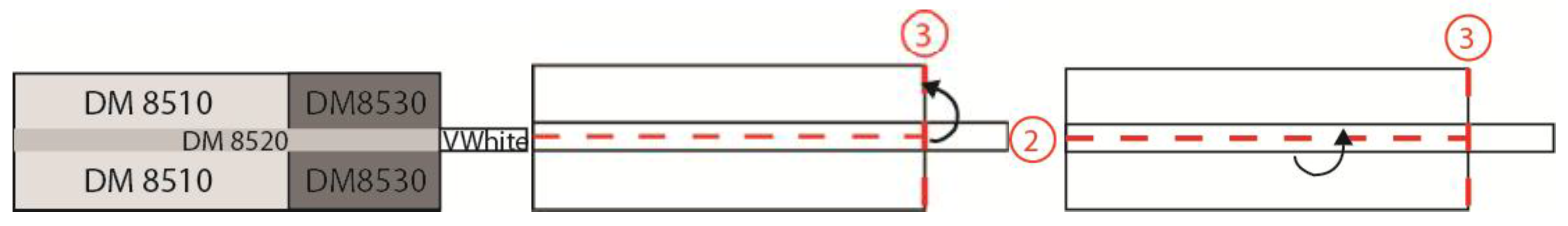
| Axis for Material Combination | Materials | Ratio | Printing Orientation |
|---|---|---|---|
| X-axis | VW, DM8510 DM8510, DM8520 DM8520, DM8530 | 50:50 50:50 50:50 | Along X-axis |
| Y-axis | VW, DM8510 DM8510, DM8520 DM8520, DM8530 | 50:50 50:50 50:50 | Along X-axis |
| Z-axis | VW, DM8510 DM8510, DM8520 DM8520, DM8530 | 50:50 50:50 50:50 | Along X-axis |
| X-axis | 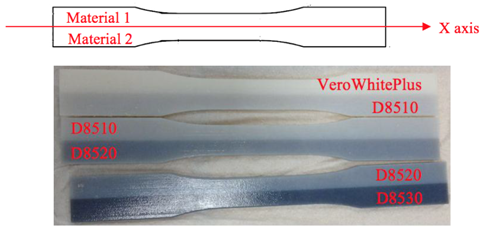 |
| Y-axis | 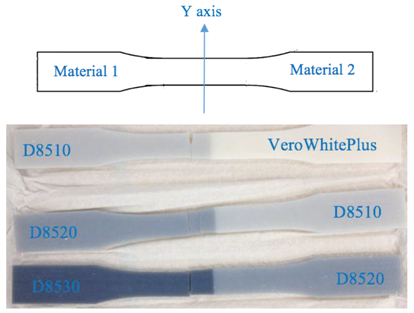 |
| Z-axis | 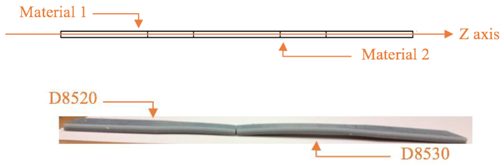 |
| Horizontal Hinge Thickness | Folding Sequence | Open Sequence | Maximum Folding Times | Crack with Visual Inspection | ||
|---|---|---|---|---|---|---|
| 0.5 mm | With hole | 2 + 1 | 1 + 2 | 3 | Less | 1 = Folding line 1 2 = Folding line 2 Maximum folding times (Number of times cross-folded) |
| 1 + 2 | ||||||
| Without hole | 2 + 1 | More | ||||
| 1 + 2 | ||||||
| 0.3 mm | With hole | 2 + 1 | 1 + 2 | 1 | Less | |
| 1 + 2 | ||||||
| Without hole | 2 + 1 | More | ||||
| 1 + 2 | ||||||
| 0.1 mm | With hole | 2 + 1 | NA | NA | Break | |
| 1 + 2 | 1 + 2 | Less | ||||
| Without hole | 2 + 1 | NA | Break | |||
| 1 + 2 | 1 + 2 | More | ||||
| Parameter | X-Axis | Y-Axis | Z-Axis | |||
|---|---|---|---|---|---|---|
| Mean | Standard Deviation | Mean | Standard Deviation | Mean | Standard Deviation | |
| Ultimate Tensile Stress (MPa) | 38.273 | ±2.2 | 38.335 | ±1.8 | 38.354 | ±2.2 |
| Maximum Strain (%) | 50.795 | ±6.3 | 46.465 | ±3.4 | 55.247 | ±12.3 |
| Young’s Modulus (MPa) | 628.281 | ±38.8 | 557.266 | ±48.6 | 552.231 | ±63.9 |
(MPa) | (MPa) | Material Combination Axis | (MPa) | (MPa) | Error | ||
| 818.479 | 742.110 | 0.5 | X | 780.295 | 628.281 ± 38.8 | 19.5% | |
| Y | 778.426 | 557.266 ± 48.6 | 28.4% | ||||
| Z | 780.295 | 552.231 ± 63.9 | 29.2% | ||||
(MPa) | (MPa) | Material Combination Axis | (MPa) | (MPa) | Error | ||
| 742.110 | 693.520 | 0.5 | X | 717.815 | 582.590 ± 48.5 | 18.8% | |
| Y | 716.993 | 499.329 ± 69.3 | 30.4% | ||||
| Z | 717.815 | 497.307 ± 53.6 | 30.7% | ||||
(MPa) | (MPa) | Material Combination Axis | (MPa) | (MPa) | Error | ||
| 693.520 | 546.235 | 0.5 | X | 619.878 | 496.813 ± 35.0 | 19.9% | |
| Y | 611.129 | 417.935 ± 60.8 | 31.6% | ||||
| Z | 619.878 | 413.767 ± 63.5 | 33.3% | ||||
| Thickness (mm) | Time to Recover (s) (Temperature: 70−73 °C) | |
|---|---|---|
| Folding Line 3 | Folding Line 2 | |
| 0.1 | N/A | 0.7 |
| 0.3 | 0.9 | 1.1 |
| 0.5 | 1.8 | 4 |
| 1 | 2 | 5 |
| 1.5 | 8 | 11 |
| 2 | 11 | Breakage |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Teoh, J.E.M.; An, J.; Feng, X.; Zhao, Y.; Chua, C.K.; Liu, Y. Design and 4D Printing of Cross-Folded Origami Structures: A Preliminary Investigation. Materials 2018, 11, 376. https://doi.org/10.3390/ma11030376
Teoh JEM, An J, Feng X, Zhao Y, Chua CK, Liu Y. Design and 4D Printing of Cross-Folded Origami Structures: A Preliminary Investigation. Materials. 2018; 11(3):376. https://doi.org/10.3390/ma11030376
Chicago/Turabian StyleTeoh, Joanne Ee Mei, Jia An, Xiaofan Feng, Yue Zhao, Chee Kai Chua, and Yong Liu. 2018. "Design and 4D Printing of Cross-Folded Origami Structures: A Preliminary Investigation" Materials 11, no. 3: 376. https://doi.org/10.3390/ma11030376
APA StyleTeoh, J. E. M., An, J., Feng, X., Zhao, Y., Chua, C. K., & Liu, Y. (2018). Design and 4D Printing of Cross-Folded Origami Structures: A Preliminary Investigation. Materials, 11(3), 376. https://doi.org/10.3390/ma11030376





