An Investigation of the Influence of Initial Roughness on the Friction and Wear Behavior of Ground Surfaces
Abstract
1. Introduction
2. Experiments
2.1. Material
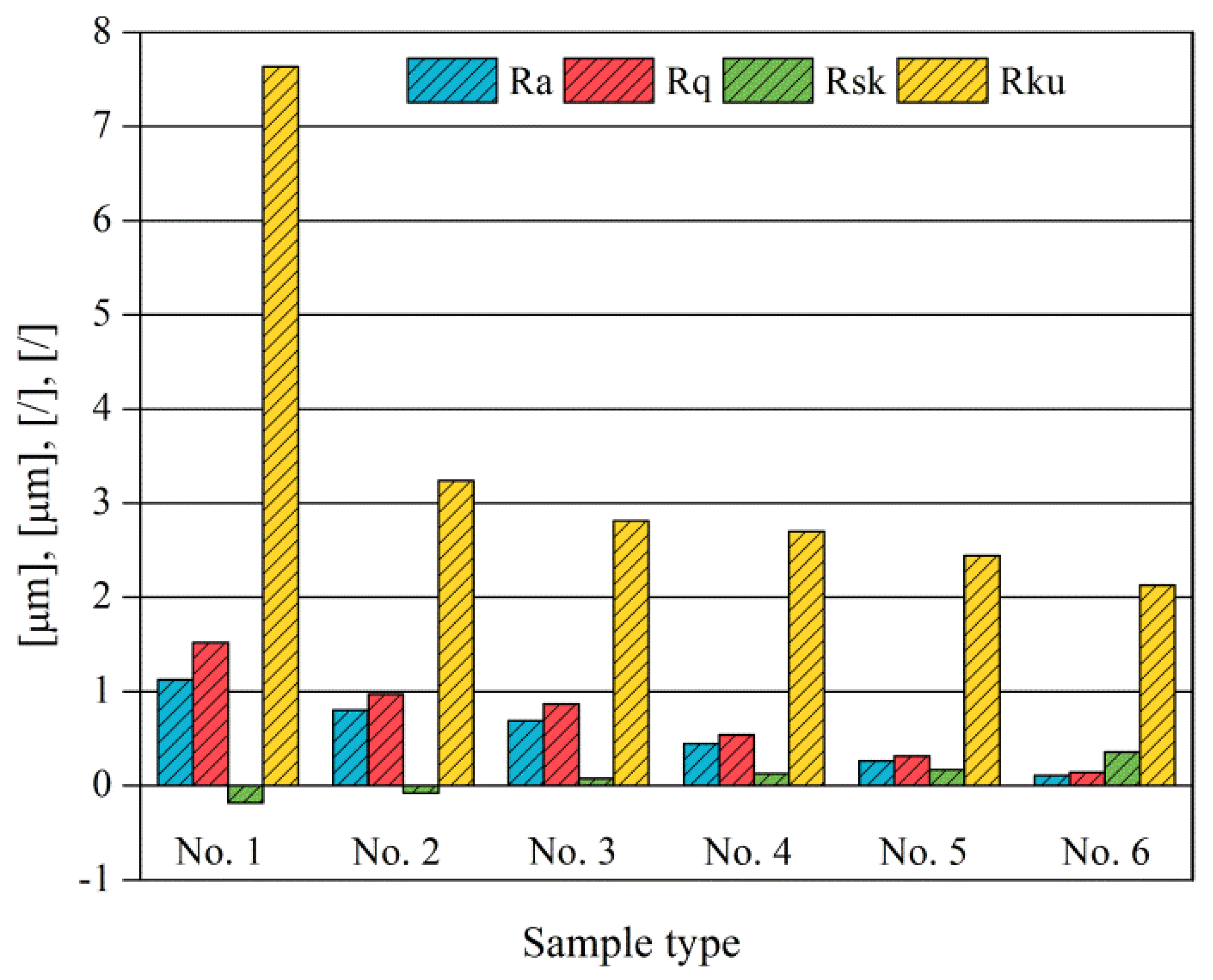
2.2. Grinding-Induced Variety of Surface Roughness
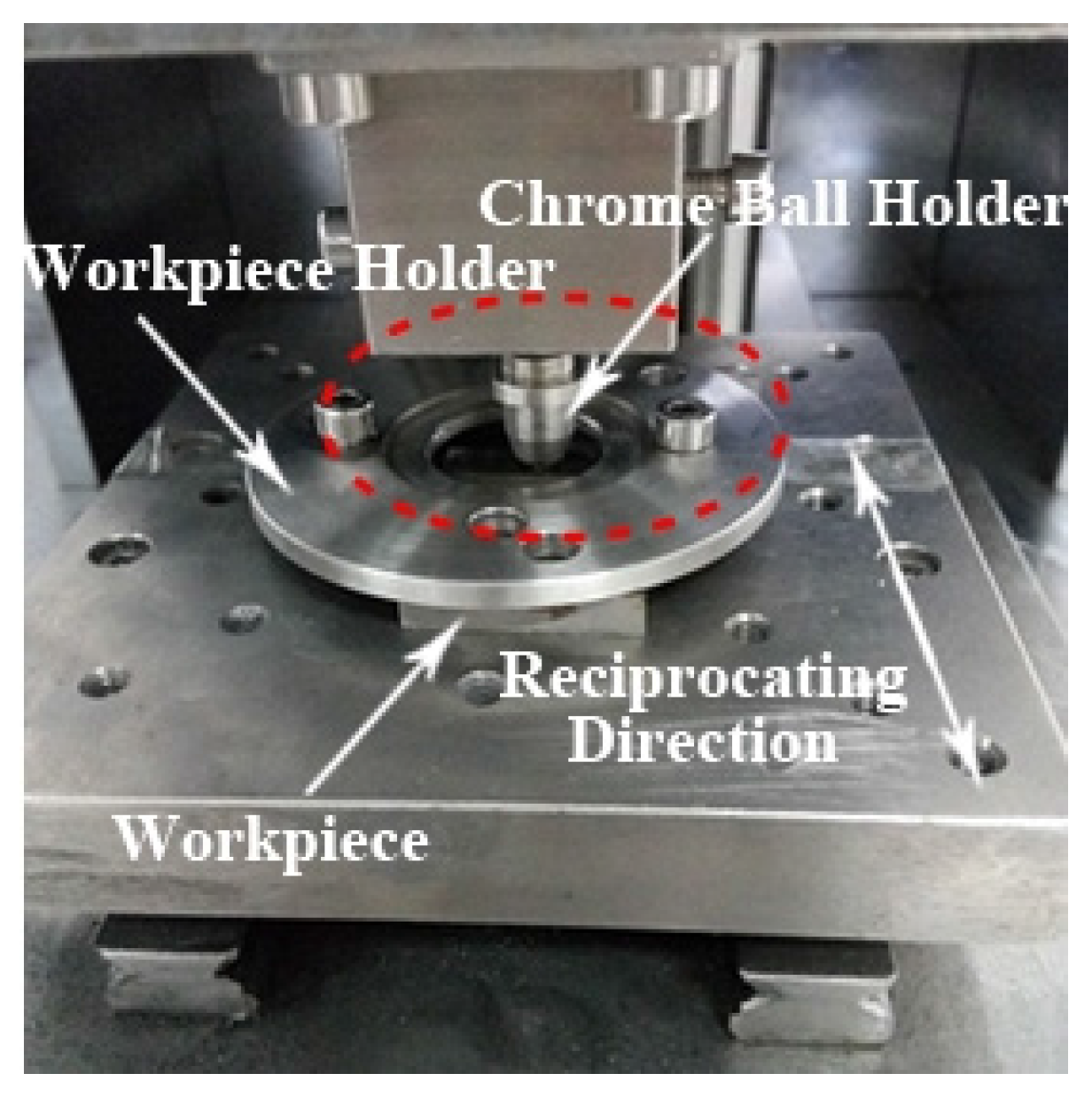
2.3. Friction and Wear Testing
3. Results and Discussion
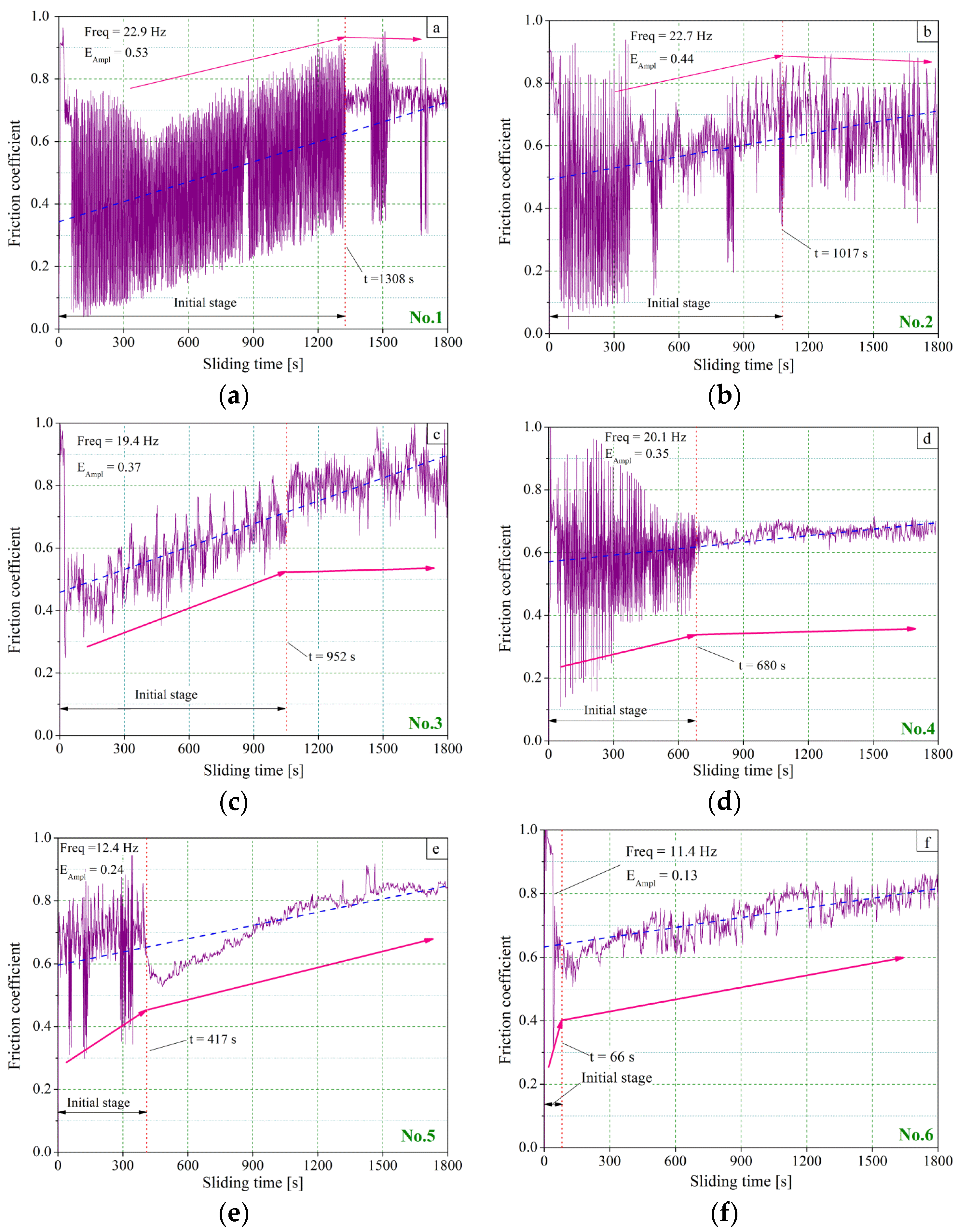
3.1. Friction Coefficient
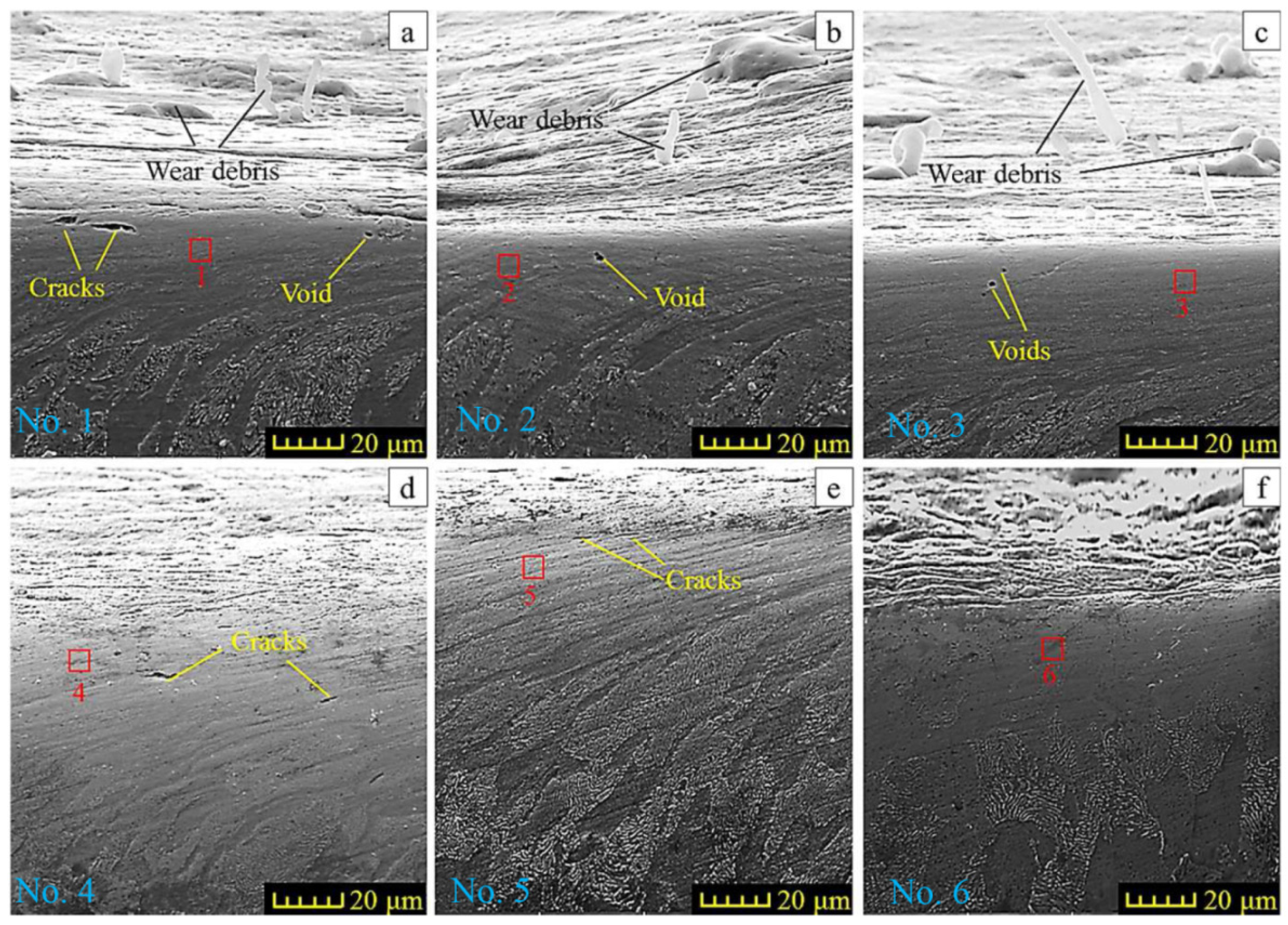
3.2. The Cross-Section Profile of Worn Surface Analysis
3.3. Analysis of Material Plastic Deformation in the Worn Layer
4. Conclusions
- (1)
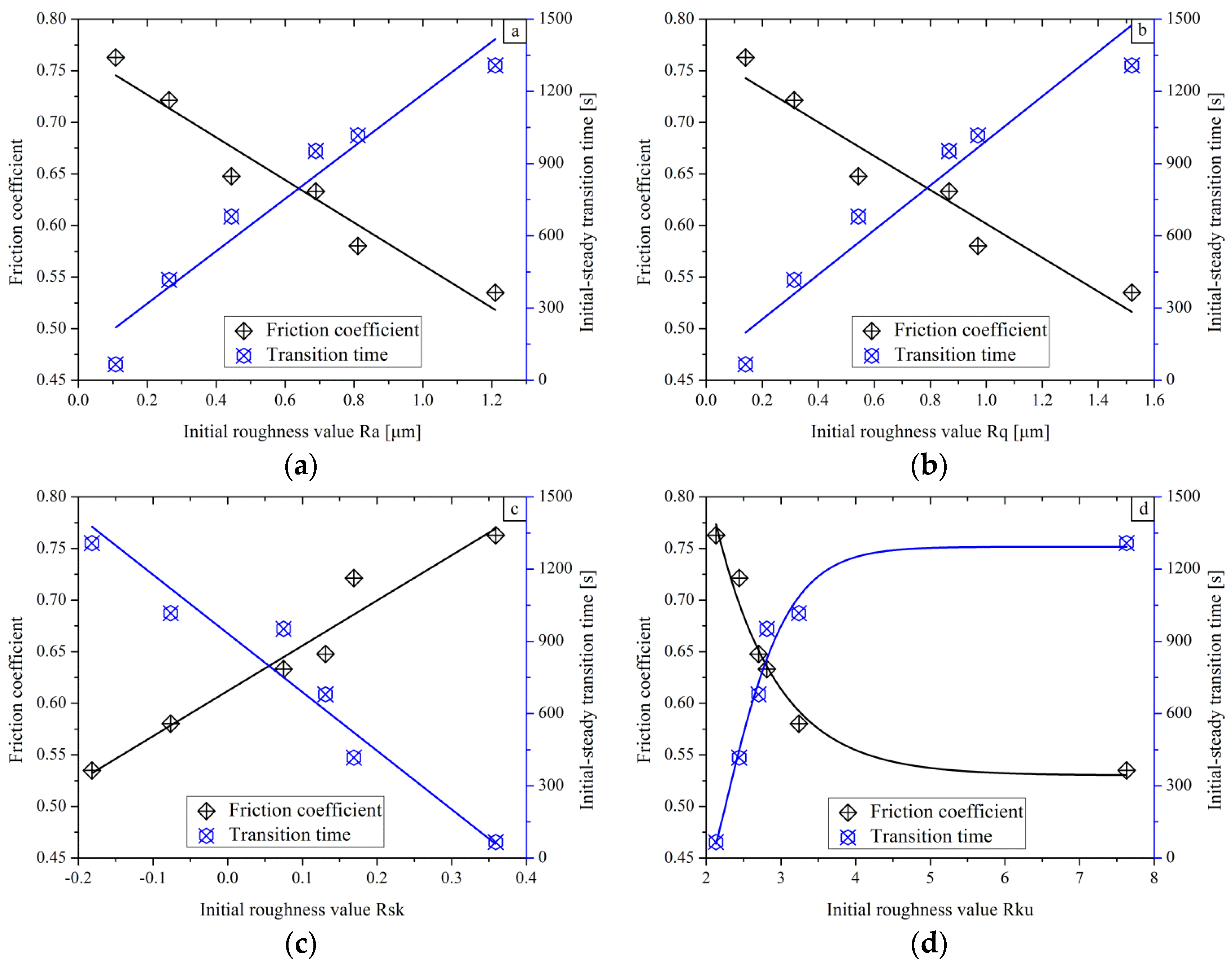
- AISI 1045 steel surfaces with higher initial roughness values of Ra, Rq and Rku result in a larger average friction coefficient and a longer initial steady wear transition period in the sliding friction tests. Surfaces with low Rsk and high Rku values have little benefit for improving the wear resistance and tribological properties in the dry sliding test.
- (2)
- For the AISI 1045 steel in dry sliding tests, it was found that the weight and the volume loss keep approximately the same level for specimens with initial roughness values below a certain limit (Ra 0.3–0.5 µm, Rq 0.1–0.4 µm). In this case, this is not preferable for improving the wear resistance by further decreasing Ra and Rq.
- (3)
- The plastic deformation mainly concentrates in the depth of 20–50 μm under the worn surface. Critical plastic deformation is generated in the samples with surface roughness parameters of higher Ra, Rq and Rku.
- (4)
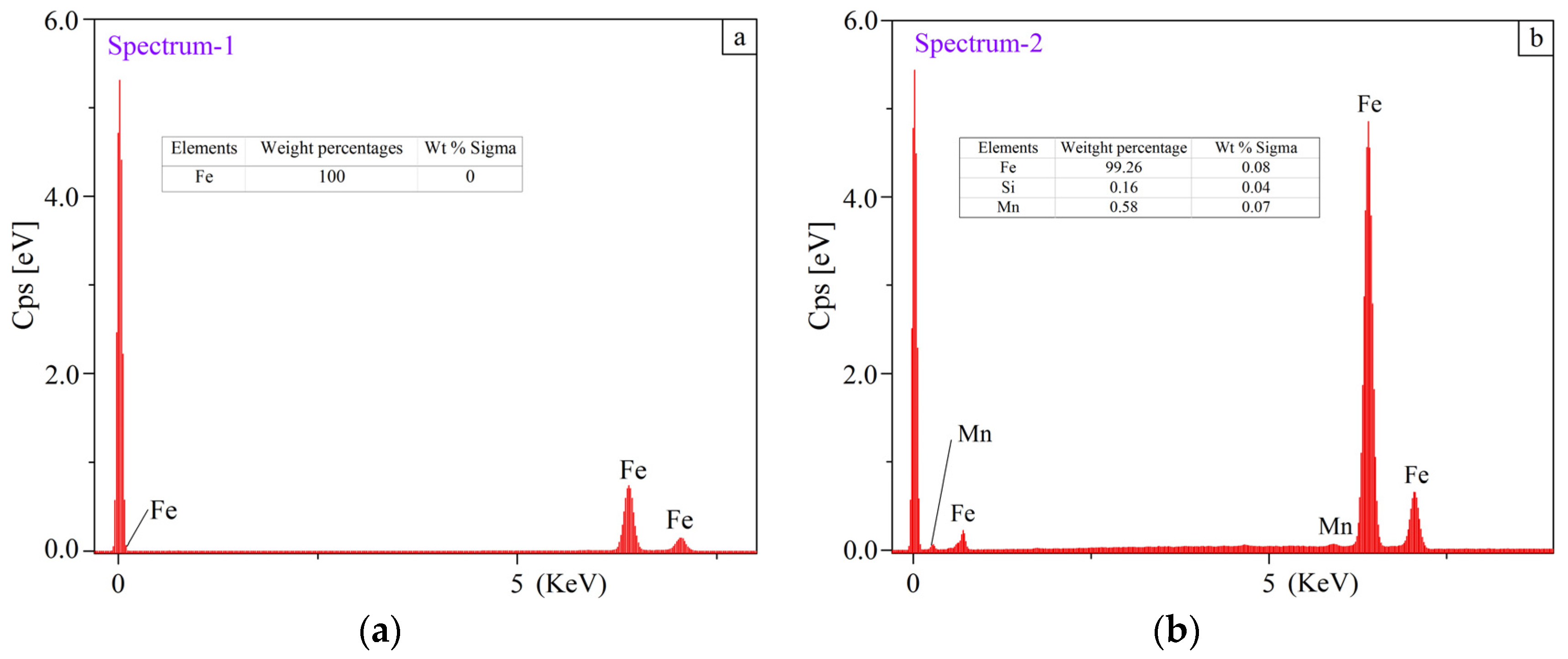
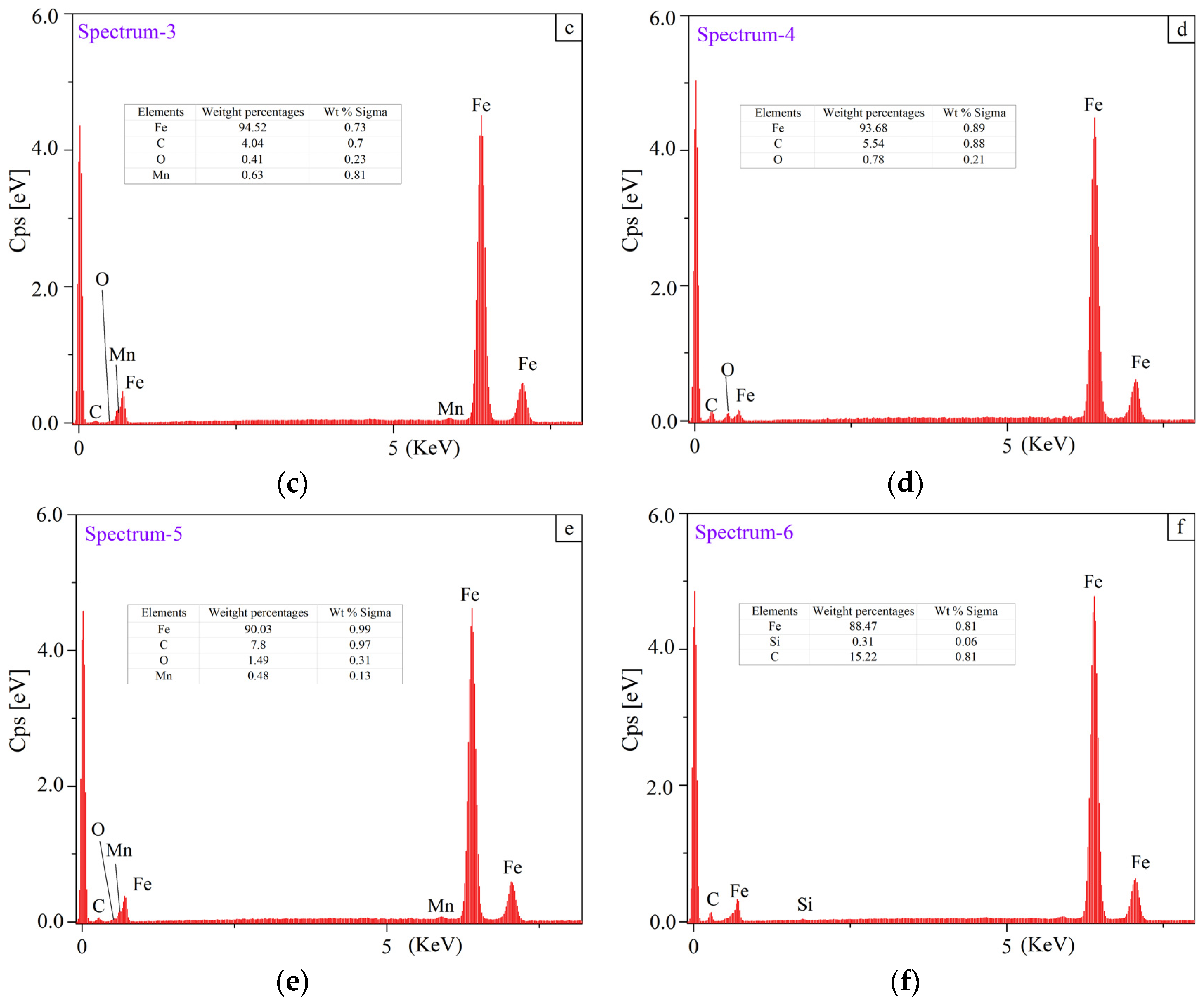
- The Fe element weight percentage measured in the fragmentation zones decreases with increasing initial roughness values of Ra and Rq, whereas a 100% content was found in the sample with the lowest initial roughness values of Ra and Rq. This indicates that the refinement of the grains took place during the sliding.
- (5)
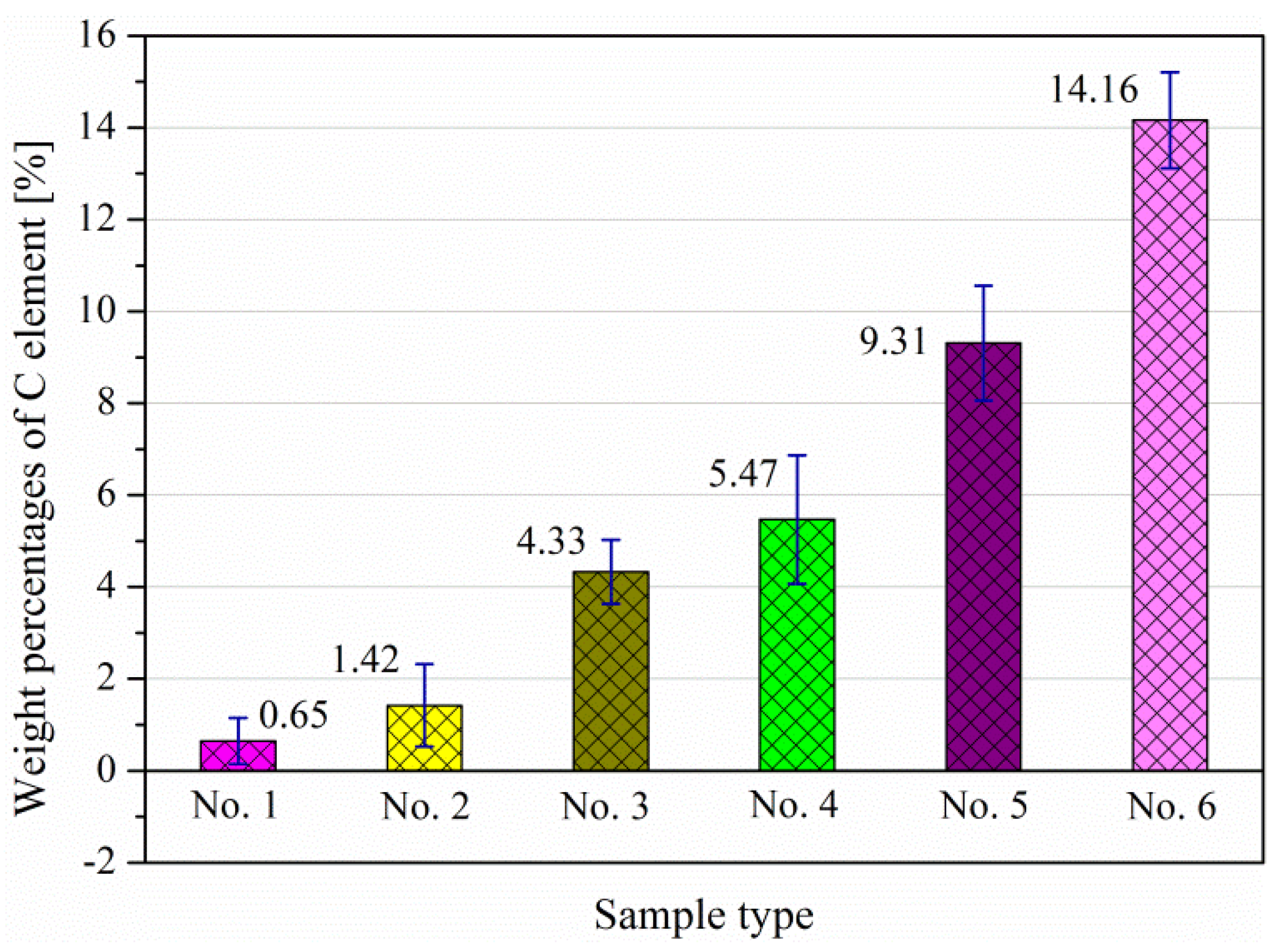
- The initial roughness parameters affect the loss of the C element in the worn surface. Surfaces with large Ra, Rq, low Rsk and high Rku values easily lose the C element in dry sliding.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Kang, R.K.; Yang, Q.F.; Wei, Q. An experimental study on creep feed grinding narrow deep groove of high temperature alloy blade with electroplated CBN wheel. Aeronaut. Manuf. Technol. 1999, 06, 16–18. [Google Scholar]
- Al-Samarai, R.A.; Haftirma; Ahmad, K.R.; Al-Douri, Y. The influence of roughness on the wear and friction coefficient under dry and lubricated sliding. Int. J. Sci. Eng. Res. 2012, 3, 1–6. [Google Scholar]
- Sedlaček, M.; Vilhena, L.M.S.; Podgornik, B.J.; Vižintin, J. Surface topography modelling for reduced friction. J. Mech. Eng. 2011, 57, 674–680. [Google Scholar] [CrossRef]
- Azis, S.A.A.; Hari, I.J.; Ahamad, W. Improving surface properties and wear behaviours of duplex stainless steel via pressure carburizing. Surf. Coat. Technol. 2012, 210, 142–150. [Google Scholar] [CrossRef]
- Saikaew, C.; Baowan, P. Surface finish improvement in ball nose end milling by optimizing operating conditions for different cutting times. India J. Eng. Mater. Sci. 2015, 22, 38–50. [Google Scholar]
- Rech, J.; Claudin, C.; D’Eramo, E. Identification of a friction model-application to the context of dry cutting of an AISI 1045 annealed steel with a TiN-coated carbide tool. Tribol. Int. 2009, 42, 738–744. [Google Scholar] [CrossRef]
- Iqbal, S.A.; Mativenga, P.T.; Sheikhj, M.A. Characterization of machining of AISI 1045 steel over a wide range of cutting speeds. Part 2: Evaluation of flow stress models and interface friction distribution schemes. Proc. Inst. Mech. Eng. B J. Eng. Manuf. 2007, 221, 917–926. [Google Scholar] [CrossRef]
- Abdelali, B.; Claudin, C.; Rech, J.; Salem, W.B.; Kapsa, P.; Dogui, A. Experimental characterization of friction coefficient at the tool-chip-workpiece interface during dry cutting of AISI 1045. Wear 2012, 286–287, 108–115. [Google Scholar] [CrossRef]
- Li, Z.J.; Wang, X. Study on friction and wear characteristics of AISI1045 steel. Eng. Mater. Appl. 2007, 34, 71–72. [Google Scholar]
- Wang, Y.; Wang, S.Q.; Wei, M.X. Study of wear behaviours and wear mechanisms of AISI 1045 steel. Mater. Heat Treat. 2010, 39, 11–14. [Google Scholar]
- Kubiak, K.J.; Liskiewicz, T.W.; Mathia, T.G. Surface morphology in engineering applications: Influence of roughness on sliding and wear in dry fretting. Tribol. Int. 2011, 44, 1427–1432. [Google Scholar] [CrossRef]
- Wang, X.; Wei, X.C.; Zhang, J.; Li, R.B.; Meng, H.; Wang, W.R. Formation of nano-crystallized structure in worn surface layer of T10 steel against 20CrMnTi steel during dry rubbing. J. Nanomater. 2016, 2016, 1–6. [Google Scholar]
- Hughes, D.A.; Dawson, D.B.; Korellis, J.S.; Weingarten, L.I. Near surface microstructures developing under large sliding loads. J. Mater. Eng. Perform. 1994, 3, 459–475. [Google Scholar] [CrossRef]
- Hughes, D.A.; Hansen, N.; Bammann, D.J. Geometrically necessary boundaries, incidental dislocation boundaries and geometrically necessary dislocations. Scr. Mater. 2003, 48, 147–153. [Google Scholar] [CrossRef]
- Hughes, D.A.; Hansen, N. Microstructure and strength of nickel at large strains. Acta Mater. 2000, 48, 2985–3004. [Google Scholar] [CrossRef]
- Lu, S.D.; Wang, Z.B.; Lu, K. Enhanced chromizing kinetics of tool steel by means of surface mechanical attrition treatment. Mater. Sci. Eng. A 2010, 527, 995–1002. [Google Scholar] [CrossRef]
- Hughes, D.A.; Hansen, N. ASM Handbook; ASM International Materials Park: Novelty, OH, USA, 2004; Volume 9, pp. 192–206. [Google Scholar]
- Tao, N.R.; Wang, Z.B.; Tong, W.P.; Sui, M.L.; Lu, J.; Lu, K. An investigation of surface nano-crystallization mechanism in Fe induced by surface mechanical attrition treatment. Acta Mater. 2002, 50, 4603–4616. [Google Scholar] [CrossRef]
- Li, W.L.; Tao, N.R.; Lu, K. Fabrication of a gradient nano-micro-structured surface layer on bulk copper by means of a surface mechanical grinding treatment. Scr. Mater. 2008, 59, 546–549. [Google Scholar] [CrossRef]
- Ni, H.; Alpas, A.T. Sub-micrometer structures generated during dry machining of copper. Mater. Sci. Eng. A 2003, 361, 338–349. [Google Scholar] [CrossRef]
- Zhou, J.M.; Bushly, V.; Peng, R.L.; Stahl, J.E. Identification of subsurface deformation in machining of Inconel 718. Appl. Mech. Mater. 2012, 117–119, 1681–1688. [Google Scholar] [CrossRef]
- Menezes, P.L.; Kishore; Kailas, S.V. Subsurface deformation and the role of surface texture-A study with Cu pins and steel plates. Sadhana-Acad. Proc. Eng. Sci. 2008, 33, 191–201. [Google Scholar]
- Marko, S.; Podgornik, B.; Vižintin, J. Correlation between standard roughness parameters skewness and kurtosis and tribological behaviour of contact surfaces. Tribol. Int. 2012, 48, 102–112. [Google Scholar]
- Sedlaček, M.; Podgornik, B.; Vižintin, J. Influence of surface preparation on roughness parameters, friction and wear. Wear 2009, 266, 482–487. [Google Scholar] [CrossRef]
- Dzierwa, A. Influence of surface preparation on surface topography and tribological behaviours. Arch. Civil. Mech. Eng. 2017, 17, 502–510. [Google Scholar] [CrossRef]
- Lu, W.; Zhang, P.; Liu, X.; Zhai, W.; Zhou, M.; Luo, J.; Zeng, W.; Jiang, X. Influence of surface topography on torsional fretting wear under flat-on-flat contact. Tribol. Int. 2017, 109, 367–372. [Google Scholar] [CrossRef]
- Hyun, S.; Pei, L.; Molinari, J.-F.; Robbins, M.O. Finite-element analysis of contact between elastic self-affine surfaces. Phys. Rev. E 2004, 70, 026117. [Google Scholar] [CrossRef] [PubMed]
- Pei, L.; Hyun, S.; Molinari, J.-F.; Robbins, M.O. Finite element modeling of elasto-plastic contact between rough surfaces. J. Mech. Phys. Sol. 2005, 53, 2385–2409. [Google Scholar] [CrossRef]
- Persson, B.N.J. Elastoplastic contact between randomly rough surfaces. Phys. Rev. Lett. 2001, 87, 116101. [Google Scholar] [CrossRef] [PubMed]
- Persson, B.N.J. Contact mechanics for randomly rough surfaces. Surf. Sci. Rep. 2006, 61, 201–227. [Google Scholar] [CrossRef]
- Almqvist, A.; Campana, C.; Prodanov, N.; Persson, B.N.J. Interfacial separation between elastic solids with randomly rough surfaces: Comparison between theory and numerical techniques. J. Mech. Phys. Solids 2011, 59, 2355–2369. [Google Scholar] [CrossRef]
- Hyun, S.; Robbins, M.O. Elastic contact between rough surfaces: Effect of roughness at large and small wavelengths. Tribol. Int. 2007, 40, 1413–1422. [Google Scholar] [CrossRef]
- Ohsaki, S.; Hono, K.; Hidaka, H.; Takaki, S. Characterization of nanocrystalline ferrite produced by mechanical milling of pearlitic steel. Scr. Mater. 2005, 52, 271–276. [Google Scholar] [CrossRef]
- Rigney, D.A. Sliding wear of metals. Annu. Rev. Mater. Sci. 1988, 18, 141–163. [Google Scholar] [CrossRef]
- Jahanmir, S.; Suh, N.P. Mechanics of subsurface void nucleation wear. Wear 1977, 44, 17–38. [Google Scholar] [CrossRef]
- Adrian, R.J. Particle imaging techniques for experimental fluid mechanics. Wear 1977, 44, 261–304. [Google Scholar] [CrossRef]
- Johnson, K.L. Contact mechanics and the wear of metals. Wear 1995, 190, 162–170. [Google Scholar] [CrossRef]
- Kopalinsky, E.M.; Oxley, P.L.B. Explaining the mechanics of metallic sliding friction and wear in terms of slipline field models of asperity deformation. Wear 1995, 190, 145–154. [Google Scholar] [CrossRef]
- Lei, L.; Weiwei, L.; Yiping, T.; Ben, S.; Webin, H. Friction and wear properties of short carbon fibre reinforced aluminium matrix composites. Wear 2009, 266, 733–738. [Google Scholar]
- Bengisu, M.T.; Akay, A. Stick-slip oscillations: Dynamics of friction and surface roughness. J. Acoust. Soc. Am. 1999, 105, 194–205. [Google Scholar] [CrossRef]
- Federici, M.; Menapace, C.; Moscatelli, A.; Gialanella, S.; Straffelini, G. Effect of roughness on the wear behavior of HVOF coatings dry sliding against a friction material. Wear 2016, 368, 326–334. [Google Scholar] [CrossRef]
- Larbi, S.; Bilek, A.; Meghlat, A. Study of the effect of speed and load on wear mechanism of a plan/plan contact with the help of a strain gage dynamometer. EPJ Web Conf. 2010, 6, 19006. [Google Scholar]
- Bayer, R.G.; Sirico, J.L. The influence of surface roughness on wear. Wear 1975, 35, 251–260. [Google Scholar] [CrossRef]
- Zmitrowicz, A. Wear debris: A review of properties and constitutive models. J. Theor. Appl. Mech. 2005, 43, 3–35. [Google Scholar]
- Ohmae, N. Analysis of the large plastic deformation involved in wear processes using the finite element method with an updated Lagrangian. J. Tribol. 1987, 109, 330–337. [Google Scholar] [CrossRef]




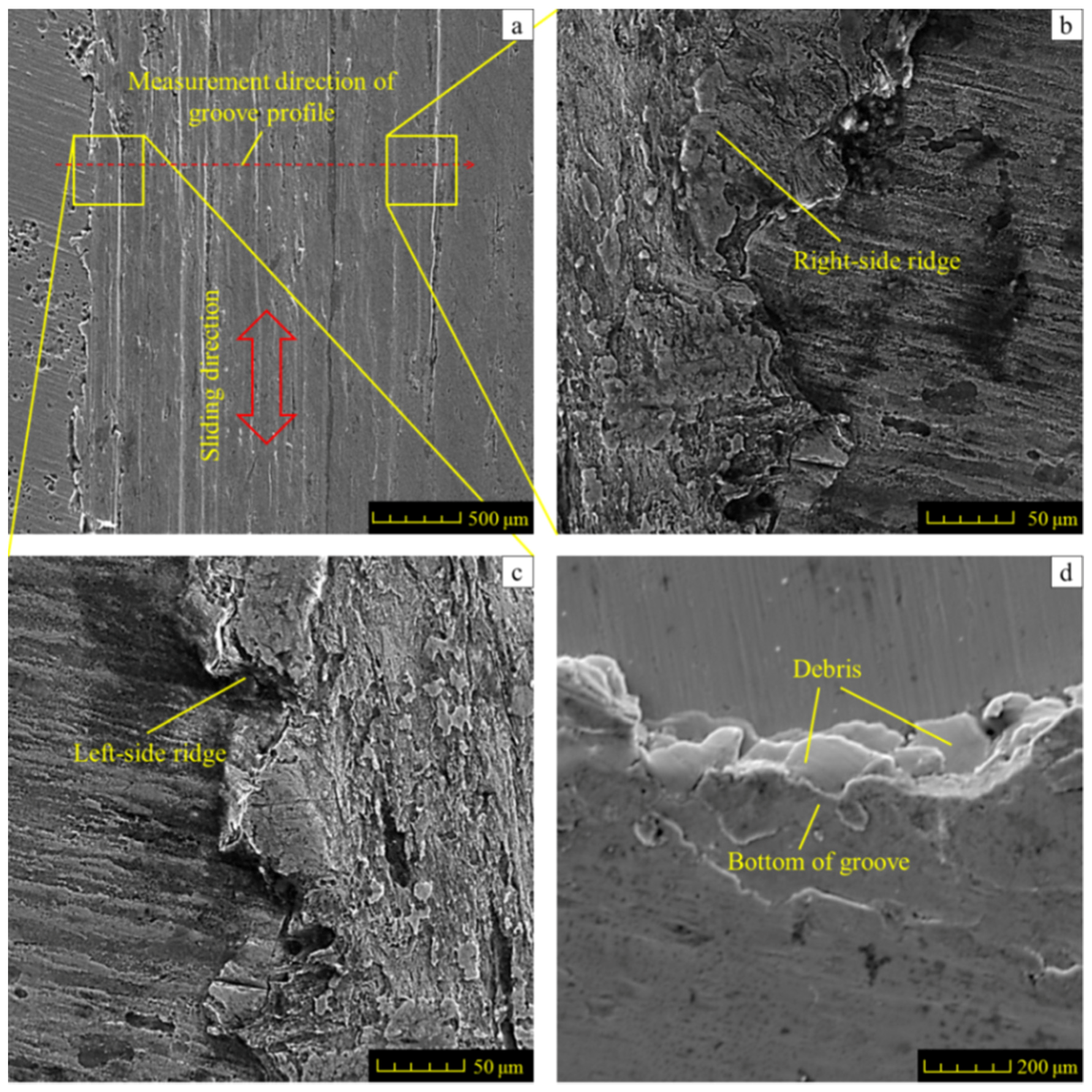
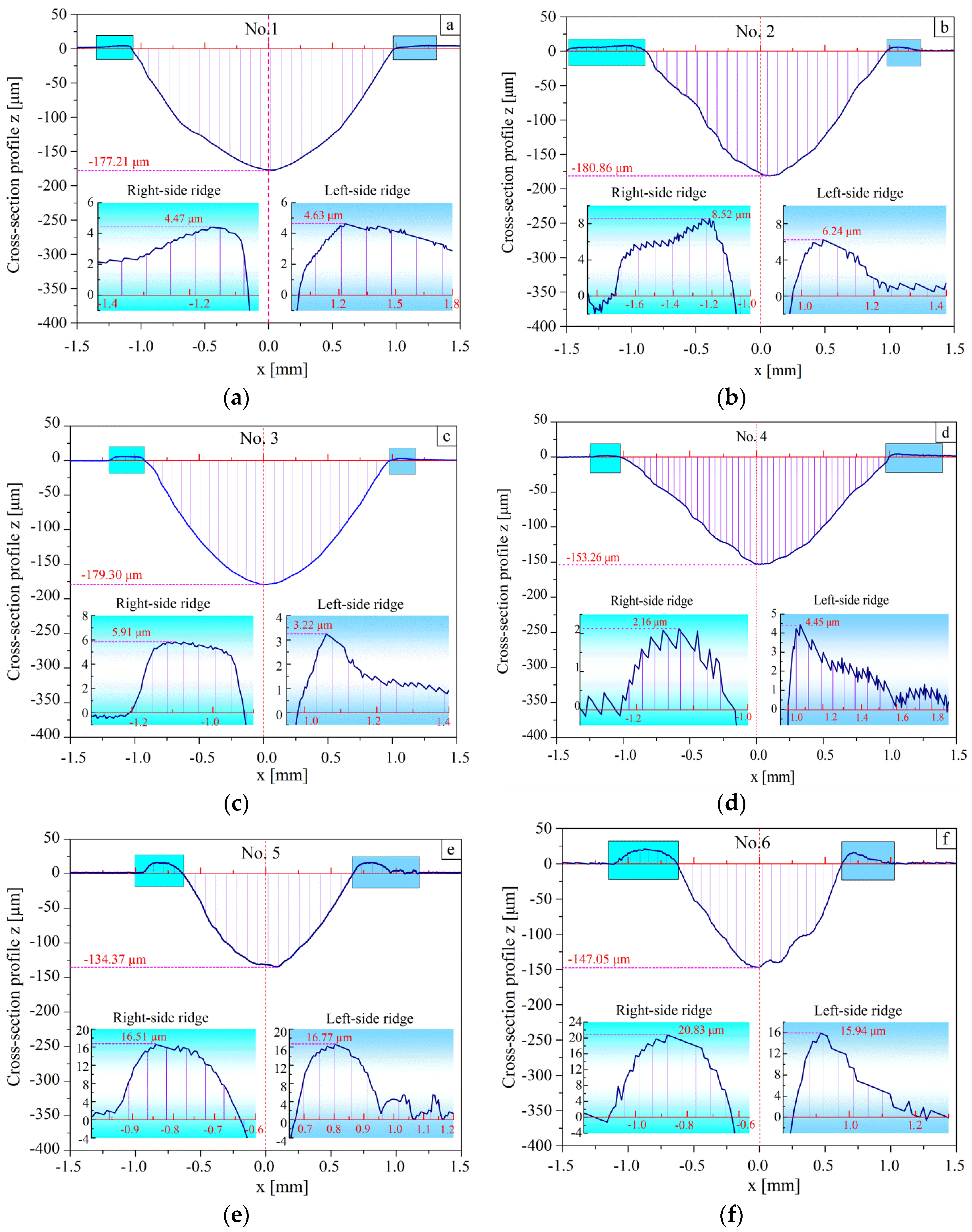
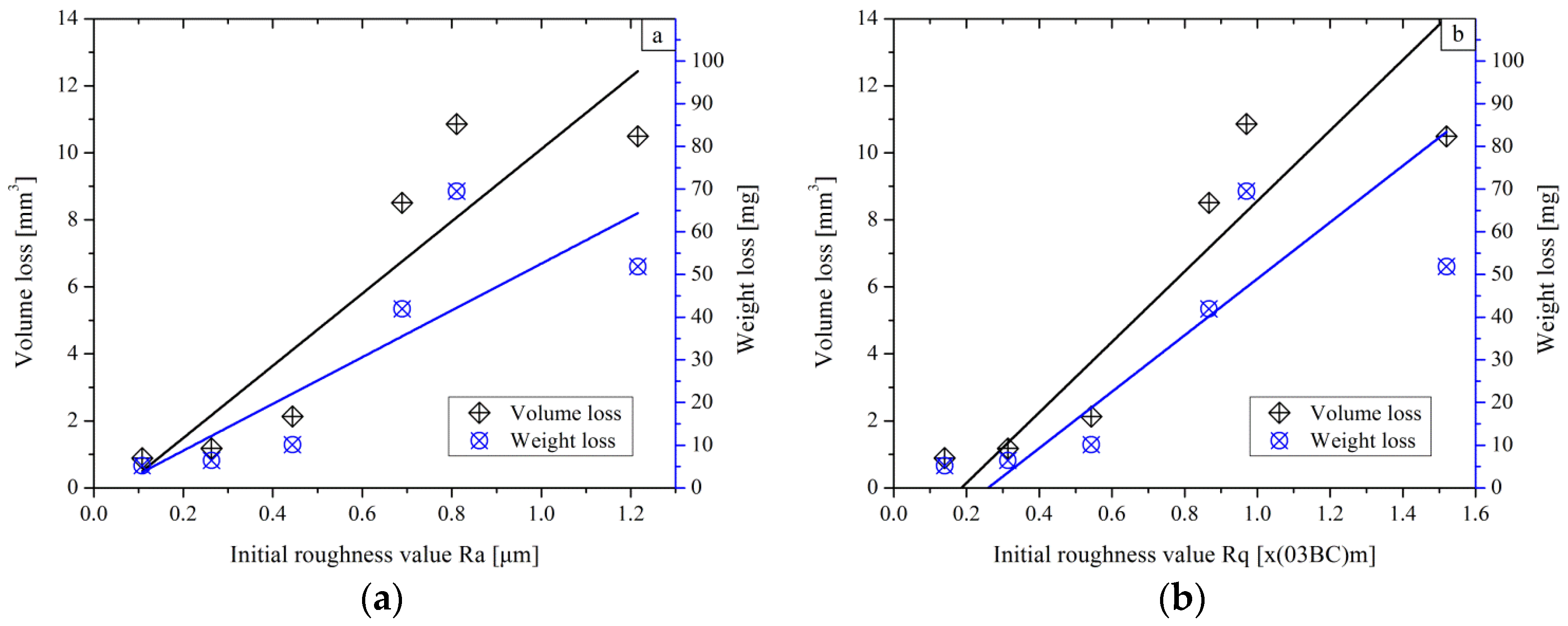
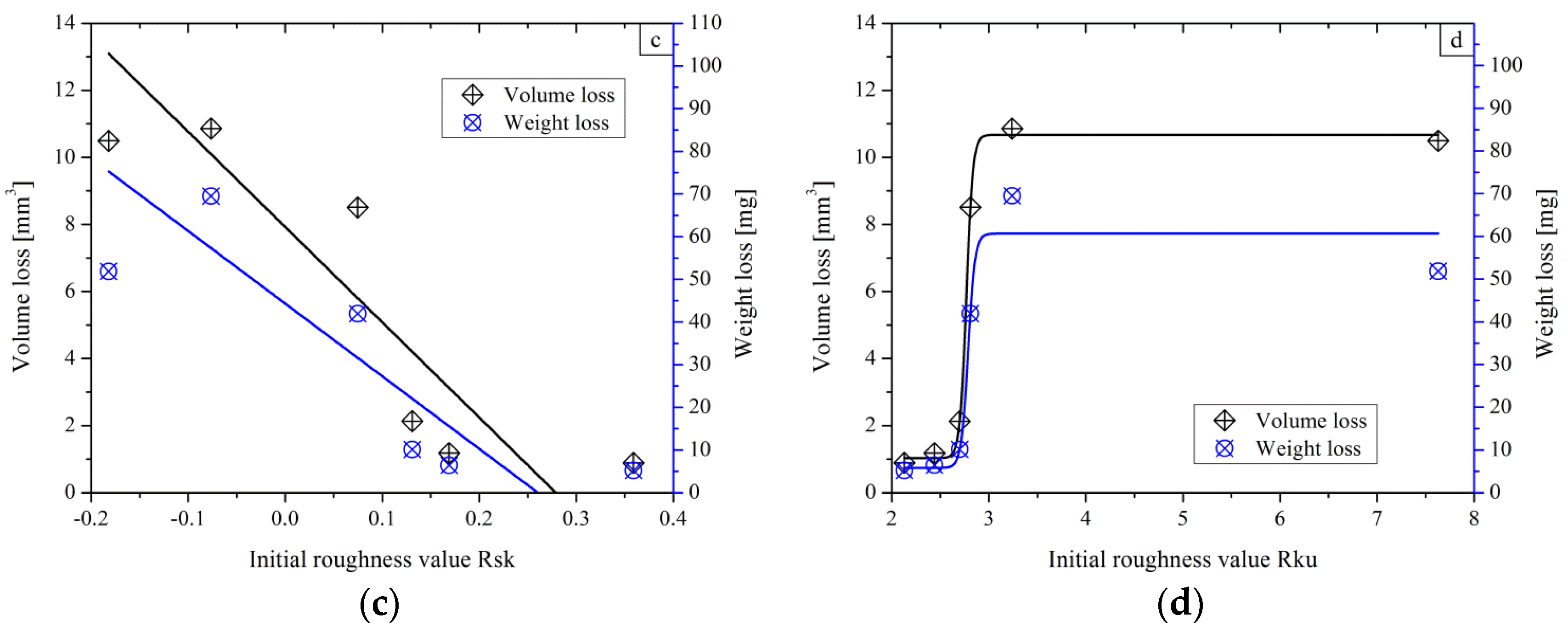

| C | P | Si | Ca | Mn | Mo | Ni | Cr | W | Fe |
|---|---|---|---|---|---|---|---|---|---|
| 0.46 | 0.03 | 0.31 | 0.4 | 0.65 | 0.08 | 0.39 | 0.04 | <0.01 | Balance |
| Samples | 3D Topography of Ground Surfaces | SEM of Ground Surfaces | Ra (μm) | Rq (μm) | Rsk | Rku |
|---|---|---|---|---|---|---|
| No. 1 |  | 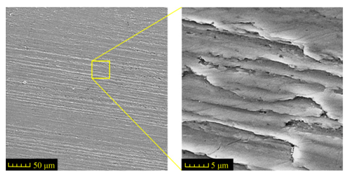 | 1.127 | 1.52 | −0.182 | 7.63 |
| No. 2 |  |  | 0.805 | 0.970 | −0.0764 | 3.24 |
| No. 3 |  | 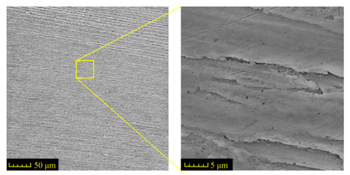 | 0.689 | 0.867 | 0.0748 | 2.81 |
| No. 4 |  |  | 0.449 | 0.543 | 0.131 | 2.70 |
| No. 5 |  | 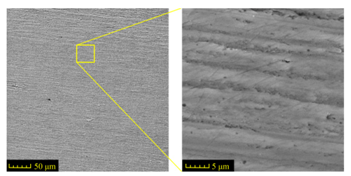 | 0.263 | 0.314 | 0.169 | 2.44 |
| No. 6 |  |  | 0.108 | 0.140 | 0.359 | 2.13 |
| C | Si | Mn | Ni | P | S | Mo | Cr | Cu |
|---|---|---|---|---|---|---|---|---|
| 0.95~1.05 | 0.15~0.35 | 0.20~0.40 | ≤0.03 | ≤0.027 | ≤0.02 | ≤0.10 | 1.3~1.65 | ≤0.025 |
© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liang, G.; Schmauder, S.; Lyu, M.; Schneider, Y.; Zhang, C.; Han, Y. An Investigation of the Influence of Initial Roughness on the Friction and Wear Behavior of Ground Surfaces. Materials 2018, 11, 237. https://doi.org/10.3390/ma11020237
Liang G, Schmauder S, Lyu M, Schneider Y, Zhang C, Han Y. An Investigation of the Influence of Initial Roughness on the Friction and Wear Behavior of Ground Surfaces. Materials. 2018; 11(2):237. https://doi.org/10.3390/ma11020237
Chicago/Turabian StyleLiang, Guoxing, Siegfried Schmauder, Ming Lyu, Yanling Schneider, Cheng Zhang, and Yang Han. 2018. "An Investigation of the Influence of Initial Roughness on the Friction and Wear Behavior of Ground Surfaces" Materials 11, no. 2: 237. https://doi.org/10.3390/ma11020237
APA StyleLiang, G., Schmauder, S., Lyu, M., Schneider, Y., Zhang, C., & Han, Y. (2018). An Investigation of the Influence of Initial Roughness on the Friction and Wear Behavior of Ground Surfaces. Materials, 11(2), 237. https://doi.org/10.3390/ma11020237






