Experimental Study on Bi-Axial Mechanical Properties of Warp-knitted Meshes with and without Initial Notches
Abstract
1. Introduction
2. Experimental
2.1. Materials
2.2. Fabrication of Warp-Knitted Meshes
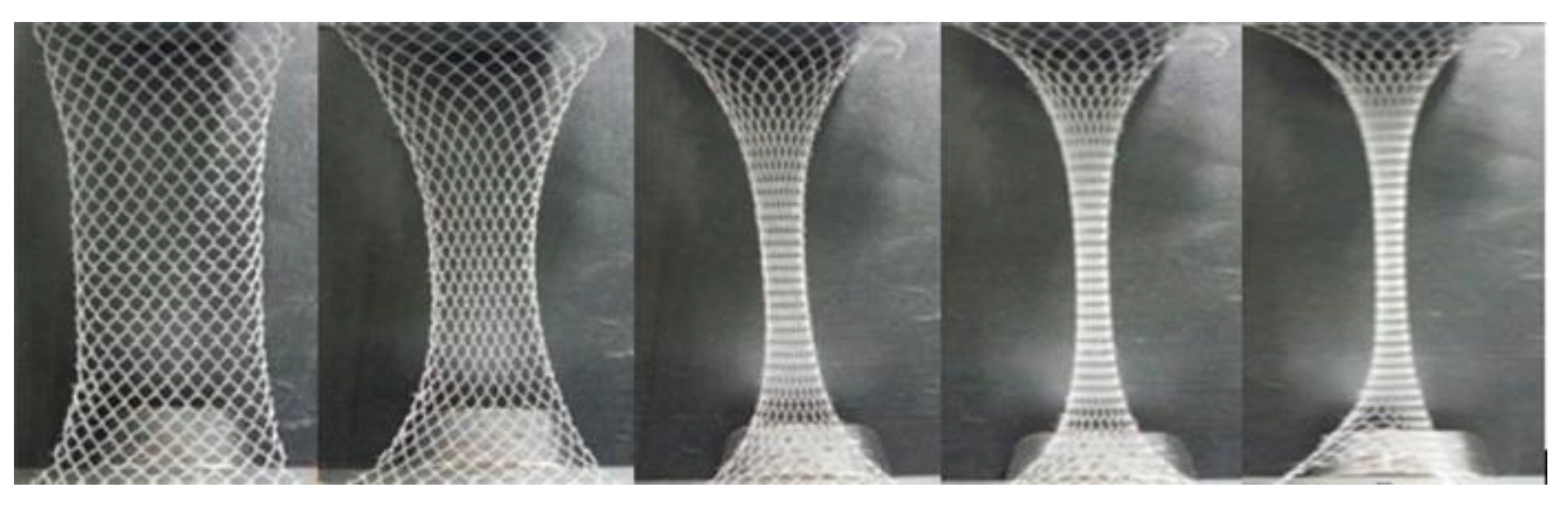
2.3. Testing Conditions
3. Results and Discussion
3.1. Mechanical Behaviors under Mono-Axial and Multi-Axial Tensile Tests
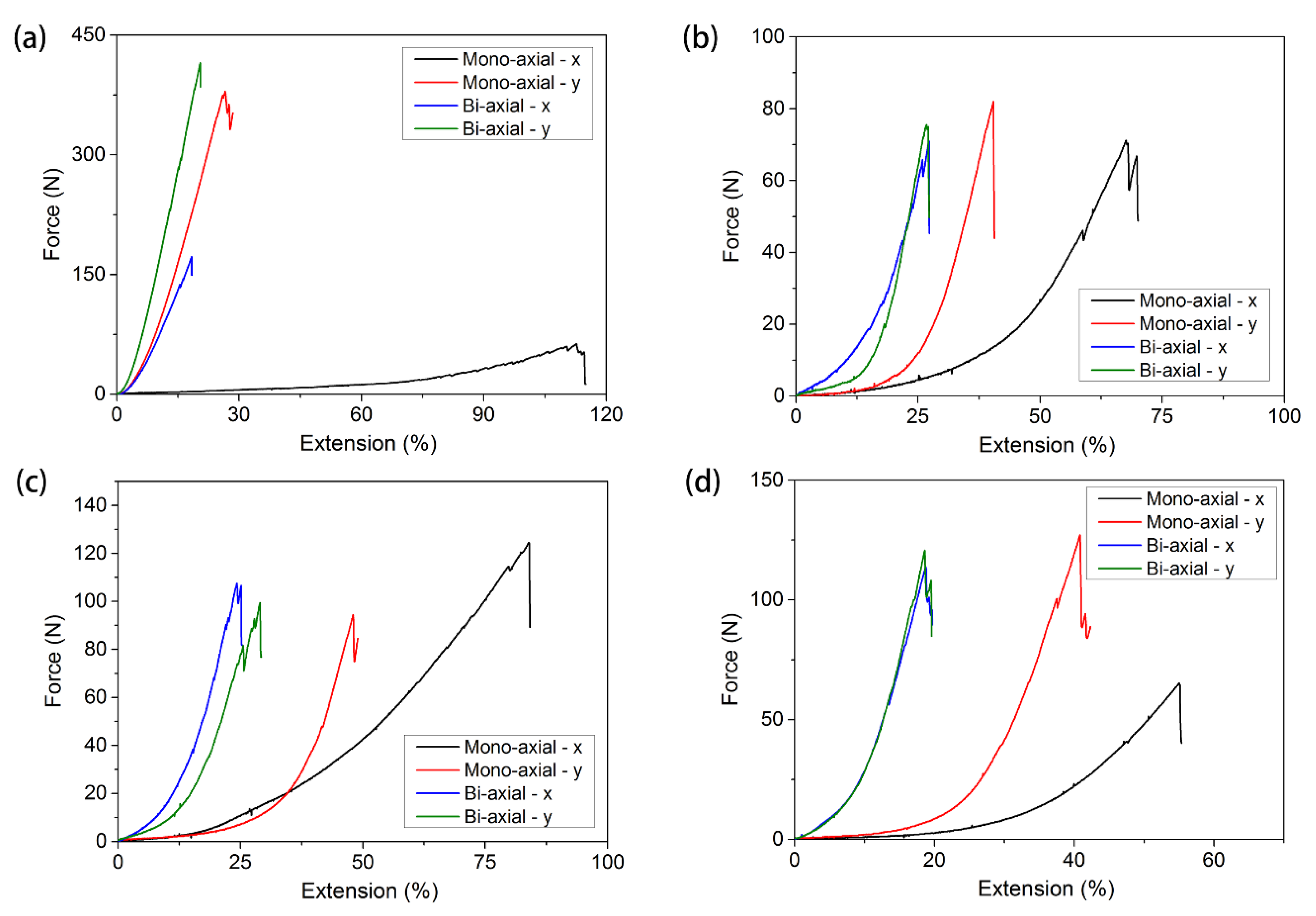
3.1.1. Mono-Axial Tensile Properties
3.1.2. Bi-Axial Tensile Properties
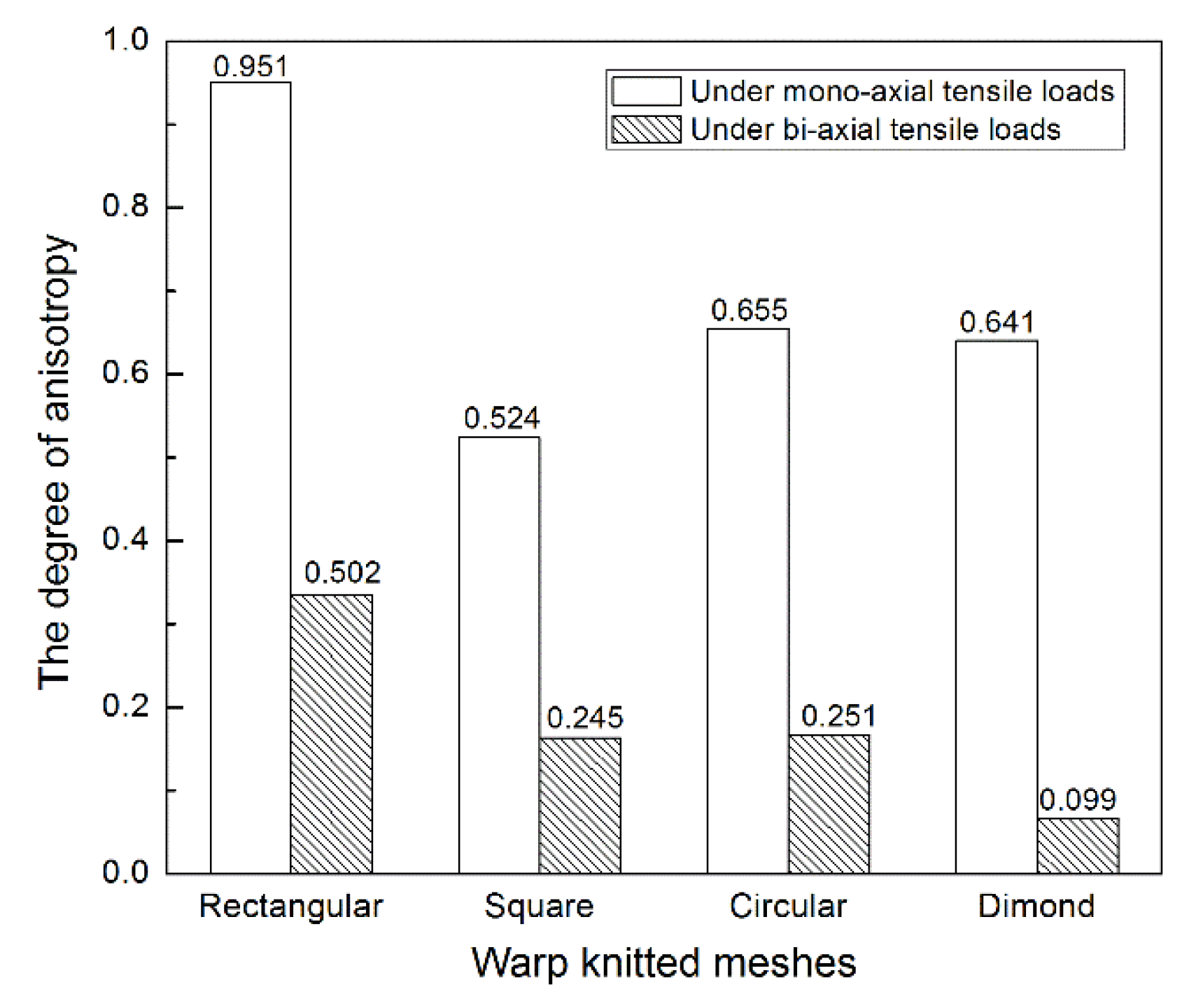
3.1.3. Mechanical Anisotropy Properties
3.2. Mechanical Behavior with Initial Cracks
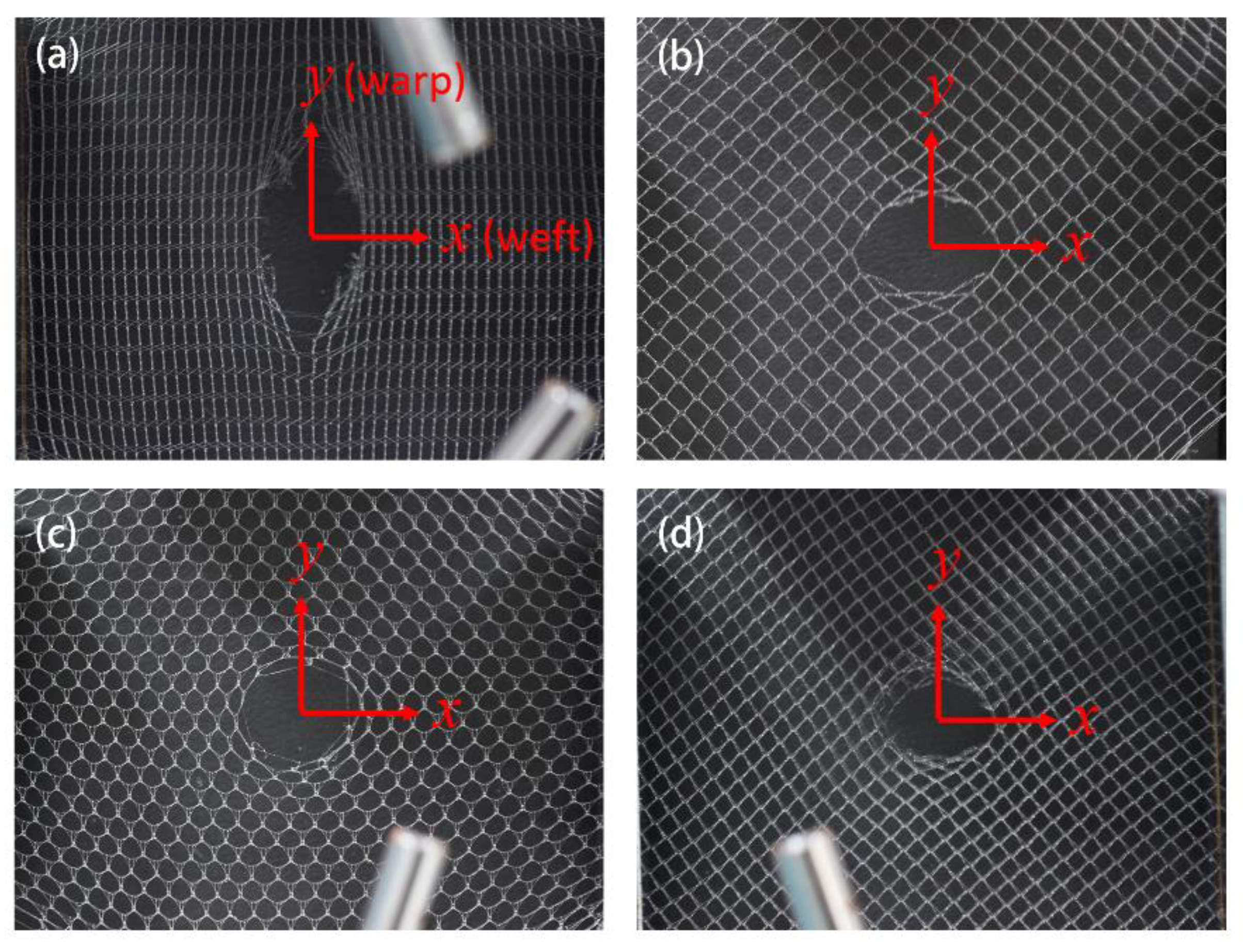
3.2.1. Notch Propagation Analysis
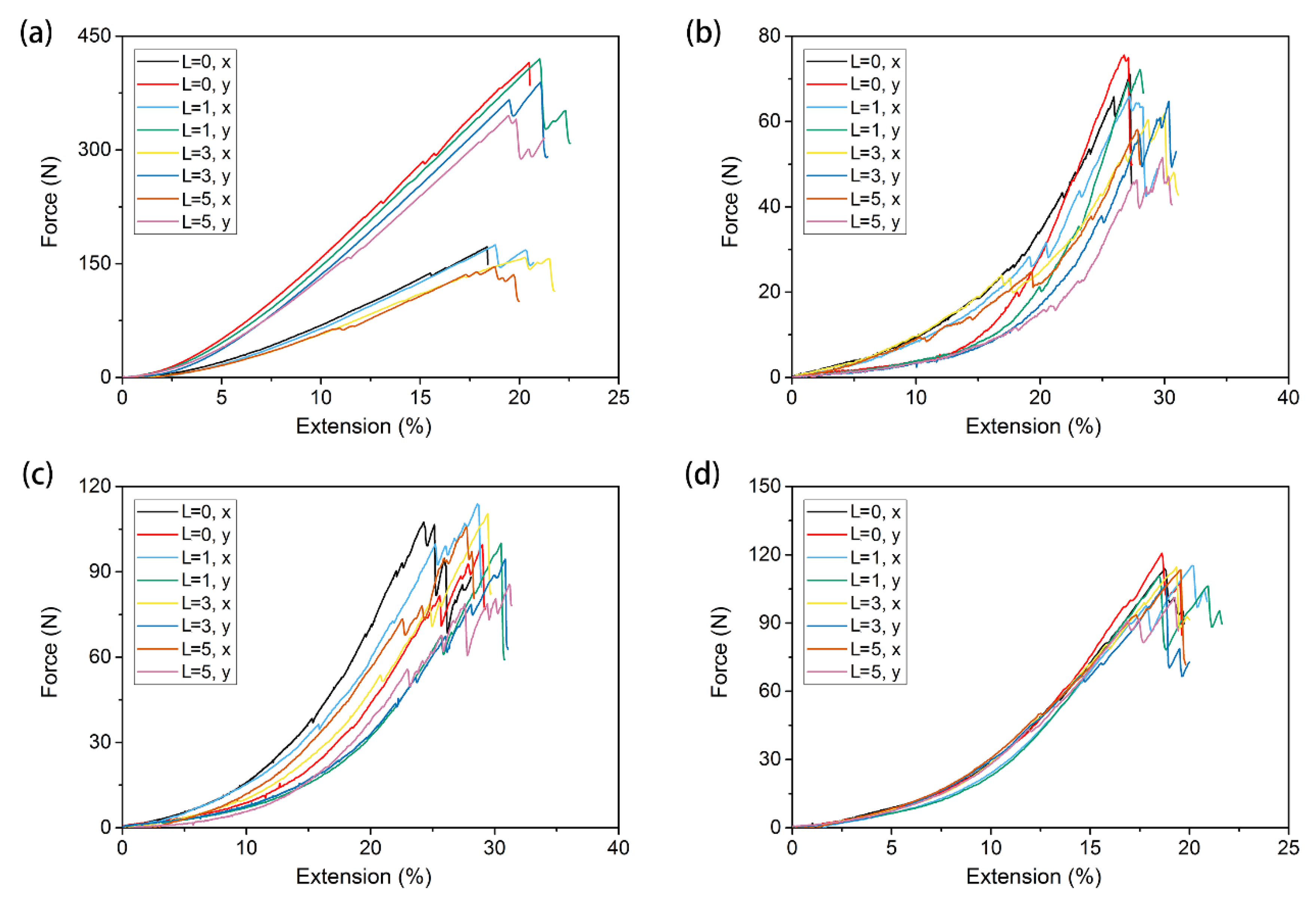
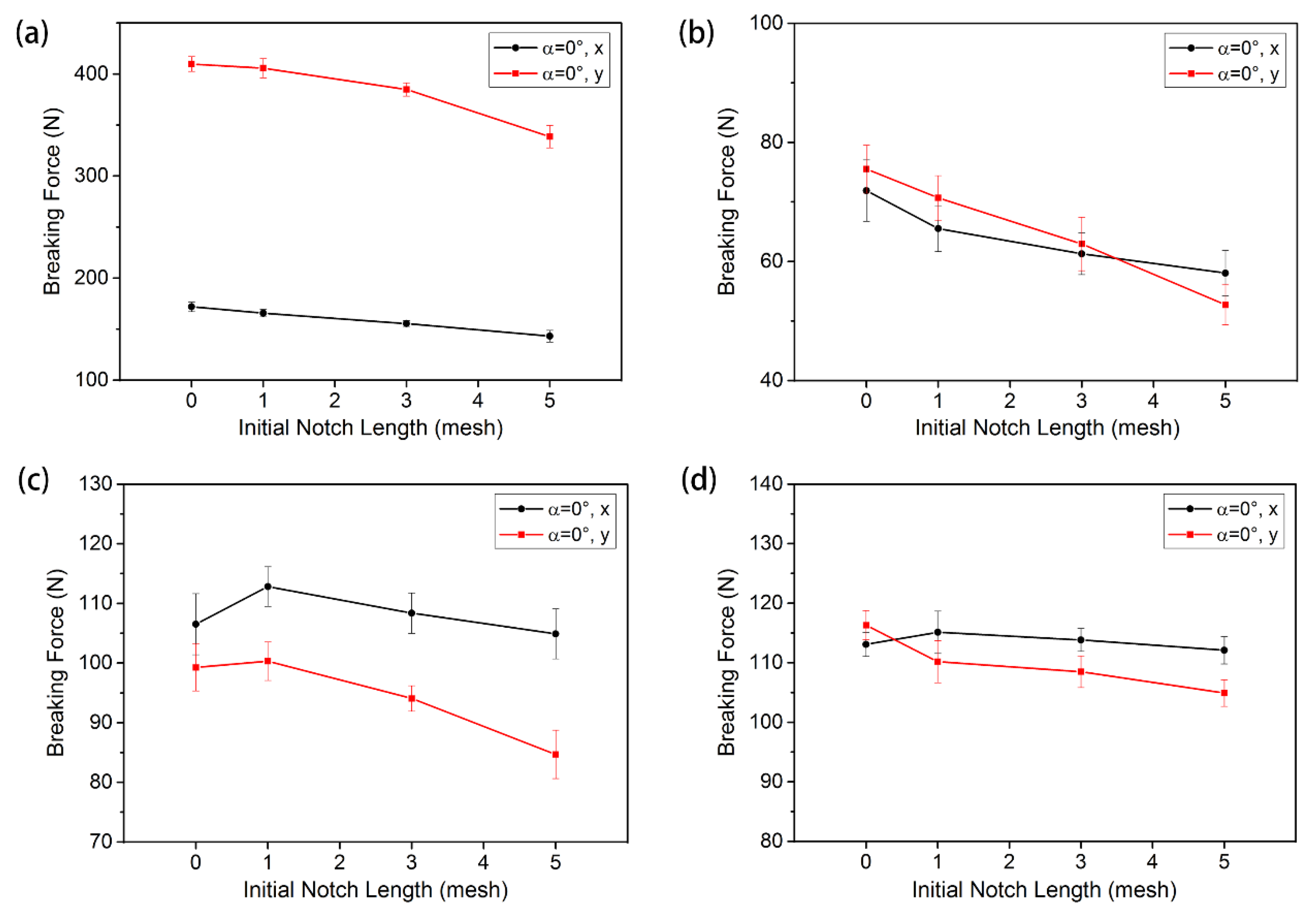
3.2.2. Effect of Initial Notch Length on Mechanical Properties
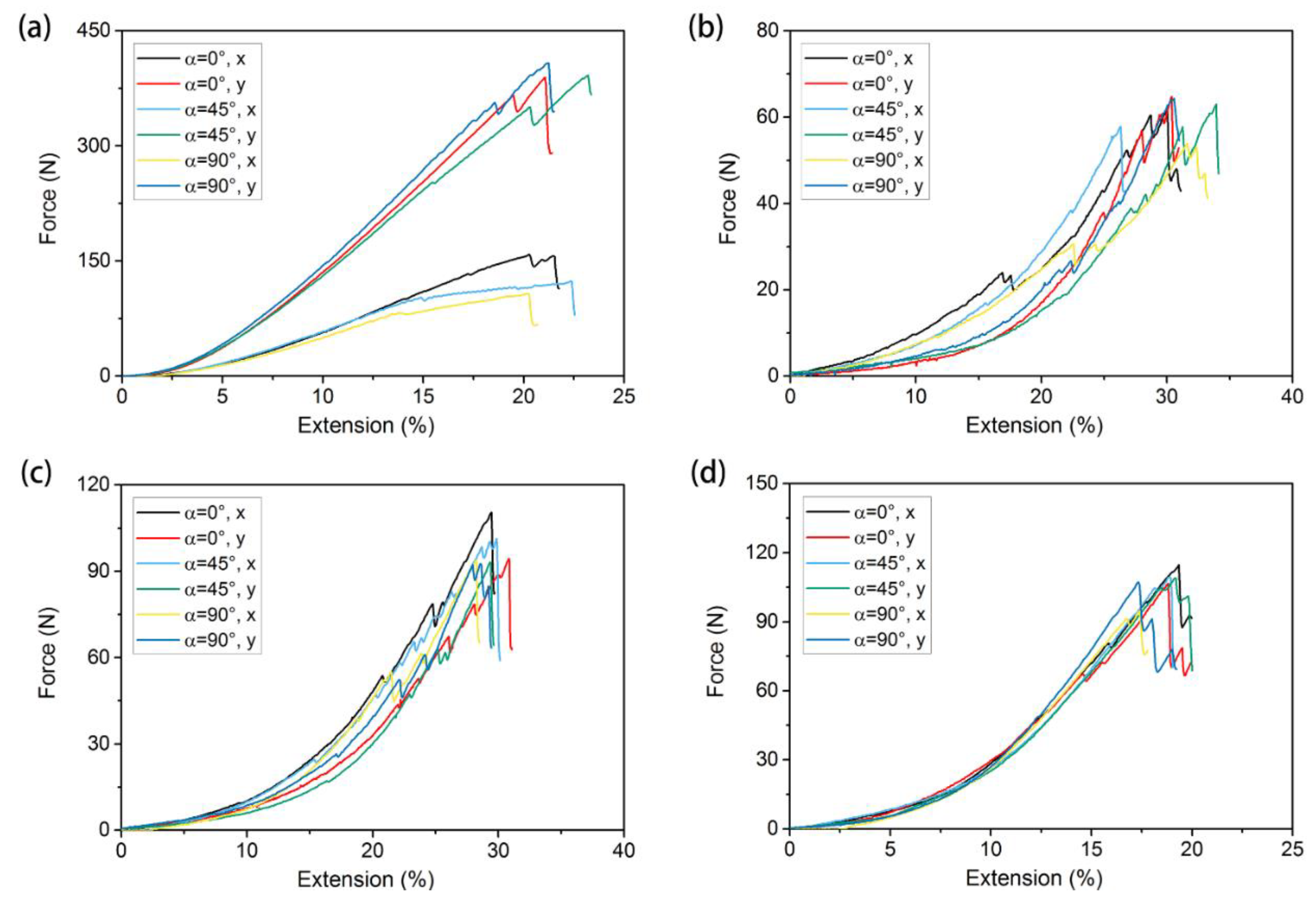
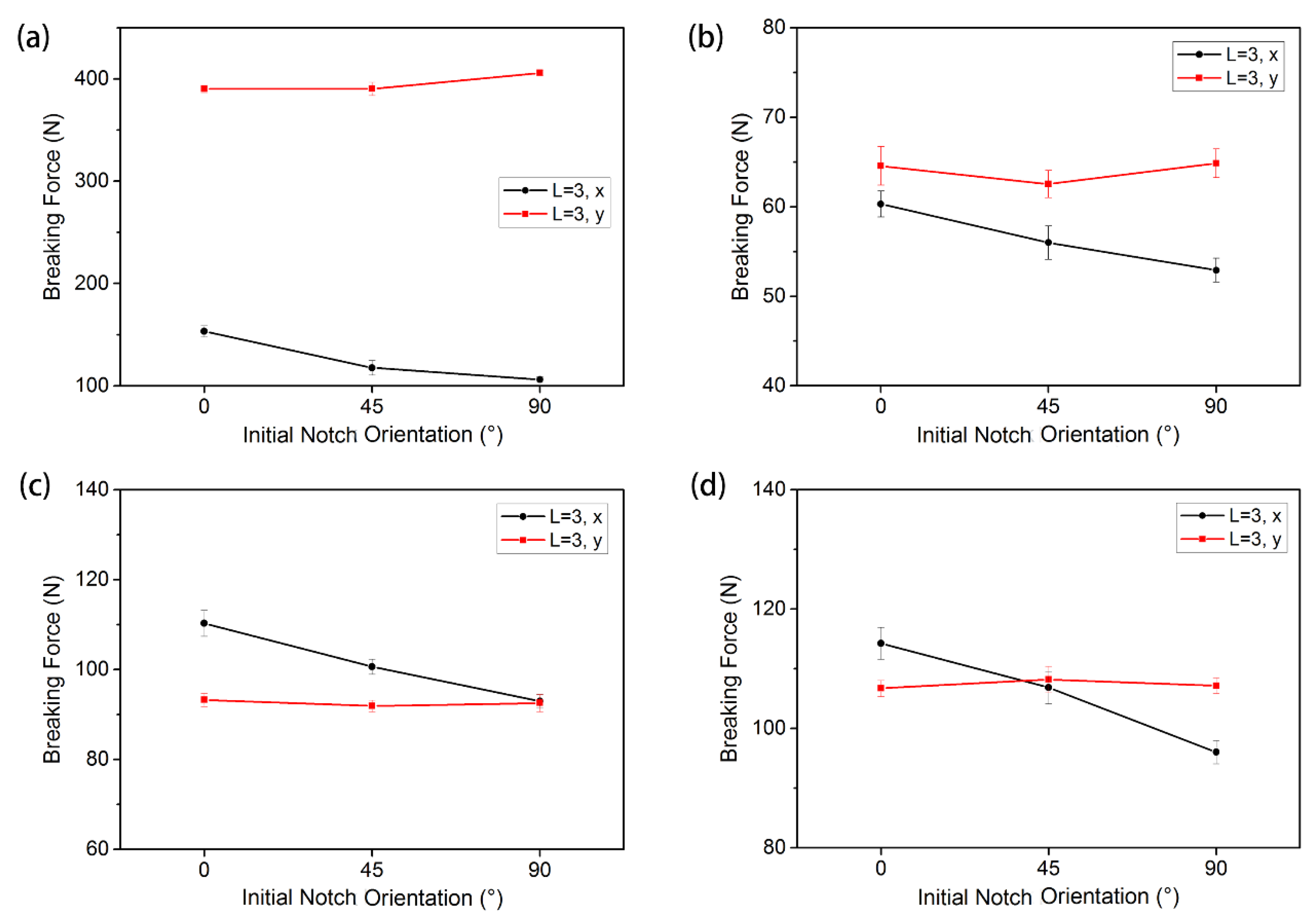
3.2.3. Effect of Crack Angle on Mechanical Properties
4. Conclusions
- (1)
- Warp-knitted meshes presented different mechanical behavior under mono-axial and bi-axial stresses. The meshes under biaxial stresses tended to be more isotropic, and the anisotropy level was decided by the fabric structure. The anisotropy degree of the diamond warp-knitted mesh was the lowest (λ = 0.099), while the rectangular one was the most anisotropic (λ = 0.502).
- (2)
- The mechanical performance of warp-knitted meshes was affected by notches. The failure mode of the meshes with notches under biaxial stresses was related to the anisotropy level. The notch on a significantly anisotropic warp-knitted mesh (rectangular mesh λ = 0.502) usually propagated along the direction of the larger modulus (ET = 16.845 MPa). For a not remarkably anisotropic mesh (λ = 0.099, 0.245, 0.251), notch propagation was probably consistent with the initial notch orientation.
- (3)
- The breaking strength of warp-knitted meshes with the same initial notch orientation decreased with the increase of notch length in both the wale and course directions. The effect varied according to the fabric structure. The diamond warp-knitted mesh had a minimal effect on the reduction of the mechanical performance. For warp-knitted meshes with the same initial notch length, the breaking force in the wale direction was stable at different notch orientations, while that in the course direction decreased remarkably with notch orientation from 0° to 90°.
Author Contributions
Funding
Conflicts of Interest
References
- Kim, D.; Ha, S. Effects of Particle Size on the Shear Behavior of Coarse Grained Soils Reinforced with Geogrid. Materials 2014, 7, 963–979. [Google Scholar] [CrossRef] [PubMed]
- Liu, X.-M.; Chen, N.-L.; Feng, X.-W. Investigation on the knittability of glass yarn. J Text. Inst. 2009, 100, 440–450. [Google Scholar] [CrossRef]
- Jiang, J.; Chen, N.; Geng, Y.; Shao, H.; Lin, F. Advanced Grid Structure-Reinforced Composites. In Porous Lightweight Composites Reinforced with Fibrous Structures; Springer: Berlin/Heidelberg, Germany, 2017; pp. 129–155. ISBN 978-3-662-53802-9. [Google Scholar]
- Russo, S. Damage assessment of GFRP pultruded structural elements. Compos. Struct. 2013, 96, 661–669. [Google Scholar] [CrossRef]
- Ghadimi, B.; Russo, S.; Rosano, M. Predicted mechanical performance of pultruded FRP material under severe temperature duress. Compos. Struct. 2017, 176, 673–683. [Google Scholar] [CrossRef]
- Leong, K.H.; Ramakrishna, S.; Huang, Z.M.; Bibo, G.A. The potential of knitting for engineering composites—A review. Compos. Part A Appl. Sci. Manuf. 2000, 31, 197–220. [Google Scholar] [CrossRef]
- Maurer, M.M.; Roehrnbauer, B.; Feola, A.; Deprest, J.; Mazza, E. Prosthetic Meshes for Repair of Hernia and Pelvic Organ Prolapse: Comparison of Biomechanical Properties. Materials 2015, 8, 2794–2808. [Google Scholar] [CrossRef]
- Lu, Y.; Zhang, P. Fabrication and evaluation of a warp knitted polypropylene/polylactic acid composite mesh for pelvic floor repair. Text. Res. J. 2017. [Google Scholar] [CrossRef]
- Mirjavan, M.; Asayesh, A.; Asgharian Jeddi, A.A. The effect of fabric structure on the mechanical properties of warp knitted surgical mesh for hernia repair. J. Mech. Behav. Biomed. Mater. 2017, 66, 77–86. [Google Scholar] [CrossRef] [PubMed]
- Zhang, L.; Jiang, J.H.; Chen, N.L. Relationship between Knitting Parameters and Mechanical Properties of Warp Knitting Mesh Fabric. Adv. Mater. Res. 2013, 627, 374–377. [Google Scholar] [CrossRef]
- Shao, G.-W.; Jiang, J.-H.; Pan, Q.-L.; Liu, Y.-Y.; Chen, N.-L. Performance of flexible warp knitting metal mesh with notch. J. Donghua Univ. (Engl. Ed.) 2014, 31, 582–588. [Google Scholar]
- Kawabata, S. Nonlinear mechanics of woven and knitted materials. In Textile Structural Composites; Elservier: New York, NY, USA, 1989; pp. 67–116. ISBN 978-0-444-42992-6. [Google Scholar]
- Minami, H.; Motobayashi, S. Experimental Research on Uniaxial and Biaxial Tensile Strength of Coated Fabrics with Variously Shaped Defects. J. Coated Fabr. 1981, 11, 24–40. [Google Scholar] [CrossRef]
- Minami, H. Strength of Coated Fabrics With Crack. J. Coat. Fabr. 1978, 7, 269–292. [Google Scholar] [CrossRef]
- Alif, N.; Carlsson, L.A.; Boogh, L. The effect of weave pattern and crack propagation direction on mode I delamination resistance of woven glass and carbon composites. Compos. Part B Eng. 1998, 29, 603–611. [Google Scholar] [CrossRef]
- Bigaud, D.; Szostkiewicz, C.; Hamelin, P. Tearing analysis for textile reinforced soft composites under mono-axial and bi-axial tensile stresses. Compos. Struct. 2003, 62, 129–137. [Google Scholar] [CrossRef]
- Wang, Z.; Chen, N.; Jiang, J. Tensile behavior of textile reinforced flexible composites with notch. Ind. Text. 2011, 62, 24–29. [Google Scholar]
- Longbin, L.; Mingyun, L.; Houdi, X. Tear strength characteristics of laminated envelope composites based on single edge notched film experiment. Eng. Fract. Mech. 2014, 127, 21–30. [Google Scholar] [CrossRef]
- Luo, Y.; Hong, H.; Fangueiro, R. Tensile and tearing properties of bi-axial warp knitted coated fabrics. Autex Res. J. 2008, 8, 17–20. [Google Scholar]
- Luo, Y.; Hu, H. Mechanical properties of PVC coated bi-axial warp knitted fabric with and without initial cracks under multi-axial tensile loads. Compos. Struct. 2009, 89, 536–542. [Google Scholar] [CrossRef]
- Triki, E.; Dolez, P.; Vu-Khanh, T. Tear resistance of woven textiles—Criterion and mechanisms. Compos. Part B Eng. 2011, 42, 1851–1859. [Google Scholar] [CrossRef]
- Triki, E.; Vu-Khanh, T.; Nguyen-Tri, P.; Boukehili, H. Mechanics and mechanisms of tear resistance of woven fabrics. Theor. Appl. Fract. Mech. 2012, 61, 33–39. [Google Scholar] [CrossRef]
- Poincloux, S.; Adda-Bedia, M.; Lechenault, F. Crackling Dynamics in the Mechanical Response of Knitted Fabrics. Phys. Rev. Lett. 2018, 121. [Google Scholar] [CrossRef] [PubMed]
- Xu, H.-Y.; Jiang, J.-H.; Cheng, N.-L.; Lin, F.-B.; Shao, H.-Q. Finite Element Modeling for the Uni-Axial Tensile Behaviour of Metallic Warp-Knitted Fabric. Fibres Text. East. Eur. 2018, 26, 49–54. [Google Scholar] [CrossRef]
- Saberski, E.R.; Orenstein, S.B.; Novitsky, Y.W. Anisotropic evaluation of synthetic surgical meshes. Hernia 2011, 15, 47–52. [Google Scholar] [CrossRef] [PubMed]
- Shepherd, J.P.; Feola, A.J.; Abramowitch, S.D.; Moalli, P.A. Uniaxial biomechanical properties of seven different vaginally implanted meshes for pelvic organ prolapse. Int. Urogynecol. J. 2012, 23, 613–620. [Google Scholar] [CrossRef] [PubMed]
- Grosberg, P. The Geometry of Warp Knitted Fabrics. J. Text. Inst. Trans. 1960, 51, T39–T48. [Google Scholar] [CrossRef]
- Xu, H.-Y.; Chen, N.-L.; Jiang, J.-H.; Jin, L.-X.; Wang, Z.-X. 3D Simulation of Warp Knitted Structures with a New Algorithm Based on NURBS. Fibres Text. East. Eur. 2015, 23, 57–60. [Google Scholar]
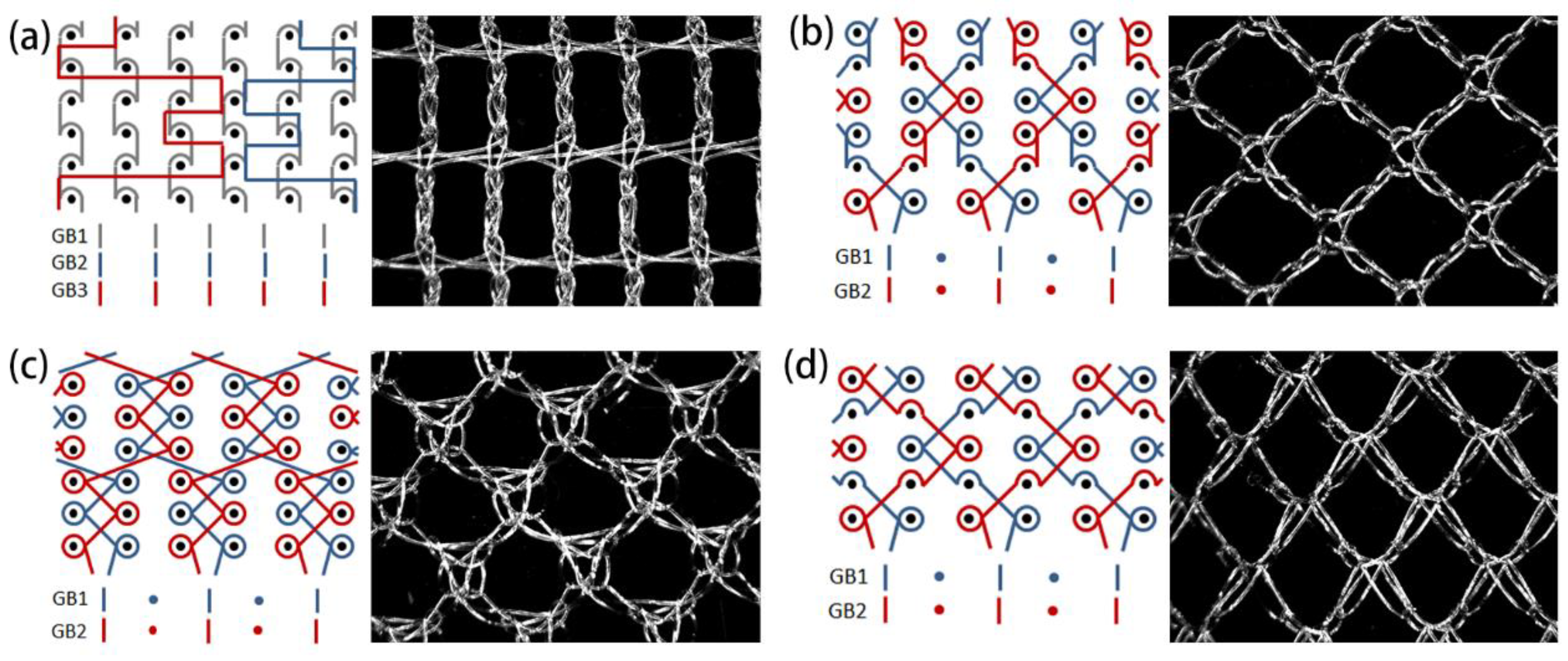


| Linear Density dtex | Modulus cN/dtex | Breaking Strength cN/dtex | Breaking Elongation % |
|---|---|---|---|
| 169 | 32.0 | 5.20 | 25.4 |
| Mesh | Draw off Density (Loops/cm) | Warp Run-in (mm/480 Courses) | Let-off Ratio | |
|---|---|---|---|---|
| GB1 | GB2 | |||
| Rectangular | 6.8 | 2620 | 920/1280 | 2.32 |
| Square | 6.8 | 2700 | 2700 | 3.89 |
| Circular | 6.8 | 2850 | 2860 | 4.06 |
| Dimond | 6.8 | 2840 | 2840 | 4.22 |
| Structures | Area Density g/m2 | Thickness mm | Porosity % |
|---|---|---|---|
| Rectangle | 32.2 | 0.693 | 72.1 |
| Square | 20.8 | 0.621 | 74.9 |
| Round | 27.6 | 0.528 | 67.8 |
| Diamond | 21.7 | 0.488 | 77.9 |
| Warp-knitted Mesh | Mono-Axial Stretch | Bi-Axial Stretch | ||
|---|---|---|---|---|
| ET/MPa | EL/MPa | ET/MPa | EL/MPa | |
| Rectangular | 13.317 | 0.654 | 16.845 | 8.385 |
| Square | 3.298 | 1.570 | 3.813 | 2.879 |
| Circular | 3.447 | 1.190 | 2.894 | 3.862 |
| Dimond | 3.894 | 1.400 | 5.344 | 4.812 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shao, H.; Li, J.; Chen, N.; Shao, G.; Jiang, J.; Yang, Y. Experimental Study on Bi-Axial Mechanical Properties of Warp-knitted Meshes with and without Initial Notches. Materials 2018, 11, 1999. https://doi.org/10.3390/ma11101999
Shao H, Li J, Chen N, Shao G, Jiang J, Yang Y. Experimental Study on Bi-Axial Mechanical Properties of Warp-knitted Meshes with and without Initial Notches. Materials. 2018; 11(10):1999. https://doi.org/10.3390/ma11101999
Chicago/Turabian StyleShao, Huiqi, Jianna Li, Nanliang Chen, Guangwei Shao, Jinhua Jiang, and Youhong Yang. 2018. "Experimental Study on Bi-Axial Mechanical Properties of Warp-knitted Meshes with and without Initial Notches" Materials 11, no. 10: 1999. https://doi.org/10.3390/ma11101999
APA StyleShao, H., Li, J., Chen, N., Shao, G., Jiang, J., & Yang, Y. (2018). Experimental Study on Bi-Axial Mechanical Properties of Warp-knitted Meshes with and without Initial Notches. Materials, 11(10), 1999. https://doi.org/10.3390/ma11101999





