2D Material Science: Defect Engineering by Particle Irradiation
Abstract
1. Introduction
2. Particle Beams Interacting with Solids
2.1. Ion Beams
2.2. Electron Beams
3. Defect Engineering by Particle Irradiation: State of the Art
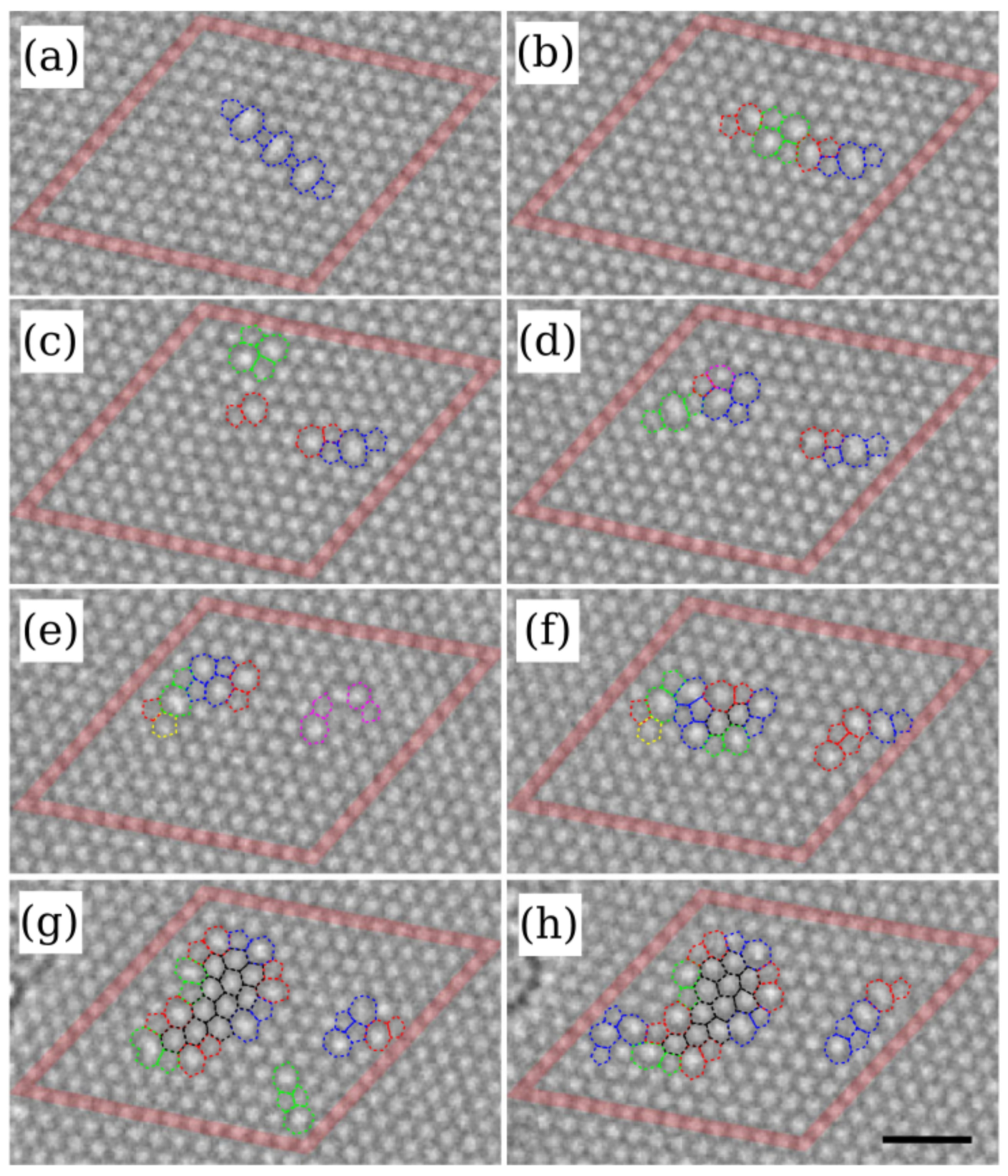
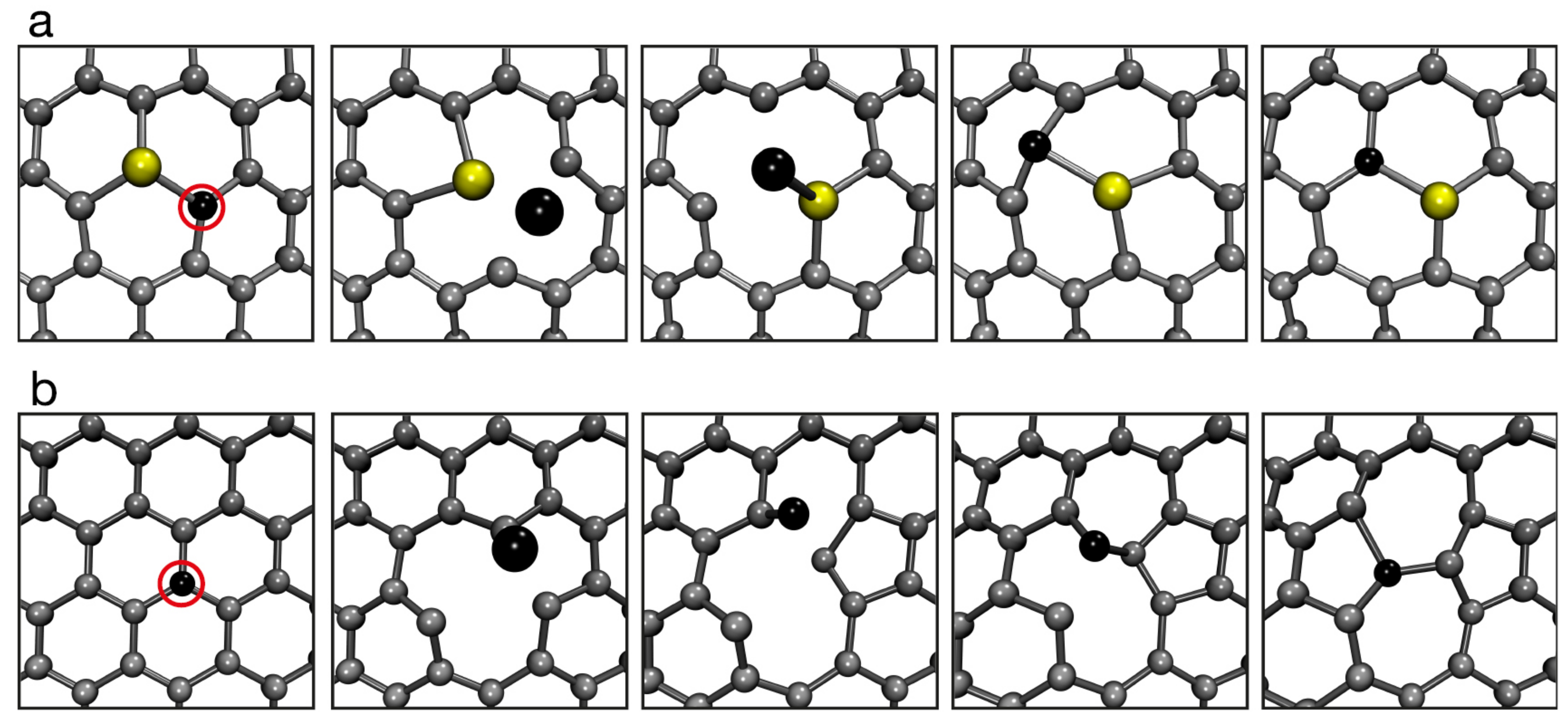
3.1. Electrons
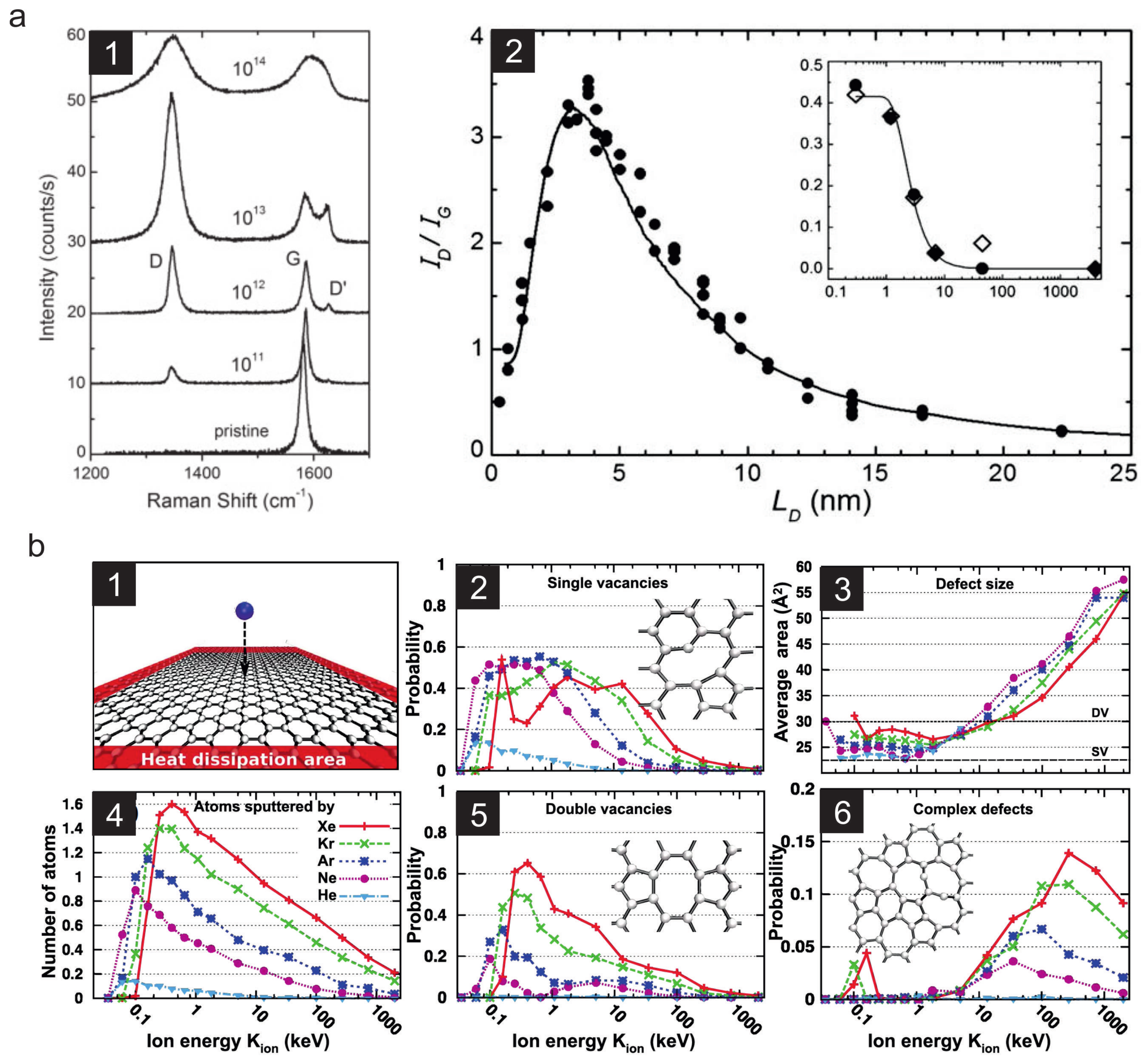
3.2. Low Energy Ions
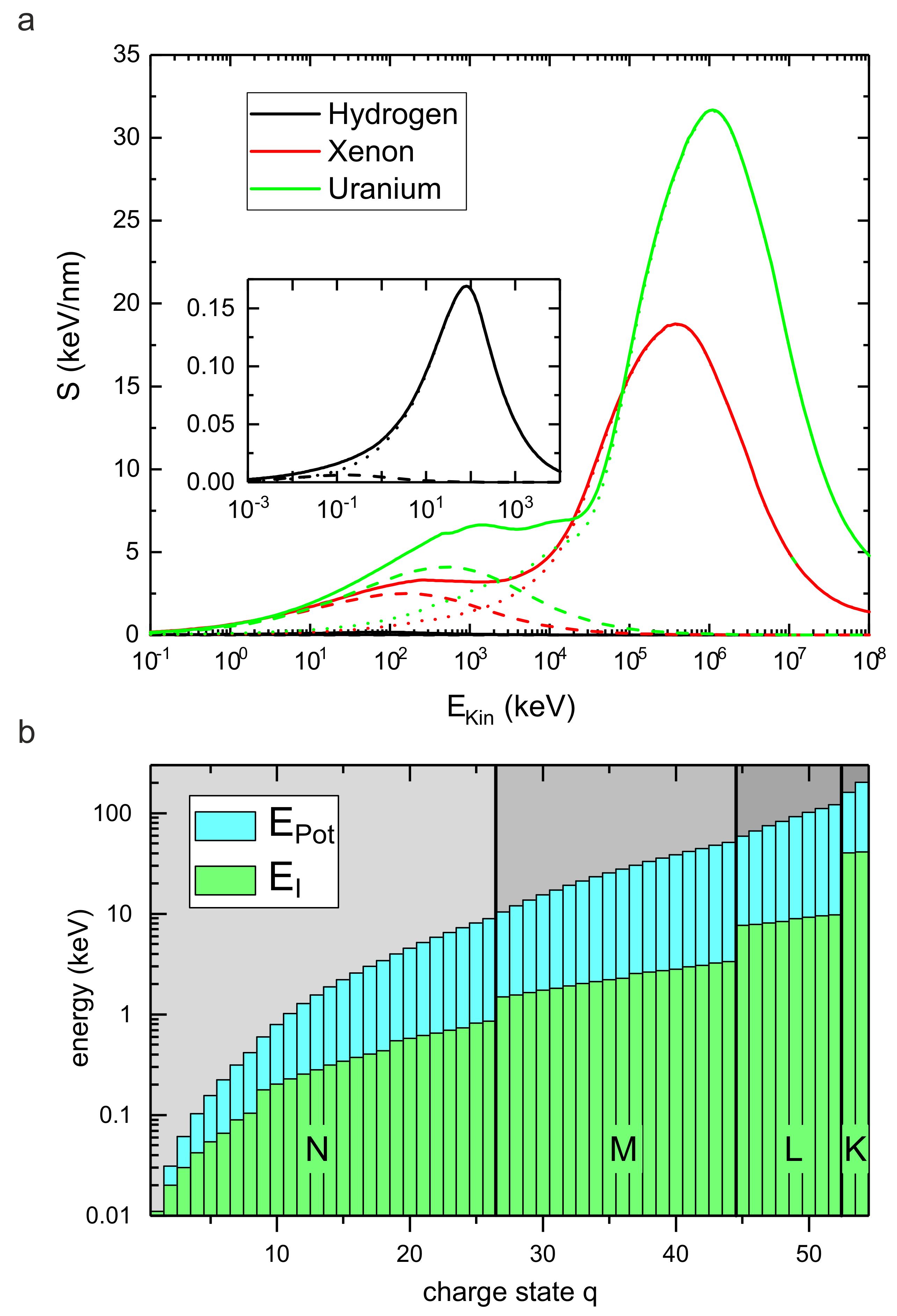
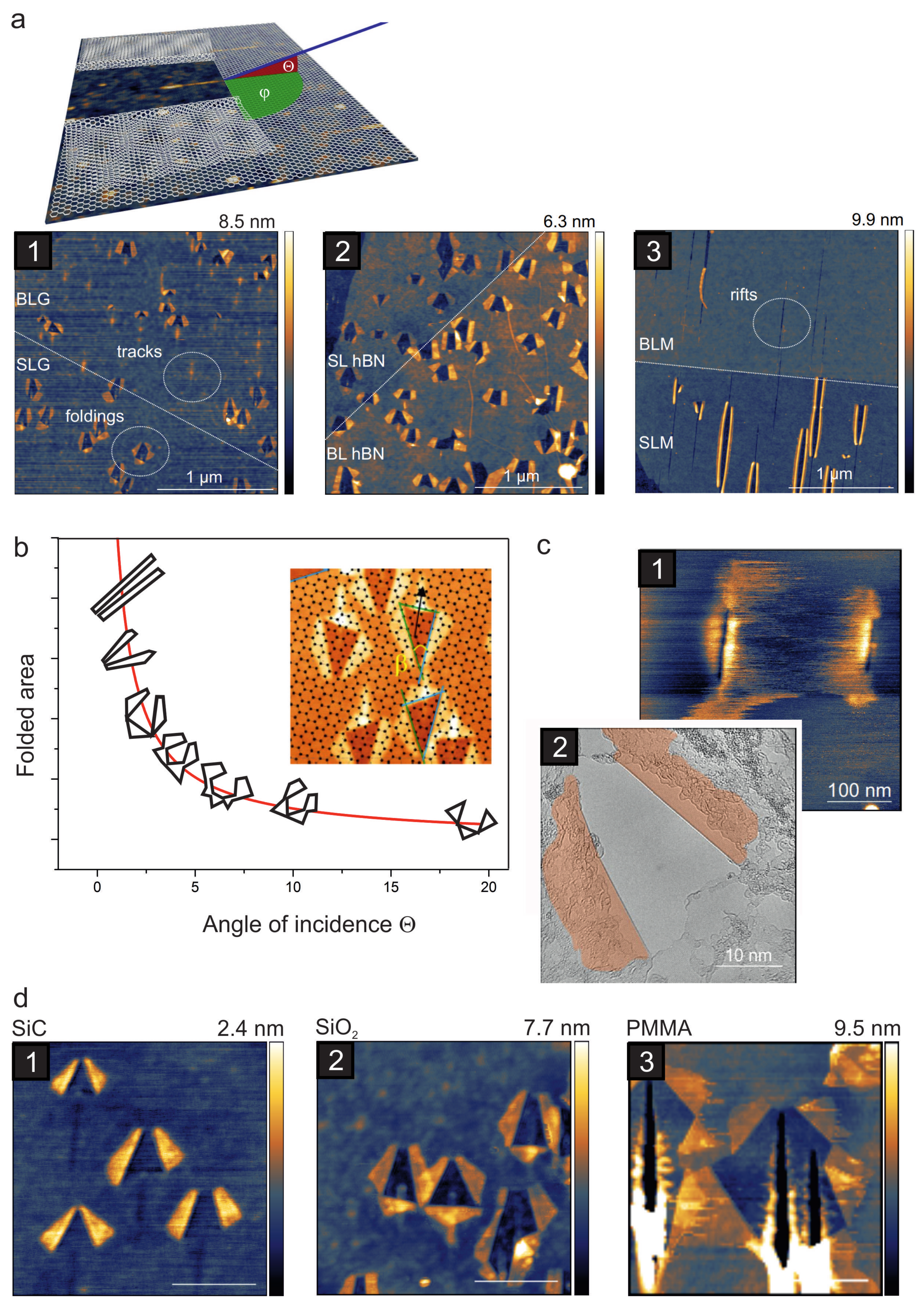
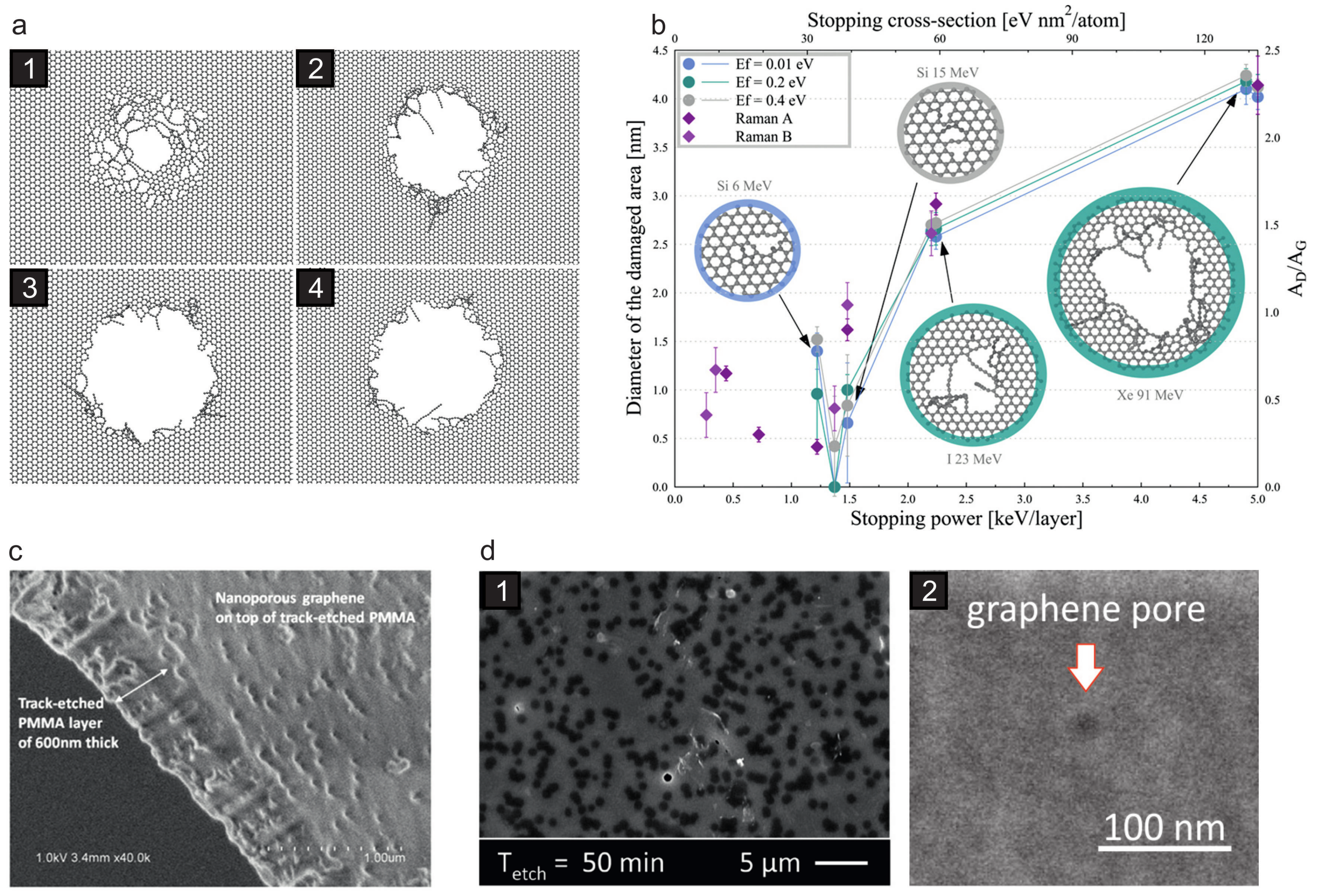
3.3. Swift Heavy Ions
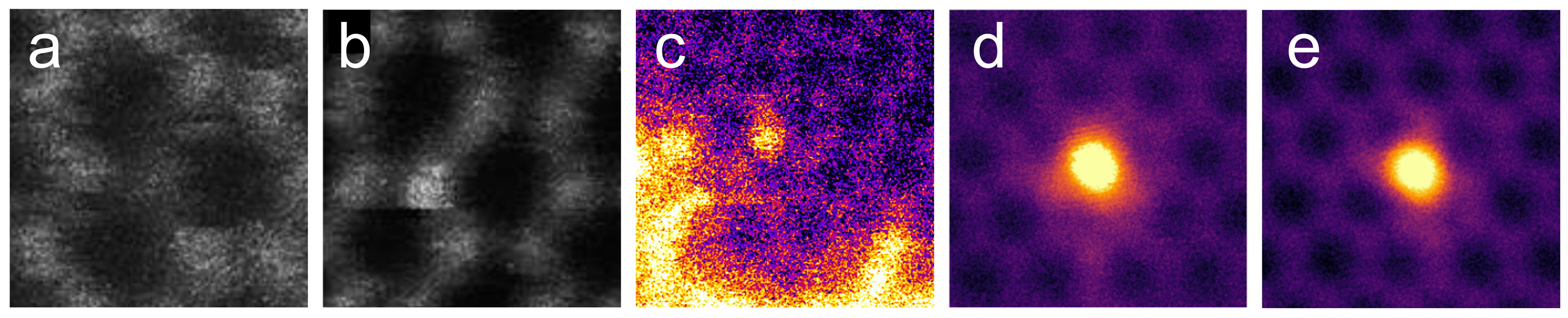
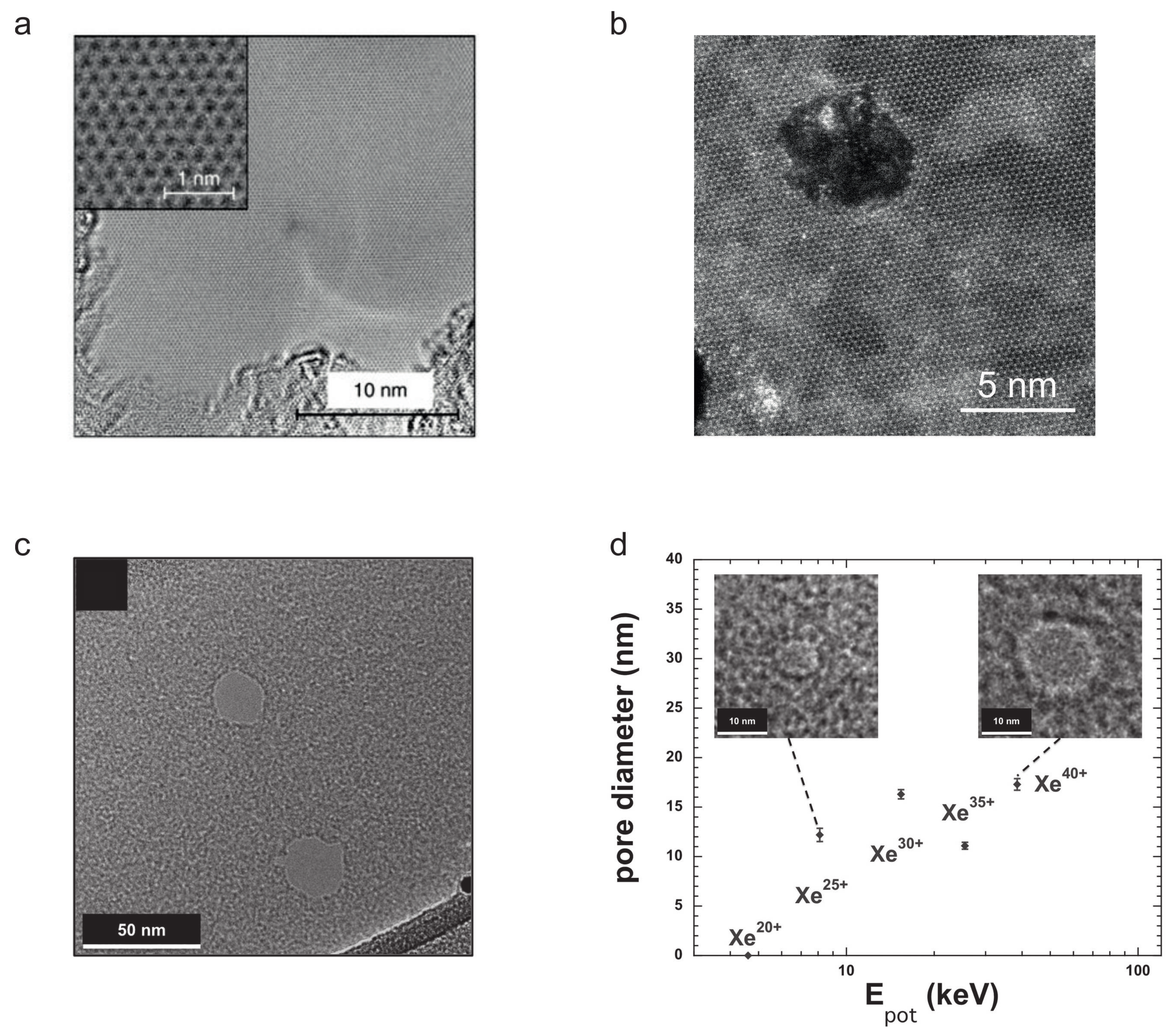
3.4. Highly Charged Ions
4. Open Problems
Author Contributions
Funding
Conflicts of Interest
References
- Fox, M.; Ispasoiu, R. Quantum Wells, Superlattices, and Band-Gap Engineering. In Springer Handbook of Electronic and Photonic Materials; Kasap, S., Capper, P., Eds.; Springer: New York, NY, USA, 2017. [Google Scholar]
- Ziegler, J. (Ed.) Implantation Science and Technology; Academic Press: Cambridge, MA, USA, 1988. [Google Scholar]
- Guo, B.; Fang, L.; Zhang, B.; Gong, J.R. Graphene Doping: A Review. Insci. J. 2011, 1, 80–89. [Google Scholar] [CrossRef]
- Agnoli, S.; Favaro, M. Doping graphene with boron: A review of synthesis methods, physicochemical characterization, and emerging applications. J. Mater. Chem. A 2016, 4, 5002. [Google Scholar] [CrossRef]
- Yadav, R.; Dixit, C. Synthesis, characterization and prospective applications of nitrogen-doped graphene: A short review. J. Sci. Adv. Mater. Devices 2017, 2, 141–149. [Google Scholar] [CrossRef]
- Geim, A.K.; Grigorieva, I.V. Van der Waals heterostructures. Nature 2013, 499, 419–425. [Google Scholar] [CrossRef] [PubMed]
- Novoselov, K.S.; Jiang, D.; Schedin, F.; Booth, T.J.; Khotkevich, V.V.; Morozov, S.V.; Geim, A.K. Two-dimensional atomic crystals. Proc. Natl. Acad. Sci. USA 2005, 102, 10451–10453. [Google Scholar] [CrossRef] [PubMed]
- Cohen-Tanugi, D.; Grossman, J. Water Desalination across Nanoporous Graphene. Nano Lett. 2012, 12, 3602–3608. [Google Scholar] [CrossRef] [PubMed]
- Dervin, S.; Dionysiou, D.D.; Pillai, S.C. 2D nanostructures for water purification: graphene and beyond. Nanoscale 2016, 8, 15115–15131. [Google Scholar] [CrossRef] [PubMed]
- Dhiman, P.; Yavari, F.; Mi, X.; Gullapalli, H.; Shi, Y.; Ajayan, P.M.; Koratkar, N. Harvesting Energy from Water Flow over Graphene. Nano Lett. 2011, 11, 3123–3127. [Google Scholar] [CrossRef] [PubMed]
- Lopez-Suarez, M.; Rurali, R.; Gammaitoni, L.; Abadal, G. Nanostructured graphene for energy harvesting. Phys. Rev. B 2012, 84, 161401. [Google Scholar] [CrossRef]
- Heerema, S.J.; Dekker, C. Graphene nanodevices for DNA sequencing. Nat. Nanotechnol. 2016, 11, 127–136. [Google Scholar] [CrossRef] [PubMed]
- Farimani, A.; Min, K.; Aluru, N. DNA base detection using a single-layer MoS2. ACS Nano 2014, 26, 7914–7922. [Google Scholar] [CrossRef] [PubMed]
- Feng, J.; Liu, K.; Bulushev, R.D.; Khlybov, S.; Dumcenco, D.; Kis, A.; Radenovic, A. Identification of single nucleotides in MoS2 nanopores. Nat. Nanotechnol. 2015, 10, 1070–1076. [Google Scholar] [CrossRef] [PubMed]
- Luan, B.; Zhou, R. Spontaneous Transport of Single-Stranded DNA through Graphene–MoS2 Heterostructure Nanopores. ACS Nano 2018, 12, 3886–3891. [Google Scholar] [CrossRef] [PubMed]
- Abanin, D.A.; Lee, P.A.; Levitov, L.S. Spin-Filtered Edge States and Quantum Hall Effect in Graphene. Phys. Rev. Lett. 2006, 96, 176803. [Google Scholar] [CrossRef] [PubMed]
- Li, G.; Zhang, D.; Qiao, Q.; Yu, Y.; Peterson, D.; Zafar, A.; Kumar, R.; Curtarolo, S.; Hunte, F.; Shannon, S.; et al. All the Catalytic Active Sites of MoS2 for Hydrogen Evolution. J. Am. Chem. Soc. 2016, 138, 16632–16638. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; Huang, S.; Ji, X.; Adepalli, K.; Yin, K.; Ling, X.; Wang, X.; Xue, J.; Dresselhaus, M.; Kong, J.; et al. Tuning Electronic Structure of Single Layer MoS2 through Defect and Interface Engineering. ACS Nano 2018, 12, 2569–2579. [Google Scholar] [CrossRef] [PubMed]
- Lin, Z.; McCreary, A.; Briggs, N.; Subramanian, S.; Zhang, K.; Sun, Y.; Li, X.; Borys, N.J.; Yuan, H.; Fullerton-Shirey, S.K. 2D materials advances: from large scale synthesis and controlled heterostructures to improved characterization techniques, defects and applications. 2D Mater. 2016, 3, 042001. [Google Scholar] [CrossRef]
- Lin, Z.; Carvalho, B.R.; Kahn, E.; Lv, R.; Rao, R.; Terrones, H.; Pimenta, M.A.; Terrones, M. Defect engineering of two-dimensional transition metal dichalcogenides. 2D Mater. 2016, 3, 022002. [Google Scholar] [CrossRef]
- Walker, R.; Shi, T.; Silva, E.; Jovanovic, I.; Robinson, J. Radiation effects on two-dimensional materials. Phys. Status Solidi 2016, 213, 3065–3077. [Google Scholar] [CrossRef]
- Li, Z.; Chen, F. Ion beam modification of two-dimensional materials: Characterization, properties, and applications. Appl. Phys. Rev. 2017, 4, 011103. [Google Scholar] [CrossRef]
- Balanzat, E.; Bouffard, S. Basic phenomena of the particle-matter interaction. Solid State Phenom. 1992, 30–31, 7–74. [Google Scholar] [CrossRef]
- Robinson, M.T.; Torrens, I.M. Computer simulation of atomic-displacement cascades in solids in the binary-collision approximation. Phys. Rev. B 1974, 9, 5008. [Google Scholar] [CrossRef]
- Spohr, R. Ion Tracks and Microtechnology: Principles and Applications; Springer: Berlin, Germany, 1990. [Google Scholar]
- Solovyov, E.A. (Ed.) Nanoscale Insights Into Ion-Beam Cancer Therapy; Springer Publishing: Basel, Switzerland, 2016. [Google Scholar]
- Bohr, N. On the Theory of the Decrease of Velocity of Moving Electrified Particles on Passing Through Matter. Philos. Mag. 1913, 25, 10–31. [Google Scholar] [CrossRef]
- Bethe, H. Zur Theorie des Durchgangs schneller Korpuskularstrahlen durch Materie. Ann. Phys. 1930, 397, 325–400. [Google Scholar] [CrossRef]
- Bloch, F. Zur Bremsung rasch bewegter Teilchen beim Durchgang durch Materie. Ann. Phys. 1933, 408, 285–320. [Google Scholar] [CrossRef]
- Lindhard, J.; Scharff, M.; Schiøtt, H.E. Range concepts and heavy ion ranges (Notes on atomic collisions, II). Medd. Dan. Vid. Selsk. 1963, 33, 1. [Google Scholar]
- Lifshitz, I.; Kaganov, M.; Tanatarov, L. On the theory of radiation-induced changes in metals. J. Nucl. Energy 1960, 12, 69–78. [Google Scholar] [CrossRef]
- Toulemonde, M.; Dufour, C.; Paumier, E. Transient thermal process after a high-energy heavy-ion irradiation of amorphous metals and semiconductors. Phys. Rev. B 1992, 46, 14362. [Google Scholar] [CrossRef]
- Osmani, O.; Medvedev, N.; Schleberger, M.; Rethfeld, B. Energy dissipation in dielectrics after swift heavy ion impact: A hybrid model. Phys. Rev. B 2011, 84, 214105. [Google Scholar] [CrossRef]
- Tombrello, P. Predicting latent track dimensions. Nucl. Instrum. Methods Phys. Res. Sect. B 1994, 94, 424–428. [Google Scholar] [CrossRef]
- Fleischer, R.L.; Price, P.; Walker, R. Ion Explosion Spike Mechanism for Formation of Charged Particle Tracks In Solids. J. Appl. Phys. 1965, 36, 3645–3652. [Google Scholar] [CrossRef]
- Itoh, N. Self-trapped exciton model of heavy-ion track registration. Nucl. Instrum. Methods Phys. Res. Sect. B 1996, 116, 33–36. [Google Scholar] [CrossRef]
- Ziegler, J.; Ziegler, M.; Biersack, J. SRIM–The stopping and range of ions in matter. Nucl. Instrum. Methods Phys. Res. Sect. B 2010, 268, 1818–1823. [Google Scholar] [CrossRef]
- Levine, M.; Marrs, R.; Henderson, J.; Knapp, D.; Schneider, M.B. The Electron Beam Ion Trap: A New Instrument for Atomic Physics Measurements. Phys. Scr. 1988, T22, 157. [Google Scholar] [CrossRef]
- Arnau, A.; Aumayr, F.; Echenique, P.M.; Grether, M.; Heiland, W.; Limburg, J.; Morgenstern, R.; Roncin, P.; Schippers, S.; Schuch, R.; et al. Interaction of slow multicharged ions with solid surfaces. Surf. Sci. Rep. 1997, 27, 113–239. [Google Scholar] [CrossRef]
- Burgdörfer, J.; Lerne, P.; Meyer, F.W. Above-surface neutralization of highly charged ions: The classical over-the-barrier model. Phys. Rev. A 1991, 9, 5674. [Google Scholar] [CrossRef]
- Gruber, E.; Wilhelm, R.; Petuya, R.; Smejkal, V.; Kozubek, R.; Hierzenberger, A.; Bayer, B.; Aldazabal, I.; Kazansky, A.; Libisch, F.; et al. Ultrafast electronic response of graphene to a strong and localized electric field. Nat. Commun. 2016, 7, 13948. [Google Scholar] [CrossRef] [PubMed]
- Wilhelm, R.A.; Gruber, E.; Schwestka, J.; Kozubek, R.; Madeira, T.I.; Marques, J.P.; Kobus, J.; Krasheninnikov, A.V.; Schleberger, M.; Aumayr, F. Interatomic Coulombic Decay: The Mechanism for Rapid Deexcitation of Hollow Atoms. Phys. Rev. Lett. 2017, 119, 103401. [Google Scholar] [CrossRef] [PubMed]
- Aumayr, F.; Facsko, S.; El-Said, A.; Trautmann, C.; Schleberger, M. Single ion induced surface nanostructures: A comparison between slow highly charged and swift heavy ions. J. Phys. Condens. Matter 2011, 23, 393001. [Google Scholar] [CrossRef] [PubMed]
- El-Said, A.; Heller, R.; Meissl, W.; Ritter, R.; Facsko, S.; Lemell, C.; Solleder, B.; Gebeshuber, I.; Betz, G.; Toulemonde, M.; et al. Creation of Nanohillocks on CaF2 Surfaces by Single Slow Highly Charged Ions. Phys. Rev. Lett. 2008, 23, 393001. [Google Scholar] [CrossRef]
- Egerton, R. Mechanisms of radiation damage in beam-sensitive specimens, for TEM accelerating voltages between 10 and 300 kV. Microsc. Res. Tech. 2012, 75, 1550–1556. [Google Scholar] [CrossRef] [PubMed]
- McKinley, W.A.; Feshbach, H. The Coulomb Scattering of Relativistic Electrons by Nuclei. Phys. Rev. 1948, 74, 1759–1763. [Google Scholar] [CrossRef]
- Banhart, F. Irradiation effects in carbon nanostructures. Rep. Prog. Phys. 1999, 62, 1181. [Google Scholar] [CrossRef]
- Iwata, T.; Nihira, T. Atomic Displacements by Electron Irradiation in Pyrolytic Graphite. J. Phys. Soc. Jpn. 1971, 31, 1761–1783. [Google Scholar] [CrossRef]
- Meyer, J.C.; Eder, F.; Kurasch, S.; Skakalova, V.; Kotakoski, J.; Park, H.J.; Chuvilin, A.; Benner, G.; Krasheninnikov, A.V.; Kaiser, U.; et al. Accurate Measurement of Electron Beam Induced Displacement Cross Sections for Single-Layer Graphene. Phys. Rev. Lett. 2012, 108, 196102. [Google Scholar] [CrossRef] [PubMed]
- Meyer, J.C.; Eder, F.; Kurasch, S.; Skakalova, V.; Kotakoski, J.; Park, H.J.; Roth, S.; Chuvilin, A.; Eyhusen, S.; Benner, G.; et al. Erratum: Accurate Measurement of Electron Beam Induced Displacement Cross Sections for Single-Layer Graphene [Phys. Rev. Lett. 108, 196102 (2012)]. Phys. Rev. Lett. 2013, 110, 239902. [Google Scholar] [CrossRef]
- Susi, T.; Hofer, C.; Argentero, G.; Leuthner, G.T.; Pennycook, T.J.; Mangler, C.; Meyer, J.C.; Kotakoski, J. Isotope analysis in the transmission electron microscope. Nat. Commun. 2016, 7, 13040. [Google Scholar] [CrossRef] [PubMed]
- Kotakoski, J.; Jin, C.; Lehtinen, O.; Suenaga, K.; Krasheninnikov, A. Electron knock-on damage in hexagonal boron nitride monolayers. Phys. Rev. B 2010, 82, 113404. [Google Scholar] [CrossRef]
- Komsa, H.P.; Kotakoski, J.; Kurasch, S.; Lehtinen, O.; Kaiser, U.; Krasheninnikov, A.V. Two-dimensional transition metal dichalcogenides under electron irradiation: Defect production and doping. Phys. Rev. Lett. 2012, 109, 035503. [Google Scholar] [CrossRef] [PubMed]
- Algara-Siller, G.; Kurasch, S.; Sedighi, M.; Lehtinen, O.; Kaiser, U. The pristine atomic structure of MoS2 monolayer protected from electron radiation damage by graphene. Appl. Phys. Lett. 2013, 103, 203107. [Google Scholar] [CrossRef]
- Kotakoski, J.; Krasheninnikov, A.V.; Kaiser, U.; Meyer, J.C. From Point Defects in Graphene to Two-Dimensional Amorphous Carbon. Phys. Rev. Lett. 2011, 106, 105505. [Google Scholar] [CrossRef] [PubMed]
- Bachmatiuk, A.; Zhao, J.; Gorantla, S.M.; Martinez, I.G.G.; Wiedermann, J.; Lee, C.; Eckert, J.; Rummeli, M.H. Low Voltage Transmission Electron Microscopy of Graphene. Small 2015, 11, 515–542. [Google Scholar] [CrossRef] [PubMed]
- Kotakoski, J.; Meyer, J.; Kurasch, S.; Santos-Cottin, D.; Kaiser, U.; Krasheninnikov, A. Stone-Wales-type transformations in carbon nanostructures driven by electron irradiation. Phys. Rev. B 2011, 83, 245420. [Google Scholar] [CrossRef]
- Kurasch, S.; Kotakoski, J.; Lehtinen, O.J.; Skakalova, V.; Smet, J.H.; Krill, C.; Krasheninnikov, A.V.; Kaiser, U. Atom-by-Atom Observation of Grain Boundary Migration in Graphene. Nano Lett. 2012, 12, 3168–3173. [Google Scholar] [CrossRef] [PubMed]
- Kotakoski, J.; Mangler, C.; Meyer, J.C. Imaging atomic-level random walk of a point defect in graphene. Nat. Commun. 2014, 5, 4991. [Google Scholar] [CrossRef] [PubMed]
- Susi, T.; Kotakoski, J.; Kepaptsoglou, D.; Mangler, C.; Lovejoy, T.C.; Krivanek, O.L.; Zan, R.; Bangert, U.; Ayala, P.; Meyer, J.C.; et al. Silicon-Carbon Bond Inversions Driven by 60-keV Electrons in Graphene. Phys. Rev. Lett. 2014, 113, 115501. [Google Scholar] [CrossRef] [PubMed]
- Susi, T.; Meyer, J.C.; Kotakoski, J. Manipulating low-dimensional materials down to the level of single atoms with electron irradiation. Ultramicroscopy 2017, 180, 163–172. [Google Scholar] [CrossRef] [PubMed]
- Dyck, O.; Kim, S.; Kalinin, S.V.; Jesse, S. Placing single atoms in graphene with a scanning transmission electron microscope. Appl. Phys. Lett. 2017, 111, 113104. [Google Scholar] [CrossRef]
- Susi, T.; Kepaptsoglou, D.; Lin, Y.C.; Ramasse, Q.M.; Meyer, J.C.; Suenaga, K.; Kotakoski, J. Towards atomically precise manipulation of 2D nanostructures in the electron microscope. 2D Mater. 2017, 4, 042004. [Google Scholar] [CrossRef]
- Susi, T.; Tripathi, M.; Meyer, J.C.; Kotakoski, J. Electron-beam manipulation of Si dopants in graphene. arXiv 2017, arXiv:1712.08755. [Google Scholar]
- Lin, Y.C.; Björkman, T.; Komsa, H.P.; Teng, P.Y.; Yeh, C.H.; Huang, F.S.; Lin, K.H.; Jadczak, J.; Huang, Y.S.; Chiu, P.W.; et al. Three-fold rotational defects in two-dimensional transition metal dichalcogenides. Nat. Commun. 2015, 6, 6736. [Google Scholar] [CrossRef] [PubMed]
- Meyer, J.C.; Chuvilin, A.; Algara-Siller, G.; Biskupek, J.; Kaiser, U. Selective sputtering and atomic resolution imaging of atomically thin boron nitride membranes. Nano Lett. 2009, 9, 2683–2689. [Google Scholar] [CrossRef] [PubMed]
- Jin, C.; Lin, F.; Suenaga, K.; Iijima, S. Fabrication of a Freestanding Boron Nitride Single Layer and Its Defect Assignments. Phys. Rev. Lett. 2009, 102, 195505. [Google Scholar] [CrossRef] [PubMed]
- Komsa, H.P.; Kurasch, S.; Lehtinen, O.; Kaiser, U.; Krasheninnikov, A.V. From point to extended defects in two-dimensional MoS2: Evolution of atomic structure under electron irradiation. Phys. Rev. B 2013, 88, 035301. [Google Scholar] [CrossRef]
- Lin, Y.C.; Dumcenco, D.O.; Huang, Y.S.; Suenaga, K. Atomic mechanism of the semiconducting-to-metallic phase transition in single-layered MoS2. Nat. Nanotechnol. 2014, 9, 391–396. [Google Scholar] [CrossRef] [PubMed]
- Elibol, K.; Susi, T.; Argentero, G.; Reza Ahmadpour Monazam, M.; Pennycook, T.J.; Meyer, J.C.; Kotakoski, J. Atomic structure of intrinsic and electron-irradiation-induced defects in MoTe2. Chem. Mater. 2018, 30, 1230–1238. [Google Scholar] [CrossRef] [PubMed]
- Lucchese, M.; Stavale, F.; Ferreira, E.M.; Vilani, C.; Moutinho, M.; Capaz, R.B.; Achete, C.; Jorio, A. Quantifying ion-induced defects and Raman relaxation length in graphene. Carbon 2010, 48, 1592–1597. [Google Scholar] [CrossRef]
- Lehtinen, O.; Kotakoski, J.; Krasheninnikov, A.V.; Tolvanen, A.; Nordlund, K.; Keinonen, J. Effects of ion bombardment on a two-dimensional target: Atomistic simulations of graphene irradiation. Phys. Rev. B 2010, 81, 153401. [Google Scholar] [CrossRef]
- Tuinstra, F.; Koenig, J. Raman spectrum of graphite. Chem. Phys. 1970, 53, 1126–1130. [Google Scholar] [CrossRef]
- Eckmann, A.; Felten, A.; Mishchenko, A.; Britnell, L.; Krupke, R.; Novoselov, K.S.; Casiraghi, C. Probing the Nature of Defects in Graphene by Raman Spectroscopy. Nano Lett. 2012, 12, 3925–3930. [Google Scholar] [CrossRef] [PubMed]
- Lehtinen, O.; Kotakoski, J.; Krasheninnikov, A.V.; Keinonen, J. Cutting and controlled modification of graphene with ion beams. Nanotechnology 2011, 22, 175306. [Google Scholar] [CrossRef] [PubMed]
- Li, W.; Liang, L.; Zhao, S.; Zhang, S.; Xue, J. Fabrication of nanopores in a graphene sheet with heavy ions: A molecular dynamics study. J. Appl. Phys. 2013, 114, 234304. [Google Scholar] [CrossRef]
- Wu, X.; Pei, Z.J. Fabrication of nanopore in graphene by electron and ion beam irradiation: Influence of graphene thickness and substrate. Comput. Mater. Sci. 2015, 102, 258–266. [Google Scholar] [CrossRef]
- Bell, D.; Lemme, M.; Stern, L.; Williams, J.; Marcus, C. Precision cutting and patterning of graphene with helium ions. Nanotechnology 2009, 20, 455301. [Google Scholar] [CrossRef] [PubMed]
- Fox, D.; Zhou, Y.; O’Neill, A.; Kumar, S.; Wang, J.; Coleman, J.; Duesberg, G.; Donegan, J.; Zhang, H. Helium ion microscopy of graphene: beam damage, image quality and edge contrast. Nanotechnology 2013, 24, 335702. [Google Scholar] [CrossRef] [PubMed]
- Archanjo, B.; Barboza, A.P.; Neves, B.R.; Malard, L.M.; Ferreira, E.H.; Brant, J.C.; Alves, E.S.; Plentz, F.; Carozo, V.; Fragneaud, B.; et al. The use of a Ga+ focused ion beam to modify graphene for device applications. Nanotechnology 2013, 23, 255305. [Google Scholar] [CrossRef] [PubMed]
- Li, H.; Daukiya, L.; Haldar, S.; Lindblad, A.; Sanyal, B.; Eriksson, O.; Aubel, D.; Hajjar-Garreau, S.; Simon, L.; Leifer, K. Site-selective local fluorination of graphene induced by focused ion beam irradiation. Sci. Rep. 2016, 6, 19719. [Google Scholar] [CrossRef] [PubMed]
- Wang, D.; Wang, Y.; Chen, X.; Zhu, Y.; Zhan, K.; Cheng, H.; Wang, X. Layer-by-layer thinning of two-dimensional MoS2 films by using a focused ion beam. Nanoscale 2016, 8, 4107–4112. [Google Scholar] [CrossRef] [PubMed]
- Thissen, N.; Vervuurt, R.; Mulders, J.; Weber, J.; Kessels, W.; Bol, A. The effect of residual gas scattering on Ga ion beam patterning of graphene. Appl. Phys. Lett. 2015, 107, 213101. [Google Scholar] [CrossRef]
- Kotakoski, J.; Brand, C.; Lilach, Y.; Cheshnovsky, O.; Mangler, C.; Arndt, M.; Meyer, J.C. Toward two-dimensional all-carbon heterostructures via ion beam patterning of single-layer graphene. Nano Lett. 2015, 15, 5944–5949. [Google Scholar] [CrossRef] [PubMed]
- Yoon, K.; Rahnamoun, A.; Swett, J.L.; Iberi, V.; Cullen, D.A.; Vlassiouk, I.V.; Belianinov, A.; Jesse, S.; Sang, X.; Ovchinnikova, O.S.; et al. Atomistic-Scale Simulations of Defect Formation in Graphene under Noble Gas Ion Irradiation. ACS Nano 2016, 10, 8376–8384. [Google Scholar] [CrossRef] [PubMed]
- Åhlgren, E.; Kotakoski, J.; Krasheninnikov, A. Atomistic simulations of the implantation of low-energy boron and nitrogen ions into graphene. Phys. Rev. B 2011, 83, 115424. [Google Scholar] [CrossRef]
- Bangert, U.; Pierce, W.; Kepaptsoglou, D.M.D.; Ramasse, Q.M.; Zan, R.; Gass, M.; Van Den Berg, J.A.; Boothroyd, C.; Amani, J.; Hofsäss, H.C. Ion implantation of graphene-towards IC compatible technologies. Nano Lett. 2013, 13, 4902–4907. [Google Scholar] [CrossRef] [PubMed]
- Zhou, W.; Kapetanakis, M.; Prange, M.; Pantelides, S.; Pennycook, S.; Idrobo, J.C. Direct Determination of the Chemical Bonding of Individual Impurities in Graphene. Phys. Rev. Lett. 2012, 109, 206803. [Google Scholar] [CrossRef] [PubMed]
- Ramasse, Q.M.; Seabourne, C.R.; Kepaptsoglou, D.M.; Zan, R.; Bangert, U.; Scott, A.J. Probing the Bonding and Electronic Structure of Single Atom Dopants in Graphene with Electron Energy Loss Spectroscopy. Nano Lett. 2013, 13, 4989–4995. [Google Scholar] [CrossRef] [PubMed]
- Nieman, R.; Aquino, A.J.A.; Hardcastle, T.P.; Kotakoski, J.; Susi, T.; Lischka, H. Structure and Electronic States of a Graphene Double Vacancy with an Embedded Si Dopant. J. Chem. Phys. 2017, 147, 194702. [Google Scholar] [CrossRef] [PubMed]
- Li, W.; Xue, J. Ion implantation of low energy Si into graphene: Insight from computational studies. RSC Adv. 2015, 5, 99920–99926. [Google Scholar] [CrossRef]
- Susi, T.; Hardcastle, T.P.; Hofsäss, H.; Mittelberger, A.; Pennycook, T.J.; Mangler, C.; Drummond-Brydson, R.; Scott, A.J.; Meyer, J.C.; Kotakoski, J. Single-atom spectroscopy of phosphorus dopants implanted into graphene. 2D Mater. 2017, 4, 021013. [Google Scholar] [CrossRef]
- Tripathi, M.; Markevich, A.V.; Boettger, R.; Facsko, S.; Besley, E.; Kotakoski, J.; Susi, T. Implanting Germanium into Graphene. ACS Nano 2018, 12, 4641–4647. [Google Scholar] [CrossRef] [PubMed]
- Bangert, U.; Stewart, A.; O’Connell, E.; Courtney, E.; Ramasse, Q.; Kepatsoglou, D.; Hofsäss, H.; Amani, J.; Tu, S.S.; Kardynal, B. Ion-beam modification of 2-D Materials-single implant atom analysis via annular dark-field electron microscopy. Ultramicroscopy 2017, 176, 31–36. [Google Scholar] [CrossRef] [PubMed]
- Ghorbani-Asl, M.; Kretschmer, S.; Spearot, D.E.; Krasheninnikov, A.V. Two-dimensional MoS2 under ion irradiation: from controlled defect production to electronic structure engineering. 2D Mater. 2017, 4, 025078. [Google Scholar] [CrossRef]
- Ma, L.; Tan, Y.; Ghorbani-Asl, M.; Boettger, R.; Kretschmer, S.; Zhou, S.; Huang, Z.; Krasheninnikov, A.V.; Chen, F. Tailoring the optical properties of atomically-thin WS2 via ion irradiation. Nanoscale 2017, 9, 11027–11034. [Google Scholar] [CrossRef] [PubMed]
- Blankenburg, S.; Bieri, M.; Fasel, R.; Müllen, K.; Pignedoli, C.A.; Passerone, D. Porous graphene as an atmospheric nanofilter. Small 2010, 18, 2266–2271. [Google Scholar] [CrossRef] [PubMed]
- Hauser, A.W.; Schwerdtfeger, P. Two-dimensional MoS2 under ion irradiation: From controlled defect production to electronic structure engineering. Phys. Chem. Chem. Phys. 2012, 14, 13292–13298. [Google Scholar] [CrossRef] [PubMed]
- Schrier, J.; McClain, J. Thermally-driven isotope separation across nanoporous graphene. Chem. Phys. Lett. 2010, 521, 118–124. [Google Scholar] [CrossRef]
- Lozada-Hidalgo, M.; Zhang, S.; Hu, S.; Esfandiar, A.; Grigorieva, I.V.; Geim, A.K. Scalable and efficient separation of hydrogen isotopes using graphene-based electrochemical pumping. Nat. Commun. 2017, 8, 15215. [Google Scholar] [CrossRef] [PubMed]
- Postma, H.W. Rapid Sequencing of Individual DNA Molecules in Graphene Nanogaps. Chem. Phys. Lett. 2010, 10, 420–425. [Google Scholar] [CrossRef] [PubMed]
- Celebi, K.; Buchheim, J.; Wyss, R.M.; Droudian, A.; Gasser, P.; Shorubalko, I.; Kye, J.I.; Lee, C.; Park, H.G. Ultimate Permeation Across Atomically Thin Porous Graphene. Science 2014, 344, 289–292. [Google Scholar] [CrossRef] [PubMed]
- O’Hern, S.C.; Boutilier, M.S.; Idrobo, J.C.; Song, Y.; Kong, J.; Laoui, T.; Atieh, M.; Karnik, R. Selective Ionic Transport through Tunable Subnanometer Pores in Single-Layer Graphene Membranes. Nano Lett. 2014, 14, 1234. [Google Scholar] [CrossRef] [PubMed]
- Surwade, S.P.; Smirnov, S.N.; Vlassiouk, I.V.; Unocic, R.R.; Veith, G.M.; Dai, S.; Mahurin, S.M. Water desalination using nanoporous single-layer graphene. Nat. Nanotechnol. 2015, 10, 459–464. [Google Scholar] [CrossRef] [PubMed]
- Huang, L.; Zhang, M.; Li, C.; Shi, G. Graphene-Based Membranes for Molecular Separation. J. Phys. Chem. Lett. 2015, 6, 2806–2815. [Google Scholar] [CrossRef] [PubMed]
- Wu, X.; Zhao, H.; Murakawa, H. The Joining of Graphene Sheets Under Ar Ion Beam Irradiation. J. Nanosci. Nanotechnol. 2014, 14, 5697–5702. [Google Scholar] [CrossRef] [PubMed]
- Ye, J.; Charnvanichborikarn, S.; Worsley, M.; Kucheyev, S.; Wood, B.; Wang, Y. Enhanced electrochemical performance of ion-beam-treated 3D graphene aerogels for lithium ion batteries. Carbon 2014, 85, 269–278. [Google Scholar] [CrossRef]
- Compagnini, G.; Giannazzo, F.; Sonde, S.; Raineri, V.; Rimini, E. Ion irradiation and defect formation in single layer graphene. Carbon 2009, 47, 3201–3207. [Google Scholar] [CrossRef]
- Fischer, B. The heavy-ion microprobe at GSI–Used for single ion micromechanics. Nucl. Instrum. Methods Phys. Res. Sect. B 1988, 30, 284–288. [Google Scholar] [CrossRef]
- Smith, R.; Karlusic, M.; Jaksic, M. Single ion hit detection set-up for the Zagreb ion microprobe. Nucl. Instrum. Methods Phys. Res. Sect. B 2012, 277, 140–144. [Google Scholar] [CrossRef]
- Akcöltekin, S.; Bukowska, H.; Peters, T.; Osmani, O.; Monnet, I.; Alzaher, I.; d’Etat, B.B.; Lebius, H.; Schleberger, M. Unzipping and folding graphene with swift heavy ions. Appl. Phys. Lett. 2011, 98, 103103. [Google Scholar] [CrossRef]
- Ochedowski, O.; Lehtinen, O.; Kaiser, U.; Turchanin, A.; Ban-d’Etat, B.; Lebius, H.; Karlusic, M.; Jaksic, M.; Schleberger, M. Nanostructuring graphene by dense electronic excitation. Nanotechnology 2015, 26, 465203. [Google Scholar] [CrossRef] [PubMed]
- Ochedowski, O. Modification of 2D-Materials by Swift Heavy Ion Irradiation. Ph.D. Thesis, Fakultät für Physik, Universität Duisburg-Essen, Duisburg, Germany, 2014. [Google Scholar]
- Akcöltekin, S. Ioneninduzierte Modifikation von Graphenschichten. Ph.D. Thesis, Fakultät für Physik, Universität Duisburg-Essen, Duisburg, Germany, 2014. [Google Scholar]
- Ochedowski, O.; Begall, G.; Scheuschner, N.; Kharrazi, M.E.; Maultzsch, J.; Schleberger, M. Graphene on Si(111)7x7. Nanotechnology 2012, 23, 405708. [Google Scholar] [CrossRef] [PubMed]
- Ochedowski, O.; Bukowska, H.; Soler, V.F.; Bröckers, L.; Ban-d’Etat, B.; Lebius, H.; Schleberger, M. Folding two dimensional crystals by swift heavy ion irradiation. Nucl. Instrum. Methods Phys. Res. Sect. B 2014, 340, 39–43. [Google Scholar] [CrossRef]
- Zhao, S.; Xue, J. Modification of graphene supported on SiO2 substrate with swift heavy ions from atomistic simulation point. Carbon 2015, 93, 169–179. [Google Scholar] [CrossRef]
- Jiang, J.W. The buckling of single-layer MoS2 under uniaxial compression. Nanotechnology 2012, 25, 355402. [Google Scholar] [CrossRef] [PubMed]
- Madauß, L.; Ochedowski, O.; Lebius, H.; Ban-d’Etat, B.; Naylor, C.; Johnson, A.; Kotakoski, J.; Schleberger, M. Defect engineering of single- and few-layer MoS2 by swift heavy ion irradiation. 2D Mater. 2017, 4, 015034. [Google Scholar] [CrossRef]
- Ochedowski, O.; Akcöltekin, S.; Ban-d’Etat, B.; Lebius, H.; Schleberger, M. Detecting swift heavy ion irradiation effects with graphene. Nucl. Instrum. Methods Phys. Res. Sect. B 2013, 314, 18–20. [Google Scholar] [CrossRef]
- Zan, R.; Ramasse, Q.; Bangert, U.; Novoselov, K. Graphene Reknits Its Holes. Nano Lett. 2012, 12, 3936–3940. [Google Scholar] [CrossRef] [PubMed]
- Zhao, S.; Xue, J.; Wang, Y.; Yan, S. Effect of SiO2 substrate on the irradiation-assisted manipulation of supported graphene: A molecular dynamics study. Nanotechnology 2012, 23, 285703. [Google Scholar] [CrossRef] [PubMed]
- Leino, A.; Daraszewicz, S.; Pakarinen, O.; Nordlund, K.; Djurabekova, F. Atomistic two-temperature modelling of ion track formation in silicon dioxide. Europhys. Lett. 2015, 110, 16004. [Google Scholar] [CrossRef]
- Muinos, H.V.; Åhlgren, E.; Ochedowski, O.; Leino, A.; Mirzayev, R.; Kozubek, R.; Lebius, H.; Karlusic, M.; Jaksic, M.; Krasheninnikov, A.; et al. Creating nanoporous graphene with swift heavy ions. Carbon 2017, 114, 511–518. [Google Scholar] [CrossRef]
- Kim, T.Y.; Park, C.-H.; Marzari, N. The Electronic Thermal Conductivity of Graphene. Nano Lett. 2016, 16, 2439–2443. [Google Scholar] [CrossRef] [PubMed]
- Clochard, M.C.; Melilli, G.; Rizza, G.; Madon, B.; Alves, M.; Wegrowe, J.E.; Toimil-Molares, M.E.; Christian, M.; Ortolani, L.; Rizzoli, R.; et al. Large area fabrication of self-standing nanoporous graphene-on-PMMA substrate. Mater. Lett. 2016, 184, 47–51. [Google Scholar] [CrossRef]
- Madauß, L.; Schumacher, J.; Ghosh, M.; Ochedowski, O.; Meyer, J.; Lebius, H.; Ban-d’Etat, B.; Toimil-Molares, M.E.; Trautmann, C.; Lammertink, R.; et al. Fabrication of nanoporous graphene/polymer composite membranes. Nanoscale 2017, 9, 10487–10493. [Google Scholar] [CrossRef] [PubMed]
- Schedin, F.; Geim, A.; Morozov, S.; Hill, E.; Blake, P.; Katsnelson, M.; Novoselov, K. Detection of individual gas molecules adsorbed on graphene. Nature Materials 2007, 6, 652–655. [Google Scholar] [CrossRef] [PubMed]
- Yuan, W.; Shi, G. Graphene-based gas sensors. J. Mater. Chem. A 2013, 1, 10078–10091. [Google Scholar] [CrossRef]
- Kumar, S.; Tripathi, A.; Singh, F.; Khan, S.A.; Baranwal, V.; Avasthi, D.K. Purification/annealing of graphene with 100-MeV Ag ion irradiation. Nanoscale Res. Lett. 2014, 9, 126. [Google Scholar] [CrossRef] [PubMed]
- Kumar, S.; Ashish Kumar and, A.T.; Tyagi, C.; Avasthi, D. Engineering of electronic properties of single layer graphene by swift heavy ionirradiation. J. Appl. Phys. 2017, 123, 161533. [Google Scholar] [CrossRef]
- Ochedowski, O.; Marinov, K.; Wilbs, G.; Keller, G.; Scheuschner, N.; Severin, D.; Bender, M.; Maultzsch, J.; Tegude, F.J.; Schleberger, M. Radiation hardness of graphene and MoS2 field effect devices against swift heavy ion irradiation. J. Appl. Phys. 2013, 113, 214306. [Google Scholar] [CrossRef]
- Kim, T.Y.; Cho, K.; Park, W.; Park, J.; Song, Y.; Hong, S.; Hong, W.K.; Lee, T. Irradiation Effects of High-Energy Proton Beams on MoS2 Field Effect Transistors. ACS Nano 2014, 8, 2774–2781. [Google Scholar] [CrossRef] [PubMed]
- Mathew, S.; Chan, T.; Zhan, D.; Gopinadhan, K.; Barman, A.; Breese, M.; Dhar, S.; Shen, Z.; Venkatesan, T.; Thong, J. Mega-electron-volt proton irradiation on supported and suspended graphene: A Raman spectroscopic layer dependent study. J. Appl. Phys. 2011, 110, 084309. [Google Scholar] [CrossRef]
- Mathew, S.; Chan, T.; Zhan, D.; Gopinadhan, K.; Barman, A.; Breese, M.; Dhar, S.; Shen, Z.; Venkatesan, T.; Thong, J. The effect of layer number and substrate on the stability of graphene under MeV proton beam irradiation. Carbon 2011, 49, 1720–1726. [Google Scholar] [CrossRef]
- Akcöltekin, E.; Peters, T.; Meyer, R.; Duvenbeck, A.; Klusmann, M.; Monnet, I.; Lebius, H.; Schleberger, M. Creation of multiple nanodots by single ions. Nat. Nanotechnol. 2007, 2, 290–294. [Google Scholar] [CrossRef] [PubMed]
- Madauß, L.; Zegkinoglou, I.; Vázquez Muiños, H.; Choi, Y.-W.; Kunze, S.; Zhao, M.-Q.; Naylor, C.H.; Ernst, P.; Pollmann, E.; Ochedowski, O.; et al. Highly Active Single-Layer MoS2 Catalyst Synthesized by Swift Heavy Ion Irradiation. Submitt. Nanoscale 2018. under review. [Google Scholar]
- Hopster, J.; Kozubek, R.; Krämer, J.; Sokolovsky, V.; Schleberger, M. Ultra-thin MoS2 irradiated with highly charged ions. Nucl. Instrum. Methods Phys. Res. Sect. B 2013, 317, 165–169. [Google Scholar] [CrossRef]
- Heller, R.; Facsko, S.; Wilhelm, R.; Möller, W. Defect mediated desorption of the KBr(001) surface induced by single highly charged ion impact. Phys. Rev. Lett. 2008, 101, 096102. [Google Scholar] [CrossRef] [PubMed]
- Hopster, J.; Kozubek, R.; Ban-d’Etat, B.; Guillous, S.; Lebius, H.; Schleberger, M. Damage in graphene due to electronic excitation induced by highly charged ions. 2D Mater. 2014, 1, 011011. [Google Scholar] [CrossRef]
- Ruan, J.A.; Bhushan, B. Atomic-Scale Friction Measurements Using Friction Force Microscopy: Part I—General Principles and New Measurement Techniques. J. Tribol. 2008, 116, 378–388. [Google Scholar] [CrossRef]
- Ernst, P.; Kozubek, R.; Madauß, L.; Sonntag, J.; Lorke, A.; Schleberger, M. Irradiation of Graphene Field Effect Transistors with Highly Charged Ions. Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. Atoms 2016, 382, 71–75. [Google Scholar] [CrossRef]
- Peng, H.; Sun, M.; Liu, F.; Yang, D.; Zhang, D.; Yuan, W.; Du, X.; Chen, H.; Wang, L.; Wang, T. Potential effect on the interaction of highly charged ion with graphene. Nucl. Instrum. Methods Phys. Res. Sect. B 2017, 407, 291–296. [Google Scholar] [CrossRef]
- Kozubek, R. Analyse von Defekstrukturen in Zweidimensionalen Materialien Nach der Interaktion Mit Hochgeladenen Ionen. Ph.D. Thesis, Fakultät für Physik, Universität Duisburg-Essen, Duisburg, Germany, 2018. [Google Scholar]
- Kozubek, R.; Philipp, E.; Herbig, C.; Michely, T.; Schleberger, M. Fabrication of Defective Single Layers of Hexagonal Boron Nitride on Various Supports for Potential Applications in Catalysis and DNA Sequencing. ACS Appl. Nano Mater. 2018, 1, 3765–3773. [Google Scholar] [CrossRef]
- Eck, W.; Küller, A.; Grunze, M.; Völkel, B.; Gölzhäuser, A. Freestanding Nanosheets from Crosslinked Biphenyl Self-Assembled Monolayers. Appl. Phys. Lett. 2005, 17, 2583–2587. [Google Scholar] [CrossRef]
- Ritter, R.; Wilhelm, R.A.; Stoger-Pollach, M.; Heller, R.; Mucklich, A.; Werner, U.; Vieker, H.; Beyer, A.; Facsko, S.; Golzhäuser, A.; et al. Fabrication of nanopores in 1 nm thick carbon nanomembranes with slow highly charged ions. Appl. Phys. Lett. 2013, 102, 063112. [Google Scholar] [CrossRef]
- Mounet, N.; Gibertini, M.; Schwaller, P.; Campi, D.; Merkys, A.; Marrazzo, A.; Sohier, T.; Castelli, I.E.; Cepellotti, A.; Pizzi, G.; et al. Two-dimensional materials from high-throughput computational exfoliation of experimentally known compounds. Nat. Nanotechnol. 2018, 13, 246–252. [Google Scholar] [CrossRef] [PubMed]
- Ding, L.; Wei, Y.; Li, L.; Zhang, T.; Wang, H.; Xue, J.; Ding, L.X.; Wang, S.; Caro, J.; Gogotsi, Y. MXene molecular sieving membranes for highly efficient gas separation. Nat. Commun. 2018, 9, 155. [Google Scholar] [CrossRef] [PubMed]
- Marrazzo, A.; Gibertini, M.; Campi, D.; Mounet, N.; Marzari, N. Prediction of a Large-Gap and Switchable Kane-Mele Quantum Spin Hall Insulator. Phys. Rev. Lett. 2018, 120, 117701. [Google Scholar] [CrossRef] [PubMed]
- Balan, A.; Radhakrishnan, S.; Woellner, C.F.; Sinha, S.K.; Deng, L.; de los Reyes, C.; Rao, B.M.; Paulose, M.; Neupane, R.; Apte, A.; et al. Exfoliation of a non-van der Waals material from iron ore hematite. Nat. Nanotechnol. 2018, 13, 602–609. [Google Scholar] [CrossRef] [PubMed]


© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Schleberger, M.; Kotakoski, J. 2D Material Science: Defect Engineering by Particle Irradiation. Materials 2018, 11, 1885. https://doi.org/10.3390/ma11101885
Schleberger M, Kotakoski J. 2D Material Science: Defect Engineering by Particle Irradiation. Materials. 2018; 11(10):1885. https://doi.org/10.3390/ma11101885
Chicago/Turabian StyleSchleberger, Marika, and Jani Kotakoski. 2018. "2D Material Science: Defect Engineering by Particle Irradiation" Materials 11, no. 10: 1885. https://doi.org/10.3390/ma11101885
APA StyleSchleberger, M., & Kotakoski, J. (2018). 2D Material Science: Defect Engineering by Particle Irradiation. Materials, 11(10), 1885. https://doi.org/10.3390/ma11101885





