Modelling and Characterization of Effective Thermal Conductivity of Single Hollow Glass Microsphere and Its Powder
Abstract
:1. Introduction
2. Hollow Glass Microspheres
3. Experiment
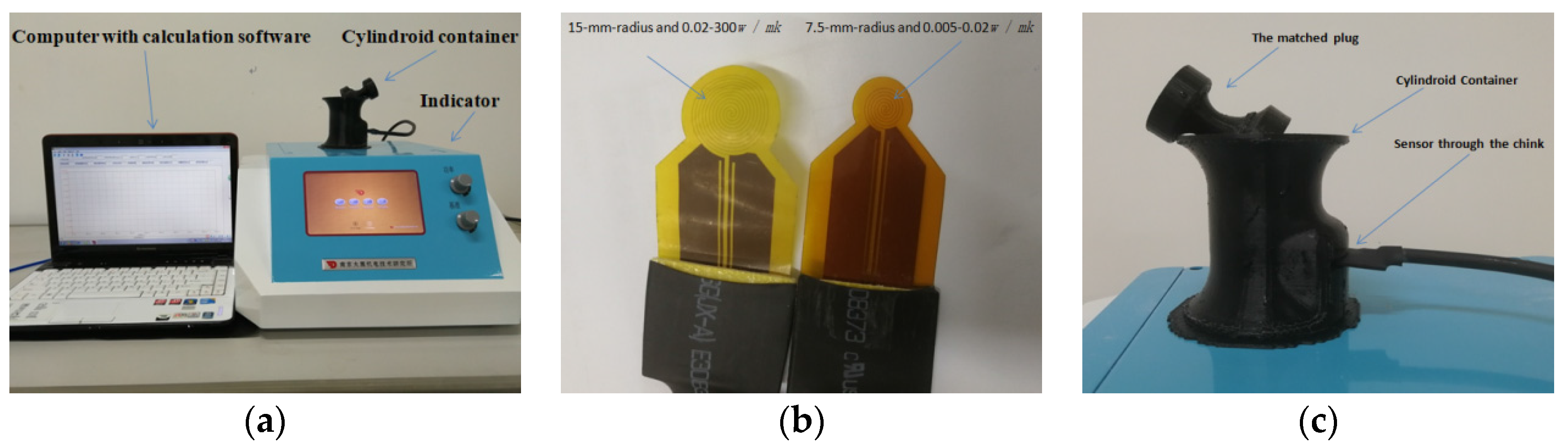
3.1. Introduction of the TPS Method
3.2. Experimental Program
4. Computational Model and Results
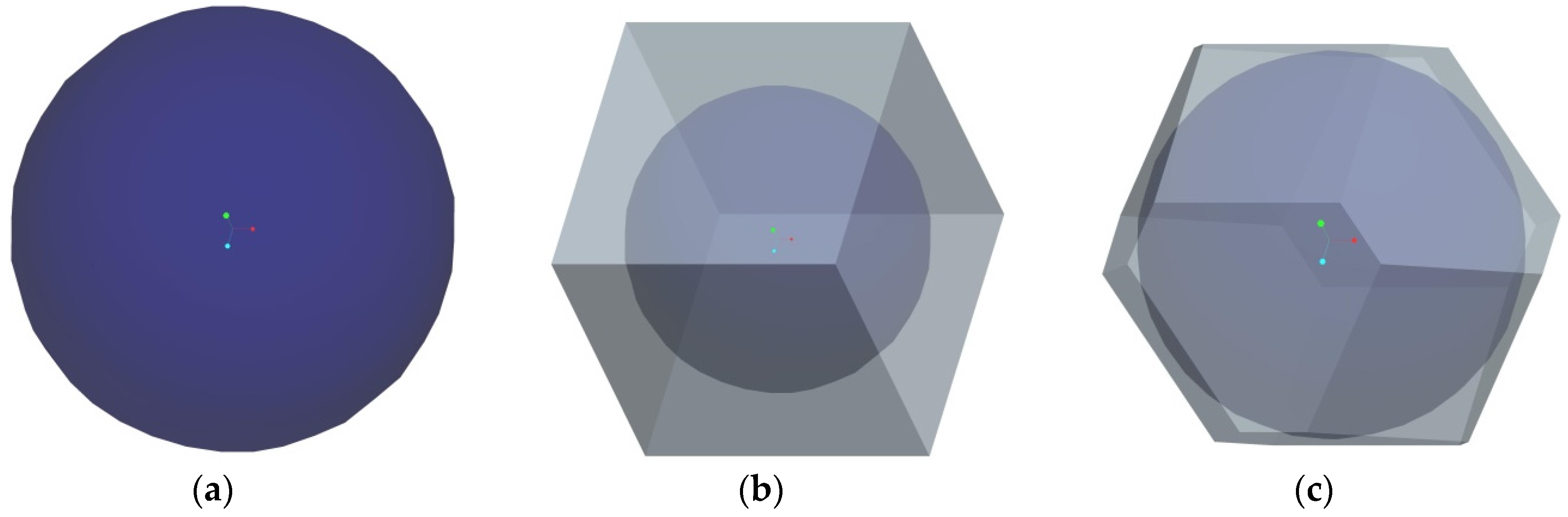
4.1. Three Different HGM Stacking Elements
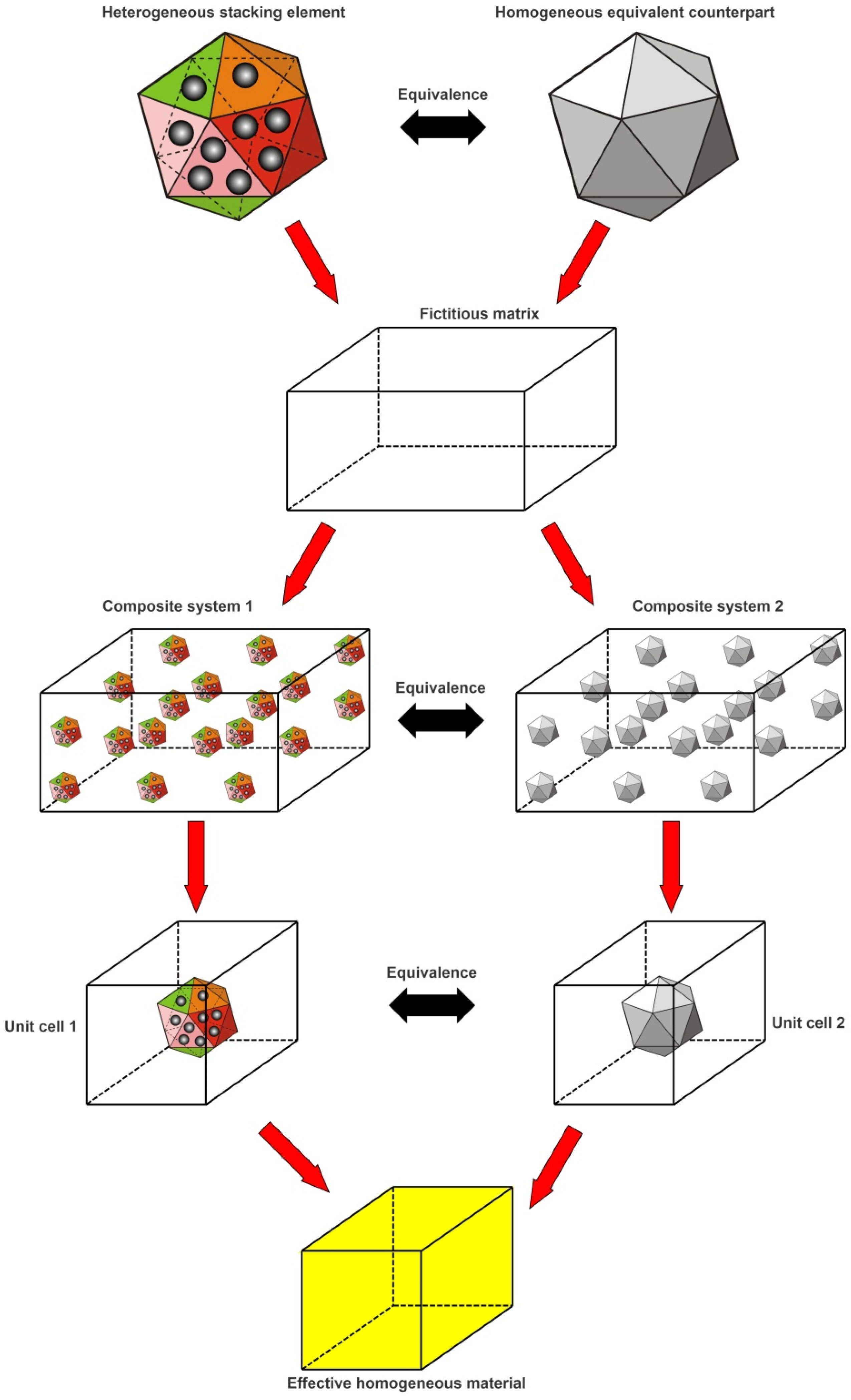
4.2. 3D Two-Step Hierarchical Computational Method
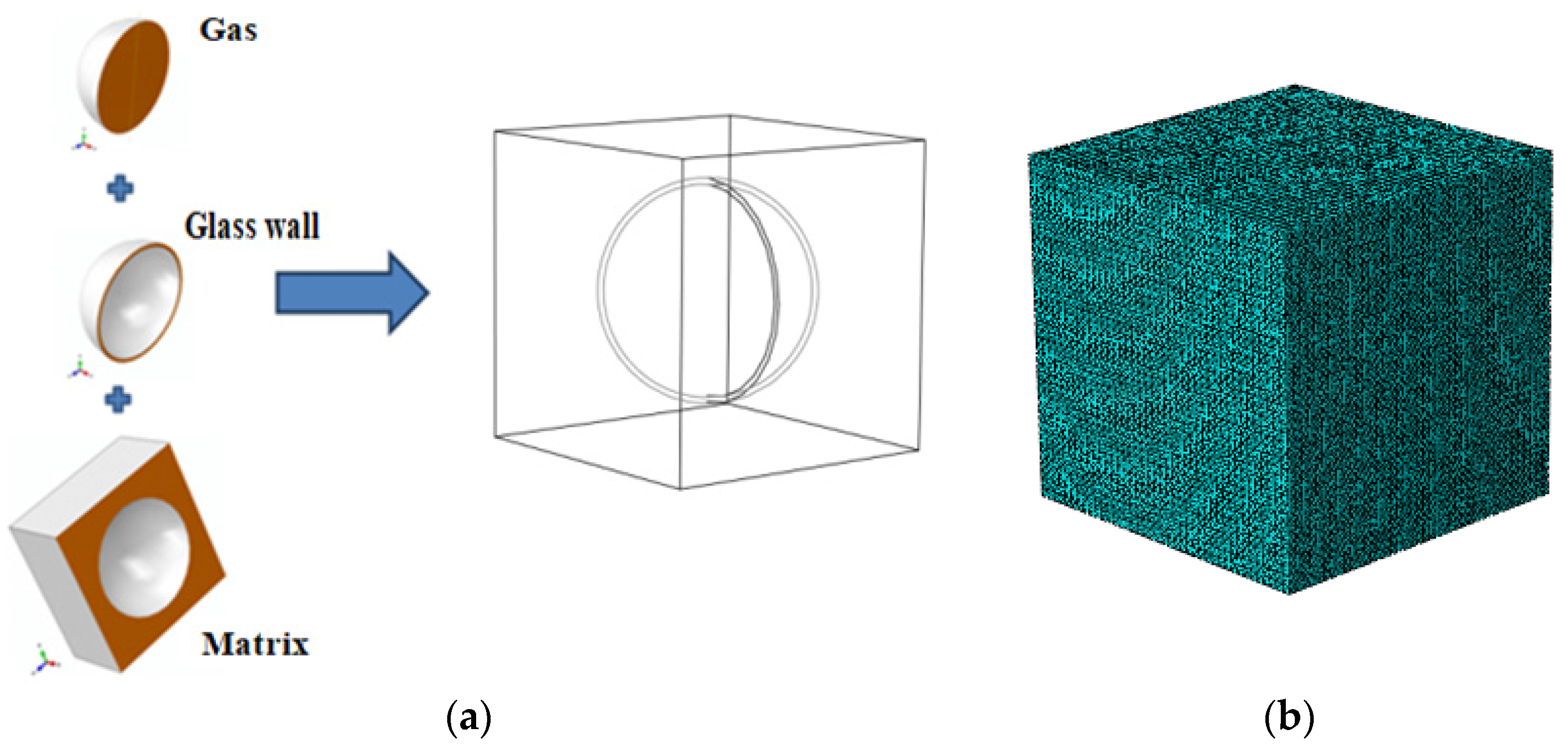
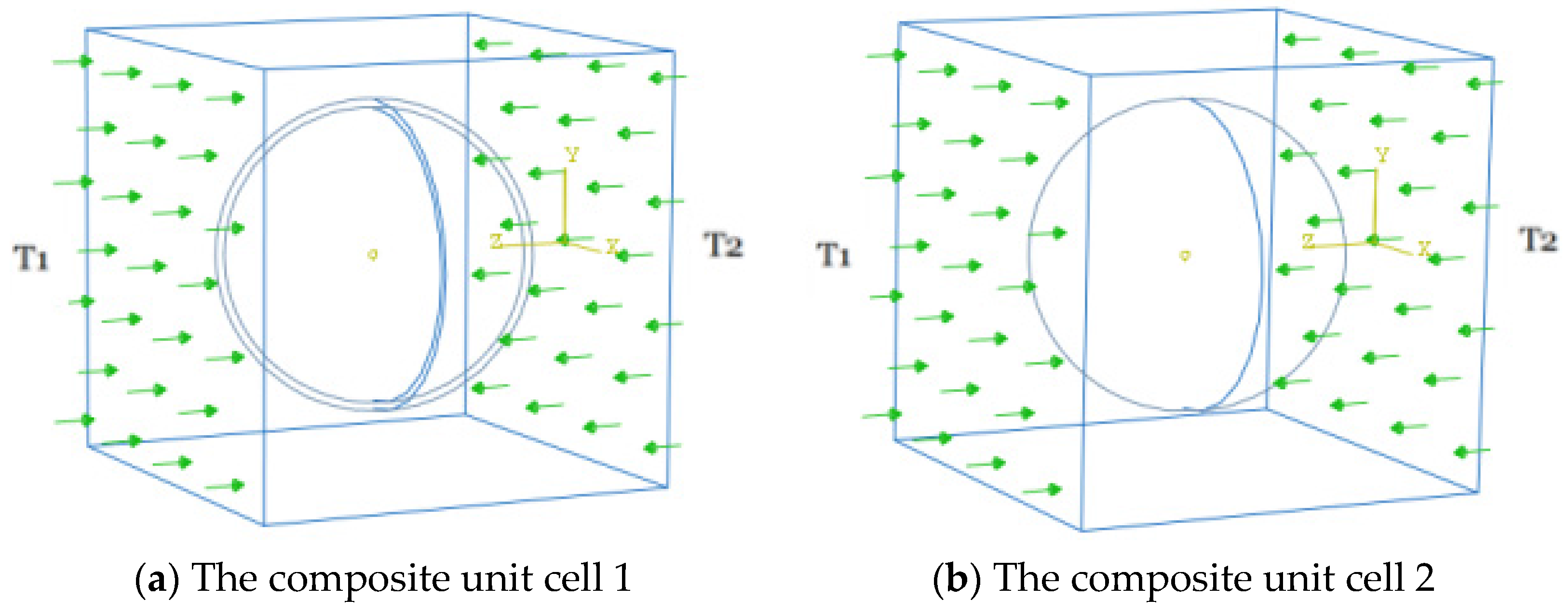
4.2.1. The Composite System with Actual Filler
4.2.2. The Composite System with Equivalent Filler
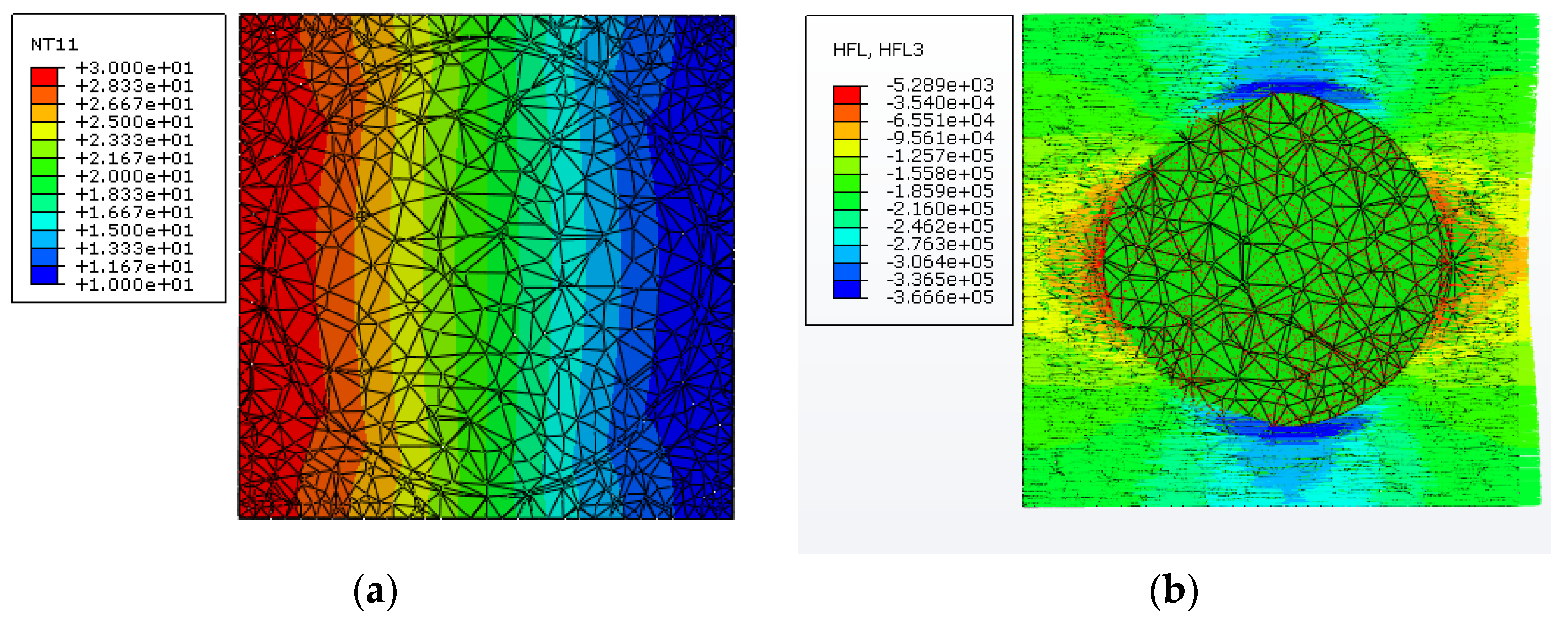
4.2.3. Basic Heat Transfer in the Two Composite Systems
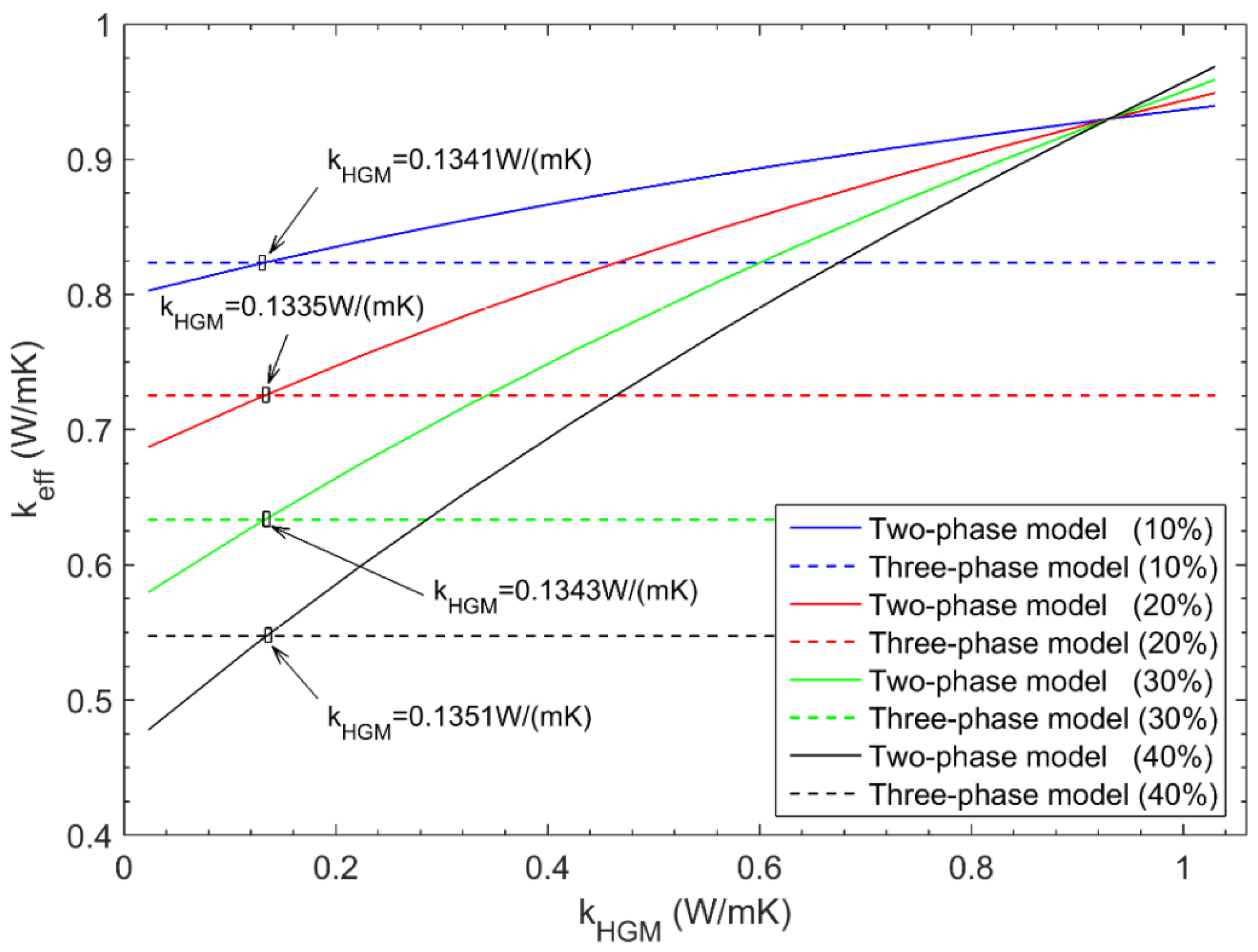
4.2.4. Results and Discussion
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Trofimov, A.; Pleshkov, L.; Back, H.L. Hollow glass microspheres for high strength composite cores. Reinf. Plast. 2006, 50, 44–50. [Google Scholar] [CrossRef]
- Ren, S.; Liu, J.C.; Guo, A.R.; Zang, W.J.; Geng, H.T.; Tao, X.; Du, H.Y. Mechanical properties and thermal conductivity of a temperature resistance hollow glass microspheres/borosilicate glass buoyance material. Mater. Sci. Eng. A 2016, 674, 604–614. [Google Scholar] [CrossRef]
- Verweij, H.; Veeneman, D.E. Hollow glass microsphere composites: Preparation and properties. J. Mater. Sci. 1985, 20, 1069–1078. [Google Scholar] [CrossRef]
- Wang, X.; Yan, H.; Xue, R.; Qi, S. A Polypyrrole/CoFe2O4/Hollow Glass Microspheres three-layer sandwich structure microwave absorbing material with wide absorbing bandwidth and strong absorbing capacity. J. Mater. Sci.-Mater. Electron. 2017, 28, 519–525. [Google Scholar] [CrossRef]
- Roquescarmes, T.; Marchal, P.; Gigante, A.; Corbel, S. Stereolithography fabrication and characterization of syntactic foams containing hollow glass microspheres. Russ. Chem. Rev. 2009, 78, 375–386. [Google Scholar] [CrossRef]
- Yakushin, V.L.; Kova, L.B.; Sevastyanova, I. Properties of rigid polyurethane foams filled with glass microspheres. Mech. Compos. Mater. 2012, 48, 579–586. [Google Scholar] [CrossRef]
- Swetha, C.; Kumar, R. Quasi-static uni-axial compression behaviour of hollow glass microspheres/epoxy based syntactic foams. Mater. Des. 2011, 32, 4152–4163. [Google Scholar] [CrossRef]
- Zhu, B.L.; Ma, J.; Wang, J.; Wu, J.; Peng, D.S. Thermal, dielectric and compressive properties of hollow glass microsphere filled epoxy-matrix composites. J. Reinf. Plast. Compos. 2012, 31, 1311–1326. [Google Scholar] [CrossRef]
- Rizzi, E.; Papa, E.; Corigliano, A. Mechanical behavior of a syntactic foam: Experiments and modeling. Int. J. Solids Struct. 2000, 37, 5773–5794. [Google Scholar] [CrossRef]
- Yung, K.C.; Zhu, B.; Yue, T.M.; Xie, C.S. Preparation and properties of hollow glass microsphere-filled epoxy-matrix composites. Compos. Sci. Technol. 2009, 69, 260–264. [Google Scholar] [CrossRef]
- Marur, P.R. Numerical estimation of effective elastic moduli of syntactic foams. Finite Elem. Anal. Des. 2010, 46, 1001–1007. [Google Scholar] [CrossRef]
- Inozemtcev, A.S.; Korolev, E.V. A method for the reduction of deformation of high-strength lightweight cement concrete. Adv. Cem. Res. 2016, 28, 92–98. [Google Scholar] [CrossRef]
- Sugama, T.; Wetzel, E.D. Microsphere-filled lightweight calcium phosphate cements. J. Mater. Sci. 1994, 29, 5165–5176. [Google Scholar] [CrossRef]
- Sugama, T.; Carciello, N.; Nayberg, T. Mullite microsphere-filled lightweight calcium phosphate cement slurries for geothermal wells: Setting and properties. Cem. Concr. Res. 1995, 25, 1305–1310. [Google Scholar] [CrossRef]
- Wang, H.; Hou, F.; Zhao, X.J. Simulation of Thermal Behavior in Hollow-glass-microsphere-filled Cement Composites. Am. J. Mater. Sci. Technol. 2015, 4, 1–11. [Google Scholar] [CrossRef]
- Lee, D.J.; Song, Y.S. Thermomechanical anisotropy and flowability of talc and glass fiber reinforced multiphase polymer composites. Compos. Struct. 2017, 174, 329–337. [Google Scholar] [CrossRef]
- Liu, K.; Lu, L.; Wang, F.; Liang, W. Theoretical and experimental study on multi-phase model of thermal conductivity for fiber reinforced concrete. Constr. Build. Mater. 2017, 148, 465–475. [Google Scholar] [CrossRef]
- Li, B.; Yuan, J.; An, Z.G.; Zhang, J.J. Effect of microstructure and physical parameters of hollow glass microsphere on insulation performance. Mater. Lett. 2011, 65, 1992–1994. [Google Scholar] [CrossRef]
- Zhu, B.L.; Zheng, H.; Wang, J.; Ma, J.; Wu, J.; Wu, R. Tailoring of thermal and dielectric properties of LDPE-matrix composites by the volume fraction, density, and surface modification of hollow glass microsphere filler. Compos. Part B-Eng. 2014, 58, 91–102. [Google Scholar] [CrossRef]
- Zhu, B.L.; Wang, J.; Zheng, H.; Ma, J.; Wu, J.; Gan, Z.H.; Liu, J. Thermal conductivity and dielectric properties of immiscible LDPE/epoxy blend filled with hybrid filler consisting of HGM and nitride particle. J. Alloys Compd. 2017, 701, 499–507. [Google Scholar] [CrossRef]
- Wang, H.; Xiao, Y.; Qin, Q.H. 2D hierarchical heat transfer computational model of natural fiber bundle reinforced composite. Sci. Iran. 2016, 23, 268–276. [Google Scholar] [CrossRef]
- Gustafsson, S.E. Transient plane source techniques for thermal conductivity and thermal diffusivity measurements of solid materials. Rev. Sci. Instrum. 1991, 62, 797–804. [Google Scholar] [CrossRef]
- Gustavsson, M.; Karawacki, E.; Gustafsson, S.E. Thermal conductivity, thermal diffusivity, and specific heat of thin samples from transient measurements with hot disk sensors. Rev. Sci. Instrum. 1994, 65, 3856–3859. [Google Scholar] [CrossRef]
- Sadeghifar, H.; Djilali, N.; Bahrami, M. Effect of Polytetrafluoroethylene (PTFE) and micro porous layer (MPL) on thermal conductivity of fuel cell gas diffusion layers: Modeling and experiments. J. Power Sources 2014, 248, 632–641. [Google Scholar] [CrossRef]
- Sadeghifar, H. Reconstruction and analysis of fuel cell gas diffusion layers using fiber spacing rather than pore size data: Questioned validity of widely-used porosity-based thermal conductivity models. J. Power Sources 2016, 307, 673–677. [Google Scholar] [CrossRef]
- Sadeghifar, H.; Bahrami, M.; Djilali, N. A statistically-based thermal conductivity model for fuel cell Gas Diffusion Layers. J. Power Sources 2013, 233, 369–379. [Google Scholar] [CrossRef]
- Sadeghifar, H.; Djilali, N.; Bahrami, M. A new model for thermal contact resistance between fuel cell gas diffusion layers and bipolar plates. J. Power Sources 2014, 266, 51–59. [Google Scholar] [CrossRef]
- Sadeghifar, H.; Djilali, N.; Bahrami, M. Thermal conductivity of a graphite bipolar plate (BPP) and its thermal contact resistance with fuel cell gas diffusion layers: Effect of compression, PTFE, micro porous layer (MPL), BPP out-of-flatness and cyclic load. J. Power Sources 2015, 273, 96–104. [Google Scholar] [CrossRef]
- Sadeghifar, H. In-plane and through-plane electrical conductivities and contact resistances of a Mercedes-Benz catalyst-coated membrane, gas diffusion and micro-porous layers and a Ballard graphite bipolar plate: Impact of humidity, compressive load and polytetrafluoroethylene. Energy Convers. Manag. 2017, 154, 191–202. [Google Scholar]
- Sadeghifar, H.; Djilali, N.; Bahrami, M. Counter-intuitive reduction of thermal contact resistance with porosity: A case study of polymer electrolyte membrane fuel cells. Int. J. Hydrog. Energy 2016, 41, 6833–6841. [Google Scholar] [CrossRef]
- Hsu, C.C.; Chang, K.C.; Huang, T.C.; Yeh, L.C.; Yeh, W.T.; Ji, W.F.; Yeh, J.M.; Tsai, T.Y. Preparation and studies on properties of porous epoxy composites containing microscale hollow epoxy spheres. Microporous Mesoporous Mater. 2014, 198, 15–21. [Google Scholar] [CrossRef]
- Qin, Q.H.; Mai, Y.W.; Yu, S.W. Effective moduli for thermopiezoelectric materials with microcracks. Int. J. Fract. 1998, 91, 359–371. [Google Scholar] [CrossRef]
- Christensen, R.M. Effective properties of composite materials containing voids. Proc. R. Soc. Lond. A 1993, 440, 461–473. [Google Scholar] [CrossRef]
- Qin, Q.H.; Swain, M.V. A micro-mechanics model of dentin mechanical properties. Biomaterials 2004, 25, 5081–5090. [Google Scholar] [CrossRef] [PubMed]
- Sadeghifar, H. In-plane and through-plane local and average Nusselt numbers in fibrous porous materials with different fiber layer temperatures: Gas diffusion layers for fuel cells. J. Power Sources 2016, 325, 311–321. [Google Scholar] [CrossRef]
- Sadeghifar, H.; Djilali, N.; Bahrami, M. A compact closed-form Nusselt formula for laminar longitudinal flow between rectangular/square arrays of parallel cylinders with unequal row temperatures. Int. J. Therm. Sci. 2016, 100, 248–254. [Google Scholar] [CrossRef]
- Kang, Y.; Morita, K. Thermal conductivity of the CaO–Al2O3–SiO2 system. ISIJ Int. 2006, 46, 420–426. [Google Scholar] [CrossRef]
- Lienhard, J.H., IV; Lienhard, J.H., V. A Heat Transfer Textbook; Phlogiston Press: Cambridge, MA, USA, 2005. [Google Scholar]
- Liang, J.Z.; Li, F.H. Simulation of heat transfer in hollow-glass-bead-filled polypropylene composites by finite element method. Polym. Test. 2007, 26, 419–424. [Google Scholar] [CrossRef]
- Qin, Q.H. Trefftz finite element method and its applications. Appl. Mech. Rev. 2005, 58, 316–337. [Google Scholar] [CrossRef]
- Wang, H.; Qin, Q.H. Hybrid FEM with fundamental solutions as trial functions for heat conduction simulation. Acta Mech. Solida Sin. 2009, 22, 487–498. [Google Scholar] [CrossRef]
- Wang, H.; Qin, Q.H.; Xiao, Y. Special n-sided Voronoi fiber/matrix elements for clustering thermal effect in natural-hemp-fiber-filled cement composites. Int. J. Heat Mass Transf. 2016, 92, 228–235. [Google Scholar] [CrossRef]
- Wang, H.; Zhao, X.J.; Wang, J.S. Interaction analysis of multiple coated fibers in cement composites by special n-sided interphase/fiber elements. Compos. Sci. Technol. 2015, 118, 117–126. [Google Scholar] [CrossRef]
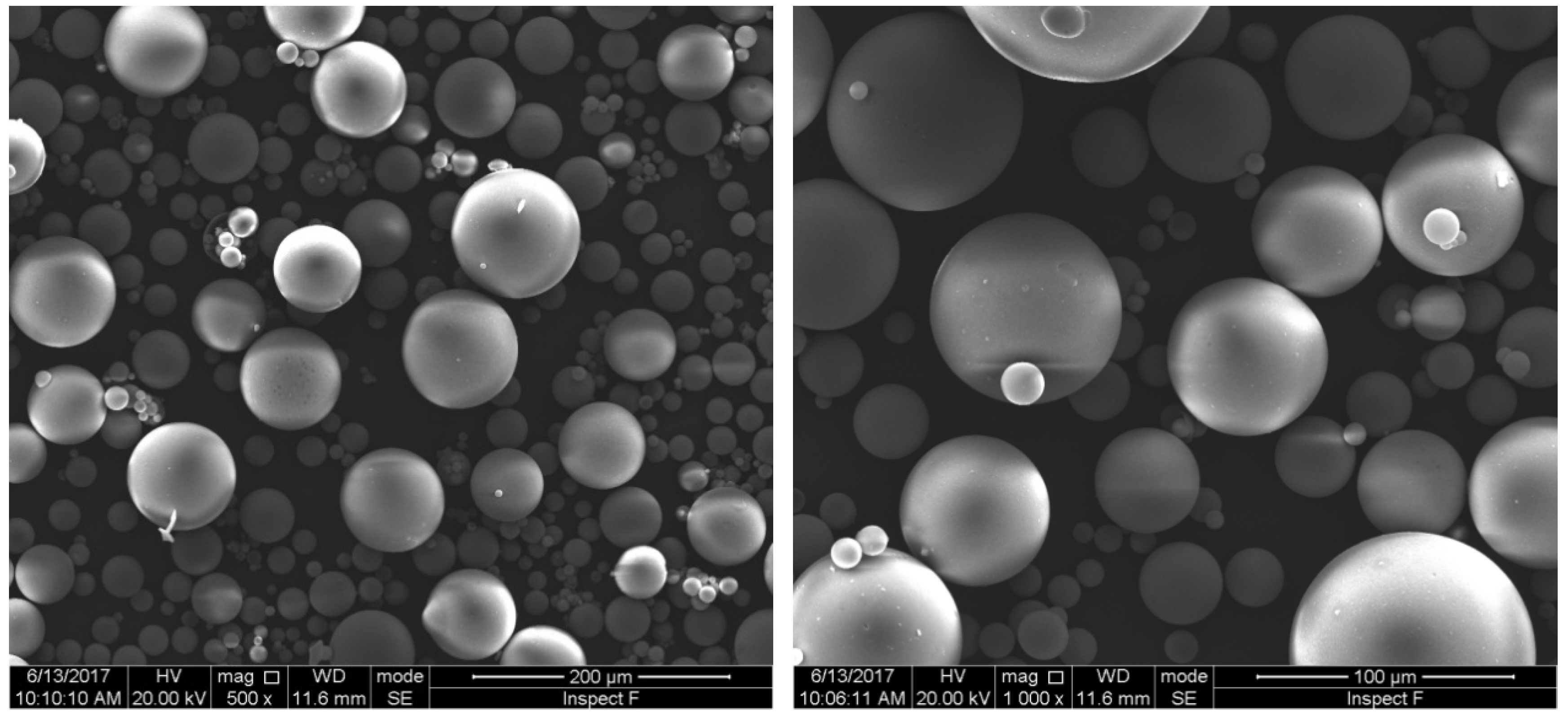
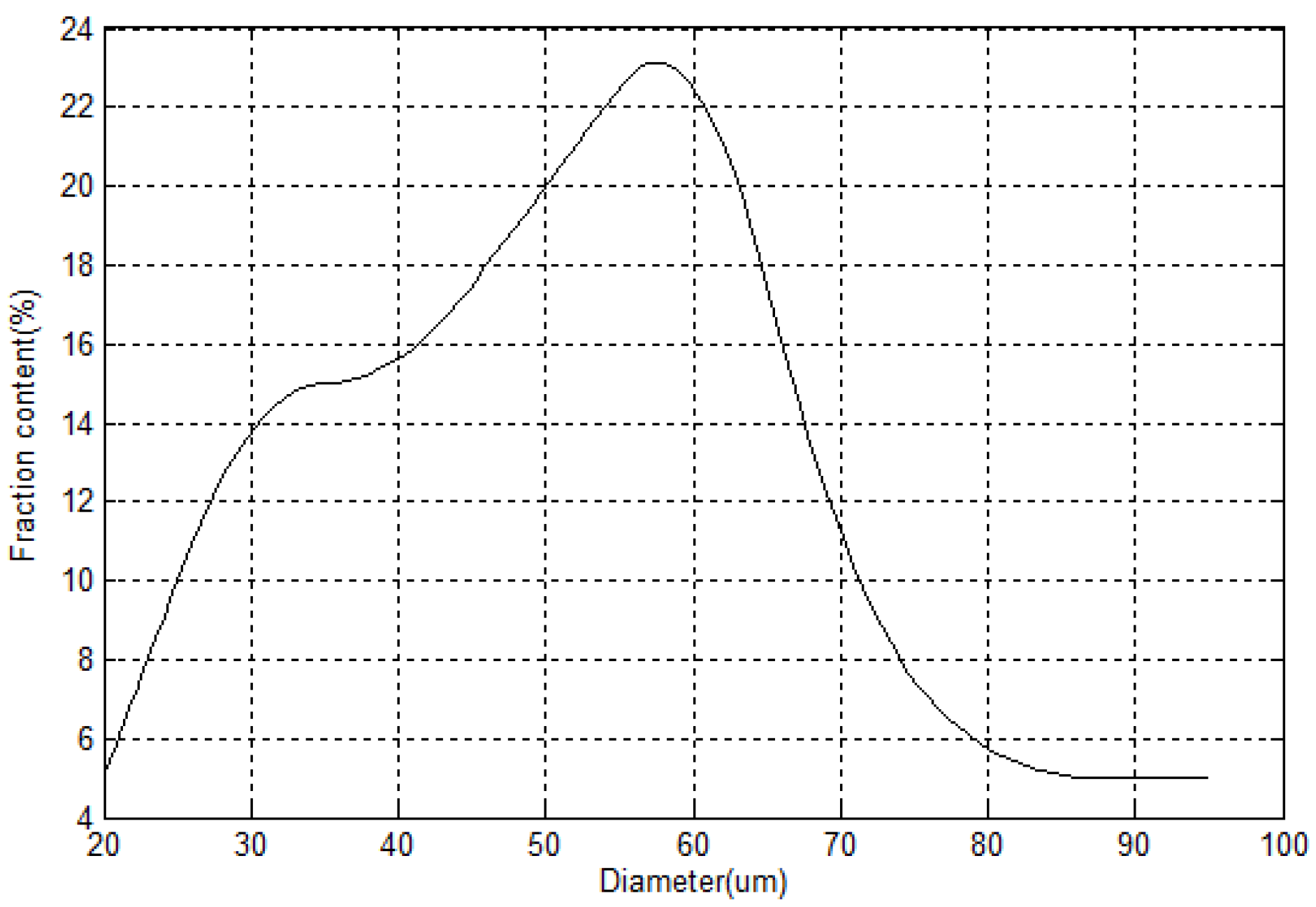
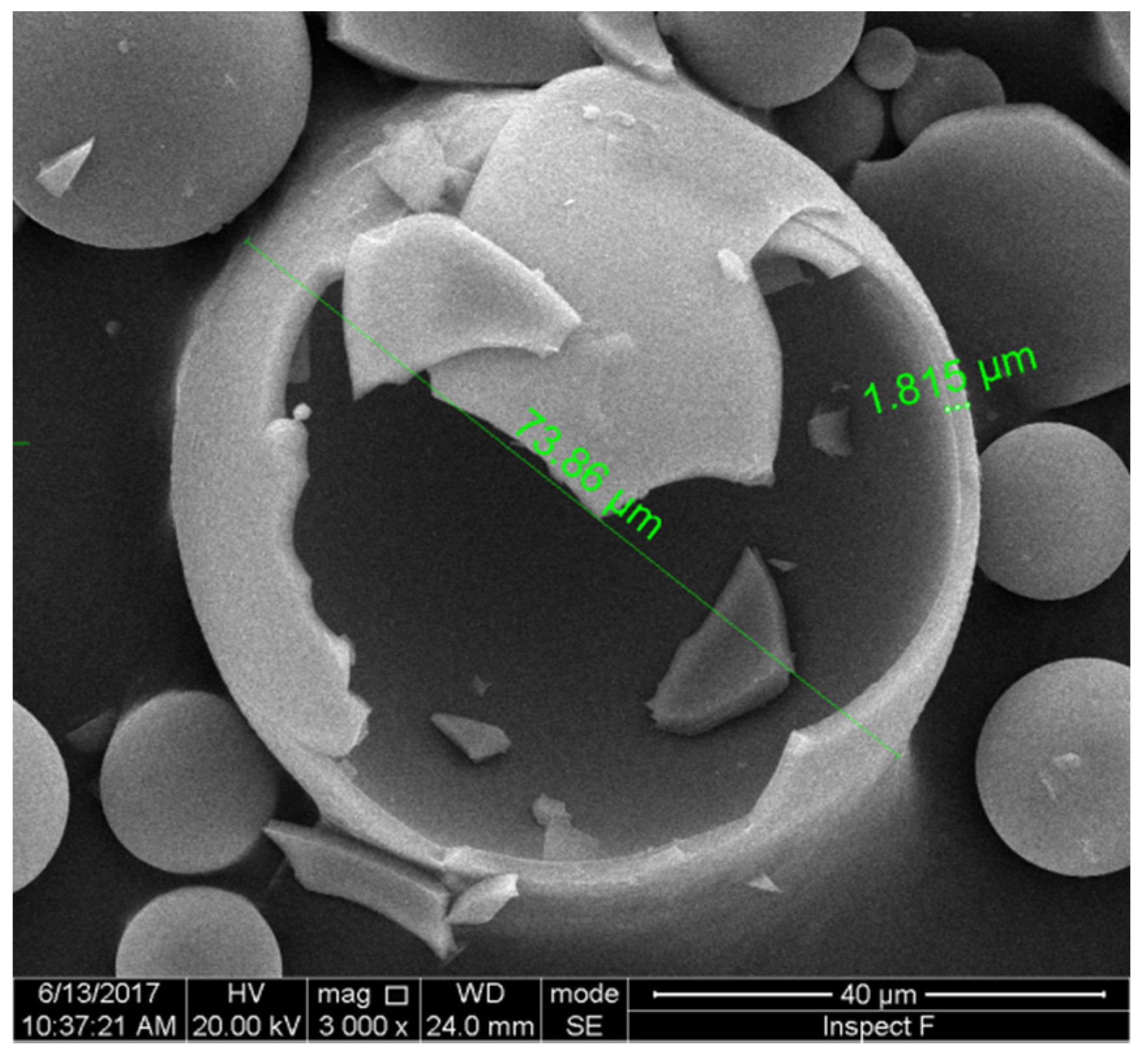
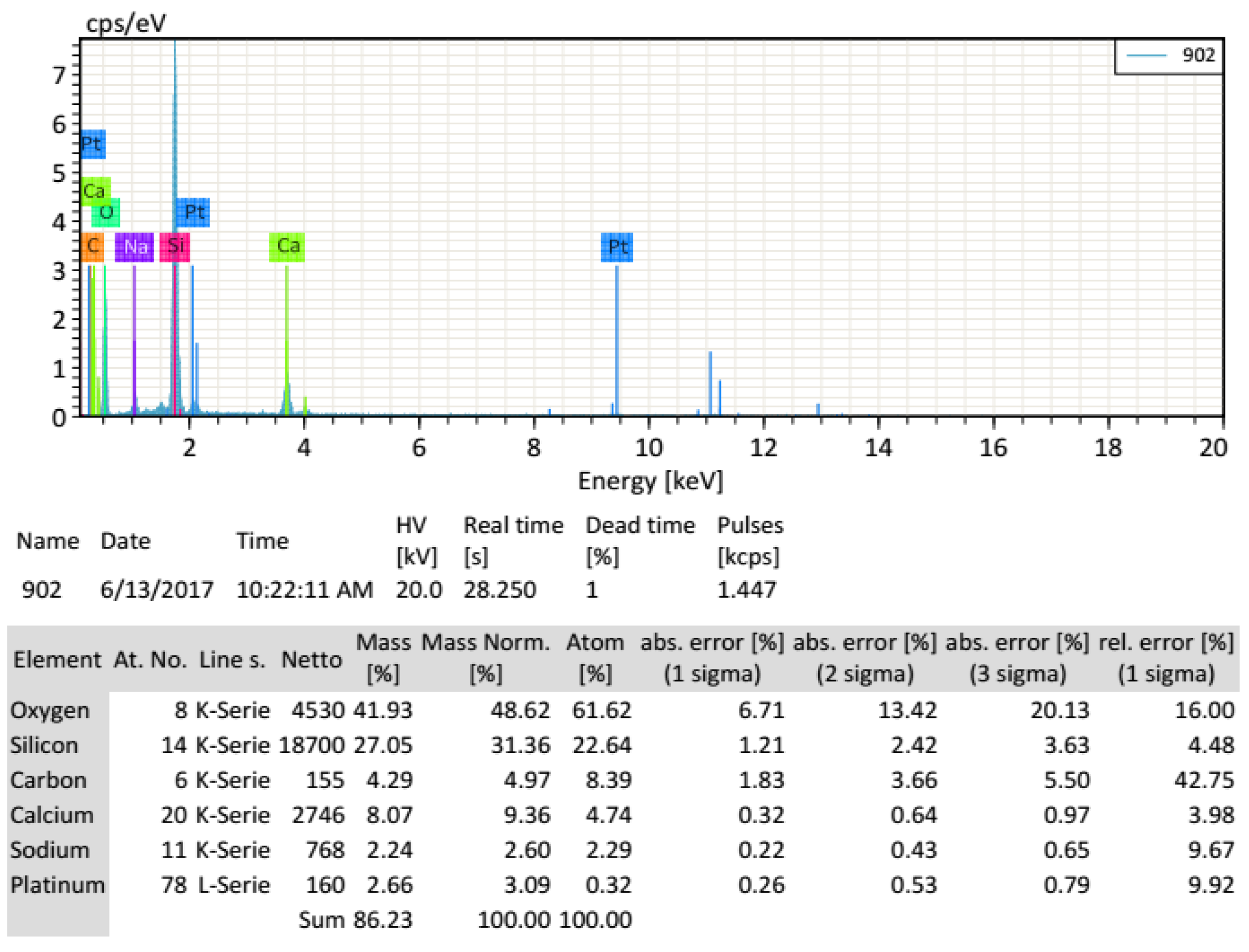




| Group | Heating Power (W) | Temperature Increase (K) | Thermal Conductivity (W/(mK)) |
|---|---|---|---|
| A | 0.061 | 282.78 | 0.0981 |
| 0.071 | 283.79 | 0.1005 | |
| 0.081 | 285.29 | 0.1014 | |
| B | 0.061 | 283.25 | 0.0877 |
| 0.071 | 284.76 | 0.0969 | |
| 0.081 | 284.39 | 0.1054 | |
| C | 0.061 | 281.81 | 0.1083 |
| 0.071 | 284.11 | 0.0943 | |
| 0.081 | 284.69 | 0.1115 | |
| D | 0.061 | 282.73 | 0.0952 |
| 0.071 | 284.30 | 0.1013 | |
| 0.081 | 285.40 | 0.1025 |
| Particle Size | Value |
|---|---|
| Average outer diameter D = () | 58.64 |
| wall thickness t () | 1.6 |
| Thermal conductivity | |
| Thermal conductivity of the gas (W/(mK)) | 0.023 [38] |
| Thermal conductivity of the solid wall (W/(mK)) | 1.03 |
| Thermal conductivity of the matrix (W/(mK)) | 0.93 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, B.; Wang, H.; Qin, Q.-H. Modelling and Characterization of Effective Thermal Conductivity of Single Hollow Glass Microsphere and Its Powder. Materials 2018, 11, 133. https://doi.org/10.3390/ma11010133
Liu B, Wang H, Qin Q-H. Modelling and Characterization of Effective Thermal Conductivity of Single Hollow Glass Microsphere and Its Powder. Materials. 2018; 11(1):133. https://doi.org/10.3390/ma11010133
Chicago/Turabian StyleLiu, Bing, Hui Wang, and Qing-Hua Qin. 2018. "Modelling and Characterization of Effective Thermal Conductivity of Single Hollow Glass Microsphere and Its Powder" Materials 11, no. 1: 133. https://doi.org/10.3390/ma11010133
APA StyleLiu, B., Wang, H., & Qin, Q.-H. (2018). Modelling and Characterization of Effective Thermal Conductivity of Single Hollow Glass Microsphere and Its Powder. Materials, 11(1), 133. https://doi.org/10.3390/ma11010133






