Electrical Double-Layer Capacitors in Hybrid Topologies —Assessment and Evaluation of Their Performance
Abstract
:1. Introduction
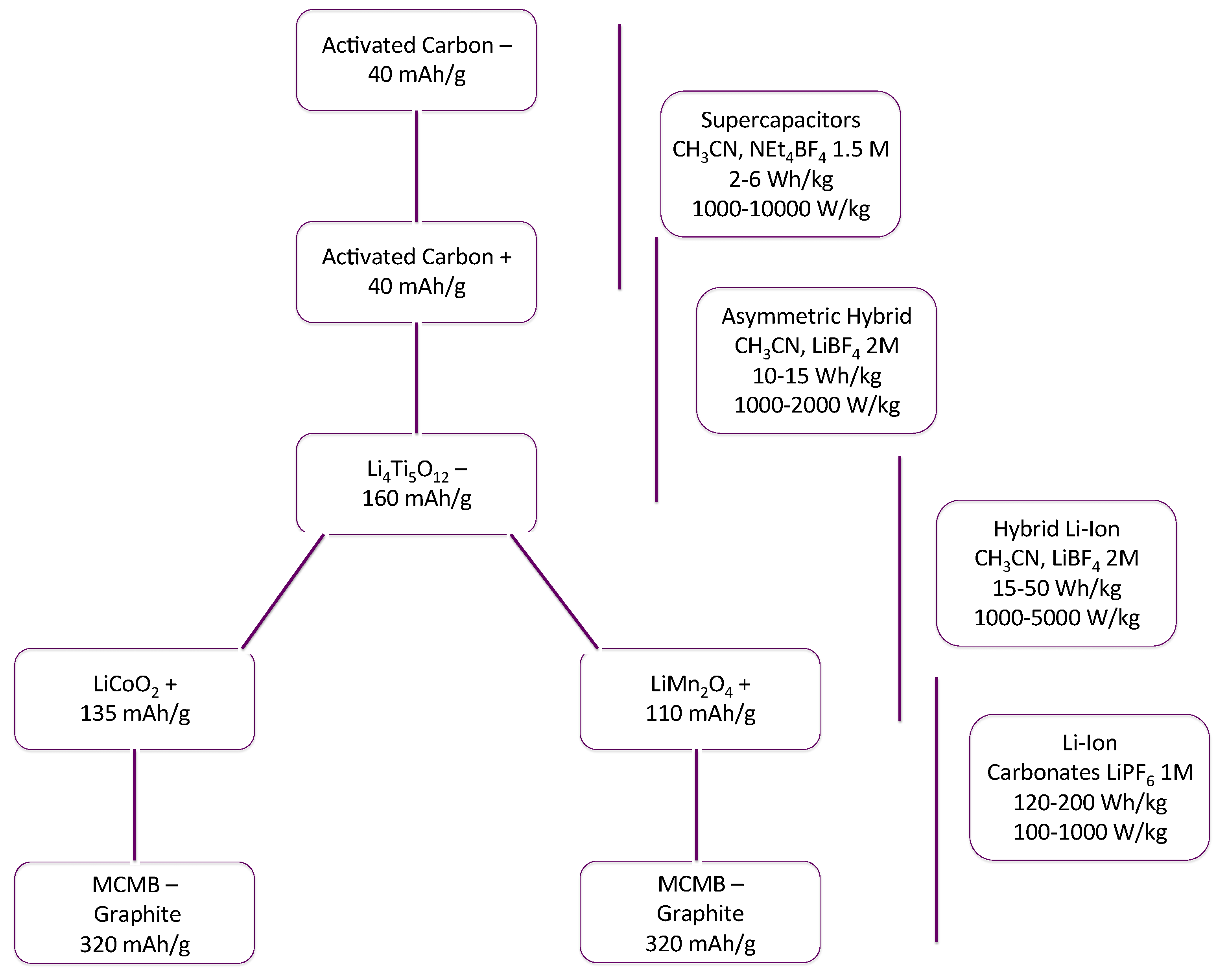
2. Hybrid Topologies

2.1. Topology “A”
2.2. Topology “B”
2.3. Topology “C”
2.4. Topology “D”
3. Simulation Tools
3.1. ADVISOR
3.2. SIMPLEV
3.3. PSAT
3.4. VSP
3.5. Modelica
3.6. Other Software Tools
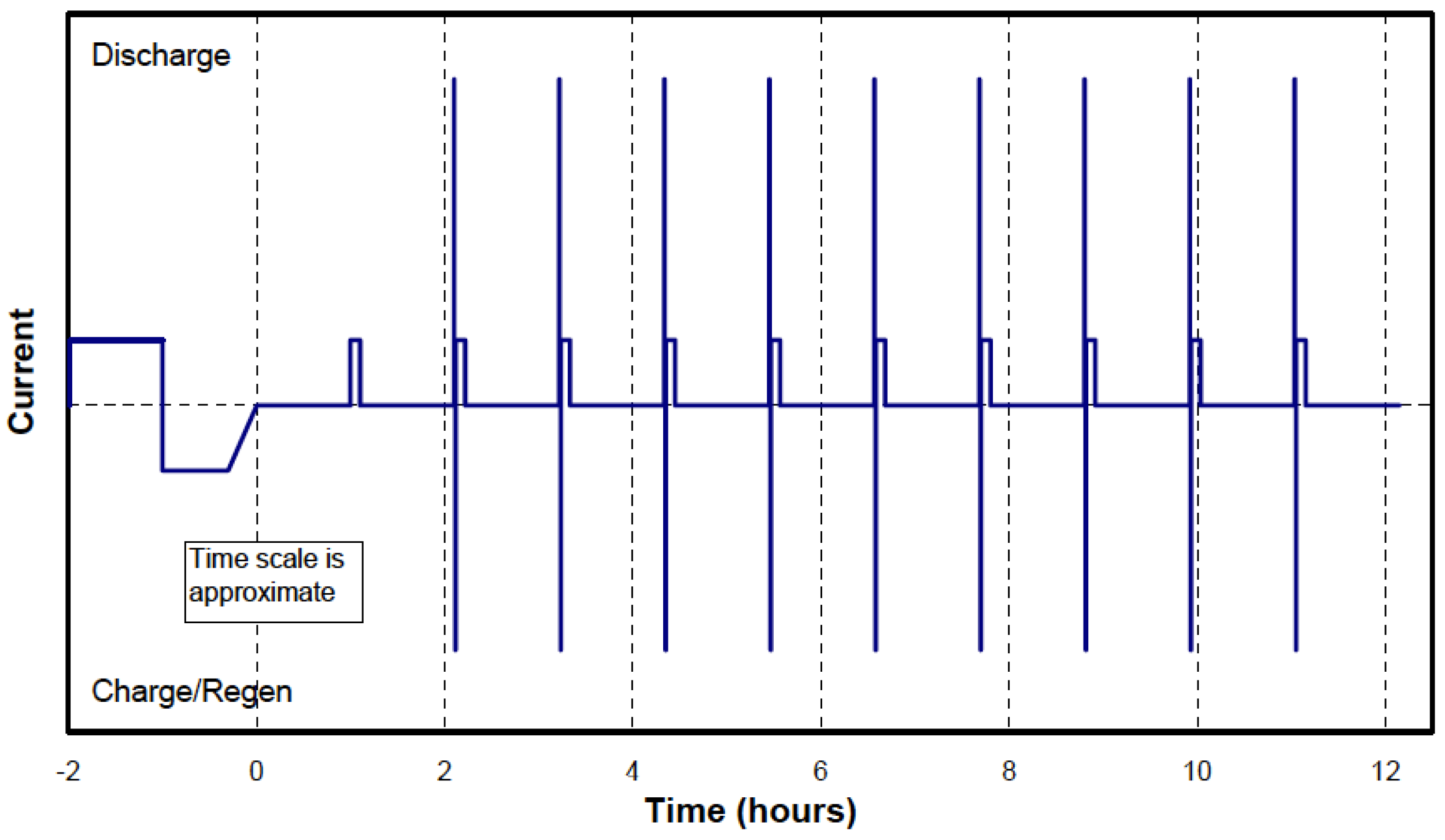
3.7. Dedicated Series Hybrid Electric Vehicle
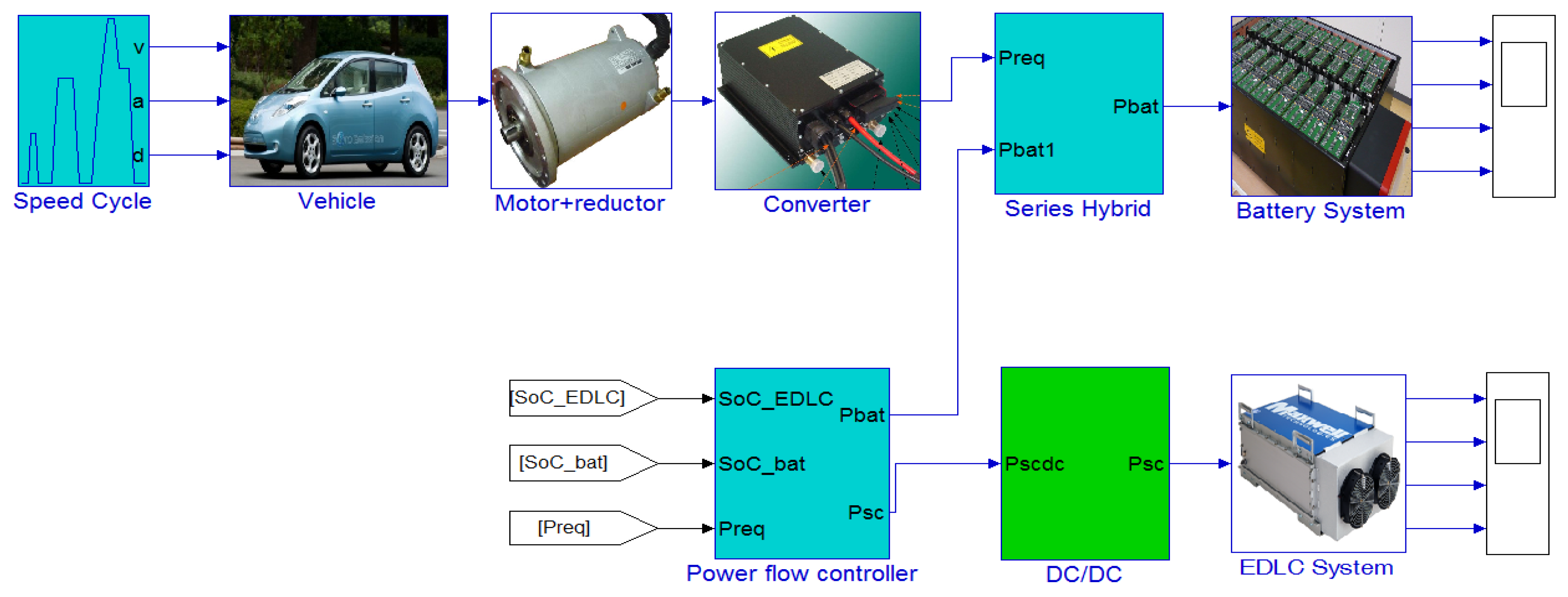
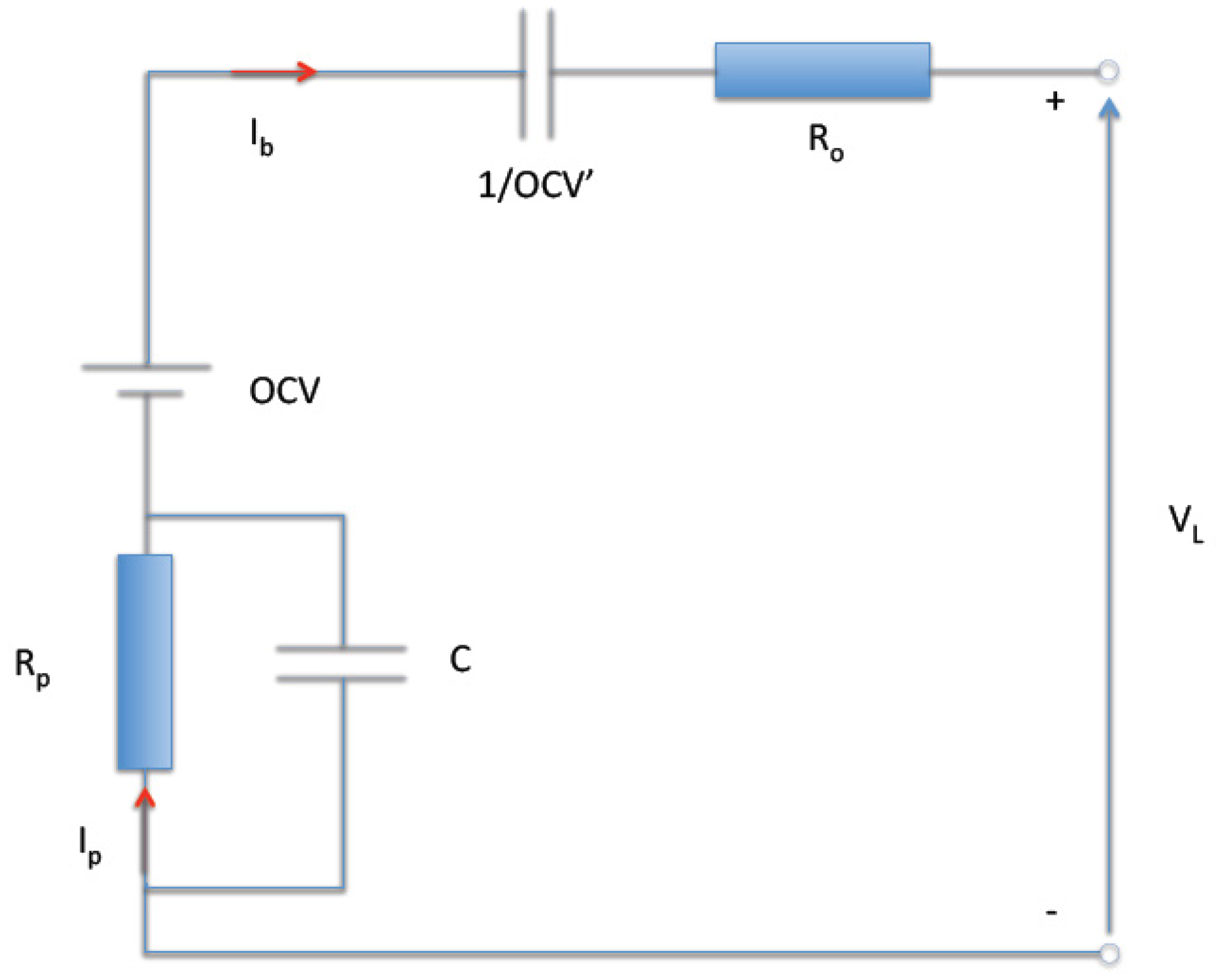
3.7.1. Battery Model

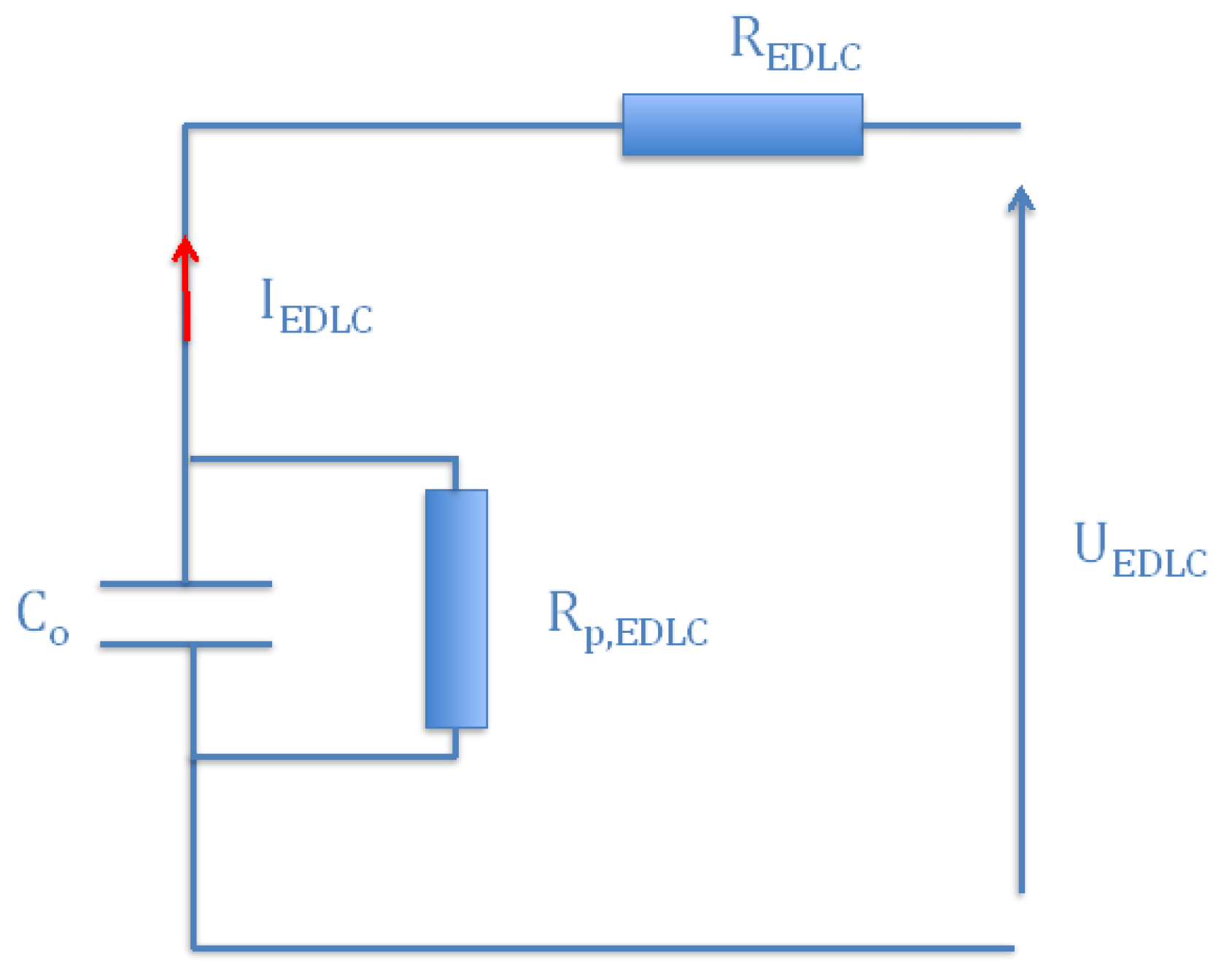
3.7.2. EDLC model
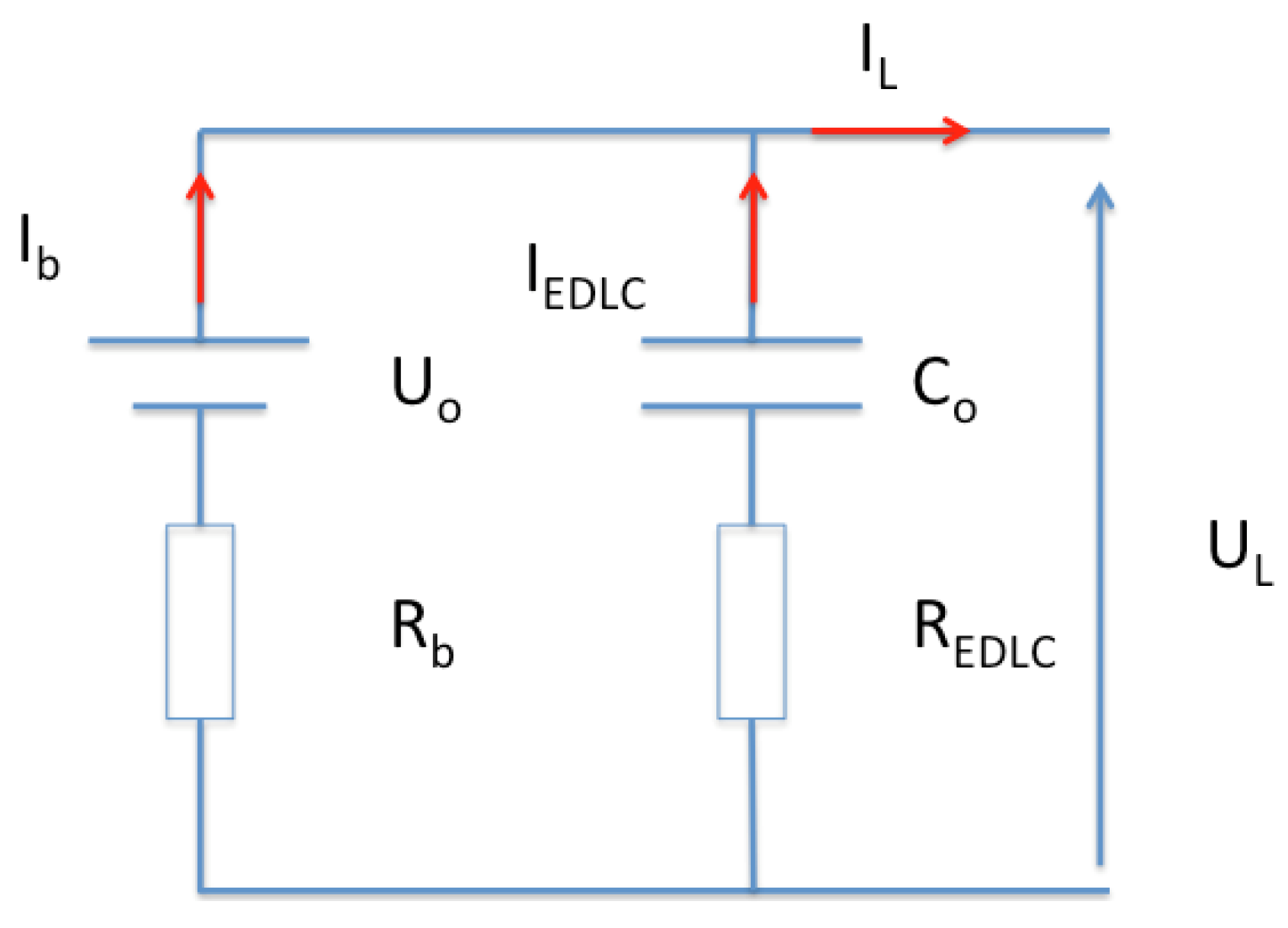
3.7.3. Converter, Motor and Reductor Models
4. Results and Discussion
4.1. Hybrid Rechargeable Energy Storage System Architecture without DC-DC Converter
| Property | Value | Unit |
|---|---|---|
| Vehicle mass (incl. battery) | 1525 | Kg |
| Aerodynamic drag coefficient | 0.29 | - |
| Rolling resistance coefficient | 0.01 | - |
| Front area | 2.27 | m2 |
| Peak power motor (AC) | 80 | kW |
| Battery energy content | 24 | kWh |
| Characteristics | Value | Unit |
|---|---|---|
| Cell voltage | 3.3 | V |
| Capacity | 45 | Ah |
| Internal resistance | 2.5 | mΩ |
| Mass | 0.99 | kg |
| Nominal pack voltage | 330 | V |
| Maximum voltage | 365 | V |
| Number cells in series | 100 | - |
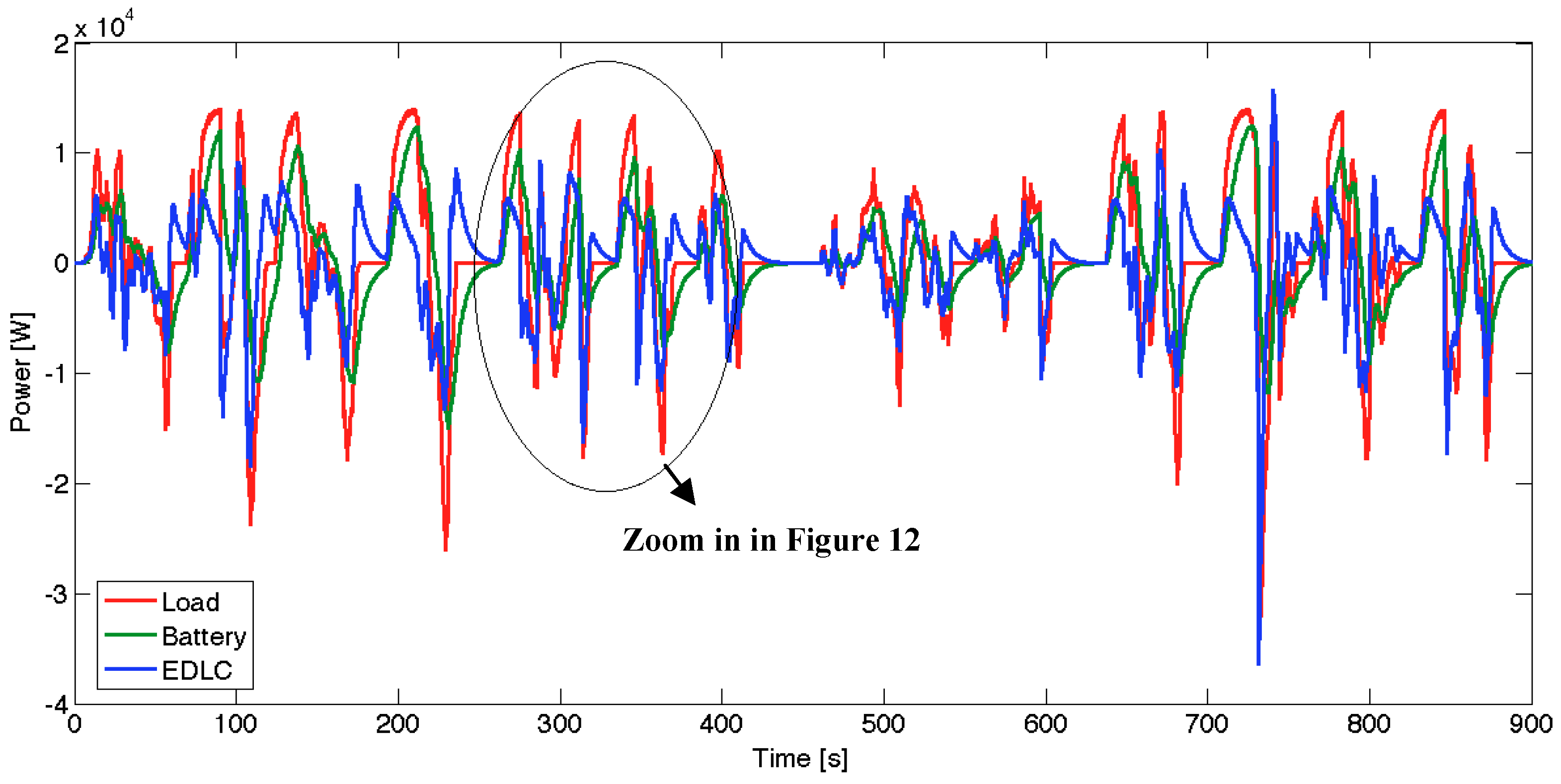
4.1.1. Simulation Results
| Characteristics | Value | Unit |
|---|---|---|
| Rated voltage | 125 | V |
| Rated capacitance | 63 | F |
| Internal resistance | 18 | mΩ |
| Mass | 60.5 | kg |
| Volume | 0.108 | m3 |
| Total mass | 181.5 | kg |
| Total volume | 0.32 | m3 |

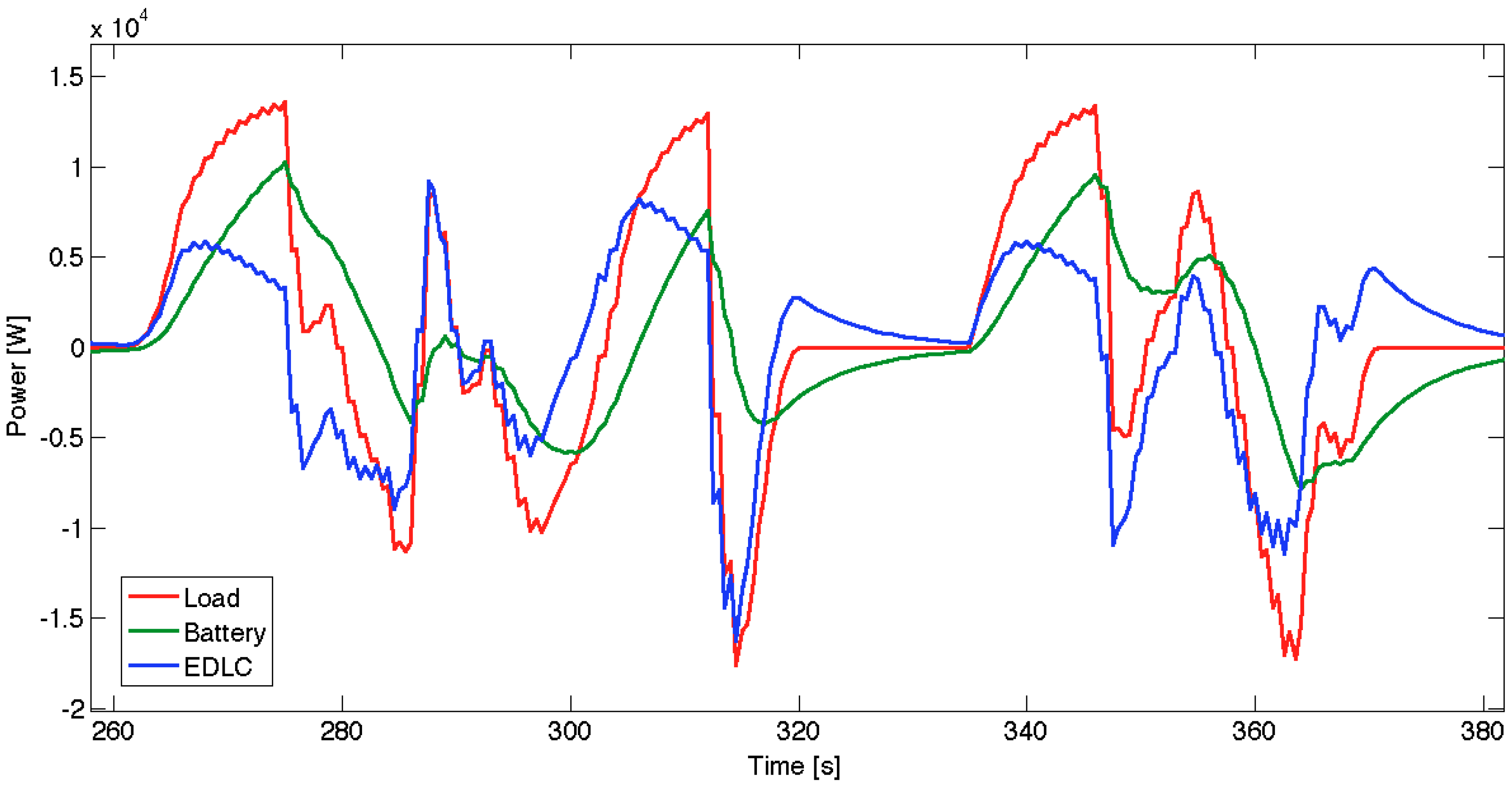
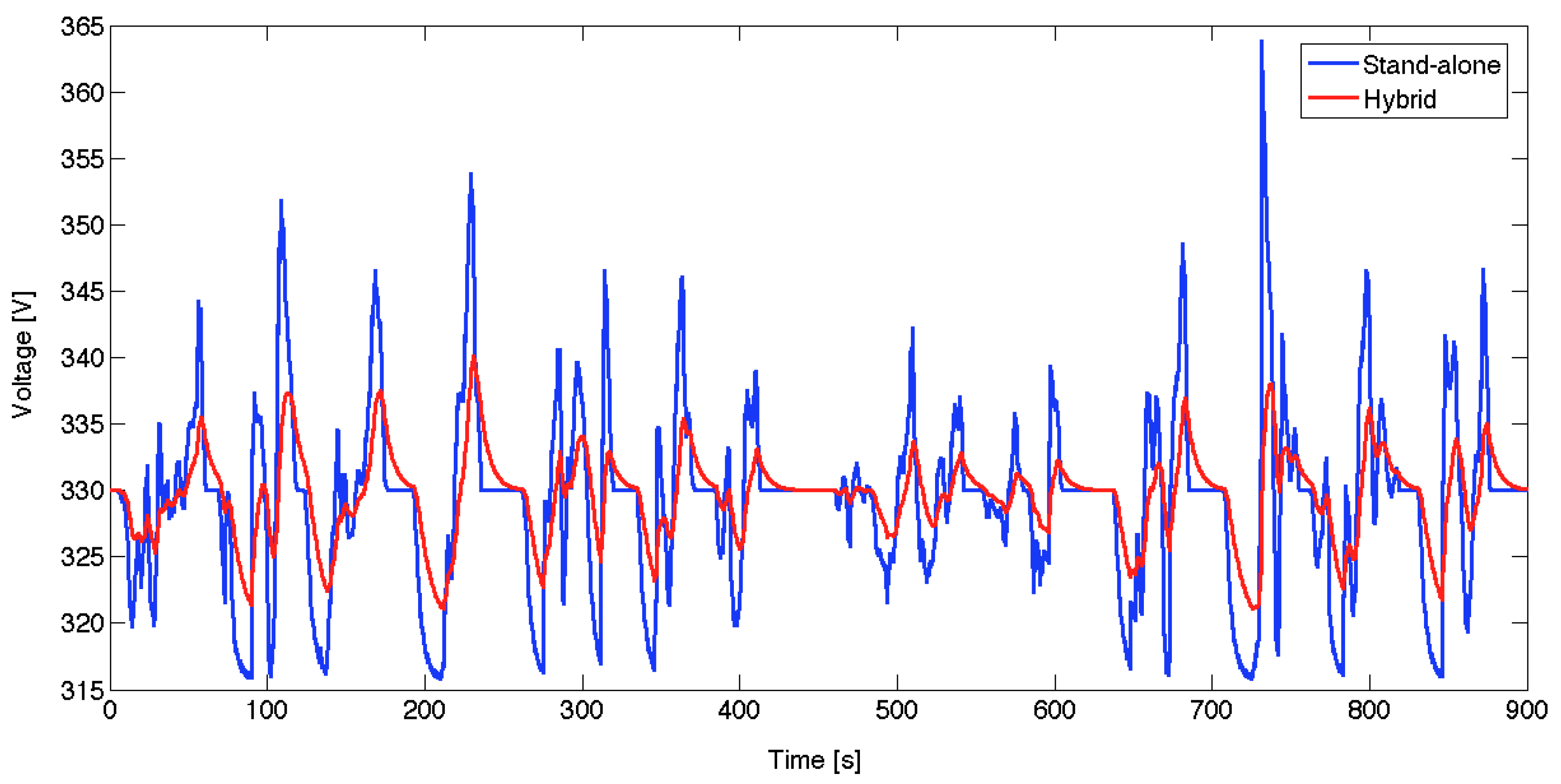

| Driving speed cycle | Range extension due to hybridization (%) | Energy | Efficiency (%) |
|---|---|---|---|
| - | - | Hybrid | Stand-alone |
| NEDC | 5 | 94.1 | 89.5 |
| DUBC | 7 | 91.3 | 84.3 |
| ECE 15 | 4 | 91.9 | 86.5 |
4.2. Hybrid Rechargeable Energy Storage System Architecture with DC-DC Converter
4.2.1. Model
4.2.2. Control Strategies
4.2.3. EDLC Package Sizing
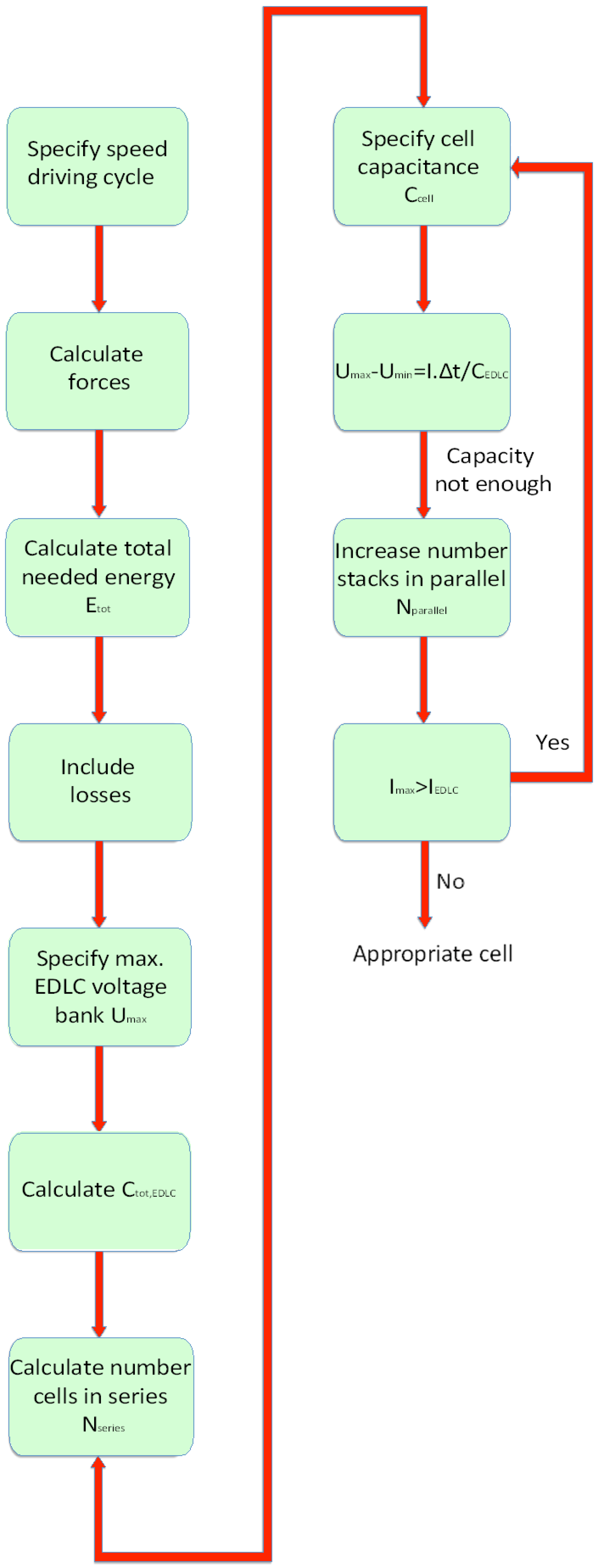
4.2.4. Simulation Results
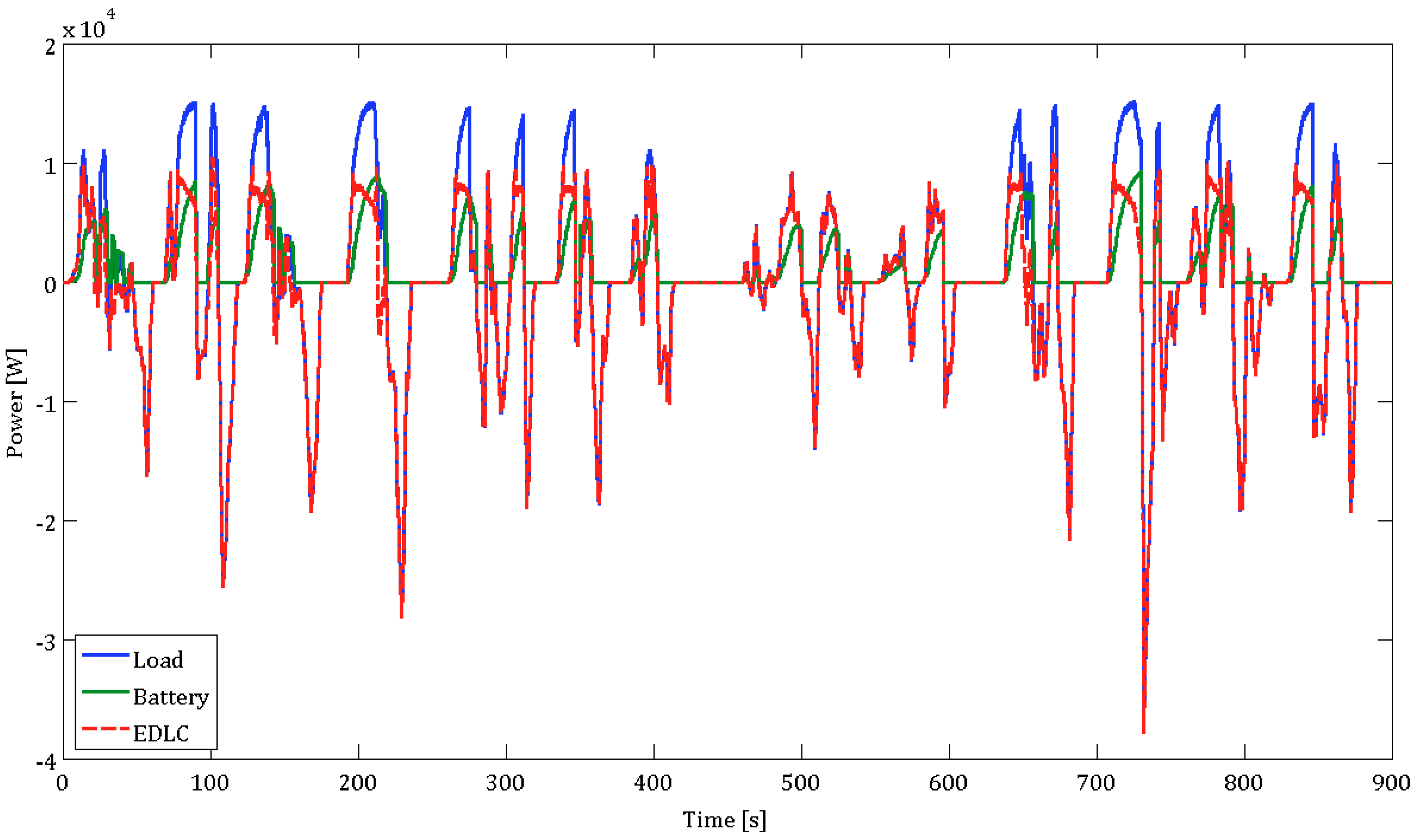
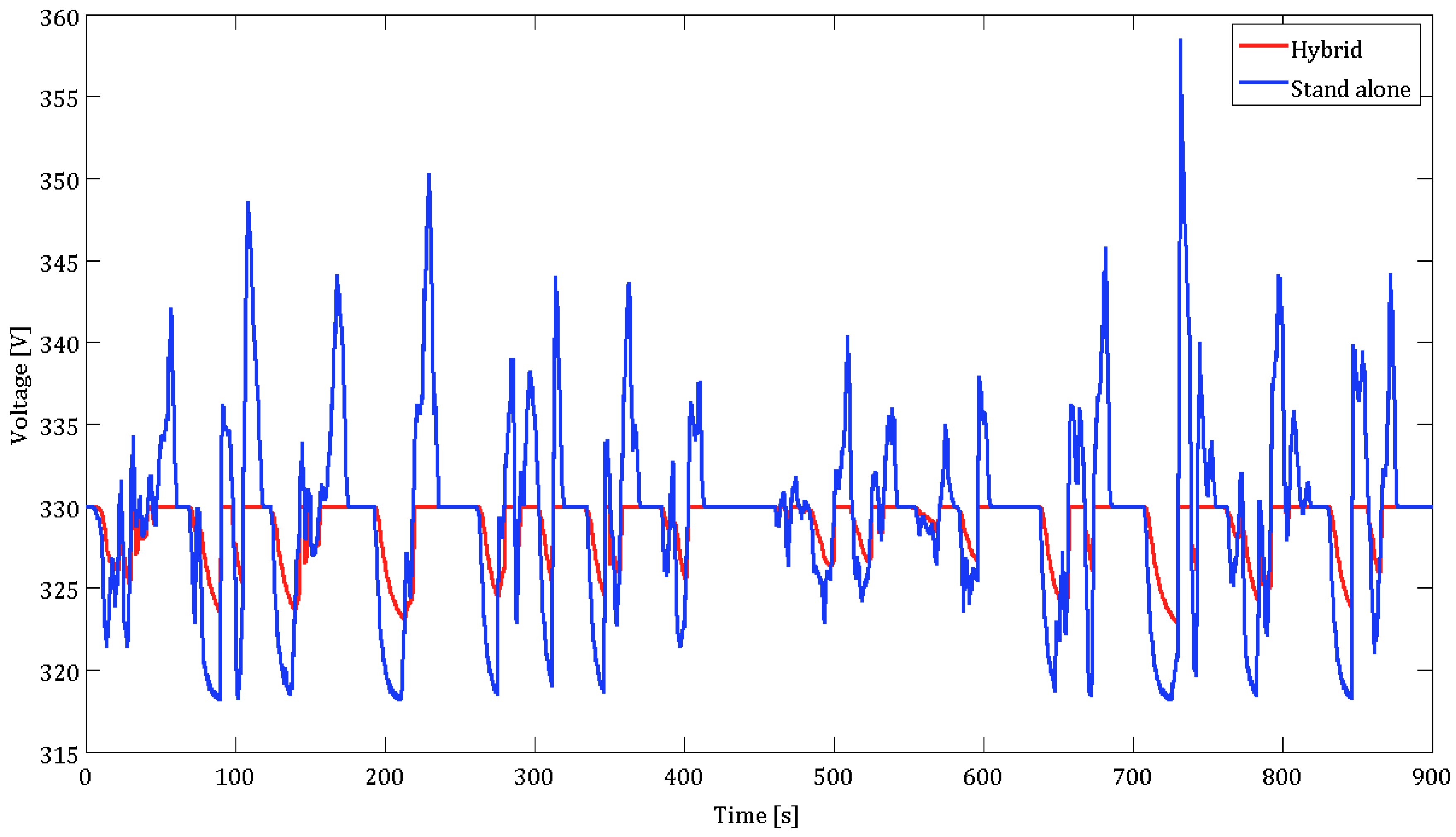
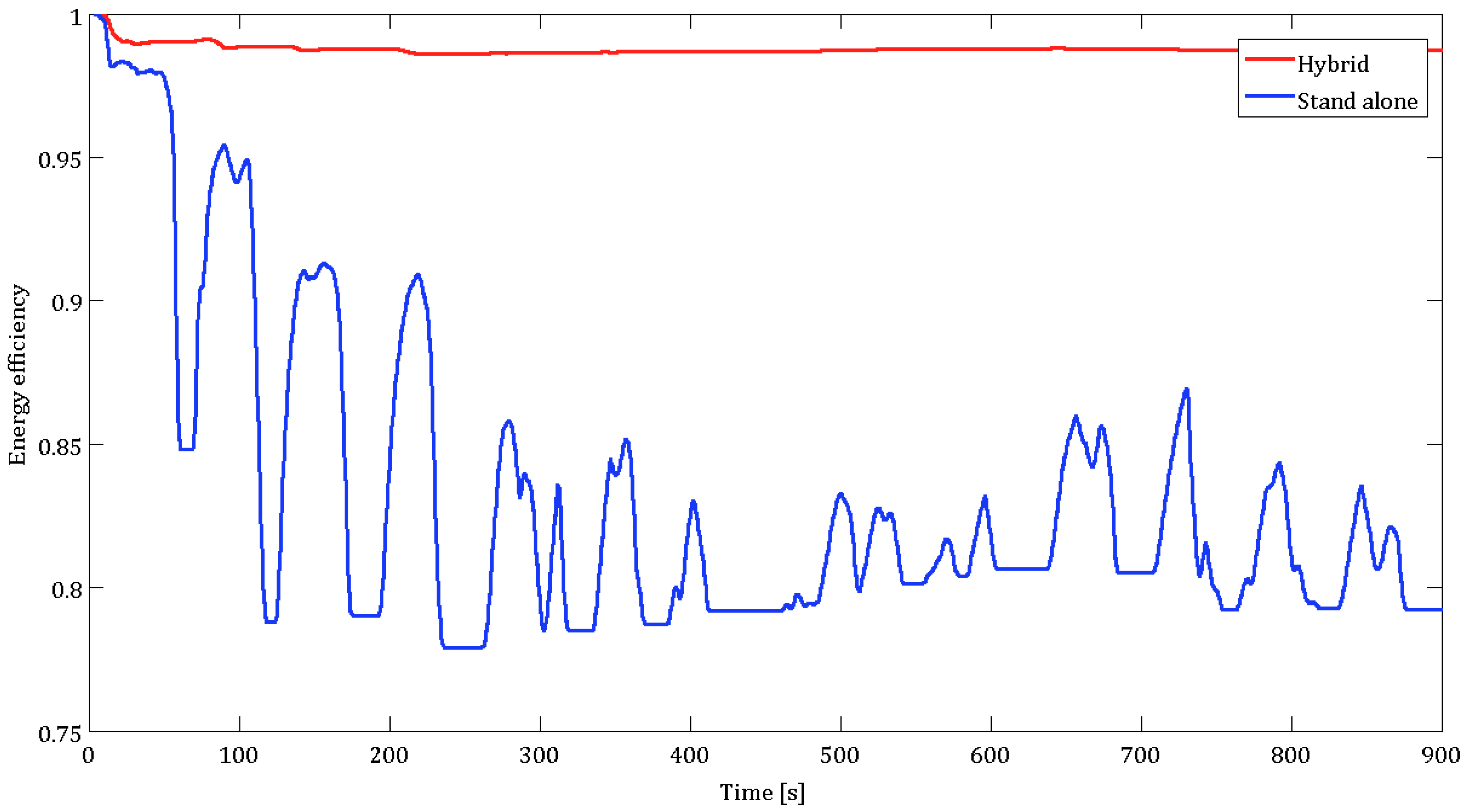

| Driving cycle | Range extension due to hybridization (%) |
|---|---|
| NEDC | 7 |
| DUBC | 8 |
| ECE 15 | 6 |
4.3. Rechargeable Hybrid Energy Storage Systems Assessment
4.3.1. Cost

4.3.2. DC-DC Converter
4.4. Novel Hybrid Topology

4.4.1. Simulation Results

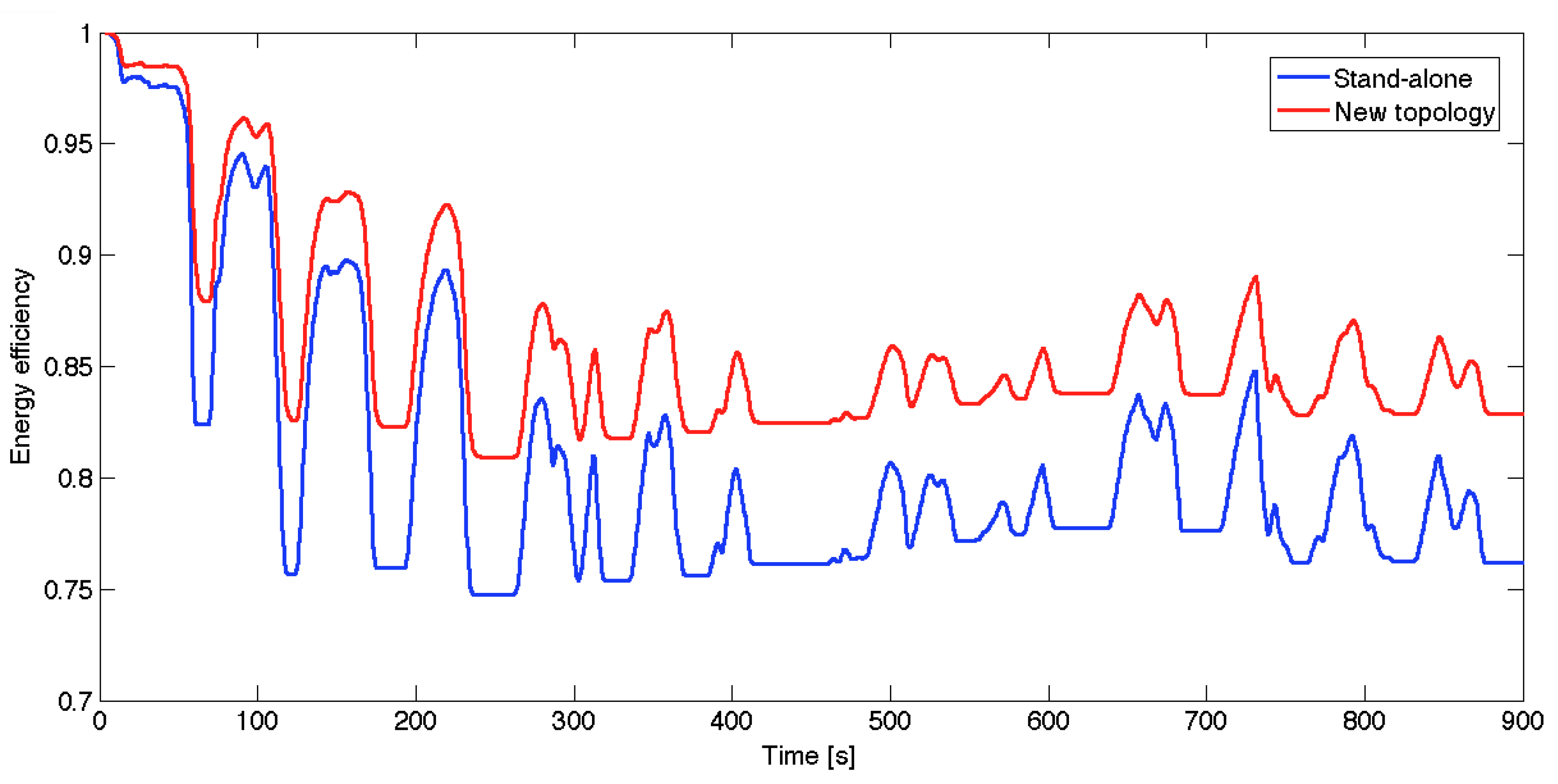
| Characteristics | Value | Unit |
|---|---|---|
| Rated cell voltage | 2.7 | V |
| Rated cell capacitance | 650 | F |
| Internal resistance | 0.8 | mΩ |
| Mass cell | 0.16 | kg |
| Volume | 0.00018 | m3 |
| Number used cells | 200 | - |
| Total mass | 32 | kg |
| Total volume | 0.036 | m3 |
| Driving speed cycle | Range extension due to hybridization (%) | Energy | Efficiency (%) |
|---|---|---|---|
| - | - | Hybrid | Stand-alone |
| NEDC | 10 | 92 | 89.5 |
| DUBC | 12 | 88 | 84.3 |
| ECE 15 | 9.5 | 90 | 86.5 |
4.4.2. Experimental Results
4.4.2.1. Capacity Improvement
| Cell | Rated voltage (V) | Rated capacitance (F) | Internal resistance (mΩ) |
|---|---|---|---|
| NESSCAP | 2.7 | 3000 | 0.29 |
| Maxwell | 2.7 | 450 | 2.4 |
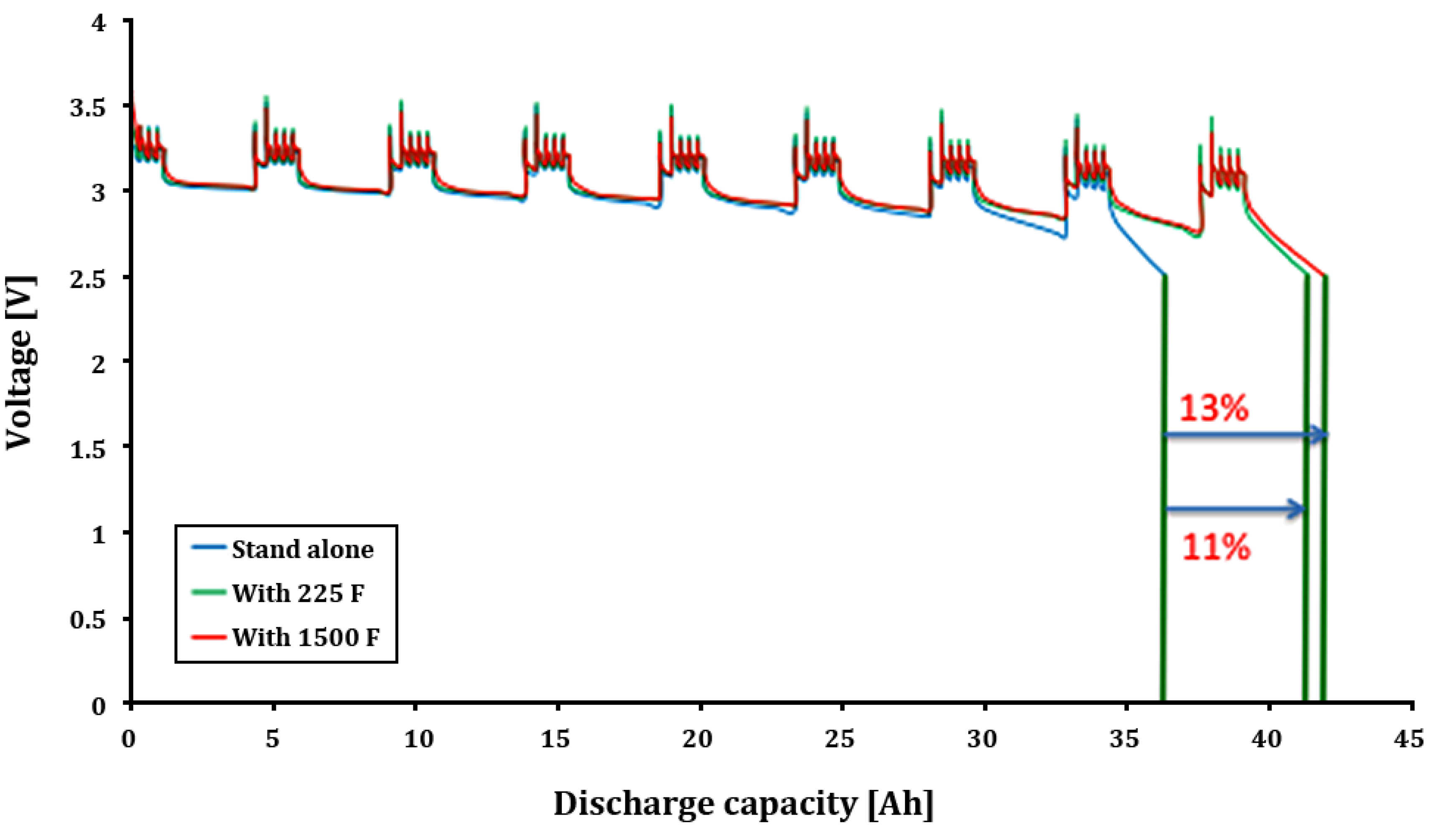

4.4.2.2. Duty Cycle

| Duty ratio (%) | Capacity improvement with 225 F bank (%) | Capacity improvement with 1500 F bank (%) |
|---|---|---|
| 25 | 8 | 12 |
| 50 | 6 | 8 |
| 75 | 5 | 7 |
| 90 | 0.5 | 1 |
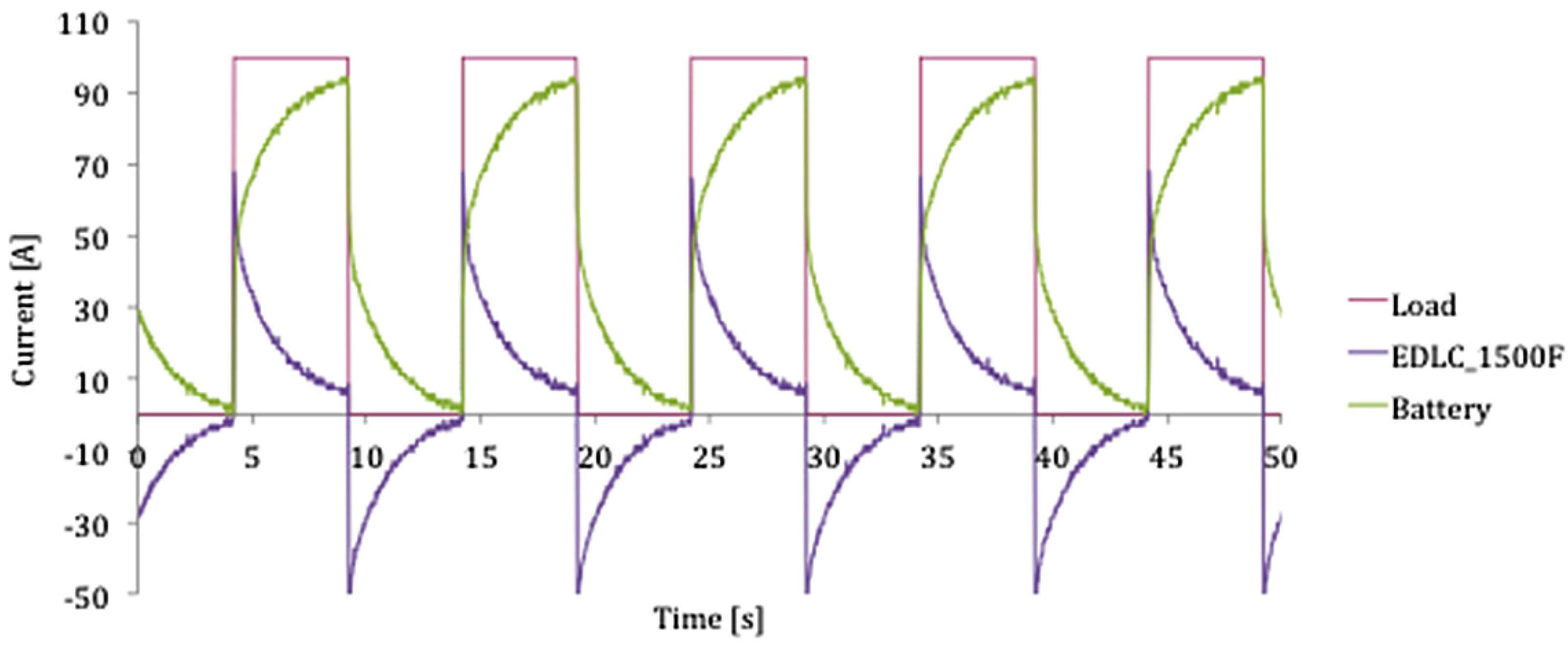
4.4.2.3. Power Improvement
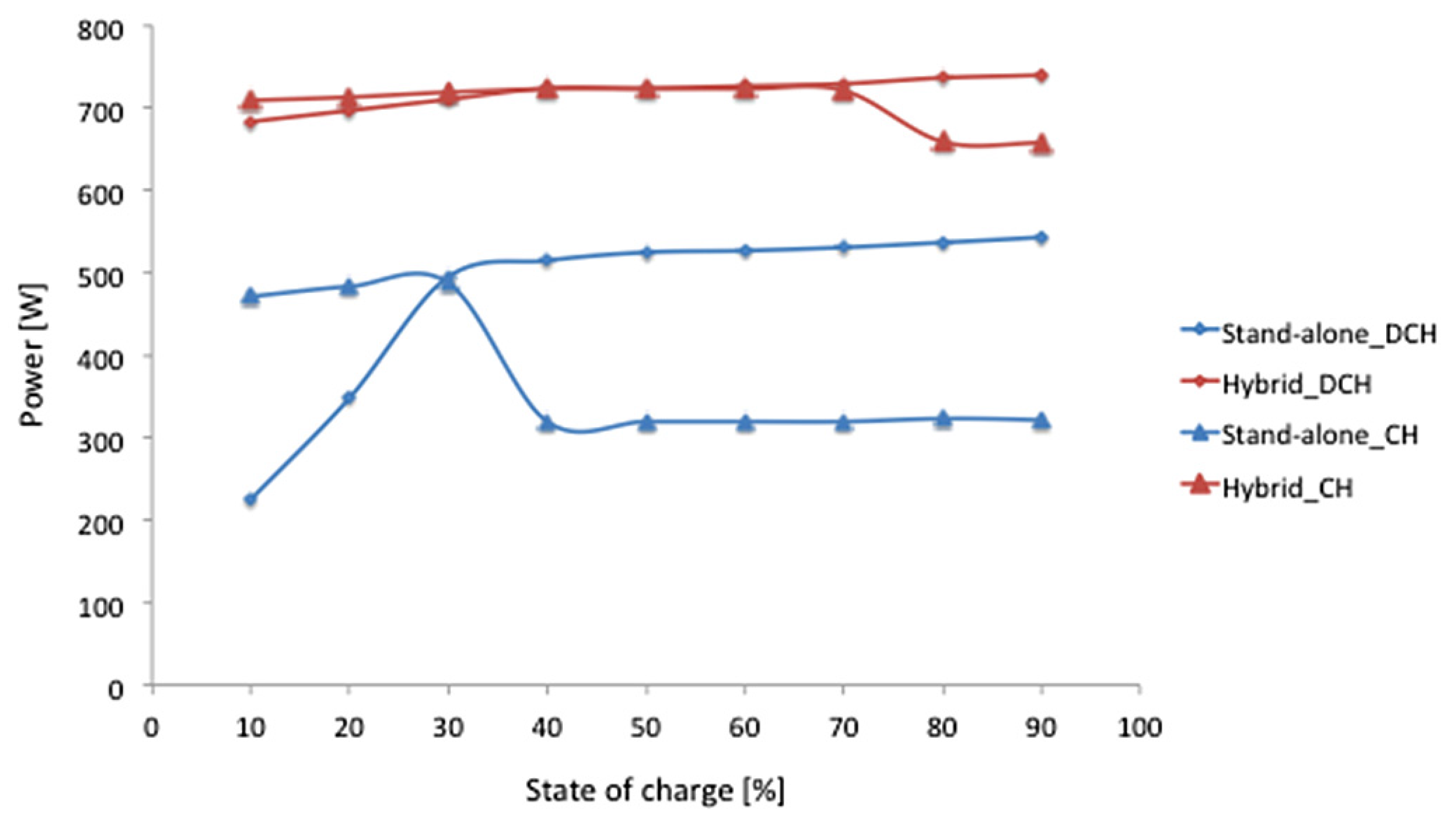
4.4.2.4. Cycle Life Analysis
- Case 1: battery stand-alone;
- Case 2: battery and two EDLC cells (450 F) in series;
- Case 3: battery and two EDLC cells (3,000 F) in series.
| System | Cycle life [cycles] | Cycle life extension [%] |
|---|---|---|
| Stand-alone | 650 | 0 |
| Hybrid (225F EDLC bank) | 850 | 31 |
| Hybrid (1500F EDLC bank) | 910 | 40 |
4.4.3. General Comparison
| Characteristics | BMOD0063-P125-B14 | BCAP0650 | BCAP0310 |
|---|---|---|---|
| Volume (m3) | 0.32 | 0.037 | 0.011 |
| Weight (kg) | 181.5 | 32 | 12 |
| Cost (€) | 10,500-12,000 | 1,300 | 620 |
| Cost DC-DC converter (€) | 2,000 | - | - |
| Characteristics | Passive | Active | New |
|---|---|---|---|
| Performances | + | ++ | + |
| Complexity | ++ | + | +++ |
| Cost | ++ | + | +++ |
| Weight | ++ | + | +++ |
| Volume | ++ | + | +++ |
5. Conclusions
References
- Maggetto, G.; van Mierlo, J. Electric vehicles, hybrid vehicles and fuel cell electric vehicles: State of art and perspectives. Annal. Chim. Sci. Mater. 2001, 26, 9–26. [Google Scholar] [CrossRef]
- Van Mierlo, J.; Maggetto, G.; Lataire, P. Which energy source for road transport in the future? A comparison of battery, hybrid and fuel cell vehicles. J. Energy Conver. Manag. 2006, 47, 2748–2760. [Google Scholar]
- Van den Bossche, P.; Vergels, F.; van Mierlo, J.; Matheys, J.; van Autenboer, W. SUBAT: An assessment of sustainable battery technology. J. Power Sources 2006, 162, 913–919. [Google Scholar] [CrossRef]
- Omar, N.; van Mulders, F.; van Mierlo, J.; van den Bossche, P. Assessment of behaviour of super capacitor-battery system in heavy hybrid lift truck vehicles. J. Asian Electr. Veh. 2009, 7, 1277–1282. [Google Scholar] [CrossRef]
- Abderrahmane, H.; Emmanuel, B. Assessment of real behavior of VHE energy storage system in heavy vehicles. In Proceedings of 3rd European Ele-Drive Transportation Conference (EET-2008), Geneva, Switzerland, 11–13 March 2008.
- Omar, N.; Verbrugge, B.; van den Bossche, P.; van Mierlo, J. Power and life enhancement of battery-electrical double layer capacitor for hybrid electric and charge-depleting plug-in vehicle applications. Electrochim. Acta 2010, 55, 7524–7531. [Google Scholar] [CrossRef]
- Cheng, J.; van Mierlo, J.; van den Bossche, P.; Lataire, P. Super capacitor based energy storage as peak power unit in the applications of hybrid electric vehicles. In Proceedings of International Conference on Power Electronics, Machines and Drives, Dublin, Ireland, 14–17 March 2006.
- Akli, C.R.; Roboam, X.; Sareni, B.; Jeunesse, A. Energy management and sizing of a hybrid locomotive. In Proceedings of European Conference on Power Electronics and Applications, Aalborg, Denmark, 2–5 September 2007.
- Pay, S.; Baghzouz, Y. Effectiveness of battery-supercapacitor combination in electric vehicles. In Proceedings of IEEE Power Technology Conference, Bologna, Italy, 23–26 June 2003.
- Wang, T.; Yu, H.; Zhu, C. Hybrid energy Sources for hybrid electric vehicle propulsion. In Proceedings of IEEE Vehicle Power and Propulsion Conference, Harbin, China, 3–5 September 2008.
- Van Mierlo, J.; Maggetto, G.; van den Bossche, P. Models of energy sources for EV and HEV: fuel cells, batteries, ultra-capacitors, flywheels and engine-generators. J. Power Sources 2004, 28, 76–89. [Google Scholar] [CrossRef]
- Douglas, H. Sizing ultracapacitors for hybrid electric vehicles. In Proceedings of Annual Conference of IEEE on Industrial Electronics Society, Raleigh, NC, USA, 6–10 November 2005.
- Conway, B.E. Electrochemical Supercapacitors Scientific Fundamentals and Technological Applications; Kluwer Academic: Amsterdam, The Netherlands, 1999. [Google Scholar]
- Du Pasquier, A.; Plitz, I.; Menocal, S.; Amatucci, G. A Comparative Study of Li-ion Battery, Supercapacitors and nonaqueous asymmetric hybrid devices for automotive applications. J. Power Sources 2003, 115, 171–178. [Google Scholar] [CrossRef]
- Amatucci, G.G.; Badway, F.; du Pasquier, A. An asymmetric hybrid nonaqueous energy storage cell. J. Electrochem. Soc. 2011, 148, A930–A939. [Google Scholar] [CrossRef]
- Burke, A. Ultracapacitor technologies and applications in hybrid and electric vehicles. Int. J. Energy Res. 2010, 34, 133–151. [Google Scholar] [CrossRef]
- Burke, A. R&D considerations for the performance and applications of electrochemical capacitors. Electrochim. Acta 2007, 53, 1083–1091. [Google Scholar] [CrossRef]
- Burke, A.; Miller, M. Testing of electrochemical capacitors: Capacitance, resistance, energy density, and power capabilities. Electrochim. Acta 2010, 55, 7438–7548. [Google Scholar]
- Omar, N.; Al Sakka, M.; Daowd, M.; Coosemans, T.; van Mierlo, J.; van den Bossche, P. Assessment of hebavior of Active EDLC-Battery system in heavy hybrid charge depleting vehicles. In Proceedings of 4th European Symposium on Super Capacitors & Applications, Bordeaux, France, 21–22 October 2010.
- Omar, N.; Daowd, M.; Mulder, G.; Timmermans, J.M.; van den Bossche, P.; van Mierlo, P.; Pauwels, S. Assessment of performance of lithium iron phophate oxide, Nickel manganese cobalt oxide and nickel cobalt aluminum oxide based cells for using in plug-in battery electric. In Proceedings of the IEEE Vehicle Power and Propulsion Conference, Chicago, IL, USA, 6–9 September 2011.
- Omar, N.; Daowd, M.; van den Bossche, P.; Hegazy, O.; Smekens, J.; Coosemans, T.; van Mierlo, J. Rechargeable energy storage systems for plug-in hybrid electric vehicles—Assessment of electrical characteristics. Energies 2012, 5, 2952–2988. [Google Scholar] [CrossRef]
- Burke, B.; Miller, M. Performance characteristics of lithium-ion batteries of various chemistries for plug-in hybrid vehicles. In Proceedings of the 24th World Battery, Hybrid and Fuel Cell Electric Vehicle Symposium, Stavanger, Norway, 11–12 May 2009.
- Kötz, R.; Carlen, M. Principles and applications of electrochemical capacitors. Electrochim. Acta 2000, 45, 2483–2498. [Google Scholar]
- Omar, N.; Daowd, M.; Hegazy, O.; Al Sakka, M.; Coosemans, T.; van den Bossche, P.; van Mierlo, J. Assessment of lithium-ion capacitor for using in battery electric vehicle and hybrid electric vehicle applications. Electrochim. Acta 2012, in press. [Google Scholar]
- Tang, Y. On the feasibility of hybrid battery/ultracapacitor energy storage systems for next generation shipboard power systems. In Proceedings of Vehicle Power and Propulsion Conference, Lille, France, 1–3 September 2010.
- Wipke, K.; Cuddy, M.; Bharathan, D.; Burch, S.; Johnson, V.; Markel, A.; Sprik, S. Advisor 2.0: A Second-Generation Advanced Vehicle Simulator for Systems Analysis; Technical Report Contract No. DE-AC369-8-GO10337; National Renewable Energy Laboratory (NREL): Golden, CO, USA, 1999. [Google Scholar]
- Cole, G.H. Simplev: A Simple Electric Vehicle Simulation Program Version 1.0; Technical Report Contract No. DE-AC077-6ID01570; U.S. Department of Energy Field Office: Albuquerque, NM, USA, 1991.
- Argonne National Laboratory. Powertrain Systems Analysis Toolkit—A Flexible, Reusable Model for Simulating Advanced Vehicles; Argonne National Laboratory: Argonne, IL, USA, 2012. Available online: http://web.anl.gov/techtransfer/pdf/PSAT.pdf (accessed on 6 November 2012).
- Argonne National Laboratory. PSAT Training. Part 1—PSAT Overview; Argonne National Laboratory: Argonne, IL, USA, 2012. Available online: http://www.transportation.anl.gov/pdfs/HV/412.pdf (accessed on 6 November 2012).
- Van Mierlo, J. Simulation Software for Comparison and Design of Electric, Hybrid Electric and Internal Combustion Vehicles with Respect to Energy, Emissions and Performances. Ph.D. Dissertation, Vrije Universiteit Brussel, Brussel, Belgium, 2000. [Google Scholar]
- Mobelica. Available online: https://www.modelica.org/education/educationalmaterial/lecturematerial/english/modelicaoverview.pdf (accessed on 6 November 2012).
- Fan, B.S.M. Modeling and Simulation of a Hybrid Electric Vehicle Using Matlab/Simulink and Adams. Master’s Thesis, University of Waterloo, Waterloo, Canada, 2007. [Google Scholar]
- Idaho National Laboratory. Battery Test Manual for Plug-In Hybrid Electric Vehicles; U.S. Department Secretary of Energy: Idaho Falls, ID, USA, 2003.
- Shi, L.; Crow, M.L. Comparison of ultracapacitor electric circuit models. In Proceedings of Power and Energy Society General Meeting—Conversion and Delivery of Electrical Energy in the 21st Century, Pittsburg, PA, USA, 20–24 July 2008.
- Karden, S.B.; Kok, D.; de Doncker, R.W. Modeling the dynamic behavior of supercapacitors using impedance spectroscopy. In Proceedings of IEEE Thirty-Sixth IAS Annual Meeting—Industry Applications Conference, Chicago, IL, USA, 30 September–4 October 2001.
- Zubieta, L.; Boner, R. Characterization of double-layer capacitors for power electronics applications. IEEE Trans. Ind. Appl. 2000, 36, 199–205. [Google Scholar] [CrossRef]
- Barrero, R.; Coosemans, T.; van Mierlo, J. Hybrid buses: Defining the power flow management strategy and energy storage system needs. In Proceedings of the 24th International Battery, Hybrid and Fuel Cell Electric Vehicle Symposium (EVS-24), Stavanger, Norway, 13–16 May 2009.
- Al Sakka, M.; van Mierlo, J.; Gualous, H.; Lataire, P. Comparison of 30 kW DC/DC converter topologies interfaces for fuel cell in hybrid electric vehicle. In Proceedings of European Conference on Power Electronics and Applications, Barcelona, Spain, 8–10 September 2009.
- Nissan Leaf. Available online: http://en.wikipedia.org/wiki/nissan-leaf (accessed on 6 November 2012).
- Angers, P. Supercapacitors—Do they improve battery life? In Proceedings of the 21st International Battery, Hybrid and Fuel Cell Electric Vehicle Symposium (EVS-21), Monaco, France, 4–7 December 2005.
- Verbrugge, M.; Liu, P.; Soukiazian, S.; Ying, R. Electrochemical energy storage systems and range-extended electric vehicles. In Proceedings of the 25th International Battery Seminar Exhibit, Fort Lauderdale, FL, USA, 11–14 March 2008.
- Sikha, G.; Popov, B.N. Performance optimization of a battery–capacitor hybrid system. J. Power Sources 2004, 134, 130–138. [Google Scholar] [CrossRef]
- Dougal, R.A.; Liu, S.; White, R.E. Power and life extension of battery-ultracapacitor hybrids. IEEE Trans. Compon. Packag. Technol. 2002, 24, 120–131. [Google Scholar] [CrossRef]
- Aharon, I.; Kuperman, A. Design of semi-active battery-ultracapacitor hybrids, electrical and electronics engineers in Israel (IEEEI). In Proceedings of IEEE 26th Convention Conference, Eliat, Israel, 17–20 November 2010.
- Kuperman, A.; Aharon, I. Battery-ultracapacitor hybrids for pulsed current loads: A review. J. Power Sources 2011, 15, 1005–1023. [Google Scholar]
- European Batteries. Available online: http://www.europeanbatteries.com/solutions/brochures (accessed on 6 November 2012).
- Carter, R.; Cruden, A. Strategies for control of a battery/supercapacitor system in an electric vehicle. In Proceedings of International Symposium on Power Electronics, Electrical Drives, Ischia, Italy, 11–13 June 2008.
- Hegazy, O.; van Mierlo, J.; Lataire, P. Analysis, modeling and implementation of multidevice interleaved DC/DC converter for fuel cell hybrid vehicles. IEEE Trans. Power Electron. 2012, 27, 4445–4458. [Google Scholar] [CrossRef]
- Hegazy, O.; van Mierlo, J.; Lataire, P. Analysis, control and comparison of DC/DC boost converter topologies for fuel cell hybrid electric vehicle applications. In Proceedings of 14th European Conference on Power Electronics and Applications, Stavanger, Norway, 29 August–2 September 2011.
- Guidi, G.; Undeland, T.; Hori, Y. Effectiveness of supercapacitors as power-assist in pure EV using a sodium nickel chloride battery as main energy storage. In Proceedings of Proceedings of the 24th International Battery, Hybrid and Fuel Cell Electric Vehicle Symposium (EVS-24), Stavanger, Norway, 13–16 May 2009.
- Aanderman, M.; Kalhammer, F.R.; MacArthur, D. Advanced Batteries for Electric Vehicles: An Assessment of Performance, Cost, and Availability; Technical Report; State of California Air Resources Board: Sacramento, CA, USA, 2000. [Google Scholar]
- Cheah, T.; Heywood, J. The cost of vehicle electrification: A literature review. In Proceedings of MIT Energy Initiative Symposium, Boston, MA, USA, April 2010.
- 4ESYS. Available online: http://www.4esys.com (accessed on 6 November 2012).
- Maxwell. Available online: http://www.maxwell.com (accessed on 6 November 2012).
- Barrero, R.; van Mierlo, J.; Lataire, P. Design of bi-directional series resonant converter as peak power unit in hybrid electric vehicles. In Proceedings of International Conference of Industrial Technology, Vina del Mar, Chile, 14–17 March 2010.
- Chandrasekaran, R.; Sikha, G.; Popov, B.N. Capacity fade analysis of a battery/super capacitor hybrid and a battery under pulse loads—Full cell studies. J. Appl. Electrochem. 2005, 35, 1005–1023. [Google Scholar] [CrossRef]
© 2012 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Omar, N.; Daowd, M.; Hegazy, O.; Bossche, P.V.d.; Coosemans, T.; Mierlo, J.V. Electrical Double-Layer Capacitors in Hybrid Topologies —Assessment and Evaluation of Their Performance. Energies 2012, 5, 4533-4568. https://doi.org/10.3390/en5114533
Omar N, Daowd M, Hegazy O, Bossche PVd, Coosemans T, Mierlo JV. Electrical Double-Layer Capacitors in Hybrid Topologies —Assessment and Evaluation of Their Performance. Energies. 2012; 5(11):4533-4568. https://doi.org/10.3390/en5114533
Chicago/Turabian StyleOmar, Noshin, Mohamed Daowd, Omar Hegazy, Peter Van den Bossche, Thierry Coosemans, and Joeri Van Mierlo. 2012. "Electrical Double-Layer Capacitors in Hybrid Topologies —Assessment and Evaluation of Their Performance" Energies 5, no. 11: 4533-4568. https://doi.org/10.3390/en5114533
APA StyleOmar, N., Daowd, M., Hegazy, O., Bossche, P. V. d., Coosemans, T., & Mierlo, J. V. (2012). Electrical Double-Layer Capacitors in Hybrid Topologies —Assessment and Evaluation of Their Performance. Energies, 5(11), 4533-4568. https://doi.org/10.3390/en5114533




