Impact of Azimuth Angle on Photovoltaic Energy Production: Experimental Analysis in Loja, Ecuador
Abstract
1. Introduction
2. Materials and Methods
2.1. Study Location
2.2. Solar Photovoltaic Energy Production Systems
- Forty-five Jinko Solar panels, model Tiger Pro JKM405M-54HL4 (Jinko Solar Co., Ltd., Shanghai, China), each with a capacity of 405 watts.
- One inverter per set of solar panels, Fronius brand, model Fronius Primo 15.0-1 208-240 WLAN/LAN/web server 4,210,078,800 (Fronius International GmbH, Pettenbach, Austria).
- Electrical protections, cabling, equipment distances, and a tilt angle of 10 degrees were kept constant across all three systems.
- The variable of interest in this study was the orientation of the solar panels. Sets 1 (W1) and 2 (W2) were oriented to the west, while set 3 (E) was oriented to the east. All panels were installed in a landscape configuration with a fixed tilt angle of 10 degrees to optimize energy capture in this equatorial region. Figure 2 shows the arrangement of the solar panels in the experimental setup.
2.3. Data and Measurement Tools
2.4. Experimental Design
2.5. Statistical Analysis Methods
- Shapiro–Wilk test: This test was applied to examine the normality of the energy production distributions from each panel. The resulting p-values indicated that the distributions were not normally distributed [34].
- Levene’s test: This test was used to assess the homogeneity of variances between the systems. The results showed no significant differences in variances between the systems with similar orientations [35].
- Central limit theorem: Despite the observed non-normality, the large sample size allowed for the sample mean distribution to approximate normality, relaxing the normality assumption in the statistical inference [36].
- One-way ANOVA: This was performed to determine if there were significant differences in energy production between the different solar panel systems. The analysis revealed no significant differences in the mean energy production among the systems [37].
- Kruskal–Wallis test: Implemented as a non-parametric alternative, this test confirmed the absence of significant differences in the median energy production among the groups [38].
- Randomized block design with replication and two-way ANOVA: This analysis was conducted to account for seasonal variations and azimuth angle. It allowed for the evaluation of both main effects and interactions [37].
- Bonferroni adjustment: This was applied to the p-values obtained from the monthly Kruskal–Wallis analysis to control for Type I errors due to multiple comparisons [39].
2.6. Data Analysis Procedures
3. Results
3.1. Solar Energy Production
3.2. Energy Production Estimation
3.3. Uncertainty Analysis
4. Discussion
4.1. Descriptive Analysis
4.2. Influence of Azimuth Angle on Energy Production
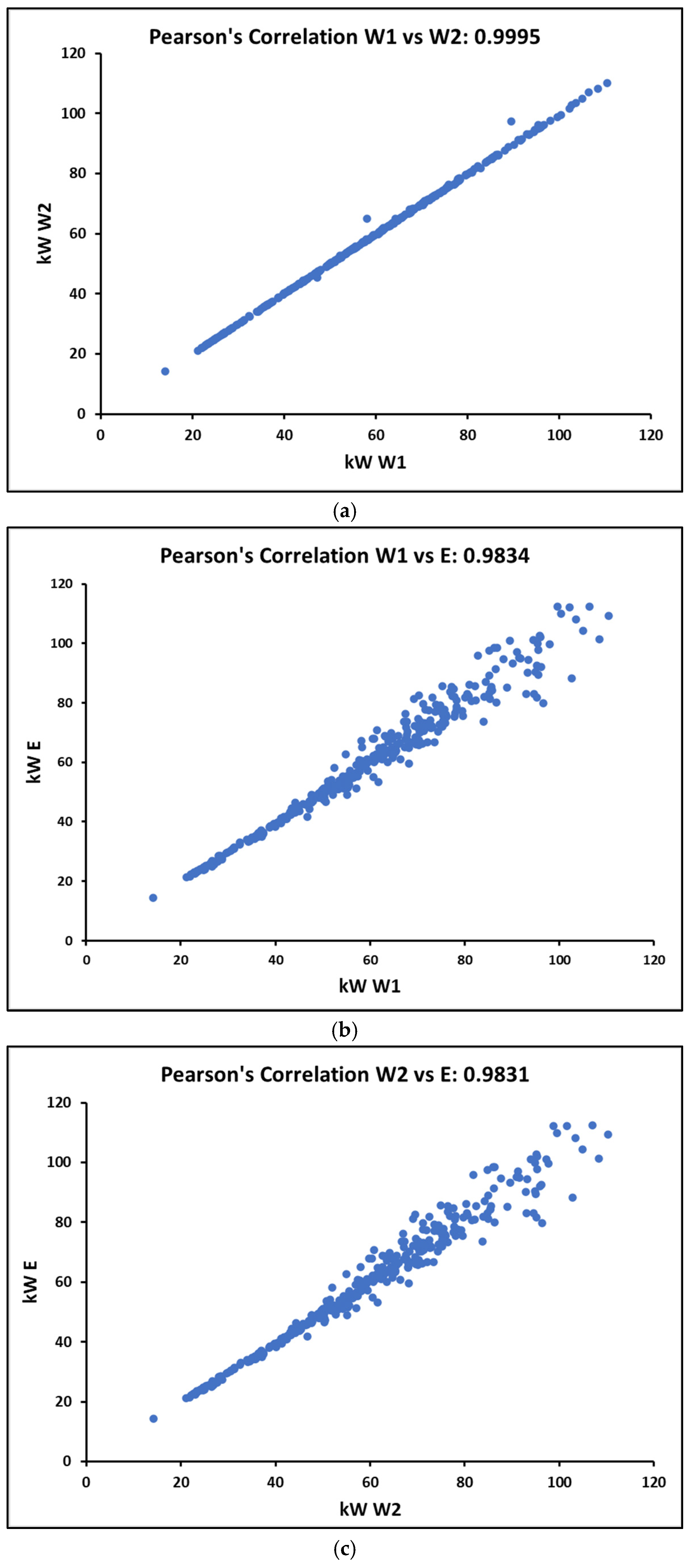
4.2.1. Correlation Evaluation
4.2.2. One-Way ANOVA
4.2.3. Randomized Block Design with Replication
- System: The absence of statistically significant differences in energy production between the solar panel systems (F = 0.028, p = 0.973) reinforces our initial conclusion that the specific orientation of the panels does not significantly influence their energy performance.
- YearMonth: The significant monthly variation in energy production (F = 31.276, p < 2 × 10−16) highlights the impact of seasonality, an expected result given the sun-dependent nature of solar energy.
- System–YearMonth interaction: The lack of significant interaction (F = 0.215, p = 1.000) suggests that seasonal differences in energy production do not depend significantly on the orientation of the solar panel systems. In other words, while energy production varies by month, this variation is consistently similar across the different systems.
4.3. Uncertainty Calculation
4.4. Comparison of Results of This Study with Others Reported in the Literature
4.5. Opportunities for Future Research
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Izam, N.S.M.N.; Itam, Z.; Sing, W.L.; Syamsir, A. Sustainable Development Perspectives of Solar Energy Technologies with Focus on Solar Photovoltaic—A Review. Energies 2022, 15, 2790. [Google Scholar] [CrossRef]
- Middelhauve, L.; Baldi, F.; Stadler, P.; Maréchal, F. Grid-Aware Layout of Photovoltaic Panels in Sustainable Building Energy Systems. Front. Energy Res. 2021, 8, 573290. [Google Scholar] [CrossRef]
- Liu, W.; Wang, J.; Wang, Y. Building solar integrated energy systems considering power and heat coordination: Optimization and evaluation. Sol. Energy 2024, 279, 112821. [Google Scholar] [CrossRef]
- Maka, A.O.M.; Alabid, J.M. Solar energy technology and its roles in sustainable development. Clean Energy 2022, 6, 476–483. [Google Scholar] [CrossRef]
- Bassam, N. El Solar energy. In Distributed Renewable Energies for Off-Grid Communities: Empowering a Sustainable, Competitive, and Secure Twenty-First Century; Elsevier: Amsterdam, The Netherlands, 2021; pp. 123–147. ISBN 9780128216057. [Google Scholar]
- Dambhare, M.V.; Butey, B.; Moharil, S.V. Solar photovoltaic technology: A review of different types of solar cells and its future trends. J. Phys. Conf. Ser. 2021, 1913, 012053. [Google Scholar] [CrossRef]
- Tapia, M.; Ramos, L.; Heinemann, D.; Zondervan, E. Power to the city: Assessing the rooftop solar photovoltaic potential in multiple cities of Ecuador. Phys. Sci. Rev. 2023, 8, 2285–2319. [Google Scholar] [CrossRef]
- Icaza, D.; Borge-Diez, D.; Galindo, S.P. Analysis and proposal of energy planning and renewable energy plans in South America: Case study of Ecuador. Renew. Energy 2022, 182, 314–342. [Google Scholar] [CrossRef]
- Barragán-Escandón, A.; Jara-Nieves, D.; Romero-Fajardoc, I.; Zalamea-Leónesteban, E.F.; Serrano-Guerrero, X. Barriers to renewable energy expansion: Ecuador as a case study. Energy Strateg. Rev. 2022, 43, 100903. [Google Scholar] [CrossRef]
- Benalcazar, P.; Lara, J.; Samper, M. Distributed Photovoltaic Generation in Ecuador: Economic Analysis and Incentives Mechanisms. IEEE Lat. Am. Trans. 2020, 18, 564–572. [Google Scholar] [CrossRef]
- Phadnis, N.; Yang, R.J.; Wijeratne, P.U.; Zhao, H.; Liu, C. The Impact of Solar PV Design Tilt and Orientation on Project Values. Smart Innov. Syst. Technol. 2019, 131, 301–310. [Google Scholar] [CrossRef]
- Danu, A.; Tanasiev, V.; Ionescu, C.; Badea, A. Influence of tilt and orientation angle of the PV panels on high-energy production in Romanian climate conditions during cold season. In Proceedings of the 2015 IEEE 15th International Conference on Environment and Electrical Engineering, EEEIC 2015-Conference Proceedings, Rome, Italy, 10–13 June 2015; Institute of Electrical and Electronics Engineers Inc.: Piscataway, NJ, USA, 2015; pp. 1599–1604. [Google Scholar]
- Naraghi, M.H.; Atefi, E. Optimum Solar Panel Orientation and Performance: A Climatic Data-Driven Metaheuristic Approach. Energies 2022, 15, 624. [Google Scholar] [CrossRef]
- Oufettoul, H.; Lamdihine, N.; Motahhir, S.; Lamrini, N.; Abdelmoula, I.A.; Aniba, G. Comparative Performance Analysis of PV Module Positions in a Solar PV Array Under Partial Shading Conditions. IEEE Access 2023, 11, 12176–12194. [Google Scholar] [CrossRef]
- Yadav, S.; Panda, S.K.; Hachem-Vermette, C. Optimum azimuth and inclination angle of BIPV panel owing to different factors influencing the shadow of adjacent building. Renew. Energy 2020, 162, 381–396. [Google Scholar] [CrossRef]
- Guo, S.-t.; Liu, J.; Qian, W.; Zhu, W.-h.; Zhang, C.-y. A review of quantum chemical methods for treating energetic molecules. Energ. Mater. Front. 2021, 2, 292–305. [Google Scholar] [CrossRef]
- Peng, H.Y.; Shen, Z.; Liu, H.J.; Dai, S.F. Wind loads on rooftop solar photovoltaic panels oriented with varying azimuth angles: A comprehensive wind tunnel analysis. J. Build. Eng. 2024, 92, 109747. [Google Scholar] [CrossRef]
- Kallioğlu, M.A.; Avcı, A.S.; Sharma, A.; Khargotra, R.; Singh, T. Solar collector tilt angle optimization for agrivoltaic systems. Case Stud. Therm. Eng. 2024, 54, 103998. [Google Scholar] [CrossRef]
- Prunier, Y.; Chuet, D.; Nicolay, S.; Hamon, G.; Darnon, M. Optimization of photovoltaic panel tilt angle for short periods of time or multiple reorientations. Energy Convers. Manag. X 2023, 20, 100417. [Google Scholar] [CrossRef]
- Alam, S.; Qadeer, A.; Afazal, M. Determination of the optimum tilt-angles for solar panels in Indian climates: A new approach. Comput. Electr. Eng. 2024, 119, 109638. [Google Scholar] [CrossRef]
- Khatib, A.T.; Samiji, M.E.; Mlyuka, N.R. Optimum Solar Collector’s North-South Tilt Angles for Dar es Salaam and their Influence on Energy Collection. Clean. Eng. Technol. 2024, 21, 100778. [Google Scholar] [CrossRef]
- Mukisa, N.; Zamora, R. Optimal tilt angle for solar photovoltaic modules on pitched rooftops: A case of low latitude equatorial region. Sustain. Energy Technol. Assess. 2022, 50, 101821. [Google Scholar] [CrossRef]
- Mahmud, Z.; Kurtz, S. Effect of solar panel orientation and EV charging profile on grid design. Renew. Energy 2024, 231, 120923. [Google Scholar] [CrossRef]
- Arslan, M.; Çunkaş, M. An experimental study on determination of optimal tilt and orientation angles in photovoltaic systems. J. Eng. Res. 2024; in press. [Google Scholar] [CrossRef]
- AL-Rousan, N.; AL-Najjar, H. Optimized deep neural network to estimate orientation angles for solar photovoltaics intelligent systems. Clean. Eng. Technol. 2024, 20, 100754. [Google Scholar] [CrossRef]
- Kerr, J.; Moores, J.E.; Smith, C.L. An improved model for available solar energy on Mars: Optimizing solar panel orientation to assess potential spacecraft landing sites. Adv. Space Res. 2023, 72, 1431–1447. [Google Scholar] [CrossRef]
- Kabeel, A.E.; Khelifa, A.; El Hadi Attia, M.; Abdelgaied, M.; Arıcı, M.; Abdel-Aziz, M.M. Optimal design and orientation of cooling technology for photovoltaic Plants: A comparative simulation study. Sol. Energy 2024, 269, 112362. [Google Scholar] [CrossRef]
- Bouguerra, S.; Yaiche, M.R.; Gassab, O.; Sangwongwanich, A.; Blaabjerg, F. The Impact of PV Panel Positioning and Degradation on the PV Inverter Lifetime and Reliability. IEEE J. Emerg. Sel. Top. Power Electron. 2021, 9, 3114–3126. [Google Scholar] [CrossRef]
- Litjens, G.B.M.A.; Worrell, E.; van Sark, W.G.J.H.M. Influence of demand patterns on the optimal orientation of photovoltaic systems. Sol. Energy 2017, 155, 1002–1014. [Google Scholar] [CrossRef]
- Li, M.; Liu, Y.; Li, J.; Li, F.; An, Y.; Gao, X. Optimizing sustainable energy integration: A novel approach using concentrated solar plant and hybrid power supply. Electr. Power Syst. Res. 2024, 237, 111050. [Google Scholar] [CrossRef]
- Sadaq, S.I.; Mehdi, S.N.; Mohinoddin, M. Experimental analysis on solar photovoltaic (SPV) panel for diverse slope angles at different wind speeds. Mater. Today Proc. 2023; in press. [Google Scholar] [CrossRef]
- Żurakowska-Sawa, J.; Gromada, A.; Trocewicz, A.; Wojciechowska, A.; Wysokiński, M.; Zielińska, A. Photovoltaic Farms: Economic Efficiency of Investments in South-East Poland. Energies 2025, 18, 170. [Google Scholar] [CrossRef]
- Alvarado, G.; Rodríguez, F.M.; Pacheco, A.; Burgueño, J.; Crossa, J.; Vargas, M.; Pérez-Rodríguez, P.; Lopez-Cruz, M.A. META-R: A software to analyze data from multi-environment plant breeding trials. Crop J. 2020, 8, 745–756. [Google Scholar] [CrossRef]
- Khatun, N. Applications of Normality Test in Statistical Analysis. Open J. Stat. 2021, 11, 113–122. [Google Scholar] [CrossRef]
- Wang, Y.; Tang, M.; Wang, P.; Liu, B.; Tian, R. The Levene test based-leakage assessment. Integration 2022, 87, 182–193. [Google Scholar] [CrossRef]
- Zhang, X.; Astivia, O.L.O.; Kroc, E.; Zumbo, B.D. How to Think Clearly About the Central Limit Theorem. Psychol. Methods 2022, 28, 1427–1445. [Google Scholar] [CrossRef]
- Okoye, K.; Hosseini, S. Analysis of Variance (ANOVA) in R: One-Way and Two-Way ANOVA. In R Program; Springer: Singapore, 2024; pp. 187–209. [Google Scholar] [CrossRef]
- Okoye, K.; Hosseini, S. Mann–Whitney U Test and Kruskal–Wallis H Test Statistics in R. In R Program; Springer: Singapore, 2024; pp. 225–246. [Google Scholar] [CrossRef]
- Kumbure, M.M.; Luukka, P.; Collan, M. A new fuzzy k-nearest neighbor classifier based on the Bonferroni mean. Pattern Recognit. Lett. 2020, 140, 172–178. [Google Scholar] [CrossRef]
- Abdullah, G.; Nishimura, H. Techno-Economic Performance Analysis of a 40.1 kWp Grid-Connected Photovoltaic (GCPV) System after Eight Years of Energy Generation: A Case Study for Tochigi, Japan. Sustainability 2021, 13, 7680. [Google Scholar] [CrossRef]
- PraveenKumar, S.; Kumar, A. Thermodynamic, environmental and economic analysis of solar photovoltaic panels using aluminium reflectors and latent heat storage units: An experimental investigation using passive cooling approach. J. Energy Storage 2025, 112, 115487. [Google Scholar] [CrossRef]
- Hamdan, M.; Abdelhafez, E.; Ajib, S.; Sukkariyh, M. Improving Thermal Energy Storage in Solar Collectors: A Study of Aluminum Oxide Nanoparticles and Flow Rate Optimization. Energies 2024, 17, 276. [Google Scholar] [CrossRef]
- Selvi, S.; Mohanraj, M.; Duraipandy, P.; Kaliappan, S.; Natrayan, L.; Vinayagam, N. Optimization of Solar Panel Orientation for Maximum Energy Efficiency. In Proceedings of the 2023 4th International Conference on Smart Electronics and Communication (ICOSEC), Trichy, India, 20–22 September 2023; pp. 159–162. [Google Scholar] [CrossRef]
- Apaolaza-Pagoaga, X.; Carrillo-Andrés, A.; Jiménez-Navarro, J.P.; Ruivo, C.R. Experimental evaluation of the performance of new Copenhagen solar cooker configurations as a function of solar altitude angle. Renew. Energy 2024, 229, 120782. [Google Scholar] [CrossRef]
- Wei, D.; Basem, A.; Alizadeh, A.; Jasim, D.J.; Aljaafari, H.A.S.; Fazilati, M.; Mehmandoust, B.; Salahshour, S. Optimum tilt and azimuth angles of heat pipe solar collector, an experimental approach. Case Stud. Therm. Eng. 2024, 55, 104083. [Google Scholar] [CrossRef]
- Kacira, M.; Simsek, M.; Babur, Y.; Demirkol, S. Determining optimum tilt angles and orientations of photovoltaic panels in Sanliurfa, Turkey. Renew. Energy 2004, 29, 1265–1275. [Google Scholar] [CrossRef]
- Mangkuto, R.A.; Tresna, D.N.A.T.; Hermawan, I.M.; Pradipta, J.; Jamala, N.; Paramita, B.; Atthaillah. Experiment and simulation to determine the optimum orientation of building-integrated photovoltaic on tropical building façades considering annual daylight performance and energy yield. Energy Built Environ. 2024, 5, 414–425. [Google Scholar] [CrossRef]
- Sameera; Tariq, M.; Rihan, M. Analysis of the impact of irradiance, temperature and tilt angle on the performance of grid-connected solar power plant. Meas. Energy 2024, 2, 100007. [Google Scholar] [CrossRef]
- Mubarak, R.; Luiz, E.W.; Seckmeyer, G. Why PV Modules Should Preferably No Longer Be Oriented to the South in the Near Future. Energies 2019, 12, 4528. [Google Scholar] [CrossRef]



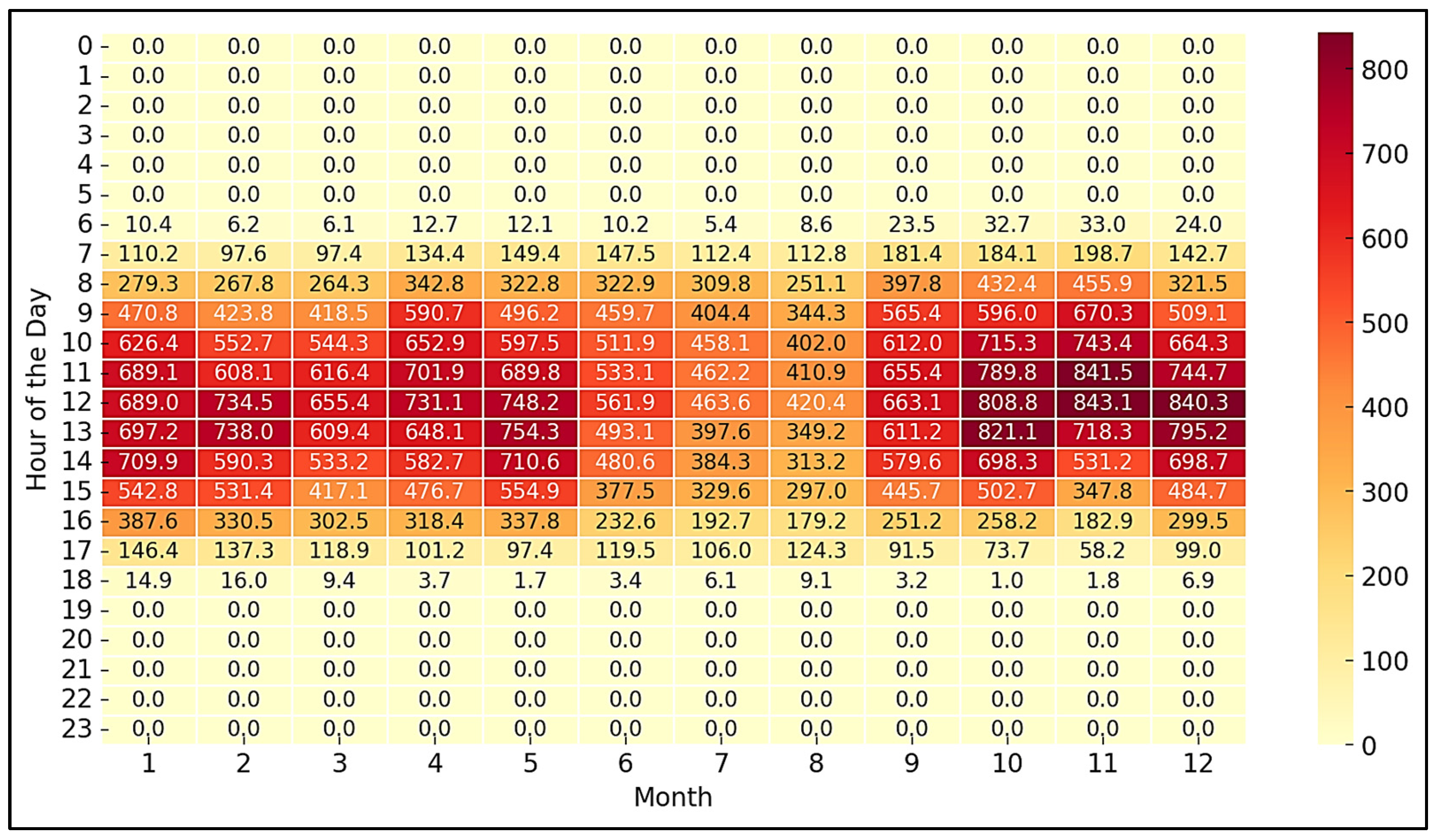
| Month | W1 (West) Production (kWh) | W2 (West) Production (kWh) | E (East) Production (kWh) |
|---|---|---|---|
| June | 1527.72 | 1525.25 | 1475.08 |
| July | 1351.64 | 1351.29 | 1337.25 |
| August | 1200.43 | 1198.67 | 1197.92 |
| September | 1833.41 | 1829.14 | 1838.85 |
| October | 2205.61 | 2199.99 | 2253.80 |
| November | 2036.65 | 2025.45 | 2158.63 |
| December | 2105.62 | 2094.65 | 2198.26 |
| January | 1997.26 | 1999.14 | 2084.71 |
| February | 1679.81 | 1691.50 | 1712.32 |
| March | 1711.78 | 1708.58 | 1699.05 |
| April | 1912.71 | 1906.94 | 1846.65 |
| May | 2039.87 | 2035.86 | 1875.66 |
| Year | 21,602.51 | 21,566.47 | 21,678.19 |
| Projected Production (kWh) | Actual Production (kWh) | Difference (kWh) | Difference (%) | |
|---|---|---|---|---|
| Actual Production E | 24,944.64 | 21,678.19 | 3266.45 | −13.09 |
| Actual Production W1 | 24,944.64 | 21,602.51 | 3342.13 | −13.40 |
| Actual Production W2 | 24,944.64 | 21,602.51 | 3342.13 | −13.40 |
| Element | Measured Energy | Uncertainty (±kWh) | Uncertainty (±%) |
|---|---|---|---|
| Investor 1 (W1) | 21,602 kWh | ±648 kWh | ±3.0% |
| Investor 2 (W2) | 21,566 kWh | ±647 kWh | ±3.0% |
| Investor 3 (E) | 21,678 kWh | ±650 kWh | ±3.0% |
| Total System | 64,847 kWh | ±1120 kWh | ±1.7% |
| Parameter | W1 | W2 | E |
|---|---|---|---|
| Number of observations (n) | 365 | 365 | 365 |
| Sum (∑xi) | 21.603 | 21.566 | 21.678 |
| Mean (X) | 59.18 | 59.09 | 59.39 |
| Median (M) | 58.18 | 58.10 | 59.07 |
| Standard deviation (SD) | 20.04 | 20.00 | 20.90 |
| Min (min xi) | 14.10 | 14.14 | 14.34 |
| Max (max xi) | 110.42 | 110.23 | 112.49 |
| Range (R) | 96.32 | 96.08 | 98.15 |
| Skew (g1) | 0.12 | 0.12 | 0.19 |
| Kurtosis (g2) | −0.49 | −0.48 | −0.48 |
| Test | Shapiro–Wilk | p-Value (Shapiro–Wilk) | Levene | p-Value (Levene) |
|---|---|---|---|---|
| W1 | ✓ | 0.00554 | - | - |
| W2 | ✓ | 0.00514 | - | - |
| E | ✓ | 0.00199 | - | - |
| W1/W2 | - | - | ✓ | 0.9611 |
| W1/E | - | - | ✓ | 0.3218 |
| W2/E | - | - | ✓ | 0.2985 |
| Source | Df | Sum Sq | Mean Sq | F Value | Pr (>F) |
|---|---|---|---|---|---|
| Systems | 2 | 18 | 8.9 | 0.022 | 0.979 |
| Residuals | 1092 | 450,787 | 412.8 | - |
| Test | Statistic | Df | p-Value |
|---|---|---|---|
| Kruskal–Wallis chi-squared | 0.009878 | 2 | 0.9951 |
| Df | Sum Sq | Mean Sq | F Value | Pr (>F) | |
|---|---|---|---|---|---|
| Panel | 2 | 18 | 9 | 0.028 | 0.973 |
| YearMonth | 11 | 110,166 | 10,015 | 31.276 | <2 × 10−16 |
| System–YearMonth | 22 | 1515 | 69 | 0.215 | 1.000 |
| Residuals | 1059 | 339,106 | 320 | - | - |
| # | Panel | Year/Month | p-Value | Statistic |
|---|---|---|---|---|
| 1 | W1 | 21-06 | 0.274 | 0.958 |
| 2 | W1 | 21-07 | 0.0850 | 0.940 |
| 3 | W1 | 21-08 | 0.00277 | 0.883 |
| … | … | … | … | … |
| 34 | E | 22-03 | 0.0507 | 0.932 |
| 35 | E | 22-04 | 0.311 | 0.960 |
| 36 | E | 22-05 | 0.251 | 0.958 |
| # | Month | KW Statistic | p-Value | p Bonferroni |
|---|---|---|---|---|
| 1 | 21-06 | 0.608644689 | 0.7376231 | 1 |
| 2 | 21-07 | 0.106272278 | 0.9482509 | 1 |
| 3 | 21-08 | 0.031970244 | 0.9841420 | 1 |
| 4 | 21-09 | 0.025006105 | 0.9875748 | 1 |
| 5 | 21-10 | 0.406314345 | 0.8161500 | 1 |
| 6 | 21-11 | 2.897094017 | 0.2349114 | 1 |
| 7 | 21-12 | 0.469457790 | 0.7907852 | 1 |
| 8 | 22-01 | 0.344676423 | 0.8416945 | 1 |
| 9 | 22-02 | 0.006842737 | 0.9965845 | 1 |
| 10 | 22-03 | 0.047114043 | 0.9767183 | 1 |
| 11 | 22-04 | 0.189401709 | 0.9096450 | 1 |
| 12 | 22-05 | 2.077357363 | 0.3539220 | 1 |
| Location | Key Findings | Implications | Ref. |
|---|---|---|---|
| Global | South-facing orientation maximizes efficiency in higher latitudes | Orientation adjustments are less impactful in equatorial regions due to consistent solar path | [1] |
| Switzerland | West orientation better aligns with daily demand | Orientation adjustments based on demand patterns improve grid performance | [2] |
| Temperate Regions | Minor deviations from optimal orientation do not significantly affect energy yield | Tilt and azimuth adjustments critical in temperate climates for maximizing output | [11] |
| Romania | Seasonal tilt adjustments increase energy capture by up to 4.8% | Seasonal tilt adjustments are crucial for maximizing output in colder climates | [12] |
| USA | South orientation with tilt equal to local latitude maximizes energy | Orientation and tilt adjustments are location-specific for optimizing yield | [13] |
| Morocco | Best performance in landscape orientation | Orientation critical for maximizing output in semi-arid climates | [14] |
| Equatorial Areas | Orientation variations have less impact due to consistent solar path | Stable solar path minimizes the impact of orientation in equatorial regions | [15] |
| Turkey | Maximum energy generation achieved with 31.33° tilt angle | Focus on specific tilt for optimizing generation in agrivoltaic systems | [18] |
| Iceland, Canada, Ecuador, and Brazil | Biannual reorientation improves output by 3–4.8% | Biannual adjustments optimize energy production in varying climates | [19] |
| Equatorial Regions | Optimal tilt angles close to latitude, requiring minimal seasonal adjustments | Supports minimal tilt adjustments in equatorial regions | [20] |
| General Analysis | East–west orientations support similar energy production to traditional setups | Orientation adjustments are less critical in consistent solar conditions | [23] |
| Various Regions | East–west orientations reduce storage needs by matching demand patterns | East–west setups improve demand alignment and reduce storage needs | [28] |
| Netherlands and Germany | Optimal orientation varies with demand patterns; 212° azimuth and 26° tilt maximize self-consumption | Demand-based orientation adjustments necessary for maximizing self-consumption | [29] |
| Equatorial Regions | Slight advantage for east-facing panels in October–November | Solar altitude variations affect panel performance seasonally | [44] |
| General Analysis | Emphasizes need for dual-axis tracking and tilt optimization | Dual-axis tracking and advanced tilt control needed for further optimization | [45] |
| Turkey | Monthly optimal tilt varies between 13° and 61° | Seasonal adjustments critical for optimizing output | [46] |
| Indonesia | Higher annual energy yield with north-facing orientation but increased uncertainty | Orientation impacts uncertainty and energy yield in tropical regions | [47] |
| India | Direct relation between irradiance and energy generation with optimal tilt close to latitude | Tilt adjustments based on irradiance critical for maximizing output | [48] |
| Southern Ecuador | Minimal differences in energy output between east- and west-facing orientations | Focus on other factors like cost, installation, and maintenance for PV optimization | This study |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Correa-Guamán, A.; Moreno-Salazar, A.; Paccha-Soto, D.; Jaramillo-Fierro, X. Impact of Azimuth Angle on Photovoltaic Energy Production: Experimental Analysis in Loja, Ecuador. Energies 2025, 18, 1998. https://doi.org/10.3390/en18081998
Correa-Guamán A, Moreno-Salazar A, Paccha-Soto D, Jaramillo-Fierro X. Impact of Azimuth Angle on Photovoltaic Energy Production: Experimental Analysis in Loja, Ecuador. Energies. 2025; 18(8):1998. https://doi.org/10.3390/en18081998
Chicago/Turabian StyleCorrea-Guamán, Angel, Alex Moreno-Salazar, Diego Paccha-Soto, and Ximena Jaramillo-Fierro. 2025. "Impact of Azimuth Angle on Photovoltaic Energy Production: Experimental Analysis in Loja, Ecuador" Energies 18, no. 8: 1998. https://doi.org/10.3390/en18081998
APA StyleCorrea-Guamán, A., Moreno-Salazar, A., Paccha-Soto, D., & Jaramillo-Fierro, X. (2025). Impact of Azimuth Angle on Photovoltaic Energy Production: Experimental Analysis in Loja, Ecuador. Energies, 18(8), 1998. https://doi.org/10.3390/en18081998









