Numerical Study of Turbulent Forced Convection in a Square Duct with Discrete X-V Inducing Turbulators (DXVIT)
Abstract
1. Introduction
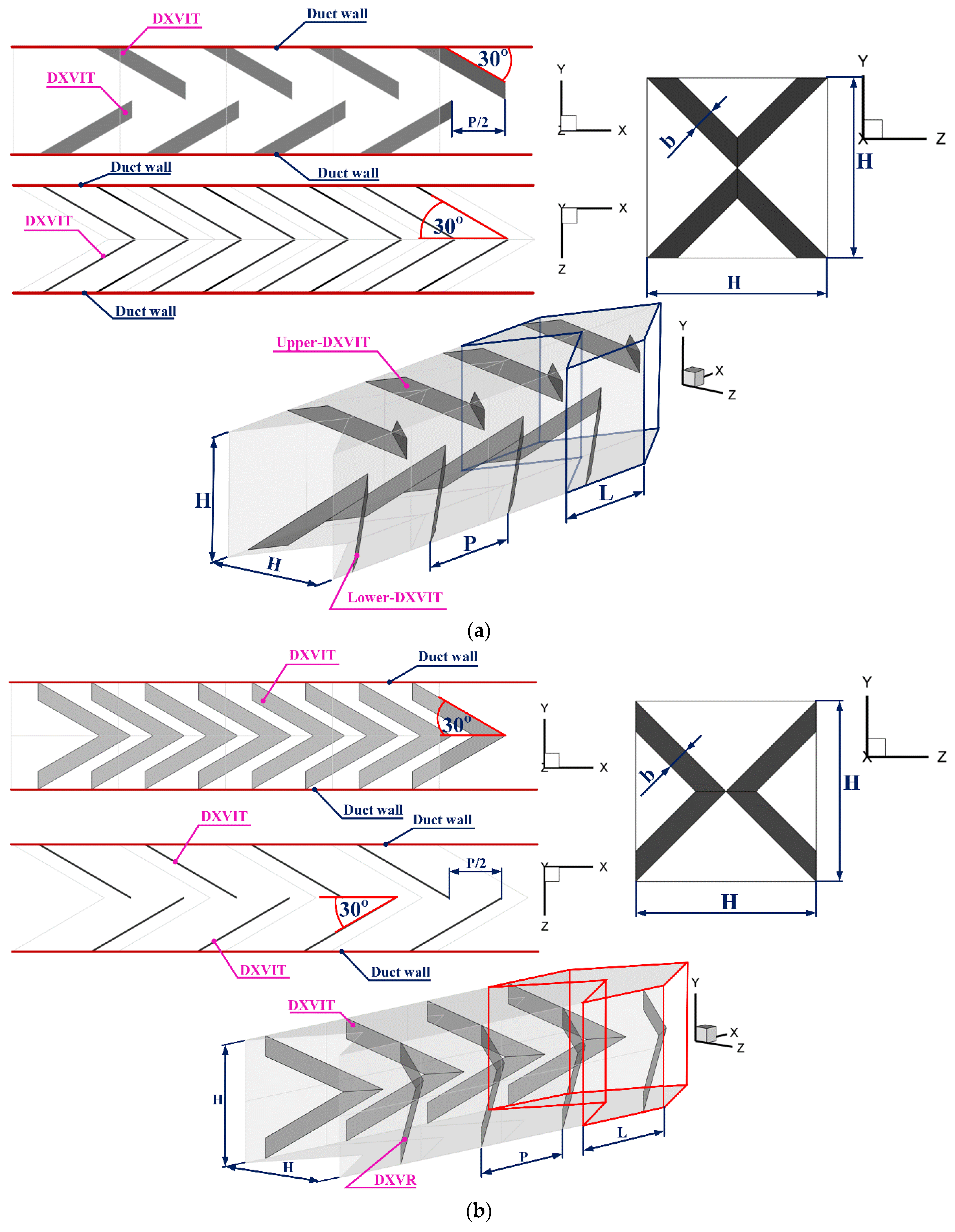
2. Physical Configuration of a Square Duct Heat Exchanger (SQHX) Equipped with DXVIT and Boundary Condition
3. CFD Model
- The flow and thermal configuration are thought to be three-dimensional (3D heat and fluid flow) and constant.
- The flow is considered incompressible, and the flow regime is turbulent. The Reynolds number is evaluated based on the conditions at the inlet of the SQHX.
- The working fluid used in the SQHX is air. The temperature variation of the air during the test does not exceed 20 Kelvin/Celsius. Therefore, the thermal properties of the fluid change very little and can be considered constant throughout the test.
- Body forces and viscous dissipation are not considered in the current study.
- Only forced convection inside the SQHX is examined in this heat transfer study because the effects of conduction, natural convection, and thermal radiation are insignificant.
4. Numerical Validation
4.1. Smooth SQHX Validation
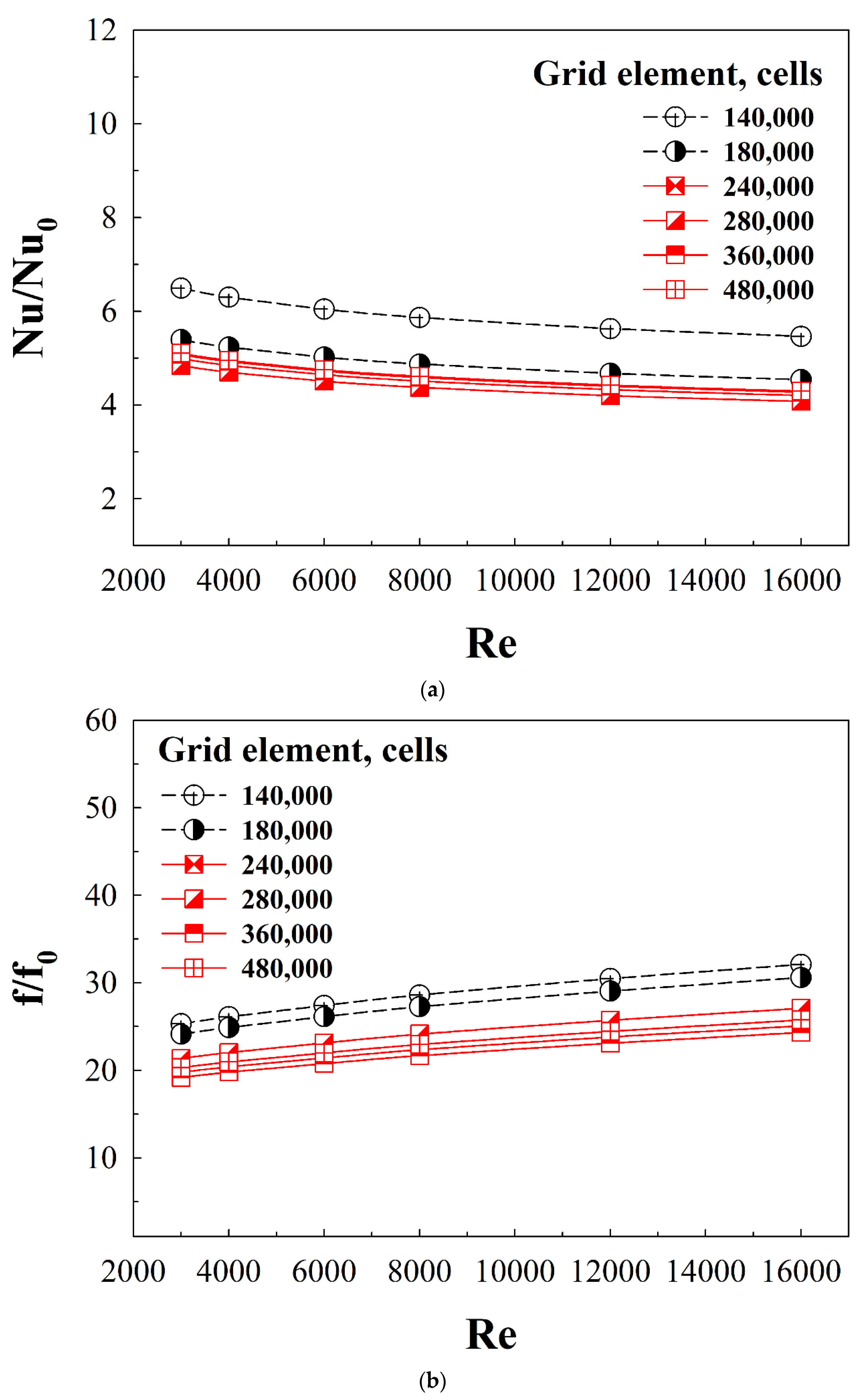
4.2. Grid Independence
4.3. Validation of the Experimental Result
5. Results and Discussion
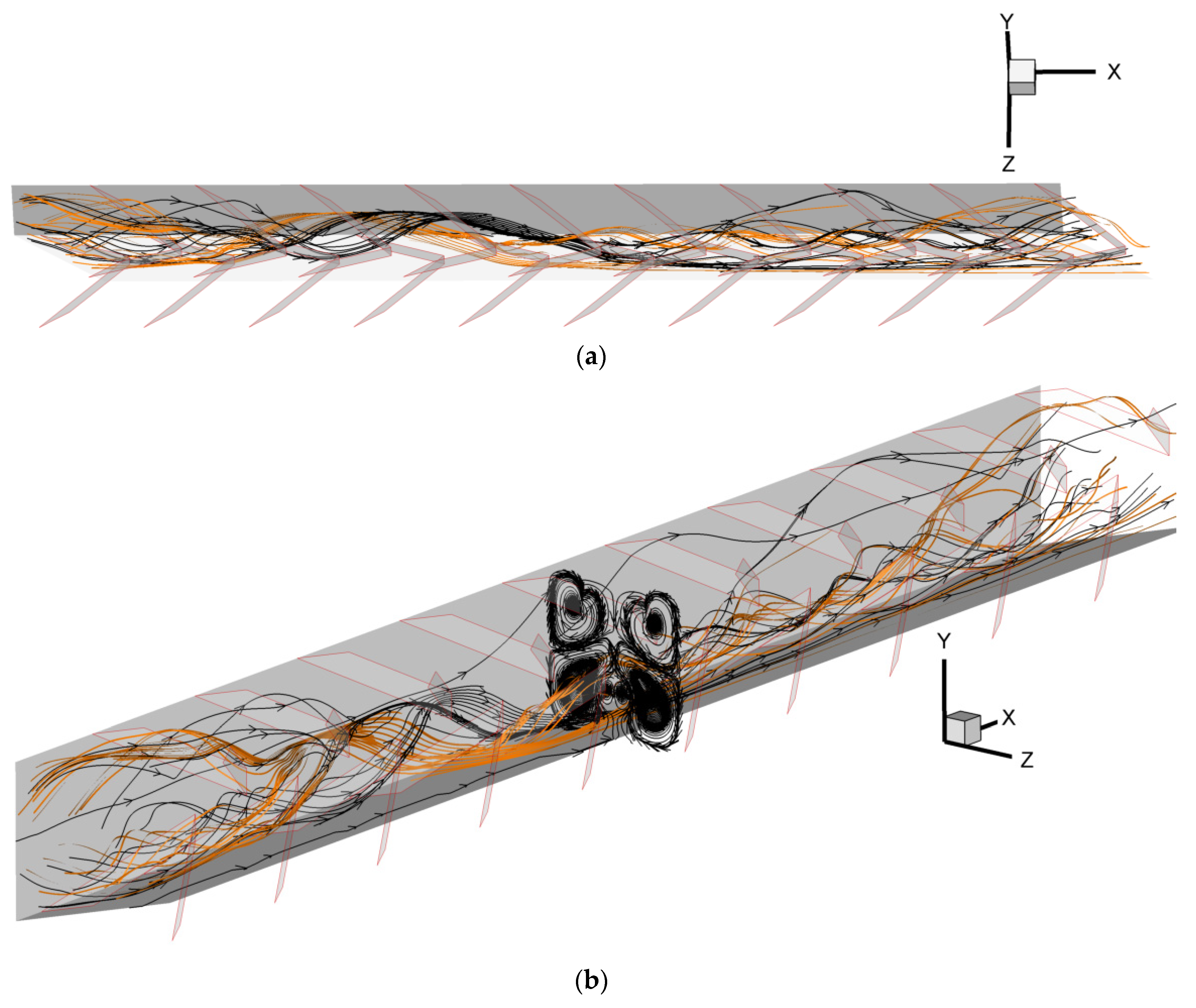
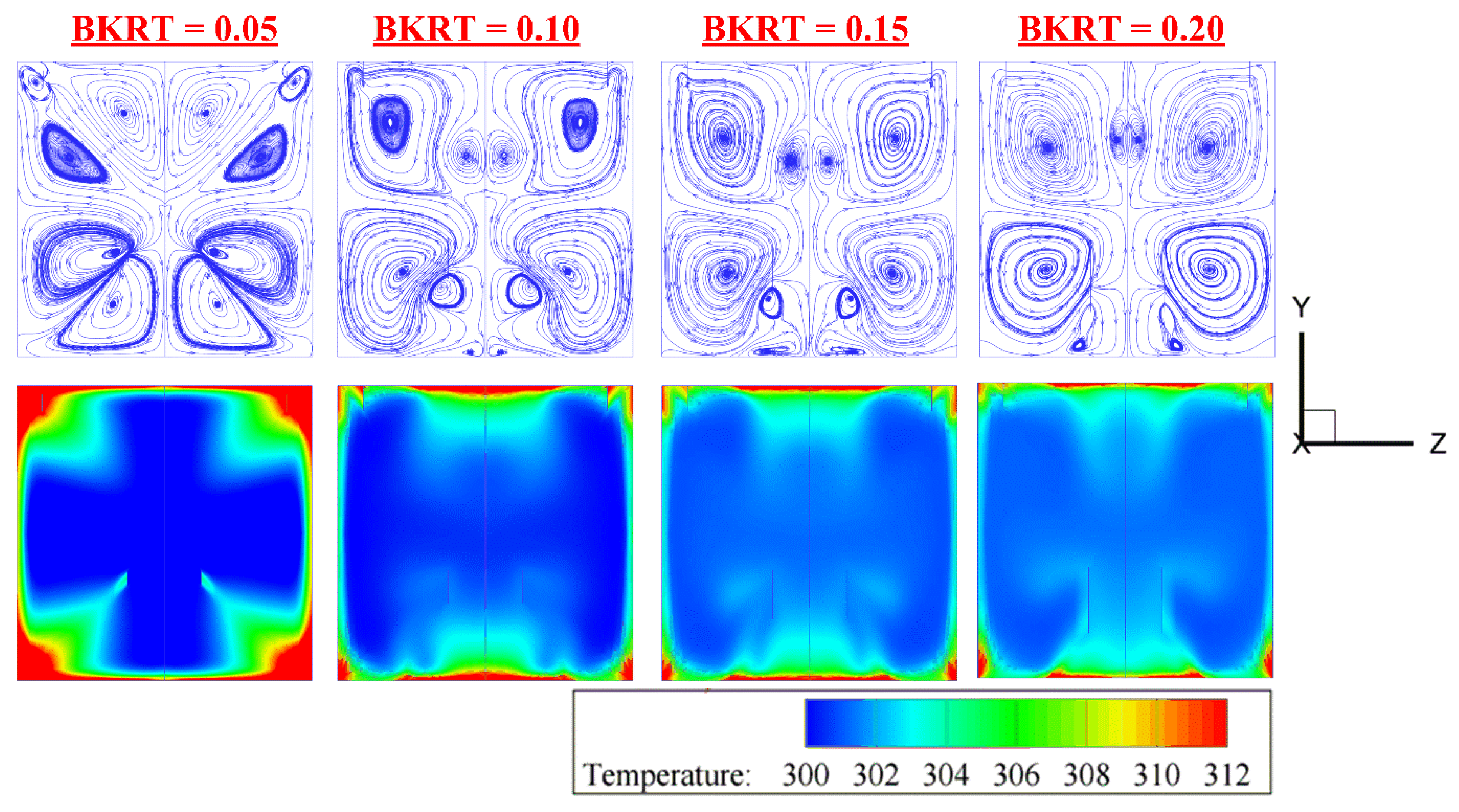
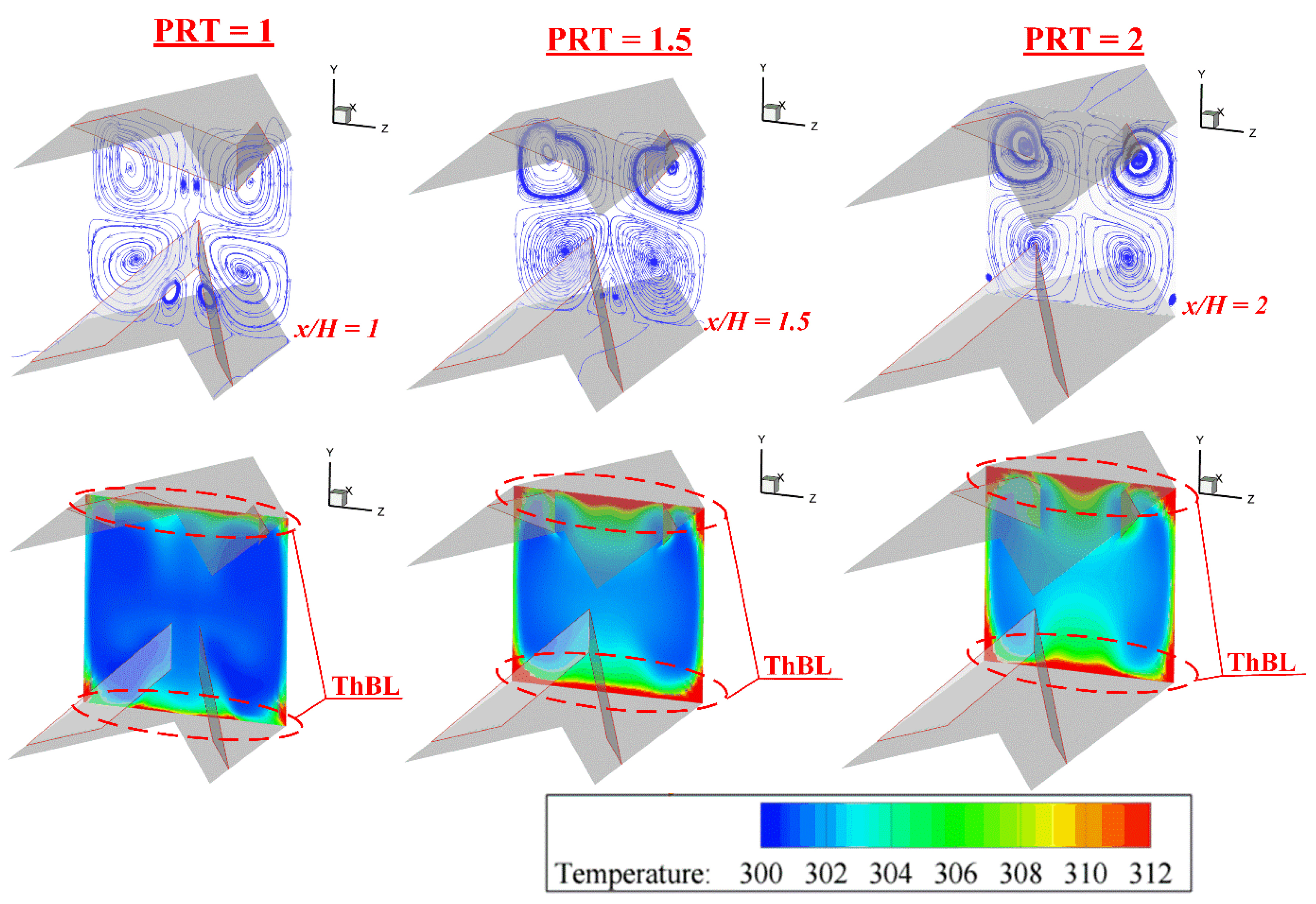
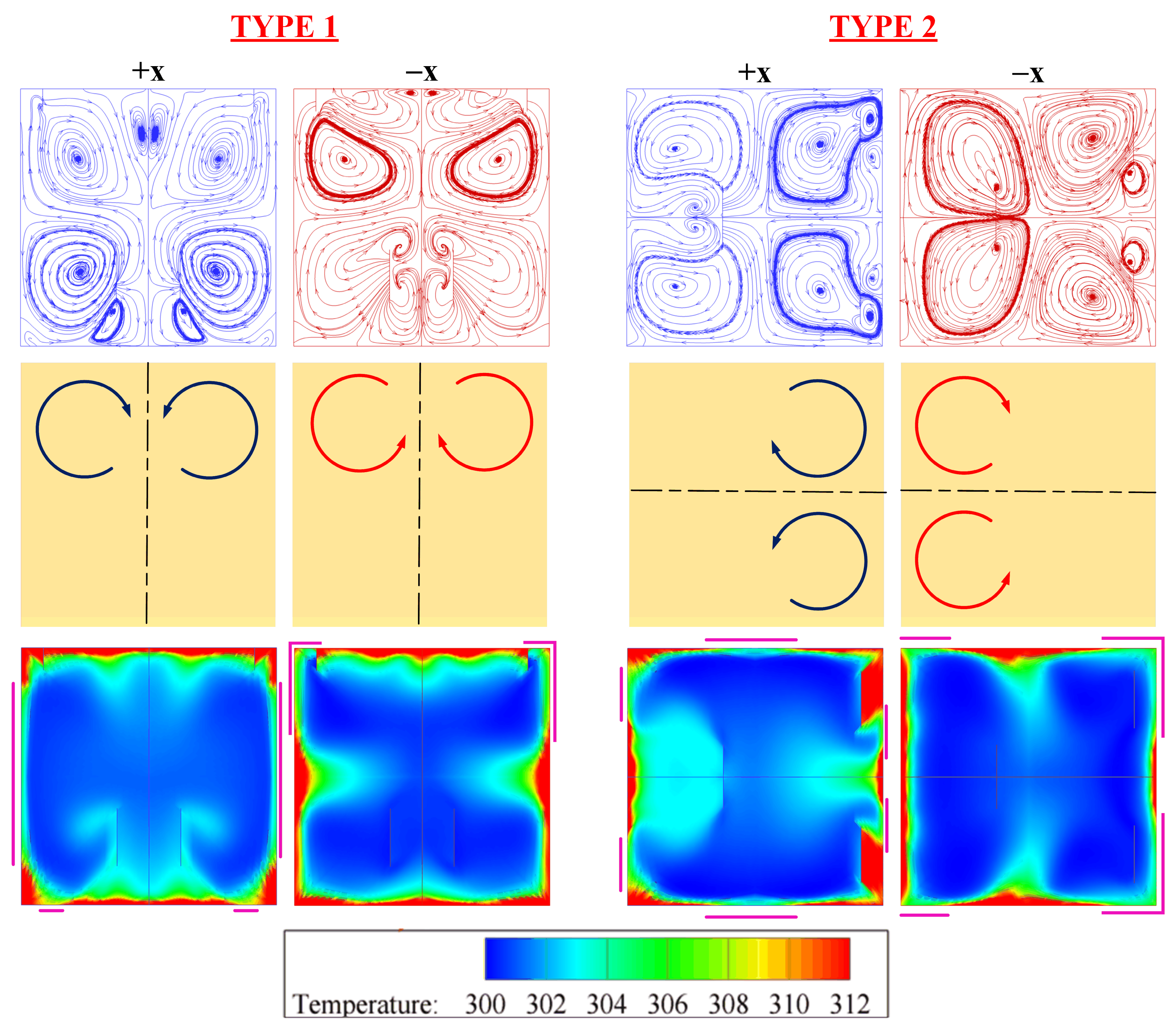
5.1. Flow and Heat Transfer Configurations
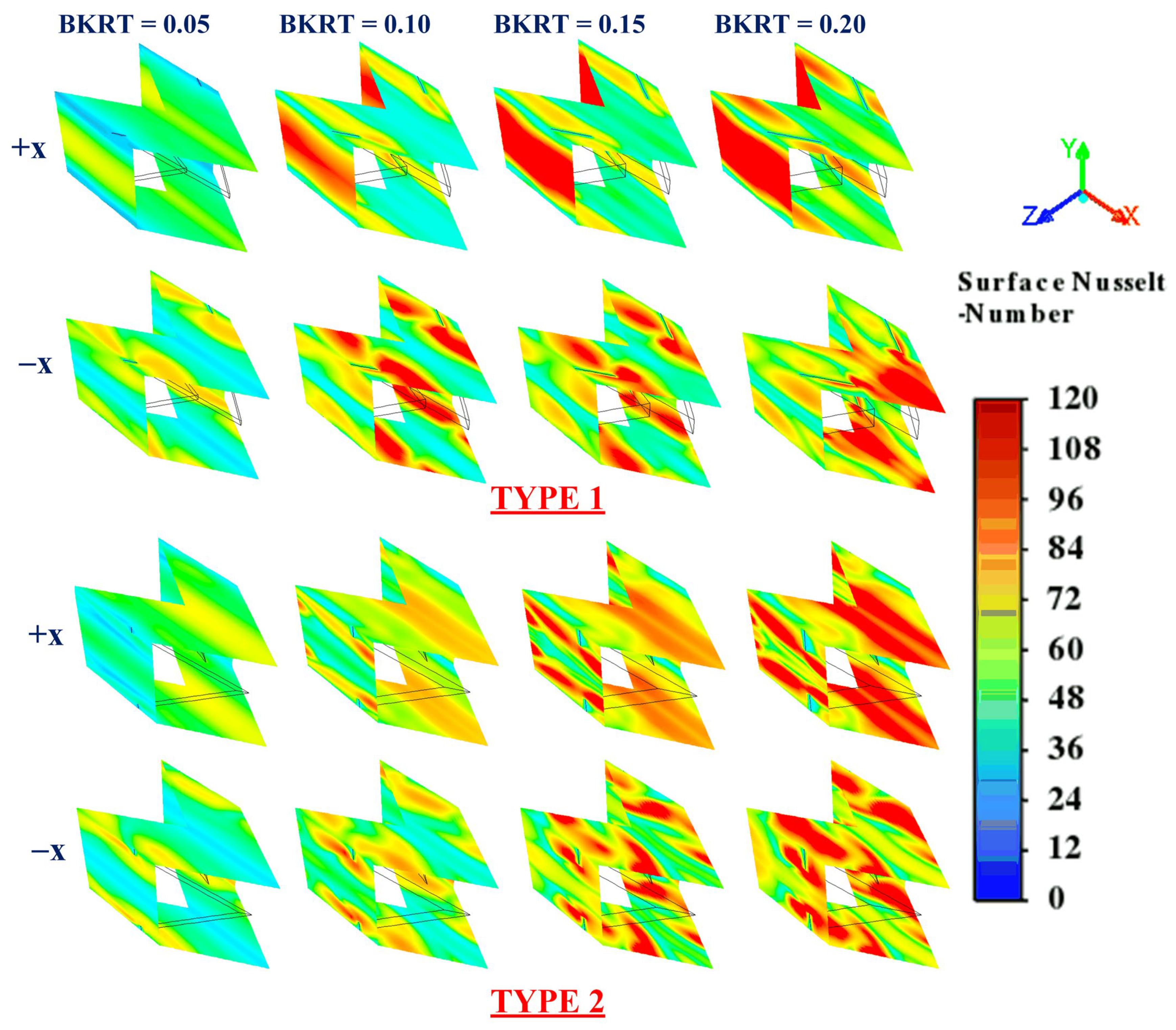
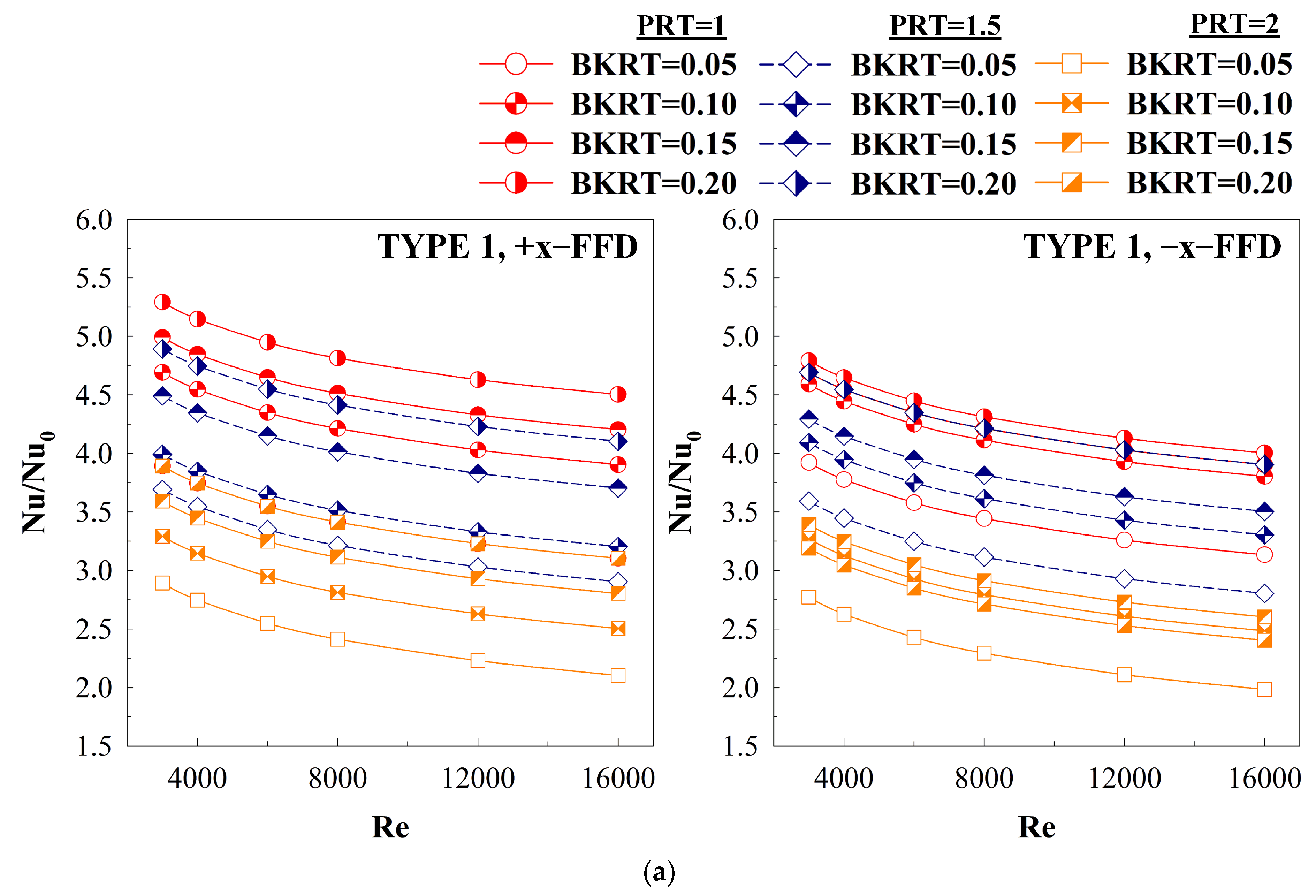
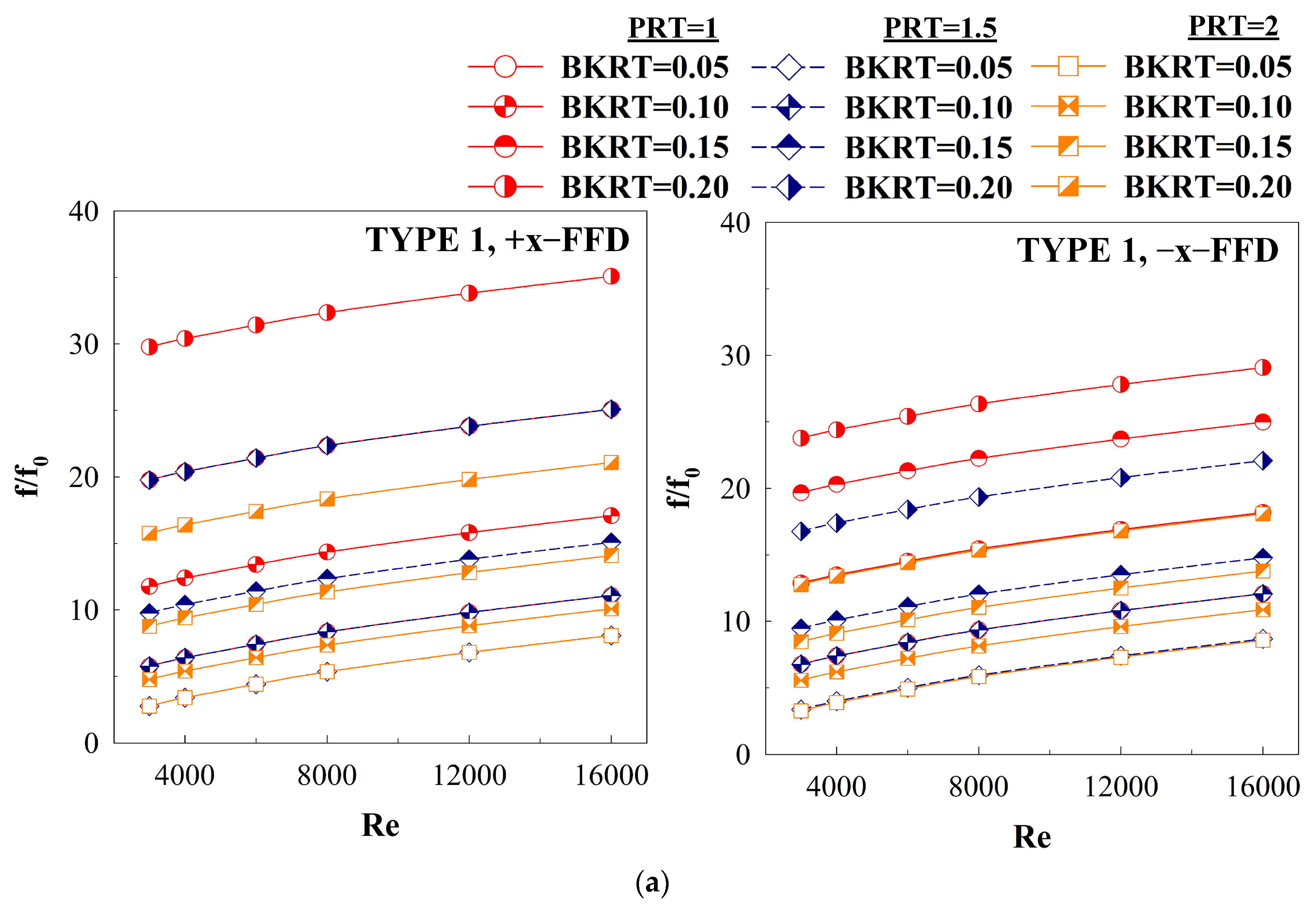
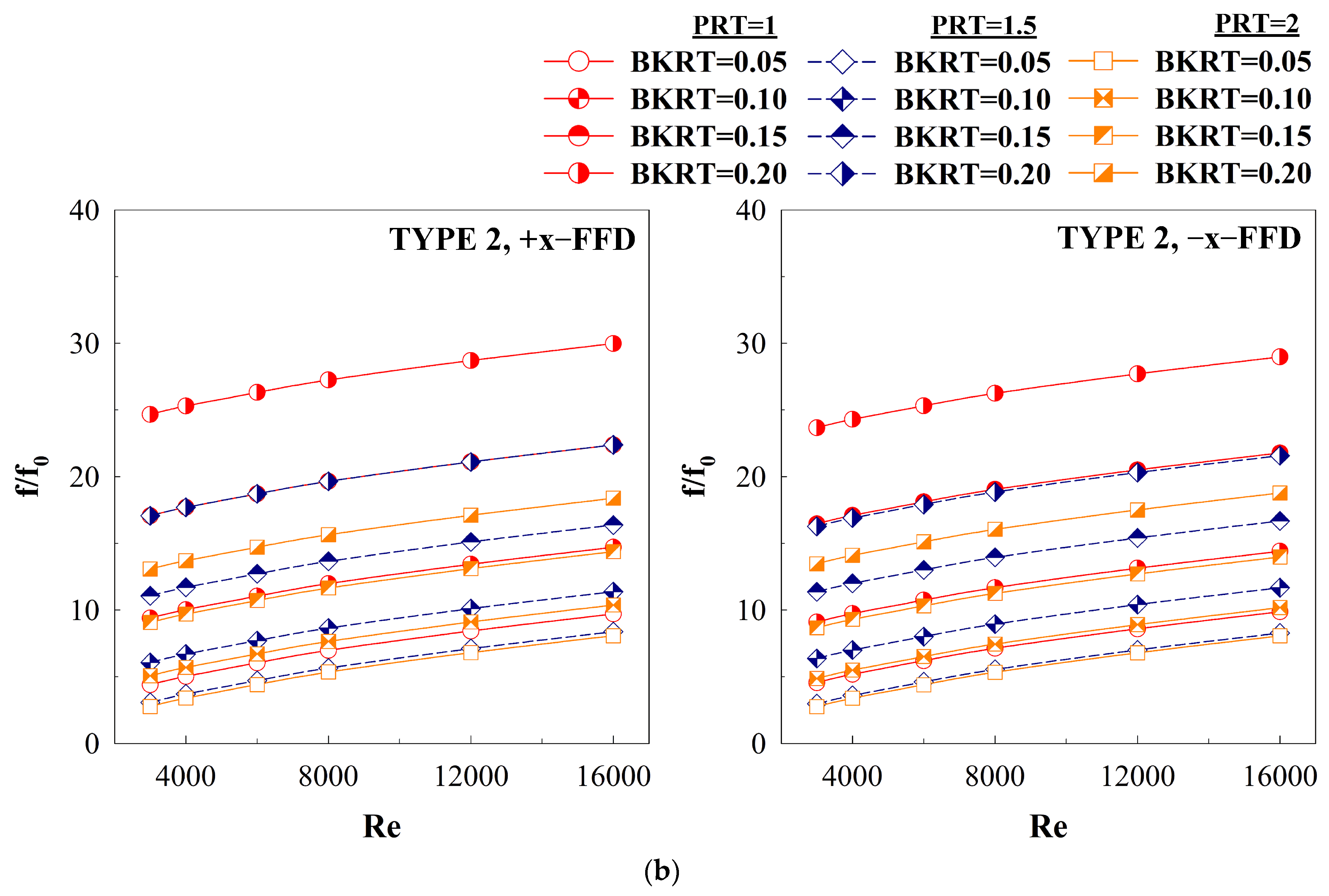
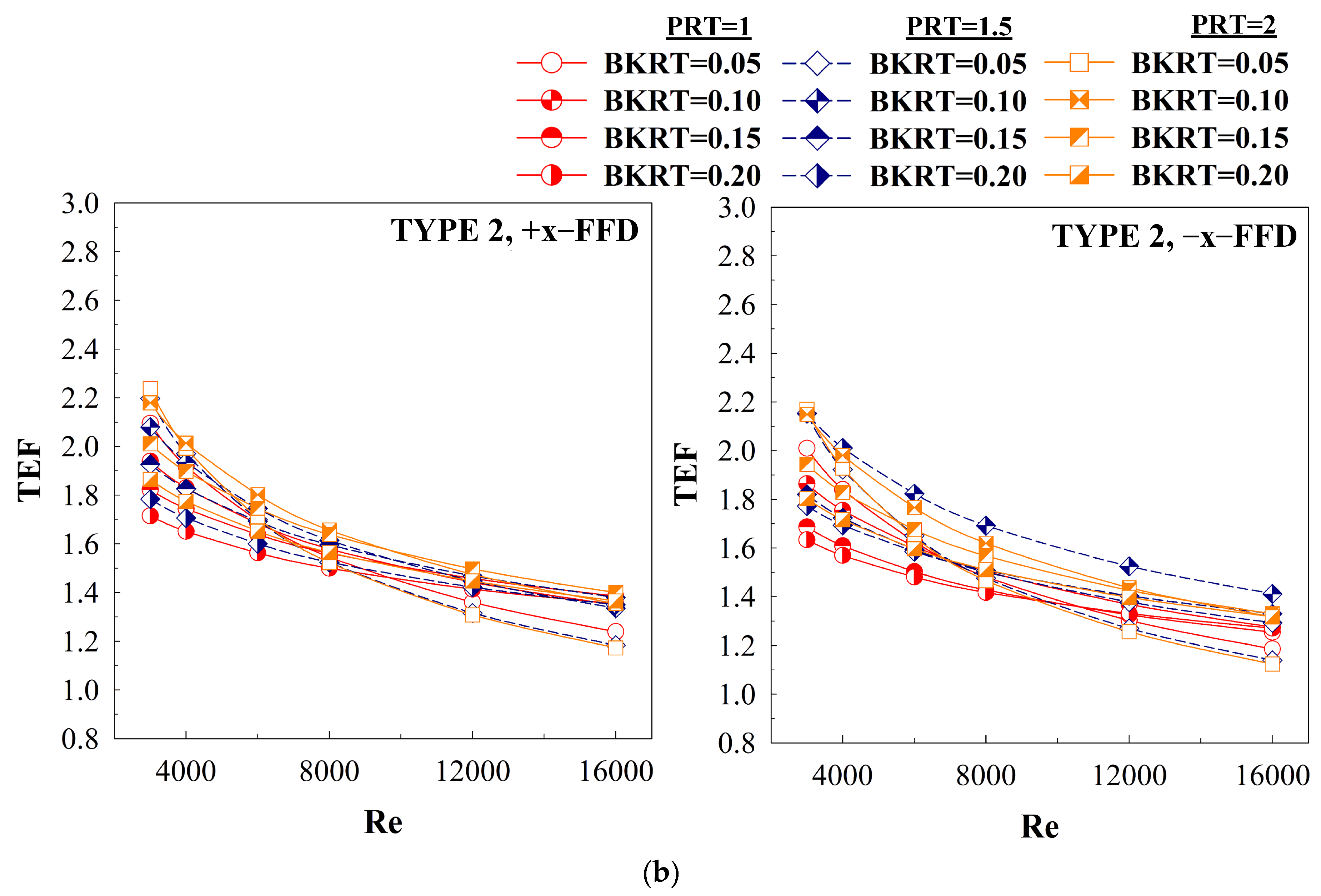
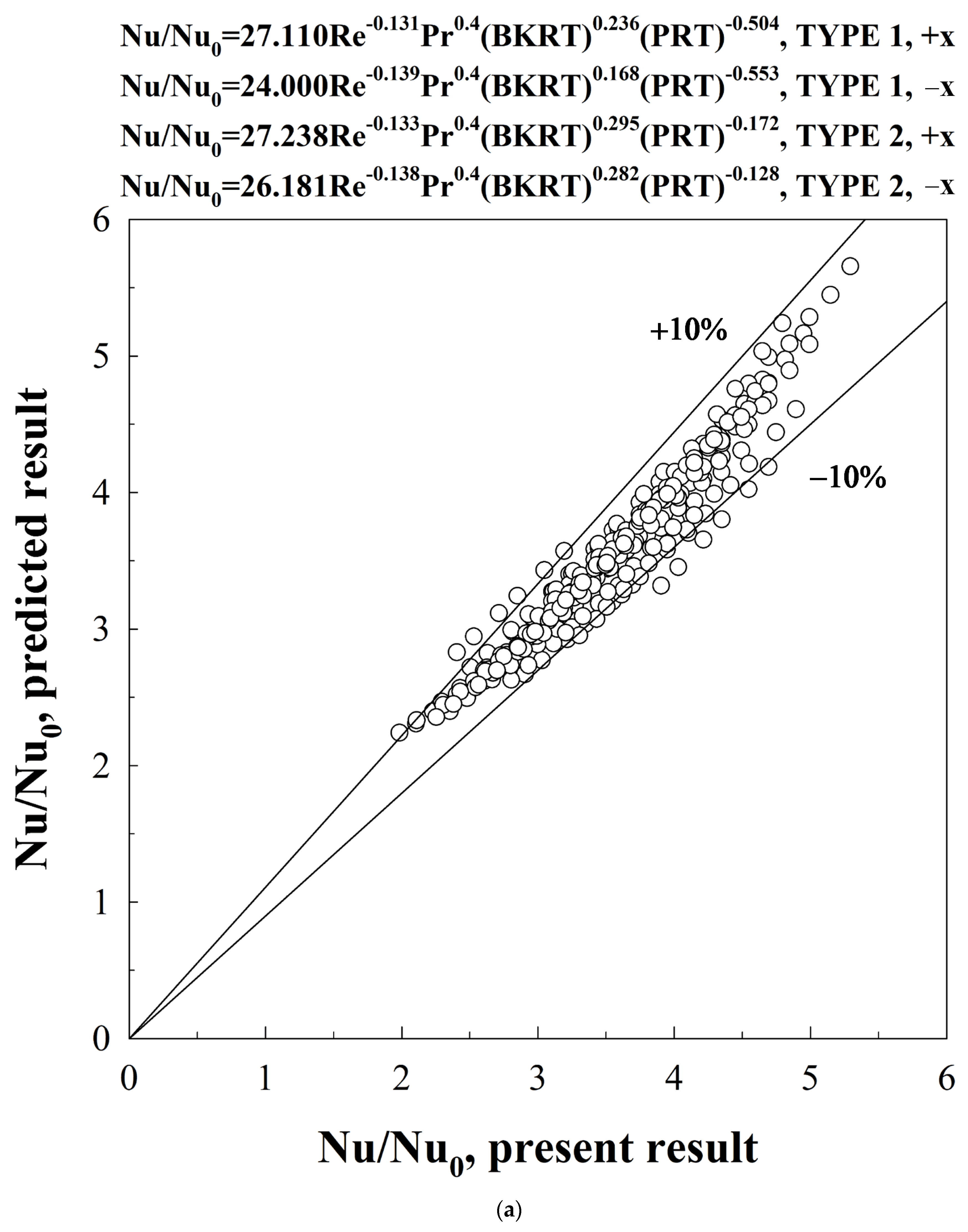
5.2. Performance Assessment
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| Symbols and abbreviations | |
| b | DXVIT thickness, m |
| constant value (=0.0845) | |
| Dh | hydraulic diameter (=H for square duct), m |
| f | friction factor, friction loss |
| h | convective heat transfer coefficient, W m−2 K−1 |
| H | duct height (=duct width), m |
| k | |
| kt | thermal conductivity, W m−1 K−1 |
| L | numerical model length/periodic length |
| Nu | Nusselt number |
| p | static pressure, Pa |
| P | pitch spacing, m |
| Pr | Prandtl number |
| Re | Reynolds number |
| T | fluid temperature, K |
| fluid mean velocity in square duct, m s−1 | |
| ui | mean component of velocity in the direction xi, m s−1 |
| u′ | fluctuating component of velocity, m s−1 |
| Greek symbol | |
| ρ | density, kg m−3 |
| µ | dynamic viscosity, kg m−1s−1 |
| effective viscosity | |
| inverse effective Prandtl number for k | |
| inverse effective Prandtl number for Γ | molecular thermal diffusivity |
| Γt | turbulent thermal diffusivity |
| a Kronecker delta | |
| Subscripts | |
| 0 | smooth duct |
| pp | driving force |
| Abbreviations | |
| BKRT | blockage ratio |
| DXVIT | discrete X-V inducing turbulator |
| PRT | pitch ratio |
| SQHX | square duct heat exchanger |
| TEF | thermal enhancement factor (=(Nu/Nu0)/(f/f0)1/3) |
| ThBL | thermal boundary layer |
References
- Mukhlif, E.A.; Abed, W.M. Influences of submerged V-broken rib geometry on the hydrothermal performance of vortex and engulfment flow regimes within a T-channel. Int. Commun. Heat Mass Transf. 2024, 159, 108325. [Google Scholar] [CrossRef]
- Kumar, A.; Pathak, M. Flow and heat transfer characteristics of gas turbine blade channels with compound V-rib-dimple structures. Int. Commun. Heat Mass Transf. 2024, 157, 107823. [Google Scholar] [CrossRef]
- Sutar, S.; Rout, S.K.; Senapati, J.R.; Barik, D.; Dennison, M.S.; Praveenkumar, S. Numerical investigation on the thermohydraulic performance of a solar air heater duct featuring parabolic rib turbulators on the absorber plate. Case Stud. Therm. Eng. 2024, 64, 105399. [Google Scholar] [CrossRef]
- Hegde, A.K.; Pai, R.; Karanth, K.V. Influence of solar insolation on energy and exergy efficiency of a rectangular duct solar air heater with various types of V rib roughness: An analytical approach. Int. Commun. Heat Mass Transf. 2024, 153, 107397. [Google Scholar] [CrossRef]
- Liu, C.; Bao, Y.; Li, B.; Lyu, P.; Liu, X.; Rao, Z. Experimental study on heat transfer enhancement and flow performance of microencapsulated phase change slurry in microchannel with different rib structures. Int. J. Heat Mass Transf. 2025, 237, 126437. [Google Scholar] [CrossRef]
- Wang, L.; Lv, C.; Liu, X.; Mao, J.; Zhang, D.; Liu, Y.; Li, M. Research on improving heat transfer performance by using wavy ribs with different cross sections. Appl. Therm. Eng. 2024, 257, 124397. [Google Scholar] [CrossRef]
- Kumar, M.S.; Abraham, S. Experimental and numerical studies of detailed heat transfer and flow characteristics in the rib turbulated annulus of a double pipe heat exchanger. Int. J. Therm. Sci. 2025, 207, 109382. [Google Scholar] [CrossRef]
- Cao, Z.; Luo, L.; Yang, X.; Yan, H.; Du, W. Effects of turning vane on flow control and heat transfer in ribbed two-pass channel with varied rib patterns. Int. J. Therm. Sci. 2025, 207, 109342. [Google Scholar] [CrossRef]
- Wang, L.; Liu, X.; Cui, T.; Mao, J.; Lv, C.; Zhang, D.; Liu, Y.; Li, M. Multi-objective analysis of the turbine blade internal cooling channel with discontinuous bionic S-shaped ribs. Int. J. Therm. Sci. 2025, 207, 109371. [Google Scholar] [CrossRef]
- Chen, L.; Weigand, B.; Yang, H.; Shi, Y.; Yang, Q. Numerical heat transfer investigation of a single impingement jet with combined V-ribs and preliminary optimization of V-rib configuration in initial crossflow. Int. J. Therm. Sci. 2024, 197, 108799. [Google Scholar] [CrossRef]
- Majmader, F.B.; Hasan, M.J. Effects of bidirectional rib arrangements on turbulent flow structure and heat transfer characteristics of a two-pass channel for turbine blade internal cooling. Int. Commun. Heat Mass Transf. 2024, 156, 107688. [Google Scholar] [CrossRef]
- Chhaparwal, G.K.; Goyal, R.; Srivastava, A.; Goyal, A.; Oza, A.D.; Siddiqui, M.I.H.; Natrayan, L.; Kumar, L.; Chandrakant, S. Numerical and experimental investigation of a solar air heater duct with circular detached ribs to improve its efficiency. Case Stud. Therm. Eng. 2024, 60, 104780. [Google Scholar] [CrossRef]
- Wang, L.; Liu, X.; Mao, J.; Lv, C.; Zhang, D.; Liu, Y.; Li, M. Analyzing the impact of sinusoidal wavy rib geometric parameters on turbine blade thermal and fluid dynamics. Int. J. Therm. Sci. 2024, 203, 109162. [Google Scholar] [CrossRef]
- Wang, Q.; Tao, J.; Cui, Z.; Zhang, T.; Chen, G. Numerical simulation of fluid and heat transfer characteristics of microchannel heat sink with fan-shaped grooves and triangular truncated ribs. Int. Commun. Heat Mass Transf. 2024, 155, 107580. [Google Scholar] [CrossRef]
- Fu, H.; Sun, H.; Luan, J.; Yang, L.; Luan, Y.; Magagnato, F. The heat transfer enhancement mechanism of W-shaped micro-ribs on impingement cooling. Appl. Therm. Eng. 2024, 252, 123658. [Google Scholar] [CrossRef]
- Wang, L.; Lv, C.; Mao, J.; Li, Y.; Zhang, D.; Liu, X. Effects of non-uniform wavy ribs on flow and heat transfer characteristics of turbine blade cooling channel. Appl. Therm. Eng. 2024, 248, 123249. [Google Scholar] [CrossRef]
- Prasad, J.S.; Datta, A.; Mondal, S. Numerical analysis of a solar air heater with offset transverse ribs placed near the absorber plate. Renew. Energy 2024, 227, 120608. [Google Scholar] [CrossRef]
- Boonloi, A.; Jedsadaratanachai, W. Thermal behaviors and flow profiles in a square channel fitted with X–V modified ribs: A numerical analysis. Case Stud. Therm. Eng. 2023, 49, 103282. [Google Scholar] [CrossRef]
- Tamang, S.; Ali, E.; Park, H. A novel concept for a rib turbulator for optimizing the cooling performance in a square channel. Int. J. Heat Mass Transf. 2024, 221, 125144. [Google Scholar] [CrossRef]
- Zhu, Q.; Wang, Z.; Zeng, J.; Wen, X.; Deng, H.; He, W.; Zhao, T. Numerical study on flow and heat transfer in a novel symmetric sinusoidal wavy microchannel heat sink with rectangular rib prisms. Int. J. Therm. Sci. 2024, 197, 108807. [Google Scholar] [CrossRef]
- Patankar, S.V.; Liu, C.H.; Sparrow, E.M. Fully developed flow and heat transfer in ducts having streamwise-periodic variations of cross-sectional area. J. Heat Transf. 1977, 99, 180–186. [Google Scholar] [CrossRef]
- Boonloi, A.; Jedsadaratanachai, W. Turbulent forced convection in a square duct heat exchanger (SDHX) equipped with combined vortex turbulators (CVT): A numerical investigation. Int. Commun. Heat Mass Transf. 2025, 161, 108421. [Google Scholar] [CrossRef]
- Cengel, Y.A.; Ghajar, A.J. Heat and Mass Transfer: Fundamentals & Applications, 5th ed.; McGraw-Hill Education: New York, NY, USA, 2015; ISBN 978-981-4595-27-8. [Google Scholar]
- Promvonge, P.; Skullong, S.; Kwankaomeng, S.; Thiangpong, C. Heat transfer in square duct fitted diagonally with angle-finned tape—Part 1: Experimental study. Int. Commun. Heat Mass Transf. 2012, 39, 617–624. [Google Scholar] [CrossRef]
- Tan, Y.; Ni, Y.; Xu, W.; Xie, Y.; Li, L.; Tan, D. Key technologies and development trends of the soft abrasive flow finishing method. J. Zhejiang Univ. Sci. A 2023, 24, 1043–1064. [Google Scholar] [CrossRef]
- Xu, P.; Li, Q.; Wang, C.; Li, L.; Tan, D.; Wu, H. Interlayer healing mechanism of multipath deposition 3D printing models and interlayer strength regulation method. J. Manuf. Process. 2025, 141, 1031–1047. [Google Scholar] [CrossRef]







Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Boonloi, A.; Jedsadaratanachai, W. Numerical Study of Turbulent Forced Convection in a Square Duct with Discrete X-V Inducing Turbulators (DXVIT). Energies 2025, 18, 1906. https://doi.org/10.3390/en18081906
Boonloi A, Jedsadaratanachai W. Numerical Study of Turbulent Forced Convection in a Square Duct with Discrete X-V Inducing Turbulators (DXVIT). Energies. 2025; 18(8):1906. https://doi.org/10.3390/en18081906
Chicago/Turabian StyleBoonloi, Amnart, and Withada Jedsadaratanachai. 2025. "Numerical Study of Turbulent Forced Convection in a Square Duct with Discrete X-V Inducing Turbulators (DXVIT)" Energies 18, no. 8: 1906. https://doi.org/10.3390/en18081906
APA StyleBoonloi, A., & Jedsadaratanachai, W. (2025). Numerical Study of Turbulent Forced Convection in a Square Duct with Discrete X-V Inducing Turbulators (DXVIT). Energies, 18(8), 1906. https://doi.org/10.3390/en18081906





