Numerical Assessment of Nuclear Cogeneration Transients with SMRs Using CATHARE 3–MODELICA Coupling
Abstract
1. Introduction
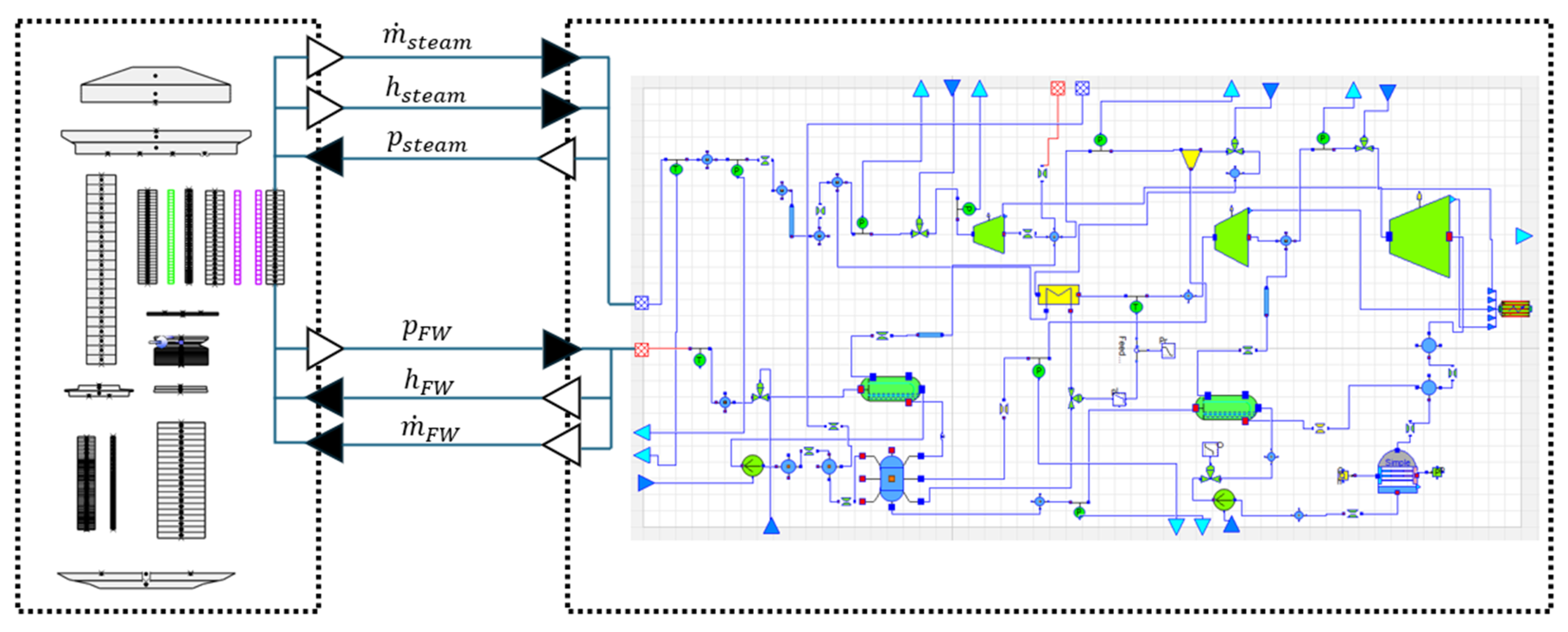
2. Description of the Adopted Models
2.1. CATHARE 3 ESMR Model Description
- Primary pressure control system, obtained through a spray valve and an electrical heater embedded in the pressuriser. The spray valve was modelled such that the enthalpy of the sprayed water is equal to the one achieved in the first node of the downcomer, where the feeding line is supposed to be connected, whereas the heater is considered simply as a point heat source.
- Average core coolant temperature control system, implemented by means of control rods. The control rods were modelled as an external reactivity source, in line with the fact that CATHARE 3 solves a point core kinetic equation. Therefore, the details of the movement of the control rods and of their axial effects were neglected in the present model. In particular, the control rod reactivity insertion was implemented following a proportional–integral (PI) control function as the one reported in Equation (1), where is the time at which the control takes place and and are the proportional and integral gains, respectively.
2.2. MODELICA BOP Model Description
- Main steam line control system: PI controller which commands the high-pressure turbine inlet valve (indicated with V1 in Figure 3) to maintain the main steam line pressure at its setpoint value.
- Medium and low-pressure turbine inlet pressure control system: PI controllers that command the opening of valves V2 and V3 to control the pressure at the inlet of the medium- and low-pressure steam turbines, respectively.
- Re-heater steam mass flow rate controller: PI controller which controls the steam temperature at the outlet of the re-heater (i.e., inlet of medium pressure turbine) acting on the valve V4.
- High-pressure pump rotational speed controller: PI controller which varies the rotational speed of the high-pressure pump (i.e., the feedwater mass flow rate entering the CSG) to keep the steam temperature constant.
- Low-pressure pump rotational speed controller: PI controller which controls the water pressure at the inlet of the degasifier by varying the rotational speed of the low-pressure pump.
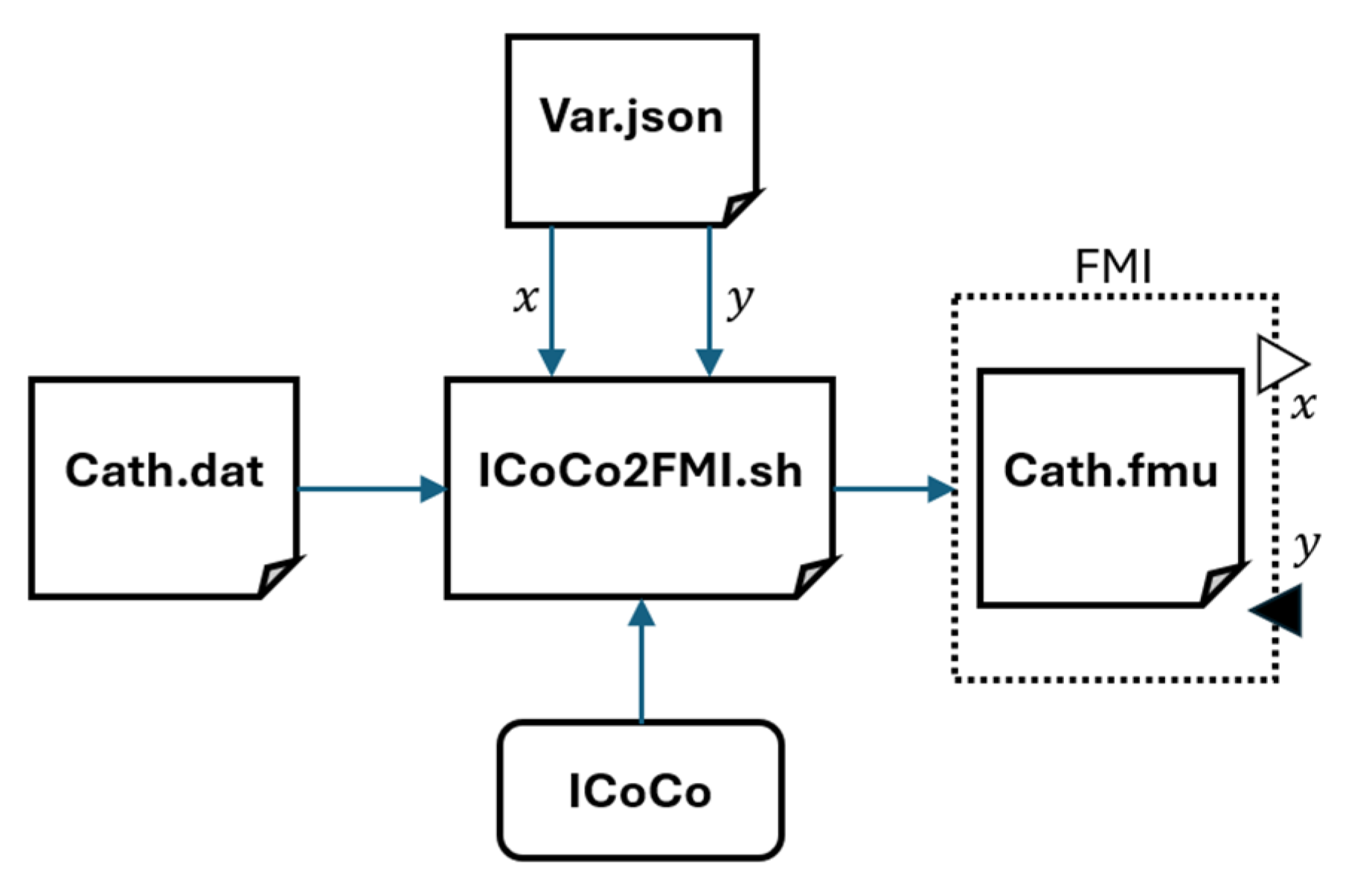
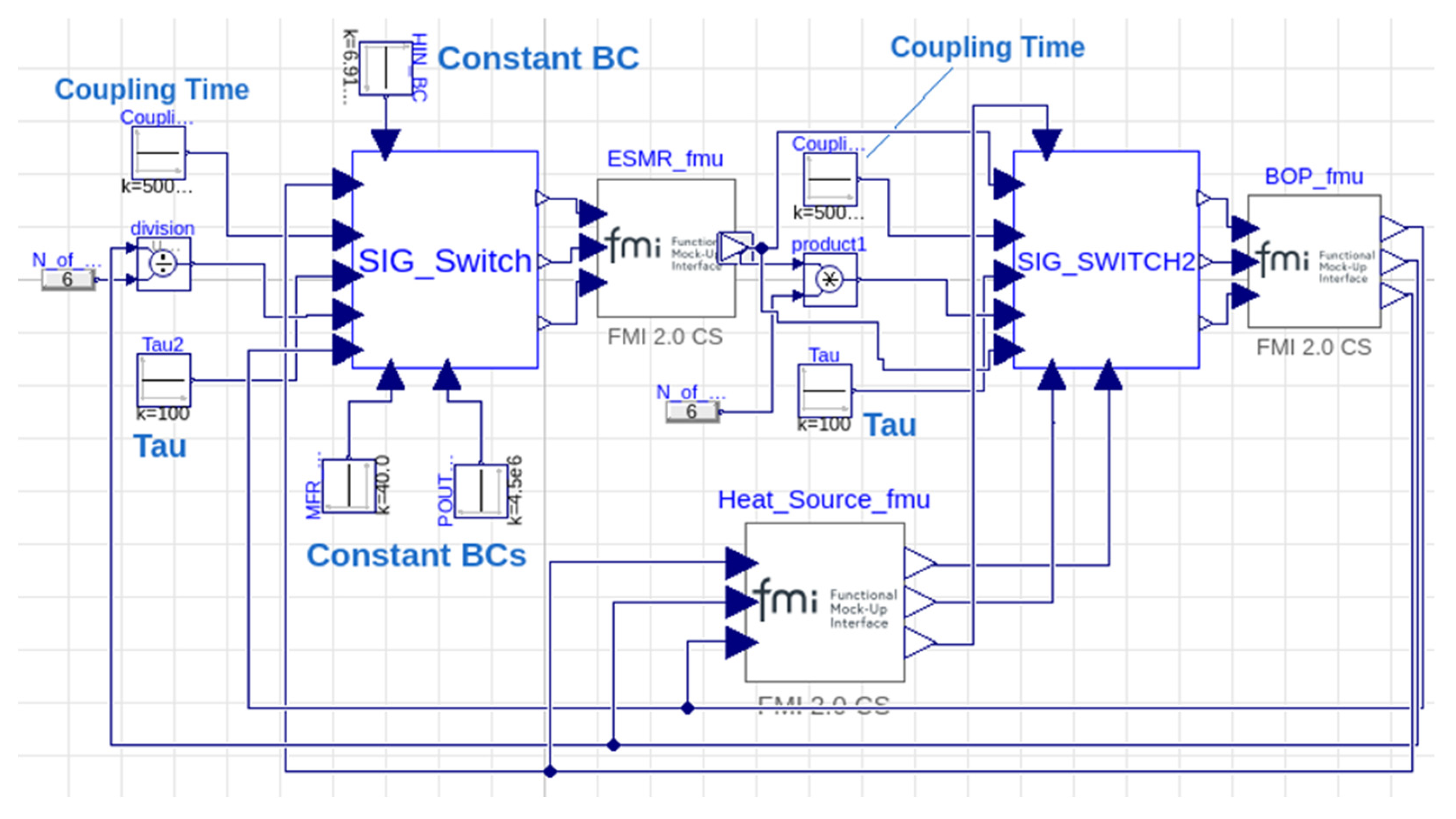
3. Description of the Coupling Strategy
4. Description of the Addressed Transient Scenarios
4.1. Cogeneration Start Transients
4.2. Core Power Variations During Cogeneration
4.3. Thermal Load Rejection
5. Obtained Results
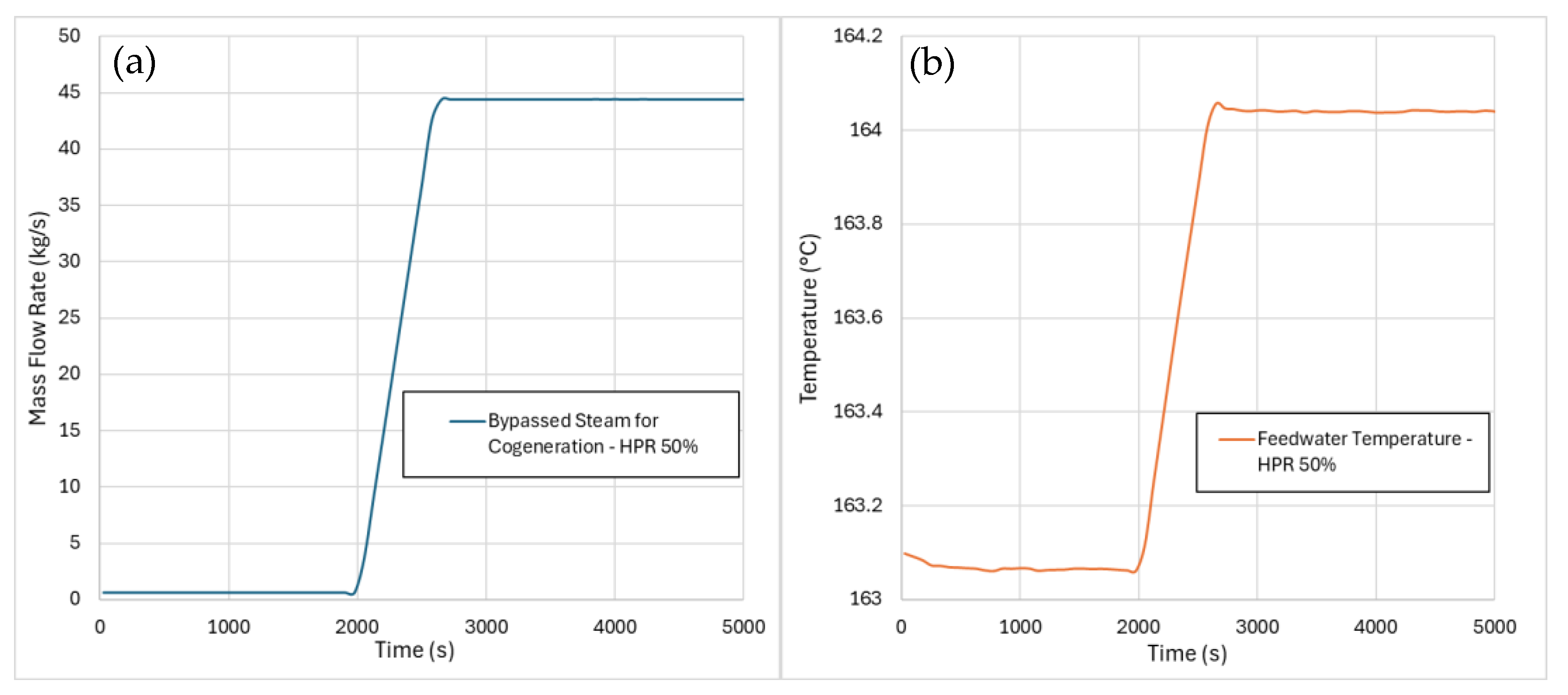
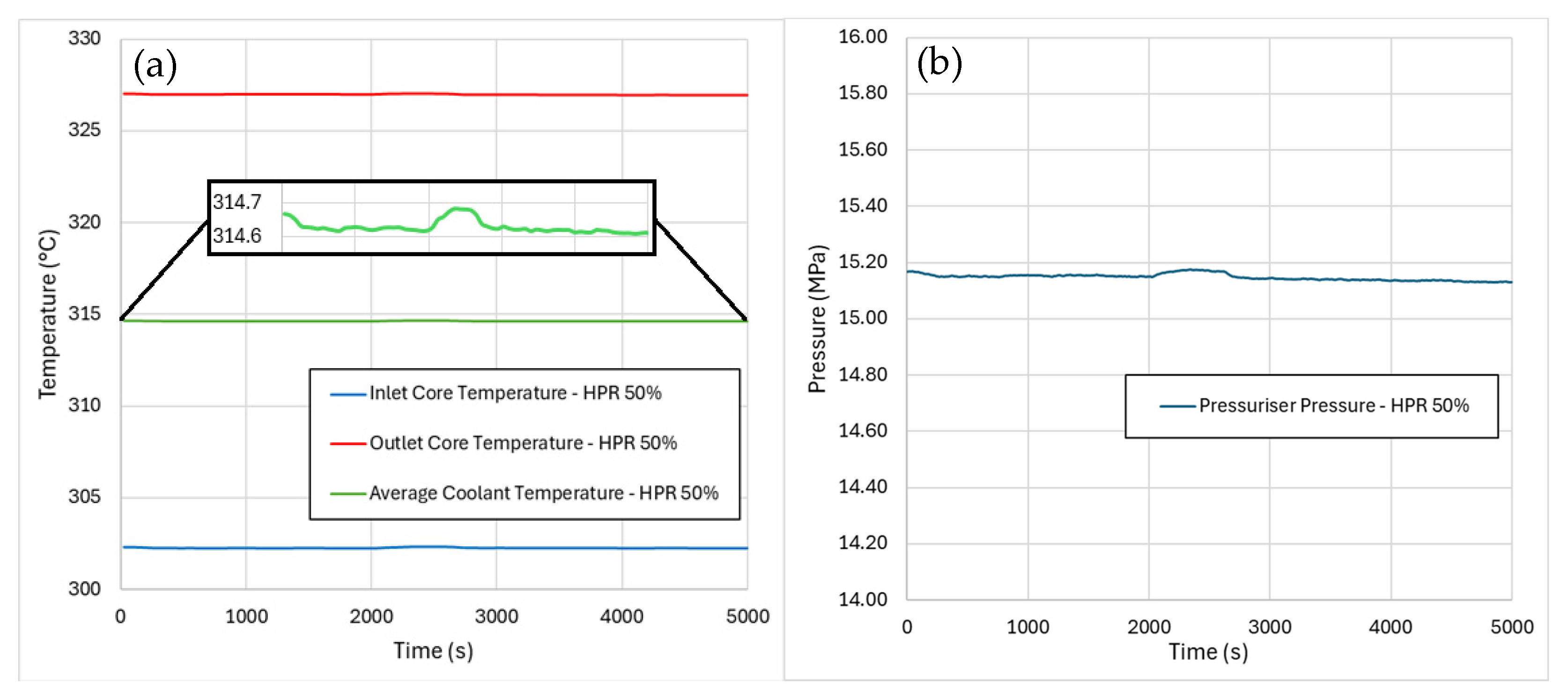
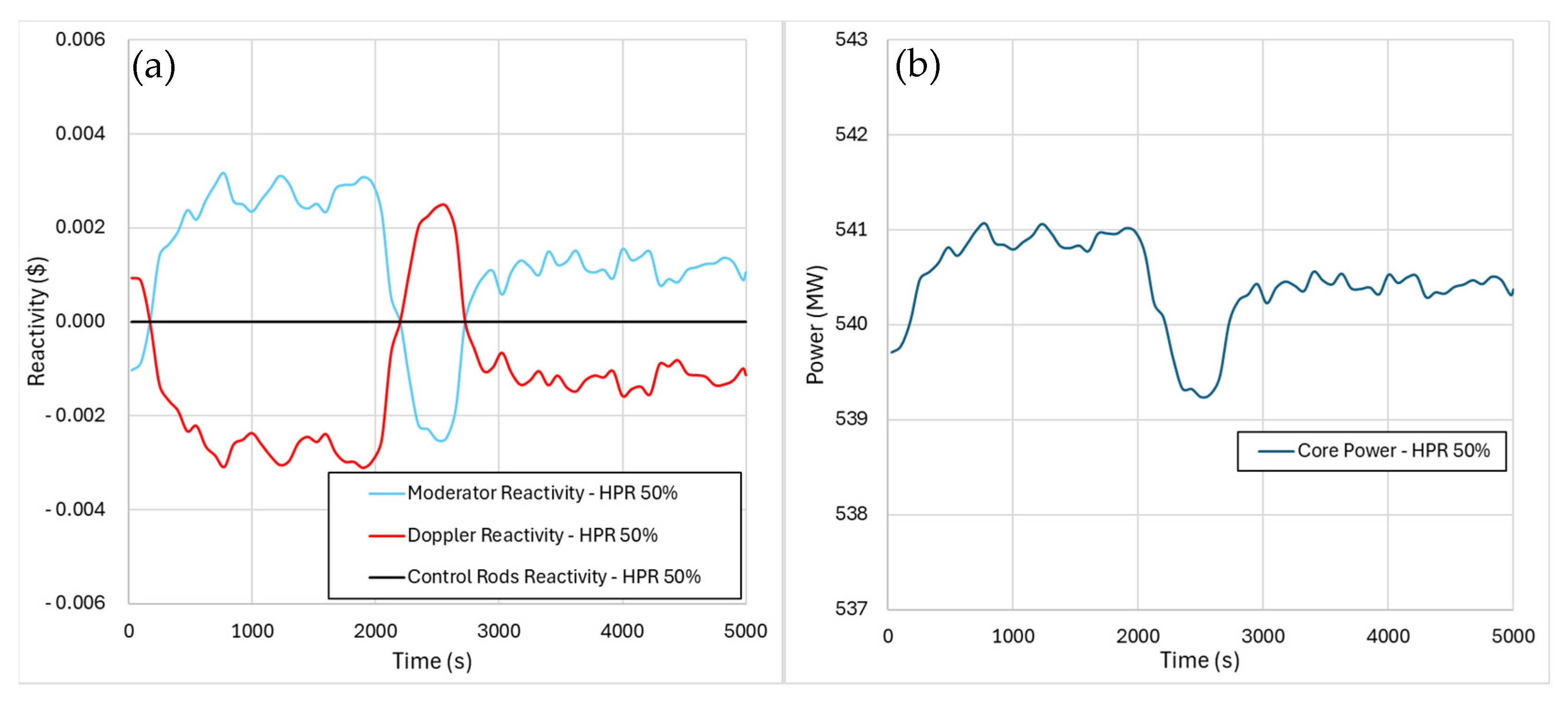
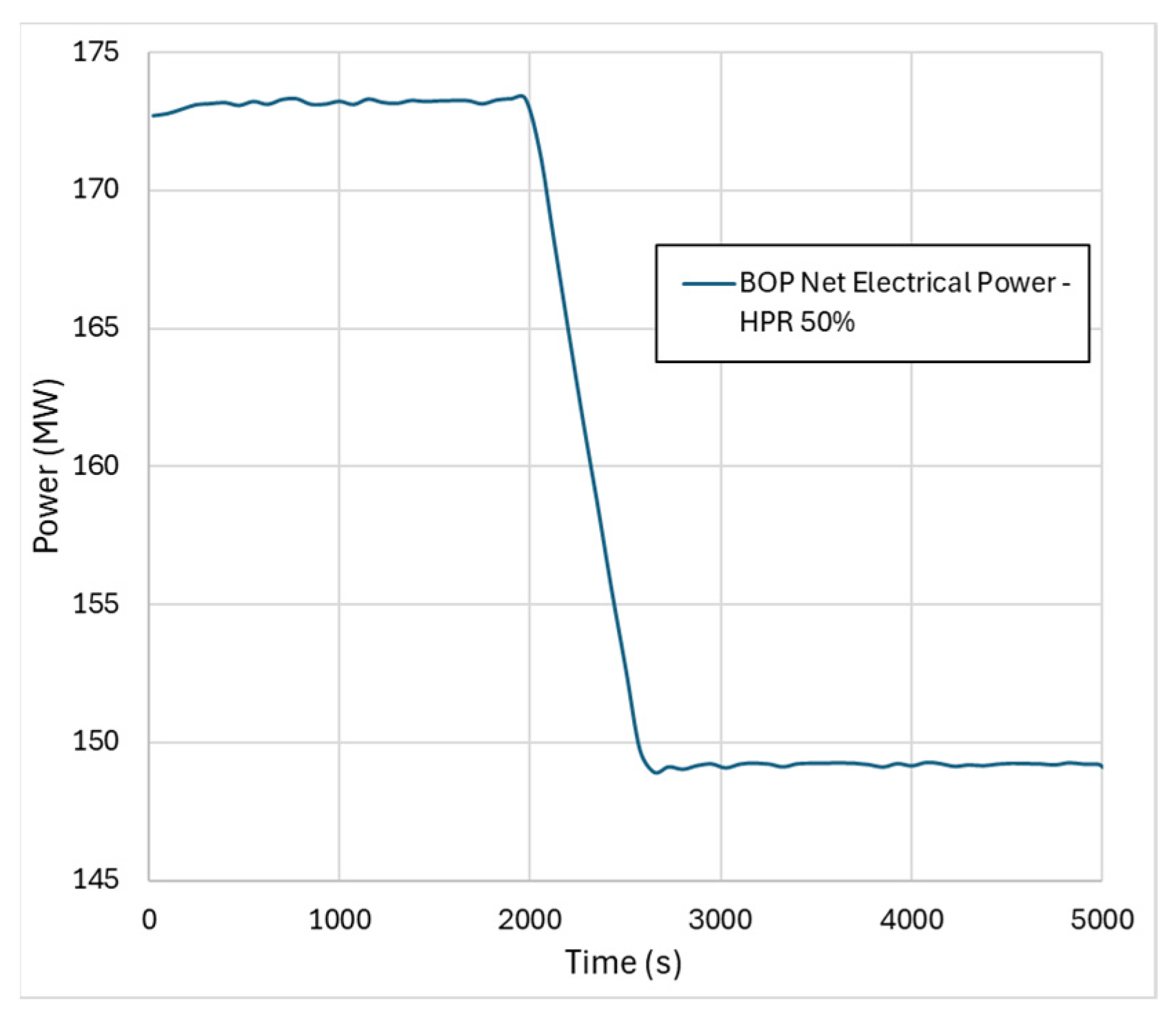
5.1. Results from Cogeneration Start Transients
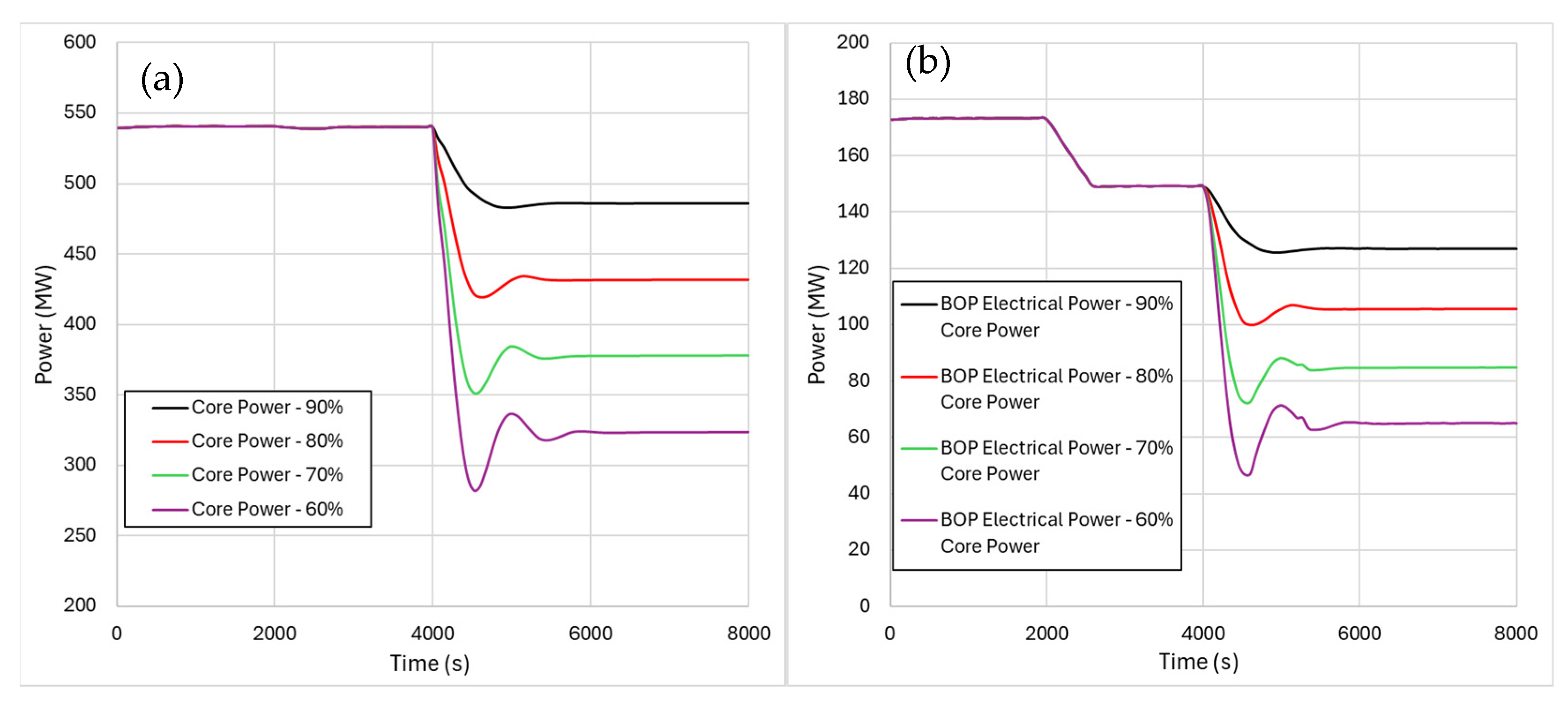
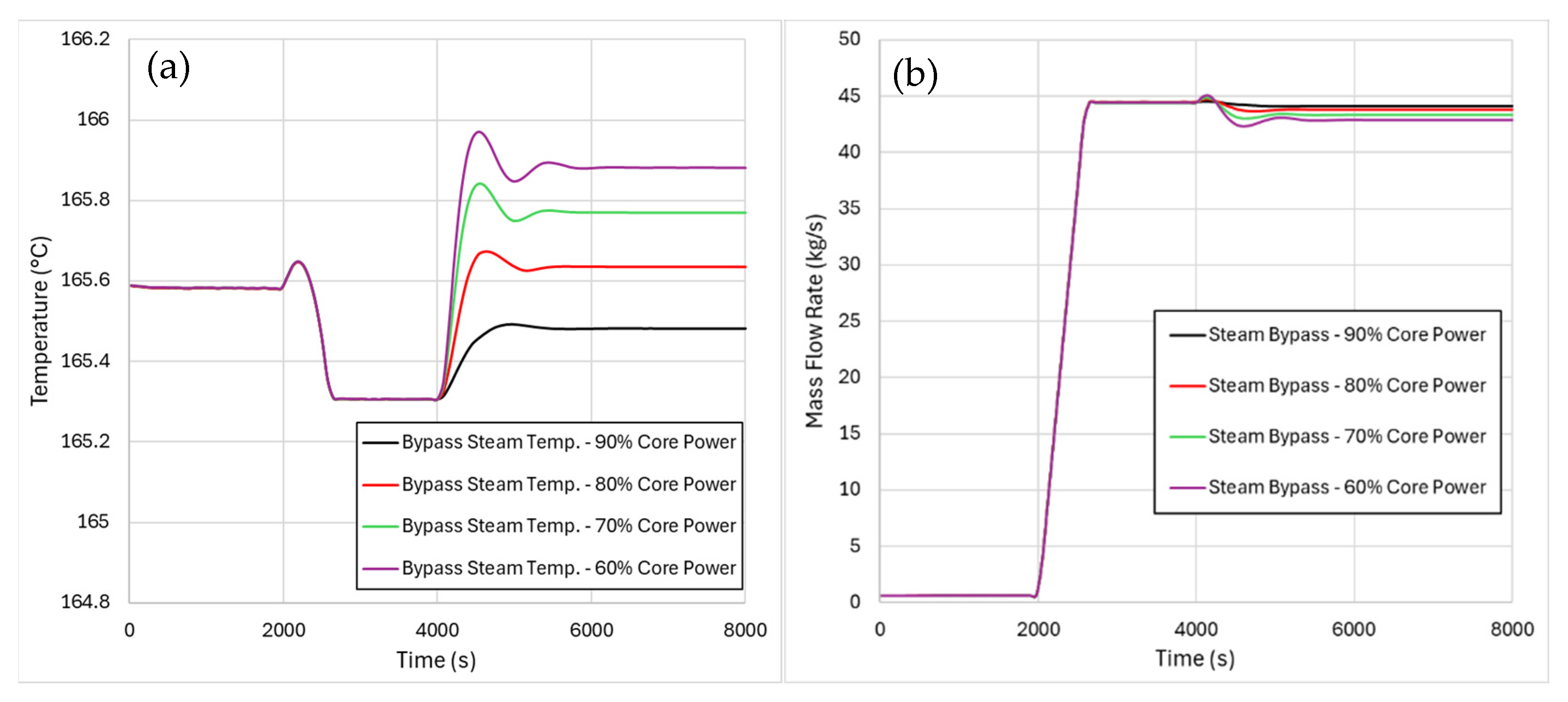
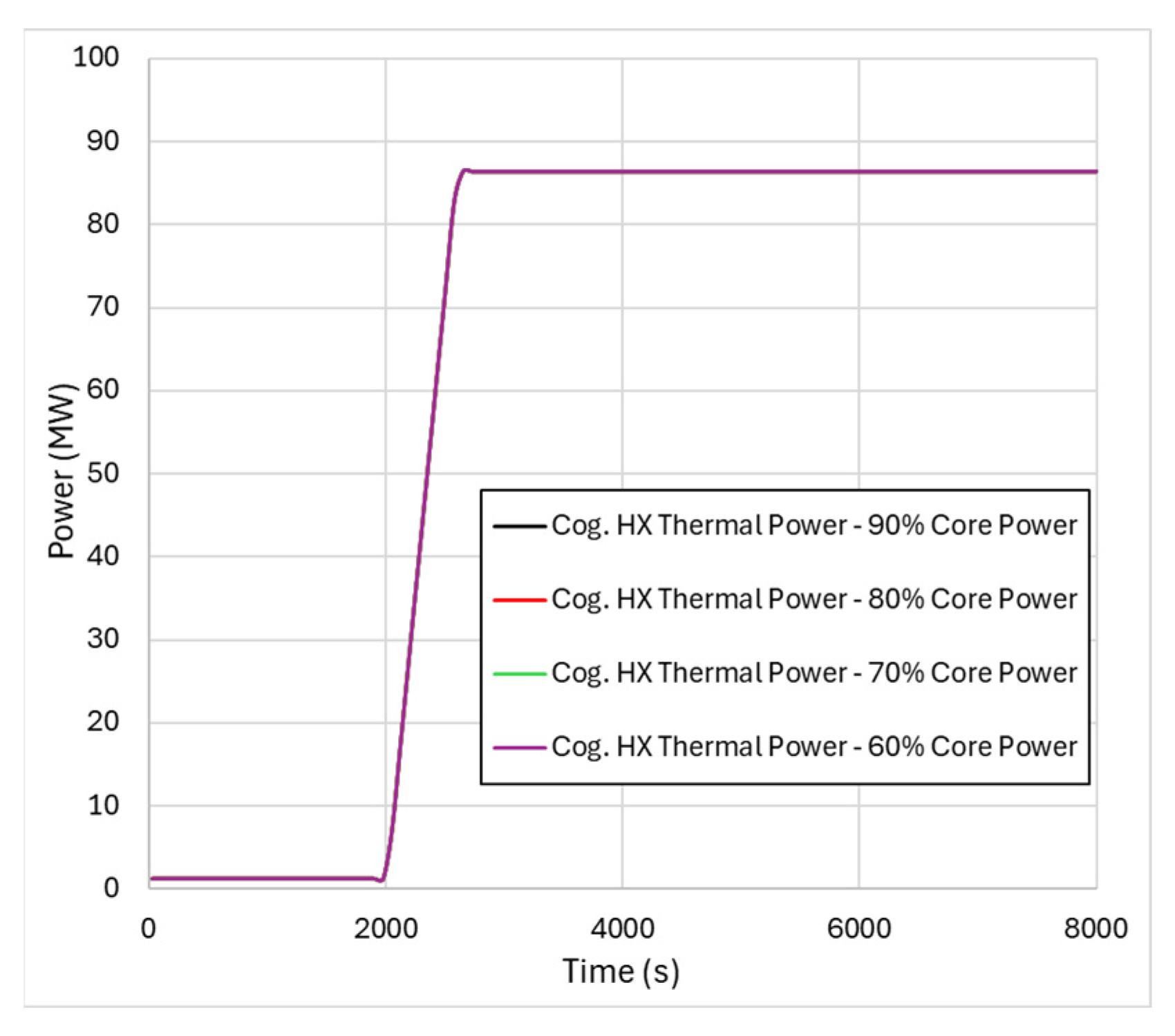
5.2. Results from Core Power Variation Transients
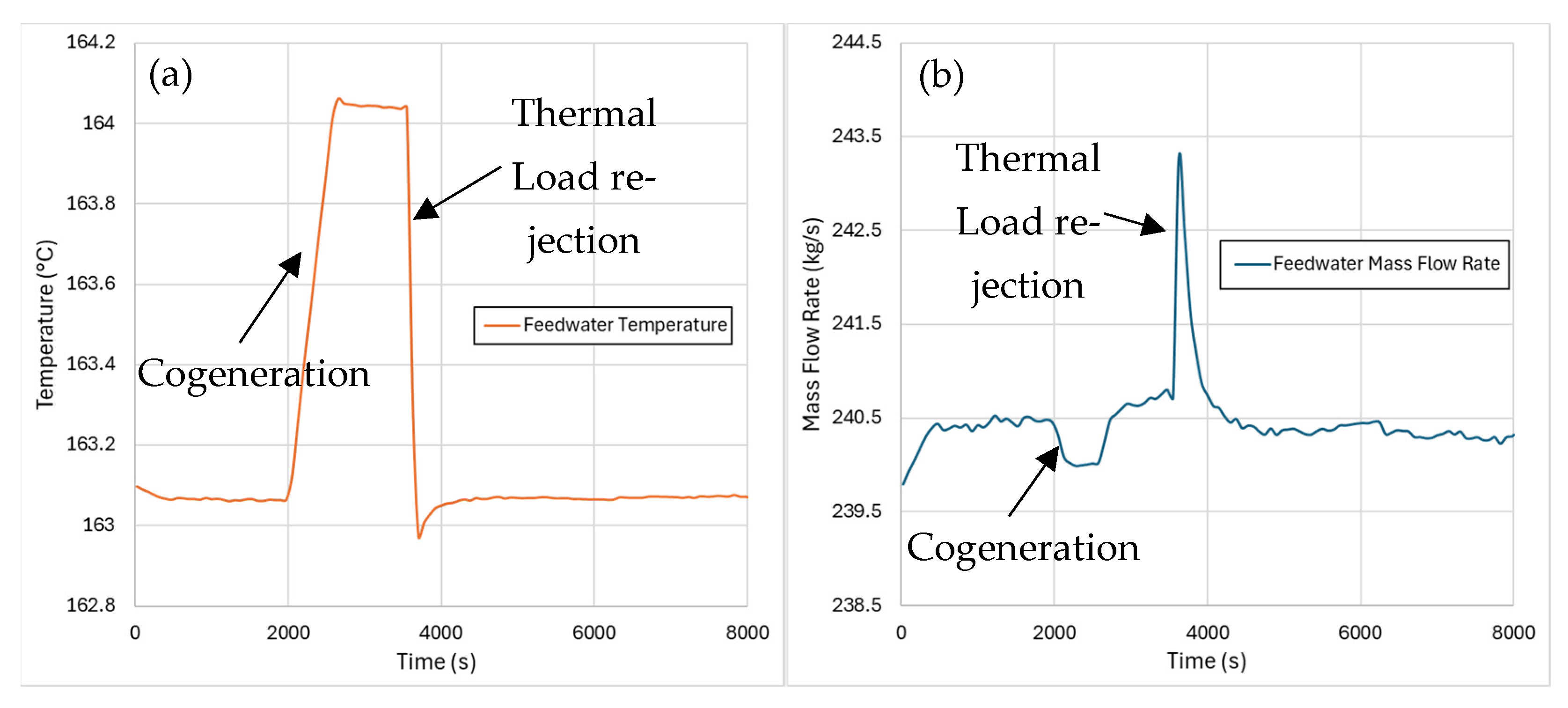
5.3. Results from Thermal Load Rejection Transients
6. Conclusions
- The cogeneration start transients show that the primary system was mildly influenced by a switch from full-electric to cogeneration operation, with different values of the heat-to-power ratio. In this regard, the highest value of HPR led to a negative core power excursion of 0.3% with respect to the reference nominal value. Moreover, as expected, the cogeneration resulted in an increase in the overall energy efficiency of the steam cycle, as a specific objective of its adoption.
- The core power variation transient results highlight the capability of the modelled nuclear power plant in handling core power variations while performing cogeneration. As a matter of fact, the BOP, thanks to the implemented control systems, was found to be capable of handling core power decreases of up to 60% of the nominal value, while keeping the thermal power provided to the heat user unchanged.
- The thermal load rejection scenario may be the case where the quasi-static assumption of the BOP may have mostly influenced the results, since the sharp change in the boundary conditions was instantaneously reflected on the output parameters and, in turn, on the primary side. However, the results further prove the stability of the developed code-coupling methodology and, in addition, they also show that the primary system was capable of handling such transient only relying on the modelled neutronic feedback.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations and Nomenclature
| Abbreviations | |
| BOP | balance of plant |
| CCV | cogeneration control valve |
| CSG | compact steam generator |
| ESMR | European Small Modular Reactor |
| FMI | Functional Mock-up Interface |
| FMU | Functional Mock-up Unit |
| HP | high pressure |
| HPR | heat-to-power ratio |
| HX | heat exchanger |
| LWR | light–water reactor |
| LW SMR | light–water small modular reactor |
| LP | low pressure |
| MP | medium pressure |
| N-R HES | Nuclear-Renewable Hybrid Energy System |
| PI | proportional integral |
| RES | renewable energy sources |
| SCSG | safety compact steam generator |
| SMR | Small Modular Reactor |
| TANDEM | Small Modular ReacTor for a European sAfe aNd Decarbonised Energy Mix |
| Roman Letters | |
| Error (-) | |
| Controller Gain (-) | |
| Pressure (MPa) | |
| Thermal Power (MW) | |
| Reactivity ($) | |
| Temperature (°C) | |
| Electrical Power (MW) | |
| Generic signals (-) | |
| Greek Letters | |
| Delayed neutron fraction (-) | |
| Decay constant (s−1) | |
| Efficiency (-) | |
References
- International Panel on Climate Change. Climate Change 2023 Synthesis Report, Contribution of Working Groups I, II and III to the Sixth Assessment Report of the Intergovernmental Panel on Climate Change; Core Writing Team, Lee, H., Romero, J., Eds.; IPCC: Geneva, Switzerland, 2023; pp. 35–115. [Google Scholar] [CrossRef]
- United Nations. Paris Agreement. 2015. Available online: https://unfccc.int/sites/default/files/english_paris_agreement.pdf (accessed on 4 April 2025).
- International Energy Agency. Net Zero by 2050: A Roadmap for the Global Energy Sector. 2021. Available online: https://iea.blob.core.windows.net/assets/deebef5d-0c34-4539-9d0c-10b13d840027/NetZeroby2050-ARoadmapfortheGlobalEnergySector_CORR.pdf (accessed on 3 April 2025).
- International Energy Agency. World Energy Outlook 2022, License: CC BY 4.0 (Report); CC BY NC SA 4.0 (Annex A). 2022. Available online: https://www.iea.org/reports/world-energy-outlook-2022 (accessed on 3 April 2025).
- International Energy Agency. CO2 Emissions in 2022, License: CC BY 4.0. 2022. Available online: https://www.iea.org/reports/co2-emissions-in-2022 (accessed on 2 April 2025).
- Joint Research Center. Technical Assessment of Nuclear Energy with Respect to the ‘Do No Significant Harm’ Criteria of Regulation (EU) 2020/852 (‘Taxonomy Regulation’); European Commission Joint Research Centre: Petten, The Netherlands, 2021; JRC124193. [Google Scholar]
- European Commission. A Clean Planet for All, A European Strategic Long-Term Vision for a Prosperous, Modern, Competitive and Climate Neutral Economy, Brussels, 28 November 2018; COM(2018) 773. Available online: https://eur-lex.europa.eu/legal-content/EN/TXT/PDF/?uri=CELEX:52018DC0773 (accessed on 26 March 2025).
- Amir, M.; Singh, K. Frequency regulation strategies in renewable energy-dominated power systems: Issues, challenges, innovations, and future trends. In Advanced Frequency Regulation Strategies in Renewable-Dominated in Power Systems; Academic Press: London, UK, 2024; Chapter 16. [Google Scholar] [CrossRef]
- Lazard. Levelized Cost of Energy Analysis v 16.0. 2023. Available online: https://www.lazard.com/media/2ozoovyg/lazards-lcoeplus-april-2023.pdf (accessed on 26 March 2025).
- Nuclear Energy Agency. The NEA Small Modular Reactor Dashboard: Second Edition, NEA No. 7671, © OECD 2024. Available online: https://oecd-nea.org/upload/docs/application/pdf/2024-02/7671_the_nea_smr_dashboard_-_second_edition.pdf (accessed on 20 March 2025).
- Pursiheimo, E.; Lindroos, T.J.; Sundell, D.; Rämä, M.; Tulkki, V. Optimal investment analysis for heat pumps and nuclear heat in decarbonised Helsinki metropolitan district heating system. Energy Storage Sav. 2022, 1, 80–92. [Google Scholar] [CrossRef]
- El-Emam, R.S.; Ozcan, H.; Bhattacharyya, R.; Awerbuch, L. Nuclear desalination: A sustainable route to water security. Desalination 2022, 542, 116082. [Google Scholar] [CrossRef]
- Locatelli, G.; Fiordaliso, A.; Boarin, S.; Ricotti, M.E. Cogeneration: An option to facilitate load following in Small Modular Reactors. Prog. Nucl. Energy 2017, 97, 153–161. [Google Scholar] [CrossRef]
- Bragg-Sitton, S.M. Hybrid Energy Systems using Small Modular Nuclear Reactors (SMRs). In Handbook of Small Modular Nuclear Reactors, 2nd ed.; Woodhead Publishing Series in Energy; Woodhead Publishing: Sawston, UK, 2021; pp. 323–356. [Google Scholar] [CrossRef]
- International Energy Agency. Energy to 2050: Scenarios for a Sustainable Future, IEA, Paris, 2004; Licence: CC BY 4.0. Available online: https://www.iea.org/reports/energy-to-2050-scenarios-for-a-sustainable-future (accessed on 23 March 2025).
- De Angelis, A.; Frignani, M.; Pucciarelli, A.; Sevbo, O.; Lapuente, M.M.; Schneidesch, C.; Vaglio-Gaudard, C.; Miss, J.; Hollands, T.; Ambrosini, W. Technology Review and Safety Assessment of Nuclear Renewable Hybrid Energy Systems with Light-Water Small Modular Reactors. In Proceedings of the 31st International Conference on Nuclear Engineering ICONE31, Prague, Czech Republic, 4–8 August 2024; ASME Digital Collection: New York, NY, USA, 2024; Volume 4, ISBN 978-0-7918-8824-7. [Google Scholar]
- Angulo, C.; Bogusch, E.; Bredimas, A.; Delannay, N.; Viala, C.; Ruer, J.; Muguerra, P.; Sibaud, E.; Chauvet, V.; Hittner, D.; et al. EUROPAIRS: The European project on coupling of High Temperature Reactors with industrial processes. Nucl. Eng. Des. 2012, 251, 30–37. [Google Scholar] [CrossRef]
- Wrochna, G.; Fütterer, M.; Hittner, D. Nuclear cogeneration with high temperature reactors. EPJ Nucl. Sci. Technol. 2020, 6, 31. [Google Scholar] [CrossRef]
- Vaglio-Gaudard, C.; Bautista, M.D.; Frignani, M.; Fütterer, M.; Goicea, A.; Hanus, E.; Hollands, T.; Lombardo, C.; Lorenzi, S.; Miss, J.; et al. The TANDEM Euratom project: Context, objectives and workplan. Nucl. Eng. Technol. 2024, 56, 993–1001. [Google Scholar] [CrossRef]
- Emonot, P.; Souyri, A.; Gandrille, J.L.; Barré, F. CATHARE-3: A new system code for thermal-hydraulic in the context of the NEPTUNE project. Nucl. Eng. Des. 2011, 231, 4476–4481. [Google Scholar] [CrossRef]
- Otter, M.; Elmqvist, H. Modelica Language, Libraries, Tools, Workshop and EU-Project RealSim. 2001. Available online: https://uweb.engr.arizona.edu/~cellier/ModelicaOverview14.pdf (accessed on 2 April 2025).
- De Angelis, A.; Olita, P.; Ambrosini, W. Preliminary Assessment of CATHARE-MODELICA Codes Coupling for Safety Assessment of Nuclear Cogeneration. In Proceedings of the 31st International Conference on Nuclear Engineering ICONE31, Prague, Czech Republic, 4–8 August 2024; ASME Digital Collection, ASME: New York, NY, USA, 2024; Volume 6, ISBN 978-0-7918-8826-1. [Google Scholar]
- MODELISAR Consortium. Functional Mock-Up Interface for Model Exchange and Co-Simulation, Version 2.0, Copyright © 2008–2011. 2014. Available online: https://fmi-standard.org/assets/releases/FMI_for_ModelExchange_and_CoSimulation_v2.0.pdf (accessed on 5 May 2025).
- Brück, D.; Elmqvist, H.; Olsson, H.; Mattsson, S.E. Dymola for Multi-Engineering Modelling and Simulation. In Proceedings of the 2nd International Modelica Conference, Oberpfaffenhofen, Germany, 18–19 March 2002; pp. 55-1–55-8. [Google Scholar]
- Simonini, G.; Hammadi, V.; Ferrara, S.; Hocine-Rastic, S.; Lorenzi, G.; Masotti, A.; Baudoux, D.; Jouret, B.; Tourneur, F.; Pappalardo, N.; et al. Modelica Models Description for the ‘TANDEM’ Library, EU TANDEM Deliverable 2.3. 2024. Available online: https://tandemproject.eu/wp-content/uploads/2024/07/D2_3_Modelica_models_description_for_the_tandem_library_V1-1.pdf (accessed on 4 April 2025).
- Tandem Modelica Library Official GitHub. Available online: https://gitlab.pam-retd.fr/tandem/tandem (accessed on 5 May 2025).
- Modelica Association, Modelica Standard Library Official GitHub Repository. Available online: https://github.com/modelica/ModelicaStandardLibrary (accessed on 5 May 2025).
- Szogradi, M.; Buchholz, S.; Lansou, S.; Lombardo, C.; Ferri, R.; Reinke, N.; De Angelis, A.; Davelaar, F.; Liegeard, C.; Bittan, J.; et al. ELSMOR—Towards European Licensing of Small Modular Reactors. In Proceedings of the European Nuclear Young Generation Forum ENYGF’23, Kraków, Poland, 8–12 May 2023. [Google Scholar]
- Lombardo, C.; Polidori, M.; Ricotti, M.; Masotti, G.; De Angelis, A.; Pucciarelli, A. CATHARE SMR Model Description, EU TANDEM Deliverable 2.6. 2024. Available online: https://tandemproject.eu/wp-content/uploads/2024/07/D2_6_cathare_smr_model_description.pdf (accessed on 27 March 2025).
- International Atomic Energy Agency. Advances in Small Modular Reactor Technology Developments, A Supplement to: IAEA Advanced Reactors Information System (ARIS). 2022. Available online: http://aris.iaea.org (accessed on 26 March 2025).
- Munoz-Cobo, J.L.; Escriva, A.; Garcıa, C. Consistent generation of point feedback reactivity parameters for stability and thermalhydraulic codes. Ann. Nucl. Energy 2006, 33, 1147–1156. [Google Scholar] [CrossRef]
- ThermoSysPro Modelica Library Official Website. Available online: https://thermosyspro.com/ (accessed on 27 March 2025).
- International Association for the Properties of Water and Steam (IAPWS). Release on the IAPWS Industrial Formulation 1997 for the Thermodynamic Properties of Water and Steam (IAPWS-IF97); IAPWS: Lucerne, Switzerland, 1997; Available online: https://www.iapws.org (accessed on 3 April 2025).
- Deville, E.; Perdu, F. Documentation of the Interface for Code Coupling: ICoCo, Commissariat à l’énergie atomique et aux énergies alternatives DEN/DANS/DM2S/STMF/LMES. 2012. Available online: https://docs.salome-platform.org/latest/extra/Interface_for_Code_Coupling.pdf (accessed on 22 March 2025).
- Fauchet, G. ICoCo2FMI Official Github Repository. 2022. Available online: https://github.com/cea-trust-platform/icoco-coupling/tree/ICoCo2FMI (accessed on 28 March 2025).
- Petzold, L.R. A description of DASSL: A differential/algebraic system solver. In Proceedings of the 10th IMACS World Congress, Montreal, QC, Canada, 8–13 August 1982. OSTI ID:5882821. [Google Scholar]
- Barre, F.; Bernard, M. The CATHARE code strategy and assessment. Nucl. Eng. Des. 1990, 124, 257–284. [Google Scholar] [CrossRef]
- Pucciarelli, A.; De Angelis, A.; Ambrosini, W.; Hollands, T.; Buchholz, S.; Wielenberg, A.; Caruso, J.; Baudrand, O.; Miss, J.; Sevbo, O.; et al. Status Report on Safety Analysis in Europe from the Operational Flexibility and Cogeneration Viewpoint, EU TANDEM Deliverable 4.1. 2023. Available online: https://tandemproject.eu/wp-content/uploads/2023/03/TANDEM_D4_1_Status_report_on_safety_analysis_in_Europe_from_the_operational_flexibility_andcogeneration_viewpoint_V1.pdf (accessed on 25 March 2025).
- Miss, J.; Vaglio-Gaudard, C. Identification of Potentially Impacted Safety Margins and Methodology for Safety Analysis of a SMR Integrated in a Hybrid System, TANDEM D4.2. 2023. Available online: https://tandemproject.eu/wp-content/uploads/2023/11/D4_2_Identification_of_potentially_impacted_safety_margins_and_methodology_for_safetyanalysis_of_a_smr_integrated_in_a_hybrid_system_V1.pdf (accessed on 27 March 2025).
- Morilhat, P.; Feutry, S.; Le Maitre, C.; Favennec, J.M. Nuclear Power Plant Flexibility at EDF, hal-01977209. 2019. Available online: https://edf.hal.science/hal-01977209/ (accessed on 26 March 2025).
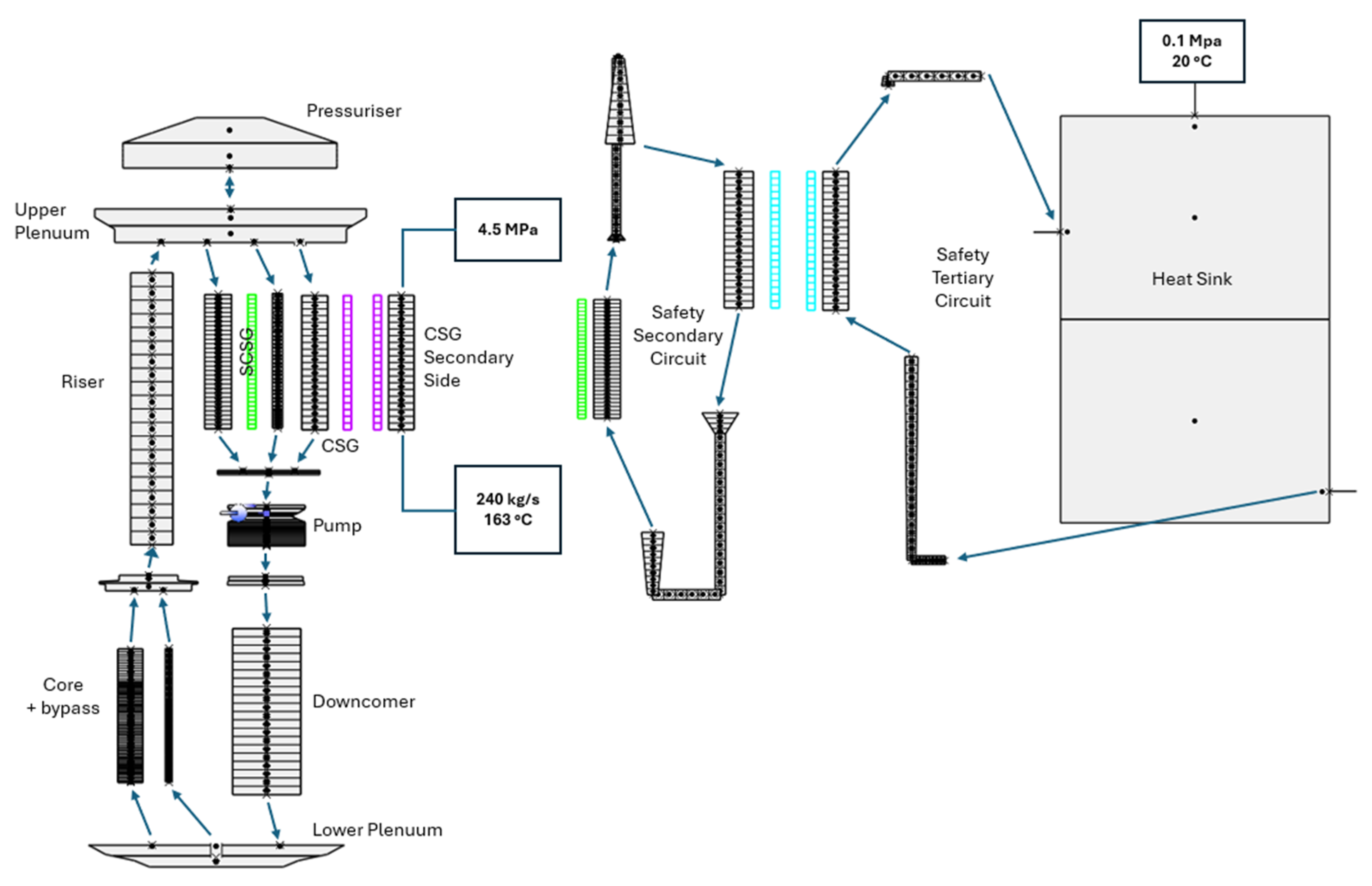
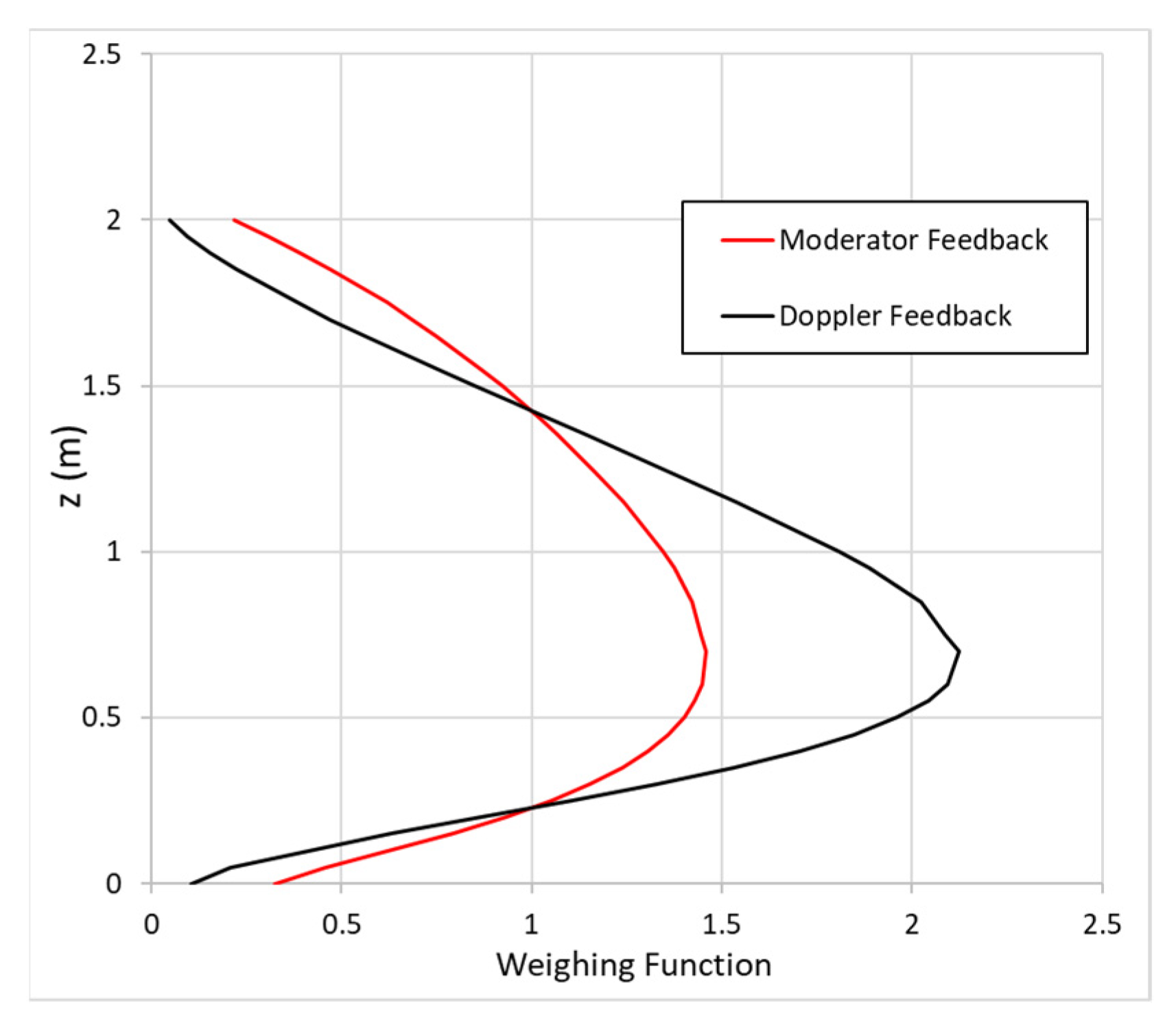
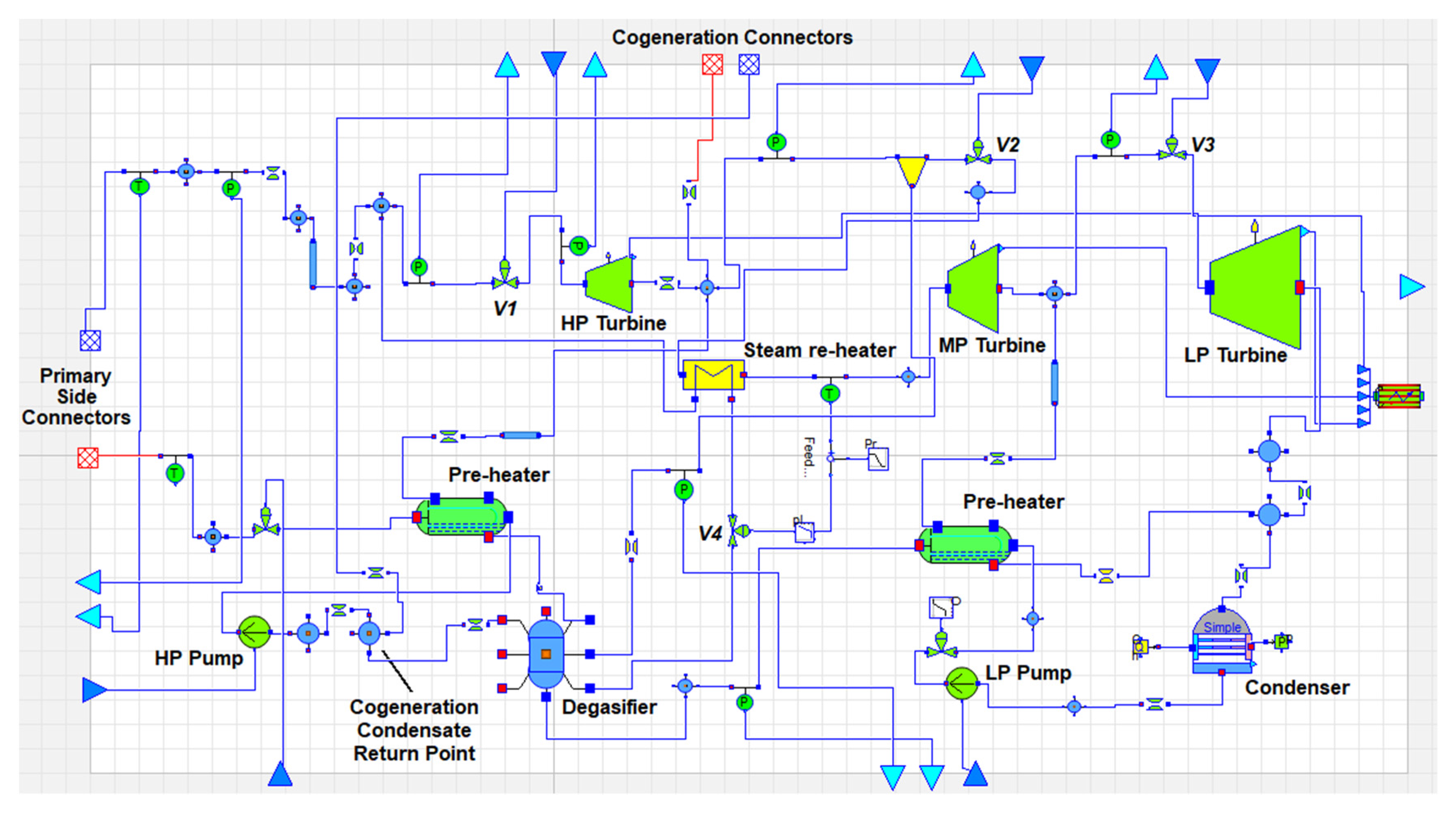
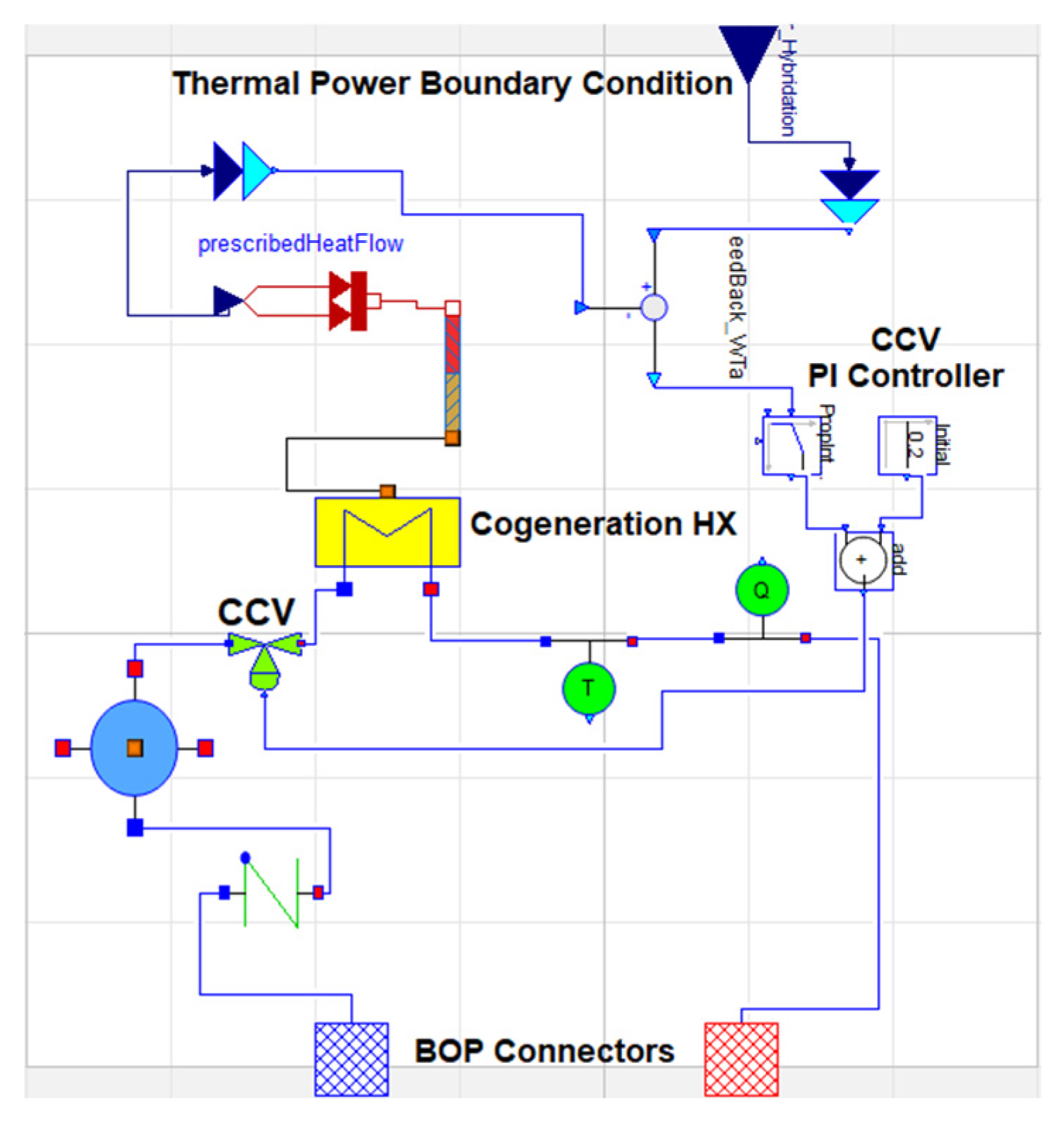







| Parameter | Value |
|---|---|
| Primary Side | |
| Core Thermal Power (MW) | 540 |
| Inlet Core Temperature (°C) | 300 |
| Core Coolant ΔT (°C) | 24.5 |
| Core Coolant Δp (MPa) | 0.58 |
| Pressuriser Pressure (MPa) | 15 |
| Coolant Mass Flow Rate (kg/s) | 3700 |
| Secondary Side | |
| Feedwater Temperature (°C) | 163 |
| SH Steam Temperature (°C) | 300 |
| Outlet Pressure (MPa) | 4.5 |
| Coolant Mass Flow Rate (kg/s) | 240 |
| Group | (-) | (s−1) |
|---|---|---|
| 1 | 1.66·10−4 | 0.0125 |
| 2 | 1.02·10−3 | 0.0314 |
| 3 | 9.63·10−4 | 0.1102 |
| 4 | 2.72·10−3 | 0.3209 |
| 5 | 8.95·10−4 | 1.3280 |
| 6 | 2.89·10−4 | 3.01 |
| TOT. | 6.053·10−3 | - |
| HPR | Heat User Thermal Power Demand (MW) |
|---|---|
| 10% | 17.3 |
| 20% | 34.6 |
| 30% | 51.9 |
| 40% | 69.1 |
| 50% | 86.5 |
| HPR | Nominal Core Power (MW) | Thermal Power Delivered to the Heat User (MW) | Core Power with Cogeneration at Steady State (MW) | Net BOP Electrical Power (MW) | Combined System Efficiency (%) |
|---|---|---|---|---|---|
| 0% | 540.89 | - | - | 173.30 | 32.0 |
| 10% | 540.89 | 17.30 | 540.66 | 168.50 | 34.3 |
| 20% | 540.89 | 34.60 | 540.62 | 163.60 | 36.6 |
| 30% | 540.89 | 51.90 | 540.55 | 158.60 | 38.9 |
| 40% | 540.89 | 69.10 | 540.50 | 153.80 | 41.2 |
| 50% | 540.89 | 86.50 | 540.39 | 149.20 | 43.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
De Angelis, A.; Alpy, N.; Olita, P.; Lombardo, C.; Ambrosini, W. Numerical Assessment of Nuclear Cogeneration Transients with SMRs Using CATHARE 3–MODELICA Coupling. Energies 2025, 18, 2539. https://doi.org/10.3390/en18102539
De Angelis A, Alpy N, Olita P, Lombardo C, Ambrosini W. Numerical Assessment of Nuclear Cogeneration Transients with SMRs Using CATHARE 3–MODELICA Coupling. Energies. 2025; 18(10):2539. https://doi.org/10.3390/en18102539
Chicago/Turabian StyleDe Angelis, Alessandro, Nicolas Alpy, Paolo Olita, Calogera Lombardo, and Walter Ambrosini. 2025. "Numerical Assessment of Nuclear Cogeneration Transients with SMRs Using CATHARE 3–MODELICA Coupling" Energies 18, no. 10: 2539. https://doi.org/10.3390/en18102539
APA StyleDe Angelis, A., Alpy, N., Olita, P., Lombardo, C., & Ambrosini, W. (2025). Numerical Assessment of Nuclear Cogeneration Transients with SMRs Using CATHARE 3–MODELICA Coupling. Energies, 18(10), 2539. https://doi.org/10.3390/en18102539






