Energy, Exergic and Economic Analyses of a Novel Hybrid Solar–Gas System for Producing Electrical Power and Cooling
Abstract
1. Introduction
2. Design of the Solar–Gas System
3. Research Methods
3.1. Modeling Method
3.2. Traditional Exergic Analysis Equations
3.3. Advanced Exergic Analysis Equations
3.3.1. Exergic Loss Splitting
3.3.2. Equation of Endogenous and Exogenous Exergic Losses
3.3.3. Equation of Avoidable and Unavoidable Exergic Losses
3.4. Economic Analysis Equations
4. Results and Discussion
4.1. Operation Performance
4.2. Traditional Exergic Analysis
4.3. Advanced Exergic Analysis
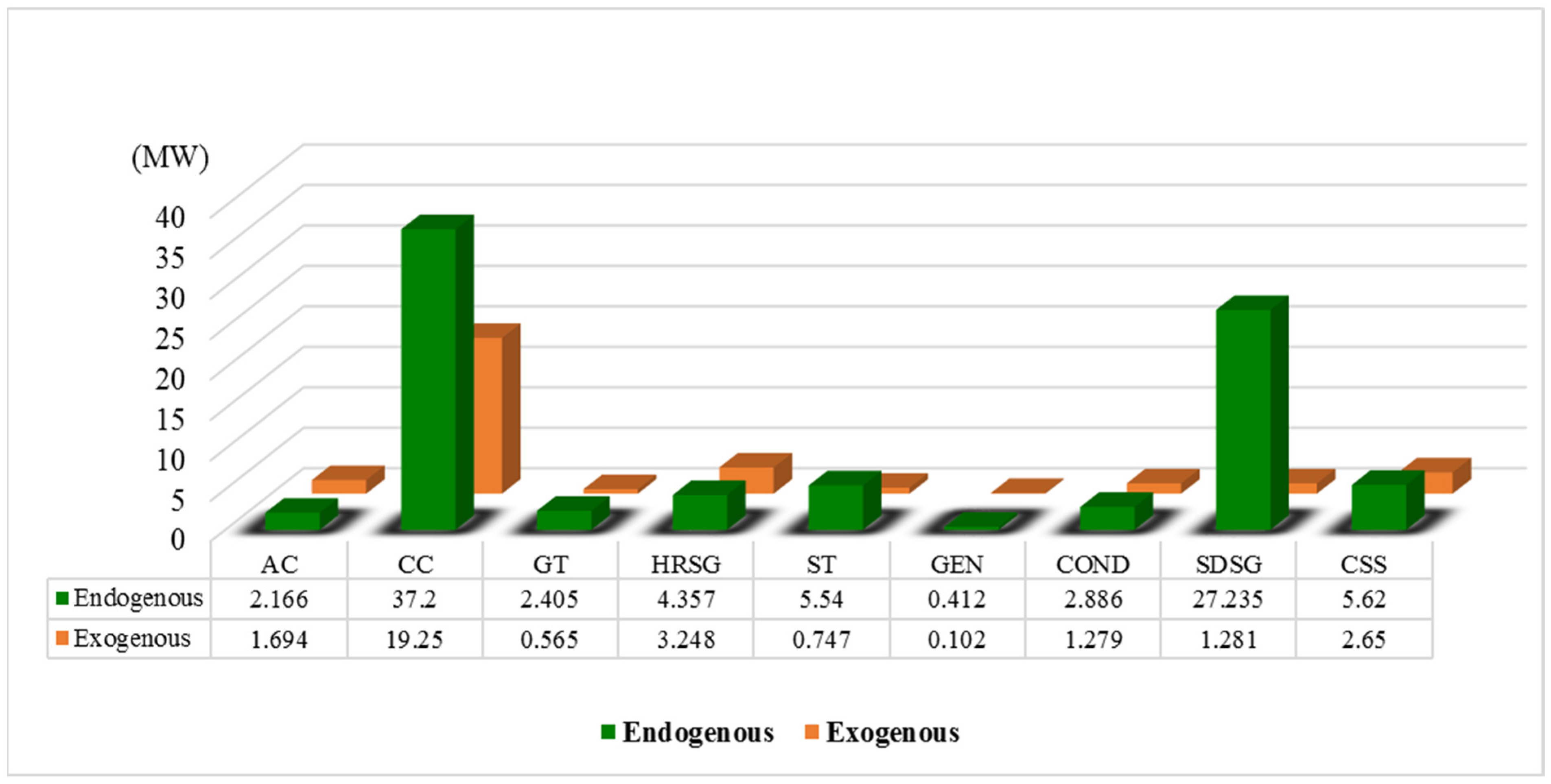
4.3.1. Endogenous and Exogenous Exergic Losses
4.3.2. Avoidable and Unavoidable Exergic Losses
4.4. Economic Analysis
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| C | Cost or income (USD) |
| egird | On-grid electricity price (USD/kwh) |
| Ed | Exergic loss (MW) |
| Ex,solar | Solar radiation exergy input of the SDSG (MW) |
| INy | Annual income (USD) |
| k | Proportion (-) |
| LCOE | Levelized cost of energy (USD/kwh) |
| My | Annual electric power generation (kwh) |
| rdis | Discount rate (-) |
| W | Power (MW) |
| Greek symbols | |
| η | Exergic efficiency (-) |
| Subscripts | |
| av | Avoidable |
| d | Exergic loss |
| en | Endogenous |
| ex | Exogenous |
| fom | Fixed operation and maintenance |
| out | Output |
| un | Unavoidable |
| vom | Varying operation and maintenance |
| x | Exergic |
| y | Annual |
| Abbreviation | |
| AC | Air compressor |
| CC | Combustion chamber |
| CH | Condensate heater |
| CP | Condensate pump |
| COND | Condenser |
| CSS | Cooling supply system |
| GEN | Power generator |
| GT | Gas turbine |
| HPD | High-pressure drum |
| HPEC | High-pressure economizer |
| HPEV | High-pressure evaporator |
| HPFP | High-pressure feedwater pump |
| HPSH | High-pressure super-heater |
| HRSG | Heat recovery steam generator |
| HS | High pressure stages of the steam turbine |
| HWH | Hot water heater |
| ISCC | Integrated solar–gas combined cycle |
| LPD | Low-pressure drum |
| LPEV | Low-pressure evaporator |
| LPSH | Low-pressure super-heater |
| LPSSP | Low-pressure steam supply pipe |
| LS | Low-pressure stages of the steam turbine |
| PTC | Parabolic trough collector |
| SDSG | Solar direct steam generator |
| ST | Steam turbine |
References
- Mokhtar, G.; Boussad, B.; Noureddine, S. A linear Fresnel reflector as a solar system for heating water: Theoretical and experimental study. Case Stud. Therm. Eng. 2016, 8, 176–186. [Google Scholar] [CrossRef]
- Wang, G.; Zhang, S.; Zou, T. Design and comparison study of a novel linear Fresnel reflector solar ORC-driven hydrogen production system using different working fluids. Case Stud. Therm. Eng. 2025, 71, 106187. [Google Scholar] [CrossRef]
- Wang, G.; Liu, J.; Cao, Y.; Chen, Z. Design and performance estimation of a novel solar multiplate mirror concentration PVT system based on nanofluid spectral filter. Renew. Energy 2024, 235, 121321. [Google Scholar] [CrossRef]
- Wang, G.; Chao, Y.; Chen, Z. Promoting developments of hydrogen powered vehicle and solar PV hydrogen production in China: A study based on evolutionary game theory method. Energy 2021, 237, 121649. [Google Scholar] [CrossRef]
- Wang, G.; Liu, J. Design and performance evaluation of a novel photovoltaic and concentrated solar thermal system using parabolic trough-shaped spectrum filter. Energy Sources Part A Recovery Util. Environ. Eff. 2025, 47, 6918–6933. [Google Scholar] [CrossRef]
- Srinivas, T.; Reddy, B.V. Hybrid solar–biomass power plant without energy storage. Case Stud. Therm. Eng. 2014, 2, 75–81. [Google Scholar] [CrossRef]
- Hong, H.; Peng, S.; Zhao, Y.; Liu, Q.; Jin, H. A Typical Solar-coal Hybrid Power Plant in China. Energy Procedia 2014, 49, 1777–1783. [Google Scholar] [CrossRef]
- Wang, G.; Zhang, Z.; Lin, J. Multi-energy complementary power systems based on solar energy: A review. Renew. Sustain. Energy Rev. 2024, 199, 114464. [Google Scholar] [CrossRef]
- Khare, V.; Nema, S.; Baredar, P. Solar–wind hybrid renewable energy system: A review. Renew. Sustain. Energy Rev. 2016, 58, 23–33. [Google Scholar] [CrossRef]
- Wang, G.; Wang, C.; Chen, Z.; Hu, P. Design and performance evaluation of an innovative solar-nuclear complementarity power system using the S-CO2 Brayton cycle. Energy 2020, 197, 117282. [Google Scholar] [CrossRef]
- Nezammahalleh, H. Exergy analysis of DSG parabolic trough collectors for the optimal integration with a combined cycle. Int. J. Exergy 2015, 16, 72–96. [Google Scholar] [CrossRef]
- Tsatsaronis, G.; Park, M.H. On avoidable and unavoidable exergy destructions and investment costs in thermal systems. Energy Convers. Manag. 2002, 43, 1259–1270. [Google Scholar] [CrossRef]
- Tsatsaronis, G.; Kelly, S.O.; Morosuk, T.V. Endogenous and Exogenous Exergy Destruction in Thermal Systems. In Proceedings of the ASME 2006 International Mechanical Engineering Congress and Exposition, Chicago, IL, USA, 5–10 November 2006. [Google Scholar]
- Cziesla, F.; Tsatsaronis, G.; Gao, Z. Avoidable thermodynamic inefficiencies and costs in an externally fired combined cycle power plant. Energy 2006, 31, 1472–1489. [Google Scholar] [CrossRef]
- Kelly, S.; Tsatsaronis, G.; Morosuk, T. Advanced exergetic analysis: Approaches for splitting the exergy destruction into endogenous and exogenous parts. Energy 2009, 34, 384–391. [Google Scholar] [CrossRef]
- Ameri, M.; Mohammadzadeh, M. Thermodynamic, thermoeconomic and life cycle assessment of a novel integrated solar combined cycle (ISCC) power plant. Sustain. Energy Technol. Assess. 2018, 27, 192–205. [Google Scholar] [CrossRef]
- Tsatsaronis, G.; Morosuk, T. Advanced exergetic analysis of a novel system for generating electricity and vaporizing liquefied natural gas. Energy 2010, 35, 820–829. [Google Scholar] [CrossRef]
- Morosuk, T.; Tsatsaronis, G. Advanced exergetic evaluation of refrigeration machines using different working fluids. Energy 2009, 34, 2248–2258. [Google Scholar] [CrossRef]
- Penkuhn, M.; Tsatsaronis, G. A decomposition method for the evaluation of component interactions in energy conversion systems for application to advanced exergy-based analyses. Energy 2017, 133, 388–403. [Google Scholar] [CrossRef]
- Acikkalp, E.; Aras, H.; Hepbasli, A. Advanced exergoeconomic analysis of an electricity-generating facility that operates with natural gas. Energy Convers. Manag. 2014, 78, 452–460. [Google Scholar] [CrossRef]
- Petrakopoulou, F.; Tsatsaronis, G.; Morosuk, T. Evaluation of a power plant with chemical looping combustion using an advanced exergoeconomic analysis. Sustain. Energy Technol. Assess. 2013, 3, 9–16. [Google Scholar] [CrossRef]
- Spelling, J.; Laumert, B. Thermo-economic Evaluation of Solar Thermal and Photovoltaic Hybridization Options for Combined-Cycle Power Plants. J. Eng. Gas Turbines Power 2015, 137, 031801. [Google Scholar] [CrossRef]
- Achour, L.; Bouharkat, M.; Behar, O. Performance assessment of an integrated solar combined cycle in the southern of Algeria. Energy Rep. 2018, 4, 207–217. [Google Scholar] [CrossRef]
- Bellos, E.; Tzivanidis, C.; Antonopoulos, K.A. Parametric analysis and optimization of a solar assisted gas turbine. Energy Convers. Manag. 2017, 139, 151–165. [Google Scholar] [CrossRef]
- Wang, S. Exergy Analysis and Operation Behavior Study of Solar-Gas Combined Cycle System. Master’s Thesis, Northeast Electric Power University, Jilin, China, 2022. [Google Scholar]
- Wang, S.; Fu, Z. Thermodynamic Investigation of an Integrated Solar Combined Cycle with an ORC System. Entropy 2019, 21, 428. [Google Scholar] [CrossRef]
- Anvari, S.; Saray, R.K.; Bahlouli, K. Conventional and advanced exergetic and exergoeconomic analyses applied to a tri-generation cycle for heat, cold and power production. Energy 2015, 91, 925–939. [Google Scholar] [CrossRef]
- Wang, S.; Fu, Z.; Zhang, G.; Zhang, T. Advanced Thermodynamic Analysis Applied to an Integrated Solar Combined Cycle System. Energies 2018, 11, 1574. [Google Scholar] [CrossRef]
- Al-Sulaiman, F.A. Exergy analysis of parabolic trough solar collectors integrated with combined steam and organic Rankine cycles. Energy Convers. Manag. 2014, 77, 441–449. [Google Scholar] [CrossRef]
- Lorenczik, S.; Keppler, J.H. Projected Costs of Generating Electricity; International Energy Agency: Paris, France, 2020.
- Morosuk, T.; Tsatsaronis, G. Advanced Exergy Analysis for Chemically Reacting Systems—Application to a Simple Open Gas-Turbine System. Int. J. Thermodyn. 2009, 12, 105–111. [Google Scholar]
- Adibhatla, S.; Kaushik, S.C. Energy, exergy and economic (3E) analysis of integrated solar direct steam generation combined cycle power plant. Sustain. Energy Technol. Assess. 2017, 20, 88–97. [Google Scholar] [CrossRef]
- Roosen, P.; Uhlenbruck, S.; Lucas, K. Pareto optimization of a combined cycle power system as a decision support tool for trading off investment vs. operating costs. Int. J. Therm. Sci. 2003, 42, 553–560. [Google Scholar] [CrossRef]
- Zhang, P.; Liu, M.; Hu, W.; Chong, D.; Yan, J. System design and thermo-economic analysis of a novel gas turbine combined cycle co-driven by methanol and solar energy. Appl. Energy 2025, 380, 125030. [Google Scholar] [CrossRef]
- Baghernejad, A.; Yaghoubi, M. Exergy analysis of an integrated solar combined cycle system. Renew. Energy 2010, 35, 2157–2164. [Google Scholar] [CrossRef]
- Benabdellah, H.M.; Ghenaiet, A. Energy, exergy, and economic analysis of an integrated solar combined cycle power plant. Eng. Rep. 2021, 3, e12404. [Google Scholar] [CrossRef]
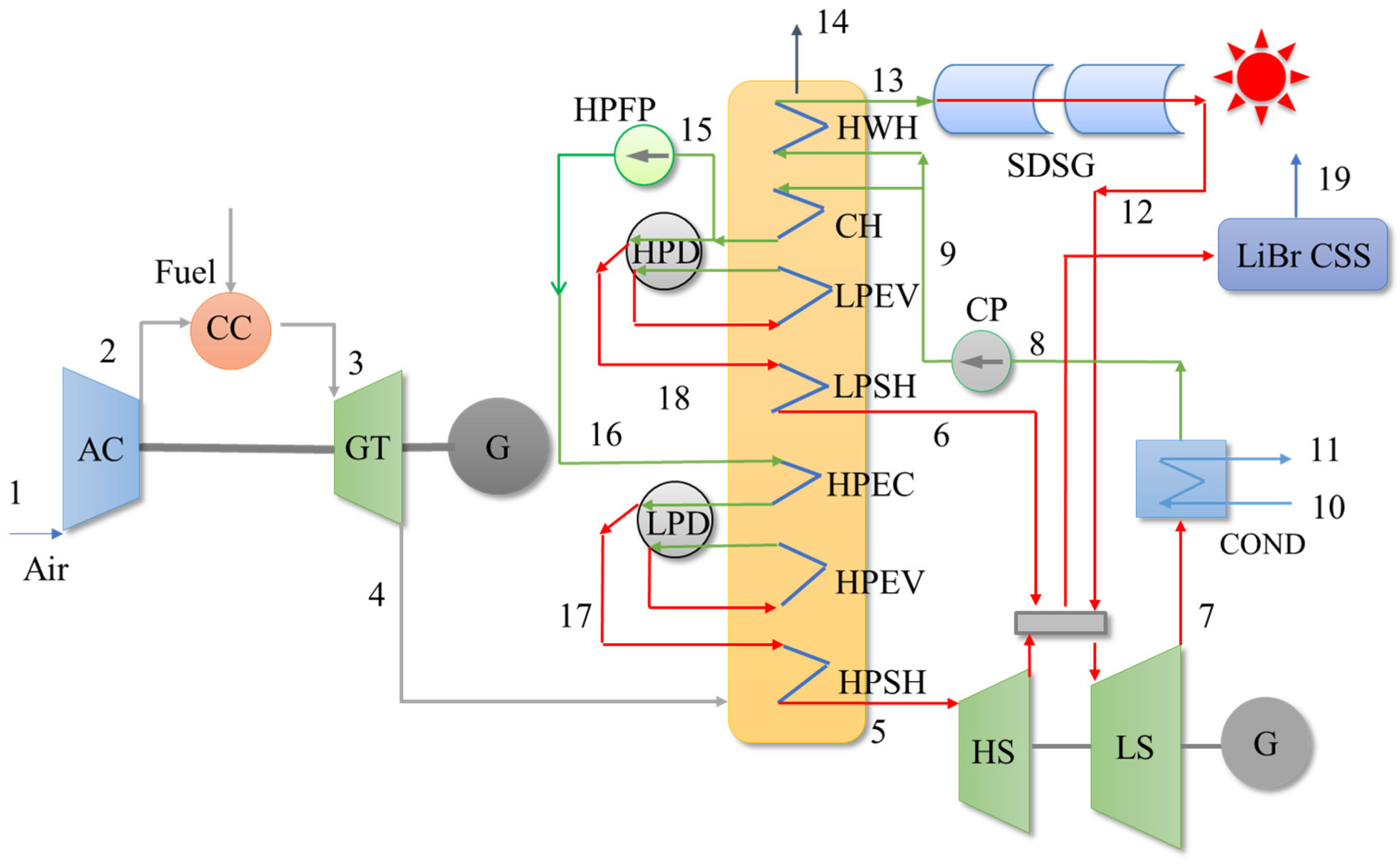
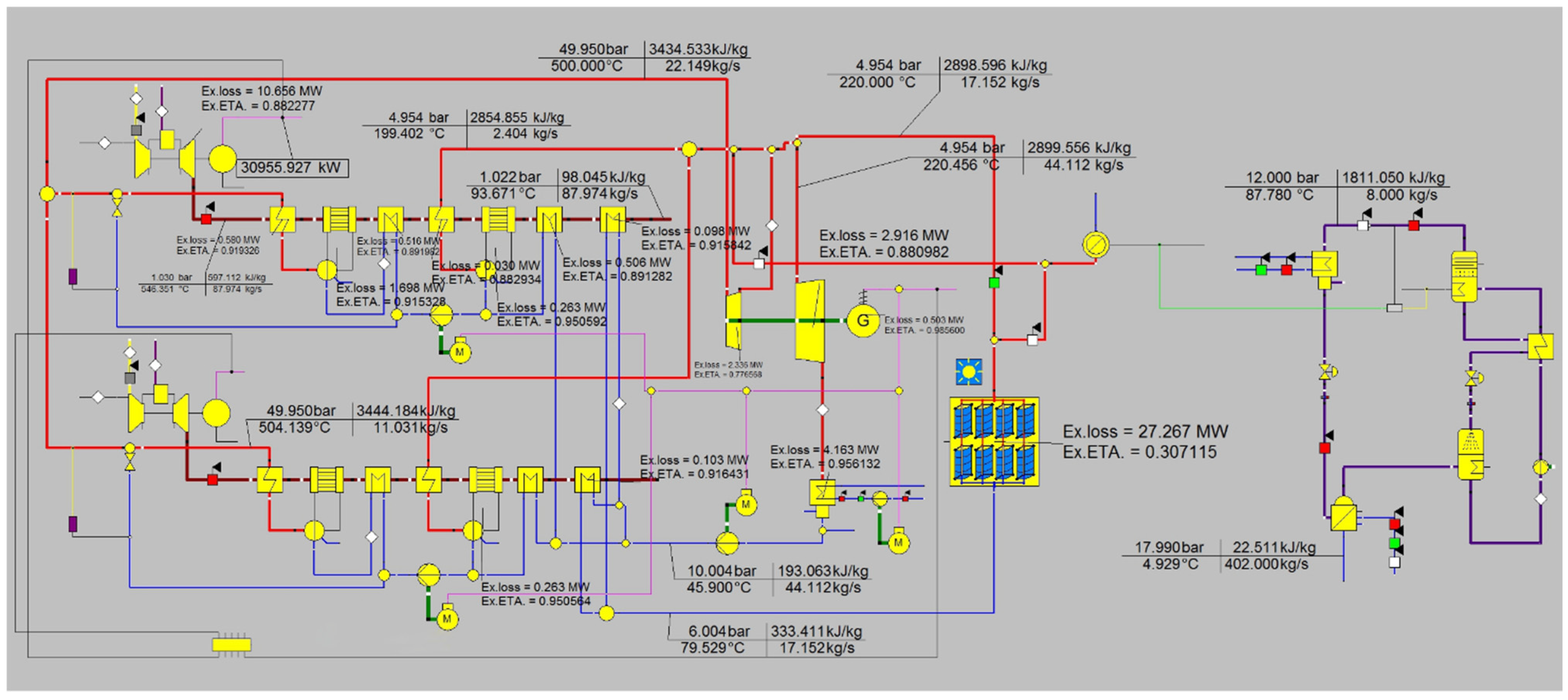



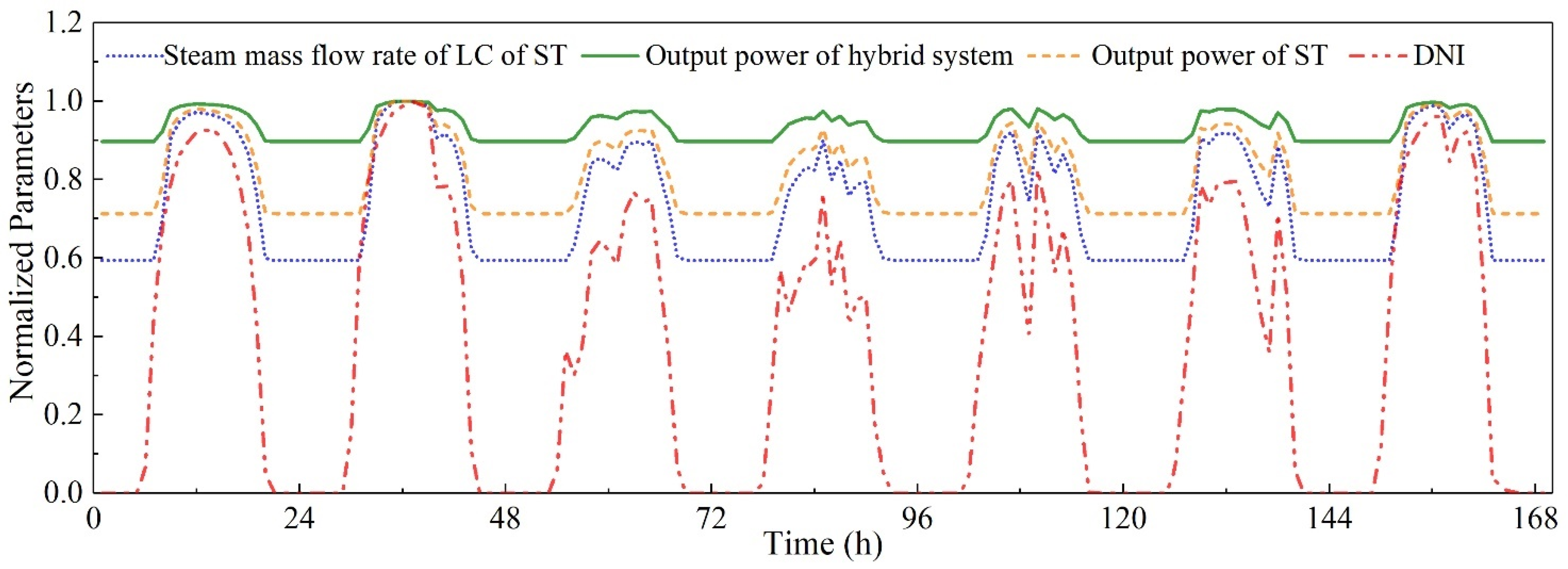
| Parameters | Referenced Values | Results in This Study |
|---|---|---|
| Total electric power/MW | 87.0 | 86.98 |
| Electric power of GT/MW | 31.0 | 30.95 |
| Electric power of ST/MW | 25.0 | 25.10 |
| Exhaust gas temperature/°C | 546.0 | 546.35 |
| High-pressure steam mass flow rate/t/h | 80.0 | 79.75 |
| High-pressure steam temperature/°C | 500.0 | 500.0 |
| High-pressure steam pressure/MPa | 5.0 | 4.95 |
| Low-pressure steam mass flow rate/t/h | 17.0 | 17.26 |
| Low-pressure steam temperature/°C | 200.0 | 199.65 |
| Low-pressure steam pressure/MPa | 0.5 | 0.5 |
| Component | Ideal Condition | Unavoidable Condition |
|---|---|---|
| AC | Isentropic efficiency = 100.0% Mechanical efficiency = 100.0% | Isentropic efficiency = 96.0% Mechanical efficiency = 99.6% |
| CC | Combustion efficiency = 1.0 Pressure loss = 0.0 bar ALAM = 2.617 | Combustion efficiency = 0.998 Pressure loss = 0.1 bar ALAM = 1.57 |
| GT | Isentropic efficiency = 100.0% Mechanical efficiency = 100.0% | Isentropic efficiency = 98.0% Mechanical efficiency = 99.6% |
| Super-heater of HRSG | Efficiency = 100.0% Pressure drop = 0.0 bar | Efficiency = 90.0% Pressure drops at hot end/cold end = 0.5 bar/0.01 bar |
| Evaporator of HRSG | Pinch-point temperature difference = 0.01 K Pressure drop = 0.0 bar | Pinch-point temperature difference = 3.0 K Pressure drops at hot end/cold end = 0.5 bar/0.01 bar |
| Economizer of HRSG | Pinch-point temperature difference = 0.01 K Pressure drop = 0.0 bar | Pinch-point temperature difference = 3.0 K Pressure drops at hot end/cold end = 0.5 bar/0.01 bar |
| ST | Isentropic efficiency = 100.0% Mechanical efficiency = 100.0% | Isentropic efficiency = 96.0% Mechanical efficiency = 99.5% |
| COND | Terminal temperature difference = 0.0 K | Terminal temperature difference = 3.0 K |
| GEN | Efficiency = 100.0% | Efficiency = 99.5% |
| SDSG | Optical efficiency = 100.0% Heat loss coefficient = 0.0 W/(m2K) Efficiency of solar receiver tubes = 100.0% | Optical efficiency = 79.9% Heat loss coefficient = 10.0 W/(m2K) Efficiency of solar receiver tubes = 85.0% |
| CSS | Efficiency of generator = 100.0% Pressure drop of condenser = 0.0 bar Efficiency of absorber = 100.0% Terminal temperature difference of evaporator = 0.1 K | Efficiency of generator = 98.0% Pressure drop of condenser =0.05 bar Efficiency of absorber = 96.0% Terminal temperature difference of evaporator = 0.5 K |
| Parameter | Value |
|---|---|
| Electric power of solar–gas system | 96.0 MW |
| Electric power of gas–steam cycle | 87.0 MW |
| Maximum cooling load | 69.66 MW |
| Exhaust gas temperature of GT | 546.0 °C |
| Inlet steam temperature/pressure of ST | 500.0 °C/5.0 MPa |
| Electric power contributed by SDSG | 9.0 MW |
| Optical efficiency of SDSG | 0.75 |
| Output steam temperature/pressure of SDSG | 220.0 °C/0.5 MPa |
| Energy conversion efficiency of solar–gas system | 45.8% |
| Energy efficiency of GT | 37.4% |
| Energy efficiency of HRSG | 82.7% |
| Solar energy proportion | 9.4% |
| Component | Ed (MW) | ηx |
|---|---|---|
| AC | 3.86 | 92.29% |
| CC | 56.45 | 61.98% |
| GT | 2.97 | 91.25% |
| HPSH | 0.591 | 87.42% |
| HPEV | 1.705 | 85.18% |
| HPEC | 0.516 | 78.39% |
| LPSH | 0.036 | 72.72% |
| LPEV | 0.256 | 88.07% |
| CH | 0.507 | 68.58% |
| HWH | 0.103 | 68.67% |
| HPFP | 0.025 | 73.96% |
| ST | 6.287 | 88.48% |
| COND | 4.045 | 76.75% |
| CP | 0.019 | 70.46% |
| SDSG | 28.516 | 36.24% |
| CSS | 8.27 | 24.61% |
| GEN | 1.022 | 97.36% |
| Node | Temperature (°C) | Pressure (MPa) | Specific Enthalpy (kJ/kg) |
|---|---|---|---|
| 1 | 25.0 | 0.103 | 25.07 |
| 2 | 510.0 | 1.02 | 560.0 |
| 3 | 1500.0 | 0.12 | 1213.0 |
| 4 | 546.0 | 1.45 | 597.0 |
| 5 | 504.5 | 4.995 | 3444.8 |
| 6 | 199.5 | 0.5 | 2854.0 |
| 7 | 45.0 | 0.01 | 2342.0 |
| 9 | 128.0 | 6.0 | 545.0 |
| 12 | 220.0 | 0.49 | 2898.6 |
| 13 | 80.0 | 0.6 | 333.4 |
| Fuel | 60.0 | 3.58 | 130.96 |
| Component | Ed (MW) | Ed,en (MW) | Ed,ex (MW) | ken |
|---|---|---|---|---|
| AC | 3.86 | 2.166 | 1.694 | 0.561 |
| CC | 56.45 | 37.2 | 19.25 | 0.659 |
| GT | 2.97 | 2.405 | 0.565 | 0.810 |
| HRSG | 7.605 | 4.357 | 3.248 | 0.573 |
| ST | 6.287 | 5.54 | 0.747 | 0.881 |
| GEN | 0.514 | 0.412 | 0.102 | 0.802 |
| COND | 4.165 | 2.886 | 1.279 | 0.693 |
| SDSG | 28.516 | 27.235 | 1.281 | 0.955 |
| CSS | 8.27 | 5.62 | 2.65 | 0.680 |
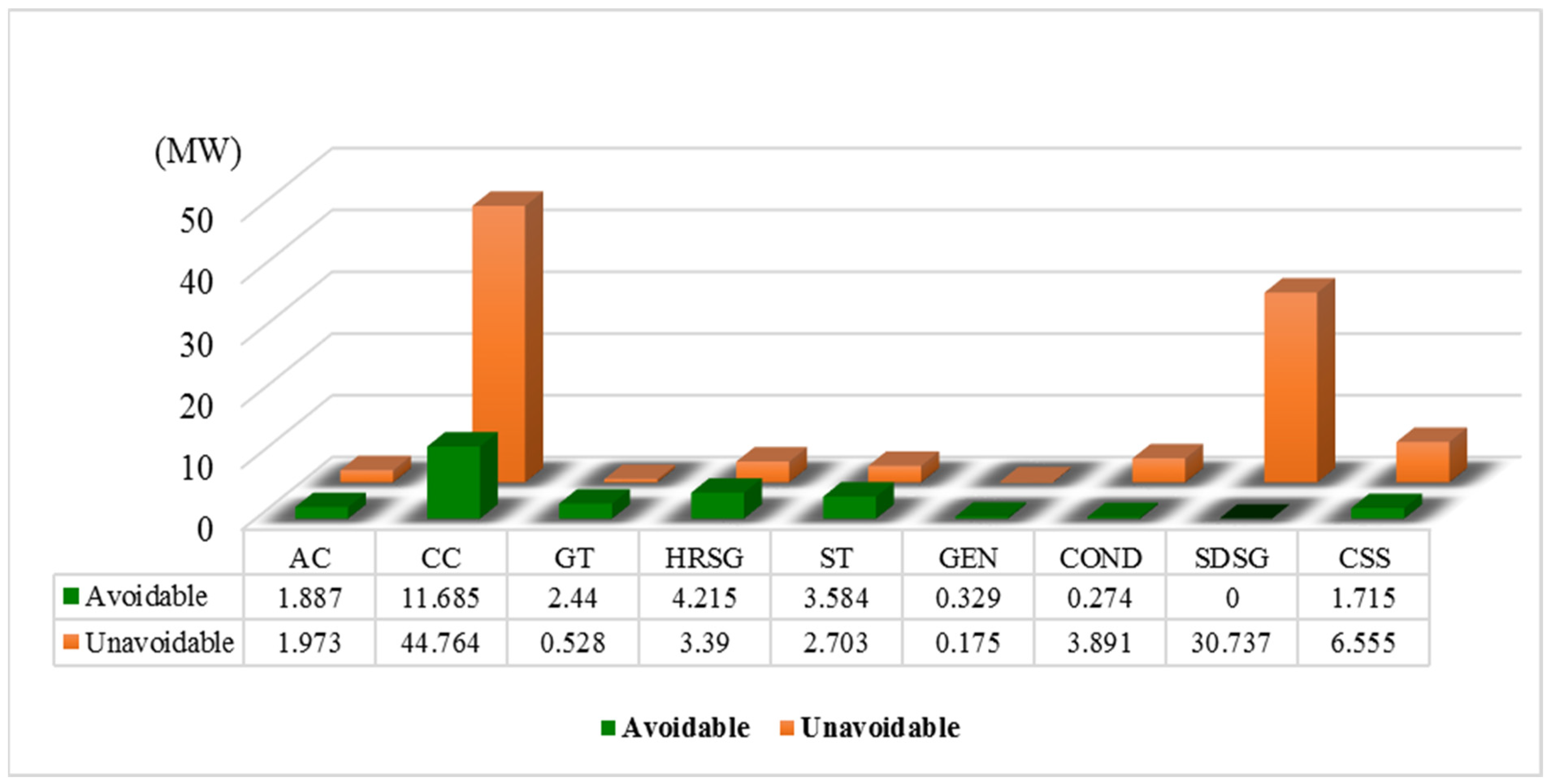
| Component | Ed (MW) | Ed,av (MW) | Ed,un (MW) | kav |
|---|---|---|---|---|
| AC | 3.86 | 1.887 | 1.973 | 0.489 |
| CC | 56.45 | 11.685 | 44.764 | 0.207 |
| GT | 2.97 | 2.44 | 0.528 | 0.822 |
| HRSG | 7.605 | 4.215 | 3.39 | 0.554 |
| ST | 6.287 | 3.584 | 2.703 | 0.570 |
| GEN | 0.514 | 0.329 | 0.175 | 0.640 |
| COND | 4.165 | 0.274 | 3.891 | 0.066 |
| SDSG | 28.516 | 0.095 | 28.611 | 0.003 |
| CSS | 8.27 | 1.715 | 6.555 | 0.207 |
| Parameter | Result |
|---|---|
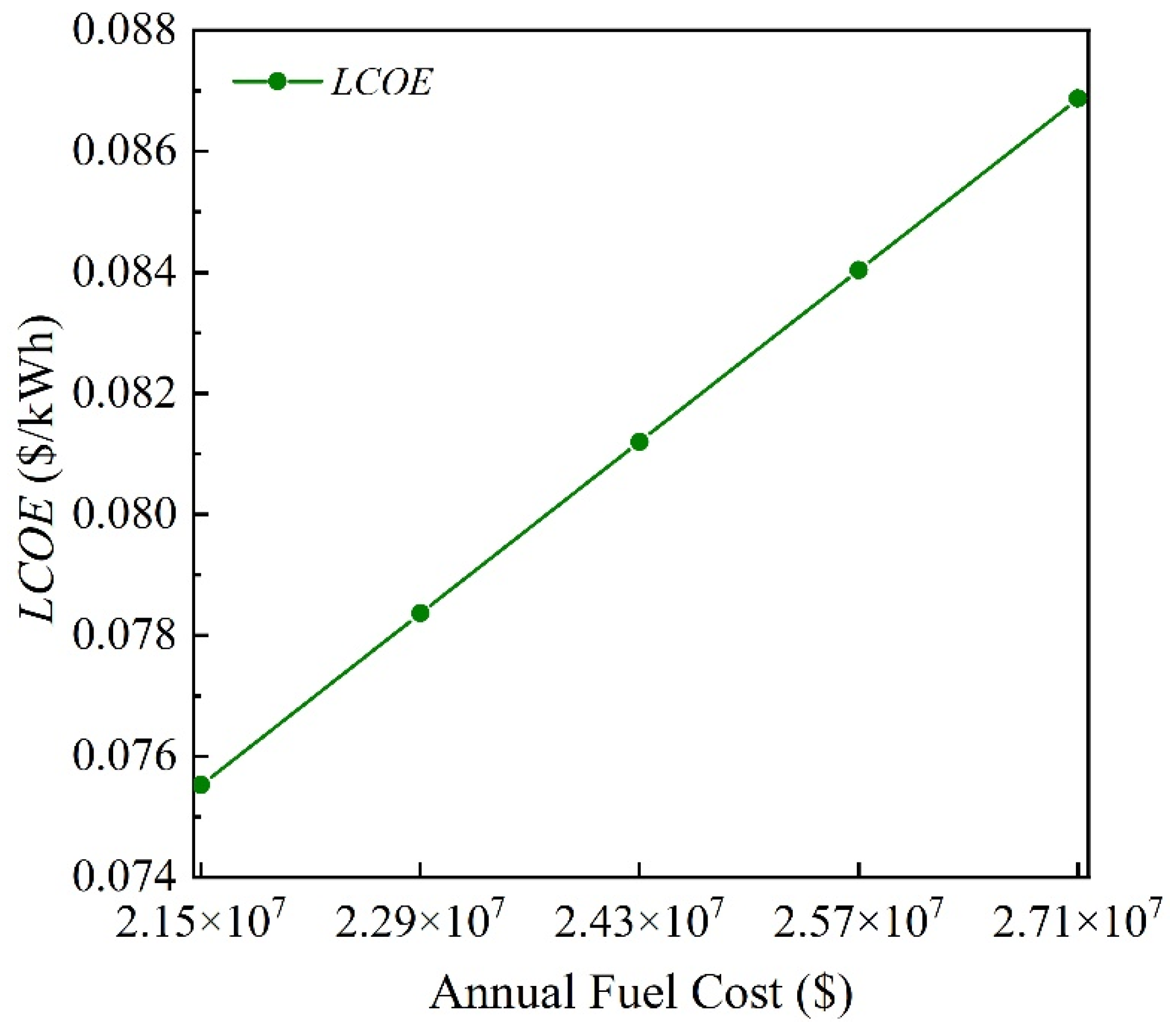
| LCOE | 0.08125 USD/kWh |
| Dynamic recycling cycle | 5.8 years |
| Net present value | USD 61,475,694.4 |
| Internal rate of return | 18.3% |
| Reference | Solar Field Type | Total Output Power (MW) | Output Power Provided by Solar Energy (MW) | Solar Proportion | Exergic Loss of the CC (MW) | Total Exergic Loss (MW) | LCOE (USD/kWh) |
|---|---|---|---|---|---|---|---|
| This work | PTC | 96.0 | 9.0 | 9.4% | 56.5 | 119.1 | 0.08125 |
| Ref. [32] | PTC | 470.3 | 39.8 | 8.5% | 297.5 | 498.4 | 0.067 |
| Ref. [34] | PTC | 402.0 | 61.7 | 15.4% | 136.8 | 278.9 | 0.1042 |
| Ref. [35] | PTC | 467.0 | 17.0 | 3.6% | 260.9 | 432.3 | -- |
| Ref. [36] | PTC | 160.0 | 22.0 | 13.8% | 75.0 | 336.7 | 0.11 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ge, Q.; Cao, X.; Guo, F.; Li, J.; Wang, C.; Wang, G. Energy, Exergic and Economic Analyses of a Novel Hybrid Solar–Gas System for Producing Electrical Power and Cooling. Energies 2025, 18, 2480. https://doi.org/10.3390/en18102480
Ge Q, Cao X, Guo F, Li J, Wang C, Wang G. Energy, Exergic and Economic Analyses of a Novel Hybrid Solar–Gas System for Producing Electrical Power and Cooling. Energies. 2025; 18(10):2480. https://doi.org/10.3390/en18102480
Chicago/Turabian StyleGe, Qun, Xiaoman Cao, Fumin Guo, Jianpeng Li, Cheng Wang, and Gang Wang. 2025. "Energy, Exergic and Economic Analyses of a Novel Hybrid Solar–Gas System for Producing Electrical Power and Cooling" Energies 18, no. 10: 2480. https://doi.org/10.3390/en18102480
APA StyleGe, Q., Cao, X., Guo, F., Li, J., Wang, C., & Wang, G. (2025). Energy, Exergic and Economic Analyses of a Novel Hybrid Solar–Gas System for Producing Electrical Power and Cooling. Energies, 18(10), 2480. https://doi.org/10.3390/en18102480






