Numerical Research on Flow Characteristics at High Radii of Rim Seals with Different Geometric Structures
Abstract
1. Introduction
2. Computational Methods
3. Results and Discussion
3.1. The Flow Characteristics of the Single-Layer Sealing Structure
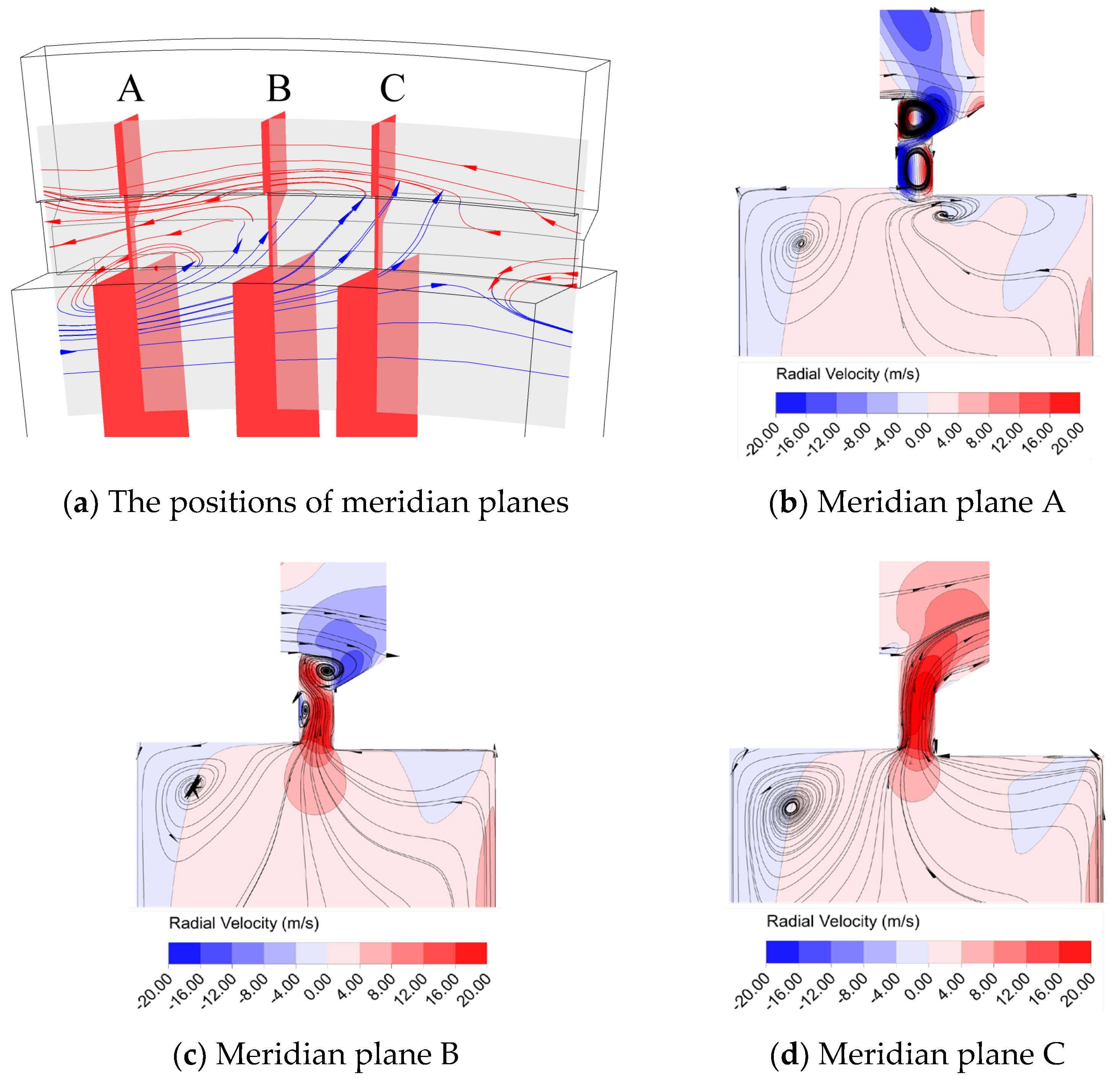
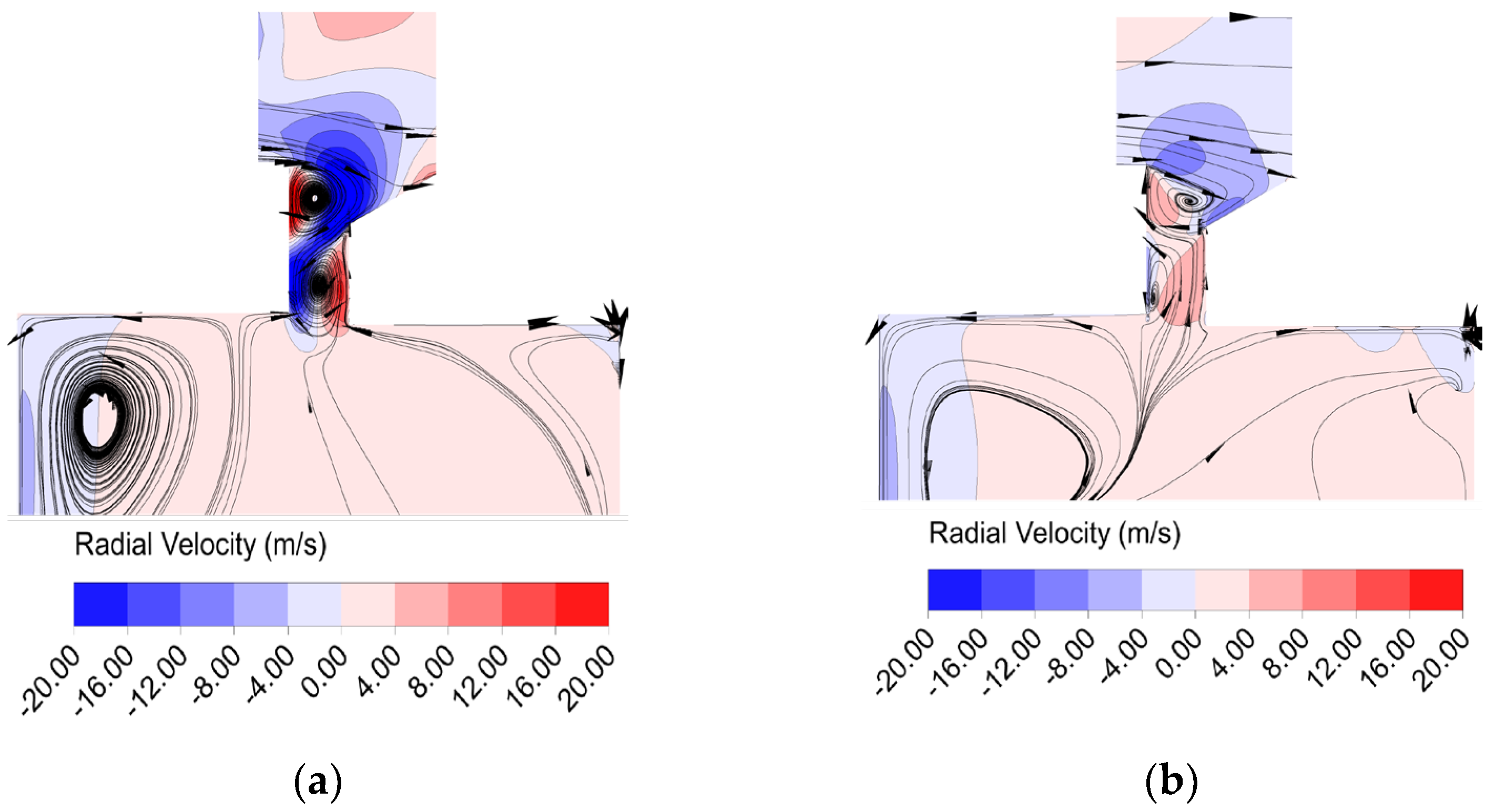
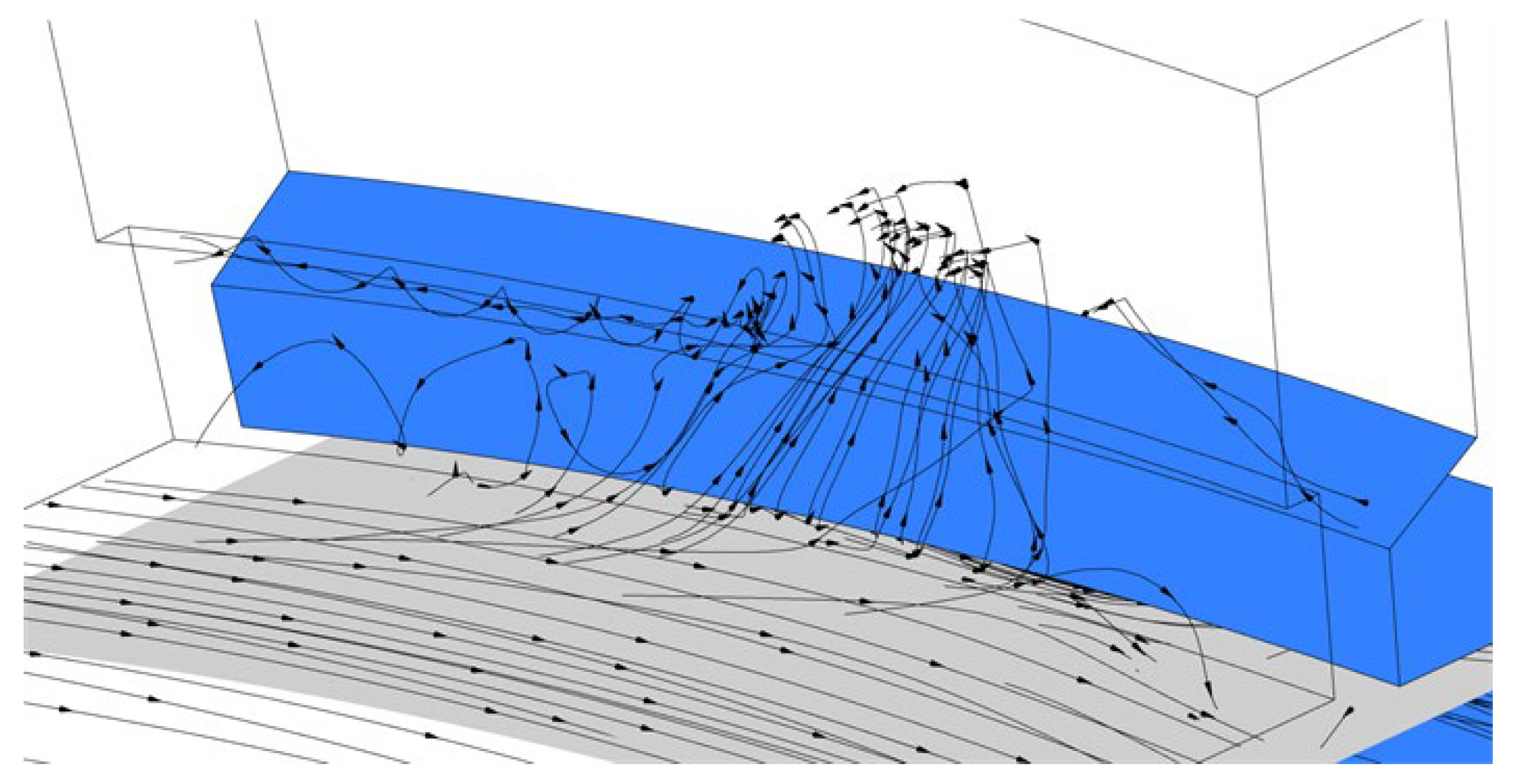
3.1.1. The Flow Characteristics of the Single-Axial Sealing Structure
3.1.2. The Flow Characteristics of the Single-Radial Sealing Structure
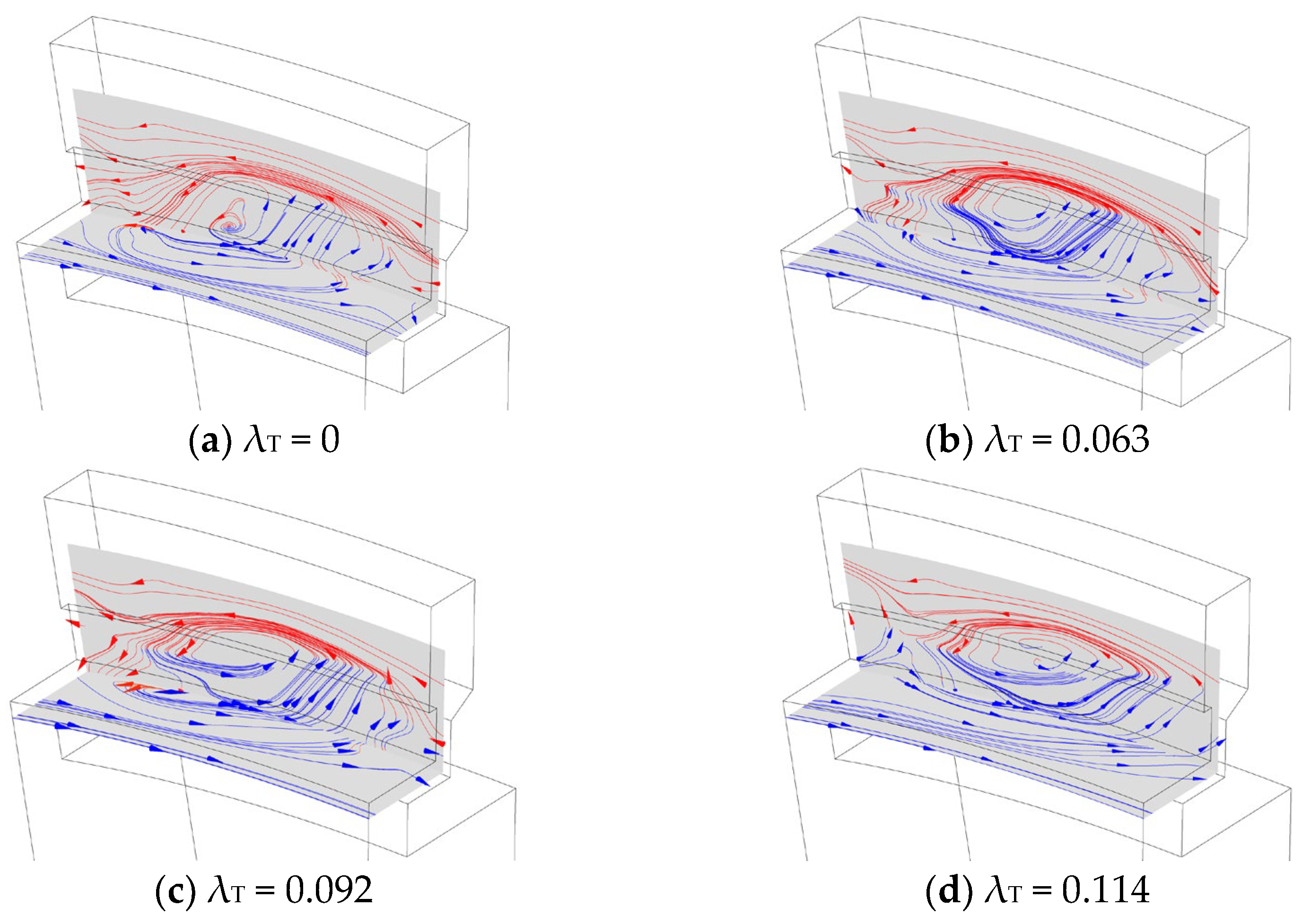
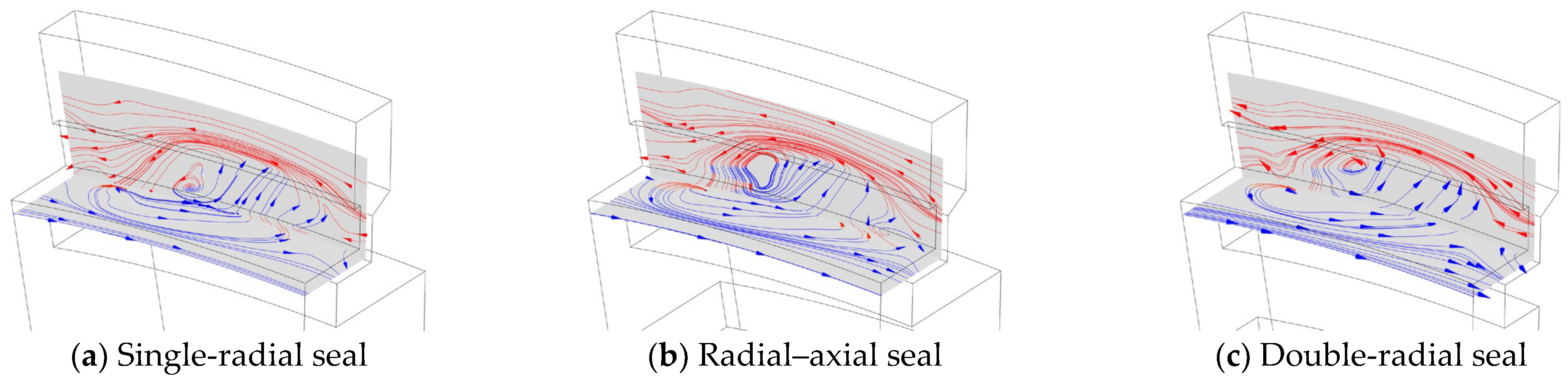
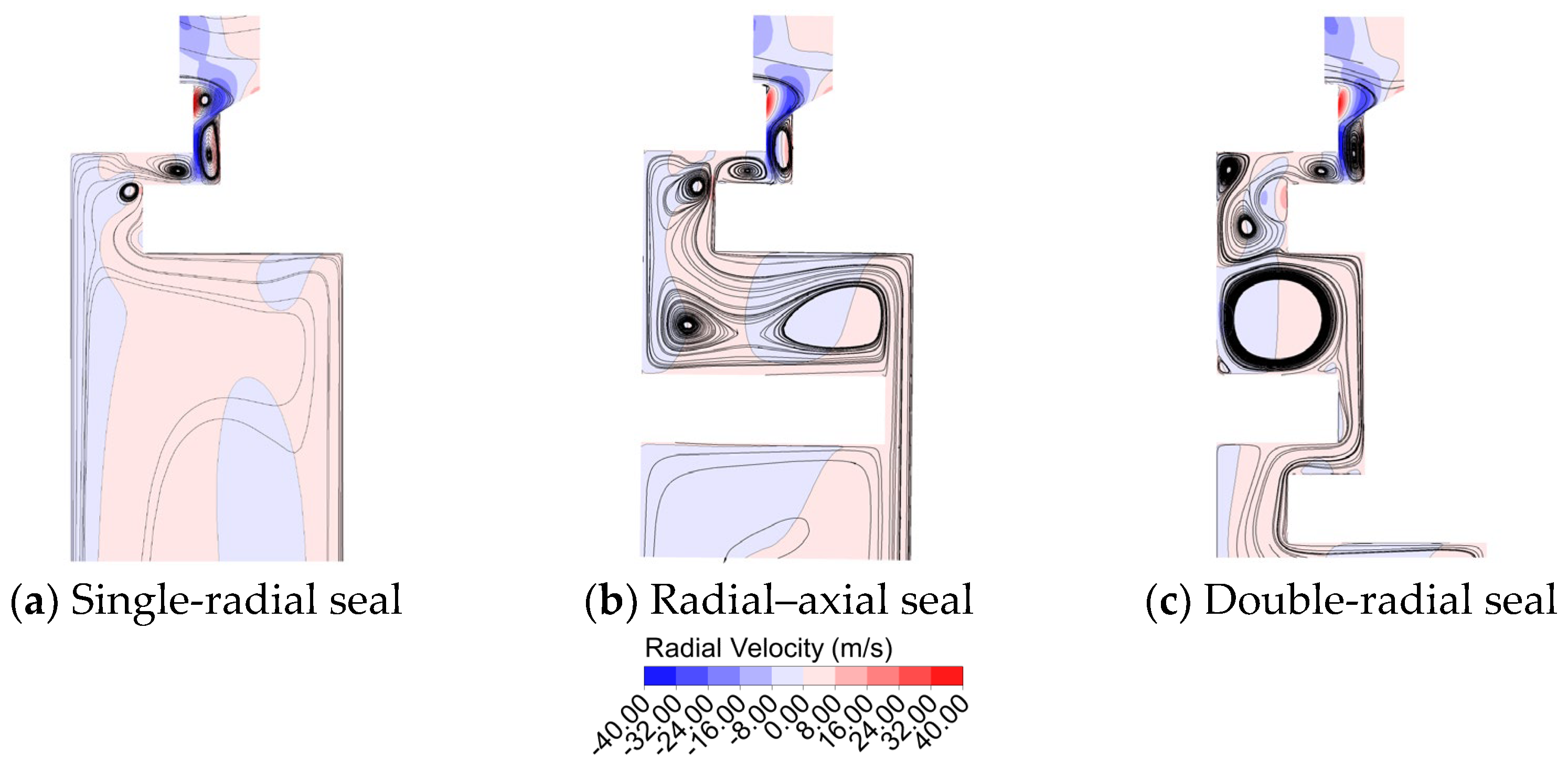
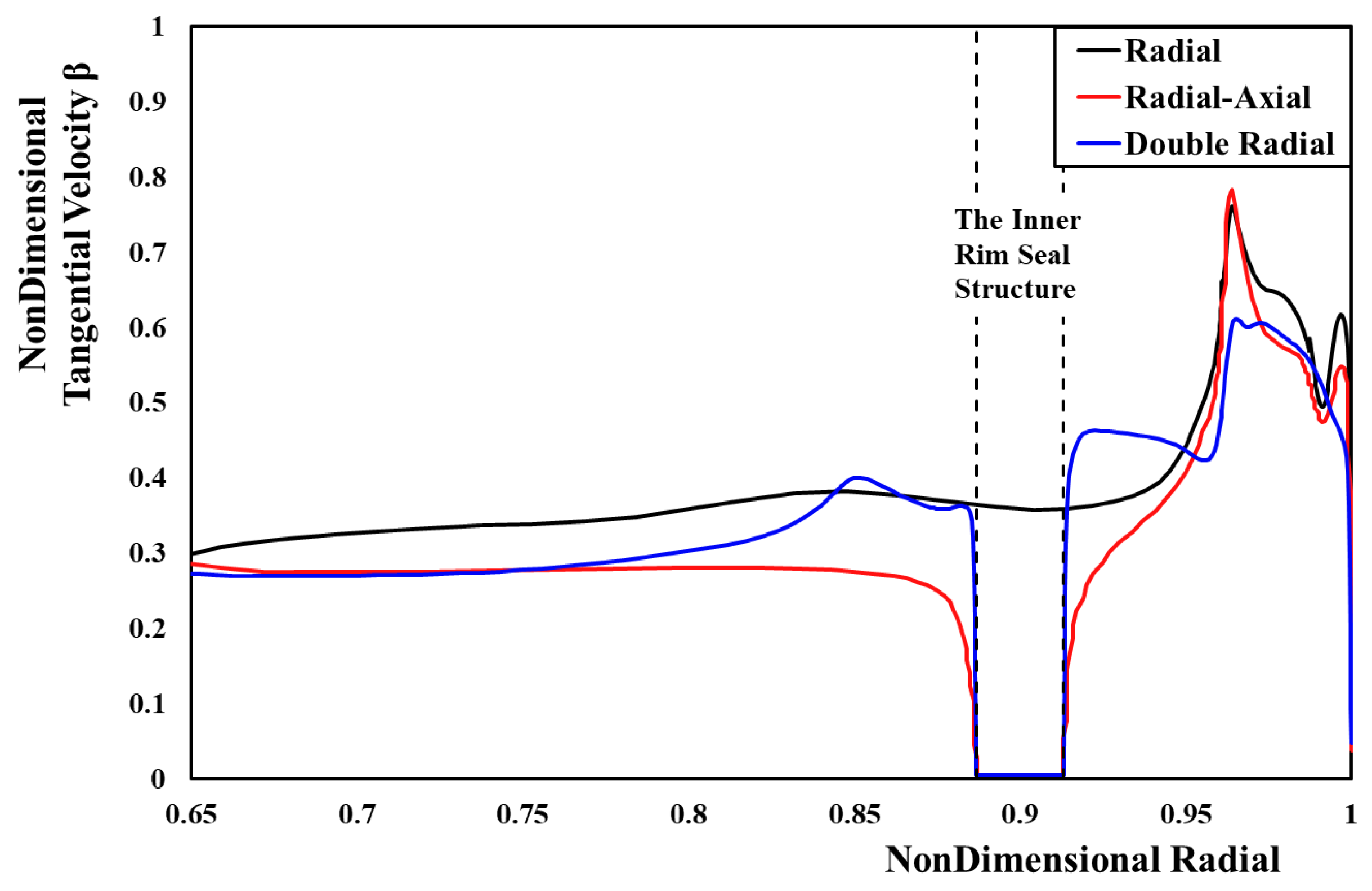
3.2. The Flow Characteristics of the Double-Layer Sealing Structure
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Batchelor, G.K. Note on a class of solutions of the navier-stokes equations representing steady rotationally-symmetric flow. Q. J. Mech. Appl. Math. 1951, 4, 29–41. [Google Scholar] [CrossRef]
- Stewartson, K. On the flow between two rotating coaxial disks. Math. Proc. Camb. Philos. Soc. 1953, 49, 333–341. [Google Scholar] [CrossRef]
- Mellor, G.L.; Chapple, P.J.; Stokes, V.K. On the flow between a rotating and stationary disk. J. Fluid Mech. Digit. Arch. 1968, 31, 95–112. [Google Scholar] [CrossRef]
- Daily, J.W.; Nece, R.E. Chamber Dimension Effects on Induced Flow and Frictional Resistance of Enclosed Rotating Disks. J. Basic Eng. 1960, 82, 217. [Google Scholar] [CrossRef]
- Rabs, M.; Benra, F.K.; Dohmen, H.J.; Schneider, O. Investigation of Flow Instabilities Near the Rim Cavity of a 1.5 Stage Gas Turbine. In Proceedings of the ASME Turbo Expo 2009: Power for Land, Sea, and Air, Orlando, FL, USA, 8–12 June 2009. [Google Scholar]
- Savov, S.S.; Atkins, N.R. A Rim Seal Ingress Model Based on Turbulent Transport. In Proceedings of the Asme Turbo Expo: Turbomachinery Technical Conference & Exposition, Charlotte, NC, USA, 26–30 June 2017; p. V05BT15A009. [Google Scholar]
- Lian, Z.; Du, Q.; Liu, G.; Wang, R.; Xie, L. Numerical Investigation on Unsteady Characteristics in Different Rim Seal Geometries: Part B. In Proceedings of the ASME Turbo Expo 2020: Turbomachinery Technical Conference and Exposition, Virtual, 21–25 September 2020. [Google Scholar]
- Bayley, F.J.; Owen, J.M. The Fluid Dynamics of a Shrouded Disk System With a Radial Outflow of Coolant. J. Eng. Gas Turbines Power 1970, 92, 335–341. [Google Scholar] [CrossRef]
- Dadkhah, S.; Turner, A.B.; Chew, J.W. Performance of Radial Clearance Rim Seals in Upstream and Downstream Rotor-Stator Wheelspaces. J. Turbomach. 1992, 114, 439–445. [Google Scholar] [CrossRef]
- Phadke, U.P.; Owen, J.M. Aerodynamic aspects of the sealing of gas-turbine rotor-stator systems: Part 3: The effect of nonaxisymmetric external flow on seal performance. Int. J. Heat Fluid Flow 1988, 9, 113–117. [Google Scholar] [CrossRef]
- Owen, J.M. Prediction of Ingestion Through Turbine Rim Seals—Part 1: Rotationally-Induced Ingress. In Proceedings of the ASME Turbo Expo 2009: Power for Land, Sea, and Air, Orlando, FL, USA, 8–12 June 2009; pp. 1071–1082. [Google Scholar]
- Owen, J.M. Prediction of Ingestion Through Turbine Rim Seals—Part 2: Externally-Induced and Combined Ingress. In Proceedings of the ASME Turbo Expo 2009: Power for Land, Sea, and Air, Orlando, FL, USA, 8–12 June 2009; pp. 1083–1093. [Google Scholar]
- Phadke, U.P.; Owen, J.M. Aerodynamic aspects of the sealing of gas-turbine rotor-stator systems: Part 1: The behavior of simple shrouded rotating-disk systems in a quiescent environment. Int. J. Heat Fluid Flow 1988, 9, 98–105. [Google Scholar] [CrossRef]
- Popović, I.; Hodson, H.P. The Effects of a Parametric Variation of the Rim Seal Geometry on the Interaction Between Hub Leakage and Mainstream Flows in High Pressure Turbines. J. Eng. Gas Turbines Power 2013, 135, 112501. [Google Scholar] [CrossRef]
- Moon, M.-A.; Lee, C.-S.; Kim, K.-Y. Effect of a rib on rim seal performance. Int. Commun. Heat Mass Transf. 2014, 59, 130–135. [Google Scholar] [CrossRef]
- Liu, D.; Tao, Z.; Luo, X.; Kang, W.; Wu, H.; Yu, X. Investigation on the Impact of Protrusion Parameter on the Efficiency of Converting Additional Windage Loss for Ingress Alleviation in Rotor–Stator System. J. Eng. Gas Turbines Power 2016, 138, 112604. [Google Scholar] [CrossRef]
- Wu, K. Research on the Mechanism of Sealing and Ingestion in Gas Turbine Rotor-Stator Rim; Power Engineering and Engineering Thermophysics; Tsinghua University: Beijing, China, 2014. [Google Scholar]
- Wang, R. Investigation on the Unsteady Mechanisms of Rim Seal Flow and the Control Methods of Hot Gas Ingestion; Science in Engineering in Power Machinery and Engineering; University of Chinese Academy of Sciences: Beijing, China, 2020. [Google Scholar]
- Xue, Q.; Li, X.; Ren, J. Numerical Investigations of Flow Structures in Radial Rim Seal and a Modification of the Orifice Model. In Proceedings of the ASME Turbo Expo 2022: Turbomachinery Technical Conference and Exposition, Rotterdam, The Netherlands, 13–17 June 2022. [Google Scholar]
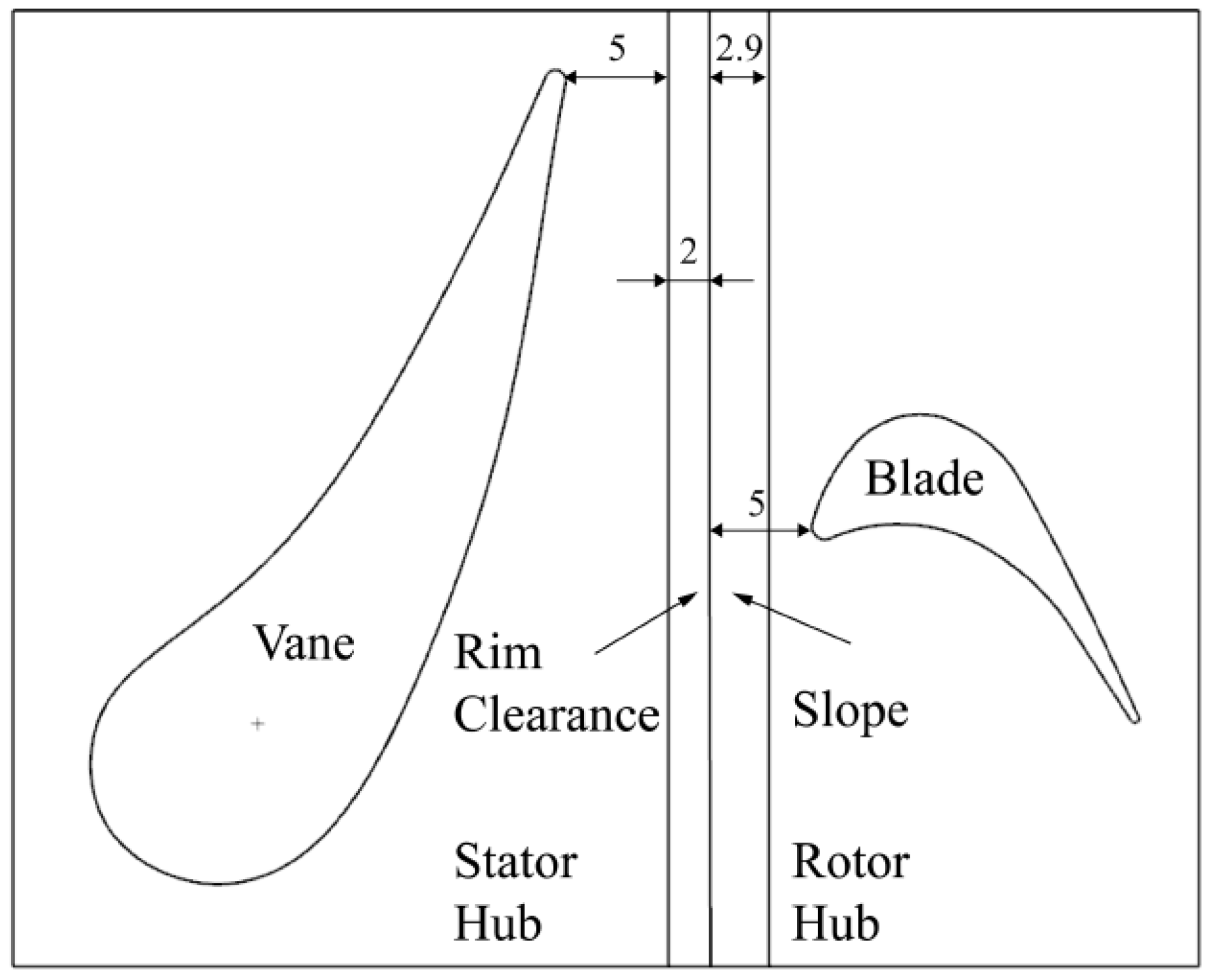
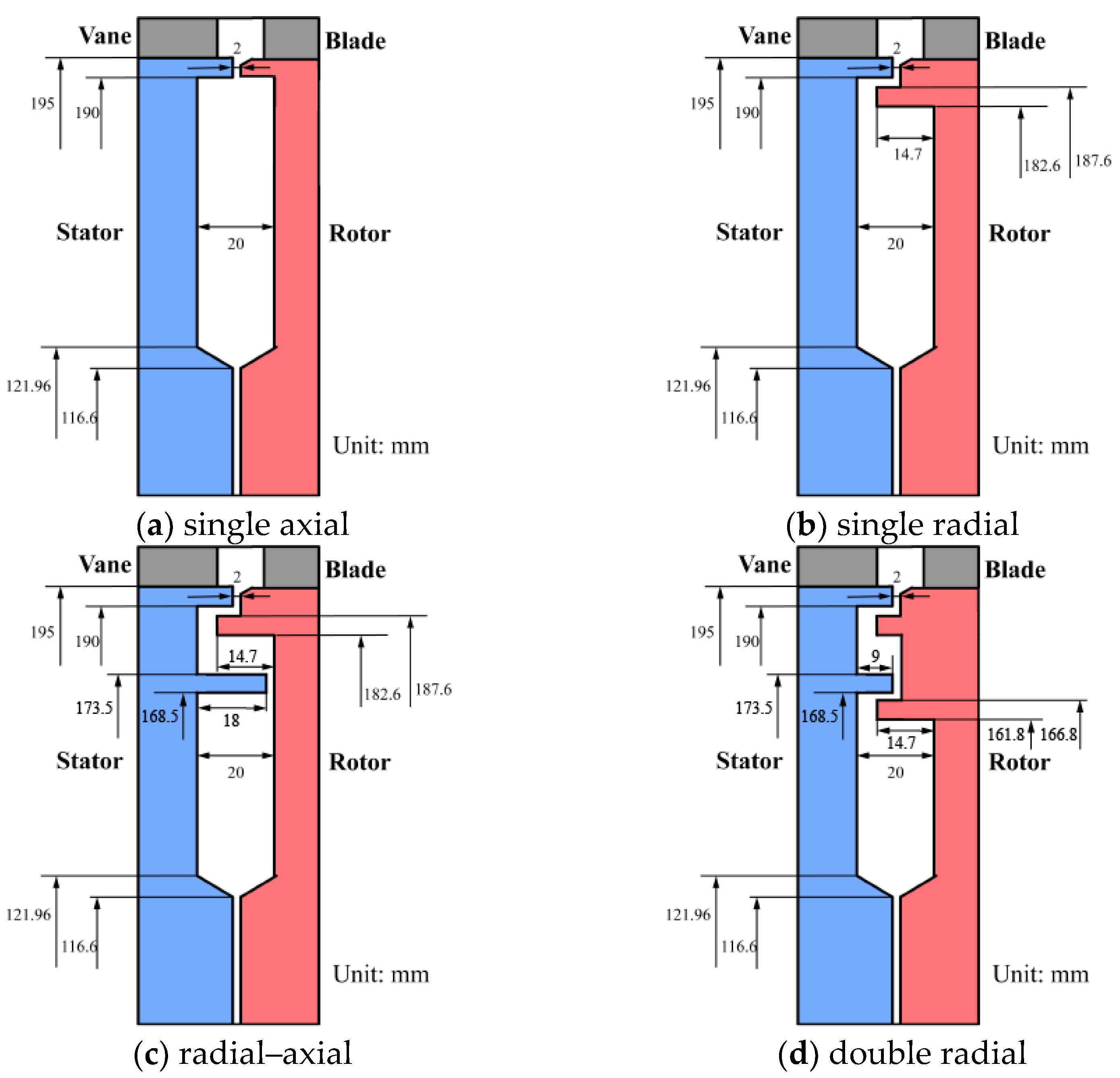


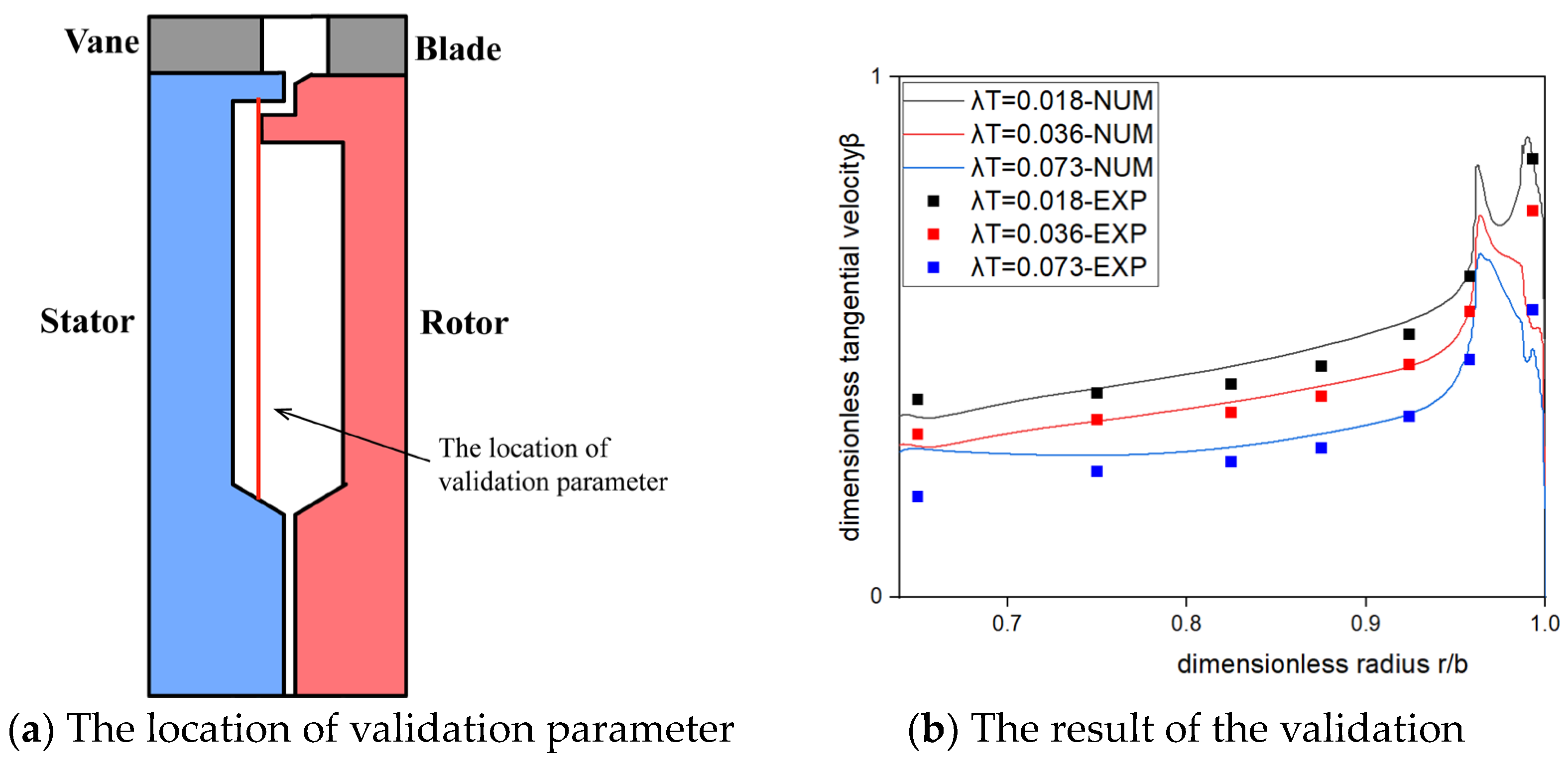

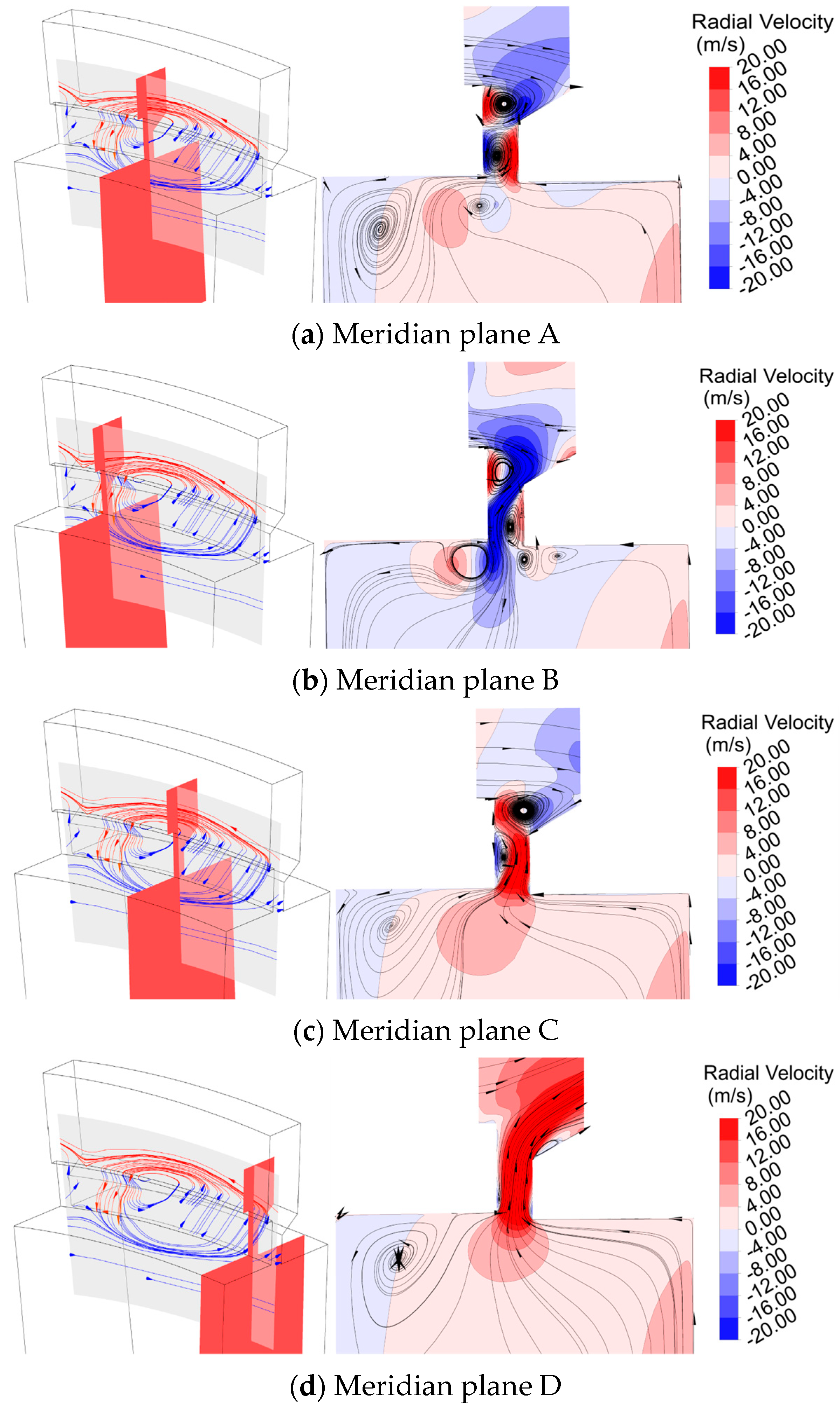



| Parameter Name | Parameter Value (mm) |
|---|---|
| Axial rim clearance | 2 |
| Radius of cavity | 190 |
| Axial disk spacing | 20 |
| Minimum radius of cavity | 116.6 |
| Height of vane and blade | 10 |
| Boundary Name | Value |
|---|---|
| Mainstream inlet | 0.0159 kg/s |
| Purge flow inlet | |
| Mainstream outlet | 1 atm |
| Rotor surface | 3000 rpm |
| Blade | 3000 rpm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xue, Q.; Li, X.; Ren, J. Numerical Research on Flow Characteristics at High Radii of Rim Seals with Different Geometric Structures. Energies 2024, 17, 1877. https://doi.org/10.3390/en17081877
Xue Q, Li X, Ren J. Numerical Research on Flow Characteristics at High Radii of Rim Seals with Different Geometric Structures. Energies. 2024; 17(8):1877. https://doi.org/10.3390/en17081877
Chicago/Turabian StyleXue, Qichao, Xueying Li, and Jing Ren. 2024. "Numerical Research on Flow Characteristics at High Radii of Rim Seals with Different Geometric Structures" Energies 17, no. 8: 1877. https://doi.org/10.3390/en17081877
APA StyleXue, Q., Li, X., & Ren, J. (2024). Numerical Research on Flow Characteristics at High Radii of Rim Seals with Different Geometric Structures. Energies, 17(8), 1877. https://doi.org/10.3390/en17081877







