Modified Multimachine Power System Design with DFIG-WECS and Damping Controller
Abstract
1. Introduction
2. Materials and Methods
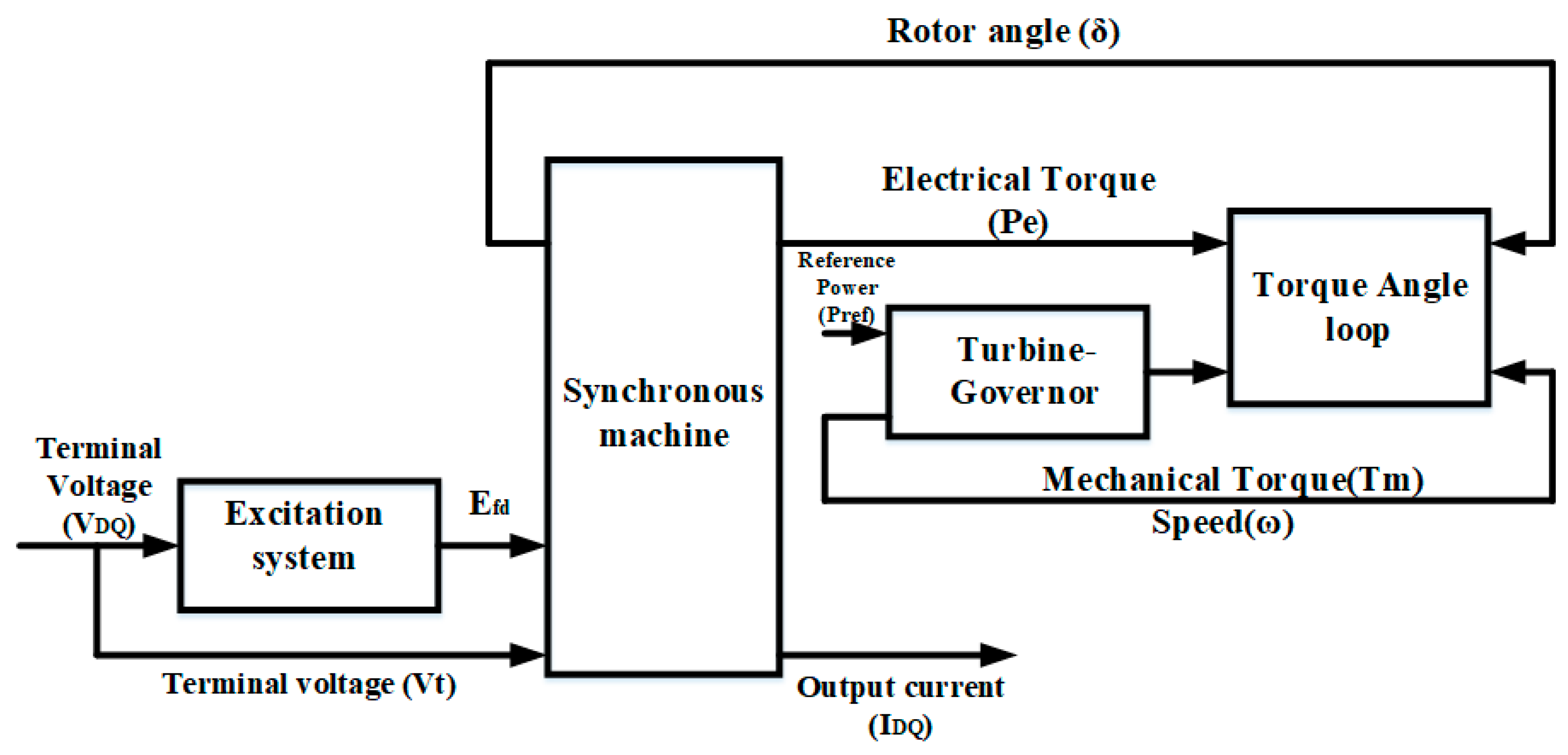
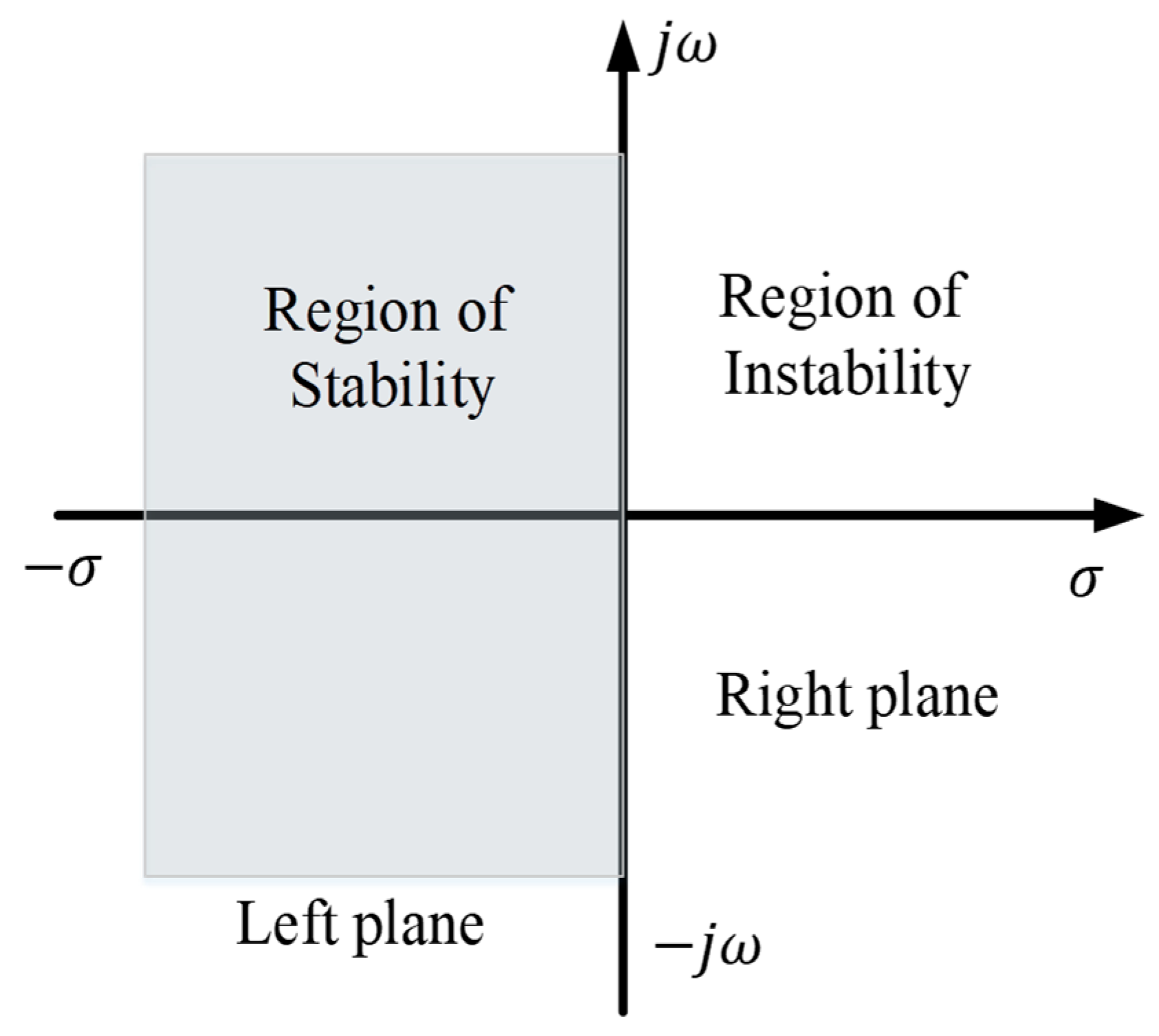
2.1. Power System Stability
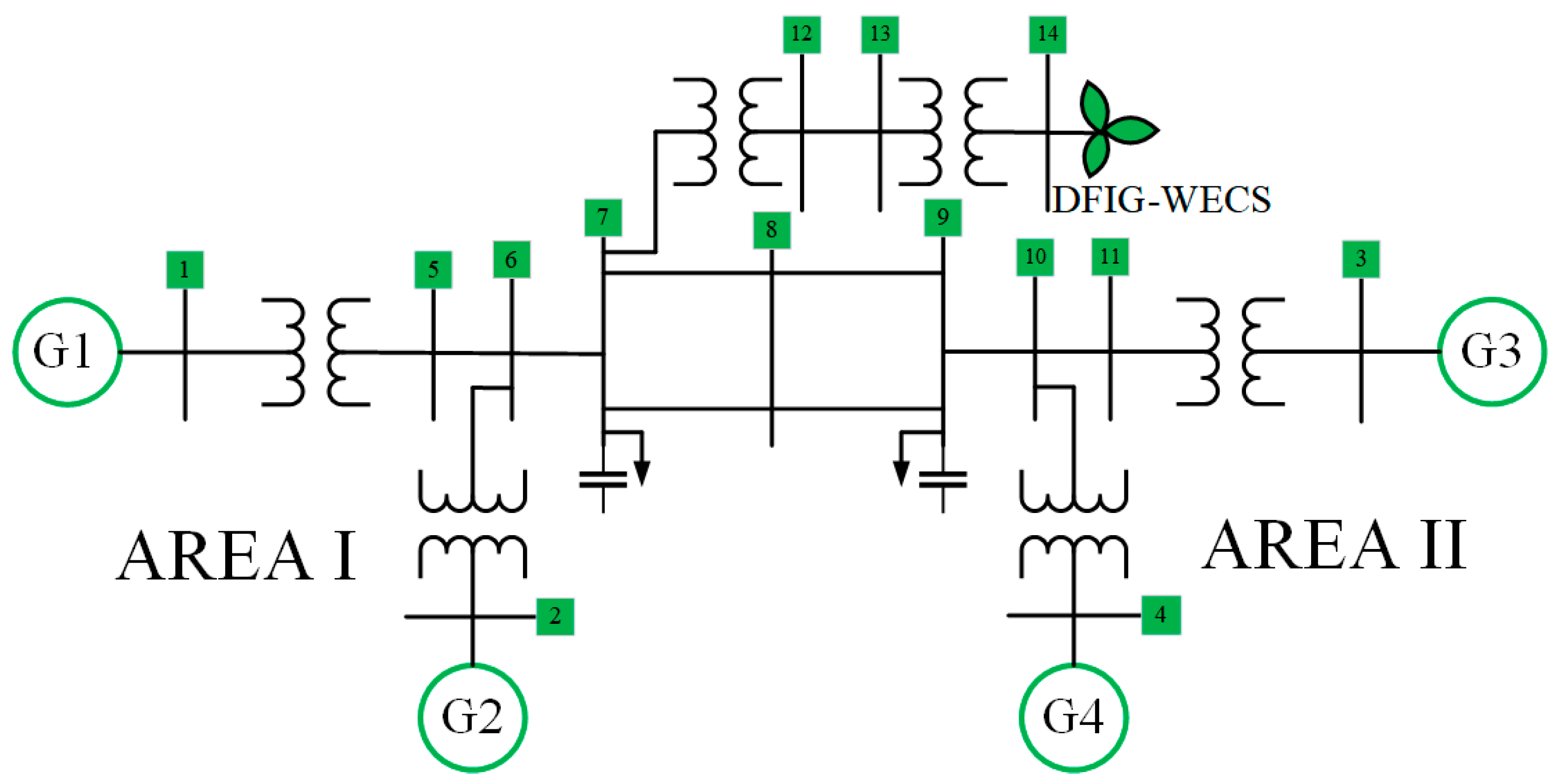
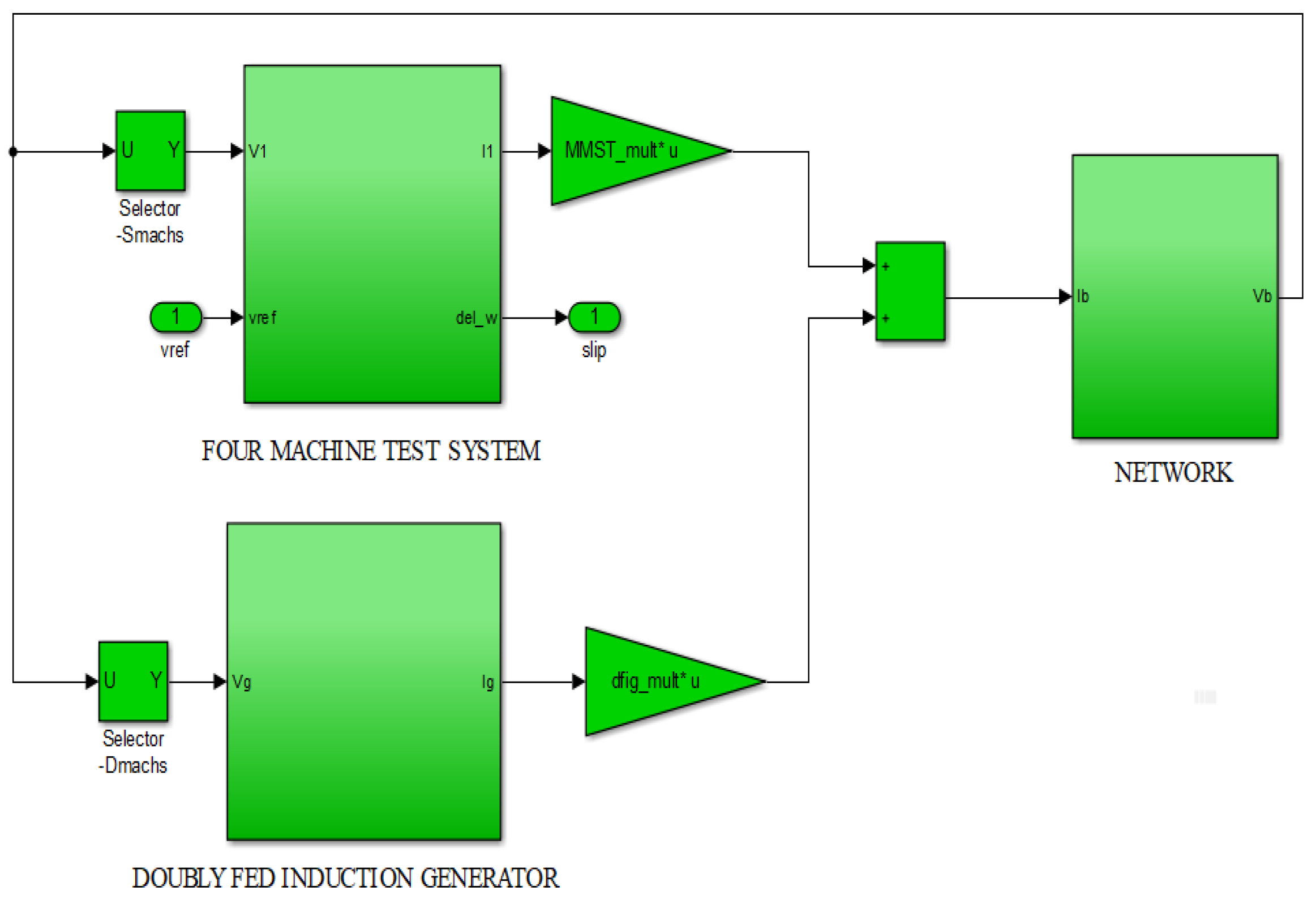
Multimachine Power Test System
2.2. Conventional Power System
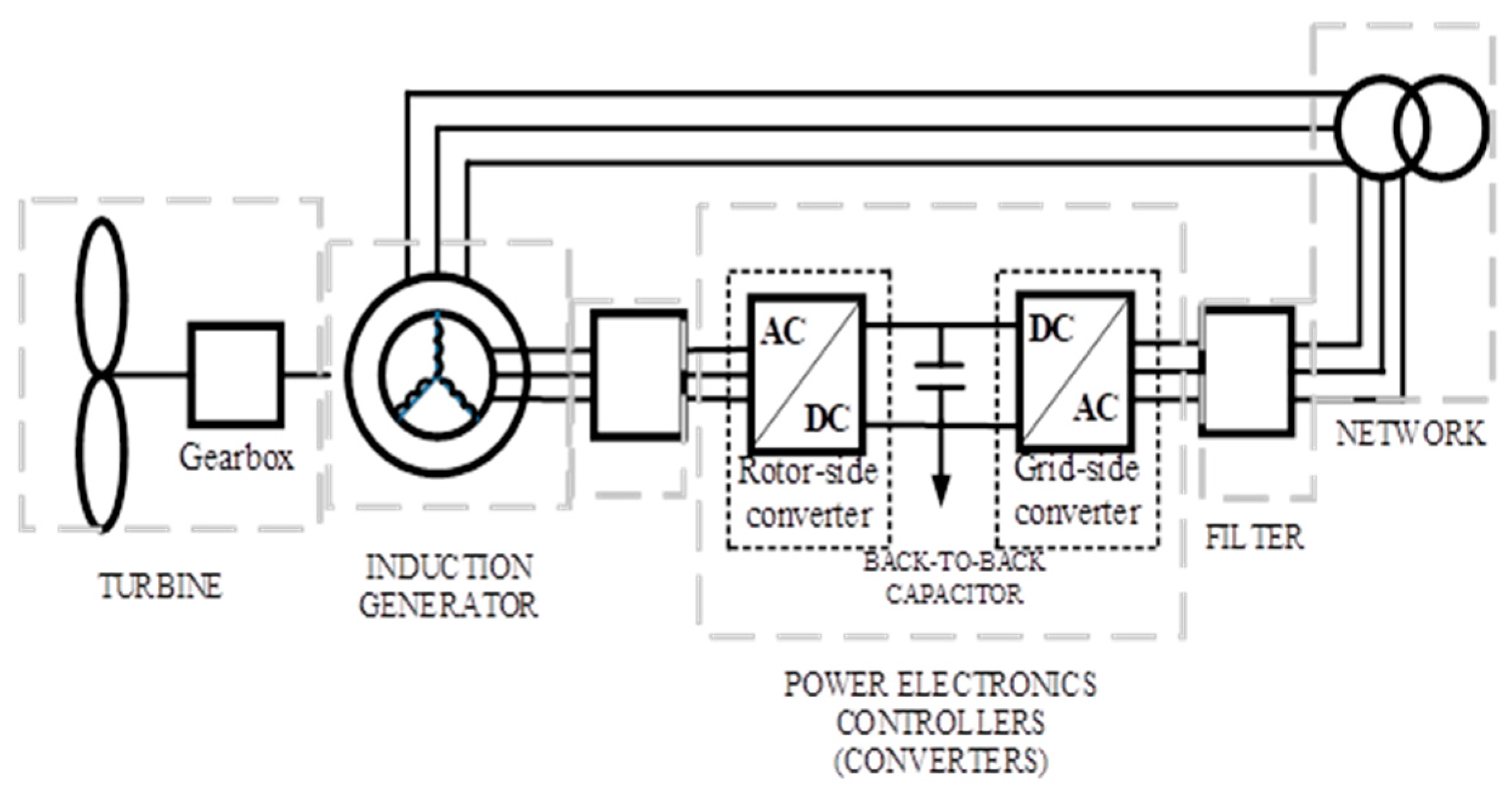
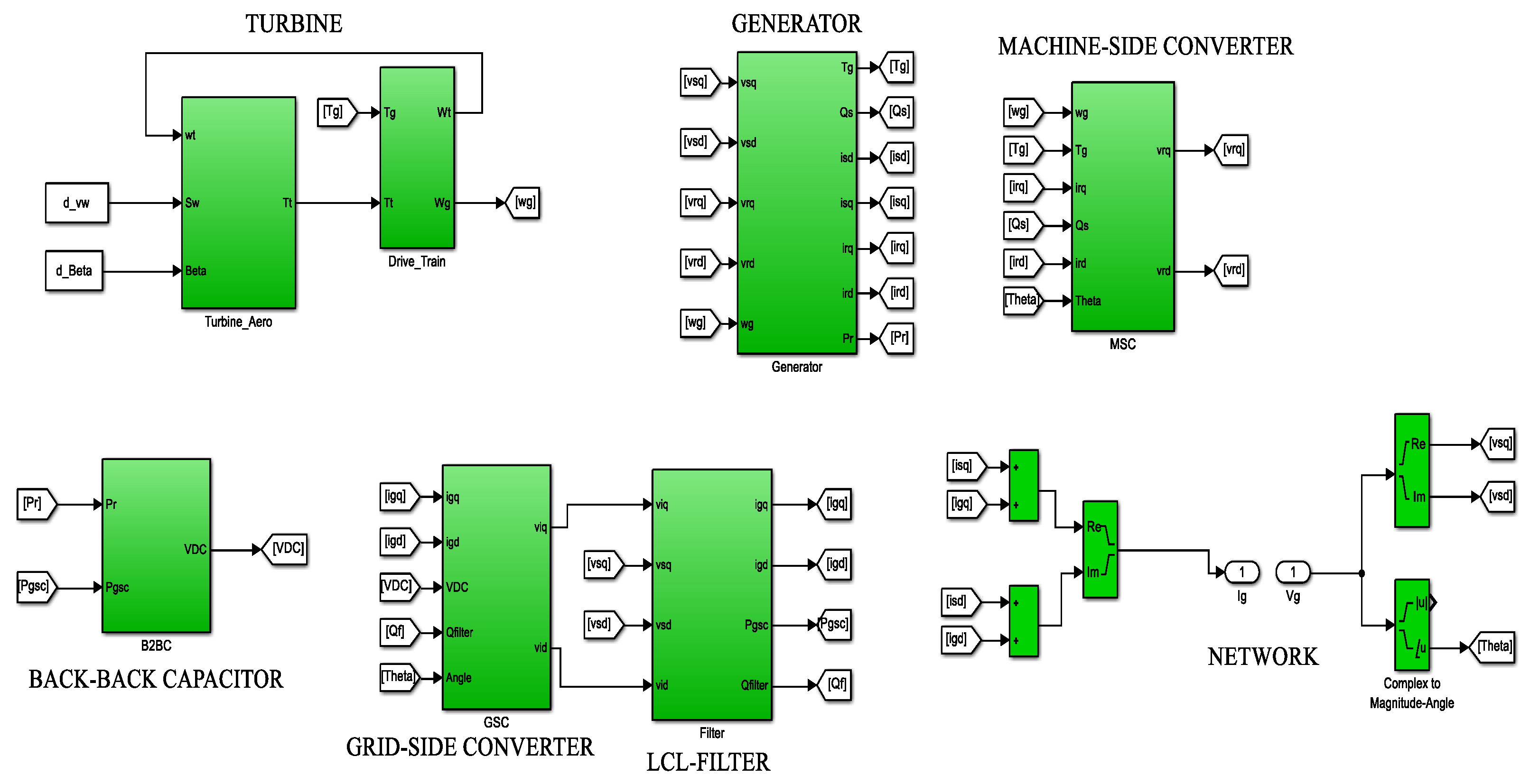
2.3. DFIG-WECS System
The DFIG-WECS Subsystems
- 1.
- Turbine: It connects the wind speed to the induction generator rotor speed, and it consists of the wind aerodynamic model and the drive train models. The wind aerodynamic model represents the wind power extraction of the rotor, and is expressed in Equation (13); it provides the mechanical power output () fed to the drive train, which depends on the wind speed (), blade length of the turbine (R), and power coefficient ():where represents the blade power coefficient, which is the part of the wind energy that can be extracted from the turbine; it is a function of . Practically, it is usually derived from the performance curve of the turbine obtained from tested field data. However, for simulation purposes, it is represented using numerical approximations, as in Equation (14), which is adapted from reference [7].The drive train, on the other hand, uses the mechanical output from the wind aerodynamic model to drive the induction generator, and is represented in Equation (15):where subscripts refer to the generator, shaft, and turbine, respectively. represents the rotor speed of the generator, is the shaft angle twist of the generator, is the generator inertia, T represents the drive train shaft stiffness, is the coefficient damping of the drive train, and is the electrical base speed.
- 2.
- Induction generator: The generator takes the rotor speed from the turbine and the bus voltage of the generator from the network model as inputs; in turn, it generates the output current and electrical torque. The name DFIG means it has two feeds, the stationary stator part and the rotating rotor part. The stator windings are arranged such that the output stator currents produce a magnetic field that turns the rotor with angular speed in the air gap. The generator adopts the dq reference frames, just like the conventional power grid system. The stator currents in these reference frames are represented by Equation (16):where and represent the stator currents in the dq frames, is the electrical base speed, the subscripts refer to the generator and shaft, respectively; thus, is the speed of the generator shaft and is the generator speed; is the transient stator inductance, and are the stator voltages in the dq frame, and are the rotor voltages in the dq frame, is a transformational parameter derived from Park’s transformation, and represent the generator voltages behind the transient impedances and are represented in Equation (17):where and relate the rotor flux and , respectively, by Equation (18):The rotor and stator fluxes themselves are given by Equation (19):where represent the rotor, mutual, and stator inductances, respectively; the generator rotor currents are given by Equation (20):The generator rotor active and reactive power, the stator active and reactive power, and the electrical torque are described by Equation (21).
- 3.
- The filter: The filter links the rotor windings of the power grid system. An inductor–capacitor–inductor (LCL) type of filter was used in this study, which is made up of two inductors (), a damping resistor (), and a capacitor (). The LCL model takes inverter voltage () and stator voltage () as inputs, and provides the current injected into the grid through the filter () as outputs. The currents entering the filter are represented by Equation (22):The currents exiting the filter are given in Equation (23):The capacitor voltage of the filter is represented by Equation (24):Lastly, the reactive and active powers exiting the filter are represented by Equation (25):
- 4.
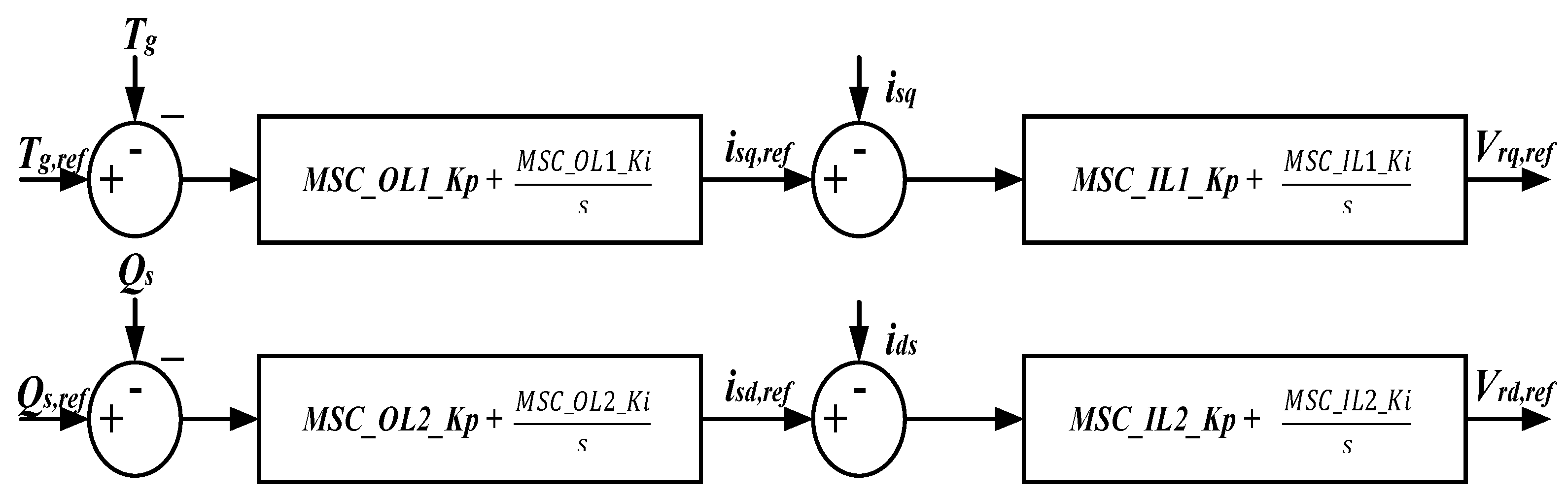
- The power electronics converters: The systems developed so far either represent the mechanical or electrical subsystems of the DFIG power test system. They are quite different from the controllers or converters in the sense that the converters are power electronics converters that contain microcontrollers that are run by some software codes. The power electronics converters include the machine-side and the grid-side converters. The controllers adopted in this study have a simple two cascaded proportional integral (PI) model. The machine-side converter receives voltages, generator rotor speed, and current in the form of electrical signals, and produces corresponding switching signals for the converter. The block diagram of the machine-side converter is shown in Figure 6.
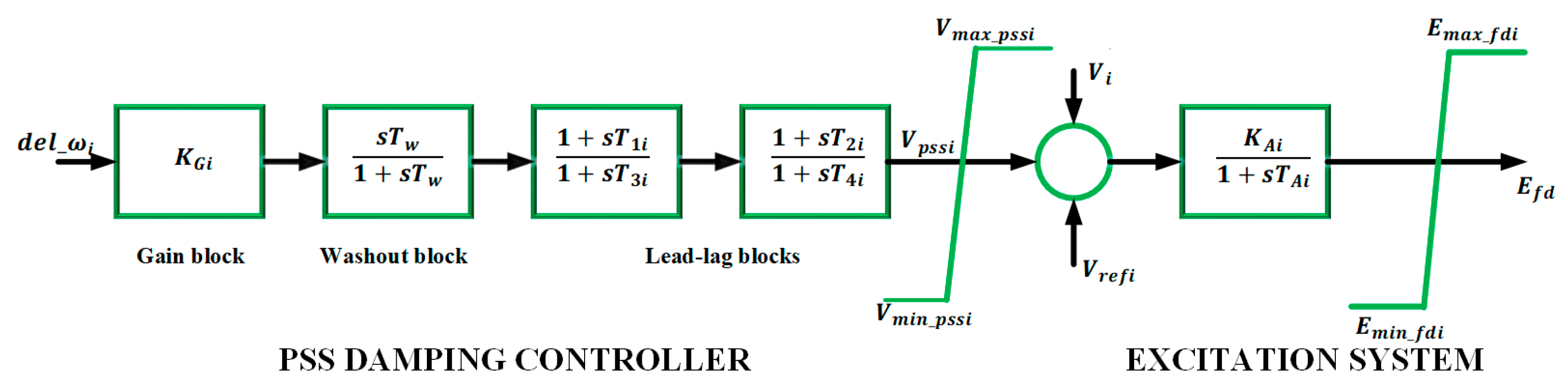
2.4. PSS Damping Controller Design
- The control gain : The control gain of the PSS determines the damping introduced by the PSS. The typical range of this value is between 0.1 and 50.
- The washout filter constant : The washout filter is a high-pass filter, and is also called a signal washout. It is normally set at 10 s.
- The phase compensators are two lead-lag blocks with parameters : The phase compensators determine the phase lag present in the system without the PSS, and then compensates for the phase lag [25].
2.5. System Linearization
2.6. Objective Function Formulation
- Minimize J
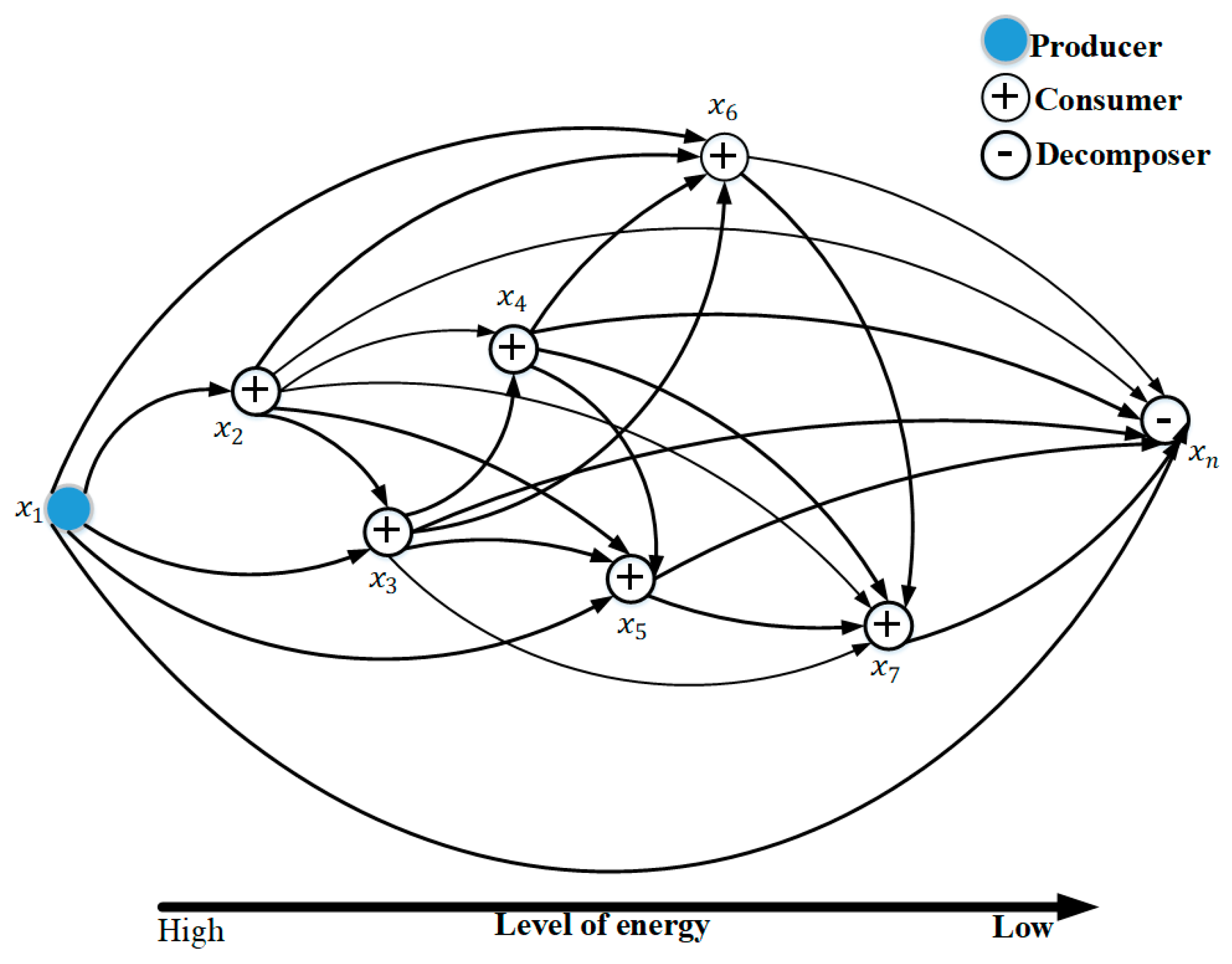
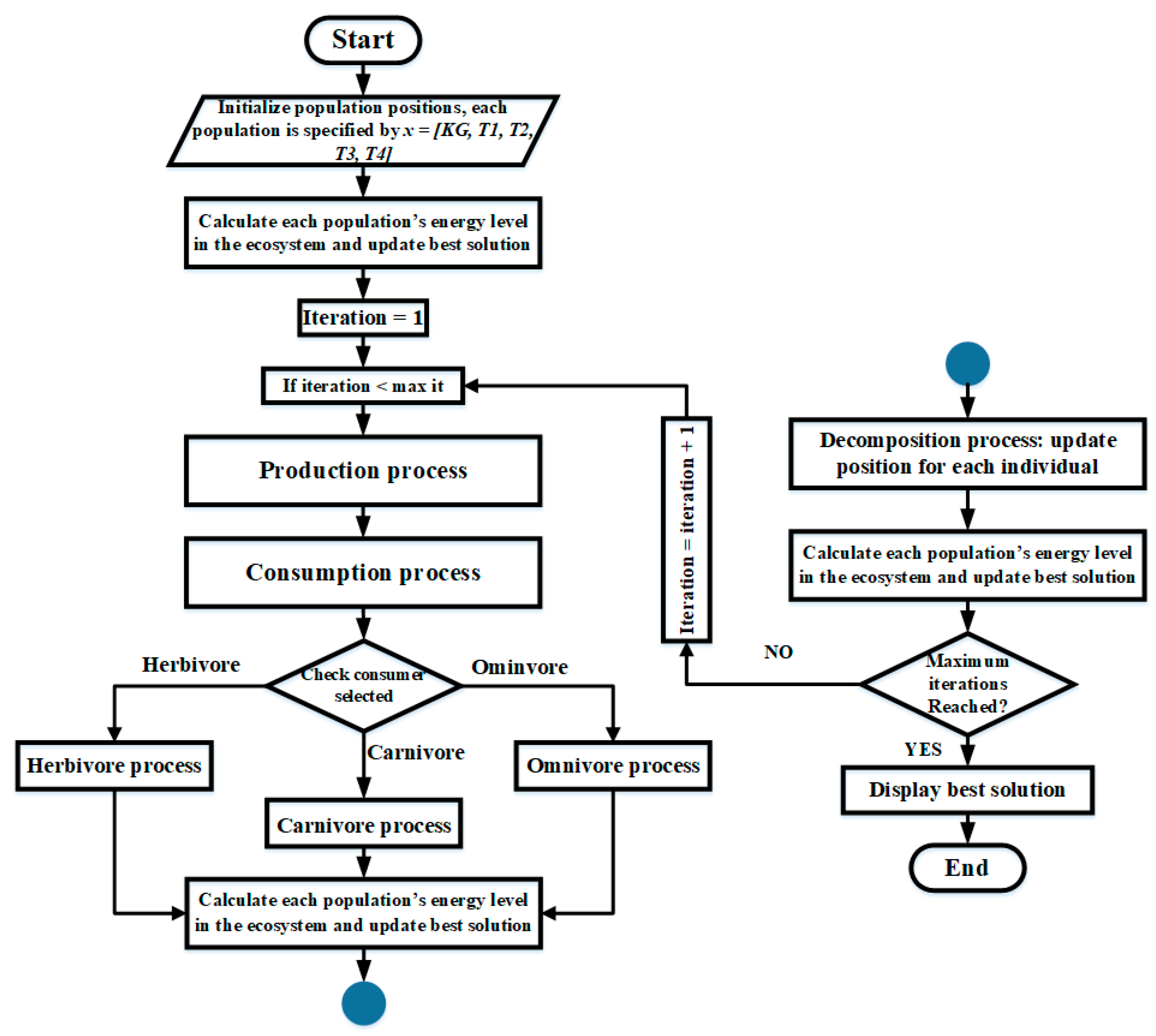
2.7. Artificial Eco-System Optimization (AEO)
- Calculate each population’s energy level in the ecosystem via the objective function in Equation (29), and update the best solution.
- Production process: using the production process, update the position for individual .
- Consumption process: Each consumer has the same probability for being selected; hence, for individuals , their position is updated using the herbivore process if the selected individuals are herbivores. If the selected individual is a carnivore, its position is updated using the carnivore process, and if they were omnivores, the omnivore process is deployed.
- Calculate each population’s energy level in the ecosystem using Equation (29), and update the result as the best solution.
- Decomposition process: Each position of is updated using the production process.
- Calculate each population’s energy level in the ecosystem via the objective function in Equation (29), and update the best solution.
- Repeat steps 3–7 until the maximum number of iterations is reached.
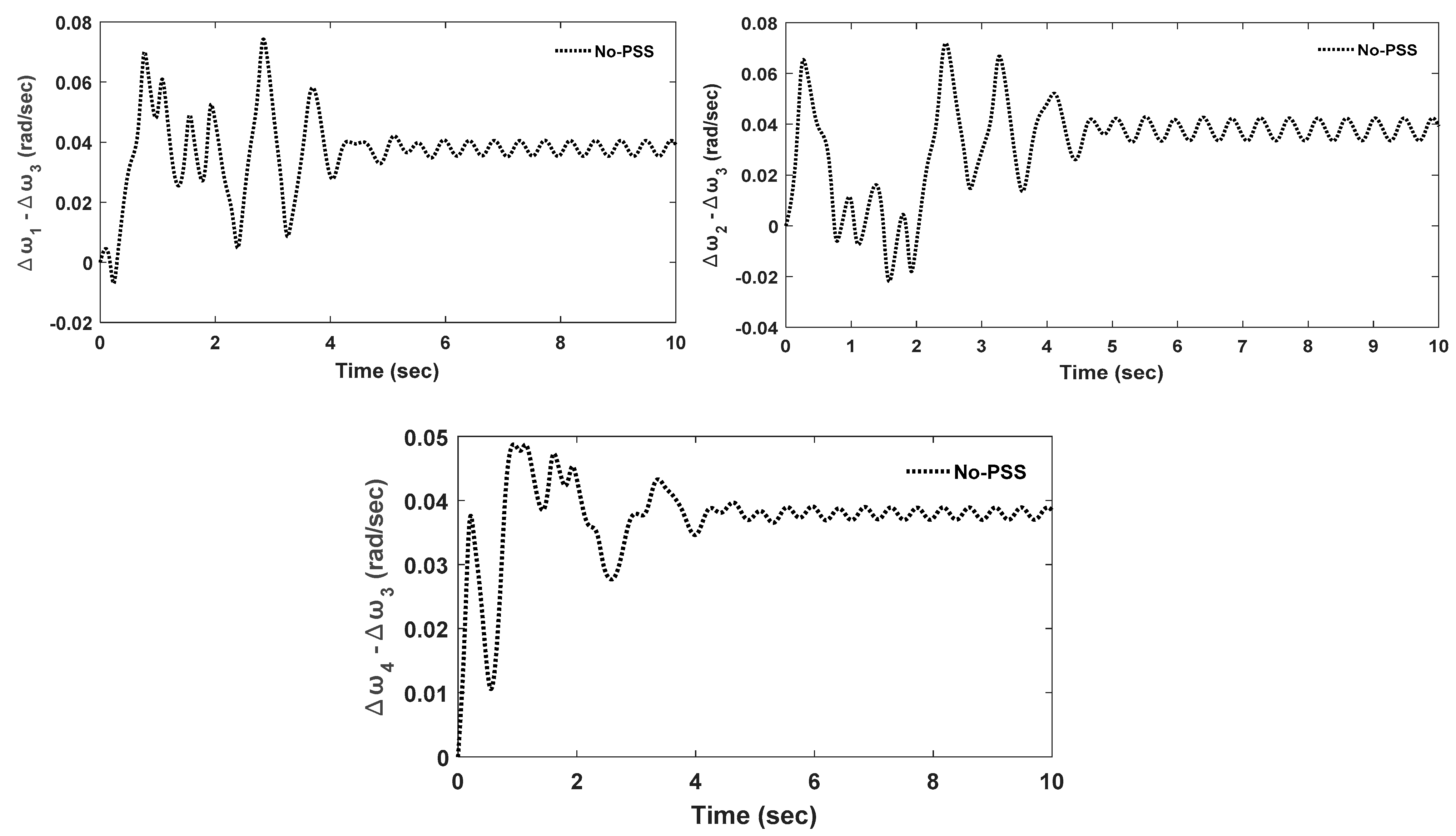
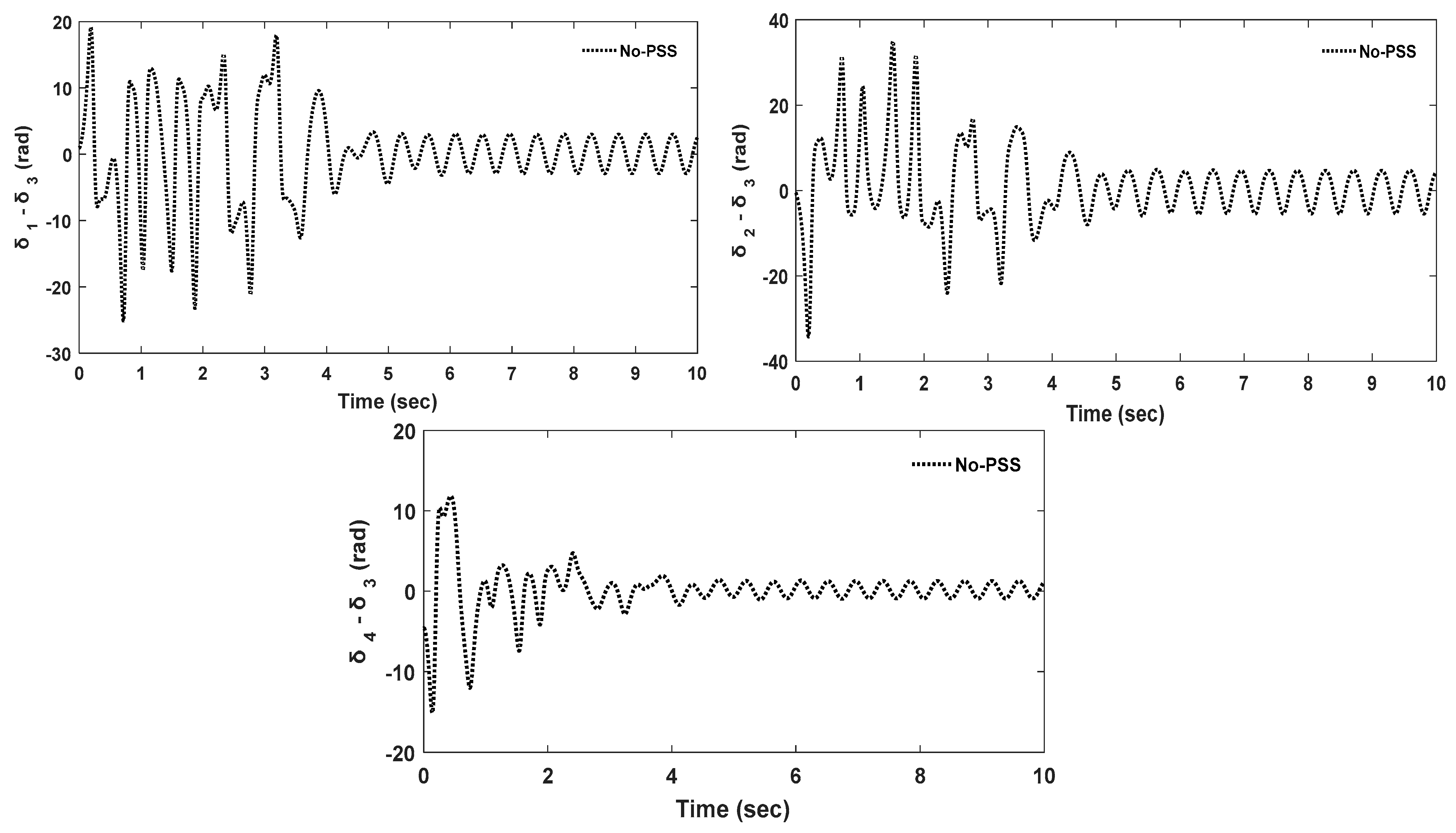
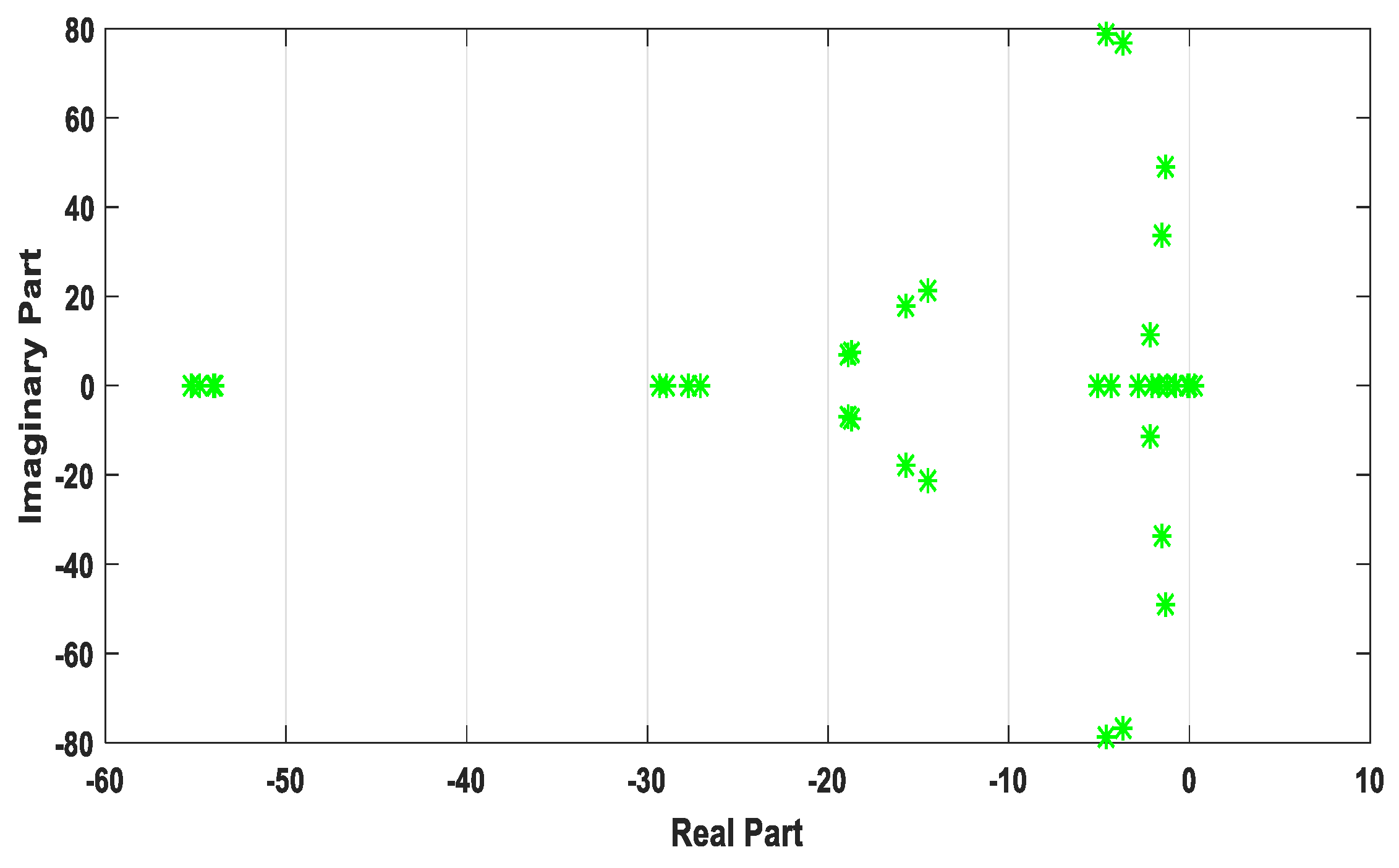
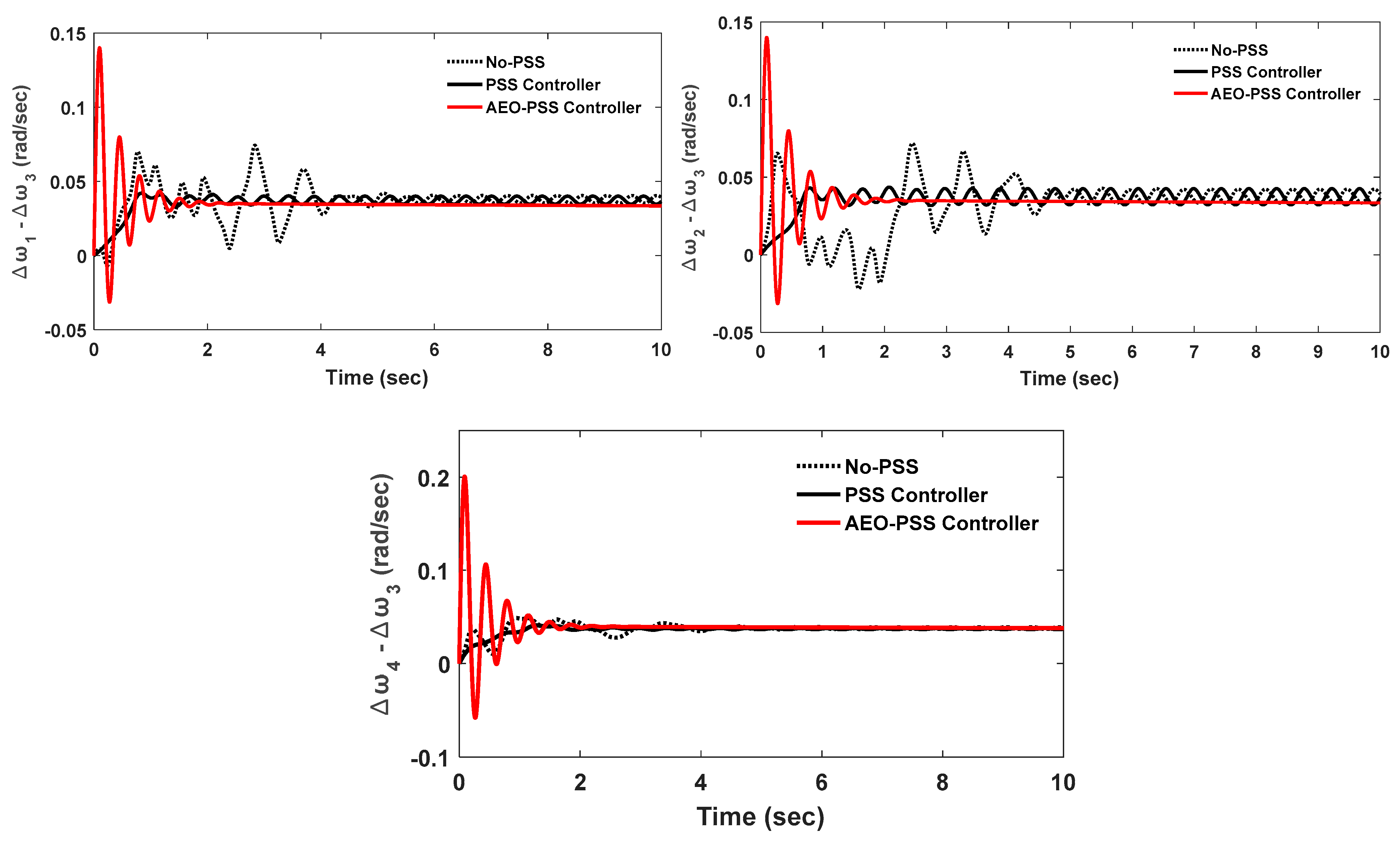
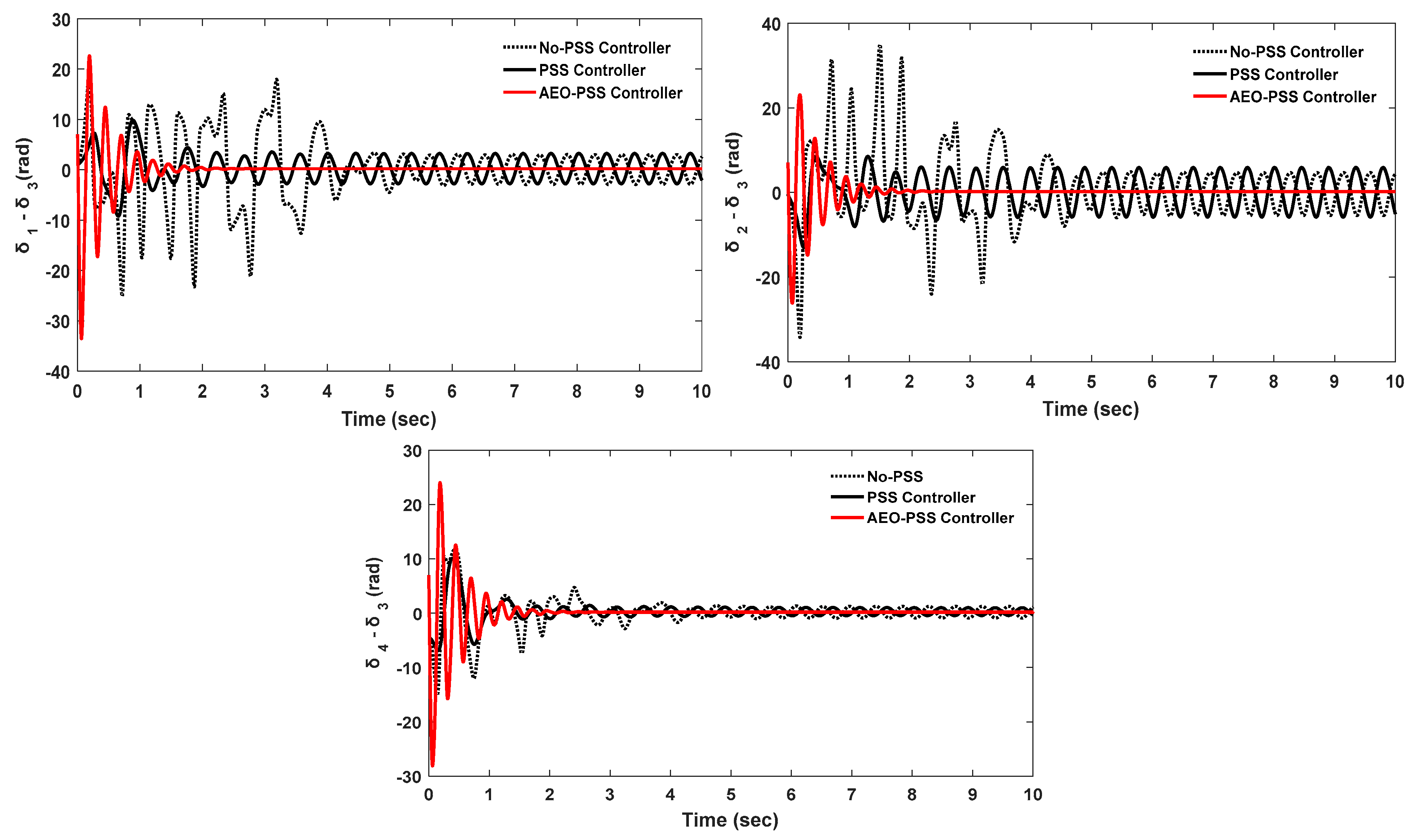
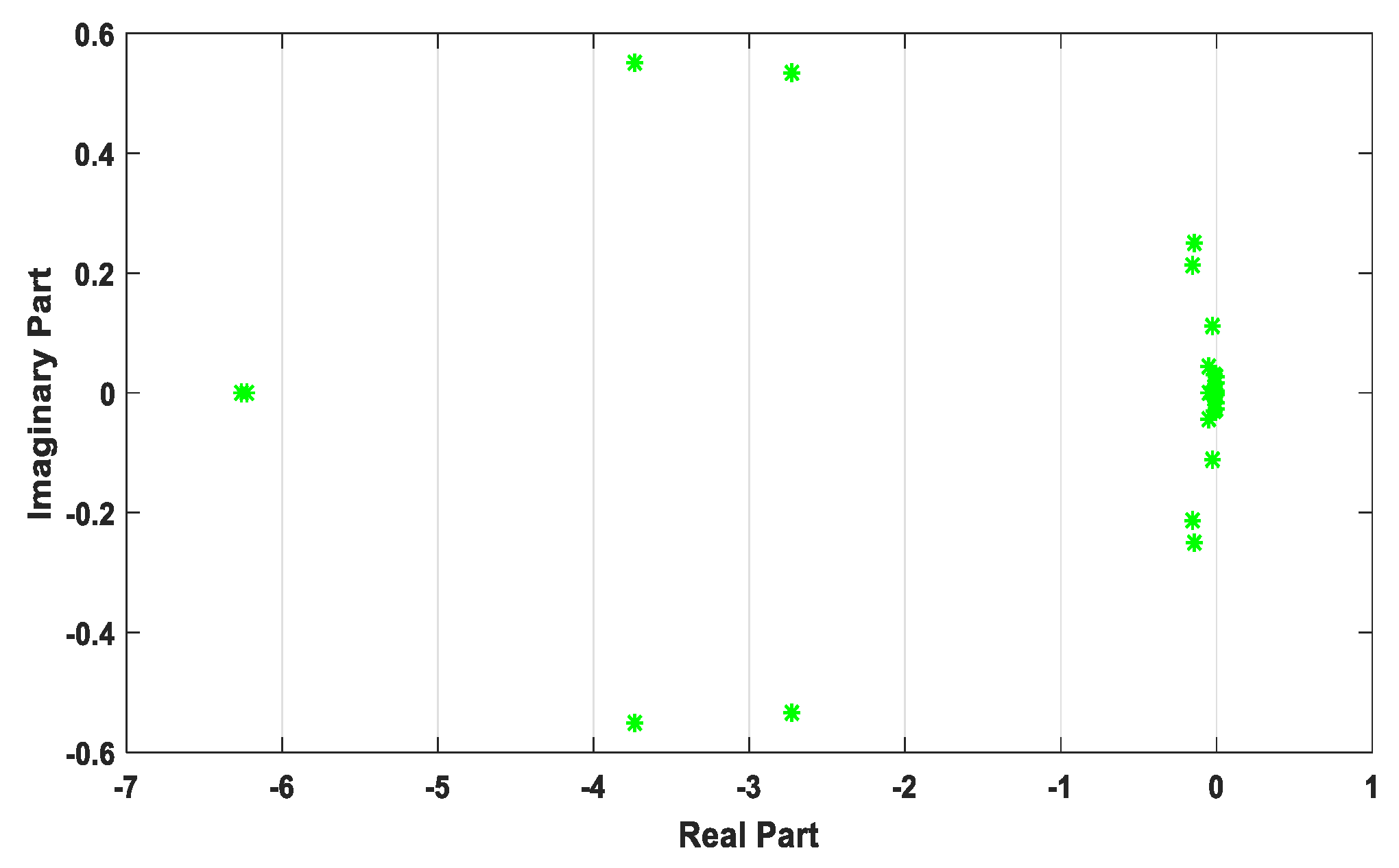
3. Results
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Elkasem, A.H.A.; Kamel, S.; Khamies, M.; Kabalci, E.; Shahinzadeh, H. Frequency stability enhancement of hybrid multi-area power grid considering high renewable energy penetration using TID controller. In Proceedings of the 2022 4th Global Power, Energy and Communication Conference (GPECOM), Nevsehir, Turkey, 14–17 June 2022; pp. 322–327. [Google Scholar]
- Sabo, A.; Kolapo, B.Y.; Odoh, T.E.; Dyari, M.; Abdul Wahab, N.I.; Veerasamy, V. Solar, Wind and Their Hybridization Integration for Multi-Machine Power System Oscillation Controllers Optimization: A Review. Energies 2023, 16, 24. [Google Scholar] [CrossRef]
- Bhukya, J.; Mahajan, V. The controlling of the DFIG based on variable speed wind turbine modeling and simulation. In Proceedings of the 2016 IEEE 6th International Conference on Power Systems (ICPS), New Delhi, India, 4–6 March 2016; pp. 1–6. [Google Scholar] [CrossRef]
- Bhukya, J.; Mahajan, V. Optimization of controllers parameters for damping local area oscillation to enhance the stability of an interconnected system with wind farm. Int. J. Electr. Power Energy Syst. 2020, 119, 105877. [Google Scholar] [CrossRef]
- Rawal, M.; Nauityal, D.C.; Rawat, M.S. Analysis of small signal stability in DFIG integrated power system. In Proceedings of the 2021 International Conference on Advances in Electrical, Computing, Communication and Sustainable Technologies (ICAECT), Bhilai, India, 19–20 February 2021. [Google Scholar] [CrossRef]
- Geng, H.; Xi, X.; Yang, G. Small-signal stability of power system integrated with ancillary-controlled largescale DFIG-based wind farm. IET Renew. Power Gener. 2017, 11, 1191–1198. [Google Scholar] [CrossRef]
- Kunjumuhammed, L.; Kuenzel, S.; Pal, B.C. Simulation of Power System with Renewables; Academic Press: Cambridge, MA, USA, 2019; ISBN 0128112549. [Google Scholar]
- Essallah, S.; Bouallegue, A.; Khedher, A. Integration of automatic voltage regulator and power system stabilizer: Small-signal stability in DFIG-based wind farms. J. Mod. Power Syst. Clean Energy 2019, 7, 1115–1128. [Google Scholar] [CrossRef]
- Sabo, A.; Odoh, T.E.; Shahinzadeh, H.; Azimi, Z.; Moazzami, M. Implementing Optimization Techniques in PSS Design for Multi-Machine Smart Power Systems: A Comparative Study. Energies 2023, 16, 2465. [Google Scholar] [CrossRef]
- Sabo, A.; Abdul Wahab, N.I.; Othman, M.L.; Mohd Jaffar, M.Z.A.; Beiranvand, H. Optimal design of power system stabilizer for multimachine power system using farmland fertility algorithm. Int. Trans. Electr. Energ Syst. 2020, 30, e12657. [Google Scholar] [CrossRef]
- Sabo, A.; Wahab, N.I.A.; Othman, M.L.; Jaffar, M.Z.A.M.; Beiranvand, H. Farmland fertility optimization for designing of interconnected multi-machine power system stabilizer. Appl. Model. Simul. 2020, 4, 183–201. [Google Scholar]
- Sabo, A.; Abdul Wahab, N.I.; Othman, M.L.; Mohd Jaffar, M.Z.A.; Beiranvand, H.; Acikgoz, H. Application of a neuro-fuzzy controller for single machine infinite bus power system to damp low-frequency oscillations. Trans. Inst. Meas. Control 2021, 43, 3633–3646. [Google Scholar] [CrossRef]
- He, P.; Zheng, M.; Li, Z.; Fang, Q.; Wu, X. Research on improving low frequency oscillation characteristics of wind power system with DFIG-PSS-VI. E3S Web Conf. 2021, 252, 2001. [Google Scholar] [CrossRef]
- Farah, A. A new design method of PSS for power system including DFIG-based wind turbines. In Proceedings of the 2021 12th International Renewable Engineering Conference (IREC), Amman, Jordan, 14–15 April 2021; pp. 1–6. [Google Scholar] [CrossRef]
- Prasad, D.K. Analysis of Small Signal and Transient Stability under the Penetration of DFIG Integrated Wind Energy Conversion System. In Proceedings of the 2019 International Conference on Electrical, Electronics and Computer Engineering (UPCON), Aligarh, India, 8–10 November 2019; pp. 1–6. [Google Scholar]
- Kamel, O.M.; Abdelaziz, A.Y.; Zaki Diab, A.A. Damping Oscillation Techniques for Wind Farm DFIG Integrated into Inter-Connected Power System. Electr. Power Compon. Syst. 2020, 48, 1551–1570. [Google Scholar] [CrossRef]
- Shah, N.N.; Joshi, S.R. Utilization of DFIG-based wind model for robust damping of the low frequency oscillations in a single SG connected to an infinite bus. Int. Trans. Electr. Energy Syst. 2019, 29, e2761. [Google Scholar] [CrossRef]
- Du, C.; Han, Y.; Li, S. Continuous twisting damping Control of DFIG-Based Wind Farm to Improve Interarea Oscillation Damping. In Proceedings of the 2021 China Automation Congress (CAC), Beijing, China, 22–24 October 2023; pp. 5432–5437. [Google Scholar] [CrossRef]
- Tan, A.; Tang, Z.; Sun, X.; Zhong, J.; Liao, H.; Fang, H. Genetic Algorithm-Based Analysis of the Effects of an Additional Damping Controller for a Doubly Fed Induction Generator. J. Electr. Eng. Technol. 2020, 15, 1585–1593. [Google Scholar] [CrossRef]
- Dhami, N.; Bhadu, M.; Bishnoi, S.K.; Sharma, K.G. Impact of PSS Tuning Methods on Grid Connected DFIG System. pp. 1–7. Available online: https://ssrn.com/abstract=3314844 (accessed on 2 April 2024).
- Hannan, M.A.; Islam, N.N.; Mohamed, A.; Lipu, M.S.H.; Ker, P.J.; Rashid, M.M.; Shareef, H. Artificial intelligent based damping controller optimization for the multi-machine power system: A review. IEEE Access 2018, 6, 39574–39594. [Google Scholar] [CrossRef]
- Kundur, P.; Paserba, J.; Ajjarapu, V.; Andersson, G.; Bose, A.; Canizares, C.; Hatziargyriou, N.; Hill, D.; Stankovic, A.; Taylor, C. Definition and classification of power system stability IEEE/CIGRE joint task force on stability terms and definitions. IEEE Trans. Power Syst. 2004, 19, 1387–1401. [Google Scholar]
- Saadat, H. Power System Analysis, 2nd ed.; McGraw Hill Higher Education: New York, NY, USA, 2009. [Google Scholar]
- Ekinci, S.; İzci, D.; Hekimoğlu, B. Implementing the Henry Gas Solubility Optimization Algorithm for Optimal Power System Stabilizer Design. Electrica 2021, 21, 250–258. [Google Scholar] [CrossRef]
- Ekinci, S.; Demiroren, A.; Hekimoglu, B. Parameter optimization of power system stabilizers via kidney-inspired algorithm. Trans. Inst. Meas. Control 2019, 41, 1405–1417. [Google Scholar] [CrossRef]
- Beiranvand, H.; Rokrok, E. General relativity search algorithm: A global optimization approach. Int. J. Comput. Intell. Appl. 2015, 14, 1550017. [Google Scholar] [CrossRef]
- Zhao, W.; Wang, L.; Zhang, Z. Artificial ecosystem-based optimization: A novel nature-inspired meta-heuristic algorithm. Neural Comput. Appl. 2020, 32, 9383–9425. [Google Scholar] [CrossRef]





| Algorithm | Parameter | Value |
|---|---|---|
| AEO | Population size | 100 |
| Maximum size | 100 | |
| Number of runs | 10 | |
| Producer | ||
| Decomposer | ||
| Consumers |
| Mode | Eigenvalue | Damping Ratio |
|---|---|---|
| 1 | 0.0471 | |
| 2 | 0.0587 | |
| 3 | 0.0271 | |
| 4 | 0.0443 | |
| 5 | 0.6864 | |
| 6 | 0.8798 | |
| 7 | 0.9287 | |
| 8 | 0.9380 | |
| 9 | 0.1938 | |
| 10 | 0.7598 | |
| 11 | 0.0062 | |
| 12 | 0.0065 | |
| 13 | −0.4061 |
| 5.1219 | 0.268 | 0.055 | 0.636 | 0.789 | |
| 25.517 | 0.444 | 0.594 | 0.001 | 0.408 | |
| 11.602 | 0.623 | 0.297 | 0.691 | 0.315 |
| Mode | Eigenvalue | Damping Ratio |
|---|---|---|
| 1 | 0.9893 | |
| 2 | 0.9813 | |
| 3 | 0.5004 | |
| 4 | 0.5829 | |
| 5 | 0.7313 | |
| 6 | 0.1902 | |
| 7 | 0.3958 | |
| 8 | 0.9997 | |
| 9 | 0.3108 | |
| 10 | 0.3226 | |
| 11 | 0.6819 | |
| 12 | 0.9991 | |
| 13 | 0.6075 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sabo, A.; Ebuka Odoh, T.; Veerasamy, V.; Abdul Wahab, N.I. Modified Multimachine Power System Design with DFIG-WECS and Damping Controller. Energies 2024, 17, 1841. https://doi.org/10.3390/en17081841
Sabo A, Ebuka Odoh T, Veerasamy V, Abdul Wahab NI. Modified Multimachine Power System Design with DFIG-WECS and Damping Controller. Energies. 2024; 17(8):1841. https://doi.org/10.3390/en17081841
Chicago/Turabian StyleSabo, Aliyu, Theophilus Ebuka Odoh, Veerapandiyan Veerasamy, and Noor Izzri Abdul Wahab. 2024. "Modified Multimachine Power System Design with DFIG-WECS and Damping Controller" Energies 17, no. 8: 1841. https://doi.org/10.3390/en17081841
APA StyleSabo, A., Ebuka Odoh, T., Veerasamy, V., & Abdul Wahab, N. I. (2024). Modified Multimachine Power System Design with DFIG-WECS and Damping Controller. Energies, 17(8), 1841. https://doi.org/10.3390/en17081841






