Measurements and Modelling of the Discharge Cycle of a Grid-Connected Hydro-Pneumatic Energy Storage System
Abstract
1. Introduction
1.1. Background
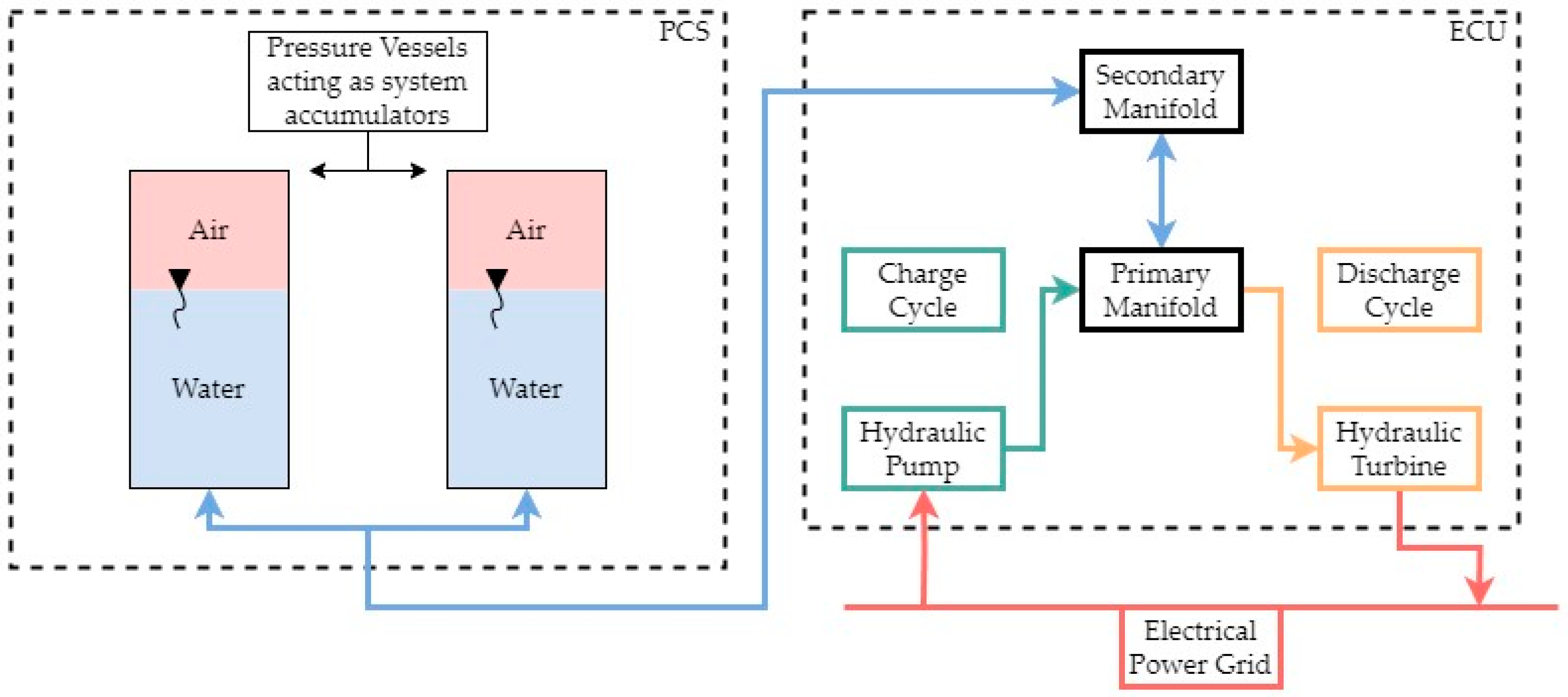
1.2. HPES System Being Developed for the MUSICA Project
1.3. Paper Overview
- The hydraulic circuit, consisting of hoses and manifolds, which supplies pressurised water from the accumulator to the Pelton turbine;
- The Pelton turbine assembly, operated at different constant speed settings;
- The coupled electrical generator and regenerative variable-speed drive.
2. Background Theory
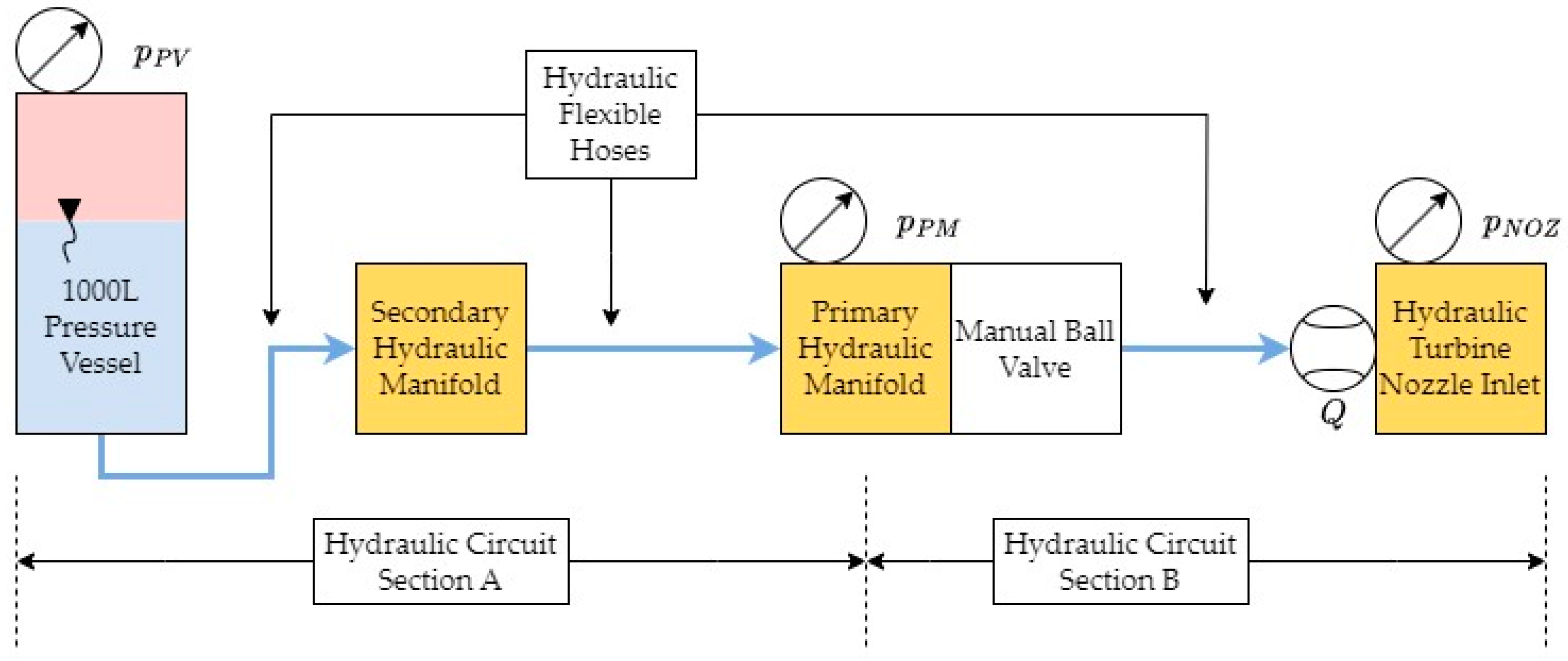
2.1. Hydraulic Circuit
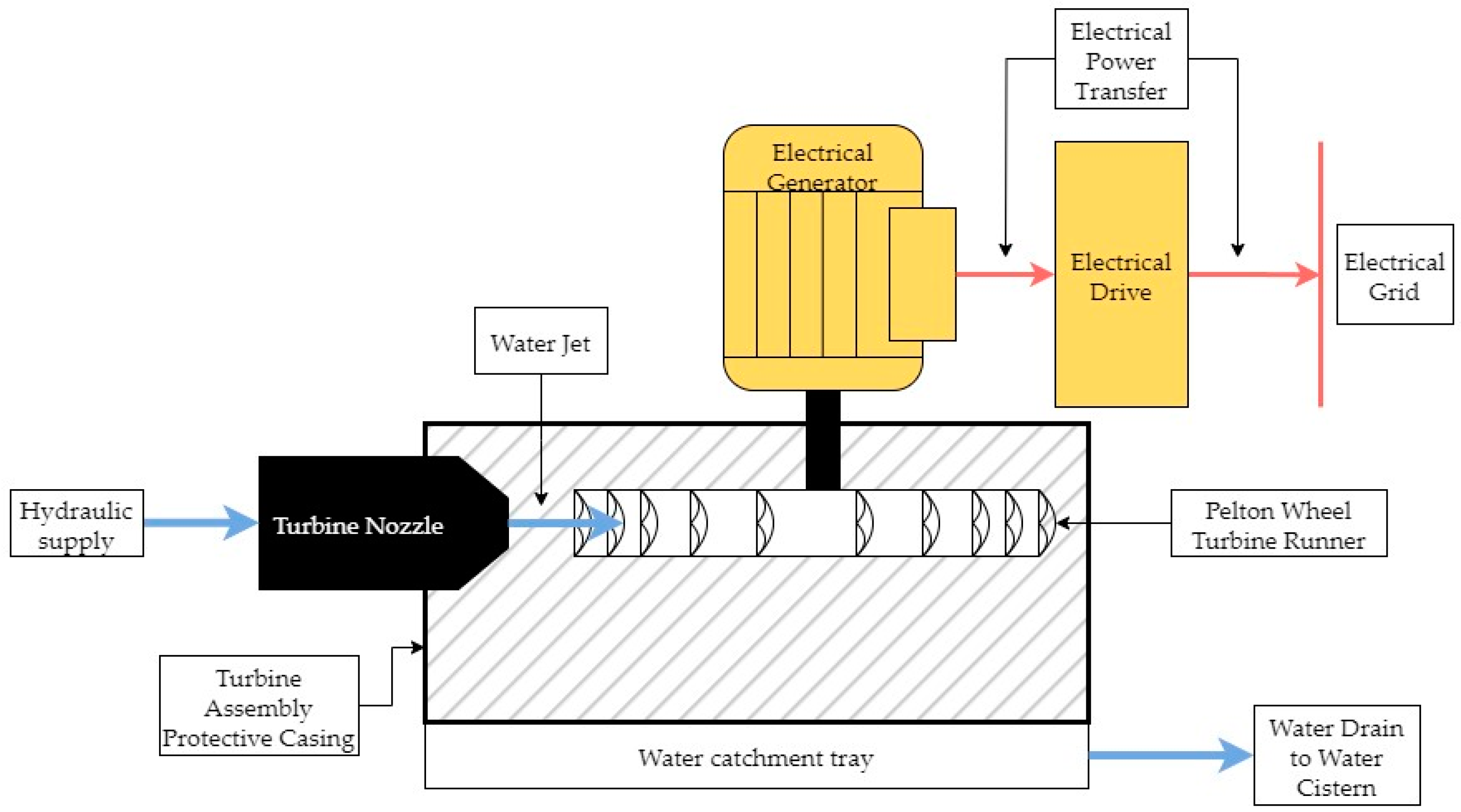
2.2. Hydraulic Turbine Assembly
2.2.1. Hydraulic Turbine Nozzle
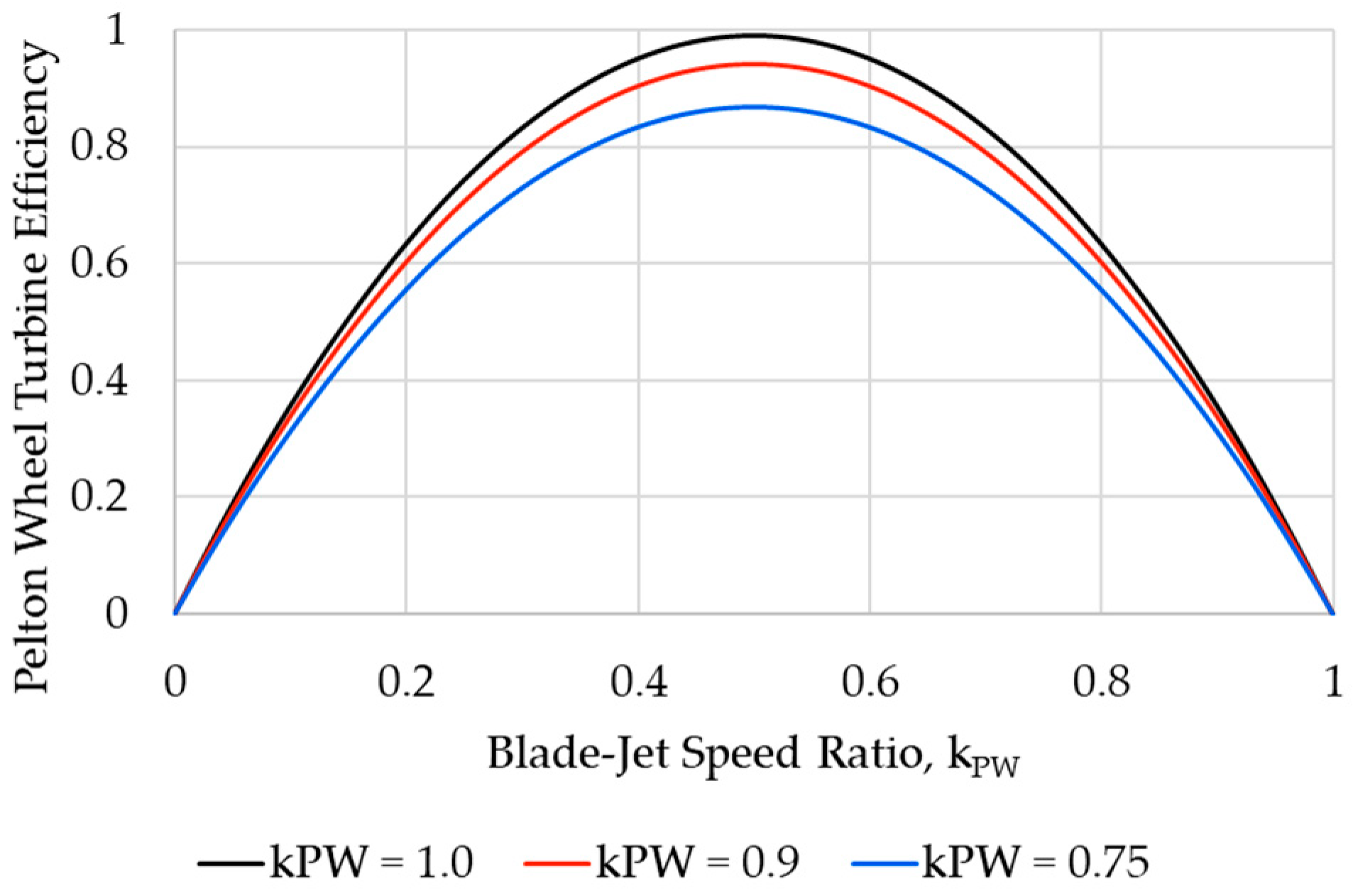
2.2.2. Pelton Wheel Turbine
2.3. Electrical Components
2.4. Overall ECU Performance
3. Equipment Setup of the Energy Conversion Unit (ECU)
- The hydraulic circuit, which provides hydraulic power, sourced from the storage accumulator, to the hydraulic turbine inlet;
- The hydraulic turbine, which converts hydraulic power from the accumulator into rotational power about a shaft;
- The main electrical components, which include an electrical generator, directly connected to the main hydraulic turbine shaft, and an electrical motor regeneration-enabled drive for controlling the generator and providing a controlled electrical power supply to the power grid.
3.1. Hydraulic Circuit Setup
- Pressure sensor at the accumulator (pressure vessel, );
- Pressure sensor at the primary manifold ();
- Pressure sensor at the turbine nozzle inlet ();
- Flow meter just before the turbine nozzle inlet ().
3.2. The Hydraulic Turbine Setup
3.3. Setup of Electrical Components
3.4. Data Logger and System Control
3.5. HPES System Operation
3.5.1. Main Discharge-Cycle Experiments
- The hydraulic turbine nozzle spear valve was set to the required position corresponding to a particular run. It should be noted that, at this point, the ball valve between the primary manifold and the hydraulic turbine was kept closed.
- The data logger and power quality analyser were initialised.
- The accumulator was prepared for a particular discharge cycle by first pre-charging it to 10 bar using an air compressor. The ECU hydraulic pump was then used to charge the pressure vessel to 30 bar.
- The hydraulic turbine was started in motoring mode from its electrical control drive using the corresponding cycle angular velocity (RPM, speed) setting.
- Once the hydraulic turbine achieved a steady angular velocity, the ball valve at the primary manifold was opened. This provided a hydraulic supply with which to operate the turbine, thus starting a discharge cycle.
- The discharge of the accumulator was allowed to continue until the system’s state of charge (SOC) approached 11 bar. At this point, the ball valve at the primary hydraulic manifold was closed, concluding that particular discharge cycle.
- At this point, the discharge cycle was concluded and all equipment, such as turbine drive and data loggers, were switched off.
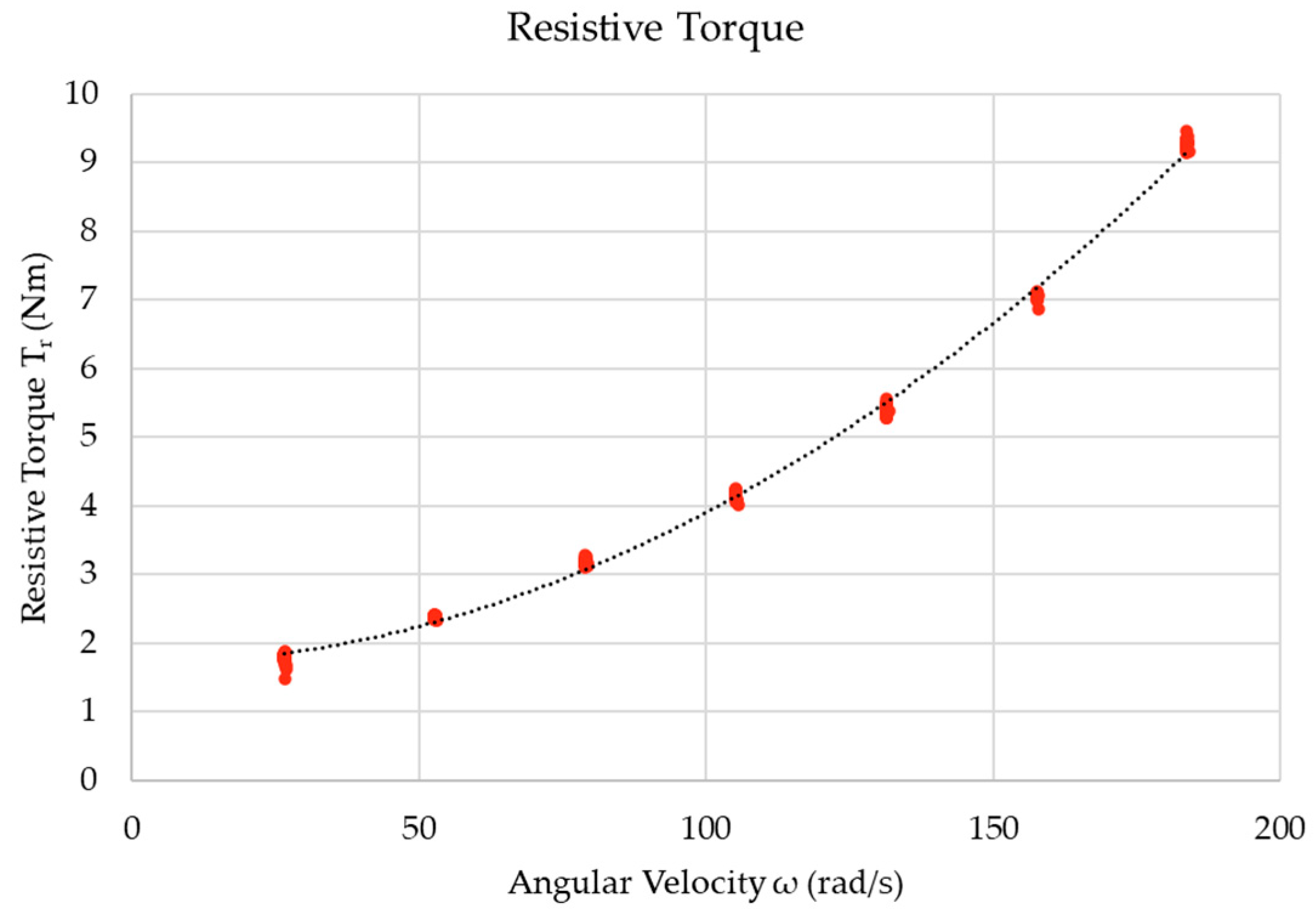
3.5.2. Steady-State Runs for Resistive Torque
4. Numerical Modelling
- A numerical model, developed using MATLAB Simulink (R2022a) to represent the full HPES ECU model used during the discharge cycle;
- CFD analyses, performed to model different hydraulic components for which no performance characteristics were available (specifically: , and ).
4.1. MATLAB Simulink Numerical Model
4.1.1. Numerical Model for the Hydraulic Circuit
4.1.2. Numerical Model Hydraulic Turbine Assembly
4.2. CFD Analysis
4.2.1. CFD Analysis Procedure and Methods
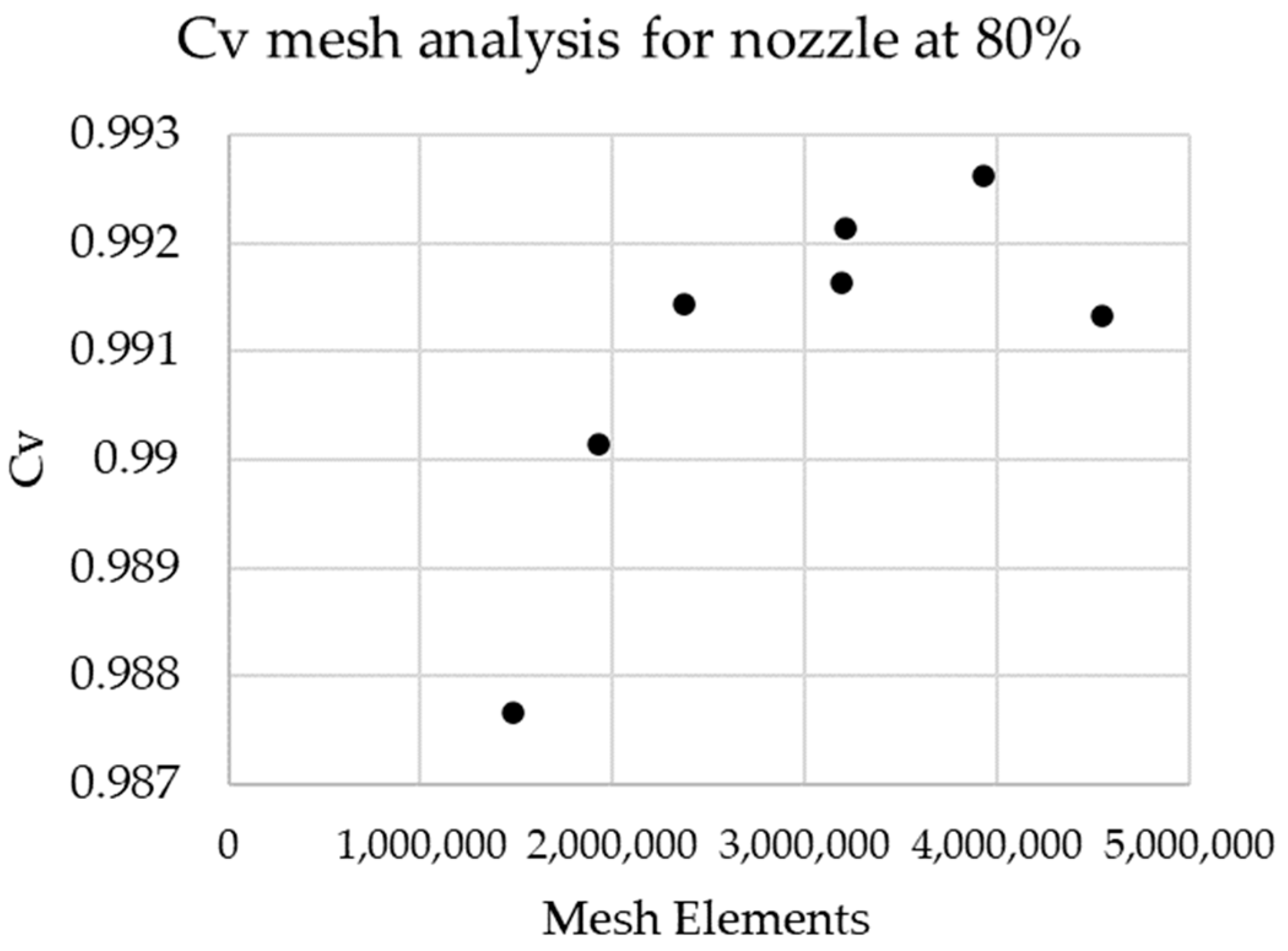
4.2.2. CFD Analysis Results
5. Results and Discussion
5.1. Hydraulic Circuit
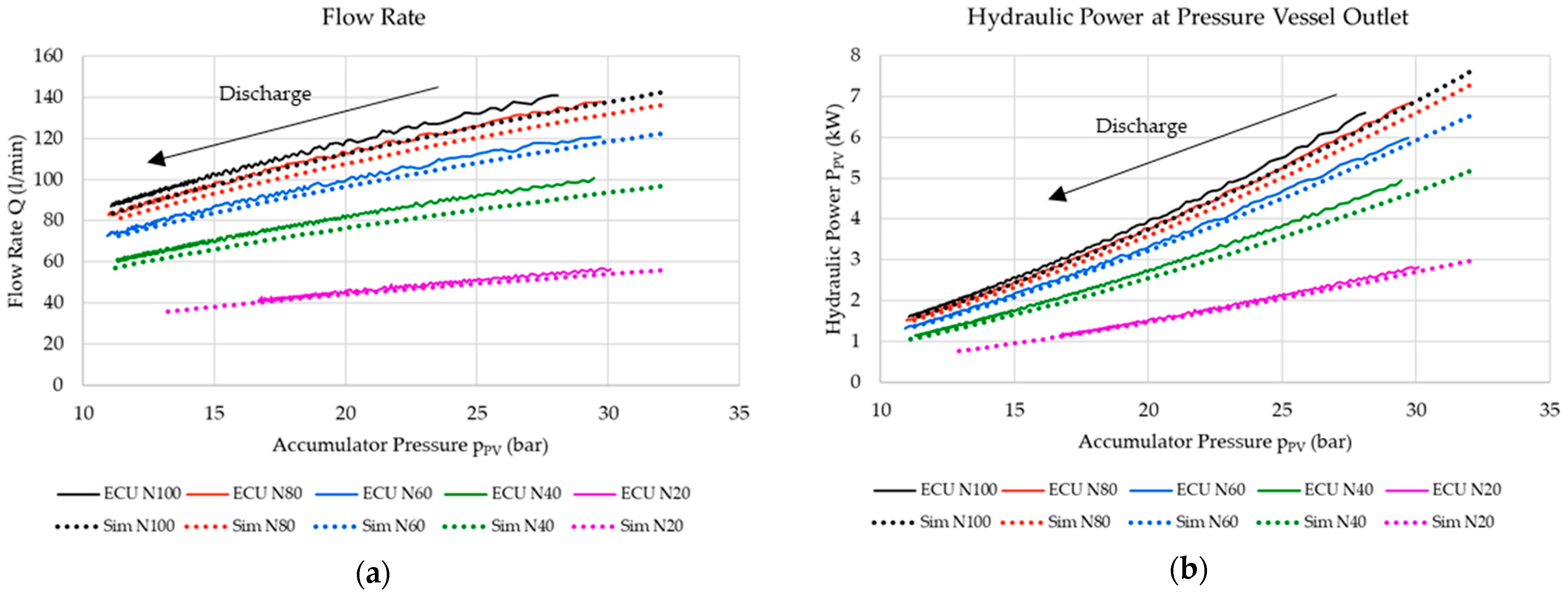
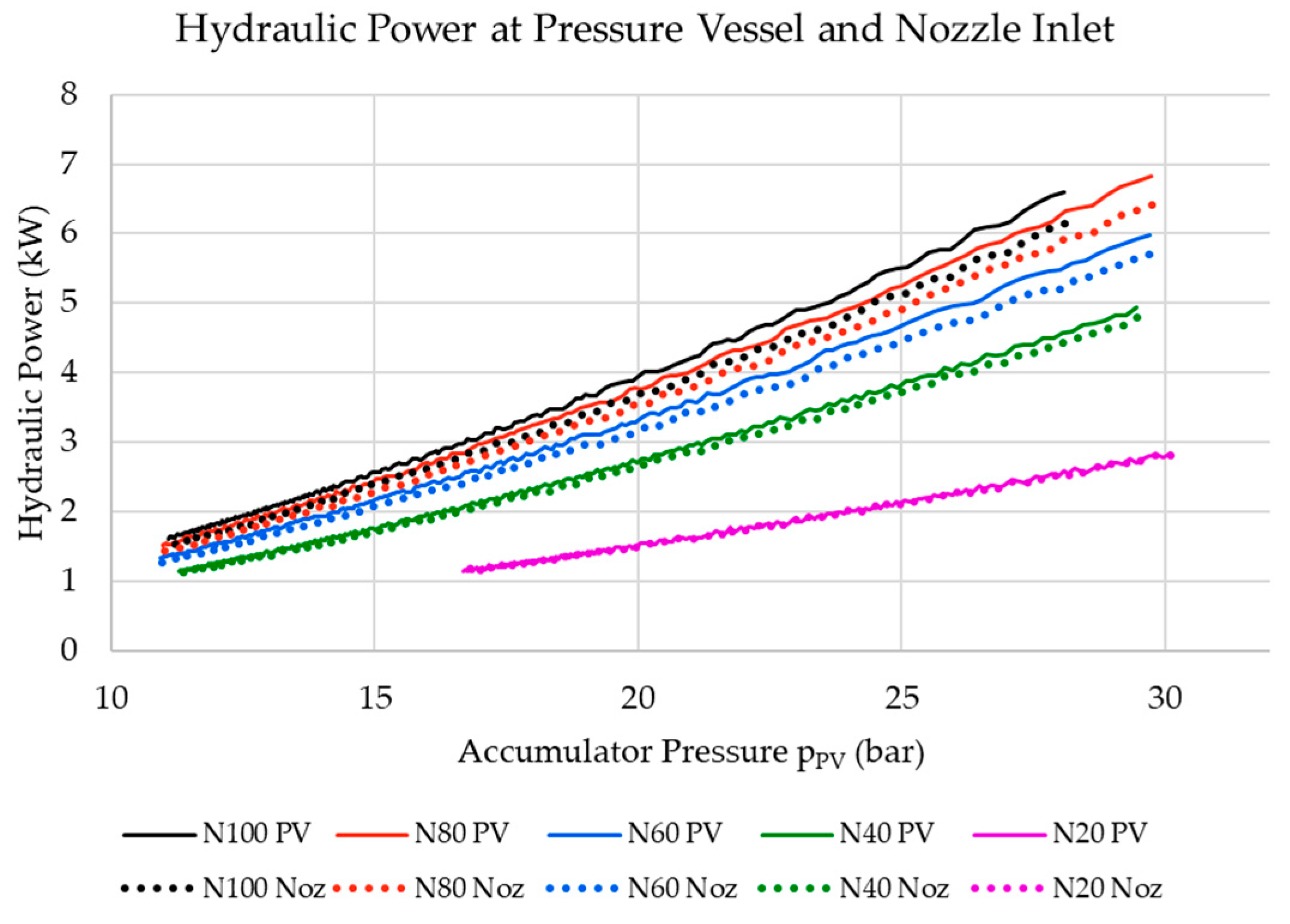
5.1.1. Hydraulic Power Supply from the Accumulator
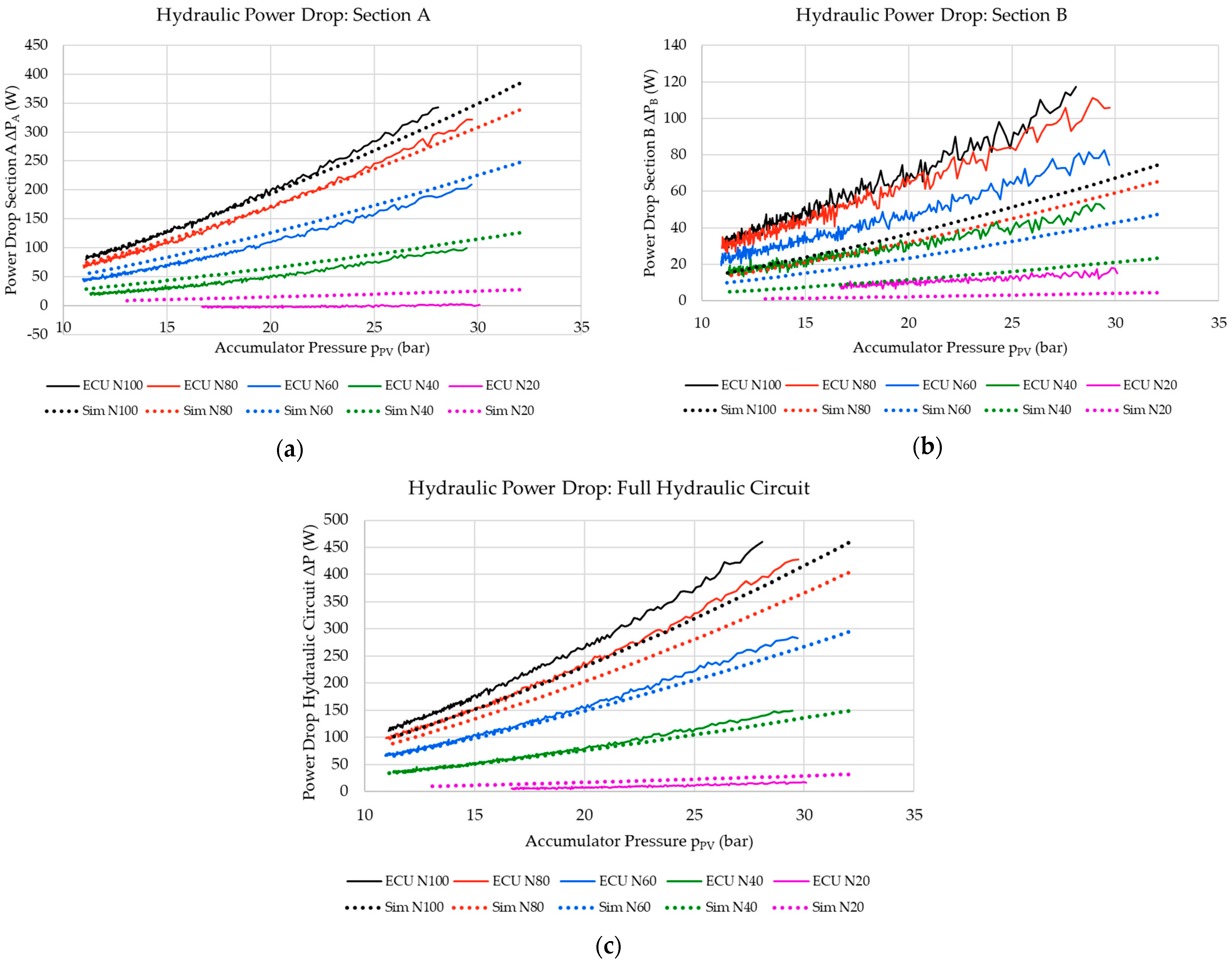
5.1.2. Pressure Drops within Hydraulic Circuit
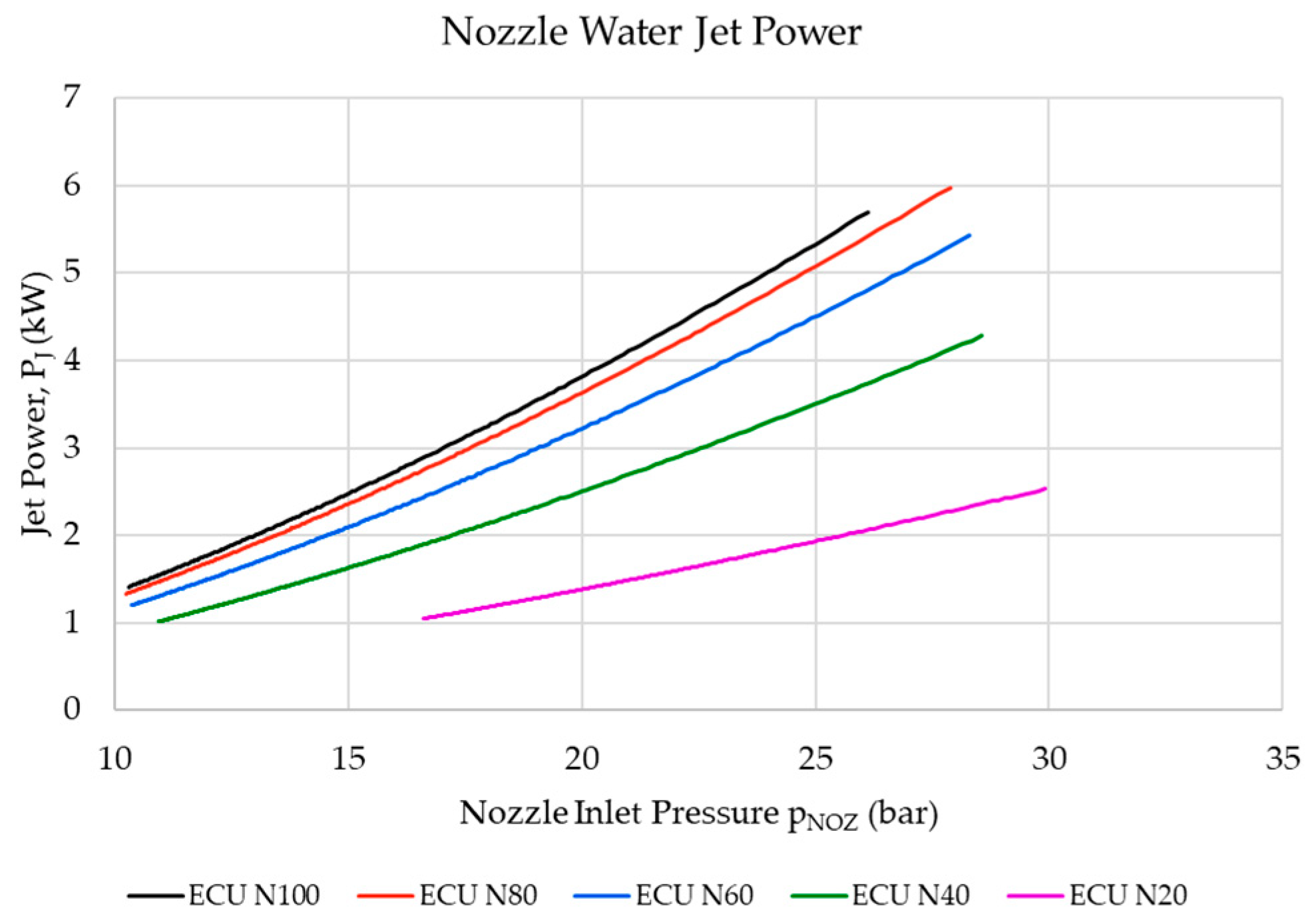
5.1.3. Power Drops within Hydraulic Circuit
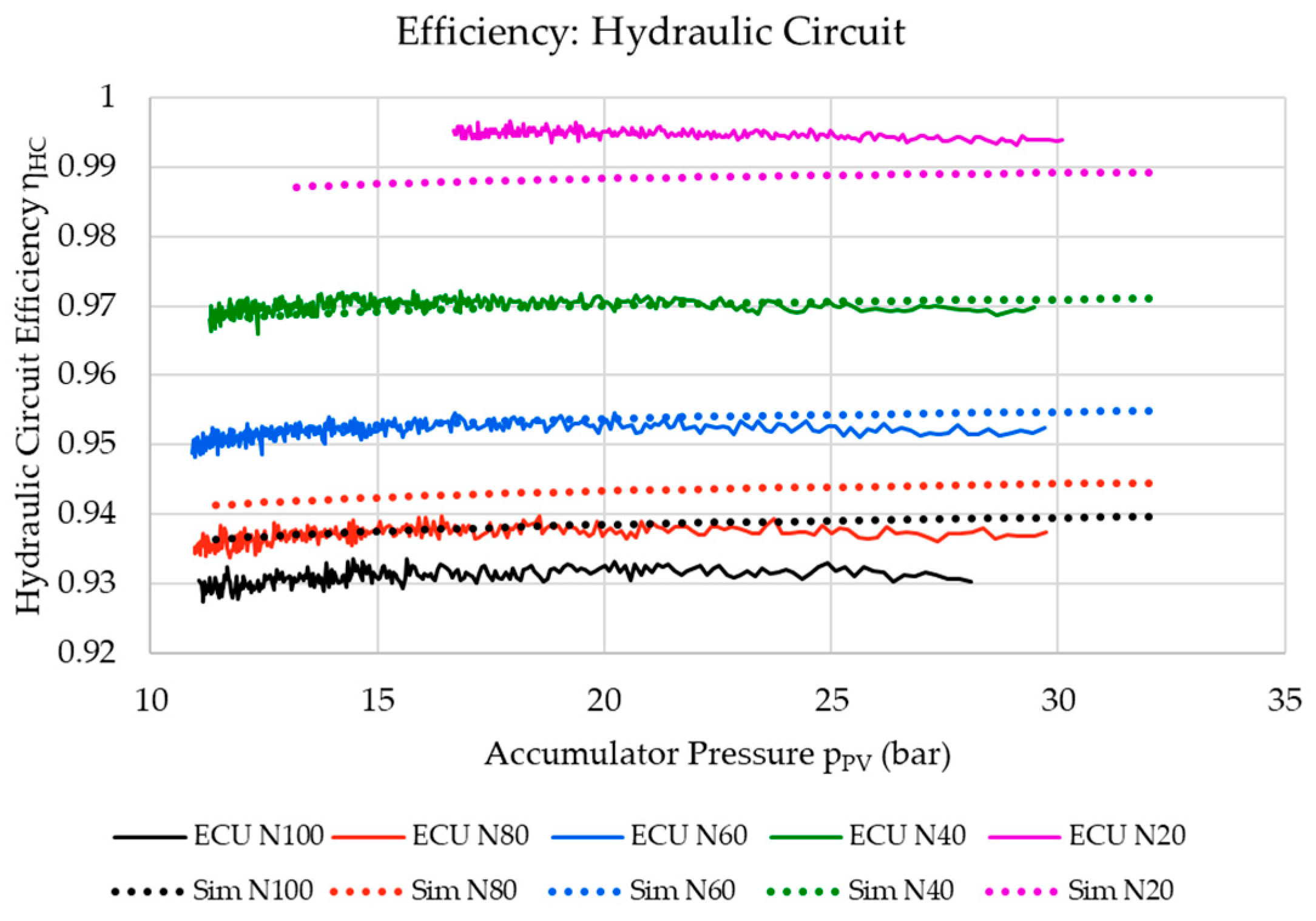
5.1.4. Hydraulic Circuit Efficiency
5.2. Hydraulic Turbine Assembly
5.2.1. The Hydraulic Turbine Nozzle
5.2.2. The Hydraulic Turbine’s Resistive Torque
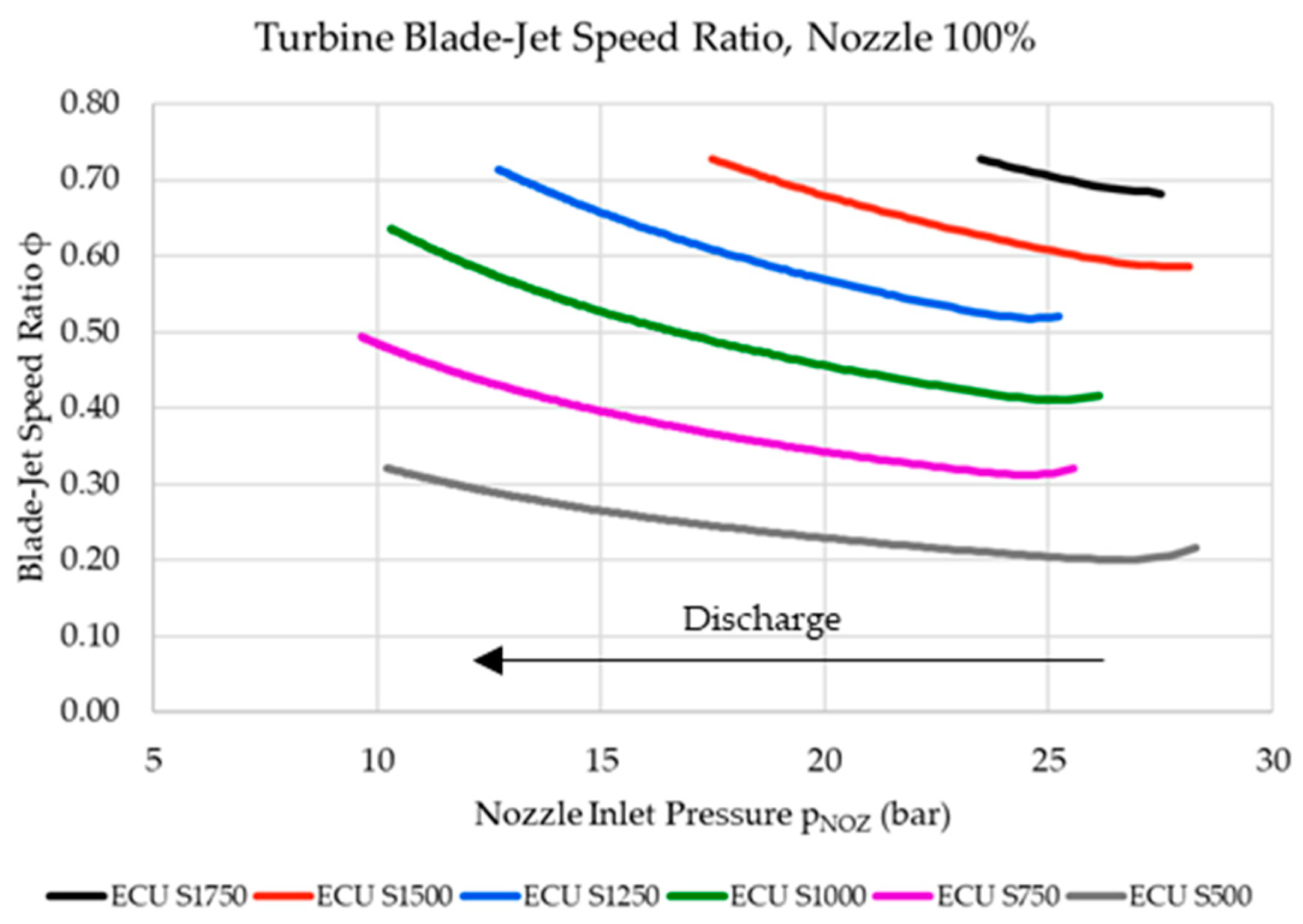
5.2.3. The Hydraulic Turbine’s Blade–Jet Speed Ratio
5.2.4. The Pelton Wheel Hydraulic Turbine
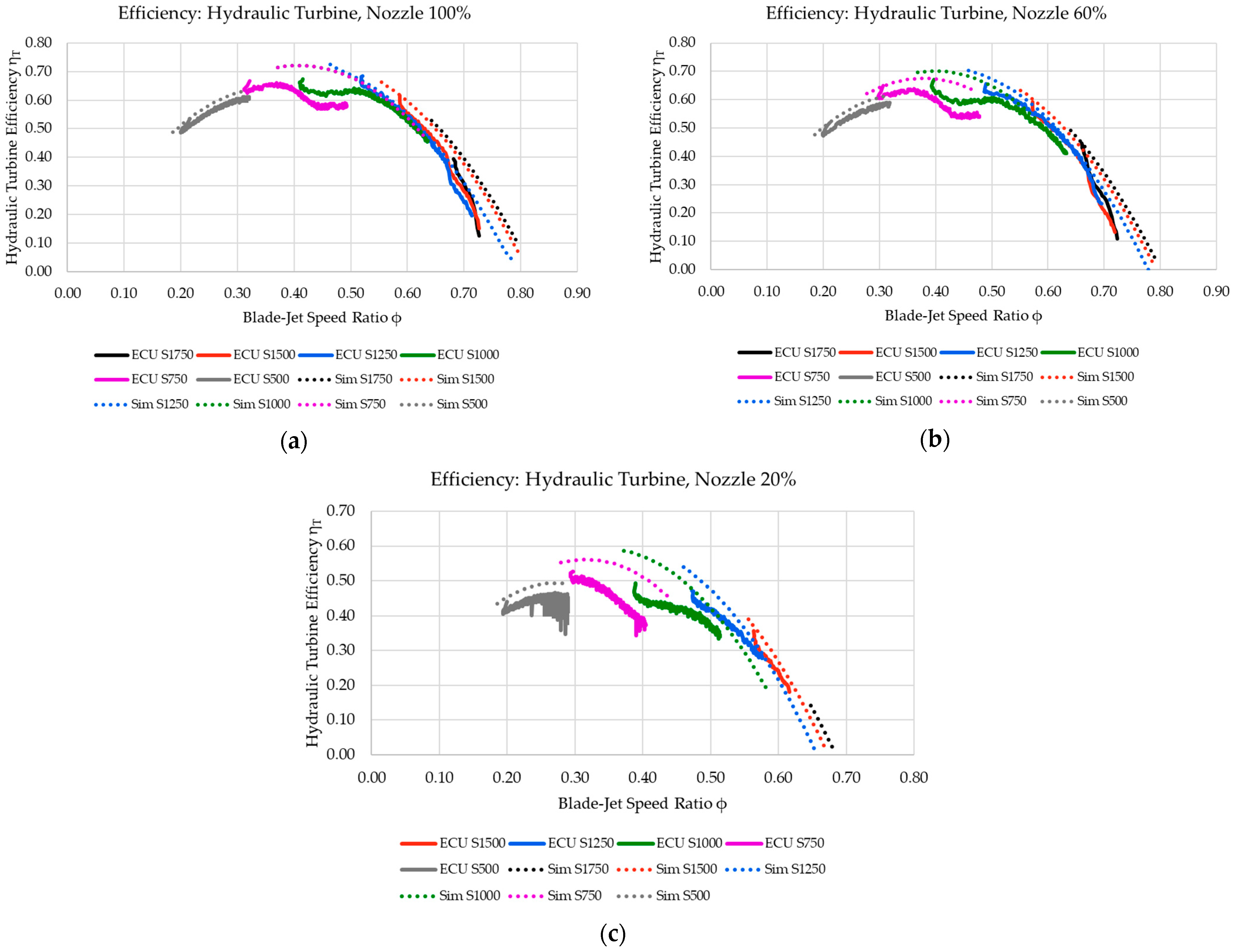
5.2.5. Hydraulic Turbine Assembly Efficiency
5.3. Electrical Components
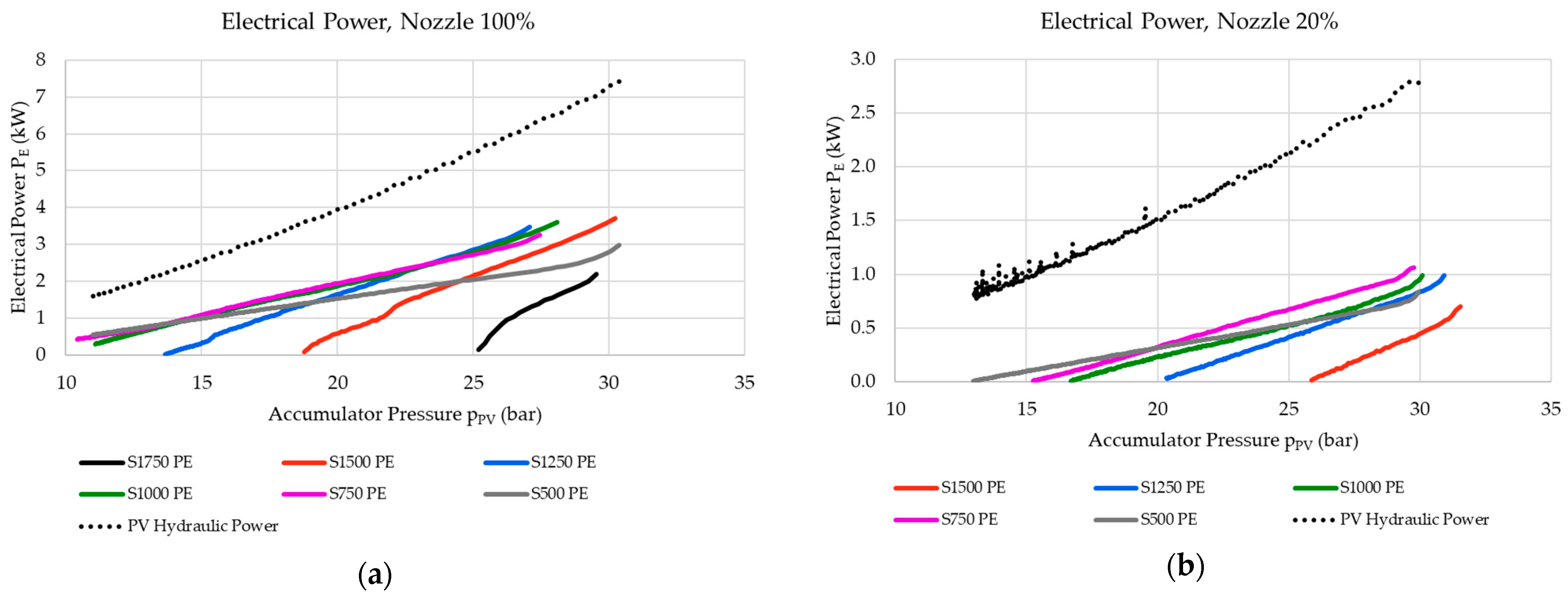
5.3.1. Electrical Power Output
5.3.2. Electrical Efficiency
5.4. The ECU’s Overall Efficiency
6. Concluding Remarks
6.1. Key Conclusions
- Overall discharge-cycle efficiency achieved by the ECU approached peaks of 55%, but only when operating under optimal bucket–jet speed ratios. The hydraulic circuit and turbine nozzle had minimal effects on the overall performance of the ECU, with the largest contributor to the overall ECU performance being the Pelton wheel turbine. The results clearly indicate that control over the turbine operational speed is essential to ensuring that operations occur in the ideal blade–jet speed ratio operational window as the overall system’s SOC decreases. This thus allows for the ECU to operate at optimal efficiency through a discharge cycle.
- When considering the full range of components, it was noticed that the ECU efficiency remains relatively constant over a particular pressure range. This indicates that, when operating close to the ideal blade–jet speed ratio, the efficiency remains relatively constant as the SOC decreases. However, in cases that diverge from ideal blade–jet speed ratio operational conditions, the efficiency drops drastically, with the system becoming increasingly sensitive to changes in the SOC.
- The numerical model achieved results that compared relatively well with the experimental results achieved for the physical ECU. However, particular operational phenomena relating to the physical ECU, such as the drop in efficiency when operating at the theoretical ideal blade–jet speed ratio or frictional effects, were not completely modelled.
- The results indicated that the electrical components selected, particularly the electrical generator, matched the expected operational characteristics of the HPES system. Whilst such components have relatively high operational efficiencies over most of their operational range, when such components are operated with low load factors, their efficiency drops drastically.
6.2. Future Work
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| AC | alternating current |
| CAES | compressed-air energy storage |
| CFD | computational fluid dynamics |
| CO2 | carbon dioxide |
| ECU | energy conversion unit |
| ESS | energy storage system |
| HPES | hydro-pneumatic energy storage system |
| MUP | multi-use platform |
| PCS | pressure containment system |
| PHES | pumped hydro energy storage |
| RES | renewable energy source |
| RPM | revolutions per minute |
| SOC | state of charge |
| UM | University of Malta |
| Nomenclature | |
| Hydraulic component hydraulic area, m2 | |
| Water jet area at hydraulic turbine nozzle outlet, m2 | |
| Hydraulic turbine nozzle coefficient of velocity | |
| Pipe hydraulic diameter, m | |
| Pipe frictional coefficient | |
| Mass moment of inertia, kg·m2 | |
| Hydraulic component pressure drop coefficients | |
| Pelton wheel turbine bucket frictional factor | |
| Pipe physical and additional lengths | |
| Mass flow rate, kg·s−1 | |
| Polytropic index | |
| Gauge pressure, Pa | |
| Pressure drop across full ECU hydraulic circuit or section/component, Pa | |
| Power drops across ECU hydraulic circuit or section/component, W | |
| Power, W | |
| Flow rate in hydraulic circuit, m3·s−1 | |
| Pelton wheel turbine bucket radial position from shaft, m | |
| Reynolds number | |
| Resistive torque at hydraulic turbine assembly, Nm | |
| Torque, Nm | |
| Time, s | |
| Pelton wheel turbine bucket linear velocity, m·s−1 | |
| Volume, m3 | |
| Water jet velocity at hydraulic turbine nozzle outlet, m·s−1 | |
| Water velocity across hydraulic component, m·s−1 | |
| Greek | |
| Pipe surface roughness, m | |
| Efficiency | |
| Pelton wheel turbine bucket angle, ° | |
| Water kinematic viscosity, m2·s−1 | |
| Water density, kg·m−3 | |
| Blade–jet speed ratio | |
| Hydraulic turbine angular velocity, rad·s−1 | |
| Subscripts | |
| A | Hydraulic circuit section A |
| B | Hydraulic circuit section B |
| E | Electrical |
| HC | Hydraulic circuit (complete circuit) |
| J | Water jet |
| PM | Primary hydraulic manifold |
| NOZ | Hydraulic turbine nozzle inlet |
| PV | Pressure vessel/Accumulator |
| PW | Pelton wheel turbine power or torque |
| PW-T | Pelton wheel turbine power or torque as per theoretical models |
| S | Shaft power or torque |
| S-T | Shaft power or torque as per theoretical models |
| T | Hydraulic turbine assembly |
References
- IRENA. Future of Wind: Deployment, Investment, Technology, Grid Integration and Socio-Economic Aspects; International Renewable Energy Agency: Abu Dhabi, United Arab Emirates, 2019. [Google Scholar]
- IRENA. Future of Solar Photovoltaic: Deployment, Investment, Technology, Grid Integration and Socio-Economic; International Renewable Energy Agency: Abu Dhabi, United Arab Emirates, 2019. [Google Scholar]
- Ellabban, O.; Abu-Rub, H.; Blaabjerg, F. Renewable energy resources: Current status, future prospects and their enabling technology. Renew. Sustain. Energy Rev. 2014, 39, 748–764. [Google Scholar] [CrossRef]
- European Commission. Available online: https://energy.ec.europa.eu/topics/renewable-energy/solar-energy_en (accessed on 24 March 2024).
- SolarPower Europe. Available online: https://www.solarpowereurope.org/press-releases/new-report-eu-solar-reaches-record-heights-of-56-gw-in-2023-but-warns-of-clouds-on-the-horizon (accessed on 24 March 2024).
- European Commission. Available online: https://energy.ec.europa.eu/topics/renewable-energy/eu-wind-energy_en (accessed on 24 March 2024).
- WindEurope. Available online: https://windeurope.org/newsroom/press-releases/the-eu-built-a-record-17-gw-of-new-wind-energy-in-2023-wind-now-19-percent-of-electricity-production/#:~:text=The%20IEA%20estimates%20that%20Europe,Europe’s%20wind%20energy%20supply%20chain (accessed on 24 March 2024).
- Jenniches, S. Assessing the regional economic impacts of renewable energy sources—A literature review. Renew. Sustain. Energy Rev. 2018, 93, 35–51. [Google Scholar] [CrossRef]
- Sinsel, S.R.; Riemke, R.L.; Hoffmann, V.H. Challenges and solution technologies for the integration of variable renewable energy sources—A review. Renew. Energy 2020, 145, 2271–2285. [Google Scholar] [CrossRef]
- Maciejowska, K. Assessing the impact of renewable energy sources on the electricity price level and variability—A quantile regression approach. Energy Econ. 2020, 85, 104532. [Google Scholar] [CrossRef]
- IRENA. Electricity Storage and Renewables: Costs and Markets to 2030; International Renewable Energy Agency: Abu Dhabi, United Arab Emirates, 2017. [Google Scholar]
- Amrouche, S.O.; Rekioua, D.; Rekioua, T.; Bacha, S. Overview of energy storage in renewable energy systems. Int. J. Hydrog. Energy 2016, 41, 20914–20927. [Google Scholar] [CrossRef]
- Ibrahim, H.; Ilinca, A.; Perron, J. Energy storage systems—Characteristics and comparison. Renew. Sustain. Energy Rev. 2008, 12, 1221–1250. [Google Scholar] [CrossRef]
- Olabi, A.G. Renewable energy and energy storage systems. Energy 2017, 136, 1–6. [Google Scholar] [CrossRef]
- Buhagiar, D.; Sant, T.; Farrugia, R.N.; Aquilina, L.; Farrugia, D.; Strati, F.M. Small-scale Experimental Testing of a Novel Marine Floating Platform with Integrated Hydro-Pneumatic Energy Storage. J. Energy Storage 2019, 24, 100774. [Google Scholar] [CrossRef]
- European Commission. Horizon 2020 Multiple-use-of Space for Island Clean Autonomy. Available online: https://cordis.europa.eu/project/id/862252 (accessed on 24 March 2024).
- Wang, J.; Lu, K.; Ma, L.; Wang, J.; Dooner, M.; Miao, S.; Li, J.; Wang, D. Overview of Compressed Air Energy Storage and Technology Development. Energies 2017, 10, 991. [Google Scholar] [CrossRef]
- Wang, H.; Wang, L.; Wang, X.; Yao, E. A novel pumped hydro combined with compressed air energy storage system. Energies 2013, 6, 1554–1567. [Google Scholar] [CrossRef]
- Camargos, T.P.; Pottie, D.L.; Ferreira, R.A.; Maia, T.A.; Porto, M.P. Experimental study of a PH-CAES system: Proof of concept. Energy 2018, 165, 630–638. [Google Scholar] [CrossRef]
- Odukomaiya, A.; Abu-Heiba, A.; Graham, S.; Momen, A.M. Experimental and analytical evaluation of a hydro-pneumatic compressed-air Ground-Level Integrated Diverse Energy Storage (GLIDES) system. Appl. Energy 2018, 221, 75–85. [Google Scholar] [CrossRef]
- White, F.M. Fluid Mechanics; McGraw-Hill: New York, NY, USA, 2011. [Google Scholar]
- Zardin, B.; Cillo, G.; Rinaldini, C.A.; Mattarelli, E.; Borghi, M. Pressure Losses in Hydraulic Manifolds. Energies 2017, 10, 310. [Google Scholar] [CrossRef]
- Moujaes, S.F.; Deshmukh, S. Three-Dimensional CFD Predications and Experimental Comparison of Pressure Drop of Some Common Pipe Fittings in Turbulent Flow. J. Energy Eng. 2006, 132, 61–66. [Google Scholar] [CrossRef]
- Stivala, D.; Rossi, M.; Renzi, M. Velocity profiles in a water jet of a Pelton nozzle: CFD simulations on both 2D and 3D geometries. IOP Conference Series. Earth Environ. Sci. 2021, 774, 12106. [Google Scholar]
- Zhang, J.; Xiao, Y.X.; Wang, J.Q.; Zhou, X.J.; Xia, M.; Zeng, C.J.; Wang, S.H.; Wang, S.W. Optimal design of a pelton turbine nozzle via 3D numerical simulation. IOP Conference Series. Earth Environ. Sci. 2018, 163, 12066. [Google Scholar]
- Aquilina, L.; Sant, T.; Farrugia, R.N. An experimental analysis for the charging cycle of a hydro-pneumatic energy storage system. In Proceedings of the 7th Offshore Energy and Storage Symposium (OSES 2023), St. Julians, Malta, 12–14 July 2023. [Google Scholar]
- Gupta, V.; Prasad, V.; Khare, R. Numerical simulation of six jet Pelton turbine model. Energy 2016, 104, 24–32. [Google Scholar] [CrossRef]
- Mathworks. Simulink. Available online: https://uk.mathworks.com/products/simulink.html (accessed on 24 March 2024).
- Neutrium. Absolulte Roughness of Pipe Materials. Available online: https://neutrium.net/fluid-flow/absolute-roughness-of-pipe-material/ (accessed on 24 March 2024).
- Burt, M.; Piao, X.; Gaudi, F.; Busch, B.; Taufik, N.F. Electric Motor Efficiency under Variable Frequencies and Loads. J. Irrig. Drain. Eng. 2008, 134, 129–136. [Google Scholar] [CrossRef]









| Sensor | Parameter | Sensor Accuracy |
|---|---|---|
| Pressure sensor: accumulator | ±0.30 bar | |
| Pressure sensor: primary hydraulic manifold | ±0.12 bar | |
| Pressure sensor: hydraulic turbine nozzle inlet | ±0.12 bar | |
| Flow meter | ±1.35 L/min | |
| Turbine drive RPM output | ±0.15 RPM | |
| Turbine drive shaft torque output | ±2.9 Nm |
| Control System/ Component | Description |
|---|---|
| Turbine electrical drive with operational RPM settings | Controls the speed at which the electrical drive operates the hydraulic turbine generator. This is applicable for both motoring and generation modes. Operational RPM was kept constant throughout particular cycles. |
| Hydraulic turbine nozzle spear position | Controls the diameter of the water jet at the turbine nozzle outlet. Position was kept constant throughout a particular cycle. |
| Primary hydraulic manifold turbine ball valve | Controls flow into turbine from accumulator. The ball valve was set to either a full closed or fully open position. |
| Hydraulic Turbine Operational RPM settings, RPM | |
| Setting | Code |
| 1750 | S1750 |
| 1500 | S1500 |
| 1250 | S1250 |
| 1000 | S1000 |
| 750 | S750 |
| 500 | S500 |
| Hydraulic Turbine Nozzle Settings | |
| Setting | Code |
| 100% open (fully open) | N100 |
| 80% open | N80 |
| 60% open | N60 |
| 40% open | N40 |
| 20% open | N20 |
| Component | Pressure Drop Coefficient | Mesh Elements |
|---|---|---|
| 90° elbow | 1.35 | 1,892,895 |
| Primary manifold | 1.719 | 2,343,069 |
| Secondary manifold | 0.508 | 2,059,028 |
| Nozzle Spear Position | (mm2) | Mesh Elements | |
|---|---|---|---|
| 100% open | 0.993 | 30.80 | 3,252,649 |
| 80% open | 0.991 | 29.45 | 3,242,369 |
| 60% open | 0.988 | 26.40 | 3,197,480 |
| 40% open | 0.984 | 20.77 | 3,395,604 |
| 20% open | 0.967 | 12.10 | 3,366,519 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aquilina, L.; Sant, T.; Farrugia, R.N.; Licari, J.; Spiteri Staines, C.; Buhagiar, D. Measurements and Modelling of the Discharge Cycle of a Grid-Connected Hydro-Pneumatic Energy Storage System. Energies 2024, 17, 1597. https://doi.org/10.3390/en17071597
Aquilina L, Sant T, Farrugia RN, Licari J, Spiteri Staines C, Buhagiar D. Measurements and Modelling of the Discharge Cycle of a Grid-Connected Hydro-Pneumatic Energy Storage System. Energies. 2024; 17(7):1597. https://doi.org/10.3390/en17071597
Chicago/Turabian StyleAquilina, Luke, Tonio Sant, Robert N. Farrugia, John Licari, Cyril Spiteri Staines, and Daniel Buhagiar. 2024. "Measurements and Modelling of the Discharge Cycle of a Grid-Connected Hydro-Pneumatic Energy Storage System" Energies 17, no. 7: 1597. https://doi.org/10.3390/en17071597
APA StyleAquilina, L., Sant, T., Farrugia, R. N., Licari, J., Spiteri Staines, C., & Buhagiar, D. (2024). Measurements and Modelling of the Discharge Cycle of a Grid-Connected Hydro-Pneumatic Energy Storage System. Energies, 17(7), 1597. https://doi.org/10.3390/en17071597










