Systematic Review of Bidirectional, Multiport Converter Structures and Their Derivatives: A Case Study of Bidirectional Dual Input Dual Output Converters
Abstract
1. Introduction
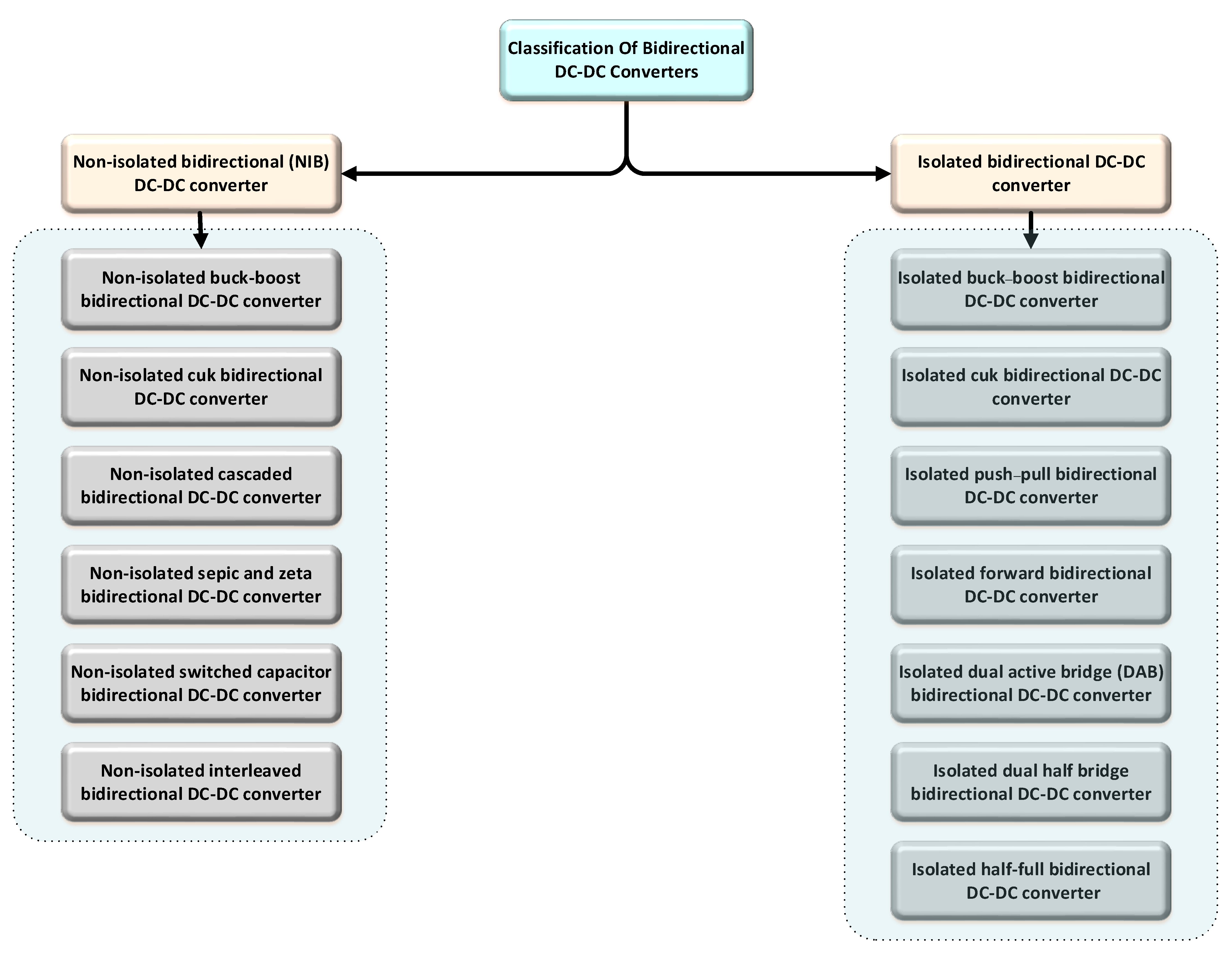
2. Classification of Bidirectional DC-DC Converters
2.1. Non-Isolated Bidirectional (NIB) DC-to-DC Converter
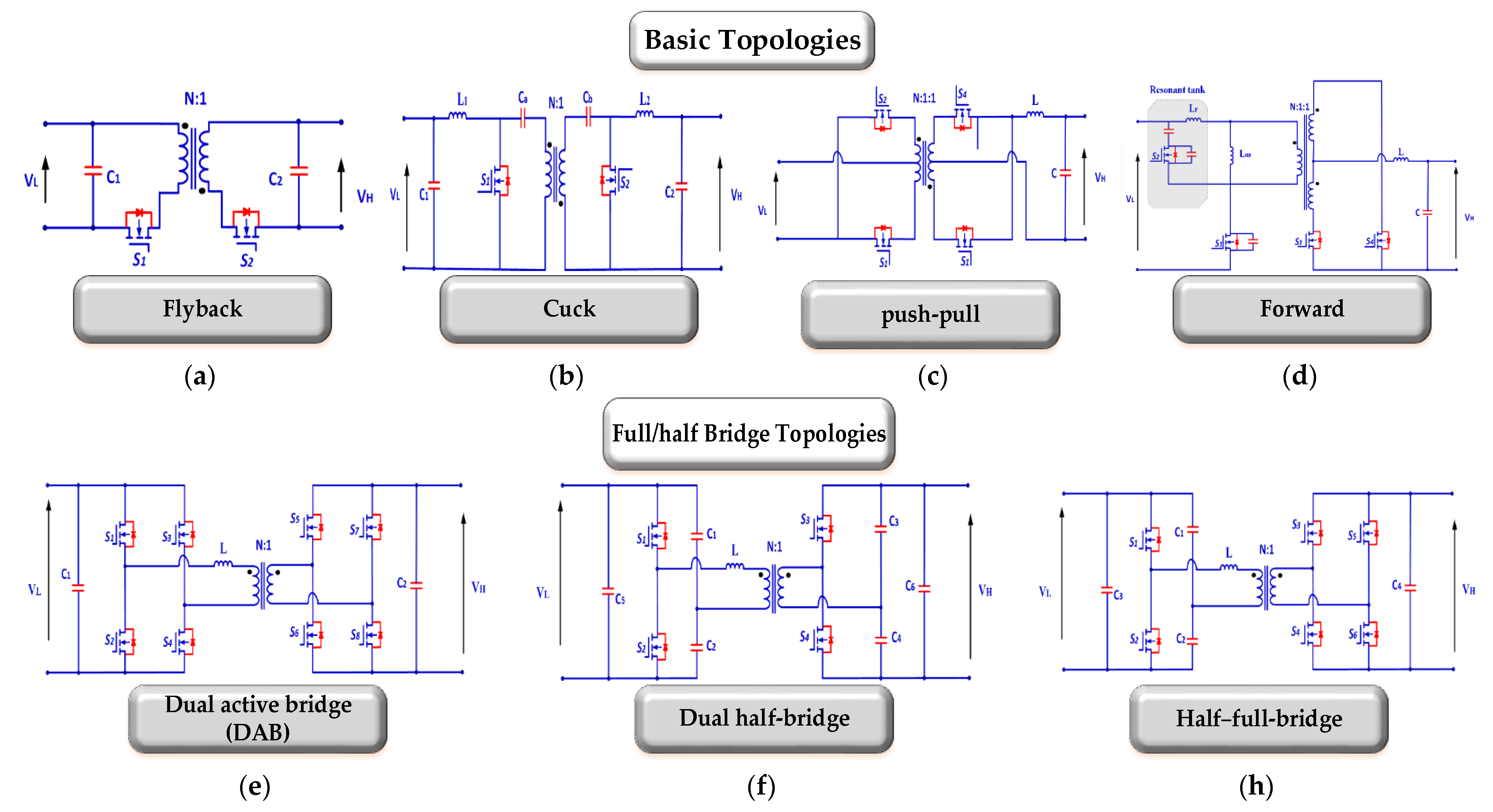
2.2. Isolated Bidirectional (IB) DC-DC Converter
3. Bidirectional Improvements towards Bidirectional Multiport Converters
- Multiport converters can handle multiple energy sources and loads simultaneously. This is particularly beneficial in systems like renewable energy, where solar, wind, and other energy sources might need to be integrated.
- These converters offer more flexibility in managing power flow between different ports, which can lead to increased overall system efficiency. They can optimally distribute power based on the demand and availability of each source.
- By integrating multiple functions into a single converter, the complexity and cost of the overall system can be reduced. This integration eliminates the need for multiple separate converters and the associated interconnections.
- Multiport converters can provide higher reliability and redundancy. If one energy source fails, the system can continue using other sources without interruption.
- These converters can implement advanced control strategies for power management, which are crucial in systems like microgrids and hybrid electric vehicles.
- With the increasing use of distributed energy resources and the need for smart grid technologies, multiport converters align well with these modern requirements, offering better integration and control capabilities.
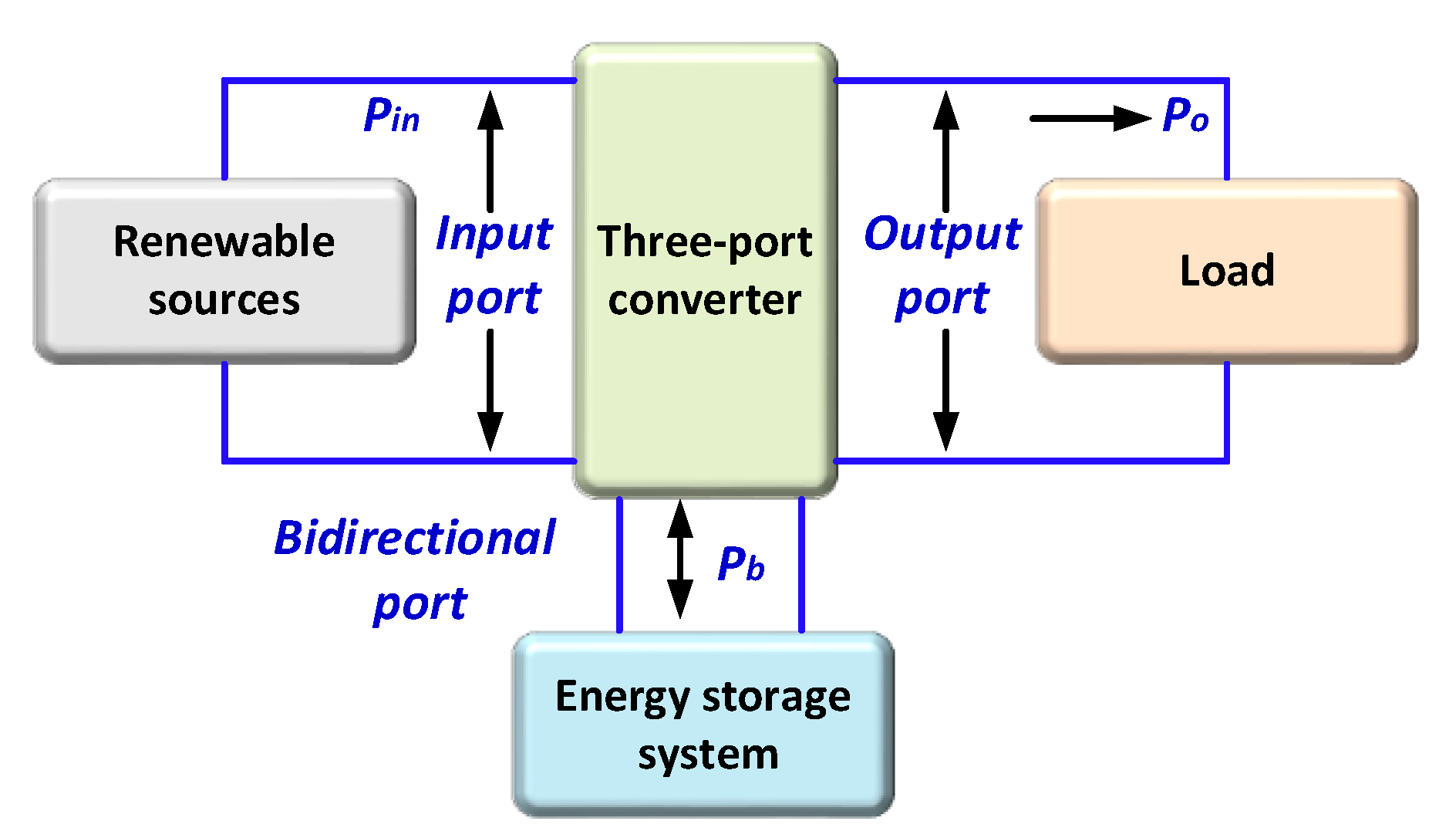
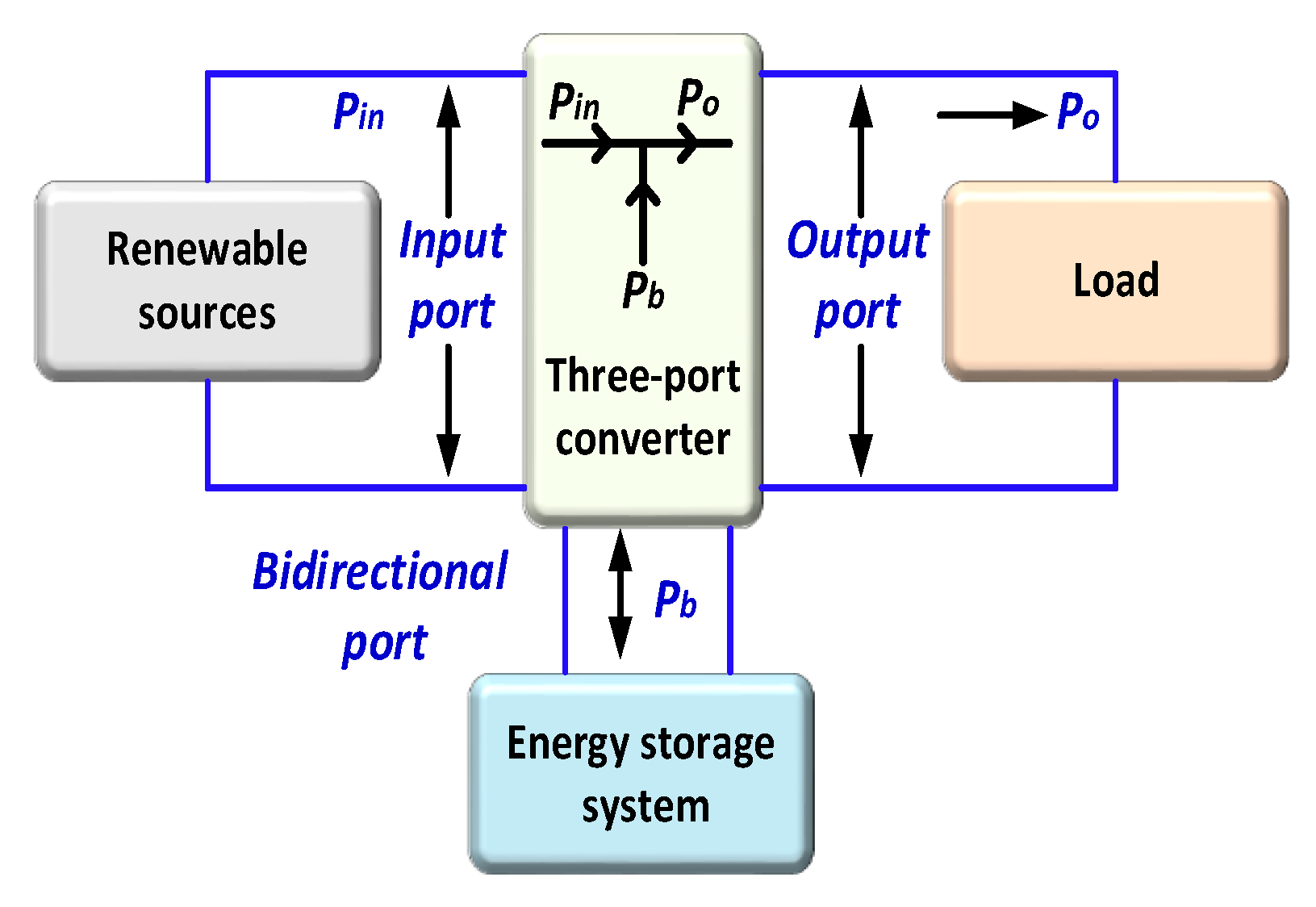
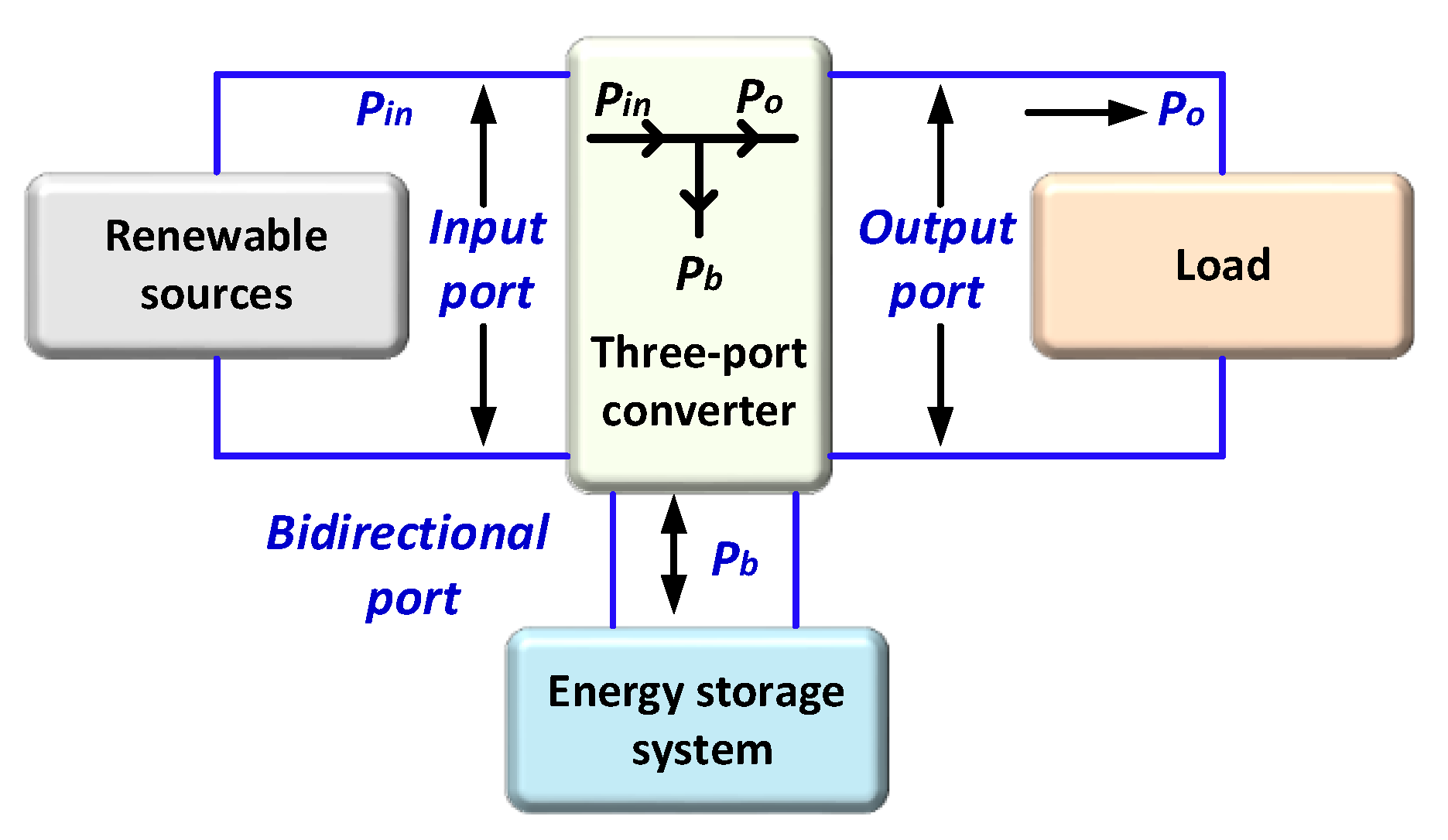
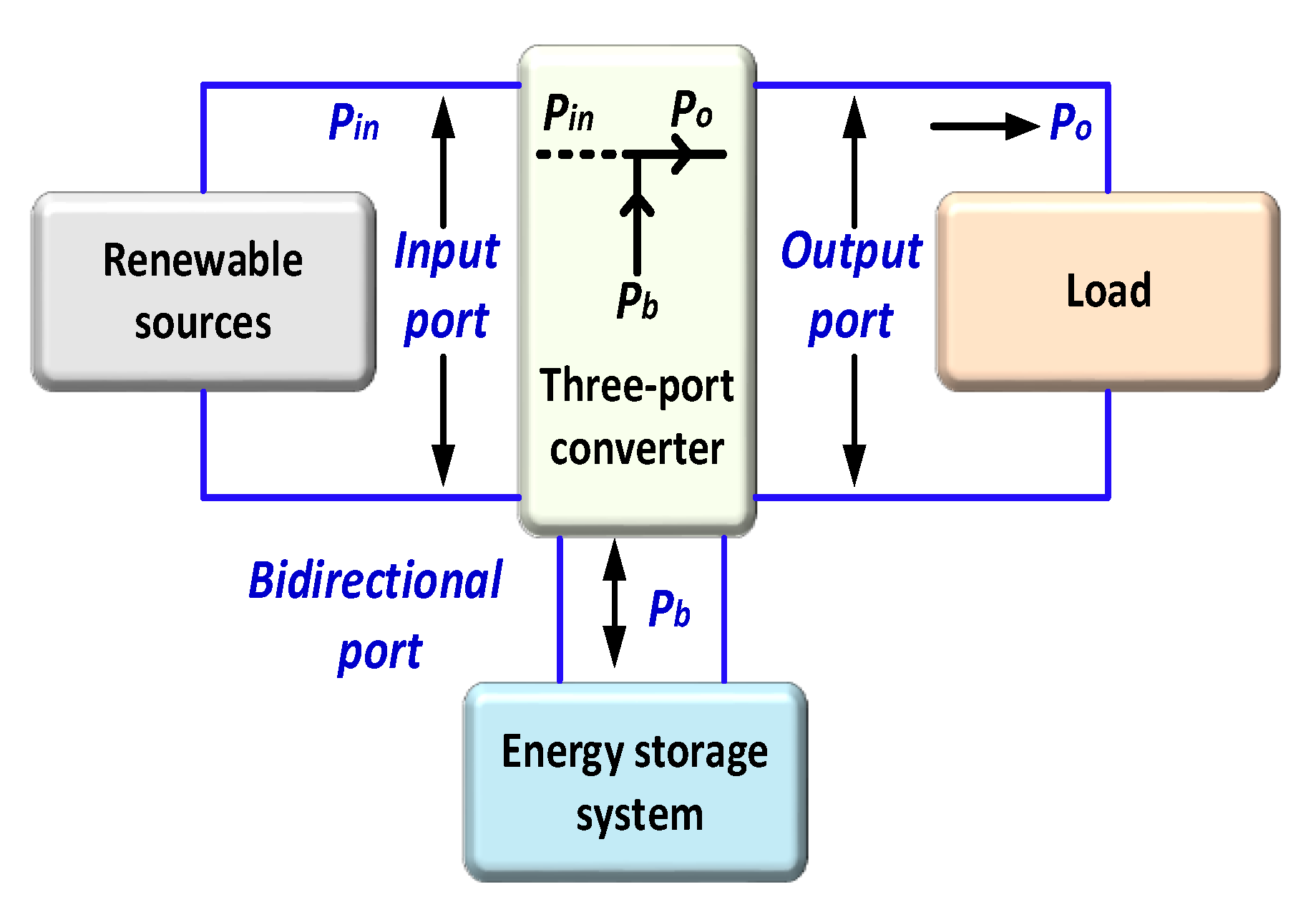
4. Multiport DC-DC Converters
4.1. The Fundamental Principles of Operation for a Three-Port DC-DC Converter
4.2. Non-Isolated Three-Port DC-DC Converters
4.2.1. Dual-Input–Single-Output DC-DC Converters (DISO)
4.2.2. Single-Input–Dual-Output DC-DC Converters (SIDO)
4.2.3. Single-Input–Single-Output Mode (SISO)
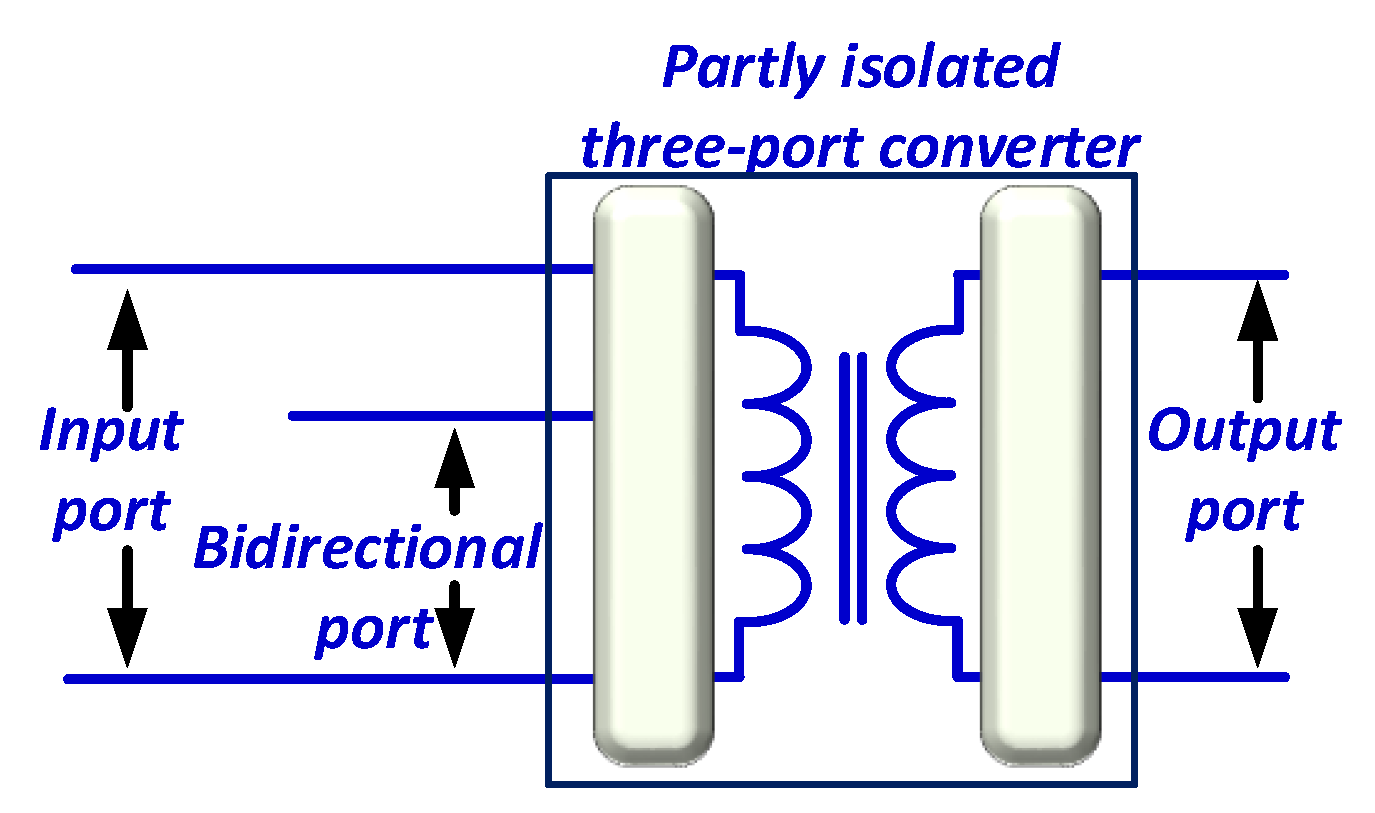
4.3. Partly Isolated Three-Port DC-DC Converters
4.4. Fully Isolated Three-Port DC-DC Converters
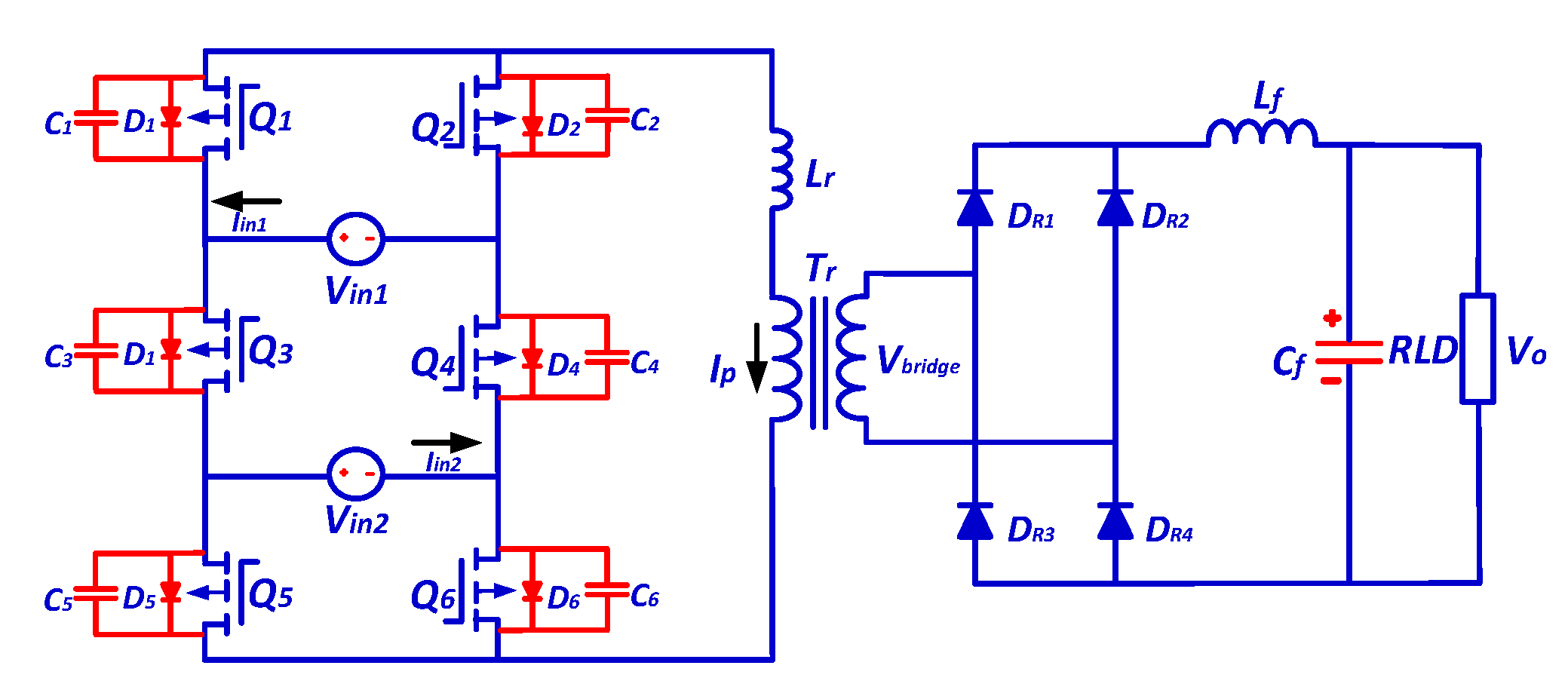
4.4.1. Multi-Input Total Bridge Converter (FBC)
- The primary and secondary turns of the transformer;
- : The first input voltage supply; : the voltage across the inductor;
- : The percentage of phase shift for Input Source 1; : The output voltage;
- : The switching time.
- The relationship between the first input source and output voltage and the second input source and output voltage can be given as follows:
4.4.2. Multi-Input Half-Bridge DC-DC Converters (HBCs)
4.4.3. Comparison of Three-Port Converters
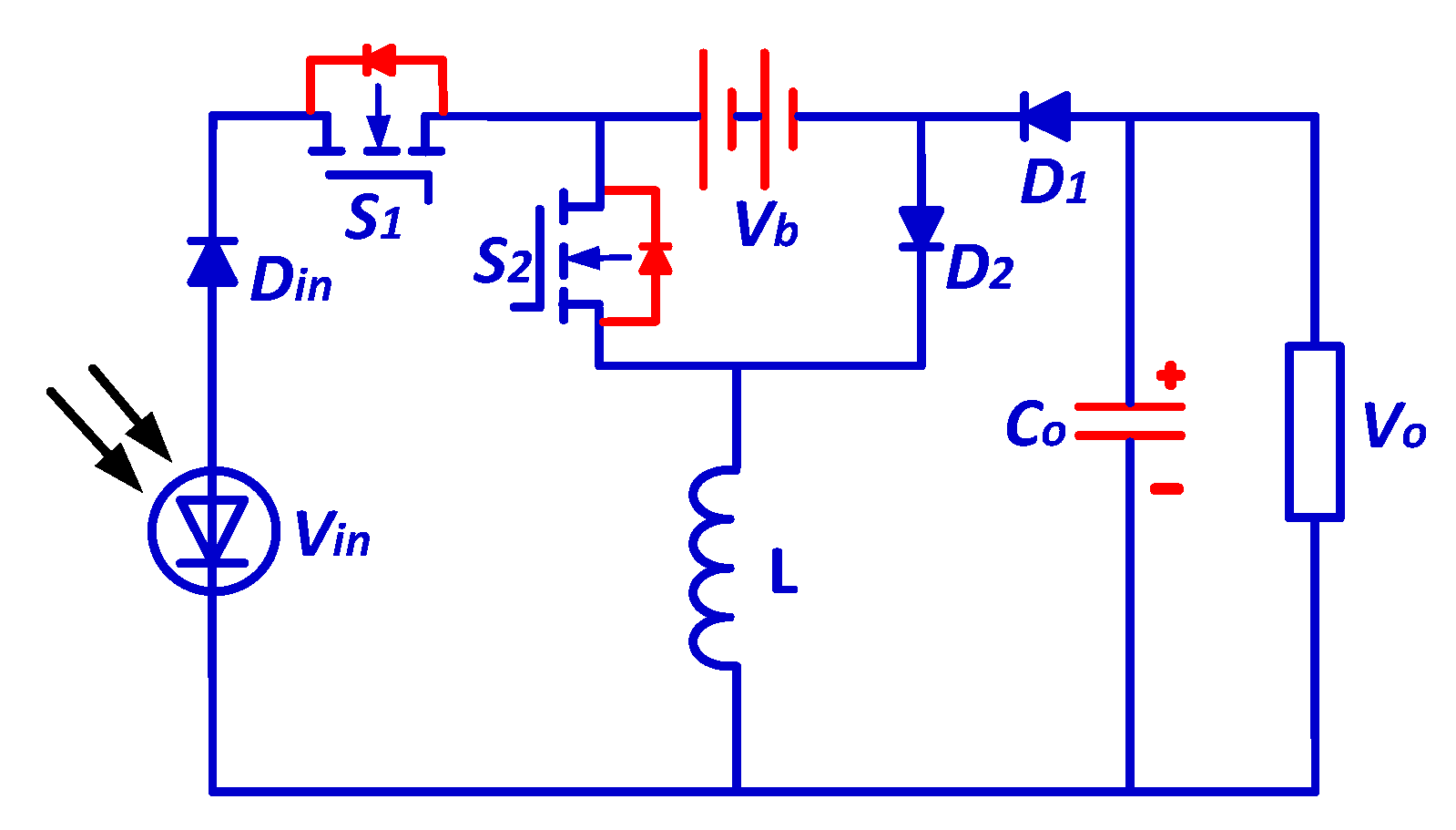
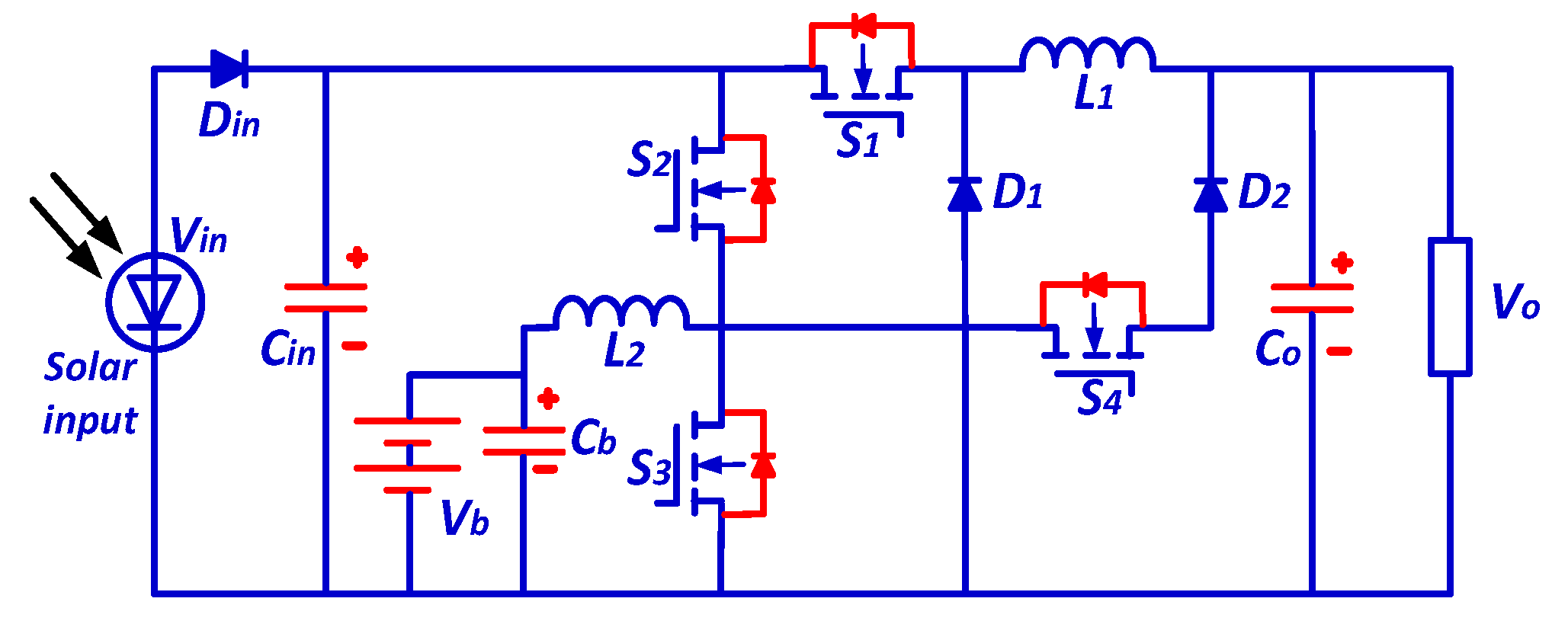
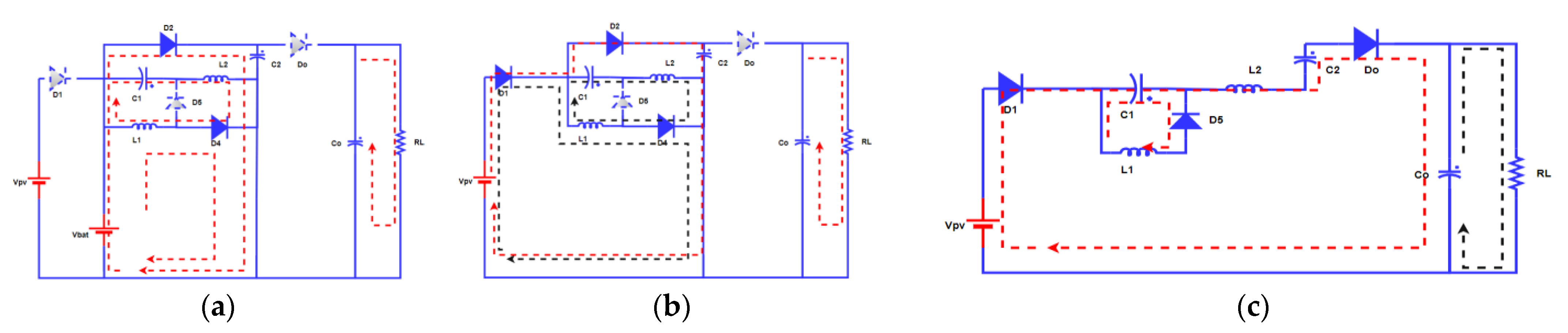
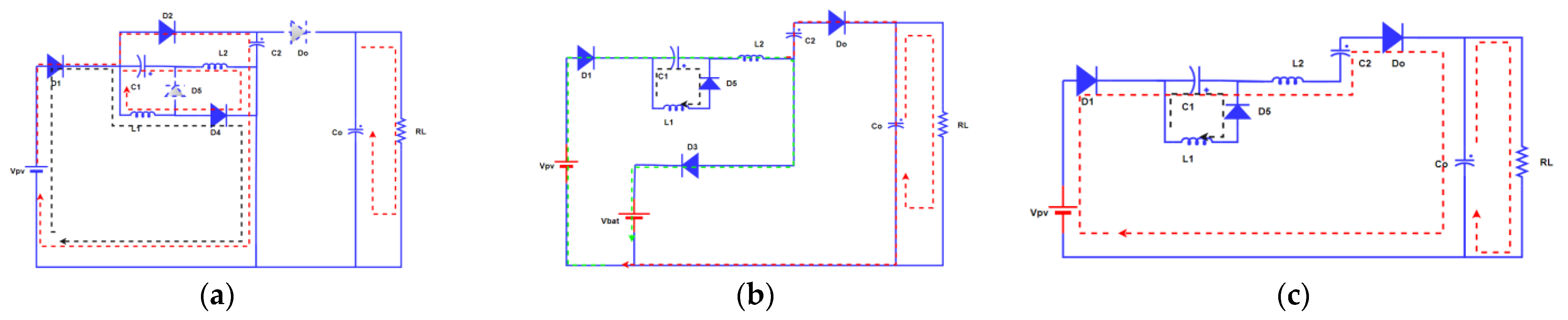
5. Proposed Three-Port DC-DC Converter
5.1. Dual Input
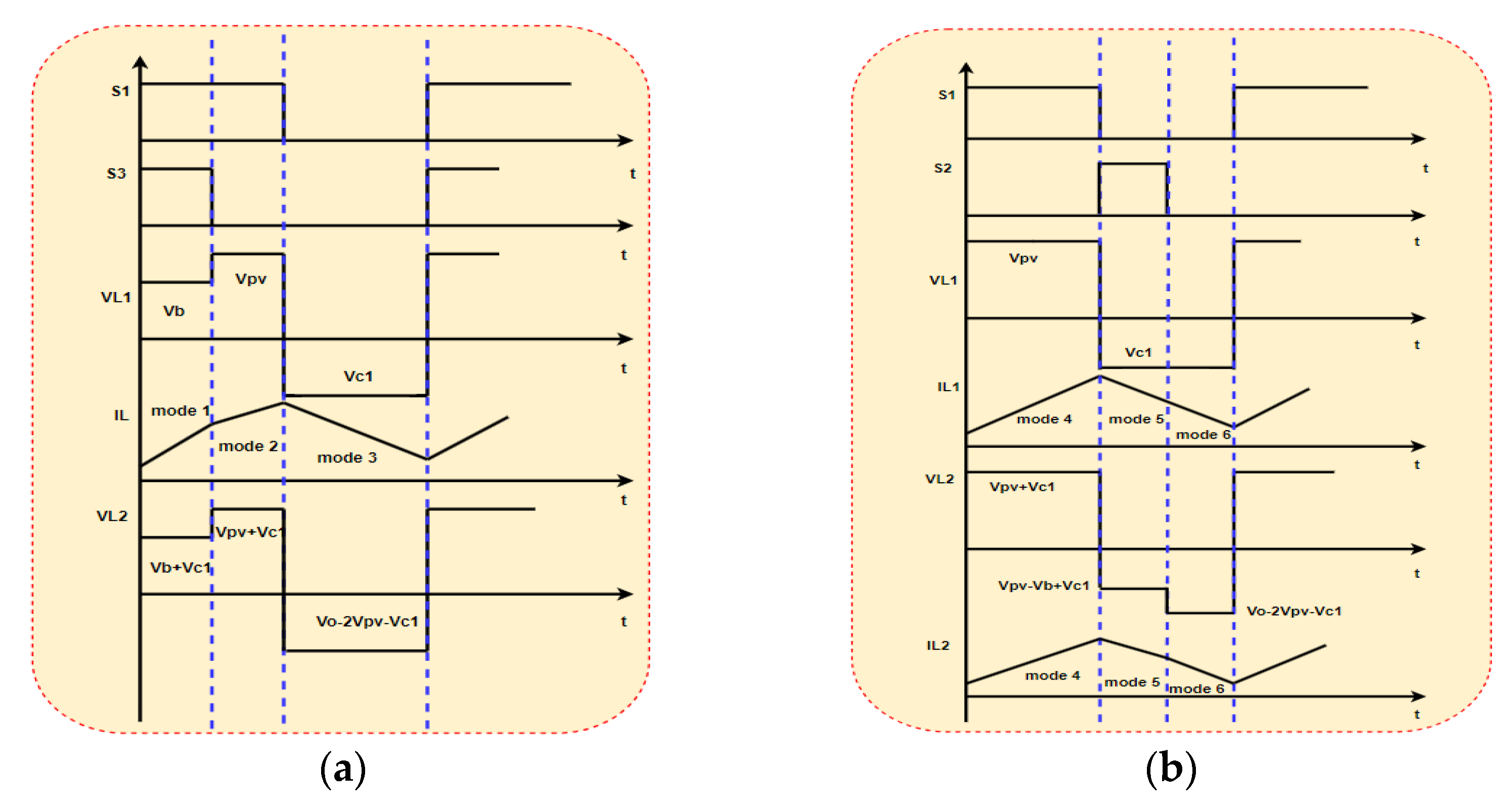
5.1.1. Mode 1
5.1.2. Mode 2
5.1.3. Mode 3
5.2. Dual Output
5.2.1. Mode 4
5.2.2. Mode 5
5.2.3. Mode 6
5.3. Current Stress Equations
5.4. Voltage Stress Equations
5.5. Design of Passive Elements
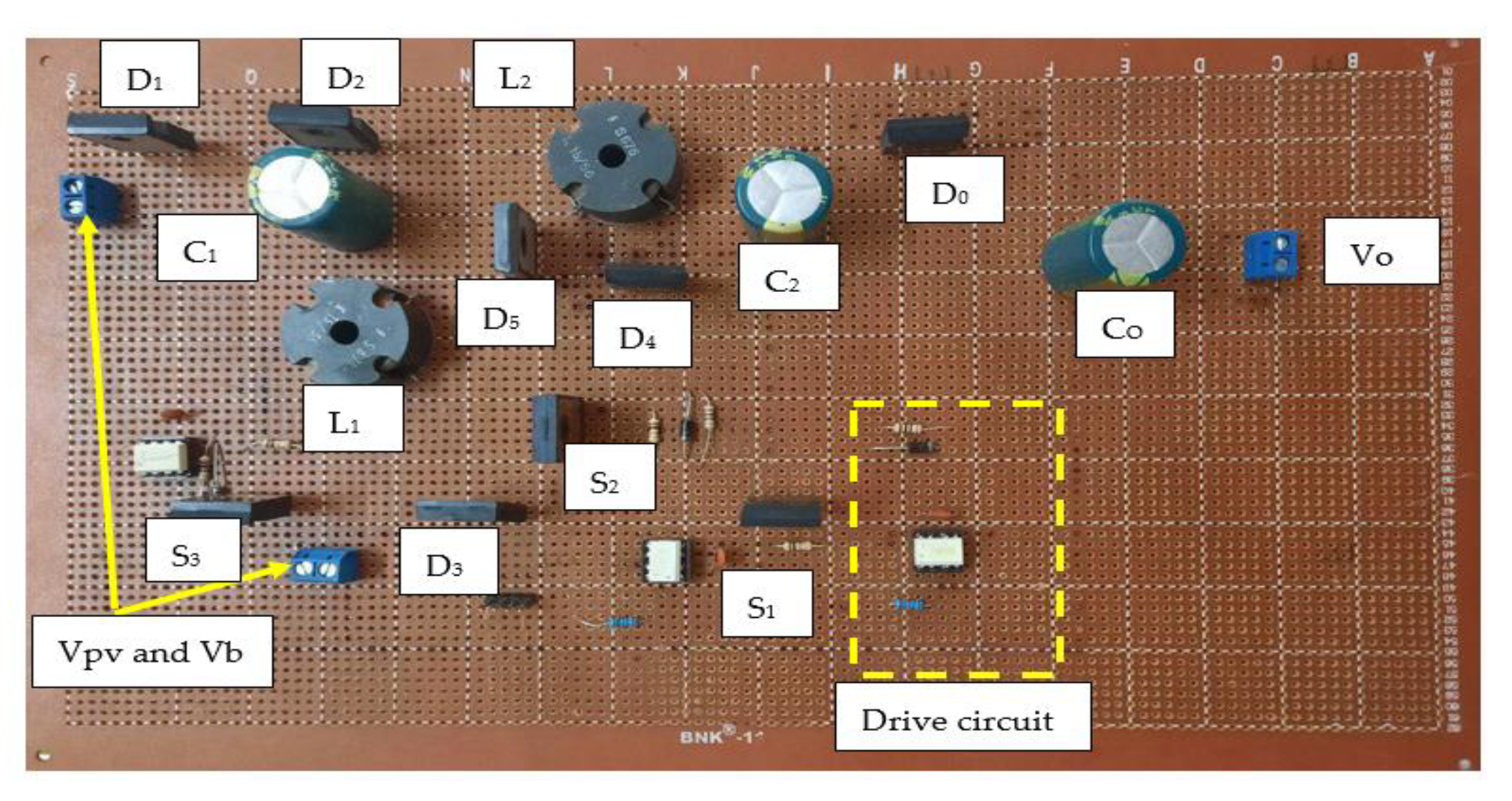
5.6. Simulation and Experimental Results
5.7. Comparative Study
6. Discussion and Future Recommendations
- Power flow management among an electric vehicle’s battery, photovoltaic system, and fuel cell can be achieved with the help of advanced control algorithms. Adaptive responses to fluctuating energy demands and storage capacities are part of this process, which also involves real-time monitoring.
- Optimal energy harvesting from PV systems, efficient energy storage in EVs, and effective use of fuel cells as a backup or supplementary power source are all areas that could benefit from better energy management.
- Emphasize a smooth integration of these systems, focusing on electric vehicles, PV modules, and fuel cells so that the converters can efficiently balance the load and source dynamics.
- Improved interoperability: Considering different protocols and standards, work on making multiport converters more compatible with electric vehicles, PV systems, and fuel cell technologies of all kinds.
- Get a feel for how these technologies will affect the environment through thorough life-cycle and sustainability analyses; the goal should be to find environmentally friendly and long-lasting solutions.
- Examining the potential interactions between these interconnected systems and the grid to enhance grid stability is an essential area of research, particularly in scenarios with a high penetration of renewable energy sources.
- Investigate novel converter topologies that can improve system performance by effectively managing the power demands and properties of electric vehicles, PV systems, and fuel cells.
- Dependability and longevity: Highlight the dependability and longevity of converters in a range of operating conditions, such as harsh weather, heavy usage, and extended operation.
- Analyze the market potential, scalability, and cost–benefit analysis of integrating these technologies to drive commercial adoption and policy support. Also, consider the economic viability of the project.
- The development and influence of standardization and regulatory frameworks is an important area to focus on to ensure these technologies’ smooth and efficient integration.
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Gorji, S.A.; Sahebi, H.G.; Ektesabi, M.; Rad, A.B. Topologies and Control Schemes of Bidirectional Dc–Dc Power Converters: An Overview. IEEE Access 2019, 7, 117997–118019. [Google Scholar] [CrossRef]
- Mughis, M.A.; Sudiharto, I.; Ferdiansyah, I.; Yanaratri, D.S. Design and Implementation of Partial M-Type Zero Voltage Resonant Circuit Interleaved Bidirectional Dc–Dc Converter (Energy Storage and Load Sharing). In Proceedings of the 2018 International Electronics Symposium on Engineering Technology and Applications (IES-ETA), Bali Island, Indonesia, 29–30 October 2018. [Google Scholar]
- Forouzesh, M.; Siwakoti, Y.P.; Gorji, S.A.; Blaabjerg, F.; Lehman, B. Step-up Dc–Dc Converters: A Comprehensive Review of Voltage-Boosting Techniques, Topologies, and Applications. IEEE Trans. Power Electron. 2017, 32, 9143–9178. [Google Scholar] [CrossRef]
- Zeng, Y.; Li, H.; Zhang, Z.; Zheng, T.Q.; Shang, Z.; Qiu, Z.; Yuan, L.; Ding, Y. A Parallel-Resonant Isolated Bidirectional Dc-Dc Converter with Low Current Ripple for Battery Storage Systems. In Proceedings of the 2019 IEEE Energy Conversion Congress and Exposition (ECCE), Baltimore, MD, USA, 29 September–3 October 2019. [Google Scholar]
- Ma, X.; Wang, P.; Bi, H.; Wang, Z. A Bidirectional Llcl Resonant Dc-Dc Converter with Reduced Resonant Tank Currents and Reduced Voltage Stress of the Resonant Capacitor. IEEE Access 2020, 8, 125549–125564. [Google Scholar] [CrossRef]
- Li, Y.; Wang, Y.; Song, H. Bidirectional Dc-Dc Converter Based on Clc Network. In Proceedings of the 2019 22nd International Conference on Electrical Machines and Systems (ICEMS), Harbin, China, 11–14 August 2019. [Google Scholar]
- Alatai, S.; Salem, M.; Ishak, D.; Das, H.S.; Alhuyi Nazari, M.; Bughneda, A.; Kamarol, M. A Review on State-of-the-Art Power Converters: Bidirectional, Resonant, Multilevel Converters and Their Derivatives. Appl. Sci. 2021, 11, 10172. [Google Scholar] [CrossRef]
- Ravi, D.; Reddy, B.M.; Shimi, S.; Samuel, P. Bidirectional Dc to Dc Converters: An Overview of Various Topologies, Switching Schemes and Control Techniques. Int. J. Eng. Technol. 2018, 7, 360–365. [Google Scholar] [CrossRef]
- Yanna, V.S.R.; Bhajana, V.S.K.; Drabek, P.; Popuri, M. A Novel Soft-Switching Bidirectional Dc-Dc Converter for Energy Storage Applications. In Proceedings of the 2020 International Conference on Applied Electronics (AE), Pilsen, Czech Republic, 8–9 September 2020. [Google Scholar]
- Wang, H.; Xu, Z.; Ge, X.; Liao, Y.; Yang, Y.; Zhang, Y.; Yao, B.; Chai, Y. A Junction Temperature Monitoring Method for Igbt Modules Based on Turn-Off Voltage with Convolutional Neural Networks. IEEE Trans. Power Electron. 2023, 38, 10313–10328. [Google Scholar] [CrossRef]
- Salem, M.; Jusoh, A.; Idris, N.R.N. Implementing Buck Converter for Battery Charger Using Soft Switching Techniques. In Proceedings of the 2013 IEEE 7th International Power Engineering and Optimization Conference (PEOCO), Langkawi Island, Malaysia, 3–4 June 2013. [Google Scholar]
- Xiao, S.; Wang, Z.; Wu, G.; Guo, Y.; Gao, G.; Zhang, X.; Cao, Y.; Zhang, Y.; Yu, J.; Liu, P. The Impact Analysis of Operational Overvoltage on Traction Transformers for High-Speed Trains Based on the Improved Capacitor Network Methodology. IEEE Trans. Transp. Electrif. 2023. [Google Scholar] [CrossRef]
- Schupbach, R.M.; Balda, J.C. Comparing Dc-Dc Converters for Power Management in Hybrid Electric Vehicles. In Proceedings of the IEEE International Electric Machines and Drives Conference, IEMDC’03, Madison, WI, USA, 1–4 June 2003. [Google Scholar]
- Cuk, S. A New Zero-Ripple Switching Dc-to-Dc Converter and Integrated Magnetics. IEEE Trans. Magn. 1983, 19, 57–75. [Google Scholar] [CrossRef]
- Zhou, S.; Zhou, G.; He, M.; Mao, S.; Zhao, H.; Liu, G. Stability Effect of Different Modulation Parameters in Voltage-Mode Pwm Control for Ccm Switching Dc-Dc Converter. IEEE Trans. Transp. Electrif. 2023. [Google Scholar] [CrossRef]
- Waffler, S.; Kolar, J.W. A Novel Low-Loss Modulation Strategy for High-Power Bidirectional Buck+Boost Converters. IEEE Trans. Power Electron. 2009, 24, 1589–1599. [Google Scholar] [CrossRef]
- Waffler, S.; Biela, J.; Kolar, J.W. Output Ripple Reduction of an Automotive Multi-Phase Bi-Directional Dc-Dc Converter. In Proceedings of the 2009 IEEE Energy Conversion Congress and Exposition, San Jose, CA, USA, 20–24 September 2009. [Google Scholar]
- Shahin, M. Analysis of Bidirectional Sepic/Zeta Converter with Coupled Inductor. In Proceedings of the 2015 International Conference on Technological Advancements in Power and Energy (TAP Energy), Kollam, India, 24–26 June 2015. [Google Scholar]
- Kim, I.-D.; Lee, Y.-H.; Min, B.-H.; Nho, E.-C.; Ahn, J.-W. Design of Bidirectional Pwm Sepic/Zeta Dc-Dc Converter. In Proceedings of the 2007 7th Internatonal Conference on Power Electronics, Daegu, South Korea, 22–26 October 2007. [Google Scholar]
- Zhou, S.; Zhou, G.; Liu, X.; Zhao, H. Dynamic Freewheeling Control for Sido Buck Converter with Fast Transient Performance, Minimized Cross-Regulation, and High Efficiency. IEEE Trans. Ind. Electron. 2022, 70, 1467–1477. [Google Scholar] [CrossRef]
- Liu, S.; Liu, C. Virtual-Vector-Based Robust Predictive Current Control for Dual Three-Phase Pmsm. IEEE Trans. Ind. Electron. 2020, 68, 2048–2058. [Google Scholar] [CrossRef]
- Alatai, S.; Salem, M.; Ishak, D.; Kamarol, M.; Jamil, M.; Bughneda, A. Design and Analysis of Five-Level Cascaded Llc Resonant Converter. In Proceedings of the 2020 IEEE International Conference on Power and Energy (PECon), Penang, Malaysia, 7–8 December 2020. [Google Scholar]
- Salem, M.; Jusoh, A.; Idris, N.R.N.; Tan, C.W.; Alhamrouni, I. Phase-Shifted Series Resonant Dc-Dc Converter for Wide Load Variations Using Variable Frequency Control. In Proceedings of the 2017 IEEE Conference on Energy Conversion (CENCON), Kuala Lumpur, Malaysia, 30–31 October 2017. [Google Scholar]
- Chung, H.S.-H.; Ioinovici, A.; Cheung, W.-L. Generalized Structure of Bi-Directional Switched-Capacitor Dc/Dc Converters. IEEE Trans. Circuits Syst. I Fundam. Theory Appl. 2003, 50, 743–753. [Google Scholar] [CrossRef]
- Li, J.; Sullivan, C.R.; Schultz, A. Coupled-Inductor Design Optimization for Fast-Response Low-Voltage Dc-Dc Converters. In Proceedings of the APEC. Seventeenth Annual IEEE Applied Power Electronics Conference and Exposition (Cat. No. 02CH37335), Dallas, TX, USA, 10–14 March 2002. [Google Scholar]
- Wong, P.-L.; Wu, Q.; Xu, P.; Yang, B.; Lee, F.C. Investigating Coupling Inductors in the Interleaving Qsw Vrm. In Proceedings of the APEC 2000. Fifteenth Annual IEEE Applied Power Electronics Conference and Exposition (Cat. No. 00CH37058), New Orleans, LA, USA, 6–10 February 2000. [Google Scholar]
- Ni, L.; Patterson, D.J.; Hudgins, J.L. High Power Current Sensorless Bidirectional 16-Phase Interleaved Dc-Dc Converter for Hybrid Vehicle Application. IEEE Trans. Power Electron. 2011, 27, 1141–1151. [Google Scholar] [CrossRef]
- Garcia, O.; Zumel, P.; De Castro, A.; Cobos, A. Automotive Dc-Dc Bidirectional Converter Made with Many Interleaved Buck Stages. IEEE Trans. Power Electron. 2006, 21, 578–586. [Google Scholar] [CrossRef]
- Garinto, D. Multi-Interleaved Zero-Ripple Vrm to Power Future Microprocessors. In Proceedings of the 2007 European Conference on Power Electronics and Applications, Aalborg, Denmark, 2–5 September 2007. [Google Scholar]
- Xu, P.; Lan, D.; Wang, F.; Shin, I.J.E. In-Memory Computing Integrated Structure Circuit Based on Nonvolatile Flash Memory Unit. Electronics 2023, 12, 3155. [Google Scholar] [CrossRef]
- Sunkara, S.; Kondrath, N. Design and Pspice Simulation of Synchronous Bidirectional Pwm Dc-Dc Buck-Boost Converter Operating in Ccm. In Proceedings of the 2013 Annual IEEE India Conference (INDICON), Mumbai, India, 13–15 December 2013. [Google Scholar]
- Kim, E.-S.; Kim, C.-J.; Kim, Y.-T. Development of Bidirectional Ac-Dc Converter for Energy Storage Systems. In Proceedings of the 2019 22nd International Conference on Electrical Machines and Systems (ICEMS), Harbin, China, 11–14 August 2019. [Google Scholar]
- Rahman, M.M.; Uddin, M.N.; Islam, M.K. Performance Enhancement of a Bi-Directional Dc-Dc Converter Using a Ćuk Converter for Electric Vehicle Applications. In Proceedings of the 2015 IEEE 28th Canadian Conference on Electrical and Computer Engineering (CCECE), Halifax, NS, Canada, 3–6 May 2015. [Google Scholar]
- Bhajana, V.S.K.; Drabek, P. A Novel Zcs Non-Isolated Bidirectional Buck-Boost Dc-Dc Converter for Energy Storage Applications. In Proceedings of the 2015 IEEE 24th International Symposium on Industrial Electronics (ISIE), Rio de Janeiro, Brazil, 3–5 June 2015. [Google Scholar]
- Lee, Y.-S.; Cheng, G.-T. Quasi-Resonant Zero-Current-Switching Bidirectional Converter for Battery Equalization Applications. IEEE Trans. Power Electron. 2006, 21, 1213–1224. [Google Scholar] [CrossRef]
- Osman, I.; Xiao, D.; Rahman, M. Analysis of a Cascaded High-Boost Non-Isolated Dc-Dc Converter with Bidirectional Power Flow. In Proceedings of the 2016 IEEE 2nd Annual Southern Power Electronics Conference (SPEC), Auckland, New Zealand, 5–8 December 2016. [Google Scholar]
- Vazquez, J.; Salmeron, P. Active Power Filter Control Using Neural Network Technologies. IEE Proc.-Electr. Power Appl. 2003, 150, 139–145. [Google Scholar] [CrossRef]
- Mu, Y.; Yang, X.; Xue, Y.; Lin, Z.; Zheng, T.Q.; Igarashi, S. A Bidirectional Switched-Capacitor Based Ac-Ac Resonant Converter. In Proceedings of the 2016 IEEE 11th Conference on Industrial Electronics and Applications (ICIEA), Hefei, China, 5–7 June 2016. [Google Scholar]
- Jangir, P.; Kumar, R. Closed-Loop Control Analysis of Interleaved Non-Isolated Bidirectional Dc-Dc Converter. In Proceedings of the 2017 IEEE International WIE Conference on Electrical and Computer Engineering (WIECON-ECE), Dehradun, India, 18–19 December 2017. [Google Scholar]
- Paul, P.; Jose, B.R.; Shahana, T.; Abraham, C.; Mathew, J. Isolated Switched Boost Dc-Dc Converter with Coupled Inductor and Transformer. In Proceedings of the TENCON 2019—2019 IEEE Region 10 Conference (TENCON), Kochi, India, 17–20 October 2019. [Google Scholar]
- Kheraluwala, M.; Gascoigne, R.W.; Divan, D.M.; Baumann, E.D. Performance Characterization of a High-Power Dual Active Bridge Dc-to-Dc Converter. IEEE Trans. Ind. Appl. 1992, 28, 1294–1301. [Google Scholar] [CrossRef]
- Sorrentino, N.; Barone, G.; Brusco, G.; Motta, M.; Menniti, D.; Pinnarelli, A.; Burgio, A. A Dual Active Bridge Dc-Dc Converter for Application in a Smart User Network. In Proceedings of the 2014 Australasian Universities Power Engineering Conference (AUPEC), Perth, Australia, 28 September–1 October 2014. [Google Scholar]
- Kumar, A.; Bhat, A.; Agarwal, P. Comparative Analysis of Dual Active Bridge Isolated Dc to Dc Converter with Flyback Converters for Bidirectional Energy Transfer. In Proceedings of the 2017 Recent Developments in Control, Automation & Power Engineering (RDCAPE), Noida, India, 26–27 October 2017. [Google Scholar]
- Kumar, B.M.; Kumar, A.; Bhat, A.; Agarwal, P. Comparative Study of Dual Active Bridge Isolated Dc to Dc Converter with Single Phase Shift and Dual Phase Shift Control Techniques. In Proceedings of the 2017 Recent Developments in Control, Automation & Power Engineering (RDCAPE), Noida, India, 26–27 October 2017. [Google Scholar]
- Peng, F.Z.; Li, H.; Su, G.-J.; Lawler, J.S. A New Zvs Bidirectional Dc-Dc Converter for Fuel Cell and Battery Application. IEEE Trans. Power Electron. 2004, 19, 54–65. [Google Scholar] [CrossRef]
- Jung, J.-H.; Kim, H.-S.; Ryu, M.-H.; Baek, J.-W. Design Methodology of Bidirectional Cllc Resonant Converter for High-Frequency Isolation of Dc Distribution Systems. IEEE Trans. Power Electron. 2012, 28, 1741–1755. [Google Scholar] [CrossRef]
- Alatai, S.; Salem, M.; Ishak, D.; Bughneda, A.; Kamarol, M.; Luta, D.N. Cascaded Multi-Level Inverter for Battery Charging-Discharging Using Buck-Boost Switch. In Proceedings of the 2021 IEEE Industrial Electronics and Applications Conference (IEACon), Penang, Malaysia, 22–23 November 2021. [Google Scholar]
- Yang, Y.; Zhang, Z.; Zhou, Y.; Wang, C.; Zhu, H. Design of a Simultaneous Information and Power Transfer System Based on a Modulating Feature of Magnetron. IEEE Trans. Microw. Theory Tech. 2022, 71, 907–915. [Google Scholar] [CrossRef]
- Zhang, F.; Yan, Y. Novel Forward-Flyback Hybrid Bidirectional Dc-Dc Converter. IEEE Trans. Power Electron. 2009, 56, 1578–1584. [Google Scholar]
- Kashif, M. Bidirectional Flyback Dc-Dc Converter for Hybrid Electric Vehicle: Utility, Working and Pspice Computer Model. In Proceedings of the 2012 Asia Pacific Conference on Postgraduate Research in Microelectronics and Electronics, Hyderabad, India, 5–7 December 2012. [Google Scholar]
- Wang, Y.; Xia, F.; Wang, Y.; Xiao, X. Harmonic Transfer Function Based Single-Input Single-Output Impedance Modeling of Lcchvdc Systems. J. Mod. Power Syst. Clean Energy 2023. [Google Scholar]
- Aboulnaga, A.; Emadi, A. Performance Evaluation of the Isolated Bidirectional Cuk Converter with Integrated Magnetics. In Proceedings of the 2004 IEEE 35th Annual Power Electronics Specialists Conference (IEEE Cat. No. 04CH37551), Aachen, Germany, 20–25 June 2004. [Google Scholar]
- Mohammadi, M.R.; Farzanehfard, H. A New Bidirectional Zvs-Pwm Cuk Converter with Active Clamp. In Proceedings of the 2011 19th Iranian Conference on Electrical Engineering, Tehran, Iran, 17–19 May 2011. [Google Scholar]
- Wang, Y.; Chen, P.; Yong, J.; Xu, W.; Xu, S.; Liu, K. A Comprehensive Investigation on the Selection of High-Pass Harmonic Filters. IEEE Trans. Power Deliv. 2022, 37, 4212–4226. [Google Scholar] [CrossRef]
- Xiangli, K.; Li, S.; Smedley, K. Analysis and Modelling of a Bidirectional Push-Pull Converter with Pwm Plus Phase-Shift Control. In Proceedings of the IECON 2016—42nd Annual Conference of the IEEE Industrial Electronics Society, Florence, Italy, 23–26 October 2016. [Google Scholar]
- Tao, H.; Duarte, J.L.; Hendrix, M.A. Three-Port Triple-Half-Bridge Bidirectional Converter with Zero-Voltage Switching. IEEE Trans. Power Electron. 2008, 23, 782–792. [Google Scholar]
- Lu, Y.-J.; Liang, T.-J.; Lin, C.-H.; Chen, K.-H. Design and Implementation of a Bidirectional Dc-Dc Forward/Flyback Converter with Leakage Energy Recycled. In Proceedings of the 2017 Asian Conference on Energy, Power and Transportation Electrification (ACEPT), Singapore, 24–26 October 2017. [Google Scholar]
- Shirkhani, M.; Tavoosi, J.; Danyali, S.; Sarvenoee, A.K.; Abdali, A.; Mohammadzadeh, A.; Zhang, C. A Review on Microgrid Decentralized Energy/Voltage Control Structures and Methods. Energy Rep. 2023, 10, 368–380. [Google Scholar] [CrossRef]
- Vieira, A.; Mazza, L.; Antunes, F.; Oliveira, D. Bidirectional Dual-Active-Bridge Dc-Dc Converter for Vehicle-to-Grid Applications in Dc Microgrids. In Proceedings of the 2018 Simposio Brasileiro de Sistemas Eletricos (SBSE), Niteroi, Brazil, 12–16 May 2018. [Google Scholar]
- Bhajana, V.S.K.; Reddy, S.R. A Novel Zvs-Zcs Bidirectional Dc-Dc Converter for Fuel Cell and Battery Application. In Proceedings of the 2009 International Conference on Power Electronics and Drive Systems (PEDS), Taipei, Taiwan, 2–5 November 2009. [Google Scholar]
- Sun, Q.; Lyu, G.; Liu, X.; Niu, F.; Gan, C. Virtual Current Compensation-Based Quasi-Sinusoidal-Wave Excitation Scheme for Switched Reluctance Motor Drives. IEEE Trans. Ind. Electron. 2023, 1–12. [Google Scholar] [CrossRef]
- Shen, Y.; Liu, D.; Liang, W.; Zhang, X. Current Reconstruction of Three-Phase Voltage Source Inverters Considering Current Ripple. IEEE Trans. Transp. Electrif. 2022, 9, 1416–1427. [Google Scholar] [CrossRef]
- Salem, M.; Richelli, A.; Yahya, K.; Hamidi, M.N.; Ang, T.-Z.; Alhamrouni, I. A Comprehensive Review on Multilevel Inverters for Grid-Tied System Applications. Energies 2022, 15, 6315. [Google Scholar] [CrossRef]
- Thummala, P.; Maksimovic, D.; Zhang, Z.; Andersen, M.A.E. Digital Control of a High-Voltage (2.5 Kv) Bidirectional Dc–Dc Flyback Converter for Driving a Capacitive Incremental Actuator. IEEE Trans. Power Electron. 2016, 31, 8500–8516. [Google Scholar] [CrossRef]
- Kim, H.-W.; Park, J.-H. Isolated Bidirectional Switched-Capacitor Flyback Converter. In Proceedings of the 2014 International Power Electronics and Application Conference and Exposition, Shanghai, China, 5–8 November 2014. [Google Scholar]
- Li, C.; Herrera, L.; Jia, J.; Fu, L.; Isurin, A.; Cook, A.; Huang, Y.; Wang, J. Design and Implementation of a Bidirectional Isolated Ćuk Converter for Low-Voltage and High-Current Automotive Dc Source Applications. IEEE Trans. Veh. Technol. 2013, 63, 2567–2577. [Google Scholar] [CrossRef]
- Ling, R.; Dan, Q.; Wang, L.; Li, D. Energy Bus-Based Equalization Scheme with Bi-Directional Isolated Cuk Equalizer for Series Connected Battery Strings. In Proceedings of the 2015 IEEE Applied Power Electronics Conference and Exposition (APEC), Charlotte, NC, USA, 15–19 March 2015. [Google Scholar]
- Kosenko, R.; Zakis, J.; Blinov, A.; Chub, A.; Veligorskyi, O. Full Soft-Switching Bidirectional Isolated Current-Fed Dual Inductor Push-Pull Dc-Dc Converter for Battery Energy Storage Applications. In Proceedings of the 2016 57th International Scientific Conference on Power and Electrical Engineering of Riga Technical University (RTUCON), Riga, Latvia, 13–14 October 2016. [Google Scholar]
- Mukhtar, N.M.; Lu, D.D.-C. An Isolated Bidirectional Forward Converter with Integrated Output Inductor-Transformer Structure. In Proceedings of the 2018 IEEE 4th Southern Power Electronics Conference (SPEC), Singapore, 10–13 December 2018. [Google Scholar]
- Park, K.-B.; Moon, G.-W.; Youn, M.-J. Two-Switch Active-Clamp Forward Converter with One Clamp Diode and Delayed Turnoff Gate Signal. IEEE Trans. Ind. Electron. 2011, 58, 4768–4772. [Google Scholar] [CrossRef]
- Das, D.; Weise, N.; Basu, K.; Baranwal, R.; Mohan, N. A Bidirectional Soft-Switched Dab-Based Single-Stage Three-Phase Ac–Dc Converter for V2g Application. IEEE Trans. Transp. Electrif. 2018, 5, 186–199. [Google Scholar] [CrossRef]
- Han, W.; Corradini, L. General Closed-Form Zvs Analysis of Dual-Bridge Series Resonant Dc–Dc Converters. IEEE Trans. Power Electron. 2018, 34, 9289–9302. [Google Scholar] [CrossRef]
- He, P.; Khaligh, A. Comprehensive Analyses and Comparison of 1 Kw Isolated Dc–Dc Converters for Bidirectional Ev Charging Systems. IEEE Trans. Transp. Electrif. 2016, 3, 147–156. [Google Scholar] [CrossRef]
- Morrison, R.; Egan, M.G. A New Power-Factor-Corrected Single-Transformer Ups Design. IEEE Trans. Ind. Appl. 2000, 36, 171–179. [Google Scholar] [CrossRef]
- Du, Y.; Lukic, S.; Jacobson, B.; Huang, A. Review of High Power Isolated Bi-Directional Dc-Dc Converters for Phev/Ev Dc Charging Infrastructure. In Proceedings of the 2011 IEEE Energy Conversion Congress and Exposition, Phoenix, AZ, USA, 17–22 September 2011. [Google Scholar]
- Yuan, J.; Dorn-Gomba, L.; Callegaro, A.D.; Reimers, J.; Emadi, A. A Review of Bidirectional on-Board Chargers for Electric Vehicles. IEEE Access 2021, 9, 51501–51518. [Google Scholar] [CrossRef]
- Bassa de los Mozos, A.; Chandra Mouli, G.R.; Bauer, P. Evaluation of Topologies for a Solar Powered Bidirectional Electric Vehicle Charger. IET Power Electron. 2019, 12, 3675–3687. [Google Scholar] [CrossRef]
- Leite, R.S.; Afonso, J.L.; Monteiro, V. A Novel Multilevel Bidirectional Topology for on-Board Ev Battery Chargers in Smart Grids. Energies 2018, 11, 3453. [Google Scholar] [CrossRef]
- Wang, H.; Sun, W.; Jiang, D.; Qu, R. A Mtpa and Flux-Weakening Curve Identification Method Based on Physics-Informed Network without Calibration. IEEE Trans. Power Electron. 2023, 38, 12370–12375. [Google Scholar] [CrossRef]
- Yang, X.; Wang, X.; Wang, S.; Wang, K.; Sial, M.B. Finite-Time Adaptive Dynamic Surface Synchronization Control for Dual-Motor Servo Systems with Backlash and Time-Varying Uncertainties. ISA Trans. 2023, 137, 248–262. [Google Scholar] [CrossRef] [PubMed]
- Hosseinpour, M.; Seifi, A.; Rahimian, M.M. A Bidirectional Diode Containing Multilevel Inverter Topology with Reduced Switch Count and Driver. Int. J. Circuit Theory 2020, 48, 1766–1785. [Google Scholar] [CrossRef]
- Kosenko, R.; Blinov, A.; Vinnikov, D.; Chub, A. Asymmetric Snubberless Current-Fed Full-Bridge Isolated Dc-Dc Converters. Sci. J. Riga. Tech. Univ.-Electr. Control Commun. Eng. 2018, 14, 5–11. [Google Scholar] [CrossRef]
- Karthikeyan, B.; Sundararaju, K.; Palanisamy, R.; Manivasagam, R.; Hossain, I.; Bajaj, M.; Shouran, M.; Kamel, S. A Dual Input Single Output Non-Isolated Dc-Dc Converter for Multiple Sources Electric Vehicle Applications. Front. Energy Res. 2022, 10, 979539. [Google Scholar] [CrossRef]
- Lu, L.; Wu, W.; Gao, Y.; Pan, C.; Yu, X.; Zhang, C.; Jin, Z. Study on Current Discrepancy and Redistribution of Hts Non-Insulation Closed-Loop Coils During Charging/Discharging and Subsequent Transient Process toward Steady-State Operation. Supercond. Sci. Technol. 2022, 35, 095001. [Google Scholar] [CrossRef]
- Gao, Y.; Doppelbauer, M.; Ou, J.; Qu, R. Design of a Double-Side Flux Modulation Permanent Magnet Machine for Servo Application. IEEE J. Emerg. Sel. Top. Power Electron. 2021, 10, 1671–1682. [Google Scholar] [CrossRef]
- Lavanya, A.; Jegatheesan, R.; Vijayakumar, K. Design of Novel Dual Input Dc–Dc Converter for Energy Harvesting System in Iot Sensor Nodes. Wirel. Pers. Commun. 2021, 117, 2793–2808. [Google Scholar] [CrossRef]
- Kim, S.-H.; Byun, H.-J.; Yi, J.; Won, C.-Y. A Bi-Directional Dual-Input Dual-Output Converter for Voltage Balancer in Bipolar Dc Microgrid. Energies 2022, 15, 5043. [Google Scholar] [CrossRef]
- Suresh, K.; Bharatiraja, C.; Chellammal, N.; Tariq, M.; Chakrabortty, R.K.; Ryan, M.J.; Alamri, B. A Multifunctional Non-Isolated Dual Input-Dual Output Converter for Electric Vehicle Applications. IEEE Access 2021, 9, 64445–64460. [Google Scholar] [CrossRef]
- Fernão Pires, V.; Cordeiro, A.; Foito, D.; Silva, J.F. A High-Voltage Gain Non-Isolated Dc–Dc Converter Designed for Bipolar Dc Microgrids. Electr. Power Compon. Syst. 2023, 51, 1171–1181. [Google Scholar] [CrossRef]
- Kim, S.; Nam, H.-T.; Cha, H.; Kim, H.-G. Investigation of Self-Output Voltage Balancing in Input-Parallel Output-Series Dc–Dc Converter. IEEE J. Emerg. Sel. Top. Power Electron. 2019, 8, 2850–2860. [Google Scholar] [CrossRef]
- Aravind, R.; Chokkalingam, B.; Mihet-Popa, L. A Transformerless Non-Isolated Multi-Port Dc–Dc Converter for Hybrid Energy Applications. IEEE Access 2023, 11, 52050–52065. [Google Scholar] [CrossRef]
- Lin, X.; Liu, Y.; Yu, J.; Yu, R.; Zhang, J.; Wen, H. Stability Analysis of Three-Phase Grid-Connected Inverter under the Weak Grids with Asymmetrical Grid Impedance by Ltp Theory in Time Domain. Int. J. Electr. Power Energy Syst. 2022, 142, 108244. [Google Scholar] [CrossRef]
- Lin, X.; Wen, Y.; Yu, R.; Yu, J.; Wen, H. Improved Weak Grids Synchronization Unit for Passivity Enhancement of Grid-Connected Inverter. IEEE J. Emerg. Sel. Top. Power Electron. 2022, 10, 7084–7097. [Google Scholar] [CrossRef]
- Mohseni, P.; Hosseini, S.H.; Sabahi, M.; Jalilzadeh, T.; Maalandish, M. A New High Step-up Multi-Input Multi-Output Dc–Dc Converter. IEEE Trans. Ind. Electron. 2018, 66, 5197–5208. [Google Scholar] [CrossRef]
- Dezhbord, M.; Mohseni, P.; Hosseini, S.H.; Mirabbasi, D.; Islam, M.R. A High Step-up Three-Port Dc–Dc Converter with Reduced Voltage Stress for Hybrid Energy Systems. IEEE J. Emerg. Sel. Top. Ind. Electron. 2022, 3, 998–1009. [Google Scholar] [CrossRef]
- Chen, C.; Wu, X.; Yuan, X.; Zheng, X. A New Technique for the Subdomain Method in Predicting Electromagnetic Performance of Surface-Mounted Permanent Magnet Motors with Shaped Magnets and a Quasi-Regular Polygon Rotor Core. IEEE Trans. Energy Convers. 2022, 38, 1396–1409. [Google Scholar] [CrossRef]
- Li, S.; Zhao, X.; Liang, W.; Hossain, T.; Zhang, Z. A Fast and Accurate Calculation Method of Line Breaking Power Flow Based on Taylor Expansion. Front. Energy Res. 2022, 10, 943946. [Google Scholar] [CrossRef]
- Zhang, X.; Gong, L.; Zhao, X.; Li, R.; Yang, L.; Wang, B. Voltage and Frequency Stabilization Control Strategy of Virtual Synchronous Generator Based on Small Signal Model. Energy Rep. 2023, 9, 583–590. [Google Scholar] [CrossRef]
- Song, X.; Wang, H.; Ma, X.; Yuan, X.; Wu, X. Robust Model Predictive Current Control for a Nine-Phase Open-End Winding Pmsm with High Computational Efficiency. IEEE Trans. Power Electron. 2023, 38, 13933–13943. [Google Scholar] [CrossRef]
- Liu, S.; Liu, C. Direct Harmonic Current Control Scheme for Dual Three-Phase Pmsm Drive System. IEEE Trans. Ind. Electron. 2021, 36, 11647–11657. [Google Scholar] [CrossRef]
- Huang, N.; Chen, Q.; Cai, G.; Xu, D.; Zhang, L.; Zhao, W. Fault Diagnosis of Bearing in Wind Turbine Gearbox under Actual Operating Conditions Driven by Limited Data with Noise Labels. IEEE Trans. Instrum. Meas. 2020, 70, 3502510. [Google Scholar] [CrossRef]
- Sun, K.; Zhang, L.; Xing, Y.; Guerrero, J.M. A Distributed Control Strategy Based on Dc Bus Signaling for Modular Photovoltaic Generation Systems with Battery Energy Storage. IEEE Trans. Power Electron. 2011, 26, 3032–3045. [Google Scholar] [CrossRef]
- Rehman, Z.; Al-Bahadly, I.; Mukhopadhyay, S. Multiinput Dc–Dc Converters in Renewable Energy Applications–An Overview. Renew. Sustain. Energy Rev. 2015, 41, 521–539. [Google Scholar] [CrossRef]
- Qian, Z.; Abdel-Rahman, O.; Al-Atrash, H.; Batarseh, I. Modeling and Control of Three-Port Dc/Dc Converter Interface for Satellite Applications. IEEE Trans. Power Electron. 2009, 25, 637–649. [Google Scholar] [CrossRef]
- Nasiri, A.; Nie, Z.; Bekiarov, S.B.; Emadi, A. An on-Line Ups System with Power Factor Correction and Electric Isolation Using Bifred Converter. IEEE Trans. Ind. Electron. 2008, 55, 722–730. [Google Scholar] [CrossRef]
- Zhang, Z.; Thomsen, O.C.; Andersen, M.A.E.; Nielsen, H.R. Dual-Input Isolated Full-Bridge Boost Dc–Dc Converter Based on the Distributed Transformers. IET Power Electron. 2012, 5, 1074–1083. [Google Scholar] [CrossRef]
- Zhang, Z.; Thomsen, O.C.; Andersen, M.A.E. Modeling and Control of a Dual-Input Isolated Full-Bridge Boost Converter. In Proceedings of the 2012 Twenty-Seventh Annual IEEE Applied Power Electronics Conference and Exposition (APEC), Orlando, FL, USA, 5–9 February 2012. [Google Scholar]
- Yalla, S.P.; Subudhi, P.S.; Ramachandaramurthy, V.K. Topological Review of Hybrid Res Based Multi-Port Converters. IET Renew. Power Gener. 2022, 16, 1087–1106. [Google Scholar] [CrossRef]
- Zhang, N.; Sutanto, D.; Muttaqi, K.M. A Review of Topologies of Three-Port Dc–Dc Converters for the Integration of Renewable Energy and Energy Storage System. Renew. Sustain. Energy Rev. 2016, 56, 388–401. [Google Scholar] [CrossRef]
- Yalamanchili, K.P.; Ferdowsi, M. Review of Multiple Input Dc-Dc Converters for Electric and Hybrid Vehicles. In Proceedings of the 2005 IEEE Vehicle Power and Propulsion Conference, Chicago, IL, USA, 7 September 2005. [Google Scholar]
- Chen, Y.-M.; Liu, Y.-C.; Lin, S.-H. Double-Input Pwm Dc/Dc Converter for High-/Low-Voltage Sources. IEEE Trans. Ind. Electron. 2006, 53, 1538–1545. [Google Scholar] [CrossRef]
- Belloni, M.; Bonizzoni, E.; Maloberti, F. On the Design of Single-Inductor Double-Output Dc–Dc Buck, Boost and Buck-Boost Converters. In Proceedings of the 2008 15th IEEE International Conference on Electronics, Circuits and Systems, St. Julian’s, Malta, 31 August–3 September 2008. [Google Scholar]
- Ray, O.; Josyula, A.P.; Mishra, S.; Joshi, A. Integrated Dual-Output Converter. IEEE Trans. Ind. Electron. 2014, 62, 371–382. [Google Scholar] [CrossRef]
- Hosseini, S.; Haghighian, S.K.; Danyali, S.; Aghazadeh, H. Multi-Input Dc Boost Converter Supplied by a Hybrid Pv/Wind Turbine Power Systems for Street Lighting Application Connected to the Grid. In Proceedings of the 2012 47th International Universities Power Engineering Conference (UPEC), London, UK, 4–7 September 2012. [Google Scholar]
- Nejabatkhah, F.; Danyali, S.; Hosseini, S.H.; Sabahi, M.; Niapour, S.M. Modeling and Control of a New Three-Input Dc–Dc Boost Converter for Hybrid Pv/Fc/Battery Power System. IEEE Trans. Ind. Electron. 2011, 27, 2309–2324. [Google Scholar] [CrossRef]
- Wu, H.; Sun, K.; Ding, S.; Xing, Y. Topology Derivation of Nonisolated Three-Port Dc–Dc Converters from Dic and Doc. IEEE Trans. Power Electron. 2012, 28, 3297–3307. [Google Scholar] [CrossRef]
- Wu, H.; Xing, Y.; Xia, Y.; Sun, K. A Family of Non-Isolated Three-Port Converters for Stand-Alone Renewable Power System. In Proceedings of the IECON 2011—37th Annual Conference of the IEEE Industrial Electronics Society, Melbourne, Australia, 7–10 November 2011. [Google Scholar]
- Ding, S.; Wu, H.; Xing, Y.; Fang, Y.; Ma, X. Topology and Control of a Family of Non-Isolated Three-Port Dc-Dc Converters with a Bidirectional Cell. In Proceedings of the 2013 Twenty-Eighth Annual IEEE Applied Power Electronics Conference and Exposition (APEC), Long Beach, CA, USA, 17–21 March 2013. [Google Scholar]
- Chen, Y.; Wen, G.; Peng, L.; Kang, Y.; Chen, J. A Family of Cost-Efficient Non-Isaolated Single-Inductor Three-Port Converters for Low Power Stand-Alone Renewable Power Applications. In Proceedings of the 2013 Twenty-Eighth Annual IEEE Applied Power Electronics Conference and Exposition (APEC), Long Beach, CA, USA, 17–21 March 2013. [Google Scholar]
- Chen, Y.; Zhang, P.; Zou, X.; Kang, Y. Dynamical Modeling of the Non-Isolated Single-Inductor Three-Port Converter. In Proceedings of the 2014 IEEE Applied Power Electronics Conference and Exposition-APEC 2014, Fort Worth, TX, USA, 16–20 March 2014. [Google Scholar]
- Vazquez, N.; Sanchez, C.; Hernandez, C.; Vazquez, E.; Lesso, R. A Three Port Converter for Renewable Energy Applications. In Proceedings of the 2011 IEEE International Symposium on Industrial Electronics, Gdańsk, Poland, 27–30 June 2011. [Google Scholar]
- Zhou, Z.; Wu, H.; Ma, X.; Xing, Y. A Non-Isolated Three-Port Converter for Stand-Alone Renewable Power System. In Proceedings of the IECON 2012—38th Annual Conference on IEEE Industrial Electronics Society, Montreal, QC, Canada, 25–28 October 2012. [Google Scholar]
- Bayat, P.; Baghramian, A. Partly Isolated Three-Port Dc-Dc Converter Based on Impedance Network. IET Power Electron. 2020, 13, 2175–2193. [Google Scholar] [CrossRef]
- Qian, Z.; Abdel-Rahman, O.; Reese, J.; Al-Atrash, H.; Batarseh, I. Dynamic Analysis of Three-Port Dc/Dc Converter for Space Applications. In Proceedings of the 2009 Twenty-Fourth Annual IEEE Applied Power Electronics Conference and Exposition, Washington, DC, USA, 15–19 February 2009. [Google Scholar]
- Qian, Z.; Abdel-Rahman, O.; Pepper, M.; Batarseh, I. Analysis and Design for Paralleled Three-Port Dc/Dc Converters with Democratic Current Sharing Control. In Proceedings of the 2009 IEEE Energy Conversion Congress and Exposition, San Jose, CA, USA, 20–24 September 2009. [Google Scholar]
- Qian, Z.; Abdel-Rahman, O.; Hu, H.; Batarseh, I. Multi-Channel Three-Port Dc/Dc Converters as Maximum Power Tracker, Battery Charger and Bus Regulator. In Proceedings of the 2010 Twenty-Fifth Annual IEEE Applied Power Electronics Conference and Exposition (APEC), Palm Springs, CA, USA, 21–25 February 2010. [Google Scholar]
- Qian, Z.; Abdel-Rahman, O.; Zhang, K.; Hu, H.; Shen, J.; Batarseh, I. Design and Analysis of Three-Port Dc/Dc Converters for Satellite Platform Power System. In Proceedings of the 2011 IEEE Energy Conversion Congress and Exposition, Phoenix, AZ, USA, 17–22 September 2011. [Google Scholar]
- Wu, H.; Chen, R.; Zhang, J.; Xing, Y.; Hu, H.; Ge, H. A Family of Three-Port Half-Bridge Converters for a Stand-Alone Renewable Power System. IEEE Trans. Power Electron. 2011, 26, 2697–2706. [Google Scholar] [CrossRef]
- Wu, H.; Xing, Y.; Chen, R.; Zhang, J.; Sun, K.; Ge, H. A Three-Port Half-Bridge Converter with Synchronous Rectification for Renewable Energy Application. In Proceedings of the 2011 IEEE Energy Conversion Congress and Exposition, Phoenix, AZ, USA, 17–22 September 2011. [Google Scholar]
- Zhang, J.; Wu, H.; Cao, F.; Xing, Y.; Ma, X. Analysis and Design of Dc Distributed Dc Power System with Modular Three-Port Converter. In Proceedings of the 2014 IEEE 23rd International Symposium on Industrial Electronics (ISIE), Istanbul, Turkey, 1–4 June 2014. [Google Scholar]
- Duarte, J.L.; Hendrix, M.; Simões, M.G. Three-Port Bidirectional Converter for Hybrid Fuel Cell Systems. IEEE Trans. Power Electron. 2007, 22, 480–487. [Google Scholar] [CrossRef]
- Tao, H.; Kotsopoulos, A.; Duarte, J.L.; Hendrix, M.A. Family of Multiport Bidirectional Dc–Dc Converters. IEE Proc.-Electr. Power Appl. 2006, 153, 451–458. [Google Scholar] [CrossRef]
- Tao, H.; Duarte, J.L.; Hendrix, M.A. Multiport Converters for Hybrid Power Sources. In Proceedings of the 2008 IEEE Power Electronics Specialists Conference, Rhodes, Greece, 15–19 June 2008. [Google Scholar]
- Liu, D.; Li, H.; Marlino, L.D. Design of a 6 Kw Multiple-Input Bi-Directional Dc-Dc Converter with Decoupled Current Sharing Control for Hybrid Energy Storage Elements. In Proceedings of the APEC 07-Twenty-Second Annual IEEE Applied Power Electronics Conference and Exposition, Anaheim, CA, USA, 25 February–1 March 2007. [Google Scholar]
- Wen, G.; Chen, Y.; Kang, Y. A Family of Cost-Efficient Integrated Single-Switch Three-Port Converters. In Proceedings of the 2013 Twenty-Eighth Annual IEEE Applied Power Electronics Conference and Exposition (APEC), Long Beach, CA, USA, 17–21 March 2013. [Google Scholar]
- Parthiban, R.; Rajambal, K. Performance Investigation of Three-Port Converter for Hybrid Energy Systems. In Proceedings of the 2014 IEEE 2nd International Conference on Electrical Energy Systems (ICEES), Chennai, India, 7–9 January 2014. [Google Scholar]
- Sun, X.; Liu, F.; Xiong, L.; Wang, B. Research on Dual Buck/Boost Integrated Three-Port Bidirectional Dc/Dc Converter. In Proceedings of the 2014 IEEE Conference and Expo Transportation Electrification Asia-Pacific (ITEC Asia-Pacific), Beijing, China, 31 August–3 September 2014. [Google Scholar]
- Yang, S.; Bryant, A.; Mawby, P.; Xiang, D.; Ran, L.; Tavner, P. An Industry-Based Survey of Reliability in Power Electronic Converters. IEEE Trans. Ind. Appl. 2011, 47, 1441–1451. [Google Scholar] [CrossRef]
- Li, W.; He, X. Review of Nonisolated High-Step-up Dc/Dc Converters in Photovoltaic Grid-Connected Applications. IEEE Trans. Ind. Electron. 2010, 58, 1239–1250. [Google Scholar] [CrossRef]
- Zhao, C.; Round, S.D.; Kolar, J.W. An Isolated Three-Port Bidirectional Dc-Dc Converter with Decoupled Power Flow Management. IEEE Trans. Power Electron. 2008, 23, 2443–2453. [Google Scholar] [CrossRef]
- Oggier, G.G.; Botalla, L.P.; García, G.O. Soft-Switching Analysis for Three-Port Bidirectional Dc-Dc Converters. In Proceedings of the 2010 9th IEEE/IAS International Conference on Industry Applications-INDUSCON 2010, São Paulo, Brazil, 8–10 November 2010. [Google Scholar]
- Piris-Botalla, L.; Oggier, G.G.; Airabella, A.M.; García, G.O. Analysis and Evaluation of Power Switch Losses for Three-Port Bidirectional Dc-Dc Converter. In Proceedings of the 2012 IEEE International Conference on Industrial Technology, Athens, Greece, 19–21 March 2012. [Google Scholar]
- Piris-Botalla, L.; Oggier, G.G.; Airabella, A.M.; García, G.O. Power Losses Evaluation of a Bidirectional Three-Port Dc–Dc Converter for Hybrid Electric System. Int. J. Electr. Power Energy Syst. 2014, 58, 1–8. [Google Scholar] [CrossRef]
- Phattanasak, M.; Gavagsaz-Ghoachani, R.; Martin, J.-P.; Nahid-Mobarakeh, B.; Pierfederici, S.; Davat, B. Control of a Hybrid Energy Source Comprising a Fuel Cell and Two Storage Devices Using Isolated Three-Port Bidirectional Dc–Dc Converters. IEEE Trans. Ind. Appl. 2014, 51, 491–497. [Google Scholar] [CrossRef]
- Krishnaswami, H.; Mohan, N. Three-Port Series-Resonant Dc–Dc Converter to Interface Renewable Energy Sources with Bidirectional Load and Energy Storage Ports. IEEE Trans. Power Electron. 2009, 24, 2289–2297. [Google Scholar] [CrossRef]
- Wang, L.; Wang, Z.; Li, H. Asymmetrical Duty Cycle Control and Decoupled Power Flow Design of a Three-Port Bidirectional Dc-Dc Converter for Fuel Cell Vehicle Application. IEEE Trans. Power Electron. 2011, 27, 891–904. [Google Scholar] [CrossRef]
- Wang, H.; Zhou, D.; Blaabjerg, F. A Reliability-Oriented Design Method for Power Electronic Converters. In Proceedings of the 2013 Twenty-Eighth Annual IEEE Applied Power Electronics Conference and Exposition (APEC), Long Beach, CA, USA, 17–21 March 2013. [Google Scholar]
- Kumaravel, S.; Achathuparambil Narayanankutty, R.; Rao, V.S.; Sankar, A. Dual Input-Dual Output Dc-Dc Converter for Solar Pv/Battery/Ultra-Capacitor Powered Electric Vehicle Application. IET Power Electron. 2019, 12, 3351–3358. [Google Scholar] [CrossRef]
- Rooholahi, B.; Siwakoti, Y.P.; Eckel, H.-G.; Blaabjerg, F.; Bahman, A.S. Enhanced Single-Inductor Single-Input Dual-Output Dc–Dc Converter with Voltage Balancing Capability. IEEE Trans. Ind. Electron. 2023. [Google Scholar] [CrossRef]
- Zhu, B.; Chen, S.; Zhang, Y.; Huang, Y. An Interleaved Zero-Voltage Zero-Current Switching High Step-up Dc-Dc Converter. IEEE Access 2020, 9, 5563–5572. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, X.; Li, X.; Yuan, H.; Xue, Y. Model Predictive Control-Based Dual-Mode Operation of an Energy-Stored Quasi-Z-Source Photovoltaic Power System. IEEE Trans. Ind. Electron. 2022, 70, 9169–9180. [Google Scholar] [CrossRef]
- Shanmugam, S.; Sharmila, A. Multiport Converters for Incorporating Solar Photovoltaic System with Battery Storage: A Pilot Survey Towards Modern Influences, Challenges and Future Scenarios. Front. Energy Res. 2022, 10, 947424. [Google Scholar] [CrossRef]

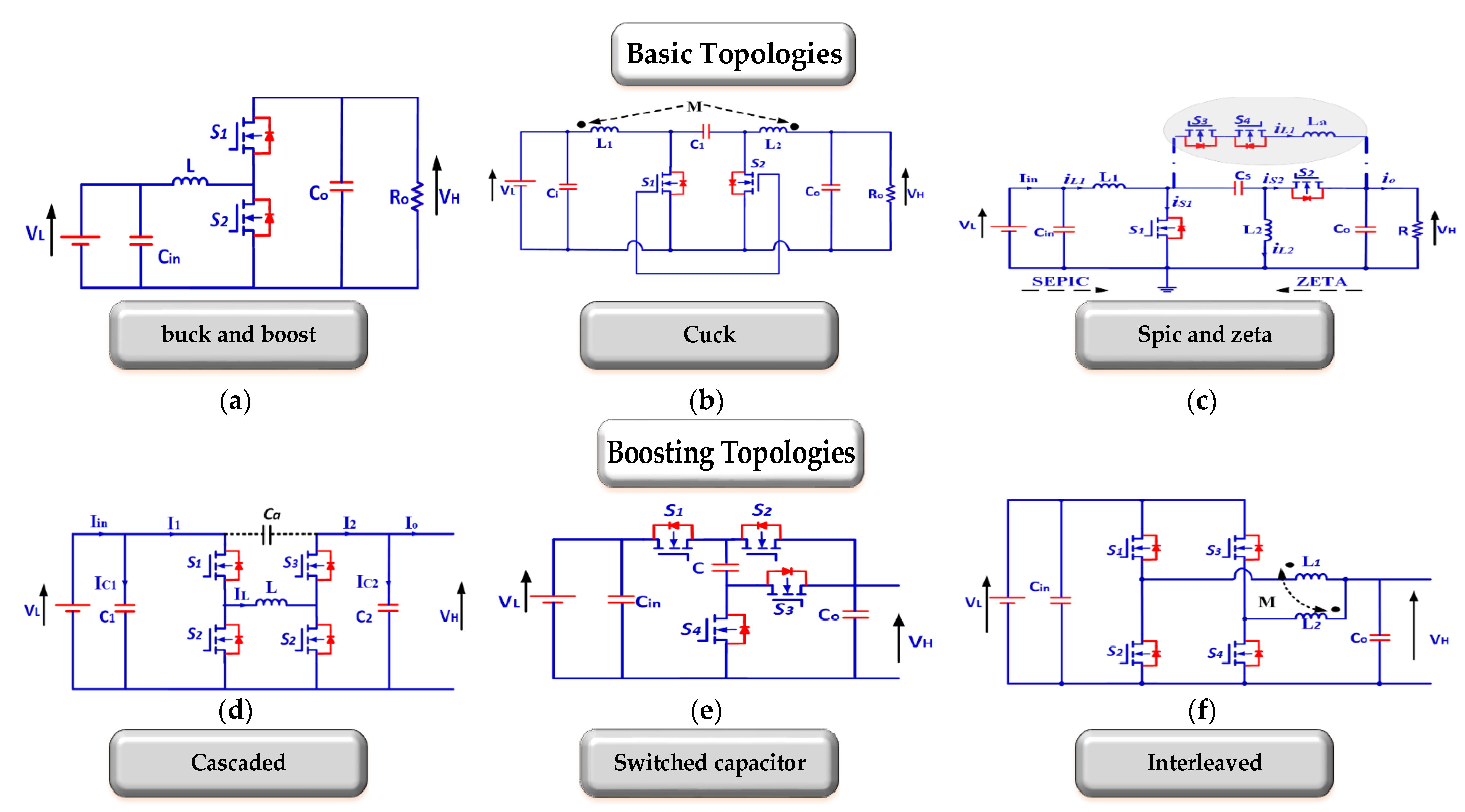





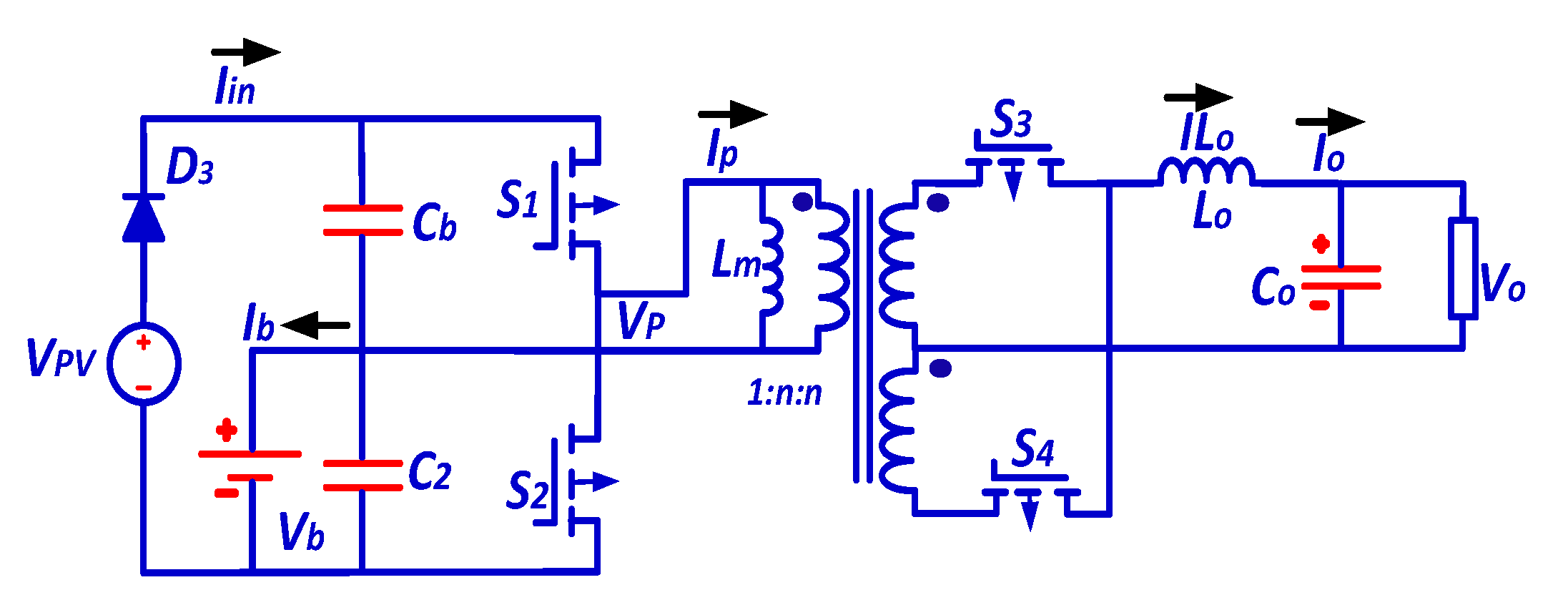

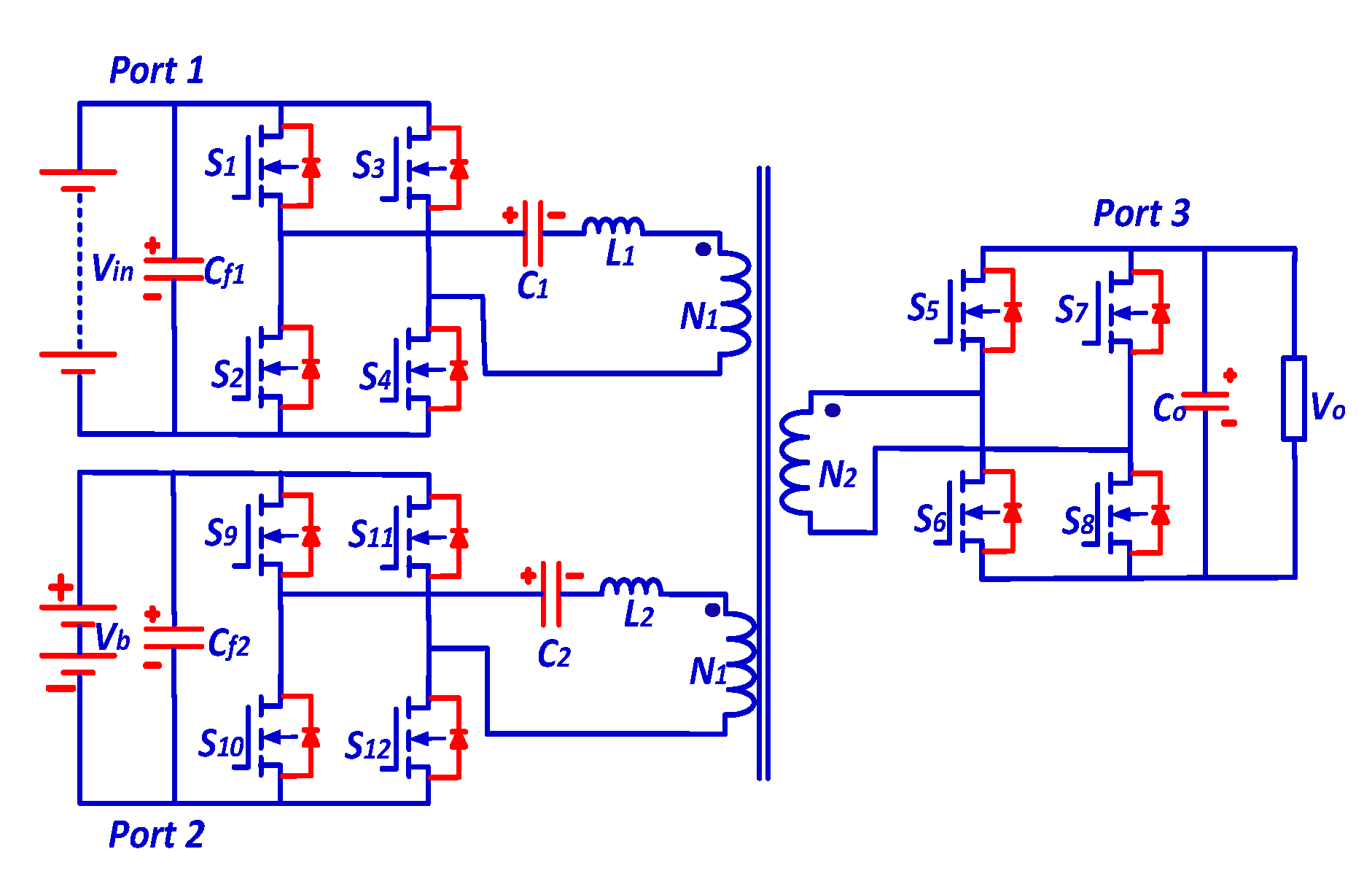
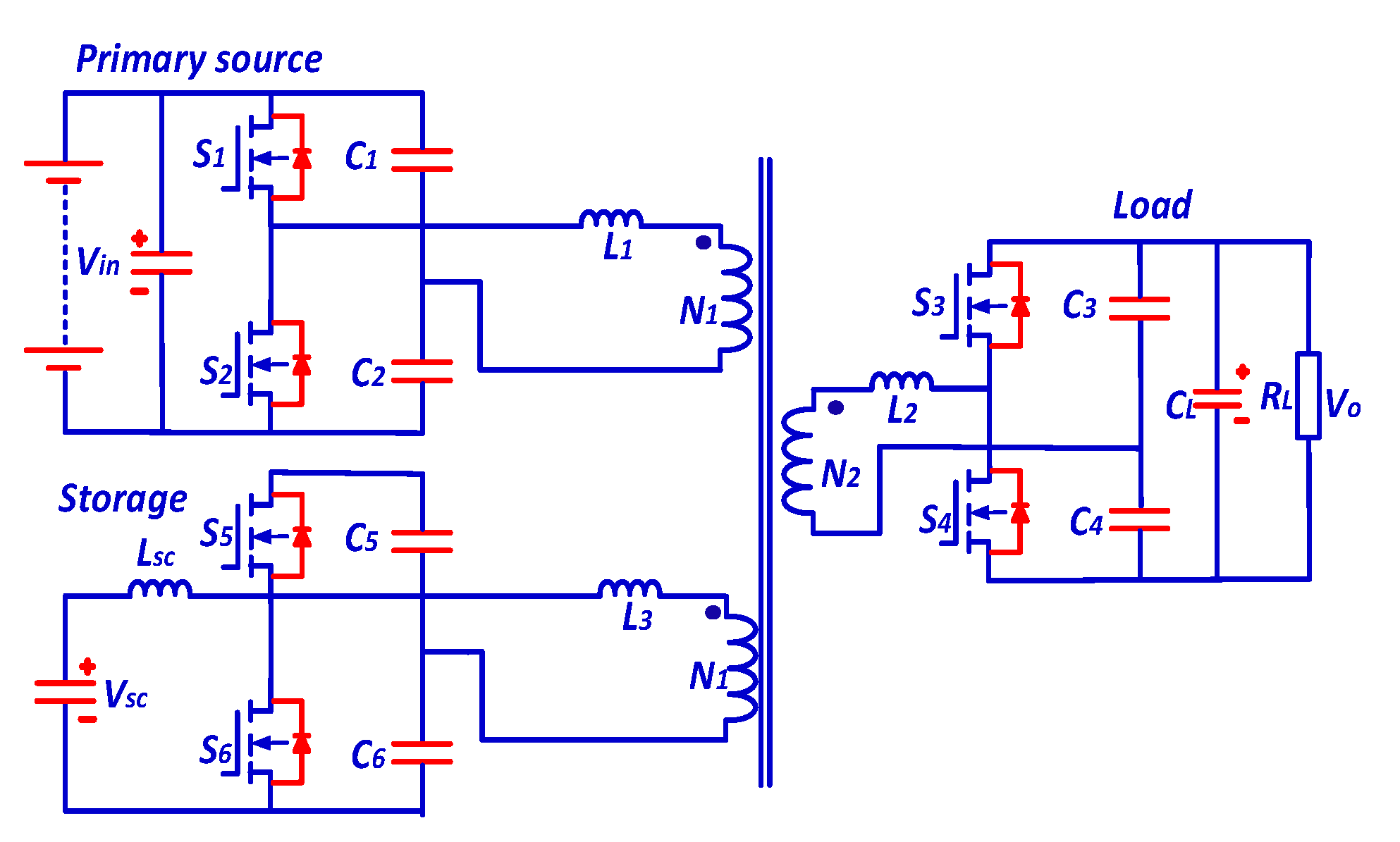
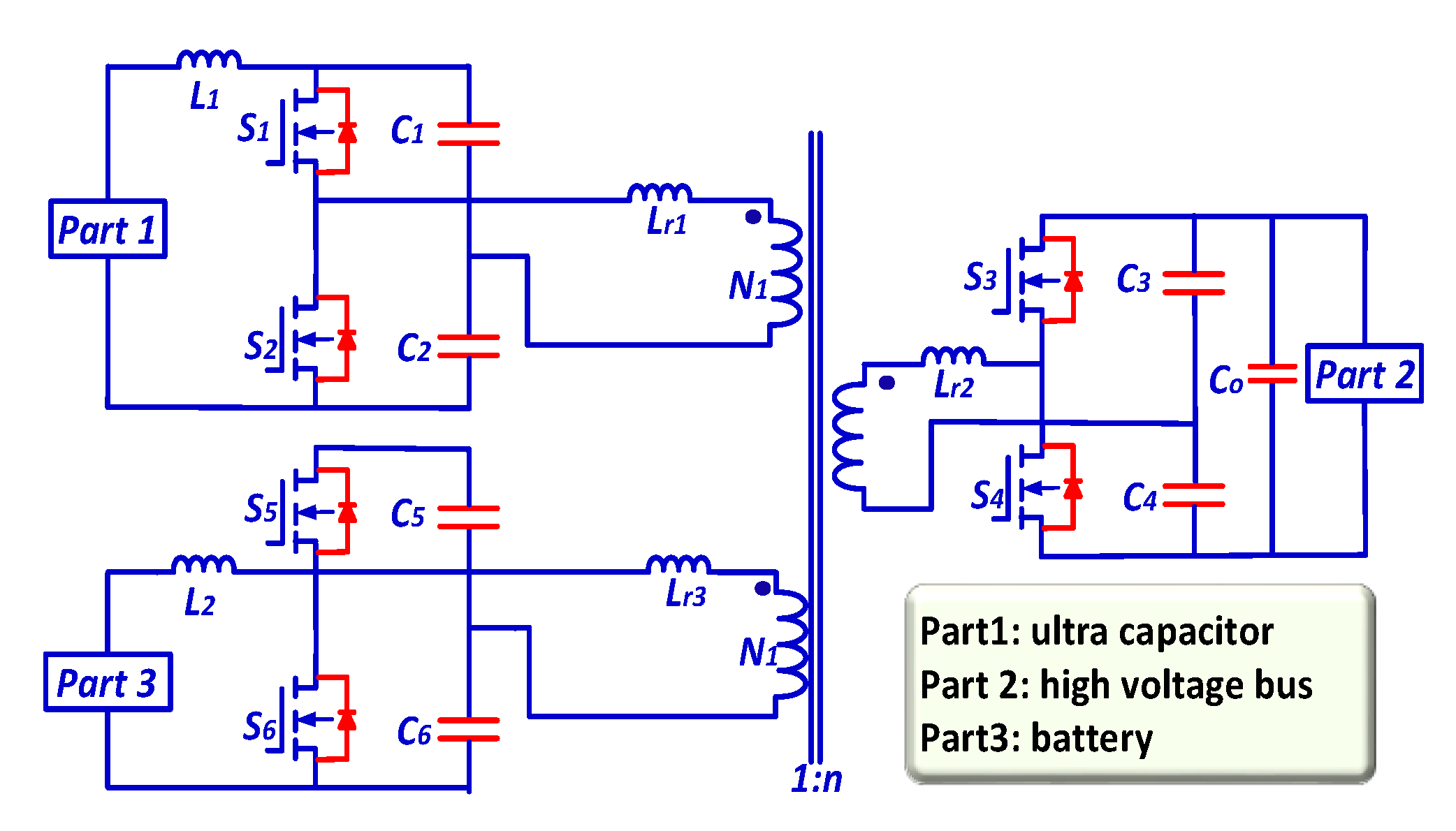


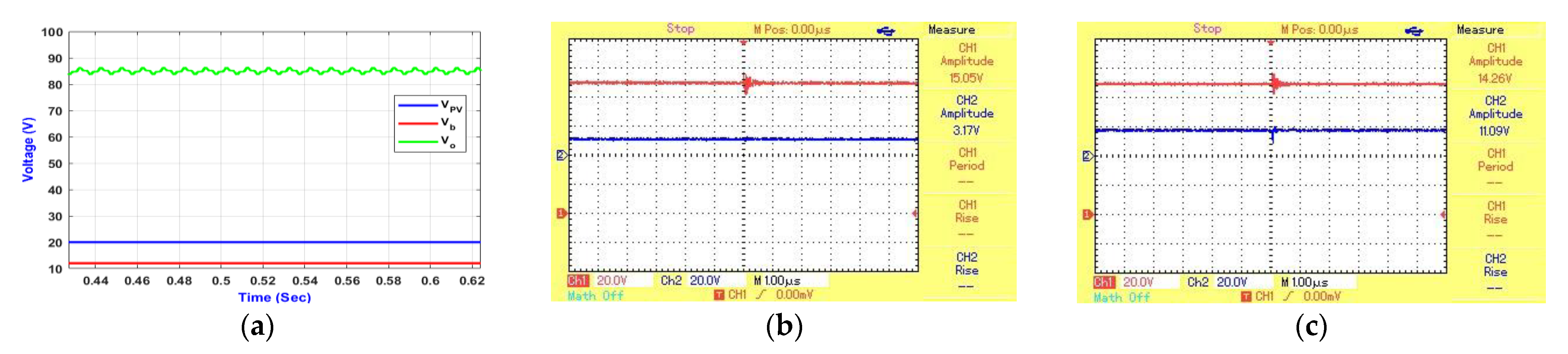

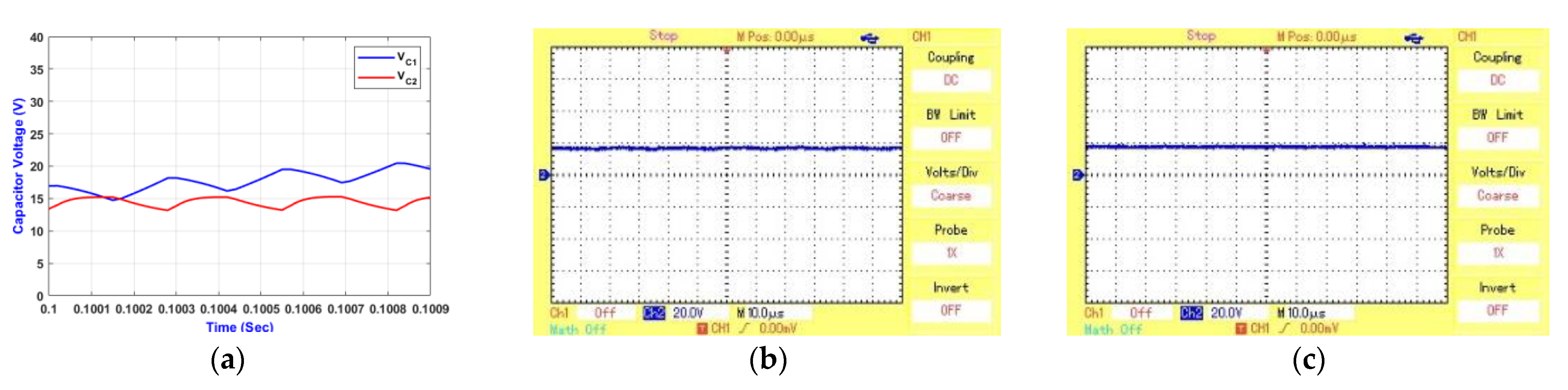
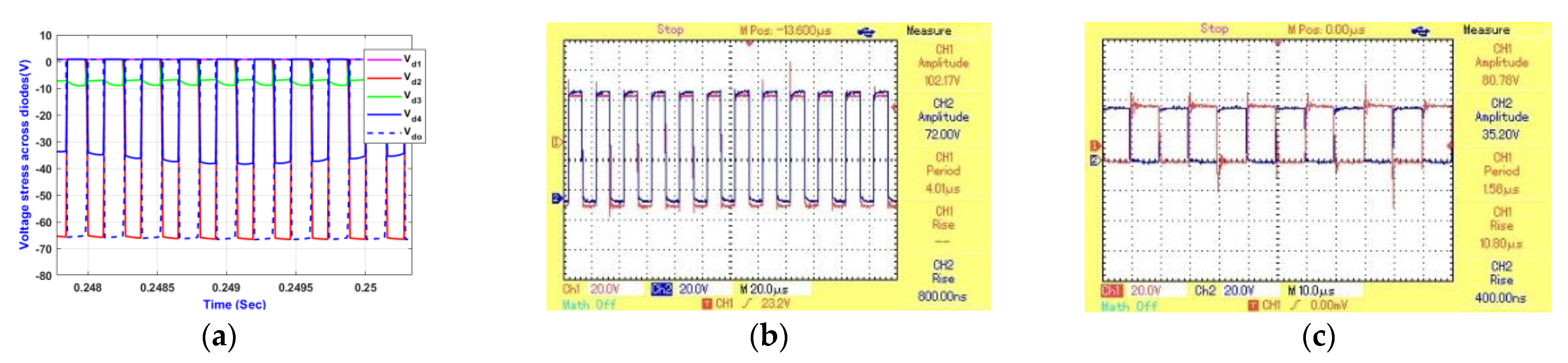

| Types of NIBDC | Voltage Gain | Number of Switches | Number of Passive Components | Characteristics | Applications |
|---|---|---|---|---|---|
| Basic buck and boost | 2 | 3 |
| Rechargeable power supply units [31]. Energy storage system [9,32]. | |
| Cuk | 4 | 5 |
| Vehicle application [33] Energy storage application [34]. Battery equalization ultracapacitor–battery interface circuits [35]. | |
| Sepic/zeta | 2 | 5 |
| Distributed power system [19]. | |
| Cascaded | 4 | 3 |
| Electric vehicle motor drive application [36]. | |
| Switched capacitor | 4 | 3 |
| Uninterruptable power supplies (UPSs) [37]. Battery charging–discharging. Automotive system voltage conversion [38]. | |
| Interleaved | 2n = 4 | 2 + n |
| Hybrid vehicle application [28]. High-power applications [39]. |
| Types of IBDC | Voltage Gain | Number of Switches | Number of Passive Components | Characteristics | Applications |
|---|---|---|---|---|---|
| Flyback | 2 | 2 | Basic isolated topology. Discontinuity of Iin. | Switched-mode power converters [64]. Low–medium power application [65]. | |
| Cuk | 2 | 6 | Continuous Iin and Io. Eliminated ripples of by input/output-coupled inductors. | Electric vehicles [49,66]; energy bus-based equalization network [67]. | |
| Push–pull | 4 | 2 | Continuous number of windings of more than two. | Energy storage [55,68]. | |
| Forward | 3 | 2 | Continuous Io. Limited D. Low-power level apps. | Energy storage system [69]. Low to medium applications [70]. | |
| DAB | Varies W.R.T control scheme | 8 | 2 | Isolated bidirectional topology is the most prevalent. Suitable for applications requiring high power or voltage. | DC microgrid [41,42,59]. Automotive applications [71]. |
| Dual half-bridge | Varies W.R.T control scheme | 4 | 6 | There are fewer semiconductors in use. A lower-power alternative to digital audio broadcasting (DAB). | Energy storage system [72]. Automotive battery [73]. |
| Half–full-bridge | Varies W.R.T control scheme | 6 | 4 | For UPS systems, this is the best. Incorporates two switch converters seamlessly. | Uninterruptable power supply (power factor-corrected) [74]. Electric vehicles [75]. |
| Structure of Converter | No. of Component | Cost | Complexity | Reliability | Efficiency |
|---|---|---|---|---|---|
| Non-isolated | Few | Low | Simple | High | Less |
| Partially isolated | Medium | Medium | Medium | High | Medium |
| Fully isolated | More | High | Complex | Low | High |
| Refs. | Structure of Converter | No. of Semiconductors | No. of Inductors | No of Winding | Capacity | Efficiency | Benefits |
|---|---|---|---|---|---|---|---|
| [116,117,118] | Non-isolated | 3 switches and 3 diodes | 1 | NW | 1 KW | 97.2% | 1—High power capacity. 2—Smaller size. 3—Higher efficiency. |
| [119,120] | Non-isolated | 2 switches and 2 diodes | 1 | NW | 24 W | - | 1—Simple computation. 2—Small size. |
| [121] | Non-isolated | 3 switches and 3 diodes | 2 | NW | 100 W | - | 1—Ease of operation. 2—Simplicity of control. |
| [117] | Non-isolated | 4 switches and 3 diodes | 2 | NW | 400 W | 92% | 1—Higher reliability. 2—Higher power capacity. |
| [135] | Non-isolated | 1 switch and 5 diodes | 2 | NW | 24W | - | 1—Only one switch. 2—Small size. 3—Long life span of the battery. 4—Cost efficient. |
| [104,124,125,126,127] | Partially isolated | 5 switches and 1 diode | 1 transformer | 3 | 200 W | - | 1—Strict load control. 2—Wide range of conduction. |
| [128,129,130] | Partially isolated | 4 switches and 1 diode | 1 transformer | 3 | 120 W | 1—High integration. 2—Simple structure. 3—Fewer no. of devices. | |
| [136] | Partially isolated | 4 switches and 4 diode | 1 transformer | 2 | 180 W | 94% | 1—Bidirectional power flow. 2—Reduced input current ripple. |
| [137] | Partially isolated | 6 switches | 2, 1 transformer | 2 | 300 W | - | 1—Minimized input current ripples. 2—Bidirectional power flow between any two ports. 3—Soft switching of all switches and cost efficient. |
| [131,140,141,142,143,144] | Isolated | 12 switches | 1 transformer | 3 | 1.5 KW | 91.7% | 1—Higher voltage conversion ratio. 2—All three ports have galvanic isolation. |
| [145] | Isolated | 12 switches | 1 transformer | 3 | 500 W | 1—High switching frequency. 2—Higher voltage conversion ratio. | |
| [56] | Isolated | 6 switches | 1 transformer | 3 | 1 KW | 92% | The voltage across the half-bridge remains constant. |
| [146] | Isolated | 6 switches | 2, 1 transformer | 3 | 2.5 KW | - | Continuous input current. |
| [147] | Isolated | 10 switches | 2, 1 transformer | 3 | - | - | 1—Small input current ripples. 2—Naturally soft switching. 3—Low voltage stresses of the primary side switches. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alrubaie, A.J.; Swadi, M.; Salem, M.; Richelli, A.; Bughneda, A.; Kamarol, M. Systematic Review of Bidirectional, Multiport Converter Structures and Their Derivatives: A Case Study of Bidirectional Dual Input Dual Output Converters. Energies 2024, 17, 1575. https://doi.org/10.3390/en17071575
Alrubaie AJ, Swadi M, Salem M, Richelli A, Bughneda A, Kamarol M. Systematic Review of Bidirectional, Multiport Converter Structures and Their Derivatives: A Case Study of Bidirectional Dual Input Dual Output Converters. Energies. 2024; 17(7):1575. https://doi.org/10.3390/en17071575
Chicago/Turabian StyleAlrubaie, Ali Jawad, Mahmood Swadi, Mohamed Salem, Anna Richelli, Ali Bughneda, and Mohamad Kamarol. 2024. "Systematic Review of Bidirectional, Multiport Converter Structures and Their Derivatives: A Case Study of Bidirectional Dual Input Dual Output Converters" Energies 17, no. 7: 1575. https://doi.org/10.3390/en17071575
APA StyleAlrubaie, A. J., Swadi, M., Salem, M., Richelli, A., Bughneda, A., & Kamarol, M. (2024). Systematic Review of Bidirectional, Multiport Converter Structures and Their Derivatives: A Case Study of Bidirectional Dual Input Dual Output Converters. Energies, 17(7), 1575. https://doi.org/10.3390/en17071575










