Numerical Study of the Thermo-Hydro-Mechanical Coupling Impacts of Shallow Geothermal Borehole Groups in Fractured Rock Mass on Geological Environment
Abstract
1. Introduction
2. THM Coupling Equations
2.1. Assumptions
2.2. Equations
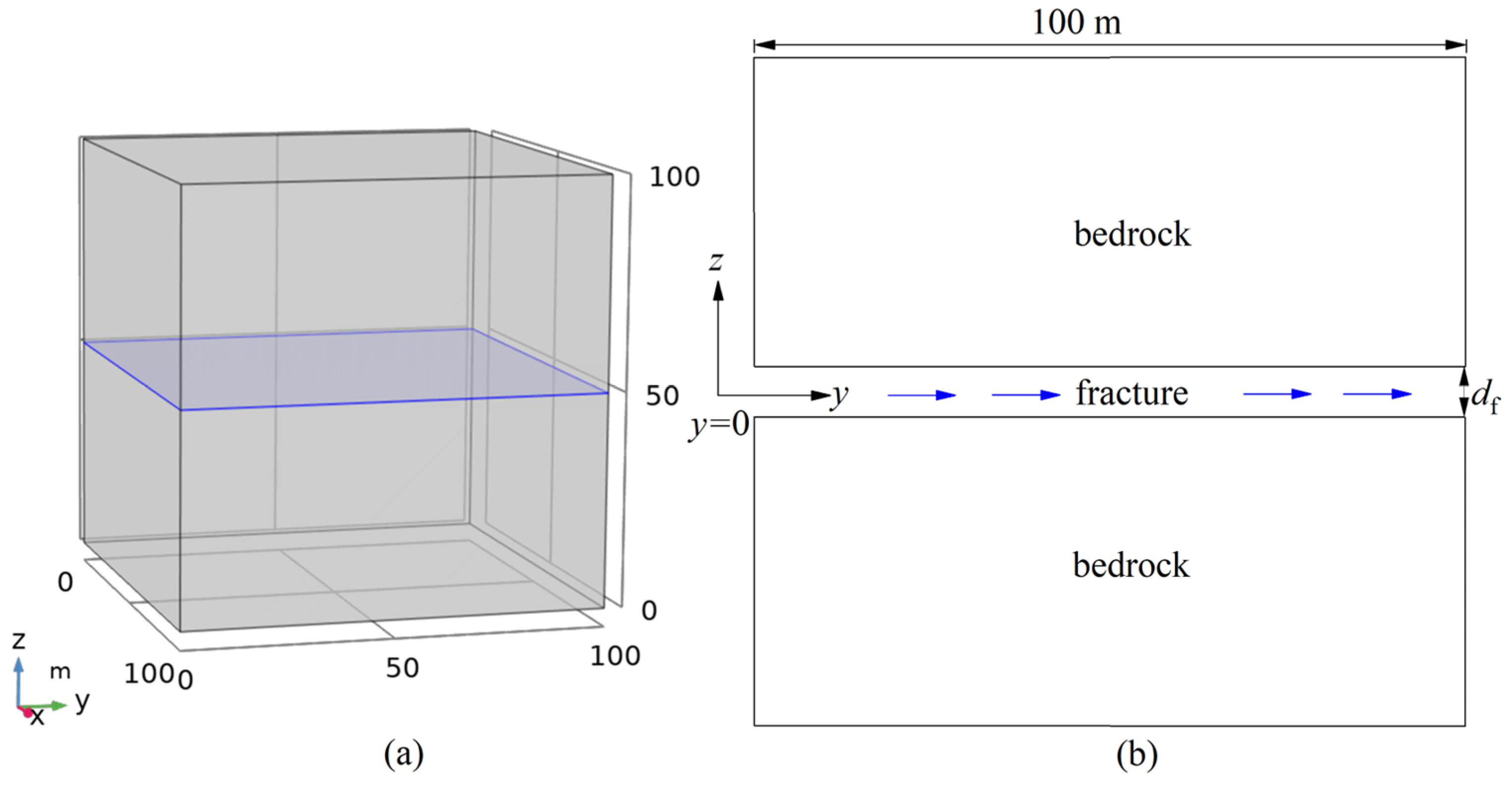
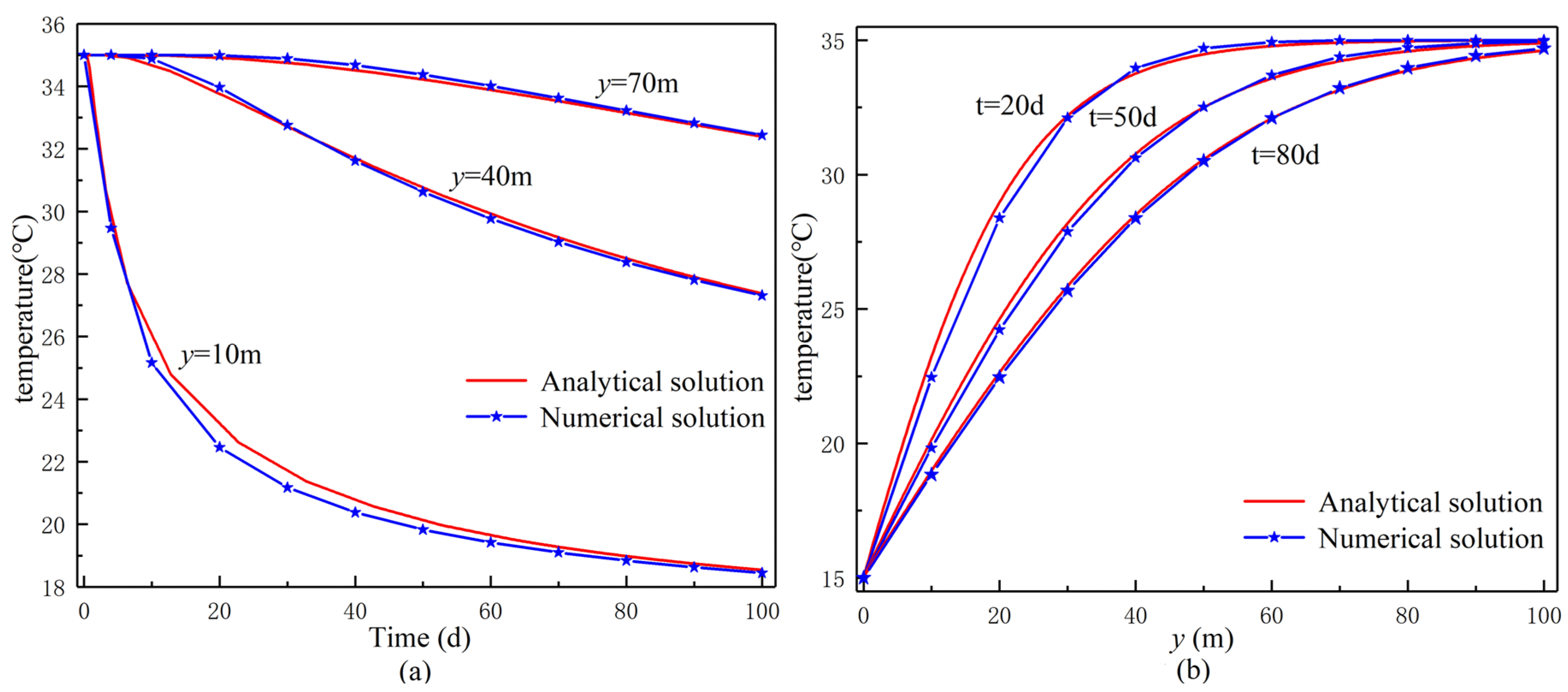
3. Model Validation
4. Establishment of Numerical Model
4.1. Geometric Model
4.2. Meshing
4.3. Initial and Boundary Conditions
5. Simulation Results
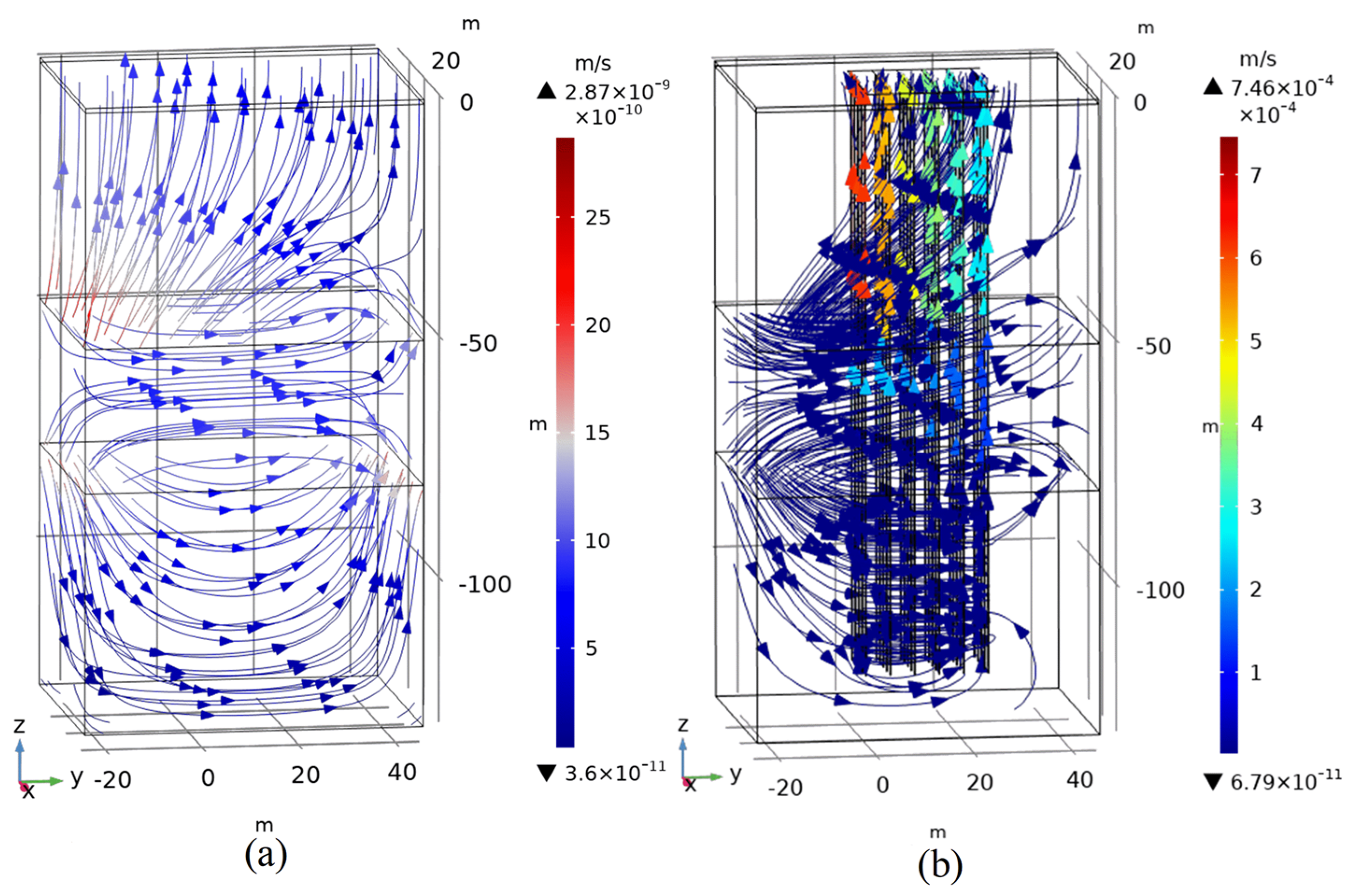
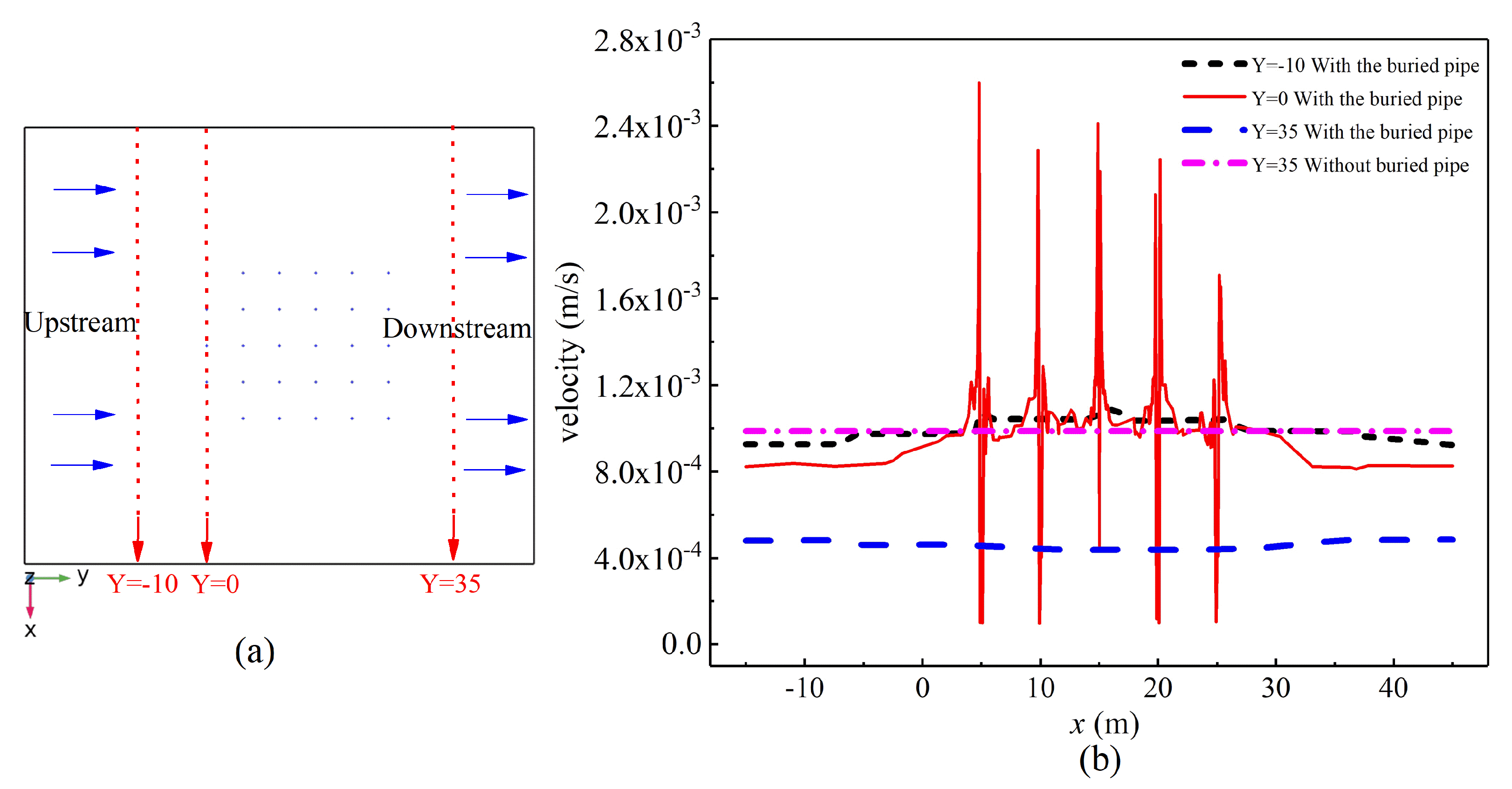
5.1. Groundwater Field
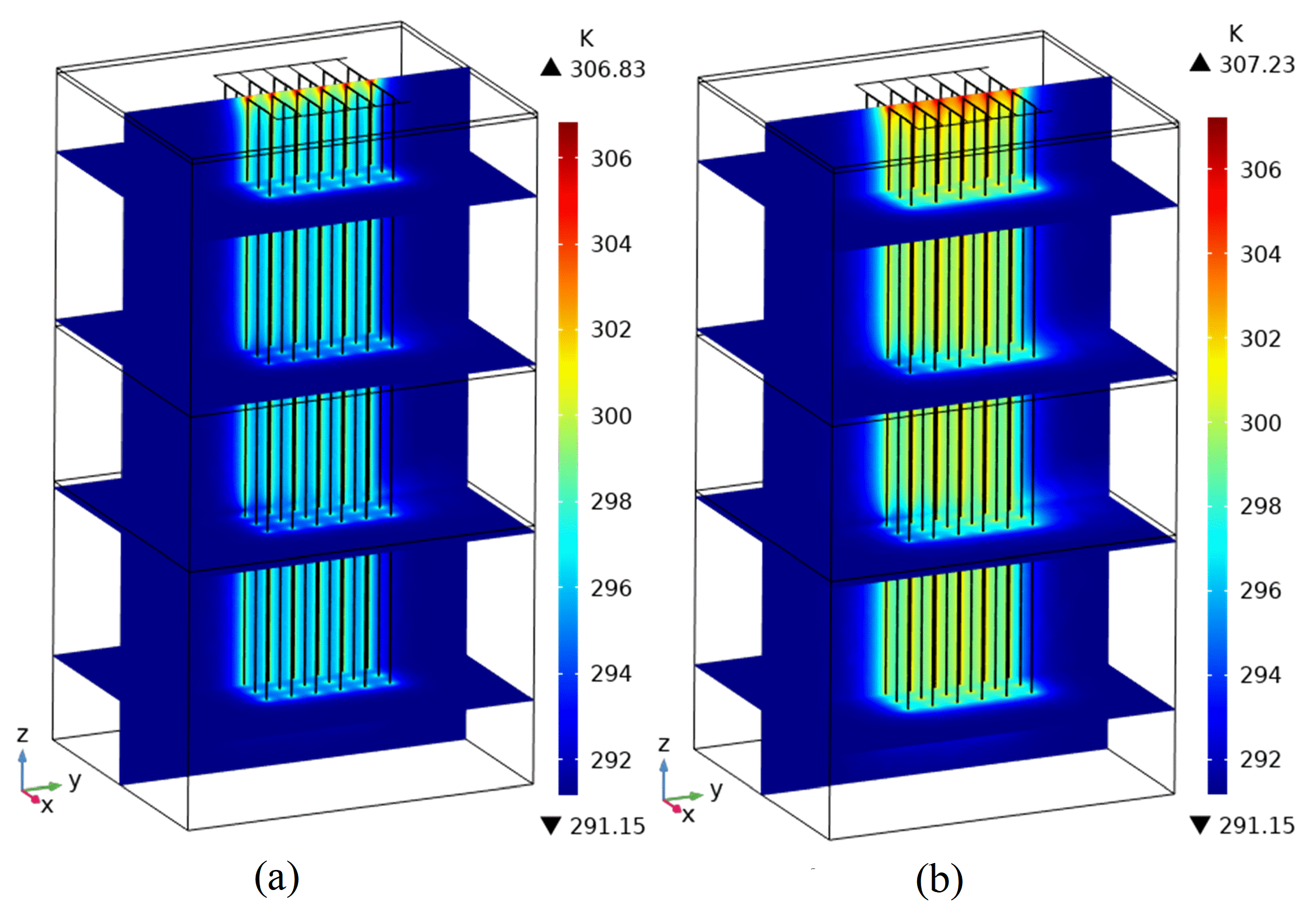
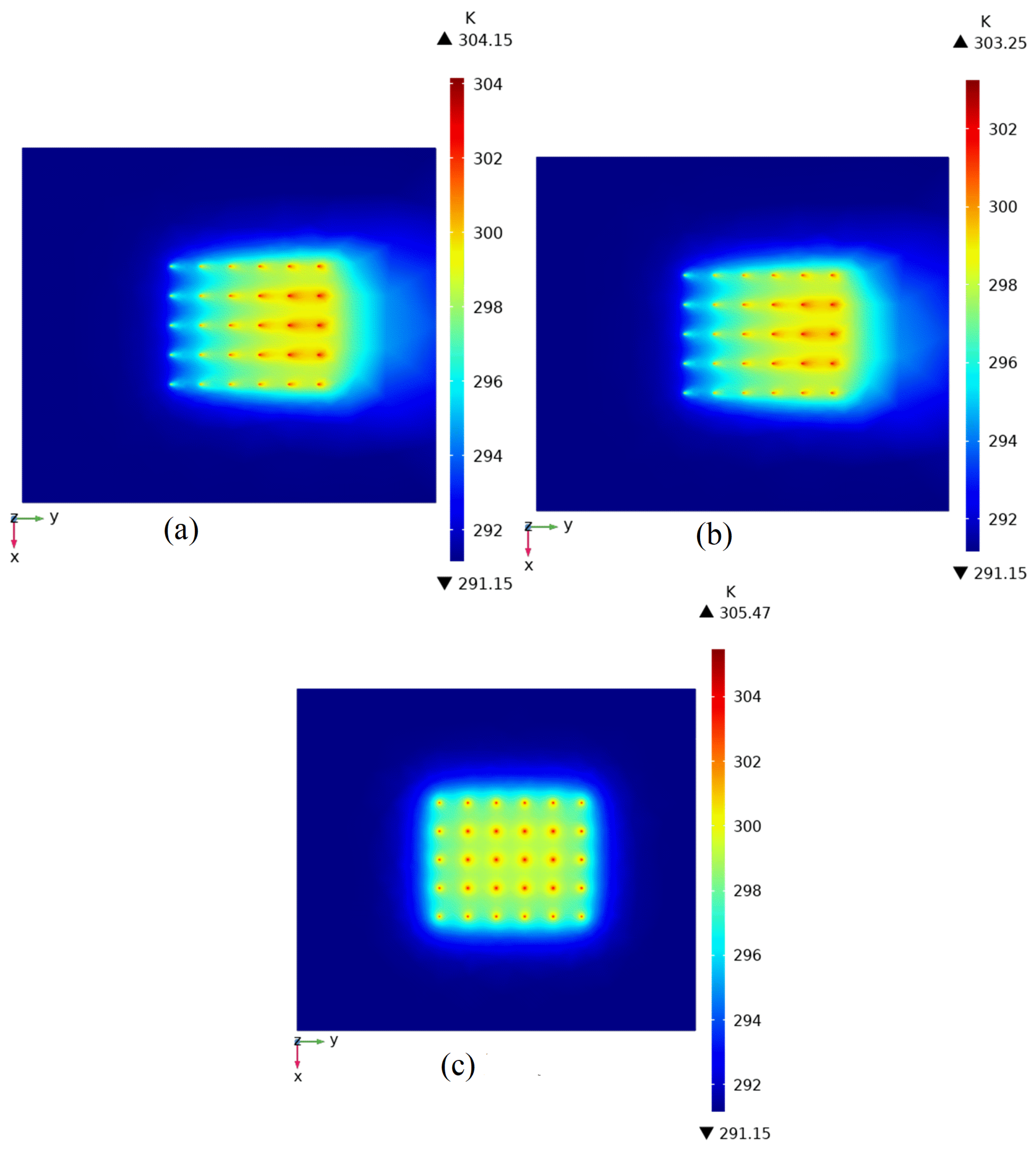
5.2. Temperature Field
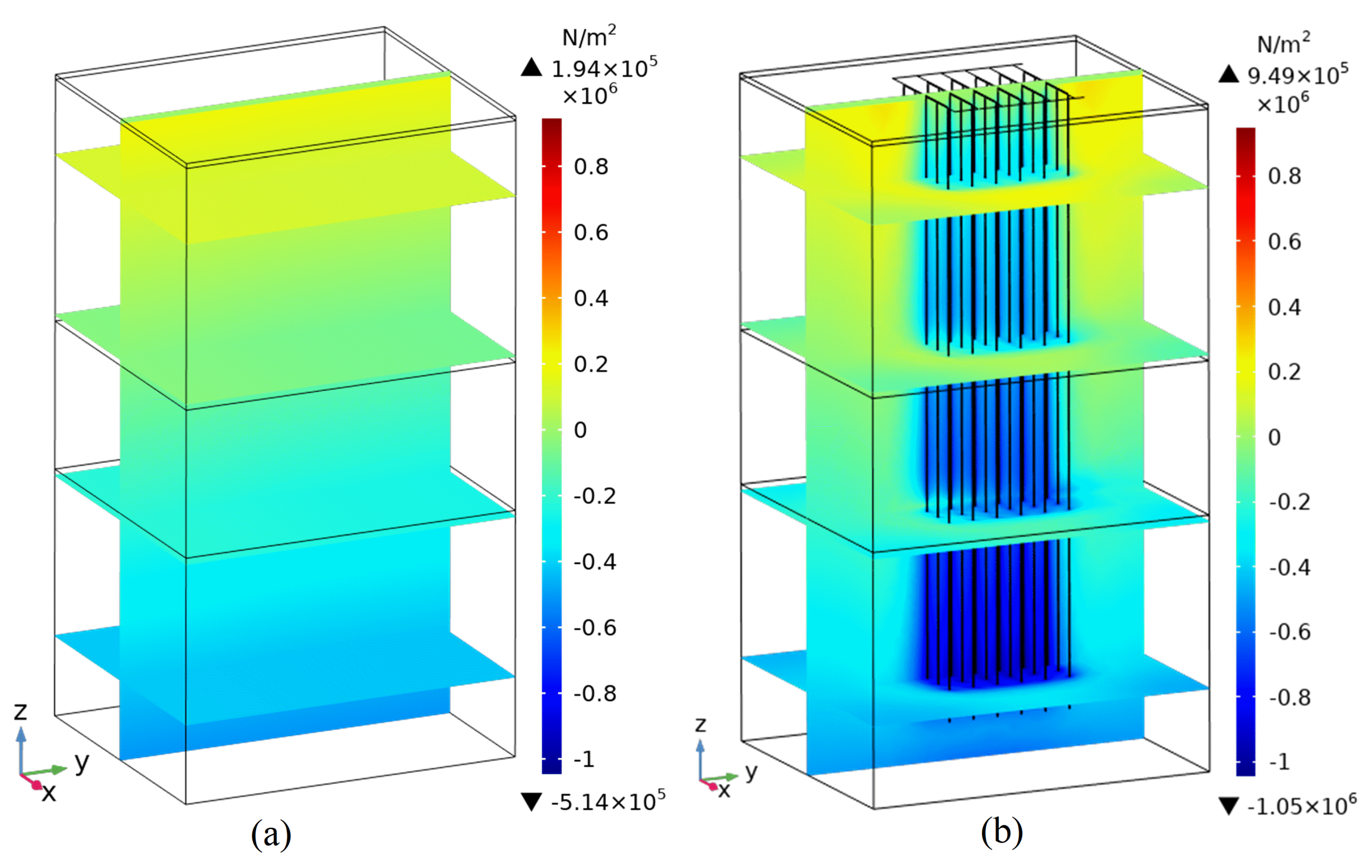
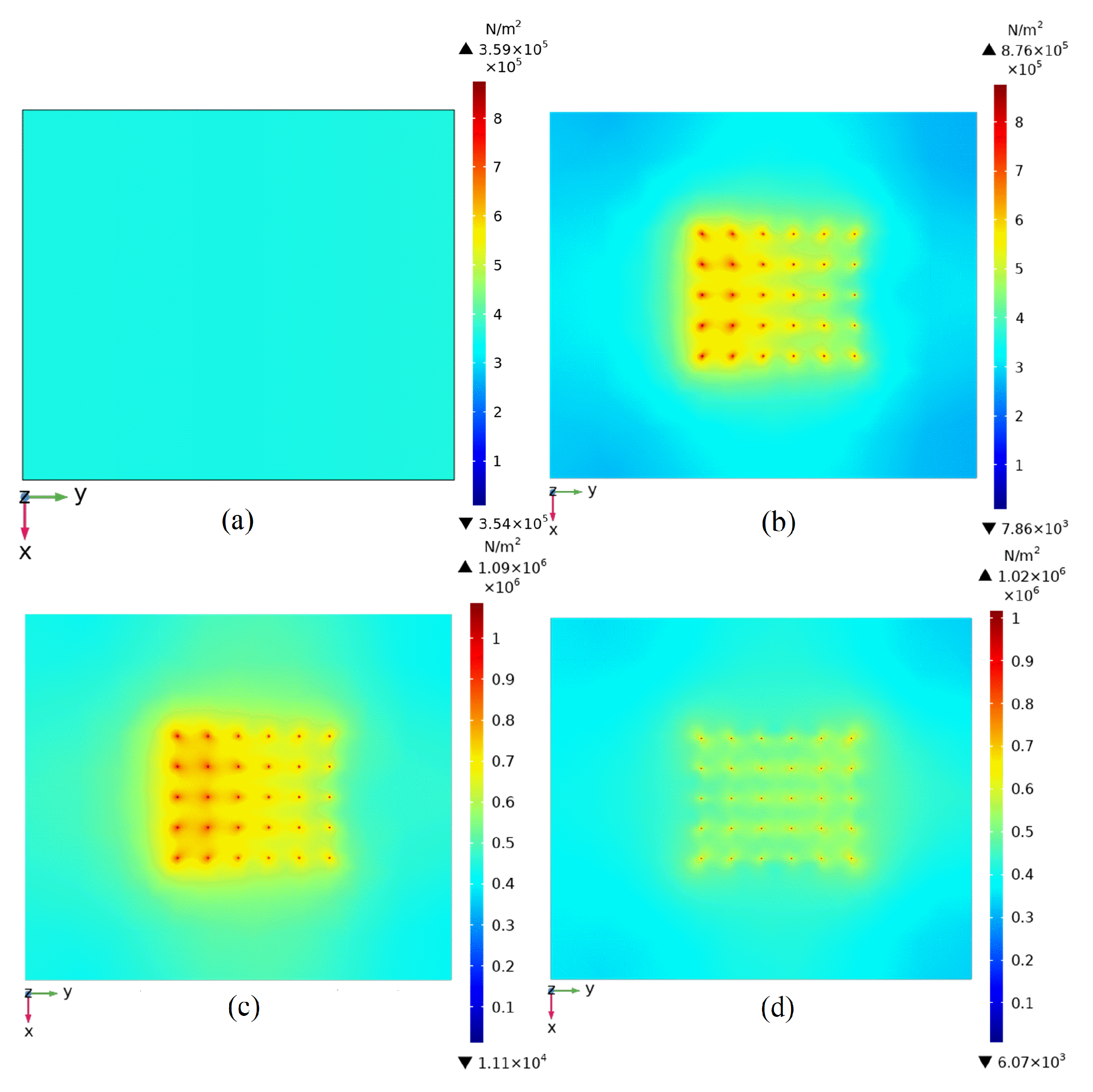
5.3. Maximum Principal Stress Distribution
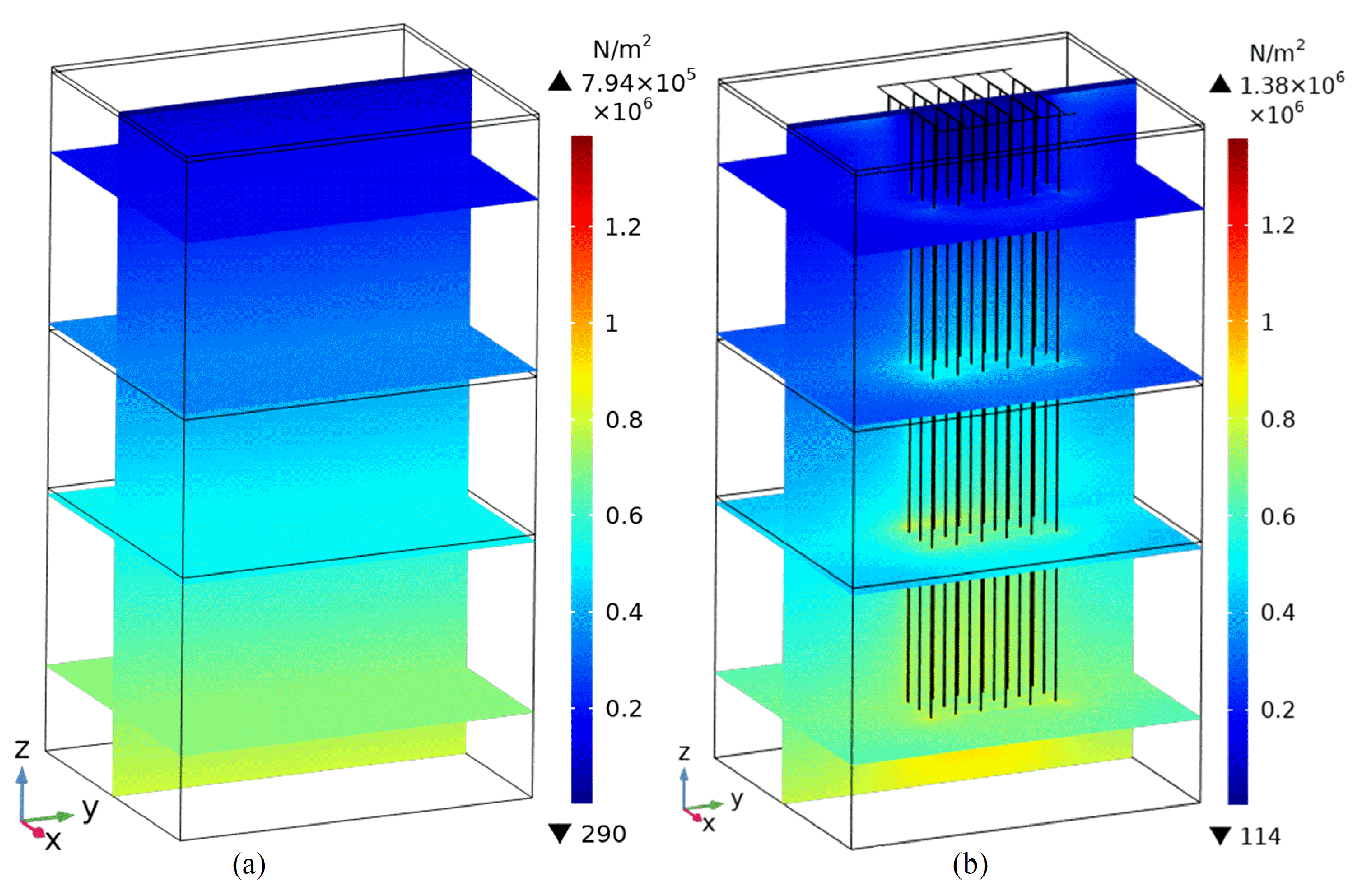
5.4. Maximum Shear Stress Distribution
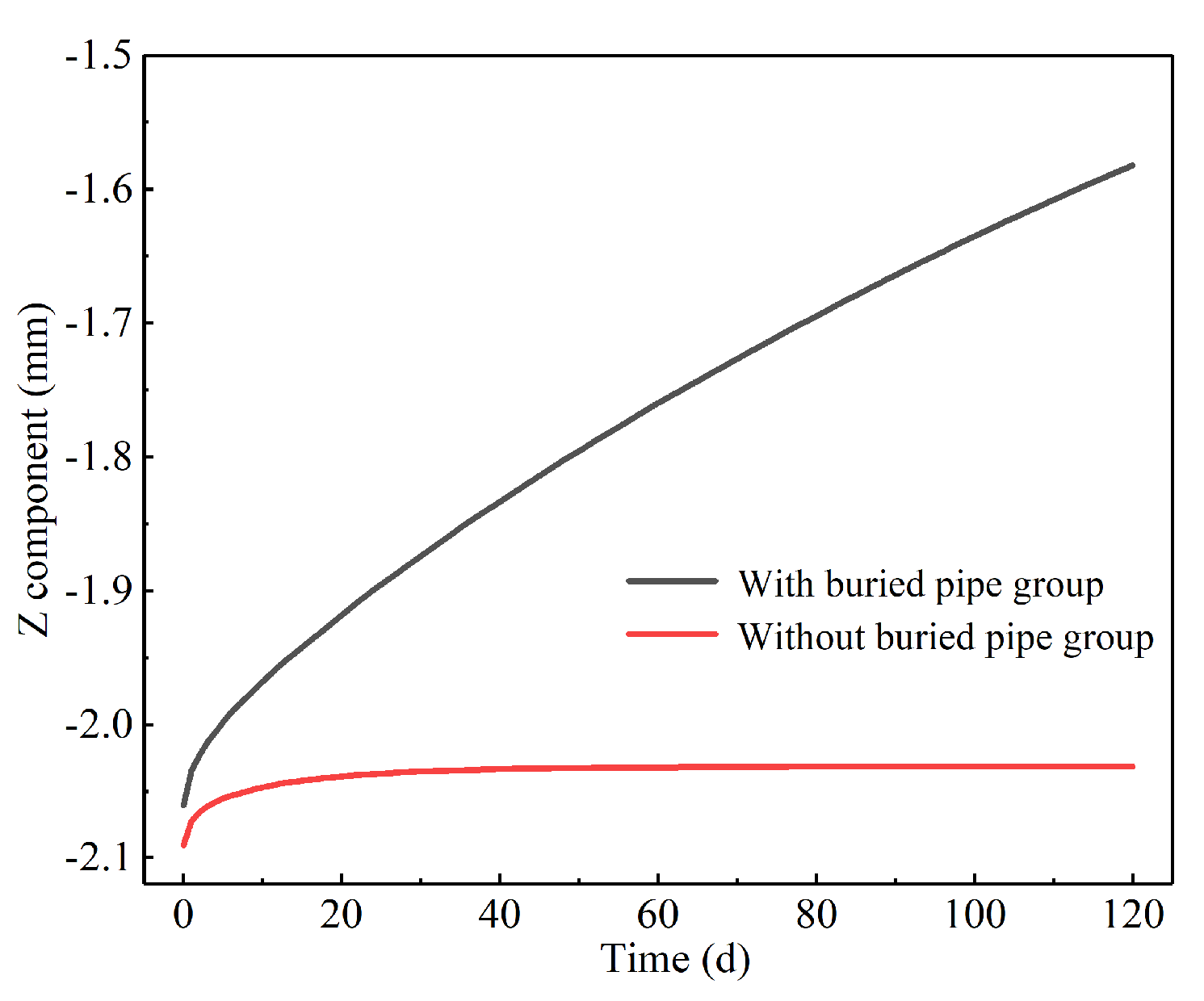
5.5. Displacement in the Rock Mass
6. Discussion
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| u | Darcy flow rate (m/s) | A | cross-sectional area of U-pipe (m2) |
| k | permeability of the porous medium (m2) | ud | fluid velocity in the pipe (m/s) |
| μ | dynamic viscosity of the fluid (Pa∙s) | fD | Darcy friction coefficient |
| p | pore pressure (Pa) | dh | average hydraulic diameter (m) |
| ρ | fluid density (kg/m3) | Qwall | external heat transfer of the pipe wall (W/m) |
| g | gravitational acceleration (m/s2) | Q0 | general heat source (W/m) |
| D | elevation (m) | h | effective value of the pipe heat transfer coefficient (W/(m2∙K)) |
| S | rock matrix water storage coefficient (1/Pa) | Z | pipe wall perimeter (m) |
| t | time (s) | TEXT | pipe outer wall temperature (K) |
| Q | seepage source term (s−1) | αBpi | water pressure effect |
| Sf | fracture water storage coefficient (1/Pa) | βTTs,i | temperature stress effect |
| kf | fracture permeability (m2) | σij | stress tensor (Pa) |
| df | fracture aperture (m) | Fi | body force (Pa) |
| Qf | flow exchange between the fracture plane and the rock matrix (W) | μs | shear modulus, also known as the second parameter of Lame |
| n | direction of the fracture plane | λ | Lame’s first parameter |
| ef | fracture plane volume strain | ui,jjuj,ji | displacement (m) |
| ▽τ | derivative along the tangential direction of the fracture | E | elastic modulus (Pa) |
| ρs | density of the rock matrix (kg/m3) | ν | Poisson’s ratio |
| λs | thermal conductivity of the rock matrix (W/(m∙K)) | αB | Biot coupling coefficient |
| w | heat source (W/m3) | βT | thermal expansion factor |
| cs | specific heat capacity of the rock matrix (J/(kg∙K)) | αT | thermal expansion coefficient (1/K) |
| Ts | block temperature (K) | Ts | rock temperature (K) |
| cf | specific heat capacity of water (J/(kg∙K)) | T0 | bedrock initial temperature (°C) |
| λf | thermal conductivity of water (W/(m∙K)) | Tin | fracture water initial temperature (°C) |
| uf | fracture water velocity (m/s) | ϕh | borehole porosity |
| Tf | fracture water temperature (K) | ϕr | rock mass porosity |
| Wf | heat absorbed by the fracture plane water from the bedrock (W/m3) | Φf | porosity of fractures 1 and 2 |
References
- Deng, F.; Pei, P.; Li, W.; Wang, L.; Ren, Y. Study on design and calculation method of borehole heat exchangers based on seasonal patterns of groundwater. Renew. Energy 2024, 220, 119711. [Google Scholar] [CrossRef]
- Wang, M. Groundwater and Geological Environment in Karst Area of Guizhou Province; Geological Publishing House: Beijing, China, 2015; ISBN 9787116094093. [Google Scholar]
- Molson, J.W.; Frind, E.O.; Palmer, C.D. Thermal energy storage in an unconfined aquifer 2. Model development, validation and application. Water Resour. Res. 1992, 28, 2857–2867. [Google Scholar] [CrossRef]
- Yoshioka, M.; Takakura, S.; Uchida, Y. Estimation of groundwater flow from temperature monitoring in a borehole heat exchanger during a thermal response test. Hydrogeol. J. 2017, 26, 853–867. [Google Scholar] [CrossRef]
- Dehkordi, S.; Schincariol, R. Effect of thermal-hydrogeological and borehole heat exchanger properties on performance and impact of vertical closed-loop geothermal heat pump systems. Hydrogeol. J. 2014, 22, 89–203. [Google Scholar] [CrossRef]
- Wang, Y.; Liu, Z.; Yuan, X.; Niu, X.; Liu, J. Investigation of the influence of groundwater seepage on the heat transfer characteristics of a ground source heat pump system with a 9-well group. Build. Simul. 2019, 12, 857–868. [Google Scholar] [CrossRef]
- Wang, Y. Three-Dimensional simulation and Experimental Study on U-Vertical Buried Pipes with Groundwater Runoff Variation. Urban Geol. 2018, 13, 41–50. [Google Scholar]
- Capozza, A.; Carli, M.D.; Zarrella, A. Investigations on the influence of aquifers on the ground temperature in ground-source heat pump operation. Appl. Energy 2013, 107, 350–363. [Google Scholar] [CrossRef]
- Samson, M.; Dallaire, J.; Gosselin, L. Influence of groundwater flow on cost minimization of ground coupled heat pump systems. Geothermics 2018, 73, 100–110. [Google Scholar] [CrossRef]
- Zeng, Z.; Xu, Y.; Lv, H.; Fan, L.; Mo, H.; Qin, H. Experimental study on ground source heat pump air conditioning system in karst area. J. Guangxi Univ. (Nat. Sci. Ed.) 2017, 42, 260–267. [Google Scholar]
- Zhu, D.; Diao, N.; Yu, M. Ground Heat Exchanger with Multi-boreholes Simplified Heat Transfer Research based on the Seepage Model. Build. Energy Environ. 2016, 35, 21–24+84. [Google Scholar]
- Li, Y.; Mao, J.; Geng, S.; Zhang, H.; Han, X. Analyis and Optimization of Multi-boreholes based on moving finite line source model. Acta Energiae Solaris Sin. 2015, 36, 1287–1293. [Google Scholar]
- Bonte, M.; Stuyfzand, P.J.; Hulsmann, A.; Beelen, P.V. Underground Thermal Energy Storage: Environmental Risks and Policy Developments in the Netherlands and European Union. Ecol. Soc. 2011, 16, 22. [Google Scholar] [CrossRef]
- Saner, D.; Juraske, R.M.; Kübert, B.P.; Hellweg, S.; Bayer, P. Is it only CO2 that matters A life cycle perspective on shallow geothermal systems. Renew. Sustain. Energy Rev. 2010, 14, 1798–1813. [Google Scholar] [CrossRef]
- Butscher, C.; Huggenberger, P.; Auckenthaler, A.; Bänninger, D. Risikoorientierte Bewilligung von ErdwrmesondenRisk-oriented approval of borehole heat exchangers. Grundwasser 2011, 16, 13–24. [Google Scholar] [CrossRef]
- Klotzbücher, T.; Kappler, A.; Straub, K.L.; Haderlein, S.B. Biodegradability and groundwater pollutant potential of organic anti-freeze liquids used in borehole heat exchangers. Geothermics 2007, 36, 348–361. [Google Scholar] [CrossRef]
- Hähnlein, S.; Bayer, P.; Ferguson, G.; Blum, P. Sustainability and policy for the thermal use of shallow geothermal energy. Energy Policy 2013, 59, 914–925. [Google Scholar] [CrossRef]
- Yang, G.; Ge, M. Research on group arrangement method of ground heat exchanger under coupled thermal conduction and groundwater seepage conditions. Renew. Energy 2014, 32, 1182–1187. [Google Scholar]
- Fleuchaus, P.; Blum, P. Damage event analysis of vertical ground source heat pump systems in Germany. Geotherm. Energy 2017, 5, 5–10. [Google Scholar] [CrossRef]
- Zhu, K.; Fang, L.; Diao, N.; Fang, Z. Potential underground environmental risk caused by GSHP systems. Procedia Eng. 2017, 205, 1477–1483. [Google Scholar] [CrossRef]
- Anbergen, H.; Frank, J.; Mueller, L.; Sass, I. Freeze-Thaw-Cycles on Borehole Heat Exchanger Grouts: Impact on the Hydraulic Properties. Geotech. Test J. 2014, 37, 639–651. [Google Scholar] [CrossRef]
- Allan, M.L.; Philippacopoulos, A.J. Ground water protection issues with geothermal heat pumps. Geotherm. Resour. Counc. Trans. 1999, 23, 101–105. [Google Scholar]
- Sun, P.; Yang, D.; Chen, Y. Introduction to Coupling Models for Multiphysics and Numerical Simulations; China Science and Technology Press: Beijing, China, 2007; ISBN 9787504646347. [Google Scholar]
- Chen, Y.; Lei, B.; Li, X.; Yang, C. Flow-Numerical Simulation of the Stability of A solid Tailing Coupling. Ind. Saf. Environ. Prot. 2020, 46, 4–6+12. [Google Scholar]
- Zhu, C.; Shan, X.; Zhang, G.; Liu, Q.; Jiao, Z. Three-dimensional thermo-hydro-mechanical coupled modeling of thermal anomalies before the 2008 Wenchuan earthquake. Geosci. J. 2020, 24, 689–699. [Google Scholar] [CrossRef]
- Bai, B. Research on Hydrodynamic Parameters in Rock Reservoir and Optimization of Geothermal System; China University of Petroleum (East China): Qingdao, China, 2018. [Google Scholar]
- Fan, Z. Study on In-Situ Stress of Tight Reservoir under Multi-Field Coupling; China University of Petroleum (East China): Qingdao, China, 2017. [Google Scholar]
- Xin, Y. Numerical Investigation of EGS with Coupling Method Based Fractal Characteristics of Fracture System; China University of Petroleum (East China): Qingdao, China, 2019. [Google Scholar]
- Salciarin, D.; Ronchi, F.; Claudio, R.; Tamagnini, C. Thermo-hydro-mechanical response of a large piled raft equipped with energy piles: A parametric study. Acta Geotech. 2017, 12, 703–728. [Google Scholar] [CrossRef]
- Laloui, L.; Nuth, M.; Vulliet, L. Experimental and numerical investigations of the behaviour of a heat exchanger pile. Int. J. Numer. Anal. Methods Geomech. 2010, 30, 763–781. [Google Scholar] [CrossRef]
- Donna, A.D.; Laloui, L. Numerical analysis of the geotechnical behaviour of energy piles. Int. J. Numer. Anal. Methods Geomech. 2015, 39, 861–888. [Google Scholar] [CrossRef]
- Sun, Z.; Zhang, X.; Xu, Y.; Yao, J.; Wang, H.; Lv, S.; Sun, Z.; Huang, Y.; Cai, M.; Huang, X. Numerical simulation of the heat extraction in EGS with thermal-hydraulic-mechanical coupling method based on discrete fractures model. Energy 2017, 120, 20–33. [Google Scholar] [CrossRef]
- Qin, F. Heat Transfer Process and Optimization of Enhanced Geothermal System (EGS) Based on Heat Flow-Solid Coupling Analysis; Kunming University of Science and Technology: Kunming, China, 2020. [Google Scholar]
- Yao, C.; Shao, Y.; Yang, J.; Huang, F.; He, C.; Jiang, Q.; Zhou, C. Effects of fracture density, roughness, and percolation of fracture network on heat-flow coupling in hot rock masses with embedded three-dimensional fracture network. Geothermics 2020, 87, 101846. [Google Scholar] [CrossRef]
- Dan, Z. Numerical Simulation Study of the Wellbore Heat Transfer and the Thermal-Hydraulic Coupling in Random Fracture Reservoirs; Northeast Petroleum University: Daqing, China, 2021. [Google Scholar]
- Kavanaugh, S.; Rafferty, K. Geothermal Heating and Cooling: Design of Ground-Source Heat Pump Systems; ASHRAE: Peachtree Corners, GA, USA, 2014. [Google Scholar]
- Jiang, W.; Zhou, H.; Li, Y.; Liu, W.; Gong, C.; Ge, X. Permeability Measurement of Karst Fractured Media Based on Groundwater Velocity and Direction Within a Borehole. Saf. Environ. Eng. 2018, 25, 1–7+18. [Google Scholar]
- Deng, F.; Pei, P.; Ren, Y.; Luo, T.; Chen, Y. Investigation and evaluation methods of shallow geothermal energy considering the influences of fracture water flow. Geotherm. Energy 2023, 11, 25. [Google Scholar] [CrossRef]









| Symbol | Parameter/Unit | Value | Symbol | Parameter/Unit | Value |
|---|---|---|---|---|---|
| ρs | Rock mass density/(kg∙m−3) | 2700 | uf | Fracture water velocity/(m∙s−1) | 0.01 |
| cs | Specific heat capacity of rock mass/(W∙m−1∙K−1) | 1000 | μ | Dynamic viscosity of fracture water/(Pa∙s) | 0.001 |
| λs | Thermal conductivity of rock mass/(W∙m−1∙K−1) | 3.0 | df | Fracture aperture/(m) | 0.0015 |
| ρfw | Fracture water density/(kg∙m−3) | 1000 | T0 | Initial temperature rock/(°C) | 35 |
| cfw | Specific heat capacity of fracture water y/(J∙kg−1∙K−1) | 4200 | Tin | Initial temperature of fracture water/(°C) | 15 |
| Symbol | Parameters/Unit | Value | Symbol | Parameters/Unit | Value |
|---|---|---|---|---|---|
| dj | Inner diameter of buried pipe/mm | 26 | ϕr | Rock mass porosity/1 | 0.01 |
| λu | Thermal conductivity of U-pipe/(W∙m−1∙K−1) | 0.42 | λf | Thermal conductivity of fractures 1 and 2/(W∙m−1∙K−1) | 3.0 |
| ϕh | Porosity in borehole/1 | 0.15 | cs | Specific heat capacity of rock mass/(J∙kg−1∙K−1) | 850 |
| λa | Thermal conductivity of borehole/(W∙m−1∙K−1) | 2.8 | ks | Rock mass permeability/m2 | 10−15 |
| ca | Specific heat capacity of borehole/(J∙kg−1∙K−1) | 820 | ν | Poisson’s ratio of rock mass/1 | 0.25 |
| ka | Permeability of borehole/m2 | 10−9 | kf | Permeability of fractures 1 and 2/(m∙s−1) | 1 × 10−9 |
| ρa | Density of borehole/kg∙m−3 | 2500 | Φf | Porosity of fractures 1 and 2/1 | 0.5 |
| Es | Young’s modulus of rock mass/Pa | 7 × 1010 | ρf | Fracture fillings density/kg∙m−3 | 2300 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ran, Y.; Peng, J.; Tian, X.; Luo, D.; Yang, B.; Pei, P.; Tang, L. Numerical Study of the Thermo-Hydro-Mechanical Coupling Impacts of Shallow Geothermal Borehole Groups in Fractured Rock Mass on Geological Environment. Energies 2024, 17, 1384. https://doi.org/10.3390/en17061384
Ran Y, Peng J, Tian X, Luo D, Yang B, Pei P, Tang L. Numerical Study of the Thermo-Hydro-Mechanical Coupling Impacts of Shallow Geothermal Borehole Groups in Fractured Rock Mass on Geological Environment. Energies. 2024; 17(6):1384. https://doi.org/10.3390/en17061384
Chicago/Turabian StyleRan, Yujin, Jia Peng, Xiaolin Tian, Dengyun Luo, Bin Yang, Peng Pei, and Long Tang. 2024. "Numerical Study of the Thermo-Hydro-Mechanical Coupling Impacts of Shallow Geothermal Borehole Groups in Fractured Rock Mass on Geological Environment" Energies 17, no. 6: 1384. https://doi.org/10.3390/en17061384
APA StyleRan, Y., Peng, J., Tian, X., Luo, D., Yang, B., Pei, P., & Tang, L. (2024). Numerical Study of the Thermo-Hydro-Mechanical Coupling Impacts of Shallow Geothermal Borehole Groups in Fractured Rock Mass on Geological Environment. Energies, 17(6), 1384. https://doi.org/10.3390/en17061384







