Numerical Simulation Study on the Gas–Solid Flow Characteristics of a Large-Scale Dual Fluidized Bed Reactor: Verification and Extension
Abstract
1. Introduction
2. CFD Model
2.1. Simulation Objects
2.1.1. Verification
2.1.2. Extension
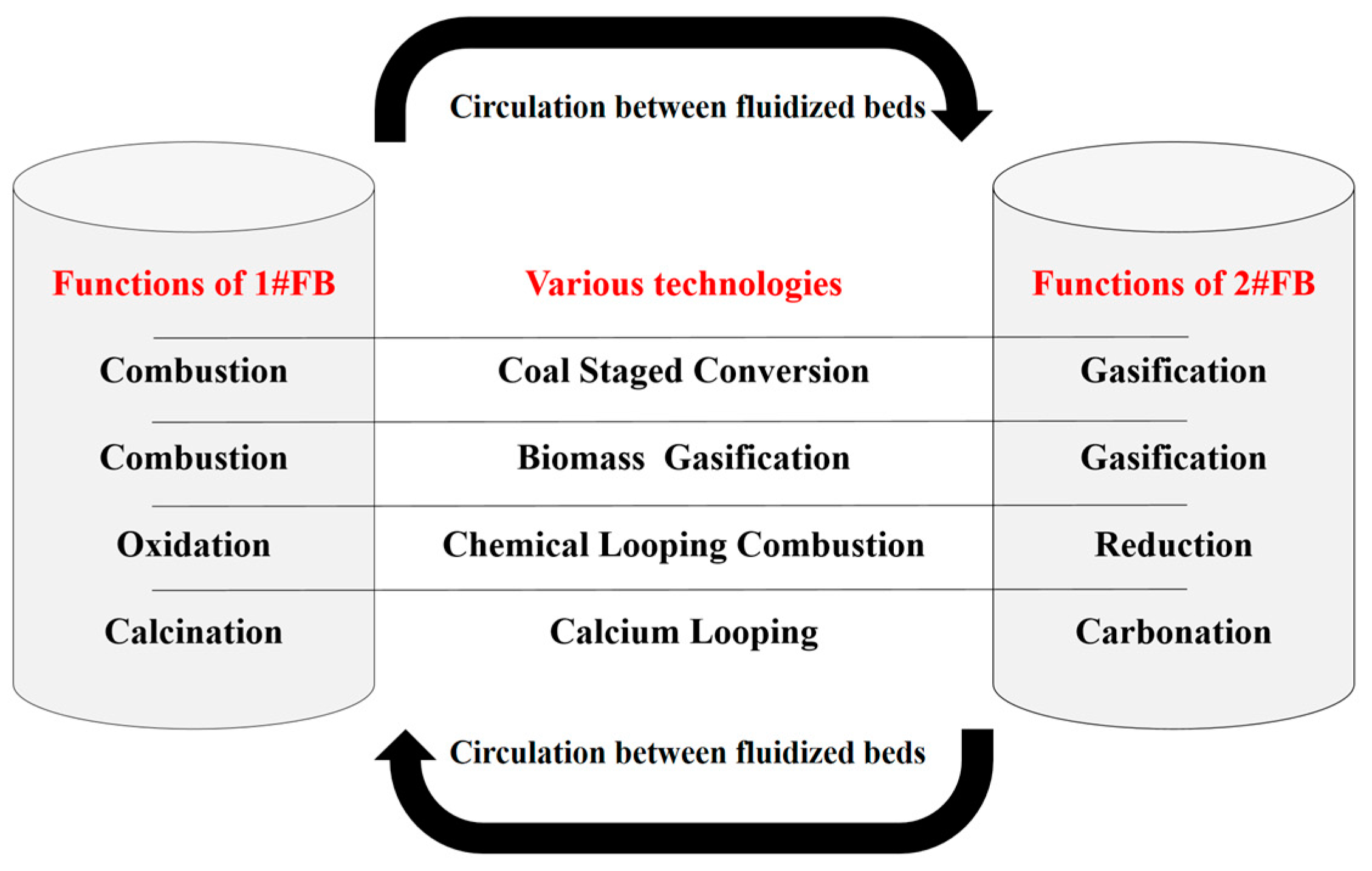
2.2. Model Structure
2.3. Simulation Considerations
2.4. Governing Equation
2.4.1. Continuity Equation
2.4.2. Momentum Equation
2.4.3. RNG k-ε Model
2.4.4. EMMS Model
3. Results and Discussion
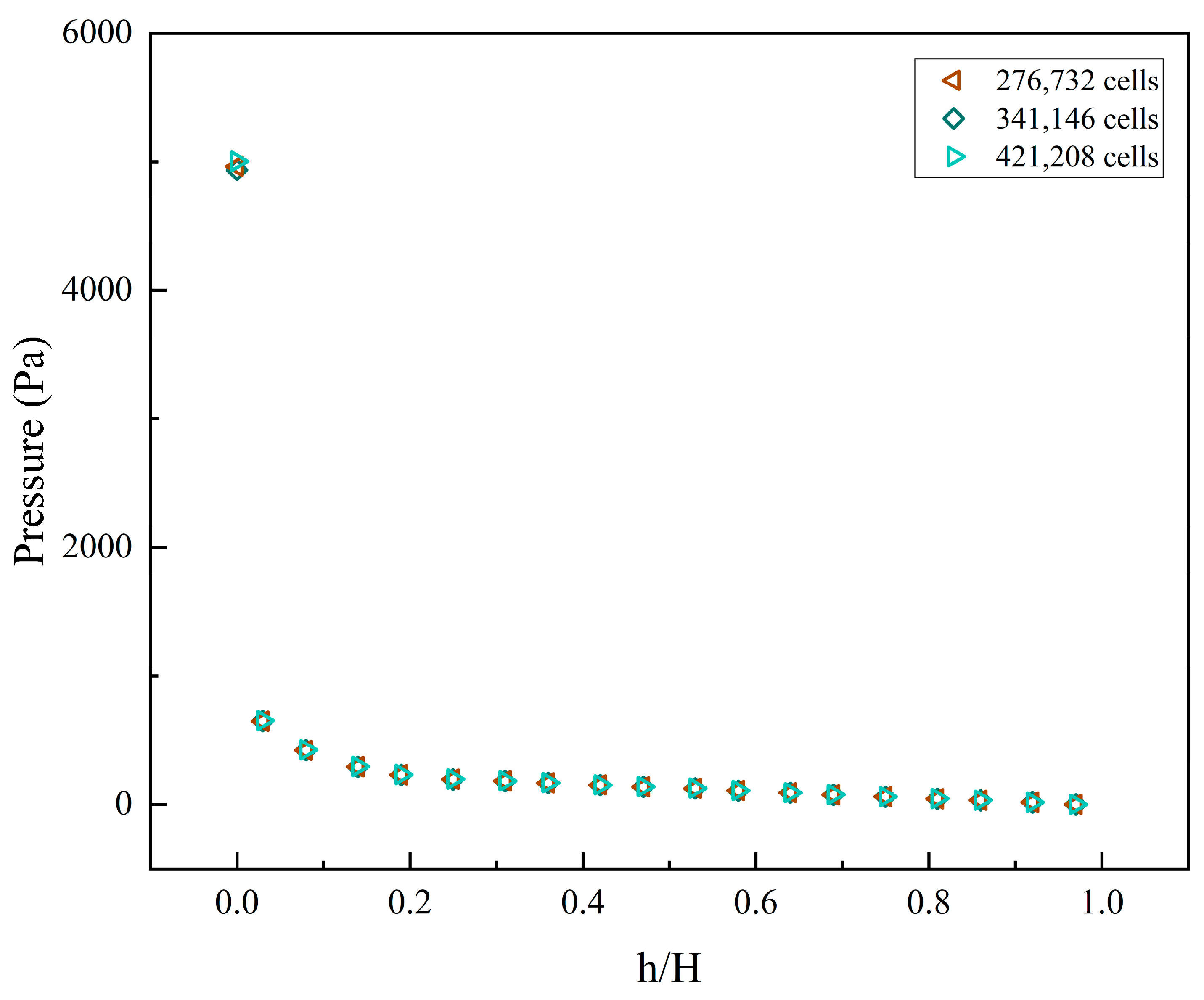
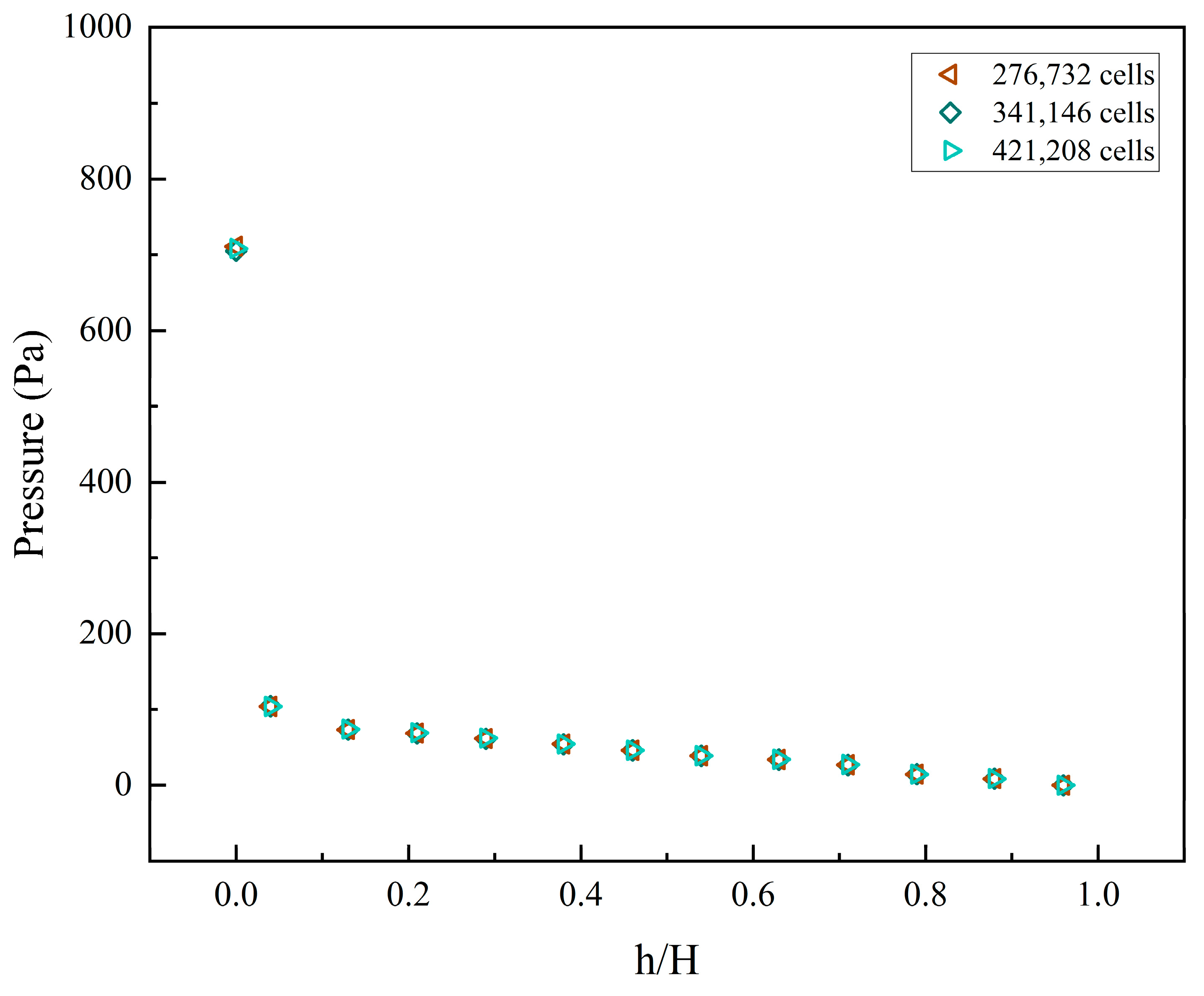
3.1. The Effect of Grid Resolution
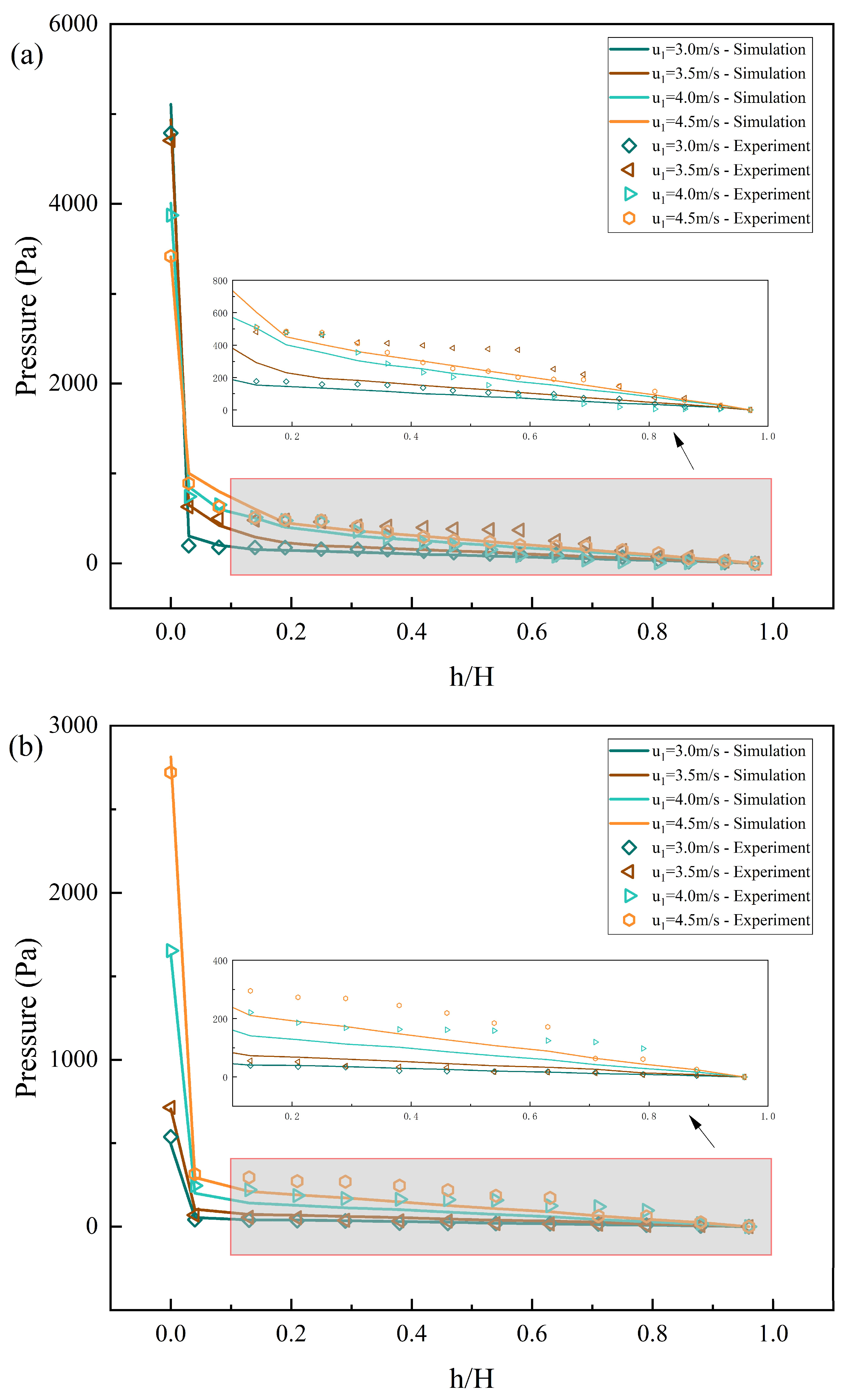
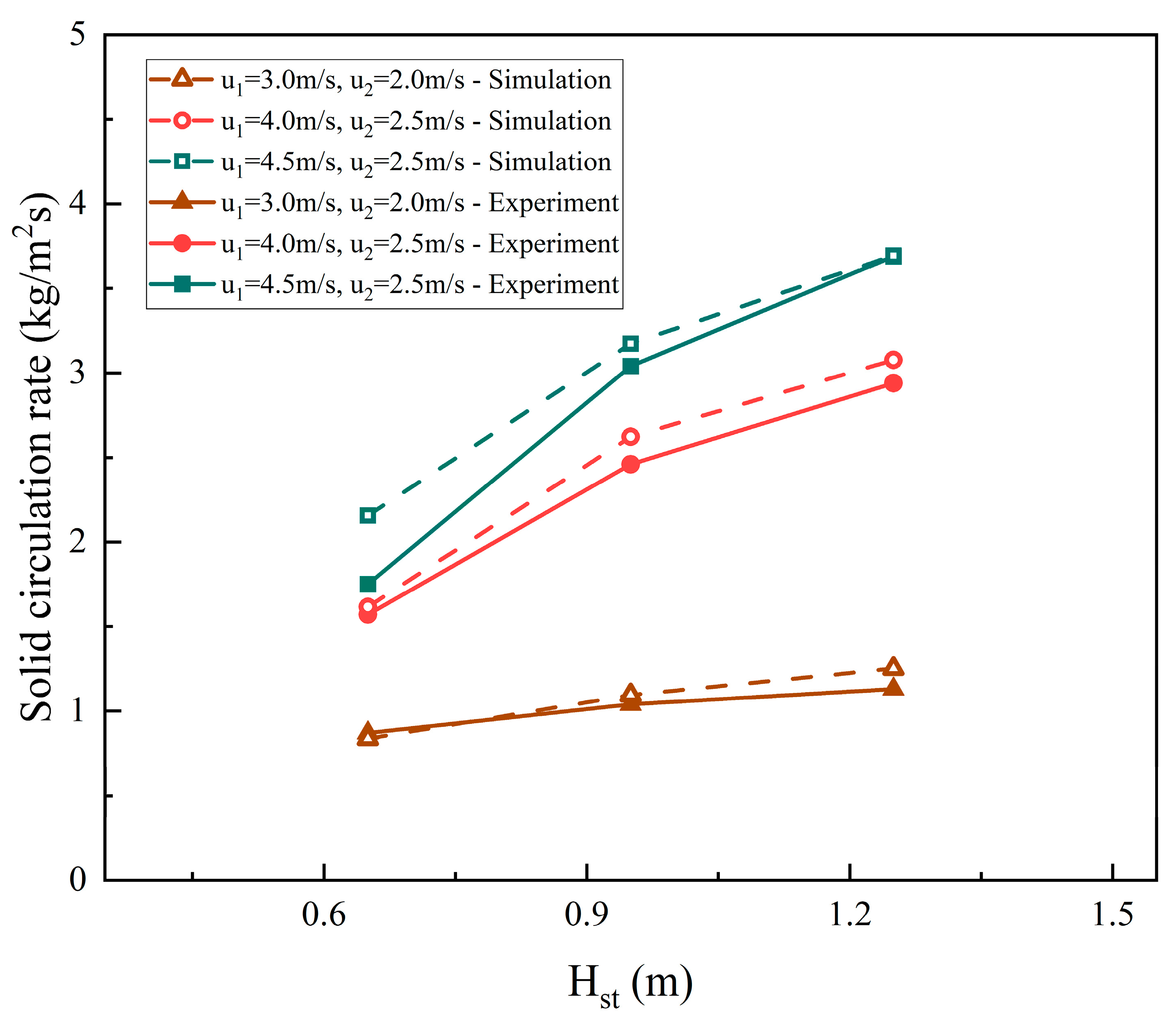
3.2. Verification
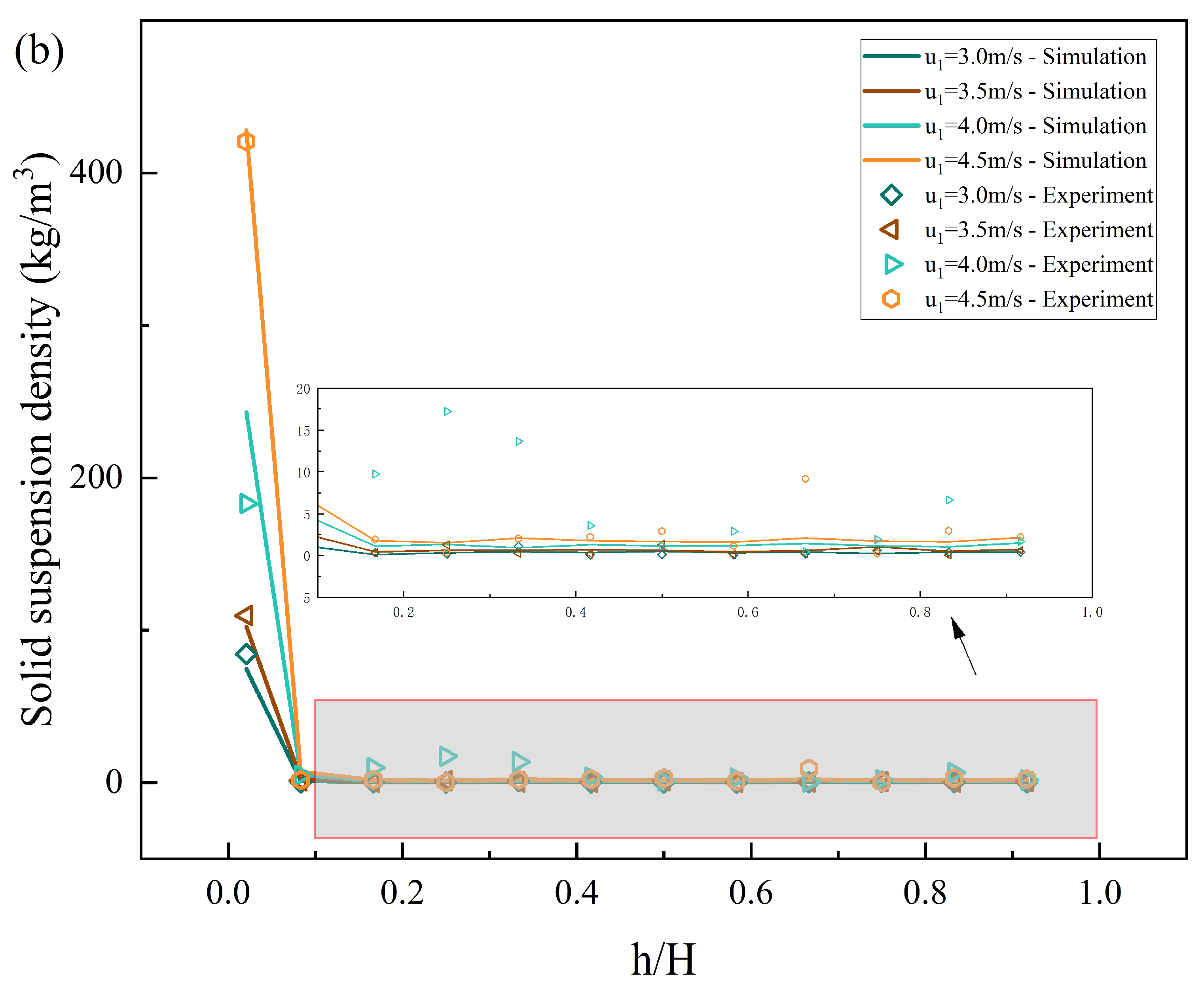
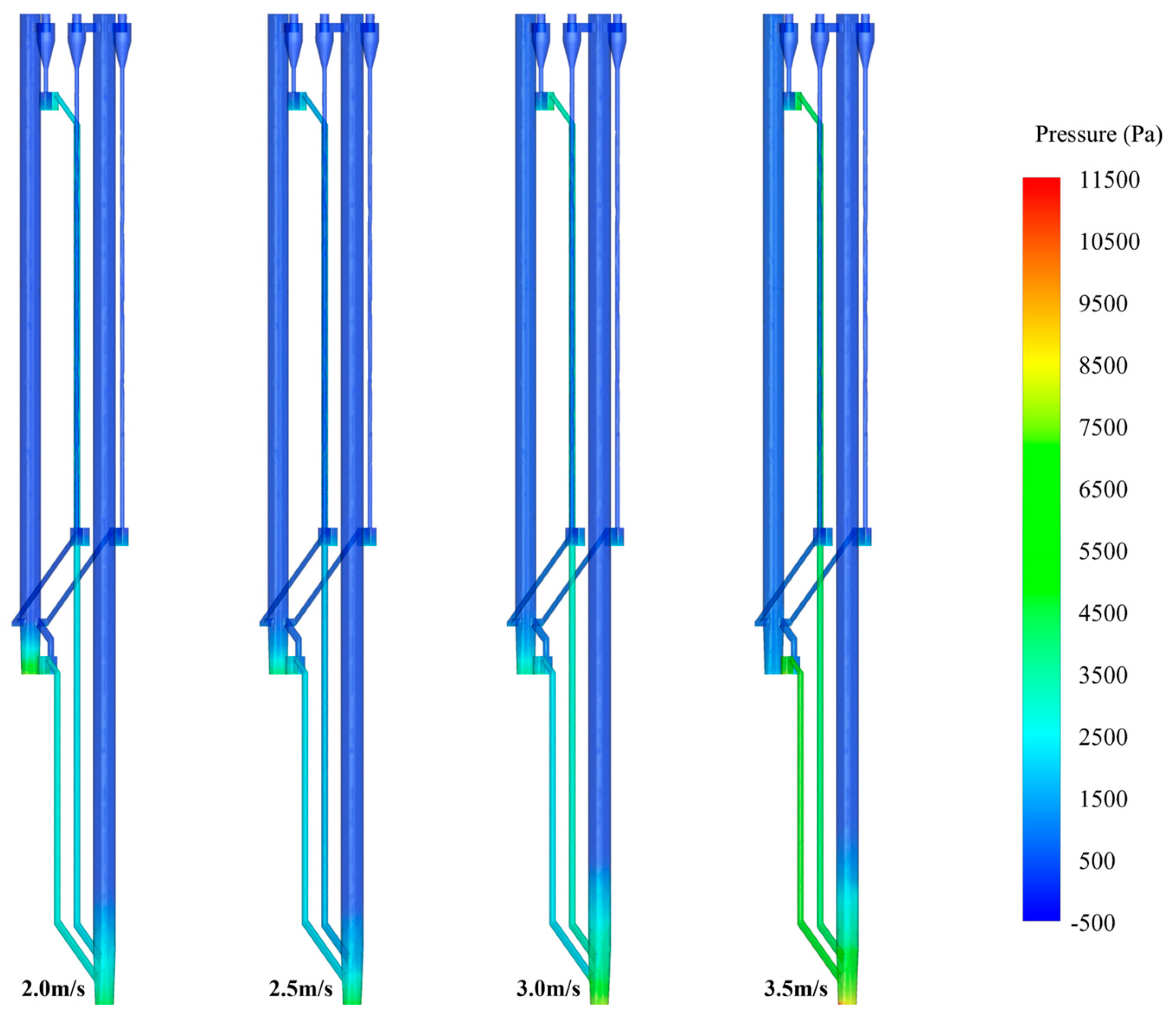
3.2.1. The Effects of Superficial Gas Velocity of 1#FB on Gas–Solid Flow Characteristics
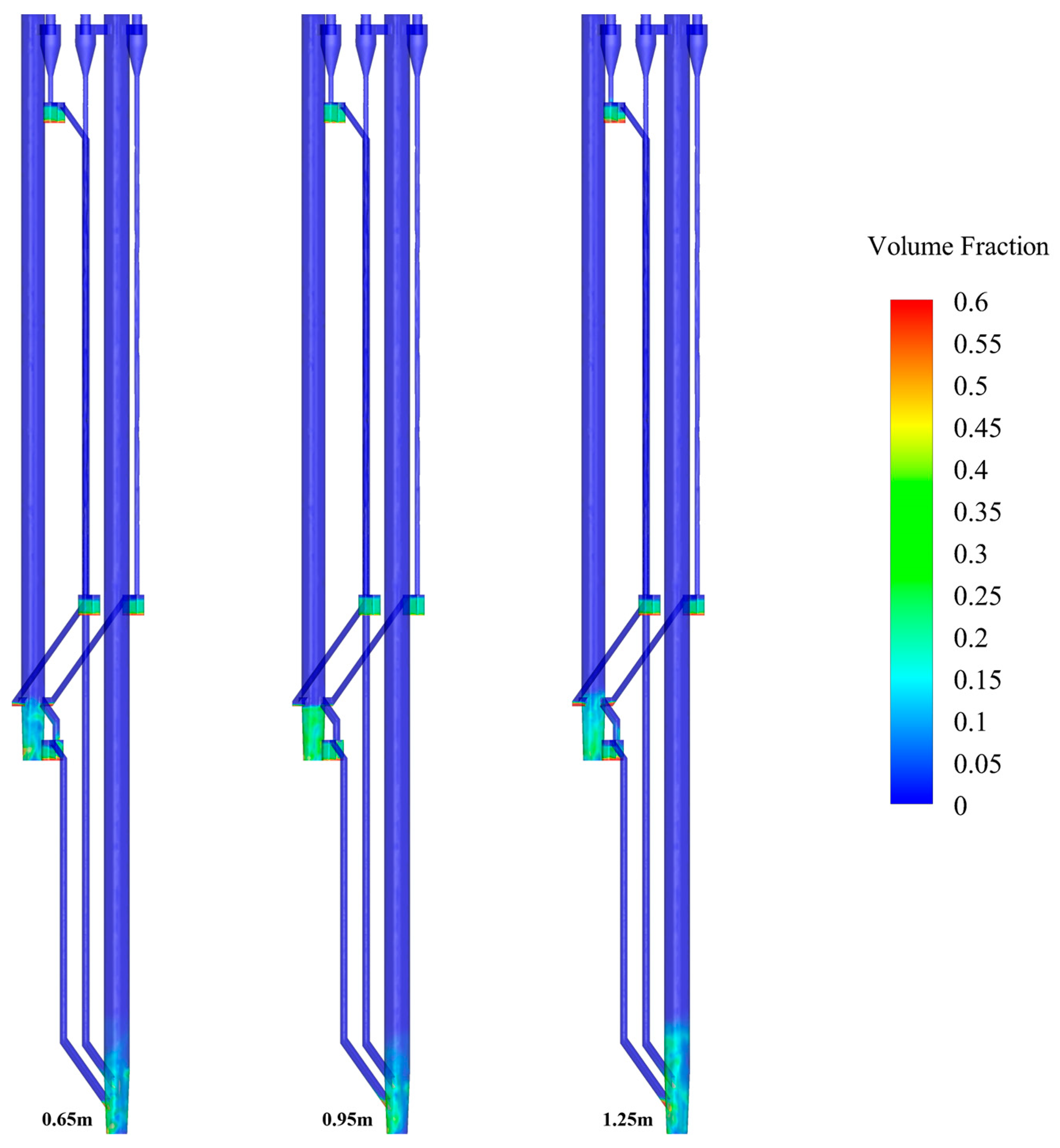
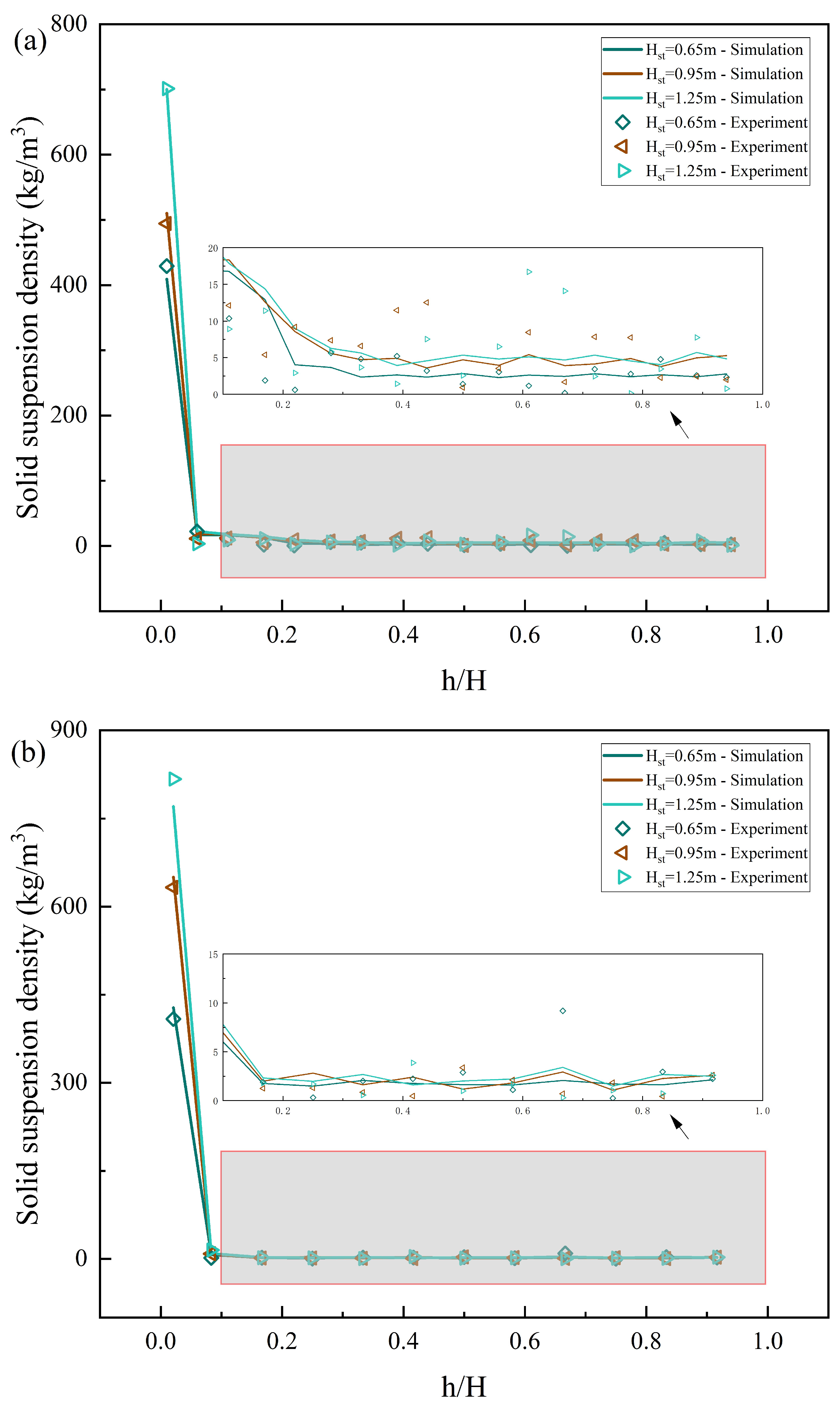
3.2.2. The Effects of Static Bed Height on Gas–Solid Flow Characteristics
3.3. Extension
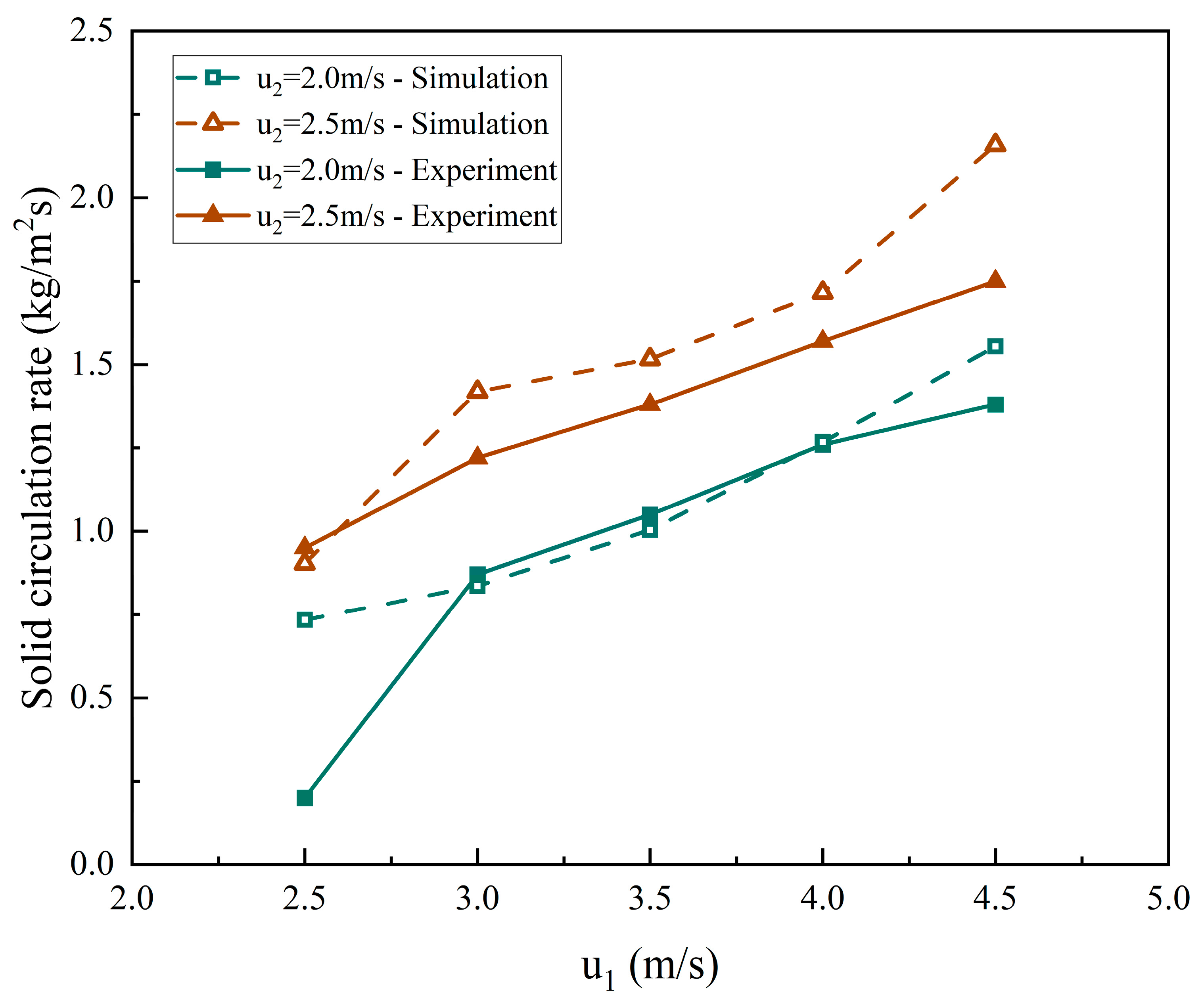
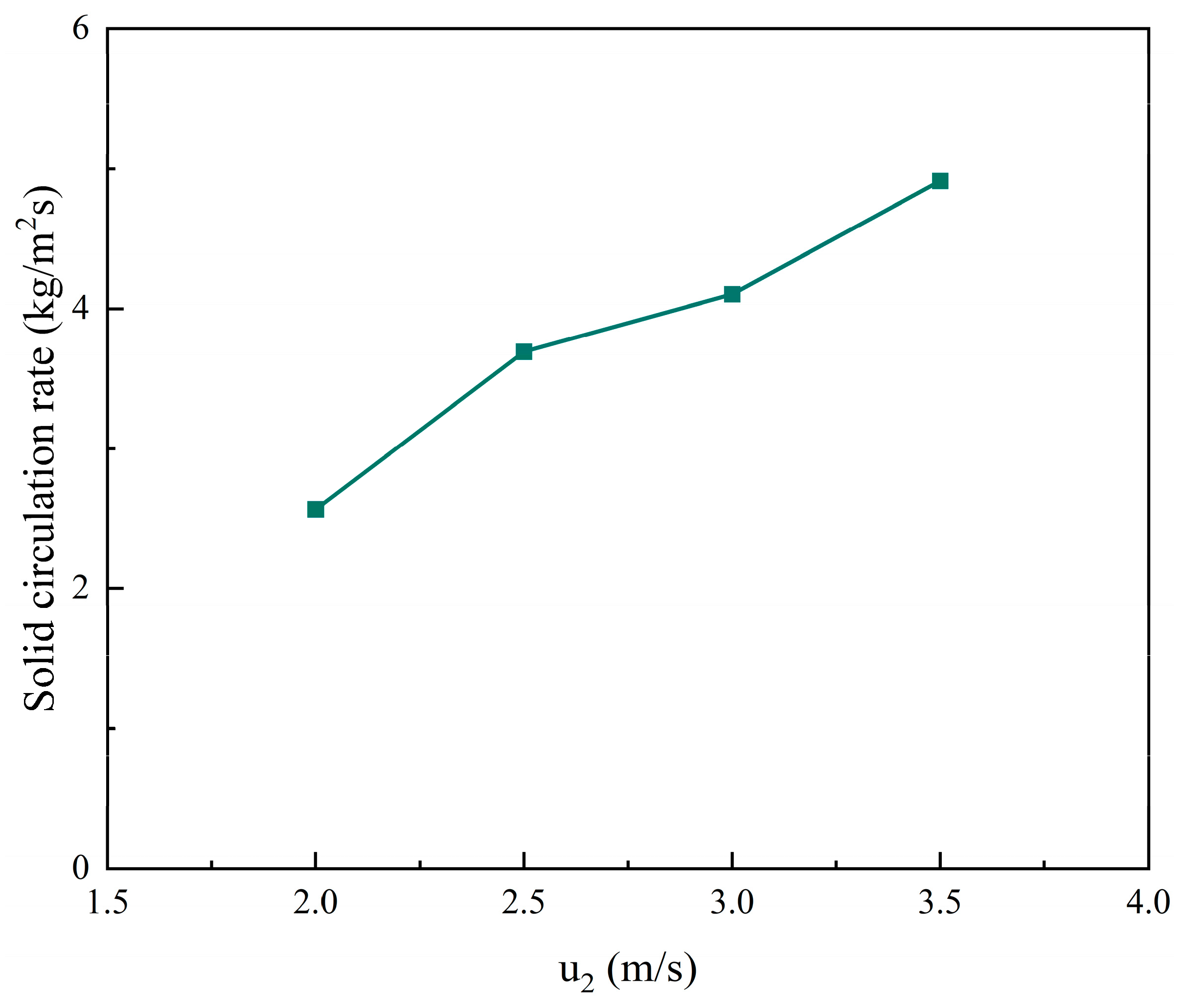
3.3.1. The Effects of Superficial Gas Velocity of 2#FB on Gas–Solid Flow Characteristics
3.3.2. The Effects of Static Bed Height on Gas–Solid Flow Characteristics
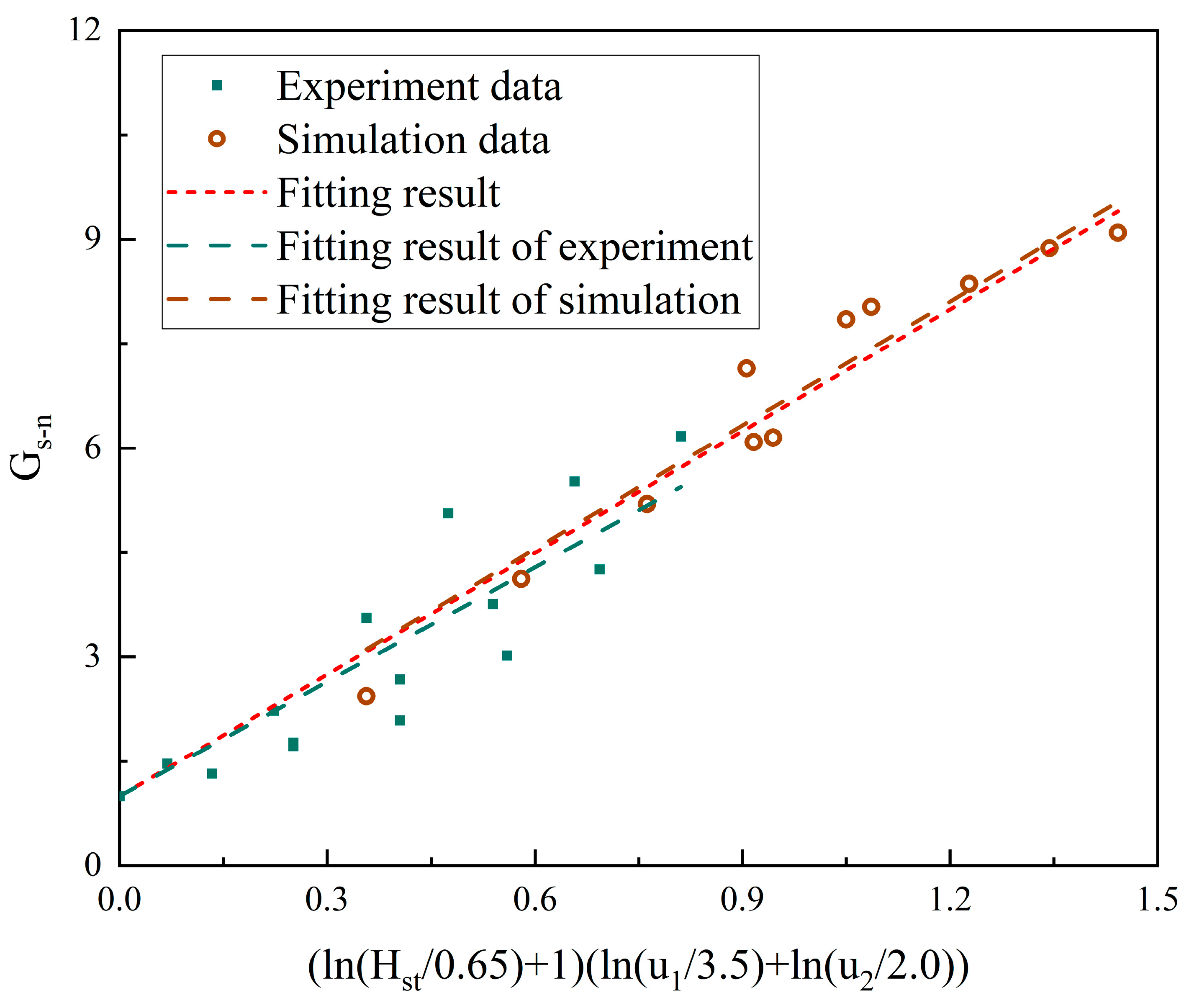
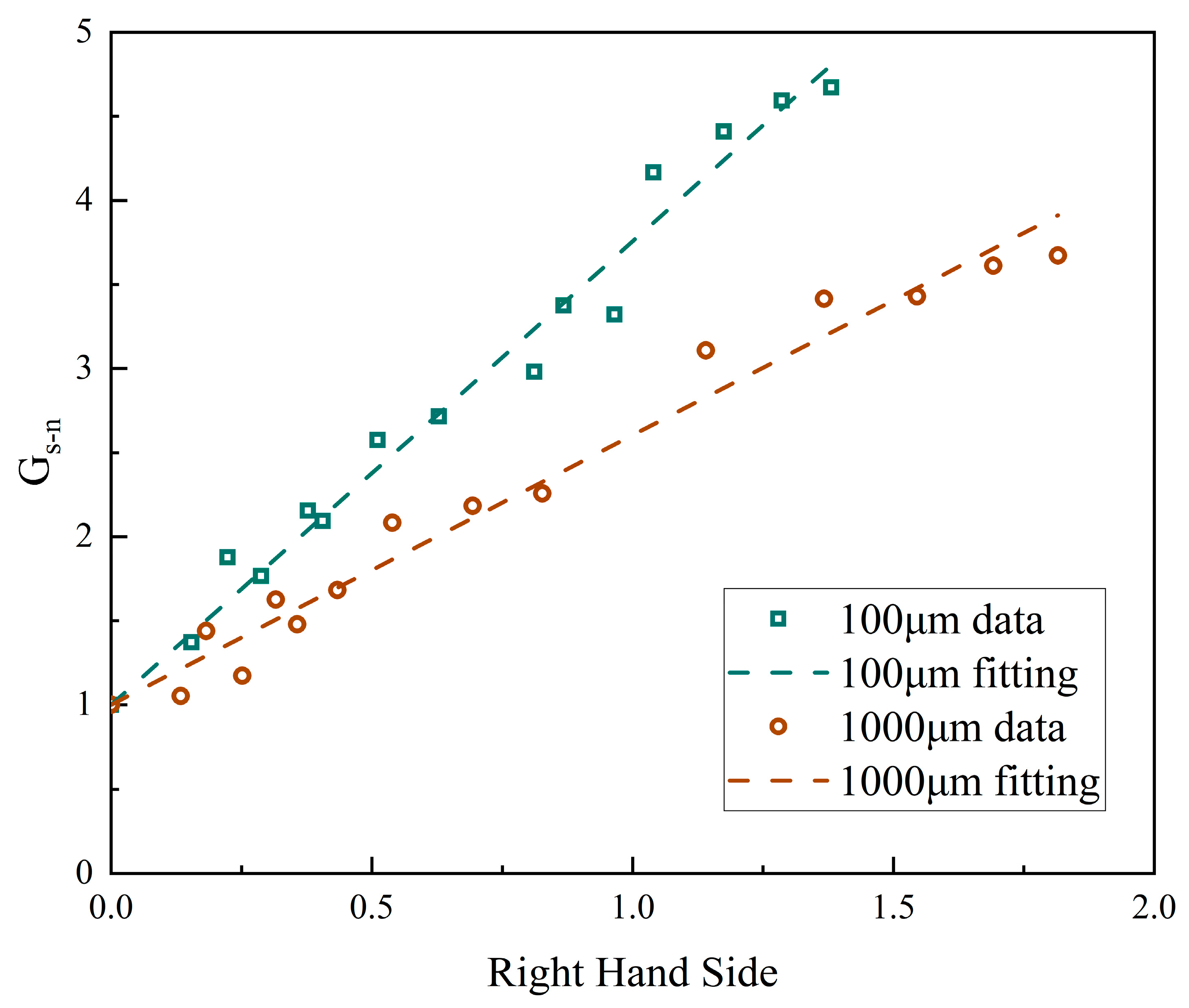
3.3.3. Empirical Formulas of Solid Circulation Rate
4. Conclusions
- The simulation results are in good agreement with the experimental data in terms of the trend, and the specific values are generally similar. Therefore, the numerical model is scientific.
- The superficial gas velocity of 1#FB, the superficial gas velocity of 2#FB, and the static bed height all have an effect on the gas–solid characteristics. The effects of the superficial gas velocity on the pressure and solid suspension density of the two furnaces are the opposite, while the static bed height has the same effect on the two furnaces. At the same time, the influences of different parameters on the gas–solid flow characteristics have marginal decreasing effects.
- According to the simulation results, the empirical formula of the solid circulation rate was fitted according to the different particle sizes. It was found that the influence of particle size on the solid circulation rate could reach three levels of magnitude, and it is the most significant among all of the parameters.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| Symbols | |
| a | inertial term, m/s2 |
| C | constant in the turbulence model |
| CD | drag coefficient |
| ds | particle diameter, m |
| dcl | cluster diameter, m |
| f | volume fraction |
| Gb | generation term of turbulent kinetic energy caused by buoyancy |
| Gk | generation term of turbulent kinetic energy caused by the mean velocity gradient |
| gravitational acceleration, m/s2 | |
| H | total height of the system, m |
| Hst | static bed height, m |
| unit tensor | |
| Kij | interphase momentum exchange coefficient from phase i to j |
| k | turbulent kinetic energy, m2/s2 |
| Nst | mass-specific energy consumption for suspending and transporting particles, W/kg |
| NT | mass-specific total energy consumption for particles, W/kg |
| p | pressure, Pa |
| t | time, s |
| u | superficial gas velocity, m/s |
| Uslip | superficial slip velocity, m/s |
| v or | velocity, m/s |
| Ym | contribution of pulsation expansion in compressible turbulence |
| Greek Letters | |
| α | volume fraction |
| αk | reciprocal of effective Prandtl number for turbulent kinetic energy |
| αε | reciprocal of effective Prandtl number for dissipation rate of turbulent kinetic energy |
| ε | dissipation rate of turbulent kinetic energy |
| εc | voidage of dense phase in the EMMS model |
| εf | voidage of dilute phase in the EMMS model |
| λ | bulk viscosity, Pa s |
| μ | viscosity, Pa s |
| μeff | effective viscosity, Pa s |
| ρ | density, kg/m3 |
| stress tensor, Pa | |
| Subscripts | |
| 1 | 1#FB |
| 2 | 2#FB |
| c | dense phase in the EMMS model |
| f | dilute phase in the EMMS model |
| g | gas phase |
| i | meso-scale interphase in the EMMS model |
| mf | minimum fluidization |
| s | solid phase |
References
- Shrestha, S.; Ali, B.S.; Binti Hamid, M.D. Cold Flow Model of Dual Fluidized Bed: A Review. Renew. Sustain. Energy Rev. 2016, 53, 1529–1548. [Google Scholar] [CrossRef]
- Gupta, S.; De, S. Investigation of Hydrodynamics and Segregation Characteristics in a Dual Fluidized Bed Using the Binary Mixture of Sand and High-Ash Coal. Adv. Powder Technol. 2021, 32, 2690–2702. [Google Scholar] [CrossRef]
- Hanchate, N.; Ramani, S.; Mathpati, C.S.; Dalvi, V.H. Biomass Gasification Using Dual Fluidized Bed Gasification Systems: A Review. J. Clean. Prod. 2021, 280, 123148. [Google Scholar] [CrossRef]
- Abuelgasim, S.; Wang, W.; Abdalazeez, A. A Brief Review for Chemical Looping Combustion as a Promising CO2 Capture Technology: Fundamentals and Progress. Sci. Total Environ. 2021, 764, 142892. [Google Scholar] [CrossRef]
- Chen, J.; Duan, L.; Ma, Y.; Jiang, Y.; Huang, A.; Zhu, H.; Jiao, H.; Li, M.; Hu, Y.; Zhou, H.; et al. Recent Progress in Calcium Looping Integrated with Chemical Looping Combustion (CaL-CLC) Using Bifunctional CaO/CuO Composites for CO2 Capture: A State-of-the-Art Review. Fuel 2023, 334, 126630. [Google Scholar] [CrossRef]
- Xu, J.; Lu, X.; Wang, Q.; Zhang, W.; Liu, C.; Xie, X.; Sun, S.; Fan, X.; Li, J. Visualization of Gas-Solid Flow Characteristics at the Wall of a 60-Meter-High Transparent CFB Riser. Powder Technol. 2018, 336, 180–190. [Google Scholar] [CrossRef]
- Bian, Q.; Yang, D.; Wu, Z.; Liu, B.; Zhang, J.; Deng, R.; Pu, H. Investigation of Particle Flow Characteristics in a CFB Recirculation System Using Electrical Capacitance Volume Tomography. Powder Technol. 2022, 408, 117765. [Google Scholar] [CrossRef]
- Stollhof, M.; Penthor, S.; Mayer, K.; Hofbauer, H. Estimation of the Solid Circulation Rate in Circulating Fluidized Bed Systems. Powder Technol. 2018, 336, 1–11. [Google Scholar] [CrossRef]
- Youn, P.-S.; Lee, D.H.; Kim, D.; Won, Y.S.; Choi, J.-H.; Joo, J.B.; Ryu, H.-J. Solids Inventory and External Solids Circulation Rate in Risers of Circulating Fluidized Beds. Adv. Powder Technol. 2022, 33, 103810. [Google Scholar] [CrossRef]
- Pröll, T.; Rupanovits, K.; Kolbitsch, P.; Bolhàr-Nordenkampf, J.; Hofbauer, H. Cold Flow Model Study on a Dual Circulating Fluidized Bed (DCFB) System for Chemical Looping Processes. Chem. Eng. Technol. 2009, 32, 418–424. [Google Scholar] [CrossRef]
- Zhou, C.; Chen, L.; Liu, C.; Wang, J.; Xing, X.; Liu, Y.; Chen, Y.; Chao, L.; Dai, J.; Zhang, Y.; et al. Interconnected Pyrolysis and Gasification of Typical Biomass in a Novel Dual Fluidized Bed. Energy Convers. Manag. 2022, 271, 116323. [Google Scholar] [CrossRef]
- Wang, F.; Zhou, W.; Chen, S.; Duan, L.; Xiang, W. Investigations on Biomass Gasification of Compact-Fast Dual Fluidized Bed Calcium Looping. J. Clean. Prod. 2023, 405, 137065. [Google Scholar] [CrossRef]
- Ohlemüller, P.; Alobaid, F.; Abad, A.; Adanez, J.; Ströhle, J.; Epple, B. Development and Validation of a 1D Process Model with Autothermal Operation of a 1 MW Th Chemical Looping Pilot Plant. Int. J. Greenh. Gas Control 2018, 73, 29–41. [Google Scholar] [CrossRef]
- Zhu, Y.; Li, K.; Wang, Q.; Cen, J.; Fang, M.; Luo, Z. Low-Rank Coal Pyrolysis Polygeneration Technology with Semi-Coke Heat Carrier Based on the Dual-Fluidized Bed to Co-Produce Electricity, Oil and Chemical Products: Process Simulation and Techno-Economic Evaluation. Fuel Process. Technol. 2022, 230, 107217. [Google Scholar] [CrossRef]
- Sun, L.; Xiao, R.; Lin, J.; Kong, D.; Luo, K.; Fan, J. Numerical Investigation of a 1MW Full-Loop Chemical Looping Combustion Unit with Dual CFB Reactors. Int. J. Greenh. Gas Control 2023, 123, 103835. [Google Scholar] [CrossRef]
- Lin, J.; Luo, K.; Hu, C.; Sun, L.; Fan, J. Full-Loop Simulation of a 1 MWth Pilot-Scale Chemical Looping Combustion System. Chem. Eng. Sci. 2022, 249, 117301. [Google Scholar] [CrossRef]
- Yakhot, V.; Orszag, S.A. Renormalization-Group Analysis of Turbulence. Phys. Rev. Lett. 1986, 57, 1722–1724. [Google Scholar] [CrossRef] [PubMed]
- Ahn, S.-H.; Xiao, Y.; Wang, Z.; Luo, Y.; Fan, H. Unsteady Prediction of Cavitating Flow around a Three Dimensional Hydrofoil by Using a Modified RNG K-ε Model. Ocean Eng. 2018, 158, 275–285. [Google Scholar] [CrossRef]
- Al-Hanaya, A.; El-Sapa, S.; Ashmawy, E.A. Axisymmetric motion of an incompressible couple stress fluid between two eccentric rotating spheres. J. Appl. Mech. Tech. Phy. 2022, 63, 790–798. [Google Scholar] [CrossRef]
- El-Sapa, S.; Albalawi, W. Interaction of Two Rigid Spheres Oscillating in an Infinite Liquid under the Control of a Magnetic Field. J. Appl. Math. 2023, 2023, 1146872. [Google Scholar] [CrossRef]
- Li, J.; Tung, Y.; Kwauk, M. Method of energy minimization in multi-scale modeling of particle-fluid two-phase flow. In Circulating Fluidized Bed Technology; Elsevier: Amsterdam, The Netherlands, 1988; pp. 89–103. ISBN 978-0-08-036225-0. [Google Scholar]
- Li, J.; Tung, Y.; Kwauk, M. Energy Transport and Regime Transition in Particle-Fluid Two-Phase Flow. In Circulating Fluidized Bed Technology; Elsevier: Amsterdam, The Netherlands, 1988; pp. 75–87. ISBN 978-0-08-036225-0. [Google Scholar]
- Ghadirian, E.; Arastoopour, H. CFD Simulation of a Fluidized Bed Using the EMMS Approach for the Gas-Solid Drag Force. Powder Technol. 2016, 288, 35–44. [Google Scholar] [CrossRef]
- Dymala, T.; Wytrwat, T.; Heinrich, S. MP-PIC Simulation of Circulating Fluidized Beds Using an EMMS Based Drag Model for Geldart B Particles. Particuology 2021, 59, 76–90. [Google Scholar] [CrossRef]
- Yang, Y.; Zhang, Q.; Pan, T.; Qu, Y.; Liu, X.; Li, X.; Cheng, Y.; Wang, L.; Liu, W. The Hydrodynamics of Low-Density Particles and Optimization of the EMMS Calculation Process. Powder Technol. 2022, 405, 117511. [Google Scholar] [CrossRef]
- Pakseresht, P.; Yao, Y.; Fan, Y.; Theuerkauf, J.; Capecelatro, J. A Critical Assessment of the Energy Minimization Multi-Scale (EMMS) Model. Powder Technol. 2023, 425, 118569. [Google Scholar] [CrossRef]











| Item | Value |
|---|---|
| The height of 1#FB | 21.60 m |
| The cross-sectional area of 1#FB | 0.30 m × 0.40 m |
| The height of 2#FB | 14.40 m |
| The cross-sectional area of 2#FB | 0.25 m × 0.40 m |
| The relative height difference of the inlets | 7.20 m |
| The design of furnace inlet | Tapered |
| Item | Settings |
|---|---|
| Density of sand | 2600 kg/m3 |
| Diameter of sand | 100, 282, 641, 1000 μm |
| Static bed height | 0.65/0.95/1.25/1.55/1.85/2.15 m |
| Inlets | Velocity-inlet type, 3.0/3.5/4.0/4.5 m/s for 1#FB; 2.0/2.5/3.0/3.5 m/s for 2#FB |
| Outlets | Pressure-outlet type, −50 Pa for both |
| Elements of mesh | 341,146 |
| Average quality of mesh | 0.83 |
| Boundary condition of wall | No-slip |
| Restitution coefficient | 0.95 |
| Packing limit | 0.6 |
| Specularity coefficient | 0.01 |
| Time step size | 0.001 s |
| Residual tolerance | 0.001 |
| Viscous model | RNG k-ε (Cμ = 0.0845, C1ε = 1.42, C2ε = 1.68, C3ε = 1.3, Pr = 0.75) |
| Turbulence multiphase model | Per phase |
| Solid shear viscosity | Syamlal-O’Brien |
| Granular bulk viscosity | Lun et al. |
| Solid pressure | Lun et al. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, Y.; Wang, Q.; Xie, G.; Fang, M.; Luo, Z. Numerical Simulation Study on the Gas–Solid Flow Characteristics of a Large-Scale Dual Fluidized Bed Reactor: Verification and Extension. Energies 2024, 17, 1304. https://doi.org/10.3390/en17061304
Lin Y, Wang Q, Xie G, Fang M, Luo Z. Numerical Simulation Study on the Gas–Solid Flow Characteristics of a Large-Scale Dual Fluidized Bed Reactor: Verification and Extension. Energies. 2024; 17(6):1304. https://doi.org/10.3390/en17061304
Chicago/Turabian StyleLin, Yubin, Qinhui Wang, Guilin Xie, Mengxiang Fang, and Zhongyang Luo. 2024. "Numerical Simulation Study on the Gas–Solid Flow Characteristics of a Large-Scale Dual Fluidized Bed Reactor: Verification and Extension" Energies 17, no. 6: 1304. https://doi.org/10.3390/en17061304
APA StyleLin, Y., Wang, Q., Xie, G., Fang, M., & Luo, Z. (2024). Numerical Simulation Study on the Gas–Solid Flow Characteristics of a Large-Scale Dual Fluidized Bed Reactor: Verification and Extension. Energies, 17(6), 1304. https://doi.org/10.3390/en17061304








