Pressure Transient Analysis on the Condenser of the HPR1000 Nuclear Power Unit
Abstract
1. Introduction
2. Numerical Model
2.1. Model Simplification Hypothesis
- (1)
- Due to the low operating pressure of the condenser, the air inside the condenser is considered an ideal gas;
- (2)
- Besides the water in the hot well, the working fluid in the steam side of the condenser is in a saturated state;
- (3)
- The temperature of the steam and air mixture in the steam side of the condenser is equal to the saturation temperature at the corresponding pressure;
- (4)
- The velocity and mass flow of the condenser circulating cooling water are evenly distributed on the tube side;
- (5)
- The water temperature change rate of the circulating cooling water in the condenser is uniform;
- (6)
- The change in metal mass, specific heat capacity, and temperature of the condenser heat exchange tube are uniform;
- (7)
- The heat dissipation of the condenser is 0, and the amount of steam extracted from the vacuum equipment is 0.
2.2. Establishment of the Mathematical Model [21]
2.2.1. Condenser Shell-Side Mathematical Model
- (1)
- Mathematical model of the steam zone
- is the steam storage capacity of the condenser, kg;
- is the exhaust steam mass of the steam turbine, kg/s;
- is the flash water added into the condenser, kg/s;
- is the dynamic evaporation amount of the hot well water surface, kg/s;
- is the dynamic condensation amount of the hot well water surface, kg/s;
- is the main amount of steam to condensate, kg/s.
- is the steam partial pressure, MPa;
- is the amount of steam in the condenser. The model uses the total volume of the shell side minus the space occupied by the hot well water for the calculation, kg;
- is the gas constant of the steam, 461.5 kJ/(kg·K);
- is the average temperature of the steam, °C;
- is the volume of steam in the condenser, m3.
- is the enthalpy of steam storage in the condenser, kJ/kg;
- is the exhaust steam enthalpy of the steam turbine, kJ/kg;
- is the enthalpy of saturated water in the condenser, kJ/kg;
- is the enthalpy of saturated steam in the condenser, kJ/kg.
- (2)
- Mathematical model of the air zone
- is the air partial pressure, MPa;
- is the amount of air in the condenser, kg;
- is the gas constant of air, 287.05 J/(kg·K);
- is the average temperature of the air, its value equal to the temperature of the steam in this paper, °C;
- is the volume of gas in the condenser, m3.
- is the normal amount of air leaking into the condenser, kg/s;
- is the amount of air entering the condenser for the vacuum damage valve, kg/s;
- is the amount of air that the shaft seal leaks into the condenser, kg/s;
- is the mixture of steam and gas pumped out of a vacuum pump, kg/s;
- is the mass fraction of air in the condenser.
- is the total amount of air, kg/s;
- is the amount of steam entering the condenser, kg/s.
- (3)
- Mathematical model of the hot water well area
- is the hot water well quality, kg;
- is the drain water amount of the low-pressure heater, kg/s;
- is the drain water amount of the high-pressure heater, kg/s;
- is the leakage of the cooling water tube; it was taken as 0, kg/s;
- is the water output of the condenser through the condensate pump, kg/s.
- is the cross-sectional area of the condenser hot well, m2.
- is the heat dissipation from the hot well to the environment, kW;
- is the heat dissipation coefficient of the hot well to the environment;
- is the enthalpy of the saturated steam storage in the condenser, kJ/kg;
- is the enthalpy of the water output of the condenser through the condensate pump, kJ/kg.
2.2.2. Condenser Tube Side Mathematical Model
- (1)
- Calculation of the metal wall temperature of the cooling water tube
- is the steam condensation heat, kW;
- is the heat of the cooling water absorption, kW;
- is the cooling water tube metal quality, kg;
- is the specific volume of the cooling water tube metal, kJ/(kg·K).
- (2)
- Calculation of the cooling water outlet temperature
- is the heat of the cooling water absorption, kW;
- is the cooling water flow rate, kg/s;
- is the amount of water stored in the cooling water tube, kg;
- is the specific volume of the cooling water, kJ/(kg·K);
- is the temperature of the outlet cooling water, °C;
- is the temperature of the inlet cooling water, °C.
2.3. The Scope and Data of the Mathematical Model
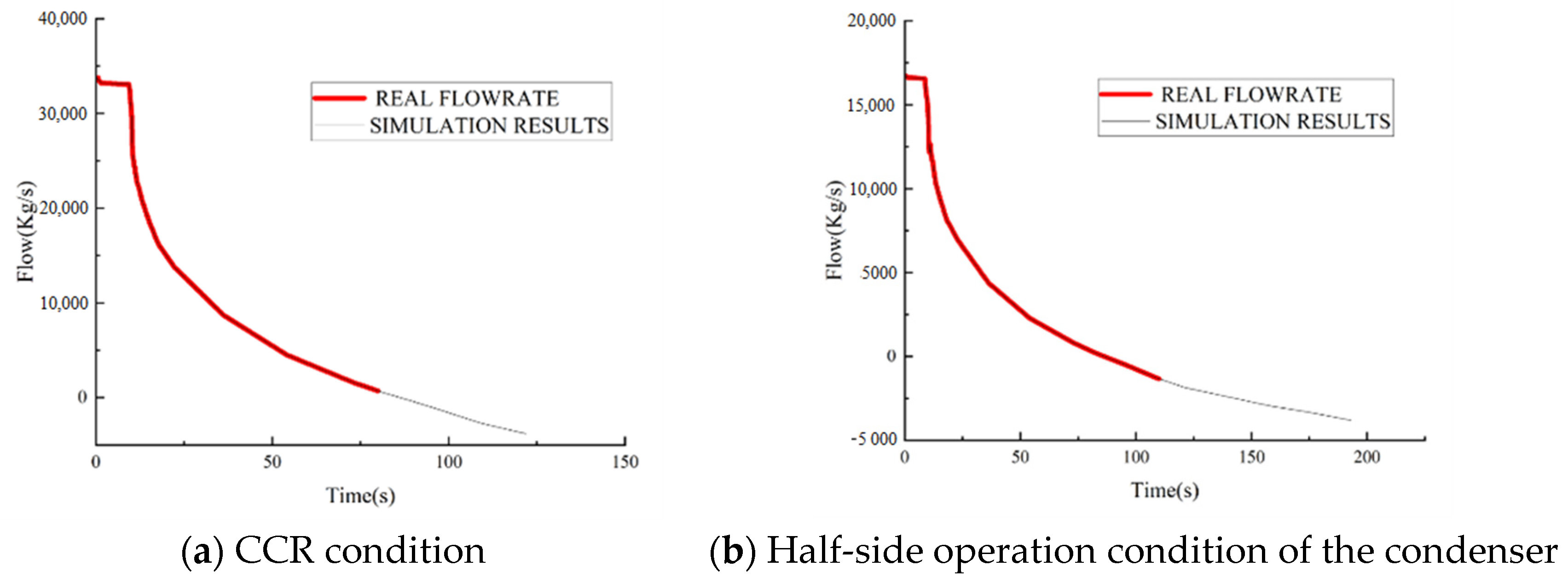
3. Verification of the Numerical Model
4. Calculated Results and Analysis
4.1. Initial Conditions and Transient Sequence
4.2. Calculation of the Boundary Conditions and Assumptions
- (1)
- In the calculation process, all kinds of drains entering the condenser are considered to maintain the full power unchanged;
- (2)
- The remaining steam of the low pressure cylinder of the steam turbine is all discharged into the condenser;
- (3)
- The circulating water pump starts to stop at 0 s, considering the change of the circulating water pumps idle;
- (4)
- After the turbine tripping, the condensate pump is shut down without considering the idleness of the condensate pump, and water flow is instantaneously at 0;
- (5)
- After the turbine tripping, the vacuum pump is shut down without considering idle running;
- (6)
- The model needs to consider the influence of the steam side volume of the condenser on the vacuum;
- (7)
- Without considering the heat dissipation of the condenser shell due to the temperature rise, the metal heat storage inside the condenser should be considered;
- (8)
- According to the steam turbine bypass steam system, two sets of valves open at the same time calculation;
- (9)
- When the steam turbine bypass valve opens, the bypass steam flow reaches the maximum flow of the valve and maintains this until the end of the simulation;
- (10)
- According to the calculation assumptions (8) and (9), the pressure changes of the two condensers are consistent during the simulation process, and the model is only simulated for one.
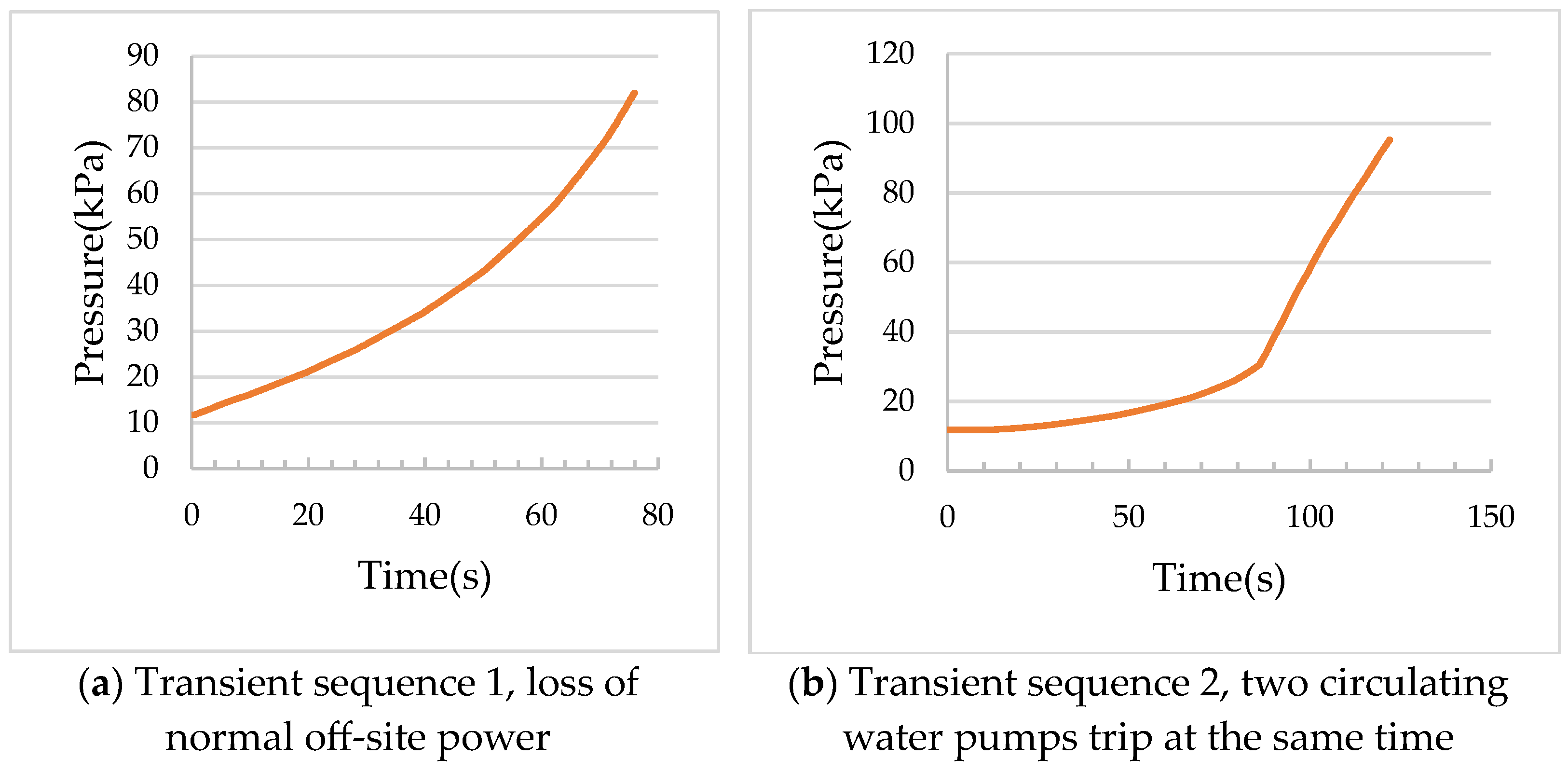
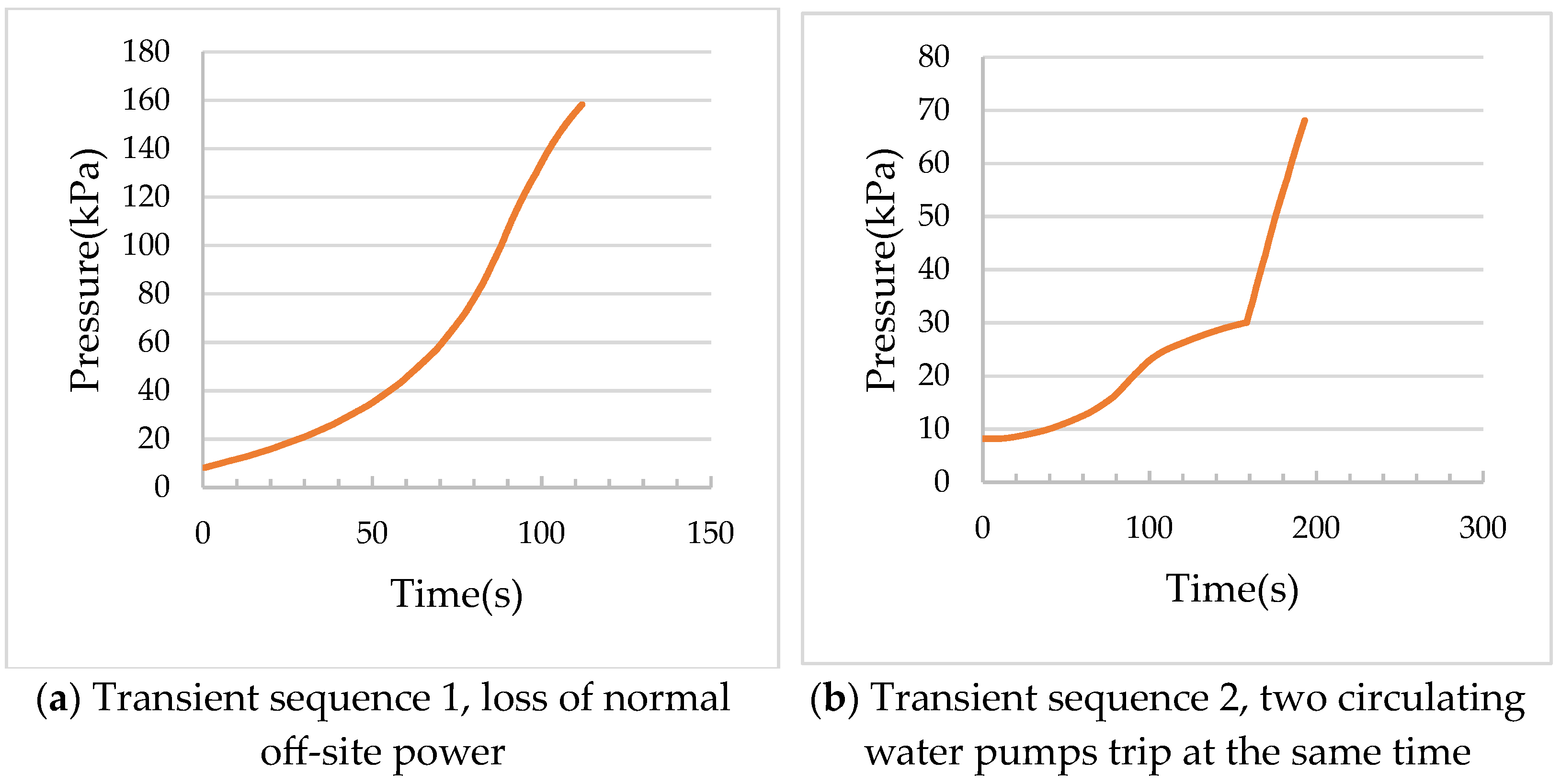
4.3. Analysis of the Simulation Results under Different Transient Conditions
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Sunder Raj, K.S. Deviations in predicted condenser performance for power plants using HEI correction factors: A case study. Eng. Gas Turbines Power 2008, 130, 023003. [Google Scholar] [CrossRef]
- Patankar, G.; Weibel, J.A.; Garimella, S.V. Patterning the condenser-side wick in ultra-thin vapor chamber heat spreaders to improve skin temperature uniformity of mobile devices. Int. J. Heat Mass Transf. 2016, 101, 927–936. [Google Scholar] [CrossRef]
- Ormiston, S.J.; Raithby, G.D.; Carlucci, L.N. Numerical modeling of power station steam condensers-Part 1: Convergence behavior of a finite-volume model. Numer. Heat Transf. 1995, 27, 81–102. [Google Scholar] [CrossRef]
- Botsch, T.W.; Stephan, K.; Alcock, J.L.; Webb, D.R. Modelling and simulation of the dynamic behavior of a shell-and-tube condenser. Int. J. Heat Mass Transf. 1997, 40, 4137–4149. [Google Scholar] [CrossRef]
- Oh, S.; Revankar, S.T. Experimental and theoretical investigation of film condensation with non-condensable gas. Int. J. Heat Mass Transf. 2006, 49, 2523–2534. [Google Scholar] [CrossRef]
- Wang, G.; Mao, X.; Hu, G.; Tian, Z.P. Development of A quasi-three-dimensional computer program for fluid flow fields and heat transfer performance of power plant condensers: PPOC3.0. Power Eng. 2001, 21, 1450–1454. [Google Scholar]
- Hou, P.; Tao, Z.; Hu, Y.; Qiao, L.; Li, Z.; Su, X. The turbine cold-end optimization of conventional island of inland AP1000 nuclear power plant. Turbine Technol. 2010, 52, 424–426. [Google Scholar]
- Hou, P.; Yan, H. A study of the turbine cold-end optimization method of conventional island double back pressure unit of inland nuclear power plant. J. Qingdao Univ. (Eng. Technol. Ed.) 2012, 27, 88–91. [Google Scholar]
- Guo, H. Simulation study on dynamic characteristics of condenser operating parameters of nuclear power steam turbine. China Meas. Test 2019, 45, 55–60. [Google Scholar]
- Silde, A.; Ylijoki, J.; Ahtinen, E. Containment model library of the Apros process simulation software: An overview of development and validation work. Prog. Nucl. Energy 2019, 116, 28–45. [Google Scholar] [CrossRef]
- Xian, L.; Yan, J.; Zhou, K.; Chen, G.; Dang, G.; Song, D. Analysis of startup process of small modular reactor with OTSGs. Nucl. Eng. Des. 2023, 415, 112669. [Google Scholar]
- Starkloff, R.; Alobaid, F.; Karner, K.; Epple, B.; Schmitz, M.; Boehm, F. Development and validation of a dynamic simulation model for a large coal-fired power plant. Appl. Therm. Eng. 2015, 91, 496–506. [Google Scholar] [CrossRef]
- Szogradi, M.; Norrman, S.; Bubelis, E. Dynamic modelling of the helium-cooled DEMO fusion power plant with an auxiliary boiler in Apros. Fusion Eng. Des. 2020, 160, 111970. [Google Scholar] [CrossRef]
- Al-Maliki WA, K.; Hadi, A.S.; Al-Khafaji, H.M.; Alobaid, F.; Epple, B. Novel feedwater preheating system for parabolic trough solar power plant. Energy Rep. 2022, 8, 10665–10687. [Google Scholar] [CrossRef]
- Patricks, R.P. Pressure Transient Analysis Circulating Cooling Water Pumps (CRF), LOT 24A; Weir Pumps Ltd.: Scotland, UK, 2006. [Google Scholar]
- Jiang, C.; Ding, J. Nuclear power station condenser unavailable calculation and analysis. Nucl. Power Eng. 2009, 30, 39–44. [Google Scholar]
- Wang, X.; Zhou, Z.; Song, Z.; Lu, Q.; Li, J. Transient characteristics of pressure variation in condensers of nuclear power station. J. Xi’an Jiaotong Univ. 2015, 49, 6–10. [Google Scholar]
- Cheng, H.; Wu, Y.; Song, D. HPR1000—Advanced pressurised water reactor with active and passive safety. Nucl. Future J. Inst. Nucl. Eng. Br. Nucl. Energy Soc. 2016, 12, 38–43. [Google Scholar]
- Sun, D.C.; Li, Y.; Xi, Z.; Zan, Y.F.; Li, P.Z.; Zhuo, W.B. Experimental evaluation of safety performance of emergency passive residual heat removal system in HPR1000. Nucl. Eng. Des. 2017, 318, 54–60. [Google Scholar] [CrossRef]
- Lu, C.; Le, Z.; Shen, Y.; Cai, W.; Yang, J.; Ji, W.; Zhang, Y. The prediction of steam generator secondary pH under SGTR condition of HPR1000. Prog. Nucl. Engergy 2021, 134, 103673. [Google Scholar] [CrossRef]
- Zhang, Z. Condenser in Large Scale Power Plant; China Machine Press: Beijing, China, 1993. [Google Scholar]

| Serial Number | Name | Unit | Design Data |
|---|---|---|---|
| Design conditions and performance | |||
| 1 | Condenser pressure under rated condition | kPa(a) | 6.4 |
| 2 | Condenser pressure when turbine trips | kPa(a) | 30 |
| 3 | Condenser pressure when bypass is prohibited from operation | kPa(a) | 60 |
| 4 | Rated temperature of condensate water | °C | 37.366 |
| 5 | Effective heat transfer area of condenser | m2 | 83,000 |
| 6 | Circulating water process | Single process | |
| 7 | Design parameters of circulating water | ||
| a | Heat exchange amount | kJ/s | 1,918,045 |
| b | Circulating water flow rate | m3/s | 68 |
| c | The average velocity of circulating water in the tube bundle | m/s | 2.399 |
| d | Cleanliness | % | 90 |
| e | Circulating water temperature rise | °C | 7 |
| f | The resistance drop of circulating water when it flows through the clean tube bundle and the water chamber | m | 5.2 |
| g | Absolute pressure (based on HEI standard section surface condenser 2.7 chapter) | kPa(a) | 6.4 |
| h | Maximum oxygen content of condensed water | ppb | 20 |
| i | Steam saturation temperature and condensation water temperature difference | °C | 0.5 |
| 8 | Absolute pressure at a bundle cleanliness of 90% | kPa(a) | 6.4 |
| Absolute pressure at 100% cleanliness of tube bundle | kPa(a) | 6.4 | |
| The pressure range is 6.8~33 °C for circulating water temperature | kPa(a) | 4~11.8 |
| Time (s) | Event |
|---|---|
| 0 | Loss of off-site electricity; the steam turbine tripped, and the main steam valve, regulating valve, reheat main steam valve, and reheat regulating valve were consequently closed. The circulating water pump, main feed water pump, and condensate pump trip at the same time. |
| 0.3 | The main steam valve, the regulating valve, the reheat main steam valve, and the reheat regulating valve are completely closed, and the exhaust volume of the steam turbine is reduced to 0 kg/s. |
| 0.5 | The bypass flow rate is 501 kg/s in 0.5 s, and the saturated steam pressure is 8.6 MPa. |
| Time (s) | Event |
|---|---|
| 0 | The circulating water pump trips, the turbine generator continues to operate at rated power, and the main feed water pump and condensate pump do not trip. When the vacuum of the condenser deteriorates and reaches the shutdown value (condenser failure pressure, 30 kPa), the turbine trips. |
| 0.3 s after the unit trip | The main steam valve, governing valve, reheat main steam valve, and reheat governing valve are completely closed, and the turbine exhaust volume is reduced to 0 kg/s. |
| 0.5 s after the unit trip | The bypass flow rate is 501 kg/s in 0.5 s, and the saturated steam pressure is 8.6 MPa. |
| CCR Operating Conditions, The Initial Back Pressure of 11.8 kPa | The Condenser Operates on the Half Side with an Initial Back Pressure of 8.2 kPa | |||
|---|---|---|---|---|
| Condenser status | Main transformer differential protection action, loss of normal external power | Two circulating water pumps tripped at the same time | Main transformer differential protection action, loss of normal external power | Two circulating water pumps tripped at the same time |
| Condenser failure 30 kPa | 34 s | 85.4 s | 43.7 s | 157.6 s |
| Condenser not available 60 kPa | 63.9 s | 100.9 s | 70.8 s | 184.9 s |
| Time interval | 29.9 s | 15.5 s | 27.1 s | 27.3 s |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lu, C.; Yang, J.; Zhang, Q. Pressure Transient Analysis on the Condenser of the HPR1000 Nuclear Power Unit. Energies 2024, 17, 1210. https://doi.org/10.3390/en17051210
Lu C, Yang J, Zhang Q. Pressure Transient Analysis on the Condenser of the HPR1000 Nuclear Power Unit. Energies. 2024; 17(5):1210. https://doi.org/10.3390/en17051210
Chicago/Turabian StyleLu, Chuntian, Jianjun Yang, and Qiang Zhang. 2024. "Pressure Transient Analysis on the Condenser of the HPR1000 Nuclear Power Unit" Energies 17, no. 5: 1210. https://doi.org/10.3390/en17051210
APA StyleLu, C., Yang, J., & Zhang, Q. (2024). Pressure Transient Analysis on the Condenser of the HPR1000 Nuclear Power Unit. Energies, 17(5), 1210. https://doi.org/10.3390/en17051210






