Control of Dielectric Parameters of Micro- and Nanomodified Epoxy Resin Using Electrophoresis
Abstract
1. Introduction
- —particle’s velocity (for constant velocity it is the distance migrated by the particle divided by the migration time).
- —permittivity of the suspending medium, εm = εr·ε0;
- —dielectric constant of the suspending medium;
- —permittivity of the vacuum (8.85 × 10−12 F·m−1);
- —zeta (electrokinetic) potential.
2. Experiment Description
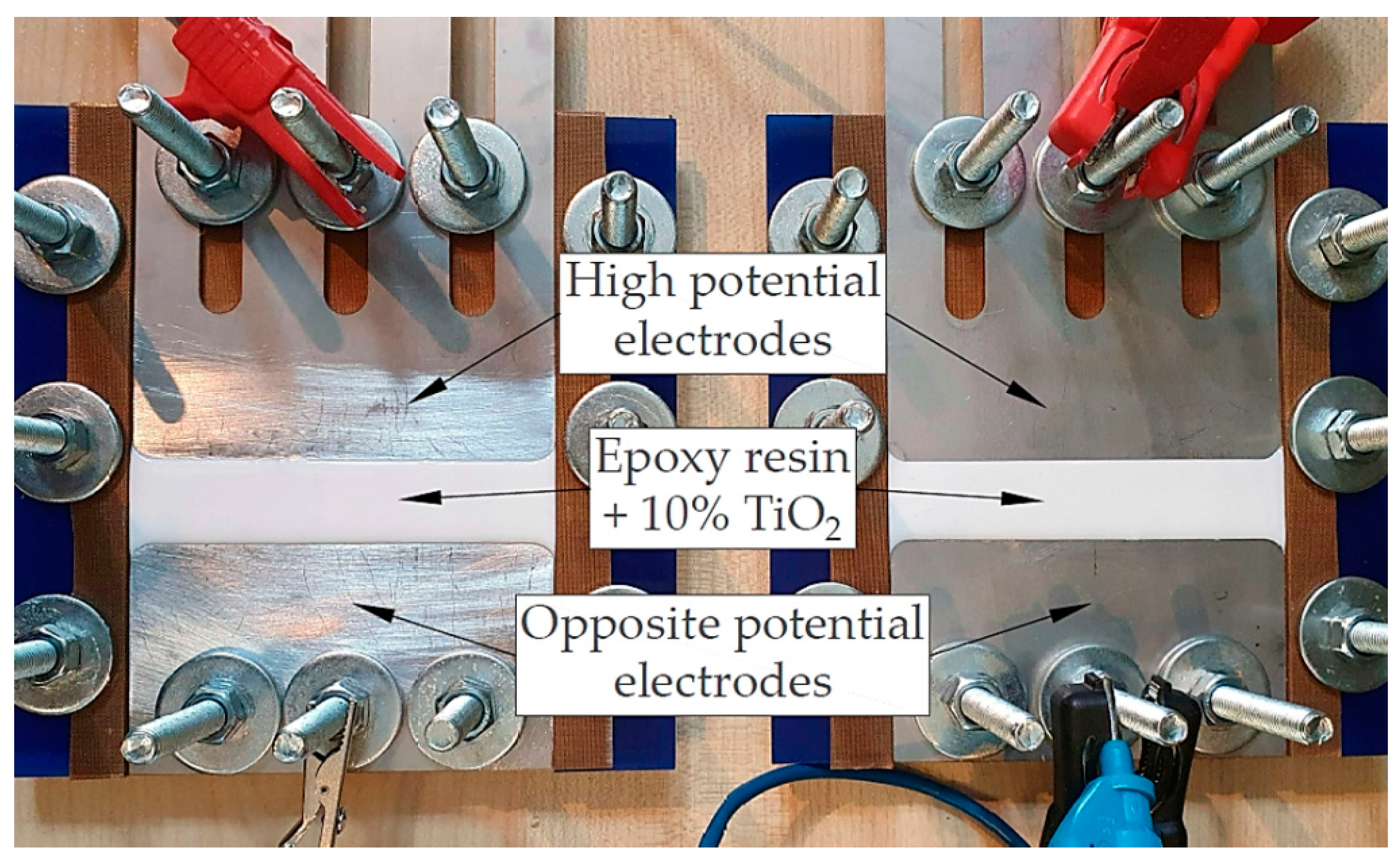
2.1. Materials for Tested Samples
2.2. Preparation and Processing of Samples
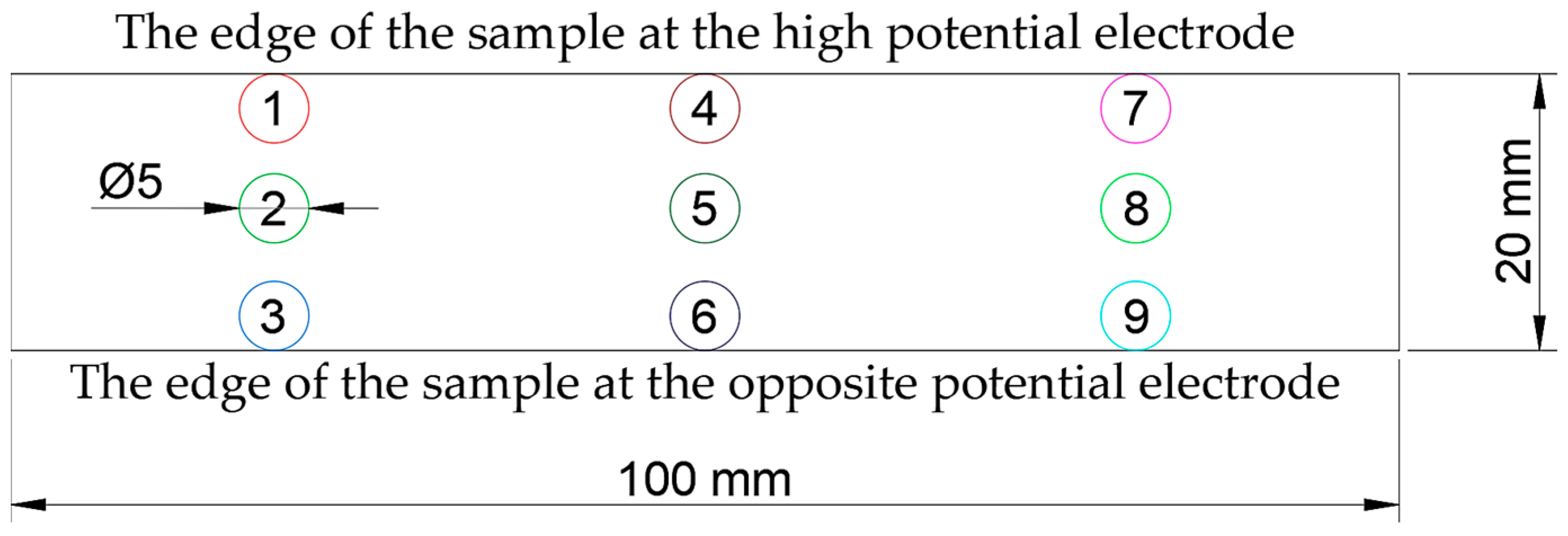
2.3. Measurement of Wideband Dielectric Properties of Composite Samples
3. Results of Processing and Measurement of Composite Samples
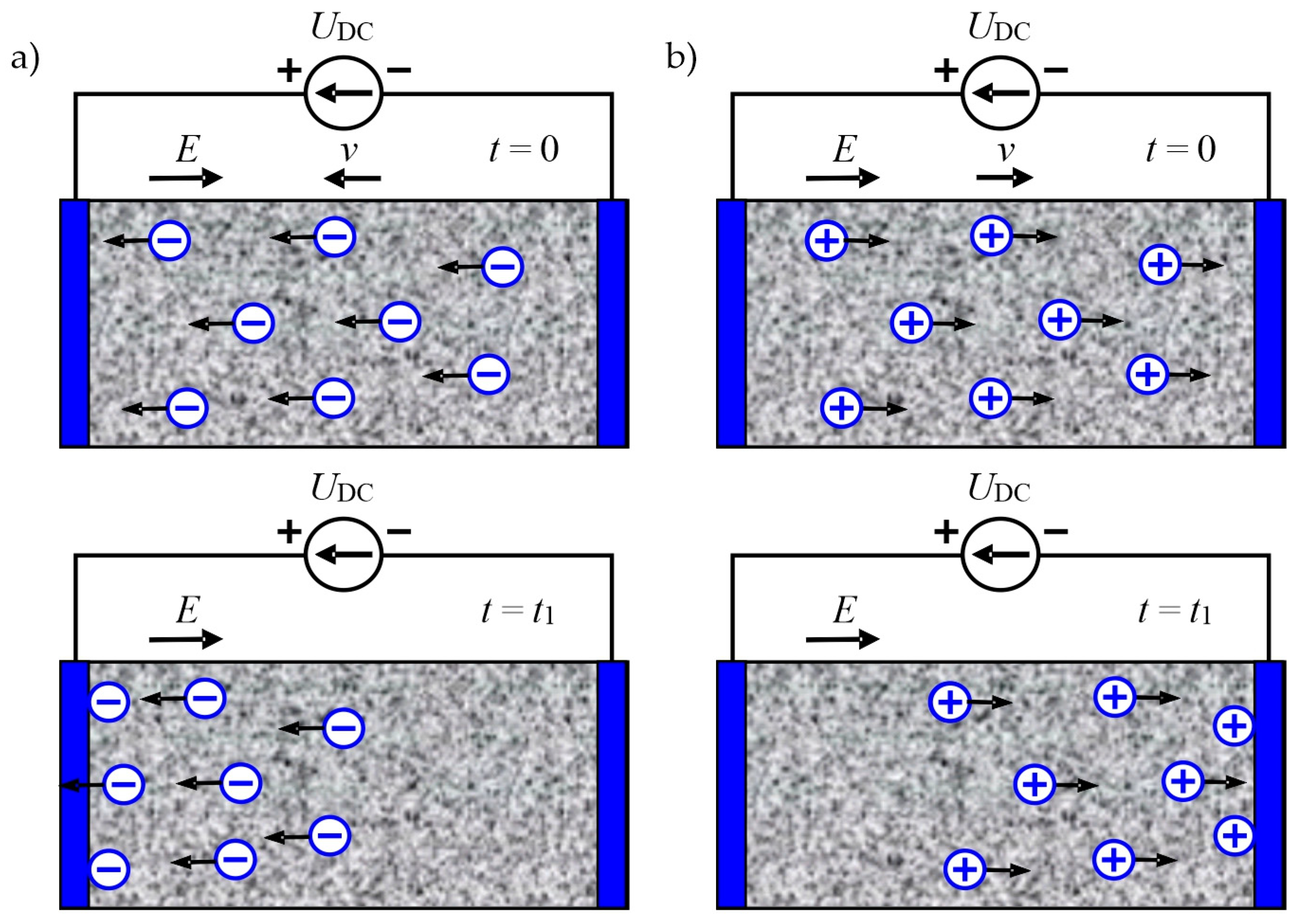
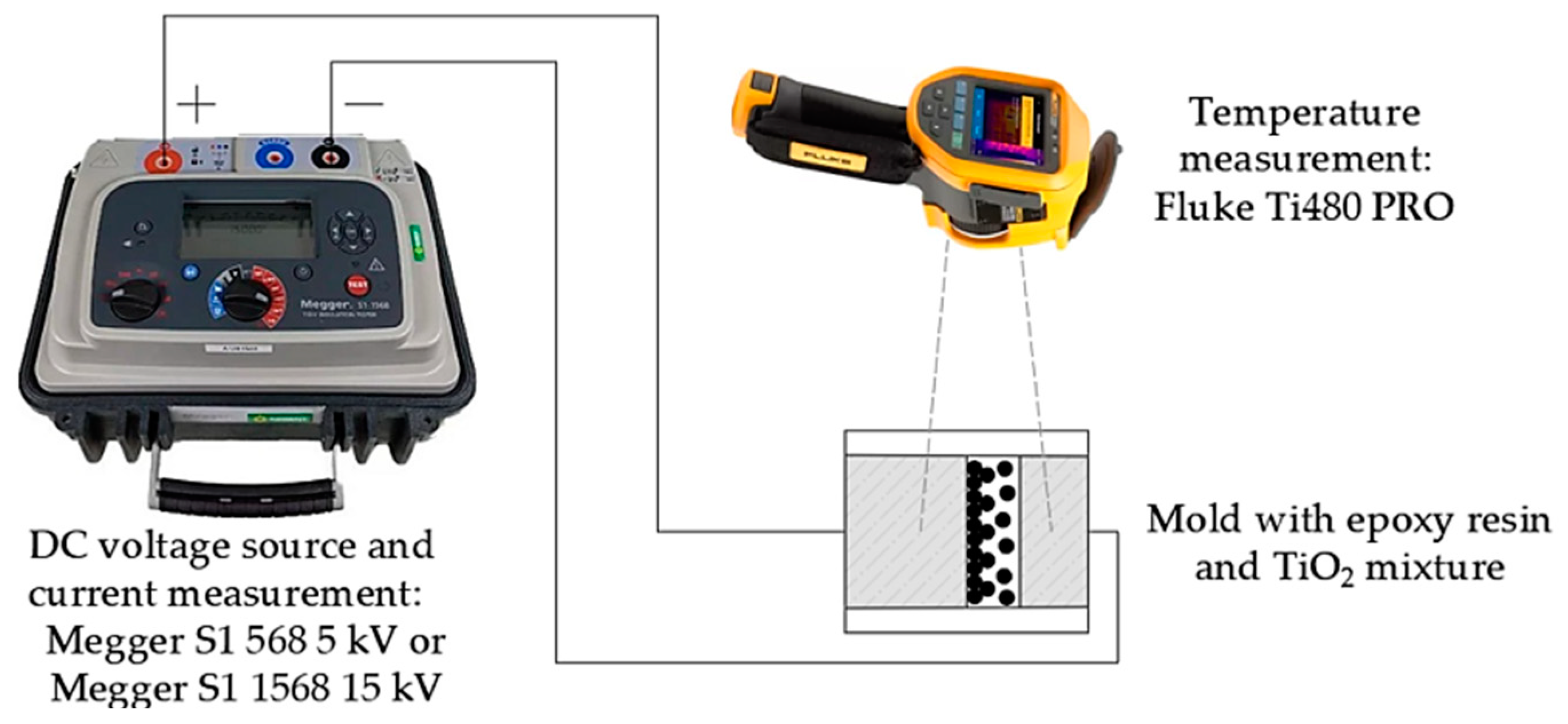
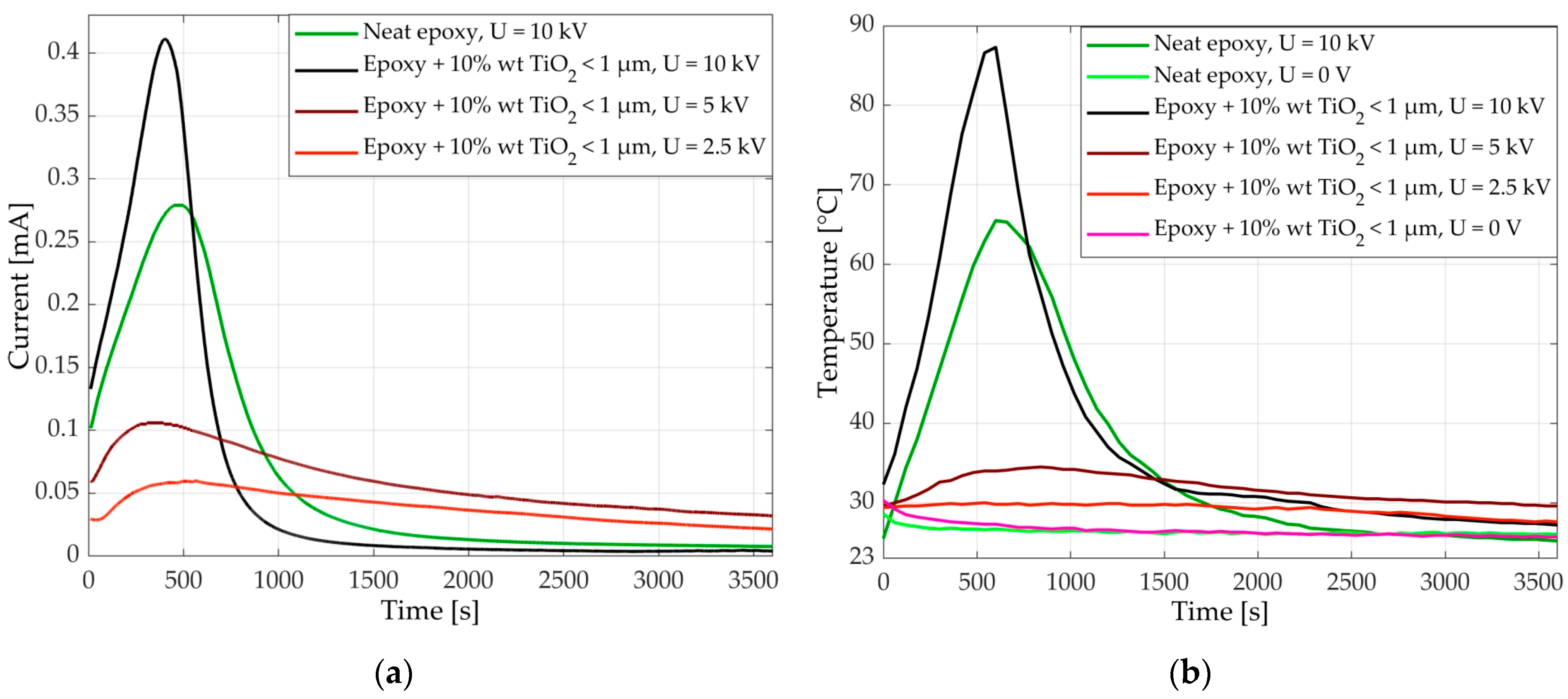
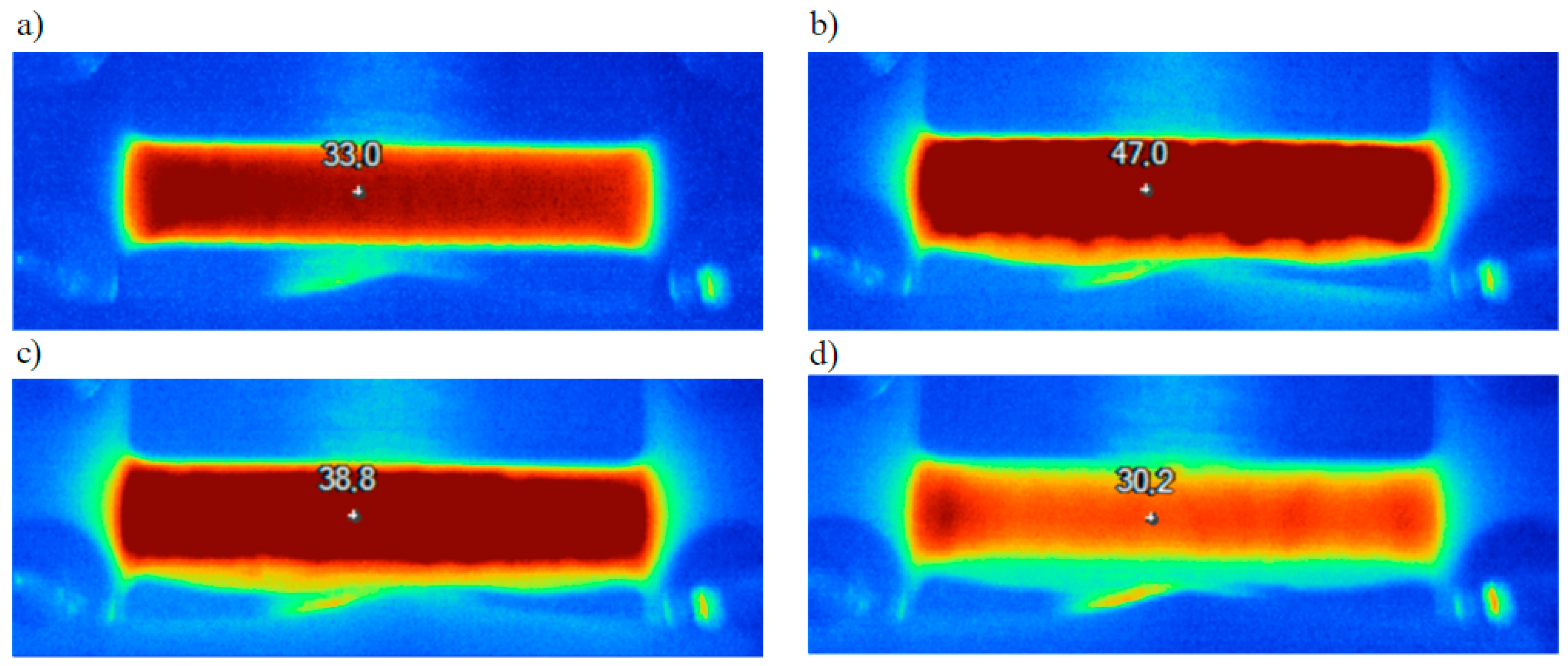
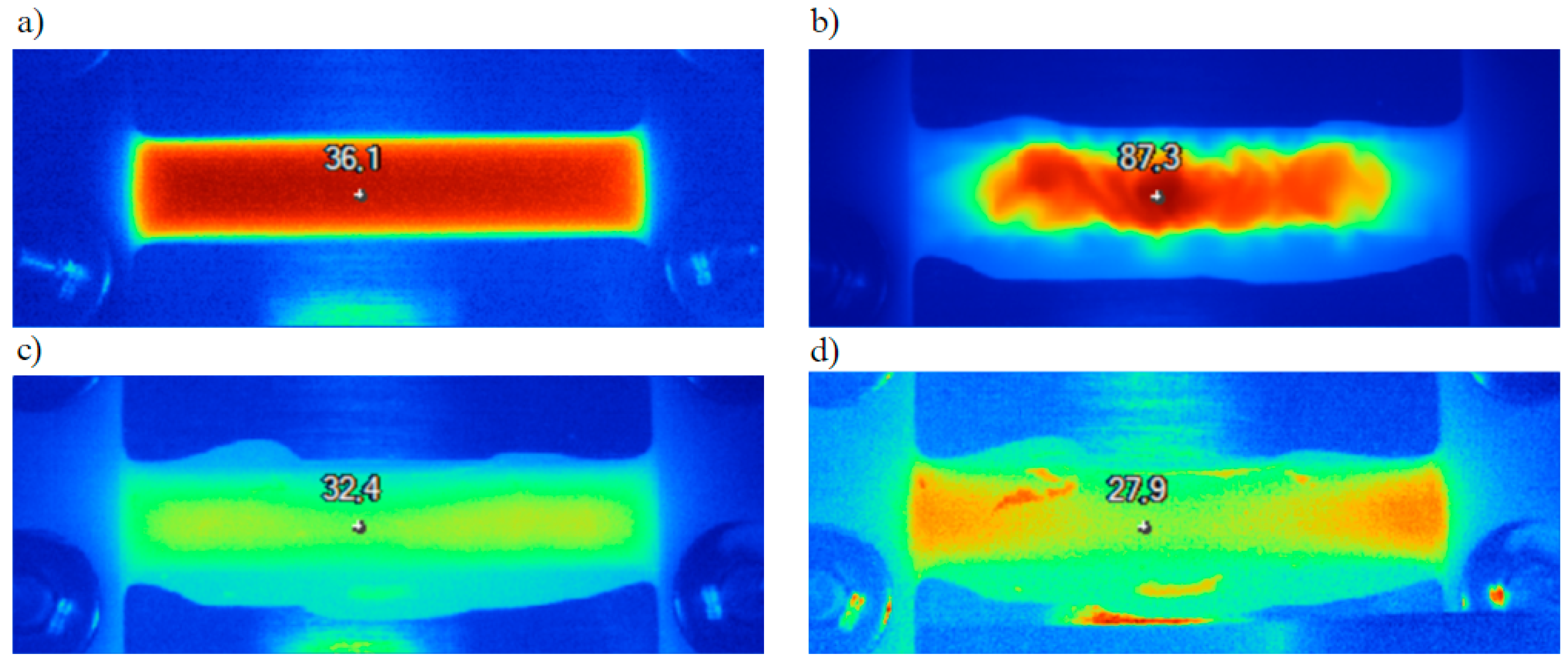
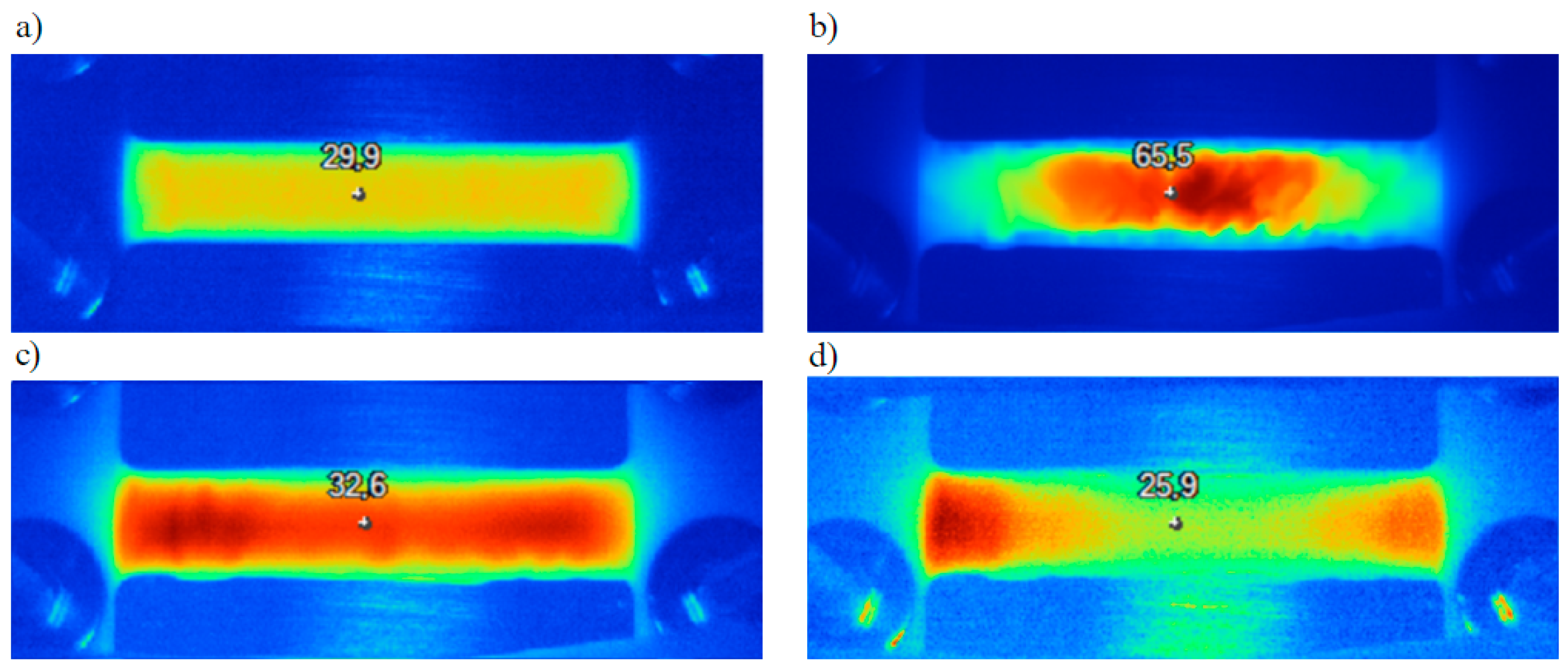
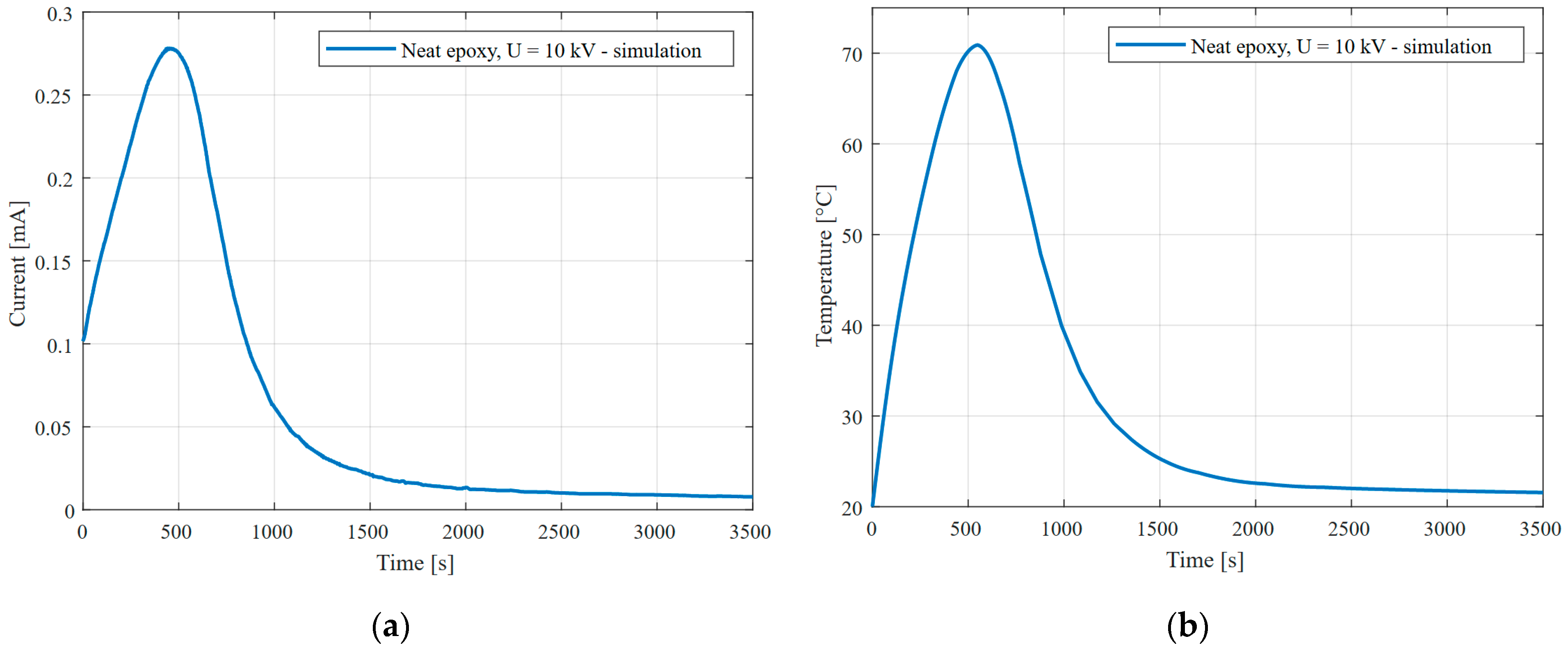
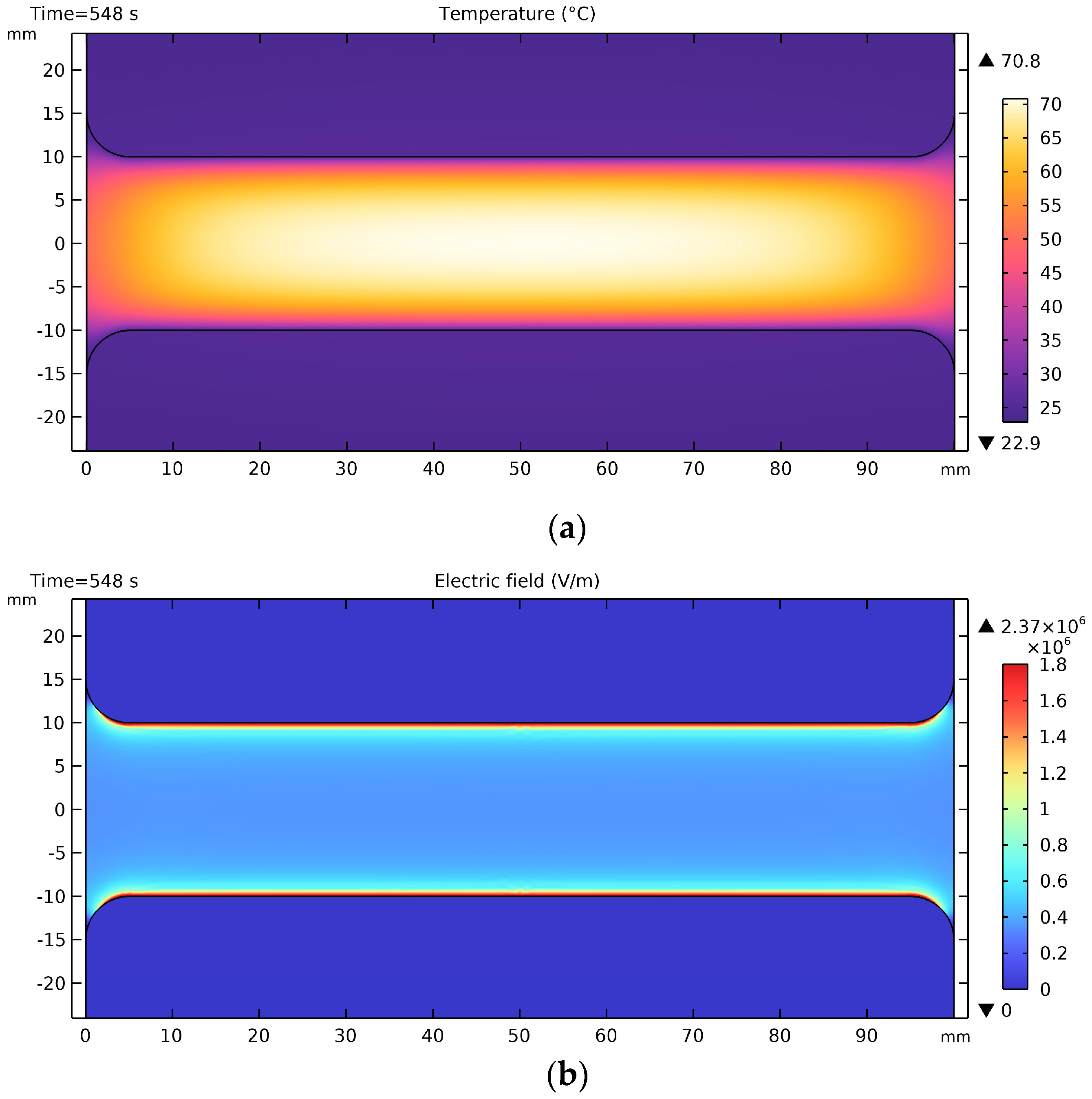
3.1. Observation of Samples Processed in a DC Electric Field
- -
- Recording the current flowing through the sample during the application of the electric field E, inducing the electrophoretic movement of charged particles;
- -
- Recording the temperature at the central point of the sample’s surface and capturing thermal images of the entire sample surface.
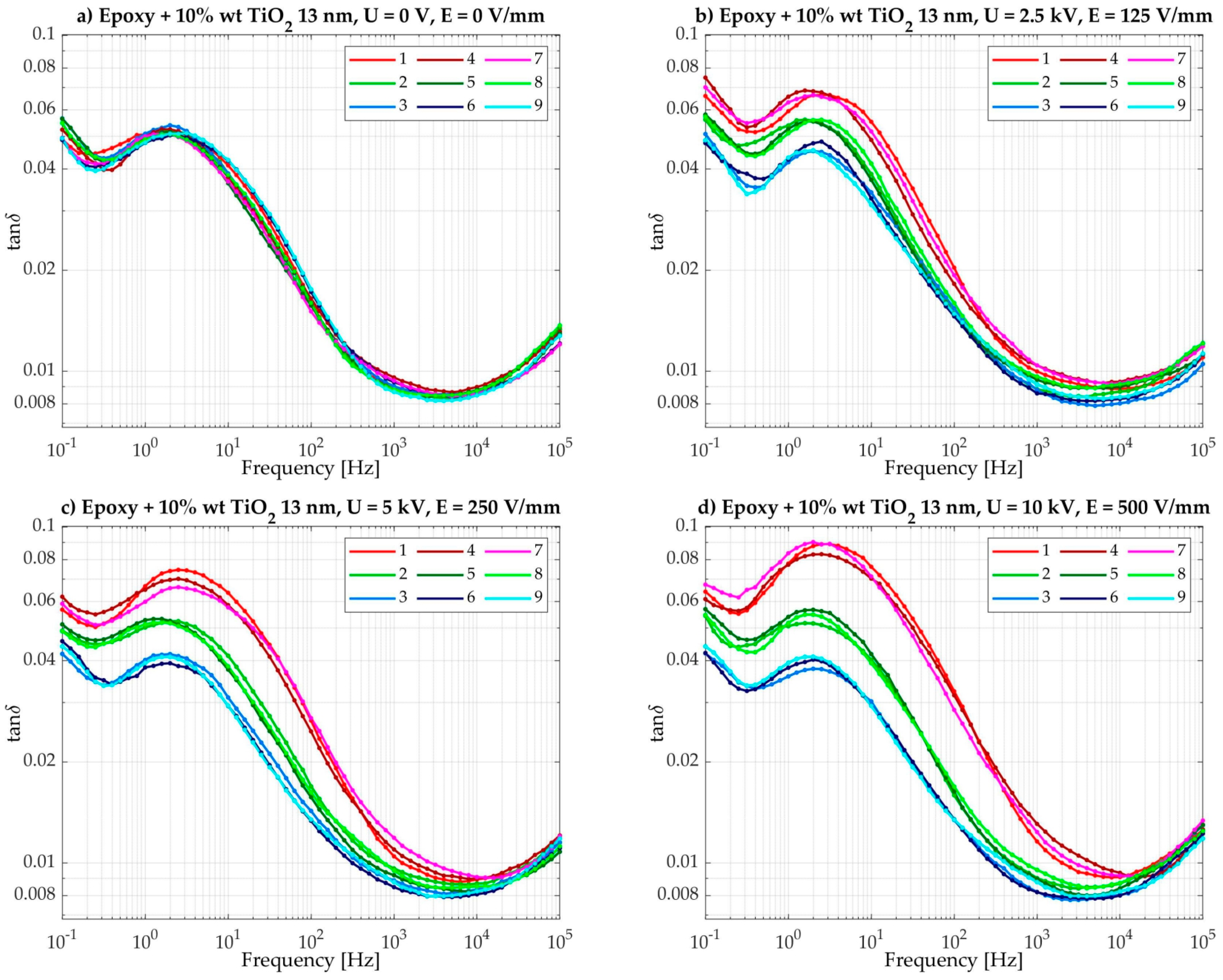
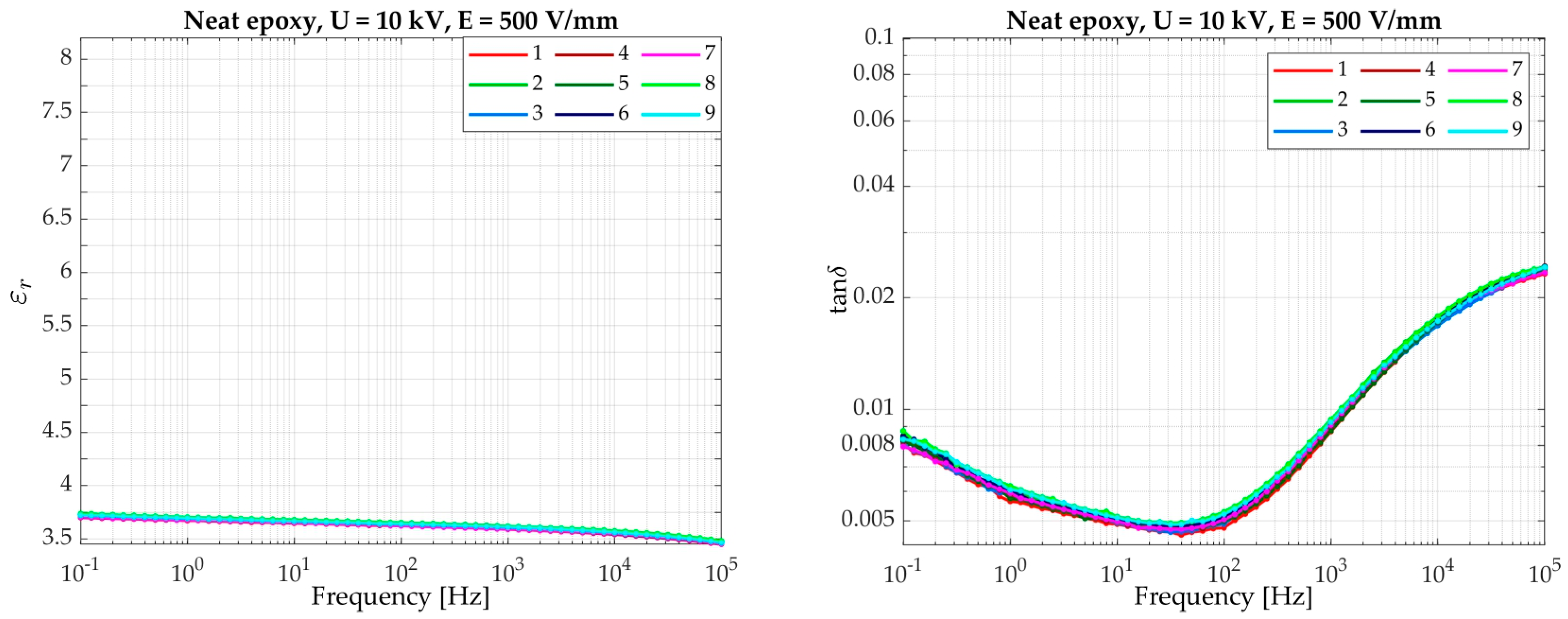
3.2. Results of Broadband Measurements of Dielectric Parameters
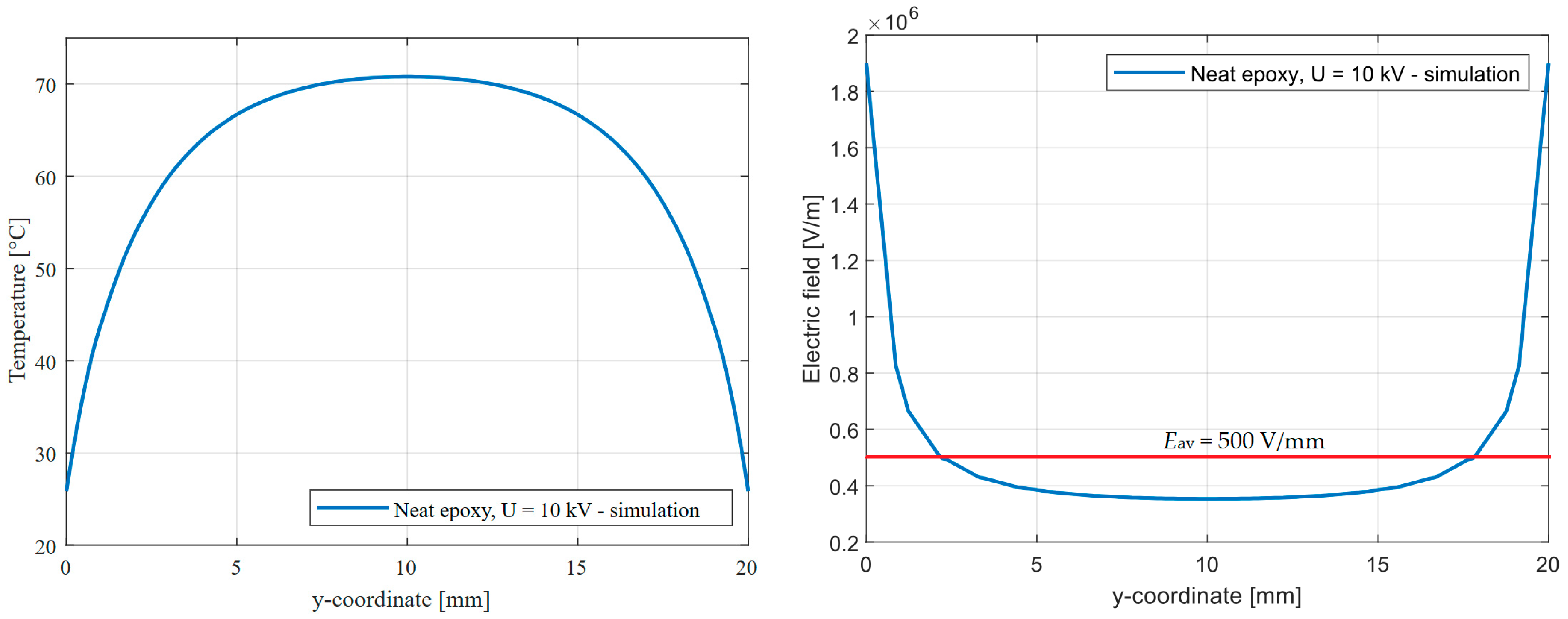
4. Analysis and Discussion of Results
- (a)
- The ratio q/r, i.e., two basic parameters describing the individual characteristics of a charged particle migrating in the suspending medium under the influence of electrophoretic force;
- (b)
- Properties of the suspending medium (i.e., cross-linking epoxy resin), characterized by a viscosity coefficient η that changes dynamically during the electrophoresis process;
- (c)
- The value of the electric field strength E forcing the movement of charged particles in the suspending medium.
- Pulse current iC(t) charging the geometric capacitance of the sample, flowing for a very short time, just after increase in voltage on the electrodes to the UDC value. Its maximum value is limited by the current capacity of the voltage source.
- Conduction current iσ(t), conditioned by the value of the time-varying bulk conductivity σ(t) of the composite. The conductivity component of the current is of the ionic type and is strongly dependent on temperature. In the progressing curing process, it also depends on the degree of cross-linking of the polymer structure of the composite and the influence of filler particles.
- Polarization current ip(t) resulting from polarization processes occurring in dielectric under the influence of an external electric field. The waveform of this current is also influenced by the phase transformations of the composite material and the formation of the cross-linked polymer structure of the sample.
- Electrophoretic current iep(t), related to the displacement of charged TiO2 filler particles in the suspension medium. This current is a small part of the conduction current flowing through the composite when UDC voltage is present between the external electrodes.
- σ0—material conductivity, S·m−1 (at E = 0.0 V·m−1 and T = 0 °C);
- T—temperature, °C;
- E—electric field strength, V·m−1;
- α—temperature factor of conductivity, °C−1;
- β—field factor of conductivity, V−1·m.
5. Conclusions
- (1)
- The material factor, resulting from the use of TiO2 filler particles of three different dimensions (13 nm, 38 nm, and <1 µm);
- (2)
- The process factor resulting from the application of four different values of electric field stress in the material sample, i.e., 0.0 Vmm−1 (without external E field) and with external E field (125 Vmm−1, 250 Vmm−1 and 500 Vmm−1), causing electrophoretic movement of filler particles, but also heating the material with Joule heat, resulting in an increase in the reaction kinetics responsible for the rate of the epoxy resin curing process.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lewis, T.J. Nanometric dielectrics. IEEE Trans. Dielectr. Electr. Insul. 1994, 5, 812–825. [Google Scholar] [CrossRef]
- Cao, Y.; Irwin, P.C.; Younsi, K. The future of nanodielectrics in the electrical power industry. IEEE Trans. Dielectr. Electr. Insul. 2004, 5, 797–807. [Google Scholar] [CrossRef]
- Nelson, J.K. Overview of nanodielectrics: Insulating materials of the future. In Proceedings of the 2007 Electrical Insulation Conference and Electrical Manufacturing Expo, Nashville, TN, USA, 22–24 October 2007; pp. 229–235. [Google Scholar] [CrossRef]
- Nelson, J.K. Dielectric Polymer Nanocomposites; Springer: New York, NY, USA, 2010. [Google Scholar] [CrossRef]
- Zhou, Y.; Peng, S.; Hu, J.; He, J. Polymeric insulation materials for HVDC cables: Development, challenges and future perspective. IEEE Trans. Dielectr. Electr. Insul. 2017, 3, 1308–1318. [Google Scholar] [CrossRef]
- Du, B. (Ed.) Polymer Insulation Applied for HVDC Transmission; Springer: Singapore, 2020. [Google Scholar] [CrossRef]
- Hemmati, R.; Wu, F.; El-Refaie, A. Survey of insulation systems in electrical machines. In Proceedings of the 2019 IEEE International Electric Machines & Drives Conference (IEMDC), San Diego, CA, USA, 12–15 May 2019; pp. 2069–2076. [Google Scholar] [CrossRef]
- Primo, V.A.; Garcia, B.; Albarracin, R. Improvement of transformer liquid insulation using nanodielectric fluids: A review. IEEE Electr. Insul. Mag. 2018, 3, 13–26. [Google Scholar] [CrossRef]
- Stone, G.C.; Boulter, E.A.; Culbert, I.; Dhirani, H. Electrical Insulation for Rotating Machines: Design, Evaluation, Aging, Testing, and Repair, 1st ed.; Wiley-IEEE Press: Milwaukee, WI, USA, 2004. [Google Scholar]
- IEC 60505:2011; Evaluation and Qualification of Electrical Insulation Systems. IEC: Genève, Switzerland, 2011.
- Chung, D.D.L. Composite Materials—Functional Materials for Modern Technologies; Springer: London, UK, 2003; ISBN 978-1-4471-3732-0. [Google Scholar]
- Tanaka, T.; Montanari, G.C.; Mülhaupt, R. Polymer nanocomposites as dielectrics and electrical insulation—Perspectives for processing technologies, material characterization and future applications. IEEE Trans. Dielectr. Electr. Insul. 2004, 11, 763–784. [Google Scholar] [CrossRef]
- Barber, P.; Balasubramanian, S.; Anguchamy, Y.; Gong, S.; Wibowo, A.; Gao, H.; Ploehn, H.J.; Zur Loye, H.-C. Polymer composite and nanocomposite dielectric materials for pulse power energy storage. Materials 2009, 2, 1697–1733. [Google Scholar] [CrossRef]
- Pleşa, I.; Noţingher, P.V.; Schlögl, S.; Sumereder, C.; Muhr, M. Properties of polymer composites used in high-voltage applications. Polymers 2016, 8, 173. [Google Scholar] [CrossRef]
- Xiao, M.; Du, B.X. Review of high thermal conductivity polymer dielectrics for electrical insulation. High Volt. 2016, 1, 34–42. [Google Scholar] [CrossRef]
- Gao, Y.; Li, J.; Li, Z.; Du, B. Polymer composites with high thermal conductivity for HVDC cable insulation: A review. IEEE Trans. Dielectr. Electr. Insul. 2023, 30, 2444–2459. [Google Scholar] [CrossRef]
- Pandey, J.C.; Singh, M. Dielectric polymer nanocomposites: Past advances and future prospects in electrical insulation perspective. SPE Polym. 2021, 2, 236–256. [Google Scholar] [CrossRef]
- Huang, X.; Tanaka, T. (Eds.) Polymer Composites for Electrical Engineering; John Wiley & Sons Ltd.: Hoboken, NJ, USA, 2022; ISBN 9781119719601. [Google Scholar]
- Li, L.; Han, L.; Hu, H.; Zhang, R. A review on polymers and their composites for flexible electronics. Mater. Adv. 2023, 4, 726. [Google Scholar] [CrossRef]
- Yao, Y.; Lu, G.-Q.; Boroyevich, D.; Ngo, K.D.T. Survey of high-temperature polymeric encapsulants for power electronics packaging. IEEE Trans. Compon. Packag. Manuf. Technol. 2015, 2, 168–181. [Google Scholar] [CrossRef]
- Diaham, S.; Valdez-Nava, Z.; Le, T.T.; Lévêque, L.; Laudebat, L.; Lebey, T. Field grading composites tailored by electrophoresis—Part 3: Application to power electronics modules encapsulation. IEEE Trans. Dielectr. Electr. Insul. 2021, 2, 348–354. [Google Scholar] [CrossRef]
- Yang, C.; Wei, H.; Guan, L.; Guo, J.; Wang, Y.; Yan, X.; Zhang, X.; Weic, S.; Guo, Z. Polymer nanocomposites for energy storage, energy saving, and anticorrosion. J. Mater. Chem. A 2015, 3, 14929–14941. [Google Scholar] [CrossRef]
- Gopal, J.; Muthu, M.; Sivanesan, I. A Comprehensive compilation of graphene/fullerene polymer nanocomposites for electrochemical energy storage. Polymers 2023, 15, 701. [Google Scholar] [CrossRef]
- Atif, R.; Shyha, I.; Inam, F. Mechanical, thermal, and electrical properties of graphene-epoxy nanocomposites—A review. Polymers 2016, 8, 281. [Google Scholar] [CrossRef]
- Zhong, S.L.; Dang, Z.M.; Zhou, W.Y.; Cai, H.W. Past and future on nanodielectrics. IET Nanodielectrics 2018, 1, 41–47. [Google Scholar] [CrossRef]
- Lewis, T.J. Interfaces are the dominant feature of dielectrics at the nanometric level. IEEE Trans. Dielectr. Electr. Insul. 2004, 11, 739–753. [Google Scholar] [CrossRef]
- Christen, T.; Donzel, L.; Greuter, F. Nonlinear resistive electric field grading Part 1: Theory and simulation. IEEE Electr. Insul. Mag. 2010, 6, 47–59. [Google Scholar] [CrossRef]
- Donzel, L.; Greuter, F.; Christen, T. Nonlinear resistive electric field grading Part 2: Materials and applications. IEEE Electr. Insul. Mag. 2011, 2, 18–29. [Google Scholar] [CrossRef]
- Yang, X.; Zhao, X.; Hu, J.; He, J. Grading electric field in high voltage insulation using composite materials. IEEE Electr. Insul. Mag. 2018, 1, 15–25. [Google Scholar] [CrossRef]
- Diaham, S.; Valdez-Nava, Z.; Lévêque, L.; Le, T.T.; Laudebat, L.; Lebey, T. Field grading composites tailored by electrophoresis—Part 1: Principle and permittivity gradient in uniform electric field. IEEE Trans. Dielectr. Electr. Insul. 2021, 2, 333–340. [Google Scholar] [CrossRef]
- Diaham, S.; Valdez-Nava, Z.; Le, T.T.; Lévêque, L.; Laudebat, L.; Lebey, T. Field grading composites tailored by electrophoresis—Part 2: Permittivity gradient in non-uniform electric field. IEEE Trans. Dielectr. Electr. Insul. 2021, 2, 341–347. [Google Scholar] [CrossRef]
- Rachmawati; Kojima, H.; Hayakawa, N.; Kato, K.; Zebouchi, N. Electric field simulation of permittivity and conductivity graded materials (ε/σ-FGM) for HVDC GIS spacers. IEEE Trans. Dielectr. Electr. Insul. 2021, 2, 736–744. [Google Scholar] [CrossRef]
- CIGRÉ Working Group D1.56. Technical Brochure 794: Field Grading in Electrical Insulation Systems; CIGRÉ: Paris, France, 2020. [Google Scholar]
- Mahamood, R.M.; Akinlabi, E.T.; Shukla, M.; Pityana, S.L. Functionally graded material: An overview. In Proceedings of the World Congress on Engineering 2012 Vol III (WCE 2012), London, UK, 4–6 July 2012. [Google Scholar]
- Shinohara, Y. Chapter 11.2.4—Functionally Graded Material. In Handbook of Advanced Ceramics, 2nd ed.; Somiya, S., Ed.; Academic Press: Cambridge, MA, USA, 2013; pp. 1179–1187. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, J. Fabrication of functionally graded porous polymer structures using thermal bonding lamination techniques. Procedia Manuf. 2017, 10, 866–875. [Google Scholar] [CrossRef]
- Kurimoto, M.; Ozaki, H.; Sawada, T.; Funabashi, T.; Kato, T.; Suzuoki, Y. FEM simulation of local field enhancement close to lamination interface of permittivity-graded material. Electron. Commun. Jpn. 2018, 6, 48–57. [Google Scholar] [CrossRef]
- Li, N.; Tian, J.; Deng, W.; Sun, H. Application of functionally graded materials for solid insulator: Fabrication, optimization design, and surface flashover of prototype samples. Appl. Mech. Mater. 2013, 291, 2308–2312. [Google Scholar] [CrossRef]
- Kurimoto, M.; Kato, K.; Hanai, M.; Hoshina, Y.; Takei, M.; Okubo, H. Application of functionally graded material for reducing electric field on electrode and spacer interface. IEEE Trans. Dielectr. Electr. Insul. 2010, 1, 256–263. [Google Scholar] [CrossRef]
- Hayakawa, N.; Ishiguro, J.; Kojima, H.; Kato, K.; Okubo, H. Fabrication and simulation of permittivity graded materials for electric field grading of gas insulated power apparatus. IEEE Trans. Dielectr. Electr. Insul. 2016, 1, 547–554. [Google Scholar] [CrossRef]
- Pradeep, A.D.; Rameshkumar, T. Review on centrifugal casting of functionally graded materials. Mater. Today Proc. 2021, 45, 729–734. [Google Scholar] [CrossRef]
- Sun, P.; Li, W.-D.; Chen, J.-H.; Wang, C.; Yin, W.; Zhang, Y.-C.; Li, J.-S.; Deng, J.-B.; Zhang, G.-J. Construction of permittivity graded insulator using topology-optimized lattice structure. IEEE Trans. Dielectr. Electr. Insul. 2023, 31, 457–465. [Google Scholar] [CrossRef]
- Matsuoka, N.; Komesu, D.; Mori, M.; Kozako, M.; Hikita, M.; Ishibe, S. Electric field grading of gas insulated switchgears with microvaristor composites. In Proceedings of the 2015 IEEE 11th International Conference on the Properties and Applications of Dielectric Materials (ICPADM), Sydney, Australia, 19–22 July 2015; pp. 332–335. [Google Scholar] [CrossRef]
- Nicholson, P.S.; Sarkar, P.; Haung, X. Electrophoretic deposition and its use to synthesize ZrO2/AI2O3 micro-laminate ceramic/ceramic composites. J. Mater. Sci. 1993, 28, 6274–6278. [Google Scholar] [CrossRef]
- Zhitomirsky, I.; Petric, A. Electrolytic and electrophoretic deposition of CeO2 films. Mater. Lett. 1999, 6, 263–268. [Google Scholar] [CrossRef]
- Besra, L.; Liu, M. A review on fundamentals and applications of electrophoretic deposition (EPD). Prog. Mater. Sci. 2007, 52, 1–61. [Google Scholar] [CrossRef]
- Belijar, G.; Fetouhi, L.; Kozako, M.; Hikita, M. Field Grading Material electrodeposition—Challenges. In Proceedings of the 2020 IEEE 3rd International Conference on Dielectrics (ICD), Valencia, Spain, 5–31 July 2020; pp. 289–292. [Google Scholar] [CrossRef]
- Zhang, X.; Le, M.-Q.; Nguyen, V.C.; Mogniotte, J.F.; Capsal, J.F.; Grinberg, D.; Cottinet, P.J.; Petit, L. Characterization of micro-ZnO/PDMS composite structured via dielectrophoresis—Toward medical application. Mater. Des. 2021, 208, 109912. [Google Scholar] [CrossRef]
- Du, B.X.; Wang, Z.H.; Li, J.; Liang, H.C.; Li, Z.H. Epoxy insulator with surface graded-permittivity by magnetron sputtering for gas-insulated line. IEEE Trans. Dielectr. Electr. Insul. 2020, 1, 197–205. [Google Scholar] [CrossRef]
- Nardi, T.; Mora, N.; Rachidi, F.; Leterrier, Y. Graded-permittivity polymer nanocomposites as superior dielectrics. Compos. Sci. Technol. 2016, 129, 1–9. [Google Scholar] [CrossRef]
- Pradhan, M.; Greijer, H.; Eriksson, G.; Unge, M. Functional behaviors of electric field grading composite materials. IEEE Trans. Dielectr. Electr. Insul. 2016, 2, 768–778. [Google Scholar] [CrossRef]
- Can-Ortiz, A.; Laudebat, L.; Valdez-Nava, Z.; Diaham, S. Nonlinear electrical conduction in polymer composites for field grading in high-voltage applications: A Review. Polymers 2021, 13, 1370. [Google Scholar] [CrossRef] [PubMed]
- Shen, Z.; Wang, X.; Zhang, T.; Jia, Z. In situ electric field driven assembly to construct adaptive graded permittivity BaTiO3/epoxy resin composites for improved insulation performance. Appl. Mater. Today 2020, 20, 100647. [Google Scholar] [CrossRef]
- Diaham, S.; Nava, Z.V.; Lévêque, L.; Le, T.T.; Laudebat, L.; Lebey, T. An original in-situ way to build field grading materials (FGM) with permittivity gradient using electrophoresis. In Proceedings of the 2018 IEEE 2nd International Conference on Dielectrics (ICD), Budapest, Hungary, 1–5 July 2018; pp. 1–4. [Google Scholar] [CrossRef]
- Michov, B. Electrophoresis—Theory and Practice; Walter de Gruyter GmbH: Berlin, Germany; Boston, MA, USA, 2020. [Google Scholar]
- Charcosset, C. Electrophoretic Mobility. In Encyclopedia of Membranes; Drioli, E., Giorno, L., Eds.; Springer: Berlin/Heidelberg, Germany, 2016. [Google Scholar] [CrossRef]
- Shallan, A.; Guijt, R.; Breadmore, M. Capillary electrophoresis: Basic principles. In Encyclopedia of Forensic Sciences; Siegel, J.A., Saukko, P.J., Houck, M.M., Eds.; Academic Press: Cambridge, MA, USA, 2013. [Google Scholar] [CrossRef]
- Ohshima, H. Electrophoretic mobility of a spherical colloidal particle in a salt-free medium. J. Colloid Interface Sci. 2002, 248, 499–503. [Google Scholar] [CrossRef]
- Murugesh, A.K.; Uthayanan, A.; Lekakou, C. Electrophoresis and orientation of multiple wall carbon nanotubes in polymer solution. Appl. Phys. A 2010, 100, 135–144. [Google Scholar] [CrossRef]
- Sarkar, P.; Datta, S.; Nicholson, P.S. Functionally graded ceramic/ceramic and metal/ceramic composites by electrophoretic deposition. Compos. Part B Eng. 1997, 28, 48–56. [Google Scholar] [CrossRef]
- Shehayeb, S. Electrophoretic Deposition of Nanoparticles for Controlled Optical Properties. Ph.D. Thesis, Material Chemistry. Laboratoire de Catalyse Organométallique et Chimie de Coordination (Liban), Montpellier, France, 2017. English. NNT: 2017MONTT195. Available online: https://theses.hal.science/tel-01872737 (accessed on 12 December 2022).
- Li, G.; Koch, D.L. Electrophoresis in dilute polymer solutions. J. Fluid Mech. 2020, 884, A9. [Google Scholar] [CrossRef]
- Andritsch, T. Epoxy Based Nanodielectrics for High Voltage DC-Applications—Synthesis, Dielectric Properties and Space Charge Dynamics. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 2010; pp. 33–35. Available online: http://eprints.soton.ac.uk/id/eprint/410506 (accessed on 13 April 2023).
- Shapagina, N.A.; Dushik, V.V. Application of electrophoretic deposition as an advanced technique of inhibited polymer films formation on metals from environmentally safe aqueous solutions of inhibited formulations. Materials 2023, 16, 19. [Google Scholar] [CrossRef] [PubMed]
- Chi, S.; Zhang, D.; Fang, R.; Liu, M.; Lin, Q. Preparation and properties of polyamido-amine dendrimer/polyurushiol composite coatings by stepwise electrophoresis deposition. Prog. Org. Coat. 2022, 166, 106779. [Google Scholar] [CrossRef]
- Elantas Europe. Epoxylite 235SG Trickle Impregnating Resin; Product information; Elantas Europe: Hamburg, Germany, 2007. [Google Scholar]
- May, C.A. Epoxy Resins: Chemistry and Technology, 2nd ed.; Marcel Dekker Inc.: New York, NY, USA, 1988. [Google Scholar]
- Ellis, B. Chemistry and Technology of Epoxy Resins; Springer Science+Business Media: Dordrecht, The Netherlands, 1993. [Google Scholar]
- Shundo, A.; Yamamoto, S.; Tanaka, K. Network formation and physical properties of epoxy resins for future practical applications. JACS Au 2022, 7, 1522–1542. [Google Scholar] [CrossRef] [PubMed]
- Alhabill, F.N.; Ayoob, R.; Andritsch, T.; Vaughan, A.S. Effect of resin/hardener stoichiometry on electrical behavior of epoxy networks. IEEE Trans. Dielectr. Electr. Insul. 2017, 24, 3739–3749. [Google Scholar] [CrossRef]
- Saeedi, I.A.; Chalashkanov, N.; Dissado, L.A.; Vaughan, A.S.; Andritsch, T. The nature of the gamma dielectric relaxation in diglycidyl ether Bisphenol-A (DGEBA) based epoxies. Polymer 2022, 249, 124861. [Google Scholar] [CrossRef]
- Li, J.; Guo, P.; Kong, X.; Wang, Y.; Li, F.; Du, B. Curing degree dependence of dielectric properties of bisphenol-A-based epoxy resin cured with methyl hexahydrophthalic anhydride. IEEE Trans. Dielectr. Electr. Insul. 2022, 29, 2072–2079. [Google Scholar] [CrossRef]
- Li, J.; Aung, H.H.; Du, B. Curing regime-modulating insulation performance of anhydride-cured epoxy resin: A review. Molecules 2023, 28, 547. [Google Scholar] [CrossRef] [PubMed]
- Titanium Dioxide (TiO2) Nanopowder/Nanoparticles, Anatase, Purity: 99.55%, Size: 13 nm. Available online: https://nanografi.com/nanoparticles/titanium-dioxide-tio2-nanopowder-nanoparticles-anatase-purity-99-55-size-13-nm/ (accessed on 9 August 2022).
- Titanium Dioxide (TiO2) Nanopowder/Nanoparticles, Anatase, Purity: 99.55%, Size: 38 nm. Available online: https://nanografi.com/nanoparticles/titanium-dioxide-tio2-nanopowder-nanoparticles-anatase-purity-99-55-size-38-nm/ (accessed on 9 August 2022).
- Acros Organics. Titanium (IV) Oxide, Cat No. 277370000; 277370010; 277370100, CAS 13463-67-7; Acros Organics BVBA: Geel, Belgium, 2014. [Google Scholar]
- Hanaor, D.A.H.; Sorrell, C. C Review of the anatase to rutile phase transformation. J. Mater. Sci. 2011, 46, 855–874. [Google Scholar] [CrossRef]
- Carp, O.; Huisman, C.L.; Reller, A. Photoinduced reactivity of titanium dioxide. Prog. Solid State Chem. 2004, 32, 33–177. [Google Scholar] [CrossRef]
- Manzoli, M.; Freyria, F.S.; Blangetti, N.; Bonelli, B. Brookite, a sometimes under evaluated TiO2 polymorph. RSC Adv. 2022, 12, 3322–3334. [Google Scholar] [CrossRef]
- Wypych, A.; Bobowska, I.; Tracz, M.; Opasinska, A.; Kadlubowski, S.; Krzywania-Kaliszewska, A.; Grobelny, J.; Wojciechowski, P. Dielectric properties and characterisation of titanium dioxide obtained by different chemistry methods. J. Nanomater. 2014, 2014, 124814. [Google Scholar] [CrossRef]
- Yan, X.; Chen, X. Titanium Dioxide Nanomaterials, Encyclopedia of Inorganic and Bioinorganic Chemistry; John Wiley & Sons: Hoboken, NJ, USA, 2015; pp. 1–33. [Google Scholar]
- Rahimi, N.; Pax, R.A.; Gray, E.M. Review of functional titanium oxides. I: TiO2 and its modifications. Prog. Solid State Chem. 2016, 3, 86–105. [Google Scholar] [CrossRef]
- Panda, J.; Singh, U.P.; Sahu, R. Synthesis, characterization of TiO2 nano particles for enhancement of electron transport application in DSSC with Cu-BPCA Dye. IOP Conf. Ser. Mater. Sci. Eng. 2018, 410, 012008. [Google Scholar] [CrossRef]
- Jia, Y.; Yang, J.; Dong, W.; Li, B.; Liu, Z. The dielectric properties and thermal conductivities of epoxy composites reinforced by titanium dioxide. J. Inorg. Organomet. Polym. 2021, 32, 1206–1212. [Google Scholar] [CrossRef]
- Fréchette, M.F.; Preda, I.; Castellon, J.; Krivda, A.; Veillette, R.; Trudeau, M.; David, E. Polymer composites with a large nanofiller content: A case study involving epoxy. IEEE Trans. Dielectr. Electr. Insul. 2014, 21, 434–443. [Google Scholar] [CrossRef]
- Kuruvilla, S.P.; Renukappa, N.M.; Rajan, J.S. Development of epoxy with nano and micro fillers for core insulation of composite insulators. In Proceedings of the 2019 International Conference on High Voltage Engineering and Technology (ICHVET), Hyderabad, India, 7–8 February 2019; pp. 1–5. [Google Scholar]
- Castellon, J.; Nguyen, H.N.; Agnel, S.; Toureille, A.; Fréchette, M.F.; Savoie, S.; Krivda, A.; Schmidt, L.E. Electrical properties analysis of micro and nano composite epoxy resin materials. IEEE Trans. Dielectr. Electr. Insul. 2011, 18, 651–658. [Google Scholar] [CrossRef]
- Yanashima, R.; Hirai, N.; Ohki, Y. Effects of addition of MgO fillers with various sizes and co-addition of nano-sized SiO2 fillers on the dielectric properties of epoxy resin. In Proceedings of the 2017 International Symposium on Electrical Insulating Materials (ISEIM), Toyohashi, Japan, 11–15 September 2017; pp. 650–653. [Google Scholar]
- Kozako, M.; Yamano, S.; Kido, R.; Ohki, Y.; Kohtoh, M.; Okabe, S.; Tanaka, T. Preparation and preliminary characteristic evaluation of epoxy/alumina nanocomposites. In Proceedings of the 2005 International Symposium on Electrical Insulating Materials, (ISEIM 2005), Kitakyushu, Japan, 5–9 June 2005; Volume 1, pp. 231–234. [Google Scholar]
- Dąda, A.; Błaut, P.; Kuniewski, M.; Zydroń, P. Analysis of selected dielectric properties of epoxy-alumina nanocomposites cured at stepwise increasing temperatures. Energies 2023, 16, 2091. [Google Scholar] [CrossRef]
- Kremer, F.; Schönhals, A. Broadband Dielectric Spectroscopy; Springer: Berlin/Heidelberg, Germany, 2003. [Google Scholar]
- Solartron Analytical. 1260 Impedance/Gain-Phase Analyzer; Operating Manual; Solartron Analytical: Farnborough, UK, 1996. [Google Scholar]
- Solartron Analytical. 1296 Dielectric Interface; Operating Manual; Solartron Analytical: Farnborough, UK, 1999. [Google Scholar]
- Fothergill, J.; Nelson, J.K.; Fu, M. Dielectric properties of epoxy nanocomposites containing TiO2, Al2O3 and ZnO fillers. In Proceedings of the Annual Report Conference on Electrical Insulation and Dielectric Phenomena (CEIDP), Boulder, CO, USA, 17–20 October 2004; pp. 406–409. [Google Scholar] [CrossRef]
- Singha, S.; Thomas, M.J. Dielectric properties of epoxy nanocomposites. IEEE Trans. Dielectr. Electr. Insul. 2008, 15, 12–23. [Google Scholar] [CrossRef]
- Roy, M.; Nelson, J.K.; MacCrone, R.K.; Schadler, L.S.; Reed, C.W.; Keefe, R. Polymer nanocomposite dielectrics—The role of the interface. IEEE Trans. Dielectr. Electr. Insul. 2005, 12, 629–643. [Google Scholar] [CrossRef]
- Singha, S.; Thomas, M.J.; Kulkarni, A. Complex permittivity characteristics of epoxy nanocomposites at low frequencies. IEEE Trans. Dielectr. Electr. Insul. 2010, 17, 1249–1258. [Google Scholar] [CrossRef]
- Singha, S.; Thomas, M.J. Permittivity and tan delta characteristics of epoxy nanocomposites in the frequency range of 1 MHz-1 GHz. IEEE Trans. Dielectr. Electr. Insul. 2008, 15, 2–11. [Google Scholar] [CrossRef]
- Tanaka, T.; Kozako, M.; Fuse, N.; Ohki, Y. Proposal of a multi-core model for polymer nanocomposite dielectrics. IEEE Trans. Dielectr. Electr. Insul. 2005, 12, 669–681. [Google Scholar] [CrossRef]
- Senturia, S.D.; Sheppard, N.F. Dielectric analysis of thermoset cure. In Epoxy Resins and Composites IV; Dušek, K., Ed.; Advances in Polymer Science; Springer: Berlin/Heidelberg, Germany, 1986; Volume 80. [Google Scholar] [CrossRef]
- Tajima, Y.A.; Crozier, D. Thermokinetic modeling of an epoxy resin I. Chemoviscosity. Polym. Eng. Sci. 1983, 4, 186–190. [Google Scholar] [CrossRef]
- Fava, R.A.; Horsfield, A.E. The interpretation of electrical resistivity measurements during epoxy resin cure. J. Phys. D Appl. Phys. 1968, 1, 117–120. [Google Scholar] [CrossRef]
- Eoll, C.K. Theory of stress distribution in insulation of high-voltage DC cables: Part I. IEEE Trans. Electr. Insul. 1975, EI-10, 27–35. [Google Scholar] [CrossRef]
- Rabenok, E.V.; Novikov, G.F.; Bogdanova, L.M.; Bukichev, Y.S.; Dzhardimalieva, G.I. Temperature dependence of direct current conductivity in TiO2/epoxy polymer dielectric nanocomposites. Russ. J. Phys. Chem. 2023, 1, 186–192. [Google Scholar] [CrossRef]
- Assar, S.T.; Abosheiasha, H.F.; Mansour, D.A.; Darwish, M.A. Preparation and investigation of epoxy/Li0.5AlxFe2.5-xO4 nanocomposites for electronic packaging applications. J. Alloys Compd. 2020, 821, 153533. [Google Scholar] [CrossRef]
- Khan, M.Z.; Wang, F.; He, L.; Shen, Z.; Huang, Z.; Mehmood, M.A. Influence of treated nano-alumina and gas-phase fluorination on the dielectric properties of epoxy resin/alumina nanocomposites. IEEE Trans. Dielectr. Electr. Insul. 2020, 27, 410–417. [Google Scholar] [CrossRef]



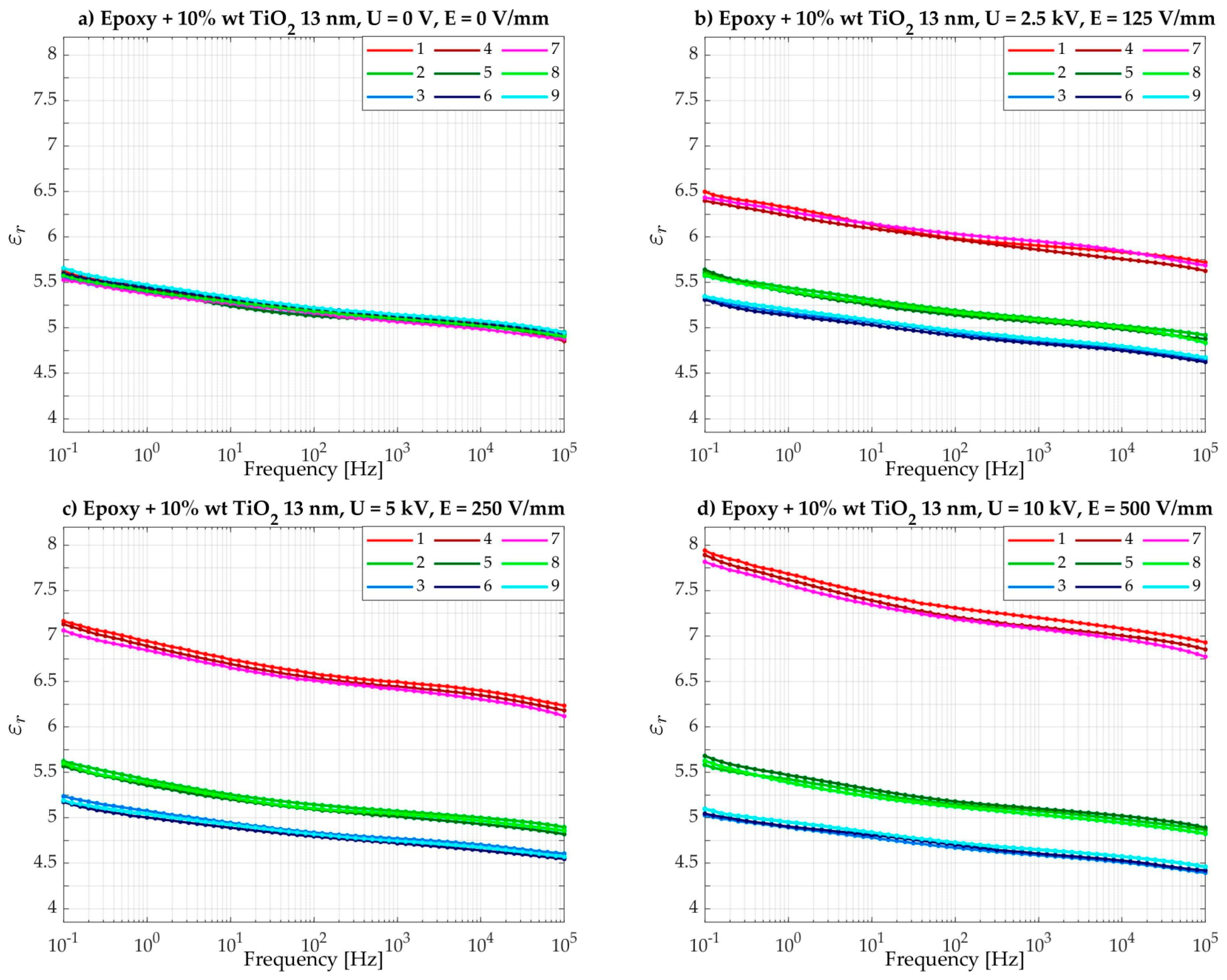
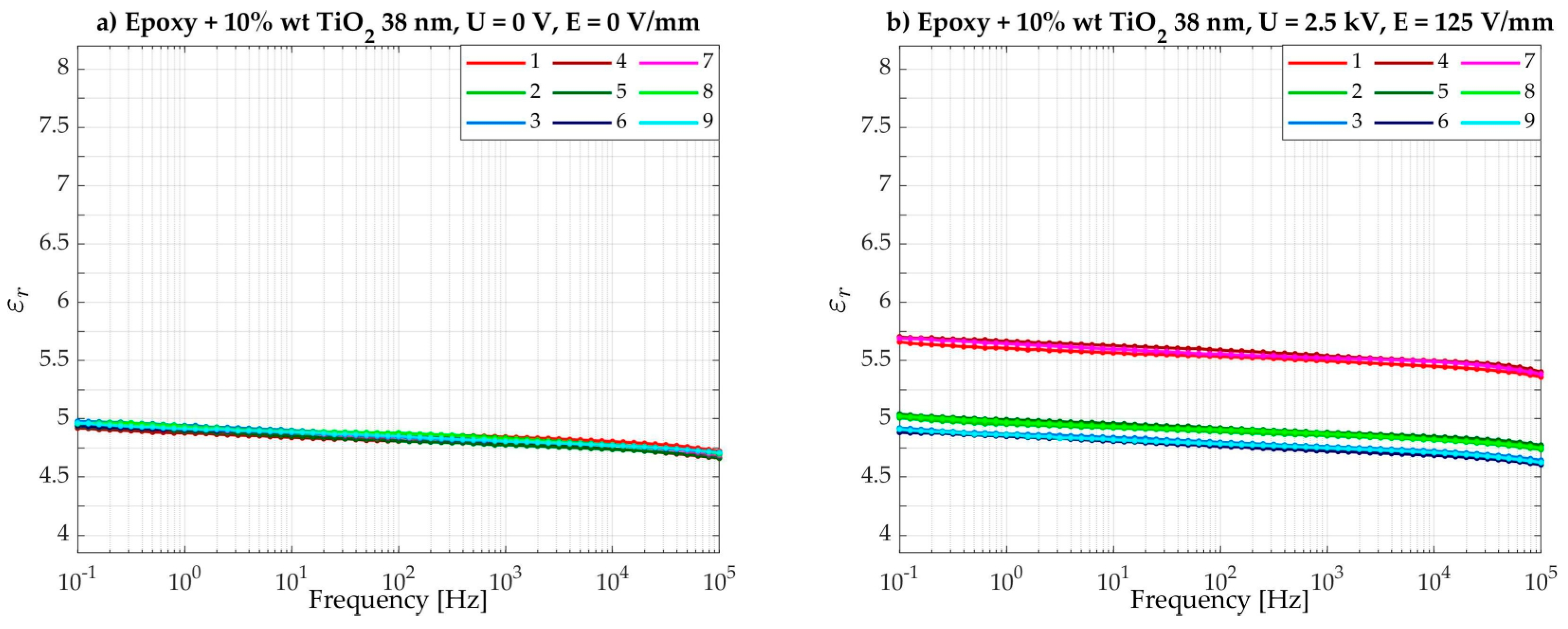
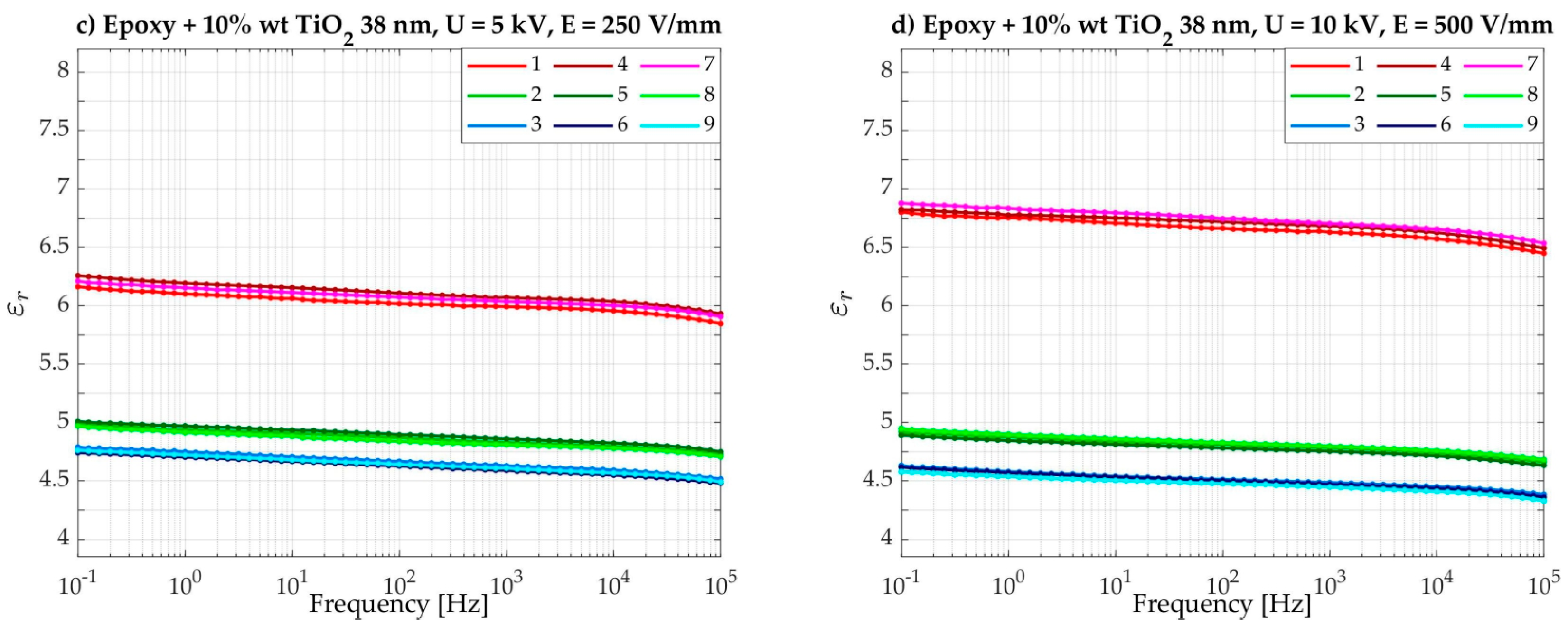
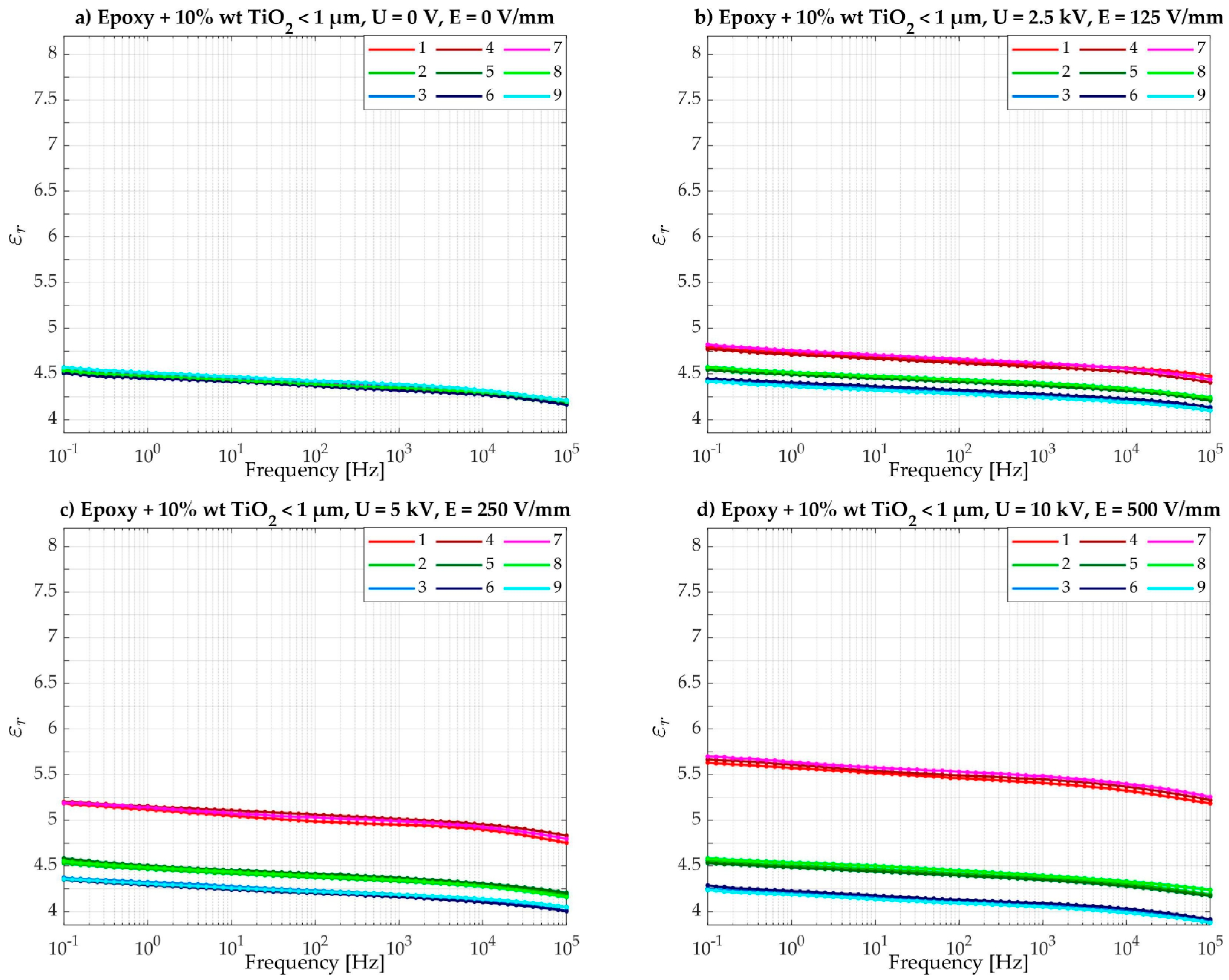



| Samples Set No. | Sample Composition | Applied Voltage UDC, kV | Average Electric Field Strength Eav, V·mm−1 |
|---|---|---|---|
| 1 | epoxy resin + 10 wt% TiO2 13 nm | 0.0 | 0.0 |
| 2 | epoxy resin + 10 wt% TiO2 13 nm | 2.5 | 125 |
| 3 | epoxy resin + 10 wt% TiO2 13 nm | 5.0 | 250 |
| 4 | epoxy resin + 10 wt% TiO2 13 nm | 10.0 | 500 |
| 5 | epoxy resin + 10 wt% TiO2 38 nm | 0.0 | 0.0 |
| 6 | epoxy resin + 10 wt% TiO2 38 nm | 2.5 | 125 |
| 7 | epoxy resin + 10 wt% TiO2 38 nm | 5.0 | 250 |
| 8 | epoxy resin + 10 wt% TiO2 38 nm | 10.0 | 500 |
| 9 | epoxy resin + 10 wt% TiO2 < 1 µm | 0.0 | 0.0 |
| 10 | epoxy resin + 10 wt% TiO2 < 1 µm | 2.5 | 125 |
| 11 | epoxy resin + 10 wt% TiO2 < 1 µm | 5.0 | 250 |
| 12 | epoxy resin + 10 wt% TiO2 < 1 µm | 10.0 | 500 |
| 13 | neat epoxy resin | 0.0 | 0.0 |
| 14 | neat epoxy resin | 2.5 | 125 |
| 15 | neat epoxy resin | 5.0 | 250 |
| 16 | neat epoxy resin | 10.0 | 500 |
| Eav, V·mm−1 | 500 | 250 | 125 | 0 | ||||
|---|---|---|---|---|---|---|---|---|
| Parameter | εr/Δεr | tanδ | εr/Δεr | tanδ | εr/Δεr | tanδ | εr | tanδ |
| Area: ➀, ➃, ➆ | 7.28/39.7% | 0.0418 | 6.59/26.5% | 0.0359 | 6.03/15.7% | 0.0258 | 5.21 | 0.0218 |
| Area: ➁, ➄, ➇ | 5.18/−0.6% | 0.0219 | 5.14/−1.3% | 0.0216 | 5.20/−0.2% | 0.0197 | ||
| Area: ➂, ➅, ➈ | 4.73/−9.2% | 0.0168 | 4.85/−6.9% | 0.0170 | 4.98/−4.4% | 0.0186 | ||
| Eav, V·mm−1 | 500 | 250 | 125 | 0 | ||||
|---|---|---|---|---|---|---|---|---|
| Parameter | εr/Δεr | tanδ | εr/Δεr | tanδ | εr/Δεr | tanδ | εr | tanδ |
| Area: ➀, ➃, ➆ | 6.72/38.6% | 0.0142 | 6.08/25.4% | 0.0131 | 5.57/14.8% | 0.0127 | 4.85 | 0.0120 |
| Area: ➁, ➄, ➇ | 4.82/−0.6% | 0.0118 | 4.88/0.6% | 0.0121 | 4.92/1.4% | 0.0120 | ||
| Area: ➂, ➅, ➈ | 4.51/−7.0% | 0.0112 | 4.66/−3.9% | 0.0115 | 4.79/−1.2% | 0.0116 | ||
| Eav, V·mm−1 | 500 | 250 | 125 | 0 | ||||
|---|---|---|---|---|---|---|---|---|
| Parameter | εr/Δεr | tanδ | εr/Δεr | tanδ | εr/Δεr | tanδ | εr | tanδ |
| Area: ➀, ➃, ➆ | 5.51/24.9% | 0.0132 | 5.04/14.3% | 0.0126 | 4.65/5.4% | 0.0120 | 4.41 | 0.0104 |
| Area: ➁, ➄, ➇ | 4.44/0.7% | 0.0105 | 4.41/0.0% | 0.0104 | 4.43/0.5% | 0.0106 | ||
| Area: ➂, ➅, ➈ | 4.12/−6.6% | 0.0092 | 4.23/−4.1% | 0.0097 | 4.32/−2.0% | 0.0100 | ||
| Parameter | Value | Unit |
|---|---|---|
| Stainless steel | ||
| Electrical conductivity, σ | 4 × 106 | S·m−1 |
| Dielectric constant, εr | 1 | - |
| Heat capacity, Cp | 475 | J·kg−1·K−1 |
| Thermal conductivity, k | 44.5 | W·m−1·K−1 |
| Density, ρ | 7850 | kg·m−3 |
| Epoxy resin | ||
| Electrical conductivity, σ | σ (α·T, t) | S·m−1 |
| Dielectric constant, εr | 4 | - |
| Heat capacity, Cp | 1380 | J·kg−1·K−1 |
| Thermal conductivity, k | 0.26 | W·m−1·K−1 |
| Density, ρ | 1130 | kg·m−3 |
| Temperature factor of epoxy conductivity, α | 0.1 | K−1 |
| PTFE | ||
| Electrical conductivity, σ | 1 × 10−16 | S·m−1 |
| Dielectric constant, εr | 2 | - |
| Heat capacity, Cp | 1050 | J·kg−1·K−1 |
| Thermal conductivity, k | 0.24 | W·m−1·K−1 |
| Density, ρ | 2200 | kg·m−3 |
| Ambience | ||
| Heat transfer coefficient, h | 10 | W·m−2·K−1 |
| Ambient temperature, Tamb | 20 | °C |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dąda, A.; Błaut, P.; Mikrut, P.; Kuniewski, M.; Zydroń, P. Control of Dielectric Parameters of Micro- and Nanomodified Epoxy Resin Using Electrophoresis. Energies 2024, 17, 1095. https://doi.org/10.3390/en17051095
Dąda A, Błaut P, Mikrut P, Kuniewski M, Zydroń P. Control of Dielectric Parameters of Micro- and Nanomodified Epoxy Resin Using Electrophoresis. Energies. 2024; 17(5):1095. https://doi.org/10.3390/en17051095
Chicago/Turabian StyleDąda, Anna, Paweł Błaut, Paweł Mikrut, Maciej Kuniewski, and Paweł Zydroń. 2024. "Control of Dielectric Parameters of Micro- and Nanomodified Epoxy Resin Using Electrophoresis" Energies 17, no. 5: 1095. https://doi.org/10.3390/en17051095
APA StyleDąda, A., Błaut, P., Mikrut, P., Kuniewski, M., & Zydroń, P. (2024). Control of Dielectric Parameters of Micro- and Nanomodified Epoxy Resin Using Electrophoresis. Energies, 17(5), 1095. https://doi.org/10.3390/en17051095






